A solution of a nonlinear Volterra integral equation with delay via a faster iteration method

1.   Introduction

Let $\mathscr{B}$ be a Banach space and $\mathscr{A}$ a nonempty closed convex subset of $\mathscr{B}$. Let $\mathscr{F}: \mathscr{A}\to \mathscr{A}$ be a mapping. A member $m^*\in \mathscr{A}$ is called a fixed points of $\mathscr{F}$ if $\mathscr{F}m^* = m^*$. We denote the set of all fixed point of $\mathscr{F}$ by $F(\mathscr{F})$.

In ^[6], Berinde introduced a new class of mappings statisfying

for all $m, q\in \mathscr{A}$, $\gamma\in(0, 1)$ and $L \geq 0$.

The author established that the class of mappings satisfying (1.1) is larger than the class of mappings introduced and studied by Zamfirescu in ^[41].

In ^[15], Imoru and Olantiwo gave a definition of a mapping considered to be generalization of the classes of mappings considered by Berinde ^[6], Osilike et al. ^[33] and some other existing contraction-type mappings as follows:

Definition 1.1. ^[15] A mapping $\mathscr{F}:\mathscr{A}\to\mathscr{A}$ is called contractive-like if there exists a constant $\gamma\in [0, 1)$ and a strictly increasing continuous function $\psi:[0, \infty)\to[0, \infty)$ with $\psi(0) = 0$ such that

for all $m, q\in \mathscr{A}$.

Remark 1.1. If $\psi(m) = Lm$, then (1.2) reduces to (1.1).

Problems in so many fields of mathematics and other subjects in sciences can be transformed into an equation for a suitable operator. Furthermore, the existence of a solution to this equation is tantamount to the existence of a fixed point of the suitable operator. Basically, fixed point theory is a nice mixture of functional analysis, topology and geometry. Translating the real-life or theoretical problem into the fixed point problem is a good approach to find the corresponding solution. In general, fixed point theory plays a vital role in almost all areas of applied sciences and engineering such as: economics, game theory, theoretical computer science, biology, chemistry, physics and many more, see e.g. ^{[8,19,20,24,25,26,27,28,29,30,31]}.

Proving the existence of a fixed point is an important step in finding a solution of a given problem, but it is also necessary to find the solution of a given problem when it exists. One valuable way of finding the desired fixed point is to utilize iterative method. For this reason, so many iterative methods for approximating the fixed points of different classes of operators have been introduced and studied by so many authors for the past two decades. Some widely used iterative methods in the literature are: Mann ^[21], Ishikawa ^[16], Noor ^[23], S ^[2], Abbas and Nazir ^[1], Tharkur ^[36] and many more.

Throughout this paper, the set of all natural numbers is denoted by $\mathbb{N}$ and the set of real numbers is donted by $\mathbb{R}$. For the sequences $\{\vartheta_n\}, \{\varpi_n\}$ and $\{\varrho_n\}$ in [0, 1], the following iterative methods are known as Noor, S and M, respectively:

Very recently, Akutsah et al. ^[4] introduced the following three steps iterative method:

where $\{\vartheta_n\}$ and $\{\varpi_n\}$ are sequences in [0, 1]. The authors analytically and numerically showed that the iterative method (1.6) converges faster than those of Ullah and Arshad ^[39], Karakaya et al. ^[18] and Thakur et al. ^[37], respectively for contractive-like mappings.

The preference of an iterative method over another is based on some crucial criteria such as fastness, stability and dependence. Data dependency of fixed points iterative methods has become an area that has attracted many researchers for several decades now. There exists several recent results on data dependency of fixed point, see e.g. ^{[3,5,10,11,14,22,25,32]}.

Due to the importance of data dependency in fixed point theory, Akutsah et al. ^[4] raised the following question:

Open Question: Is it possible to obtain the data dependence result of the iterative method (1.6) for contractive-like mappings?

On other hand, a fixed point iterative method is said to be numerically stable if small modifications in the initial data involved in a computation process will produce a small impact on the computed value of the fixed point. The concept of stability was first considered by Ostrowski ^[34] for Banach contraction mappings. In 1988, Harder and Hicks ^[12,13] illustrated the importance of studying the stability of various iterative methods.

Definition 1.2. ^[12,13] Let $\{t_n\}$ be any sequence in $\mathscr{A}$. Then, an iterative method $t_{n+1} = f(\mathscr{F}, t_n)$, which converges to fixed point $m^*$, is said to be $\mathscr{F}$-stable or stable with respect to $\mathscr{F}$, if for $\varepsilon_n = \|t_{n+1}-f(\mathscr{F}, t_n)\|$, $\forall\, n\in\mathbb{N}$, we have

In the last few years, many authors have studied the stability of several iterative methods for different classes of operators (see e.g. ^{[11,17,22,25]} and the references in them). Very recently, Akutsah et al. ^[4] established the following convergence and stability results for contractive-like mapping in Banach spaces.

Theorem 1.1. ^[4] Let $\mathscr{A}$ be a nonempty closed convex subset of a uniformly convex Banach space $\mathscr{B}$. Let $\mathscr{F}$ be a mapping satisfying (1.2). Let $\{m_n\}$ be the iterative method defined in (1.6) with sequences $\{\vartheta_n\}$, $\{\varpi_n\}\in [0, 1]$ such that $\sum\limits_{n = 0}^{\infty}\varpi_n = \infty$. Then, $\{m_n\}$ converges strongly to a unique fixed point of $\mathscr{F}$.

Theorem 1.2. ^[4] Let $\mathscr{A}$ be a nonempty closed convex subset of a uniformly convex Banach space $\mathscr{B}$. Let $\mathscr{F}$ be a mapping satisfying (1.2). Let $\{m_n\}$ be the iterative method defined by $(1.6)$ with sequences $\{\vartheta_n\}$ and $\{\varpi_n\}$ in [0, 1] such that $\sum\limits_{n = 0}^{\infty}\varpi_n = \infty$. Then, $\{m_n\}$ is $\mathscr{F}$-stable.

In 2007, Berinde ^[8] showed throughout an example that taking an arbitrary sequence $\{t_n\}$ in Definition 1.2 led to an inconsistency. For this reason, Berinde ^[8] redefined the Definition 1.2 and gave a weaker, but more natural notion of stability known as weak stability. According the author, an approximate sequence of $\{m_n\}$ instead of arbitrary sequence $\{t_n\}$ in Definition 1.2 should be taken.

In 2012, Timi ^[38] introduced a wider concept of stability known as weak $w^2$-stability by adopting equivalent sequences instead of arbitrary sequences in Definition 1.2.

Definition 1.3. ^[38] Let $\{m_n\}$ be an iterative sequence given by $m_{n+1} = f (\mathscr{F}, m_n)$. Assume that $\{m_n\}$ converges to an $m^* \in F(\mathscr{F})$. Set

If for any equivalent sequence $\{t_n\}\subset \mathscr{A}$ of $\{m_n\}$,

then the iterative sequence $\{m_n\}$ is said to be weak $w^2$-stable with respect to $\mathscr{F}$.

Motivated by the above results, we prove the strong convergence theorem of the iterative method (1.6) for contractive-like mappings without the necessity of the assumption: $\sum_{n = 0}^{\infty}\varpi_n = \infty$ as considered in ^[4]. Also, we demonstrate the numerical convergence of (1.6) using a nontrivial example in higher dimensional space. Further, we prove analytically that the iterative method (1.6) is $w^2$-stable and the analytical proof is supported with some illustrative examples. Also, we give an affirmative answer to the open question above which was raised in ^[4] by showing that the iterative method (1.6) is data dependent for contractive-like mappings. Furthermore, by applying our main results, we establish the existence, uniqueness and approximation results for the solutions of a nonlinear Volterra integral equation with delay. We also provide an example which supports the application of our results.

This paper is organized as follows: In Section 2, we list some definitions and lemmmas which will be used for further proof. In Section 3, we establish the convergence result of the iterative method (1.6) for contractive-like mappings. A supporting example is also provided. In Section 4, we show that the iterative algorithm (1.6) is weak $w^2$-stable with respect to contractive-like mappings. The analytic result is supported with a numerical example. In Section 5, we prove the data dependence result of the iterative algorithm (1.6). In Section 6, we apply our main results to solve a nonlinear Volterra integral equation with delay and Section 7 gives the conclusion of our artilce.

2.   Preliminaries

In this section, we give some definitions and lemmas that will be useful in proving our main results.

Definition 2.1. ^[8] Let $\{m_n\}$ and $\{t_n\}$ be sequences in $\mathscr{A}$. We say that $\{t_n\}$ is an approximate sequence of $\{m_n\}$, if for any $r\in \mathbb{N}$, there exists $\varepsilon(r)$ such that

Definition 2.2. ^[7] Let $\mathscr{F}$, $\mathscr{\tilde{F}}:\mathscr{A}\to \mathscr{A}$ be two operators. We say that $\mathscr{\tilde{F}}$ is an approximate operator for $\mathscr{F}$ if for some $\varepsilon > 0$, we have

Definition 2.3. ^[9] Let $\{m_n\}$ and $\{t_n\}$ be sequences in $\mathscr{A}$. We say that these sequences are equivalent if

Lemma 2.1. ^[40] Let $\{ \mathfrak{\theta}_n\}$ and $\{\lambda_n\}$ be nonnegative real sequences satisfying the following inequalities:

where $\sigma_n\in (0, 1)$ for all $n\in \mathbb{N}$, $\sum\limits_{n = 0}^{\infty}\sigma_n = \infty$ and $\lim\limits_{n\to\infty}\frac{ \mathfrak{\lambda}_n}{\sigma_n} = 0$, then $\lim\limits_{n\to\infty} \mathfrak{\theta}_n = 0$.

Lemma 2.2. ^[35] Let $\{ \mathfrak{\theta}_n\}$ be a nonnegative real sequence such that for all $n\geq n_0\in \mathbb{N}$, the following condition holds:

where $\sigma_n\in (0, 1)$ for all $n\in \mathbb{N}$, $\sum\limits_{n = 0}^{\infty}\sigma_n = \infty$ and $\lambda_n\geq0$ for all $n\in \mathbb{N}$, then

3.   Convergence result

In this section, we will prove a strong convergence result of the iterative method (1.6) for contractive-like mapping by weakening some conditions imposed on the control parameters by Akutsah et al. ^[4]. We also provide an example in three dimensional space to compare the convergence of various iterative methods.

Theorem 3.1. Let $\mathscr{F}$ be a mapping satisfying (1.2) defined on a nonempty closed convex subset $\mathscr{A}$ of a Banach space $\mathscr{B}$ with $F(\mathscr{F})\neq\emptyset$. Let $\{m_n\}$ be the iterative sequence defined by (1.6), then $\{m_n\}$ converges strongly to the unique fixed point of $\mathscr{F}$.

Proof. From (1.6), for any $m^*\in F(\mathscr{F})$, we have

Since $\{\vartheta_n\}$, $\{\varpi_n\}\in [0, 1]$ and $\gamma\in [0, 1)$, then it follows that $(1-(1-\gamma)\vartheta_n) < 1$ and $(1-(1-\gamma)\varpi_n) < 1$. Hence, (3.3) yields

Taking limit on both sides of the above inequality (3.4), we get $\lim\limits_{n\to\infty}\|m_{n}-m^*\| = 0$. Indeed, $\gamma \in [0, 1)$ and so $\lim\limits_{n\to\infty}\gamma^{2n} = 0$.

Remark 3.1. Clearly, the condition $\sum\limits_{n = 0}^{\infty}\varpi_n = \infty$ on the sequence $\{\varpi_n\} \in (0, 1)$ in Theorem 1.1 is superfluous.

Now we give the following example to validate the analytical proof in Theorem 6.1 and also carry out a numerical experiment to test the efficiency of the iterative method (1.6).

Example 3.1. Let $\mathscr{A} = [0, 8]\times [0, 8]\times [0, 8]$ be the subset of a Banach space $\mathscr{B} = \mathbb{R}^3$ with the texicap norm. Let $\mathscr{F}: \mathscr{A}\to \mathscr{A}$ be defined by

Clearly, the only fixed point of $\mathscr{F}$ is $(0, 0, 0)$. We will now show that $\mathscr{F}$ is a contraction-like mapping. To see this, we define a function $\psi:\mathbb{R}^+\to \mathbb{R}^+$ by $\psi(m) = \frac{m}{12}$. Clearly, $\psi$ is a strictly increasing and continuous function satisfying $\psi(0) = 0$. We show that

for all $p, q\in \mathscr{A}$ and $\gamma\in [0, 1)$. It will be useful to note the following. If $m = (m_1, m_2, m_3)\in [0, 4)\times[0, 4)\times [0, 4)$, then

and

Similarly, if $m = (m_1, m_2, m_3)\in [4, 8]\times[4, 8]\times [4, 8]$, we have

and

Next, we consider the following cases:

Case Ⅰ: If $m = (m_1, m_2, m_3), q = (q_1, q_2, q_3)\in [0, 4)\times[0, 4)\times [0, 4)$, then using (3.6), we have

Case Ⅱ: If $m = (m_1, m_2, m_3), q = (q_1, q_2, q_3)\in [4, 8]\times[4, 8]\times [4, 8]$, then using (3.7), we get

Case Ⅲ: If $m = (m_1, m_2, m_3)\in [0, 4)\times[0, 4)\times [0, 4)$ and $q = (q_1, q_2, q_3)\in [4, 8]\times[4, 8]\times [4, 8]$, then using (3.6), we have

Case Ⅳ: If $m = (m_1, m_2, m_3)\in [4, 8]\times[4, 8]\times [4, 8]$ and $q = (q_1, q_2, q_3)\in [0, 4)\times[0, 4)\times [0, 4)$, then using (3.6), we get

So, (3.5) is fulfilled with $\gamma = \frac{1}{7}$. Thus, $\mathscr{F}$ is a contractive-like mapping.

It is worthy mentioning that the above example is more interesting and not as simple as that of Akutsah et al. ^[4].

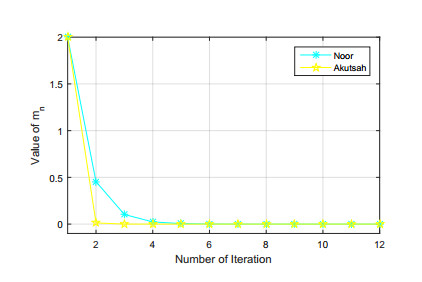
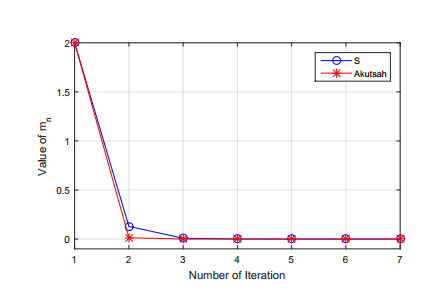
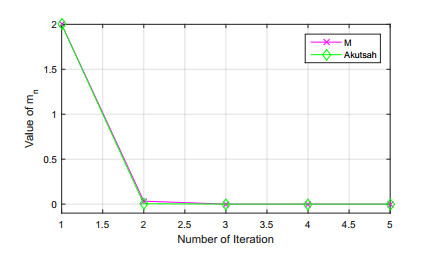
Using MATLAB R2015a, we obtain the following Tables 1–3 and Figures 1–3. Clearly, for control sequences $\varpi_n = \vartheta_n = \varrho_n = 0.8$ and starting value $m_0 = (2, 2.5, 3.5)$, the iterative method (1.6) converges faster than a number of iterative methods. We also notice that M, Thakur and Karakaya iterative methods converge almost at the same rate.

4.   Stability result

In this section, we prove a stability result and provide some numerical examples to support our analytical proof.

Theorem 4.1. Let $\mathscr{F}$ be a mapping satisfying (1.2) defined on a nonempty closed convex subset $\mathscr{A}$ of a Banach space $\mathscr{B}$ with $F(\mathscr{F})\neq0$. Let $\{m_n\}$ be the iterative sequence defined by (1.6), then $\{m_n\}$ is weak $w^2$-stable with respect to $\mathscr{F}$.

Proof. Let $\{t_n\}\in \mathscr{A}$ be an equivalent sequence of $\{m_n\}$. Define a sequence $\{\epsilon_n\}$ in $\mathbb{R}^+$ by

where $\{\vartheta_n\}$ and $\{\varpi_n\}$ are sequences in [0, 1]. Let $\lim\limits_{n\to\infty}\epsilon_n = 0$, then from (1.2), (1.6) and (4.1), we have

Using (4.2)–(4.4), we have

Since $\{t_n\}\in\mathscr{A}$ and its equivalence to $\{m_n\}$ yields $\lim\limits_{n\to\infty}\|m_n-t_n\| = 0$. We have shown in Theorem 3.1 that $\|m_n-m^*\| = 0$, consequently $\lim\limits_{n\to\infty}\|m_{n+1}-m^*\| = 0$. This implies that

Following similar argument as above, we have that

Since $\lim\limits_{n\to\infty} \|m_n-\mathscr{F}m_n\| = \psi(\lim\limits_{n\to\infty} \|m_n-\mathscr{F}m_n\|) = 0.$ It follows that

Also,

Therefore, from (4.8), we have

Thus, taking the limit on both sides of (4.5), we get

Hence, $\{m_n\}$ is weak $w^2$-stable with respect to $\mathscr{F}$.

Now, we furnish the following examples in support of the above claim.

Example 4.1. Let $\mathscr{B} = \mathbb{R}$ with the usual norm and $\mathscr{A} = [0, 1]$ be a subset of $\mathscr{B}$. Define a mapping $\mathscr{F}:\mathscr{A}\to\mathscr{A}$ by

Clearly, zero is the fixed point of $\mathscr{F}$. Now we show that $\mathscr{F}$ is a weak contractive-like mapping satisfying (1.2). For this, let $\gamma = \frac{1}{2}$ and given as increasing function $\psi:[0, \infty)\to[0, \infty)$ with $\psi(0) = 0$, then we obtain

The iterative method (1.6) corresponding to the operator $\mathscr{F}$ in (4.11) is defined as follows:

where $\{\vartheta_n\}$ and $\{\varpi_n\}$ are sequences in [0, 1].

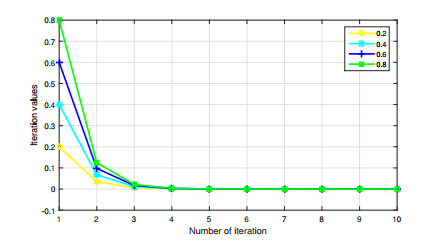
Let $\vartheta_n = \varpi_n = \frac{1}{n+2}$. Using MATLAB R2015a, the following Table 4 and Figure 4 are obtained. They show that the iterative method $\{m_n\}$ in (4.12) converges to $m^* = 0$ for different choices of starting point $m_1\in [0, 1]$.

From the above table and graph, it is evident that $\lim\limits_{n\to\infty}m_n = 0 = m^*\in F(\mathscr{F})$. On the other hand, we obtain $\lim\limits_{n\to\infty}\|m_n\| = \|\lim\limits_{n\to\infty}m_n\| = 0$. If we take the sequence $\{t_n\}$ as $t_n = \frac{1}{n+3}$ for all $n\in \mathbb{N}$, then we have

which yields $\lim\limits_{n\to\infty}\|m_n-t_n\| = 0$. This implies that the sequences $\{m_n\}$ and $\{t_n\}$ are equivalent.

Now, we show that (1.6) is weak $w^2$-stable with respect to $\mathscr{F}$.

Clearly, $\lim\limits_{n\to\infty}\epsilon_n = 0$. Therefore, the iterative method $\{m_n\}$ is weak $w^2$-stable with respect to $\mathscr{F}$.

Example 4.2. Let $\mathscr{B} = \mathbb{R}$ with the usual norm and $\mathscr{A} = [0, 1]$ be a subset of $\mathscr{B}$. Define a mapping $\mathscr{F}:\mathscr{A}\to\mathscr{A}$ by

Apparently, zero is the fixed point of $\mathscr{F}$ and $\mathscr{F}$ satisfies (1.2) with $\gamma = \frac{1}{5}$.

Now, we show that the iterative sequence $\{m_n\}^\infty_{n = 0}$ in (1.6) converges to $p = 0\in F(\mathscr{F})$ for different choices of real sequences in $\{\varpi_n\}, \{\vartheta_n\}$ in [0, 1]. Let $\left\{m^{(1)}_n\right\}^\infty_{n = 0}, \left\{m^{(2)}_n\right\}^\infty_{n = 0}, \left\{m^{(3)}_n\right\}^\infty_{n = 0}$ be the iterative method (1.6) with control parameters $\left(\varpi_n = \vartheta_n = \frac{1}{n+1}\right)$, $\left(\varpi_n = \vartheta_n = \frac{1}{2n+1}\right)$ and $\left(\varpi_n = \frac{1}{n+2}, \vartheta_n = \frac{n+1}{2n+1}\right)$ for all $n\in \mathbb{N}$, respectively.

For arbitrary $x_1\in [0, 1]$, it follows that

Also,

Finally,

From (4.14)–(4.16), set

Then clearly, $\sigma^{(i)}_n\in (0, 1)$ for each $i\in \{1, 2, 3\}$ and $\sum\limits_{n}^{\infty}\sigma^{(i)}_n = \infty$ for each $i\in \{1, 2, 3\}$. Thus, by Lemma 2.1, we have that $\lim\limits_{n\to\infty}m^{(i)}_n = p = 0\in F(\mathscr{F})$ for each $i\in \{1, 2, 3\}$.

On the other hand, we have $\lim\limits_{n\to\infty}\left\|m^{(i)}\right\| = \left\|\lim\limits_{n\to\infty}m^{(i)}\right\| = 0$ for each $i\in \{1, 2, 3\}$. Taking the sequence $\{t_n\}^\infty_{n = 0}$ to be $t_n = \frac{1}{n+4}$ for all $n\in \mathbb{N}$, then we get

which shows that $\lim\limits_{n\to\infty}\left\|m^{(i)}-t_n\right\| = 0$ for each $i\in \{1, 2, 3\}$, in other words, each of $\left\{m^{(i)}_n\right\}^\infty_{n = 0}$, $i\in \{1, 2, 3\}$ and $\{t_n\}^\infty_{n = 0} = \left\{\frac{1}{n+4}\right\}^\infty_{n = 0}$ are equivalent sequences.

Let $\epsilon^{(1)}_n$, $\epsilon^{(2)}_n$ and $\epsilon^{(3)}_n$ be the corresponding sequences to the iterative algorithms $\left\{m^{(1)}_n\right\}^\infty_{n = 0}$, $\left\{m^{(2)}_n\right\}^\infty_{n = 0}$ and $\left\{m^{(3)}_n\right\}^\infty_{n = 0}$, respectively. Then we have

Obviously, $\lim\limits_{n\to\infty} \epsilon^{(i)}_n = 0$ for each $i \in\{1, 2, 3\}$. Hence, all the iterative sequences $\left\{m^{(i)}_n\right\}^\infty_{n = 0}$, $i \in\{1, 2, 3\}$ are $w^2$-stable with respect to $\mathscr{F}$.

5.   Data dependence result

In this section, the data dependence result of (1.6) for contractive-like mapping is obtained, hence, giving an affirmative answer to the above open question raised by Akutsah et al. ^[4].

Theorem 5.1. Let $\mathscr{\tilde{F}}$ be an approximate operator of a mapping $\mathscr{F}$ satisfying (1.2). Let $\{m_n\}$ be an iterative method generated by (1.6) for $\mathscr{F}$ and define an iterative method as follows:

where $\{\vartheta_n\}$ and $\{\varpi_n\}$ are sequences in $[0, 1]$ satisfying the following conditions:

(i) $\frac{1}{2}\leq \varpi_n, \, \forall\, n\in\mathbb{N}$,

(ii) $\sum\limits _{n = 0}^{\infty}\varpi_n = \infty$.

If $\mathscr{F}m^* = m^*$ and $\mathscr{\tilde{F}}\tilde{m^*} = \tilde{m^*}$ such that $\lim\limits_{n\to\infty}\tilde{m}_n = \tilde{m^*}$, we have

where $\epsilon > 0$ is a fixed number.

Proof. Using (1.2), (1.6) and (5.1), we obtain

Using (5.3) and (5.4),

Putting (5.5) into (5.6), we obtain

Since $\varpi_n$, $\vartheta_n$ $\in[0, 1]$ and $\gamma\in[0, 1)$, it follows that

From (5.7) and (5.8), we have

By our assumption (ⅰ), we have

This yields

Set

From Theorem 3.1, we know that $\lim\limits_{n\to\infty}m_m = m^*$ and since $\mathscr{F}m^* = m^*$, then from (4.7), (4.9) and Lemma 2.2, we obtain

Since by Theorem 3.1, we have that $\lim\limits_{n\to\infty}m_n = m^*$ and using the hypothesis $\lim\limits_{n\to\infty}\tilde{m}_n = \tilde{m^*}$, it follows from (5.11) that

6.   Application

In this section, we discuss an application to nonlinear Volterra integral equation with delay. Consider the integral equation

with initial function

where $\phi\in C[a-\tau, a], \mathbb{R})$, $a, b\in \mathbb{R}$ and $\tau > 0$.

Let $C[a, b]$ denote the set all of continuous functions defined on $[a, b]$ endowed with infinity norm $\|m-p\|_\infty = \max\limits_{a\leq t\leq b}\|m(t)-p(t)\|$. It is well known that $(C[a, b], \mathbb{R}), \|\cdot\|_\infty)$ is a Banach space.

Theorem 6.1. Let $\mathscr{K}$ be a nonempty closed convex subset of a Banach space $\mathscr{M} = (C([a, b], \mathbb{R}), \|\cdot\|_\infty)$. Let $\{m_n\}$ be the iterative method (1.6) with $\varpi_n, \vartheta_n\in [0, 1]$. Let $\mathscr{F}:\mathscr{K}\to \mathscr{K}$ be the operator defined by

Suppose the following assumptions hold:

(a) $g:I\to \mathbb{R}$ is continuous;

(b) $f:I\times I\times\mathbb{R}\times\mathbb{R}\to\mathbb{R}$ is continuous in the sense that there exists a constant $L_f > 0$ such that

for all $t, s\in I$, $u_i, v_i\in \mathbb{R}\, \, (i=1, 2)$;

(d) $2\lambda L_f(b-a) < 1$.

Then, the problem (6.1) with (6.2) has a unique solution, say $m^*\in C[a, b]$. Moreover, if $\mathscr{F}$ is a mapping satisfying (1.2). Then, $\{m_n\}$ converges strongly to $m^*$.

Proof. Now, using the contraction principle, we show that $\mathscr{F}$ has a fixed point. Note that

Next, for any $t\in I$, we have

therefore,

From condition (d), the operator $\mathscr{F}$ is a contraction and using the contraction principle we deduce that the operator $\mathscr{F}$ has a unique fixed point, $F(\mathscr{F}) = \{m^*\}$, i.e. the problem (6.1) with (6.2) has a unique solution $m^*\in C [a, b]$.

Next, we show that $\{m_n\}$ converges strongly to $m^*$. For $m, p\in \mathscr{A}$, we have

therefore,

From (6.4), it is clear that $\mathscr{F}$ is a mapping satisfying (1.1). Set $\gamma = 2\lambda L_f(b-a)$, and by assumption (d), we have $\gamma < 1$. Thus, the operator $\mathscr{F}$ is a contractive-like mapping satisfying (1.2) on $\mathscr{K}$ with $L = 1$ since for $\psi(m) = Lm$, (1.2) reduces to (1.1). Taking $\mathscr{A} = \mathscr{K}$ and $\mathscr{B} = \mathscr{M}$, then all the assumptions of Theorem 4.2 are satisfied. Therefore, the sequence $\{m_n\}$ defined by the iterative algorithm (1.6) converges strongly to the unique solution of the problem (6.1) with (6.2).

7.   Conclusions

In this study, we have proved the strong convergence results of the iterative scheme (1.6) for fixed points of contractive-like mapping under mild conditions. We have presented some interesting and nontrivial examples in three dimensional space to compare the efficiency of the iterative method (1.6) with some existing iterative methods. Also, we have shown analytically and numerically that the iterative method (1.6) is $w^2$-stable for different choices of parameters and initial guesses. Again, our data dependence result gives an affirmative answer to the open question raised by Akutsah et al ^[4]. As an application of our results, we have established the existence, uniqueness and approximation results for the solutions of a nonlinear Volterra integral equation with delay (6.1) with (6.2).

Acknowledgements

The work was supported by Princess Nourah bint Abdulrahman University Researchers Supporting Project (No. PNURSP2022R14). The authors A. Khan and T. Abdeljawad would like to thank Prince Sultan University for paying the APC and for the support through the TAS research lab.

Conflict of interest

The authors declare no conflicts of interest.