Bifurcation control strategy for a fractional-order delayed financial crises contagions model

1.   Introduction

The financial crisis will have a great impact on the economic order of China and even the whole world. During the past several decades, the international financial crises have continually burst out and spread to many countries or regions quickly. For example, "tequila crisis" of Latin American countries in 1994, "Russian virus" in 1998, and the financial crises of Southeast Asian in 1997, etc. ^[1]. The financial crises is very harmful and will lead to great disorder of economic development. Thus it is an important task for us to deal with the various financial models to reveal their inherent change law in order to control vicious economic development and serve human beings. At present, there are many valuable works on all kinds of finance models. For example, Yu et al. ^[2] reported the bifurcation and its control issue for a hyperchaotic finance model, Cao ^[3] investigated the chaos control of a hyperchaotic finance model, Liao et al. ^[4] revealed the impact of policy lag on the Hopf bifurcation and chaos for a macroeconomic model. For more related studies, one can see ^[5-11]

Hopf bifurcation is an important dynamical phenomenon in delayed systems. In particular, Hopf bifurcation and its control in economic systems plays a vital role in maintaining economic stability and virtuous circle of development. Thus it is important for us to explore this topic in economic or financial models.

In 2011, Chen and Ying ^[1] investigated the following financial crises contagions model:

where $u_1, u_2$ denote the stock return rates of country I and country II, respectively, $\alpha > 0$ represents the increasing rate of the average stock returns of country I under the normal situation, and $\beta > 0$ represents the decreasing rate of the stock returns of country II. In details, one can see ^[1]. By virtue of the stability theory of ordinary differential equation, Chen and Ying ^[1] systematically analyzed the stability of different equilibrium points of model (1.1).

Considering that the stock return rate of country I is affected by the stock return rate of country II during the past time and the stock return rate of country II is affected by the stock return rate of country I during the past time, we think that it is more suitable for us to introduce the time delay into model (1.1), then we can establish the following delayed financial crises contagions model:

where $u_1, u_2$ denote the stock return rates of country I and country II, respectively, $\alpha > 0$ represents the increasing rate of the average stock returns of country I under the normal situation, and $\beta > 0$ represents the decreasing rate of the stock returns of country II, $\sigma$ is a delay.

From a mathematical point of view, fractional-order dynamical model is more efficient instrument to describe the real financial phenomenon in economics than integer-order ones since fractional-order dynamical model possesses the memory trait and hereditary peculiarity for all kinds of economic variables and inherent development process ^[12,17-23], Inspired by this idea, we modify the delayed financial crises contagions model (1.2) as the following fractional-order form:

where $0 < \mu < 1$ is a constant, $u_1, u_2$ denote the stock return rates of country I and country II, respectively, $\alpha > 0$ represents the increasing rate of the average stock returns of country I under the normal situation, and $\beta > 0$ represents the decreasing rate of the stock returns of country II, $\sigma$ is a delay. The fractional-order financial crises contagions model (1.3) owns greater advantages in describing economic laws than the integer-order ones and Hopf bifurcation property can effectively depict the stock return rates of country I and country II. Motivated by this idea, we think that it is necessary to deal with the Hopf bifurcation and its control issue for model (1.3). In particular, the key object is to discuss the stability and Hopf bifurcation of system (1.3) and analyze the Hopf bifurcation control issue of system (1.3). In addition, we still reveal the effect of delay on Hopf bifurcation of system (1.3).

The key contributions of this work are as follow: (1) A novel fractional-order delayed financial crises contagions model is built. (2) A delay-independent sufficient condition guaranteeing the stability and the creation of Hopf bifurcation for the involved fractional-order delayed financial crises contagions model is obtained. (3) A suitable delayed feedback controller is successfully designed to control the Hopf bifurcation of the involved fractional-order delayed financial crises contagions model.

The work is arranged as follows. The requisite theory about fractional-order differential system is prepared in Section 2. The delay-independent stability and bifurcation criteria remaining the stability and the onset of Hopf bifurcation for fractional-order delayed financial crises contagions model are built in Section 3. The delay-independent stability and bifurcation criteria maintaining the stability and the onset of Hopf bifurcation for fractional-order delayed controlled financial crises contagions model are built in Section 4. The computer simulations substantiating the studied key results are performed in Section 5. The conclusion is drawn in Section 6.

2.   Requisite knowledge

In this section, some necessary important definitions and lemmas about fractional-order dynamical system are given.

Definition 2.1. [12] The fractional integral of order $\mu$ of the function $g(\eta)$ is given by

where $\eta\geq \eta_0, \mu > 0,$ and $\Gamma(s) = \int_0^\infty \eta^{s-1}e^{-\eta}d\eta$ denotes Gamma function.

Definition 2.2. [12] Let $g(\eta)\in C([\eta_0, \infty), R)$. Define the Caputo fractional-order derivative of order $\mu$ of $g(\eta)$ as follows:

where $\eta\geq \eta_0$ and $m$ denotes a positive integer which satisfies $m-1\leq \mu < m.$ Furthermore, when $0 < \mu < 1$, then

Definition 2.3. [13] For the given system:

where $\mu\in(0, 1], x_l(t) = (x_1(t), x_2(t), \cdots, x_k(t)), h_l(t) = (h_1(t), h_2(t), \cdots, h_k(t)).$ If $h_l(x_l^*) = 0,$ then $(x_1^*, x_2^*, \cdots, x_k^*)$ is said to be the equilibrium point of system (2.1).

Lemma 2.1. [14] For the given fractional order system ${\mathcal{D}}^\mu y = {\mathcal{L}}y, y(0) = y_0$ where $0 < \mu < 1, y\in R^k, {\mathcal{L}}\in R^{k\times k}.$ Assume that $\lambda_h(h = 1, 2, \cdots, k)$ is the root of the characteristic equation of ${\mathcal{D}}^\mu y = {\mathcal{L}}y$. Then system ${\mathcal{D}}^\mu y = {\mathcal{L}}y$ is said to be asymptotically stable $\Leftrightarrow$ $|\mbox{arg}(\lambda_h)| > \frac{\mu\pi}{2} (h = 1, 2, \cdots, k)$. Besides, this system is said to be stable $\Leftrightarrow$ $|\mbox{arg}(\lambda_h)| > \frac{\mu\pi}{2} (h = 1, 2, \cdots, k)$ and every critical eigenvalue that satisfies $|\mbox{arg}(\lambda_h)| = \frac{\mu\pi}{2} (h = 1, 2, \cdots, k)$ has geometric multiplicity one.

Lemma 2.2. [15] For the given fractional order system ${\mathcal{D}}^\mu w(t) = {\mathcal{T}}_1w(t)+{\mathcal{T}}_2w(t-\sigma)$, where $w(t) = \phi(t), t\in[-\sigma, 0], \mu\in(0, 1], w\in R^n, {\mathcal{T}}_1, {\mathcal{T}}_2\in R^{n\times n}, \mu\in R^{+(n\times n)}$. The characteristic equation of the system can be expressed as $\det |s^\mu {\mathcal{I}}-{\mathcal{T}}_1-{\mathcal{T}}_2e^{-s\sigma}| = 0.$ Then the zero solution of the system is asymptotically stable if each root of the equation $\det |s^\mu {\mathcal{I}}-{\mathcal{T}}_1-{\mathcal{T}}_2e^{-s\sigma}| = 0$ owns negative real part.

3.   Bifurcation of financial crises model (1.3)

In this section, we are to analyze the influence of time delay $\sigma$ on Hopf bifurcation for the fractional-order delayed financial crises contagions model (1.3).

Let $(u_{1*}, u_{2*})$ be the equilibrium point of model (1.3), then

It follows from (3.1) that system (1.3) has the unique positive equilibrium point $U(u_{1*}, u_{2*})$ where $u_{1*} = \frac{\beta^2}{\alpha}, u_{2*} = \frac{\alpha}{\beta}.$

let

then system (1.3) is expressed as the following form:

The linear system of (3.3) near $(0, 0)$ owns the expression:

Let ${u}_i$ denote $\tilde{u}_i(i = 1, 2)$, then system (3.4) becomes

The characteristic equation of Eq (3.5) is given by

which leads to

where

Assume that

holds.

Lemma 3.1. For system (1.3), the positive equilibrium point $U(u_{1*}, u_{2*})$ is locally asymptotically stable provided that $(K_1)$ holds true.

Proof. If $\sigma = 0,$ then (3.7) becomes

It follows from $(K_1)$ that every root $\lambda_h$ of (3.7) satisfies $|\mbox{arg}(\lambda_h)| > \frac{\mu\pi}{2} (h = 1, 2).$ By Lemma 3.1, one knows that the positive equilibrium point $U(u_{1*}, u_{2*})$ is locally asymptotically stable. The proof completes.

Assume that $s = i\gamma = \gamma\left(\cos\frac{\pi}{2}+i\sin\frac{\pi}{2}\right)$ is a root of Eq. (3.7), then

According to (3.10), we have

and

which leads to

where

Denote

Suppose that

By $(K_2)$, one derives $\epsilon_4 < 0$. Notice that $\frac{d\Psi(\gamma)}{d\gamma} > 0$, for each $\gamma > 0$, then Eq (3.13) has at least one positive real root. So, Eq (3.7) has at least a pair of purely roots.

Suppose that Eq (3.15) has four real roots (say $\gamma_h > 0(h = 1, 2, 3, 4)$. By (3.11), we have

where $l = 0, 1, 2, \cdots, h = 1, 2, 3, 4.$ Let

Assume that

where

Lemma 3.2. Suppose that $s(\sigma) = \rho_1(\sigma)+i\rho_2(\sigma)$ is the root of (3.7) near $\sigma = \sigma_{0}$ such that $\rho_1(\sigma_{0}) = 0, \rho_2(\sigma_{0}) = \gamma_{0}$, then $Re\left[\frac{ds}{d\sigma}\right]_{\sigma = \sigma_{0}, \gamma = \gamma_{0}} > 0.$

Proof. It follows from (3.7) that

It follows from (3.19) that

which leads to

Thus

Applying $(K_3)$, we have

which ends the proof.

By means of the analysis above, the following assertion holds.

Theorem 3.1. If $(K_1)$–$(K_3)$ are satisfied, then the positive equilibrium point $(u_{1*}, u_{2*})$ of system (1.3) is locally asymptotically stable if $0\leq\sigma < \sigma_{0}$ and system (1.3) generates Hopf bifurcation around the positive equilibrium point $(u_{1*}, u_{2*})$ when $\sigma$ passes through the delay value $\sigma_{0}$.

4.   Bifurcation control for the financial crises model (1.3)

In this section, we are to analyze the influence of time delay $\sigma$ on Hopf bifurcation for the fractional-order delayed controlled financial crises contagions model. we design a time delay feedback controller ^[16] which takes the form:

where $\theta$ is feedback gain coefficient.

Clearly, system (4.2) has the unique positive equilibrium point $U(u_{1*}, u_{2*})$ where $u_{1*} = \frac{\beta^2}{\alpha}, u_{2*} = \frac{\alpha}{\beta}.$

let

then system (4.2) is expressed as the following form:

The linear system of (4.4) near $(0, 0)$ owns the expression:

Let ${u}_i$ denote $\bar{u}_i(i = 1, 2)$, then system (4.5) becomes

The characteristic equation of Eq (4.6) is given by

which leads to

where

Assume that

holds.

Lemma 4.1. For system (4.2), the positive equilibrium point $U(u_{1*}, u_{2*})$ is locally asymptotically stable provided that $(K_4)$ holds true.

Proof. If $\sigma = 0,$ then (4.8) becomes

It follows from $(K_4)$ that every root $\lambda_j$ of (4.8) satisfies $|\mbox{arg}(\lambda_j)| > \frac{\mu\pi}{2} (j = 1, 2).$ By Lemma 3.1, one knows that the positive equilibrium point $U(u_{1*}, u_{2*})$ is locally asymptotically stable. The proof completes.

By (4.8), we have

Let $s = i\varrho = \varrho\left(\cos\frac{\pi}{2}+i\sin\frac{\pi}{2}\right)$ be the root of Eq. (4.11), then

where

It follows from (4.12) that

which leads to

For the convenience of calculation, we deal with this formula (4.13) properly. Let

then (4.13) becomes

Notice that

where

By (4.15) and (4.18), we get

where

Denote

Suppose that

By $(K_5)$, one derives $\upsilon_9 < 0$. Notice that $\frac{d\Phi(\varrho)}{d\varrho} > 0$, for each $\varrho > 0$, then Eq (4.20) has at least one positive real root. So, Eq (4.11) has at least a pair of purely roots.

Suppose that Eq (4.11) owns eight real roots (say $\varrho_j > 0(j = 1, 2, \cdots, 8)$. By (4.14), we have

where $i = 0, 1, 2, \cdots, j = 1, 2, \cdots, 8.$ Let

Assume that

where

Lemma 4.2. Suppose that $s(\sigma) = \eta_1(\sigma)+i\eta_2(\sigma)$ is the root of (4.11) near $\sigma = \sigma_{0}$ such that $\eta_1(\sigma_{0}) = 0, \eta_2(\sigma_{0}) = \varrho_{0}$, then $Re\left[\frac{ds}{d\sigma}\right]_{\sigma = \sigma_{0}, \varrho = \varrho_{0}} > 0.$

Proof. It follows from (4.11) that

It follows from (4.26) that

where

Then

Thus

Applying $(K_6)$, we have

which ends the proof.

By means of the analysis above, the following assertion holds.

Theorem 4.1. If $(K_4)$-$(K_6)$ are satisfied, then the positive equilibrium point $(u_{1*}, u_{2*})$ of system (4.2) is locally asymptotically stable if $0\leq\sigma < \sigma_{0}$ and system (4.2) generates Hopf bifurcation around the positive equilibrium point $(u_{1*}, u_{2*})$ when $\sigma$ passes through the delay value $\sigma_{0}$.

5.   Numerical examples

Example 5.1 Consider the following fractional-order financial crises contagions model:

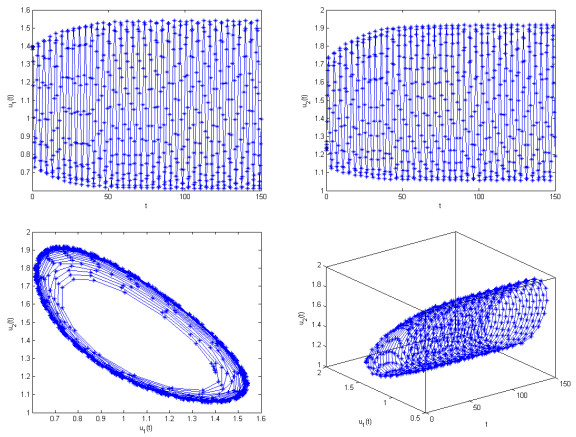
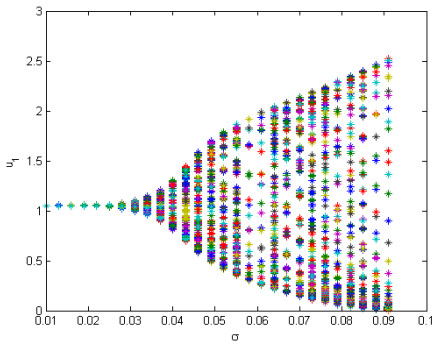
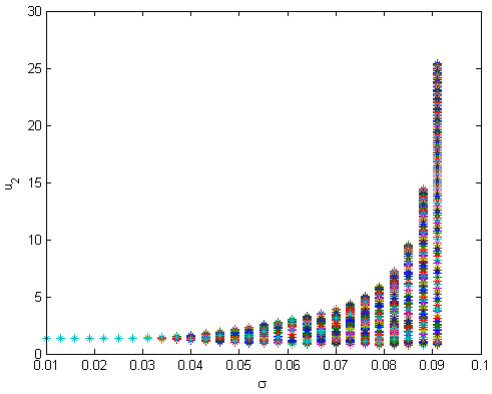
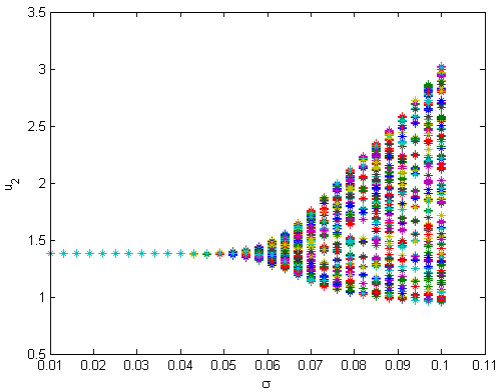
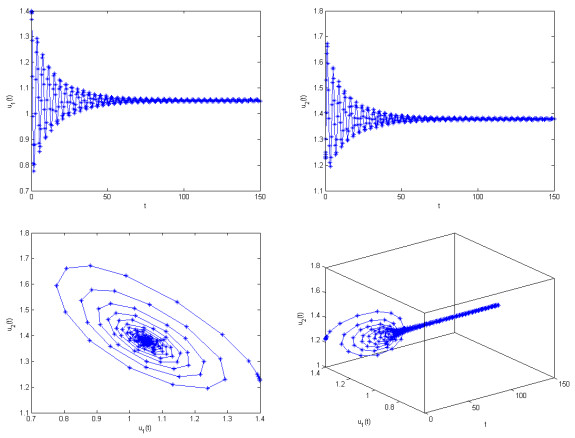
Apparently, system (5.1) owns the unique positive equilibrium point $(1.0513, 1.3793)$. Making use of Matlab software, we get $\gamma_0 = 0.5091$ and $\sigma_{0} = 0.0332$. The conditions $(K_1)$–$(K_3)$ of Theorem 3.1 are fulfilled. In order to check the stability of the positive equilibrium point $(1.0513, 1.3793)$ and the appearance of Hopf bifurcation of system (5.1), we choose two unequal delay values. Let $\sigma = 0.025 < \sigma_0 = 0.0332$, we obtain the computer simulation results that are presented in Figure 1. According to Figure 1, one can clearly see that the positive equilibrium point $(1.0513, 1.3793)$ keeps locally asymptotically stable situation. Figure 1 contains 4 subfigures. Subfigure 1 of Figure 1 shows that the state variable $u_1\rightarrow1.0513$ when the time increases. Subfigure 2 of Figure 1 implies that the state variable $u_2\rightarrow1.3793$ when the time increases. Subfigure 3 of Figure 1 manifests the numerical relation of $u_1$ and $u_2$. Subfigure 4 of Figure 1 display the numerical relation of $t$-$u_1$-$u_2$. Let $\sigma = 0.045 > \sigma_0 = 0.0332$, we get the computer simulation results which are presented in Figure 2. According to Figure 2, we can clearly see that a Hopf bifurcation arises around the positive equilibrium point $(1.0513, 1.3793)$. Figure 2 contains 4 subfigures. Subfigure 1 of Figure 2 implies that the state variable $u_1$ will keep a periodic oscillatory level around the value $1.0513$ when the time increases. Subfigures 2 of Figure 2 implies that the state variable $u_2$ will keep a periodic oscillatory state near the value $1.3793$ when the time increases. Subfigures 3 of Figure 2 manifests the numerical relation of $u_1$ and $u_2$. Subfigures 4 of Figure 2 displays the numerical relation of $t$-$u_1$-$u_2$. The correlation for $\mu$, $\gamma_0$ and $\sigma_{0}$ is listed in Table 1. Also, the bifurcation plots are presented to show that the bifurcation value is approximately equal to 0.0332 (see Figures 3 and 4).

Example 5.2 Consider the following fractional-order controlled financial crises contagions model with delays:

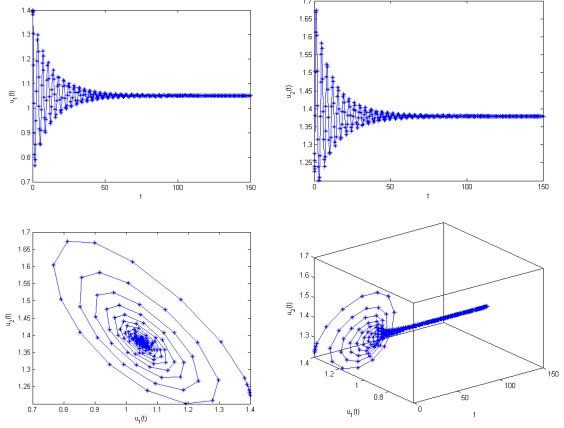
Apparently, system (5.2) owns the unique positive equilibrium point $(1.0513, 1.3793)$. Let $\theta = 3$. Making use of Matlab software, one gets $\varrho_0 = 0.9012$ and $\sigma_{0} = 0.0583$. The conditions $(K_4)$-$(K_6)$ in Theorem 4.1 are satisfied. In order to check the stability of the positive equilibrium point $(1.0513, 1.3793)$ and the onset of Hopf bifurcation of system (5.2), we choose two unequal delay values. Let $\sigma = 0.045 < \sigma_0 = 0.0583$, we get the computer simulation results that are presented in Figure 5. According to Figure 1, one can distinctly see that the equilibrium point $(1.0513, 1.3793)$ keeps locally asymptotically stable situation. Figure 5 includes 4 subfigures. Subfigure 1 of Figure 1 shows that the state variable $u_1\rightarrow1.0513$ when the time increases. Subfigure 2 of Figure 5 implies that the state variable $u_2\rightarrow1.3793$ when the time increases. Subfigure 3 of Figure 5 manifests the numerical relation of $u_1$ and $u_2$. Subfigure 4 of Figure 5 display the numerical relation of $t$-$u_1$-$u_2$. Let $\sigma = 0.075 > \sigma_0 = 0.0583$, we obtain the computer simulation results which are presented in Figure 6. According to Figure 6, we can clearly see that a Hopf bifurcation arises around the positive equilibrium point $(1.0513, 1.3793)$. Figure 2 contains 4 subfigures. Subfigure 1 of Figure 6 implies that the state variable $u_1$ will keep a periodic oscillatory level around the value $1.0513$ when the time increases. Subfigures 2 of Figure 6 implies that the state variable $u_2$ will keep a periodic oscillatory state near the value $1.3793$ when the time increases. Subfigures 3 of Figure 6 manifests the numerical relation of $u_1$ and $u_2$. Subfigures 4 of Figure 6 displays the numerical relation of $t$-$u_1$-$u_2$. The correlation for $\mu$, $\varrho_0$ and $\sigma_{0}$ is listed in Table 2. Also, the bifurcation plots are presented to show that the bifurcation value is approximately equal to 0.0583 (see Figures 7 and 8).

Remark 5.1 From the computer numerical simulation results of Example 5.1 and Example 5.2, we know that the stability region of system (5.1) is $[0, \sigma_{0} = 0.0332)$ and the Hopf bifurcation value is $0.0332$, the stability region of system (5.2) is $[0, \sigma_{0} = 0.0583)$ and the Hopf bifurcation value is $0.0583$. Thus the stability region of system (5.1) is enlarged and the time of the onset Hopf bifurcation is postponed by designing a suitable delayed feedback controller.

6.   Conclusions

Fractional-order differential system has displayed underlying application prospect in the economic sphere. Based on the previous publications, we propose a new fractional-order delayed financial crises contagions model. By virtue of the stability theory and bifurcation knowledge of fractional-order differential equation, we derive a novel delay-independent stability and bifurcation condition to remain the stability and generate Hopf bifurcation for the involved fractional-order delayed financial crises contagions model. Through designing an appropriate delayed feedback controller, we can successfully control the stability region and the time of onset of Hopf bifurcation for the involved fractional-order delayed financial crises model. The investigated fruits are helpful for us to grasp the inherent law of economic operation and then serve mankind effectively. Also, the research approach can be applied to control the dynamical peculiarity of numerous other dynamical models in lots of disciplines.

Acknowledgments

This work is supported by National Natural Science Foundation of China (No.61673008, No.62062018), Project of High-level Innovative Talents of Guizhou Province ([2016]5651), Key Project of Hunan Education Department (17A181), University Science and Technology Top Talents Project of Guizhou Province (KY[2018]047), Foundation of Science and Technology of Guizhou Province ([2019]1051), Guizhou University of Finance and Economics(2018XZD01). The authors would like to thank the referees and the editor for helpful suggestions incorporated into this paper.

Conflict of interest

The authors declare that they have no conflict of interest.