Pythagorean fuzzy sets in UP-algebras and approximations

Akarachai Satirad; Ronnason Chinram; Aiyared Iampan; Akarachai Satirad; Ronnason Chinram; Aiyared Iampan

doi:10.3934/math.2021354

AIMS Mathematics

2021, Volume 6, Issue 6: 6002-6032. doi: 10.3934/math.2021354

Previous Article Next Article

Research article

Pythagorean fuzzy sets in UP-algebras and approximations

1.
Department of Mathematics, School of Science, University of Phayao, Mae Ka, Phayao 56000, Thailand
2.
Algebra and Applications Research Unit, Division of Computational Science, Faculty of Science, Prince of Songkla University, Hat Yai, Songkhla 90110, Thailand

Received: 20 November 2020 Accepted: 25 March 2021 Published: 31 March 2021
MSC : 03E72, 03G25, 08A72

The aim of this paper is to apply the concept of Pythagorean fuzzy sets to UP-algebras, and then we introduce five types of Pythagorean fuzzy sets in UP-algebras. In addition, we will also discuss the relationship between some assertions of Pythagorean fuzzy sets and Pythagorean fuzzy UP-subalgebras (resp., Pythagorean fuzzy near UP-filters, Pythagorean fuzzy UP-filters, Pythagorean fuzzy UP-ideals, Pythagorean fuzzy strong UP-ideals) in UP-algebras and study upper and lower approximations of Pythagorean fuzzy sets.

Keywords:

Citation: Akarachai Satirad, Ronnason Chinram, Aiyared Iampan. Pythagorean fuzzy sets in UP-algebras and approximations[J]. AIMS Mathematics, 2021, 6(6): 6002-6032. doi: 10.3934/math.2021354

Related Papers:

[1]	Abdul Razaq, Ghaliah Alhamzi . On Pythagorean fuzzy ideals of a classical ring. AIMS Mathematics, 2023, 8(2): 4280-4303. doi: 10.3934/math.2023213
[2]	Supriya Bhunia, Ganesh Ghorai, Qin Xin . On the fuzzification of Lagrange's theorem in $(\alpha, \beta)$ -Pythagorean fuzzy environment. AIMS Mathematics, 2021, 6(9): 9290-9308. doi: 10.3934/math.2021540
[3]	Ayesha Shareef, Uzma Ahmad, Saba Siddique, Mohammed M. Ali Al-Shamiri . Pythagorean fuzzy incidence graphs with application in illegal wildlife trade. AIMS Mathematics, 2023, 8(9): 21793-21827. doi: 10.3934/math.20231112
[4]	Misbah Rasheed, ElSayed Tag-Eldin, Nivin A. Ghamry, Muntazim Abbas Hashmi, Muhammad Kamran, Umber Rana . Decision-making algorithm based on Pythagorean fuzzy environment with probabilistic hesitant fuzzy set and Choquet integral. AIMS Mathematics, 2023, 8(5): 12422-12455. doi: 10.3934/math.2023624
[5]	Supriya Bhunia, Ganesh Ghorai, Qin Xin . On the characterization of Pythagorean fuzzy subgroups. AIMS Mathematics, 2021, 6(1): 962-978. doi: 10.3934/math.2021058
[6]	Hifza, Muhammad Gulistan, Zahid Khan, Mohammed M. Al-Shamiri, Muhammad Azhar, Asad Ali, Joseph David Madasi . A new fuzzy decision support system approach; analysis and applications. AIMS Mathematics, 2022, 7(8): 14785-14825. doi: 10.3934/math.2022812
[7]	Li Li, Mengjing Hao . Interval-valued Pythagorean fuzzy entropy and its application to multi-criterion group decision-making. AIMS Mathematics, 2024, 9(5): 12511-12528. doi: 10.3934/math.2024612
[8]	D. Ajay, P. Chellamani, G. Rajchakit, N. Boonsatit, P. Hammachukiattikul . Regularity of Pythagorean neutrosophic graphs with an illustration in MCDM. AIMS Mathematics, 2022, 7(5): 9424-9442. doi: 10.3934/math.2022523
[9]	Saleem Abdullah, Muhammad Qiyas, Muhammad Naeem, Mamona, Yi Liu . Pythagorean Cubic fuzzy Hamacher aggregation operators and their application in green supply selection problem. AIMS Mathematics, 2022, 7(3): 4735-4766. doi: 10.3934/math.2022263
[10]	Esmail Hassan Abdullatif Al-Sabri, Muhammad Rahim, Fazli Amin, Rashad Ismail, Salma Khan, Agaeb Mahal Alanzi, Hamiden Abd El-Wahed Khalifa . Multi-criteria decision-making based on Pythagorean cubic fuzzy Einstein aggregation operators for investment management. AIMS Mathematics, 2023, 8(7): 16961-16988. doi: 10.3934/math.2023866

Abstract

1. Introduction and preliminaries

For the study of many algebraic structures, algebras of logic form important class of algebras. Examples of these are BCK-algebras ^[15], BCI-algebras ^[16], BE-algebras ^[19], UP-algebras ^[11], fully UP-semigroups ^[12], topological UP-algebras ^[27], UP-hyperalgebras ^[13], extension of KU/UP-algebras ^[24] and others.

The concept of rough sets was first introduced by Pawlak ^[22] in 1982. After the concept was introduced, several researchers were conducted on the generalizations of the concept of rough sets and application to many algebraic structures such as: in 2002, Jun ^[18] and Dudek et al. ^[7] applied rough set theory to BCK/BCI-algebras. In 2019-2020, Ansari et al. ^[2] and Klinseesook et al. ^[20] applied rough set theory to UP-algebras.

The concept of fuzzy sets was first introduced by Zadeh ^[35] in 1965. The fuzzy set theories developed by Zadeh and others have found many applications in the domain of mathematics and elsewhere. After the introduction of the concept of fuzzy sets by Zadeh ^[35], Atanassov ^[3] defined new concept called intuitionistic fuzzy set which is a generalization of fuzzy set, Yager ^[33] introduce a new class of non-standard fuzzy subsets called Pythagorean fuzzy subsets and the related idea of Pythagorean membership grades, and Satirad and Iampan ^[25] introduced several types of subsets and of fuzzy sets of fully UP-semigroups, and investigated the algebraic properties of fuzzy sets under the operations of intersection and union.

The concept of Pythagorean fuzzy sets was applied to semigroups, ternary semigroups, and many logical algebras such as: In 2019, Hussain et al. ^[9] present the concept of rough Pythagorean fuzzy ideals in semigroups. Then, this idea is extended to the lower and upper approximations of Pythagorean fuzzy left (resp., right) ideals, bi-ideals, interior ideals, (1, 2)-ideals in semigroups and some important properties related to these concepts are given. Jansi and Mohana ^[17] introduced the concepts of bipolar Pythagorean fuzzy A-ideals of BCI-algebras and investigated their properties. Also, relationship between bipolar Pythagorean fuzzy subalgebras, bipolar Pythagorean fuzzy ideals, and bipolar Pythagorean fuzzy A-ideals are analyzed. In 2020, Chinram and Panityakul ^[5] studied rough Pythagorean fuzzy ideals in ternary semigroups. This idea is extended to the lower and upper approximations of Pythagorean fuzzy ideals.

In this paper, we apply the concept of Pythagorean fuzzy sets to UP-algebras and investigate their properties. Also, we discuss the relationship between the Pythagorean UP-subalgebras, Pythagorean fuzzy near UP-filters, Pythagorean fuzzy UP-filters, Pythagorean fuzzy UP-ideals, and Pythagorean fuzzy strong UP-ideals. This idea is extended to the lower and upper approximations of Pythagorean fuzzy sets in UP-algebras.

Before we begin our study, let's review the definition of UP-algebras.

Definition 1.1. ^[11] An algebra $U = (U, \ast, 0)$ of type $(2, 0)$ is said to be a UP-algebra, where $U$ is a nonempty set, $\ast$ is a binary operation on $U$ , and $0$ is a fixed element of $U$ if it fulfills the following axioms:

(UP-1) $(\text{for all } x, y, z\in U)((y\ast z)\ast((x\ast y)\ast(x\ast z)) = 0)$ ,

(UP-2) $(\text{for all } x\in U)(0\ast x = x)$ ,

(UP-3) $(\text{for all } x\in U)(x\ast0 = 0)$ , and

(UP-4) $(\text{for all } x, y\in U)(x\ast y = 0, y\ast x = 0\Rightarrow x = y)$ ,

and is said to be a KU-algebra if it fulfills axioms (UP-2), (UP-3), (UP-4), and the following axiom:

(KU) $(\text{for all } x, y, z\in U)((x\ast y)\ast((y\ast z)\ast(x\ast z)) = 0)$ .

From ^[11], we know that the concept of UP-algebras is a generalization of KU-algebras (see ^[23]).

Example 1.2. ^[29] Let $U$ be a nonempty set and let $X\in\mathcal{P}(U)$ , where $\mathcal{P}(U)$ means the power set of $U$ . Let $\mathcal{P}_{X}(U) = \{A\in\mathcal{P}(U)\mid X\subseteq A\}$ . Define a binary operation $\vartriangle$ on $\mathcal{P}_{X}(U)$ by putting $A\vartriangle B = B\cap (A^{C}\cup X)$ for all $A, B\in\mathcal{P}_{X}(U)$ , where $A^{C}$ means the complement of a subset $A$ . Then $(\mathcal{P}_{X}(U), \vartriangle, X)$ is a UP-algebra. Let $\mathcal{P}^{X}(U) = \{A\in\mathcal{P}(U)\mid A\subseteq X\}$ . Define a binary operation $\blacktriangle$ on $\mathcal{P}^{X}(U)$ by putting $A\blacktriangle B = B\cup (A^{C}\cap X)$ for all $A, B\in\mathcal{P}^{X}(U)$ . Then $(\mathcal{P}^{X}(U), \blacktriangle, X)$ is a UP-algebra.

Example 1.3. ^[6] Let $\mathbb{N}_{0}$ be the set of all natural numbers with zero. Define two binary operations $\circ$ and $\star$ on $\mathbb{N}_{0}$ by

$\begin{align*} (\text{for all } m,n\in \mathbb{N}_{0})\left(m\circ n = \left\{ \begin{array}{ll} n & \text{if}\; m < n, \\ 0 & \text{otherwise} \end{array} \right.\right) \end{align*}$

and

$\begin{align*} (\text{for all } m,n\in \mathbb{N}_{0})\left( m\star n = \left\{ \begin{array}{ll} n & \text{if}\; m > n\; \text{or}\; m = 0, \\ 0 & \text{otherwise} \end{array} \right.\right). \end{align*}$

Then $(\mathbb{N}_{0}, \circ, 0)$ and $(\mathbb{N}_{0}, \star, 0)$ are UP-algebras.

For more examples of UP-algebras, see ^{[1,2,4,12,14,28,29,30,31]}.

In a UP-algebra $U = (U, \ast, 0)$ , the following axioms are valid (see ^[11,12]).

$\begin{align} & (\text{for all } x\in U)(x\ast x = 0), \end{align}$

(1)

$\begin{align} & (\text{for all } x,y,z\in U)(x\ast y = 0, y\ast z = 0 \Rightarrow x\ast z = 0), \\ & (\text{for all } x,y,z\in U)(x\ast y = 0 \Rightarrow (z\ast x)\ast (z\ast y) = 0), \end{align}$

(2)

$\begin{align} & (\text{for all } x,y,z\in U)(x\ast y = 0 \Rightarrow (y\ast z)\ast (x\ast z) = 0), \end{align}$

(3)

$\begin{align} & (\text{for all } x,y\in U)(x\ast (y\ast x) = 0), \end{align}$

(4)

$\begin{align} & (\text{for all } x,y\in U)((y\ast x)\ast x = 0 \Leftrightarrow x = y\ast x), \\ & (\text{for all } x,y\in U)(x\ast (y\ast y) = 0), \\ & (\text{for all } a,x,y,z\in U)((x\ast(y\ast z))\ast(x\ast((a\ast y)\ast (a\ast z))) = 0), \\ & (\text{for all } a,x,y,z\in U)((((a\ast x)\ast (a\ast y))\ast z)\ast((x\ast y)\ast z) = 0), \\ & (\text{for all } x,y,z\in U)(((x\ast y)\ast z)\ast(y\ast z) = 0), \\ & (\text{for all } x,y,z\in U)(x\ast y = 0 \Rightarrow x\ast (z\ast y) = 0), \\& (\text{for all } x,y,z\in U)(((x\ast y)\ast z)\ast(x\ast(y\ast z)) = 0),\, \text{and} \\ & (\text{for all } a,x,y,z\in U)(((x\ast y)\ast z)\ast(y\ast(a\ast z)) = 0). \end{align}$

(5)

From ^[11], the binary relation $\leq$ on a UP-algebra $U = (U, \ast, 0)$ is defined as follows:

$\begin{equation*} (\text{for all } x,y\in U)(x\leq y \Leftrightarrow x\ast y = 0). \end{equation*}$

In a KU-algebra $U = (U, \ast, 0)$ , the following axioms are valid (see ^[21]).

$\begin{align} & (\text{for all } x,y,z\in U)(x\ast(y\ast z) = y\ast(x\ast z)),\, \text{and} \\ & (\text{for all } x,y\in U)(y\ast((y\ast x)\ast x) = 0). \end{align}$

(6)

Theorem 1.4. ^[11] In a UP-algebra $U = (U, \ast, 0)$ , the following statements are equivalent:

(1) $U$ is a KU-algebra,

(2) $(\mathit{\text{for all}} \;x, y, z\in U)(x\ast(y\ast z) = y\ast(x\ast z))$ , and

(3) $(\mathit{\text{for all}} \;x, y, z\in U)(x\ast(y\ast z) = 0\Rightarrow y\ast(x\ast z) = 0)$ .

For a nonempty subset $S$ of a UP-algebra $U = (U, \ast, 0)$ which fulfills the following assertion:

$\begin{equation} (\text{for all } x,y\in U)(y\in S\Rightarrow x\ast y\in S). \end{equation}$

(7)

Then the constant $0$ of $U$ is in $S$ . Indeed, let $x\in S$ . By (1) and (7), we have $0 = x\ast x\in S$ .

Definition 1.5. ^[8,10,11,32] A nonempty subset $S$ of a UP-algebra $U = (U, \ast, 0)$ is said to be

(1) a UP-subalgebra of $U$ if it fulfills the following assertion:

$\begin{equation*} \label{Ss4_1} (\text{for all } x,y\in S)(x\ast y\in S), \end{equation*}$

(2) a near UP-filter of $U$ if it fulfills the assertion (7),

(3) a UP-filter of $U$ if it fulfills the following assertions:

$\begin{gather} \text{the constant}\; 0\; \text{of}\; U\; \text{is in}\; S, \\ (\text{for all } x,y\in U)(x\ast y\in S,x\in S\Rightarrow y\in S), \end{gather}$

(8)

(4) a UP-ideal of $U$ if it fulfills the assertion (8) and the following assertion:

$\begin{equation*} \label{Ss2_1} (\text{for all } x,y,z\in U)(x\ast(y\ast z)\in S,y\in S\Rightarrow x\ast z\in S), \end{equation*}$

(5) a strong UP-ideal of $U$ if it fulfills the assertion (8) and the following assertion:

$\begin{equation*} \label{Ss3_1} (\text{for all } x,y,z\in U)((z\ast y)\ast(z\ast x)\in S,y\in S\Rightarrow x\in S). \end{equation*}$

Guntasow et al. ^[8] and Iampan ^[10] proved that the concept of UP-subalgebras is a generalization of near UP-filters, near UP-filters is a generalization of UP-filters, UP-filters is a generalization of UP-ideals, and UP-ideals is a generalization of strong UP-ideals. Furthermore, they proved that the only strong UP-ideal of a UP-algebra $U$ is $U$ .

Definition 1.6. ^[35] A fuzzy set $\mathrm{F}$ in a nonempty set $U$ (or a fuzzy subset of $U$ ) is described by its membership function $\mathrm{f_{F}}$ . To every point $x\in U$ , this function associates a real number $\mathrm{f_{F}}(x)$ in the closed interval $[0, 1]$ . The real number $\mathrm{f_{F}}(x)$ is interpreted for the point as a degree of membership of an object $x\in U$ to the fuzzy set $\mathrm{F}$ , that is, $\mathrm{F}: = \{(x, \mathrm{f_{F}}(x))\mid x\in U\}$ . We say that a fuzzy set $\mathrm{F}$ in $U$ is constant if its membership function $\mathrm{f_{F}}$ is constant.

Definition 1.7. ^[35] Let $\mathrm{F}$ be a fuzzy set in a nonempty set $U$ . The complement of $\mathrm{F}$ , denoted by $\mathrm{\widetilde{F}}$ , is described by its membership function $\mathrm{f_{\widetilde{F}}}$ which defined as follows:

$\begin{equation*} (\text{for all } x\in U)(\mathrm{f_{\widetilde{F}}}(x) = 1-\mathrm{f_{F}}(x)). \end{equation*}$

The following two propositions are easy to verify.

Proposition 1.8. Let $\mathrm{F}$ be a fuzzy set in a nonempty set $U$ . Then following assertions are valid:

(1) $(\mathit{\text{for all}} \;x, y\in U)(\mathrm{f_{F}}(x)\leq\mathrm{f_{F}}(y)\Leftrightarrow\mathrm{f_{\widetilde{F}}}(x)\geq\mathrm{f_{\widetilde{F}}}(y))$ ,

(2) $(\mathit{\text{for all}} \;x, y\in U)(\mathrm{f_{F}}(x) = \mathrm{f_{F}}(y)\Leftrightarrow\mathrm{f_{\widetilde{F}}}(x) = \mathrm{f_{\widetilde{F}}}(y))$ ,

(3) $\mathrm{\widetilde{\widetilde{F}}} = \mathrm{F}$ , and

(4) $(\mathit{\text{for all}} \;x, y\in U)(1-\min\{\mathrm{f_{F}}(x), \mathrm{f_{F}}(y)\} = \max\{\mathrm{f_{\widetilde{F}}}(x), \mathrm{f_{\widetilde{F}}}(y)\})$ .

Proposition 1.9. Let $\{\mathrm{F}_{i}\}_{i\in I}$ be a nonempty family of fuzzy sets in a nonempty set $U$ , where $I$ is an arbitrary index set. Then following assertions are valid:

(1) $(\mathit{\text{for all}}\; x, y\in U)(\inf\limits_{i\in I}\{\min\{\mathrm{f_{F}}_{i}(x), \mathrm{f_{F}}_{i}(y)\}\} = \min\{\inf\limits_{i\in I}\{\mathrm{f_{F}}_{i}(x)\}, \inf\limits_{i\in I}\{\mathrm{f_{F}}_{i}(y)\}\})$ ,

(2) $(\mathit{\text{for all}}\; x, y\in U)(\sup\limits_{i\in I}\{\max\{\mathrm{f_{F}}_{i}(x), \mathrm{f_{F}}_{i}(y)\}\} = \max\{\sup\limits_{i\in I}\{\mathrm{f_{F}}_{i}(x)\}, \sup\limits_{i\in I}\{\mathrm{f_{F}}_{i}(y)\}\})$ ,

(3) $(\mathit{\text{for all}}\; x, y\in U)(\inf\limits_{i\in I}\{\max\{\mathrm{f_{F}}_{i}(x), \mathrm{f_{F}}_{i}(y)\}\}\geq\max\{\inf\limits_{i\in I}\{\mathrm{f_{F}}_{i}(x)\}, \inf\limits_{i\in I}\{\mathrm{f_{F}}_{i}(y)\}\})$ ,

(4) $(\mathit{\text{for all}}\; x, y\in U)(\sup\limits_{i\in I}\{\min\{\mathrm{f_{F}}_{i}(x), \mathrm{f_{F}}_{i}(y)\}\}\leq\min\{\sup\limits_{i\in I}\{\mathrm{f_{F}}_{i}(x)\}, \sup\limits_{i\in I}\{\mathrm{f_{F}}_{i}(y)\}\})$ ,

(5) $(\mathit{\text{for all}}\; x\in U)((\sup\limits_{i\in I}\{\mathrm{f}_{\mathrm{F}_{i}}(x)\})^{2} = \sup\limits_{i\in I}\{\mathrm{f}_{\mathrm{F}_{i}}(x)^{2}\})$ ,

(6) $(\mathit{\text{for all}}\; x\in U)((\inf\limits_{i\in I}\{\mathrm{f}_{\mathrm{F}_{i}}(x)\})^{2} = \inf\limits_{i\in I}\{\mathrm{f}_{\mathrm{F}_{i}}(x)^{2}\})$ ,

(7) $(\mathit{\text{for all}}\; x\in U)(1-\sup\limits_{i\in I}\{\mathrm{f}_{\mathrm{F}_{i}}(x)\} = \inf\limits_{i\in I}\{1-\mathrm{f}_{\mathrm{F}_{i}}(x)\})$ , and

(8) $(\mathit{\text{for all}}\; x\in U)(1-\inf\limits_{i\in I}\{\mathrm{f}_{\mathrm{F}_{i}}(x)\} = \sup\limits_{i\in I}\{1-\mathrm{f}_{\mathrm{F}_{i}}(x)\})$ .

For a fuzzy set $\mathrm{F}$ in a UP-algebra $U = (U, \ast, 0)$ which fulfills the following assertion:

$\begin{equation} (\text{for all } x,y\in U)(\mathrm{f_{F}}(x\ast y)\geq\mathrm{f_{F}}(y)). \end{equation}$

(9)

Then

$\begin{equation*} (\text{for all } x\in U)(\mathrm{f_{F}}(0)\geq\mathrm{f_{F}}(x)). \end{equation*}$

Indeed, let $x\in U$ . By (1) and (9), we have $\mathrm{f_{F}}(0) = \mathrm{f_{F}}(x\ast x)\geq\mathrm{f_{F}}(x)$ .

Definition 1.10. ^[8,26,32] A fuzzy set $\mathrm{F}$ in a UP-algebra $U = (U, \ast, 0)$ is said to be

(1) a fuzzy UP-subalgebra of $U$ if it fulfills the following assertion:

$\begin{equation} (\text{for all } x,y\in U)(\mathrm{f_{F}}(x\ast y)\geq \min\{\mathrm{f_{F}}(x),\mathrm{f_{F}}(y)\}), \end{equation}$

(10)

(2) a fuzzy near UP-filter of $U$ if it fulfills the assertion (9),

(3) a fuzzy UP-filter of $U$ if it fulfills the following assertions:

$\begin{gather} (\text{for all } x\in U)(\mathrm{f_{F}}(0)\geq \mathrm{f_{F}}(x)), \end{gather}$

(11)

$\begin{gather} (\text{for all } x,y\in U)(\mathrm{f_{F}}(y)\geq \min\{\mathrm{f_{F}}(x\ast y),\mathrm{f_{F}}(x)\}), \end{gather}$

(12)

(4) a fuzzy UP-ideal of $U$ if it fulfills the assertion (11) and the following assertion:

$\begin{equation} (\text{for all } x,y,z\in U)(\mathrm{f_{F}}(x\ast z)\geq \min\{\mathrm{f_{F}}(x\ast(y\ast z)),\mathrm{f_{F}}(y)\}), \end{equation}$

(13)

(5) a fuzzy strong UP-ideal of $U$ if it fulfills the assertion (11) and the following assertion:

$\begin{equation} (\text{for all } x,y,z\in U)(\mathrm{f_{F}}(x)\geq\min\{\mathrm{f_{F}}((z\ast y)\ast(z\ast x)),\mathrm{f_{F}}(y)\}). \end{equation}$

(14)

Guntasow et al. ^[8], and Satirad and Iampan ^[26] proved that the concept of fuzzy UP-subalgebras is a generalization of fuzzy near UP-filters, fuzzy near UP-filters is a generalization of fuzzy UP-filters, fuzzy UP-filters is a generalization of fuzzy UP-ideals, and fuzzy UP-ideals is a generalization of fuzzy strong UP-ideals. Furthermore, they proved that fuzzy strong UP-ideals and constant fuzzy sets coincide in a UP-algebras $U$ .

2. Pythagorean fuzzy sets in UP-algebras

In 2013, Yager ^[33] and Yager and Abbasov ^[34] introduced the concept of Pythagorean fuzzy sets for the first time.

Definition 2.1. ^[33,34] A Pythagorean fuzzy set $\mathrm{P}$ in a nonempty set $U$ is described by their membership function $\mu_{\mathrm{P}}$ and non-membership function $\nu_{\mathrm{P}}$ . To every point $x\in U$ , these functions associate real numbers $\mu_{\mathrm{P}}(x)$ and $\nu_{\mathrm{P}}(x)$ in the closed interval $[0, 1]$ , with the following assertion:

$\begin{equation*} \label{PFS_1} (\text{for all } x\in U)(0\leq\mu_{\mathrm{P}}(x)^{2}+\nu_{\mathrm{P}}(x)^{2}\leq1). \end{equation*}$

The real numbers $\mu_{\mathrm{P}}(x)$ and $\nu_{\mathrm{P}}(x)$ are interpreted for the point as a degree of membership and non-membership of an object $x\in U$ , respectively, to the Pythagorean fuzzy set $\mathrm{P}$ , that is, $\mathrm{P}: = \{(x, \mu_{\mathrm{P}}(x), \nu_{\mathrm{P}}(x))\mid x\in U\}$ . For the sake of simplicity, a Pythagorean fuzzy set $\mathrm{P}$ is denoted by $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ . We say that a Pythagorean fuzzy set $\mathrm{P}$ in $U$ is constant if their membership function $\mu_{\mathrm{P}}$ and non-membership function $\nu_{\mathrm{P}}$ are constant.

We apply the concept of Pythagorean fuzzy sets to UP-algebras and introduce the five types of Pythagorean fuzzy sets in UP-algebras.

Definition 2.2. A Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ in a UP-algebra $U = (U, \ast, 0)$ is said to be

(1) a Pythagorean fuzzy UP-subalgebra of $U$ if it fulfills the following assertions:

$\begin{gather} (\text{for all } x,y\in U)(\mu_{\mathrm{P}}(x\ast y)\geq \min\{\mu_{\mathrm{P}}(x),\mu_{\mathrm{P}}(y)\}), \end{gather}$

(15)

$\begin{gather} (\text{for all } x,y\in U)(\nu_{\mathrm{P}}(x\ast y)\leq \max\{\nu_{\mathrm{P}}(x),\nu_{\mathrm{P}}(y)\}), \end{gather}$

(16)

(2) a Pythagorean fuzzy near UP-filter of $U$ if it fulfills the following assertions:

$\begin{gather} (\text{for all } x,y\in U)(\mu_{\mathrm{P}}(x\ast y)\geq\mu_{\mathrm{P}}(y)), \end{gather}$

(17)

$\begin{gather} (\text{for all } x,y\in U)(\nu_{\mathrm{P}}(x\ast y)\leq \nu_{\mathrm{P}}(y)), \end{gather}$

(18)

(3) a Pythagorean fuzzy UP-filter of $U$ if it fulfills the following assertions:

$\begin{gather} (\text{for all } x\in U)(\mu_{\mathrm{P}}(0)\geq\mu_{\mathrm{P}}(x)), \end{gather}$

(19)

$\begin{gather} (\text{for all } x\in U)(\nu_{\mathrm{P}}(0)\leq \nu_{\mathrm{P}}(x)), \end{gather}$

(20)

$\begin{gather} (\text{for all } x,y\in U)(\mu_{\mathrm{P}}(y)\geq\min\{\mu_{\mathrm{P}}(x\ast y),\mu_{\mathrm{P}}(x)\}), \end{gather}$

(21)

$\begin{gather} (\text{for all } x,y\in U)(\nu_{\mathrm{P}}(y)\leq\max\{\nu_{\mathrm{P}}(x\ast y),\nu_{\mathrm{P}}(x)\}), \end{gather}$

(22)

(4) a Pythagorean fuzzy UP-ideal of $U$ if it fulfills the assertions (19) and (20) and the following assertions:

$\begin{gather} (\text{for all } x,y,z\in U)(\mu_{\mathrm{P}}(x\ast z)\geq \min\{\mu_{\mathrm{P}}(x\ast(y\ast z)),\mu_{\mathrm{P}}(y)\}), \end{gather}$

(23)

$\begin{gather} (\text{for all } x,y,z\in U)(\nu_{\mathrm{P}}(x\ast z)\leq \max\{\nu_{\mathrm{P}}(x\ast(y\ast z)),\nu_{\mathrm{P}}(y)\}), \end{gather}$

(24)

(5) a Pythagorean strong fuzzy UP-ideal of $U$ if it fulfills the assertions (19) and (20) and the following assertions:

$\begin{gather} (\text{for all } x,y,z\in U)(\mu_{\mathrm{P}}(x)\geq\min\{\mu_{\mathrm{P}}((z\ast y)\ast(z\ast x)),\mu_{\mathrm{P}}(y)\}), \end{gather}$

(25)

$\begin{gather} (\text{for all } x,y,z\in U)(\nu_{\mathrm{P}}(x)\leq\max\{\nu_{\mathrm{P}}((z\ast y)\ast(z\ast x)),\nu_{\mathrm{P}}(y)\}). \end{gather}$

(26)

From now on, we shall let $U$ be a UP-algebra $U = (U, \ast, 0)$ .

Theorem 2.3. A Pythagorean fuzzy set in $U$ is a Pythagorean fuzzy strong UP ideal if and only if it is constant.

Proof. Assume that $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy strong UP ideal of $U$ . Then it fulfills (19) and (20). Thus for all $x\in U$ ,

$\begin{align*} \mu_{\mathrm{P}}(x) &\geq\min\{\mu_{\mathrm{P}}((x\ast 0)\ast(x\ast x)),\mu_{\mathrm{P}}(0)\}&\text{by (25)}\\ & = \min\{\mu_{\mathrm{P}}(0\ast(x\ast x)),\mu_{\mathrm{P}}(0)\}&\text{by (UP-3)}\\ & = \min\{\mu_{\mathrm{P}}(x\ast x),\mu_{\mathrm{P}}(0)\}&\text{by (UP-2)}\\ & = \min\{\mu_{\mathrm{P}}(0),\mu_{\mathrm{P}}(0)\}&\text{by (1)}\\ & = \mu_{\mathrm{P}}(0) \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(x) &\leq\max\{\nu_{\mathrm{P}}((x\ast 0)\ast(x\ast x)),\nu_{\mathrm{P}}(0)\}&\text{by (26)}\\ & = \max\{\nu_{\mathrm{P}}(0\ast(x\ast x)),\nu_{\mathrm{P}}(0)\}&\text{by (UP-3)}\\ & = \max\{\nu_{\mathrm{P}}(x\ast x),\nu_{\mathrm{P}}(0)\}&\text{by (UP-2)}\\ & = \max\{\nu_{\mathrm{P}}(0),\nu_{\mathrm{P}}(0)\}&\text{by (1)}\\ & = \nu_{\mathrm{P}}(0). \end{align*}$

Since $\mu_{\mathrm{P}}(0)\geq\mu_{\mathrm{P}}(x)$ and $\nu_{\mathrm{P}}(0)\leq\nu_{\mathrm{P}}(x)$ , we have $\mu_{\mathrm{P}}(x) = \mu_{\mathrm{P}}(0)$ and $\nu_{\mathrm{P}}(x) = \nu_{\mathrm{P}}(0)$ for all $x\in U$ . Hence, $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ are constant, that is, $\mathrm{P}$ is constant.

The converse is evident because $\mathrm{P}$ is constant.

Theorem 2.4. Every Pythagorean fuzzy near UP-filter of $U$ is a Pythagorean fuzzy UP-subalgebra.

Proof. Let $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ be a Pythagorean fuzzy near UP-filter of $U$ . Then for all $x, y\in U$ ,

$\begin{align*} \mu_{\mathrm{P}}(x\ast y) \geq\mu_{\mathrm{P}}(y)\;\;\;\;\;\;\;\;\text{by (17)} \geq\min\{\mu_{\mathrm{P}}(x),\mu_{\mathrm{P}}(y)\} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(x\ast y) \leq \nu_{\mathrm{P}}(y)\;\;\;\;\;\;\;\;\text{by (18)} \leq\max\{\nu_{\mathrm{P}}(x),\nu_{\mathrm{P}}(y)\}. \end{align*}$

Therefore, $\mathrm{P}$ is a Pythagorean fuzzy UP-subalgebra of $U$ .

The converse of Theorem 2.4 does not hold in general. This is shown by the following example.

Example 2.5. Let $U = \{0, 1, 2, 3\}$ be a UP-algebra with a fixed element $0$ and a binary operation $\ast$ defined by the following Cayley table:

$\begin{equation*} \begin{array}{c|cccc} \ast & 0 & 1 & 2 & 3 \\ \hline 0 & 0 & 1 & 2 & 3 \\ 1 & 0 & 0 & 1 & 3 \\ 2 & 0 & 0 & 0 & 3 \\ 3 & 0 & 1 & 1 & 0 \end{array} \end{equation*}$

We define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 0.9 & 0.7 & 0.8 & 0.5 \\ \nu_{\mathrm{P}} & 0 & 0.4 & 0.1 & 0.6 \end{array} \end{equation*}$

Then $\mathrm{P}$ is a Pythagorean fuzzy UP-subalgebra of $U$ . Since $\mu_{\mathrm{P}}(3\ast2) = \mu_{\mathrm{P}}(1) = 0.7\not\geq0.8 = \mu_{\mathrm{P}}(2)$ , we have $\mathrm{P}$ is not a Pythagorean fuzzy near UP-filter of $U$ .

Theorem 2.6. Every Pythagorean fuzzy UP-filter of $U$ is a Pythagorean fuzzy near UP-filter.

Proof. Let $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ be a Pythagorean fuzzy UP-filter of $U$ . Then for all $x, y\in U$ ,

$\begin{align*} \mu_{\mathrm{P}}(x\ast y) \geq\min\{\mu_{\mathrm{P}}(y\ast(x\ast y)),\mu_{\mathrm{P}}(y)\} = \min\{\mu_{\mathrm{P}}(0),\mu_{\mathrm{P}}(y)\}\;\;\;\;\;\;\;\;\text{by (21), (4)} = \mu_{\mathrm{P}}(y) \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(x\ast y) \leq\max\{\nu_{\mathrm{P}\nu}(y\ast(x\ast y)),\nu_{\mathrm{P}}(y)\} = \max\{\nu_{\mathrm{P}}(0),\nu_{\mathrm{P}}(y)\}\;\;\;\;\;\;\;\;\text{by (22), (4)} = \nu_{\mathrm{P}}(y). \end{align*}$

Therefore, $\mathrm{P}$ is a Pythagorean fuzzy near UP-filter of $U$ .

The converse of Theorem 2.6 does not hold in general. This is shown by the following example.

Example 2.7. Let $U = \{0, 1, 2, 3\}$ be a UP-algebra with a fixed element $0$ and a binary operation $\ast$ defined by the following Cayley table:

$\begin{equation*} \begin{array}{c|cccc} \ast & 0 & 1 & 2 & 3 \\ \hline 0 & 0 & 1 & 2 & 3 \\ 1 & 0 & 0 & 2 & 3 \\ 2 & 0 & 0 & 0 & 3 \\ 3 & 0 & 0 & 0 & 0 \end{array} \end{equation*}$

We define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 1 & 0.7 & 0.8 & 0.75 \\ \nu_{\mathrm{P}} & 0 & 0.6 & 0.3 & 0.4 \end{array} \end{equation*}$

Then $\mathrm{P}$ is a Pythagorean fuzzy near UP-filter of $U$ . Since $\mu_{\mathrm{P}}(1) = 0.7\not\geq0.75 = \min\{1, 0.75\} = \min\{\mu_{\mathrm{P}}(0), \mu_{\mathrm{P}}(3)\} = \min\{\mu_{\mathrm{P}}(3\ast1), \mu_{\mathrm{P}}(3)\}$ , we have $\mathrm{P}$ is not a Pythagorean fuzzy UP-filter of $U$ .

Theorem 2.8. Every Pythagorean fuzzy UP-ideal of $U$ is a Pythagorean fuzzy UP-filter.

Proof. Let $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ be a Pythagorean fuzzy UP-ideal of $U$ . It is sufficient to prove the assertions (21) and (22). Then for all $x, y\in U$ ,

$\begin{align*} \mu_{\mathrm{P}}(y) = \mu_{\mathrm{P}}(0\ast y) \geq\min\{\mu_{\mathrm{P}}(0\ast(x\ast y)),\mu_{\mathrm{P}}(x)\} = \min\{\mu_{\mathrm{P}}(x\ast y),\mu_{\mathrm{P}}(x)\}\;\;\;\;\;\;\;\;\text{by (UP-2), (23)} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(y) = \nu_{\mathrm{P}}(0\ast y) \leq\max\{\nu_{\mathrm{P}}(0\ast(x\ast y)),\nu_{\mathrm{P}}(x)\} = \max\{\nu_{\mathrm{P}}(x\ast y),\nu_{\mathrm{P}}(x)\}.\;\;\;\;\;\;\;\;\text{by (UP-2), (24)} \end{align*}$

Therefore, $\mathrm{P}$ is a Pythagorean fuzzy UP-filter of $U$ .

The converse of Theorem 2.8 does not hold in general. This is shown by the following example.

Example 2.9. Let $U = \{0, 1, 2, 3\}$ be a UP-algebra with a fixed element $0$ and a binary operation $\ast$ defined by the following Cayley table:

$\begin{equation*} \begin{array}{c|cccc} \ast & 0 & 1 & 2 & 3 \\ \hline 0 & 0 & 1 & 2 & 3 \\ 1 & 0 & 0 & 2 & 2 \\ 2 & 0 & 1 & 0 & 2 \\ 3 & 0 & 1 & 0 & 0 \end{array} \end{equation*}$

We define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 0.9 & 0.5 & 0.2 & 0.2 \\ \nu_{\mathrm{P}} & 0.1 & 0.4 & 0.5 & 0.5 \end{array} \end{equation*}$

Then $\mathrm{P}$ is a Pythagorean fuzzy UP-filter of $U$ . Since $\mu_{\mathrm{P}}(2\ast3) = \mu_{\mathrm{P}}(2) = 0.2\not\geq0.5 = \min\{0.9, 0.5\} = \min\{\mu_{\mathrm{P}}(0), \mu_{\mathrm{P}}(1)\} = \min\{\mu_{\mathrm{P}}(2\ast(1\ast3)), \mu_{\mathrm{P}}(1)\}$ , we have $\mathrm{P}$ is not a Pythagorean fuzzy UP-ideal of $U$ .

Theorem 2.10. Every Pythagorean fuzzy strong UP-ideal of $U$ is a Pythagorean fuzzy UP-ideal.

Proof. Let $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ be a Pythagorean fuzzy strong UP-ideal of $U$ . By Theorem 2.3, we have $\mathrm{P}$ is constant. Therefore, it is evident that $\mathrm{P}$ is a Pythagorean fuzzy UP-ideal of $U$ .

The converse of Theorem 2.10 does not hold in general. This is shown by the following example.

Example 2.11. Let $U = \{0, 1, 2, 3\}$ be a UP-algebra with a fixed element $0$ and a binary operation $\ast$ defined by the following Cayley table:

$\begin{equation*} \begin{array}{c|cccc} \ast & 0 & 1 & 2 & 3 \\ \hline 0 & 0 & 1 & 2 & 3 \\ 1 & 0 & 0 & 2 & 3 \\ 2 & 0 & 1 & 0 & 3 \\ 3 & 0 & 1 & 2 & 0 \end{array} \end{equation*}$

We define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 1 & 0.5 & 0.2 & 0.7 \\ \nu_{\mathrm{P}} & 0 & 0.6 & 0.8 & 0.4 \end{array} \end{equation*}$

Then $\mathrm{P}$ is a Pythagorean fuzzy UP-ideal of $U$ . But $\mathrm{P}$ is not constant and by Theorem 2.3, we have $\mathrm{P}$ is not a Pythagorean fuzzy strong UP-ideal of $U$ .

Theorem 2.12. Let $\mathrm{F}$ be a fuzzy set in $U$ . Then the following statements hold:

(1) $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy set in $U$ ,

(2) $\mathrm{F}$ is a fuzzy UP-subalgebra of $U$ if and only if $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy UP-subalgebra of $U$ ,

(3) $\mathrm{F}$ is a fuzzy near UP-filter of $U$ if and only if $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy near UP-filter of $U$ ,

(4) $\mathrm{F}$ is a fuzzy UP-filter of $U$ if and only if $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy UP-filter of $U$ ,

(5) $\mathrm{F}$ is a fuzzy UP-ideal of $U$ if and only if $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy UP-ideal of $U$ , and

(6) $\mathrm{F}$ is a fuzzy strong UP-ideal of $U$ if and only if $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy strong UP-ideal of $U$ .

Proof. (1) Let $x\in U$ . Then $0\leq\mathrm{f_{F}}(x)^{2}+\mathrm{f_{\widetilde{F}}}(x)^{2} = \mathrm{f_{F}}(x)^{2}+(1-\mathrm{f_{F}}(x))^{2}\leq\mathrm{f_{F}}(x)+(1-\mathrm{f_{F}}(x)) = 1$ . Hence, $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy set in $U$ .

(2) Assume that $\mathrm{F}$ is a fuzzy UP-subalgebra of $U$ . Then for all $x, y\in U$ ,

$\begin{align*} \mathrm{f_{F}}(x\ast y)\geq\min\{\mathrm{f_{F}}(x),\mathrm{f_{F}}(y)\}\;\;\;\;\;\;\;\;\text{by (10)} \end{align*}$

and

$\begin{align*} \mathrm{f_{\widetilde{F}}}(x\ast y) = 1-\mathrm{f_{F}}(x\ast y) \leq1-\min\{\mathrm{f_{F}}(x),\mathrm{f_{F}}(y)\} = \max\{\mathrm{f_{\widetilde{F}}}(x),\mathrm{f_{\widetilde{F}}}(y)\}.\;\;\;\;\;\;\;\;\text{by Proposition 1.8 (4), (10)} \end{align*}$

This implies that $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy UP-subalgebra of $U$ .

Conversely, assume that $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy UP-subalgebra of $U$ . Then $\mathrm{F}$ fulfills the assertion (15). Hence, $\mathrm{F}$ is a fuzzy UP-subalgebra of $U$ .

(3) Assume that $\mathrm{F}$ is a fuzzy near UP-filter of $U$ . Then for all $x, y\in U$ ,

$\begin{align*} \mathrm{f_{F}}(x\ast y)\geq\mathrm{f_{F}}(y)\;\;\;\;\;\;\;\;\text{by (9)} \end{align*}$

and

$\begin{align*} \mathrm{f_{\widetilde{F}}}(x\ast y)\leq \mathrm{f_{\widetilde{F}}}(y).\;\;\;\;\;\;\;\;\text{by Proposition 1.8 (1)} \end{align*}$

This implies that $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy near UP-filter of $U$ .

Conversely, assume that $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy near UP-filter of $U$ . Then $\mathrm{F}$ fulfills the assertion (17). Hence, $\mathrm{F}$ is a fuzzy near UP-filter of $U$ .

(4) Assume that $\mathrm{F}$ is a fuzzy UP-filter of $U$ . Then for all $x, y\in U$ ,

$\begin{gather*} \mathrm{f_{F}}(0)\geq\mathrm{f_{F}}(x),&\text{by (11)}\\ \mathrm{f_{\widetilde{F}}}(0)\leq\mathrm{f_{\widetilde{F}}}(x),&\text{by Proposition 1.8 (1)}\\ \mathrm{f_{F}}(y)\geq\min\{\mathrm{f_{F}}(x\ast y),\mathrm{f_{F}}(x)\},&\text{by (12)} \end{gather*}$

and

$\begin{align*} \mathrm{f_{\widetilde{F}}}(y) = 1-\mathrm{f_{F}}(y) \leq1-\min\{\mathrm{f_{F}}(x\ast y),\mathrm{f_{F}}(x)\} = \max\{\mathrm{f_{\widetilde{F}}}(x\ast y),\mathrm{f_{\widetilde{F}}}(x)\}.\;\;\;\;\;\;\;\;\text{by (12), Proposition 1.8 (4)} \end{align*}$

This implies that $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy UP-filter of $U$ .

Conversely, assume that $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy UP-filter of $U$ . Then $\mathrm{F}$ fulfills the assertions (19) and (21). Hence, $\mathrm{F}$ is a fuzzy UP-filter of $U$ .

(5) Assume that $\mathrm{F}$ is a fuzzy UP-ideal of $U$ . Then for all $x, y\in U$ ,

$\begin{gather*} \mathrm{f_{F}}(0)\geq\mathrm{f_{F}}(x),&\text{by (11)}\\ \mathrm{f_{\widetilde{F}}}(0)\leq\mathrm{f_{\widetilde{F}}}(x),&\text{by Proposition 1.8 (1)}\\ \mathrm{f_{F}}(x\ast z)\geq\min\{\mathrm{f_{F}}(x\ast(y\ast z)),\mathrm{f_{F}}(y)\},&\text{by (13)} \end{gather*}$

and

$\begin{align*} \mathrm{f_{\widetilde{F}}}(x\ast z) = 1-\mathrm{f_{F}}(x\ast z) \leq1-\min\{\mathrm{f_{F}}(x\ast(y\ast z)),\mathrm{f_{F}}(y)\} = \max\{\mathrm{f_{\widetilde{F}}}(x\ast(y\ast z)),\mathrm{f_{\widetilde{F}}}(y)\}.\;\;\;\;\;\;\;\;\text{by (13), Proposition 1.8 (4)} \end{align*}$

This implies that $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy UP-ideal of $U$ .

Conversely, assume that $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy UP-ideal of $U$ . Then $\mathrm{F}$ fulfills the assertions (19) and (23). Hence, $\mathrm{F}$ is a fuzzy UP-ideal of $U$ .

(6) Assume that $\mathrm{F}$ is a fuzzy strong UP-ideal of $U$ . Then $\mathrm{f_{F}}$ is constant and so $\mathrm{f_{\widetilde{F}}}$ is constant. By Theorem 2.3, we have $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy strong UP-ideal of $U$ .

Conversely, assume that $(\mathrm{f_{F}}, \mathrm{f_{\widetilde{F}}})$ is a Pythagorean fuzzy strong UP-ideal of $U$ . By Theorem 2.3, we have $\mathrm{f_{F}}$ is constant. Hence, $\mathrm{F}$ is a fuzzy strong UP-ideal of $U$ .

3. Properties of Pythagorean fuzzy sets in UP-algebras

In this section, we shall find some properties and examples for study the generalizations of Pythagorean fuzzy sets in UP-algebras.

Proposition 3.1. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy UP-subalgebra of $U$ , then it fulfills the assertions (19) and (20).

Proof. Let $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ be a Pythagorean fuzzy UP-subalgebra of $U$ . Then for all $x\in U$ ,

$\begin{align*} \mu_{\mathrm{P}}(0) = \mu_{\mathrm{P}}(x\ast x)\geq\min\{\mu_{\mathrm{P}}(x),\mu_{\mathrm{P}}(x)\} = \mu_{\mathrm{P}}(x)\;\;\;\;\;\;\;\;\text{by (1) and (15)} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(0)& = \nu_{\mathrm{P}}(x\ast x)\leq\max\{\nu_{\mathrm{P}}(x),\nu_{\mathrm{P}}(x)\} = \nu_{\mathrm{P}}(x).\;\;\;\;\;\;\;\;\text{by (1) and (16)} \end{align*}$

Hence, $\mathrm{P}$ fulfills the assertions (19) and (20).

Proposition 3.2. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy UP-filter of $U$ , then

$\begin{gather} (\mathit{\text{for all}}\; x,y \in U)(x\leq y\Rightarrow \mu_{\mathrm{P}}(x)\leq \mu_{\mathrm{P}}(y)), \end{gather}$

(27)

$\begin{gather} (\mathit{\text{for all}}\; x,y \in U)(x\leq y\Rightarrow \nu_{\mathrm{P}}(x)\geq \nu_{\mathrm{P}}(y)). \end{gather}$

(28)

Proof. Let $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ be a Pythagorean fuzzy UP-filter of $U$ and let $x, y\in U$ be such that $x\leq y$ . Then $x\ast y = 0$ , so

$\begin{align*} \mu_{\mathrm{P}}(y)\geq\min\{\mu_{\mathrm{P}}(x\ast y),\mu_{\mathrm{P}}(x)\} = \min\{\mu_{\mathrm{P}}(0),\mu_{\mathrm{P}}(x)\} = \mu_{\mathrm{P}}(x)\;\;\;\;\;\;\;\;\text{by (21)} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(y)\leq\max\{\nu_{\mathrm{P}}(x\ast y),\nu_{\mathrm{P}}(x)\} = \max\{\nu_{\mathrm{P}}(0),\nu_{\mathrm{P}}(x)\} = \nu_{\mathrm{P}}(x).\;\;\;\;\;\;\;\;\text{by (22)} \end{align*}$

Hence, $\mathrm{P}$ fulfills the assertions (27) and (28).

Corollary 3.3. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy UP-filter of $U$ , then

$\begin{gather*} \label{PPFS3_1} (\mathit{\text{for all}}\; x,y \in U)(\mu_{\mathrm{P}}(y)\leq \mu_{\mathrm{P}}(x\ast y)),\\ \label{PPFS3_2} (\mathit{\text{for all}}\; x,y \in U)(\nu_{\mathrm{P}}(y)\geq \nu_{\mathrm{P}}(x\ast y)). \end{gather*}$

Proof. By (4), we have $y\ast(x\ast y) = 0$ , that is, $y\leq x\ast y$ . By (27) and (28), we have $\mu_{\mathrm{P}}(y)\leq \mu_{\mathrm{P}}(x\ast y)$ and $\nu_{\mathrm{P}}(y)\geq \nu_{\mathrm{P}}(x\ast y)$ . Hence, $\mathrm{P}$ fulfills the assertions (3.3) and (3.3).

Proposition 3.4. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the following assertions:

$\begin{gather} (\mathit{\text{for all}}\; x,y,z\in U)(z\leq x\Rightarrow \mu_{\mathrm{P}}(x\ast y)\geq\min\{\mu_{\mathrm{P}}(z),\mu_{\mathrm{P}}(y)\}), \end{gather}$

(29)

$\begin{gather} (\mathit{\text{for all}}\; x,y,z\in U)(z\leq x\Rightarrow \nu_{\mathrm{P}}(x\ast y)\leq\max\{\nu_{\mathrm{P}}(z),\nu_{\mathrm{P}}(y)\}), \end{gather}$

(30)

then it is a Pythagorean fuzzy UP-subalgebra of $U$ .

Proof. Let $x, y\in U$ . By (1), we have $x\leq x$ . It follows from (29) and (30) that $\mu_{\mathrm{P}}(x\ast y)\geq\min\{\mu_{\mathrm{P}}(x), \mu_{\mathrm{P}}(y)\}$ and $\nu_{\mathrm{P}}(x\ast y)\leq\max\{\nu_{\mathrm{P}}(x), \nu_{\mathrm{P}}(y)\}$ . Hence, $\mathrm{P}$ is a Pythagorean fuzzy UP-subalgebra of $U$ .

Theorem 3.5. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the assertions (29) and (30), then it fulfills the assertions (19) and (20).

Proof. It is straightforward by Proposition 3.4.

In general, the converse of Theorem 3.5 may be not true by the following example.

Example 3.6. From Example 2.9, we define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 1 & 0.5 & 0.1 & 0.7 \\ \nu_{\mathrm{P}} & 0 & 0.5 & 0.6 & 0.4 \end{array} \end{equation*}$

Then $\mathrm{P}$ fulfills the assertions (19) and (20) but it does not satisfy the assertions (29) and (30). Indeed, $1\leq1$ but $\mu_{\mathrm{P}}(1\ast3) = \mu_{\mathrm{P}}(2) = 0.1\not\geq0.5 = \min\{0.5, 0.7\} = \min\{\mu_{\mathrm{P}}(1), \mu_{\mathrm{P}}(3)\}$ and $\nu_{\mathrm{P}}(1\ast3) = \nu_{\mathrm{P}}(2) = 0.6\not\leq0.5 = \max\{0.5, 0.4\} = \max\{\nu_{\mathrm{P}}(1), \nu_{\mathrm{P}}(3)\}$ .

Proposition 3.7. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the following assertions:

$\begin{gather} (\mathit{\text{for all}}\; x,y,z\in U)(\mu_{\mathrm{P}}(x\ast y)\geq\min\{\mu_{\mathrm{P}}(z),\mu_{\mathrm{P}}(y)\}), \end{gather}$

(31)

$\begin{gather} (\mathit{\text{for all}}\; x,y,z\in U)(\nu_{\mathrm{P}}(x\ast y)\leq\max\{\nu_{\mathrm{P}}(z),\nu_{\mathrm{P}}(y)\}), \end{gather}$

(32)

then it fulfills the assertions (29) and (30).

In general, the converse of Proposition 3.7 may be not true by the following example.

Example 3.8. Let $U = \{0, 1, 2, 3\}$ be a UP-algebra with a fixed element $0$ and a binary operation $\ast$ defined by the following Cayley table:

$\begin{equation*} \begin{array}{c|cccc} \ast & 0 & 1 & 2 & 3 \\ \hline 0 & 0 & 1 & 2 & 3 \\ 1 & 0 & 0 & 3 & 3 \\ 2 & 0 & 1 & 0 & 0 \\ 3 & 0 & 1 & 2 & 0 \end{array} \end{equation*}$

We define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 0.8 & 0.1 & 0.3 & 0.2 \\ \nu_{\mathrm{P}} & 0.4 & 0.9 & 0.6 & 0.8 \end{array} \end{equation*}$

Then $\mathrm{P}$ fulfills the assertions (29) and (30) but it does not satisfy the assertions (31) and (32). Indeed, $\mu_{\mathrm{P}}(1\ast2) = \mu_{\mathrm{P}}(3) = 0.2\not\geq0.3 = \min\{0.8, 0.3\} = \min\{\mu_{\mathrm{P}}(0), \mu_{\mathrm{P}}(2)\}$ and $\nu_{\mathrm{P}}(1\ast2) = \nu_{\mathrm{P}}(3) = 0.8\not\leq0.6 = \max\{0.4, 0.6\} = \max\{\nu_{\mathrm{P}}(0), \nu_{\mathrm{P}}(2)\}$ .

Proposition 3.9. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the assertions (27) and (28), then it is a Pythagorean fuzzy near UP-filter of $U$ .

Proof. Let $x, y\in U$ . By (4), we have $y\leq x\ast y$ . It follows from (27) and (28) that $\mu_{\mathrm{P}}(x\ast y)\geq\mu_{\mathrm{P}}(y)$ and $\nu_{\mathrm{P}}(x\ast y)\leq \nu_{\mathrm{P}}(y)$ . Hence, $\mathrm{P}$ is a Pythagorean fuzzy near UP-filter of $U$ .

Theorem 3.10. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the assertions (27) and (28), then it fulfills the assertions (31) and (32).

Proof. Let $x, y, z\in U$ . By (4), we have $y\leq x\ast y$ . It follows from (27) and (28) that $\mu_{\mathrm{P}}(x\ast y)\geq\mu_{\mathrm{P}}(y)\geq\min\{\mu_{\mathrm{P}}(z), \mu_{\mathrm{P}}(y)\}$ and $\nu_{\mathrm{P}}(x\ast y)\leq \nu_{\mathrm{P}}(y)\leq\max\{\nu_{\mathrm{P}}(z), \nu_{\mathrm{P}}(y)\}$ . Hence, $\mathrm{P}$ fulfills the assertions (31) and (32).

In general, the converse of Theorem 3.10 may be not true by the following example.

Example 3.11. From Example 2.7, we define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 0.8 & 0.3 & 0.4 & 0.7 \\ \nu_{\mathrm{P}} & 0.2 & 0.7 & 0.5 & 0.4 \end{array} \end{equation*}$

Then $\mathrm{P}$ fulfills the assertions (31) and (32) but it does not satisfy the assertions (27) and (28). Indeed, $3\leq1$ but $\mu_{\mathrm{P}}(3) = 0.7\not\leq0.3 = \mu_{\mathrm{P}}(1)$ and $\nu_{\mathrm{P}}(3) = 0.4\not\geq0.7 = \nu_{\mathrm{P}}(1)$ .

Theorem 3.12. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy UP-subalgebra of $U$ fulfilling the following assertions:

$\begin{gather} (\mathit{\text{for all}}\; x,y\in U)(x\ast y\neq0\Rightarrow \mu_{\mathrm{P}}(x)\geq\mu_{\mathrm{P}}(y)), \end{gather}$

(33)

$\begin{gather} (\mathit{\text{for all}}\; x,y\in U)(x\ast y\neq0\Rightarrow \nu_{\mathrm{P}}(x)\leq \nu_{\mathrm{P}}(y)), \end{gather}$

(34)

then it is a Pythagorean fuzzy near UP-filter of $U$ .

Proof. Let $x, y\in U$ .

Case 1: $x\ast y = 0$ . By Proposition 3.1, we have $\mu_{\mathrm{P}}(x\ast y) = \mu_{\mathrm{P}}(0)\geq\mu_{\mathrm{P}}(y)$ and $\nu_{\mathrm{P}}(x\ast y) = \nu_{\mathrm{P}}(0)\leq \nu_{\mathrm{P}}(y)$ .

Case 2: $x\ast y\neq0$ . By (33) and (34), we have $\mu_{\mathrm{P}}(x\ast y)\geq\min\{\mu_{\mathrm{P}}(x), \mu_{\mathrm{P}}(y)\} = \mu_{\mathrm{P}}(y)$ and $\nu_{\mathrm{P}}(x\ast y)\leq\max\{\nu_{\mathrm{P}}(x), \nu_{\mathrm{P}}(y)\} = \nu_{\mathrm{P}}(y)$ . Hence, $\mathrm{P}$ is a Pythagorean fuzzy near UP-filter of $U$ .

Proposition 3.13. A Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ in $U$ fulfills the following assertions:

$\begin{gather} (\mathit{\text{for all}}\; x,y,z\in U)(z\leq x\ast y\Rightarrow \mu_{\mathrm{P}}(y)\geq\min\{\mu_{\mathrm{P}}(z),\mu_{\mathrm{P}}(x)\}), \end{gather}$

(35)

$\begin{gather} (\mathit{\text{for all}}\; x,y,z\in U)(z\leq x\ast y\Rightarrow \nu_{\mathrm{P}}(y)\leq\max\{\nu_{\mathrm{P}}(z),\nu_{\mathrm{P}}(x)\}) \end{gather}$

(36)

if and only if it is a Pythagorean fuzzy UP-filter of $U$ .

Proof. Let $x\in U$ . By (UP-3), we have $x\leq x\ast 0$ . It follows from (35) and (36) that $\mu_{\mathrm{P}}(0)\geq\min\{\mu_{\mathrm{P}}(x), \mu_{\mathrm{P}}(x)\} = \mu_{\mathrm{P}}(x)$ and $\nu_{\mathrm{P}}(0)\leq\max\{\nu_{\mathrm{P}}(x), \nu_{\mathrm{P}}(x)\} = \nu_{\mathrm{P}}(x)$ . Next, let $x, y\in U$ . By (1), we have $x\ast y\leq x\ast y$ . It follows from (35) and (36) that $\mu_{\mathrm{P}}(y)\geq\min\{\mu_{\mathrm{P}}(x\ast y), \mu_{\mathrm{P}}(x)\}$ and $\nu_{\mathrm{P}}(y)\leq\max\{\nu_{\mathrm{P}}(x\ast y), \nu_{\mathrm{P}}(x)\}$ . Hence, $\mathrm{P}$ is a Pythagorean fuzzy UP-filter of $U$ .

Conversely, let $x, y, z \in U$ be such that $z\leq x\ast y$ . Then $z\ast(x\ast y) = 0$ , so

$\begin{align*} \mu_{\mathrm{P}}(x\ast y)\geq\min\{\mu_{\mathrm{P}}(z\ast(x\ast y)),\mu_{\mathrm{P}}(z)\} = \min\{\mu_{\mathrm{P}}(0),\mu_{\mathrm{P}}(z)\} = \mu_{\mathrm{P}}(z)\;\;\;\;\;\;\;\;\text{by (21)} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(x\ast y)\leq\max\{\nu_{\mathrm{P}}(z\ast(x\ast y)),\nu_{\mathrm{P}}(z)\} = \max\{\nu_{\mathrm{P}}(0),\nu_{\mathrm{P}}(z)\} = \nu_{\mathrm{P}}(z).\;\;\;\;\;\;\;\;\text{by (22)} \end{align*}$

Thus

$\mu_{\mathrm{P}}(y)\geq\min\{\mu_{\mathrm{P}}(x\ast y),\mu_{\mathrm{P}}(x)\}\geq \min\{\mu_{\mathrm{P}}(z),\mu_{\mathrm{P}}(x)\}$

and

$\nu_{\mathrm{P}}(y)\leq\max\{\nu_{\mathrm{P}}(x\ast y),\nu_{\mathrm{P}}(x)\}\leq\max\{\nu_{\mathrm{P}}(z),\nu_{\mathrm{P}}(x)\}$ .

Hence, $\mathrm{P}$ fulfills the assertions (35) and (36).

Theorem 3.14. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the assertions (35) and (36), then it fulfills the assertions (27) and (28).

Proof. Let $x, y\in U$ be such that $x\leq y$ . By (5), we have $x\leq x\ast y$ . It follows from (35) and (36) that $\mu_{\mathrm{P}}(y)\geq\min\{\mu_{\mathrm{P}}(x), \mu_{\mathrm{P}}(x)\} = \mu_{\mathrm{P}}(x)$ and $\nu_{\mathrm{P}}(y)\leq\max\{\nu_{\mathrm{P}}(x), \nu_{\mathrm{P}}(x)\} = \nu_{\mathrm{P}}(x)$ . Hence, $\mathrm{P}$ fulfills the assertions (27) and (28).

In general, the converse of Theorem 3.14 may be not true by the following example.

Example 3.15. Let $U = \{0, 1, 2, 3\}$ be a UP-algebra with a fixed element $0$ and a binary operation $\ast$ defined by the following Cayley table:

$\begin{equation*} \begin{array}{c|cccc} \ast & 0 & 1 & 2 & 3 \\ \hline 0 & 0 & 1 & 2 & 3 \\ 1 & 0 & 0 & 2 & 2 \\ 2 & 0 & 1 & 0 & 1 \\ 3 & 0 & 0 & 0 & 0 \end{array} \end{equation*}$

We define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 0.7 & 0.3 & 0.5 & 0.1 \\ \nu_{\mathrm{P}} & 0.3 & 0.7 & 0.5 & 0.8 \end{array} \end{equation*}$

Then $\mathrm{P}$ fulfills the assertions (27) and (28) but it does not satisfy the assertions (35) and (36). Indeed, $2\leq1\ast3$ but $\mu_{\mathrm{P}}(3) = 0.1\not\geq0.3 = \min\{0.5, 0.3\} = \min\{\mu_{\mathrm{P}}(2), \mu_{\mathrm{P}}(1)\}$ and $\nu_{\mathrm{P}}(3) = 0.8\not\leq0.7 = \max\{0.5, 0.7\} = \max\{\nu_{\mathrm{P}}(2), \nu_{\mathrm{P}}(1)\}$ .

Theorem 3.16. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy near UP-filter of $U$ fulfilling the following assertions:

$\begin{gather} (\mathit{\text{for all}}\; x,y\in U)(\mu_{\mathrm{P}}(x\ast y) = \mu_{\mathrm{P}}(y)), \end{gather}$

(37)

$\begin{gather} (\mathit{\text{for all}}\; x,y\in U)(\nu_{\mathrm{P}}(x\ast y) = \nu_{\mathrm{P}}(y)), \end{gather}$

(38)

then it is a Pythagorean fuzzy UP-filter of $U$ .

Proof. Let $x, y\in U$ . By Theorem 2.4 and Proposition 3.1, we have $\mathrm{P}$ is a Pythagorean fuzzy UP-subalgebra of $U$ which fulfills the assertions (19) and (20). By (37) and (38), we have $\mu_{\mathrm{P}}(y)\geq\min\{\mu_{\mathrm{P}}(y), \mu_{\mathrm{P}}(x)\} = \min\{\mu_{\mathrm{P}}(x\ast y), \mu_{\mathrm{P}}(x)\}$ and $\nu_{\mathrm{P}}(y)\leq\max\{\nu_{\mathrm{P}}(y), \nu_{\mathrm{P}}(x)\} = \max\{\nu_{\mathrm{P}}(x\ast y), \nu_{\mathrm{P}}(x)\}$ . Hence, $\mathrm{P}$ is a Pythagorean fuzzy UP-filter of $U$ .

Proposition 3.17. A Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ in $U$ fulfills the following assertions:

$\begin{gather} (\mathit{\text{for all}} a,x,y,z\in U)(a\leq x\ast(y\ast z)\Rightarrow \mu_{\mathrm{P}}(x\ast z)\geq\min\{\mu_{\mathrm{P}}(a),\mu_{\mathrm{P}}(y)\}), \end{gather}$

(39)

$\begin{gather} (\mathit{\text{for all}} a,x,y,z\in U)(a\leq x\ast(y\ast z)\Rightarrow \nu_{\mathrm{P}}(x\ast z)\leq\max\{\nu_{\mathrm{P}}(a),\nu_{\mathrm{P}}(y)\}) \end{gather}$

(40)

if and only if it is a Pythagorean fuzzy UP-ideal of $U$ .

Proof. Let $x\in U$ . By (UP-3), we have $x\leq x\ast(x\ast 0)$ . Then

$\begin{align*} \mu_{\mathrm{P}}(0) = \mu_{\mathrm{P}}(x\ast 0)\geq\min\{\mu_{\mathrm{P}}(x),\mu_{\mathrm{P}}(x)\} = \mu_{\mathrm{P}}(x)\;\;\;\;\;\;\;\;\text{by (UP-3) and (39)} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(0) = \nu_{\mathrm{P}}(x\ast 0)\leq\max\{\nu_{\mathrm{P}}(x),\nu_{\mathrm{P}}(x)\} = \nu_{\mathrm{P}}(x).\;\;\;\;\;\;\;\;\text{by (UP-3) and (40)} \end{align*}$

Let $x, y, z\in U$ . By (1), we have $x\ast(y\ast z)\leq x\ast(y\ast z)$ . Then

$\begin{align*} \mu_{\mathrm{P}}(x\ast z)\geq\min\{\mu_{\mathrm{P}}(x\ast(y\ast z)),\mu_{\mathrm{P}}(y)\}\;\;\;\;\;\;\;\;\text{by (39)} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(x\ast z)\leq\max\{\nu_{\mathrm{P}}(x\ast(y\ast z)),\nu_{\mathrm{P}}(y)\}.\;\;\;\;\;\;\;\;\text{by (40)} \end{align*}$

Hence, $\mathrm{P}$ is a Pythagorean fuzzy UP-ideal of $U$ .

Conversely, let $a, x, y, z\in U$ be such that $a\leq x\ast(y\ast z)$ . By (27) and (28), we have $\mu_{\mathrm{P}}(a)\leq\mu_{\mathrm{P}}(x\ast(y\ast z))$ and $\nu_{\mathrm{P}}(a)\geq \nu_{\mathrm{P}}(x\ast(y\ast z))$ . Thus

$\begin{align*} \mu_{\mathrm{P}}(x\ast z)\geq\min\{\mu_{\mathrm{P}}(x\ast(y\ast z)),\mu_{\mathrm{P}}(y)\}\geq\min\{\mu_{\mathrm{P}}(a),\mu_{\mathrm{P}}(y)\}\;\;\;\;\;\;\;\;\text{by (23)} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(x\ast z)\leq\max\{\nu_{\mathrm{P}}(x\ast(y\ast z)),\nu_{\mathrm{P}}(y)\}\leq\max\{\nu_{\mathrm{P}}(a),\nu_{\mathrm{P}}(y)\}.\;\;\;\;\;\;\;\;\text{by (24)} \end{align*}$

Hence, $\mathrm{P}$ fulfills the assertions (39) and (40).

Proposition 3.18. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy UP-ideal of $U$ , then

$\begin{gather} (\mathit{\text{for all}} a,x,y,z\in U)(a\leq x\ast(y\ast z)\Rightarrow \mu_{\mathrm{P}}(a\ast z)\geq\min\{\mu_{\mathrm{P}}(x),\mu_{\mathrm{P}}(y)\}), \end{gather}$

(41)

$\begin{gather} (\mathit{\text{for all}} a,x,y,z\in U)(a\leq x\ast(y\ast z)\Rightarrow \nu_{\mathrm{P}}(a\ast z)\leq\max\{\nu_{\mathrm{P}}(x),\nu_{\mathrm{P}}(y)\}). \end{gather}$

(42)

Proof. Let $a, x, y, z\in U$ such that $a\leq x\ast(y\ast z)$ . Then $a\ast(x\ast(y\ast z)) = 0$ , so

$\begin{align*} \mu_{\mathrm{P}}(a\ast(y\ast z))\geq\min\{\mu_{\mathrm{P}}(a\ast(x\ast(y\ast z))),\mu_{\mathrm{P}}(x)\} = \min\{\mu_{\mathrm{P}}(0),\mu_{\mathrm{P}}(x)\} = \mu_{\mathrm{P}}(x)\;\;\;\;\;\;\;\;\text{by (23)} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(a\ast(y\ast z))\leq\max\{\nu_{\mathrm{P}}(a\ast(x\ast(y\ast z))),\nu_{\mathrm{P}}(x)\} = \max\{\nu_{\mathrm{P}}(0),\nu_{\mathrm{P}}(x)\} = \nu_{\mathrm{P}}(x).\;\;\;\;\;\;\;\;\text{by (24)} \end{align*}$

Thus

$\begin{align*} \mu_{\mathrm{P}}(a\ast z)\geq \min\{\mu_{\mathrm{P}}(a\ast(y\ast z)),\mu_{\mathrm{P}}(y)\}\geq \min\{\mu_{\mathrm{P}}(x),\mu_{\mathrm{P}}(y)\}\;\;\;\;\;\;\;\;\text{by (23)} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(a\ast z)\leq\max\{\nu_{\mathrm{P}}(a\ast(y\ast z)),\nu_{\mathrm{P}}(y)\}\leq\max\{\nu_{\mathrm{P}}(x),\nu_{\mathrm{P}}(y)\}.\;\;\;\;\;\;\;\;\text{by (24)} \end{align*}$

Hence, $\mathrm{P}$ fulfills the assertions (41) and (42).

Corollary 3.19. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the assertions (39) and (40), then it fulfills the assertions (41) and (42).

Proof. It is straightforward by Propositions 3.17 and 3.18.

Theorem 3.20. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the assertions (6), (41), and (42), then it fulfills the assertions (39) and (40).

Proof. Let $a, x, y, z\in U$ be such that $a\leq x\ast(y\ast z)$ . By (6), we have $0 = a\ast(x\ast(y\ast z)) = x\ast(a\ast(y\ast z))$ , that is, $x\leq a\ast(y\ast z)$ . It follows from (41) and (42) that $\mu_{\mathrm{P}}(x\ast z)\geq\min\{\mu_{\mathrm{P}}(a), \mu_{\mathrm{P}}(y)\}$ and $\nu_{\mathrm{P}}(x\ast z)\leq\max\{\nu_{\mathrm{P}}(a), \nu_{\mathrm{P}}(y)\}$ . Hence, $\mathrm{P}$ fulfills the assertions (39) and (40).

Theorem 3.21. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the assertions (41) and (42), then it fulfills the assertions (35) and (36).

Proof. Let $x, y, z\in U$ be such that $z\leq x\ast y$ . By (1) and (2), we have $0 = z\ast z\leq z\ast(x\ast y)$ . By (UP-2), (41), and (42), we have $\mu_{\mathrm{P}}(y) = \mu_{\mathrm{P}}(0\ast y)\geq\min\{\mu_{\mathrm{P}}(z), \mu_{\mathrm{P}}(x)\}$ and $\nu_{\mathrm{P}}(y) = \nu_{\mathrm{P}}(0\ast y)\leq\max\{\nu_{\mathrm{P}}(z), \nu_{\mathrm{P}}(x)\}$ . Hence, $\mathrm{P}$ fulfills the assertions (35) and (36).

Corollary 3.22. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the assertions (39) and (40), then it fulfills the assertions (35) and (36).

Proof. It is straightforward by Corollary 3.19 and Theorem 3.21.

In general, the converse of Theorem 3.21 may be not true by the following example.

Example 3.23. From Example 3.8, we define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 0.7 & 0.3 & 0.2 & 0.2 \\ \nu_{\mathrm{P}} & 0.3 & 0.7 & 0.75 & 0.75 \end{array} \end{equation*}$

Then $\mathrm{P}$ fulfills the assertions (35) and (36) but it does not satisfy the assertions (41) and (42). Indeed, $3\leq1\ast(0\ast2)$ but $\mu_{\mathrm{P}}(3\ast2) = \mu_{\mathrm{P}}(2) = 0.2\not\geq0.3 = \min\{0.3, 0.7\} = \min\{\mu_{\mathrm{P}}(1), \mu_{\mathrm{P}}(0)\}$ and $\nu_{\mathrm{P}}(3\ast2) = \nu_{\mathrm{P}}(2) = 0.75\not\leq0.7 = \max\{0.7, 0.3\} = \max\{\nu_{\mathrm{P}}(1), \nu_{\mathrm{P}}(0)\}$ .

The following example shows that Pythagorean fuzzy set in a UP-algebra which fulfills the assertions (39) and (40) is not constant.

Example 3.24. From Example 2.11, we define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 1 & 0.8 & 0.5 & 0.5 \\ \nu_{\mathrm{P}} & 0 & 0.3 & 0.6 & 0.6 \end{array} \end{equation*}$

Then $\mathrm{P}$ fulfills the assertions (39) and (40) but it is not constant.

Theorem 3.25. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy UP-filter of $U$ fulfilling the assertion (6), then it is a Pythagorean fuzzy UP-ideal of $U$ .

Proof. Let $\mathrm{P}$ be a Pythagorean fuzzy UP-filter of $U$ . Then for all $x, y, z\in U$ ,

$\begin{align*} \mu_{\mathrm{P}}(x\ast z)\geq\min\{\mu_{\mathrm{P}}(y\ast(x\ast z)),\mu_{\mathrm{P}}(y)\} = \min\{\mu_{\mathrm{P}}(x\ast(y\ast z)),\mu_{\mathrm{P}}(y)\}\;\;\;\;\;\;\;\;\text{by (21) and (6)} \end{align*}$

and

$\begin{align*} \nu_{\mathrm{P}}(x\ast z)\leq\max\{\nu_{\mathrm{P}}(y\ast(x\ast z)),\nu_{\mathrm{P}}(y)\} = \max\{\nu_{\mathrm{P}}(x\ast(y\ast z)),\nu_{\mathrm{P}}(y)\}.\;\;\;\;\;\;\;\;\text{by (22) and (6)} \end{align*}$

Hence, $\mathrm{P}$ is a Pythagorean fuzzy UP-ideal of $U$ .

Proposition 3.26. A Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ in $U$ fulfills the following assertions:

$\begin{gather} (\mathit{\text{for all}} a,x,y,z\in U)(a\leq(z\ast y)\ast(z\ast x)\Rightarrow \mu_{\mathrm{P}}(x)\geq\min\{\mu_{\mathrm{P}}(a),\mu_{\mathrm{P}}(y)\}), \end{gather}$

(43)

$\begin{gather} (\mathit{\text{for all}} a,x,y,z\in U)(a\leq(z\ast y)\ast(z\ast x)\Rightarrow \nu_{\mathrm{P}}(x)\leq\max\{\nu_{\mathrm{P}}(a),\nu_{\mathrm{P}}(y)\}) \end{gather}$

(44)

if and only if it is a Pythagorean fuzzy strong UP-ideal of $U$ .

Proof. Let $x\in U$ . By (UP-3), we have $x\leq0 = x\ast0 = (0\ast x)\ast(0\ast 0)$ . By (43) and (44), we have $\mu_{\mathrm{P}}(0)\geq \min\{\mu_{\mathrm{P}}(x), \mu_{\mathrm{P}}(x)\} = \mu_{\mathrm{P}}(x)$ and $\nu_{\mathrm{P}}(0)\leq \max\{\nu_{\mathrm{P}}(x), \nu_{\mathrm{P}}(x)\} = \nu_{\mathrm{P}}(x)$ . Next, let $x, y, z\in U$ . By (1), we have $(z\ast y)\ast(z\ast x)\leq(z\ast y)\ast(z\ast x)$ . By (43) and (44), we have $\mu_{\mathrm{P}}(x)\geq\min\{\mu_{\mathrm{P}}((z\ast y)\ast(z\ast x)), \mu_{\mathrm{P}}(y)\}$ and $\nu_{\mathrm{P}}(x)\leq\max\{\nu_{\mathrm{P}}((z\ast y)\ast(z\ast x)), \nu_{\mathrm{P}}(y)\}$ . Hence, $\mathrm{P}$ is a Pythagorean fuzzy strong UP-ideal of $U$ .

The converse is evident because $\mathrm{P}$ is constant by Theorem 2.3.

Theorem 3.27. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the following assertions:

$\begin{gather} (\mathit{\text{for all}}\; x,y,z\in U)(z\leq x\ast y\Rightarrow \mu_{\mathrm{P}}(z)\geq\min\{\mu_{\mathrm{P}}(x),\mu_{\mathrm{P}}(y)\}), \end{gather}$

(45)

$\begin{gather} (\mathit{\text{for all}}\; x,y,z\in U)(z\leq x\ast y\Rightarrow \nu_{\mathrm{P}}(z)\leq\max\{\nu_{\mathrm{P}}(x),\nu_{\mathrm{P}}(y)\}), \end{gather}$

(46)

then it fulfills the assertions (29) and (30).

Proof. Let $x, y, z\in U$ be such that $z\leq x$ . By (3), we have $x\ast y\leq z\ast y$ . By (45) and (46), we have $\mu_{\mathrm{P}}(x\ast y)\geq\min\{\mu_{\mathrm{P}}(z), \mu_{\mathrm{P}}(y)\}$ and $\nu_{\mathrm{P}}(x\ast y)\leq\max\{\nu_{\mathrm{P}}(z), \nu_{\mathrm{P}}(y)\}$ . Hence, $\mathrm{P}$ fulfills the assertions (29) and (30).

Proposition 3.28. A Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ in $U$ fulfills the assertions (45) and (46) if and only if it is a Pythagorean fuzzy strong UP-ideal of $U$ .

Proof. Let $x\in U$ . By (UP-3), we have $x\leq0 = 0\ast0$ . By (45) and (46), we have $\mu_{\mathrm{P}}(x)\geq\min\{\mu_{\mathrm{P}}(0), \mu_{\mathrm{P}}(0)\} = \mu_{\mathrm{P}}(0)$ and $\nu_{\mathrm{P}}(x)\leq\max\{\nu_{\mathrm{P}}(0), \nu_{\mathrm{P}}(0)\} = \nu_{\mathrm{P}}(0)$ . By Theorem 3.27, we have $\mathrm{P}$ fulfills (29) and (30). Thus $\mathrm{P}$ is a Pythagorean fuzzy UP-subalgebra of $U$ by Proposition 3.4. It follows from Proposition 3.1 that $\mu_{\mathrm{P}}(0)\geq\mu_{\mathrm{P}}(x)$ and $\nu_{\mathrm{P}}(0)\leq \nu_{\mathrm{P}}(x)$ , so $\mu_{\mathrm{P}}(x) = \mu_{\mathrm{P}}(0)$ and $\nu_{\mathrm{P}}(x) = \nu_{\mathrm{P}}(0)$ for all $x\in U$ , that is, $\mathrm{P}$ is constant. By Theorem 2.3, we have $\mathrm{P}$ is a Pythagorean fuzzy strong UP-ideal of $U$ .

The converse is evident because $\mathrm{P}$ is constant by Theorem 2.3.

Theorem 3.29. If $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy set in $U$ fulfilling the following assertions:

$\begin{gather} (\mathit{\text{for all}}\; x,y,z\in U)(z\leq x\ast y\Rightarrow \mu_{\mathrm{P}}(z)\geq\mu_{\mathrm{P}}(y)), \end{gather}$

(47)

$\begin{gather} (\mathit{\text{for all}}\; x,y,z\in U)(z\leq x\ast y\Rightarrow \nu_{\mathrm{P}}(z)\leq \nu_{\mathrm{P}}(y)), \end{gather}$

(48)

then it fulfills the assertions (29) and (30).

Proof. Let $x, y, z\in U$ be such that $z\leq x$ . By (3), we have $x\ast y\leq z\ast y$ . It follows from (47) and (48) that $\mu_{\mathrm{P}}(x\ast y)\geq\mu_{\mathrm{P}}(y)\geq\min\{\mu_{\mathrm{P}}(z), \mu_{\mathrm{P}}(y)\}$ and $\nu_{\mathrm{P}}(x\ast y)\leq \nu_{\mathrm{P}}(y)\leq\max\{\nu_{\mathrm{P}}(z), \nu_{\mathrm{P}}(y)\}$ . Hence, $\mathrm{P}$ fulfills the assertions (29) and (30).

Proposition 3.30. A Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ in $U$ fulfills the assertions (47) and (48) if and only if it is a Pythagorean fuzzy strong UP-ideal of $U$ .

Proof. Let $x\in U$ . By (UP-3), we have $x\leq0 = 0\ast0$ . By (47) and (48), we have $\mu_{\mathrm{P}}(x)\geq\mu_{\mathrm{P}}(0)$ and $\nu_{\mathrm{P}}(x)\leq \nu_{\mathrm{P}}(0)$ . By Theorem 3.27, we have $\mathrm{P}$ fulfills (29) and (30). Thus $\mathrm{P}$ is a Pythagorean fuzzy UP-subalgebra of $U$ by Proposition 3.4. It follows from Proposition 3.1 that $\mu_{\mathrm{P}}(0)\geq\mu_{\mathrm{P}}(x)$ and $\nu_{\mathrm{P}}(0)\leq \nu_{\mathrm{P}}(x)$ , so $\mu_{\mathrm{P}}(x) = \mu_{\mathrm{P}}(0)$ and $\nu_{\mathrm{P}}(x) = \nu_{\mathrm{P}}(0)$ for all $x\in U$ , that is, $\mathrm{P}$ is constant. By Theorem 2.3, we have $\mathrm{P}$ is a Pythagorean fuzzy strong UP-ideal of $U$ .

The converse is evident because $\mathrm{P}$ is constant by Theorem 2.3.

We get the diagram of the properties of Pythagorean fuzzy sets in UP-algebras, which is shown with Figure 1.

Figure 1. Properties of Pythagorean fuzzy sets in UP-algebras.

DownLoad: Full-Size Img PowerPoint

4. Approximations

Let $U$ be a set and $\rho$ an equivalence relation on $U$ . If $x\in U$ , then the $\rho$ -class of $U$ is the set $(x)_{\rho}$ defined as follows:

$(x)_{\rho} = \{y\in U\mid (x,y)\in\rho\}.$

An equivalence relation $\rho$ on a UP-algebra $U = (U, \ast, 0)$ is said to be a congruence relation if

$(\text{for all } x,y,z\in U)((x,y)\in\rho\Rightarrow (x\ast z,y\ast z)\in\rho,(z\ast x,z\ast y)\in\rho).$

Definition 4.1. For nonempty subsets $A$ and $B$ of a UP-algebra $U = (U, \ast, 0)$ , we denote

$AB = A\ast B = \{a\ast b\mid a\in A\; \text{and}\; b\in B\}.$

If $\rho$ is a congruence on a UP-algebra $U = (U, \ast, 0)$ , then

$\begin{align*} (\text{for all } x,y\in U)((x)_{\rho}(y)_{\rho}\subseteq(x\ast y)_{\rho}).\;\;\;\;\;\;\;\;\text{see [20]} \end{align*}$

A congruence relation $\rho$ on a UP-algebra $U = (U, \ast, 0)$ is said to be complete if

$(\text{for all } x,y\in U)((x)_{\rho}(y)_{\rho} = (x\ast y)_{\rho}).$

Example 4.2. Let $U = \{0, 1, 2, 3\}$ be a UP-algebra with a fixed element $0$ and a binary operation $\ast$ defined by the following Cayley table:

$\begin{equation*} \begin{array}{c|cccc} \ast & 0 & 1 & 2 & 3 \\ \hline 0 & 0 & 1 & 2 & 3 \\ 1 & 0 & 0 & 2 & 3 \\ 2 & 0 & 0 & 0 & 1 \\ 3 & 0 & 0 & 0 & 0 \end{array} \end{equation*}$

Let

$\rho = \{(0,0),(1,1),(2,2),(3,3),(0,1),(1,0),(2,3),(3,2)\}.$

Then $\rho$ is a congruence relation on $U$ . Thus

$(0)_{\rho} = (1)_{\rho} = \{0,1\},(2)_{\rho} = (3)_{\rho} = \{2,3\}.$

We consider

$\{0,1\} = \{0,1\}\{0,1\} = (0)_{\rho}(0)_{\rho} = (0\ast0)_{\rho} = (0)_{\rho} = \{0,1\},$

$\{0,1\} = \{0,1\}\{0,1\} = (0)_{\rho}(1)_{\rho} = (0\ast1)_{\rho} = (1)_{\rho} = \{0,1\},$

$\{2.3\} = \{0,1\}\{2,3\} = (0)_{\rho}(2)_{\rho} = (0\ast2)_{\rho} = (2)_{\rho} = \{2,3\},$

$\{2,3\} = \{0,1\}\{2,3\} = (0)_{\rho}(3)_{\rho} = (0\ast3)_{\rho} = (3)_{\rho} = \{2,3\},$

$\{0,1\} = \{0,1\}\{0,1\} = (1)_{\rho}(0)_{\rho} = (1\ast0)_{\rho} = (0)_{\rho} = \{0,1\},$

$\{0,1\} = \{0,1\}\{0,1\} = (1)_{\rho}(1)_{\rho} = (1\ast1)_{\rho} = (0)_{\rho} = \{0,1\},$

$\{2.3\} = \{0,1\}\{2,3\} = (1)_{\rho}(2)_{\rho} = (1\ast2)_{\rho} = (2)_{\rho} = \{2,3\},$

$\{2,3\} = \{0,1\}\{2,3\} = (1)_{\rho}(3)_{\rho} = (1\ast3)_{\rho} = (3)_{\rho} = \{2,3\},$

$\{0,1\} = \{2,3\}\{0,1\} = (2)_{\rho}(0)_{\rho} = (2\ast0)_{\rho} = (0)_{\rho} = \{0,1\},$

$\{0,1\} = \{2,3\}\{0,1\} = (2)_{\rho}(1)_{\rho} = (2\ast1)_{\rho} = (0)_{\rho} = \{0,1\},$

$\{0,1\} = \{2,3\}\{2,3\} = (2)_{\rho}(2)_{\rho} = (2\ast2)_{\rho} = (0)_{\rho} = \{0,1\},$

$\{0,1\} = \{2,3\}\{2,3\} = (2)_{\rho}(3)_{\rho} = (2\ast3)_{\rho} = (1)_{\rho} = \{0,1\},$

$\{0,1\} = \{2,3\}\{0,1\} = (3)_{\rho}(0)_{\rho} = (3\ast0)_{\rho} = (0)_{\rho} = \{0,1\},$

$\{0,1\} = \{2,3\}\{0,1\} = (3)_{\rho}(1)_{\rho} = (3\ast1)_{\rho} = (0)_{\rho} = \{0,1\},$

$\{0,1\} = \{2,3\}\{2,3\} = (3)_{\rho}(2)_{\rho} = (3\ast2)_{\rho} = (0)_{\rho} = \{0,1\},$

$\{0,1\} = \{2,3\}\{2,3\} = (3)_{\rho}(3)_{\rho} = (3\ast3)_{\rho} = (0)_{\rho} = \{0,1\}.$

Hence, $\rho$ is a complete congruence relation on $U$ .

Definition 4.3. Let $\rho$ be an equivalence relation on a nonempty set $U$ and $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ a Pythagorean fuzzy set in $U$ . The upper approximation is defined by

$\rho^{+}(\mathrm{P}) = \{(x,\overline{\mu}_{\mathrm{P}}(x),\overline{\nu}_{\mathrm{P}}(x))\mid x\in U\},$

where $\overline{\mu}_{\mathrm{P}}(x) = \sup_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)\}$ and $\overline{\nu}_{\mathrm{P}}(x) = \inf\limits_{a\in(x)_{\rho}}\{\nu_{\mathrm{P}}(a)\}$ . The lower approximation is defined by

$\rho^{-}(\mathrm{P}) = \{(x,\underline{\mu}_{\mathrm{P}}(x),\underline{\nu}_{\mathrm{P}}(x))\mid x\in U\},$

where $\underline{\mu}_{\mathrm{P}}(x) = \inf\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)\}$ and $\underline{\nu}_{\mathrm{P}}(x) = \sup_{a\in(x)_{\rho}}\{\nu_{\mathrm{P}}(a)\}$ .

Theorem 4.4. Let $\rho$ be an equivalence relation on a nonempty set $U$ and $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ a Pythagorean fuzzy set in $U$ . Then the following statements hold:

(1) $\rho^{+}(\mathrm{P})$ is a Pythagorean fuzzy set in $U$ , and

(2) $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy set in $U$ .

Proof. Let $x\in U$ .

(1) We consider

$\begin{align*} 0&\leq\overline{\mu}_{\mathrm{P}}(x)^{2}+\overline{\nu}_{\mathrm{P}}(x)^{2}\\ & = (\sup\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)\})^{2}+(\inf\limits_{a\in(x)_{\rho}}\{\nu_{\mathrm{P}}(a)\})^{2}\\ & = \sup\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)^{2}\}+\inf\limits_{a\in(x)_{\rho}}\{\nu_{\mathrm{P}}(a)^{2}\}&\text{by Proposition 1.9 (6)}\\ &\leq\sup\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)^{2}\}+\inf\limits_{a\in(x)_{\rho}}\{1-\mu_{\mathrm{P}}(a)^{2}\}\\ & = \sup\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)^{2}\}+1-\sup\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)^{2}\}&\text{by Proposition 1.9 (7)}\\ & = 1. \end{align*}$

This implies that $0\leq\overline{\mu}_{\mathrm{P}}(x)^{2}+\overline{\nu}_{\mathrm{P}}(x)^{2}\leq1$ . Therefore, $\rho^{+}(\mathrm{P})$ is a Pythagorean fuzzy set in $U$ .

(2) The proof is similar to the proof of (1).

Lemma 4.5. If $\rho$ is an equivalence relation on a nonempty set $U$ and $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ a Pythagorean fuzzy set in $U$ , then

$\begin{gather} (\mathit{\text{for all}}\; x,y\in U)(x\rho y\Rightarrow \overline{\mu}_{\mathrm{P}}(x) = \overline{\mu}_{\mathrm{P}}(y)), \end{gather}$

(49)

$\begin{gather} (\mathit{\text{for all}}\; x,y\in U)(x\rho y\Rightarrow \overline{\nu}_{\mathrm{P}}(x) = \overline{\nu}_{\mathrm{P}}(y)), \end{gather}$

(50)

$\begin{gather} (\mathit{\text{for all}}\; x,y\in U)(x\rho y\Rightarrow \underline{\mu}_{\mathrm{P}}(x) = \underline{\mu}_{\mathrm{P}}(y)), \\ (\mathit{\text{for all}}\; x,y\in U)(x\rho y\Rightarrow \underline{\nu}_{\mathrm{P}}(x) = \underline{\nu}_{\mathrm{P}}(y)). \nonumber \end{gather}$

(51)

Proof. Let $x, y\in U$ be such that $x\rho y$ . Then

$\begin{eqnarray*} \overline{\mu}_{\mathrm{P}}(x) = \sup\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)\}& = &\sup\limits_{b\in(y)_{\rho}}\{\mu_{\mathrm{P}}(b)\} = \overline{\mu}_{\mathrm{P}}(y),\\ \overline{\nu}_{\mathrm{P}}(x) = \inf\limits_{a\in(x)_{\rho}}\{\nu_{\mathrm{P}}(a)\}& = &\inf\limits_{b\in(y)_{\rho}}\{\nu_{\mathrm{P}}(b)\} = \overline{\nu}_{\mathrm{P}}(y),\\ \underline{\mu}_{\mathrm{P}}(x) = \inf\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)\}& = &\inf\limits_{b\in(y)_{\rho}}\{\mu_{\mathrm{P}}(b)\} = \underline{\mu}_{\mathrm{P}}(y),\\ \underline{\nu}_{\mathrm{P}}(x) = \sup\limits_{a\in(x)_{\rho}}\{\nu_{\mathrm{P}}(a)\}& = &\sup\limits_{b\in(y)_{\rho}}\{\nu_{\mathrm{P}}(b)\} = \underline{\nu}_{\mathrm{P}}(y). \end{eqnarray*}$

We complete the proof.

Theorem 4.6. Let $\rho$ be an congruence relation on a UP-algebra $U = (U, \ast, 0)$ and $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ a Pythagorean fuzzy set in $U$ . Then the following statements hold:

(1) if $\mathrm{P}$ is a Pythagorean fuzzy UP-subalgebra of $U$ and $\rho$ is complete, then $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy UP-subalgebra of $U$ ,

(2) if $\mathrm{P}$ is a Pythagorean fuzzy near UP-filter of $U$ and $\rho$ is complete, then $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy near UP-filter of $U$ ,

(3) if $\mathrm{P}$ is a Pythagorean fuzzy UP-filter of $U$ and $(0)_{\rho} = \{0\}$ , then $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy UP-filter of $U$ ,

(4) if $\mathrm{P}$ is a Pythagorean fuzzy UP-ideal of $U$ , $(0)_{\rho} = \{0\}$ , and $\rho$ is complete, then $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy UP-ideal of $U$ , and

(5) if $\mathrm{P}$ is a Pythagorean fuzzy strong UP-ideal of $U$ , then $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy strong UP-ideal of $U$ .

Proof. (1) Assume that $\mathrm{P}$ is a Pythagorean fuzzy UP-subalgebra of $U$ and $\rho$ is complete. Then for all $x, y\in U$ ,

$\begin{align*} \underline{\mu}_{\mathrm{P}}(x\ast y) & = \inf\limits_{c\in(x\ast y)_{\rho}}\{\mu_{\mathrm{P}}(c)\}\\ & = \inf\limits_{c\in(x)_{\rho}(y)_{\rho}}\{\mu_{\mathrm{P}}(c)\}\\ & = \inf\limits_{a\ast b\in(x)_{\rho}(y)_{\rho}}\{\mu_{\mathrm{P}}(a\ast b)\}\\ &\geq\inf\limits_{a\in(x)_{\rho},b\in(y)_{\rho}}\{\min\{\mu_{\mathrm{P}}(a),\mu_{\mathrm{P}}(b)\}\}&\text{by (15)}\\ & = \min\{\inf\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)\},\inf\limits_{b\in(y)_{\rho}}\{\mu_{\mathrm{P}}(b)\}\}&\text{by Proposition 1.9 (1)}\\ & = \min\{\underline{\mu}_{\mathrm{P}}(x),\underline{\mu}_{\mathrm{P}}(y)\} \end{align*}$

and

$\begin{align*} \underline{\nu}_{\mathrm{P}}(x\ast y) & = \sup\limits_{c\in(x\ast y)_{\rho}}\{\nu_{\mathrm{P}}(c)\}\\ & = \sup\limits_{c\in(x)_{\rho}(y)_{\rho}}\{\nu_{\mathrm{P}}(c)\}\\ & = \sup\limits_{a\ast b\in(x)_{\rho}(y)_{\rho}}\{\nu_{\mathrm{P}}(a\ast b)\}\\ &\leq\sup\limits_{a\in(x)_{\rho},b\in(y)_{\rho}}\{\max\{\nu_{\mathrm{P}}(a),\nu_{\mathrm{P}}(b)\}\}&\text{by (16)}\\ & = \max\{\sup\limits_{a\in(x)_{\rho}}\{\nu_{\mathrm{P}}(a)\},\sup\limits_{b\in(y)_{\rho}}\{\nu_{\mathrm{P}}(b)\}\}&\text{by Proposition 1.9 (2)}\\ & = \max\{\underline{\nu}_{\mathrm{P}}(x),\underline{\nu}_{\mathrm{P}}(y)\}. \end{align*}$

Hence, $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy UP-subalgebra of $U$ .

(2) Assume that $\mathrm{P}$ is a Pythagorean fuzzy near UP-filter of $U$ and $\rho$ is complete. Then for all $x, y\in U$ ,

$\begin{align*} \underline{\mu}_{\mathrm{P}}(x\ast y) = \inf\limits_{c\in(x\ast y)_{\rho}}\{\mu_{\mathrm{P}}(c)\} = \inf\limits_{c\in(x)_{\rho}(y)_{\rho}}\{\mu_{\mathrm{P}}(c)\} = \inf\limits_{a\ast b\in(x)_{\rho}(y)_{\rho}}\{\mu_{\mathrm{P}}(a\ast b)\} \geq\inf\limits_{b\in(y)_{\rho}}\{\mu_{\mathrm{P}}(b)\}\;\;\;\;\;\;\;\;\text{by (17)} = \underline{\mu}_{\mathrm{P}}(y) \end{align*}$

and

$\begin{align*} \underline{\nu}_{\mathrm{P}}(x\ast y) = \sup\limits_{c\in(x\ast y)_{\rho}}\{\nu_{\mathrm{P}}(c)\} = \sup\limits_{c\in(x)_{\rho}(y)_{\rho}}\{\nu_{\mathrm{P}}(c)\} = \sup\limits_{a\ast b\in(x)_{\rho}(y)_{\rho}}\{\nu_{\mathrm{P}}(a\ast b)\} \leq\sup\limits_{b\in(y)_{\rho}}\{\nu_{\mathrm{P}}(b)\}\;\;\;\;\;\;\;\;\text{by (18)} = \underline{\nu}_{\mathrm{P}}(y). \end{align*}$

Hence, $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy near UP-filter of $U$ .

(3) Assume that $\mathrm{P}$ is a Pythagorean fuzzy UP-filter of $U$ and $(0)_{\rho} = \{0\}$ . Then for all $x, y\in U$ ,

$\underline{\mu}_{\mathrm{P}}(0) = \inf\limits_{a\in(0)_{\rho}}\{\mu_{\mathrm{P}}(a)\} = \mu_{\mathrm{P}}(0)\geq\mu_{\mathrm{P}}(b)\geq\inf\limits_{b\in(x)_{\rho}}\{\mu_{\mathrm{P}}(b)\} = \underline{\mu}_{\mathrm{P}}(x),$

$\underline{\nu}_{\mathrm{P}}(0) = \sup\limits_{a\in(0)_{\rho}}\{\nu_{\mathrm{P}}(a)\} = \nu_{\mathrm{P}}(0)\leq\nu_{\mathrm{P}}(b)\leq\sup\limits_{b\in(x)_{\rho}}\{\nu_{\mathrm{P}}(b)\} = \underline{\nu}_{\mathrm{P}}(x),$

$\begin{align*} \underline{\mu}_{\mathrm{P}}(y) & = \inf\limits_{b\in(y)_{\rho}}\{\mu_{\mathrm{P}}(b)\}\\ &\geq\inf\limits_{a\ast b\in(x)_{\rho}(y)_{\rho},a\in(x)_{\rho}}\{\min\{\mu_{\mathrm{P}}(a\ast b),\mu_{\mathrm{P}}(a)\}\}&\text{by (21)}\\ &\geq\inf\limits_{a\ast b\in(x\ast y)_{\rho},a\in(x)_{\rho}}\{\min\{\mu_{\mathrm{P}}(a\ast b),\mu_{\mathrm{P}}(a)\}\}\\ & = \min\{\inf\limits_{a\ast b\in(x\ast y)_{\rho}}\{\mu_{\mathrm{P}}(a\ast b)\},\inf\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)\}\}&\text{by Proposition 1.9 (1)}\\ & = \min\{\underline{\mu}_{\mathrm{P}}(x\ast y),\underline{\mu}_{\mathrm{P}}(x)\}, \end{align*}$

and

$\begin{align*} \underline{\nu}_{\mathrm{P}}(y) & = \sup\limits_{b\in(y)_{\rho}}\{\nu_{\mathrm{P}}(b)\}\\ &\leq\sup\limits_{a\ast b\in(x)_{\rho}(y)_{\rho},a\in(x)_{\rho}}\{\max\{\nu_{\mathrm{P}}(a\ast b),\nu_{\mathrm{P}}(a)\}\}&\text{by (22)}\\ &\leq\sup\limits_{a\ast b\in(x\ast y)_{\rho},a\in(x)_{\rho}}\{\max\{\nu_{\mathrm{P}}(a\ast b),\nu_{\mathrm{P}}(a)\}\}\\ & = \max\{\sup\limits_{a\ast b\in(x\ast y)_{\rho}}\{\nu_{\mathrm{P}}(a\ast b)\},\sup\limits_{a\in(x)_{\rho}}\{\nu_{\mathrm{P}}(a)\}\}&\text{by Proposition 1.9 (2)}\\ & = \max\{\underline{\nu}_{\mathrm{P}}(x\ast y),\underline{\nu}_{\mathrm{P}}(x)\}. \end{align*}$

Hence, $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy UP-filter of $U$ .

(4) Assume that $\mathrm{P}$ is a Pythagorean fuzzy UP-ideal of $U$ , $\rho$ is complete, and $(0)_{\rho} = \{0\}$ . Then for all $x, y, z\in U$ ,

$\begin{align*} \underline{\mu}_{\mathrm{P}}(x\ast z) & = \inf\limits_{d\in(x\ast z)_{\rho}}\{\mu_{\mathrm{P}}(d)\}\\ & = \inf\limits_{d\in(x)_{\rho}(z)_{\rho}}\{\mu_{\mathrm{P}}(d)\}\\ & = \inf\limits_{a\ast c\in(x)_{\rho}(z)_{\rho}}\{\mu_{\mathrm{P}}(a\ast c)\}\\ &\geq\inf\limits_{a\ast(b\ast c)\in(x)_{\rho}((y)_{\rho}(z)_{\rho}),b\in(y)_{\rho}}\{\min\{\mu_{\mathrm{P}}(a\ast(b\ast c)),\mu_{\mathrm{P}}(b)\}\}&\text{by (23)}\\ & = \inf\limits_{a\ast(b\ast c)\in(x\ast(y\ast z))_{\rho},b\in(y)_{\rho}}\{\min\{\mu_{\mathrm{P}}(a\ast(b\ast c)),\mu_{\mathrm{P}}(b)\}\}\\ & = \min\{\inf\limits_{a\ast(b\ast c)\in(x\ast(y\ast z))_{\rho}}\{\mu_{\mathrm{P}}(a\ast(b\ast c))\},\inf\limits_{b\in(y)_{\rho}}\{\mu_{\mathrm{P}}(b)\}\}&\text{by Proposition 1.9 (1)}\\ & = \min\{\underline{\mu}_{\mathrm{P}}(x\ast(y\ast z)),\underline{\mu}_{\mathrm{P}}(y)\}, \end{align*}$

and

$\begin{align*} \underline{\nu}_{\mathrm{P}}(x\ast z) & = \sup\limits_{d\in(x\ast z)_{\rho}}\{\nu_{\mathrm{P}}(d)\}\\ & = \sup\limits_{d\in(x)_{\rho}(z)_{\rho}}\{\nu_{\mathrm{P}}(d)\}\\ & = \sup\limits_{a\ast c\in(x)_{\rho}(z)_{\rho}}\{\nu_{\mathrm{P}}(a\ast c)\}\\ &\leq\sup\limits_{a\ast(b\ast c)\in(x)_{\rho}((y)_{\rho}(z)_{\rho}),b\in(y)_{\rho}}\{\max\{\nu_{\mathrm{P}}(a\ast(b\ast c)),\nu_{\mathrm{P}}(b)\}\}&\text{by (24)}\\ & = \sup\limits_{a\ast(b\ast c)\in(x\ast(y\ast z))_{\rho},b\in(y)_{\rho}}\{\max\{\nu_{\mathrm{P}}(a\ast(b\ast c)),\nu_{\mathrm{P}}(b)\}\}\\ & = \max\{\sup\limits_{a\ast(b\ast c)\in(x\ast(y\ast z))_{\rho}}\{\nu_{\mathrm{P}}(a\ast(b\ast c))\},\sup\limits_{b\in(y)_{\rho}}\{\nu_{\mathrm{P}}(b)\}\}&\text{by Proposition 1.9 (2)}\\ & = \max\{\underline{\nu}_{\mathrm{P}}(x\ast(y\ast z)),\underline{\nu}_{\mathrm{P}}(y)\}. \end{align*}$

Hence, $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy UP-ideal of $U$ .

(5) Assume that $\mathrm{P}$ is a Pythagorean fuzzy strong UP-ideal of $U$ . By Theorem 2.3, we have $\mathrm{P}$ is constant. Then for all $x, y, z\in U$ ,

$\underline{\mu}_{\mathrm{P}}(0) = \inf\limits_{a\in(0)_{\rho}}\{\mu_{\mathrm{P}}(a)\} = \inf\limits_{b\in(x)_{\rho}}\{\mu_{\mathrm{P}}(b)\} = \underline{\mu}_{\mathrm{P}}(x),$

$\underline{\nu}_{\mathrm{P}}(0) = \sup\limits_{a\in(0)_{\rho}}\{\nu_{\mathrm{P}}(a)\} = \sup\limits_{b\in(x)_{\rho}}\{\nu_{\mathrm{P}}(b)\} = \underline{\nu}_{\mathrm{P}}(x),$

$\begin{align*} \underline{\mu}_{\mathrm{P}}(x) & = \inf\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)\}\\ &\geq\inf\limits_{(c\ast b)\ast(c\ast a)\in((z)_{\rho}(y)_{\rho})((z)_{\rho}(x)_{\rho}),b\in(y)_{\rho}}\{\min\{\mu_{\mathrm{P}}((c\ast b)\ast(c\ast a)),\mu_{\mathrm{P}}(b)\}\}&\text{by (25)}\\ &\geq\inf\limits_{(c\ast b)\ast(c\ast a)\in((z\ast y)\ast(z\ast x))_{\rho},b\in(y)_{\rho}}\{\min\{\mu_{\mathrm{P}}((c\ast b)\ast(c\ast a)),\mu_{\mathrm{P}}(b)\}\}\\ & = \min\{\inf\limits_{(c\ast b)\ast(c\ast a)\in((z\ast y)\ast(z\ast x))_{\rho}}\{\mu_{\mathrm{P}}((c\ast b)\ast(c\ast a))\},\inf\limits_{b\in(y)_{\rho}}\{\mu_{\mathrm{P}}(b)\}\}&\text{by Proposition 1.9 (1)}\\ & = \min\{\underline{\mu}_{\mathrm{P}}((z\ast y)\ast(z\ast x)),\underline{\mu}_{\mathrm{P}}(y)\}, \end{align*}$

and

$\begin{align*} \underline{\nu}_{\mathrm{P}}(x) & = \sup\limits_{a\in(x)_{\rho}}\{\nu_{\mathrm{P}}(a)\}\\ &\leq\sup\limits_{(c\ast b)\ast(c\ast a)\in((z)_{\rho}(y)_{\rho})((z)_{\rho}(x)_{\rho}),b\in(y)_{\rho}}\{\max\{\nu_{\mathrm{P}}((c\ast b)\ast(c\ast a)),\nu_{\mathrm{P}}(b)\}\}&\text{by (26)}\\ &\leq\sup\limits_{(c\ast b)\ast(c\ast a)\in((z\ast y)\ast(z\ast x))_{\rho},b\in(y)_{\rho}}\{\max\{\nu_{\mathrm{P}}((c\ast b)\ast(c\ast a)),\nu_{\mathrm{P}}(b)\}\}\\ & = \max\{\sup\limits_{(c\ast b)\ast(c\ast a)\in((z\ast y)\ast(z\ast x))_{\rho}}\{\nu_{\mathrm{P}}((c\ast b)\ast(c\ast a))\},\sup\limits_{b\in(y)_{\rho}}\{\nu_{\mathrm{P}}(b)\}\}&\text{by Proposition 1.9 (2)}\\ & = \max\{\underline{\nu}_{\mathrm{P}}((z\ast y)\ast(z\ast x)),\underline{\nu}_{\mathrm{P}}(y)\}. \end{align*}$

Hence, $\rho^{-}(\mathrm{P})$ is a Pythagorean fuzzy strong UP-ideal of $U$ .

The following example shows that Theorem 4.6 (3) may be not true if $(0)_{\rho}\neq\{0\}$ .

Example 4.7. Let $U = \{0, 1, 2, 3\}$ be a UP-algebra with a fixed element $0$ and a binary operation $\ast$ defined by the following Cayley table:

$\begin{equation*} \begin{array}{c|cccc} \ast & 0 & 1 & 2 & 3 \\ \hline 0 & 0 & 1 & 2 & 3 \\ 1 & 0 & 0 & 2 & 0 \\ 2 & 0 & 1 & 0 & 3 \\ 3 & 0 & 1 & 2 & 0 \end{array} \end{equation*}$

We define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 0.7 & 0.4 & 0.6 & 0.6 \\ \nu_{\mathrm{P}} & 0.2 & 0.6 & 0.3 & 0.3 \end{array} \end{equation*}$

Then $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy UP-filter of $U$ . Let

$\rho = \{(0,0),(1,1),(2,2),(3,3),(0,1),(1,0),(0,3),(3,0)\}.$

Then $\rho$ is a congruence relation on $U$ . Thus

$(0)_{\rho} = (1)_{\rho} = (3)_{\rho} = \{0,1,3\},(2)_{\rho} = \{2\}.$

Since $\underline{\mu}_{\mathrm{P}}(0) = \min\{\mu_{\mathrm{P}}(0), \mu_{\mathrm{P}}(1), \mu_{\mathrm{P}}(3)\} = \min\{0.7, 0.4, 0.6\} = 0.4\not\geq0.6 = \mu_{\mathrm{P}}(2) = \underline{\mu}_{\mathrm{P}}(2)$ and $\underline{\nu}_{\mathrm{P}}(0) = \max\{\nu_{\mathrm{P}}(0), \nu_{\mathrm{P}}(1), \nu_{\mathrm{P}}(3)\} = \max\{0.2, 0.6, 0.3\} = 0.6\not\leq0.3 = \nu_{\mathrm{P}}(2) = \underline{\nu}_{\mathrm{P}}(2)$ , we have $\rho^{-}(\mathrm{P})$ is not a Pythagorean fuzzy UP-filter of $U$ .

The following example shows that Theorem 4.6 (4) may be not true if $(0)_{\rho}\neq\{0\}$ and $\rho$ is not complete.

Example 4.8. From Example 2.11, we define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 1 & 0.2 & 0.1 & 0.5 \\ \nu_{\mathrm{P}} & 0 & 0.6 & 0.9 & 0.4 \end{array} \end{equation*}$

Then $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy UP-ideal of $U$ . Let

$\rho = \{(0,0),(1,1),(2,2),(3,3),(0,2),(2,0)\}.$

Then $\rho$ is a congruence relation on $U$ . Thus

$(0)_{\rho} = (2)_{\rho} = \{0,2\},(1)_{\rho} = \{1\},(3)_{\rho} = \{3\}.$

Since $\underline{\mu}_{\mathrm{P}}(0) = \min\{\mu_{\mathrm{P}}(0), \mu_{\mathrm{P}}(2)\} = \min\{1, 0.1\} = 0.1\not\geq0.2 = \mu_{\mathrm{P}}(1) = \underline{\mu}_{\mathrm{P}}(1)$ and $\underline{\nu}_{\mathrm{P}}(0) = \max\{\nu_{\mathrm{P}}(0), \nu_{\mathrm{P}}(2)\} = \max\{0, 0.9\} = 0.9\not\leq0.6 = \nu_{\mathrm{P}}(1) = \underline{\nu}_{\mathrm{P}}(1)$ , we have $\rho^{-}(\mathrm{P})$ is not a Pythagorean fuzzy UP-ideal of $U$ .

Problem 4.9. Is the lower approximation $\rho^{-}(\mathrm{P})$ a Pythagorean fuzzy UP-ideal of $U$ if $\mathrm{P}$ is a Pythagorean fuzzy UP-ideal, $(0)_{\rho}\neq\{0\}$ , and $\rho$ is complete?

Lemma 4.10. If $\rho$ is an congruence relation on a UP-algebra $U = (U, \ast, 0)$ and $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ a Pythagorean fuzzy UP-subalgebra of $U$ , then the upper approximation $\rho^{+}(\mathrm{P})$ fulfills the following assertions:

$\begin{gather} (\mathit{\text{for all}}\; x\in U)(\overline{\mu}_{\mathrm{P}}(0)\geq\overline{\mu}_{\mathrm{P}}(x)), \end{gather}$

(52)

$\begin{gather} (\mathit{\text{for all}}\; x\in U)(\overline{\nu}_{\mathrm{P}}(0)\leq\overline{\nu}_{\mathrm{P}}(x)). \end{gather}$

(53)

Proof. Let $x\in U$ . Then

$\begin{gather*} \overline{\mu}_{\mathrm{P}}(0) = \sup\limits_{a\in(0)_{\rho}}\{\mu_{\mathrm{P}}(a)\} \geq\mu_{\mathrm{P}}(0) \geq\sup\limits_{b\in(x)_{\rho}}\{\mu_{\mathrm{P}}(b)\}\;\;\;\;\;\;\;\;\text{by (19)} = \overline{\mu}_{\mathrm{P}}(x) \end{gather*}$

and

$\begin{gather*} \overline{\nu}_{\mathrm{P}}(0) = \inf\limits_{a\in(0)_{\rho}}\{\nu_{\mathrm{P}}(a)\} \leq\nu_{\mathrm{P}}(0) \leq\inf\limits_{b\in(x)_{\rho}}\{\nu_{\mathrm{P}}(b)\}\;\;\;\;\;\;\;\;\text{by (20)} = \overline{\nu}_{\mathrm{P}}(x). \end{gather*}$

Hence, $\rho^{+}(\mathrm{P})$ fulfills the assertions (52) and (53).

Theorem 4.11. Let $\rho$ be an congruence relation on a UP-algebra $U = (U, \ast, 0)$ and $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ a Pythagorean fuzzy set in $U$ . Then the following statements hold:

(1) If $\mathrm{P}$ is a Pythagorean fuzzy UP-subalgebra of $U$ , then $\rho^{+}(\mathrm{P})$ is a Pythagorean fuzzy UP-subalgebra of $U$ ,

(2) If $\mathrm{P}$ is a Pythagorean fuzzy near UP-filter of $U$ , then $\rho^{+}(\mathrm{P})$ is a Pythagorean fuzzy near UP-filter of $U$ , and

(3) If $\mathrm{P}$ is a Pythagorean fuzzy strong UP-ideal of $U$ , then $\rho^{+}(\mathrm{P})$ is a Pythagorean fuzzy strong UP-ideal of $U$ .

Proof. (1) Assume that $\mathrm{P}$ is a Pythagorean fuzzy UP-subalgebra of $U$ . Then for all $x, y\in U$ ,

Case 1: $x = y$ . Then

$\begin{gather*} \overline{\mu}_{\mathrm{P}}(x\ast y) = \overline{\mu}_{\mathrm{P}}(0) \geq\overline{\mu}_{\mathrm{P}}(x)\;\;\;\;\;\;\;\;\text{by (1), (52)} \geq\min\{\overline{\mu}_{\mathrm{P}}(x),\overline{\mu}_{\mathrm{P}}(y)\} \end{gather*}$

and

$\begin{gather*} \overline{\nu}_{\mathrm{P}}(x\ast y) = \overline{\nu}_{\mathrm{P}}(0) \leq\overline{\nu}_{\mathrm{P}}(x)\;\;\;\;\;\;\;\;\text{by (1), (53)} \leq\max\{\overline{\nu}_{\mathrm{P}}(x),\overline{\nu}_{\mathrm{P}}(y)\}. \end{gather*}$

Case 2: $x\neq y$ .

Case 2.1: $x\ast y = x$ or $y$ . It is sufficient to assume that $x\ast y = x$ . Then

$\overline{\mu}_{\mathrm{P}}(x\ast y) = \overline{\mu}_{\mathrm{P}}(x)\geq\min\{\overline{\mu}_{\mathrm{P}}(x),\overline{\mu}_{\mathrm{P}}(y)\}$

and

$\overline{\nu}_{\mathrm{P}}(x\ast y) = \overline{\nu}_{\mathrm{P}}(x)\leq\max\{\overline{\nu}_{\mathrm{P}}(x),\overline{\nu}_{\mathrm{P}}(y)\}.$

Case 2.2: $x\ast y\neq x$ and $x\ast y\neq y$ . Assume that there exists $z\in U$ be such that $x\ast y = z$ . If $z\rho 0$ , then

$\begin{align*} \overline{\mu}_{\mathrm{P}}(x\ast y) = \overline{\mu}_{\mathrm{P}}(z) = \overline{\mu}_{\mathrm{P}}(0)\geq\min\{\overline{\mu}_{\mathrm{P}}(x),\overline{\mu}_{\mathrm{P}}(y)\}\;\;\;\;\;\;\;\;\text{by (49)} \end{align*}$

and

$\begin{align*} \overline{\nu}_{\mathrm{P}}(x\ast y) = \overline{\nu}_{\mathrm{P}}(z) = \overline{\nu}_{\mathrm{P}}(0)\leq\max\{\overline{\nu}_{\mathrm{P}}(x),\overline{\nu}_{\mathrm{P}}(y)\}\;\;\;\;\;\;\;\;\text{by (50)}. \end{align*}$

If $x\rho0$ or $y\rho0$ , it is sufficient to assume that $x\rho0$ . Since $\rho$ is a congruence relation on $U$ , we have $xy\rho0y$ , that is, $z\rho y$ . Therefore,

$\begin{gather*} \overline{\mu}_{\mathrm{P}}(x\ast y) = \overline{\mu}_{\mathrm{P}}(z) = \overline{\mu}_{\mathrm{P}}(y)\;\;\;\;\;\;\;\;\text{by (49), (52)} = \min\{\overline{\mu}_{\mathrm{P}}(0),\overline{\mu}_{\mathrm{P}}(y)\} = \min\{\overline{\mu}_{\mathrm{P}}(x),\overline{\mu}_{\mathrm{P}}(y)\} \end{gather*}$

and

$\begin{gather*} \overline{\nu}_{\mathrm{P}}(x\ast y) = \overline{\nu}_{\mathrm{P}}(z) = \overline{\nu}_{\mathrm{P}}(y)\;\;\;\;\;\;\;\;\text{by (50), (53)} = \min\{\overline{\nu}_{\mathrm{P}}(0),\overline{\nu}_{\mathrm{P}}(y)\} = \max\{\overline{\nu}_{\mathrm{P}}(x),\overline{\nu}_{\mathrm{P}}(y)\}. \end{gather*}$

Hence, $\rho^{+}(\mathrm{P})$ is a Pythagorean fuzzy UP-subalgebra of $U$ .

(2) Assume that $\mathrm{P}$ is a Pythagorean fuzzy near UP-filter of $U$ . Then for all $x, y\in U$ ,

$\begin{gather*} \overline{\mu}_{\mathrm{P}}(x\ast y) = \sup\limits_{c\in(x\ast y)_{\rho}}\{\mu_{\mathrm{P}}(c)\} \geq\sup\limits_{c\in(x)_{\rho}(y)_{\rho}}\{\mu_{\mathrm{P}}(c)\} = \sup\limits_{a\ast b\in(x)_{\rho}(y)_{\rho}}\{\mu_{\mathrm{P}}(a\ast b)\} \geq\sup\limits_{b\in(y)_{\rho}}\{\mu_{\mathrm{P}}(b)\}\;\;\;\;\;\;\;\;\text{by (17)} = \overline{\mu}_{\mathrm{P}}(y) \end{gather*}$

and

$\begin{gather*} \overline{\nu}_{\mathrm{P}}(x\ast y) = \inf\limits_{c\in(x\ast y)_{\rho}}\{\nu_{\mathrm{P}}(c)\} \leq\inf\limits_{c\in(x)_{\rho}(y)_{\rho}}\{\nu_{\mathrm{P}}(c)\} = \inf\limits_{a\ast b\in(x)_{\rho}(y)_{\rho}}\{\nu_{\mathrm{P}}(a\ast b)\} \leq\inf\limits_{b\in(y)_{\rho}}\{\nu_{\mathrm{P}}(b)\}\;\;\;\;\;\;\;\;\text{by (18)} = \overline{\nu}_{\mathrm{P}}(y). \end{gather*}$

Hence, $\rho^{+}(\mathrm{P})$ is a Pythagorean fuzzy near UP-filter of $U$ .

(3) Assume that $\mathrm{P}$ is a Pythagorean fuzzy strong UP-ideal of $U$ . By Theorem 2.3, we have $\mathrm{P}$ is constant. Then for all $x, y, z\in U$ ,

$\overline{\mu}_{\mathrm{P}}(0) = \sup\limits_{a\in(0)_{\rho}}\{\mu_{\mathrm{P}}(a)\} = \sup\limits_{b\in(x)_{\rho}}\{\mu_{\mathrm{P}}(b)\} = \overline{\mu}_{\mathrm{P}}(x),$

$\overline{\nu}_{\mathrm{P}}(0) = \inf\limits_{a\in(0)_{\rho}}\{\nu_{\mathrm{P}}(a)\} = \inf\limits_{b\in(x)_{\rho}}\{\nu_{\mathrm{P}}(b)\} = \overline{\nu}_{\mathrm{P}}(x),$

$\begin{align*} \overline{\mu}_{\mathrm{P}}(x) & = \sup\limits_{a\in(x)_{\rho}}\{\mu_{\mathrm{P}}(a)\}\\ &\geq\sup\limits_{(c\ast b)\ast(c\ast a)\in((z)_{\rho}(y)_{\rho})((z)_{\rho}(x)_{\rho}),b\in(y)_{\rho}}\{\min\{\mu_{\mathrm{P}}((c\ast b)\ast(c\ast a)),\mu_{\mathrm{P}}(b)\}\}&\text{by (25)}\\ & = \sup\limits_{(c\ast b)\ast(c\ast a)\in((z\ast y)\ast(z\ast x))_{\rho},b\in(y)_{\rho}}\{\min\{\mu_{\mathrm{P}}((c\ast b)\ast(c\ast a)),\mu_{\mathrm{P}}(b)\}\}\\ & = \min\{\sup\limits_{(c\ast b)\ast(c\ast a)\in((z\ast y)\ast(z\ast x))_{\rho}}\{\mu_{\mathrm{P}}((c\ast b)\ast(c\ast a))\},\sup\limits_{b\in(y)_{\rho}}\{\mu_{\mathrm{P}}(b)\}\}&\text{by $\mathrm{P}$ is constant}\\ & = \min\{\overline{\mu}_{\mathrm{P}}((z\ast y)\ast(z\ast x)),\overline{\mu}_{\mathrm{P}}(y)\}, \end{align*}$

and

$\begin{align*} \overline{\nu}_{\mathrm{P}}(x) & = \inf\limits_{a\in(x)_{\rho}}\{\nu_{\mathrm{P}}(a)\}\\ &\leq\inf\limits_{(c\ast b)\ast(c\ast a)\in((z)_{\rho}(y)_{\rho})((z)_{\rho}(x)_{\rho}),b\in(y)_{\rho}}\{\max\{\nu_{\mathrm{P}}((c\ast b)\ast(c\ast a)),\nu_{\mathrm{P}}(b)\}\}&\text{by (26)}\\ & = \inf\limits_{(c\ast b)\ast(c\ast a)\in((z\ast y)\ast(z\ast x))_{\rho},b\in(y)_{\rho}}\{\max\{\nu_{\mathrm{P}}((c\ast b)\ast(c\ast a)),\nu_{\mathrm{P}}(b)\}\}\\ & = \max\{\inf\limits_{(c\ast b)\ast(c\ast a)\in((z\ast y)\ast(z\ast x))_{\rho}}\{\nu_{\mathrm{P}}((c\ast b)\ast(c\ast a))\},\inf\limits_{b\in(y)_{\rho}}\{\nu_{\mathrm{P}}(b)\}\}&\text{by $\mathrm{P}$ is constant}\\ & = \max\{\overline{\nu}_{\mathrm{P}}((z\ast y)\ast(z\ast x)),\overline{\nu}_{\mathrm{P}}(y)\}. \end{align*}$

Hence, $\rho^{+}(\mathrm{P})$ is a Pythagorean fuzzy strong UP-ideal of $U$ .

The following example shows that if $\mathrm{P}$ is a Pythagorean fuzzy UP-filter of $U$ , then the upper approximation $\rho^{+}(\mathrm{P})$ is not a Pythagorean fuzzy UP-filter in general.

Example 4.12. From Example 3.8, we define a Pythagorean fuzzy set $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ with $\mu_{\mathrm{P}}$ and $\nu_{\mathrm{P}}$ as follows:

$\begin{equation*} \begin{array}{c|cccc} U & 0 & 1 & 2 & 3 \\ \hline \mu_{\mathrm{P}} & 0.6 & 0.5 & 0.3 & 0.3 \\ \nu_{\mathrm{P}} & 0.3 & 0.4 & 0.7 & 0.7 \end{array} \end{equation*}$

Then $\mathrm{P} = (\mu_{\mathrm{P}}, \nu_{\mathrm{P}})$ is a Pythagorean fuzzy UP-filter of $U$ . Let

$\rho = \{(0,0),(1,1),(2,2),(3,3),(3,0),(0,3)\}.$

Then $\rho$ is a congruence relation on $U$ . Thus

$(0)_{\rho} = (3)_{\rho} = \{0,3\},(1)_{\rho} = \{1\},(2)_{\rho} = \{2\}.$

Since $\overline{\mu}_{\mathrm{P}}(2) = \mu_{\mathrm{P}}(2) = 0.3\not\geq0.5 = \min\{\max\{\mu_{\mathrm{P}}(0), \mu_{\mathrm{P}}(3)\}, \mu_{\mathrm{P}}(1)\}\} = \min\{\overline{\mu}_{\mathrm{P}}(3), \overline{\mu}_{\mathrm{P}}(1)\} = \min\{\overline{\mu}_{\mathrm{P}}(1\ast2), \overline{\mu}_{\mathrm{P}}(1)\}$ . we have $\rho^{+}(\mathrm{P})$ is not a Pythagorean fuzzy UP-filter of $U$ .

Problem 4.13. Is the upper approximation $\rho^{+}(\mathrm{P})$ a Pythagorean fuzzy UP-filter of $U$ if $\mathrm{P}$ is a Pythagorean fuzzy UP-filter of $U$ ?

5. Conclusions and future works

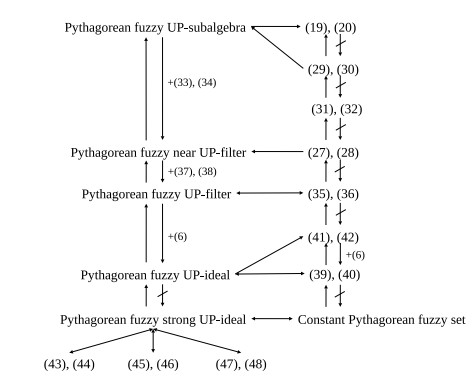
In this paper, we have introduced the concept of Pythagorean fuzzy sets in UP-algebras, and then we have introduced five types of Pythagorean fuzzy sets in UP-algebras, namely Pythagorean fuzzy UP-subalgebras, Pythagorean fuzzy near UP-filters, Pythagorean fuzzy UP-filters, Pythagorean fuzzy UP-ideals, and Pythagorean fuzzy strong UP-ideals. Further, we have discussed the relationship between some assertions of Pythagorean fuzzy sets and Pythagorean fuzzy UP-subalgebras (resp., Pythagorean fuzzy near UP-filters, Pythagorean fuzzy UP-filters, Pythagorean fuzzy UP-ideals, Pythagorean fuzzy strong UP-ideals) in UP-algebras and have studied upper and lower approximations of Pythagorean fuzzy sets. Hence, we get the diagram of generalization of Pythagorean fuzzy sets in UP-algebras, which is shown with Figure 2.

Figure 2. Pythagorean fuzzy sets in UP-algebras.

DownLoad: Full-Size Img PowerPoint

Some important topics for our future study of UP-algebras are as follows:

(1) to get more results in Pythagorean fuzzy sets,

(2) to define new types of Pythagorean fuzzy sets,

(3) to get more results and examples in upper approximation and lower approximation,

(4) to study the roughness of Pythagorean fuzzy sets, and

(5) to study the soft set theory of Pythagorean fuzzy sets.

Acknowledgments

This work was supported by the Unit of Excellence in Mathematics, University of Phayao.

Conflict of interest

The authors declare no conflict of interest.

References

[1]	M. A. Ansari, A. Haidar, A. N. A. Koam, On a graph associated to UP-algebras, Math. Comput. Appl., 23 (2018), 1–12.
[2]	M. A. Ansari, A. N. A. Koam, A. Haider, Rough set theory applied to UP-algebras, Ital. J. Pure Appl. Math., 42 (2019), 388–402.
[3]	K. T. Atanassov, Intuitionistic fuzzy sets, Fuzzy Set. Syst., 20 (1986), 87–96. doi: 10.1016/S0165-0114(86)80034-3
[4]	R. Chinram, A. Iampan, Codewords generated by UP-valued functions, AIMS Mathematics, 6 (2021), 4771–4785. doi: 10.3934/math.2021280
[5]	R. Chinram, T. Panityakul, Rough Pythagorean fuzzy ideals in ternary semigroups, J. Math. Comput. Sci., 20 (2020), 303–312.
[6]	N. Dokkhamdang, A. Kesorn, A. Iampan, Generalized fuzzy sets in UP-algebras, Ann. Fuzzy Math. Inform., 16 (2018), 171–190. doi: 10.30948/afmi.2018.16.2.171
[7]	W. A. Dudek, Y. B. Jun, H. S. Kim, Rough set theory applied to BCI-algebras, Quasigroups Relat. Syst., 9 (2002), 45–54.
[8]	T. Guntasow, S. Sajak, A. Jomkham, A. Iampan, Fuzzy translations of a fuzzy set in UP-algebras, J. Indones. Math. Soc., 23 (2017), 1–19. doi: 10.22342/jims.23.2.371.1-19
[9]	A. Hussain, T. Mahmood, M. I. Ali, Rough Pythagorean fuzzy ideals in semigroups, Comput. Appl. Math., 38 (2019), 1–15. doi: 10.1007/s40314-019-0767-y
[10]	A. Iampan, Multipliers and near UP-filters of UP-algebras, Journal of Discrete Mathematical Sciences and Cryptography, 2019, 1–14.
[11]	A. Iampan, A new branch of the logical algebra: UP-algebras, J. Algebra Relat. Top., 5 (2017), 35–54.
[12]	A. Iampan, Introducing fully UP-semigroups, Discuss. Math. Gen. Algebra Appl., 38 (2018), 297–306. doi: 10.7151/dmgaa.1290
[13]	A. Iampan, A. Satirad, M. Songsaeng, A note on UP-hyperalgebras, J. Algebr. Hyperstruct. Log. Algebr., 1 (2020), 77–95. doi: 10.29252/hatef.jahla.1.2.7
[14]	A. Iampan, M. Songsaeng, G. Muhiuddin, Fuzzy duplex UP-algebras, Eur. J. Pure Appl. Math., 13 (2020), 459–471. doi: 10.29020/nybg.ejpam.v13i3.3752
[15]	Y. Imai, K. Iséki, On axiom systems of propositional calculi. XIV, Proc. Japan Acad., 42 (1966), 19–22.
[16]	K. Iséki, An algebra related with a propositional calculus, Proc. Japan Acad., 42 (1966), 26–29.
[17]	R. Jansi, K. Mohana, Bipolar Pythagorean fuzzy A-ideals of BCI-algebra, International Journal of Innovative Science, Engineering & Technology, 6 (2019), 2348–7968.
[18]	Y. B. Jun, Roughness of ideals in BCK-algebras, Sci. Math. Japonica. Online, 7 (2002), 115–119.
[19]	H. S. Kim, Y. H. Kim, On BE-algebras, Sci. Math. Japonica. Online, 2006 (2006), 1299–1302.
[20]	T. Klinseesook, S. Bukok, A. Iampan, Rough set theory applied to UP-algebras, Journal of Information and Optimization Sciences, 41 (2020), 705–722. doi: 10.1080/02522667.2018.1552511
[21]	S. M. Mostafa, M. A. A. Naby, M. M. M. Yousef, Fuzzy ideals of KU-algebras, Int. Math. Forum, 63 (2011), 3139–3149.
[22]	Z. Pawlak, Rough sets, Internat. J. Comput. Inform. Sci., 11 (1982), 341–356. doi: 10.1007/BF01001956
[23]	C. Prabpayak, U. Leerawat, On ideals and congruences in KU-algebras, Sci. Magna, 5 (2009), 54–57.
[24]	A. Satirad, R. Chinram, A. Iampan, Four new cnocepts of extension of KU/UP-algebras, Missouri J. Math. Sci., 32 (2020), 138–157.
[25]	A. Satirad, A. Iampan, Fuzzy sets in fully UP-semigroups, Ital. J. Pure Appl. Math., 42 (2019), 539–558.
[26]	A. Satirad, A. Iampan, Fuzzy soft sets over fully UP-semigroups, Eur. J. Pure Appl. Math., 12 (2019), 294–331. doi: 10.29020/nybg.ejpam.v12i2.3412
[27]	A. Satirad, A. Iampan, Topological UP-algebras, Discuss. Math. Gen. Algebra Appl., 39 (2019), 231–250. doi: 10.7151/dmgaa.1317
[28]	A. Satirad, P. Mosrijai, A. Iampan, Formulas for finding UP-algebras, Int. J. Math. Comput. Sci., 14 (2019), 403–409.
[29]	A. Satirad, P. Mosrijai, A. Iampan, Generalized power UP-algebras, Int. J. Math. Comput. Sci., 14 (2019), 17–25.
[30]	T. Senapati, Y. B. Jun, K. P. Shum, Cubic set structure applied in UP-algebras, Discrete Math. Algorithms Appl., 10 (2018), 1850049. doi: 10.1142/S1793830918500490
[31]	T. Senapati, G. Muhiuddin, K. P. Shum, Representation of UP-algebras in interval-valued intuitionistic fuzzy environment, Ital. J. Pure Appl. Math., 38 (2017), 497-517.
[32]	J. Somjanta, N. Thuekaew, P. Kumpeangkeaw, A. Iampan, Fuzzy sets in UP-algebras, Ann. Fuzzy Math. Inform., 12 (2016), 739–756.
[33]	R. R. Yager, Pythagorean fuzzy subsets, 2013 Joint IFSA World Congress NAFIPS Annual Meeting (IFSA/NAFIPS), 2013, 57–61.
[34]	R. R. Yager, A. M. Abbasov, Pythagorean member grades, complex numbers, and decision making, Int. J. Intell. Syst., 28 (2013), 436–452. doi: 10.1002/int.21584
[35]	L. A. Zadeh, Fuzzy sets, Inform. Contr., 8 (1965), 338–353. doi: 10.1016/S0019-9958(65)90241-X

This article has been cited by:

1.	Rukchart Prasertpong, Roughness of soft sets and fuzzy sets in semigroups based on set-valued picture hesitant fuzzy relations, 2022, 7, 2473-6988, 2891, 10.3934/math.2022160
2.	Harish Garg, Gia Sirbiladze, Zeeshan Ali, Tahir Mahmood, Hamy Mean Operators Based on Complex q-Rung Orthopair Fuzzy Setting and Their Application in Multi-Attribute Decision Making, 2021, 9, 2227-7390, 2312, 10.3390/math9182312
3.	Muhammad Kamran, Rashad Ismail, Shahzaib Ashraf, Nadeem Salamat, Seyma Ozon Yildirim, Ismail Naci Cangul, Decision support algorithm under SV-neutrosophic hesitant fuzzy rough information with confidence level aggregation operators, 2023, 8, 2473-6988, 11973, 10.3934/math.2023605
4.	Yixiong Feng, Kaiyue Cui, Zhaoxi Hong, Zhiwu Li, Weiyu Yan, Jianrong Tan, Disassembly Sequence Planning of Product Structure With an Improved QICA Considering Expert Consensus for Remanufacturing, 2023, 19, 1551-3203, 7201, 10.1109/TII.2022.3231922

Reader Comments

Your name:*

Email:*
© 2021 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(3276) PDF downloads(297) Cited by(4)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(2)

AIMS Mathematics

Pythagorean fuzzy sets in UP-algebras and approximations

Related Papers:

Abstract

1. Introduction and preliminaries

2. Pythagorean fuzzy sets in UP-algebras

3. Properties of Pythagorean fuzzy sets in UP-algebras

4. Approximations

5. Conclusions and future works

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Pythagorean fuzzy sets in UP-algebras and approximations

Related Papers:

Abstract

1. Introduction and preliminaries

2. Pythagorean fuzzy sets in UP-algebras

3. Properties of Pythagorean fuzzy sets in UP-algebras

4. Approximations

5. Conclusions and future works

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog