Nomenclature
Greek symbols
1.
Introduction
In modern times, electro-osmosis is a preeminent and stimulating mechanism due to its involvement in several kinds of devices at macro and nano scales. An abundant range of its practical applications includes bio-pharmaceutical inspections, industrial operations, chemical separation procedures, and biochemistry. The migration of a liquid caused due to the imposition of electric power about mediums with porous holes, blood vessels, micro-channels, layers of cells, or any other fluid conveying systems is termed as electro-osmosis. The credit for this electrifying mechanism is attributed to Reuss [1]. In the initial decade of 19th century, he introduced the idea of electro-osmosis on water transportation through clay during his pioneering experimental research. About four decades later, following the theme of Reuss, Wiedemann [2] reconfigured the experiment and discussed the formal mathematics involved in this phenomenon. Mainly, electro-osmotic flows involve the transport of ions, and they are described with the assistance of some fundamental theories and principles. A few of them are Helmholtz-Smudchowski theory [3], Schmid's theory, Debye-Huckel approximation, and ion-hydration theory [4,5]. In recent times, various imperative devices of microfluidics operate on the principle of electro-osmosis to achieve the rigorous delivery of the involved fluids. The exceptional role of microfluidics can be found in nanotechnology, energy harvesting, micro-propulsion, material processing, determining prognosis, and development of Deoxyribonucleic acid chips. Additionally, microfluidic flows specify some stimulating consequences and unintuitive features. For instance, there are minute chances for the traditional mixing of different fluids because, at the micro-scale, these flows become laminar. Besides this, such flows ensure the high specificity of physical and chemical characteristics, which leads to produce better reactive conditions [6]. However, the major complication faced in microfluidics is the actuation of fluid flow that is usually procured through the ability of fluid to integrate with surface-tension of conduits or due to the combined action of electrokinetic and capillary forces. The said complication arises as a consequence of size depletion of section geometry to micro-scale because, for such cases, the size of the channel strongly influences the velocity of the fluid. An adequate alternative to overcome this issue is the external pumping of fluids via peristalsis, mechanical pumps, electro-osmosis, syringe pumps, or some other exterior pressure sources. The primary reason to operate electro-osmosis as the preferred external pumping means is that the conduit measurements have no effects on the velocity of the fluid. Some supplementary advantages of electro-osmosis are accurate mobility, better control, enhanced efficiency, easily implementable, non-moving mechanical parts, and inexpensive fabrication of devices [7,8]. Observing the broad utility range of electro-osmosis, numerous engineers and scientists are focused to explore the vast effectiveness of this phenomenon for various working liquids such as blood, polymeric arrangements, salt solutions, industrial adhesives, colloidal suspensions, and so forth. In this regard, multitudinous numerical [9,10,11,12], theoretical [13,14,15,16], and experimental [17] investigations have been performed to mature the available facts.
Keeping view of the significant relevance of electro-osmotic flows in the development of microfluidic equipment, Hadian et al. [18] adapted an analytic technique to evaluate the temperature distribution associated with electro-osmotic flow established through a slit microchannel and to find the relative contribution of imposed electric strength and channel height in development of the temperature distribution. Dejam [19] computed an analytic expression to discuss the dispersion phenomenon for the electro-osmotic flow of a viscoelastic fluid inside a channel, which possesses porous walls. Mishra and Sinha [20] considered the biological problem of heat transfer in blood carriage through an artery with Navier slip as a practical-life demonstrative example of electro-osmosis. Recently, Ponalagusamy and Manchi [21] reported that the combined application of magnetic and electric fields substantially controls Jeffrey fluid transport through a stenosed duct with blood cell dispersion. Azari et al. [22] presented analytic solutions to investigate an exclusive coupling between heat transmission and surface charge asymmetries for an electro-osmosis flow inside a circular channel. A computational model to analyze electro-osmosis through a microfluidic porous channel subject to various flow profiles is formulated by Dejam [23]. Moghadam [24] examined the thermal efficiency of some electro-osmotically established flows in an annulus to study the influence of several alternatively appearing electric fields.
The fluids accounting mass and heat transmission phenomena at the same time are of broad utilization in several industrial and chemical engineering problems such as sample separation, temperature preservation, climate control, and food processing. Heat and mass transfer collectively take place due to the simultaneous impacts of species and energy gradients. In many complex engineering situations, the combined occurrence of these phenomena in electromagnetically established flows possesses a crucial contribution. Particularly mass transfer is considered a fundamental unit for multiple biological, aeronautical, and chemical problems. For instance, the transfer of ions inside the kidney through membranes and oxygenation of the human body are sub-disciplines of biological processes. Meanwhile, the formation of semiconducting thin films, water purification, coating of a silicon wafer, and polymer production are some common examples of chemical processes [25]. To examine the main impacts of a chemical reaction and activation energy, Khan et al. [26] derived the dual solutions for magneto cross liquid migration close to a moving wedge. Systematic research was carried out to scrutinize the mass transfer mechanism of magnetohydrodynamic (MHD) Casson fluid under variable energy and solutal surface constraints by Kataria and Patel [27]. Zhao [28] operated a hybrid numerical method to explore the consequences of species diffusion on the free convectional transport of an Oldroyd-B nanofluid. Moving forward, it is worth considering that the heat transmission process can be maneuvered through radiation and induction of some heat injector/absorbent to achieve the desired result. The applications of radiative flows are witnessed at various industrial and environmental stages. For example, water evaporation from open channels, cooling and heating chambers, nuclear plants, combustion, and astrophysical flows [29]. Likewise, the installation of a heat injector/absorbent is an efficient solution for several complex problems like cooling of chips, heat treatments, ventilation, better performance of microprocessors, and storage of foodstuff [30]. Recently proposed studies on radiative flows including injection or absorption of heat have opened many new horizons. Saeed et al. [31] discussed the Maxwell fluid migration over an extending cylinder to highlight the productivity of heat injection/absorption and radiation for convective flows. Baslem et al. [32] utilized a numerical approach to analyze the improvement in the thermal behavior of some nanofluids resulted due to radiation phenomenon. Hayat et al. [33] theoretically addressed the role of nonlinear radiative flux and heat injector/absorbent to minimize the entropy generation. The effects of radiation on thermal functioning of chemically reactive Casson fluid during mixed convective flow near an inclined surface were deliberated by Sulochana et al. [34].
The idea of fractional calculus came into existence on the basis of a query made by L'Hospital in 1965. In a letter, he inquired from Leibniz about the best practical description of the nth derivative of an arbitrary function F(x) when n=1/2. In modern times, it is a well-recognized fact that L'Hospital's letter was the first discussion about fractional derivatives. To achieve the differentiation cause in an efficient manner, the utilization of fractional derivatives is more effectual as equated with regular derivatives. Mainly there are two key reasons firstly, there is no restriction on the choice of fractional parametric value, which leads to produce exceptional accordance between experimental results and theoretical information. Secondly, fractional derivatives encounter both current and previous time steps, and this characteristic makes them more adequate to restore and communicate the memory effects. Fractional derivatives have a remarkable history and a vast range of applications in multitudinous disciplines of economics, signal processing, biotechnology, medical, viscoelasticity, and many more [35,36,37]. Kumar et al. [38] constructed a fractional model to anticipate the expansion of malaria and to propose the medication via sprays, pills, and vaccination. Ahmed et al. [39] analyzed the principal reliability extent of lockdown to mitigate the dissemination of novel coronavirus by proposing a fractional model based on the Caputo derivative. Ullah and Khan [40] formulated a non-integer order model to evaluate the dynamics of hepatitis B and to develop the strategies for limiting the spread of the disease. Acay et al. [41] performed a comprehensive investigation to explore multiple aspects of different economical problems with the help of diverse fractional derivatives.
The development of different fractional operators is based on the convolution of classical derivatives and some particular kernels. Several kernels have been advised in the literature, which possess practical advantages on each other coupled with particular limitations. For example, the differentiation of a constant value is not zero in the case of the operator proposed by Reiman and Liouville [42]. Caputo [43] eliminated this limitation by proposing a different fractional operator, which later encountered the observations of having a singular kernel. To address the marked fault, Caputo and Fabrizio [44] further advised a model with a special exponential kernel. After its utilization in a variety of analyses, the disadvantage of possessing a local kernel came into sight. In particular, the incapability of a local kernel to exhibit the memory effect is the primary concern here. Atangana and Baleanu [45] circumvented the shortcomings of the aforementioned operator by propounding a novel operator containing a unique kernel that is neither local nor singular. In fluid mechanics, there are extensive applications of fractional operators to delineate the elastic, thermal, material, and viscous properties of various fluids. Furthermore, some of the essential rheological qualities of industrial liquids are precisely anticipated only through non-integer order simulations. Gemant [46] initiated the use of fractional calculus in viscoelasticity. With the assistance of semi-analytic expressions, Aman et al. [47] explored the physical behavior of Maxwell fluid subject to second-order slip flow. Awan et al. [48] operated a non-singular kernel to scrutinize electro-osmotic slip transportation of second grade fluid contained by two parallel unbounded walls. Jiang et al. [49] analyzed the electromagnetically developed circular motion of fractional Oldroyd-B fluid by employing Hankel and Laplace transformation methods. Asjad et al. [50] constructed a model with a non-integer order to explore the thermal behavior of a chemically reactive nanofluid composed of carbon nanotubes and Carboxyl methylcellulose.
The literature review discloses that piece-wise time-dependent boundary conditions are not jointly operated for momentum, concentration, and energy equations of a non-Newtonian fluid. Particularly, there is no fractional model in terms of Atangana-Baleanu fractional derivative, which studies the flow behavior of a chemically reactive and thermally radiative Walters' B fluid under heat injection/absorption effects. Moreover, there are very few investigations that simultaneously examine electro-osmotic flows with heat transfer phenomenon. The novelty of this work is the development of a fractional model based on Atangana-Baleanu fractional derivative to study the electro-osmotic transport mechanism of Walters' B fluid together with mass and heat transfer. In addition to this, piece-wise time-dependent boundary conditions are applied at the boundary wall to establish foregoing phenomena. The vertical wall is assumed to be nested in a porous material subject to the imposition of a magnetic influence. Laplace transform method is employed to produce integral form exact solutions of the modeled problem. These solutions are further utilized in determining the gradients of velocity, concentration, and energy functions to obtain the expressions for skin friction coefficient, Sherwood number, and Nusselt number respectively. A detailed illustrative and tabular analysis is performed to explore the quantities of physical significance and interest.
The article is arranged in the following order: Section 2 contains a description of the problem and formulation of governing equations, Section 3 presents the generalization of the developed model to fractional model along with analytic solutions, Section 4 discusses graphical and tabular results, and concluding remarks are provided in Section 5.
2.
Description and modeling of the problem
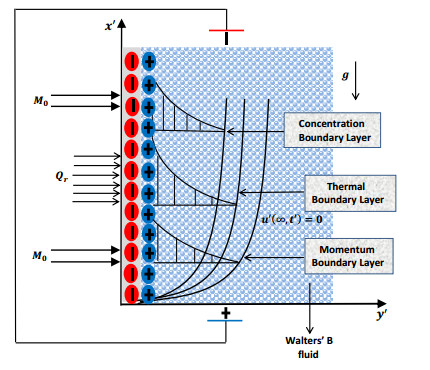
In this investigation, we have considered the boundary driven MHD flow of a chemically reacted and electrically insulated Walters' B fluid close to an unbounded surface. The upright wall situated along the vertical direction (marked as x′-axis) is encountering the radiative effects in the horizontal direction (labeled as y′-axis). The geometry of the considered problem is configured in Figure 1 in terms of the Cartesian system. Initially, for t′=0, the wall and fluid are static with concentration Φ′∞ and temperature Θ′∞. Later for 0<t′≤t′0, the boundary wall develops the flow of fluid due to its time-controlled motion with velocity ℑ′0(t′/t′0). For the same time interval, concentration and energy levels of the wall are maintained as Φ′∞+ΔΦ′(t′/t′0) and Θ′∞+ΔΘ′(t′/t′0) respectively. After this time duration, the wall expresses a uniform movement with velocity ℑ′0, and concentration and temperature are respectively changed to constant values as Φ′w and Θ′w, for t′>t′0.
Using expressions from the appendix, the final form of momentum equation is given along with energy and concentration equations
In the modeled equations, Cp is the heat capacitance, κ2 denotes the thermal conductance, Θ′ represents the fluid's temperature, φ is the porosity parameter, Q1 is the thermal injection/absorption coefficient, Qr stands for thermal radiative flux, D accounts for the mass diffusion, R′ specifies the rate of chemical reaction, u′ is the fluid's velocity, ℑ′0 indicates the characteristic velocity, σ is the electrical conductance, κ1 is the porosity of medium, κ0 is the parameter associated with Walters' B fluid. Moreover, ρ and g are standard notations presenting density and gravitational pull respectively. The mathematical presentation of connected initial conditions and ramped surface conditions is
Under Rosseland approximation [51], the total thermal radiation flux is approximated as
The above expression is nonlinear in terms of temperature function (Θ′4). This term is expanded around Θ′∞ through the Taylor series to obtain a linearized version of temperature function. Assuming sufficiently small temperature differences yields
Providing Eqs (2.8) and (2.9) in Eq (2.2) yields
To make the problem independent of geometry and to reduce the number of parameters, the following unit-free quantities are employed
into Eqs (2.1), (2.3)–(2.7), and (2.10). This substitution gives the following modified model
where a1=1+WfK, a2=M+1K, and κ3=κeμρℑ′0 are constants, K=κ1ℑ′20ν2φ specifies the permeability parameter, CR=νR′ℑ′20 is the parameter of chemical reaction, Q=μQ1ρ2ℑ′20Cp accounts for the heat injected or absorbed, M=σM20νρℑ′20 denotes the magnetic strength, Gr1=gΔΘ′νβ1ℑ′30 is the thermal Grashof number, Sc=νD is the Schmidt number, Pr=μCpκ2 represents the Prandtl number, Gr2=gΔΦ′νβ1ℑ′30 is the mass Grashof number, R=16εrΘ′3∞3κ2κr characterizes the radiation effects, Es=νExεκ2eψwρℑ′30 deals with electro-osmosis effects, and Wf=ℑ′20k0ν2ρ is the term associated with Walters' B fluid.
3.
Generalization of model and computation of exact solutions
3.1. Fractional-order equations
The obtained unit-free model is transmuted to fractional form by substituting the integer-order time derivative (∂∂t) with Atangana-Baleanu fractional derivative (ABDψt). This substitution gives the following version of Eqs (2.12)–(2.14)
For any fractional order ψ and an arbitrary function F(t), expressions for Atangana-Baleanu derivative and its Laplace transform (LT) are presented as [45]
where generalized Mittag-Leffler function is presented as
3.2. Solutions of fractional equations
Applying the LT on Eq (3.2) and solving as
The general solution of Eq (3.4) is determined by employing the method of undetermined coefficients as
The Laplace transformed boundary conditions are used to find the following values of involved constants C1 and C2
Providing the values of C1 and C2 in Eq (3.5), we acquire
Similar steps are adapted to construct a solution of the concentration field (Eq (3.3)) however, avoiding the writing repetition, the final form of general concentration solution is presented as
where
Now incorporating the LT into Eq (3.1) gives us the following velocity equation
Plugging Eqs (3.6) and (3.7) in Eq (3.8) and solving the consequent equation, the following general velocity expression is evaluated
Determining the constants D1 and D2 through related boundary conditions and substituting them in Eq (3.9) yields
where
Equation (3.10) can be simplified as
where
The more adequate form of Eqs (3.6), (3.7), and (3.11) for Laplace inversion is stated as
Equations (3.12), (3.13), and (3.14) are transmuted back in primary coordinates (ζ,t) by employing the Laplace inversion technique. The real-time solutions are furnished as
where * denotes the convolution product and
3.3. Some significant quantities
Proceeding for the effective measurement of shear stress and rates of mass and heat transmission, the coefficient of skin friction (Cf), Sherwood number (Sh), and Nusselt number (Nu) are described as
where mass flux h∗ and heat flux q∗ are expressed as
Providing relevant values from Eq (2.11) and substituting Eq (3.19), Eq (3.18) becomes
4.
Results and discussion
In this investigation, the concept of time-fractional Atangana-Baleanu derivative is applied to achieve the purpose of improved thermal efficiency of the electro-osmotic flow of Walters' B fluid over a permeable upright wall that expresses a ramped motion. This study also encounters thermal radiation, MHD, and heat injection/absorption phenomena. Besides this, the mass diffusion mechanism is also explored under first-order chemical reaction. After reviewing the literature, it is important to mention that ramped velocity, concentration, and energy boundary conditions are first time simultaneously employed for a non-Newtonian fluid. Operating these non-uniform conditions at the same time produces multiple intricate functions in terms of Laplace frequency, which sometimes restricts the execution of analytic Laplace inversion. However, in this work, exact real domain versions of all the primary functions (velocity, concentration, and temperature) are procured in integral form employing the analytic Laplace reversal technique. In this section, results of numerical simulations are provided through graphical illustrations and tabular arrangements to deeply probe the computational and physical attributes of the under observation problem. Some physical arguments are discussed to justify the portrayed fluctuations in behaviors of velocity, concentration, and temperature distributions subject to changes in correlated parameters. Since the levied boundary conditions are piece-wise functions of time, therefore, all the tables and plots are constructed for a wide range of time values. In the end, the prominent contribution of fractional and other associated parameters in determining the shear stress and mass and heat transfer rates is anatomized by precisely computing the velocity, concentration, and energy gradients at the bounding wall.
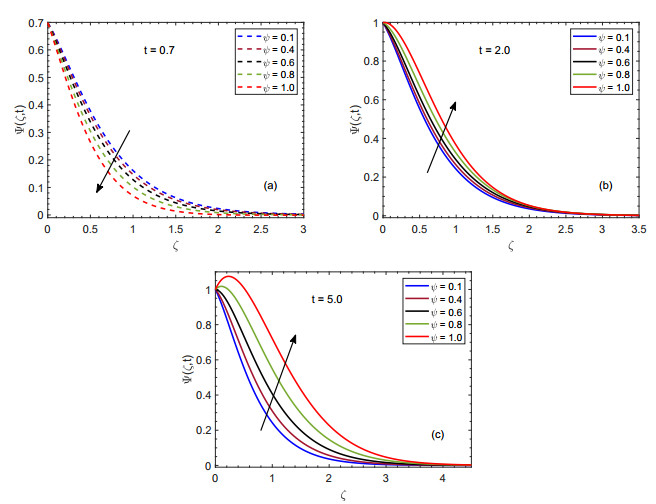
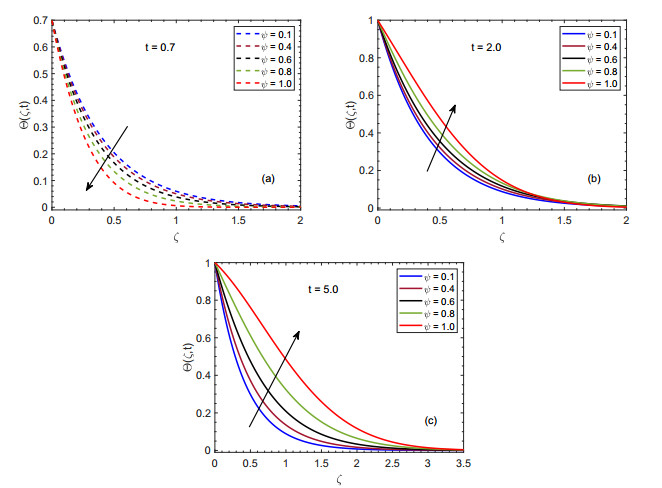
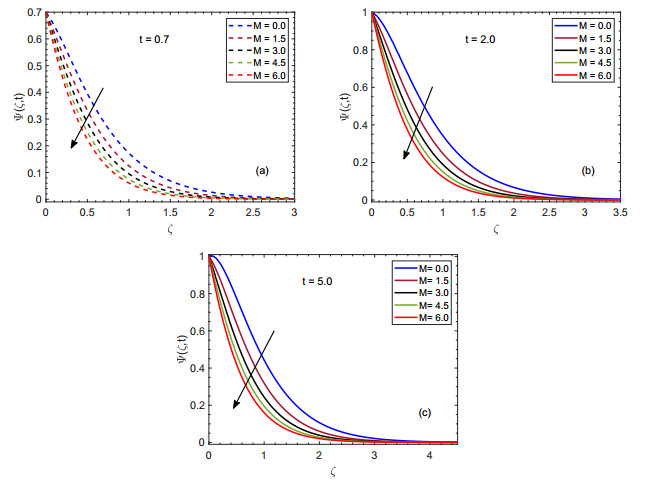
Figures 2-4 respectively display the velocity, concentration, and energy solution profiles for fractional and classical models. These profiles are graphed and discussed for three values of the time. The first value of time is selected as t=0.7, which highlights the behavior of functions for the time-dependent part of the corresponding boundary condition i.e., t<1. The other two values of time are t=2.0 and t=5.0. These values account for that time portion of the boundary conditions for which the functions are independent of time and have constant value 1 i.e., t>1. It is evident from Figures 2-4 that fractional parameter ψ declines the profiles of all three functions for t<1 whereas, for t>1, this trend reverses completely and functions exhibit increasing profiles. Moreover, it is witnessed that for smaller values of time, the fundamental functions involved in this study are maximum for the fractional model whereas, for t>1, the classical model provides the highest profiles of these functions. The primary reason behind the aforementioned observations is that ψ exerts a dual nature influence on boundary layer thicknesses. For ramped condition, it attenuates the momentum boundary layer but for uniform surface condition, it causes to expand the thickness of the momentum boundary layer. This interpretation is also true for the expansion and attenuation of thermal and concentration boundary layers. These explanations certify that the graphical illustrations are in accordance with physical behaviors. The changes produced in the flow pattern due to the presence of magnetic lines of action are portrayed in Figure 5. Unlike ψ, the parameter M has uniform effects for all three values of t. It is recognized that the imposition of a magnetic field causes to slow down the flow of Walters' B fluid. The physical argument for this retardation is the generation of a highly effective viscous force, which drags the fluid in an anti-flow direction. This force is commonly termed as Lorentz force. The Lorentz force enables the viscous forces to eliminate the impacts of flow supportive forces, which leads to contract the momentum boundary layer thickness. In consequence, the curve associated with the velocity function continuously declines for enhancing domain of M.
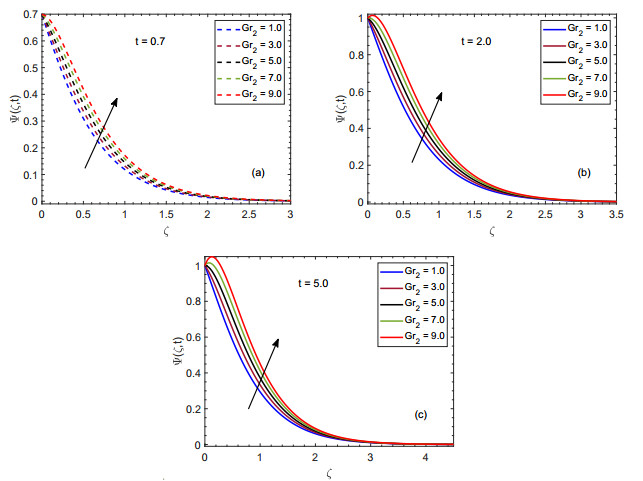
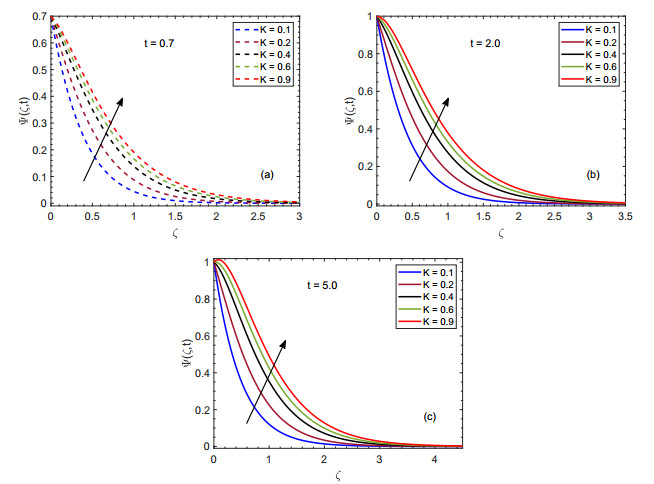
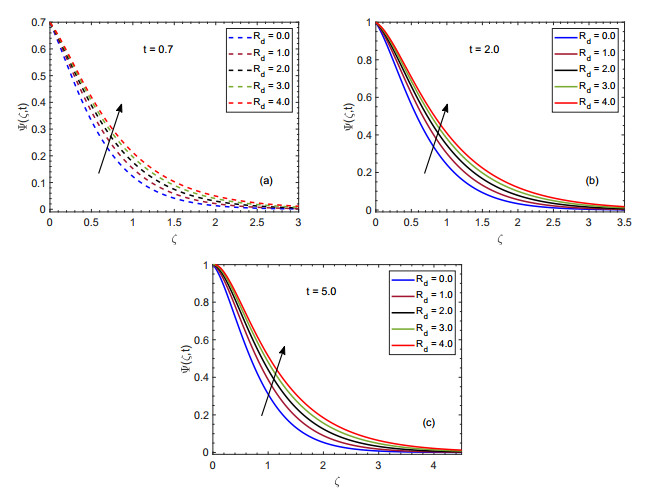
The response of the velocity function for alteration in Gr1 and Gr2 is reported in Figures 6 and 7, respectively. It is deduced that both parameters have an identical influence on the flow pattern. The Walters' B fluid gets accelerated due to a rise in the magnitudes of Gr1 and Gr2. In the physical sense, Gr1 and Gr2 share an inverse correspondence with viscous effects and direct relation with buoyancy forces. In an alternative way, the Grashof number is a means of quantifying the two opposite forces. The buoyancy forces are credited as flow accelerated forces, which depend upon the temperature and concentration gradients. The rising values of Gr1 and Gr2 signify these gradients, and consequently, the buoyancy forces vanquish the control of viscous effects on the velocity function. These phenomena expand the thickness of corresponding boundary layers, and accelerated flow patterns are witnessed. Figure 8 features the velocity function to inspect the influence of the material parameter of Walters' B fluid Wf on the flow profile. The parameter Wf characterizes the elastic and viscous features of the material, and it is one of the key factors for the intensified viscoelastic nature of the Walters' B fluid. Hence, the rising magnitude of Wf increases the viscoelasticity of the fluid, which diminishes the flow speed and brings down the corresponding curve. The respective figure describes another stimulating result that the flow of the Newtonian fluid (Wf=0) is more expeditious as equated to the flow of Walters' B fluid (Wf≠0). The specific factor responsible for this outcome is the lower viscosity of a Newtonian fluid. With the aid of Figure 9, ramifications of nesting the upright ramped wall in a porous medium are discussed for the flow phenomenon. This figure indicates the existence of direct proportionality between fluid velocity and permeability parameter K. It means that the speed of the flow enhances for growing inputs of K, and there is a continuous rise in the associated curve. An enlargement in diameter of the permeable holes of the medium provides the physical supportive ground for this rise in the velocity. Additionally, one of the reasons for speedy flow is that more amount of fluid can penetrate through the porous medium due to the increased size of the holes. The observed outcome is also justified by the fact that the fluid confronts weak resistance type forces because of the greater volume capacity of the medium. The modulating consequences of Rd on flow patterns are analyzed through Figure 10. The anticipation of heat radiative gradient by means of Rosseland approximation communicates that the extended domain of Rd restricts the considered fluid to discharge the energy. Subsequently, the fluid particles possess high energy due to a greater amount of heat transmitted at the solid-fluid interface. The augmented energy level excites the particles, due to which they start performing rapid collisions. As a consequence of such collisions, cohesive forces become ineffective because of the breakage of inter-particle bonds. The existence of this mechanism implies that the resistive potential of fluid particles is weak. Hence, the fluid performs a high-speed motion for amplified inputs of Rd.
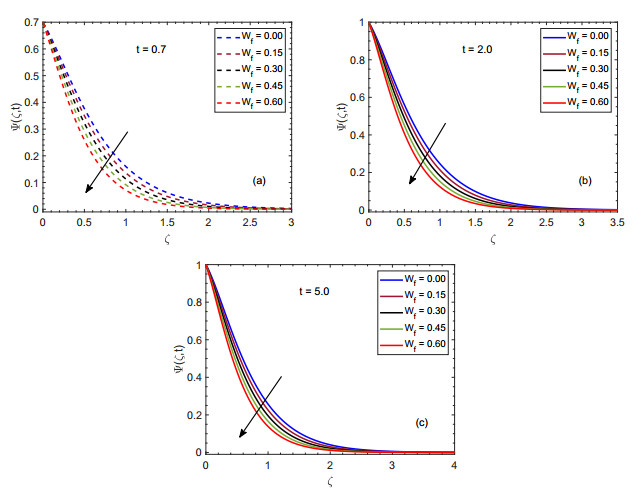
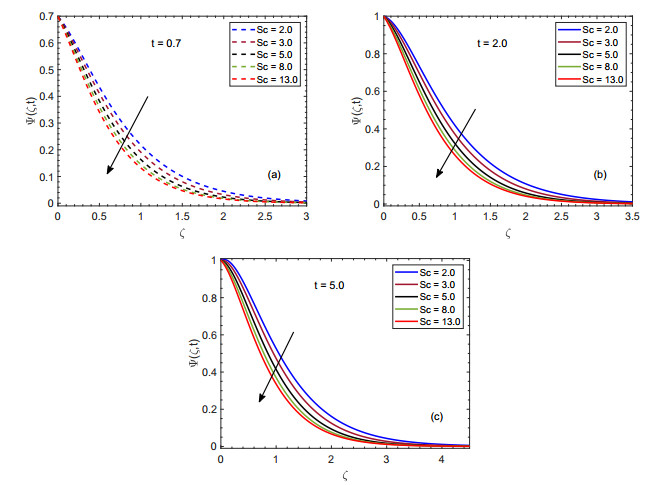
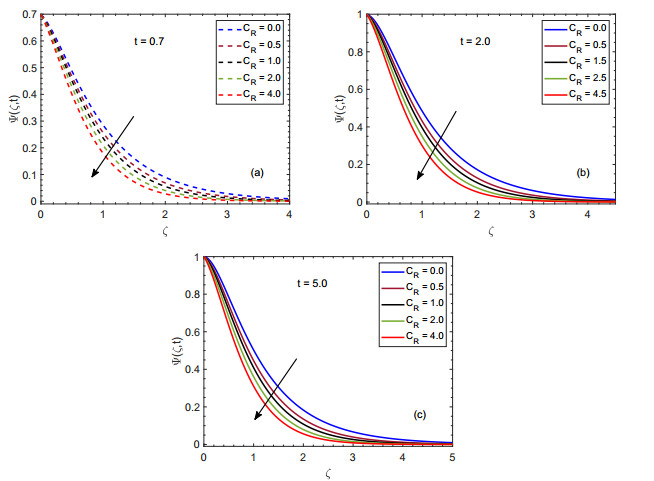
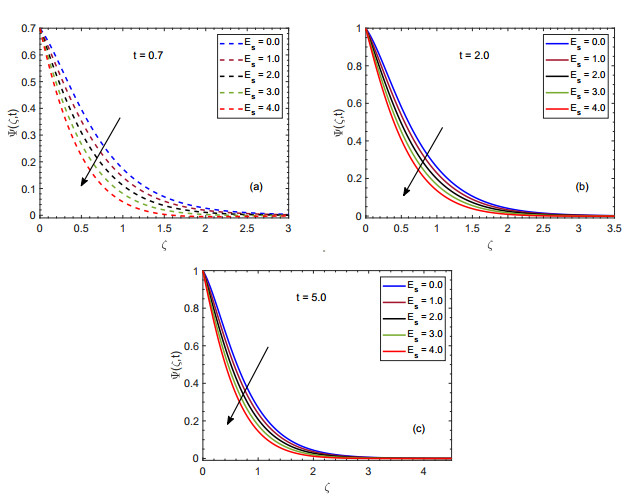
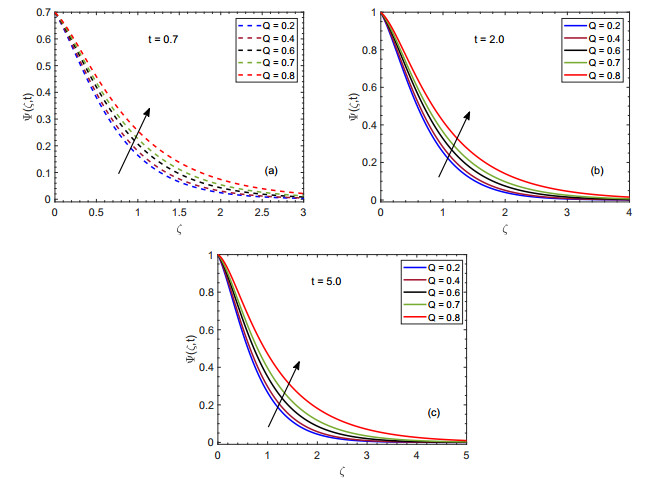
Figures 11 and 12 are drawn to feature the influences of Sc and CR on the flow profile. These parameters appear in the velocity term because of the partial coupling of the flow and concentration equations. These figures describe that both the considered parameters have similar impacts on fluid velocity. Particularly these parameters lead to slow down the flow speed. Since Sc and viscous forces are directly linked to each other, therefore the deceleration in the flow is pretty obvious for this parameter because it signifies the viscous strength of the fluid and dwindles the mass diffusion. Identically, raising the value of the parameter CR associated with corrosive chemical reaction minimizes the species concentration, which results in weaker solutal buoyancy forces. In this way, the velocity of the fluid is suppressed and a decaying profile is observed from the relevant figure. Figure 13 is a graphical demonstration of the contribution of Pr in setting the fluid flow. The thicknesses of momentum and thermal boundary layer are significantly influenced by Pr and also it is considered one of the vital parameters to examine the heat transfer problems. Physically, it inculcates the relative effects of viscosity and fluid's heat conduction capacity. From Figure 13, a decelerating flow field is witnessed for a rising variation of Pr. This is because the conduction capacity of fluids with large Pr is dominated by those having small Pr values. Moreover, the larger Pr values have the tendency to augment the momentum diffusivity, which enhances the thickness of fluids and make them more viscous. Taking everything into account, it can be stated that viscous influence permits the fluids to exhibit a controlled flow with restraint velocity. Hence, we perceive a dropping velocity profile in response to Pr maximization. The changes produced in the flow field for modification of Es are recorded in Figure 14. It is discovered that the application of electro-osmotic force on Walters' B fluid provokes decelerating effects on the flow. From a physical view, the electric double layer attains more charges in consequence of the enhanced strength of the imposed electric field. Because of this charge intensification, the fluid gathers dominant resistive forces that restrict the fluid velocity. This control of electro-osmosis on the liquid flow has gathered vital applications in sub-branches of medical sciences, biochemical, and engineering. For example, separated fluids through electro-osmosis are effective as drug carriers, electronic controlling of liquid motions, minimizing the deterioration and dampness of very thick wall structures [52], and materials processing [53]. The consequences of including a heat injector in the considered model on the velocity function are evaluated in Figure 15. This evaluation is conducted by choosing diverse values for the injection parameter Q, while magnitudes of other parameters are considered fixed. It is found that the velocity function escalates for growing values of Q. Since the energy of fluid particles is augmented for the boosted rate of heat injection at the solid-fluid interface, therefore the temperature of the particles rises. Following this reason, the forces functioning against the separation of fluid particles from each other become inefficient. Accordingly, the fluid describes a swift flow velocity.
The computations for Nu and Sh are arranged in Tables 1 and 2 to perform a detailed numerical study about rates of heat transfer and mass transfer respectively. To clearly observe the impacts of imposed boundary conditions and fractional parameter ψ, these tables showcase the results for a wide range of values of time t and ψ. The reported results show that ψ and t offer dual nature contribution to the aforementioned processes. Precisely stating, subject to ramped conditions (t<1), augmented values of ψ result in enhancement of Nu and Sh, and maximum values are obtained for integer-order expressions. Whereas, for isothermal conditions (t>1), completely opposite trends are witnessed, which indicate that Nu and Sh decline for rising values of ψ. In this case, integer-order expressions produce minimum values. In a likewise manner, time advancement up to t<1 augments the transfer rates, and after that, they keep reducing for t>1. These results suggest that the combination of isothermal conditions with a fractional model is more efficient to attain the improved transfer rates as equated to classical model and ramped conditions. Table 3 is bestowed to perceive and interpret the variations in Sh and Nu for the physical and thermal parameters. It is noticed that the parameters CR and Sc act in a similar fashion for Sh and diminish the mass transfer rate. Nusselt number follows inverse trends for extending domains of Rd and Pr. Extensively, Nu is a decelerating function of Rd and an escalating function of Pr. Besides this, the induction of a heat injector depreciates the Nu, whereas the existence of a heat absorbent helps to transfer the heat more swiftly. The numerical outcomes for Cf are enumerated in Tables 4 and 5 for extensive inspection of the shear stress. Shear stress is a key feature for several practical problems, and usually, boosted shear stress is seen as a leading deficiency for technical analyses. To determine an adequate solution of this drawback for the considered problem, the behavior of shear stress for several pertinent parameters is discussed with the help of Tables 4 and 5. Table 4 indicates that the use of ramped boundary condition is more suitable to control the shear stress. Moreover, it is recognized from Table 5 that dominant magnetic and electro-osmotic forces can suppress shear stress. Simultaneously, a favorable choice of the parameters Gr1, Gr2, and K is another effective method to serve the desired cause. Finally, it is viewed that the response of Cf under variation of ψ and t is exactly opposite to the responses of Nu and Sh. In the end, some key advantages of conducting this analysis are briefly elaborated. For instance, the current work is purely based on exact solutions and they can be utilized for verification of the several numerical techniques. Moreover, the considered problem is modeled in terms of fractional derivative, which leads to providing more general results. A suitable adjustment of the fractional parameter not only yields a perfect agreement between experimental data and theoretical outcomes but also the results for classical models can be evaluated. Several existing results can be traced through a simple modification of some parameters. Furthermore, simultaneous application of piece-wise time-dependent boundary conditions to capture flow, mass, and heat transfer dynamics through Atangana-Baleanu fractional derivative is not yet investigated. So, in this context, this study contributes to the literature extensively. However, it is also significant to discuss some limitations of this study, which will help readers to easily understand this work. Based on these limitations, this work can be modified and extended in the future. The limitations and assumptions of this work are as follows:
● The flow is incompressible, laminar, and one-dimensional.
● A linear chemical reaction is considered for the mass equation.
● Induced magnetic effects are ignored. Moreover, the influence of thermal radiation and the magnetic field is assumed negligible along the vertical axis.
● Impacts of viscous dissipation are considered insignificant for the energy equation.
5.
Summary and conclusions
The core motive of this research piece is to examine the influence of electro-osmotic forces on MHD fractional flow of a Walters' B fluid close to a vertical wall nested in a porous material. Besides this, mass transfer rate and radiative thermal performance are analyzed subject to ramped concentration and energy of the bounding wall. The impacts of first-order chemical reaction and heat injection/absorption are also evaluated in this work. A time-controlled velocity condition is imposed, which leads to develop the flow of the considered fluid. The unit-less conventional equations of the considered problem are generalized to the fractional form operating the non-singular and non-local kernel-based Atangana-Baleanu derivative. The consequent equations are solved analytically, and exact solutions are determined by exercising the Laplace transform technique. To analyze the features of influential parameters, effects of generalizing the model, and advantages of using the ramped surface conditions, a comprehensive tabular and graphical study is performed. The extracted key results of the current work are summarized as
● The velocity of flow drops due to extension in the domain of the parameter Es. This behavior of the velocity profile has exceptional applications in minimizing the deterioration and dampness of wall structures [52]. At the atomic level, the electro-osmosis phenomenon is also used in fluids' separation processes, which can be efficiently utilized as medication dischargers [54].
● Heat and mass transfer rates reveal interesting features for variation in ψ and t. More specifically, for t<1, an escalation in ψ and t enhances the observed rates. Later, for t>1, mass and heat transfer rates act in a reverse fashion and show decreasing behavior for an upsurge in t and ψ.
● Application of the ramping strategy on the bounding wall is more efficient when augmentation of the heat transfer rate and reduction of shear stress are the primary goals.
● The fractional parameter ψ specifies a dual role for ramped and isothermal conditions. Precisely, for t<1, it declines the velocity, concentration, and energy profiles, but for t>1, these quantities keep increasing as a result of a rise in ψ.
● For t<1, the fundamental functions involved in this study are maximum for the fractional model, whereas, for t>1, the classical model provides the highest profiles of these functions.
● Electro-osmotic and magnetic effects cause to reduce the shear stress at the bounding wall.
● The comparative analysis of solutions for isothermal and ramped conditions indicate that curves representing concentration, velocity, and energy functions are higher for isothermal conditions.
● Time-controlled boundary conditions possess vital significance in order to gain the swiftest cooling rate and improved flow control.
Acknowledgments
The authors acknowledge the financial support provided by the Center of Excellence in Theoretical and Computational Science (TaCS-CoE), KMUTT. Moreover, this research project is supported by Thailand Science Research and Innovation (TSRI) Basic Research Fund: Fiscal year 2021 under project number 64A306000005. The first and third authors appreciate the support provided by Petchra Pra Jom Klao Ph.D. Research Scholarship (Grant No. 25/2563 and 14/2562).
Conflict of interest
The authors declare no conflict of interest.
Appendix
The velocity vector for the problem under observation is described as
The momentum equation under Boussinesq's approximation [55] is developed as [56,57]
where →J×→M is the product of current density and net magnetic field, →Ex deals with the externally imposed electric field, β1 and β2 are the thermal and mass expansion coefficients respectively, →R shows the force of porous medium's resistance, and ρe specifies the total electric charge density. The terms ρe and T are described as [57]
Here, the kinematic tensors B1 and B2 are defined as [58]
Some of the terms in flow equation have the following form









 DownLoad:
DownLoad: