A modified iteration for total asymptotically nonexpansive mappings in Hadamard spaces

1.   Introduction

Let $\mathcal{C}$ be a nonempty closed subset of a CAT(0) space, $\mathcal{M}$ and $\mathcal{T}$ be a self map defined on $\mathcal{C}$. Then $\mathcal{T}$ is said to be:

$(i)$ nonexpansive if $d(\mathcal{T}u, \mathcal{T}v)\leq d(u, v), \; \forall u, v \in \mathcal{C}$;

$(ii)$ asymptotically nonexpansive if there exists a sequence $\{\zeta_n\}$ in $[1, \infty)$ with $\lim_{n \rightarrow \infty} \zeta_n = 1$ such that $d(\mathcal{T}^{n}u, \mathcal{T}^{n}v)\leq \zeta_nd(u, v) \; \forall u, v \in \mathcal{C}$ and $\forall n \geq 1$;

$(iii)$ uniformly $\mathcal{L}$-Lipschitzian if there exists a constant $\mathcal{L} > 0$ such that $d(\mathcal{T}^{n}u, \mathcal{T}^{n}v) \leq \mathcal{L} d(u, v) \; \forall u, v \in \mathcal{C}$ and $\forall n \geq 1$.

In 2006, Alber et al. ^[3] introduced a new generalized mapping named as total asymptotically nonexpansive mapping, defined as follows:

Definition 1.1. A self mapping $\mathcal{T}$ on $\mathcal{C}$ is called $(\{\vartheta_n\}, \{\kappa_n\}, \varphi)$ total asymptotically nonexpansive mapping if there exist nonnegative real sequences $\{\vartheta_n\}$ and $\{\kappa_n\}$ with $\vartheta_n \rightarrow 0$, $\kappa_n \rightarrow 0$ as $n \rightarrow \infty$ and a continuous strictly increasing function $\varphi : [0, \infty)\rightarrow [0, \infty)$ with $\varphi(0) = 0$ such that

$\forall u, v \in \mathcal{C}$ and $n\geq 1$.

They showed that this mapping generalizes several classes of mappings which are extensions of asymptotically nonexpansive mappings and also approximated fixed points of the above mapping by using modified Mann iteration process.

It can be directly seen by above definitions that, asymptotically nonexpansive mappings contain nonexpansive mappings with $\{\zeta_n = 1\}$, $\forall n \geq 1$ and total asymptotically nonexpansive mappings contain asymptotically nonexpansive mappings with $\{\vartheta_n = \zeta_n -1\}$, $\{\kappa_n = 0\}$, $\forall n \geq 1$ and $\varphi(t) = t$, $t \geq 0$. Furthermore, every asymptotically nonexpansive mapping is a uniformly $\mathcal{L}$-Lipschitzian mapping with $\mathcal{L} = \sup_{n\in N}\{\zeta_n\}$.

A mapping $\mathcal{T}$ is said to have a fixed point $\rho$ if $\mathcal{T}\rho = \rho$ and a sequence $\{u_n\}$ is said to be asymptotic fixed point sequence if $\lim_{n \rightarrow \infty} d(u_n, \mathcal{T}u_n) = 0.$

In the background of iteration processes, Mann ^[20], Ishikawa ^[10] and Halpern ^[8] are the three basic iterations utilized to approximate the fixed points of nonexpansive mapping.

After these three basic iterative schemes, several researchers came up with the idea of generalized iterative schemes to approximate the fixed points of nonlinear mappings. Here, we have few iterations among the number of new itarative schemes, Noor iteration ^[21], Agarwal et al. iteration (S-iteration) ^[2], Abbas and Nazir iteration ^[1], Thakur New iteration ^[28], Garodia and Uddin ^[12], Garodia et al.^[13] and so on.

In 2015, Cholamjiak ^[4] proposed a modified proximal point algorithm for solving minimization problems in CAT(0) spaces.

In the same year, Thakur et al. ^[28] presented modified Picard-Mann hybrid iteration process $\{u_n\}$ to approximate the fixed points of total asymptotically nonexpansive mappings in the framework of Hadamard spaces and the sequence $\{u_n\}$ is defined as follows:

$\forall n \geq 1$, where $\{\eta_n\}$ is an appropriate sequence in the interval $(0, 1)$. They also proved its convergence analysis under some certain conditions.

In 2017, Suparatulatorn et al. ^[26] proposed a modified proximal point algorithm using Halpern's iteration process for nonexpansive mappings in CAT(0) spaces and prove some convergence theorems. For more details see (^[9,14,15]) and refences therein.

Recently, Kuman et al. ^[18] presented modified Picard-S hybrid iteration process $\{u_n\}$ as follows:

$\forall n \geq 1$, where $\{\eta_n\}$ and $\{\varsigma_n\}$ are appropriate sequences in the interval $(0, 1)$ and they established some convergence theorems to approximate the fixed points of total asymptotically nonexpansive mapping in the setting CAT(0) spaces.

Motivated by above work, we introduce a new iterative scheme, which is defined as follows:

for all $n \geq 1$, where $\{\eta_n\}$ and $\{\varsigma_n\}$ are appropriate sequences in the interval $(0, 1)$. We prove some convergence theorems of the sequence generated by iterative scheme (1.3) to approximate the fixed point of total asymptotically nonexpansive mapping in Hadamard space. We also provide a numerical experiment to show the convergence rate of iterative scheme (1.3) and its fastness over the other existing iterative processes.

2.   Preliminaries

This section contains some well-known concepts and results which will be used frequently in the paper.

Lemma 2.1.(^[6]) Let $\mathcal{M}$ be a CAT(0) space, $x, y\in \mathcal{M}$ and $t\in[0, 1].$ Then

Let $\{u_n\}$ be a bounded sequence in $\mathcal{M}$, complete CAT(0) spaces. For $u\in \mathcal{M}$ set:

The asymptotic radius $r(\{u_n\})$ is given by

and the asymptotic center $\mathcal{A}(\{u_n\})$ of $\{u_n\}$ is defined as:

$\mathcal{A}(\{u_n\})$ consists of exactly one point in CAT(0) spaces see (^[5], Proposition 7).

A sequence $\{u_n\}$ in $\mathcal{M}$ is said to $\Delta$-converges to $u\in \mathcal{M}$ if $u$ is the unique asymptotic center for every subsequence $\{z_n\}$ of $\{u_n\}$. In this case we write $\Delta-\lim_{n} {u_n} = u$ and read as $u$ is the $\Delta-$limit of $\{u_n\}.$

Lemma 2.2.(^[7]) Let $\mathcal{M}$ be a complete CAT(0) space and $\{u_n\}$ be a bounded sequence in $\mathcal{M}$. If $\mathcal{A}(\{u_n\}) = \{\rho\}$, $\{z_n\}$ is a subsequence of $\{u_n\}$ such that $\mathcal{A}(\{z_n\}) = \{z\}$ and $d(u_n, z)$ converges, then $\rho = z$.

Recalling the existence theorem for the fixed point and demiclosedness principle for the mappings satisfy Definition 1.1 in CAT(0) spaces due to Karapinar et al. ^[11].

Lemma 2.3. (^[11]) Let a self map $\mathcal{T}$ defined on a convex closed nonempty and bounded set, $\mathcal{C}$ of $\mathcal{M}$, a complete CAT(0) space. Let $\mathcal{T}$ be uniformly continuous and total asymptotically nonexpansive mapping. Then, $\mathcal{T}$ has a fixed point, and set of fixed points $\mathcal{F}(\mathcal{T})$ is convex and closed.

Lemma 2.4. (^[11]) Let $\mathcal{T}$ be a self map defined on $\mathcal{C}$, a nonempty closed, convex subset of $\mathcal{M}$, a complete CAT(0) space. Let $\mathcal{T}$ be a uniformly continuous and total asymptotically nonexpansive mapping. For every bounded sequence $\{u_n\}\in \mathcal{C}$ such that, $\lim_{n\rightarrow\infty} d(u_n, \mathcal{T}u_n) = 0$ and $\lim_{n\rightarrow\infty} u_n = q$ implies that $\mathcal{T}q = q$.

The next lemma due to Schu ^[23] is useful in our subsequent discussion.

Lemma 2.5. (^[23]) Let $\mathcal{M}$ be a complete $CAT(0)$ space and let $u\in \mathcal{M}$. Suppose $\{t_n\}$ is a sequence in $[b, c]$ for some $b, c \in (0, 1)$ and $\{u_n\}$, $\{v_n\}$ are sequences in $\mathcal{M}$ such that $\limsup\limits_{n\to\infty} d(u_n, u)\leq r$, $\limsup\limits_{n\to\infty} d(v_n, u) \leq r$, and $\lim\limits_{n\to\infty} d((1- t_n)u_n \oplus t_n v_n, u) = r$ for some $r\geq 0$. Then

Lemma 2.6.(^[22]) Let $\{\alpha_n\}$, $\{\beta_n\}$ and $\{\xi_n\}$ be the sequences of nonnegative numbers such that

for all $n\geq 1$. If $\sum_{n = 1}^{\infty}\beta_n < \infty$ and $\sum_{n = 1}^{\infty}\xi_n < \infty$, then $\lim\limits_{n\to\infty}\alpha_n$ exists. Whenever, if there exists a subsequence $\{\alpha_{n_{k}}\}\subseteq \{\alpha_n\}$ such that $\alpha_{n_{k}}\rightarrow 0$ as $k \rightarrow \infty$, then $\lim\limits_{n\to\infty}\alpha_n = 0.$

3.   Main results

Theorem 3.1. Let $\mathcal{C}$ be a closed bounded and convex subset of $\mathcal{M}$, a complete CAT(0) space and a self map $\mathcal{T}$ defined on $\mathcal{C}$ is uniformly $\mathcal{L}$-Lipschitzian and $(\{\vartheta_n\}, \{\kappa_n\}, \varphi)$-total asymptotically nonexpansive mapping. Assume that the following conditions hold:

$(a) \; \sum_{n = 1}^{\infty}\vartheta_n < \infty \text{and} \sum_{n = 1}^{\infty}\kappa_n < \infty$;

$(b)$ there exist constants $m$, $n$ with $0 < m\leq\eta_n\leq n < 1$ for each $n\in N$;

$(c)$ there exist constants $p$, $q$ with $0 < p\leq\varsigma_n\leq q < 1$ for each $n\in N$;

$(d)$ there exist a constant $M_1$ such that $\varphi(\omega)\leq M_1\omega$ for each $\omega\geq0$.

Then the sequence $\{u_n\}$ defined by (1.3) $\triangle$-converges to a point of $\mathcal{F}(\mathcal{T})$.

Proof. By using Lemma 2.5, we have $\mathcal{F}(\mathcal{T})\neq\emptyset$. We start with proving that $\lim_{n\rightarrow \infty}d(u_n, \rho)$ exists for any $\rho\in \mathcal{F}(\mathcal{T})$, where $\{u_n\}$ is defined by (1.3).

Let $\rho\in \mathcal{F}(\mathcal{T})$. Then we have

for each $n\in N$. Also we have

for each $n\in N$. From (1.3), (3.1) and (3.2), we get

where

By assumption $(a)$, we have

By assertion (3.3), (3.4) and Lemma 2.8, we obtain $\lim_{n\rightarrow \infty}d(u_n, \rho)$ exists.

Next, we prove that $\lim_{n\rightarrow \infty}d(u_n, \mathcal{T}u_n) = 0$.

Suppose

From (3.1), we have

Since $\mathcal{T}$ satisfies Definition 1.1

From (3.6) and (3.7), we have

In the same way, we get

Since

By taking limit infimum both sides, we obtain,

From (3.6) and (3.10), we obtain

By using (3.5) and (3.9), we have

Applying (3.12) and (3.13), we have,

By using (3.5), (3.9), (3.14) and Lemma 2.5, we can conclude that

We also have,

By taking limit infimum both sides, we obtain

By (3.2), we have

By taking limit suprimum both sides, we obtain

By using (3.16) and (3.17), we get

Also we have

By using (3.8), (3.11), (3.20) and Lemma 2.5, we can conclude that

Since $\mathcal{T}$ is $(\{\vartheta_n\}, \{\kappa_n\}, \varphi)$-total asymptotically nonexpansive mapping.

By taking limit ${n\rightarrow \infty}$ and using (3.15), we get

We have

By taking limit as ${n\rightarrow \infty}$ and using (3.21), we obtain

From (3.15), (3.22) and (3.23), we get

Since $\mathcal{T}$ satisfies Definition 1.1 and uniformly $\mathcal{L}$-Lipshitzian, we obtain

Let $x \in W_\Delta(u_n)$. Then, there exists a subsequence $\{z_n\}$ of $\{u_n\}$ such that $\mathcal{A}(\{z_n\}) = \{x\}$. By using Lemma 2.3, there exists a subsequence $\{y_n\}$ of $\{z_n\}$ such that $\{y_n\} \; \Delta$-converges to $y\in \mathcal{C}$. By Lemma 2.4, $y\in \mathcal{F}(\mathcal{T})$. Since $\{d(z_n, y)\}$ converges, by Lemma 2.2, x = y. This implies that $W_\Delta(u_n)\subseteq \mathcal{F}(\mathcal{T})$.

Next we will prove that $W_\Delta(u_n)$ consists of exactly one point. Let $\{z_n\}$ be a subsequence of $\{u_n\}$ with $\mathcal{A}(\{z_n\}) = \{x\}$ and $\mathcal{A}(\{u_n\}) = \{u\}$. We have seen that $x = y$ and $y\in \mathcal{F}(\mathcal{T})$. Finally, since $\{d(u_n, y)\}$ converges, by Lemma 2.2, we have $u = y\in \mathcal{F}(\mathcal{T})$. This shows that $W_\Delta(u_n) = \{u\}$.

Theorem 3.2. Let $\mathcal{M}$, $\mathcal{T}$, $\mathcal{C}$, $(a)$, $(b)$, $(c)$, $(d)$, $\{\eta_n\}$, $\{\varsigma_n\}$ same as in Theorem 3.1. Then, the sequence $\{u_n\}$, defined by $(1.3)$ strongly converges to a fixed point of $\mathcal{T}$ iff

where $d(x, \mathcal{F}(\mathcal{T})) = \inf\{d(x, \rho) : \rho \in \mathcal{F}(\mathcal{T})\}$.

Senter and Dotson ^[24] introduced a mapping satisying condition (Ⅰ) as follows:

A mapping $\mathcal{T}$ defined on $\mathcal{C}$ is said to satisfy the Condition (Ⅰ) (^[24]) if there exists a nondecreasing function $f:[0, \infty) \rightarrow [0, \infty)$ with $f(0) = 0$ and $f(\omega) > 0$ for all $\omega\in (0, \infty)$ such that $\|u - \mathcal{T}u\| \geq f(d(u, \mathcal{F}(\mathcal{T})))$ for all $u \in \mathcal{C},$ where $d(u, \mathcal{F}(\mathcal{T})) = \text{inf}\{\|u - \rho\| : \rho \in \mathcal{F}(\mathcal{T})\}.$

By using the similar technique as in the proof of Theorem 3.3 by Thakur et. al ^[28], we get the following result:

Theorem 3.3. Let $\mathcal{M}$, $\mathcal{T}$, $\mathcal{C}$, $(a)$, $(b)$, $(c$), $(d)$, $\{\eta_n\}$, $\{\varsigma_n\}$ be same as in Theorem 3.1 with $\mathcal{T}$ satisfies Condition (I). Then, $\{u_n\}$, defined by $(1.3)$ converges to a point of $\mathcal{F}(\mathcal{T})$.

Recalling the definition of semi-compact mapping;

A map $\mathcal{T}$ defined on $\mathcal{C}$ is said to be semi-compact ^[27] if for a sequence $\{u_n\}$ in $\mathcal{C}$ with $\lim\limits_{n\to\infty}d(u_n, \mathcal{T}u_n) = 0$, there exists a subsequence $\{u_{n_{j}}\}$ of $\{u_n\}$ such that $u_{n_{j}}\rightarrow \rho\in \mathcal{C}.$

By using the same steps used by Karapinar et al. ^[11] in the proof of Theorem 22, we get the next result.

Theorem 3.4. Let $\mathcal{M}$, $\mathcal{T}$, $\mathcal{C}$, $(a)$, $(b)$, $(c)$, $(d)$, $\{\eta_n\}$, $\{\varsigma_n\}$ be same as in Theorem 3.1. Let $\mathcal{T}$ be semi-compact. Then the sequence $\{u_n\}$ defined by $(1.3)$ converges to a point of $\mathcal{F}(\mathcal{T})$.

4.   Numerical example

Example 4.1. Let $\mathcal{M} = \mathbb{R}$ with usual metric and $\mathcal{C} = [1, 10]$. Let a self map $\mathcal{T}$ on $\mathcal{C}$ as follows:

for all $u \in \mathcal{C}$.

It can be clearly seen that $\mathcal{T}$ is a continuous uniformly $\mathcal{L}$-Lipschitzian mapping with $\mathcal{F}(\mathcal{T}) = \{2\}$. Next, we will show that $\mathcal{T}$ satisfies Definition 1.1 on [1, 10].

We notice that the function $g(u) = \sqrt[3]{(u^{2}+4)}-u, \; \; \forall u\in [1, 10]$ has the derivative

for all $u \in [1, 10]$. Since $u\geq 1$, we have $g'(u) = \frac{1}{3}\Big(\frac{1}{(u^{2}+4)^{2/3}}\Big)(2u)\leq 1$ and hence

for all $u\in [1, 10]$ which shows that the above function is decreasing on $[1, 10]$. Let $u, v \in [1, 10]$ with $u\leq v$ shows that

we get

Hence, we get

This shows that $\mathcal{T}$ satisfies Definition 1.1 as it is nonexpansive mapping.

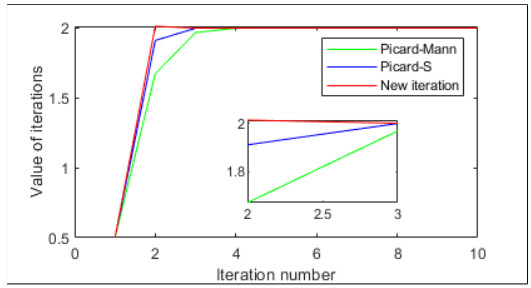
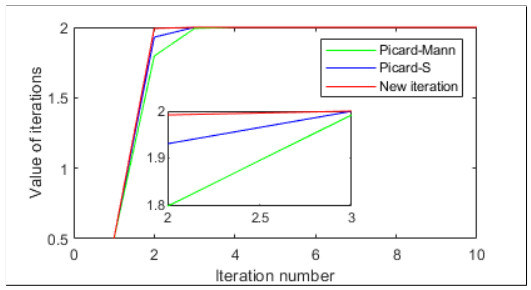
By using the initial value $u_1 = 0.5$ and setting the stopping criteria $\|u_n-2\|\leq 10^{-15}$, reckoning the iterative values of (1.1), (1.2) and (1.3) for two choices, Choice 1:$\; \; \; \eta_n = 1-\frac{n}{\sqrt[2]{n^{2}+1}}, \varsigma_n = \frac{n}{n+1}$ and Choice 2:$\; \; \; \; \eta_n = 1-\frac{n}{3n+1}, \varsigma_n = \frac{n}{16n+1}$, as shown in Tables 1 and 2 respectively.

Figures 1 and 2 clearly shows the fastness of sequence (1.3) over the other existing iterative schemes with different control conditions.

5.   Conclusions

In this article, we have presented a new type of iteration procedure for total asymptotically nonexpansive mapping under some new conditions in CAT(0) spaces. We showed that our new type of iteration are more efficient than some of the existing iteration. Also, we have provided the reader with a numerical experiment to support our claim.

Acknowledgements

We wish to pay our sincere thanks to learned referees for pointing out many omission and motivating us to study deeply. The second author is also grateful to University Grants Commission, India for providing financial assistance in the form of Junior Research Fellowship. The authors N. Mlaiki and T. Abdeljawad would to thank Prince Sultan University for funding this work through research group Nonlinear Analysis Methods in Applied Mathematics (NAMAM) Group No. RG-DES-2017-01-17.

Conflict of interest

The authors declare that they have no competing interests.