Some curve pairs according to types of Bishop frame

1.   Introduction

There are lots of interesting problems in the theory of curves at differential geometry. One of these problems determines the characterization of a regular curve. Numerous studies of curves are carried out in $3-$dimensional Euclidean space. Two curves which have some special properties at their corresponding points are called associated curves or curve pairs. Hence, curve pairs are attracted the attention of many researchers ^[1,2,3,4]. The most famous curve pairs are Bertrand partner curves, involute-evolute curves and Mannheim partner curves. The Bertrand curves were firstly described by Bertrand Russell in 1850. He studied curves whose principal normals are the principal normals of another curve ^[5]. Involute-evolute curves were discovered by C. Huggens while trying to build a more accurate clock ^[6]. Later, the relations Frenet apparatus of involute-evolute curve couple were given in ^[7] in the Euclidean $3-$space. Turgut and Erdogan examined involute-evolute curve couple in $n-$dimensional Euclidean space ^[8]. The other famous curve pairs are the Mannheim partner curves. These curves are defined by Mannheim with the equality $\kappa ^{2}+\tau ^{2} = w^{2} =$constant. Another characterization can be made as two curves $\alpha$ and $\gamma$ in $\mathbb{E}^{3}$ which are called Manneim partner curves if the principal normal vector fields of $\alpha$ coincide with the binormal vector fields of $\gamma$ at the corresponding points of curves ^[9,10,11].

On the other hand Bishop frame was introduced by L.R. Bishop in 1975 by means of parallel vector fields ^[12]. Recently, this frame is attracted the attention of many researchers. For example, in ^[13,14] the authors introduced a new version of Bishop frame and an application to spherical images and they studied in $\mathbb{E}_{1}^{3}$, respectively. In ^[15], the authors studied associated curves in Euclidean $3-$space according to Bishop frame.

In this study, we examine the evolute curves, Bertrand curves and Mannheim curves of the normal indicatrix of a regular curve. On the other hand, using the Frenet frame of the normal indicatrix of a regular curve, we obtain the versions of Bishop frame. We achieve this new types of Bishop frame by rotating around the Frenet elements of the normal indicatrix. Considering these associated curves with together the versions of Bishop frame, we say that elements of the version frames correspond to evolute, Bertrand and Mannheim curves of the normal indicatrix of a regular curve.

2.   Materials and method

In this section, we recall some definitions and concepts of space curves in the Euclidean $3-$space. We denote by $\mathbb{E}^{3}$ the Euclidean $3-$ space, with the usual metric.

Let $\alpha = \alpha (s):I\subset R\rightarrow \mathbb{E}^{3}$ be a regular curve in $\mathbb{E}^{3}$, we also assume that it is parametrized by arc-length $s.$ In each point of a space curve we have a moving frame. The Frenet frame $\left \{ T(s), N(s), B(s)\right \}$ is an orthonormal frame where $T(s)$ is the tangent, $N(s)$ is the principal normal and $B(s)$ is the binormal vector of $\alpha (s)$, respectively. Also $\kappa (s)$ and $\tau (s)$ are the curvature and torsion of the curve $\alpha (s),$ respectively. Then, the Frenet equations are given by the following relations;

One of the other moving frame is the Bishop frame. One can express parallel transport of an orthonormal frame along a curve simply by parallel transporting each component of the frame. For the tangent vector, normal vector and binormal vector are applicable. The Bishop trihedra $\left \{ T(s), M_{1}(s), M_{2}(s)\right \}$ is expressed;

where $k_{1}(s)$ and $k_{2}(s)$ are the Bishop curvatures.

Definition 2.1. Let $\alpha :I\subset R\rightarrow \mathbb{E}^{3}$ and $\gamma :I\subset R\rightarrow \mathbb{E}^{3}$ be two curves in the Euclidean $3-$space, with the Frenet frame $\{T_{\alpha }, N_{\alpha }, B_{\alpha }\}$ and $\{T_{\gamma }, N_{\gamma }, B_{\gamma }\}$ respectively.

i. $\gamma$ is called the evolute curve of $\alpha$ if and only if the principal normal vector field of $\gamma$ is equal to tangent vector field of $\alpha$.

ii. $\gamma$ is called the Bertrand curve of $\alpha$ if and only if the principal normal vector field of $\gamma$ is equal to the principal normal vector field of $\alpha$.

iii. $\gamma$ is called the Mannheim curve of $\alpha$ if and only if the principal normal vector field of $\gamma$ is equal to binormal vector field of $\alpha$, ^[16].

Definition 2.2. Let $\alpha :I\subset R\rightarrow \mathbb{E}^{3}$ be a regular curve in the Euclidean $3-$space. If we translate of the principal normal vector field to the center of unit sphere $S^{2}$, we obtain a spherical curve $\alpha _{N}(s_{N}) = N(s)$. This curve is called normal indicatrix of the curve $\alpha = \alpha (s)$, ^[16].

Let $\{T_{N}, N_{N}, B_{N}\}$ be the Frenet frame of $\alpha _{N}$ and $\{\kappa _{N}, \tau _{N}\}$ be its curvatures with the parameter $s_{N}$. Then, the following equations are available;

3.   Some curve pairs according to types of Bishop frame

Let $\alpha = \alpha (s)$ be a curve in the Euclidean $3-$space with the Frenet frame $\{T, N, B\}$ and $\alpha _{N} = \alpha _{N}(s_{N})$ be its normal indicatrix with $\{T_{N}, N_{N}, B_{N}\}.$ For the normal indicatrix $\alpha _{N}$, consider a vector field $X$ given by

where $s_{N}$ is arc-length parameter of $\alpha _{N}$ and $x, y$, $z$ are real functions. We compute our results for unit vector field $X$, then we get

By differentiating above equation, we get

Definition 3.1. Let $\alpha$ be a curve in the Euclidean $3-$space, $\alpha _{N}$ be the normal indicatrix of a curve $\alpha$ and $X$ be the unit vector field satisfies equations $\left(3.1\right)$ and $\left(3.2\right)$. The integral curve of $X$ is called $X-$direction curve of $\alpha _{N}$, ^[17].

Let $\gamma = \gamma (s_{\gamma })$ be a $X-$direction curve of $\alpha_{N}$ with the Frenet apparatus $\{T_{\gamma }, N_{\gamma }, B_{\gamma }, \kappa _{\gamma }, \tau _{\gamma }\}.$ From the Definition 3.1, we know that

where $s_{\gamma }$ is the arc-length parameter of $\gamma.$ Without loss of generality, we assume that $s_{\gamma } = s_{N}.$ By differentiating $\left(3.1\right)$, we get the following equation

Definition 3.2. Let $\alpha$ be a curve in the Euclidean $3-$space$, \; \alpha _{N}$ be normal indicatrix of $\alpha$ according to Frenet frame $\left \{ T_{N}(s_{N}), N_{N}(s_{N}), B_{N}(s_{N})\right \}.$ If we rotate the Frenet frame around the $T_{N}(s_{N})-$axis up to $\theta (s_{N})$, we obtain the frame $\left \{ T_{N}(s_{N}), (M_{1})_{N}(s_{N}), (M_{2})_{N}(s_{N})\right \}$ as follows;

We called this frame as the type of Bishop frame (type-1) of normal indicatrix $\alpha _{N}$.

Theorem 3.1. Let $\alpha$ be a curve$, \; \alpha _{N}$ be normal indicatrix of $\alpha$ according to $\left \{ T_{N}, N_{N}, B_{N}, \kappa _{N}, \tau _{N}\right \}$ and $\gamma$ be $X-$direction curve of $\alpha _{N}.$ If we rotate the Frenet frame in $\mathbb{E}^{3}$ around the $\left(T_{N}\right) -$axis up to $\theta (s_{N}) = \int \tau _{N}(s_{N})ds_{N}$, elements of the version Bishop frame (type-1) $(M_{1})_{N}$ and $(M_{2})_{N}$ are the evolute curves of $\alpha _{N}$.

Proof. Let $\gamma$ be $X-$direction curve of $\alpha _{N}$ with $\left \{ T_{\gamma }(s_{\gamma }), N_{\gamma }(s_{\gamma }), B_{\gamma }(s_{\gamma }), \kappa _{\gamma }(s_{\gamma }), \tau _{\gamma }(s_{\gamma })\right \}$ and $\gamma$ be its evolute. We have the following differential equations system by using $\left(3.4\right);$

If necessary algebraic operations are done, we obtain $x = 0$. Since $y^{2}+z^{2} = 1$, we have

Using $x = 0$ in $\left(3.6\right)$ and using $\left(3.7\right)$, we get

Hence, we can easily see that

or

So, $X-$direction curves as follows are called evolute curves of $\alpha _{N}$.

On the other hand, from the Eq $\left(3.5\right),$ we have

If necessary arrangements are made, $\left(M_{1}\right) _{N}$ and $\left(M_{2}\right) _{N}$ vectors are obtained as follows;

For $\theta (s_{N}) = \int \tau _{N}(s_{N})ds_{N},$

Consequently, from the Eqs $\left(3.8\right)$ and $\left(3.9\right),$ elements of the frame $\left(M_{1}\right) _{N}$ and $\left(M_{2}\right) _{N}$ are the evolute curves of $\alpha _{N}$ the normal indicatrix of a curve $\alpha.$

Definition 3.3. Let $\alpha$ be a curve in the Euclidean $3-$space and $\alpha _{N}$ be normal indicatrix of $\alpha$ according to Frenet frame $\left \{ T_{N}(s_{N}), N_{N}(s_{N}), B_{N}(s_{N})\right \}.$ If we rotate the Frenet frame around the $N_{N}(s_{N})-$axis up to $\theta (s_{N})$, we obtain the frame $\left \{ \left(L_{1}\right) _{N}(s_{N}), N_{N}(s_{N}), \left(L_{2}\right) _{N}(s_{N})\right \}$ as follows;

We called this frame as the type of Bishop frame (type-2) of normal indicatrix $\alpha _{N}$.

Theorem 3.2. Let $\alpha$ be a curve in the Euclidean $3-$space, $\alpha _{N}$ be normal indicatrix of $\alpha$ according to $\left \{ T_{N}, N_{N}, B_{N}, \kappa _{N}, \tau _{N}\right \}$ and $\gamma$ be $X-$direction curve of $\alpha _{N}.$ If we rotate the Frenet frame around the $\left(N_{N}\right) -$axis up to $\theta (s_{N}) = -\theta$ constant angle$,$ elements of the version Bishop frame (type-2) $\left(L_{1}\right) _{N}$ and $\left(L_{2}\right) _{N}$ are the Bertrand curves of $\alpha _{N}$.

Proof. Let $\gamma$ be $X-$direction curve of $\alpha _{N}$ and $\gamma$ be its Bertrand. By using $\left(3.4\right),$ we have the following

If we solve this differential equations system, we obtain $y = 0, \; x = c_{1}, \; z = c_{2}$ where $c_{1}$ and $c_{2}$ are constant real numbers. Since $x^{2}+z^{2} = 1$, we have

where $\theta$ is a constant angle between the tangent vector of the curve $\alpha _{N}$ and the tangent vector of the curve $\gamma.$ Hence, $X-$direction curves are called Bertrand curves of $\alpha _{N}$ as follows;

On the other hand, from the Eq $\left(3.10\right),$ we get

So, $\left(L_{1}\right) _{N}$ and $\left(L_{2}\right) _{N}$ vectors are obtained as follows;

For $\theta (s_{N}) = -\theta$ constant angle$,$

As a result, using the Eqs $\left(3.12\right)$ and $\left(3.13\right), \ $we can easily say that $\left(L_{1}\right) _{N}$ and $\left(L_{2}\right) _{N}$ curves are the Bertrand curves of $\alpha _{N}.$

Definition 3.4. Let $\alpha$ be a curve in the Euclidean $3-$space$, \; \alpha _{N}$ be normal indicatrix of $\alpha$ according to Frenet frame $\left \{ T_{N}(s_{N}), N_{N}(s_{N}), B_{N}(s_{N})\right \}.$ If we rotate the Frenet frame around the $B_{N}(s_{N})-$axis up to $\theta (s_{N})$, we obtain the frame $\left \{ \left(S_{1}\right) _{N}(s_{N}), \left(S_{2}\right) _{N}(s_{N}), B_{N}(s_{N})\right \}$ as follows;

We called this frame as the type of Bishop frame (type-3) of normal indicatrix $\alpha _{N}$.

Theorem 3.3. Let $\alpha$ be a curve in the Euclidean $3-$space$, \; \alpha _{N}$ be normal indicatrix of $\alpha$ according to $\left \{ T_{N}, N_{N}, B_{N}, \kappa _{N}, \tau _{N}\right \}$ and $\gamma$ be $X-$ direction curve of $\alpha _{N}.$ If we rotate the Frenet frame around the $\left(B_{N}\right) -$axis up to $\theta (s_{N}) = \int \kappa _{N}(s_{N})ds_{N},$ elements of the version Bishop frame (type-3) $\left(S_{1}\right) _{N}$ and $\left(S_{2}\right) _{N}$ curves are the Mannheim curves of $\alpha _{N}$.

Proof. Let $\gamma$ be $X-$direction curve of $\alpha _{N}$ and $\gamma$ be its Mannheim. We obtain the following equations using $\left(3.4\right),$

If necessary regulation is made, we obtain $z = 0$ and

By giving $z$ the value $0$ in $\left(3.15\right)$ and using $\left(3.16\right)$, we get

Hence the vector coordinates of $X(s_{N})$ are given by

and

Then, $X-$direction curves are called Mannheim curves of $\alpha _{N}$ as follows;

On the other hand, using Eq $\left(3.14\right),$ we have

If necessary arrangements are made, $\left(S_{1}\right) _{N}$ and $\left(S_{2}\right) _{N}$ vectors are obtained as;

For $\theta (s_{N}) = \int \kappa _{N}(s_{N})ds_{N},$

Then, from the Eqs $\left(3.17\right)$ and $\left(3.18 \right),$ we can easily see that elements of the version frame $\left(S_{1}\right) _{N}$ and $\left(S_{2}\right) _{N}$ are the Mannheim curves of $\alpha _{N}.$

Example 3.1. Let $\alpha (s)$ be a unit speed curve and $N(s)$ be the principal normal vector field of $\alpha$ as follows;

and

Using the $s_{N} = 4s$, the normal indicatrix of the curve $\alpha$ is obtained as

If necessary algebraic operations are made, we get the Serret-Frenet apparatus of normal indicatrix as follows;

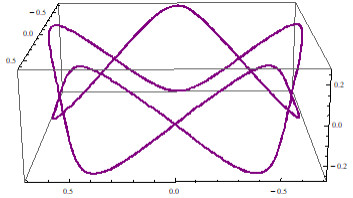
Graphs of the curve $\alpha (s)$ and its normal indicatrix curve $\alpha _{N}(s_{N})$ are shown in Figure 1 and Figure 2.

Let $(M_{1})_{N}, (L_{1})_{N}$ and $(S_{1})_{N}$ be evolute curve, Bertrand curve and Mannheim curve of normal indicatrix $\alpha _{N}$ of the curve $\alpha,$ respectively. In accordance with the theory explained in this study, these curves are obtained as follows;

If we give $\theta _{1} = \frac{\pi }{4}$ and $\theta _{2} = \frac{\pi }{4}$ in above equations, we obtain the $\left(M_{1}\right) _{N}$ and $\left(L_{1}\right) _{N}$ curves as illustrated in the Figure 3;

In addition to these, the integral curves of the vector fields $(M_{1})_{N}, (L_{1})_{N}$ and $(S_{1})_{N}$ are called evolute-direction curve, Bertrand-direction curve and Mannheim-direction curve, respectively. These direction curves obtained as,

If we give $\theta _{1} = \frac{\pi }{4}$, $\theta _{2} = \frac{\pi }{4}, \; c_{i} = 1 \; (i = 1, 2, ..., 9)$ in above equations, we obtain the $\gamma _{\left(M_{1}\right) _{N}}, \gamma _{\left(L_{1}\right) _{N}}$ and $\gamma _{\left(S_{1}\right) _{N}}$ direction-curves as illustrated in Figure 4 and Figure 5.

4.   Conclusions

There have been many studies on evolute curves, Bertrand curves and Mannheim curves to date. In this study, these curves are considered together with the Bishop frame. As a result of this study, we see that the associated curves are the elements of the versions of Bishop frame in Euclidean $3-$space. So, this study offers a new contribution to the literature in this respect.

Conflict of interest

The author declares no conflicts of interest in this paper.