Surface family pair with Bertrand pair as mutual geodesic curves in Euclidean 3-space $\mathbb{E}^{3}$

1.   Introduction

The geodesic among two points on a surface is located as the curve embedded in the surface that relates the points with minimal distance ^[1,2]. Geodesic also has been vastly utilized in different industries, such as cutting and painting path, tent manufacturing, fiberglass tape windings in pipe manufacturing, and textile manufacturing ^[3,4,5,6,7]. Generally, the study in geodesic concentrated on how to find and describe geodesic on the given surfaces, and there were a lots of papers employing on such a problem ^[8,9,10]. In the designing industry of garments, shoes, and so on, it is oftentimes wanted for designers to establish a family of surfaces from a specific spatial geodesic curve, through which they can choose those fulfilling the fashion tastes of customers. This may be considered as the reverse problem of the above-mentioned. In ^[11], Wang et al. considered the problem of constructing a family of surfaces from a specified spatial geodesic curve, through which each surface can be a nominee for style designing. They proved the necessary and sufficient condition for the coefficients to be content with both the geodesic and the isoparametric requirements. Stimulate by Wang et al. ^[11], researchers obtained restrictions for a prescribed curve to be a distinct curve on designed surfaces ^{[12,13,14,15,16,17,18,19,20,21,22,23,24,25]}.

For the theory of space curves, the symmetrical relationship among the curves is an interesting problem. Bertrand curve is one of the classical private curves. Two curves are named Bertrand pair if there exists linearly relationship of their principal normal vectors at the corresponding points ^[1,2]. The Bertrand curve can be considered as the generalization of the helix. The helix, as a specific type of curve, has drawn the awareness of mathematicians as well as scientists because of its different implementations, for example, clarification of DNA, carbon nano-tube, nano-springs, a-helices, the geometrical shaping of linear chained polymers stabilized as helices and the eigenproblems interpreted for collocation of molecules (see ^{[26,27,28,29]}). Moreover, the Bertrand curves perform special examples of offset curves which are applied in computer-aided manufacture (CAM), and computer-aided design (CAD) (see ^[30,31]). However, for our knowledge, there is no work to constructing surface family pair interpolating curve pair to be geodesic curves in Euclidean 3-space $\mathbb{E}^{3}$. This work is intend to serve such a need, we take into consideration Bertrand pair as geodesic curves to constructing surface family pair in $\mathbb{E}^{3}$.

The major advantage of this work is to establish surface family pair from given Bertrand pair. Hence, the sufficient and necessary conditions for the given Bertrand pair to be the geodesic pair are given in details. As an application, some representative Bertrand pair are selected to form their corresponding surface family pair that have such Bertrand pair as geodesic curves. We extended the study to ruled surface family pair.

2.   Preliminaries

The ambient space is the Euclidean space $\mathbb{E}^{3}$ and for our work we have used ^[1,2] as general references. A curve is regular if it confess a tangent line at each point of the curve. In the following, all curves are supposed to be regular. Given a spatial curve ${\boldsymbol{\alpha }}(s)$, which is expressed by arc length parameter $s$. We assume $\overset{..}{{\boldsymbol{ \alpha }}}{\bf{(}}s{\bf{)}}\neq$ $0$ for all $s$ $\in$ $[0, L]$, since this would give us a straight line. In this paper, $\overset{.}{{\boldsymbol{ \alpha }}}(s)$ and ${\boldsymbol{\alpha }}^{^{\prime }}(r)$ indicate the derivatives of ${\boldsymbol{\alpha }}$ with respect to arc-length parameter $s$ and arbitrary parameter $r$, respectively. For each point of ${\boldsymbol{\alpha }}(s)$, the set $\{ {\bf{t}}(s),$ ${\bf{n}}(s)$, ${\bf{b}}(s)\}$ is named the Serret-Frenet frame on ${\boldsymbol{\alpha }}(s)$, where ${\bf{t}} (s) = \overset{.}{{\boldsymbol{\alpha }}}(s)$, ${\bf{n}}(s)$ $= \overset{..}{ {\boldsymbol{\alpha }}}(s)/\left \Vert \overset{..}{{\boldsymbol{\alpha }}}(s)\right \Vert$ and ${\bf{b}}(s) = {\bf{t}}(s)\times {\bf{n}}(s)$ are the unit tangent, principal normal, and binormal vectors of the curve at the point ${\boldsymbol{\alpha }}(s)$, respectively. The arc-length derivative of the Serret-Frenet frame is governed by the relations ^[1]:

where the curvature $\kappa (s)$ and torsion $\tau (s)$ are specified by

Although the parameter of arc-length is simple for analyzing, in the majority of practical situations, the parameter of a given curve is commonly not in arc-length parametrization. We can represent the given curve by employing arc-length representation. Given the curve

where the parameter $r$ is not the arc-length. The synthesis of the Serret-Frenet frame are specified by ^[1]:

and the Serret-Frenet formula are

We utilize basic notification on Bertrand pair from ^[1,2]. Let ${\boldsymbol{\alpha }}(s)$, and $\widehat{{\boldsymbol{\alpha }}}(s)$ be two curves in $\mathbb{E }^{3}$, ${\bf{n}}(s)$ and$\; \widehat{{\bf{n}}}(s)$ are principal normal vectors of them respectively, the pair {${\boldsymbol{\alpha }}(s)$, $\widehat{ {\boldsymbol{\alpha }}}(s)$} is named Bertrand pair if ${\bf{n}}(s)$ and $\widehat{{\bf{n}}}(s)$ are linearly dependent at the corresponding points, ${\boldsymbol{\alpha }}(s)$ is named the Bertrand mate of $\widehat{{\boldsymbol{\alpha }}}({s})$, and

where $f$ is a stationary. Therefore, the formulae the Serret-Frenet frame of ${\boldsymbol{\alpha }}(s)$ with that of $\widehat{{\boldsymbol{\alpha }}}(s)$ are

where $\psi$ is a constant angle.

We signalize a surface $M$ by

If ${\bf{y}}_{j}(s, t) = \frac{\partial {\bf{y}}}{\partial j}$, the surface normal is

which is orthogonal to each of the vectors ${\bf{y}}_{s}$ and ${\bf{y}} _{t}$.

Remark 2.1. ^[1,2] A curve on a surface is a geodesic if and only if the principal normal vector of the curve is everywhere parallel to surface normal

A curve ${\boldsymbol{\alpha }}(s)$ on a surface ${\bf{y}}(s, t)$ is an isoparametric curve if it has a constant $s$ or $t$-parameter value. In other words, there exists a parameter $t_{0}$ such that ${\boldsymbol{\alpha }}(s) = {\bf{y}}(s, t_{0})$ or ${\boldsymbol{\alpha }}(t) = {\bf{y}}(s_{0}, t)$. Given a parametric curve ${\boldsymbol{\alpha }}(s)$, we call it an isogeodesic of the surface ${\bf{y}}(s, t)$ if it is both an geodesic and a parameter curve on ${\bf{y}}(s, t)$.

3.   Main results

This section presents a new approach for constructing surface family pair interpolating Bertrand pair as mutual geodesic curves in $\mathbb{E}^{3}$. To do this, we take into account a Bertrand pair, such that the surfaces tangent planes are coincident with the curves rectifying planes.

Let ${\boldsymbol{\alpha }}(s)$ be a curve with $\left \Vert \overset{..}{{\boldsymbol{ \alpha }}}(s)\right \Vert \neq 0$, $\widehat{{\boldsymbol{\alpha }}}(s)$ is Bertrand mate of ${\boldsymbol{\alpha }}(s)$, and $\{ \widehat{\kappa }(s)$, $\widehat{\tau } (s)$, $\widehat{{\bf{t}}}(s), \widehat{{\bf{n}}}(s)$, $\widehat{{\bf{b}} }(s)\}$ is the Frenet-Serret apparatus of $\widehat{{\boldsymbol{\alpha }}}(s)$ as in Eq (2.1). The surface family $M$ interpolating ${\boldsymbol{\alpha }}(s)$ can be written as ^[1]:

and the surface family $\widehat{M}$ interpolating $\widehat{{\boldsymbol{\alpha }} }(s)$ is

Here $a(s, t),$ $b(s, t)\in C^{1}$ are named marching-scale functions.

In order to obtain the $\widehat{M}$ interpolating $\widehat{{\boldsymbol{\alpha }} }(s)$ as a mutual geodesic curve, according to Eqs (3.1) and (3.2), we discuss what the marching-scale functions should satisfy. To do this, we have

and

Since $\widehat{{\boldsymbol{\alpha }}}(s)$ is an isoparametric on $M$, there exists a value $t = t_{0}\in \lbrack 0, T]$ such that $\widehat{{\bf{y}}} (s, t_{0}) = \widehat{{\boldsymbol{\alpha }}}(s)$, that is,

Thus, when $t = t_{0}$, i.e., over $\widehat{{\boldsymbol{\alpha }}}(s)$, we have

Coincidence of the rectifying plane of $\widehat{{\boldsymbol{\alpha }}}(s)$ with the tangent plane of the surface $\widehat{M}$ identifies the curve as a geodesic curve. Then from Eqs (3.2)–(3.6), we get the following theorem.

Theorem 3.1. The surface family pair $\{ M$, $\widehat{M} \}$ interpolate Bertrand pair $\{ {\boldsymbol{\alpha }}(s)$, $\widehat{{\boldsymbol{ \alpha }}}(s) \}$ as mutual geodesic curves if and only if

As in ^[8], for the intents of facilitation and inspection, we also address the case when the marching-scale functions $a(s, t)$, and $b(s, t)$ can be display into two factors:

Here $l({s})$, $m({s}), A(t)$ and $B(t)$ are $C^{1}$ functions are not identically vanish. Then, from Theorem 3.1, we gain

Corollary 3.1. The surface family pair $\{ M$, $\widehat{M} \}$ interpolate Bertrand pair $\{ {\boldsymbol{\alpha }}(s)$, $\widehat{{\boldsymbol{ \alpha }}}(s) \}$ as mutual geodesic curves if and only if

In Eq (2.5), if $\psi = 0$ and $\psi = \pi /2$ then the pair $\{ M$, $\widehat{ M} \}$ are named oriented pair, and right pair, respectively. Further to acquire $\{ M$, $\widehat{M} \}$, interpolate Bertrand pair $\{ {\boldsymbol{\alpha }} (s)$, $\widehat{{\boldsymbol{\alpha }}}(s) \}$, we can first design the marching-scale functions in Eq (3.9), and then use them to Eqs (3.1) and (3.2) to derive the parameterization. For suitability in practice, the $a(s, t)$, and $b(s, t)$ can be moreover constrained to be in extra limited forms and still possess sufficient degrees of freedom to specify large family pair interpolate Bertrand pair $\{ {\boldsymbol{\alpha }}(s)$, $\widehat{ {\boldsymbol{\alpha }}}(s) \}$ as mutual geodesic curves. Therefore, let us assume that $a(s, t)$, and $b(s, t)$ can be displayed two different forms:

(1) If we choose

Thus, we can simply express the sufficient condition for which the $\{ {\boldsymbol{\alpha }}(s)$, $\widehat{{\boldsymbol{\alpha }}}(s) \}$ are geodesic curves on the surface family pair $\{ M$, $\widehat{M} \}$ as

where $l(s),$ $m(s),$ $A(t), B(t)$ $\in C^{1}$, $a_{ij}$, $b_{ij}\in \mathbb{R}$ $(i = 1, 2;j = 1, 2, ..., p)$ and $l(s)$, and $m(s)$ are not identically zero.

(2) If we choose

then

where $l(s),$ $m(s),$ $A(t), B(t)\in C^{1}$, $a_{ij}$, $b_{ij}\in \mathbb{R}$ $(i = 1, 2;j = 1, 2, ..., p)$ and $l(s)$, and $m(s)$ are not identically zero.

Now, we are dealing with and constructing some representative examples to verify the approach. They also serve to confirm the correctness of the formulae obtained above.

Example 3.1. If ${\bf{q}}_{0} = (0, 0, 0), \ {\bf{q}} _{1} = (0, 1, 1)$ and ${\bf{q}}_{2} = (1, 2, 0)$ are points in the Euclidean 3-space $\mathbb{E}^{3}$, then the quadratic Bézier curve can be specified as

where

are the blending functions of the curve ${\boldsymbol{\alpha }}(r)$. It is easy to show that

After simple computation, we get

where $\rho (r) = \sqrt{5r^{2}-4r+2}$. Choosing $a(r, t) = -4rt$, $b(r, t) = -t$, $\gamma \neq 0$, and $t_{0} = 0$. Obviously, Eq (3.9) is satisfied, and the parametric surface specified by Eq (3.1) is

Let $f = \sqrt{6}$ in Eq (2.7), we get

Via Eq (2.5), we find

Then, we have

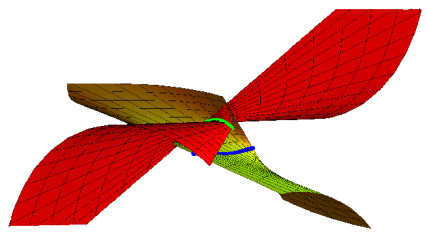
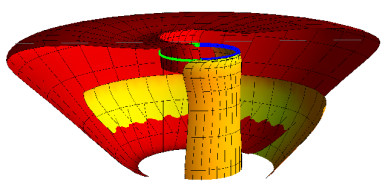
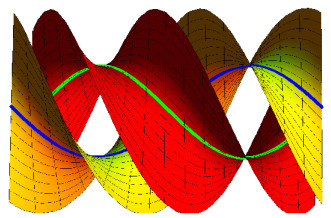
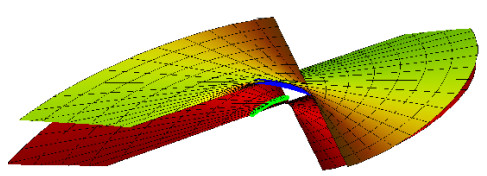
For $\beta = \gamma = -1$ the oriented pair, and the right pair, respectively, are shown in Figures 1 and 2, where $0\leq r\leq 1$, and $-15\leq t\leq 15$.

Example 3.2. Given a helix

The Serret-Frenet frame is

Then, the parametric surface defined by Eq (3.1) is

Let $f = \sqrt{2}$ in Eq (2.7), we get

Via Eq (2.5), we find

Then, we have

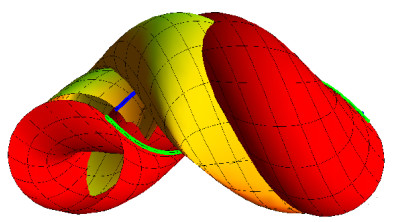
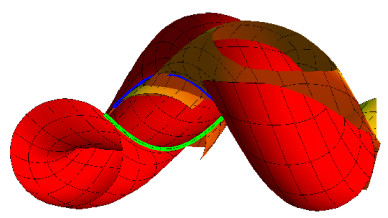
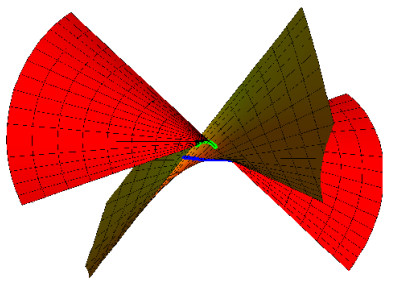
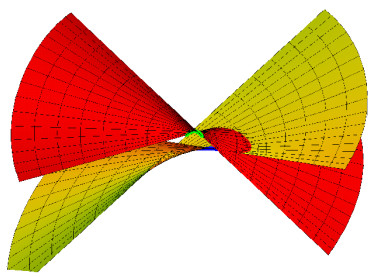
If $a(s, t) = \sin t$, $b(s, t) = 1-\cos t$, $t_{0} = 0$, the oriented pair, and the right pair, respectively, are shown in Figures 3 and 4, where $0\leq s, t\leq 2\pi$.

Example 3.3. Let

Then,

Then, the surface specified by Eq (3.1) is

Let $f = 2$ in Eq (2.7), we get

Similarly, we find

and

If we choose

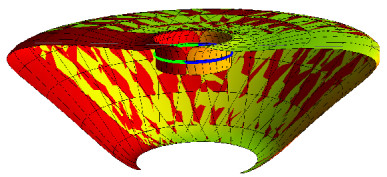
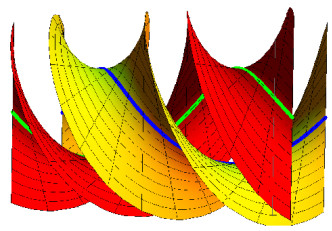
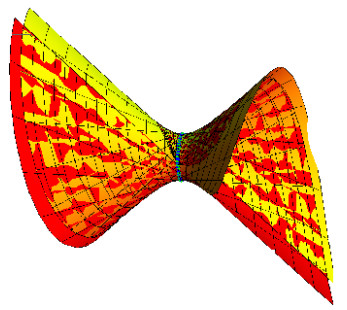
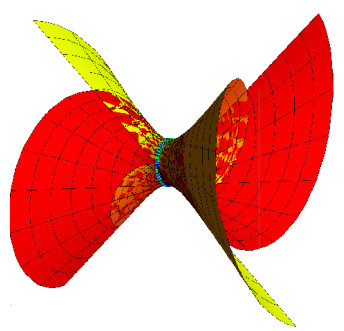
where $t_{0} = 0$, $\frac{3\pi }{2}$, $a_{1k}$, $b_{1k}\in \mathbb{R}$, and $0\leq t\leq 2\pi$, then Eq (3.11) is satisfied. Therefore, the oriented pair, and the right pair, respectively, are shown in Figure 5 and 6, where $0\leq s\leq \pi$, and $0\leq t\leq 5$.

3.1.   Ruled surface family pair with Bertrand pair as mutual geodesic curves

A ruled surface is a special surface created by a continuous movable of a line (ruling) on a curve, which acts as the base curve. In this subsection, we will address the construction of ruled surface family pair with Bertrand pair as mutual geodesic curves. For the ease of search, let us consider that $\widehat{{\boldsymbol{\alpha }}}(s)$ be a unit speed curve. Suppose that $\widehat{{\bf{y}}}(s, t)$ is a ruled surface with the base $\widehat{ {\boldsymbol{\alpha }}}(s)$ and $\widehat{{\boldsymbol{\alpha }}}(s)$ is also an isoparametric curve of $\widehat{{\bf{y}}}(s, t)$, then there exists $t_{0}$ such that $\widehat{{\bf{y}}}(s, t_{0}) = \widehat{{\boldsymbol{\alpha }}}(s)$. This follows that the surface can be specified as

where $\widehat{{\bf{e}}}(s)$ is a unit vector specify the orientation of the rulings. Via the Eq (3.2), we have

which is a system of two equations with two unknown functions $a(s, t)$, and $b(s, t)$. To solve the functions $a(s, t)$, and $b(s, t)$ we have

Equation (3.15) is exactly the necessary and sufficient conditions for ${\bf{ y}}(s, t)$ is a ruled surface.

First, we need to examine if $\widehat{{\boldsymbol{\alpha }}}(s)$ is also geodesic on $\widehat{M}$ by employing the Theorem 3.1. It is apparent that in this case, these follows that

Then, at any point on $\widehat{{\boldsymbol{\alpha }}}(s)$, the ruling orientation $\widehat{{\bf{e}}}$ should be in the rectifying plane. Also, the $\widehat{{\bf{e}}}$, and $\widehat{{\bf{t}}}$ must not be parallel. This follows that

Substituting Eq (3.17) into the Eq (3.15), we attain

Then, the ruled surface family with the mutual geodesic $\widehat{{\boldsymbol{ \alpha }}}(s)$ can be specified as

where $x(s),$ $y(s)\neq 0$, $\ 0\leq s\leq L,$ and$\ 0\leq t\leq T$. However, the normal vector to $\widehat{M}$ along the curve $\widehat{ {\boldsymbol{\alpha }}}(s)$ is

which show that $\widehat{{\boldsymbol{\alpha }}}(s)$ is a geodesic curve on $\widehat{M}$. Then the following theorem can be stated.

Theorem 3.2. The ruled surface family pair $\{ M$, $\widehat{M} \}$ interpolate Bertrand pair $\{ {\boldsymbol{\alpha }}(s)$, $\widehat{ {\boldsymbol{\alpha }}}(s) \}$ as mutual geodesic curves if and only if there exist a parameter $t_{0}\in \lbrack 0, T]$, and the functions $x(s),$ $y(s)\neq 0$, so that $\widehat{M}$, and $M$, respectively, parametrized by Eq (3.19), and

It must be pointed out in Eqs (3.19) and (3.21), there exist two geodesic curves crossing during every point on the curves $\widehat{{\boldsymbol{\alpha }}} (s)$(${\boldsymbol{\alpha }}(s))$ one is $\widehat{{\boldsymbol{\alpha }}}$ itself and the other is a line in the orientation $\widehat{{\bf{e}}}(s)$ as given in Eq (3.17). Every constituent of the isoparametric ruled surface family with the mutual geodesic $\widehat{{\boldsymbol{\alpha }}}$ is established by two set functions $x(s),$ $y(s)\neq 0$.

Example 3.4. In view of Example 3.1, for $x(r) = y(r) = -1$, the ruled oriented pair $\{ M$, $\widehat{M} \}$, and the ruled right pair $\{ M$, $\widehat{M} \}$, respectively, are shown in Figures 7 and 8, where $0\leq r\leq 1$, and $-15\leq t\leq 15$.

Example 3.5. In view of Example 3.2, for $x(s) = y(s) = 1$, the ruled oriented pair $\{ M$, $\widehat{M} \}$, and the ruled right pair $\{ M$, $\widehat{M} \}$, respectively, are shown in Figures 9 and 10, where $0\leq s\leq 2\pi$, and $-1\leq t\leq 1$.

Example 3.6. In view of Example 3.3, for $x(s) = y(s) = s$, the ruled oriented pair $\{ M$, $\widehat{M} \}$, and the ruled right pair $\{ M$, $\widehat{M} \}$, respectively, are shown in Figures 11 and 12, where $0\leq s\leq 2\pi$, and $-1\leq t\leq 1$.

4.   Conclusions

In this work, we constructed the surface family pair and ruled surface family pair having Bertrand pair as mutual geodesic curve in Euclidean 3-space $\mathbb{E}^{3}$. Meanwhile, some curves are selected to organize the surface family pair and ruled surface family pair which have the Bertrand pair $\{ \widehat{{\boldsymbol{\alpha }}}(s)$, ${\boldsymbol{\alpha }}(s) \}$ as mutual geodesic curves. Hopefully, these results will be advantageous to the work in computer-aided manufacture and those exploring the manufacturing. There are several opportunities for further work. The authors plans to register the study in different spaces and examining the classification of singularities.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors would like to acknowledge the Princess Nourah bint Abdulrahman University Researchers Supporting Project number (PNURSP2023R337), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia

Conflict of interest

The authors declare that there is no conflict of interest regarding the publication of this paper.