Dynamical transition for a 3-component Lotka-Volterra model with diffusion

1.   Introduction

In this paper, we consider the following 3-component Lotka-Volterra model with diffusion

where $u$, $v$ are the population densities of two competing prey and $w$ is the population density of its predator. The habitat $\Omega\subset\mathbb{R}^{n}$ is a bounded domain with smooth boundary $\partial\Omega$. $r, a_{i}, b_{i}, c_{i}, \alpha_{i}, k_{i} (i = 1, 2)$ and $d_{j}(j = 1, 2, 3)$ are positive constants. $a_{1}$ and $a_{2}$ represent the intrinsic growth rate, $b_{i}$ and $c_{i}(i = 1, 2)$ represent the intra-specific and inter-specific competition rates of $u$ and $v$, $k_{1}$ and $k_{2}$ are the predation rate of $w$, $\alpha_{1}$ and $\alpha_{2}$ are the transformation rate of predation, and $r$ is the death rate of $w$. $d_{1}$, $d_{2}$ and $d_{3}$ are represent the diffusion rates of $u$, $v$ and $w$ respectively.

Here, we focus on the system $(1.1)$ supplemented with the following initial condition:

and the Neumann boundary condition:

where $\frac{\partial }{\partial n}$ is the outward normal derivative on $\partial\Omega$. The Neumann boundary condition in $(1.3)$ was interpreted as the condition that the system is self-contained with zero population flux across the boundary.

For two species Lotka-Volterra (LV) systems, there has been largely discussed in the past several decades. Kuto and Tsujikawa ^[1] considered a general stationary Lotka-Volterra competition model with diffusion. They obtained the existence of nonconstant solutions by the Leray-Schauder degree theory and derived a limiting system as diffusion of one of the species tending to infinity. Eilbeck et al.^[2] studied the two species Lotka-Volterra competition model and obtained the existence and non-uniqueness of coexistence solutions for a wide range of parameters. Other results related to the two species Lotka-Volterra competition model, we refer to other studies ^[3,4,5,6] and the references therein.

However, very little result is known about the three species Lotka-Volterra model. In general, three species systems are very complicated even in the ordinary differential equations case. In recent years, the three species Lotka-Volterra model with diffusion was studied by some investigators. Ni et al.^[7] considered the role of cross-diffusion in the 3 $\times$ 3 Lotka-Volterra competition model, and obtained the existence of non-constant steady states created by cross-diffusion in 3 $\times$ 3 systems. In ^[8], Pang and Wang studied a strongly coupled system of a two-predator-one-prey ecosystem, they demonstrated the emergence of stationary patterns for this system, and showed that it is due to the cross diffusion that arises naturally in the model. Moreover, Ali et al.^[9] studied the prey-predator-top-predator system, in addition, they point out that the system exhibits Bogdanov-Takens bifurcation, saddle-node bifurcation, Hopf bifurcation for suitable choice of the relevant parameters. There are other related works on three species model, see ^{[10,11,12,13,14,15,16,17]} and references therein.

Although considerable work has been done concerning three competition model and two-predator-one-prey ecosystem, it is worth noting that it is interesting to investigate two prey and one predator system. In some circumstances, predation may have a tendency to increase species diversity in competitive communities, which is called predation mediated coexistence. For instance, in ^[10], the authors considered the coexistence problem of two competing species mediated by the presence of predator, and speculate that the possibility. Furthermore, Kan-on and Mimura ^[11] proved the existence of stable spatially inhomogeneous positive stationary solutions of $(1.1)$. In addition, Yukio Kan-on ^[12] studied the positive stationary solutions by using the singular perturbation method and the associated singular limit spectral analysis. Moreover, Wang ^[13] considered the strongly coupled version of $(1.1)$ and established the existence and non-existence of non-constant positive solutions.

Motivated by the above papers, what we are concerned in this paper is to describe the dynamic stability and transition for the system $(1.1)$. The technical method for the analysis is the dynamical transition theory, which has been developed by Ma and Wang ^[18,19,20] and has been used to solve many interesting mathematical and physical problems, see ^{[21,22,23,24,25,26]}. As is well known, for the system $(1.1)$, due to non-selfadjoint linear operator, the transition can be caused by real or complex eigenvalues crossing the imaginary axis. Let $\delta_0$ and $\delta_1$ be critical values given by $(3.17)$ and $(3.20)$. $\delta_0$ is derived from $AB-C = 0$, which determine the signs of the eigenvalues of the linearized eigenvalue equations $(3.7)$. The same is $\delta_1$, which is derived from $C_k = 0$. When $\delta_0 > \delta_1$, the first eigenvalue is real and simple, and all three types of transition are possible depending on a non-dimensional number exactly given in terms of the system parameters. In particular, the transition is mixed if $\int_{\Omega}e_{k_0}^3dx\neq 0$, and in the case where $\int_{\Omega}e_{k_0}^3dx = 0$ we show that the transition of the system is continuous as $\tilde{\theta} < 0$, and is jump as $\tilde{\theta} > 0$ where $\tilde{\theta}$ is defined in $(4.43)$. when $\delta_0 < \delta_1$, the first eigenvalues are complex, and we show that the system undergoes a continuous or jump transition. In the small oscillation frequency limit, the transition is always continuous and the time periodic rolls are stable after the transition.

The rest of this paper is organized as follows. In Section 2, we present some preliminaries on dynamical transition theory. Section 3 recapitulates (1) the nondimensional form and the nonnegative basic states of the steady-state equations for the system $(1.1)$, (2) an abstract form for $(1.1)$, and (3) linear theory and principle of exchange of stabilities(PES). The main theorems of this artical are stated and proved in Section 4.

2.   Preliminaries

In this section, we introduce the dynamical transition theory for nonlinear dissipative systems developed by Ma and Wang ^[18,19,20], which provides the basic method for the following research of this paper.

Let $H$ and $H_{1}$ be two Hilbert spaces, $H_{1}\subset H$ be a compact and dense inclusion. Consider the following abstract nonlinear equation defined on $H$, given by

where $\chi(t)$ is an unknown function, $L_{\lambda}: H_{1}\rightarrow H$ is a linear operator, and $G: H_{1}\rightarrow H$ is a nonlinear operator, $\lambda$ is the system parameter.

Assume that $L_{\lambda}: H_{1}\rightarrow H$ is a parameterized linear completely continuous field depending continuously on $\lambda$. which satisfies

Furthermore, we assume that the nonlinear term $G: H_{\sigma}\rightarrow H(0\le\sigma < 1)$ is a $C^{r}$ bounded operators $(r\ge 1)$ and depending continuously on $\lambda$, where $H_{\sigma}$ is the fractional order space, and

Hereafter, we always assume the conditions $(2.2)$ and $(2.3)$ hold true, which imply that the system $(2.1)$ has a dissipative structure.

At first, we recall the mathematical definition of transition for the system $(2.1)$.

Definition 2.1. (^[20]) We say that the system $(2.1)$ has a transition from $(\chi, \lambda) = (0, \lambda_{0})$ at $\lambda_{0}$ if the following two conditions hold true:

$(1)$ if $\lambda < \lambda_{0}$, $\chi = 0$ is locally asymptotically stable for $(2.1)$, and

$(2)$ if $\lambda > \lambda_{0}$, there exists a neighborhood $U\subset H$ of $\chi = 0$ independent of $\lambda$, such that for any $\chi_{0}\in U\setminus\Gamma_{\lambda}$ the solution $\chi_{\lambda}(t, \chi_0)$ of $(2.1)$ satisfies that

where $\Gamma_{\lambda}$ is the stable manifold of $\chi = 0$, with codim $\Gamma_{\lambda}\ge 1$ in $H$ for $\lambda > \lambda_0$.

Let the eigenvalues (counting multiplicity) of $L_{\lambda}$ be given by $\{\beta_{j}(\lambda)\in\mathbb{C}|j = 1, 2, \cdots\}$, and let

The following theorem is a basic principle of transitions from equilibrium states, which provides sufficient conditions and a basic classification for transitions of nonlinear dissipative systems. The proof of this Lemma is given in Ma and Wang ^[19,20].

Lemma 2.1. Let the conditions $(2.5)$ and $(2.6)$ hold true. Then the system $(2.1)$ must have a transition from $(\chi, \lambda) = (0, \lambda_{0})$, and there is a neighborhood $U\subset H$ of $\chi = 0$ such that the transition is one of the following three types:

$(1)$ Continuous transition: There exists an open and dense set $\widetilde{U_{\lambda}}\subset U$ such that for any $\chi_0\in\widetilde{U_{\lambda}}$, the solution $\chi_{\lambda}(t, \chi_0)$ of (2.1)satisfies

$(2)$ Jump transition: For any $\lambda_{0} < \lambda < \lambda_{0}+\varepsilon$ with some $\varepsilon > 0$, there is an open and dense set $U_{\lambda}\subset U$ such that for any $\chi_0\in U_{\lambda}$,

$(3)$ Mixed transition: For any $\lambda_{0} < \lambda < \lambda_{0}+\varepsilon$ with some $\varepsilon > 0$, $U$ can be decomposed into two open sets $U_{1}^{\lambda}$ and $U_{2}^{\lambda}$ ($U_{i}^{\lambda}$ not necessarily connected): $\bar{U} = \bar{U}_{1}^{\lambda}+\bar{U}_{2}^{\lambda}, U_{1}^{\lambda}\cap U_{2}^{\lambda} = \emptyset$, such that

where $U_{1}^{\lambda}$ and $U_{2}^{\lambda}$ are called metastable domain.

3.   Mathematical setting and linear problem

3.1.   Mathematical setting

The forthcoming analysis is to formulate the evolution equations given in $(1.1)$ using an abstract functional setting that is standard in the framework of dynamic transitions.

First, we introduce a set of the following to nondimensionalize the system $(1.1)$:

where the prime denotes nondimensionalized variables. Substituting these nondimensional variables into $(1.1)$ and neglecting the prime for all variables for convenience, we obtain

here

and the unknown functions are $u$, $v$, $w\ge 0$, $\Omega\subset\mathbb{R}^{n}$ is a bounded domain, the parameters are positive constants:

Furthermore, let

Then we define the following function spaces,

where $\chi = (u, v, w)$.

Define the operators $L_{\lambda} = A_{\lambda}+B_{\lambda}$ and $G_{\lambda}:H_{1}\rightarrow H$ by

Obviously, $L_{\lambda}:H_{1}\rightarrow H$ is a parameterized linear completely continuous field depending continuously on $\lambda$, and $G_{\lambda}:H_{1}\rightarrow H$ represents the nonlinear terms of the Eq $(3.1)$.

Thus, the Eqs $(3.1)$ with $(1.2)$ and $(1.3)$, take the following operator form:

where $\lambda = (\varepsilon_{1}, \varepsilon_{2}, \varepsilon_{3}, \bar{a}_{1}, \bar{a}_{2}, \alpha, \gamma, \kappa, \sigma, q)\in \mathbb{R}_{+}^{10}$.

On the other hand, we study the steady-state solutions for the system $(3.1)$. It is easy to check that the system $(3.1)$ admits seven biologically realistic constant steady-state solutions:

where

Biologically, only positive solutions $(u_{0} > 0, v_{0} > 0, w_{0} > 0)$ are of interest in the competition of biological population. Hence, we make the natural assumption: $u_{0} > 0, v_{0} > 0, w_{0} > 0$.

In this paper, we mainly focus on the bifurcation and transition problem of $(3.1)$ at the more general positive steady-state solution $\varphi_{6}$ in $(3.3)$.

For this purpose, we take the translation

Omitting the primes, the system $(3.1)$ with $(1.2)$–$(1.3)$ becomes

with the initial-boundary conditions

Then it suffices to study the bifurcation solution of $(3.5)$ at the steady-state solution $\chi = (0, 0, 0)^{T}$.

3.2.   Linear theory and principle of exchange of stabilities (PES)

The linearized eigenvalue equations of $(3.5)$ are given by

Let $\rho_k$ and $e_k$ be the $k$th eigenvalue and eigenvector of the Laplace operator $\Delta$ with the Neumann boundary condition:

Let $M_{k}$ be the matrix given by

Thus, all eigenvalues $\beta(\lambda) = \beta_{ki}(\lambda)$ of $(3.7)$ satisfy

where $\eta_{ki}\in\mathbb{R}^{3}$ is the eigenvector of $M_{k}$ corresponding to $\beta_{ki}(\lambda)$. Hence, the eigenvector $\psi_{ki}$ of $(3.7)$ corresponding to $\beta_{ki}(\lambda)$ is

where $e_{k}(x)$ is as in $(3.8)$.

In particular, $\rho_{1} = 0$ and $e_{1}$ is a constant, and

By simple calculation, it is not difficult to find that the eigenvalues $\beta(\lambda) = \beta_{1i}(\lambda)(i = 1, 2, 3)$ satisfy the following equation:

where

It is known that all solutions of $(3.13)$ have negative real parts if and only if

If we suppose $q(\gamma-\kappa)-(1-\kappa\alpha) < 0$ in $(3.3)$, then, direct calculation indicates that these two parameters $A$ and $C$ in $(3.14)$ are positive

Note that

which implies that all real eigenvalues of $(3.12)$ do not change their signs, and at least one of these real eigenvalues is negative.

In addition, let $\delta = \alpha\gamma = \frac{c_1c_2}{b_1b_2}$, and we can derive from $AB-C = 0$, the critical number

It is then clear that

Next, we check the other eigenvalues $\beta_{kj}(\lambda)$ with $j = 1, 2, 3, \; k\ge 2$. By calculation, the eigenvalues $\beta_{kj}(\lambda)(j = 1, 2, 3, k\ge 2)$ of $(3.9)$ satisfy

where

We introduce another critical number

where

The following lemma characterizes the principle of exchange stability (PES) for the eigenvalue Eq $(3.7)$.

Lemma 3.1. Assume that $q(\gamma-\kappa)-(1-\kappa\alpha) < 0$, let $\delta_{0}$ and $\delta_{1}$ be the numbers given by $(3.17)$ and $(3.20)$, then the eigenvalues $\beta_{ki} (i = 1, 2, 3, k\ge 1)$ of $L_{\lambda}$ satisfy the following properties:

$(1)$ If $\delta_{1} < \delta_{0}$ and $k_{0}$ be the integer that $\delta_{1}$ in $(3.20)$ reaches its minimum at $\rho_{k_0}$, then $\beta_{k_{0}1}$ is a real eigenvalue of $(3.7)$, and

$(2)$ If $\delta_{1} > \delta_{0}$, then $\beta_{11}(\delta) = \bar{\beta}_{12}(\delta)$ are a pair of complex eigenvalues of $(3.7)$, and

Proof. According to the assumption, $C$ is positive. We see that

By the direct calculation, we can see that

As $\delta_{1} < \delta_{0}$, we infer from $(3.16)$ and $(3.18)$ that

In addition, it is clear that there must exist a $k_0$ satisfying $(3.20)$, furthermore,

thus, assertion (1) is approved.

As $\delta_{1} > \delta_{0}$, through the analysis of above, we know that $C_k > 0$ at $\delta = \delta_0$ for all $k\ge 2$. Since all real eigenvalues $\beta_{1j} (1\le j \le 3)$ of $(3.12)$ do not change their signs, and at least one of these real eigenvalues is negative, so the condition $(3.18)$ implies that there exists a pair of complex eigenvalues $\beta_{11} = \bar{\beta}_{12}$ crossing the imaginary axis at $\delta = \delta_{0}$. Then assertion (2) follows. The Lemma is proved.

4.   Main results and proofs

The following theorems will show the types of transition that the system $(3.5)$ undergoes as the bifurcation parameter $\delta$ crosses the critical value $\delta_0$ or $\delta_1$ basing on Lemma $3.1$. Hereafter, we will give different transition theorems basing on Lemma $3.1$.

First, we consider the case that $\delta_{0} < \delta_{1}$.

4.1.   Transitions from complex eigenvalues

By Lemma $3.1$, as $\delta_{0} < \delta_{1}$, the first critical eigenvalues will be a pair of complex numbers $\beta_{11}$ and $\beta_{12}$, the problem $(3.5)$ undergoes a dynamic transition to a periodic solution from $\delta_{0}$. To determine the types of transition, we introduce a parameter $b_{0}$ which is defined by $(4.30)$ as follows:

where

Here $A$ is as in $(3.14)$, and $a, b, \tilde{a}, \tilde{b}$ are as in $(4.6)$ and $(4.8)$.

Then, we have the following dynamic transition theorem.

Theorem 4.1. Consider $b_0$ which is given by $(4.1)$. Let $\delta_{0} < \delta_{1}$. Assume $q(\gamma-\kappa)-(1-\kappa\alpha) < 0$ and assume that the critical index are $(k, j) = (1, 1)$ and $(k, j) = (1, 2)$, then the problem $(3.5)$ undergoes a transition to periodic solutions at $\delta = \delta_{0}$, and the following assertions holds true:

$(1)$ If $b_0 < 0$, then the transition of $(3.5)$ is continuous, and the system bifurcates to a periodic solution on $\delta > \delta_{0}$, which is an attractor.

$(2)$ If $b_0 > 0$, then the transition of $(3.5)$ is jump, and the system bifurcates on $\delta < \delta_{0}$ to a unique unstable periodic orbit.

Proof. We shall prove the theorem in the following two steps.

Step 1. Calculate the critical eigenvectors.

By Lemma $3.1$, at $\delta_{0}$ there is a pair of imaginary eigenvalues $\beta_{11} = \bar{\beta}_{12} = -i\rho$ of $(3.7)$. Let $z = \xi+i\eta$ and $z^{*} = \xi^{*}+i\eta^{*}$ be the eigenvectors and conjugate eigenvectors of $(3.7)$ corresponding to $-i\rho$, i.e. $z$ and $z^{*}$ satisfy that

For $z = (z_{1}, z_{2}, z_{3})$, from the first equation of $(4.3)$ we obtain

Thus, we derive from $(4.4)$ the eigenvectors $z = \xi+i\eta$ as follows:

where

In the same fashion, we derive from the second equation of $(4.3)$ the conjugate eigenvectors $z^{*} = \xi^{*}+i\eta^{*}$ as

where

It is easy to show that

It is known that functions $\psi_{1}^{*}+i\psi_{2}^{*}$ given by

also satisfy the second equation of $(4.3)$ with

On the other hand, we know that

In addition, because $\pm i\rho$ are solutions of $(3.13)$, and $AB-C = 0$ at $\delta_{0}$, we deduce that

Then, we obtain

From the equation

we derive the eigenvector

In the same fashion, from

we derive the conjugate eigenvector of $\beta_{13}$ as follows:

Step 2. Derivation of evolution equation.

Let $\chi\in H$ be a solution of $(3.5)$ expressed as

where $\Phi(x, y)$ is the center manifold function of $(3.5)$ at $\delta_{0}$.

Based on the center manifold reduction, the reduced equations of $(3.5)$ on the center manifold are given by

where $G(\chi) = G(\chi, \chi)$ is the bilinear operator defined by

for $\chi = (u_1, v_1, w_1)$, $\chi_1 = (u_2, v_2, w_2)\in H_1$.

Now we are in position to solve the center manifold function $\Phi(x, y)$. By the approximation formula (B.4.1) in ^[20], the center manifold function $\Phi$ satisfy

where

here $P_2:H\rightarrow E_2$ is the canonical projection, $E_2$ is the orthogonal complement of $E_1 = span\{\xi, \eta\}$, and $\ell$ is the linearized operator of $(3.5)$.

Remark 4.1. In $(4.21)$, $o(2)$ represent $o(|(x, y)|^2)$. hereafter, we make the following convention

Direct calculation shows that

and $D_0, D_1, D_2, D_3, D_4$ are as in $(4.2)$.

By $(4.5)$, $(4.18)$ and $(4.20)$, it is clear that

Hence, $\Phi_1, \Phi_2, \Phi_3\in span\{\zeta\}$, which implies

We infer from $(4.21)$–$(4.25)$ the center manifold function as follows:

where $F_1$, $F_2$, $F_3$ are as in $(4.2)$.

Inserting $(4.26)$ into $(4.19)$, by direct circulation, we have

where $D^2 = \langle \xi, \xi^{*}\rangle^2+\langle \xi, \eta^{*}\rangle^2$.

In view of $(4.9)$, Eqs $(4.27)$ and $(4.28)$ become

where

The transition of $(3.5)$–$(3.6)$ is determined by the sign of the following number; see ^[20],

which is the same as that given by $(4.1)$. From $(4.1)$ and $(4.2)$, it is easy to show that $b_0 < 0$ in the limit of small $\rho$. Thus the proof is complete.

Second, we consider the case that $\delta_{0} > \delta_{1}$.

4.2.   Transitions from real eigenvalues

Thanks to Lemma $3.1$, for $\delta_{0} > \delta_{1}$, the transition of the system $(3.5)$ occurs at $\delta_{1}$, which is from real eigenvalues. The following theorems will show the types of transition that the system $(3.5)$ undergoes as the bifurcation parameter $\delta$ crosses the critical value $\delta_{1}$

Let $\delta_{1}$ achieve it minimum at $\rho_{k_0}$, $\rho_{k_0}$ be the eigenvalues of $(3.8)$, $e_{k_0}$ be the eigenvector of $(3.8)$ corresponding to $\rho_{k_0}$. Assume that $\beta_{k_{0}1}$ is simple near $\delta_{1}$. Hereafter, we will give different transition theorems basing on the two cases about $e_{k_0}$.

First, we consider the case where

For simplicity, let

where

and $a, b, c, d, e$ in $(4.33)$ are given by

Then, under the condition $(4.31)$, we have the second dynamic transition theorem.

Theorem 4.2. Assume that $q(\gamma-\kappa)-(1-\kappa\alpha) < 0$. Let $\delta_{0} > \delta_{1}$. If $\theta\ne 0$, then we have the following assertions:

$(1)$ The transition of $(3.5)$ at $\delta = \delta_{1}$ is mixed. More precisely, there exists a neighborhood $U\subset H_{1}$ of $\chi = 0$ such that $U$ is separated into two disjoint open sets $U_{1}$ and $U_{2}$ by the stable manifold $\Gamma$ of $\chi = 0$ satisfying

$(a)$ $U = U_{1}+U_2+\Gamma$,

$(b)$ the transition in $U_{1}$ is jump, and in $U_{2}$ is continuous.

$(2)$ The system $(3.5)$ bifurcates in $U_{2}$ to a unique singular point $\bar{\chi}$ on $\delta > \delta_{1}$, which is an attractor such that for any initial value $\varphi\in U_{2}$,

$(3)$ The system $(3.5)$ bifurcates on $\delta < \delta_{1}$ to a unique saddle point $\bar{\chi}$.

$(4)$ The bifurcated singular point $\bar{\chi}$ can be expressed as

where $\theta$ is as in $(4.32)$ and $\xi = (\xi_{1}, \xi_{2}, \xi_{3})$ are given by

Proof. We shall prove the theorem in the following two steps.

Step 1. Calculate the critical eigenvectors and decompose space.

Let $\xi$ and $\xi^{*}\in \mathbb{R}^{3}$ be the eigenvectors of $M_{k_0}$ and $M_{k_0}^{*}$ corresponding to $\beta_{k_{0}1}(\delta_{1}) = 0$, i.e.

where $M_{k_0}$ is the matrix $(3.9)$ with $k = k_{0}$. It is easy to see that $\xi$ is as in $(4.35)$, and

On the other hand, based on the spectral theory of linear completely continuous field, the spaces $H$ and $H_{1}$ can be decomposed into the following form:

where $E_{1} = span\{\xi e_{k_0}\}$, $E_{2} = E_{1}^{\bot}$. Then near $\delta_{1}$, the solution of the Eq $(3.5)$ can be expressed as

where $x\xi e_{k_0} \in E_{1}$, $z \in E_{2}$. $\psi_{kj} (j = 1, 2, 3, k\ne k_0)$ is the eigenvector corresponding to the eigenvalue $\beta_{kj}$.

Thus, in the space $E_{1}$, the Eq $(3.5)$ can be reduced to

Note that $\langle \cdot, \cdot \rangle$ denotes the inner product in $H$.

Step 2. Derivation of evolution equation.

According to the condition $(4.31)$, we do not need to consider the influence of the center manifold function. That is to say, we let $\chi = x\xi e_{k_0}$ in $(4.38)$.

Hence, we derive the following reduced bifurcation equation

For the operator $G$, we can derive that

where $\theta$ is as in $(4.32)$.

Thus, the Eq $(4.39)$ can be rewritten as

It is known that the transition of the Eq $(3.5)$ and its local topological structure are determined completely by $(4.40)$. If $\theta\ne 0$, it is clear that $(4.40)$ has exactly a bifurcated solution as follows:

Therefore,

is the bifurcated singular point of $(3.5)$.

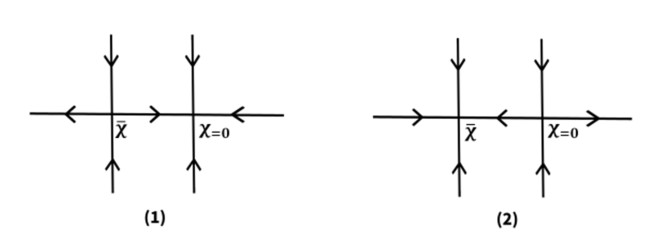
Obviously, $\bar{\chi}$ is a locally asymptotically stable singular point on $\delta > \delta_{1}$, which implies the problem $(3.5)$–$(3.6)$ has a continuous transition in $U_2$. Meanwhile, the original equilibrium state loses its stability and the problem $(3.5)$–$(3.6)$ has a jump transition in $U_1$. And $\bar{\chi}$ is an unstable saddle point on $\delta < \delta_{1}$ (see Figure 1). Thus, the Theorem is proved.

In the following, we consider the case that

Let

here

$Q_k$ is as in (4.33), $\phi = (\phi_1, \phi_2, \phi_3)$ satisfies

and the operators $L$ and $G$ are defined by

Then we have the following theorem,

Theorem 4.3. Assume that $q(\gamma-\kappa)-(1-\kappa\alpha) < 0$. Let $\tilde{\theta}\ne 0$ be the number given by $(4.43)$, and $\delta_{0} > \delta_{1}$. Then the transition of $(3.5)$ at $\delta_{1}$ is continuous as $\tilde{\theta} < 0$, and is jump as $\tilde{\theta} > 0$. Moreover, we have the following assertions:

$(1)$ When $\tilde{\theta} > 0$, the system $(3.5)$ bifurcates from $(\chi, \delta) = (0, \delta_{1})$ to two steady-state solutions $\chi_{+}$ and $\chi_{-}$ on $\delta < \delta_{1}$, which are saddles, and no bifurcation solutions on $\delta > \delta_{1}$.

$(2)$ When $\tilde{\theta} < 0$, the system $(3.5)$ bifurcates from $(\chi, \delta) = (0, \delta_{1})$ to two steady-state solutions $\chi_{+}$ and $\chi_{-}$ on $\delta > \delta_{1}$, which are attractors, and no bifurcation solutions on $\delta < \delta_{1}$.

$(3)$ The bifurcated solutions $\chi_{\pm}$ can be expressed as

where $\xi$ is as in $(4.35)$, $\beta_{k_01}$ as in Lemma $3.1$.

Proof. We shall prove the theorem in the following two steps.

Step 1.We deduce the evolution equation.

Space decomposition is the same as the step 1 in the proof of Theorem $4.2$, so we omit it.

Analogously, in the space $E_{1}$, the Eq $(3.5)$ can be reduced to

Let

and $\Phi(x)$ is the center manifold function. To evaluate the last term in $(4.46)$, we need to know the center manifold function $\Phi: E_{1}\rightarrow E_{2}$. Let $\Phi = x^{2}\phi = o(2)$, then by the approximation formula of center manifolds (see (A.10) in^[27]), $\phi$ satisfies

Direct circulation to get

Hence, we have

where $\tilde{\theta}$ is defined by $(4.43)$.

Combining $(4.46)$ and $(4.49)$, we deduce that the following reduced bifurcation equation:

Step 2. Bifurcation analysis.

Obviously, when $\tilde{\theta} > 0$, the Eq $(4.50)$ bifurcates two saddle points on $\delta < \delta_{1}$, and when $\tilde{\theta} < 0$, the Eq $(4.50)$ bifurcates two stable singular points on $\delta > \delta_{1}$. The bifurcated solutions can be expressed as

It is known that the transition and local topological structure of Eq $(3.5)$ are determined completely by $(4.50)$. Therefore,

and

are the bifurcated singular points of $(3.5)$. The stability of $\chi_{+}$ and $\chi_{-}$ are the same as that of $x_{\pm}$, see Figure 2. The Theorem is proved.

5.   Conclusions

In this work, we study the dynamical transition for a 3-component Lotka-Volterra model with diffusion from the perspective of dynamic transition recently developed by Ma and Wang ^[18,19,20]. By using the Principle of Exchange of Stabilities condition for 3-component Lotka-Volterra model, we note that the model produces stationary periodic in space patterns for $\delta < \min\{\delta_0, \delta_1\}$, where $\delta_0$ and $\delta_1$ are determined by parameters $\lambda = (\varepsilon_{1}, \varepsilon_{2}, \varepsilon_{3}, \bar{a}_{1}, \bar{a}_{2}, \alpha, \gamma, \kappa, \sigma, q)\in \mathbb{R}_{+}^{10}.$ However, when $\delta > \min\{\delta_0, \delta_1\}$, i.e., the ratio of the inter-specific competition rates and the intra-specific rates are greater than the specified value, the stability is broken. Then we obtained a few main characteristics are as follows.

First, when $\delta_0 < \delta_1$, the first eigenvalues are complex, and we show that the system undergoes a continuous or jump transition. In the small oscillation frequency limit, the transition is always continuous and the time periodic rolls are stable after the transition. That provide some sufficient conditions for the stable coexistence equilibrium and periodic solution.

Second, when $\delta_0 > \delta_1$, it was demonstrated that chaotic coexistence bifurcates from the periodic when $\int_{\Omega}e_{k_0}^3dx\neq 0$. If $\int_{\Omega}e_{k_0}^3dx = 0$, we show that the permanent coexistence was existed for the two-prey-one-predator model with intra-specific competition for predator.

Acknowledgments

Limei Li acknowledges the support from the National Science Foundation of China (NSFC) Grant No. 11701399. The authors are also grateful to the reviewer for the constructive comments.

Conflict of interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.