1.
Introduction
1.1. Problem statement
We consider a singular no-sign obstacle problem of the type
where a>1, χD is the characteristic function of D, B1⊂Rn is the unit ball and B+1=B1∩{x1>0}. The equation is considered in the weak form,
for all φ∈W1,20(B+1). This problem, when the non-negativity assumption u≥0 is imposed, is already studied in [9]. The above no-sign problem, as a general semilinear PDE with non-monotone r.h.s., introduces certain difficulties and to some extent some challenges. To explain this we shall give a very short review of the existing results and methods for similar type of problems (see also the book [6] and Caffarelli's review of the classical obstacle problem [2]). The general methodology of approaching such problems lies in using the so-called ACF-monotonicity formula (see [8]) or alternatively using John Andersson's dichotomy (see [1] or [3]). Although there are still some chances that both these methods will work for our problem above, we shall introduce a third method here which relies on a softer version of a monotonicity formula (which has a wider applicability) in combination with some elaborated analysis. We refer to this as a Weiss-type monotonicity formula, see (2.1) below.
1.2. Notation
For clarity of exposition we shall introduce some notations and definitions here that are used frequently in the paper. Throughout this paper, Rn will be equipped with the Euclidean inner product x⋅y and the induced norm |x|, Br(x0) will denote the open n-dimensional ball of center x0, radius r with the boundary ∂Br(x0). In addition, Br=Br(0) and ∂Br=∂Br(0). Rn+ stands for half space {x∈Rn:x1>0} as well as B+r=Br∩Rn+. Moreover, in the text we use the n-dimensional Hausdorff measure Hn. For a multi-index μ=(μ1,⋯,μn)∈Zn+, we denote the partial derivative with ∂μu=∂μ1x1⋯∂μnxnu and |μ|1=μ1+⋯+μn.
For a domain Ω⊂Rn+ and 1≤p<∞, we use the notation Lp(Ω) and Wm,p(Ω) as the standard spaces. However, we need some new notation for the weighted spaces
where θ∈R. For m∈N, we define the weighted Sobolev space Wm,p(Ω;xθ1) as the closure of C∞(¯Ω) with the following norm,
It is noteworthy that for θ=0, we have Lp(Ω;1)=Lp(Ω) but Wm,p(Ω;1)⊋Wm,p(Ω). Generally, the trace operator has no sense for θ>−1, while functions in W1,p(Ω;xθ1) have zero traces on {x1=0} for θ≤−1. (Theorem 6 in [7]).
1.3. Main results
We consider u∈W1,p(B+1,xθ1) for some θ<−n and n<p to be a weak solution of (1.1). This condition provides the continuity of x(θ+n)/p1u up to the boundary according to Sobolev embedding Theorem 3.1 in [5]. First, we prove the following a priori regularity result.
Proposition 1.1. (Appendix A) Let u∈W1,p(B+1,xθ1) be a solution of (1.1) for some θ<−n, n<p and f∈L∞(B+1). Then for each max{0,1+θ+np}<β<1 there exists C=C(β,n,a) such that for r≤1/2,
for all x0∈{x1=0}.
In Appendix A we will prove this proposition. Our main result in this paper concerns the optimal growth rate of solution u of (1.1) at touching free boundary points, which is stated in the following theorem.
Theorem 1.2. Suppose u∈W1,p(B+1,xθ1) is a solution of (1.1) for some θ<−n, n<p and x0∈∂{u=0}∩{x1=0}∩B+1/4. Moreover, if f∈Cα(¯B+1) for some α∈(0,1), then
for a universal constant C=C(a,n,[f]0,α).
2.
Monotonicity formula
Our main tool in proving optimal decay for solutions from the free boundary points is Weiss-monotonicity formula, combined with some elaborated techniques. We define the balanced energy functional
Considering the scaling ur,x0=ur(x)=u(rx+x0)r2, Φx0(r,u)=Φ0(1,ur). In what follows we prove almost-monotonicity of the energy.
Lemma 2.1 (Almost-Monotonicity Formula). Let u solve (1.1) and be as in Proposition 1.1 and assume that ∇u(x0)=0 for some x0∈{x1=0} and f∈Cα(¯B+1) for some α∈(0,1). Then u satisfies, for r≤r0 such that B+r0(x0)⊆B+1,
where C depends only on ‖f‖Cα(¯B+1) and the constant C(β,n,a) in Proposition 1.1.
Proof. Let ur(x):=u(rx+x0)r2, then
Note that the second integral
as |{ur=0∧∇ur≠0}|=0 and ∂rur=0 on {ur=0∧∇ur=0}. Since |∂rur|≤Crβ−2 we infer that
and conclude that
Definition 2.2. Let HP2 stand for the class of all two-homogeneous functions P∈W1,2(B+1;xa−21) satisfying div(xa1∇P)=0 in Rn+ with boundary condition P=0 on x1=0. We also define the operator Π(v,r,x0) to be the projection of vr,x0 onto HP2 with respect to the inner product
We will use the following extension of [10,Lemma 4.1].
Lemma 2.3. Assume that div(xa1∇w)=0 in B+1 with boundary condition w=0 on x1=0, and w(0)=|∇w(0)|=0. Then
and equality implies that w∈HP2, i.e., it is homogeneous of degree two.
Proof. We define an extension of the Almgren frequency,
Moreover, if N(w,r)=κ for ρ<r<σ, it implies that w is homogeneous of degree κ in Bσ∖Bρ.
Now supposing towards a contradiction that N(w,s)<2 for some s∈(0,1], and defining wr(x):=w(rx)‖w(rx)‖L2(∂B+1,xb1), we infer from N(w,s)<2 that ∇wr is bounded in L2(B+1;xa1) and so ∇wrm⇀∇w0 weakly in L2(B+1;xa1) and wrm→w0 strongly in L2(∂B+1;xa1) as a sequence rm→0. Consequently, w0 satisfies div(xa1∇w0)=0 in B+1, w0(0)=|∇w0(0)|=0 and w0=0 on x1=0 as well as ‖w0‖L2(∂B+1;xa1)=1. Furthermore, for all r∈(0,1) we have
and so w0 must be a homogeneous function of degree κ:=N(w,0+)<2. Note that for every multi-index μ∈{0}×Zn−1+, the higher order partial derivative ζ=∂μw0 satisfies the equation div(xa1∇ζ)=0 in Rn+. From the integrability and homogeneity we infer that ∂μw0≡0 for κ−|μ|1<−n2, otherwise
can not be bounded. Thus x′↦w0(x1,x′) is a polynomial, and we can write w0(x1,x′)=xκ1p(x′x1). Consider the multi-index μ such that |μ|1=degp, so ∂μw0=xκ−|μ|11∂μp is a solution of div(xa1∇ζ)=0 in Rn+. Therefore, ∂μw0∈W1,2(B+1;xθ1) for −1<θ according to Proposition A.1, which implies that 2(κ−|μ|1)+θ>−1. So, degp<κ+θ+12.
Substituting w0(x)=xκ−11(αx1+ℓ⋅x′) for κ>1 in the equation and comparing with w0(0)=|∇w0(0)|=0 we arrive at the only nonzero possible case being κ+a=2, which contradicts a>1. The case κ<1 leads to degp=0 and w0(x)=αxκ1, which implies κ+a=1 and a contradiction to a>1.
3.
Decay rate of solutions close to degenerate points
Proposition 3.1. Let f∈Cα(¯B+1) and u be solution of (1.1) satisfying the condition in Proposition 1.1. Then the function
is bounded on (0,1/8), uniformly in x0∈∂{u=0}∩{x1=0}∩B1/8.
Proof. Let us divide the proof into steps.
Step 1 We claim that there exists a constant C1<∞ such that for all x0∈∂{u=0}∩{x1=0}∩B1/8 and r≤1/8,
where ux0,r:=u(rx+x0)r2. To prove this we observe that w:=ux0,r satisfies
Moreover, for ϕ(ρ):=ρ−n−a+1∫∂B+ρxa1w(x)dHn−1 we have
If f(x0)≥18α[f]0,α then fr≥0 for r≤1/8. Therefore ϕ is increasing and ϕ(ρ)≥ϕ(0)=0 (recall that w(0)=0). Similarly, if f(x0)≤−18α[f]0,α, we obtain that ϕ(ρ)≤0. Therefore the claim is true for C1=0 in these cases.
In the case |f(x0)|≤18α[f]0,α, then |fr(x)|≤28α[f]0,α and then
So, |ϕ(ρ)|≤2−3α[f]0,αρ2 and
Step 2 We claim that there exists a constant C2<∞ such that
for every x0∈∂{u=0}∩{x1=0}∩B1/8, r≤1/8. Suppose towards a contradiction that this is not true, then there exists a sequence um, xm→ˉx and rm→0 such that
Let um:=umxm,rm and pm=Π(um,rm,xm) and wm=um−pmMm. Then, since um(0)=|∇um(0)|=0 and by the monotonicity formula and the result of previous step, we find that
Passing to a subsequence such that ∇wm⇀∇w in {\mathcal L}^2(B^+_{1};x_1^a) as m\to\infty , the compact embedding on the boundary implies that \|w_\|{ {\mathcal L}^{2}(\partial B_{1}\cap \mathbb{R}_+^n; x_1^a)} = 1 , and
and that
Since \operatorname{div}(x_1^a\nabla w_{m}) = \frac{x_1}{M_{m}}f(x^m+r\cdot)\chi_{\{u_m\neq0\}} , it follows that \operatorname{div}(x_1^a\nabla w) = 0 in B_{1}^+ . Moreover, we obtain from L^{p} -theory that w_{m}\to w in C_{\text{loc}}^{1, \alpha}(B^+_{1}) for each \alpha\in(0, 1) as m\to\infty . Consequently w(0) = \lvert\nabla w(0)\rvert = 0 . Thus we can apply Lemma 2.3 and obtain from (3.2) that w is homogeneous of degree 2, contradicting (3.3) and \|w_\|{ {\mathcal L}^{2}(\partial B_{1})} = 1. This proves the claim.
Step 3 We will show that there exists constant C_2 such that for all x^0\in\partial\{u = 0\}\cap\{x_1 = 0\} satisfying
we have
In order to see this, we can observe that
Now by condition (3.4), consider a sequence r_m \rightarrow0 such that \frac{|B_{r_m}^+(x^0)\cap\{v = 0\}| }{|B_{r_m}^+|} \rightarrow0 and assume that \nabla(u_{x^0, r_m}-p_{x^0, r_m})\rightharpoonup \nabla w in {\mathcal L}^2(B_1^+; x_1^a) as m \rightarrow\infty . Observe now \operatorname{div}(x_1^a w) = f(x^0) in B_1^+ and by similar calculation as above we will have
On the other hand,
Step 4 In this step, we prove the proposition for the points satisfying condition (3.4). For these points, we have
Thus r\mapsto\partial_{r}\left[\int_{\partial B_{1}^+}x_1^au_{x^0, r}^{2}{\mathop{}\!d} {\mathcal H}^{n-1}\right] is integrable and we obtain uniform boundedness of \int_{\partial B_{1}^+}x_1^au_{x^0, r}^{2}d {\mathcal H}^{n-1} = r^{-n-3-b}\int_{\partial B_{r}(x^0)^+}x_1^au^{2}{\mathop{}\!d} {\mathcal H}^{n-1} for all points with property (3.4). It follows that the boundedness holds uniformly on the closure of those points x^0 .
Step 5 We now consider the case
Let us assume towards a contradiction that there are sequences u^m , r_{m} and x^m such that and M_{m} = \|{u_{x^m, r_m}}\|_{ {\mathcal L}^{2}(\partial B_{1}^+)}\to+\infty as m\to\infty . Setting w_{m} = \frac{u^m_{x^m, r_{m}}}{M_{m}} we obtain, as in Step 2, that a subsequence of w_{m} converges weakly in W^{1, 2}(B_{1}^+; x_1^{a-2}) to a function w , with \|w_\|{ {\mathcal L}^{2}(\partial B_{1}^+; x_1^a)} = 1 , w(0) = \lvert\nabla w(0)\rvert = 0 , \operatorname{div}(x_1^a\nabla w) = 0 and
According to Lemma 2.3, w\in\mathbb{HP}_2 . In addition we now know that
This however contradicts the analyticity of w inside B_1^+ , knowing that \|w_\|{ {\mathcal L}^{2}(\partial B_{1};x_1^a)} = 1 .
Now we are ready to prove the main result of the article.
Proof of Theorem 1.2. From Theorem 8.17 in [4], we know that if \operatorname{div}(b(x)\nabla w) = g such that 1\leq b(x)\leq 5^a , then there exists a universal constant C = C(a, n) such that
Now for x^0 \in \partial\{u = 0\}\cap\{x_1 = 0\}\cap B_{1/8}^+ and an arbitrary point y\in \partial B_r^+(x^0) , we apply the above estimate for R = 2\delta/3 , w = (\delta/3)^au and equation \operatorname{div}\left(b(x)\nabla w\right) = x_1^af\chi_{\{u\neq0\}} , where \delta = y_1 and b(x) = \frac{x_1^a}{(\delta/3)^a} . Note that 1\leq b(x)\leq 5^a in B_{2\delta/3}(y) and
According to Proposition 3.1,
Hence,
From this theorem it follows that solutions have quadratic growth inside cones.
Corollary 3.2. Suppose u is a solution of (1.1) satisfying the condition in Proposition 1.1 and x^0\in \partial\{u = 0\}\cap\{x_1 = 0\}\cap B_{1/8}^+ . Then, for every constant \tau > 0 ,
where {\mathcal C} : = \{x: x_1\geq \tau |x-x^0|\} .
Acknowledgments
This paper was prepared while M. Fotouhi was visiting KTH Royal Institute of Technology. A. Minne was supported by the Knut and Alice Wallenberg Foundation. H. Shahgholian was supported by Swedish Research Council.
Conflict of interest
The authors declare no conflict of interest.
A.
A priori regularity of the problem
Let u be a solution of (1.1) for f\in {\mathcal L}^\infty(B_1^+) . We are going to show a priori regularity for solutions to (1.1). Consider the operator {\mathcal L}_{a, c}u: = x_1^2\Delta u+ax_1\partial_1u-cu . The following proposition is the regularity result related to this operator which has been proven by Krylov [5,Theorem 2.7,Theorem 2.8].
Proposition A.1. i) For any a\in {\mathbb R}, p > 1 and \theta\in {\mathbb R} there exists a constant c_0 > 0 such that for any c\geq c_0 the operator {\mathcal L}_{a, c} is a bounded one-to-one operator from W^{2, p}({\mathbb R}^n_+; x_1^\theta) onto {\mathcal L}^p({\mathbb R}^n_+; x_1^\theta) and its inverse is also bounded, in particular for any u\in W^{2, p}({\mathbb R}^n_+; x_1^\theta)
where C is independent of u and c .
ii) The statement in i) is valid for the operator {\mathcal L}_{a, 0} when -1 < \theta < a-2 and a > 1 or either a-2 < \theta < -1 and a < 1 .
Now we can deduce a priori regularity result for u as follows.
Proof of Proposition 1.1. Notice that x_1^{\beta-1}u\in C(\overline{B_1^+}) due to Sobolev embedding Theorem 3.1 in [5]. Then if the statement of proposition fails, there exists a sequence u_j of solutions (1.1), x^j\in \{x_1 = 0\} and r_j\rightarrow0 such that
In particular, the function \tilde u_j(x) = \frac{u_j(x^j+r_jx)}{jr_j^{1+\beta/2}} , satisfies
and with equality for R = 1 , along with
where c_0 is defined in Proposition A.1. According to (A.1), the right hand side of (A.2) is uniformly bounded in {\mathcal L}^p(B_R^+; x_1^\theta) for p(\beta-1)-1 < \theta\leq-1 . From here and Proposition A.1 we conclude that \{\tilde u_j\} is bounded in W^{2, p}(B_{R}^+; x_1^\theta) for some \theta\leq-1 and there is a convergent subsequence, tending to a function u_0 with properties
as well as the condition \theta\leq-1 insures that the trace operator is well defined and u_0 is zero on \{x_1 = 0\} . The Liouville type theorem in [9,Lemma 20]) implies that
Therefore, u_0 is a linear function, which contradicts (A.3).











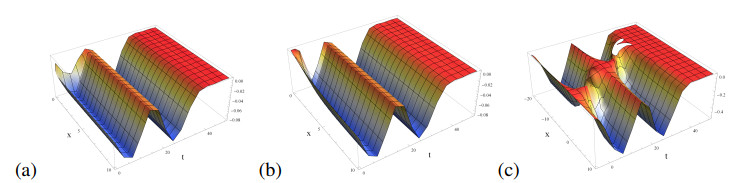
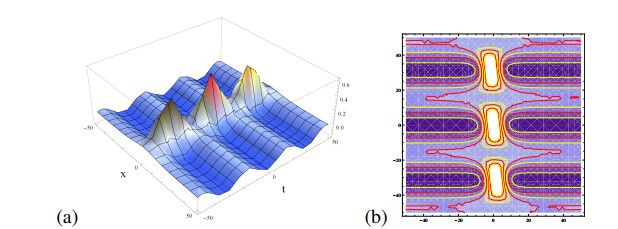
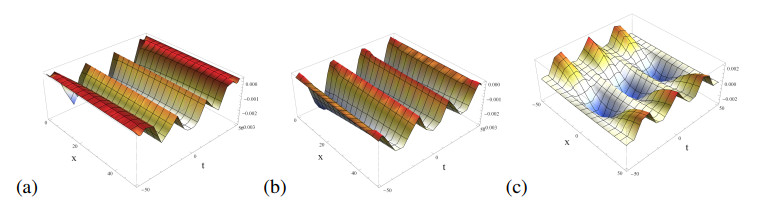

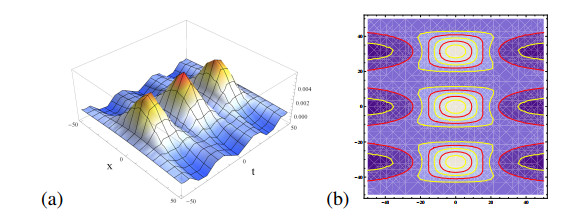


 DownLoad:
DownLoad: