Stochastic comparisons of series and parallel systems with dependent and heterogeneous Topp-Leone generated components

1.   Introduction

Order statistics play a significant role in many areas such as statistics, reliability, auction theory, risk management and many other branches of applied probability. For $n$ random variables ${X_{1}, \dots, X_{n}}$, denote $X_{k:n}$ the $k$th smallest order statistic of ${X_{1}, \dots, X_{n}}$. Then $X_{1:n}\leq\dots\leq X_{n:n}$, and $X_{k:n}$ represents the lifetime of $(n-k+1)$-out-of-$n$ system. In particularly, $X_{1:n}$ and $X_{n:n}$ respectively represent the lifetimes of series and parallel systems. Thus, to investigate the lifetime of $k$-out-of-$n$ system is equivalent to study the stochastic properties of the order statistics. For more details, one may refer to Balakrishnan and Rao ^[1], Khaledi and Kochar ^[2], Zhao and Balakrishnan ^[3] and Fang and Zhang ^[4]. In the past few decades, stochastic comparisons of order statistics have been studied by many scholars, for example, Barlow and Proschan ^[5], Bartoszewicz ^[6], Boland et al. ^[7], Kundu et al. ^[8] and Balakrishnan et al. ^[9].

In the past decades, most of the work is developed on the independent and identically distributed random variables. For comprehensive references, interested readers may refer to Kochar ^[10] and Balakrishnan and Zhao ^[11]. Further, a considerable amount of work has also been carried out comparing systems with independent heterogeneous components under specific distributions. For more on this topic, please see Dykstra et al. ^[12], Balakrishnan et al. ^[13] and Torrado ^[14]. However, the components of the system share many complex factors when the system is functioning, such as environmental conditions and work stress. For these reasons, it would be practicable to consider dependent lifetimes of components. Recently, the dependence structure of the components is investigated by researchers with the help of copula theory. Archimedean copula has been considered by many scholars due to its flexibility, for instance, Clayton copula, Ali-Mikhail-Haq copula, and Gumbel-Hougaard copula. For example, Navarro and Spizzichino ^[15] studied the stochastic ordering of series and parallel systems with components sharing a common copula. Li and Li ^[16] considered ordering properties of the smallest order statistics of Weibull samples having a common Archimedean copula. Li et al. ^[17] discussed stochastic comparisons of extreme order statistics from scaled and interdependent random variables. Fang et al. ^[18] presented conditions to stochastically compare the extreme order statistics from dependent and heterogeneous random variables. Kundu and Chowdhury ^[19] discussed the lifetimes of two series and parallel systems with location-scale components assembled with some kind of Archimedean copulas under different stochastic orders. Fang et al. ^[20] obtained various ordering results for comparing the lifetimes of the series and the parallel systems, where each component follows scale proportional hazard or reversed hazard models with Archimedean copula. For proportional hazard rate and proportional reversed hazard rate models, Li and Li ^[21] developed ordering properties of extreme order statistics from heterogeneous dependent random variables in the sense of the hazard rate and the reversed hazard rate orders.

In this manuscript, we study the stochastic comparisons of series and parallel systems having Topp-Leone generated components with different scale and shape parameters. The Topp-Leone generated family of distribution was given by Sadegh Rezaei ^[22] as a generalization of Topp and Leone's distribution. It has the property to model bathtub-shaped hazard rates depending on the values of parameters and can be used for lifetime modeling. For more applications of the distribution, one may refer to Sadegh Rezaei ^[22]. A random variable $X$ is said to be Topp-Leone generated ($TL-G$) family of distribution if its cumulative distribution function is

where $\theta$ is the scale parameter, $\alpha$ is the shape parameter, $G(x; \xi)$ is the baseline distribution function, and $\xi$ is the parameter specifying the baseline distribution, and denote $X\sim TL-G(\alpha, \theta, \xi)$. For convenience, $G(x; \xi)$ is written by $G(x)$. $TL-G(\alpha, \theta, \xi)$ is reduced to Topp and Leone's distribution when $G(x)\sim U(0, 1)$ and $\theta = 1$.

The organization of the paper is as follows. In Section 2, we present some fundamental definitions and lemmas. In Section 3, we develop the usual stochastic and the reversed hazard rate orders of series and parallel systems with dependent heterogeneous components under Archimedean copulas. Some numerical examples are provided to illustrate theoretical findings. Section 4 concludes the paper.

2.   Preliminaries

In this section, we first recall some basic definitions of some well-known notions of stochastic orders, majorization orders, and Archimedean copula, and introduce some lemmas may be used in the sequel. Denote $\mathbb{R} = (-\infty, +\infty)$ and $\mathbb{R}^{n} = (-\infty, +\infty)^{n}$.

Consider two absolutely continuous random variables $X$ and $Y$ have distribution functions $F(x)$ and $G(x)$, survival functions $\bar F(x) = 1-F(x)$ and $\bar G(x) = 1-G(x)$, density functions $f(x)$ and $g(x)$, hazard rate functions $h_{X}(x) = f(x)/\bar F(x)$ and $h_{Y}(x) = g(x)/\bar G(x)$, and reversed hazard rate functions $\tilde{\gamma}_{X}(x) = f(x)/F(x)$ and $\tilde{\gamma}_{Y}(x) = g(x)/G(x)$, respectively.

Definition 1. $X$ is smaller than $Y$ in the

(ⅰ) usual stochastic order (denoted by $X\leq_{st}Y$) if $\bar F(x)\leq\bar G(x)$ for all $x\in\mathbb{R}_{};$

(ⅱ) hazard rate order (denoted by $X\leq_{hr}Y$) if $\bar G(x)/\bar F(x)$ is increasing in $x\in\mathbb{R}_{}$, or $h_{X}(x)\geq h_{Y}(x)$ for all $x\in\mathbb{R}_{};$

(ⅲ) reversed hazard rate order (denoted by $X\leq_{rh}Y$) if $G(x)/F(x)$ is increasing in $x\in\mathbb{R}_{}$, or $\tilde{\gamma}_{X}(x)\leq\tilde{\gamma}_{Y}(x)$ for all $x\in\mathbb{R}_{}$.

$X\leq_{st}Y$ means $X$ is less likely than $Y$ to take on large values, where "large" means any value greater than $x$, and that this is the case for all $x's$. The reversed hazard rate could be understood as the probability intensity of a component survival to the last moment $t$ given that its lifetime does not exceed $t$ (cf. Yan and Luo^[23]). The hazard rate is well known and has been widely applied. As a dual concept of the hazard rate, the reversed hazard rate is far less popular and frequently used. Recently, the reversed hazard rate order has received great attention because it is more appropriate and effective than the hazard rate order for the study of some particular problems such as assessing waiting time, hidden failures, inactivity times, etc. (cf. Veres-Ferrer and Pavia^[24]).

It is well known that the hazard rate order or the reversed hazard rate order implies the usual stochastic order, and not conversely. For more detailed discussions and applications of stochastic orders, please refer to Shaked and Shanthikumar ^[25]. In addition, some scholars recently have compared order statistics under some weaker orders such as the second-order stochastic dominance, the details can be referred to Lando et al.^[26,27].

Let $x_{1:n}\leq x_{2:n}\leq \dots\leq x_{n:n}$ and $y_{1:n}\leq y_{2:n}\leq \dots\leq y_{n:n}$ be the increasing arrangements of the elements of vectors ${\bf {x}} = (x_1, \dots, x_n)$ and ${\bf {y}} = (y_1, \dots, y_n)$, respectively. In particular, ${\bf {x}}\leq {\bf {y}}$ means $x_{i}\leq y_{i},$ for all $i = 1, \dots, n$.

Definition 2. For two vectors ${\bf{x}} = (x_1, \dots, x_n)$ and ${\bf{y}} = (y_1, \dots, y_n)$, ${\bf{x}}$ is said to

(ⅰ) majorize ${\bf{y}}$ (written as ${\bf{x}}\overset{m}\succeq{\bf{y}}$) if $\sum_{i = 1}^{j}x_{i:n}\leq\sum_{i = 1}^{j}y_{i:n}, \; \; j = 1, \dots, n-1$, and $\sum_{i = 1}^{n}x_{i:n} = \sum_{i = 1}^{n}y_{i:n};$

(ⅱ) supermajorize ${\bf{y}}$ (written as ${\bf{x}}\overset{w}\succeq{\bf{y}}$) if $\sum_{i = 1}^{j}x_{i:n}\leq\sum_{i = 1}^{j}y_{i:n}, \; \; j = 1, \dots, n;$

(ⅲ) submajorize ${\bf{y}}$ (written as ${\bf {x}}\succeq_{w}{\bf {y}}$) if $\sum_{j = i}^{n}x_{j:n}\geq\sum_{j = i}^{n}y_{j:n}, \; \; i = 1, \dots, n.$

The majorization, the weak supermajorization order, and the weak submajorization order are widely used to establish various stochastic inequalities. For more details on the notions and basic properties of majorization orders, one may refer to Marshall ^[28].

The following we recall the notions of copula.

Definition 3. For a random vector $\boldsymbol X = (X_{1}, X_{2}, \dots, X_{n})$ with joint distribution function $F$, joint survival function $\bar{F}$, univariate distribution functions $F_{1}, F_{2}, \dots, F_{n}$ and univariate survival functions $\bar{F}_{1}, \dots, \bar{F}_{n}$. If there exist some function $C:[0, 1]^{n}\rightarrow[0, 1]$ and $\widehat{C}:[0, 1]^{n}\rightarrow[0, 1]$ such that, for all $x_{i}, i = 1, \dots, n$,

then $C$ and $\widehat{C}$ are called the copula and survival copula of random vector $\boldsymbol X$, respectively.

Definition 4. For a decreasing and continuous function $\psi:[0, \infty)\rightarrow[0, 1]$ such that $\psi(0) = 1$ and $\psi(+\infty) = 0,$ let $\phi = \psi^{-1}$ be its pseudo-inverse. Then,

is called an Archimedean copula with generator $\psi,$ if $(-1)^k{\psi}^{(k)}(x)\geq0$ for $k = 1, 2\dots, n-2$ and $(-1)^{n-2}{\psi}^{(n-2)}(x)$ is decreasing and convex.

For more on Archimedean copula, readers may refer to Nelsen ^[29]. For convenience, from now on, we denote

Definition 5. A function $f$ is said to be super-additive if $f(x+y)\geq f(x)+f(y)$, for all $x$ and $y$ in the domain of $f$.

Definition 6. A real-valued function $\varphi$ defined on a set $\mathbb{A}\subseteq \mathbb{R}^{n}$ is said to be Schur-convex $[$Schur-concave$]$ on $\mathbb{A}$ if ${\bf{x}}\overset{m}\succeq{\bf{y}}$ implies $\varphi({\bf{x}})\geq[\leq]\varphi({\bf{y}})$ on $\mathbb{A}$.

In the following, we present some lemmas will be used in proving the main results.

Lemma 1. (Li and Fang ^[30]. Lemma A.1) For two n-dimensional Archimedean copulas $C_{\psi_{1}}$ and $C_{\psi_{2}}$, if $\phi_{2}\circ\psi_{1}$ is super-additive, then $C_{\psi_{1}}({\bf{u}})\leq C_{\psi_{2}}({\bf{u}})$ for all ${\bf{u}} = (u_{1}, \dots, u_{n})\in [0, 1]^{n}$.

Lemma 2. (Marshall et al. ^[28]. Theorem 3.A.4) Suppose $J\subset\mathbb{R}$ is an open interval and $\phi:J^{n}\rightarrow\mathbb{R}$ is continuously differentiable. Then, $\phi$ is Schur-convex $[$Schur-concave$]$ on $J^{n}$ if and only if

(ⅰ) $\phi$ is symmetric on $J^{n};$ and

(ⅱ) for all $i\neq j$ and all ${\bf{x}}\in J^{n}$,

where $\frac{\partial\phi({\bf{x}})}{\partial x_{i}}$ represents the partial derivative of $\phi$ with respect to its $i$-th argument.

Lemma 3. (Marshall et al. ^[28]. Theorem 3.A.8) A real valued function $\phi$ on $\mathbb{R}^n,$ satisfies

if and only if $\phi$ is decreasing and Schur-convex $[$Schur-concave$]$ on $\mathbb{R}^n.$ Similarly, $\phi$ satisfies

if and only if $\phi$ is increasing and Schur-convex $[$Schur-concave$]$ on $\mathbb{R}^n.$

Lemma 4. (Das and Kayal ^[31]. Lemma 2.6) Let the function $h:(0, 1)\rightarrow(-\infty, 0)$ be defined as $h(u) = \frac{u\ln u}{1-u}.$ Then, $h(u)$ is decreasing in $u$ for all $u\in (0, 1)$.

Lemma 5. (Li and Li ^[21]. Lemma 2.3) If $\boldsymbol {\lambda}\overset{w}\preceq\boldsymbol {\mu}, \psi$ is log-concave and $(\psi\ln \psi)/\psi'$ is increasing and concave, then

for any $H(x)\in [0, 1]$, where $\mathcal{L}({\bf{u}}) = \frac{\psi'(\sum_{i = 1}^{n}u_{i})}{\psi(\sum_{i = 1}^{n}u_{i})}\sum_{i = 1}^{n}\frac{\psi(u_{i})\ln\psi(u_{i})}{\psi'(u_{i})}, u_{i}\in [0, 1], i = 1, \dots, n$ and $\phi = \psi^{-1}$ is the pseudo-inverse of $\psi.$

Throughout this paper, the terms increasing and decreasing are used for non-decreasing and non-increasing, respectively. It is also assumed that all the random variables are non-negative and absolutely continuous.

3.   Main results

In this section, we compare two system lifetimes with dependent heterogeneous components following the Topp-Leone generated distribution. The usual stochastic order and the reversed hazard rate order are obtained for series and parallel systems. Let $\boldsymbol{X} = (X_1, \dots, X_n)$ be the dependent heterogeneous TL-G distributed random vectors, we denote $\boldsymbol{X}\sim \text{TL-G}(\mathit{\boldsymbol{\alpha}}, \mathit{\boldsymbol{\theta}}, F)$, where $F$ is the baseline distribution function, $\psi_1$ is generator of the associated Archimedean copula, and $\mathit{\boldsymbol{\alpha}} = (\alpha_{1}, \dots, \alpha_{n})$ and $\mathit{\boldsymbol{\theta}} = (\theta_{1}, \dots, \theta_{n})$ are the tilt parameter vector. Similarly, denote $\boldsymbol{Y}\sim \text{TL-G}(\mathit{\boldsymbol{\beta}}, \mathit{\boldsymbol{\delta}}, G)$. For convenience, we denote the distribution(survival) function of random variable $X$ by $H_{X}(x)(\bar H_{X}(x))$. Then, the distribution functions of the parallel systems $X_{n:n}$ and $Y_{n:n}$ are given by

and

respectively. And the reliability functions of series systems $X_{1:n}$ and $Y_{1:n}$ can be written as

and

respectively, where $\phi_{1} = \psi_{1}^{-1}$ and $\phi_{2} = \psi_{2}^{-1}$.

First, we develop sufficient conditions for the usual stochastic order between parallel systems with dependent components. In the following theorem, we consider the case of the heterogeneous shape parameters and common scale parameters.

Theorem 1. Suppose that $X$ and $Y$ are two random variables with distribution functions $F$ and $G$, respectively. Define $\boldsymbol{X}\sim \mathit{\text{TL-G}}(\boldsymbol{\alpha}, \boldsymbol{\theta}, F)$ with generator $\psi_1$ and $\boldsymbol{Y}\sim \mathit{\text{TL-G}}(\boldsymbol{\beta}, \boldsymbol{\theta}, G)$ with generator $\psi_2$, such that $\boldsymbol {\alpha}, \boldsymbol {\beta}$ and $\boldsymbol{\theta}\in \epsilon_{+}$. If $X\geq_{st}Y$, $\phi_{2}\circ\psi_{1}$ is super-additive, and either $\psi_{1}$ or $\psi_{2}$ is log-convex. Then, $\boldsymbol {\alpha}\overset{w}\succeq\boldsymbol {\beta}\Rightarrow X_{n:n}\geq_{st}Y_{n:n}.$

Proof. Without loss of generality, assume that $\psi_{2}$ is log-convex, it follows from Lemma 1 that

By the decreasing property of $\psi_{2}$ and $\phi_{2}$, and note that $X\geq_{st}Y,$ we have

Combining (3.1) and (3.2), we conclude

Thus, to prove the required result, it is sufficient to show that

Denote

On differentiating (3.3) with respect to $\alpha_{i}$ ($i = 1, 2, \dots, n$), we obtain

Hence, $\varphi_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2})$ is decreasing in $\alpha_{i}$ ($i = 1, 2, \dots, n$). After simplifications, we have

Under the assumption of $\boldsymbol {\alpha}, \boldsymbol{\theta}\in \epsilon_{+}$, we have $\alpha_{i}\leq\alpha_{j}, \theta_{i}\leq\theta_{j}$ ($1\leq i\leq j\leq n$), which implies that $\big(G^{\theta_i}(2-G^{\theta_i})\big)^{\alpha_{i}}\geq\big(G^{\theta_j}(2-G^{\theta_j})\big)^{\alpha_{j}}.$ From the log-convexity of $\psi_{2}$ that holds

It is obvious that

It follows immediately from Lemma 2 that $\varphi_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2})$ is Schur-concave in $\boldsymbol{\alpha}$. Note that $\boldsymbol {\alpha}\overset{w}\succeq\boldsymbol {\beta}$, we obtain that $\varphi_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2}) \leq \varphi_2(\boldsymbol{\beta}, \boldsymbol{\theta}, G, \psi_{2})$ by Lemma 3. The proof is completed.

Remark 1. (i) Note that the super-additivity of $\phi_{2}\circ\psi_{1}$ can be roughly interpreted as follows: Kendall's $\tau$ of the copula with generator $\psi_{2}$ is larger than that with generator $\psi_{1}$ and hence is more positive dependent (refer to Li and Fang ^[30]). Theorem 1 shows that the less positive dependence, and the more heterogeneous shape parameters (in the weakly supermajorized order) lead to the stochastically large lifetime of the parallel system and higher reliability could be achieved.

(ii) It is vital to note that the super-additivity of $\phi_{2}\circ\psi_{1}(x)$ in Theorem 1 is easy to check for many well-known Archimedean copulas. For example, for the $Clayton$ copula with generator $\psi(x) = (ax+1)^{-\frac{1}{a}}$ for $a\in (0, \infty)$, it is easy to verify that $\psi(x) = -\frac{1}{a}\ln(ax+1)$ is log-convex in $x\in[0, 1].$ Let $\psi_{1}(x) = (a_{1}x+1)^{-\frac{1}{a_{1}}}$ and $\psi_{2}(x) = (a_{2}x+1)^{-\frac{2}{a_{2}}}$. It can be observed that $\phi_{2}\circ\psi_{1}(x) = ((a_{1}x+1)^{\frac{a_{2}}{a_{1}}}-1)/a_{2}$. Taking twice derivative of $\phi_{2}\circ\psi_{1}(x)$ with respect to x, it can be seen that $[\phi_{2}\circ\psi_{1}(x)]''\geq0$ for any $a_{2}\geq a_{1} > 0$, which implies the super-additivity of $\phi_{2}\circ\psi_{1}(x)$.

(iii) To display the whole curves of distribution functions of random variables defined on $[0, +\infty)$, we take the transformation $(x+1)^{-1} : [0, +\infty)\mapsto (0, 1]$ for variable $X$. Then $X\leq_{st}Y$ is equivalent to $(X+1)^{-1}\geq_{st}(Y+1)^{-1}$. Through this transformation, we can display the graphs of the CDFs in the $(0, 1]$ interval and further realize the panoramic observation of random variables.

The following example 1 illustrates the theoretical result of Theorem 1.

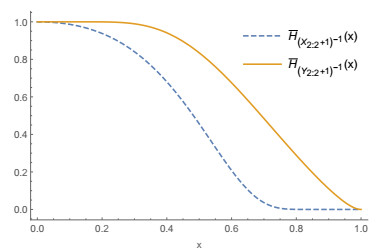
Example 1. Consider $F(x) = e^{-\frac{2}{x}}$ and $G(x) = 1-\frac{1}{1+x}, x > 0$. It is obvious that $X\geq_{st}Y$. Let $X_{i}\sim \text{TL-G}(\alpha_{i}, \theta_{i}, F)$ and $Y_{i}\sim \text{TL-G}(\beta_{i}, \delta_{i}, G), i = 1, 2$. Set $(\alpha_{1}, \alpha_{2}) = (2, 6)\overset{w}\succeq (4, 5) = (\beta_{1}, \beta_{2}), (\theta_{1}, \theta_{2}) = (0.6, 0.1) = (\delta_{1}, \delta_{2})$. We further take $\psi_{1}(x) = e^{\frac{1-e^{x}}{2}}$ and $\psi_{2}(x) = (0.1x+1)^{-\frac{1}{0.1}}, x > 0$. It is clear that $\psi_{2}$ is log-convex but $\psi_{1}$ is log-concave. We can easily show that $\phi_{2}\circ\psi_{1}(x)$ is convex in $x$ and $\phi_{2}\circ\psi_{1}(0) = 0$, and thus $\phi_{2}\circ\psi_{1}(x)$ is super-additive. As is seen in Figure 1, the survival curve of $(X_{2:2}+1)^{-1}$ beneath that of $(Y_{2:2}+1)^{-1}$ confirms $X_{2:2}(x)\geq_{st} Y_{2:2}(x)$. This validates the result in Theorem 1.

The following result provides some sufficient conditions for the usual stochastic order of series systems under the weakly submajorized order. We consider equal scale parameter vectors.

Theorem 2. Suppose that $X$ and $Y$ are two random variables with distribution functions $F$ and $G$, respectively. Define $\boldsymbol{X}\sim \mathit{\text{TL-G}}(\boldsymbol{\alpha}, \boldsymbol{\theta}, F)$ with generator $\psi_1$ and $\boldsymbol{Y}\sim \mathit{\text{TL-G}}(\boldsymbol{\beta}, \boldsymbol{\theta}, G)$ with generator $\psi_2$, such that $\boldsymbol {\alpha}, \boldsymbol {\beta}$ and $\boldsymbol{\theta} \in \epsilon_{+}$. If $X\leq_{st}Y$, and $\phi_{2}\circ\psi_{1}$ is super-additive, and either $\psi_{1}$ or $\psi_{2}$ is log-convex. Then, $\boldsymbol {\alpha}\succeq_{w}\boldsymbol {\beta}\Rightarrow X_{1:n}\leq_{st}Y_{1:n}.$

Proof. Consider the case of $\psi_{2}$ is log-convex. The super-additivity of $\phi_{2}\circ\psi_{1}$ implies that

Note that $\psi_{2}$, $\phi_{2}$ are decreasing, by $X\leq_{st}Y,$ we have

Combining (3.4) and (3.5), we get

Hence, to prove the required results, it is sufficient to show that

Denote

The partial derivative of (3.6) with respect to $\alpha_{i}$($i = 1, \dots, n$) can be given by

Thus, $\vartheta_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2})$ is increasing in $\alpha_{i}$ ($i = 1, 2, \dots, n$). We obtain

Consider that $\alpha_{i}\leq\alpha_{j}, \theta_{i}\leq\theta_{j}$ ($1\leq i\leq j\leq n$), which implies that $(G^{\theta_i}(2-G^{\theta_i}))^{\alpha_{i}}\geq(G^{\theta_j}(2-G^{\theta_j}))^{\alpha_{j}}$. It follows from log-convexity of $\psi_{2}$ that

By Lemma 4, it holds that

For convenience, denote

and

Note that $A_{1}\leq A_{2} < 0, B_{1}\leq B_{2} < 0, C_{1}\leq C_{2} < 0.$ Then, equation (3.7) is equivalent to

Thus

It follows from Lemma 2 that $\vartheta_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2})$ is Schur-concave in $\boldsymbol {\alpha}$. Based on $\boldsymbol {\alpha}\succeq_{w}\boldsymbol {\beta}$ and by Lemma 3, we conclude $\vartheta_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2}) \leq \vartheta_2(\boldsymbol{\beta}, \boldsymbol{\theta}, G, \psi_{2})$. This completes the proof.

Remark 2. In accordance with Theorem 2, for a series system, the more positive dependence, and the less heterogeneous (in the weakly subermajorized order) the shape parameters are, the stochastically longer lifetime of the system and hence higher reliability will be achieved. It should be pointed out that Theorem 2 expands Theorem 3.5 of Chanchal et al. ^[32] to the case of the heterogeneous components. It might be of great interest to study the hazard rate order between $X_{1:n}$ and $Y_{1:n}$, which is left as an open problem.

The following example 2 illustrates that the theoretical result of Theorem 2.

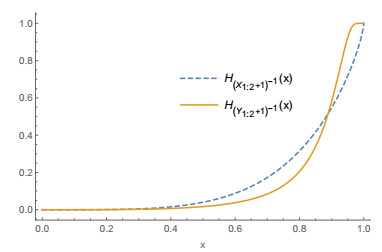
Example 2. Consider $F(x) = 1-\frac{1}{1+x}$ and $G(x) = e^{-\frac{1}{x}}, x > 0$. It is obvious that $X\leq_{st}Y$. Let $\psi_{1}(x) = e^{1-(1+x)^{5}}$ and $\psi_{2}(x) = e^{-x^{0.3}}, x > 0$, and $X_{i}\sim \text{TL-G}(\alpha_{i}, \theta_{i}, F)$ and $Y_{i}\sim \text{TL-G}(\beta_{i}, \delta_{i}, G), i = 1, 2$, and set $(\alpha_{1}, \alpha_{2}) = (2, 8)\succeq_{w} (3, 4) = (\beta_{1}, \beta_{2}), (\theta_{1}, \theta_{2}) = (1.2, 3) = (\delta_{1}, \delta_{2})$. It is easy to verify that $\psi_{2}$ is log-convex but $\psi_{1}$ is log-concave, and $\phi_{2}\circ\psi_{1}(x)$ is convex in $x$ and $\phi_{2}\circ\psi_{1}(0) = 0$, which implies $\phi_{2}\circ\psi_{1}(x)$ is super-additive. Then, the conditions of Theorem 2 are satisfied. Figure 2 plots distribution functions of $(X_{1:2}+1)^{-1}$ and $(Y_{1:2}+1)^{-1},$ from which it can be observed that $H_{(X_{1:2}+1)^{-1}}(x)$ is always smaller than $H_{(Y_{1:2}+1)^{-1}}(x)$, and this verifies $X_{1:2}\leq_{st}Y_{1:2}$.

The next theorem establishes some sufficient conditions for the usual stochastic order, here, we consider different scale parameter vectors.

Theorem 3. Suppose that $X$ and $Y$ are two random variables with distribution functions $F$ and $G$, respectively. Define $\boldsymbol{X}\sim \mathit{\text{TL-G}}(\boldsymbol{\alpha}, \boldsymbol{\theta}, F)$ with generator $\psi_1$ and $\boldsymbol{Y}\sim \mathit{\text{TL-G}}(\boldsymbol{\alpha}, \boldsymbol{\delta}, G)$ with generator $\psi_2$, such that $\boldsymbol {\theta}, \boldsymbol {\delta}$ and $\boldsymbol{\alpha} \in \epsilon_{+}$. If $X\geq_{st}Y$, $\phi_{2}\circ\psi_{1}$ is super-additive, and either $\psi_{1}$ or $\psi_{2}$ is log-convex. Then, $\boldsymbol {\theta}\overset{w}\succeq\boldsymbol {\delta}\Rightarrow X_{n:n}\geq_{st}Y_{n:n}.$

Proof. First assume that $\psi_{2}$ is log-convex. Similar to the arguments as in the proof of Theorem 1, we notice that to prove the present theorem, it is enough to show that

Denote

On differentiating (3.9) with respect to $\theta_{i}$ ($i = 1, 2, \dots, n$), we have

It follows that $\varphi_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2})$ is decreasing in $\theta_{i}$ ($i = 1, 2, \dots, n$). We further get

Further, we have $\alpha_{i}\leq\alpha_{j}, \theta_{i}\leq\theta_{j}$ ($1\leq i\leq j\leq n$). Therefore $\big(G^{\theta_i}(2-G^{\theta_i})\big)^{\alpha_{i}}\geq\big(G^{\theta_j}(2-G^{\theta_j})\big)^{\alpha_{j}}$. By the log-convexity of $\psi_{2}$, we conclude

and

For convenience, denote

and

Note that $A'_{2}\leq A'_{1} < 0, 0 < B'_{1}\leq B'_{2}, 0 < C'_{1}\leq C'_{2}$. Then, Eq (3.9) is equivalent to

Thus

Thus $\varphi_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2})$ is Schur-concave in $\boldsymbol {\theta}$. Based on $\boldsymbol {\alpha}\overset{w}\succeq\boldsymbol {\beta}$, we obtain $\varphi_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2}) \leq \varphi_2(\boldsymbol{\alpha}, \boldsymbol{\delta}, G, \psi_{2})$ by Lemma 3. The desired result is proved.

Remark 3. Theorem 3 manifests that the less positive dependence, and the more heterogeneous scale parameters (in the weakly supermajorized order) lead to the stochastically large lifetime of the parallel system and higher reliability could be achieved. It should be noted that Theorem 3 generalizes Theorem 2 in Chanchal et al. ^[32] to the case of the dependent components. It might be of great interest to establish the (reversed) hazard rate order between $X_{n:n}$ and $Y_{n:n}$ of Theorem 3, which is left as an open problem.

The following example 3 illustrates the theoretical result of Theorem 3.

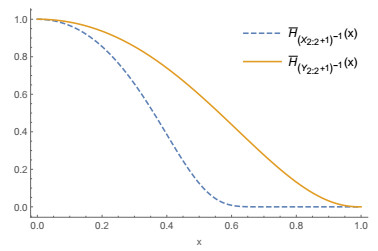
Example 3. For $F(x) = e^{-\frac{1}{x}}$ and $G(x) = 1-e^{-x}, x > 0$. It is obvious that $X\geq_{st}Y$. Let $\psi_{1}(x) = e^{1-(1+x)^{5}}$ and $\psi_{2}(x) = \frac{0.2}{e^{x}-0.8}, x > 0$, and $X_{i}\sim \text{TL-G}(\alpha_{i}, \theta_{i}, F)$ and $Y_{i}\sim \text{TL-G}(\beta_{i}, \delta_{i}, G), i = 1, 2$. Further set $(\theta_{1}, \theta_{2}) = (0.2, 0.6)\overset{w}\succeq(0.4, 0.5) = (\delta_{1}, \delta_{2})$, $(\alpha_{1}, \alpha_{2}) = (2, 3) = (\beta_{1}, \beta_{2})$. It is easy to show that $\psi_{2}$ is log-convex but $\psi_{1}$ is log-concave, and $\phi_{2}\circ\psi_{1}(x)$ is convex in $x$ and $\phi_{2}\circ\psi_{1}(0) = 0$, which implies $\phi_{2}\circ\psi_{1}(x)$ is super-additive. Then, the conditions of Theorem 3 are satisfied. Figure 3 plots these survival functions of $(X_{2:2}+1)^{-1}$ and $(Y_{2:2}+1)^{-1}$, from which it can be observed that $\bar{H}_{(X_{2:2}+1)^{-1}}(x)$ is always smaller than $\bar{H}_{(Y_{2:2}+1)^{-1}}(x)$, and this confirms that $X_{2:2}\geq_{st}Y_{2:2}$.

The theorem given below provides sufficient conditions for the usual stochastic order, here, we assume that the shape parameter vectors are the same.

Theorem 4. Suppose that $X$ and $Y$ are two random variables with distribution functions $F$ and $G$, respectively. Define $\boldsymbol{X}\sim \mathit{\text{TL-G}}(\boldsymbol{\alpha}, \boldsymbol{\theta}, F)$ with generator $\psi_1$ and $\boldsymbol{Y}\sim \mathit{\text{TL-G}}(\boldsymbol{\alpha}, \boldsymbol{\delta}, G)$ with generator $\psi_2$, such that $\boldsymbol {\theta}, \boldsymbol {\delta}$ and $\boldsymbol{\alpha} \in \epsilon_{+}$. If $X\leq_{st}Y$ and $\phi_{2}\circ\psi_{1}$ is super-additive, Then, $\boldsymbol {\theta }\leq \boldsymbol {\delta}\Rightarrow X_{1:n}\leq_{st}Y_{1:n}.$

Proof. Similar to the proof of Theorem 2, we only need to prove that

Denote

On differentiating (3.10) with respect to $\theta_{i}$ ($i = 1, \dots, n$) give rises to

Thus, $\vartheta_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2})$ is increasing in $\theta_{i}$ ($i = 1, \dots, n$). When $\boldsymbol {\theta }\leq \boldsymbol {\delta}$, we obtain that $\vartheta_2(\boldsymbol{\alpha}, \boldsymbol{\theta}, G, \psi_{2}) \leq \vartheta_2(\boldsymbol{\alpha}, \boldsymbol{\delta}, G, \psi_{2})$, hence the proof is finished.

Remark 4. It should be mentioned that the condition $\phi_{2}\circ\psi_{1}(x)$ is super-additive in Theorem 4 is quite general and easy to be constructed for many well-known Archimedean copulas. For example, consider Ali-Mikhail-Haq(AMH) copula with generator $\psi(x) = \frac{1-a}{e^{x}-a}$ for $a\in [0, 1)$, it is easy to see that $\ln\psi(x) = \ln(1-a)-\ln(e^{x}-a)$ is convex in $x\in[0, 1].$ Let $\psi_{1}(x) = \frac{1-a_{1}}{e^{x}-a_{1}}$ and $\psi_{2}(x) = \frac{1-a_{2}}{e^{x}-a_{2}}$. It can be observed that $\phi_{2}\circ\psi_{1}(x) = \ln[\frac{1-a_{2}}{1-a_{1}}(e^{x}-a_{1})+a_{2}]$. Taking derivative of $\phi_{2}\circ\psi_{1}(x)$ twice with respect to x, it can be seen that $[\phi_{2}\circ\psi_{1}(x)]''\geq0$ for $1 > a_{2} > a_{1}\geq0$, which implies the super-additivity of $\phi_{2}\circ\psi_{1}(x)$.

It is natural to ask whether the condition in Theorem 4 can be weakened? The following numerical example provides a negative answer. We show that if we take $\boldsymbol {\theta}\overset{m}\preceq\boldsymbol{\delta}$, then the result in Theorem 4 does not hold.

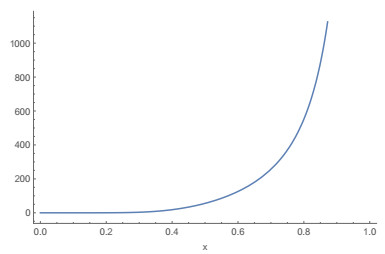
Counterexample 1. Let $X_{i}\sim \mathit{\text{TL-G}}(\alpha_{i}, \theta_{i}, F)$ and $Y_{i}\sim \mathit{\text{TL-G}}(\beta_{i}, \delta_{i}, G), i = 1, 2$. For $F(x) = 1-\frac{1}{1+x}$ and $G(x) = e^{-\frac{1}{x}}, x > 0$. It is obvious that $X\leq_{st}Y$. Further set $(\theta_{1}, \theta_{2}) = (0.4, 0.6)\overset{m}\preceq(0.1, 0.9) = (\delta_{1}, \delta_{2})$, $(\alpha_{1}, \alpha_{2}) = (2, 3) = (\beta_{1}, \beta_{2})$. Suppose we choose the Ali-Mikhail-Haq(AMH) copula with parameters $a_{1} = 0.2, a_{2} = 0.8$. We plot the graphs of the distribution functions of $(X_{1:2}+1)^{-1}$ and $(Y_{1:2}+1)^{-1}$ in Figure 4. The graphs cross each other. This implies that the usual stochastic ordering as mentioned in Theorem 4 does not hold.

Next, we switch our focus to the reversed hazard rate order between two parallel systems having dependent Topp-Leone generated distributed components. The following theorem presents sufficient conditions for the comparison of two parallel systems with the same scale parameters.

Theorem 5. Let $\boldsymbol{X}\sim \mathit{\text{TL-G}}(\boldsymbol{\alpha}, \boldsymbol{\theta}, F)$ and $\boldsymbol{Y}\sim \mathit{\text{TL-G}}(\boldsymbol{\beta}, \boldsymbol{\theta}, F)$ have the common generator $\psi$, such that $\boldsymbol {\alpha}, \boldsymbol {\beta}$ and $\boldsymbol{\theta}\in \epsilon_{+}$. If $\psi$ is log-concave, and $(\psi\ln \psi)/\psi'$ is increasing and concave. Then $\boldsymbol {\alpha}\overset{w}\preceq\boldsymbol {\beta}\Rightarrow X_{n:n}\geq_{rh}Y_{n:n}.$

Proof. The reversed hazard rate function of parallel system $X_{n:n}$ is

Denote

then $\tilde{\gamma}_{X_{n:n}}(x)$ can be represented as

Similarly, we have

Notice that for $\theta > 0, F^{\theta}(2-F^{\theta})\in [0, 1]$. Since $\psi$ is log-concave, $\boldsymbol {\alpha}\overset{w}\preceq\boldsymbol {\beta}$, and note that $(\psi\ln\psi)/\psi'$ is increasing concave. It follows from Lemma 5 that

$\tilde{\gamma}_{X_{n:n}}(x)\geq \tilde{\gamma}_{Y_{n:n}}(x)$ holds immediately from $(1-F^{\theta})/(2-F^{\theta})\geq 0$ and $\ln(F^{\theta}(2-F^{\theta}))\leq 0$. The proof is completed.

Remark 5. Theorem 5 states that less heterogeneous shape parameters in the weakly supermajorized order lead to a large lifetime of the parallel system in the sense of the reversed hazard rate order. It might be of great interest to study the likelihood ratio order between $X_{n:n}$ and $Y_{n:n}$, which is left as an open problem.

The following example 4 illustrates that the theoretical results of Theorem 5.

Example 4. Let $\psi(x) = e^{4-4e^{x}}, x > 0$. It is obvious that $\psi(x)$ is log-concave in $x > 0$, and $(\psi(x)\ln \psi(x))/\psi'(x) = (e^{x}-1)/e^{x}$ is increasing concave. Assume $X_{i}\sim \text{TL-G}(\alpha_{i}, \theta_{i}, F)$ and $Y_{i}\sim \text{TL-G}(\beta_{i}, \theta_{i}, F), i = 1, 2$, $F(x) = [1-e^{-x}]^{0.5}, x > 0$. Further set $(\alpha_{1}, \alpha_{2}) = (4, 5)\overset{w}\preceq \; (2, 6) = (\beta_{1}, \beta_{2})$, $(\theta_{1}, \theta_{2}) = (2, 3)$. The difference between the hazard rate functions of $(X_{2:2}+1)^{-1}$ and $(Y_{2:2}+1)^{-1}$ is plotted in Figure 5, from which one can observe that $h_{(X_{2:2}+1)^{-1}}(x)$ is always larger than $h_{(Y_{2:2}+1)^{-1}}(x)$, and this confirms that $X_{2:2}\geq_{rh}Y_{2:2}$.

4.   Conclusion

In this paper, we study stochastic comparisons of series and parallel systems with heterogeneous Topp-Leone generated components with Archimedean (survival) copulas. We established the usual stochastic order of the series and parallel systems, and the reversed hazard rate order of the parallel system. These results generalize some existing results in the literature (see Chanchal et al. ^[32]) to the case of dependent components in the $TL$-$G$ family.

Acknowledgments

The authors thank the editor and two anonymous reviewers for their insightful and valuable comments, which significantly helped in improving the presentation of this paper. This research was supported by the National Natural Science Foundation of China (11861058, 71471148).

Conflict of interest

The authors declare no conflict of interest.