The fractional-order unified chaotic system: A general cascade synchronization method and application

Hongli An; Dali Feng; Li Sun; Haixing Zhu; Hongli An; Dali Feng; Li Sun; Haixing Zhu

doi:10.3934/math.2020277

AIMS Mathematics

2020, Volume 5, Issue 5: 4345-4356. doi: 10.3934/math.2020277

Previous Article Next Article

Research article

The fractional-order unified chaotic system: A general cascade synchronization method and application

1.
College of Sciences, Nanjing Agricultural University, Nanjing 210095, PRC
2.
College of Economics and Management, Nanjing Forestry University, Nanjing 210037, PRC

Received: 23 December 2019 Accepted: 26 April 2020 Published: 09 May 2020
MSC : 34D06, 34H10

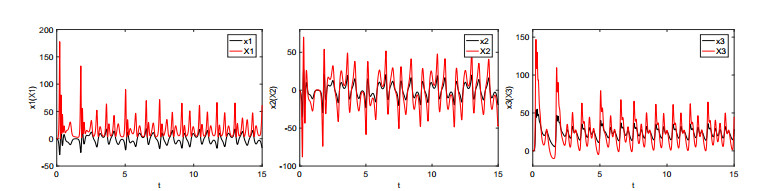
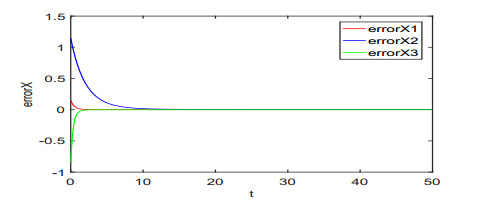
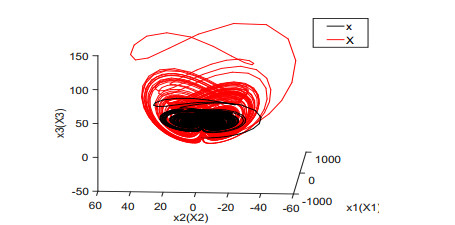
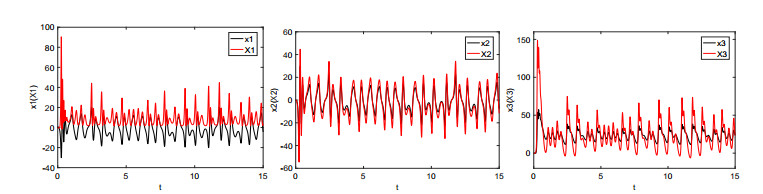
In this paper, we extend the cascade synchronization of integer-order chaotic systems to function cascade synchronization of fractional-order chaotic systems. The nice feature of our method is that a fractional-order chaotic system will synchronize to another system via a scaling function. The rich choices of the scaling function turn our method more general than some existing methods. As applications, we take a fractional-order unified chaotic system as an illustrative example to test the effectiveness.

Keywords:

Citation: Hongli An, Dali Feng, Li Sun, Haixing Zhu. The fractional-order unified chaotic system: A general cascade synchronization method and application[J]. AIMS Mathematics, 2020, 5(5): 4345-4356. doi: 10.3934/math.2020277

Related Papers:

[1]	Alessandro P. Delitala . Autoimmunity in latent autoimmune diabetes in adults. AIMS Medical Science, 2019, 6(2): 132-139. doi: 10.3934/medsci.2019.2.132
[2]	Melissa R. Bowman Foster, Ali Atef Hijazi, Raymond C. Sullivan Jr, Rebecca Opoku . Hydroxyurea and pyridostigmine repurposed for treating Covid-19 multi-systems dysfunctions. AIMS Medical Science, 2023, 10(2): 118-129. doi: 10.3934/medsci.2023010
[3]	Louis Papageorgiou, Dimitrios Vlachakis . Antisoma Application: A Fully Integrated V-Like Antibodies Platform. AIMS Medical Science, 2017, 4(4): 382-394. doi: 10.3934/medsci.2017.4.382
[4]	Teruo Sekimoto, Takamasa Tanaka, Tatsuya Shiraki, Renu Virmani, Aloke V. Finn . How does atherosclerotic plaque become calcified, and why?. AIMS Medical Science, 2024, 11(4): 421-438. doi: 10.3934/medsci.2024029
[5]	Michiro Muraki . Sensitization to cell death induced by soluble Fas ligand and agonistic antibodies with exogenous agents: A review. AIMS Medical Science, 2020, 7(3): 122-203. doi: 10.3934/medsci.2020011
[6]	Gasim Dobie, Sarah Abutalib, Wafa Sadifi, Mada Jahfali, Bayan Alghamdi, Asmaa Khormi, Taibah Alharbi, Munyah Zaqan, Zahra M Baalous, Abdulrahim R Hakami, Mohammed H Nahari, Abdullah A Mobarki, Muhammad Saboor, Mohammad S Akhter, Abdullah Hamadi, Denise E Jackson, Hassan A Hamali . The correlation between severe complications and blood group types in COVID-19 patients; with possible role of T polyagglutination in promoting thrombotic tendencies. AIMS Medical Science, 2023, 10(1): 1-13. doi: 10.3934/medsci.2023001
[7]	Rena Rahimova . Relationship between CTLA4, TNF-α and PTPN22 gene polymorphism and the serum levels of antithyroglobulin and antiperoxidase antibodies in autoimmune thyroiditis. AIMS Medical Science, 2023, 10(1): 14-23. doi: 10.3934/medsci.2023002
[8]	Kwon Yong, Martin Brechbiel . Application of ²¹²Pb for Targeted α-particle Therapy (TAT): Pre-clinical and Mechanistic Understanding through to Clinical Translation. AIMS Medical Science, 2015, 2(3): 228-245. doi: 10.3934/medsci.2015.3.228
[9]	Ilana S. Golub, Angela Misic, Lucia P. Schroeder, Jairo Aldana-Bitar, Srikanth Krishnan, Sina Kianoush, Travis Benzing, Keishi Ichikawa, Matthew J. Budoff . Calcific coronary lesions: management, challenges, and a comprehensive review. AIMS Medical Science, 2024, 11(3): 292-317. doi: 10.3934/medsci.2024021
[10]	K.E. Khalid, Hamdi Nouri Nsairat, Jingwu Z. Zhang . The Presence of Interleukin 18 Binding Protein Isoforms in Chinese Patients with Rheumatoid Arthritis. AIMS Medical Science, 2016, 3(1): 103-113. doi: 10.3934/medsci.2016.1.103

Abstract

1. Introduction

We study the following first-order coupled nonlinear system,

$\begin{equation} \left\{ \begin{array}{c} z^{\prime }(t) = f\left( t, z(t), w(t)\right), \\ w^{\prime }(t) = g\left( t, z(t), w(t)\right), \end{array} \right. \end{equation}$

(1.1)

with $t\in \left[0, T\right]$ $(T > 0)$ and $f, g:\left[0, T\right] \times \mathbb{R}^{2}\rightarrow \mathbb{R}$ $L^{1}$ -Carathéodory functions, together with the boundary conditions

$\begin{equation} \begin{array}{c} z(0) = z(T), \\ w(0) = w(T). \end{array} \end{equation}$

(1.2)

The motivation for the study of systems (1.1) and (1.2) arise from the application of nonlinear models to several phenomena in different domains of life, namely population dynamics ^[1], neuroscience ^[2], biological systems ^[3], ecology ^[4], electronic circuits ^[5], celestial mechanics ^[6], among others.

Periodic solutions are usually found in differential equations. For example:

In ^[7], the authors present sufficient conditions for the solvability of a class of singular Sturm-Liouville equations with periodic boundary value conditions. The problem describes the periodic oscillations of the axis of a satellite in the plane of the elliptic orbit around its center of mass.

In ^[8], the authors study the stability of two coupled Andronov oscillators sharing a mutual discrete periodic interaction. The study was motivated by the phenomenon of synchronization observed in Huygens' clocks ^[9].

In ^[10], the authors prove the existence of positive periodic solutions in a generalized Nicholson's blowflies model, using Krasnoselskii cone fixed point theorem.

In ^[11], it is considered the dynamics of hematopoietic stem cell populations under some external periodic regulatory factors at the cellular cycle level. The existence of periodic solutions in the respective nonautonomous differential system is typically associated to the presence of some hematological disease.

However, nonlinear systems with non-oscillatory nonlinearities can hamper the search for periodic solutions.

To overcome that difficulty, in ^[12] the authors use well-ordered lower and upper solutions to guarantee the existence of solutions of a first-order fully coupled system. In ^[13], the authors introduce the idea of well-ordered coupled lower and upper solutions, to obtain an existence and localization result for periodic solutions of first-order coupled non-linear systems of $n$ ordinary differential equations.

Motivated by these works, in this paper we present some novelties: A new translation technique to deal with lower and upper solutions, not necessarily well-ordered; A method to provide sufficient conditions to obtain existence and localization results, combining the adequate coupled lower and upper solutions definition with the monotonicity of the nonlinearities.

This work is organized as follows. In Section 2 we present the definitions and the solvability conditions for problems (1.1) and (1.2). In Section 3, we present the main results regarding the existence and localization of solutions for the problem, using the lower and upper solutions technique, together with Schauder's fixed point theorem, and a numerical example. In Section 4 we adapt the solvability conditions of Section 2 to the lack of monotonicity criteria in the nonlinearities. We find coupled lower and upper solutions for the original problem. Finally, in Section 5, we present an application to a real case scenario of population dynamics.

2. Definitions

In this work we consider the space of continuous functions in $[0, T],$ $\left(C[0, T]\right)^2,$ with the norm $\left\Vert (z, w) \right\Vert = \max \left\{ \left\Vert z \right\Vert, \left\Vert w \right\Vert \right\}$ , with $\left\Vert u \right\Vert = \max_{t\in \lbrack 0, T]}\left\vert u (t) \right\vert$ .

So, $(C[0, T])^2$ is a Banach space.

The nonlinearities assumed will be $L^{1}-$ Carathéodory functions, that is:

Definition 2.1. The function $h:[0, T]\times \mathbb{R}^{2}\rightarrow \mathbb{R},$ is a $L^{1}-$ Carathéodory if it verifies

$(i)$ for each $\left(y_{1}, y_{2}\right) \in \mathbb{R}^{2}$ , $t\mapsto h(t, y_{1}, y_{2})$ is measurable in $[0, T];$

$(ii)$ for almost every $t\in \lbrack 0, T],$ $\left(y_{1}, y_{2}\right) \mapsto h(t, y_{1}, y_{2})$ is continuous in $\mathbb{R} ^{2};$

$(iii)$ for each $L > 0$ , there exists a positive function $\psi _{L}\in L^{1}[0, T],$ such that, for $\max \left\{ \left\Vert y_{i}\right\Vert, i = 1, 2\right\} < L$ ,

$\begin{equation} \left\vert h(t, y_{1}, y_{2})\right\vert \leq \psi _{L}(t), \text{ }a.e.\text{ } t\in \lbrack 0, T]. \end{equation}$

(2.1)

A key argument in this work is the lower and upper solutions method, but not necessarily well-ordered as it is standard in the literature. This issue is overcome by a new type of lower and upper solutions' definition with some adequate translations:

Definition 2.2. Consider $C^{1}$ -functions $\alpha _{i}, \beta _{i}:[0, T]\rightarrow \mathbb{R},$ $i = 1, 2.$ The functions $\left(\alpha _{1}, \alpha _{2}\right)$ are lower solutions of the periodic problems (1.1) and (1.2) if

$\begin{equation} \left\{ \begin{array}{c} \alpha _{1}^{\prime }(t)\leq f\left( t, \alpha _{1}^{0}(t), \alpha _{2}^{0}(t)\right), \\ \alpha _{2}^{\prime }(t)\leq g\left( t, \alpha _{1}^{0}(t), \alpha _{2}^{0}(t)\right), \end{array} \right. \end{equation}$

(2.2)

with

$\begin{equation} \alpha _{i}^{0}(t): = \alpha _{i}(t)-\left\Vert \alpha _{i}\right\Vert , \text{ }i = 1, 2, \end{equation}$

(2.3)

and

$\begin{equation} \alpha _{i}(0)\leq \alpha _{i}(T), \text{ }i = 1, 2. \end{equation}$

(2.4)

The functions $\left(\beta _{1}, \beta _{2}\right)$ are upper solutions of the periodic problems (1.1) and (1.2) if

$\begin{equation*} \left\{ \begin{array}{c} \beta _{1}^{\prime }(t)\geq f\left( t, \beta _{1}^{0}(t), \beta _{2}^{0}(t)\right), \\ \beta _{2}^{\prime }(t)\geq g\left( t, \beta _{1}^{0}(t), \beta _{2}^{0}(t)\right), \end{array} \right. \label{eq: beta_i '} \end{equation*}$

with

$\begin{equation} \beta _{i}^{0}(t): = \beta _{i}(t)+\left\Vert \beta _{i}\right\Vert , \text{ } i = 1, 2, \end{equation}$

(2.5)

and

$\begin{equation*} \beta _{i}(0)\geq \beta _{i}(T), \text{ }i = 1, 2. \end{equation*}$

By solution we mean $(C^1[0, T])^2$ functions verifying (1.1) and (1.2). The existence tool is the Schauder's fixed-point theorem (Theorem 2.A of ^[14]):

Theorem 2.1. Let $Y$ be a nonempty, closed, bounded and convex subset of a Banach space $X$ , and suppose that $P:Y\rightarrow Y$ is a compact operator. Then $P$ has at least one fixed point in $Y$ .

3. Existence and localization theorem

This method allows to obtain an existence and localization result for periodic non-trivial solutions in presence of well ordered lower and upper solutions:

Theorem 3.1. Let $\left(\alpha _{1}, \alpha _{2}\right)$ and $\left(\beta _{1}, \beta _{2}\right)$ be lower and upper solutions of (1.1) and (1.2), respectively $.$ Assume that $f$ is a $L^{1}$ -Carathéodory function in the set

$\begin{equation*} \left\{ \left( t, y_{1}, y_2\right) \in \lbrack 0, T]\times \mathbb{R}^2 :\alpha _{1}^{0}(t)\leq y_{1}\leq \beta _{1}^{0}(t)\right\} , \end{equation*}$

$g$ is a $L^{1}$ -Carathéodory function in

$\begin{equation*} \left\{ \left( t, y_1, y_{2}\right) \in \lbrack 0, T]\times \mathbb{R}^2 :\alpha _{2}^{0}(t)\leq y_{2}\leq \beta _{2}^{0}(t)\right\} , \end{equation*}$

with

$\begin{equation} f\left( t, y_{1}, y_{2}\right) \ \mathit{\text{nondecreasing in}}\ y_{2}, \end{equation}$

(3.1)

for fixed $t\in \lbrack 0, T]$ and $y_{1}\in \mathbb{R}$ , and

$\begin{equation*} g\left( t, y_{1}, y_{2}\right) \ \mathit{\text{nondecreasing in}}\ y_{1}, \end{equation*}$

for fixed $t\in \lbrack 0, T]$ and $y_{2}\in \mathbb{R}$ . Then problems (1.1) and (1.2) has, at least, a solution $(z, w)\in \left(C^1[0, T]\right) ^{2}$ such that

$\begin{eqnarray*} \alpha _{1}^{0}(t) &\leq &z(t)\leq \beta _{1}^{0}(t), \\ \alpha _{2}^{0}(t) &\leq &w(t)\leq \beta _{2}^{0}(t), \ \mathit{\text{for all}}\ t\in \lbrack 0, T]. \end{eqnarray*}$

Proof. For $i = 1, 2,$ define the continuous functions $\delta_i^0:[0, T]\times \mathbb{R} \rightarrow \mathbb{R}$ , given by

$\begin{equation} \delta _{1}^{0}(t, z) = \left\{ \begin{array}{ccc} \alpha _{1}^{0}(t) & \text{if} & z < \alpha _{1}^{0}(t), \\ z & \text{if} & \alpha _{1}^{0}(t)\leq z\leq \beta _{1}^{0}(t), \\ \beta _{1}^{0}(t) & \text{if} & z > \beta _{1}^{0}(t), \end{array} \right. \end{equation}$

(3.2)

and

$\begin{equation*} \delta _{2}^{0}(t, w) = \left\{ \begin{array}{ccc} \alpha _{2}^{0}(t) & \text{if} & w < \alpha _{2}^{0}(t), \\ w & \text{if} & \alpha _{2}^{0}(t)\leq w\leq \beta _{2}^{0}(t), \\ \beta _{2}^{0}(t) & \text{if} & w > \beta _{2}^{0}(t), \end{array} \right. \end{equation*}$

and consider the auxiliary problem composed by

$\begin{equation} \left\{ \begin{array}{c} z^{\prime }(t)+z(t) = f\left( t, \delta _{1}^{0}(t, z), \delta _{2}^{0}(t, w)\right) +\delta _{1}^{0}(t, z), \\ w^{\prime }(t)+w(t) = g\left( t, \delta _{1}^{0}(t, z), \delta _{2}^{0}(t, w)\right) +\delta _{2}^{0}(t, w), \end{array} \right. \end{equation}$

(3.3)

together with the periodic boundary conditions (1.2).

Step 1. Integral form of (3.3) and (1.2).

The general solution of (3.3) and (1.2) is

$\begin{equation*} \left\{ \begin{array}{c} z(t) = e^{-t}\Big (C_z + \int_{0}^{t}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds\Big ), \\ \\ w(t) = e^{-t}\Big (C_w + \int_{0}^{t}e^{s}q_{2}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds\Big ), \\ \end{array} \right. \end{equation*}$

with

$\begin{equation} \begin{array}{c} q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0}): = f(s, \delta _{1}^{0}(s, z(s)), \delta _{2}^{0}(s, w(s)))+\delta _{1}^{0}(s, z(s)), \\ q_{2}(s, \delta _{1}^{0}, \delta _{2}^{0}): = g(s, \delta _{1}^{0}(s, z(s)), \delta _{2}^{0}(s, w(s)))+\delta _{2}^{0}(s, w(s)). \end{array} \end{equation}$

(3.4)

and, by (1.2),

$\begin{equation*} \left\{ \begin{array}{c} C_z = \frac{e^{-T}}{1-e^{-T}}\int_{0}^{T}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds, \\ C_w = \frac{e^{-T}}{1-e^{-T}}\int_{0}^{T}e^{s}q_{2}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds.\\ \end{array} \right. \end{equation*}$

Therefore, the integral form of (3.3) and (1.2) is

$\begin{equation} \left\{ \begin{array}{c} z(t) = e^{-t}\Big (\frac{e^{-T}}{1-e^{-T}}\int_{0}^{T}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds+\int_{0}^{t}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds\Big ), \\ \\ w(t) = e^{-t}\Big (\frac{e^{-T}}{1-e^{-T}}\int_{0}^{T}e^{s}q_{2}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds+\int_{0}^{t}e^{s}q_{2}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds\Big ).\\ \end{array} \right. \end{equation}$

(3.5)

Define the operator $T:(C[0, T])^{2}\rightarrow (C[0, T])^{2}$ such that

$\begin{equation} T(z, w)(t) = (T_{1}(z, w)(t), T_{2}(z, w)(t)), \end{equation}$

(3.6)

with

$\begin{equation} \left\{ \begin{array}{c} T_{1}(z, w)(t) = e^{-t}\Big (\frac{e^{-T}}{1-e^{-T}}\int_{0}^{T}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds+\int_{0}^{t}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds\Big ), \\ \\ T_{2}(z, w)(t) = e^{-t}\Big (\frac{e^{-T}}{1-e^{-T}}\int_{0}^{T}e^{s}q_{2}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds+\int_{0}^{t}e^{s}q_{2}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds\Big ). \\ \end{array} \right. \end{equation}$

(3.7)

Following Definition 2.1, the norm of the operator $T$ , defined in (3.6), is given by

$\begin{equation} \begin{split} & \lVert T(z, w)\rVert = \max\limits_{i = 1, 2}\big\{\lVert T_{1}(z, w)\rVert , \;\lVert T_{2}(z, w)\rVert \big\} = \max \big \{\max\limits_{t\in \lbrack 0, T]}\lvert T_{1}(z(t), w(t))\rvert , \;\max\limits_{t\in \lbrack 0, T]}\lvert T_{2}(z(t), w(t))\rvert \big \}. \end{split} \end{equation}$

(3.8)

Step 2. $T$ has a fixed point.

The conditions for Theorem 2.1 require the existence of a nonempty, bounded, closed and convex subset $D\subset \left(C\left[0, T \right] \right) ^{2}$ such that $TD\subset D$ .

As $f,$ $g$ are $L^{1}$ -Carathéodory functions, by Definition 2.1, there are positive $L^{1}[0, T]$ functions $\psi _{iL}, i = 1, 2$ , such that

$\begin{equation} \begin{array}{c} \lvert f(t, \delta _{1}^{0}(t, z), \delta _{2}^{0}(t, w))\rvert \leq \psi _{1L}(t), \\ \lvert g(t, \delta _{1}^{0}(t, z), \delta _{2}^{0}(t, w))\rvert \leq \psi _{2L}(t), \end{array} \quad a.e.\;t\in \lbrack 0, T], \end{equation}$

(3.9)

with

$\begin{equation} L: = \max \{|\alpha _{1}^{0}(t)|, |\alpha _{2}^{0}(t)|, \beta _{1}^{0}(t), \beta _{2}^{0}(t)\}. \end{equation}$

(3.10)

Consider the closed ball of radius $K$ ,

$\begin{equation} D: = \{(z, w)\in (C[0, T])^{2}:\lVert (z, w)\rVert \leq K\}, \end{equation}$

(3.11)

with $K$ given by

$\begin{equation} K = \max \Bigg\{ \begin{array}{c} \frac{e^{T}}{1-e^{-T}}\Big(\int_{0}^{T}\psi _{1L}(s)\, ds+LT\Big), \frac{e^{T}}{1-e^{-T}}\Big(\int_{0}^{T}\psi _{2L}(s)\, ds+LT\Big) \end{array} \Bigg\}. \end{equation}$

(3.12)

For $t\in \lbrack 0, T]$ ,

$\begin{equation} \begin{split} \lvert & T_{1}(z, w)(t)\rvert = \bigg\lvert e^{-t}\Big (\frac{e^{-T}}{1-e^{-T}} \int_{0}^{T}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds+\int_{0}^{t}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds \Big )\bigg\rvert \\ & \qquad \qquad \leq \frac{e^{-T}}{1-e^{-T}}\int_{0}^{T}|e^{s}|\;|q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})|\, ds+\int_{0}^{t}|e^{s}|\;|q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})|\, ds \\ & \qquad \qquad \leq \frac{e^{T}}{1-e^{-T}}\int_{0}^{T}|q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})|\, ds \\ & \qquad \qquad \leq \frac{e^{T}}{1-e^{-T}}\int_{0}^{T}(|f(s, \delta _{1}^{0}, \delta _{2}^{0})|+L)\, ds \\ & \qquad \qquad = \frac{e^{T}}{1-e^{-T}}\Big(\int_{0}^{T}|f(s, \delta _{1}^{0}, \delta _{2}^{0})|\, ds+LT\Big) \\ & \qquad \qquad \leq \frac{e^{T}}{1-e^{-T}}\Big(\int_{0}^{T}\psi _{1L}(s)\, ds+LT\Big)\leq K. \end{split} \end{equation}$

(3.13)

Similarly,

$\begin{equation} \lvert T_{2}(z, w)(t)\rvert < \frac{e^{T}}{1-e^{-T}}\Big(\int_{0}^{T}\psi _{2L}(s)\, ds+LT\Big)\leq K, \quad \forall t\in \lbrack 0, T]. \end{equation}$

(3.14)

Since $T_{1}$ and $T_{2}$ are uniformly bounded, so is $T$ .

Consider $t_{1}, t_{2}\in \lbrack 0, T]$ and $t_{1} < t_{2}$ , without loss of generality. Then,

$\begin{equation} \begin{split} \big\lvert & T_{1}(z, w)(t_{1})-T_{1}(z, w)(t_{2})\big\rvert \\ & = \Bigg\lvert e^{-t_{1}}\Big (\frac{e^{-T}}{1-e^{-T}} \int_{0}^{T}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds+\int_{0}^{t_{1}}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds\Big ) \\ & \qquad \qquad -e^{-t_{2}}\Big (\frac{e^{-T}}{1-e^{-T}} \int_{0}^{T}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds+\int_{0}^{t_{2}}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds\Big )\Bigg\rvert \\ & = \Bigg\lvert\big(e^{-t_{1}}-e^{-t_{2}}\big)\Big (\frac{e^{-T}}{1-e^{-T}} \int_{0}^{T}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds+\int_{0}^{t_{1}}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds\Big ) \\ & \qquad \qquad -e^{-t_{2}}\int_{t_{1}}^{t_{2}}e^{s}q_{1}(s, \delta _{1}^{0}, \delta _{2}^{0})\, ds\Bigg\rvert\xrightarrow[t_1\rightarrow t_2]{}0, \end{split} \end{equation}$

(3.15)

proving that $T_{1}$ is equicontinuous.

In the same way, it can be proved that $\big\lvert T_{2}(z(t_{1}), w(t_{1}))-T_{2}(z(t_{2}), w(t_{2}))\big\rvert \xrightarrow[t_1 \rightarrow t_2]{}0.$

Therefore, $T$ is equicontinuous.

By (3.12), it is clear that $TD\subset D$ , then, by Schauder's Fixed Point Theorem, $T$ has a fixed point $(z^{\ast }(t), w^{\ast }(t))\in (C[0, T])^{2}$ , which is a solution of (3.3) and (1.2).

Step 3. The pair $(z^{\ast }(t), w^{\ast }(t))$ , solutions of (3.3) and (1.2), is a solution of the initial problems (1.1) and (1.2).

To prove that $(z^{\ast }, w^{\ast })\in (C[0, T])^{2}$ is a solution of the original problem (1.1), (1.2), it is enough to prove that

$\begin{equation} \alpha _{1}^{0}(t)\leq z^{\ast }(t)\leq \beta _{1}^{0}(t), \quad \alpha _{2}^{0}(t)\leq w^{\ast }(t)\leq \beta _{2}^{0}(t), \quad \forall t\in \lbrack 0, T]. \end{equation}$

(3.16)

In the first case, suppose, by contradiction, that there exists some $t\in \lbrack 0, T]$ such that

$\begin{equation*} z^{\ast }(t) < \alpha _{1}^{0}(t), \end{equation*}$

and define

$\begin{equation} \min\limits_{t\in \lbrack 0, T]}(z^{\ast }-\alpha _{1}^{0})(t): = z^{\ast }(t_{0})-\alpha _{1}^{0}(t_{0}) < 0. \end{equation}$

(3.17)

If $t_{0}\in]0, T[$ , then

$\begin{equation} (z^{\ast })^{\prime }(t_{0}) = (\alpha _{1}^{0})^{\prime }(t_{0}), \end{equation}$

(3.18)

and, by (3.17) and (3.1) and Definition 2.2, the following contradiction with (3.18) holds:

$\begin{equation*} \begin{split} (z^{\ast })^{\prime }(t_{0})& = f(t_{0}, \delta _{1}^{0}(t_{0}, z^{\ast }(t_{0})), \delta _{2}^{0}(t_{0}, w(t_{0})))+\delta _{1}^{0}(t_{0}, z^{\ast }(t_{0}))-z^{\ast }(t_{0}) \\ & = f(t_{0}, \alpha _{1}^{0}(t_{0}), \delta _{2}^{0}(t_{0}, w(t_{0})))+\alpha _{1}^{0}(t_{0})-z^{\ast }(t_{0}) \\ & > f(t_{0}, \alpha _{1}^{0}(t_{0}), \delta _{2}^{0}(t_{0}, w(t_{0}))) \\ & \geq f(t_{0}, \alpha _{1}^{0}(t_{0}), \alpha _{2}^{0}(t_{0})) \\ & \geq {\alpha _{1}}^{\prime }(t_{0}). \end{split} \end{equation*}$

If $t_{0} = 0$ , then, by (1.2) and Definition 2.2,

$\begin{equation*} \begin{split} z^{\ast }(0)-\alpha _{1}^{0}(0) = & z^{\ast }(T)-(\alpha _{1}(0)-\Vert \alpha \Vert ) \\ = & z^{\ast }(T)-\alpha _{1}(0)+\Vert \alpha \Vert \\ \geq & z^{\ast }(T)-\alpha _{1}(T)+\Vert \alpha \Vert \\ = & z^{\ast }(T)-(\alpha _{1}(T)-\Vert \alpha \Vert ) \\ = & z^{\ast }(T)-\alpha _{1}^{0}(T). \end{split} \end{equation*}$

Then, by (3.17),

$\begin{equation*} z^{\ast }(0)-\alpha _{1}^{0}(0) = z^{\ast }(T)-\alpha _{1}^{0}(T), \end{equation*}$

and

$\begin{equation} (z^{\ast })^{\prime }(T)-(\alpha _{1})^{\prime }(T)\leq 0. \end{equation}$

(3.19)

Therefore, by (3.17) and (3.1) and Definition 2.2,

$\begin{equation*} \begin{split} (z^{\ast })^{\prime }(T)& = f(T, \delta _{1}^{0}(T, z^{\ast }(T)), \delta _{2}^{0}(T, w(T)))+\delta _{1}^{0}(T, z^{\ast }(T))-z^{\ast }(T) \\ & = f(T, \alpha _{1}^{0}(T), \delta _{2}^{0}(T, w(T)))+\alpha _{1}^{0}(T)-z^{\ast }(T) \\ & > f(T, \alpha _{1}^{0}(T), \delta _{2}^{0}(T, w(T))) \\ & \geq f(T, \alpha _{1}^{0}(T), \alpha _{2}^{0}(T))\\ &\geq \alpha _{1}^{\prime }(T), \end{split} \end{equation*}$

which contradicts (3.19).

Therefore, $z^{\ast }(t)\geq \alpha _{1}^{0}(t), \forall t\in \lbrack 0, T]$ . The same arguments can be used to prove the other inequalities of (3.16).

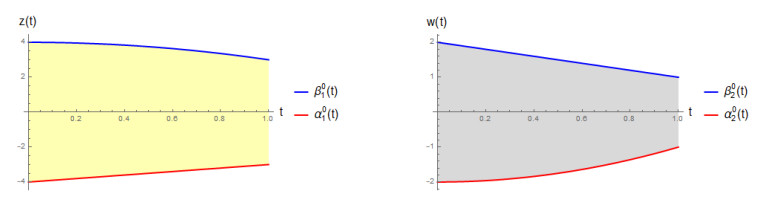
Example 3.1. Consider the following system, for $t\in \lbrack 0, 1],$

$\begin{equation} \left\{ \begin{split} z^{\prime }(t)& = w(t)-\left( z(t)\right) ^{3}, \\ w^{\prime }(t)& = 1-t+\arctan \left( z(t)\right) -4\sqrt[3]{w(t)}, \end{split} \right. \end{equation}$

(3.20)

together with the periodic boundary conditions (1.2).

The functions $\alpha _{i}, \beta _{i}:\left[0, 1\right] \rightarrow \mathbb{R},$ $i = 1, 2,$ given by

$\begin{eqnarray*} \alpha _{1}(t) & = &t-2, \qquad \beta _{1}(t) = 2-t^{2}, \\ \alpha _{2}(t) & = &t^{2}-1, \qquad \beta _{2}(t) = 1-t, \end{eqnarray*}$

are, respectively, lower and upper solutions of problems (3.20) and (1.2), according to Definition 2.2, with

$\begin{equation*} \alpha _{1}^{0}(t) = t-4, \qquad \alpha _{2}^{0}(t) = t^{2}-2, \end{equation*}$

and

$\begin{equation*} \beta _{1}^{0}(t) = 4-t^{2}, \qquad \beta _{2}^{0}(t) = 2-t. \end{equation*}$

The above problem is a particular case of (1.1) and (1.2), where

$\begin{equation*} f\left( t, z(t), w(t)\right) = w(t)-\left( z(t)\right) ^{3} , \end{equation*}$

and

$\begin{equation*} g\left( t, z(t), w(t)\right) = 1-t+\arctan \left( z(t)\right) -4\sqrt[3]{w(t)}. \end{equation*}$

As the assumptions of Theorem 3.1 are verified, then the system (3.20), (1.2) has, at least, a solution $(z^*, w^*)\in (C[0, 1])^{2}$ such that

$\begin{equation} \begin{split} t-4& \leq z^*(t)\leq 4-t^{2}, \\ t^{2}-2& \leq w^*(t)\leq 2-t, \quad \forall t\in \lbrack 0, 1], \end{split} \end{equation}$

(3.21)

as shown in Figure 1.

Figure 1.

$(z^*, w^*)$ -solution localization.

DownLoad: Full-Size Img PowerPoint

Moreover, this solution is not trivial, since constants are not solutions of (3.20).

4. Ordered lower and upper-solutions and monotonicity

In this section we present a technique to deal with the lack of monotonicity requirements in Theorem 3.1 on the nonlinearities, by defining coupled lower and upper solutions $\alpha _{i}$ and $\beta _{i},$ conveniently, as in the next definition:

Definition 4.1. Consider the $C^{1}$ -functions $\alpha _{i}, \beta _{i}:[0, T]\rightarrow \mathbb{R},$ $i = 1, 2.$ The functions $\left(\alpha _{1}, \alpha _{2}\right)$ are coupled lower solutions of the periodic problems (1.1) and (1.2) if

$\begin{equation*} \left\{ \begin{array}{c} \alpha _{1}^{\prime }(t)\geq f\left( t, \alpha _{1}(t), \alpha_{2}(t)\right), \\ \alpha _{2}^{\prime }(t)\leq g\left( t, \beta_{1}(t), \alpha _{2}(t)\right), \end{array} \right. \label{eq: a1' < f, a2' > g} \end{equation*}$

and

$\begin{equation*} \begin{array}{c} \alpha_{1}(0)\geq \alpha_{1}(T), \\ \alpha_{2}(0)\leq \alpha_{2}(T). \end{array} \end{equation*}$

The functions $\left(\beta _{1}, \beta _{2}\right)$ are coupled upper solutions of the periodic problems (1.1) and (1.2) if

$\begin{equation*} \left\{ \begin{array}{c} \beta _{1}^{\prime }(t)\leq f\left( t, \beta _{1}(t), \beta _{2}(t)\right), \\ \beta _{2}^{\prime }(t)\geq g\left( t, \alpha _{1}(t), \beta _{2}(t)\right), \end{array} \right. \end{equation*}$

and

$\begin{equation*} \begin{array}{c} \beta_{1}(0)\leq \beta_{1}(T), \\ \beta_{2}(0)\geq \beta_{2}(T). \end{array} \end{equation*}$

Theorem 4.1. Let $\left(\alpha _{1}, \alpha _{2}\right)$ and $\left(\beta _{1}, \beta _{2}\right)$ be lower and upper solutions of (1.1) and (1.2), respectively, according to Definition 4.1, such that

$\begin{equation*} \alpha _{1}(t)\geq \beta _{1}(t)\ \mathit{\text{and}}\ \alpha _{2}(t)\leq \beta _{2}(t), \mathit{\text{}}\forall t\in \left[ 0, T\right] . \end{equation*}$

Assume that $f$ is a $L^{1}$ -Carathéodory function in the set

$\begin{equation*} \left\{ \left( t, y_{1}, y_2\right) \in \lbrack 0, T]\times \mathbb{R}^2 :\beta _{1}(t)\leq y_{1}\leq \alpha _{1}(t)\right\} , \end{equation*}$

$g$ is a $L^{1}$ -Carathéodory function in

$\begin{equation*} \left\{ \left( t, y_1, y_{2}\right) \in \lbrack 0, T]\times \mathbb{R}^2 :\alpha _{2}(t)\leq y_{2}\leq \beta _{2}(t)\right\} , \end{equation*}$

with

$\begin{equation} f\left( t, y_{1}, y_{2}\right) \ \mathit{\text{nonincreasing in}}\ y_{2}, \end{equation}$

(4.1)

for $t\in \lbrack 0, T]$ , $y_1 \in \mathbb{R}$ , and

$\begin{equation} g\left( t, y_{1}, y_{2}\right) \ \mathit{\text{nondecreasing in}}\ y_{1}, \end{equation}$

(4.2)

for $t\in \lbrack 0, T]$ , $y_2 \in \mathbb{R}$ . Then problems (1.1) and (1.2) has, at least, a solution $(z, w)\in \left(C^1[0, T]\right) ^{2}$ such that

$\begin{equation} \begin{split} &\beta _{1}(t) \leq z(t)\leq \alpha _{1}(t), \\ &\alpha _{2}(t) \leq w(t)\leq \beta _{2}(t),\ \mathit{\text{for all}}\ t\in \lbrack 0, T]. \end{split} \end{equation}$

(4.3)

Remark 4.1. This method relating the definition of coupled lower and upper-solutions, and the monotonicity properties of the nonlinearities, can be applied to other types of monotonicity.

Proof. For $i = 1, 2,$ define the continuous functions $\delta_i:[0, T]\times \mathbb{R} \rightarrow \mathbb{R}$ , given by

$\begin{equation*} \delta_{1}(t, z) = \left\{ \begin{array}{ccc} \beta _{1}(t) & \text{if} & z < \beta _{1}(t), \\ z & \text{if} & \beta _{1}(t)\leq z\leq \alpha _{1}(t), \\ \alpha_{1}(t) & \text{if} & z > \alpha_{1}(t), \end{array} \right. \end{equation*}$

and

$\begin{equation*} \delta _{2}(t, w) = \left\{ \begin{array}{ccc} \alpha_{2}(t) & \text{if} & w < \alpha_{2}(t), \\ w & \text{if} & \alpha_{2}(t)\leq w\leq \beta_{2}(t), \\ \beta_{2}(t) & \text{if} & w > \beta_{2}(t), \end{array} \right. \end{equation*}$

and consider the auxiliary problem composed by

$\begin{equation} \left\{ \begin{array}{c} z^{\prime }(t)+z(t) = f\left( t, \delta _{1}(t, z), \delta _{2}(t, w)\right) +\delta _{1}(t, z), \\ w^{\prime }(t)+w(t) = g\left( t, \delta _{1}(t, z), \delta _{2}(t, w)\right) +\delta _{2}(t, w), \end{array} \right. \end{equation}$

(4.4)

together with the periodic boundary conditions (1.2).

Problems (4.4) and (1.2) can be addressed using the arguments for the proof of Theorem 3.1. The corresponding operator has a fixed point $(\bar{z}, \bar{w})$ .

The delicate step, however, is to show that $(\bar{z}, \bar{w})$ is a solution of (1.1) and (1.2), such that

$\begin{equation} \beta{1}(t)\leq \bar{z}(t)\leq \alpha_{1}(t), \quad \alpha_{2}(t)\leq \bar{w}(t)\leq \beta_{2}(t), \quad \forall t\in [0, T]. \end{equation}$

(4.5)

To prove the case for $\beta_1(t)\leq \bar{z}(t), \forall t \in [0, T]$ , we suppose, by contradiction, that there exists some $t\in \lbrack 0, T]$ such that

$\begin{equation*} \bar{z}(t) < \beta_{1}(t), \end{equation*}$

and define

$\begin{equation} \min\limits_{t\in \lbrack 0, T]}(\bar{z}-\beta_{1})(t): = \bar{z}(t_{0})-\beta_{1}(t_{0}) < 0. \end{equation}$

(4.6)

If $t_{0}\in]0, T[$ , then

$\begin{equation} \bar{z}'(t_{0}) = \beta_1'(t_{0}), \end{equation}$

(4.7)

and, by (4.6), (4.1) and Definition 4.1, the following contradiction with (4.7) holds:

$\begin{equation*} \begin{split} \bar{z}'(t_0) & = f(t_0, \delta_1(t_0, \bar{z}(t_0)), \delta_2(t_0, w(t_0)))+\delta_1(t_0, \bar{z}(t_0))-\bar{z}(t_0) \\ & = f(t_0, \beta_1(t_0), \delta_2(t_0, w(t_0)))+\beta_1(t_0)-\bar{z}(t_0) \\ & > f(t_0, \beta_1(t_0), \delta_2(t_0, w(t_0))) \\ & \geq f(t_0, \beta_1(t_0), \beta_2(t_0)) \\ & \geq \beta_1'(t_0). \end{split} \end{equation*}$

If $t_{0} = 0$ , then, by (1.2) and Definition 4.1,

$\begin{equation*} \begin{split} \bar{z}(0)-\beta_{1}(0) = \bar{z}(T)-\beta_{1}(0)\geq \bar{z}(T)-\beta_{1}(T). \end{split} \end{equation*}$

Then, by (4.6),

$\begin{equation*} \bar{z}(0)-\beta_{1}(0) = \bar{z}(T)-\beta_{1}(T), \end{equation*}$

and

$\begin{equation} \bar{z}'(T)-\beta_{1}'(T)\leq 0. \end{equation}$

(4.8)

But by (4.6), (4.1) and Definition 4.1,

$\begin{equation*} \begin{split} \bar{z}'(T) & = f(T, \delta_1(T, \bar{z}(T)), \delta_2(T, w(T)))+\delta_1(T, \bar{z}(T))-\bar{z}(T) \\ & = f(T, \beta_1(T), \delta_2(T, w(T)))+\beta_1(T)-\bar{z}(T) \\ & > f(T, \beta_1(T), \delta_2(T, w(T))) \\ &\geq f(T, \beta_1(T), \beta_2(T))\\ & \geq \beta_1'(T), \end{split} \end{equation*}$

which contradicts (4.8).

Therefore, $\bar{z}(t)\geq \beta_1(t), \forall t\in [0, T]$ . The same arguments can be applied to prove the inequality $\bar{z}(t)\leq \alpha_1(t)$ .

Using the same technique, one can prove that

$\begin{equation*} \alpha_{2}(t) \leq \bar{w}(t) \leq \beta_2(t), \forall t\in [0, T]. \end{equation*}$

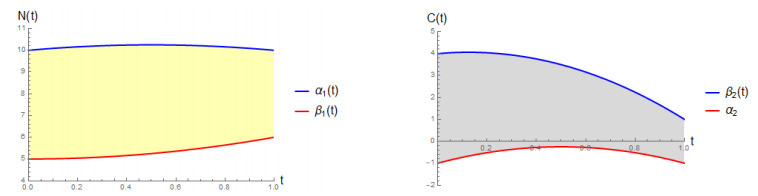
5. Application to a criminal vs non-criminal population dynamics

In ^[15], the authors present an analysis of the dynamics of the interaction between criminal and non-criminal populations based on the pray-predator Lotka-Volterra models. Motivated by this work, we consider a variant of the constructed model with a logistic growth of the non-criminal population and a law enforcement term, that is,

$\begin{equation} \left\{ \begin{array}{c} N^{\prime }(t) = \mu N(t)\Big(1-\frac{1}{K}N(t)\Big)-aN(t)C(t), \\ C^{\prime }(t) = -\gamma C(t)-l_{c}C(t)+bN(t)C(t), \end{array} \right. \end{equation}$

(5.1)

where $C$ and $N$ are, respectively, the criminal and non-criminal population as functions of time, $\mu$ is the growth rate of $N$ in the absence of $C$ , $a$ and $b$ are the variation rates of $N$ and $C$ , respectively, due to their interaction, $\gamma$ is the natural mortality rate of $C$ , $K$ is the carrying capacity for $N$ in the absence of $C$ , and $l_{c}$ is the measure of enforced law on $C$ .

We note that the interaction term could have different effects in each of the populations, hence, the, eventually, different multiplying factors $a$ and $b$ .

As a numerical example, we consider the parameter set:

$\begin{equation} \begin{array}{c} \mu = 2.5, \text{ }a = 0.1, \text{ }b = 0.01, \\ \gamma = 2.5, \text{ }K = 8, \text{ }l_{c} = 5. \end{array} \end{equation}$

(5.2)

We choose a normalized period $T = 1$ , representing the evolution dynamics in one year, and the periodic boundary conditions

$\begin{equation} \begin{array}{c} N(0) = N(1), \\ C(0) = C(1). \end{array} \end{equation}$

(5.3)

Notice that the problem (5.1) and (5.3), with (5.2), is a particular case of (1.1) and (1.2) with

$\begin{equation*} \begin{array}{c} f\left( t, N(t), C(t)\right) = 2.5N(t)\Big(1-\frac{1}{8}N(t)\Big)-0.1N(t)C(t), \\ g\left( t, N(t), C(t)\right) = -2.5C(t)-5C(t)+0.01N(t)C(t), \end{array} \end{equation*}$

and the quadratic functions

$\begin{eqnarray*} \alpha_{1}(t)& = &-t^{2}+t+10, \text{ } \\ \alpha_{2}(t) & = & -3t^{2}+3t-1, \\ \beta_{1}(t)& = &t^{2}+5, \text{ }\\ \beta_{2}(t)& = &-4t^{2}+t+4, \end{eqnarray*}$

are, respectively, coupled lower and upper solutions of (5.1) and (5.3), for the parameters (5.2), according to Definition 4.1.

As all the assumptions of Theorem 4.1 are verified, then there is at least a solution $(N, C)$ of (5.1), moreover,

$\begin{equation*} t^{2}+5\leq N(t)\leq -t^{2}+t+10, \end{equation*}$

and

$\begin{equation*} -3t^{2}+3t-1\leq C(t)\leq -4t^{2}+t+4. \end{equation*}$

The only constant solutions $(N, C)$ admissible by problem (5.1) are $(0, 0)$ , $(750, 0)$ , $(0, -2318.75)$ and $(750, -2318.75)$ , which are beyond the lower and upper bounds $\alpha_i, \beta_i$ . Hence, the region of solutions shown in Figure 2 contains only non-trivial solutions.

Figure 2.

$(N, C)$ -solution localization.

DownLoad: Full-Size Img PowerPoint

6. Discussion

The existence of periodic solutions for problems where the nonlinearities have no periodicity at all is scarce in the literature, not only on differential equations but also on coupled systems of differential equations. This work aims to contribute towards fulfilling this gap, by presenting sufficient conditions to guarantee the existence of periodic solutions in the presence of lower and upper solutions and some adequate monotonicity properties on the nonlinearities.

The localization ensured by the lower and upper solutions method has been undervalued, as well as the estimation and some qualitative properties that it provides for the oscillation and variation of the solutions. The introduction of a practical example, and an application to a real social phenomenon, was intended to stress the potentialities of this technique.

7. Conclusions

In the literature, in general, the lower and upper solutions method requires the well-ordered case, that is, the lower function below the upper one, and a specific local monotonicity on the nonlinearity. This work suggests techniques to overcome both restrictions, so that the search for functions that verify lower and upper solutions properties becomes easier.

For the first issue, we apply a translation, such that, regardless of the order relation between the lower and upper solutions, the shifted functions are always well-ordered.

Section 4 presents a technique that combines the definition of coupled lower and upper-solutions (Definition 4.1) with the monotonicity properties verified by the nonlinearities. In this way, this method can be applied to other problems where the different types of monotonicity hold.

Acknowledgments

This work research was supported by national funds through the Fundação para a Ciência e Tecnologia, FCT, under the project UIDB/04674/2020.

Conflict of interest

The authors declare no conflicts of interest.

References

[1]	T. L. Carroll, L. M. Pecora, Synchronizing nonautonomous chaotic circuits, IEEE Trans. Circuits Syst. II. Analog Digital Signal Proc., 40 (1993), 646-650. doi: 10.1109/82.246166
[2]	U. Parlitz, L. Junge, L. Kocarev, Subharmonic entrainment of unstable period orbits and generalized synchronization, Phys. Rev. Lett., 79 (1997), 3158-3161. doi: 10.1103/PhysRevLett.79.3158
[3]	A. O. Alamodi, K. H. Sun, W. Ai, et al. Design new chaotic maps based on dimension expansion, Chin. Phys. B, 28 (2019), 20503.
[4]	Z. Y. Yan, Chaos Q-S synchronization between Rössler system and the new unified chaotic system, Phys. Lett. A, 334 (2005), 406-412. doi: 10.1016/j.physleta.2004.11.042
[5]	H. T. Yu, J. Wang, B. Deng, et al. Adaptive synchronization control of coupled chaotic neurons in an external electrical stimulation, Chin. Phys. B, 22 (2013), 58701.
[6]	Y. H. Sun, X. P. Wu, L. Q. Bai, et al. Finite-time synchronization control and parameter identification of uncertain permanent magnet synchronous motor, Neurocomputing, 207 (2016), 511-518. doi: 10.1016/j.neucom.2016.05.036
[7]	Z. Li, C. Z. Han, Adaptive synchronization of Rossler and Chen chaotic systems, Chin. Phys. B, 11 (2002), 666-669. doi: 10.1088/1009-1963/11/7/304
[8]	C. Li, X. Liao, K. Wong, Lag synchronization of hyperchaos with application to secure communications, Chaos Solitons Fractals, 23 (2004), 183-193.
[9]	G. H. Li, An active control synchronization for two modified Chua circuits, Chin. Phys. B, 3 (2005), 472-475.
[10]	J. Q. Yang, Y. T. Chen, F. L. Zhu, Associated observer-based synchronization for uncertain chaotic systems subject to channel noise and chaos-based secure communication, Neurocomputing, 167 (2015), 587-595. doi: 10.1016/j.neucom.2015.04.030
[11]	J. Fu, M. Yu, T. D. Ma, Modified impulsive synchronization of fractional order hyperchaotic systems, Chin. Phys. B, 20 (2011), 120508.
[12]	N. Rulkov, M. Sushchik, L. Tsimring, et al. Generalized synchronization of chaos in directionally coupled chaotic systems, Phys. Rev. E, 51 (1995), 980-994. doi: 10.1103/PhysRevE.51.980
[13]	S. Yang, C. Duan, Generalized synchronization in chaotic systems, Chaos Solitons Fractals, 9 (1998), 1703-1707. doi: 10.1016/S0960-0779(97)00149-5
[14]	Q. Han, C. X. Liu, L. Sun, et al. A fractional order hyperchaotic system derived from Liu system and its circuit realization, Chin. Phys. B, 22 (2013), 020502.
[15]	R. X. Zhang, S. P. Yang, Adaptive lag synchronization and parameter identification of fractional order chaotic systems, Chin. Phys. B, 20 (2011), 090512.
[16]	T. L. Carroll, L. M. Pecora, Cascading synchronized chaotic systems, Phys. D, 67 (1993), 126-140. doi: 10.1016/0167-2789(93)90201-B
[17]	J. M. González-Miranda, Synchronization of symmetric chaotic systems, Phys. Rev. E, 53 (1996), 5656-5669. doi: 10.1103/PhysRevE.53.5656
[18]	G. R. Wang, X. L. Yu, S. G. Chen, Chaos control, synchronization and its application, Beijing: National Defence Industry Publishing House, 2001.
[19]	H. L. An, Y. Chen, A function cascade synchronization method with unknown parameters and applications, Chin. Phys. B, 17 (2008), 98-104. doi: 10.1088/1674-1056/17/1/018
[20]	S. Bhalekar, V. Daftardar-Gejji, Synchronization of different fractional order chaotic systems using active control, Commun. Nonlinear Sci. Numer. Simul., 15 (2010), 3536-3546. doi: 10.1016/j.cnsns.2009.12.016
[21]	D. Kusnezov, A. Bulgac, G. Dang, Quantum Levy processes and fractional kinetics, Phys. Rev. Lett., 82 (1999), 1136-1139. doi: 10.1103/PhysRevLett.82.1136
[22]	G. Si, Z. Sun, Y. Zhang, et al. Projective synchronization of different fractional-order chaotic systems with non-identical orders, Nonlinear Anal-Real., 13 (2012), 1761-1771. doi: 10.1016/j.nonrwa.2011.12.006
[23]	S. Wang, Y. Yu, H. Wang, et al. Function projective lag synchronization of fractional-order chaotic systems, Chin. Phys. B, 23 (2014), 040502.
[24]	Y. Xu, Q. Li, W. Li, Periodically intermittent discrete observation control for synchronization of fractional-order coupled systems, Commun. Nonlinear Sci. Numer. Simul., 74 (2019), 219-235. doi: 10.1016/j.cnsns.2019.03.014
[25]	G. Erjaee, S. Momani, Phase synchronization in fractional differential chaotic systems, Phys. Lett. A, 372 (2008), 2350-2354. doi: 10.1016/j.physleta.2007.11.065
[26]	Y. Xu, W. Li, Synchronization for fractional-order multi-linked complex network with two kinds of topological structure via periodically intermittent control, Math. Meth. Appl. Sci., 42 (2019), 2379-2397. doi: 10.1002/mma.5516
[27]	J. Wang, Y. Zhang, Designing synchronization schemes for chaotic fractional-order unified systems, Chaos Solitons Fractals, 30 (2006), 1265-1272. doi: 10.1016/j.chaos.2005.09.027
[28]	Y. Chen, Y. Q. Yang, Chaos in the fractional order generalized Lorenz canonical form, Chin. Phys. Lett., 26 (2009), 100501.
[29]	W. Deng, C. Li, J. Lu, Stability analysis of linear fractional differential system with multiple time delays, Nonlinear Dyn., 48 (2007), 409-416. doi: 10.1007/s11071-006-9094-0
[30]	H. Tirandaz, A. Hajipour, Adaptive synchronization and anti-synchronization of TSUCS and La unified chaotic systems with unknown parameters, Optik, 130 (2017), 543-549. doi: 10.1016/j.ijleo.2016.10.093
[31]	X. Huang, Z. Zhao, Z. Wang, et al. Chaos and hyperchaos in fractional-order cellular neural networks, Neurocomputing, 94 (2012), 13-21. doi: 10.1016/j.neucom.2012.01.011
[32]	I. Podlubny, Fractional differential equations, San Diego: Academic Press, 1999.
[33]	R. Hilfer, Applications of fractional calculus in physics, New Jersey: World Scientific, 2000.
[34]	K. Diethelm, The Analysis of fractional differential equations: An application-oriented exposition using differential operators of caputo type, Berlin: Springer, 2010.
[35]	I. Grigorenko, E. Grigorenko, Chaotic dynamics of the fractional Lorenz system, Phys. Rev. Lett., 91 (2003), 034101-034108. doi: 10.1103/PhysRevLett.91.034101
[36]	W. H. Deng, C. P. Li, Chaos synchronization of the fractional Lü system, Phys. A, 353 (2005), 61-72. doi: 10.1016/j.physa.2005.01.021
[37]	C. P. Li, G. J. Peng, Chaos in Chen's system with a fractional order, Chaos Solitons Fractals, 22 (2004), 443-450. doi: 10.1016/j.chaos.2004.02.013

This article has been cited by:

1.	Andreas Fröberg, How children and young people can stay physically active during the novel coronavirus pandemic while take into account safety measures and precautions, 2020, 10, 2228-6497, 295, 10.34172/hpp.2020.47
2.	Patricia Polero, Carmen Rebollo-Seco, José C. Adsuar, Jorge Pérez-Gómez, Jorge Rojo-Ramos, Fernando Manzano-Redondo, Miguel Ángel Garcia-Gordillo, Jorge Carlos-Vivas, Physical Activity Recommendations during COVID-19: Narrative Review, 2020, 18, 1660-4601, 65, 10.3390/ijerph18010065
3.	Adrianos Golemis, Panteleimon Voitsidis, Eleni Parlapani, Vasiliki A Nikopoulou, Virginia Tsipropoulou, Panayiota Karamouzi, Aikaterini Giazkoulidou, Aikaterini Dimitriadou, Christina Kafetzopoulou, Vasiliki Holeva, Ioannis Diakogiannis, Young adults’ coping strategies against loneliness during the COVID-19-related quarantine in Greece, 2021, 0957-4824, 10.1093/heapro/daab053
4.	Peng Gu, Zeheng Liang, Hao Zhang, Dazhi Zhang, Effects of Attitudes towards Exercise Behaviour, Use of Sports Apps and COVID-19 on Intentions to Exercise, 2022, 12, 2075-4426, 1434, 10.3390/jpm12091434
5.	Rastegar Hoseini, Zahra Hoseini, Elahe Bahmani, Mahsa Ahmadi Darmian, The Association Between Physical Activity Level of CVD Patients and Rate of Covid-19 Incidence, 2022, 9, 2383-1413, 10.5812/iji.118853
6.	Ruofei Lin, Xiaoli Hu, Lige Guo, Junpei Huang, Zulkarnain Jaafar, The health benefit of physical exercise on COVID-19 pandemic: Evidence from mainland China, 2022, 17, 1932-6203, e0275425, 10.1371/journal.pone.0275425
7.	Mahnaz Azmodeh, Rastegar Hoseini, Ehsan Amiri, Physical Activity Levels and Self-perception of Fitness in COVID-19-Recovered Individuals, 2022, 16, 1735-8639, 10.5812/ijpbs.115038
8.	L Ambrosio, D Lambrick, J Faulkner, MC Portillo, Accessibility and applicability of physical activity guidelines and recommendations for adults living with long term conditions during COVID-19, 2022, 0960-3123, 1, 10.1080/09603123.2022.2066071
9.	Yong Wang, Correlation analysis between the quality of physical activity and public health based on artificial intelligence technology, 2024, 9, 2444-8656, 10.2478/amns.2023.2.00486
10.	Hossein Pirani, Omid Reza Salehi, Sarieh Shahraki, Adeleh Khodabakhsi Fard, Kimia Khoramipour, Effect of COVID-19 on Public Health: The Latest Physical Activity and Diet Update for Counteracting New Emerging COVID-19 Strains (Omicron) and Stay Active and Do Not Lay on the Vaccine Alone: A Narrative Review, 2023, 12, 2322-1593, 10.5812/jcrps-138081
11.	Tereza Lais Menegucci Zutin, Flávia Vilas Boas Ortiz Carli, Mara Silvia Foratto Marconato, Camila Maria De Arruda, Eduardo Federighi Baisi Chagas, MODIFICAÇÕES NO ESTILO DE VIDA DURANTE A PANDEMIA DE COVID-19 E CONHECIMENTO DA DOENÇA, 2024, 4, 2447-0961, e3107, 10.56083/RCV4N3-001

Reader Comments

Your name:*

Email:*
© 2020 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)