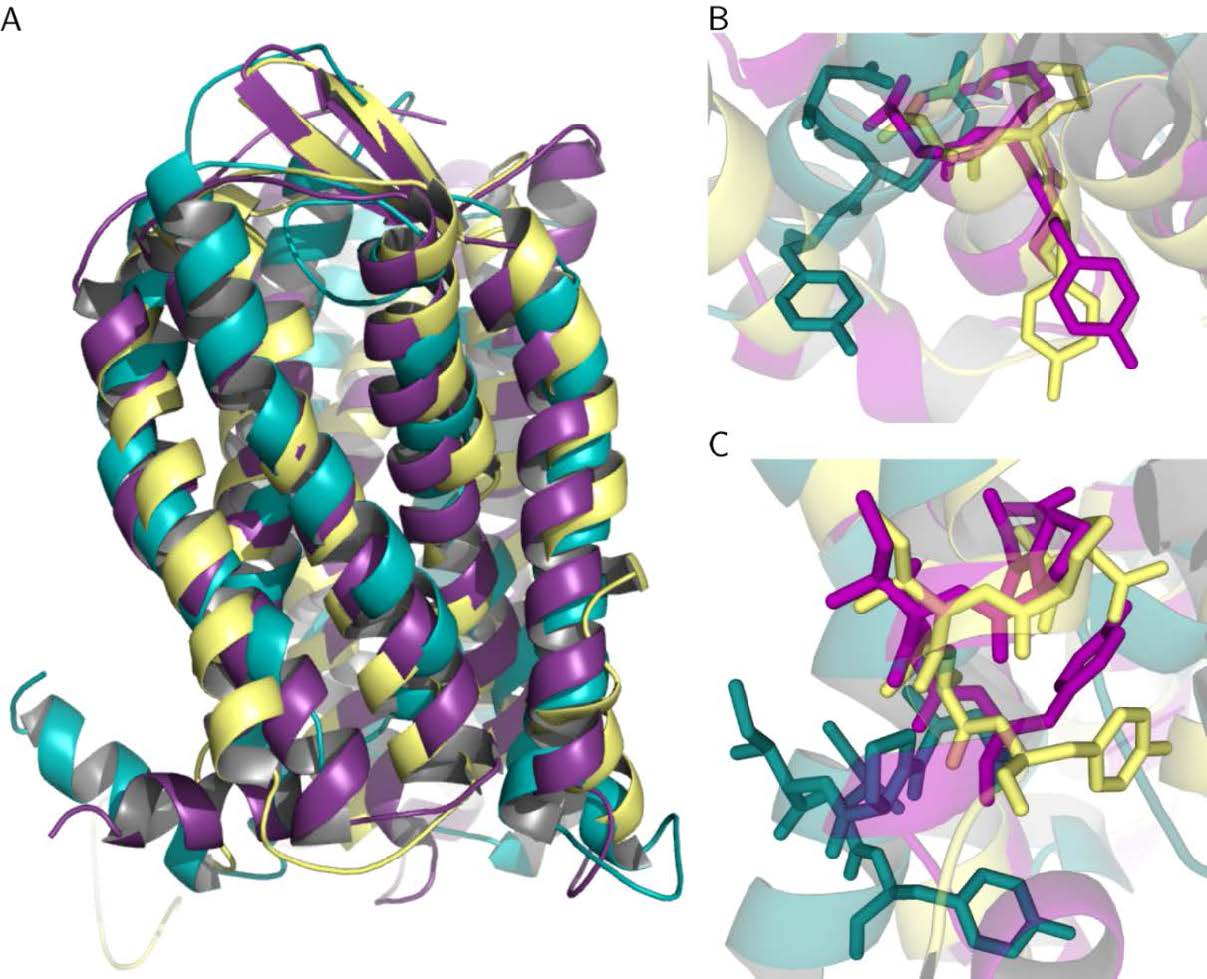
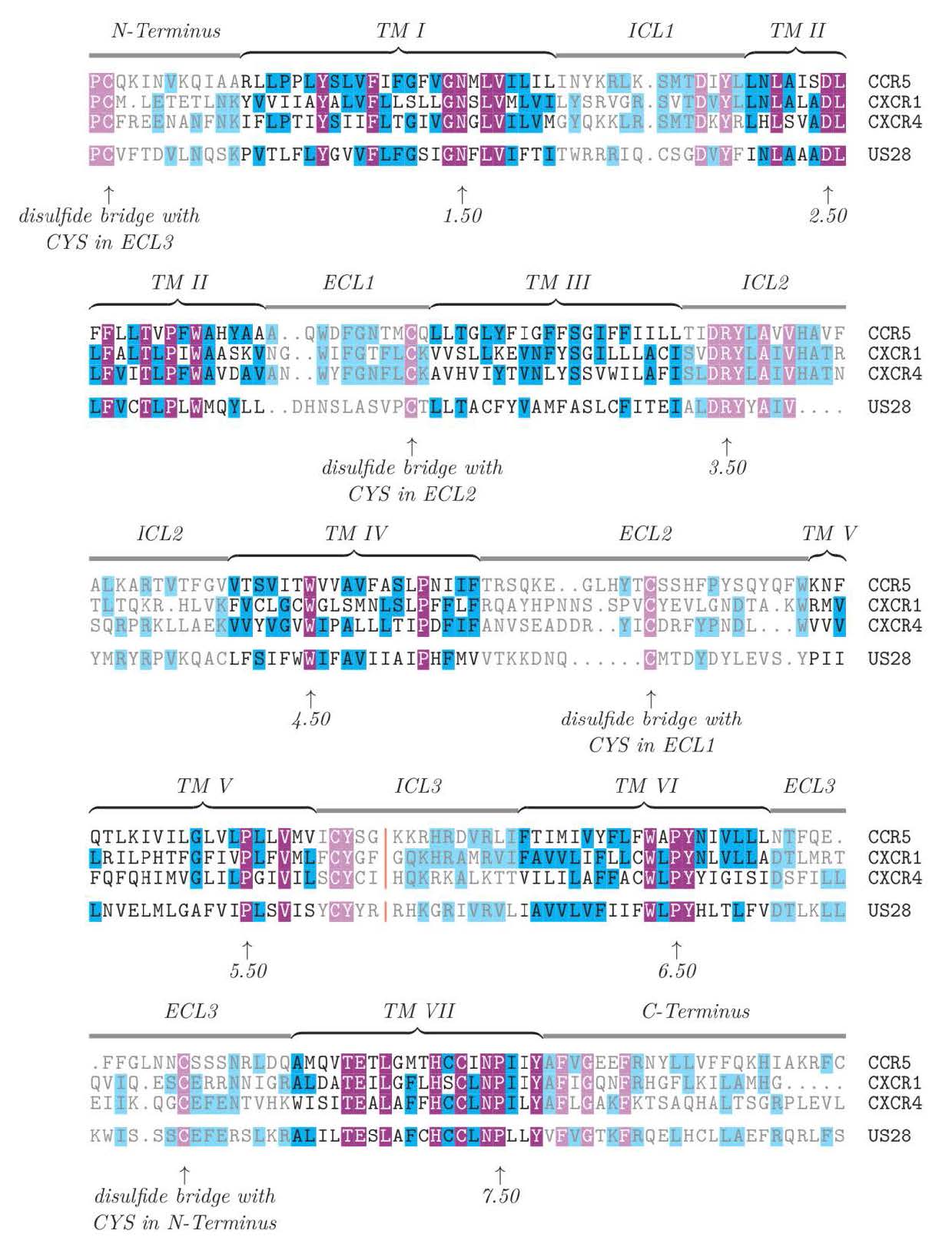
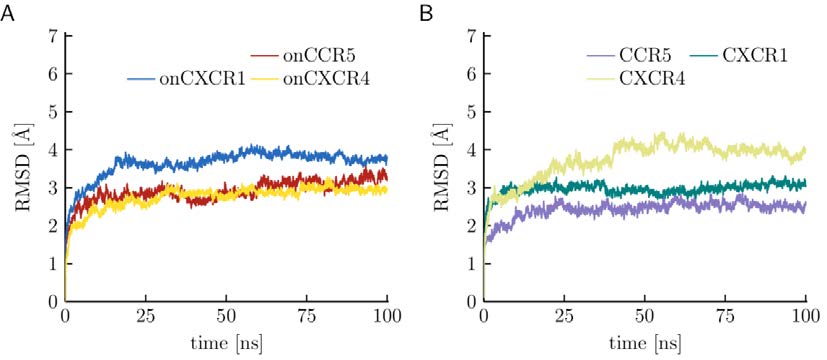
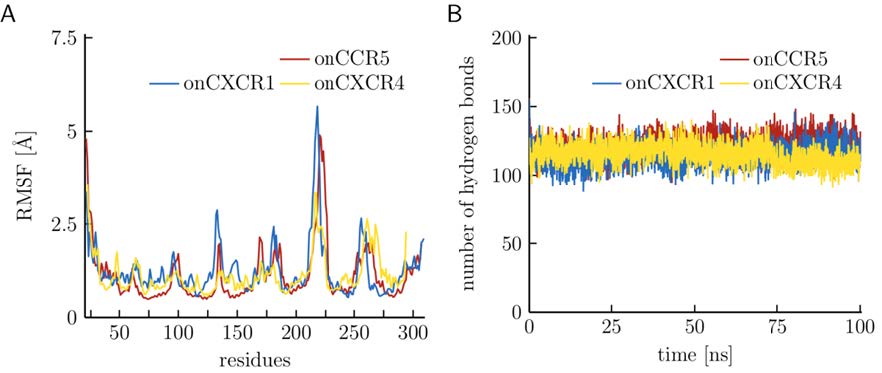
Cell responses can be triggered via G-protein coupled receptors (GPCRs) that interact with small molecules, peptides or proteins and transmit the signal over the membrane via structural changes to activate intracellular pathways. GPCRs are characterized by a rather low sequence similarity and exhibit structural differences even for functionally closely related GPCRs. An accurate structure prediction for GPCRs is therefore not straightforward. We propose a computational approach that relies on the generation of several independent models based on different template structures, which are subsequently refined by molecular dynamics simulations. A comparison of their conformational stability and the agreement with GPCR-typical structural features is then used to select a favorable model. This strategy was applied to predict the structure of the herpesviral chemokine receptor US28 by generating three independent models based on the known structures of the chemokine receptors CXCR1, CXCR4, and CCR5. Model refinement and evaluation suggested that the model based on CCR5 exhibits the most favorable structural properties. In particular, the GPCR-typical structural features, such as a conserved water cluster or conserved non-covalent contacts, are present to a larger extent in the model based on CCR5 compared to the other models. A final model validation based on the recently published US28 crystal structure confirms that the CCR5-based model is the most accurate and exhibits 80.8% correctly modeled residues within the transmembrane helices. The structural agreement between the selected model and the crystal structure suggests that our modeling strategy may also be more generally applicable to other GPCRs of unknown structure.
1.
Introduction
In this paper, we consider the global solution to the Cauchy problem of fractional drift diffusion system with power-law nonlinearity,
where $ m\geq 1 $ is an integer, $ v(x, t), w(x, t) $ are the densities of negatively and positively charged particles, $ \phi(x, t) $ is the electric potential determined by the Poisson equation $ \Delta\phi = v-w $. The difficulties mainly come from higher-order nonlinear couplings.
By the fundamental solution of Laplacian:
where $ \omega(N) $ denotes the volume of the unit ball in $ \mathbb{R}^N $, the electric potential $ \phi $ can be expressed by the convolution:
$ \Lambda = \sqrt{-\Delta} $ is the Calderón-Zygmund operator, and the fractional Laplacian $ \Lambda^{\alpha} = (-\Delta)^{\frac{\alpha}{2}} $ with $ 1 < \alpha < 2N $ is a non-local fractional differential operator defined as Eq (1.4)
where $ \mathcal{F} $ and $ \mathcal{F}^{-1} $ are the Fourier transform and its inverse [1].
In probabilistic terms, replacing the Laplacian $ \Delta $ with its fractional power $ -\Lambda^{\alpha} = -(-\Delta)^{\frac{\alpha}{2}} $, it leads to interesting and largely open questions of extensions of results for Brownian motion driven stochastic equations to those driven by Lévy $ \alpha- $stable flights.
In the physical literature, such fractal anomalous diffusions have been recently enthusiastically embraced by a slew of investigators in the context of hydrodynamics, acoustics, trapping effects in surface diffusion, statistical mechanics, relaxation phenomena, and biology [2].
An important technical difficulty is that the densities of the semigroups generated by $ -\Lambda^{\alpha} = -(-\Delta)^{\frac{\alpha}{2}} $ do not decay rapidly in $ x\in \mathbb{R}^{N} $ as is the case of the heat semigroup $ S(t) = e^{t\Delta} $ $ (\alpha = 2) $, the Gauss-Weierstrass kernel $ K_{t}(x) = \mathcal{F}^{-1}(e^{-t|\xi|^{2}}) $ decays exponentially while the densities $ \mathcal{F}^{-1}(e^{-t|\xi|^{\alpha}})(0 < \alpha < 2) $ of non-Gaussian Lévy $ \alpha- $stable semigroups $ S_{\alpha}(t) = e^{-t(-\Delta)^{\frac{\alpha}{2}}} $ have only an algebraic decay rate $ |x|^{-N-\alpha} $.
For a more general nonlinear term in Eq (1.1), the motivation is the Keller-Segel model [3,4], a prototype of cross-diffusion models related to pattern formation, it describes the time and space dynamics of the density of cells (or organisms) $ n(t, x) $ interacting with a chemoattractant $ S(t, x) $ according to the following system:
where $ F $ and $ G $ are the source terms related to interactions [5]. The positive definite nonlinear terms $ D_{n}(n, s) $ and $ D_{s}(n, s) $ are the diffusivity of the chemoattractant and of the cells, respectively. In many applications the cross-diffusion function $ \chi(n, s) $ has a complicated structure, and even it has a very simple structure, for example, a polynomial $ \chi(n, s) = n^{m} $, it fails to satisfy a global Lipschitz condition.
For $ m = 1 $, Eq (1.1) becomes a fractional drift-diffusion system Eq (1.6),
Zhao-Liu [6] established global well-posedness and asymptotic stability of mild solutions for the Cauchy problem Eq (1.5) with small initial data in critical Besov spaces, and proved the regularizing-decay rate estimates which imply that mild solutions are analytic in space variables. Ogawa-Yamamoto [7] considered the global existence and asymptotic behavior of solutions for the Cauchy problem Eq (1.5), they showed that the time- global existence of the solutions with large initial data in Lebesgue space $ L^{p}(\mathbb{R}^N) $ and Sobolev space $ W^{\alpha, p}(\mathbb{R}^N) $ and obtained the asymptotic expansion of the solution up to the second terms as $ t\rightarrow +\infty $.
For $ \alpha = 2 $, Eq (1.6) corresponds to the usual drift-diffusion system,
it has been studied widely [8,9,10,11,12,13,14]. Karch [15] considered the Cauchy problem of a scalar equation with a bilinear operator $ B $
For $ w = 0 $, Eq (1.6) corresponds to the generalized Keller-Segel model of chemotaxis:
For $ 1 < \alpha < 2 $, Escudero [16] proved that Eq (1.8) admits a one-dimensional global solution (the same result also holds for $ \alpha = 2 $), Biler-Karch [17] studied the Blowup solutions to generalized Keller-Segel model, and Biler-Wu [18] considered two-dimensional chemotaxis models with fractional diffusion. For $ \alpha = 2 $, Biler-Boritchev-Karch et al., considered the concentration phenomena [19] and gave sharp Sobolev estimates for concentration of solution [20] to the diffusive aggregation model:
with the Poisson kernel function $ K $ from the equation $ \Delta\phi = v $.
Wu-Zheng [21] considered the parabolic-parabolic system corresponding to the parabolic-elliptic system Eq (1.8), the Keller-Segel system with fractional diffusion generalizing the Keller-Segel model of chemotaxis
for initial data $ (u_{0}, v_{0}) $ in the critical Fourier-Herz space $ \dot{B}^{2-2\alpha}_{q}(\mathbb{R}^N)\times \dot{B}^{2-\alpha}_{q}(\mathbb{R}^N) $ with $ 2\leq q\leq \infty $ for $ \varepsilon > 0 $ and $ 1 < \alpha\leq 2 $.
For the fractional evolution equations with higher order nonlinearity, Miao-Yuan-Zhang [22] studied the Cauchy problem for the semilinear fractional power dissipative equation
with the nonlinear term $ F(u) = f(u) $ or $ F(u) = Q(D)f(u) $, where $ Q(D) $ is a homogeneous pseudo differential operator and $ f(u) = |u|^{b}u $ or $ |u|^{b_{1}}u+|u|^{b_{2}}u $ with $ b > 0, b_{1} > 0 $ and $ b_{2} > 0 $. For example, the equation in Eq (1.10) contains the semilinear fractional power dissipative equation $ \partial_t u+\Lambda^{\alpha}u = \pm |u|^{b}u $, the generalized convection-diffusion equation $ \partial_t u+\Lambda^{\alpha}u = a\cdot\nabla(|u|^{b}u) $, and so on.
Following the idea of Karch [15], due to the fractional heat semigroup $ S_{\alpha}(t) = e^{-t\Lambda^{\alpha}} $ and the well-known Duhamel principle, we rewrite the system Eq (1.1) as a system of integral equations
where
A solution of Eq (1.11) and Eq (1.12) is called a mild solution of Eq (1.1).
Inspired by the contributions summarized in the above items, we aim to extend the results to the system Eq (1.1) with higher-order nonlinear terms $ \nabla\cdot(v^m \nabla \phi) $ and $ \nabla\cdot(w^m \nabla \phi) $. The goal of this article is to prove the global well-posedness of mild solutions to the Cauchy problem Eq (1.1) with small initial data in critical Besov spaces. When $ m = 1 $ in the higher order nonlinear term $ \nabla\cdot(v^m \nabla \phi) $, we recover the result proved in [6]. The outline of the rest of the article is as follows. In Section 2 we give the definition of homogeneous Besov space by the fractional heat semigroup operator and present some useful estimates. In Section 3 we establish the global existence and uniqueness of the mild solution. In Section 4 we discuss the asymptotic stability of the mild solution. In Section 5 we give the regularizing-decay rate estimates of the mild solution. In Section 6 we consider a fractional drift diffusion system with a generalized electric potential equation and we also give the global existence and asymptotic stability of the mild solution.
2.
Preliminaries
Let $ \mathcal{S}(\mathbb{R}^N) $ be the Schwartz space and $ \mathcal{S}'(\mathbb{R}^N) $ be its dual. Now, we introduce a definition of the homogeneous Besov space by the semigroup operator $ S_{\alpha}(t) = e^{-t\Lambda^{\alpha}} $.
Definition 2.1. [6] Let $ l > 0 $ and $ 1\leq p\leq \infty $. Define
with the norm
$ (\dot{B}^{-l}_{p, \infty}(\mathbb{R}^N), ||\cdot||_{\dot{B}^{-l}_{p, \infty}}) $ is a Banach space.
If $ (v(x, t), w(x, t)) $ is a solution of the Cauchy problem Eq (1.1), for any $ \lambda > 0 $, denote
$ (v_{\lambda}(x, t), w_{\lambda}(x, t)) $ is also a solution of the Cauchy problem Eq (1.1) with the initial data
then $ (v_{\lambda}(x, t), w_{\lambda}(x, t)) $ is called a self-similar solution to Eq (1.1). We can verify that $ \dot{B}^{-\frac{\alpha}{m}+\frac{n}{p}}_{p, \infty}(\mathbb{R}^n) $ is a critical space, i.e., the self-similar solution is invariant under the norm $ ||\cdot||_{\dot{B}^{-\frac{\alpha}{m}+\frac{n}{p}}_{p, \infty}} $, which defined in [6], for initial data $ (v_{0}(x), w_{0}(x)) $ of the system Eq (1.1). In the case the index $ s_c: = \frac{n}{p}-\frac{\alpha}{m} $ provides the minimal regularity for the initial data to ensure the well-posedness of the Cauchy problem Eq (1.1). In order to find a critical space for the solutions of the Cauchy problem Eq (1.1), we define some time-weighted space-time space.
Let $ X $ be a Banach space and $ I $ be a finite or infinite interval. We define the time-weighted space-time Banach space,
with the norm $ ||f||_{C_{\sigma}(I; X)} = \sup_{t > 0}t^{\frac{1}{\sigma}}||f(t)||_{X} $. The corresponding homogeneous time-weighted space-time Banach space,
We denote $ C_{*}([0, \infty); X) $ by the set of bounded maps from $ [0, \infty) $ to $ X $ which are continuous for $ t > 0 $ and weakly continuously for $ t = 0 $.
For initial data $ (v_{0}(x), w_{0}(x)) $ in critical homogeneous Besov space $ \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $ with minimal regularity, we want to find a mild solution of the Cauchy problem Eq (1.1) and discuss the global existence of mild solution in the following critical space,
with the norm
For the Laplacian operator $ \Delta $ and the Calderón-Zygmund operator $ \Lambda = \sqrt{-\Delta} $, we have the following classical Hardy-Littlewood-Sobolev inequality.
Lemma 2.2. [23,24] Let $ 1 < p < N $, the nonlocal operator $ (-\Delta)^{-\frac{1}{2}} $ is bounded from $ L^{p}(\mathbb{R}^N) $ to $ L^{\frac{Np}{N-p}}(\mathbb{R}^N) $, i.e., $ \forall f\in L^{p}(\mathbb{R}^N) $,
For the fractional power operator $ \Lambda^{\alpha} = (-\Delta)^{\frac{\alpha}{2}} $ and the semigroup operator $ S_{\alpha}(t) = e^{-t\Lambda^{\alpha}} $, we first consider the Cauchy problem for the homogeneous linear fractional heat equation
By the Fourier transform the solution can be written as:
where the fractional heat kernel Eq (2.12),
the function $ K(x)\in L^{\infty}(\mathbb{R}^N)\cap C_{0}(\mathbb{R}^N) $, where $ C_{0}(\mathbb{R^{N}}) $ denotes the space of functions $ f\in C(\mathbb{R}^{N}) $ satisfying that $ \lim_{|x|\rightarrow \infty}f(x) = 0 $.
For the semigroup operator $ S_{\alpha}(t) $ we have $ L^{p}-L^{q} $ estimates
Lemma 2.3. [9] Let $ 1\leq p\leq q\leq\infty $. Then, $ \forall f\in L^{p}(\mathbb{R}^N) $,
for $ \alpha > 0 $ and $ \gamma > 0 $.
Following the work of Kato [25,26] and Lemarie-Rieusset [23] for the Navier-Stokes problem, Miao-Yuan [27] gave a general existence and uniqueness result for an abstract operator equation via a contraction argument.
Lemma 2.4. [27] Let $ X $ be a Banach space and $ B: X\times X\times\cdots\times X\rightarrow X $ be a $ (m+1)- $linear continuous operator satisfying
$ \forall u_{1}, u_{2}, \cdots, u_{m+1}\in X $ for some constant $ K > 0 $. Let $ \varepsilon > 0 $ be such that $ (m+1)(2\varepsilon)^{m}K < 1 $. Then for every $ y\in X $ with $ ||y||_{X}\leq \varepsilon $ the equation
has a unique solution $ u\in X $ satisfying that $ ||u||_{X}\leq 2\varepsilon $. Moreover, the solution $ u $ depends continuously on $ y $ in the sense that, if $ ||y||_{X}\leq \varepsilon $ and $ v = y_{1}+B(v, v, \cdots, v) $, $ ||v||_{X}\leq 2\varepsilon $, then
We will use the Lemma to prove the global-in-time existence and uniqueness of the mild solutions to the Cauchy problem Eq (1.1) in the mixed time-space Besov space.
3.
Global existence and uniqueness in Besov space
In this section we give the global existence and uniqueness of mild solution to the Cauchy problem Eq (1.1).
Theorem 3.1. Let $ N\geq2 $ be a positive integer, $ 1 < \alpha\leq 2N $ and
If $ (v_{0}, w_{0})\in \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $, there exists $ \varepsilon > 0 $ such that if $ ||(v_{0}, w_{0})||_{\dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}}\leq\varepsilon $, the Cauchy problem Eq (1.1) has a unique global mild solution $ (v, w)\in \mathcal{X} $ such that $ ||(v, w)||_{\mathcal{X}}\leq2\varepsilon $. Moreover, the solution depends continuously on initial data in the following sense: let $ (\tilde{v}, \tilde{w})\in \mathcal{X} $ be the solution of Eq (1.1) with initial data $ (\tilde{v}_{0}, \tilde{w}_{0}) $ such that $ ||(\tilde{v}_{0}, \tilde{w}_{0})||_{\dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N)}\leq\varepsilon $, then there is a constant $ C $ such that
For the integral system Eqs (1.11) and (1.12) we first consider the term $ S_{\alpha}(t)v_{0} = e^{-t\Lambda^{\alpha}}v_{0} $.
Lemma 3.2. Let $ v_{0}(x)\in \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $ and Eq (3.1) hold true. Then $ S_{\alpha}(t)v_{0}\in \mathcal{X} $ and
Proof. According to the definition of the norm $ ||\cdot||_{\dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N)} $ and $ L^{p}-L^{q} $ estimates for the semigroup operator $ S_{\alpha}(t) = e^{-t\Lambda^{\alpha}} $, we have
and
Therefore, we have
Moreover, following the method of [23] (Proposition 4.4, P33) we obtain that
On the other hand, from $ v_{0}(x)\in \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $ and Definition 2.1, we have
Hence, we have $ S_{\alpha}(t)v_{0}\in \mathcal{X} $ and Eq (3.2) holds true.
Lemma 3.3. Let $ (v, w)\in \mathcal{X} $ and Eq (3.1) hold true. Then $ B(v, \cdots, v, w)\in \mathcal{X} $ and
Proof. According to the definition of the norm $ ||\cdot||_{\dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N)} $, we have
by the expression Eq (1.12) of $ B(v, \cdots, v, w)(t) $, that is,
hence, by the Minkowski inequality, we get
For $ 0 < s\leq t-\tau $, using the $ L^{p}-L^{q} $ estimates Eq (2.13) and Eq (2.14) for the semigroup operator $ S_{\alpha}(t) = e^{-t\Lambda^{\alpha}} $, we have
the last inequality comes from the Hölder inequality for the product $ v\cdot v\cdots v\cdot (v-w) $ and $ \frac{m}{p}+\frac{N-p}{Np} = \frac{(m+1)N-p}{Np} $. Using the classical Hardy-Littlewood-Sobolev inequality Eq (2.8) and Eq (2.9), we have Eq (3.6):
For $ s > t-\tau $, using the $ L^{p}-L^{q} $ estimates Eq (2.13) and Eq (2.14) for the semigroup operator $ S_{\alpha}(t) = e^{-t\Lambda^{\alpha}} $, we have
From the condition Eq (3.1): $ \max\{1, \frac{mN}{\alpha}\} < p < \min\{N, \frac{m(m+1)N}{\alpha}\} $ and $ s > t-\tau $, the function $ f(s) = s^{\frac{1}{m}-\frac{N}{\alpha p}}(t+s-\tau)^{-\frac{mN}{\alpha p}} $ has the maximum
where $ C $ is a constant, by Eq (2.9) we have
Together with Eq (3.6) and Eq (3.7) we have:
Putting Eq (3.8) into Eq (3.5), we have
in the last inequality we use the fact that the Beta function
converges to a constant, since the condition Eq (3.1) implies that
Therefore, we have
Next, we consider the estimate of $ ||B(v, \cdots, v, w)(t)||_{L^{p}} $. From Eq (1.12) we have
thus,
In order to prove that $ B(v, \cdots, v, w)\in \mathcal{X} $, it suffices to prove that $ B(v, \cdots, v, w) $ is continuous for $ t > 0 $ and weakly continuous for $ t = 0 $ in $ \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $, and it is continuous for $ t\geq0 $ in $ L^{p}(\mathbb{R}^N) $.
For any $ 0 < t_{1} < t_{2} $, due to Eq (3.4) we have
Similar to the estimate of $ ||B(v, \cdots, v, w)(t)||_{\dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N)} $, we have
the condition Eq (3.1) implies that $ 1+\frac{1}{m}-\frac{(m+1)N}{\alpha p} > 0 $, hence as $ t_{2}\rightarrow t_{1} $,
According to the property of semigroup,
for $ \phi = (-\Delta)^{-1}(w-v) $ we get
by the $ L^{p}-L^{q} $ estimates Eq (2.13) and Eq (2.14) for the semigroup operator $ S_{\alpha}(t) = e^{-t\Lambda^{\alpha}} $, we have
For $ 0 < s\leq t_{1}-\tau $, we have
For $ s > t_{1}-\tau $, we have
Putting Eqs (3.15)–(3.17) into Eq (3.14), we have
where $ C = C(N, \alpha, p) $ the Beta function $ B = \mathcal{B}(\frac{1}{m}-\frac{N}{\alpha p}, \frac{(m+1)N}{\alpha p}-\frac{1}{m}) $ converges due to the condition Eq (3.1), thus we have
that is,
Putting Eq (3.12) and Eq (3.20) into Eq (3.11) we have
This means that $ B(v, \cdots, v, w) $ is continuous for $ t > 0 $ in $ \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $.
Similarly, we can prove that $ B(v, \cdots, v, w) $ is weakly continuous for $ t = 0 $ in $ \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $ and it is continuous for $ t\geq0 $ in $ L^{p}(\mathbb{R}^N) $. Therefore, we have
that is, $ B(v, \cdots, v, w)\in \mathcal{X} $ and Eq (3.3) holds true, i.e.,
This ends the proof of Lemma 3.3.
The proof of Theorem 3.1. Now for the integral system Eq (1.11) and Eq (1.12) from the Cauchy problem Eq (1.1), we have
in Lemma 3.2 and Lemma 3.3 we deal with the terms $ S_{\alpha}(t)(v_{0}, w_{0}) $ and
respectively. For the Banach space $ \mathcal{X} $ and multi-linear operator $ B(v, \cdots, v, w) $, which satisfies the estimate Eq (3.23), following the Lemma 2.4, for every $ (v_{0}, w_{0})\in \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $, there exists $ \varepsilon > 0 $ such that $ (m+1)(2\varepsilon)^{m}C(N, \alpha, p) < 1 $, then Eq (3.24) has a unique solution $ (v, w)\in \mathcal{X} $ such that $ ||(v, w)||_{X}\leq 2\varepsilon $. Therefore, the Cauchy problem Eq (1.1) has a unique global-in-time mild solution in the mixed time-space Besov space. This completes the proof of Theorem 3.1.
4.
Asymptotic stability analysis
Theorem 4.1. Let $ N $ be a positive integer, $ 1 < \alpha\leq 2N $ and Eq (3.1) hold true and $ (v, w) $ and $ (\tilde{v}, \tilde{w}) $ be two mild solutions of the Cauchy problem Eq (1.1) described in Theorem 3.1 corresponding to initial conditions $ (v_{0}, w_{0}) $ and $ (\tilde{v}_{0}, \tilde{w}_{0}) $, respectively. If $ (v_{0}, w_{0}), (\tilde{v}_{0}, \tilde{w}_{0})\in \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $ such that
then, we have the following asymptotic stability
Proof. Since $ (v_{0}, w_{0}), (\tilde{v}_{0}, \tilde{w}_{0})\in \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $, by Theorem 3.1, there exists a constant $ \varepsilon > 0 $ such that if $ ||(v_{0}, w_{0}), (\tilde{v}_{0}, \tilde{w}_{0})||_{\dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}}\leq\varepsilon $, then the mild solutions $ (v, w) $ and $ (\tilde{v}, \tilde{w}) $ satisfy that $ ||(v, w), (\tilde{v}, \tilde{w})||_{\mathcal{X}}\leq2\varepsilon $. From Eq (1.11) and Eq (1.12) we have
where
By the definition of $ \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N)- $norm, we have
where
For a constant $ \theta\in (0, 1) $ determined in later we have
In the procedure of estimate of Eq (3.5), instead of the product $ v\cdot v\cdots v\cdot (v-w) $ with $ m+1 $ exponents such that $ \frac{m}{p}+\frac{N-p}{Np} = \frac{(m+1)N-p}{Np} $, use the Hölder inequality for the product $ (v-\tilde{v})v^k\tilde{v}^{m-1-k}(v-w) $ with $ m+1 $ exponents such that $ \frac{1}{p}+\frac{k}{p}+\frac{m-1-k}{p}+\frac{N-p}{Np} = \frac{(m+1)N-p}{Np} $, we can prove that
and
Together Eq (4.7) with Eq (4.8) we have
Similarly we have
We next consider the term $ ||v-\tilde{v}||_{L^{p}(\mathbb{R}^N)} $:
where
For the first term we have
For the term $ J_{k} $ and $ \phi = (-\Delta)^{-1}(w-v) $, we have
Similarly, for the term $ J_{m} $ we have
Together Eq (4.5) with Eq (4.11) we have
For $ w-\tilde{w} $ we can get the same estimate similar to Eq (4.15).
For the convenience we denote
Due to the condition Eq (3.1), $ \max\{1, \frac{mN}{\alpha}\} < p < \min\{N, \frac{m(m+1)N}{\alpha}\} $, we have
then, we obtain that $ Q(\theta) $ converges and $ \lim\limits_{\theta\rightarrow 0}Q(\theta) = 0 $.
Due to the condition Eq (4.1) we have $ \lim\limits_{t\rightarrow +\infty}F(t) = 0 $ and $ F(t)\in L^{\infty}[0, +\infty) $. Passing the limit in Eq (4.15) we get
Choosing $ \theta $ and $ \varepsilon $ small enough such that $ Q(\theta) < 1 $ and $ 2C(N, \alpha, p)\varepsilon^{m} < 1 $ respectively, then Eq (4.16) implies that $ M = 0 $. That is, Eq (4.2) holds true. The proof is complete.
5.
Regularizing-decay rate estimates
In this section we consider the regularizing decay rate estimates of the mild solutions to the system Eq (1.1). Compared to the case $ m = 1 $, the main difficulty is caused by the power-law nonlinearity term $ v^{m} $ as $ m > 1 $ in the first two equations of Eq (1.1). To overcome this difficulty, we will apply multiple Leibniz's rule. For the regularizing-decay rate estimates of mild solutions to the Navier-Stokes equations, we refer the reader to [6,28,29,30].
In what follows, for $ x = (x_{1}, \cdots, x_{N})\in\mathbb{R}^{N} $ and $ \beta = (\beta_{1}, \cdots, \beta_{N})\in\mathbb{N}^{N}_{0} $, where $ \mathbb{N}_{0} = \mathbb{N}\bigcup{\{0\}} $ and $ \mathbb{N} = \{1, 2, \cdots\} $, we denote $ \partial^{\beta}_{x} = \partial^{\beta_{1}}_{x_{1}} \cdots \partial^{\beta_{N}}_{x_{N}} $ and $ |\beta| = \beta_{1}+\cdots+\beta_{N} $.
We first describe the main result on regularizing-decay rate estimates of the mild solutions to the system Eq (1.1).
Theorem 5.1. Let $ N\geq2 $ be a positive integer, $ 1 < \alpha\leq 2N $. Assume that $ p $ satisfies Eq (3.1) and $ (v_{0}, w_{0})\in \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $, and $ (v, w) $ is the mild solution to the system Eq (1.1) with initial data $ (v_{0}, w_{0}) $. Furthermore, assume that there exist two positive constants $ M_{1} $ and $ M_{2} $ such that
Then, there exist two positive constants $ K_{1} $ and $ K_{2} $ depending only on $ M_{1} $, $ M_{2} $, $ N $, $ \alpha $, $ m $ and $ p $, such that
for all $ p\leq q \leq \infty $, $ t\in(0, T) $ and $ \beta\in\mathbb{N}^{N}_{0} $.
Remark 1. In fact, Eq (5.3) is equivalent to the claim
for some $ \delta\in(1, 2] $ and sufficiently large constants $ K_{1} $ and $ K_{2} $.
Let us first prepare the refined $ L^{p}-L^{q} $ estimate for semigroup operator $ S_{\alpha}(t) $.
Lemma 5.2. Let $ 1\leq p\leq q\leq \infty $. Then for any $ f\in\dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^{N}) $, we have
for all $ t > 0, \beta\in\mathbb{N}^{N}_{0} $, and $ C_{0} $ is a constant depending only on $ N $ and $ \alpha $.
Proof. As $ S_{\alpha}(t) $ is the convolution operator with fractional heat kernel $ K_{t}(x) = \mathcal{F}^{-1}(e^{-t|\xi|^{\alpha}}) $, by scaling we see that
where $ K(x) = (2\pi)^{-\frac{n}{2}}\int_{\mathbb{R}^{N}}e^{ix\cdot\xi}e^{-|\xi|^{\alpha}}d\xi $. It is clear that [22] (Lemma 2.2)
thus, the Young inequality implies that
By the semigroup property of $ S_{\alpha}(t) $ and the commutativity between semigroup and differential operators, we get
Combining Eq (5.6) and Eq (5.7), and using Definition 2.1, we obtain
where $ \|\mathbf{T}\|_{\mathcal{L}(L^{p}, L^{q})} $ denotes the norm of linear operator $ \mathbf{T} $ from $ L^{p} $ to $ L^{q} $. This proves the Lemma 5.2.
Next we recall some useful results.
Lemma 5.3. [31,Lemma 2.1] Let $ \delta > \frac{1}{2} $. Then there exists a positive constant $ C $ depending only on $ \delta $, such that
Here the notation $ \alpha < \beta $ means that $ \alpha_{i} < \beta_{i}, \forall i\in \mathbb{N} $, $ \left(βα
\right) = \prod\limits^{N}_{i = 1}\frac{\beta_{i}!}{\alpha_{i}!(\beta_{i}-\alpha_{i})!} $, and the dependence of $ C(\delta) $ on $ \delta $ is merely of the form $ \sum^{\infty}_{j = 1}j^{-\delta-\frac{1}{2}} $.
Lemma 5.4. [28] Let $ \psi_{0} $ be a measurable and locally bounded function in $ (0, \infty) $ and $ \{\psi_{j}\}^{\infty}_{j = 1} $ be a sequence of measurable functions in $ (0, \infty) $. Assume that $ \alpha\in\mathbb{R} $ and $ \mu, \nu > 0 $ satisfying $ \mu+\nu = 1 $. Let $ B_{\eta} > 0 $ be a number depending on $ \eta\in(0, 1) $ and $ B_{\eta} $ be non-increasing with respect to $ \eta $. Assume that there is a positive constant $ \sigma $ such that
for all $ j\geq 0 $, $ t > 0 $ and $ \eta\in(0, 1) $. Let $ \eta_{0} $ be a unique positive number such that
Then, for any $ 0 < \eta\leq\eta_{0} $, we have
We now prove the Theorem 5.1. Following the idea in Giga-Sawada [28], we first prove the Remark 1, a variant of Theorem 5.1 under extra regularity assumption.
Proposition 1. Under the same assumptions in Theorem 5.1. Assume further that
for all $ p\leq q\leq\infty $ and $ \beta\in\mathbb{N}^{N}_{0} $. Then for any $ \delta\in (1, 2] $, there exist two positive constants $ K_{1} $ and $ K_{2} $ depending only on $ M_{1} $, $ M_{2} $, $ N $, $ \alpha $, $ m $ and $ p $, such that
for all $ p\leq q \leq \infty $, $ t\in(0, T) $ and $ \beta\in\mathbb{N}^{N}_{0} $.
Proof. We split the proof into the following two steps by an induction $ |\beta| = m $.
Step 1. We will prove Eq (5.12) for $ m = 0 $. Equation (5.2) implies that Eq (5.12) is trivial if $ q = p $, thus it suffices to consider $ q\in (p, \infty] $. Let $ \eta\in(0, 1) $ be a constant to be determined later, we take $ L^{q}- $norm of the first equation in Eq (1.11) and split the time integral into two parts as follows:
We will estimate term by term.
For $ E_{1} $, by Lemma 5.2 and Eq (5.1), one can easily see that
For $ E_{2} $ and $ E_{3} $, by Lemma 2.2, Lemma 2.3 and Eq (5.2), we have
Combining Eqs (5.14)–(5.16), and setting $ \bar{B}_{\eta} = C_{1}(N, \alpha, M_{1})+C_{2}(N, \alpha, p, M_{2})\eta^{-1-\frac{1}{m}} $, the inequality Eq (5.13) yields that
The estimate for $ w(t) $ can be done analogously as Eq (5.17). Hence, we have
where $ B_{\eta} = 2\bar{B}_{\eta} $ and $ C_{4} = 2C_{3}(N, \alpha, p, M_{2}) $.
By applying Lemma 5.4, we get the desired estimate Eq (5.12) for $ |\beta| = k = 0 $ with $ K_{1} = 2B_{\eta_{0}} $ for some $ \eta_{0} = \eta_{0}(N, \alpha, p, m, M_{1}, M_{2})\in(0, 1) $.
Step 2. Next we prove Eq (5.12) for $ |\beta| = k\geq1 $. Due to the appearance of nonlocal function $ \phi $, we use a different argument to prove Eq (5.12) for $ p\leq q < N $ and $ N\leq q\leq\infty $, thus we split the proof into the following two cases.
Case 1: $ p\leq q < N $. In this case, we first differentiate the first equation of Eq (1.11) to obtain the identity
We take the $ L^{q}- $norm of $ \partial^{\beta}_{x}v $, for some $ \eta\in(0, 1) $ to be chosen later, we split the time integral into the following two parts:
We next estimate $ F_{i}(i = 1, 2, 3) $ term by term.
For $ F_{1} $, Lemma 5.2 implies that
For $ F_{2} $, using Lemma 5.2, Lemma 2.3 and Eq (5.2), we have
where $ k = k_{1}+k_{2}+\dots+ k_{N} $ and $ k_{i} = |\beta_{i}|(i = 1, 2, \dots, N) $.
Using Leibniz's rule, we split $ F_{3} $ into the following three parts:
Here, the notation $ \gamma < \beta $ means that $ \gamma\leq\beta $ and $ |\gamma| < |\beta| $.
Now, we establish the estimates for $ F_{3j}(j = 1, 2, 3) $. For $ F_{31} $, using Leibniz's rule again, we can split $ F_{31} $ into two parts as follows:
where we denote $ \sum_{\beta} = \sum_{0 = \beta_{0}\leq\beta_{1}\leq\dots\leq\beta_{m-1} < \beta_{m} = \beta} $.
For $ G_{2} $, using Lemma 2.2, Lemma 2.3 and Eq (5.2), we have
For $ G_{1} $, using Lemma 2.2, Lemma 2.3, Lemma 5.3, Eq (5.2) and Eq (5.12), we have
where
For $ F_{32} $, using the same arguments as $ G_{1} $, we have
according to the property of semigroup we get
where $ \sum_{\gamma} $ is defined the same as that in estimating $ F_{31} $ and $ I(\eta) $ is defined in Eq (5.27).
For $ F_{33} $, analogously we have
where $ I(\eta) $ is defined in Eq (5.27).
Combining the above estimates Eqs (5.20)$ - $(5.29) and setting $ \bar{B}_{\eta} $ by
and
we obtain
Similarly, we can deal with $ \partial^{\beta}_{x}w(t) $. Hence, we conclude that
where $ B_{\eta} = 2\bar{B}_{\eta} $ and $ C_{13} = 2C_{8}(N, \alpha, m, p) $.
Let $ \eta_{k} = \frac{1}{2k} $. It is clear that $ I(\eta_{k}) $ is strictly monotone decreasing in $ k $ and $ I(\eta_{k})\rightarrow 0 $ as $ k\rightarrow \infty $. Choosing $ k_{0} $ sufficiently large, such that $ I(\frac{1}{2k})\leq\frac{1}{2C_{13}} $ for all $ k\geq k_{0} $, applying Lemma 5.4, we get
for all $ t > 0 $ and $ |\beta| = k $. Note that from Eq (5.33), we can choose $ K_{1} $ and $ K_{2} $ sufficiently large such that Eq (5.12) holds for all $ \beta $ satisfying $ |\beta|\leq k_{0} $. Hence, it suffices to prove that it is possible to choose $ K_{1} $ and $ K_{2} $ such that $ 2B_{\frac{1}{2k}}\leq K_{1}(K_{2}k)^{2k-\delta} $ for all $ k > k_{0} $. Since
we can calculate $ 2B_{\frac{1}{2k}} $ as follows:
Obviously, there exists a constant $ C_{14} > C_{0} $ such that $ C^{k}_{0}+2^{\frac{k}{\alpha}+1+\frac{1}{m}}C_{0}^{k}k^{1+\frac{1}{m}+\delta}\leq C_{14}^{2k-\delta} $. Hence,
where $ C_{12} $ is defined in Eq (5.30).
Choosing $ K_{1}: = 8(M_{1}+C_{5}) $ and $ K_{2}: = \max\{C_{14}, 32(C_{9}+C_{10})K_{1}, 32C_{11}K_{1}^{\frac{m}{2}}\} $, we obtain Eq (5.12). This completes the proof of Proposition 1 for $ p\leq q < N $.
Case 2: $ N\leq q\leq\infty $. Now we are in a position to establish the estimate of $ \|\partial^{\beta}_{x}v(t)\|_{L^{q}} $ for $ N\leq q\leq\infty $. For $ p $ satisfying Eq (3.1), using the Gagliardo-Nirenberg inequality [32], we have
Now, from Eq (5.35) and the result of Case 1 we see that
It is clear that there exists a constant $ C_{15}\geq2 $ such that $ k^{4}\leq C_{15}^{2k-\delta} $, thus we have
Hence, we can choose $ K_{1} $ and $ K_{2} $ sufficiently large such that Eq (5.12) holds for all $ p\leq q\leq\infty $. This completes the proof of Proposition 1.
Finally, let us show that under the assumptions of Theorem 5.1, the mild solution $ (v(t), w(t)) $ of Eq (1.1) always satisfies the regularity condition Eq (5.12).
Proposition 2. Under the assumptions of Theorem 5.1, the mild solution $ (v(t), $ $ w(t)) $ satisfies that
for all $ p\leq q\leq\infty $, $ t\in(0, T) $ and $ \beta\in\mathbb{N}^{N}_{0} $, where $ \tilde{K}_{1} $ and $ \tilde{K}_{2} $ are constants depending only on $ M_{1}, M_{2}, m, N, \alpha, p $ and $ \delta $.
Proof. Since the mild solution $ (v(t), w(t)) $ is the limit function of the sequence $ (v_{j}(t), w_{j}(t)) $ of appropriate Picard iterations as follows:
Step 1. We first show that
When $ j = 1 $, following from Eq (5.1) we have
Hence Eq (5.38) holds for $ j = 1 $.
When $ j\geq2 $, using Lemma 2.2 and Lemma 2.3, we have
where $ B = \int^{1}_{0}(1-\tau)^{-\frac{mN}{\alpha p}}\tau^{-1-\frac{1}{m}+\frac{(m+1)N}{\alpha p}}d\tau = \mathcal{B}(1-\frac{mN}{\alpha p}, -\frac{1}{m}+\frac{(m+1)N}{\alpha p}) $ is the standard Beta function which is obviously finite.
For $ w_{j}(t) $ we have the analogous estimate. Then, for $ j = 2, 3, \cdots $, we get
where the constant $ C(N, \alpha, p, m, M_{1}, B) $ is always finite. Therefore Eq (5.38) holds true.
Step 2. To apply the Lemma 5.4, we need to show that $ \|(\partial^{\beta}_{x}v_{1}(t), \partial^{\beta}_{x}w_{1}(t))\|_{L^{q}} $ is locally bounded in $ (0, T) $. Using Lemma 2.3 and Eq (5.1), we have
Similarly, we have a similar estimate on $ w_{j}(t) $. Then $ \|(\partial^{\beta}_{x}v_{1}(t), \partial^{\beta}_{x}w_{1}(t))\|_{L^{q}} $ is locally bounded in $ (0, T) $.
Step 3. Similarly to the proof of Proposition 1, let $ \psi_{j}(t) = \|\partial^{\beta}_{x}v_{j}(t)\|_{L^{q}} $, for all $ j\geq1 $ and $ t\in(0, T) $, we have
Using Lemma 5.4 (the version of sequences), we can choose appropriate constants $ \tilde{K}_{1} $ and $ \tilde{K}_{2} $ such that
For $ w_{j}(t) $ we have the similar estimate. Hence we complete the proof of Proposition 2.
The proof of Theorem 5.1. Now Theorem 5.1 follows immediately from Proposition 1 and Proposition 2. We complete the proof of Theorem 5.1.
6.
A generalized fractional drift diffusion system
In this section, we consider a fractional drift diffusion system with generalized electric potential equation
where $ c $ is a constant and $ b(x, y) $ is the kernel function of nonlocal linear integral operator $ \mathcal{K} $.
For $ \mathcal{K} = (-\Delta)^{-1} $ which comes from the Poisson equation $ \Delta\phi = v-w $, Eq (6.1) becomes the fractional drift diffusion system Eq (1.1). For instance,
where $ c $ is a constant. If $ c < 0 $, the equation $ u_{t} = \Delta u+\nabla\cdot(u\nabla \mathcal{K}(u)) $ models the Brownian diffusion of charge carriers interacting via Coulomb forces. If $ c > 0 $, the operator $ \mathcal{K} $ reflects the mutual gravitational attraction of particles. Furthermore, Biler-Woyczynski [33] considered the equation $ u_{t} = \Lambda^{\alpha} u+\nabla\cdot(u\nabla \mathcal{K}(u)) $.
We also give the global existence and asymptotic stability of the mild solution to the Cauchy problem Eq (6.1).
Theorem 6.1. Let $ N $ be a positive integer, $ 1 < \alpha\leq 2N $ and Eq (3.1) hold true. Assume that $ (v_{0}, w_{0})\in \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $. If the derivative of kernel function $ b(x, y) $ satisfies
then there exists $ \varepsilon > 0 $ such that if $ ||(v_{0}, w_{0})||_{\dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}}\leq\varepsilon $, the Cauchy problem Eq (6.1) has a unique global mild solution $ (v, w)\in \mathcal{X} $ such that $ ||(v, w)||_{\mathcal{X}}\leq2\varepsilon $. Moreover, the solution depends continuously on initial data in the following sense: let $ (\tilde{v}, \tilde{w})\in \mathcal{X} $ be the solution of Eq (6.1) with initial data $ (\tilde{v}_{0}, \tilde{w}_{0}) $ such that $ ||(\tilde{v}_{0}, \tilde{w}_{0})||_{\dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N)}\leq\varepsilon $, then there is a constant $ C $ such that
Proof. After a few modifications of the proof to Theorem 3.1, we can prove this theorem. Here we just give the main difference in the proof.
By the fractional heat semigroup $ S_{\alpha}(t) = e^{-t\Lambda^{\alpha}} $, we rewrite the system Eq (6.1) as a system of integral equations
where
Similar to Eqs (3.4)–(3.8), we have
due to the condition Eq (6.3): $ |Db(x, y)|\leq C|x-y|^{-N+1} $, use Hardy-Littlewood-Sobolev inequality for the integral $ \int_{\mathbb{R}^{N}}|x-y|^{-N+1}|v-w|dy $, we have
then
therefore, we have
Similarly, we have
Following the main estimates Eq (6.10) and Eq (6.11) and the proof of Theorem 3.1, the Cauchy problem Eq (6.1) has a unique global-in-time mild solution in the mixed time-space Besov space. This completes the proof of Theorem 6.1.
Using the same method we can prove that the mild solution of the Cauchy problem Eq (6.1) has the following asymptotic stability.
Theorem 6.2. Let $ N\geq2 $ be a positive integer, $ 1 < \alpha\leq 2N $, Eq (3.1) and Eq (6.3) hold true. Assume that $ (v, w) $ and $ (\tilde{v}, \tilde{w}) $ are two mild solutions of the Cauchy problem Eq (6.1) described in Theorem 6.1 corresponding to initial conditions $ (v_{0}, w_{0}) $ and $ (\tilde{v}_{0}, \tilde{w}_{0}) $, respectively. If $ (v_{0}, w_{0}), (\tilde{v}_{0}, \tilde{w}_{0})\in \dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $ such that
then, we have the following asymptotic stability
Theorem 6.3. Let $ N\geq2 $ be a positive integer, $ 1 < \alpha\leq 2N $, Eq (3.1) and Eq (6.3) hold true. Assume that $ (v_{0}, w_{0})\in\dot{B}^{-\frac{\alpha}{m}+\frac{N}{p}}_{p, \infty}(\mathbb{R}^N) $, and $ (v, w) $ is the mild solution to the system Eq (6.1) with initial data $ (v_{0}, w_{0}) $. Furthermore, assume that there exist two positive constants $ M_{1} $ and $ M_{2} $ such that
Then, there exist two positive constants $ K_{1} $ and $ K_{2} $ depending only on $ M_{1} $, $ M_{2} $, $ N $, $ \alpha $, $ m $ and $ p $, such that
for all $ p\leq q \leq \infty $, $ t\in(0, T) $ and $ \beta\in\mathbb{N}^{N}_{0} $.
Acknowledgments
The authors are grateful to the anonymous referees for helpful comments and suggestions that greatly improved the presentation of this paper. The research of C. Gu is partially supported by the CSC under grant No. 202006160118. The research of C. Gu and Y. Tang is supported by the NNSF of China (Nos. 12171442 and 11971188).
Conflict of interest
The authors have no conflicts in this paper.

















 DownLoad:
DownLoad: