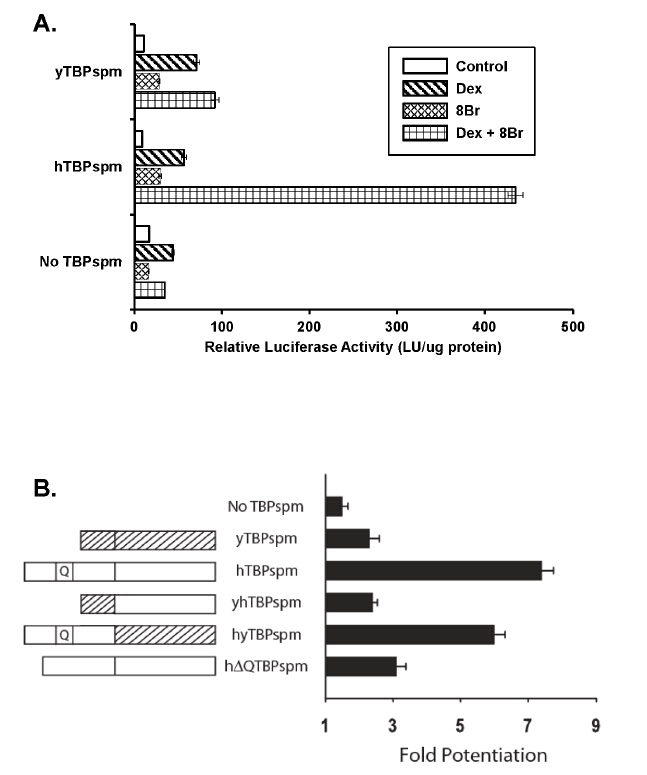
Transcriptional induction by steroid receptors is coupled to cellular signal transduction pathways although,in general,the mechanisms governing these events are not well defined. Using TATA-binding protein (TBP) specificity mutants that recognize a TGTA box,we show that yeast TBP expressed in mammalian cells can support steroid-mediated gene induction to a similar degree as human TBP,however yeast TBP does not support the 8-Bromo-cAMP-mediated potentiation of glucocorticoid receptor (GR)-dependent transactivation. Chimeras between yeast and human TBP reveal that it is the non-conserved N-terminus of TBP that governs the potentiation of GR action. While the conserved core of TBP is sufficient for TATA-element binding and preinitiation complex formation,the role of the N-terminus has remained elusive. Our results suggest a role of the N-terminus of human TBP in coupling cell signaling events to steroid-mediated transcription,thereby establishing one of the few described functional roles of this polypeptide domain in a physiological process.
1.
Introduction
Delle Monache and Goatin developed in [20] a macroscopic model aiming at describing the situation in which a slow moving large vehicle – a bus for instance – reduces the road capacity and thus generates a moving bottleneck for the surrounding traffic flow. Their model is given by a Cauchy problem for Lightwill-Whitham-Richards scalar conservation law in one space dimension with local point constraint. The constraint is prescribed along the slow vehicle trajectory $ (y(t),t) $, the unknown $ y $ being coupled to the unknown $ \rho $ of the constrained LWR equation. Point constraints were introduced in [19,17] to account for localized in space phenomena that may occur at exits and which act as obstacles. The constraint in the model of [20] depends upon the slow vehicle speed $ \dot y $, where its position $ y $ verifies the following ODE
Above, $ \rho = \rho(x,t) \in [0,R] $ is the traffic density and $ \omega: [0,R] \to \mathbb{R}^{+} $ is a nonincreasing Lipschitz continuous function which links the traffic density to the slow vehicle velocity. Delle Monache and Goatin proved an existence result for their model in [20] with a wave-front tracking approach in the $ \mathbf{BV} $ framework. Adjustments to the result were recently brought by Liard and Piccoli in [28]. Despite the step forward made in [21], the uniqueness issue remained open for a time. Indeed, the appearance of the trace $ \rho(y(t)^{+},t) $ makes it fairly difficult to get a Lipschitz continuous dependency of the trajectory $ y = y(t) $ from the solution $ \rho = \rho(x,t) $. Nonetheless, a highly nontrivial uniqueness result was achieved by Liard and Piccoli in [27]. To describe the influence of a single vehicle on the traffic flow, the authors of [26] proposed a PDE-ODE coupled model without constraint on the flux for which they proposed in [9] two convergent schemes. In the present paper, we consider a modified model where the point constraint becomes nonlocal, making the velocity of the slow vehicle depend on the mean density evaluated in a small vicinity ahead the driver. More precisely, instead of A, we consider the relation
where $ \mu \in \mathbf{BV}( \mathbb{R} ; \mathbb{R}^{+}) $ is a weight function used to average the density. From the mathematical point of view, this choice makes the study of the new model easier. Indeed, the authors of [3,4,5] put forward techniques for full well-posedness analysis of similar models with nonlocal point constraints. From the modeling point of view, considering B makes sense for several reasons outlined in Section 3.5.
The paper is organized as follows. Sections 2 and 3 are devoted to the proof of the well-posedness of the model. In Section 4 we introduce the numerical finite volume scheme and prove its convergence. An important step of the reasoning is to prove a $ \mathbf{BV} $ regularity for the approximate solutions. It serves both in the existence proof and it is central in the uniqueness argument. In that optic, the appendix is essential. Indeed, it is devoted to the proof of a $ \mathbf{BV} $ regularity for entropy solutions to a large class of limited flux models. Let us stress that we highlight the interest of the $ \mathbf{BV}_{\mathbf{loc}} $ discrete compactness technique of Towers [33] in the context of general discontinuous-flux problems. In the numerical section 5, first we perform numerical simulations to validate our model. Then we investigate both qualitatively and quantitatively the proximity between our model – in which we considered B – as $ \delta \to \mu_{0^+} $ and the model of [20] in which the authors considered A.
2.
Model, notion of solution and uniqueness
2.1. Model in the bus frame
Note that we find it convenient to study the problem in the bus frame, which means setting $ X = x-y(t) $ in the model of Delle Monache and Goatin in [20]. Keeping in mind what we said above about the nonlocal constraint, the problem we consider takes the following form:
Above, $ \rho = \rho(x,t) $ denotes the traffic density, of which maximum attainable value is $ R > 0 $, and
denotes the normal flux through the curve $ x = y(t) $. We assume that the flux function $ f : [0,R] \to \mathbb{R} $ is Lipschitz continuous and bell-shaped, which are commonly used assumptions in traffic dynamics:
In [20], the authors chose the function $ {{Q(s) = \alpha \times \left(\frac{1-s}{2} \right)^2}} $ to prescribe the maximal flow allowed through a bottleneck located at $ x = 0 $. The parameter $ \alpha \in (0, 1) $ was giving the reduction rate of the road capacity due to the presence of the slow vehicle. We use the $ s $ variable to stress that the value of the constraint is a function of the speed of the slow vehicle. In the sequel the $ s $ variable will refer to quantities related to the slow vehicle velocity. Regarding the function $ Q $, we can allow for more general choices. Specifically,
can be any Lipschitz continuous function. It is a well known fact that in general, the total variation of an entropy solution to a constraint Cauchy problem may increase (see [17,Section 2] for an example). However, this increase can be controlled if the constraint level does not reach the maximum level. A mild assumption on $ Q $ – see Assumption (17) below – will guarantee availability of $ \mathbf{BV} $ bounds, provided we suppose that
2.2. Notion of solution
Throughout the paper, we denote by
the entropy fluxes associated with the Kružkov entropy $ \rho \mapsto |\rho-\kappa| $, for all $ \kappa \in [0,R] $, see [25]. Following [20,17,6,15], we give the following definition of solution for Problem (1).
Definition 2.1. A couple $ (\rho,y) $ with $ \rho \in \mathbf{L}^{{\infty}}( \mathbb{R} \times (0,T)) $ and $ y \in {\mathbf{W}^{{1},{\infty}}}((0,T)) $ is an admissible weak solution to (1) if
(ⅰ) the following regularity is fulfilled:
(ⅱ) for all test functions $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R} \times \mathbb{R}^{+}), \varphi \geq 0 $ and $ \kappa \in [0,R] $, the following entropy inequalities are verified for all $ 0 \leq \tau < \tau' \leq T $:
where
(ⅲ) for all test functions $ \psi \in \mathbf{C}^{{\infty}}([0,T]), \psi \geq 0 $ and some given $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R}) $ which verifies $ \varphi(0) = 1 $, the following weak constraint inequalities are verified for all $ 0 \leq \tau < \tau' \leq T $:
(ⅳ) the following weak ODE formulation is verified for all $ t \in [0,T] $:
Definition 2.2. We will call $ \mathbf{BV} $-regular solution any admissible weak solution $ (\rho,y) $ to the Problem (1) which also verifies
Remark 1. It is more usual to formulate (4) with $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R} \times [0,T)) $, $ \tau = 0 $ and $ \tau' = T $. The equivalence between the two formulations is due to the regularity (3).
Remark 2. As it happens, the time-continuity regularity (3) is actually a consequence of inequalities (4). Indeed, we will use the result [12,Theorem 1.2] which states that if $ \Omega $ is an open subset of $ \mathbb{R} $ and if for all test functions $ \varphi \in \mathbf{C}_c^{{\infty}}(\Omega \times [0,T)), \varphi \geq 0 $ and $ \kappa \in [0,R] $, $ \rho $ satisfies the following entropy inequalities:
then $ {{\rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}(\Omega))}} $. Moreover, since $ \rho $ is bounded and $ \overline{\Omega} \backslash \Omega $ has a Lebesgue measure $ 0 $, $ \rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}(\overline{\Omega})) $. We will use this remark several times in the sequel of the paper, with $ \Omega = \mathbb{R}^{*} $.
Remark 3. Any admissible weak solution $ (\rho,y) $ to Problem (1) is also a distributional solution to the conservation law in (1). Therefore, inequalities (5) imply the following ones for all $ 0 \leq \tau < \tau' \leq T $:
where $ \varphi $ and $ \psi $ are such as described in Definition 2.1 (ⅲ).
The interest of weak formulations (5)-(6) for the flux constraint and for the ODE governing the slow vehicle lies in their stability with respect to $ \rho $. Formulation (4) – (6) is well suited for passage to the limit of a.e. convergent sequences of exact or approximate solutions.
2.3. Uniqueness of the BV-regular solution
In this section, we prove stability with respect to the initial data and uniqueness for $ \mathbf{BV} $-regular solutions to Problem (1). We start with the
Lemma 2.3. If $ (\rho,y) $ is an admissible weak solution to Problem (1), then $ \dot y \in {\mathbf{W}^{{1},{\infty}}}((0,T)) $. In particular, $ \dot y \in \mathbf{BV}([0,T]) $.
Proof. Denote for all $ t \in [0,T] $,
Since $ \mu \in \mathbf{L}^{{1}}( \mathbb{R}) \cap \mathbf{L}^{{\infty}}( \mathbb{R}) $ and $ \rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $, $ s $ is continuous on $ [0,T] $. By definition, $ y $ satisfies the weak ODE formulation (6). Consequently, for a.e. $ t \in (0,T) $, $ \dot y(t) = s(t) $. We are going to prove that $ s $ is Lipschitz continuous on $ [0,T] $, which will ensure that $ \dot y \in {\mathbf{W}^{{1},{\infty}}}((0,T)) $. Since $ \mu \in \mathbf{BV}( \mathbb{R}) $, there exists a sequence $ {{(\mu_{n})_{n \in \mathbb{N}} \subset \mathbf{BV}( \mathbb{R}) \cap \mathbf{C}_c^{{\infty}}( \mathbb{R})}} $ such that:
Introduce for all $ n \in \mathbb{N} $ and $ t \in [0,T] $, the function
Fix $ \psi \in \mathbf{C}_c^{{\infty}}((0,T)) $. Since $ \rho $ is a distributional solution to the conservation law in (1), we have for all $ n \in \mathbb{N} $,
which means that for all $ n \in \mathbb{N} $, $ \xi_{n} $ is differentiable in the weak sense, and that for a.e. $ t \in (0,T) $,
In particular, since both the sequences $ (\|\mu_{n}\|_{\mathbf{L}^{{1}}})_{n} $ and $ ( \mathbf{TV}(\mu_{n}))_{n} $ are bounded – say by $ C > 0 $ – we also have for all $ n \in \mathbb{N} $,
Therefore, the sequence $ (\xi_{n})_{n} $ is bounded in $ {\mathbf{W}^{{1},{\infty}}}((0,T)) $. Now, for all $ t,\tau \in [0,T] $ and $ n \in \mathbb{N} $, triangle inequality yields:
Letting $ n \to + \infty $, we get that for all $ t,\tau \in [0,T] $, $ {{|s(t) - s(\tau)| \leq K|t - \tau|}} $, which proves that $ s $ is Lipschitz continuous on $ [0,T] $. The proof of the statement is completed.
Before stating the uniqueness result, we make the following additional assumption:
This ensures that for all $ s \in [0,\|\omega\|_{\mathbf{L}^{{\infty}}}] $, the function $ F(s,\cdot) $ verifies the bell-shaped assumptions (53). For example, when considering the flux $ f(\rho) = \rho(R-\rho) $, (7) reduces to $ \|\omega\|_{\mathbf{L}^{{\infty}}} < R $, which only means that the maximum velocity of the slow vehicle is lesser than the maximum velocity of the cars.
Theorem 2.4. Suppose that $ f $ satisfies (2) and (7). Fix $ \rho_{0}^{1}, \rho_{0}^{2} \in \mathbf{L}^{{1}}( \mathbb{R}; [0,R]) \cap \mathbf{BV}( \mathbb{R}) $ and $ y_{0}^{1}, y_{0}^{2} \in \mathbb{R} $. We denote by $ (\rho^{1},y^{1}) $ a $ \mathbf{BV} $-regular solution to Problem (1) corresponding to initial data $ (\rho_{0}^{1}, y_{0}^{1}) $, and by $ (\rho^{2},y^{2}) $ an admissible weak solution with initial data $ (\rho_{0}^{2},y_{0}^{2}) $. Then there exist constants $ \alpha,\beta,\gamma > 0 $ such that
and
In particular, Problem (1) admits at most one $ \mathbf{BV} $-regular solution.
Proof. Since $ (\rho^1 ,y^1) $ is a $ \mathbf{BV} $-regular solution to Problem (1), there exists $ C \geq 0 $ such that
Lemma 2.3 ensures that $ \dot y^1, \dot y^2 \in \mathbf{BV}([0,T] ; \mathbb{R}^+) $. We can use result (54) to obtain that for a.e. $ t \in (0,T) $,
Moreover, since for a.e. $ t \in (0,T) $,
Gronwall's lemma yields (8) with $ {{\alpha = \left( 2 \|Q'\|_{\mathbf{L}^{{\infty}}} + 2R + C \right) \|\omega'\|_{\mathbf{L}^{{\infty}}}\|\mu\|_{\mathbf{L}^{{\infty}}}}} $. Then for all $ t \in [0,T] $,
where
The uniqueness of a $ \mathbf{BV} $-regular solution is then clear.
Remark 4. Up to inequality (10), our proof was very much following the one of [21,Theorem 2.1]. However, the authors of [21] faced an issue to derive a Lipschitz stability estimate between the car densities and the slow vehicle velocities starting from
For us, due to the nonlocality of our problem, it was straightforward to obtain the bound
Remark 5. A noteworthy consequence of Theorem 2.4 is that existence of a $ \mathbf{BV} $-regular solution will ensure uniqueness of an admissible weak one.
3.
Two existence results
3.1. Time-splitting technique
In [20], to prove existence for their problem, the authors took a wave-front tracking approach. We choose here to use a time-splitting technique. The main advantage of this technique is that it relies on a ready-to-use theory. More precisely, at each time step, we will deal with exact solutions to a conservation law with a flux constraint, which have now become standard, see [17,6,15].
Fix $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) $ and $ y_{0} \in \mathbb{R} $. Let $ \delta > 0 $ be a time step, $ N \in \mathbb{N} $ such that $ T \in [N \delta, (N+1)\delta) $ and denote for all $ n \in \{0, \ldots, N+1\} $, $ t^{n} = n \delta $. We initialize with
Fix $ n \in \{1, \ldots ,N+1\} $. First, we define for all $ t \in (t^{n-1},t^{n}] $,
Since both $ q^n $ and $ \rho^{n-1}(\cdot, t^{n-1}) $ are bounded, [6,Theorem 2.11] ensures the existence and uniqueness of a solution $ \rho^n \in \mathbf{L}^{{\infty}}( \mathbb{R} \times [t^{n-1},t^{n}]) $ to
in the sense that $ \rho^n $ satisfies entropy/constraint inequalities analogous to (4)-(5) with suitable flux/constraint function and initial data, see Definition A.1. Taking also into account Remark 2, $ \rho^{n} \in \mathbf{C}^{{}}([t^{n-1},t^{n}] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $. We then define the following functions:
First, let us prove that $ (\rho_{\delta},y_{\delta}) $ solves an approximate version of Problem (1).
Proposition 1. The couple $ (\rho_{\delta},y_{\delta}) $ is an admissible weak solution to
in the sense that $ \rho_\delta \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $ and satisfies entropy/constraint inequalities analogous to (4)-(5) with flux $ F(s_{\delta}(\cdot), \cdot) $, constraint $ q_\delta $, and initial data $ \rho_0(\cdot + y_0) $; and $ y_{\delta} $ satisfies, instead of (6), the following weak ODE formulation:
Proof. By construction, for all $ n \in \{1, \ldots, N+1\}, \ \rho^{n} \in \mathbf{C}^{{}}([t^{n-1},t^{n}] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $. Combining this with the "stop-and-restart" conditions $ \rho^{n}(\cdot,t^{n-1}) = \rho^{n-1}(\cdot,t^{n-1}) $, one ensures that $ \rho_{\delta} \in \mathbf{C}^{{}}([0, T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $. Let $ t \in [0,T] $ and $ n \in \{1, \ldots, N+1\} $ such that $ t \in [t^{n-1},t^{n}) $. Then,
which proves that $ \dot y_\delta $ solves the ODE in (11) in the weak sense. Fix now $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R} \times \mathbb{R}^{+}), \varphi \geq 0 $ and $ \kappa \in [0,R] $. By construction of the sequence $ ((\rho^{k},y^{k}))_{k} $, we have for all $ n,m \in \{ 0, \ldots, N+1\} $,
It is then straightforward to prove that for all $ 0 \leq \tau < \tau' \leq T $,
Proving that $ \rho_\delta $ satisfies constraint inequalities is very similar so we omit the details. One has to start from
and make use once again of the construction of the sequence $ ((\rho^{k},y^{k}))_{k} $ to obtain
This concludes the proof.
Remark 6. Remark that we have for all $ \delta > 0 $,
This means that the sequence $ (y_{\delta})_{\delta} $ is bounded in $ {\mathbf{W}^{{1},{\infty}}}((0,T)) $. Then the compact embedding of $ {\mathbf{W}^{{1},{\infty}}}((0,T)) $ in $ \mathbf{C}^{{}}([0,T]) $ yields a subsequence of $ (y_{\delta})_{\delta} $, which we do not relabel, which converges uniformly on $ [0,T] $ to some $ y \in \mathbf{C}^{{}}([0,T]) $.
At this point, we propose two ways to obtain compactness for the sequence $ (\rho_{\delta})_{\delta} $, which will lead to two existence results.
3.2. The case of a nondegenerately nonlinear flux
Theorem 3.1. Fix $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0;R]) $ and $ y_{0} \in \mathbb{R} $. Suppose that $ f $ is Lipschitz continuous, satisfies (2)-(7) and the following nondegeneracy assumption
Then Problem (1) admits at least one admissible weak solution.
Proof. Condition (15) combined with the obvious uniform $ \mathbf{L}^{{\infty}} $ bound
and the results proved by Panov in [30,31] ensure the existence of a subsequence – which we do not relabel – that converges in $ \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R}^{*} \times (0,T)) $ to some $ \rho \in \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R}^{*} \times (0,T)) $; and a further extraction yields the almost everywhere convergence on $ \mathbb{R} \times (0,T) $ and also the fact that $ \rho \in \mathbf{L}^{{\infty}}( \mathbb{R} \times (0,T) ; [0,R]) $. We now show that the couple $ (\rho,y) $ constructed above is an admissible weak solution to (1) in the sense of Definition 2.1.
For all $ \delta > 0 $ and $ t \in [0,T] $,
The last term vanishes as $ \delta \to 0 $ since $ \omega $ is bounded. Then, Lebesgue theorem combined with the continuity of $ \omega $ gives, for all $ t \in [0,T] $,
This last quantity is also equal to $ y(t) $ due to the uniform convergence of $ (y_{\delta})_{\delta} $ to $ y $. This proves that $ y $ verifies (6). Now, we aim at passing to the limit in (13) and (14). With this in mind, we prove the a.e. convergence of the sequence $ (\sigma_{\delta})_{\delta} $ towards $ \dot y $. Since $ \mu \in \mathbf{BV}( \mathbb{R}) $, there exists a sequence of smooth functions $ (\mu_{n})_{n \in \mathbb{N}} \subset \mathbf{BV}( \mathbb{R}) \cap \mathbf{C}_c^{{\infty}}( \mathbb{R}) $ such that:
Introduce for every $ \delta > 0 $ and $ n \in \mathbb{N} $, the function
Since for all $ \delta > 0 $, $ \rho_{\delta} $ is a distributional solution to the conservation law in (11), one can show – following the proof of Lemma 2.3 for instance – that for every $ n \in \mathbb{N} $, $ \xi_{\delta}^{n} \in {\mathbf{W}^{{1},{\infty}}}((0,T)) $, and that for a.e. $ t \in (0,T) $,
Moreover, since both the sequences $ \left( \|\mu_{n}\|_{\mathbf{L}^{{1}}} \right)_{n} $ and $ ( \mathbf{TV}(\mu_{n}))_{n} $ are bounded, it is clear that $ \left(\xi_{\delta}^{n}\right)_{\delta,n} $ is uniformly bounded in $ {\mathbf{W}^{{1},{\infty}}}((0,T)) $, therefore so is $ \left(\omega (\xi_{\delta}^{n})\right)_{\delta,n} $. Consequently, for all $ n \in \mathbb{N} $, $ \delta > 0 $ and almost every $ t \in (0,T) $, triangle inequality yields:
which proves that $ (\sigma_{\delta})_{\delta} $ converges a.e. on $ (0,T) $ to $ \dot y $. To prove the time-continuity regularity, we first apply inequality (13) with $ \tau = 0 $, $ \tau' = T $ (which is licit since $ \rho_\delta $ is continuous in time), $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R}^{*} \times [0,T)), \varphi \geq 0 $ and $ \kappa \in [0,R] $:
Then, we let $ \delta \to 0 $ to get
Consequently, $ \rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $, see Remark 2. Finally, the a.e. convergences of $ (\sigma_{\delta})_{\delta} $ and $ (\rho_{\delta})_\delta $ to $ \dot y $ and $ \rho $, respectively, are enough to pass to the limit in (13). This ensures that for all test functions $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R} \times \mathbb{R}^{+}), \varphi \geq 0 $ and $ \kappa \in [0,R] $, the following inequalities hold for a.e. $ 0 \leq \tau < \tau' \leq T $:
Observe that the expression in the left-hand side of the previous inequality is a continuous function of $ (\tau,\tau') $ which is almost everywhere greater than the continuous function $ 0 $. By continuity, this expression is everywhere greater than $ 0 $, which proves that $ \rho $ satisfies the entropy inequalities (4). Using similar arguments, we show that $ \rho $ satisfies the constraint inequalities (5). This proves the couple $ (\rho,y) $ is an admissible weak solution to Problem (1), and this concludes the proof.
In this section, we proved an existence result for $ \mathbf{L}^{{\infty}} $ initial data, but we have no guarantee of uniqueness since a priori we have no information regarding the $ \mathbf{BV} $ regularity of such solutions.
Assumption (15) ensures the compactness for sequences of entropy solutions to conservation laws with flux function $ F $. However, it prevents us from using flux functions with linear parts – which corresponds to constant traffic velocity for small densities – whereas such fundamental diagrams are often used in traffic modeling. The results of the next section will extend to this interesting case, under the extra $ \mathbf{BV} $ assumption on the data.
3.3. Well-posedness for BV data
To obtain compactness for $ (\rho_\delta)_\delta $, an alternative to the setting of Section 3.2 is to derive uniform $ \mathbf{BV} $ bounds.
Theorem 3.2. Fix $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) \cap \mathbf{BV}( \mathbb{R}) $ and $ y_{0} \in \mathbb{R} $. Suppose that $ f $ satisfies (2) and (7). Suppose also that
where $ \overline{\rho}_s = \underset{\rho \in [0,R]}{{\rm{argmax}}} \ F(s,\rho) $. Finally assume that $ Q $ satisfies the condition
Then Problem (1) admits a unique admissible weak solution, which is also $ \mathbf{BV} $-regular.
Proof. Fix $ \delta > 0 $. Recall that $ (\rho_{\delta},y_{\delta}) $ is an admissible weak solution to (11). In particular, $ \rho_\delta $ is an admissible weak solution to the constrained conservation law in (11), in the sense of Definition A.1. It is clear from the splitting construction that for a.e. $ t \in (0,T) $,
Following the steps of the proof of Lemma 2.3, we can show that for all $ \delta > 0 $, $ \sigma_{\delta} \in \mathbf{BV}([0,T]; \mathbb{R}^+) $. Even more than that, by doing so we show that the sequence $ \left( \mathbf{TV}(\sigma_{\delta}) \right)_{\delta} $ is bounded. Therefore, the sequence $ \left( \mathbf{TV}(s_{\delta}) \right)_{\delta} $ is bounded as well. Moreover, since $ Q $ verifies (17), all the hypotheses of Corollary 2 are fulfilled. Combining this with Remark 15, we get the existence of a constant $ \mathbf{C}^{{ \varepsilon}} = \mathbf{C}^{{ \varepsilon}} (\| \partial_s F \|_{\mathbf{L}^{{\infty}}}) $ such that for all $ t \in [0,T] $,
Consequently for all $ t \in [0,T] $, the sequence $ (\rho_{\delta}(t))_{\delta} $ is bounded in $ \mathbf{BV}( \mathbb{R}) $. A classical analysis argument – see [24,Theorem A.8] – ensures the existence of $ \rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $ such that
With this convergence, we can follow the proof of Theorem 3.1 to show that $ (\rho,y) $ is an admissible weak solution to (1). Then, when passing to the limit in (18), the lower semi-continuity of the $ \mathbf{BV} $ semi-norm ensures that $ (\rho,y) $ is also $ \mathbf{BV} $-regular. By Remark 5, it ensures uniqueness and concludes the proof.
3.4. Stability with respect to the weight function
To end this section, we now study the stability of Problem (1) with respect to the weight function $ \mu $. More precisely, let $ \left(\mu^{\ell}\right)_{\ell} \subset \mathbf{BV}( \mathbb{R} ; \mathbb{R}^{+}) $ be a sequence of weight functions that converges to $ \mu $ in the weak $ \mathbf{L}^{{1}} $ sense:
Let $ (y_{0}^{\ell})_{\ell} \subset \mathbb{R} $ be a sequence of real numbers that converges to some $ y_{0} $ and let $ (\rho_{0}^{\ell})_{\ell} \subset \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) $ be a sequence of initial data that converges to $ \rho_{0} $ in the strong $ \mathbf{L}^{{1}} $ sense. We suppose that the flux function $ f $ satisfies Assumptions (2)-(7)-(15). Theorem 3.1 allows us to define or all $ \ell \in \mathbb{N} $, the couple $ (\rho^{\ell},y^{\ell}) $ as an admissible weak solution to the problem
Remark 7. Using the same arguments as in Remark 6 and as in the proof of Theorem 3.1, we get that up to the extraction of a subsequence, $ (y^{\ell})_{\ell} $ converges uniformly on $ [0,T] $ to some $ y \in \mathbf{C}^{{}}([0,T]) $ and $ (\rho^{\ell})_{\ell} $ converges a.e. on $ \mathbb{R} \times (0,T) $ to some $ \rho \in \mathbf{L}^{{\infty}}( \mathbb{R} \times (0,T)) $.
Theorem 3.3. The couple $ (\rho,y) $ constructed above is an admissible weak solution to Problem (1).
Proof. The sequence $ (\mu^{\ell})_{\ell} $ converges in the weak $ \mathbf{L}^{{1}} $ sense and is bounded in $ \mathbf{L}^{{1}}( \mathbb{R}) $; by the Dunford-Pettis theorem, this sequence is equi-integrable:
and
Fix $ t \in (0,T) $ and $ \varepsilon > 0 $. Fix $ \alpha,X > 0 $ given by (20) and (21). Egoroff theorem yields the existence of a measurable subset $ E_{t} \subset [-X,X] $ such that
For a sufficiently large $ \ell \in \mathbb{N} $,
which proves that for a.e. $ t \in (0,T) $,
We get that $ y $ verifies the weak ODE formulation (6) by passing to the limit in
By definition, for all $ \ell \in \mathbb{N} $, the couple $ (\rho^{\ell},y^{\ell}) $ satisfies the analogue of entropy/constraint inequalities (4)-(5) with suitable flux/constraint functions. Applying these inequalities with $ \tau = 0 $, $ \tau' = T $, $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R}^{*} \times [0,T)), \varphi \geq 0 $ and $ \kappa \in [0,R] $, we get
The continuity of $ \omega $ and the convergence (22) ensure that $ (\dot y^{\ell})_{\ell} $ converges a.e. to $ \dot y $. This combined with the a.e. convergence of $ (\rho^{\ell})_{\ell} $ to $ \rho $ and Riesz-Frechet-Kolmogorov theorem – $ \left( \rho_{0}^{\ell} \right)_{\ell} $ being strongly compact in $ \mathbf{L}^{{1}}( \mathbb{R}) $ – is enough to show that when letting $ \ell \to +\infty $ in the inequality above, we get, up to the extraction of a subsequence, that
Consequently $ \rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $, see Remark 2. Finally, the combined a.e. convergences of $ (\dot y^{\ell})_{\ell} $ and $ (\rho^{\ell})_{\ell} $ to $ \dot y $ and $ \rho $, respectively, guarantee that $ (\rho,y) $ verifies inequalities (4)-(5) for almost every $ 0 \leq \tau < \tau' \leq T $. The same continuity argument we used in the proof Theorem 3.1 holds here to ensure that $ (\rho,y) $ actually satisfies the inequalities for all $ 0 \leq \tau < \tau' \leq T $. This concludes the proof of our stability claim.
3.5. Discussion
The last section concludes the theoretical analysis of Problem (1). The nonlocality in space of the constraint delivers an easy proof of stability with respect to the initial data in the $ \mathbf{BV} $ framework. Although a proof of existence using a fixed point theorem was possible (cf. [5]), we chose to propose a proof based on a time-splitting technique. The stability with respect to $ \mu $ is a noteworthy feature, which shows a certain sturdiness of the model. However, the case we had in mind – namely $ \mu \to \delta_{0^{+}} $ – is not reachable with the assumptions we used to prove the stability, especially (19). We will explore this singular limit numerically, after having built a robust convergent numerical scheme for Problem (1). Let us also underline that unlike in [27,28] where the authors required a particular form for the function $ \omega $ to prove well-posedness for their model, our result holds as long as $ \omega $ is Lipschitz continuous.
As evoked earlier, the nonlocality in space of the constraint makes the mathematical study of the model easier. But in the modeling point of view, this choice also makes sense for several reasons. First of all, one can think that the velocity $ \dot y $ of the slow moving vehicle – unlike its acceleration – is a rather continuous value. Even if the driver of the slow vehicle suddenly applies the brakes, the vehicle will not decelerate instantaneously. Note that the LWR model allows for discontinuous averaged velocity of the agents, however while modeling the slow vehicle we are concerned with an individual agent and can model its behavior more precisely. Moreover, considering the mean value of the traffic density in a vicinity ahead of the driver could be seen at taking into account both the driver anticipation and a psychological effect. For example, if the driver sees – several dozens of meters ahead of him/her – a speed reduction on traffic, he/she will start to slow down. This observation can be related to the fact that, compared to the fluid mechanics models where the typical number of agents is governed by the Avogadro constant, in traffic models the number of agents is at least $ 10^{20} $ times less. Therefore, a mild nonlocality (evaluation of the downstream traffic flow via averaging over a handful of preceding cars) is a reasonable assumption in the macroscopic traffic models inspired by fluid mechanics. This point of view is exploited in the model of [16]. Note that it is feasible to substitute the basic LWR equation on $ \rho $ by the nonlocal LWR introduced in [16] in our nonlocal model for the slow vehicle. Such mildly nonlocal model remains close to the basic local model of [20]. It can be studied combining the techniques of [16] and the ones we developed in this section.
4.
Numerical approximation of the model
In this section, we aim at constructing a finite volume scheme and at proving its convergence toward the $ \mathbf{BV} $-regular solution to (1). We will use the notations:
Fix $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) $ and $ y_{0} \in \mathbb{R} $.
4.1. Finite volume scheme in the bus frame
For a fixed spatial mesh size $ \Delta x $ and time mesh size $ \Delta t $, let $ x_{j} = j\Delta x $, $ t^{n} = n \Delta t $. We define the grid cells $ \mathbf{K}_{j+1/2} = (x_{j},x_{j+1}) $. Let $ N \in \mathbb{N} $ such that $ T \in [t^N, t^{N+1}) $. We write
We choose to discretize the initial data $ \rho_{0}(\cdot + y_{0}) $ and the weight function $ \mu $ with $ \left(\rho_{j+1/2}^{0}\right)_{j \in \mathbb{Z}} $ and $ \left(\mu_{j+1/2}\right)_{j \in \mathbb{Z}} $ where for all $ j \in \mathbb{Z} $, $ \rho_{j+1/2}^{0} $ and $ \mu_{j+1/2} $ are their mean values on the cell $ \mathbf{K}_{j+1/2} $.
Remark 8. Others choice could be made, for instance in the case $ \rho_{0} \in \mathbf{C}^{{}}( \mathbb{R}) $ such that $ {{\lim_{|x| \to +\infty} \rho_{0}(x)}} $ exists (in which case, the limit is zero due to the integrability assumption), the values $ \rho_{j+1/2}^{0} = \rho_{0}\left(x_{j+1/2} + y_{0}\right) $ can be used. The only requirements are
Fix $ n \in \{0, \ldots, N-1\} $. At each time step we first define an approximate velocity of the slow vehicle $ s^{n+1} $ and a constraint level $ q^{n+1} $:
With these values, we update the approximate traffic density with the marching formula for all $ j \in \mathbb{Z} $:
where, following the recipe of [6,15],
$ \mathbf{F}^{n+1} $ being a monotone consistent and Lipschitz numerical flux associated to $ F(s^{n+1},\cdot) $. We will also use the notation
where $ \mathbf{H}_{j}^{n+1} $ is given by the expression in the right-hand side of (24). We then define the functions
Let $ \Delta = (\Delta x, \Delta t) $. For our convergence analysis, we will assume that $ \Delta \to 0 $, with $ \lambda = \Delta t / \Delta x $ verifying the CFL condition
where $ \mathbf{F}^s = \mathbf{F}^s(a, b) $ is the numerical flux, associated to $ F(s,\cdot) $, we use in (24).
Remark 9. When considering the Rusanov flux or the Godunov one, (27) is guaranteed when
4.2. Stability and discrete entropy inequalities
Proposition 2 ($ \mathbf{L}^{{\infty}} $ stability). The scheme (26) is
(i) monotone: for all $ n \in \{0, \ldots, N-1\} $ and $ j \in \mathbb{Z} $, $ \mathbf{H}_{j}^{n+1} $ is nondecreasing with respect to its three arguments;
(ii) stable:
Proof. (i) In the classical case – $ j \notin \{-1,0\} $ – we simply differentiate the Lipschitz function $ \mathbf{H}^{n+1}_{j} $ and make use of both the CFL condition (27) and the monotonicity of $ \mathbf{F}^{n+1} $. For $ j \in \{-1,0\} $, note that the authors of [6] pointed out (in Proposition 4.2) that the modification done in the numerical flux (25) does not change the monotonicity of the scheme.
(ii) The $ \mathbf{L}^{{\infty}} $ stability is a consequence of the monotonicity and also of the fact that $ 0 $ and $ R $ are stationary solutions of the scheme. Indeed, as in [6,Proposition 4.2] for all $ n \in \{ 0, \ldots, N \} $ and $ j \in \mathbb{Z} $,
In order to show that the limit of $ (\rho_{\Delta})_{\Delta} $ – under the a.e. convergence up to a subsequence – is a solution of the conservation law in (1), we derive discrete entropy inequalities. These inequalities also contain terms that will help to pass to the limit in the constrained formulation of the conservation law, as soon as the sequence $ (q_{\Delta})_\Delta $ of constraints is proved convergent as well.
Proposition 3 (Discrete entropy inequalities). The numerical scheme (26) fulfills the following inequalities for all $ n \in \{0, \ldots, N-1\} $, $ j \in \mathbb{Z} $ and $ \kappa \in [0,R] $:
where $ \Phi_{j}^{n} $ and $ \Phi_{int}^{n} $ denote the numerical entropy fluxes:
and
Proof. This result is a direct consequence of the scheme monotonicity. When the constraint does not enter the calculations i.e. $ j \notin \{-1,0\} $, the proof follows [23,Lemma 5.4]. The key point is not only the monotonicity, but also the fact that in the classical case, all the constants $ \kappa \in [0,R] $ are stationary solutions of the scheme. This observation does not hold when the constraint enters the calculations. For example if $ j = -1 $,
Consequently, we have both
and
By substracting these last two inequalities, we get
which is exactly (29) in the case $ j = -1 $. The case $ j = 0 $ is similar so we omit the details of the proof for this case.
Starting from (24) and (29), we can obtain approximate versions of (4) and (5). Let us introduce the functions:
Proposition 4 (Approximate entropy/constraint inequalities). (i) Fix $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R} \times \mathbb{R}^{+}), \varphi \geq 0 $ and $ \kappa \in [0,R] $. Then there exists a constant $ \mathbf{C}^{{\varphi}}_1 = \mathbf{C}^{{\varphi}}_1(R,T,\mathbf{L}^{{}}) $, nondecreasing with respect to its arguments, such that the following inequalities hold for all $ 0 \leq \tau < \tau' \leq T $:
(ii) Fix $ \psi \in \mathbf{C}^{{\infty}}([0,T]), \psi \geq 0 $ and $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R}) $ such that $ \varphi(0) = 1 $. Then there exists a constant $ \mathbf{C}^{{\varphi, \psi}}_2 = \mathbf{C}^{{\varphi, \psi}}_2 (R,T,\mathbf{L}^{{}},\|Q\|_{\mathbf{L}^{{\infty}}}) $, nondecreasing with respect to its arguments, such that for all $ 0 \leq \tau < \tau' \leq T $:
Proof. Fix $ k,m \in \mathbb{N} $ such that $ \tau \in [t^{k},t^{k+1}) $ and $ \tau' \in [t^{m},t^{m+1}) $.
(i) Define for all $ n \in \mathbb{N} $ and $ j \in \mathbb{Z} $, $ {{\varphi_{j+1/2}^{n} = \frac{1}{\Delta x \Delta t} \iint_{ \mathcal{P}_{j+1/2}^{n}} \varphi(x,t) \mathinner{\mathrm{d}{x}} \mathinner{\mathrm{d}{t}}}} $. Multiplying the discrete entropy inequalities (29) by $ \varphi_{j+1/2}^{n} $, then summing over $ n \in \{k,\ldots,m-1\} $ and $ j \in \mathbb{Z} $, one obtains after reorganization of the sums (using in particular the Abel/"summation-by-parts" procedure)
with
Inequality (30) follows from (32) with
making use of the bounds:
(ii) In this case, the constant $ \mathbf{C}^{{\varphi, \psi}}_2 $ reads
Following the proof of (30), define for all $ n \in \mathbb{N} $ and $ j \in \mathbb{Z} $,
multiply the scheme (24) by $ \varphi_{j+1/2} \psi^{n} $, then take the sum over $ n \in \{k,\ldots,m-1\} $ and $ j \geq 0 $. Since the proof is very similar to the one of (i), we omit the details.
The final step is to obtain compactness for the sequences $ (\rho_{\Delta})_{\Delta} $ and $ (y_{\Delta})_{\Delta} $ in order to pass to the limit in (30)-(31). We start with $ (y_{\Delta})_{\Delta} $.
Proposition 5. For all $ t \in [0,T] $,
Consequently, there exists $ y \in \mathbf{C}^{{}}([0,T]) $ such that up to an extraction, $ (y_{\Delta})_{\Delta} $ converges uniformly to $ y $ on $ [0,T] $.
Proof. For all $ t \in [0,T] $, if $ t \in [t^{n},t^{n+1}) $ for some $ n \in \{0, \ldots, N\} $, then we can write
Let us also point out that from (23), we get that for all $ \Delta $ and almost every $ t \in (0,T) $,
Combining (33) and (34), we obtain that for all $ \Delta $,
The sequence $ (y_{\Delta})_{\Delta} $ is therefore bounded in $ {\mathbf{W}^{{1},{\infty}}}((0,T)) $. Making use of the compact embedding of $ {\mathbf{W}^{{1},{\infty}}}((0,T)) $ in $ \mathbf{C}^{{}}([0,T]) $, we get the existence of $ y \in \mathbf{C}^{{}}([0,T]) $ such that up to the extraction of subsequence, $ (y_{\Delta})_{\Delta} $ converges uniformly to $ y $ on $ [0,T] $.
The presence of a time dependent flux in the conservation law of (1) complicates the obtaining of compactness for $ (\rho_{\Delta})_{\Delta} $. In particular, the techniques used in [10,11] to derive localized $ \mathbf{BV} $ estimates don't apply here since our problem lacks time translation invariance. In the present situation, it would be possible to derive weak $ \mathbf{BV} $ estimates ([6,23]). We choose different options. Similarly to what we did in Section 3, we propose two ways to obtain compactness, which will lead to two convergence results.
4.3. Compactness via one-sided Lipschitz condition technique
First, we choose to adapt techniques and results put forward by Towers in [33]. With this in mind, we suppose in this section that $ f \in \mathbf{C}^{{2}}([0,R]) $ and strictly concave. Therefore,
Though this assumption is stronger than the nondegeneracy one (15), since $ f $ is bell-shaped, these two assumptions are similar in their spirit. We will also assume, following [33], that
To be precise, the choice made for the numerical flux at the interface – i.e. when $ j = 0 $ in (25) – does not play any role. What is important is that away from the interface, one chooses either the Engquist-Osher flux or the Godunov one. We denote for all $ n \in \{0, \ldots, N+1\} $ and $ j \in \mathbb{Z} $,
We will also use the notation
In [33], the author dealt with a discontinuous in both time and space flux and the specific "vanishing viscosity" coupling at the interface. The discontinuity in space was localized along the curve $ \{x = 0\} $. Here, we deal with only a discontinuous in time flux, but we also have a flux constraint along the curve $ \{x = 0\} $ since we work in the bus frame. The applicability of the technique of [33] for our case with moving interface and flux-constrained interface coupling relies on the fact that one can derive a bound on $ \mathbf{D}_{j}^{n} $ as long as the "interface" does not enter the calculations for $ \mathbf{D}_{j}^{n} $ i.e. $ j \in \hat{\mathbb{Z}} $. This is what the following lemma points out under Assumptions (35)-(36). For readers' convenience and in order to highlight the generality of the technique of Towers [33], let us provide the key elements of the argumentation leading to compactness.
Lemma 4.1. Let $ n \in \{0, \ldots, N-1\} $ and $ j \in \hat{ \mathbb{Z}} $. Then if $ {{a = \frac{\lambda \alpha}{4}}} $, we have
and
Proof. (Sketched) Inequality (38) is an immediate consequence of inequality (37), see [33,Lemma 4.3]. Obtaining inequality (37) however, is less immediate. Let us give some details of the proof.
First, note that by introducing the function $ \psi : z \mapsto z - az^{2} $, inequality (37) can be stated as:
Then, one can show – only using the monotonicity of both the scheme and the function $ \psi $ – that under the assumption inequality (39) holds when
it follows that inequality (39) holds for all cases. And finally in [33,Page 23], the author proves that if the flux considered is either the Engquist-Osher flux or the Godunov flux, then (40) holds.
The following lemma is an immediate consequence of inequality (38).
Lemma 4.2. Fix $ 0 < \varepsilon < X $. Let $ i,J \in \mathbb{N}^{*} $ such that $ \varepsilon \in \mathbf{K}_{i+1/2} $ and $ X \in \mathbf{K}_{J-1/2} $. Then if $ \Delta x / \varepsilon $ is sufficiently small, there exists a constant $ {{ \mathbf{B} = \mathbf{B}\left(R,X,\frac{1}{a},\frac{1}{ \varepsilon}\right)}} $, nondecreasing with respect to its arguments, such that for all $ n \geq i-1 $,
and
Proposition 6. There exists $ \rho \in \mathbf{L}^{{\infty}}( \mathbb{R} \times (0,T)) $ such that up to the extraction of a subsequence, $ (\rho_{\Delta})_{\Delta} $ converges almost everywhere to $ \rho $ in $ \mathbb{R} \times (0,T) $.
Proof. Fix $ 0 < \varepsilon < X $ and $ t > \lambda \varepsilon $. Denote by $ {{\Omega(X, \varepsilon) = (-X,- \varepsilon) \cup ( \varepsilon, X)}} $. Introduce $ i,J,n \in \mathbb{N} $ such that $ \varepsilon \in \mathbf{K}_{i+1/2} $, $ X \in \mathbf{K}_{J-1/2} $ and $ t \in [t^{n},t^{n+1}) $. Remark that
i.e. $ n \geq i-1 $. Then if we suppose that $ \Delta x / \varepsilon $ is sufficiently small, we can use Lemma 4.2. From (41), we get
and from (42), we deduce
Combining (43)-(44) and the $ \mathbf{L}^{{\infty}} $ bound (28), a functional analysis result ([24,Theorem A.8]) ensures the existence of a subsequence which converges almost everywhere to some $ \rho $ on $ \Omega(X, \varepsilon) \times (\lambda \varepsilon,T) $. By a standard diagonal process we can extract a further subsequence (which we do not relabel) such that $ (\rho_{\Delta})_{\Delta} $ converges almost everywhere to $ \rho $ on $ \mathbb{R} \times (0,T) $.
Theorem 4.3. Fix $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) $ and $ y_{0} \in \mathbb{R} $. Suppose that $ f \in \mathbf{C}^{{2}} $ satisfies Assumptions (2)-(7)-(35). Suppose also that in (25), we use the Engquist-Osher flux or the Godunov one when $ j \neq 0 $ and any other monotone consistent and Lipschitz numerical flux when $ j = 0 $. Then under the CFL condition (27), the scheme (23) – (25) converges to an admissible weak solution to Problem (1).
Proof. We have shown that – up to the extraction of a subsequence – $ y_{\Delta} $ converges uniformly on $ [0,T] $ to some $ y \in \mathbf{C}^{{}}([0,T]) $ and that $ \rho_{\Delta} $ converges a.e. on $ \mathbb{R} \times (0,T) $ to some $ \rho \in \mathbf{L}^{{\infty}}( \mathbb{R} \times (0,T)) $. We now prove that this couple $ (\rho,y) $ is an admissible weak solution to Problem (1) in the sense of Definition 2.1.
Recall that for all $ \Delta $ and $ t \in [0,T] $,
When letting $ \Delta \to 0 $, the dominated convergence theorem ensures that $ y $ satisfies (6). Apply inequality (30) with $ \tau = 0 $, $ \tau' = T $, $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R}^{*} \times [0,T)), \varphi \geq 0 $ and $ \kappa \in [0,R] $ to obtain
Then the a.e. convergence of $ (s_{\Delta})_{\Delta} $ to $ \dot y $ – coming from (34) – and the a.e. convergence of $ (\rho_{\Delta})_{\Delta} $ to $ \rho $ ensure that when letting $ \Delta \to 0 $, we get
and consequently $ \rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $, see Remark 2. Now, we pass to the limit in (30) and (31) using the a.e. convergence of $ (s_{\Delta})_{\Delta} $ to $ \dot y $ and of $ (\rho_{\Delta})_{\Delta} $ to $ \rho $ as well as the continuity of $ Q $ and $ \omega $. Consequently, for all test functions $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R} \times \mathbb{R}^{+}), \varphi \geq 0 $ and $ \kappa \in [0,R] $, the following inequalities hold for almost every $ 0 \leq \tau < \tau' \leq T $:
To conclude, note that the expression in the left-hand side of the previous inequality is a continuous function of $ (\tau,\tau') $ which is almost everywhere greater than the continuous function $ 0 $. By continuity, this expression is everywhere greater than $ 0 $, which proves that $ \rho $ satisfies the entropy inequalities (4). Using similar arguments, one shows that $ \rho $ also satisfies the constraint inequalities (5). This shows that the couple $ (\rho,y) $ is an admissible weak solution to (1), and that concludes the proof of convergence.
We proved than in the $ \mathbf{L}^{{\infty}} $ framework, the scheme converges to an admissible weak solution, but note that there is no guarantee of uniqueness in this construction. Also stress that we cannot extend this result to general consistent monotone numerical fluxes beyond hypothesis (36).
4.4. Compactness via global BV bounds
The following result is the discrete version of Lemma 2.3 so it is consistent that the proof uses the discrete analogous arguments of the ones we used in the proof of Lemma 2.3.
Lemma 4.4. Introduce for all $ \Delta > 0 $ the function $ \xi_{\Delta} $ defined for all $ t \in [0,T] $ by
Then $ \xi_{\Delta} $ has bounded variation and consequently, so does $ s_{\Delta} $.
Proof. Since $ \mu \in \mathbf{BV}( \mathbb{R}) $, there exists a sequence of smooth functions $ (\mu_{\ell})_{\ell \in \mathbb{N}} \subset \mathbf{BV}( \mathbb{R}) \cap \mathbf{C}_c^{{\infty}}( \mathbb{R}) $ such that
Introduce for all $ \ell \in \mathbb{N} $ and $ t \in [0,T] $, the function $ {{\xi_{\Delta, \ell}(t) = \int_{ \mathbb{R}} \rho_{\Delta}(x,t) \mu_{\ell}(x) \mathinner{\mathrm{d}{x}}}} $ and let $ K > 0 $ such that
For all $ \ell \in \mathbb{N} $ and $ t,s \in [0,T] $, if $ t \in [t^{k},t^{k+1}) $ and $ s \in [t^{m},t^{m+1}) $, we have
Consequently, for all $ \ell \in \mathbb{N} $, $ \Delta > 0 $ and $ t,\tau \in [0,T] $, the triangle inequality yields:
Letting $ \ell \to +\infty $, we get that for all $ \Delta > 0 $ and $ t,\tau \in [0,T] $,
which leads to
This proves that $ \xi_{\Delta} \in \mathbf{BV}([0,T]) $. Since $ \omega $ is Lipschitz continuous, $ s_{\Delta} $ also has bounded variation.
Theorem 4.5. Fix $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) \cap \mathbf{BV}( \mathbb{R}) $ and $ y_{0} \in \mathbb{R} $. Suppose that $ f $ satisfies (2)-(7)-(16) and that $ Q $ satisfies (17). Suppose also that in (25), we use the Godunov flux when $ j = 0 $ and any other monotone consistent and Lipschitz numerical flux when $ j \neq 0 $. Then under the CFL condition (27), the scheme (23) – (25) converges to a $ \mathbf{BV} $-regular solution to Problem (1).
Proof. All the hypotheses of Lemma A.4 are fulfilled. Consequently, there exists a constant $ \mathbf{C}^{{ \varepsilon}} > 0 $ such that for all $ n \in \{0, \ldots, N-1\} $,
Making use of Lemma 4.4, we obtain that for all $ n \in \{0, \ldots, N\} $,
where the constant $ K $ was introduced in the proof of Lemma 4.4. The two last inequalities imply that for all $ t \in [0,T] $, we have
Therefore, the sequence $ (\rho_{\Delta})_{\Delta} $ is uniformly in time bounded in $ \mathbf{BV}( \mathbb{R}) $. Using [22,Appendix], we get the existence of $ \rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $ such that
Following the proof of Theorem 4.3, we show that $ (\rho,y) $ is an admissible weak solution. Then passing to the limit in (46), the lower semi-continuity of the $ \mathbf{BV} $ semi-norm ensures that $ (\rho,y) $ is also $ \mathbf{BV} $-regular.
Remark 10. Note the complementarity of the hypotheses made in the above theorem with the ones of Theorem 4.3. Recall that in Theorem 4.3, we needed the Godunov flux only away from the interface.
5.
Numerical simulations
In this section we present some numerical tests performed with the scheme analyzed in Section 4. In all the simulations we take the uniformly concave flux $ f(\rho) = \rho(1-\rho) $ (the maximal car velocity and the maximal density are assumed to be equal to one). Following the hypotheses of Theorem 4.5, we choose the Godunov flux at the interface, and the Rusanov one away from the interface. We will use weight functions of the kind
for one (in Section 5.1) or several (in Section 5.3) values of $ k \in \mathbb{N}^* $.
5.1. Validation of the scheme
In this section, consider a two-lane road on which a bus travels with a speed given by the function
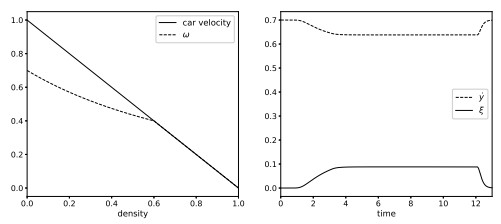
where $ \alpha $ and $ \beta $ are chosen so that $ \omega(0) = 0.7 $ and $ \omega(0.6) = 0.4 $, as illustrated in Figure 1 (left). The set-up of the experiment is the following. Consider a domain of computation $ [0, 11] $, the weight function $ \mu_4 $ and the following data:
The idea behind the choice of $ Q $ is that in average (between the two lanes), the presence of the slow vehicle reduces by $ 25\% $ the maximum traffic flow. As we can see in Figure 1 (right), the slow vehicle nearly always travels at maximum velocity. It makes sense because even though we can see that cars are overtaking it (Figure 1, right and Figure 2), the density $ \xi $ ahead of it is never sufficiently important to make it go slower.
Remark 11. The function $ \omega $ we chose above is not of the form as required in [27,28]. Once again, let us stress that the particular form $ \omega(\rho) = \min\left\{V_{\text{bus}}; 1-\rho \right\} $, where $ V_{\text{bus}} $ is the maximum bus velocity, is crucial for the well-posedness result of [27,28] to hold. Indeed, it is essential in the analysis of [27,28] that the velocity of the bus be constant (equal to $ V_{\text{bus}}) $ across the nonclassical shocks. Our nonlocal model is not bound to this restriction.
5.2. Convergence analysis
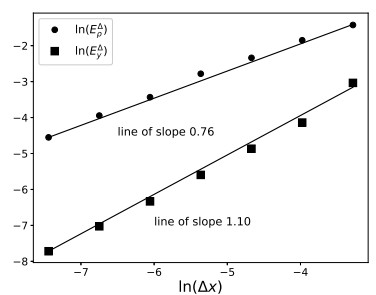
We also perform a convergence analysis for this test. In Table 1, we computed the relative errors
for different number of space cells at the final time $ T = 13 $. We see that those ratio converge with convergence orders approximately equal to $ 0.76 $ for the car density and approximately equal to $ 1.1 $ for the slow moving vehicle position.
5.3. Comparisons with experiments on the local model
Now we confront the numerical tests performed with our model with the tests done by the authors in [14] approximating the original problem of [20]. We deal with a road of length $ 1 $ parametrized by the interval $ [0,1] $ and choose the weight function $ \mu_3 $. Moreover,
First, consider the initial datum
The numerical solution is composed of two classical shocks separated by a nonclassical discontinuity, as illustrated in Figure 4 (left).
Next, we choose
The values of the initial condition create a rarefaction wave followed by a nonclassical and classical shocks, as illustrated in Figure 4 (right).
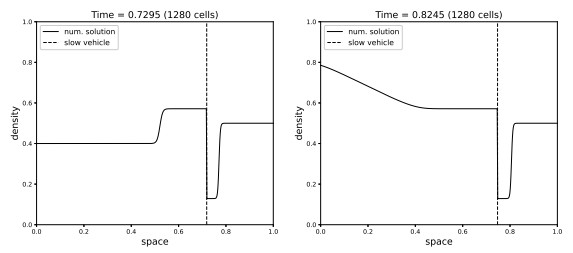
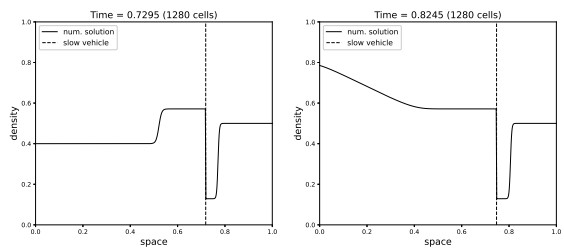
Finally, still following [14], we consider
Here the solution is composed of a rarefaction wave followed by nonclassical and classical shocks on the density that are created when the slow vehicle approaches the rarefaction and initiates a moving bottleneck, as illustrated in Figure 5.
With these three tests, we can already see – in a qualitative way – the resemblance between the numerical approximations to the solutions to our model and the numerical approximations of [14]. One way to quantify their proximity is for example to evaluate the $ \mathbf{L}^{{1}} $ error between the car densities and the $ \mathbf{L}^{{\infty}} $ error between the bus positions. More precisely, denote by $ (\rho_{\Delta},y_{\Delta}) $ the approximation of the $ \mathbf{BV} $-regular solution to (1) obtained with the scheme (23) – (25), and denote by $ (\overline{\rho}_{\Delta},\overline{y}_{\Delta}) $ the couple obtained with this same scheme but
Let us precise that this is not the scheme the authors of [14] proposed. However, this scheme is consistent with the problem
and behaves in a stable way in the calculations we performed. Therefore, the couple $ (\overline{\rho}_{\Delta},\overline{y}_{\Delta}) $ is expected to give a reasonable approximation of the solution to (50). With this in mind, for the case (49) and still with the weight function $ \mu_3 $, we computed in Table 2 the measured errors
These calculations indicate that for a sufficiently large number of cells $ J \geq 40960 $,
This indicates the discrepancy between our nonlocal and the local model (50) of [20]. The idea is now to fix the number of cells $ J = 40960 $ and to make the length of the weight function support go to zero. In Table 3, we have computed, for different weight functions, the error between the approximations of the two models. This error corresponds, as in the above calculation, to the residual error observed starting from a sufficiently small $ \Delta x $.
Remark 12. The previous simulations show a closeness between our model as $ \mu \to \delta_{0^{+}} $ and (50). Let us however point that the nonlocality in space for the slow vehicle introduces an undesirable artefact into the model. In the rarefaction regime one may observe that the large vehicle may move a bit faster that the surrounding flow. The situation where this effect becomes truly perceptible is when considering initial data of the type
Indeed, for such data, there exists a small time interval $ [0, \delta] $ in which $ \dot y(t) > v(\rho(y(t)^+)) = 0 $, which would suggest that the slow vehicle moves forward while the cars in front of it do not. This time interval is in fact quite small due to the narrowness of the support of the weight function. The local model does not develop such phenomena. This qualitative artefact precludes us from giving a microscopic interpretation to the model, which main output is the global influence of the slow vehicle on the flow; however, let us stress that the phenomenon becomes quantitatively negligible for larger times. Indeed, Ole\u{ı}nik estimate on decay of positive waves ensures that data of the type (51) evolve into rarefaction waves and do not appear while driving: the classical LWR model precludes the formation of rarefaction waves focused at positive time. The modification of the classical LWR brought by the constraint may produce nonclassical waves at positive times; while these waves are downward jumps in density like in (51), they are situated precisely at the location of the constraint and not slightly behind it, like in (51).
Even if we are unable, at this time, to rigorously link our problem (1) with $ \mu \to \delta_{0^{+}} $ and the original problem (50) of the authors in [20], this last experiment corroborates the conjecture that the local model (50) is the singular limit of our model in the case $ \omega $ is of the form $ \omega(\rho) = \min\left\{V_{\text{bus}} ; 1-\rho \right\} $. The other interesting question is whether the local model is well posed beyond this particular choice of $ \omega $.
Acknowledgments
The author is most grateful to Boris Andreianov for his constant support and many enlightening discussions.
Appendix A.
On BV bounds for limited flux models
We focus on the study of the following class of models:
where $ s \in \mathbf{BV}([0,T]; [0,\Sigma]) $ for some $ \Sigma > 0 $ and $ q \in \mathbf{BV}([0,T] ; \mathbb{R}^{+}) $. We suppose that $ F \in \mathbf{C}^{{1}}([0, \Sigma] \times [0,R]) $ and that for all $ s \in [0,\Sigma] $, $ F(s, \cdot) $ is bell-shaped i.e.
This framework covers the particular case when $ F $ takes the form:
with bell-shaped $ f : [0,R] \to \mathbb{R}^+ $, which our model (1) is based on. This class of models is well known, especially when the flux function is not time dependent, cf. [17,6]. In this appendix, we establish in passing the well-posedness of Problem (52), but our main interest lies in the $ \mathbf{BV} $ in space regularity of the solutions. More precisely, we aim at obtaining a bound on the total variation of the solutions to (52), using a finite volume approximation which allows for sharp control of the variation at the constraint. Note that the alternative offered by wave-front tracking would be cumbersome because of the explicit time-dependency in (52). In the general case, entropy solutions to limited flux problems like (52) do not belong to $ \mathbf{L}^{{\infty}}((0,T) ; \mathbf{BV}( \mathbb{R})) $, see [1]. We will show that it is the case under a mild assumption on the constraint function $ q $ – see Assumption (59) below – and provided that
Throughout the appendix, for all $ s \in [0,\Sigma] $ and $ a,b \in [0,R] $, we denote by
the classical Kružkov entropy flux associated with the Kružkov entropy $ \rho \mapsto |\rho-\kappa| $, for all $ \kappa \in [0,R] $, see [25].
A.1. Equivalent definitions of solution and uniqueness
Let us first recall the following definition.
Definition A.1. A bounded function $ \rho \in \mathbf{L}^{{\infty}}( \mathbb{R} \times (0,T)) $ is an admissible weak solution to (52) if
(ⅰ) the following regularity is fulfilled: $ {{\rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R}))}} $;
(ⅱ) for all test functions $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R} \times \mathbb{R}^{+}), \varphi \geq 0 $ and $ \kappa \in [0,R] $, the following entropy inequalities are verified for all $ 0 \leq \tau < \tau' \leq T $:
where
(ⅲ) for all test functions $ \psi \in \mathbf{C}^{{\infty}}([0,T]), \psi \geq 0 $ and some given $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R}) $ which verifies $ \varphi(0) = 1 $, the following weak constraint inequalities are verified for all $ 0 \leq \tau < \tau' \leq T $:
Definition A.2. An admissible weak solution $ \rho $ will be called $ \mathbf{BV} $-regular if it verifies $ {{\rho \in \mathbf{L}^{{\infty}}((0,T) ; \mathbf{BV}( \mathbb{R}))}} $.
As we pointed out before, this notion of solution is well suited for passage to the limit of a.e. convergent sequences of exact or approximate solutions. However, it is not so well-adapted to prove uniqueness. An equivalent notion of solution, based on explicit treatment of traces of $ \rho $ at the constraint, was introduced by the authors of [7]. This notion of solution leads to the following stability estimate.
Theorem A.3. Fix $ s^1, s^2 \in \mathbf{BV}([0,T] ; [0,\Sigma]) $, $ \rho_{0}^1,\rho_{0}^2 \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) \cap \mathbf{BV}( \mathbb{R}) $ and $ q^1,q^2 \in \mathbf{BV}([0,T] ; \mathbb{R}^+) $. Denote by $ \rho^1 $ a $ \mathbf{BV} $-regular solution to (52) with data $ \rho_0^1, q^1, s^1 $ and $ \rho^2 $ an admissible weak solution to (52) with data $ \rho_0^2, q^2, s^2 $. Suppose that the flux functions $ (t,\rho) \mapsto F(s^1(t),\rho),F(s^2(t),\rho) $ satisfy (53). Then for a.e. $ t \in (0,T) $, we have:
In particular, Problem (52) admits at most one $ \mathbf{BV} $-regular solution.
Proof. Since our interest to details lies rather on the numerical approximation point of view, we do not fully prove this statement but we give the essential steps leading to this stability result.
● Definition of solution. First, the authors of [7] introduce a subset of $ \mathbb{R}^2 $ called germ, which can be seen as the set of all the possible traces of a solution to (52). Then, they say that $ \rho $ is a solution to (52) if it satisfies entropy inequalities away from the interface – i.e. with $ \varphi \in \mathbf{C}_c^{{\infty}}( \mathbb{R}^* \times \mathbb{R}^+) $ in the entropy inequalities – and if the couple constituted of left-side and the right-side traces of $ \rho $ belongs to this so-called germ.
● Equivalence of the two definitions. The next step is to prove that this latter definition of solution is equivalent to Definition A.1. This part is done using good choices of test functions, see [7,Theorem 3.18] or [6,Proposition 2.5,Theorem 2.9].
● First stability estimate. One first shows that if $ s^1 = s^2 $, then for a.e. $ t \in (0,T) $, one has
The proof starts with the classical doubling of variables method of Kružkov [25,Theorem 1] and then uses the germ structure, what the authors of [7] called $ \mathbf{L}^{{1}} $-dissipativity, see [7,Definition 3.1] and [6,Lemma 2.7].
● Proof of estimate (54). The proof is based upon estimate (55) and elements borrowed from [8,18]. Most details can be found in the proof of [21,Theorem 2.1].
Remark 13. Though the definition of solutions with the germ explicitly involves the traces of $ \rho $, we did not discuss the existence of such traces. A first way to ensure such existence is to deal with $ \mathbf{BV} $-regular solutions. That way, traces do exist and are to be understood in the sense of $ \mathbf{BV} $ functions. Outside the $ \mathbf{BV} $ framework, existence of strong traces for solutions to (52) is ensured provided an assumption on the fundamental diagram like (15), see [2,29]. Finally, if one does not want to impose such a condition on the flux, (which is our case in this appendix), one can follow what the authors of [7] proposed (in Section 2) and consider the "singular mapping traces."
A.2. Existence of BV-regular solutions
We now turn to the proof of the existence of $ \mathbf{BV} $-regular solutions by the means of a finite volume scheme.
Fix $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) $. For a fixed spatial mesh size $ \Delta x $ and time mesh size $ \Delta t $, let $ x_{j} = j\Delta x $, $ t^{n} = n \Delta t $. We define the grid cells $ \mathbf{K}_{j+1/2} = (x_{j},x_{j+1}) $ and $ N \in \mathbb{N}^{*} $ such that $ T \in [t^N, t^{N+1}) $. We write
We choose to discretize the initial data $ \rho_{0} $ and the functions $ s,q $ with $ \left( \rho_{j+1/2}^{0}\right)_{j} $, $ (s^{n})_{n} $ and $ (q^{n})_{n} $ where for all $ j \in \mathbb{Z} $ and $ n \in \{0 ,\ldots, N\} $), $ \rho_{j+1/2}^{0} $, $ s^{n} $ and $ q^n $ are their mean values on each cell $ \mathbf{K}_{j+1/2} $ and $ [t^{n},t^{n+1}) $. Following [6], the marching formula of the scheme is the following: for all $ n \in \{0 ,\ldots, N-1\} $ and $ j \in \mathbb{Z} $:
where
$ \mathbf{F}^{n} $ being a monotone consistent and Lipschitz numerical flux associated to $ F(s^{n}, \cdot) $. We then define
Let $ \Delta = (\Delta x, \Delta t) $. For the convergence analysis, we will assume that $ \Delta \to 0 $, with $ \lambda = \Delta t / \Delta x $, verifying the CFL condition
where $ \mathbf{F}^s = \mathbf{F}^s(a, b) $ is the numerical flux – associated to $ F(s,\cdot) $ – we use in the scheme (56). From now, the analysis of the scheme follows the same path as in Section 4. In that order, we prove that the scheme (56)-(57) is $ \mathbf{L}^{{\infty}} $ stable, satisfies discrete entropy inequalities similar to (29) and approximate entropy/constraint inequalities similar to (30)-(31). Only the compactness for $ (\rho_{\Delta})_{\Delta} $ is left to obtain since the $ \mathbf{L}_{\mathbf{loc}}^{{1}} $ compactness for the sequences $ (s_{\Delta})_{\Delta} $ and $ (q_{\Delta})_{\Delta} $ is clear. One way to do so is to derive uniform $ \mathbf{BV} $ bounds.
Lemma A.4. We suppose that $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) \cap \mathbf{BV}( \mathbb{R}) $ and that $ q $ verifies the assumption
Then there exists a constant $ \mathbf{C}^{{ \varepsilon}} = \mathbf{C}^{{ \varepsilon}}(\| \partial_s F \|_{\mathbf{L}^{{\infty}}}) $ nondecreasing with respect to its argument such that for all $ n \in \{0, \ldots, N-1\} $,
where $ \rho_{\Delta} = \left( \rho^{n}_{j+1/2} \right)_{n,j} $ is the finite volume approximation constructed with the scheme (56)-(57), using the Godunov numerical flux when $ j = 0 $ in (57).
Proof. Fix $ n \in \{0, \ldots N-1\} $. With this set up we can follow the proofs of [13,Section 2] to obtain the following estimate:
where for all $ k \in \{0, \ldots, n \} $, the couple $ \left( \widehat{\rho}_{s^k}(q^{k}), \breve{\rho}_{s^k}(q^{k}) \right) \in [0,R]^{2} $ is uniquely defined by the conditions
Denote by $ \Omega( \varepsilon) $ the open subset
where for all $ s \in [0,\Sigma] $, $ {{\Omega_{s}( \varepsilon) = \left( \breve{\rho}_{s}(q_ \varepsilon (s)), \widehat{\rho}_{s}(q_ \varepsilon (s)) \right)}} $. By Assumption (59), the continuous function $ (s,\rho) \mapsto | \partial_{\rho}F(s,\rho)| $ is positive on the compact subset $ [0,\Sigma] \times [0,R] \backslash \Omega( \varepsilon) $. Hence, it attains its minimal value $ \text{C}_{0} > 0 $. Consequently, for all $ s \in [0,\Sigma] $, if one denotes by $ I_{s} : [0,\breve{\rho}_{s}(q_ \varepsilon (s))] \to [0,q_{ \varepsilon}(s)] $ the increasing part of $ F(s,\cdot) $, this function carries out a $ \mathbf{C}^{{1}} $-diffeomorphism. Moreover,
Then, for all $ k \in \{0, \ldots, n\} $,
Using the same techniques, one can show that the same inequality holds when considering $ {{\left| \widehat{\rho}_{s^{k+1}}(q^{k+1}) - \widehat{\rho}_{s^{k}}(q^{k}) \right|}} $. Therefore, inequality (60) follows with
Remark 14. Recall we suppose that $ F : [0,\Sigma] \times [0,R] $ is continuously differentiable, but if we look in the details of the proof above, we actually need $ F = F(s,\rho) $ to be continuously differentiable with respect to $ s $ and
Corollary 1. Fix $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) \cap \mathbf{BV}( \mathbb{R}) $, $ s \in \mathbf{BV}([0,T], [0,\Sigma]) $ and $ q \in \mathbf{BV}([0,T], \mathbb{R}^+) $. Suppose that $ q $ verifies Assumption (59). Let $ \rho_{\Delta} = \left( \rho^{n}_{j+1/2} \right)_{n,j} $ be the finite volume approximate solution constructed with the scheme (56)-(57), using the Godunov numerical flux when $ j = 0 $ in (57), and any other monotone consistent and Lipschitz numerical flux when $ j \neq 0 $. Then there exists $ \rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $ such that
Proof. Since $ s $ and $ q $ have bounded variation, inequality (60) leads to an uniform in time $ \mathbf{BV} $ bound for the sequence $ \left( \rho_{\Delta} \right)_\Delta $. Then the result from [22,Appendix] establish the compactness statement.
Theorem A.5. Fix $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) \cap \mathbf{BV}( \mathbb{R}) $, $ s \in \mathbf{BV}([0,T] ; [0,\Sigma]) $, $ F \in \mathbf{C}^{{1}}([0,\Sigma] \times [0,R]) $ verifying (53) and $ q \in \mathbf{BV}([0,T] ; \mathbb{R}^{+}) $. Suppose that in (57), we use the Godunov flux when $ j = 0 $ and any other monotone consistent and Lipschitz numerical flux when $ j \neq 0 $. Finally, suppose that $ q $ satisfies (59). Then under the CFL condition (58), the scheme (56)-(57) converges to an admissible weak solution $ \rho $, to (52), which is also $ \mathbf{BV} $-regular. More precisely, there exists a constant $ \mathbf{C}^{{ \varepsilon}} = \mathbf{C}^{{ \varepsilon}}(\| \partial_s F \|_{\mathbf{L}^{{\infty}}}) $ nondecreasing with respect to its argument such that
Proof. From the scheme (56), one can derive approximate entropy/constraint inequalities analogous to (30)-(31) of Section 4. Let $ \rho $ be the limit to the finite volume scheme, the compactness of $ \left( \rho_{\Delta} \right)_\Delta $ coming from the last corollary. We already know that $ \rho \in \mathbf{C}^{{}}([0,T] ; \mathbf{L}_{\mathbf{loc}}^{{1}}( \mathbb{R})) $. By passing to the limit in the approximate entropy/constraint inequalities verified by $ \left( \rho_{\Delta} \right)_\Delta $ we get that $ \rho $ satisfies the entropy/constraint inequalities of Definition A.1. This shows that $ \rho $ is an admissible weak solution to Problem (52). Finally, from (60), the lower semi-continuity of the $ \mathbf{BV} $ semi-norm ensures that $ \rho \in \mathbf{L}^{{\infty}} ([0,T] ; \mathbf{BV}( \mathbb{R})) $ and verifies (61). This concludes the proof.
Corollary 2. Fix $ \rho_{0} \in \mathbf{L}^{{1}}( \mathbb{R} ; [0,R]) \cap \mathbf{BV}( \mathbb{R}) $, $ s \in \mathbf{BV}([0,T] ; [0,\Sigma]) $, $ F \in \mathbf{C}^{{1}}([0,\Sigma] \times [0,R]) $ verifying (53) and $ q \in \mathbf{BV}([0,T] ; \mathbb{R}^{+}) $. Suppose that $ q $ satisfies Assumption (59). Then Problem (52) admits a unique $ \mathbf{BV} $-regular solution $ \rho $. Moreover, $ \rho $ satisfies the bound (61).
Proof. Uniqueness comes from Theorem 54, the existence and the $ \mathbf{BV} $ bound comes from Theorem A.5.
Remark 15. Under the hypotheses of Corollary 2, if we prove the existence of an other admissible weak solution $ \overline{\rho} $ to (11) (by another method, splitting for instance), then Theorem A.3 ensures that $ \overline{\rho} = \rho $.









 DownLoad:
DownLoad: