Mesoscopic glass transition model: Influence of the cooling rate on the structure refinement

Vladimir Ankudinov; Konstantin Shklyaev; Mikhail Vasin; Vladimir Ankudinov; Konstantin Shklyaev; Mikhail Vasin

doi:10.3934/math.20241078

AIMS Mathematics

2024, Volume 9, Issue 8: 22174-22196. doi: 10.3934/math.20241078

Previous Article Next Article

Research article Special Issues

Mesoscopic glass transition model: Influence of the cooling rate on the structure refinement

Vereshchagin Institute of High Pressure Physics, Russian Academy of Sciences, 108840 Moscow, Russia

Received: 25 March 2024 Revised: 18 June 2024 Accepted: 24 June 2024 Published: 16 July 2024
MSC : 82-XX, 82Cxx, 82B26

The process of glass transition during the quenching in the domain with the cold wall has been numerically simulated. We have implemented the temperature-dependent form of the previously proposed theoretical model, which combined the heat transfer in the domain and the gauge theory of glass transition, assuming the presence of topologically stable distortions (disclinations) in the forming solid. The competition between crystallization (formation of polycrystalline structure) and the formation of the amorphous disordered phase has been shown. At the relatively slow cooling rates corresponding to the formation of the crystalline phase, we observed a columnar to equiaxed transition qualitatively similar to the observed in many metallic alloys. The moving front followed the equilibrium isotherm corresponding to the equilibrium temperature of transition in the disclinations subsystem, although front drag resulted in the effect of kinetic undercooling and the emergence of the maximum velocity of the crystallization front. High thermal conductivity values associated with the substantial heat flux lead to the bulk amorphous state. The dynamics of the coarsening of the primary amorphous structure depended on the annealing temperature.

Keywords:

Citation: Vladimir Ankudinov, Konstantin Shklyaev, Mikhail Vasin. Mesoscopic glass transition model: Influence of the cooling rate on the structure refinement[J]. AIMS Mathematics, 2024, 9(8): 22174-22196. doi: 10.3934/math.20241078

Related Papers:

[1]	K. Maqbool, S. Shaheen, A. M. Siddiqui . Effect of nano-particles on MHD flow of tangent hyperbolic fluid in a ciliated tube: an application to fallopian tube. Mathematical Biosciences and Engineering, 2019, 16(4): 2927-2941. doi: 10.3934/mbe.2019144
[2]	Benchawan Wiwatanapataphee, Yong Hong Wu, Thanongchai Siriapisith, Buraskorn Nuntadilok . Effect of branchings on blood flow in the system of human coronary arteries. Mathematical Biosciences and Engineering, 2012, 9(1): 199-214. doi: 10.3934/mbe.2012.9.199
[3]	B. Wiwatanapataphee, D. Poltem, Yong Hong Wu, Y. Lenbury . Simulation of Pulsatile Flow of Blood in Stenosed Coronary Artery Bypass with Graft. Mathematical Biosciences and Engineering, 2006, 3(2): 371-383. doi: 10.3934/mbe.2006.3.371
[4]	Yi Ren, Guolei Zhang, Longbin Yang, Yanwei Hu, Xiaojing Nie, Zhibin Jiang, Dawei Wang, Zhifan Wu . Study on seafloor hydrothermal systems circulation flow and heat transfer characteristics. Mathematical Biosciences and Engineering, 2022, 19(6): 6186-6203. doi: 10.3934/mbe.2022289
[5]	Chii-Dong Ho, Jr-Wei Tu, Hsuan Chang, Li-Pang Lin, Thiam Leng Chew . Optimizing thermal efficiencies of power-law fluids in double-pass concentric circular heat exchangers with sinusoidal wall fluxes. Mathematical Biosciences and Engineering, 2022, 19(9): 8648-8670. doi: 10.3934/mbe.2022401
[6]	Huu Thuan Nguyen, Tu Anh Do, Benoît Cosson . Numerical simulation of submerged flow bridge scour under dam-break flow using multi-phase SPH method. Mathematical Biosciences and Engineering, 2019, 16(5): 5395-5418. doi: 10.3934/mbe.2019269
[7]	Alberto M. Gambaruto, João Janela, Alexandra Moura, Adélia Sequeira . Sensitivity of hemodynamics in a patient specific cerebral aneurysm to vascular geometry and blood rheology. Mathematical Biosciences and Engineering, 2011, 8(2): 409-423. doi: 10.3934/mbe.2011.8.409
[8]	Oualid Kafi, Nader El Khatib, Jorge Tiago, Adélia Sequeira . Numerical simulations of a 3D fluid-structure interaction model for blood flow in an atherosclerotic artery. Mathematical Biosciences and Engineering, 2017, 14(1): 179-193. doi: 10.3934/mbe.2017012
[9]	Alberto Gambaruto, João Janela, Alexandra Moura, Adélia Sequeira . Shear-thinning effects of hemodynamics in patient-specific cerebral aneurysms. Mathematical Biosciences and Engineering, 2013, 10(3): 649-665. doi: 10.3934/mbe.2013.10.649
[10]	Honghui Zhang, Jun Xia, Yinlong Yang, Qingqing Yang, Hongfang Song, Jinjie Xie, Yue Ma, Yang Hou, Aike Qiao . Branch flow distribution approach and its application in the calculation of fractional flow reserve in stenotic coronary artery. Mathematical Biosciences and Engineering, 2021, 18(5): 5978-5994. doi: 10.3934/mbe.2021299

Abstract

1. Introduction

Increased blood viscosity may damage blood vessels and increase the risk of heart attacks and vascular strokes, the leading causes of death globally. Scientists have commonly used drugs like aspirin to reduce blood viscosity. However, taking aspirin in high doses results in various side effects such as blood clots, stomach bleeding, ulcers, and even ringing in the ears. Magnetic field therapy may be a potent way to lower the risk of heart attacks. For decades, magnetic fields have been used in medical diagnostics, such as magnetic resonance imaging (MRI). In hospitals, high-strength magnets of 1.5 to 3 teslas have been used with no harmful effects on the body and improve nerve signals. However, more magnetic therapy research for a health and safety solution under various physiological and experimental conditions is required for correct treatment protocols to prevent heart attack and vascular stroke.

Blood is a predominantly shear-thinning fluid primarily related to the rheological properties of red blood cells (RBC) ^[1,2]. RBC aggregation is reversible and shear dependent. This aggregation is reformed at low shear rates and is dispersed at high shear. According to Baieth ^[3] and Connes et al. ^[4], increasing shear rates decreases blood viscosity exponentially. Blood is a non-Newtonian homogeneous fluid treated as an electrically conducting magnetic fluid. It exhibits magnetohydrodynamic (MHD) properties. A wide range of research was conducted practically and theoretically to study the influence of the magnetic field on blood viscosity and blood flow characteristics. Yamamoto et al. ^[5] experimented with investigating the impact of a magnetic field on the change of blood viscosity. It is found that a 1.5-tesla making a change in blood oxygenation causes an increment in blood viscosity and a shear rate of blood flow. The relative blood viscosity decreases as a function of the applied magnetic field. Some researchers ^[6,7] used rheometers with varying shear rates to measure blood viscosity in a static magnetic field. A change in blood viscosity alters blood vessels' velocity and flow rate. According to experimental results from Haik et al. ^[8], when exposed to a high magnetic field of 10 tesla, the blood flow rate can be reduced by 30% due to increased blood viscosity. Mathematical models have been developed for various situations under a transverse magnetic field ^{[9,10,11,12,13,14]}. They pointed out that increasing the intensity of the transverse magnetic field reduces blood flow rate. Moreover, Tzirakis et al. ^[15] presented a Symmetric Weighted Interior Penalty formulation to study the impact of the magnetoviscous effects on the generated viscosity of non-Newtonian biomagnetic fluid flow. Gayathri and Shailendhra ^[16] studied blood flow in human arteries under a magnetic field. The results indicated that there was no significant change in velocity and parameters such as relative residence time, wall shear stress (WSS), and oscillatory shear index were observed up to 3 teslas in small arteries.

Several studies on the influence of magnetic fields on temperature were conducted under various conditions. Misra et al. ^[17] examined the flow and heat transfer in a parallel plate channel with stretching walls of a viscoelastic electrically conducting fluid under a magnetic field effect. They showed that the magnetic field enhances the blood temperature. According to Dar ^[18], thermal radiation and an angled magnetic field affect the peristaltic transport of blood flow with variable viscosity. It shows that increasing the thermal radiation parameter and the magnetic strength decreases the axial speed, while increasing the heat source/sink parameter or the angle of inclination of the magnetic field increases the axial speed. Alimohamadi and Sadeghy ^[19] demonstrated that a bi-directional magnetic field can lower the wall shear stress and temperature of hot spots on porous plaque in stenotic arteries.

Additionally, much research focusing on the impact of temperature-dependent viscosity has been carried out. Nadeem and Akbar ^[20] reported that the viscosity variation depending on temperature for blood and oil is more prominent than other impacts. Also, Makinde and Onyejekwe ^[21] examined the MHD steady flow and heat transfer between two parallel plates of an electrically conducting fluid with temperature-dependent variable viscosity. They discovered that as viscosity increases, the heat transfer rate at the stationary wall decreases. Shit and Majee ^[22] studied unsteady blood flow and heat transfer characteristics with various viscosity values subjected to magnetic and vibration environments. The results show that an increase in the temperature-dependent viscosity increases the flow rate and temperature.

On the other hand, the magnetic field can improve blood circulation while relaxing blood vessels. In Tao-Huang experiment ^[23], velocity of blood is set to be in the same direction as the magnetic field lines inside a tool measuring blood viscosity. They reported that the exposed magnetic field of 1.3 teslas for at least 1 minute could dramatically reduce the blood viscosity by 20 to 30%. A similar study was carried out by Kadhim ^[24]. The viscosity of blood samples was determined under a 1.5-tesla MRI magnetic field using a U-tube viscometer and a mathematical formula. It is observed that the blood viscosity drops as the time of magnetic field exposure increases. This viscosity reduction is significant for 1 minute and 15-minute exposure samples. Furthermore, Keshtkar et al. ^[25] used the finite element method to simulate fluctuations of blood velocity in pulse electromagnetic fields. The results show that strong pulses of transient electromagnetic fields enhance fluid velocity with lower fluctuations at various temperatures. Javadzadegan et al. ^[26] reported the magnetic effects on blood viscosity and wall shear rate. An increase in high or ultra-high magnetic field strengths results in beneficial and adverse effects, such as lower viscosity in smaller areas of low wall shear stress and increased maximum wall shear stress.

This paper presents a MHD blood flow and heat transfer through a system of coronary arteries with LAD stenosis. The system of coronary arteries consists of the base of the aorta, the right coronary artery (RCA), and the left coronary artery (LCA), with two branches, including left circumflex (LCX) and left anterior descending (LAD) coronary arteries. According to the literature, this study hypothesizes that the exerted magnetic field parallel to the blood flow direction can reduce blood viscosity and enhance blood flow. The imposed magnetic field strength-dependent and temperature-dependent variables have been considered for a modified non-Newtonian blood viscosity. The work is structured as follows. The mathematical model of the study problem is presented in Section 2, and the numerical technique is illustrated in Section 3. Section 4 concerns a numerical example. The conclusion and discussion are summarized in Section 5.

2. Mathematical model

To investigate the effect of the magnetic field on blood flow behavior through the coronary system. Blood is assumed to be an incompressible non-Newtonian fluid. The applied magnetic field has an impact on fluid viscosity after 60 seconds ^[23]. Based on a non-Newtonian model, blood viscosity $(\eta)$ depends on shear rate, temperature, and magnet strength ^[27,28]. Here, the viscosity of the incompressible MHD non-Newtonian fluid is

$\begin{equation} \eta = \left\{\begin{array}{ll} H(T)\hat{\eta}, & t < 60s\\ H(T)\hat{\eta}(1 - (c_1+c_2 |\textbf{B}_0|)|\textbf{B}_0|), & \text{otherwise.} \end{array}\right. \end{equation}$

(2.1)

As blood loses viscosity and decreases surface tension on the arterial wall when the blood temperature rises with high activation energy, we thus define the term $H(t)$ by

$\begin{equation} H(T) = e^{\alpha \left( \frac{1}{T_\alpha-T_s} - \frac{1}{T - T_s}\right)}, \end{equation}$

(2.2)

where $T$ is the blood temperature, $\alpha \in [0, \; 0.4]$ is Arrhenius activation energy ^[29], $T_\alpha = 37 ^\circ$ C denotes the tissue temperature and $T_s = 25^\circ$ C is the surrounding temperature. The modified Carreau-Yasuda viscosity model is used for blood viscosity. In Eq (2.1), $\hat{\eta}$ is determined by

$\begin{equation} \hat{\eta} = \left\{ \begin{array}{ll} \eta_{max} & \dot{\gamma} < \epsilon \\ \eta_\infty + (\eta_0-\eta_\infty)[1+(\lambda \dot{\gamma})^p]^{(n-1)/p} & \epsilon \leq \dot{\gamma} < 327 \\ \eta_{min} & \dot{\gamma} \geq 327 \end{array} \right. \end{equation}$

(2.3)

with shear rate given by

$\dot{\gamma} = \sqrt{2 \frac{ \partial{} u_i}{ \partial{} x_j}S_{ij}};\; \;\;\; S_{ij} = \frac{1}{2} \left[\frac{ \partial{} u_i}{ \partial{} x_j}+\frac{ \partial{} u_j}{ \partial{} x_i} \right].$

In Eq (2.1), $\textbf{B}_0$ is the external magnetic field, $(c_1+c_2 |\textbf{B}_0|) < 1$ and other model parameters are constants.

The pulsatile MHD flow and heat transfer of blood through the system of coronary arteries with stenosis are governed by a set of partial differential equations, including the continuity Eq (2.4), the momentum Eq (2.5), the energy Eq (2.6) and the Maxwell's equation for magnetic field (2.9):

$\begin{equation} \nabla \cdot \textbf{u} = 0, \end{equation}$

(2.4)

$\begin{equation} \rho\bigg(\frac{ \partial{} \textbf{u}}{ \partial{} t}+(\textbf{u}\cdot\nabla) \textbf{u}\bigg) = \nabla \cdot \eta \nabla \textbf{u}-\nabla p+\textbf{F}, \end{equation}$

(2.5)

$\begin{equation} \rho c_p\bigg(\frac{ \partial{} T}{ \partial{} t}+(\textbf{u}\cdot\nabla) T\bigg) = \nabla \cdot k \nabla T + S_T, \end{equation}$

(2.6)

where $\textbf{u} = (u_1, u_2, u_3)^T$ is the velocity vector, $p, \; T$ denote respectively pressure and temperature of blood, and $\rho, \; c_p, \; k$ denote the density, the heat capacity and thermal conductivity. $\textbf{F}$ is the Lorentz force term, determined by

$\begin{equation} \textbf{F} = \textbf{J} \times \textbf{B}, \end{equation}$

(2.7)

where $\textbf{J} = \sigma (\textbf{E}+\textbf{u} \times \textbf{B})$ denotes the induced current density vector, $\textbf{E}$ denotes the electric field, and $\textbf{B}$ is the magnetic field that is assumed to be in the form of

$\begin{equation} \textbf{B} = \textbf{B}_0+\textbf{b}, \end{equation}$

(2.8)

with the induced magnetic field $\textbf{b}$ which can be calculated based on Ohm's law and Maxwell's equation by

$\begin{equation} \frac{ \partial{} \textbf{b}}{ \partial{} t}+(\textbf{u} \cdot \nabla ) \textbf{b} = \nabla \cdot \frac{1}{\mu \sigma} \nabla^2 \textbf{b} + S_b, \end{equation}$

(2.9)

where $\mu$ and $\sigma$ denote the media's magnetic permeability and electrical conductivity, respectively. The heat source term or the Joule heating rate, $S_T$ in Eq (2.6), and the magnetic source term $S_b$ in Eq (2.9) are respectively determined by

$\begin{equation} S_T = \frac{1}{\sigma}\textbf{J} \cdot \textbf{J}; \;\;\;\; S_b = \left( \textbf{B} \cdot \nabla \right)\textbf{u} -(\textbf{u} \cdot \nabla )\textbf{B}_0. \end{equation}$

(2.10)

To define the above unsteady state MHD problem completely, boundary and initial conditions are required. Here, we set the behavior of blood flow as the pulsatile conditions for pressure and flow rate in the terms of periodic functions $P(t) = P(t+mT_c)$ and $Q(t) = Q(t+mT_c)$ for $m = 1, 2, 3, \ldots, M$ with cardiac period $T_c = 0.80$ s. The waveforms of $P(t)$ and $Q(t)$ can be represented by the following Fourier series.

$\begin{align} P(t)& = \overline{p}+\sum\limits_{m = 1}^M\alpha_m^p\cos(m\omega t)+\beta_m^p\sin(m\omega t), \end{align}$

(2.11)

$\begin{align} Q(t)& = \overline{Q}+\sum\limits_{m = 1}^M\alpha_m^Q\cos(m\omega t)+\beta_m^Q\sin(m\omega t), \end{align}$

(2.12)

where $\overline{p}$ represents the mean pressure, $\overline{Q}$ is the mean flow rate, $\omega = \frac{2\pi}{T_c}$ is the angular frequency with the period $T_c$ , and $M$ denotes the number of harmonics considered.

At the aorta inlet $(\partial \Omega_{in})$ with an inlet cross-sectional area of $A$ ,

● the velocity and temperature are defined by

$\begin{equation} \textbf{u}(\textbf{x},t) = \frac{Q(t)}{A};\;\;\;T(\textbf{x},t)\; = T_0; \end{equation}$

(2.13)

● the magnetic flux is set to zero: $\int_{ \partial \Omega_{in}} \textbf{B} \cdot \textbf{n}\; ds = 0$ .

At the arterial wall $(\partial \Omega_{\Gamma})$ with a unit outward normal vector $\textbf{n}$ ,

● the no-slip condition and the thermal convection condition are applied:

$\begin{equation} \left\{ \begin{array}{ccl} \textbf{u}(\textbf{x},t) & = & 0 \\ -k\frac{ \partial{} T(\textbf{x},t)}{ \partial{} \textbf{n}} & = &h_\infty(T(\textbf{x},t)-T_{\alpha}) \end{array} \right. \end{equation}$

(2.14)

● the thin wall condition of induced magnetic field is applied:

$\begin{equation} \textbf{B}(\textbf{x},t) = \textbf{B}(r_p(\textbf{x}_c) + h) - \textbf{B}(r_p(\textbf{x}_c) - h), \end{equation}$

(2.15)

where $r_p(\textbf{x}_c)$ is mean radius of the arterial cross section $c$ along the flow channel and $h$ is the arterial wall thickness.

On the arterial outlets $(\partial \Omega_{out})$ with a unit outward normal vector $\textbf{n}$ ,

● the pulsatile pressure is imposed to the outlet boundary $(\partial \Omega_{out})$ , i.e.,

$\begin{equation} p(\textbf{x},t) = P(t);\;\;\;(\eta\nabla \textbf{u}+(\nabla \textbf{u})^T)\cdot\textbf{n} = 0, \end{equation}$

(2.16)

● the temperature gradient is set to zero: $\frac{ \partial T}{ \partial \textbf{n}} = 0,$

● the magnetic flux across the outlet cross section area is set to zero : $\int_{ \partial \Omega_{out}} \textbf{B} \cdot \textbf{n}\; ds = 0$ .

For initial conditions, we set, for all $\textbf{x} = (x, y, z) \in \Omega$ ,

$\begin{equation} \left\{ \begin{array}{ccc} \textbf{u}(\textbf{x},0) & = & 0 \\ T(\textbf{x},0) & = & T_0 \\ \textbf{b}(\textbf{x},0) & = & 0. \end{array} \right. \end{equation}$

(2.17)

Thus, the MHD blood flow and heat transfer in the coronary artery system with LAD stenosis is governed by the following initial boundary value problem (IBVP).

IBVP: Find vector functions $\textbf{u}(t)$ and $\textbf{b}(t)$ , and scalar functions $p(t)$ and $T$ such that the field Eqs (2.4)–(2.6) and (2.9), and all boundary conditions are satisfied.

3. Numerical approximation

3.1. Variational statement

The variational statement corresponding to the above initial boundary value problem is:

Find $\textbf{u}, \; \textbf{b} \in [H^1(\Omega)]^3$ and $p, \; T\in H^1(\Omega)$ such that all essential boundary conditions of $\textbf{b}, \; \textbf{u}, p, \; T$ are satisfied and

$\begin{equation} \left\{ \begin{array}{ll} a(\textbf{b},\;v_b) = L(v_b) &\forall v_b \in [H^1_{b0}(\Omega)]^3\\ \\ a(\textbf{u},\;v_p) = L(v_p) &\forall v_p \in H^1_{p0}(\Omega)\\ \\ a(\textbf{U},\;v) = L(v) &\forall v \in [H^1_0 (\Omega)]^3\\ \\ a(T,\;v_T) = L(v_T) &\forall v_T \in H^1_{T0}(\Omega) \end{array}\right. \end{equation}$

(3.1)

where $H^1(\Omega)\; = \; \left \{v\; |\; v, \frac{ \partial{} v}{ \partial{} x}, \frac{ \partial{} v}{ \partial{} y}, \frac{ \partial{} v}{ \partial{} z} \in L^2(\Omega) \right \}$ is a Sobolev space class and $H^1(\Omega) = \text{span}\{\phi_i\}_{i = 1}^\infty$ , with the norm $\parallel v\parallel^2_{L^2(\Omega)}$ and $H^1_v = \{ v \in H^1\; |\; v\; \text{is vanished on boundary}\}$ .

The notations $a(u, v)$ and $L(v)$ in Eq (3.1) represent respectively a bilinear form of $u$ and $v$ , and a linear form of $v$ :

$\begin{equation} \begin{array}{ccl} a(\textbf{b},\;v_b) & = & \int_{\Omega} v_b\dot{\textbf{b}} + v_b (\textbf{u} \cdot \nabla) \textbf{b}+\frac{1}{\mu \sigma} \nabla^2 \textbf{b} \cdot \nabla v_b\;d\Omega - \frac{1}{\mu \sigma}\int_{ \partial{} \Omega} v_b \nabla^2 \textbf{b} \cdot \textbf{n}\;ds\\\\ a(\textbf{u},\;v_p) & = & \int_{ \partial{} \Omega_{out}} v_p (\textbf{u} \cdot \textbf{n})\; ds - \int_{\Omega} \textbf{u} \cdot(\nabla v_p) \;d\Omega\\\\ a(\textbf{U},\;v) & = & \int_{\Omega} v\dot{\textbf{u}} + v (\textbf{u} \cdot \nabla) \textbf{u} + \frac{1}{\rho} v \nabla p + \frac{1}{\rho} \eta \nabla \textbf{u} \cdot \nabla v\;d\Omega- \frac{1}{\rho}\int_{ \partial{} \Omega_{out}} v \eta \nabla \textbf{u} \cdot \textbf{n}\;ds\\\\ a(T,\;v_T) & = & \int_{\Omega} v_T\dot{T} + v_T (\textbf{u} \cdot \nabla) T +\frac{k}{\rho c_p }\nabla T \cdot \nabla v_T\;d\Omega- \frac{k}{\rho c_p }\int_{ \partial{} \Omega_{\Gamma}} v_T \nabla T \cdot \textbf{n}\;ds\\\\ \end{array} \end{equation}$

(3.2)

with

$\begin{equation} \begin{array}{lcl} L(v_b) & = & \int_{\Omega} v_b S_b \; d\Omega\\ L(v_p) & = & 0\\ L(v) & = &\int_{\Omega}\;\frac{1}{\rho}v\textbf{F} \; d\Omega\\ L(v_T) & = & \int_{\Omega} \frac{1}{\rho c_p }v_T S_T \; d\Omega. \end{array} \end{equation}$

(3.3)

3.2. Galerkin Finite Volume Formulation

Based on standard Galerkin method, let $H^h(\Omega) = \text{span}\{ \phi_n\}_{n = 1}^N \subset H^1(\Omega)$ and $H^h_{0} = \text{span}\{ \phi_n\}_{n = 1}^N \subset H^1_0$ , the problem (3.1) becomes

Find $\textbf{b}_h, \; \textbf{u}_h \in [H^h(\Omega)]^3$ , and $p_h, \; T_h\in H^h(\Omega)$ such that all essential boundary conditions are satisfied and

$\begin{equation} \left\{ \begin{array}{ll} a(\textbf{b}_h,\;v_{b_h}) = L(v_{b_h}) &\forall v_{b_h} \in [H^1_{b0_h}(\Omega)]^3\\ \\ a(\textbf{u}_h,\;v_{p_h}) = L(v_{p_h}) &\forall v_{p_h} \in H^1_{p0_h}(\Omega)\\ \\ a(\textbf{U}_h,\;v_h) = L(v_h) &\forall v_h \in [H^1_{0_h} (\Omega)]^3\\ \\ a(T_h,\;v_{T_h}) = L(v_{T_h}) &\forall v_{T_h} \in H^1_{T0_h}(\Omega) \end{array}\right. \end{equation}$

(3.4)

with

$\begin{align} & \textbf{u} \approx \textbf{u}_h = \sum\limits_{n = 1}^N\phi_n(\textbf{x})\textbf{u}_n(t), \; \; \; p\approx p_h = \sum\limits_{n = 1}^N\phi_n(\textbf{x}) p_n(t), \end{align}$

(3.5)

$\begin{align} &T\approx T_h = \sum\limits_{n = 1}^N\phi_n(\textbf{x}) T_n(t), \;\;\; \textbf{b} \approx \textbf{b}_h = \sum\limits_{n = 1}^N\phi_n(\textbf{x}) \textbf{b}_n(t), \;\;\; v\approx v_h = \sum\limits_{n = 1}^N\psi_n(\textbf{x}) v_n. \end{align}$

(3.6)

The error distribution over $\Omega$ depends on the selection for the basis function $\psi_n$ . To construct a control volume method, we choose nodal basis functions $\psi_n = \phi_n$ at coordinate $\textbf{x}_n$ of the element $n$ :

$\phi_n(\textbf{x}_m) = \begin{cases} 1,& \it{n}\; = \;\it{m} ,\\ 0, & \text{otherwise}. \end{cases}$

Applying the gradient and divergence theorems with the approximate solutions yields, for $i, j = 1, 2, 3$ ,

$\begin{equation} \left\{ \begin{array}{lcll} \sum\nolimits_{n = 1}^N |\Omega_n| \dot{b}_{i_n} + \sum\nolimits_{n = 1}^N\left( \int_{ \partial{} \Omega_n}\phi_n \phi_{j_n} u_{j_n} \hat{n}_j \;ds -\frac{1}{\mu \sigma} \int_{ \partial{}\Omega_n} \frac{ \partial{}^2\phi_n}{ \partial{} x_j^2} \hat{n}_j\right)\;b_{i_n} & = & L(\Omega_n), \\ \\ \sum\nolimits_{n = 1}^{N} \int_{ \partial{} \Omega_n} \phi_{i_n}\hat{n}_j ds\; u_{j_n}& = &0,\; \\ \\ \sum\nolimits_{n = 1}^N |\Omega_n| \dot{u}_{i_n} + \sum\nolimits_{n = 1}^N \left( \int_{ \partial{} \Omega_n} \phi_n \phi_{j_n} u_{j_n} \hat{n}_j \;ds -\frac{1}{\rho} \int_{\Omega_n} \eta \frac{ \partial{} \phi_n }{ \partial{} x_j} \hat{n}_j\;ds \right)u_{i_n}\;+ \sum\nolimits_{n = 1}^N\int_{ \partial{} \Omega_n} \phi_n \hat{n}_i\;ds\;p_n & = &L'(\Omega_n), \\ \\ \sum\nolimits_{n = 1}^N |\Omega_n| \dot{T}_n + \sum\nolimits_{n = 1}^N\left( \int_{ \partial{} \Omega_n}\phi_n \phi_{j_n} u_{j_n} \hat{n}_j \;d{\Omega} - \frac{h_{\infty}}{\rho c_p}\int_{ \partial{} \Omega_{\Gamma_n}} \phi_n \;ds \right)\;T_n & = & L''(\Omega_n), \end{array} \right. \end{equation}$

(3.7)

with

$\begin{equation} \begin{array}{lcl} L(\Omega_n) & = & \sum\nolimits_{n = 1}^N |\Omega_n| S_{b_n} \;d\Omega,\\\\ L'(\Omega_n) & = & \frac{1}{\rho}\sum\nolimits_{n = 1}^N |\Omega_n| F_{n} \;d\Omega,\\\\ L''(\Omega_n) & = & \frac{1}{\rho c_p}\sum\nolimits_{n = 1}^N |\Omega_n| (S_{T_n} \;d\Omega + h_{\infty}T_{\alpha}), \end{array} \end{equation}$

(3.8)

where $\vert\Omega_n\vert$ is the volume of element $\Omega_n.$

Thus, the system of Eq (3.7) can be written in matrix form as

$\begin{align} \dot{\textbf{B}}+\textbf{D}_b\textbf{B}& = \textbf{S}_b, \end{align}$

(3.9)

$\begin{align} \textbf{C}^T\textbf{U}& = \textbf{0}, \end{align}$

(3.10)

$\begin{align} \dot{\textbf{U}}+\textbf{D}_u\textbf{U}+\textbf{A}\textbf{P}& = \textbf{F}, \end{align}$

(3.11)

$\begin{align} \dot{\textbf{T}}+\textbf{D}_T\textbf{T}& = \textbf{S}_T, \end{align}$

(3.12)

where $\textbf{U}, \textbf{B}, \textbf{T}$ and $\textbf{P}$ are global vectors with $U_n, B_n, T_n$ and $P_n$ denoting the velocity, induced magnetic fields, temperature, and pressure at element $n$ , and all coefficient matrices refer to global matrices. Matrices $\textbf{D}_b$ , $\textbf{D}_u$ and $\textbf{D}_T$ correspond to convection-diffusion terms. The matrix $A$ corresponds to the pressure term. Matrices $\textbf{F}, \textbf{S}_T$ and $\textbf{S}_b$ are the forcing terms.

4. Numerical example

4.1. Computation domain and Model parameters

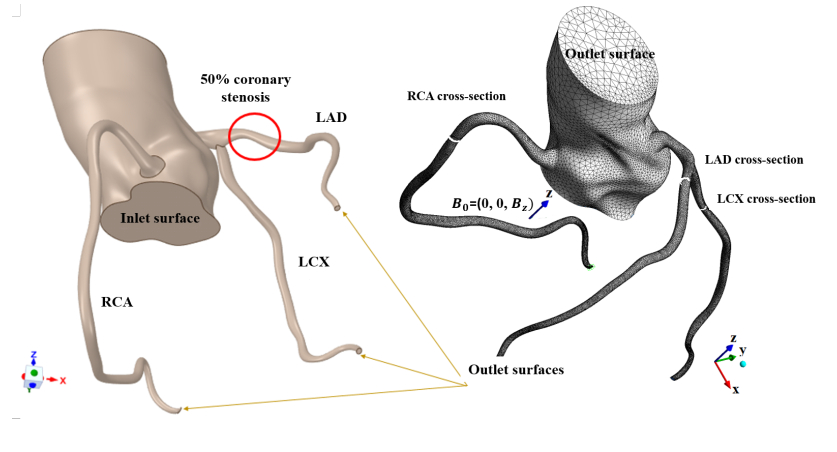
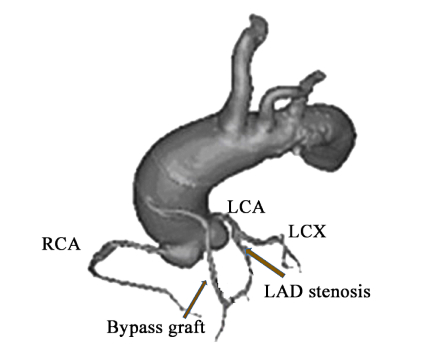
Based on the conventional angiogram of a coronary system having a LAD stenosis with bypass grafting as shown in Figure 1, our computational domain is the system of coronary arteries, including the regular RCA, the stenosed LAD, and the normal LCX, as shown in Figure 2. The ANSYS 2020 R1 software was used to conduct the simulation study. To speed up blood flow in the coronary arteries, we applied the external magnetic field in the same direction as inlet blood flow through the aorta, i.e.,

$\begin{equation} \textbf{B}_0 = (0, 0, B_z) \end{equation}$

(4.1)

Figure 1. The coronary system with LAD stenosis.

DownLoad: Full-Size Img PowerPoint

Figure 2. Computational domain with three investigated cross sections of the RCA, the LAD and the LCX.

DownLoad: Full-Size Img PowerPoint

which creates the Lorentz force opposing pulsatile blood flow in the aorta but supports the flow rate of blood in the RCA, the LAD and the LCX.

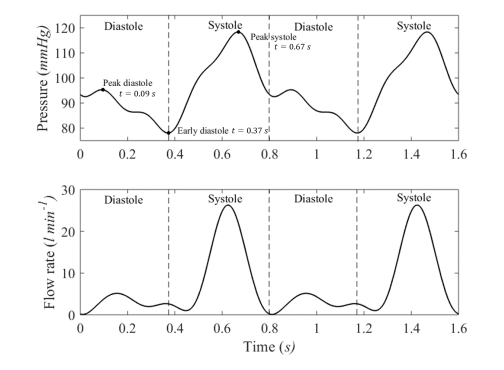
In the human cardiovascular system, heartbeat induces pulsatile pressure. During ventricular systole, with the ventricular wall contracted, the pressure within the ventricular wall exceeds that at the aortic root. When the ventricle relaxes during diastole, the aortic valve closes and the pressure within the wall falls below diastolic pressure. Consequently, blood is pumped from the aorta to the right and the left coronary arteries. shows the inflow periodic waveforms of blood pressure and flow rate with a cardiac cycle of 0.8 $\; s$ , which is typically within the valid range ^{[30,31,32,33]}. The general model parameters used in this numerical simulation are shown in Table 1 ^[33] and ^{[16,34,35,36]}. presents the values of $\alpha_m^p, \beta_m^p, \alpha_m^Q$ and $\beta_m^Q$ used in Eqs (2.11) and (2.12). Properties of blood and coronary artery are shown in Table 2.

Figure 3. The periodic blood pressure and flow rate waveforms for two cardiac cycles at the inflow surface of the aorta.

DownLoad: Full-Size Img PowerPoint

Table 1. Coefficients of pulsatile pressure and flow rate functions ^[33].

Coronary artery	m	$\alpha_m^p$	$\beta_m^p$	$\alpha_m^Q$	$\beta_m^Q$
Aorta	1	8.1269	–12.4156	1.7048	–7.5836
$\overline{Q}=5.04\times 10^{-4}\; m^3\cdot s^{-1}$	2	–6.1510	–1.1072	–6.7035	–2.1714
$\overline{p}=95\; mmHg$	3	–1.333	–0.3849	–2.6389	2.6462
$A = 6.7204\times 10^{-4}m^2$	4	–2.9473	1.1603	0.7198	0.7198
RCA/LAD/LCX	1	–3.3107	–2.2932	0.1007	0.0764
$\overline{p}=65\; mmHg$	2	–9.8639	8.0487	–0.0034	–0.0092
	3	3.0278	3.8009	0.0294	0.0337
	4	2.2476	–3.2564	0.0195	–0.0129

| Show Table

DownLoad: CSV

Table 2. Model parameters.

Parameter	Notation	Value		Unit
Parameter	Notation	Blood	Vessel wall	Unit
Density	$\rho$	1050	1102	$kg \cdot m^{-3}$
Heat capacity	$c_p$	3.85	3.306	$kJ \cdot kg^{-1}K^{-1}$
Thermal conductivity	$k$	0.52	0.460	$w \cdot m^{-1} K^{-1}$
Heat transfer coefficient	$h_\infty$	-	1000	$w \cdot m^{-2} K^{-1}$
Electrical conductivity	$\sigma$	0.7	0.232	$S\cdot m^{-1}$
Magnetic permeability	$\mu=\mu_0\mu_r$	$1.257 \times 10^{-6}$	$4\pi \times 10^{-7}$	$H \cdot m^{-1}$
Maximum shear viscosity	$\eta_{max}$	0.554712		$kg \cdot (m\cdot s)^{-1}$
Minimum shear viscosity	$\eta_{min}$	0.00345		$kg \cdot (m\cdot s)^{-1}$
Infinite shear viscosity	$\eta_{\infty}$	0.00345		$kg \cdot (m\cdot s)^{-1}$
Zero shear viscosity	$\eta_{0}$	0.16		$kg \cdot (m\cdot s)^{-1}$
Time constant	$\lambda$	8.2		$s$
Shape parameter	$p$	0.64
Consistency index	$n$	0.2128
External magnetic field intensity	$\|\textbf{B}_0\|$	1–3	-	$tesla$
Coefficient of magnetic-field-dependent viscosity	$c_1, \; c_2$	0.0974333, 0.102567

| Show Table

DownLoad: CSV

4.2. Numerical results

Three values of the imposed magnetic field, $|{\textbf{B}}_0| = 1, \; 2$ and $3$ teslas, are chosen and applied parallel to the aorta. The impacts of the magnetic field on the magnitude of induced magnetic field (b), the magnitude of Lorentz force (F), blood shear rate ( $\dot{\gamma}$ ), the percentage of viscosity reduction, speed, and temperature distribution are presented in the following graphical and tabular forms. The results are considered at the $76^{th}$ cardiac cycle after applying the external magnetic fields with the absence of temperature-dependent viscosity.

4.2.1. Induced magnetic field and Lorentz force

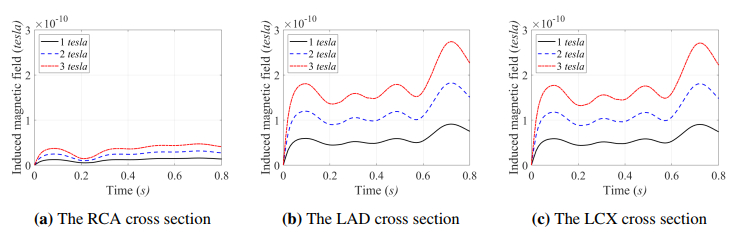
By increasing the magnetic intensity, B $_0$ , the magnitude of induced magnetic field, b, increases significantly for each artery cross section, as shown in Figure 4. The smallest magnitude of induced magnetic field occurs at the RCA cross section since this cross section is parallel to the inlet aorta, whereas the other cross sections are inclined towards it.

Figure 4. The effect of the imposed magnetic fields (B

$_0$ ) on the magnitude of induced magnetic field (b) at three investigated cross sections of the RCA, the LAD, and the LCX arteries.

DownLoad: Full-Size Img PowerPoint

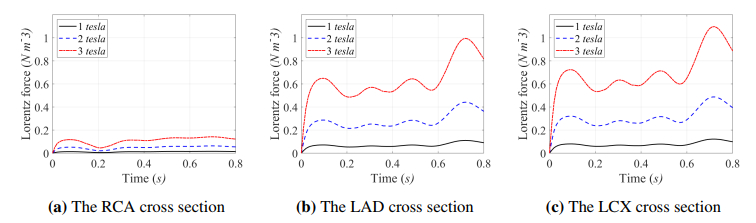
Applying a magnetic field to fluid results in the Lorentz force, which slows down the fluid in the aorta but speeds up the fluid in the RCA, the LAD and the LCX arteries. illustrates the magnitude of Lorentz force, F, obtained from the model with different $\vert \textbf{B}_0 \vert$ values of 1, 2 and 3 teslas. It is noted that the force increases as the magnetic field increases.

Figure 5. The effect of the imposed magnetic fields (B

$_0$ ) on the magnitude of Lorentz force (F) at three investigated cross sections of the RCA, the LAD, and the LCX arteries.

DownLoad: Full-Size Img PowerPoint

4.2.2. Characteristics of blood flow and temperature

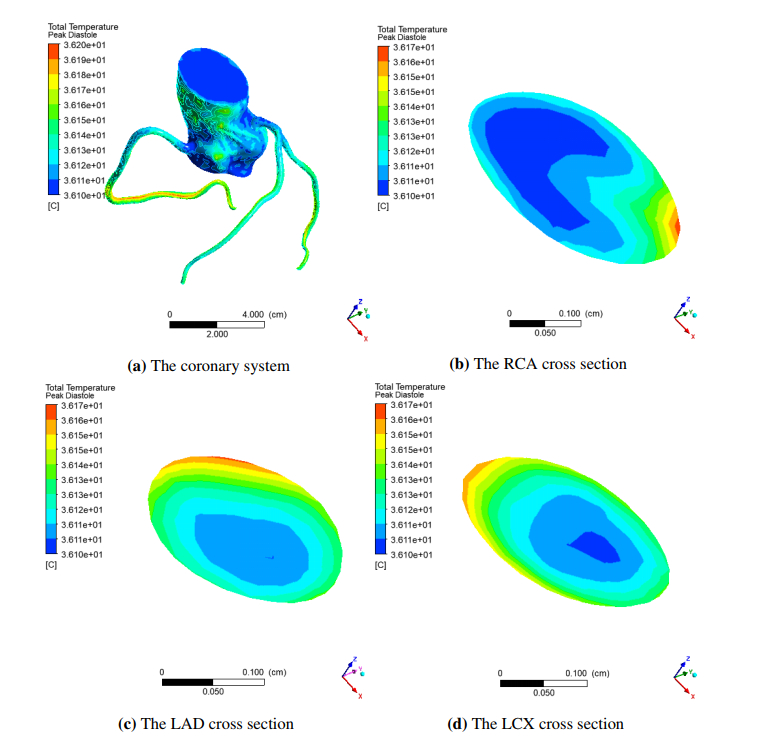
Since we focus on the coronary arteries, we thus consider the temperature distribution during diastole, which is the relaxation phase of the heart. To investigate the effect of magnetic field on temperature distribution using an initial blood temperature of $37 ^\circ$ C, temperature distribution on the RCA, the LAD and the LCX cross sections of the coronary system is examined. The results as shown in show the surface plots of temperature distributions obtained from the model with magnetic field strength of 1 tesla at the peak diastole. Temperatures vary between 36.1 and 36.2 $^\circ$ C on these three cross sections and the presence of a high temperature appears to be associated with arterial curvature.

Figure 6. Temperature distribution on the coronary system and three investigated cross sections of the RCA, the LAD, and the LCX arteries at the peak diastole for

$|\textbf{B}_0| = 1 \; tesla.$ .

DownLoad: Full-Size Img PowerPoint

Table 3 presents percentage of viscosity reduction obtained from the model with and without magnetic field. The percentage of viscosity reduction is determined from the results obtained from the unapplied and imposed magnetic fields. Three percentage values of viscosity reduction including average, minimum, and maximum viscosity for each cross section at different times are used to evaluate the magnetic effect. According to Table 3, the blood viscosity reduces as magnetic field strength increases. A high-intensity magnetic field produces a high percentage of viscosity reduction. For magnetic field intensities of 1, 2, and 3 tesla, the LAD cross section produces the average viscosity reduction in the ranges of [6.97%, 9.18%], [17.78%, 23.78%] and [22.22%, 29.75%], respectively. It is noted that percentage of viscosity reduction has a wide range of minimum values varying from about 6.91 to 74.18%, but a small range of maximum values varying from 23.15 to 31.62%. Our results align to the findings of the Tao-Huang experiment ^[23] and numerical studies ^[24,25,26].

Table 3. Effect of the imposed magnetic field on the percentage of viscosity reduction in average, minimum, and maximum values at three investigated cross sections on the RCA, the LAD and the LCX arteries.

Cross section	Investigated time	Viscosity reduction (%)
		From $\|\textbf{B}_0\| = 0$ to 1 $tesla$		From $\|\textbf{B}_0\| = 0$ to 2 $tesla$		From $\|\textbf{B}_0\| = 0$ to 3 $tesla$
		average	(min, max)	average	(min, max)	average	(min, max)
	early systole	9.22	(7.87, 21.82)	24.30	(51.08, 31.52)	29.69	(73.37, 31.52)
RCA	peak systole	7.98	(7.84, 22.26)	20.67	(52.29, 29.68)	25.73	(73.91, 29.68)
	peak diastole	8.98	(8.19, 22.22)	23.35	(51.27, 31.88)	28.91	(73.48, 31.88)
	early systole	9.18	(7.49, 23.39)	23.78	(52.31, 31.62)	29.75	(74.18, 31.62)
LAD	peak systole	6.97	(7.66, 23.15)	17.78	(50.38, 26.67)	22.22	(73.10, 26.67)
	peak diastole	7.81	(6.91, 23.29)	20.54	(51.38, 28.27)	25.33	(72.54, 28.27)
	early systole	4.98	(7.64, 21.90)	12.96	(50.91, 21.90)	16.15	(72.52, 21.90)
LCX	peak systole	3.44	(7.88, 17.11)	8.79	(50.32, 17.11)	11.26	(69.98, 17.11)
	peak diastole	4.02	(7.37, 19.28)	10.76	(52.03, 19.28)	13.17	(71.89, 19.28)

| Show Table

DownLoad: CSV

Although each investigated cross section shaped like an ellipse with major and minor axes, we present model variables including the shear rate and the speed of blood only along the major axis.

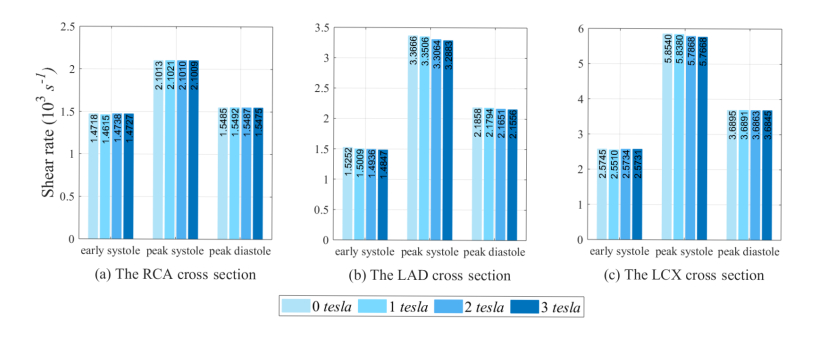
Figures 7 and 8 are bar plots of the minimum and maximum values of shear rate. The results indicate that the minimum shear rate increases as the magnetic field intensity increases. The minimum and maximum shear rates along the major axis of the LCX cross section for all choices of the magnetic strength are higher compared to other cross sections. For the model with a 3-tesla strength at the peak systole, the minimum shear rate along the major axis of the LCX artery is about 44.52 and 63.42% higher than those of the LAD artery and the RCA, respectively. This may relate to the greater curvature of the LCX artery with higher blood speed. However, there is no significant difference in maximum shear rate obtained from the model with different magnetic intensity as shown in Figure 8. Similarly, the maximum shear rate along the major axis of the LCX artery obtained from the model with a 3-tesla strength at the peak systole is about 42.98 and 63.57% higher than those of the LAD artery and the RCA.

Figure 7. Minimum shear rate (

$s^{-1}$ ) at three different times obtained from the model with various imposed magnetic fields at each cross section: (a) the RCA cross section; (b) the LAD cross section; (c) the LCX cross section.

DownLoad: Full-Size Img PowerPoint

Figure 8. Maximum shear rate (

DownLoad: Full-Size Img PowerPoint

For wall shear stress (WSS) investigation, the results are analysed along the total distance around the shape of each investigated cross section. The speed and flow rate are examined from the investigated cross sections. The comparison of the influence of magnetic fields on values of WSS, speed, and flow rate at the peak systole and the peak diastole on three cross sections with varying magnetic intensities ranging from 0 to 3 tesla is demonstrated in Table 4. The results reveal a decrease in maximum WSS as an increase in the magnetic field strength. The maximum values of WSS and speed at the peak systole are greater than those at the peak diastole. For the LAD artery, the WSS rises from 13.0838 to 13.4209 Pa at the peak systole and from 8.3849 to 8.4894 Pa at the peak diastole when magnetic intensity drops from 3 to 0 tesla.

Table 4. Effect of the imposed magnetic field on wall shear stress (

$Pa$ ), speed (

$m\cdot s^{-1}$ ), and flow rate (

$ml\cdot min^{-1}$ ) at the peak systole and the peak diastole on three investigated cross sections on the RCA, the LAD, and the LCX arteries.

Cross section	$\vert \textbf{B}_0 \vert$	The peak systole			The peak diastole
		WSS	Speed	flow rate	WSS	Speed	flow rate
		max	max	max	max	max	max
RCA	0	8.7091	0.5196	44.32	6.2620	0.4272	36.44
	1	8.7077	0.5230	44.61	6.2605	0.4309	36.75
	2	8.6926	0.5291	45.13	6.2459	0.4380	37.36
	3	8.6796	0.5322	45.40	6.2306	0.4415	37.66
LAD	0	13.4209	0.6407	3.15	8.4794	0.4931	2.42
	1	13.3531	0.6425	3.16	8.4577	0.4965	2.44
	2	13.1688	0.6444	3.17	8.4090	0.5030	2.47
	3	13.0838	0.6461	3.18	8.3849	0.5068	2.49
LCX	0	23.3705	0.8914	135.94	14.7041	0.6719	10.25
	1	23.3086	0.8913	135.92	14.7056	0.6737	10.27
	2	23.1064	0.8890	135.57	14.7000	0.6770	10.32
	3	23.0271	0.8887	135.53	14.6959	0.6788	10.35

| Show Table

DownLoad: CSV

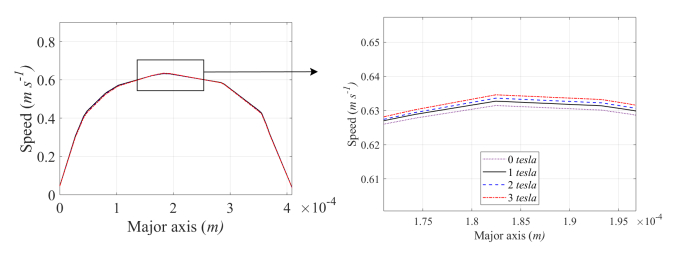
The blood speed in a full-wave cycle along the major axes of the RCA, the LCA, and the LCX cross sections obtained from the model with the magnetic intensity of a 3-tesla is investigated. The results show that the maximum speed along all investigated major axes is at the peak systole as shown in . It is observed that the velocity profile of blood over the investigated cross section is parabolic. Blood speed along the LAD major axis reaches a maximum of 0.3976, 0.6461, and 0.5068 m $\cdot$ s $^{-1}$ during the early systole, the peak systole, and the peak diastole, respectively. Comparison of blood speed along major axes of LAD cross section for various values of magnetic field intensity at the peak systole and the peak diastole are made. The model with increasing amounts of magnetic field intensity improves blood flow with higher speed in the system of coronary artery. It is observed that higher magnetic strength produces greater blood speed as shown in Figures 10 and 11. As flow rate is directly proportional to the fluid speed. The flow rates across the investigated cross sections are also investigated. An increase in the magnetic field strength increases blood flow rate. It is more noticeable that maximum flow rates through LAD cross section increase about 0.83% at the peak systole and 2.78% at the peak diastole for the model with a 3-tesla magnetic intensity, compared to the one without a magnetic field.

Figure 9. Velocity profile along the major axes of the RCA, the LAD, and the LCX cross sections at the early systole, the peak systole, and the peak diastole obtained from the model with the imposed magnetic field of a 3-tesla.

DownLoad: Full-Size Img PowerPoint

Figure 10. The effect of the imposed magnetic fields (B

$_0$ ) on the velocity profile along the LAD major axis at the peak systole.

DownLoad: Full-Size Img PowerPoint

Figure 11. The effect of the imposed magnetic fields (B

$_0$ ) on the velocity profile along the LAD major axis at the peak diastole.

DownLoad: Full-Size Img PowerPoint

In general, the blood flow rate is normally 10 ml $\cdot$ min $^{-1}$ with associated wall shear rates varying from 100 to 32,000 s $^{-1}$ (equivalent to wall shear stress (WSS) between 0.35 and 112 Pa) depending on the presence of stenosis and the dimensions of flow channels ^[38]. The results here are acceptable as we obtain blood flow through the regular LCX artery with a normal flow rate while in the LAD artery with stenosis, a small flow rate of blood is obtained.

5. Conclusions and discussion

Coronary artery disease (CAD), the most predominant form of heart disease, is one of the most common causes of death worldwide. Recent advances in treating patients with the CAD show promising effects in managing the CAD. These treatments combine different scientific disciplines of biotechnology and tissue engineering and have led to the development of novel therapeutic strategies. Magnetic therapy is one of the practical approaches widely used nowadays in medicine for preventing CAD, particularly myocardial infarction. Thus, it is essential to understand the impact on blood flow during acute exposure to a magnetic field. Recently, the effect of magnetic fields on blood flow has been a crucial topic for biomedical engineers.

The novelty of this paper is to introduce a new suggestion for variation imposed magnetic field effects on three-dimensional pulsatile MHD flow of blood in the three-dimensional system of coronary arteries with coronary stenosis. Blood is assumed to be incompressible non-Newtonian fluid through the system of coronary arteries, including the base of the aorta, the RCA, and two branches (LAD and LCX) of the LCA. Based on Carreau Model, blood viscosity depends on the blood temperature and Arrhenius activation energy. This study investigates the impact of magnetic field exposure on blood flow and temperature distribution in the system of the coronary arteries, with coronary stenosis appearing at the proximal LAD artery.

Findings from our study confirm that the magnetic field can control the heat and blood flow characteristics through the system of coronary arteries with coronary stenosis. The heat created from the magnetic field improves blood temperature. There is a change in the viscosity in the presence of a magnetic field. A stronger magnetic field reduces blood viscosity and enhances blood speed. However, other assumptions involved in the proposed three-dimensional pulsatile MHD flow model are also unrealistic to some extent. For example,

● Artery components of the RCA, the LAD, and the LCX arteries are not included in our computation domain.

● Although blood can flow through the inner layer of the arterial wall, in this study, the impact of the magnetic field on blood in this innermost layer is not considered.

● Blood is a biomagnetic fluid. It is necessary to select a magnetic field with an acceptable range of influence on the blood flow. However, this study only chose the magnetic field in a small range between 1 and 3 teslas.

These flaws restrict the implementation of the proposed model. However, the proposed model is still a powerful tool to estimate blood speed and temperature distribution in the system of coronary arteries with coronary stenosis. In future research, the arterial wall and branches of the RCA, the LAD, and the LCX arteries must be included in the computation domain. Blood flow through both the flow channel and the inner layer of the arterial wall must be investigated. In addition, the effect of the magnetic field intensity in small, medium, and high ranges on blood flow must be analysed numerically.

Acknowledgments

The first author would like to thank the Science Achievement Scholarship of Thailand (SAST) and the Faculty of Graduate Studies, Mahidol University, Thailand. The first author also thanks you to the School of Electrical Engineering, Computing and Mathematical Sciences, Curtin University, Australia, for all facilities supported during one year of student visit in 2020.

Data Availability

The data used to support the findings of this study are available from the corresponding authors upon request.

Conflict of interest

The authors declare there is no conflict of interest.

References

[1]	D. M. Herlach, P. K. Galenko, D. H. Moritz, Metastable solids from undercooled melts, Elsevier, 2007. https://doi.org/10.4028/www.scientific.net/MSF.539-543.1977
[2]	N. Provatas, K. Elder, Phase-field methods in materials science and engineering, Wiley-VCH, 2010.
[3]	M. I. Ojovan, Viscosity and glass transition in amorphous oxides, Adv. Cond. Matter Phys., 2008 (2008), 817829. https://doi.org/10.1155/2008/817829 doi: 10.1155/2008/817829
[4]	M. Sperl, E. Zaccarelli, F. Sciortino, P. Kumar, H. E. Stanley, Disconnected glass-glass transitions and diffusion anomalies in a model with two repulsive length scales, Phys. Rev. Lett., 104 (2010), 145701. https://doi.org/10.1103/PhysRevLett.104.145701 doi: 10.1103/PhysRevLett.104.145701
[5]	L. Xu, S. V. Buldyrev, N. Giovambattista, H. E. Stanley, Liquid-liquid phase transition and glass transition in a monoatomic model system, Int. J. Mol. Sci., 11 (2010), 5184–5200. https://doi.org/10.3390/ijms11125184 doi: 10.3390/ijms11125184
[6]	H. Tanaka, T. Kawasaki, H. Shintani, K. Watanabe, Critical-like behaviour of glass-forming liquids, Nat. Mater., 9 (2010), 324–331. https://doi.org/10.1038/nmat2634. doi: 10.1038/nmat2634
[7]	D. M. Herlach, Non-equilibrium solidification of undercooled metallic metls, Mat. Sci. Eng. R, 12 (1994), 177–272. https://doi.org/10.1016/0927-796X(94)90011-6 doi: 10.1016/0927-796X(94)90011-6
[8]	W. Gotze, L. Sjogren, Relaxation processes in supercooled liquids, Rep. Prog. Phys., 55 (1992), 241–376. https://doi.org/10.1088/0034-4885/55/3/001 doi: 10.1088/0034-4885/55/3/001
[9]	J. Jackle, Models of the glass transition, Rep. Prog. Phys., 49 (1986), 171. https://dx.doi.org/10.1088/0034-4885/49/2/002 doi: 10.1088/0034-4885/49/2/002
[10]	P. K. Galenko, D. Jou, Rapid solidification as non-ergodic phenomenon, Phys. Rep., 818 (2019), 1–70. https://doi.org/10.1016/j.physrep.2019.06.002 doi: 10.1016/j.physrep.2019.06.002
[11]	R. E. Ryltsev, N. M. Chtchelkatchev, V. N. Ryzhov, Superfragile glassy dynamics of a one-component system with isotropic potential: Competition of diffusion and frustration, Phys. Rev. Lett., 110 (2013), 025701. https://doi.org/10.1103/PhysRevLett.110.025701 doi: 10.1103/PhysRevLett.110.025701
[12]	M. G. Vasin, Glass transition as a topological phase transition, Phys. Rev. E, 106 (2022), 044124. https://doi.org/10.1103/PhysRevE.106.044124 doi: 10.1103/PhysRevE.106.044124
[13]	E. Kats, V. Lebedev, A. Muratov, Weak crystallization theory, Phys. Rep., 228 (1993), 1–91. https://doi.org/10.1016/0370-1573(93)90119-X doi: 10.1016/0370-1573(93)90119-X
[14]	R. Prieler, D. Li, H. Emmerich, Nucleation and successive microstructure evolution via phase-field and phase-field crystal method, J. Cryst. Growth, 312 (2010), 1434–1436. http://dx.doi.org/10.1016/j.jcrysgro.2009.09.022 doi: 10.1016/j.jcrysgro.2009.09.022
[15]	D. Tourret, H. Liu, J. LLorca, Phase-field modeling of microstructure evolution: Recent applications, perspectives and challenges, Prog. Mater. Sci., 123 (2022), 100810. https://doi.org/10.1016/j.pmatsci.2021.100810 doi: 10.1016/j.pmatsci.2021.100810
[16]	D. Jou, J. C. Vázquez, G. Lebon, Extended irreversible thermodynamics, 4 Eds., Springer Berlin Heidelberg, 2010. https://doi.org/10.1007/978-90-481-3074-0
[17]	P. Galenko, D. Jou, Diffuse-interface model for rapid phase transformations in nonequilibrium systems, Phys. Rev. E, 71 (2005), 046125. https://doi.org/10.1103/PhysRevE.71.046125 doi: 10.1103/PhysRevE.71.046125
[18]	M. D. Krivilyov, E. V. Kharanzhevskii, V. G. Lebedev, D. A. Danilov, E. V. Danilova, P. K. Galenko, Synthesis of composite coatings using rapid laser sintering of metallic powder mixtures, Phys. Met. Metallogr.+, 114 (2013), 799–820. https://doi.org/10.1134/S0031918X13080073 doi: 10.1134/S0031918X13080073
[19]	H. Fu, M. Dehsara, M. Krivilyov, S. D. Mesarovic, D. P. Sekulic, Kinetics of the molten Al-Si triple line movement during a brazed joint formation, J. Mater. Sci., 51 (2016), 1798–1812. https://doi.org/10.1007/s10853-015-9550-7 doi: 10.1007/s10853-015-9550-7
[20]	I. Steinbach, L. Zhang, M. Plapp, Phase-field model with finite interface dissipation, Acta Mater., 60 (2012), 2689–2701. https://doi.org/10.1016/j.actamat.2012.01.035 doi: 10.1016/j.actamat.2012.01.035
[21]	H. Wang, P. K. Galenko, X. Zhang, W. Kuang, F. Liu, D. M. Herlach, Phase-field modeling of an abrupt disappearance of solute drag in rapid solidification, Acta Mater., 90 (2015), 282–291. https://doi.org/10.1016/j.actamat.2015.02.021 doi: 10.1016/j.actamat.2015.02.021
[22]	D. A. Danilov, V. G. Lebedev, P. K. Galenko, A grand potential approach to phase-field modeling of rapid solidification, J. Non-Equil. Thermody., 39 (2014), 93–111. https://doi.org/10.1515/jnetdy-2013-0032 doi: 10.1515/jnetdy-2013-0032
[23]	U. M. B. Marconi, P. Tarazona, Dynamic density functional theory of fluids, J. Chem. Phys., 110 (1999), 8032–8044. https://doi.org/10.1063/1.478705 doi: 10.1063/1.478705
[24]	S. Van Teeffelen, R. Backofen, A. Voigt, H. Löwen, Derivation of the phase-field-crystal model for colloidal solidification, Phys. Rev. E, 79 (2009), 1–10. https://doi.org/10.1103/PhysRevE.79.051404 doi: 10.1103/PhysRevE.79.051404
[25]	V. Ankudinov, I. Starodumov, N. P. Kryuchkov, E. V. Yakovlev, S. O. Yurchenko, P. K. Galenko, Correlated noise effect on the structure formation in the phase-field crystal model, Math. Method. Appl. Sci., 44 (2021), 12185–12193. https://doi.org/10.1002/mma.6887 doi: 10.1002/mma.6887
[26]	J. Berry, K. R. Elder, M. Grant, Simulation of an atomistic dynamic field theory for monatomic liquids: Freezing and glass formation, Phys. Rev. E, 77 (2008), 061506. https://doi.org/10.1103/PhysRevE.77.061506 doi: 10.1103/PhysRevE.77.061506
[27]	A. J. Archer, M. J. Robbins, U. Thiele, E. Knobloch, Solidification fronts in supercooled liquids: How rapid fronts can lead to disordered glassy solids, Phys. Rev. E, 86 (2012), 031603. https://doi.org/10.1103/PhysRevE.86.031603 doi: 10.1103/PhysRevE.86.031603
[28]	J. Berry, M. Grant, Modeling multiple time scales during glass formation with phase-field crystals, Phys. Rev. Lett., 106 (2011), 175702. https://doi.org/10.1103/PhysRevLett.106.175702 doi: 10.1103/PhysRevLett.106.175702
[29]	S. Abdalla, A. J. Archer, L. Gránásy, G. I. Tóth, Thermodynamics, formation dynamics, and structural correlations in the bulk amorphous phase of the phase-field crystal model, J. Chem. Phys., 157 (2022), 164502. https://doi.org/10.1063/5.0114705 doi: 10.1063/5.0114705
[30]	S. Tang, J. C. Wang, B. Svendsen, D. Raabe, Competitive bcc and fcc crystal nucleation from non-equilibrium liquids studied by phase-field crystal simulation, Acta Mater., 139 (2017), 196–204. https://doi.org/10.1016/j.actamat.2017.08.015 doi: 10.1016/j.actamat.2017.08.015
[31]	T. Wang, E. Napolitano, A phase-field model for phase transformations in glass-forming alloys, Metall. Mater. Trans. A, 43 (2012), 2662–2668. https://doi.org/10.1007/s11661-012-1136-2 doi: 10.1007/s11661-012-1136-2
[32]	A. Ericsson, M. Fisk, H. Hallberg, Modeling of nucleation and growth in glass-forming alloys using a combination of classical and phase-field theory, Comput. Mater. Sci., 165 (2019), 167–179. https://doi.org/10.1016/j.commatsci.2019.04.008 doi: 10.1016/j.commatsci.2019.04.008
[33]	P. Bruna, E. Pineda, D. Crespo, Phase-field modeling of glass crystallization: Change of the transport properties and crystallization kinetic, J. Non-Cryst. Solids, 353 (2007), 1002–1004. https://doi.org/10.1016/j.jnoncrysol.2006.12.086 doi: 10.1016/j.jnoncrysol.2006.12.086
[34]	P. K. Galenko, V. Ankudinov, K. Reuther, M. Rettenmayr, A. Salhoumi, E. V. Kharanzhevskiy, Thermodynamics of rapid solidification and crystal growth kinetics in glass-forming alloys, Philos. T. Roy. Soc. A, 377 (2019), 20180205. https://doi.org/10.1098/rsta.2018.0205 doi: 10.1098/rsta.2018.0205
[35]	P. K. Galenko, R. Wonneberger, S. Koch, V. Ankudinov, E. V. Kharanzhevskiy, M. Rettenmayr, Bell-shaped "dendrite velocity-undercooling" relationship with an abrupt drop of solidification kinetics in glass forming Cu-Zr(-Ni) melts, J. Cryst. Growth, 532 (2020), 125411. https://doi.org/10.1016/j.jcrysgro.2019.125411 doi: 10.1016/j.jcrysgro.2019.125411
[36]	M. V. Dudorov, A. D. Drozin, A. V. Stryukov, V. E. Roshchin, Mathematical model of solidification of melt with high-speed cooling, J. Phys.-Condens. Mat., 34 (2022), 444002. https://doi.org/10.1088/1361-648X/ac8c12 doi: 10.1088/1361-648X/ac8c12
[37]	P. A. Gamov, A. D. Drozin, M. V. Dudorov, V. E. Roshchin, Model for nanocrystal growth in an amorphous alloy, Russ. Metall.+, 2012 (2012), 1002–1005. https://doi.org/10.1134/S0036029512110055 doi: 10.1134/S0036029512110055
[38]	M. M. A. Rafique, Modelling and simulation of solidification phenomena during additive manufacturing of bulk metallic glass matrix composites (BMGMC)—A brief review and introduction of technique, J. Encapsulat. Adsorpt. Sci., 8 (2018), 67–116. https://doi.org/10.4236/jeas.2018.82005 doi: 10.4236/jeas.2018.82005
[39]	X. H. Wu, G. Wang, D. C. Zeng, Z. W. Liu, Prediction of the glass-forming ability of Fe-B binary alloys based on a continuum-field-multi-phase-field model, Comput. Mater. Sci., 108 (2015), 27–33. https://doi.org/10.1016/j.commatsci.2015.06.004 doi: 10.1016/j.commatsci.2015.06.004
[40]	X. Wu, G. Wang, D. Zeng, Prediction of the glass forming ability in a Fe-25%B binary amorphous alloy based on phase-field method, J. Non-Cryst. Solids, 466–467 (2017), 52–57. https://doi.org/10.1016/j.jnoncrysol.2017.03.043 doi: 10.1016/j.jnoncrysol.2017.03.043
[41]	S. Ganorkar, Y. H. Lee, S. Lee, Y. C. Cho, T. Ishikawa, G. W. Lee, Unequal effect of thermodynamics and kinetics on glass forming ability of Cu-Zr alloys, AIP Adv., 10 (2020), 045114. https://doi.org/10.1063/5.0002784 doi: 10.1063/5.0002784
[42]	C. Cammarota, G. Gradenigo, G. Biroli, Confinement as a tool to probe amorphous order, Phys. Rev. Lett., 111 (2013), 1–5. https://doi.org/10.1103/PhysRevLett.111.107801 doi: 10.1103/PhysRevLett.111.107801
[43]	A. A. Novokreshchenova, V. G. Lebedev, Determining the phase-field mobility of pure nickel based on molecular dynamics data, Tech. Phys., 62 (2017), 642–644. https://doi.org/10.1134/S1063784217040181 doi: 10.1134/S1063784217040181
[44]	A. Z. Patashinskii, V. L. Pokrovskii, Fluctuation theory of phase transitions, Oxford/New York: Pergamon Press, 1979. http://doi.org/10.1002/bbpc.19800840723
[45]	A. N. Vasilev, Quantum-field renormalization groups in the cases of critical-behavior and stochastical dynamics, Russia: PIYAF Publ., Saint-Petersburg, 1998.
[46]	M. G. Vasin, V. M. Vinokur, Description of glass transition kinetics in 3D XY model in terms of gauge field theory, Phys. A, 525 (2019), 1161–1169. https://doi.org/10.1016/j.physa.2019.04.065 doi: 10.1016/j.physa.2019.04.065
[47]	G. Toulouse, Theory of the frustration effect in spin glasses: Ⅰ, Commun. Phys., 2 (1977), 115–119.
[48]	J. C. Dyre, Colloquium: The glass transition and elastic models of glass-forming liquids, Rev. Mod. Phys., 78 (2006), 953–972. https://doi.org/10.1103/RevModPhys.78.953 doi: 10.1103/RevModPhys.78.953
[49]	J. Villain, Two-level systems in a spin-glass model: Ⅱ. Three-dimensional model and effect of a magnetic field, J. Phys. C, 11 (1978), 745. https://doi.org/10.1088/0022-3719/11/4/018 doi: 10.1088/0022-3719/11/4/018
[50]	E. Fradkin, B. A. Huberman, S. H. Shenker, Gauge symmetries in random magnetic systems, Phys. Rev. B, 18 (1978), 4789–4814. https://link.aps.org/doi/10.1103/PhysRevB.18.4789
[51]	N. R. Blackett, Disclination lines in glasses, Philos. Mag., 40 (1979), 859–868. https://doi.org/10.1080/01418617908234879 doi: 10.1080/01418617908234879
[52]	D. R. Nelson, Order, frustration, and defects in liquids and glasses, Phys. Rev. B, 28 (1983), 5515–5535. https://doi.org/10.1103/PhysRevB.28.5515 doi: 10.1103/PhysRevB.28.5515
[53]	I. E. Dzyaloshinskii, G. E. Volovick, Poisson brackets in condensed matter physics, Ann. Phys., 125 (1980), 67–97. https://doi.org/10.1016/0003-4916(80)90119-0 doi: 10.1016/0003-4916(80)90119-0
[54]	I. E. Dzyaloshinskii, S. P. Obukhov, Topological phase transition in the XY model of a spin glass, Zh. Eksp. Teor. Fiz, 83 (1982), 13–832.
[55]	M. Baggioli, I. Kriuchevskyi, T. W. Sirk, A. Zaccone, Plasticity in amorphous solids is mediated by topological defects in the displacement field, Phys. Rev. Lett., 127 (2021), 015501. https:/doi.org/10.1103/PhysRevLett.127.015501 doi: 10.1103/PhysRevLett.127.015501
[56]	M. Baggioli, M. Landry, A. Zaccone, Deformations, relaxation, and broken symmetries in liquids, solids, and glasses: A unified topological field theory, Phys. Rev. E, 105 (2022), 024602. https://doi.org/10.1103/PhysRevE.105.024602 doi: 10.1103/PhysRevE.105.024602
[57]	J. Frenkel, Kinetic theory of liquids, Oxford: Oxford University Press, 1947.
[58]	M. Grimsditch, R. Bhadra, L. M. Torell, Shear waves through the glass-liquid transformation, Phys. Rev. Lett., 62 (1989), 2616. https://doi.org/10.1103/PhysRevLett.62.2616 doi: 10.1103/PhysRevLett.62.2616
[59]	T. Pezeril, C. Klieber, S. Andrieu, K. A. Nelson, Optical generation of gigahertz-frequency shear acoustic waves in liquid glycerol, Phys. Rev. Lett., 102 (2009), 107402. https://doi.org/10.1103/PhysRevLett.102.107402 doi: 10.1103/PhysRevLett.102.107402
[60]	Y. H. Jeong, S. R. Nagel, S. Bhattacharya, Ultrasonic investigation of the glass transition in glycerol, Phys. Rev. A, 34 (1986), 602. https://doi.org/10.1103/PhysRevA.34.602 doi: 10.1103/PhysRevA.34.602
[61]	S. Hosokawa, M. Inui, Y. Kajihara, K. Matsuda, T. Ichitsubo, W. C. Pilgrim, et al., Transverse acoustic excitations in liquid Ga, Phys. Rev. Lett., 102 (2009), 105502. https://doi.org/10.1103/PhysRevLett.102.105502 doi: 10.1103/PhysRevLett.102.105502
[62]	V. M. Giordano, G. Monaco, Inelastic x-ray scattering study of liquid Ga: Implications for the short-range order, Phys. Rev. B, 84 (2011), 052201. https://doi.org/10.1103/PhysRevB.84.052201 doi: 10.1103/PhysRevB.84.052201
[63]	T. Scopigno, G. Ruocco, F. Sette, Microscopic dynamics in liquid metals: The experimental point of view, Rev. Mod. Phys., 77 (2005), 881–933. https://doi.org/10.1103/RevModPhys.77.881 doi: 10.1103/RevModPhys.77.881
[64]	E. Pontecorvo, M. Krisch, A. Cunsolo, G. Monaco, A. Mermet, R. Verbeni, et al., High-frequency longitudinal and transverse dynamics in water, Phys. Rev. E, 71 (2005), 011501. https://doi.org/10.1103/PhysRevE.71.011501 doi: 10.1103/PhysRevE.71.011501
[65]	W. C. Pilgrim, C. Morkel, State dependent particle dynamics in liquid alkali metals, J. Phys.-Condens. Mat., 18 (2006), R585. https://doi.org/10.1088/0953-8984/18/37/R01 doi: 10.1088/0953-8984/18/37/R01
[66]	M. G. Vasin, V. Ankudinov, Phase-field model of glass transition: Behavior under uniform quenching, Phase Transit., 2024, 1–19. https://dx.doi.org/10.1080/01411594.2024.2353297
[67]	P. Minnhagen, The two-dimensional Coulomb gas, vortex unbinding, and superfluid-superconducting films, Rev. Mod. Phys., 59 (1987), 1001. https://doi.org/10.1103/RevModPhys.59.1001 doi: 10.1103/RevModPhys.59.1001
[68]	M. Vasin, V. Ankudinov, Soft model of solidification with the order-disorder states competition, Math. Method. Appl. Sci., 45 (2022), 8082–8095. https://doi.org/10.1002/mma.8035 doi: 10.1002/mma.8035
[69]	M. Vasin, V. Ankudinov, Competition of glass and crystal: Phase-field model, Math. Method. Appl. Sci., 47 (2024), 6798–6809. https://doi.org/10.1002/mma.9207 doi: 10.1002/mma.9207
[70]	P. C. Hohenberg, B. I. Halperin, Theory of dynamic critical phenomena, Rev. Mod. Phys., 49 (1977), 435–479. https://doi.org/10.1103/RevModPhys.49.435 doi: 10.1103/RevModPhys.49.435
[71]	www.comsol.com, COMSOL Multiphysics® v.6.0, www.comsol.com, COMSOL AB, Stockholm, Sweden.
[72]	R. C. Budhani, T. C. Goel, K. L. Chopra, Melt-spinning technique for preparation of metallic glasses, B. Mater. Sci., 4 (1982), 549–561. https://doi.org/10.1007/BF02824962 doi: 10.1007/BF02824962
[73]	J. Schroers, Processing of bulk metallic glass, Adv. Mater., 22 (2010), 1566–1597. https://doi.org/10.1002/adma.200902776 doi: 10.1002/adma.200902776
[74]	M. Vasin, V. Lebedev, V. Ankudinov, K. Shklyaev, The phase-field model of the glass transition, Chem. Phys. Mesoscopy, 25 (2023), 14. https://doi.org/10.15350/17270529.2023.4.46 doi: 10.15350/17270529.2023.4.46
[75]	P. A. Geslin, C. H. Chen, A. M. Tabrizi, A. Karma, Dendritic needle network modeling of the Columnar-to-Equiaxed transition. Part Ⅰ: Two dimensional formulation and comparison with theory, Acta Mater., 202 (2021), 42–54. https://doi.org/10.1016/j.actamat.2020.10.009 doi: 10.1016/j.actamat.2020.10.009
[76]	W. Kurz, C. Bezençon, M. Gäumann, Columnar to equiaxed transition in solidification processing, Sci. Technol. Adv. Mat., 2 (2001), 185–191. https://doi.org/10.1016/S1468-6996(01)00047-X doi: 10.1016/S1468-6996(01)00047-X
[77]	B. R. Bird, E. S. Warren, N. E. Lightfoot, Transport phenomena, John Wiley & Sons, 2007.
[78]	Z. Wang, B. Riechers, P. M. Derlet, R. Maaß, Atomic cluster dynamics causes intermittent aging of metallic glasses, Acta Mater., 267 (2024), 119730. https://doi.org/10.1016/j.actamat.2024.119730 doi: 10.1016/j.actamat.2024.119730
[79]	V. I. Tkatch, A. M. Grishin, V. V. Maksimov, Estimation of the heat transfer coefficient in melt spinning process, J. Phys., 144 (2009), 012104. https://doi.org/10.1088/1742-6596/144/1/012104 doi: 10.1088/1742-6596/144/1/012104
[80]	R. E. Napolitano, H. Meco, The role of melt pool behavior in free-jet melt spinning, Metall. Mater. Trans. A, 35 A (2004), 1539–1553. https://doi.org/10.1007/s11661-004-0261-y doi: 10.1007/s11661-004-0261-y
[81]	A. B. Lysenko, T. V. Kalinina, A. M. V. Tomina, Y. V. Vishnevskaya, O. Popil, Production conditions and fine structure parameters of metallic glasses based on light rare-earth elements, In: IOP Conference Series: Materials Science and Engineering, IOP Publishing, 1256 (2022), 012011. https://doi.org/10.1088/1757-899X/1256/1/012011
[82]	O. Gusakova, V. Shepelevich, D. Alexandrov, I. Starodumov, Rapid quenching effect on the microstructure of Al-Si eutectic Zn-doped alloy, J. Cryst. Growth, 531 (2020), 125333. https://doi.org/10.1016/j.jcrysgro.2019.125333. doi: 10.1016/j.jcrysgro.2019.125333
[83]	S. A. Tavolzhanskii, I. N. Pashkov, G. A. Aleksanyan, Analysis of the production of ribbons of Copper-Phosphorus solder by the lateral flow of a melt onto a rotating roller mold, Metallurgist, 59 (2016), 843–850. http://link.springer.com/10.1007/s11015-016-0182-1.
[84]	A. A. Shirzadi, T. Kozieł, G. Cios, P. Bała, Development of Auto Ejection Melt Spinning (AEMS) and its application in fabrication of cobalt-based ribbons, J. Mater. Process. Tech., 264 (2019), 377–381. https://doi.org/10.1016/j.jmatprotec.2018.09.028 doi: 10.1016/j.jmatprotec.2018.09.028
[85]	R. Prasad, G. Phanikumar, Martensite and nanocrystalline phase formation in rapidly solidified Ni $_2$ MnGa alloy by melt-spinning, Mater. Sci. Forum, 649 (2010), 35–40. https://doi.org/10.4028/www.scientific.net/MSF.649.35 doi: 10.4028/www.scientific.net/MSF.649.35
[86]	A. K. Demyanetz, M. Bamberger, M. Regev, Quantitative microstructure study of melt-spun Mg $_{65}$ Cu $_{25}$ Y $_{10}$ , SN Appl. Sci., 2 (2020), 1–10. https://doi.org/10.1007/s42452-020-03522-3 doi: 10.1007/s42452-020-03522-3
[87]	V. I. Tkatch, A. I. Limanovskii, S. N. Denisenko, S. G. Rassolov, The effect of the melt-spinning processing parameters on the rate of cooling, Mater. Sci. Eng. A, 323 (2002), 91–96. https://doi.org/10.1016/S0921-5093(01)01346-6 doi: 10.1016/S0921-5093(01)01346-6
[88]	N. Liu, T. Ma, C. Liao, G. Liu, R. M. O. Mota, J. Liu, et al., Combinatorial measurement of critical cooling rates in aluminum-base metallic glass forming alloys, Sci. Rep., 11 (2021), 3903. https://doi.org/10.1038/s41598-021-83384-w doi: 10.1038/s41598-021-83384-w
[89]	H. Fiedler, H. Mühlbach, G. Stephani, The effect of the main processing parameters on the geometry of amorphous metal ribbons during planar flow casting (PFC), J. Mater. Sci., 19 (1984), 3229–3235. https://doi.org/10.1007/BF00549809 doi: 10.1007/BF00549809
[90]	H. S. Chen, C. E. Miller, Centrifugal spinning of metallic glass filaments, Mater. Res. Bull., 11 (1976), 49–54. https://doi.org/10.1016/0025-5408(76)90213-0 doi: 10.1016/0025-5408(76)90213-0

This article has been cited by:

1.	G. Dharmaiah, J.L. Rama Prasad, K.S. Balamurugan, I. Nurhidayat, Unai Fernandez-Gamiz, S. Noeiaghdam, Performance of magnetic dipole contribution on ferromagnetic non-Newtonian radiative MHD blood flow: An application of biotechnology and medical sciences, 2023, 9, 24058440, e13369, 10.1016/j.heliyon.2023.e13369
2.	Majid Siavashi, Ava Bina, Mojtaba Sayadnejad, Borhan Beigzadeh, Fluid-solid interaction analysis of blood flow in the atherosclerotic carotid artery using the Eulerian-Lagrangian approach, 2024, 31, 2095-2899, 151, 10.1007/s11771-023-5395-4
3.	Ahmed Elhanafy, Yasser Abuouf, Samir Elsagheer, Shinichi Ookawara, Mahmoud Ahmed, Effect of external magnetic field on realistic bifurcated right coronary artery hemodynamics, 2023, 35, 1070-6631, 10.1063/5.0152322
4.	Yan Wang, Zhen‐Gang Zhao, Zheng Chai, Jian‐Cheng Fang, Mao Chen, Electromagnetic field and cardiovascular diseases: A state‐of‐the‐art review of diagnostic, therapeutic, and predictive values, 2023, 37, 0892-6638, 10.1096/fj.202300201RR
5.	Bahram Vaziri, Saadat Zirak, Mohammad Azadi, Amir Keshmiri, Nima Shokri, Effect of stent struts angle on body vessel shear stress at different heat fluxes, 2025, 1388-6150, 10.1007/s10973-024-13961-4

Reader Comments

Your name:*

Email:*
© 2024 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)