Optimization of compression moulding parameters of multiwall carbon nanotube/synthetic graphite/epoxy nanocomposites with respect to electrical conductivity

1.   Introduction

Bipolar plates are the main components of polymer electrolyte membrane (PEM) fuel cells, constituting 80% of PEM fuel cells by weight ^[1,2]. Conductive polymer composites (CPCs) are potential bipolar plate materials, because these materials can be produced according to the desired shape at relatively inexpensive prices ^[3,4,5]. However, these materials have low electrical conductivity.

Several attempts have been made by researchers to improve the electrical conductivity of CPCs by adding conductive fillers of various shapes, sizes and contents. Expanded graphite (EG), graphite (G), carbon black (CB), carbon fibres (CF), and carbon nanotubes (CNTs) have been frequently used to improve the electrical conductivity of CPCs ^{[6,7,8,9,10,11,12,13,14]}. However, if higher contents of these conductive fillers are not well dispersed in the matrix (forming agglomerations), then the electrical conductivity of the resulting CPC material decreases ^[15]. The Department of Energy of the United States has provided requirements for CPCs as bipolar plate materials, namely, that the electrical conductivity must be > 100 S/cm, and the flexural strength must be > 25 MPa.

Du et al. ^[16] conducted research on the properties of CPCs using thin epoxy resin as a binder and expanded graphite as a conductive filler. The optimum electrical conductivity for the bipolar plate composite is obtained from an expanded graphite content of 70 wt%, that is, 119.8 S/cm. Suherman et al. ^[14] used hybrid fillers, with graphite as the main conductive filler, carbon nanotubes as a second conductive filler, and epoxy resin as a binder at a content of 20 weight percent (wt%). These researchers obtained an optimum composition of (75/5/20 wt%), respectively. The moulding parameters that significantly influence the bipolar plate composite properties are the moulding temperature, moulding time, and moulding pressure. San et al. ^[17] used a thermosetting material as a matrix and synthetic graphite and carbon as conductive materials. These researchers used response surface methodology (RSM) to optimize the parameters to produce a bipolar plate material. These researchers found that the maximum electrical conductivity of the bipolar plate composite was 107.4 S/cm. Akhtar et al. ^[18] used multifillers of multiwall carbon nantube (MWCNT) and natural graphite (NG) to produce bipolar plates, by using epoxy resins as a matrix. The electrical conductivity of the bipolar plate composite material produced was 126 S/cm. Selamat et al. ^[19] conducted a study using the Taguchi method to optimize the multiple responses of the compression moulding parameters of a polypropylene–graphite composite. These researchers found that the Taguchi method obtained a balance between the flexural strength and electrical conductivity. However, the electrical conductivity and flexural strength were still low at 16.61 S/cm and 19.82 MPa, respectively, and it did not meet the U.S. Department of Energy requirements.

Previous research shows that very few researchers use an optimization method to obtain optimum properties for CPC materials. Therefore, the orthogonal array (OA)-Taguchi method and signal to noise ratio (SNR) analysis were used ^[20,21,22] to obtain the optimal electrical conductivity while reducing the time and cost ^[20,23,24]. Thus, the optimization of the compression moulding parameters of MWCNT/SG/epoxy nanocomposites for bipolar plates with respect to electrical conductivity was investigated using the Taguchi method.

2.   Materials and methods

2.1.   Materials

Synthetic graphite (SG) and multiwalled carbon nanotubes (MWCNTs) were used as the primary and secondary fillers in this study, respectively. SG with particle size of 74 μm and surface area of 1.5 m²/g was obtained from Asbury Carbons, New Jersey. MWCNTs with surface area of 300 m²/g, diameter of 9.5 nm, length of 1.5 mm, and purity of 90% were obtained from Nanocyl Carbon, Belgium. The 635 thin epoxy resin at a viscosity of 6 Poise and the hardener (4-Aminophenylsulphone) were obtained from US Composites. The ratio between the epoxy resin and hardener was 3:1; these properties were based on the manufacturer descriptions.

2.2.   Nanocomposite manufacturing process

The composition in weight percentage (wt%) used was 75/5/20 synthetic graphite (SG), multiwalled carbon nanotubes (MWCNTs), and epoxy, respectively. There are three stages in the manufacturing process of the MWCNT/SG/epoxy nanocomposites. (1) SG and MWCNTs were mixed using a stainless steel ball mill (ball diameter, 10 mm) at 200 rpm for one hour. The ball to powder (graphite and MWCNTs) ratio was 4:1. (2) Hardener and epoxy resin were mixed using a mixer (model RM 20-KIKA-WERK) for 40 seconds at 1200 rpm. An epoxy resin mixture with a ratio of 3:1 was used as suggested by the manufacturer. (3) The filler and epoxy resin were mixed using a Haake Rheomix internal mixer at 20 rpm for 15 minutes at 35 ℃. Next, the mixture of MWCNTs/SG/epoxy was poured into steel moulds at various moulding parameters: moulding temperature (A) = 110, 130, and 150 ℃, moulding pressure (B) = 1200, 1500, and 1800 psi, and moulding time (C) = 60, 75, and 90 minutes.

2.3.   Characterization

The electrical conductivity of the nanocomposites was tested by a Jandel four-point probe with a RM3 test unit, and the fracture surface was measured using an FESEM (field emission scanning electron microscope), Model Supra 55/55VP. The FESEM sample was fractured from a sample with dimensions of 100 × 12.7 × 2.5 mm³. The bottom of the fault was cut to 4 mm (thickness) to observe the scattering of the conductive fillers in the matrix. The voltage and magnifications used for observation were 3 kV and 200 to 10, 000 times, respectively.

2.4.   Selection of control factors

The compression moulding parameters varied in this study were the moulding time, moulding temperature, and moulding pressure. Table 1 shows the levels and control factors of these moulding parameters. Each control factor consisted of three levels, moulding temperature (A) = 110, 130, and 150 ℃, moulding pressure (B) = 1200, 1500, and 1800 psi, and moulding time (C) = 60, 75, and 90 minutes. Each moulding parameter used was first tested to ensure that the specimens produced using these parameters were well formed. For example, the shortest moulding time selected was 60 minutes because the 45 minutes moulding time used in the preliminary experiments did not produce CPC material well. Therefore, 60 minutes was selected as the lowest moulding time.

The influence of each compression moulding parameter on the electrical conductivity of the nanocomposites was estimated. Three different temperatures of 110, 130 and 150 ℃ were used to obtain the optimum moulding temperature for the electrical conductivity. The moulding pressure was limited by the viscosity of the mixture of resin and conductive filler at 1800 psi. Therefore, the compression moulding pressures applied were 1200, 1500, and 1800 psi to obtain the optimum combination. Compression moulding pressures exceeding 1800 psi caused the mixture of resin and conductive filler to overflow from the mould. Three different compression moulding times (60, 75 and 90 minutes) were used to obtain the optimal moulding time to produce nanocomposites. The levels of the parameters were set to obtain the optimum electrical conductivity, customized with the Taguchi orthogonal array (OA) L9 (3³) design. Analysis of variance (ANOVA) was conducted for the electrical conductivity for each compression moulding parameter in each replicate experiment.

2.5.   Nanocomposite orthogonal array (OA) L9 (3³)

The optimization of the compression moulding parameters was conducted by producing nine nanocomposite plates with dimensions of 100 mm × 100 mm × 2.5 mm. The number of nanocomposite plates produced was based on the factors and levels of the compression moulding parameters (Table 2). The electrical conductivity was measured at three different points: the middle, far right, and left side of each sample.

The OA with L9 (3³) with nine experiment runs was used to obtain optimum compression moulding parameters. Although this number of experiments is the minimum number of runs for an OA with three control factors and three levels, the minimum number of runs successfully produced the optimum combination parameters for this study ^[22,25,26].

2.6.   Analysis of Variance (ANOVA)

ANOVA is a statistical technique for comparing datasets, or it can be used as an exploratory tool to explain observations. In this study, the analysis of variance was used to determine whether the compression moulding parameters significantly affect the electrical conductivity and to obtain the percentage contribution of each parameter through certain steps (Eq 1 to Eq 8) ^[27,28] as follows:

1. The electrical conductivity data from the experimental results are separated by the influences of the control factors and levels, namely, the moulding temperature (A1, A2, and A3), moulding pressure (B1, B2, and B3), and moulding time (C1, C2, and C3).

2. The sum of squares for each factor control (S_n(i)), total sum of squares(SS_T), and sum of squares error (SS_E) is calculated using the following formula:

where X is the experimental data, N is the total number of experiments, and i is the control factors (A, B, and C) at levels of j (level 1, 2, and 3).

3. The variance (V_n) of each control factor is obtained from S_n divided by DoF_n:

The variance error (V_e) is obtained from the results of the SS error (SS_e) divided by the DoF errors (DoF_e) according to the following formula:

The number of DoF (degrees of freedom) of the control factors is the number of data points in the collection minus one. The total number of DoF is obtained from the total of the experiments minus one, while the DoF error is the total number of DoF minus the number of DoF of the control factors of A, B, and C.

4. The variance ratio (F_n) test examines the combined effect of the control factors and is obtained by dividing the variance of each control factor by the variance error:

5. The critical value (F) is obtained from the distribution of F (α, d₁, and d₂), where α is the level of significance, d₁ is the numerator (number of degrees of freedom of the control factors), and d₂ is the denominator (number of degrees of freedom of the error). This study uses a significance level of 99.5%, so α is 0.005. The critical value (F) is necessary to determine whether the control factors significantly influence the electrical conductivity produced.

6. P_n is the contribution percentage of each control factor and is calculated using the formula:

Because the total contribution percentage (P_t) is 100, the error contribution percentage (P_e) is the total contribution percentage minus the contribution percentages of all the control factors.

2.7.   Signal to noise ratio (SNR) analysis

The SNR calculates how the response varies relative to the nominal value or target in various noise conditions. In this study, the SNR for the electrical conductivity was maximized; therefore, Eqs 9 and 10 are used ^[27] as follows:

The MSD is the squared deviation of the average value, as in the following equation:

where n is the number of experimental replications, carried out for each control factor combination, while y_i is the electrical conductivity of the nanocomposite for test repetition.

3.   Results and discussion

3.1.   Electrical conductivity of nanocomposites

The electrical conductivity and SNR of each experiment are shown in Table 3. The electrical conductivity of the MWCNT/SG/epoxy nanocomposites is the major focus of this study. The Taguchi method with orthogonal arrays (OA) of L9 (3³) was used. The significant level, influence of each factor, and bilateral reliability was determined using analysis of variance (ANOVA).

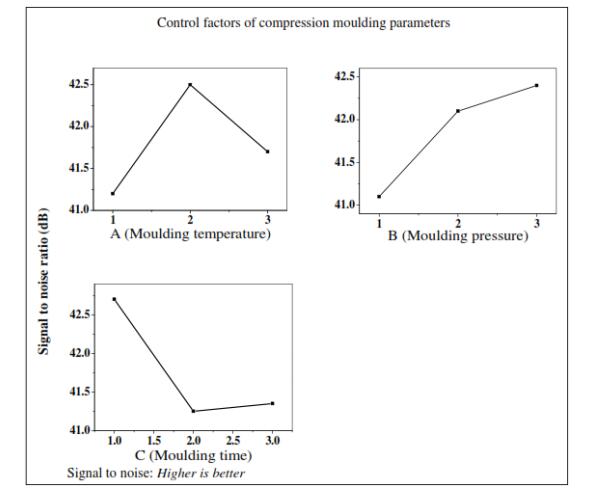
The average electrical conductivity and signal to noise ratio (SNR) for the different compression moulding parameters are shown in Figures 1 and 2.

Increasing the moulding temperature from 110 to 130 ℃ improves the electrical conductivity of the MWCNT/SG/epoxy nanocomposites produced. The epoxy resin viscosity decreases as the moulding temperature increases, facilitating the formation of an electrical conductivity network between the flake shaped primary conductive filler (SG) and the micro sized and tubular elongated shaped (nano sized) secondary conductive filler (MWCNTs) ^[12,29]. Increasing the moulding pressure from 1200 to 1500 to 1800 psi effectively increases the electrical conductivity of nanocomposites. This effect is due to the decrease in the number of voids produced during the manufacturing process with increasing moulding pressure. The electrical conductivity increases as the number of voids decreases ^[30]. Figures 1 and 2 show that the optimum combination of compression moulding parameters for the electrical conductivity based on the highest SNR is the moulding temperature (A2), moulding pressure (B3) and moulding time (C1).

3.2.   Analysis of variance (ANOVA) of the nanocomposite electrical conductivity

The ANOVA of the nanocomposite electrical conductivity is shown in Table 4. The table shows that the variance ratio (F_n) is higher than the critical value (F) at the highest significant level of α = 0.005 (99.5% confidence level) for the moulding temperature (A), moulding pressure (B), and moulding time (C). This result proves that the predetermined compression moulding parameters significantly affect the electrical conductivity of the nanocomposites produced.

The moulding time (C) provides the highest percentage contribution to the electrical conductivity at 42.24%, followed by the moulding temperature (A) at 25.49%. The moulding pressure (B) contributes a percentage of 20.23% to the electrical conductivity. Bin et al. ^[31] and Hui et al. ^[32] reported that the moulding temperature, moulding time, and moulding pressure effectively affect the electrical conductivity of the composite produced.

The highest percentage contribution (P_n) of the moulding time to the electrical conductivity of the nanocomposites is 42.24% due to the decrease in the contact resistance between the conductive filler particles during the compression moulding process ^[31]. The ANOVA error, at 12.4%, shows that almost all the significant factors were covered in the Taguchi approach. This result has a much smaller error than that reported by Zakaria et al. ^[33] for a CB/G/EP composite of 80/20, namely, 44%.

3.3.   Nanocomposite verification tests

The optimum electrical conductivity for the nanocomposites was obtained through the forecast equation ($\sigma_{\text {forecast }}$) and verification testing (experimental study). The optimum parameters were obtained from the average SNR, namely, parameters A2, B3, and C1. The forecast equation was used to predict the SNR and electrical conductivity with the optimum compression moulding parameters, using Eq 11 ^[22].

$\mathcal{J}$ = the average SNR of the electrical conductivity from the experiments. The SNR is maximized (Figure 2) with A2 (moulding temperature, 130 ℃), B3 (moulding pressure, 1800 psi), and C1 (moulding time, 60 minutes).The average predictive values for the electrical conductivity and SNR of the nanocomposites using Eq 11 are 155 S/cm and 43.73 dB, respectively. Then, the optimum combination obtained from the average of the compression moulding parameters and SNR was confirmed through experiments. The experiments were carried out by preparing samples with the optimum combination of compression moulding parameters, that is, 130 ℃ moulding temperature, 1800 psi moulding pressure, and 60 minutes moulding time.

The summary of the results of the initial combination of parameters, optimum combination of parameters and $\sigma_{\text {forecast }}$ are shown in Table 5. The average value of the electrical conductivity and SNR for the initial combination parameters of A1, B1, and C1 are 120 S/cm and 41.55 dB, respectively. Meanwhile, the electrical conductivity and SNR for the optimum combination of factors and levels of A2, B3, and C1 are 163 S/cm and 44.26 dB, respectively. Compared with the initial combination, the confirmation test of the optimum combination shows an increase in the electrical conductivity of the nanocomposites as high as 36.21%. This result occurs because the optimum combination of compression moulding parameters is capable of producing a more even dispersion of the conductive filler material in the polymer matrix. Therefore, the optimum combination of parameters for the nanocomposites produces electrical conductivity higher than that of the initial combination of parameters. Thus, the higher average SNR for each optimum compression moulding parameter effectively increases the electrical conductivity of the nanocomposites. The differences between the average electrical conductivity and SNR of the optimum combination and those of the optimum $\sigma_{\text {forecast }}$ are only 5.37% and 1.21%, respectively, which shows that the prediction equation appropriately predicted the electrical conductivity and SNR of the optimum nanocomposites.

3.4.   Nanocomposite microstructures

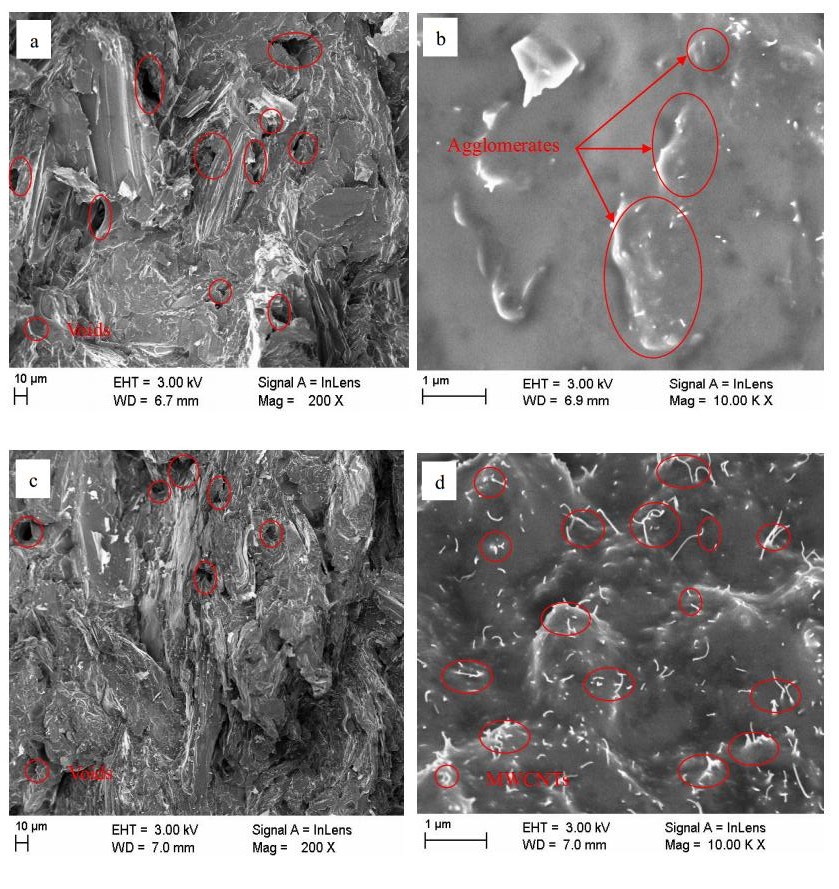
The primary conductive filler (synthetic graphite) and secondary conductive filler (MWCNT) dispersions in the epoxy matrix were observed by a scanning electron microscope (SEM). Figure 3 shows the microstructure of the MWCNT/SG/epoxy nanocomposites with optimized compression moulding parameters. The microstructure contains a conductive filler dispersion within a polymer matrix. The MWCNTs have tubular geometry and are a brighter colour (white) than the synthetic graphite particles (SG), which have flake geometries.

The microstructure of both optimization processes showed voids between the SG as the primary conductive filler material (Figure 3a, c) in the initial fracture surface testing. These voids reduce the electrical conductivity of the nanocomposite generated. The number of voids decreased in the confirmation test (Figure 3c) due to the MWCNTs, which exhibited an elongated tube geometry, effectively filling the voids and conducting electricity between the larger SG particles. The increasing contact between the conductive filler particles increased the electrical conductivity of the nanocomposites produced.

Agglomeration occurred in the initial testing of the epoxy matrix for both optimization compression moulding parameters that used MWCNTs as a secondary filler, as shown in Figure 3b. The agglomeration increased the distance between the conductive filler materials, decreasing the electrical conductivity of the MWCNT/SG/epoxy nanocomposites produced. The electrical conductivity network formation occurred not only by direct contact between the conductive filler particles but also by distances of a few micrometres between particles, thus enabling electrons to jump across the gaps between the conductive filler materials ^[28]. The optimization of the compression moulding parameters effectively reduced the agglomeration of the MWCNTs within the polymer matrix, so that the MWCNTs could be dispersed more evenly in the epoxy. An even dispersion of the conductive filler in the matrix produces a higher electrical conductivity in MWCNT/SG/epoxy nanocomposites compared with an uneven dispersion of the conductive filler material ^[12,34,35]. This situation was observed in the confirmation tests (Figure 3c, d).

4.   Conclusions

The compression moulding parameters of MWCNT/SG/epoxy nanocomposites were investigated with respect to electrical conductivity. Based on the results, certain conclusions can be drawn:

1. The moulding temperature (A), moulding pressure (B), and moulding time (C) were selected as control factors using the Taguchi method. Experimental trials based on an L9 (3³) orthogonal array were carried out.

2. The analysis of variance (ANOVA) results show that all the control factors significantly influence the electrical conductivity produced. The moulding time (C) provides the highest percentage contribution to the resulting electrical conductivity at 42.24%, the moulding temperature (A) at 25.49% and the moulding pressure (B) at 20.23%.

3. The electrical conductivity of the initial combination at factors and levels of A1, B1, C1 is 120 S/cm. The optimum combination at factors and levels of A2, B3, C1 is 163 S/cm.

4. The optimum electrical conductivity of the MWCNT/SG/epoxy nanocomposites is 163 S/cm. This value meets the U.S. Department of Energy requirements for bipolar plate applications.

Acknowledgements

The authors gratefully acknowledge the financial assistance of the Directorate General of Higher Education for this research, with contract number: SP DIPA-042.06.1.401516/2019, 5 December 2018.

Conflicts of interest

The authors have no conflicts of interest.