What is the coverage of your health insurance plan? An audit of hospital billing

Aswin Sugunan; Rajasekharan Pillai K; Anice George; Aswin Sugunan; Rajasekharan Pillai K; Anice George

doi:10.3934/publichealth.2024052

AIMS Public Health

2024, Volume 11, Issue 4: 1013-1029. doi: 10.3934/publichealth.2024052

Previous Article Next Article

Research article

What is the coverage of your health insurance plan? An audit of hospital billing

1.
School of Management, The Apollo University, Chittoor, India
2.
Manipal Academy of Higher Education, Manipal, India
3.
Welcomgroup Graduate School of Hotel Administration, Manipal Academy of Higher Education, Manipal, India
4.
Manipal College of Nursing, Manipal Academy of Higher Education, Manipal, India

Received: 20 December 2023 Revised: 19 April 2024 Accepted: 26 April 2024 Published: 24 September 2024

The provocative advice of health policymakers in endorsing private health insurance, as a critical tool for health reforms, is well-reckoned as a deterrent to mounting healthcare expenditure in the wake of the public health insurance quagmire. However, scholarly evidence has condemned the ineffectiveness of private health insurance in containing out-of-pocket expenditure. In this backdrop, we carried out a nuanced investigation of the coverage pattern of private health insurance policies. We examined the one-year billing information of private health insurance holders hospitalized in a multi-specialty teaching hospital. We found that private health insurance fails to provide full coverage, leading to underinsurance though minimal financial protection was extended. Moreover, reimbursement patterns under various cost heads are also discussed. We conclude by emphasizing the need for future research to fill the knowledge gap. We claim methodological novelty in its approach to data collection.

Keywords:

Citation: Aswin Sugunan, Rajasekharan Pillai K, Anice George. What is the coverage of your health insurance plan? An audit of hospital billing[J]. AIMS Public Health, 2024, 11(4): 1013-1029. doi: 10.3934/publichealth.2024052

Related Papers:

[1]	Deniz Sevinç . Volatility spillovers among MIST stock markets. Data Science in Finance and Economics, 2022, 2(2): 80-95. doi: 10.3934/DSFE.2022004
[2]	Nurun Najwa Bahari, Hafizah Bahaludin, Munira Ismail, Fatimah Abdul Razak . Network, correlation, and community structure of the financial sector of Bursa Malaysia before, during, and after COVID-19. Data Science in Finance and Economics, 2024, 4(3): 362-387. doi: 10.3934/DSFE.2024016
[3]	Moses Khumalo, Hopolang Mashele, Modisane Seitshiro . Quantification of the stock market value at risk by using FIAPARCH, HYGARCH and FIGARCH models. Data Science in Finance and Economics, 2023, 3(4): 380-400. doi: 10.3934/DSFE.2023022
[4]	Jiamin Cheng, Yuanying Jiang . How can carbon markets drive the development of renewable energy sector? Empirical evidence from China. Data Science in Finance and Economics, 2024, 4(2): 249-269. doi: 10.3934/DSFE.2024010
[5]	Kuo-Shing Chen . Interlinkages between Bitcoin, green financial assets, oil, and emerging stock markets. Data Science in Finance and Economics, 2024, 4(1): 160-187. doi: 10.3934/DSFE.2024006
[6]	Dachen Sheng, Opale Guyot . Market power, internal and external monitoring, and firm distress in the Chinese market. Data Science in Finance and Economics, 2024, 4(2): 285-308. doi: 10.3934/DSFE.2024012
[7]	Zimei Huang, Zhenghui Li . What reflects investor sentiment? Empirical evidence from China. Data Science in Finance and Economics, 2021, 1(3): 235-252. doi: 10.3934/DSFE.2021013
[8]	Katleho Makatjane, Ntebogang Moroke . Examining stylized facts and trends of FTSE/JSE TOP40: a parametric and Non-Parametric approach. Data Science in Finance and Economics, 2022, 2(3): 294-320. doi: 10.3934/DSFE.2022015
[9]	Samuel Asante Gyamerah, Collins Abaitey . Modelling and forecasting the volatility of bitcoin futures: the role of distributional assumption in GARCH models. Data Science in Finance and Economics, 2022, 2(3): 321-334. doi: 10.3934/DSFE.2022016
[10]	Nitesha Dwarika . The risk-return relationship in South Africa: tail optimization of the GARCH-M approach. Data Science in Finance and Economics, 2022, 2(4): 391-415. doi: 10.3934/DSFE.2022020

Abstract

1. Introduction

When searching the literature, we noticed that several propositions have been made by several researchers to find kernels that can be used to obtain fractional differential operators. The main reason for this is that, real world problems show signs of processes resembling behaviors of some mathematical functions. Riemann, Liouville, Cauchy and Abel works have led to a fractional calculus with a power law kernel. Their work was later modified by Caputo; this version has been used in many fields of science because of its ability to allow classical initial conditions ^[1]. Prabhakar suggested a different kernel as a product of power-law and the generalized Mittag-Leffler function with three parameters. This version has also attracted the attention of many researchers, studies have been done on theory as well as on applications. Indeed, the two kernels have their specific values, for example, power-law helps only to replicate processes exhibiting power-law behavior, while the product of the power-law and the generalized three-parameters Mittag-Leffler has also its field of application ^[2]. As nature is complex, a new kernel was suggested by Caputo and Fabrizio, a special exponential kernel with Delta Dirac properties. A differential operator that is in fashion nowadays due to its ability to replicate processes following fading memory. Indeed, this kernel brought a new shift into fractional calculus, as the concept of the fractional derivative with a non-singular kernel was introduced ^[3]. A point made by some researcher about the non-fractionality of the kernel led to a new kernel, the generalized Mittag-Leffler function with one parameter. This version was suggested by Atangana and Baleanu, constituting another new step forward in the field of fractional calculus. The operators have been used in several fields of studies with great success ^[4]. In ^[5], the basic theory of fractional differentiation, existence-uniqueness theorems and analytical numerical methods of solution of fractional differential equations are presented. In ^[6], the authors examined Noether's theorems of fractional generalized Birkhoffian systems in terms of classical and combined Caputo derivatives. The development of the time-fractional damage model for hyperelastic body is considered in ^[7]. While looking at nature and its complexities, one can with no doubt conclude that these suggested kernels are not enough to predict all complex behaviors of our universe. On this note, one will proceed to search for a different kernel or modified kernel, or a class of functions that will be used to introduce new differential operators. Sabatier recently presented some variants of kernels that will also open new doors for investigation ^[8]. In addition to these outstanding contributions, several other ideas have been suggested, for example the concept of short memory was suggested and a fractional derivative in the Caputo sense has been defined for different values of fractional orders. The idea is to have a different type of variable-order derivative unlike the well-known version that considers a fractional orders. The idea is initiated to have a different type of variable order derivative unlike the well-known version that considers a fractional-order to be a function of time. This case was suggested by Wu et al. ^[9] and applied in chaos. On the other hand, researchers have noticed that several real-world problems exhibit processes with different behaviors as a function of time and space. A particular case is a passage from deterministic to stochastic, or from power law to exponential decay. It was noted that existing differential operators may not be able to account for these behaviors, thus piecewise differential and integral operators were introduced to deal with problems exhibiting crossover behaviors ^[10]. The main aim of this note is to provide a critical analysis of the possible applications, advantages, and disadvantages of these two concepts.

2. Motivation for piecewise derivatives

We will illustrate the motivation with some examples.

Death body decay in different temperatures. Consider a corpse found in a snowy place, and assume that such body has been found after 20 days. The corpse is taken and brought to the house and kept in a normal temperature for a few days and later put in a mortuary and buried. The main aim here is to replicate the process of decay. The first part will provide a very low decay. The second part will provide a fast decay, the third part will again be a fast process and finally, a slow process. Indeed, slow and fast processes can be characterized by some mathematical functions. The power-law function is

$\begin{equation} t^{-\alpha }. \end{equation}$

(2.1)

The exponential decay function with a Dirac delta property is

$\begin{equation} \frac{1}{1-\alpha }\exp \left[ -\frac{\alpha }{1-\alpha }t\right] . \end{equation}$

(2.2)

However, a crossover from fast to slow decay processes can be modeled using the following Mittag-Leffler function with Delta-Dirac property

$\begin{equation} \frac{1}{1-\alpha }E_{\alpha }\left[ -\frac{\alpha }{1-\alpha }t^{\alpha } \right] . \end{equation}$

(2.3)

The process can be divided into several intervals to capture each behavior. In the first part, one can have

$\begin{equation} _{0}^{C}D_{t}^{\alpha }y\left( t\right) = -\lambda _{1}y\left( t\right) \;{\rm{ if }}\;0 < t\leq T_{1}. \end{equation}$

(2.4)

The second processes can be

$\begin{equation} _{T_{1}}^{CF}D_{t}^{\alpha }y\left( t\right) = -\lambda _{2}y\left( t\right) \;{\rm{ if }}\;T_{1} < t\leq T_{2}. \end{equation}$

(2.5)

The two last parts will be characterized by

$\begin{equation} _{T_{2}}^{ABC}D_{t}^{\alpha }y\left( t\right) = -\lambda _{3}y\left( t\right) \; {\rm{ if }}\;T_{2} < t\leq T. \end{equation}$

(2.6)

Thus the whole process will be a system with the following crossover behaviors

$\begin{equation} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha }y\left( t\right) = -\lambda _{1}y\left( t\right) \;{\rm{ if }}\;0 < t\leq T_{1}, \\ _{T_{1}}^{CF}D_{t}^{\alpha }y\left( t\right) = -\lambda _{2}y\left( t\right) \;{\rm{ if }}\;T_{1} < t\leq T_{2}, \\ _{T_{2}}^{ABC}D_{t}^{\alpha }y\left( t\right) = -\lambda _{3}y\left( t\right) \;{\rm{ if }}\;T_{2} < t\leq T. \end{array} \right. \end{equation}$

(2.7)

Therefore in general the concept of piecewise derivative has been introduced.

3. Short memory concept

In this section, we present the definitions of both short memory and piecewise differentiation. For the short memory case, the idea has already been discussed; for example a paper published by Deng in 2007 has already discussed the short memory principle ^[11], which has also been called the fixed memory principle and logarithmic memory principle. Wu et al. ^[9] presented the concept of a fractional variable-order derivative where the fractional order changes within an interval. The suggested definition is given below as:

$\begin{equation} \left\{ \begin{array}{c} _{t_{0}}^{C}D_{t}^{\alpha _{0}}y\left( t\right)=f\left( y,t\right) \text{ for }t\in \left[ t_{0},t_{1}\right] , \\ _{t_{1}}^{C}D_{t}^{\alpha _{1}}y\left( t\right)=f\left( y,t\right) \text{ for }t\in \left[ t_{1},t_{2}\right] , \\ _{t_{2}}^{C}D_{t}^{\alpha _{2}}y\left( t\right)=f\left( y,t\right) \text{ for }t\in \left[ t_{2},t_{3}\right] . \end{array} \right. \end{equation}$

(3.1)

Here Caputo power law derivative is used, which is known to have a singularity at the origin for a class of functions. The author did not give explicitly space of the functions as the derivative was defined in general, therefore, if we assume a class of the functions for which $\frac{dy}{dt}$ is continuous then at each $t=t_{i},$ $f\left( y,t\right)$ should be zero.

On the other hand, the piecewise concept is defined as follows.

$1)$ A piecewise derivative of $f$ with classical, power-law and stochastic processes is given by

$\begin{equation} \left\{ \begin{array}{c} Dy\left( t\right) = f\left( t,y\left( t\right) \right) \;{\rm{ if }}\;0 < t \leq t_{1}, \\ _{t_{1}}^{C}D_{t}^{\alpha }y\left( t\right) = f\left( t,y\left( t\right) \right) \;{\rm{ if }}\;t_{1} < t\leq t_{2}, \\ dy\left( t\right) = f\left( t,y\left( t\right) \right) dt+\sigma y\left( t\right) dB\left( t\right)\; {\rm{ if }}\;t_{2} < t \leq t_{3}. \end{array} \right. \end{equation}$

(3.2)

$2)$ A piecewise derivative of $f$ with power, exponential and Mittag-Leffler laws is given as

$\begin{equation} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha }y\left( t\right) = f\left( t,y\left( t\right) \right) \;{\rm{ if }}\;0 < t \leq t_{1}, \\ _{t_{1}}^{CF}D_{t}^{\alpha }y\left( t\right) = f\left( t,y\left( t\right) \right) \;{\rm{ if }}\;t_{1} < t \leq t_{2}, \\ _{t_{1}}^{ABC}D_{t}^{\alpha }y\left( t\right) = f\left( t,y\left( t\right) \right) \;{\rm{ if }}\;t_{2} < t \leq t_{3}. \end{array} \right. \end{equation}$

(3.3)

Several definitions can be found in ^[10] $.$

In the next section, we will provide a deep analysis of these concepts.The aim of the section is to present advantages and disadvantages of these two independent concepts. We note that

$\begin{eqnarray} _{0}^{C}D_{t}^{\alpha }{\rm{ }}f\left( t\right) & = &\frac{1}{\Gamma \left( 1-\alpha \right) }\int\limits_{0}^{t}\frac{d}{d\tau }f\left( \tau \right) \left( t-\tau \right) ^{-\alpha }d\tau ,{\rm{ \ }} \end{eqnarray}$

(3.4)

$\begin{eqnarray} _{0}^{CF}D_{t}^{\alpha }f\left( t\right) & = &\frac{1}{1-\alpha } \int\limits_{0}^{t}\frac{d}{d\tau }f\left( \tau \right) \exp \left[ -\frac{ \alpha }{1-\alpha }\left( t-\tau \right) \right] d\tau , \end{eqnarray}$

(3.5)

$\begin{eqnarray} _{0}^{ABC}D_{t}^{\alpha }f\left( t\right) & = &\frac{1}{1-\alpha } \int\limits_{0}^{t}\frac{d}{d\tau }f\left( \tau \right) E_{\alpha }\left[ - \frac{\alpha }{1-\alpha }\left( t-\tau \right) ^{\alpha }\right] d\tau . \end{eqnarray}$

(3.6)

4. Analysis and difference

Here, we present a deep analysis that will help the readers to see the difference between the two concepts. Let us start with short memory. A deep look inside the short memory concept defined by gives the following:

$1)$ The short memory principle considers a change in constant variable order; however, it uses a single kernel. Indeed, this can be viewed as variable order where the order is changed within shorter intervals. Nonetheless, it describes some process and in the case of Caputo derivation, this process will only describe a power-law process that is, this process will not have crossover behavior.

$2)$ The concept is unable to capture classical processes because the fractional order should change in each interval.

On a serious note, however, if we have $f\left(t, y\left(t\right) \right) \neq 0, \forall t\in \left[ 0, T\right].$ So at each $t_{j}$

$\begin{equation} \lim\limits_{t\rightarrow t_{j}}{\rm{ }}_{t_{j}}^{C}D_{t}^{\alpha }y\left( t\right) = f\left( t,y\left( t\right) \right) \mid _{t = t_{j}}. \end{equation}$

(4.1)

Since $\frac{dy}{dt}$ is continuous,

$\begin{equation} \lim\limits_{t\rightarrow t_{j}}{\rm{ }}_{t_{j}}^{C}D_{t}^{\alpha }y\left( t\right) = f\left( t_{j},y\left( t_{j}\right) \right) \neq 0. \end{equation}$

(4.2)

However the right hand side produces $f\left( t,y\left( t\right) \right) \neq 0$ , which is a contradiction. It infers that $f\left( t_{j},y\left( t_{j}\right) \right) =0.$ This may be used to explain the process with a renewal force that follows the power-law process. For example, the trajectory of a flea with a constant jump. There are fewest real world problems that present these behaviors. However, due to the power-law singularity the definition suggested by Wu may have some problems at the boundaries. Nonetheless, as a result of using non-singular kernels, the concept of short memory will be well-posed since the conditions at the boundaries will be well controlled and the renewal processes would be well-posed

On the other hand, however, the concept of piecewise was introduced for different purposes. The following example will give light to the situation. We consider evaluating the velocity of water within a geological formation with fractures. We record the velocity as a function of time. The velocity will obviously be low in the rock's matrix; however as the water reaches the fracture, there will be a crossover behavior because the velocity will suddenly increase. The first part of this process follows behaviors resembling a declining process and, later, an almost constant high velocity. Now if one was to withdraw water from such a geological formation and record the water level, one will observe a fading groundwater level in earlier times; then when water is being taken from the fracture, we observe a steady groundwater level. Thus, there is a crossover behavior from the fading process to long range, which can be captured using an exponential function, then the power law. Therefore, the differential operator able to replicate this process is

$\begin{equation} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha }h\left( r,t\right) = f\left( t,r,h\left( r,t\right) \right) \;{\rm{ if }}\;0 < t\leq T_{1}, \\ _{T_{1}}^{CF}D_{t}^{\alpha }h\left( r,t\right) = f_{1}\left( t,r,h\left( r,t\right) \right) \;{\rm{ if }}\;T_{1} < t\leq T. \end{array} \right. \end{equation}$

(4.3)

Therefore, one can see a clear difference and objectives of both concepts. Piecewise differential operators are thus conceived to capture processes exhibiting different patterns, or crossover behaviors. Here the order does not change within an interval as changing order does not change the process but only the memory with the same pattern, rather, the kernels change to bring into the mathematical formulation the crossover behaviors by each kernel.

For example, using the piecewise derivative the decay equation

$\begin{equation} \left\{ \begin{array}{c} y\prime \left( t\right) = -\lambda y\left( t\right) \;{\rm{ if }}\;0 < t\leq T_{1}, \\ _{T_{1}}^{C}D_{t}^{\alpha }y\left( t\right) = -\lambda y\left( t\right) \;{\rm{ if }}\;T_{1} < t\leq T_{2}, \\ dy\left( t\right) = -\lambda y\left( t\right) dt+\sigma y\left( t\right) dB\left( t\right) \;{\rm{ if }}\;T_{2} < t \leq T \end{array} \right. \end{equation}$

(4.4)

which will lead to decay with crossover behaviors. From $0 < t < T_{1}$ , we shall observe a normal decay process. From $T_{1} < t < T_{2}$ , we shall observe power-law decay or the Mittag-Leffler decay. From $T_{2} < t < T$ , we shall observe decay with randomness. On the other hand, with the short memory

$\begin{equation} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha _{1}}y\left( t\right) = -\lambda y\left( t\right) \;{\rm{ if }}\;0 < t \leq T_{1}, \\ _{T_{1}}^{C}D_{t}^{\alpha _{2}}y\left( t\right) = -\lambda y\left( t\right) \; {\rm{ if }}\;T_{1} < t \leq T_{2}, \\ _{T_{2}}^{C}D_{t}^{\alpha _{3}}y\left( t\right) = -\lambda y\left( t\right) \;{\rm{ if }}\;T_{2} < t \leq T \end{array} \right. \end{equation}$

(4.5)

which leads only to a power-law process or the Mittag-Leffler process.

5. Application to real world problems

In this section, we shall present clearly the difference between the two concepts by applying them to some problems. We shall start with a simple problem about the decay problem with two intervals.

Example 1. We consider a simple decay model within $\left[ 0, T_{1} \right]$ and $\left[ T_{1}, T_{2}\right].$ In the case of short memory, we have

$\begin{equation} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha _{1}}y\left( t\right) = -\lambda y\left( t\right) \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ _{T_{1}}^{C}D_{t}^{\alpha _{2}}y\left( t\right) = -\lambda y\left( t\right) \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right]. \end{array} \right. \end{equation}$

(5.1)

In the case of piecewise, we can consider the following

$\begin{equation} \left\{ \begin{array}{c} \frac{dy\left( t\right) }{dt} = -\lambda y\left( t\right) \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ _{T_{1}}^{C}D_{t}^{\alpha }y\left( t\right) = -\lambda y\left( t\right) \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right] \end{array} \right. \end{equation}$

(5.2)

$\begin{equation} \left\{ \begin{array}{c} _{0}^{ABC}D_{t}^{\alpha }y\left( t\right) = -\lambda y\left( t\right) \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ \frac{dy\left( t\right) }{dt} = -\lambda y\left( t\right) \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right] \end{array} \right. \end{equation}$

(5.3)

$\begin{equation} \left\{ \begin{array}{c} _{0}^{CF}D_{t}^{\alpha }y\left( t\right) = -\lambda y\left( t\right) \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ _{T_{1}}^{C}D_{t}^{\alpha }y\left( t\right) = -\lambda y\left( t\right) \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right]. \end{array} \right. \end{equation}$

(5.4)

Using the Laplace transform on the above system yields in the case of short memory

$\begin{equation} \left\{ \begin{array}{c} y\left( t\right) = y\left( 0\right) E_{\alpha _{1}}\left[ -\lambda t^{\alpha _{1}}\right] \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ y\left( t\right) = y\left( T_{1}\right) E_{\alpha _{2}}\left[ -\lambda \left( t-T_{1}\right) ^{\alpha _{2}}\right] \;{\rm{ if }}\;t\in \left[ T_{1},T_{2} \right]. \end{array} \right. \end{equation}$

(5.5)

In the case of piecewise, we have the following solution

$\begin{equation} \left\{ \begin{array}{c} y\left( t\right) = y\left( 0\right) \exp \left[ -\lambda t\right] \;{\rm{ if }}\; t\in \left[ 0,T_{1}\right] , \\ y\left( t\right) = y\left( T_{1}\right) E_{\alpha }\left[ -\lambda \left( t-T_{1}\right) ^{\alpha }\right] \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right] \end{array} \right. \end{equation}$

(5.6)

$\begin{equation} \left\{ \begin{array}{c} y\left( t\right) = y\left( 0\right) \;{\rm{ if }}\;t = 0, \\ y\left( t\right) = \frac{y\left( 0\right) }{1+\lambda \left( 1-\alpha \right) }E_{\alpha }\left[ -\frac{\lambda \alpha }{1+\lambda \left( 1-\alpha \right) }t^{\alpha }\right] b\;{\rm{ if }}\;t\in \left] 0,T_{1}\right] , \\ y\left( t\right) = y\left( T_{1}\right) \exp \left[ -\lambda \left( t-T_{1}\right) \right] \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right] \end{array} \right. \end{equation}$

(5.7)

$\begin{equation} \left\{ \begin{array}{c} y\left( t\right) = y\left( 0\right) \;{\rm{ if }}\;t = 0, \\ y\left( t\right) = \frac{y\left( 0\right) }{1+\lambda \left( 1-\alpha \right) }\exp \left[ -\frac{\lambda \alpha }{1+\lambda \left( 1-\alpha \right) } t^{\alpha }\right] b\;{\rm{ if }}\;t\in \left] 0,T_{1}\right] , \\ y\left( t\right) = y\left( T_{1}\right) E_{\alpha }\left[ -\lambda \left( t-T_{1}\right) ^{\alpha }\right] \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right]. \end{array} \right. \end{equation}$

(5.8)

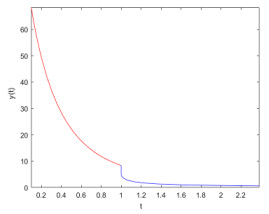
$T_{1} = 1$ where the crossover occurs and $T_{2} = 2.4$ is the final time. The numerical simulations are presented in Figures 1–4 below.

Figure 1. Decay model with short memory for

$\lambda = 3, \alpha _{1} = 0.9$ and

$\alpha _{2} = 0.6$ .

DownLoad: Full-Size Img PowerPoint

Figure 2. Decay model with piecewise derivative for

$\lambda = 3$ .

DownLoad: Full-Size Img PowerPoint

Figure 3. Decay model with piecewise derivative for

$\lambda = 3$ .

DownLoad: Full-Size Img PowerPoint

Figure 4. Decay model with piecewise derivative for

$\lambda = 3$ .

DownLoad: Full-Size Img PowerPoint

Example 2. We consider the spread of an infectious disease. We consider a Susceptible, Exposed, Infected and Recovered (SEIR) model. It was proven by Atangana and Seda that such a model may not be able to predict waves ^[12]. Thus to introduce waves, in particular two waves, we consider the spread to be in the periods $\left[ 0, T_{1} \right]$ and $\left[ T_{1}, T_{2}\right].$ In the case of short memory, we have

$\begin{eqnarray} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha }S = \mu N-\mu S-\frac{\beta SI}{N} \\ _{0}^{C}D_{t}^{\alpha }E = \frac{\beta SI}{N}-\left( \mu +a\right) E \\ _{0}^{C}D_{t}^{\alpha }I = aE-\left( \gamma +\mu \right) I \\ _{0}^{C}D_{t}^{\alpha }R = \gamma I-\mu R \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} _{T_{1}}^{C}D_{t}^{\beta }S = \mu N-\mu S-\frac{\beta SI}{N} \\ _{T_{1}}^{C}D_{t}^{\beta }E = \frac{\beta SI}{N}-\left( \mu +a\right) E \\ _{T_{1}}^{C}D_{t}^{\beta }I = aE-\left( \gamma +\mu \right) I \\ _{T_{1}}^{C}D_{t}^{\beta }R = \gamma I-\mu R \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] . \end{eqnarray}$

(5.9)

In the case of piecewise several scenarios can be obtained. So we can have

$\begin{eqnarray} \left\{ \begin{array}{c} \overset{\cdot }{S} = \mu N-\mu S-\frac{\beta SI}{N} \\ \overset{\cdot }{E} = \frac{\beta SI}{N}-\left( \mu +a\right) E \\ \overset{\cdot }{I} = aE-\left( \gamma +\mu \right) I \\ \overset{\cdot }{R} = \gamma I-\mu R \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} dS = \left( \mu N-\mu S-\frac{\beta SI}{N}\right) dt+\sigma _{1}SdB_{1}\left( t\right) \\ dE = \left( \frac{\beta SI}{N}-\left( \mu +a\right) E\right) dt+\sigma _{2}EdB_{2}\left( t\right) \\ dI = \left( aE-\left( \gamma +\mu \right) I\right) dt+\sigma _{3}IdB_{3}\left( t\right) \\ dR = \left( \gamma I-\mu R\right) dt+\sigma _{4}RdB_{4}\left( t\right) \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] \end{eqnarray}$

(5.10)

$\begin{eqnarray} \left\{ \begin{array}{c} dS = \left( \mu N-\mu S-\frac{\beta SI}{N}\right) dt+\sigma _{1}SdB_{1}\left( t\right) \\ dE = \left( \frac{\beta SI}{N}-\left( \mu +a\right) E\right) dt+\sigma _{2}EdB_{2}\left( t\right) \\ dI = \left( aE-\left( \gamma +\mu \right) I\right) dt+\sigma _{3}IdB_{3}\left( t\right) \\ dR = \left( \gamma I-\mu R\right) dt+\sigma _{4}RdB_{4}\left( t\right) \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} _{T_{1}}^{C}D_{t}^{\alpha }S = \mu N-\mu S-\frac{\beta SI}{N} \\ _{T_{1}}^{C}D_{t}^{\alpha }E = \frac{\beta SI}{N}-\left( \mu +a\right) E \\ _{T_{1}}^{C}D_{t}^{\alpha }I = aE-\left( \gamma +\mu \right) I \\ _{T_{1}}^{C}D_{t}^{\alpha }R = \gamma I-\mu R \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] . \end{eqnarray}$

(5.11)

Noting that several more scenarios can be considered, however, we will only consider these two in the case of piecewise. Before proceeding with the analysis of these models, we shall first provide an interpolation of each case in terms of wave behaviors. In the case of short memory, the first and second waves show the non-Gaussian distribution associated to the power-law variables $t^{-\alpha _{1}}$ and $t^{-\alpha _{2}}$ . Therefore, one will observe different long tails for $t^{-\alpha _{1}}$ and $t^{-\alpha _{2}}$ . On the other hand, however, the first considered model shows that the first wave has a normal distribution, while the second wave has random behavior. The second model shows that the first wave has a long tail spread while the second has random behavior.

We now present the numerical solutions of the considered models. We shall consider the numerical scheme suggested by Ghanbari et al. ^[13] for fractional cases. The short memory case can be simplified to

$\begin{equation} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha }y_{i}\left( t\right) = F_{i}\left( t,y_{i}\left( t\right) \right) \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ _{T_{1}}^{C}D_{t}^{\beta }y_{i}\left( t\right) = F_{i}\left( t,y_{i}\left( t\right) \right) \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right]. \end{array} \right. \end{equation}$

(5.12)

Applying the Ghanbari method yields

$\begin{equation} \left\{ \begin{array}{c} y_{i}^{n_{1}} = y_{i}\left( 0\right) +h^{\alpha }\left( \begin{array}{c} \widetilde{a}_{n_{1}}^{\left( \alpha \right) }F_{i}\left( t_{0},y_{i}\left( t_{0}\right) \right) \\ +\sum\limits_{k = 2}^{n_{1}}\widetilde{a}_{n_{1}-k}^{\left( \alpha \right) }F_{i}\left( t_{k},y_{i}\left( t_{k}\right) \right) \end{array} \right) \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ y_{i}^{n_{2}} = y_{i}\left( T_{1}\right) +h^{\beta }\left( \begin{array}{c} \widetilde{a}_{n_{2}}^{\left( \beta \right) }F_{i}\left( T_{1},y_{i}\left( T_{1}\right) \right) \\ +\sum\limits_{j = n_{1}+2}^{n_{2}}a_{n_{2}-j}^{\left( \beta \right) }F_{i}\left( t_{j},y_{i}\left( t_{j}\right) \right) \end{array} \right) \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right] \end{array} \right. \end{equation}$

(5.13)

where

$\begin{eqnarray} \widetilde{a}_{n_{1}}^{\left( \alpha \right) } & = &\frac{\left( n_{1}-1\right) ^{\alpha +1}-n_{1}^{\alpha }\left( n_{1}-\alpha -1\right) }{ \Gamma \left( \alpha +2\right) }, \\ a_{n_{2}}^{\left( \beta \right) } & = &\left\{ \begin{array}{c} \frac{1}{\Gamma \left( \beta +2\right) }\;{\rm{ if }}\;n = 0, \\ \frac{\left( n_{2}-1\right) ^{\beta +1}-2n_{2}^{\beta +1}+\left( n_{2}+1\right) ^{\beta +1}}{\Gamma \left( \beta +2\right) }\;{\rm{ if }}\;n\geq 1. \end{array} \right. \end{eqnarray}$

(5.14)

and

$\begin{eqnarray} \widetilde{a}_{n_{2}}^{\left( \beta \right) } &=&\frac{\left( n_{2}-1\right) ^{\beta +1}-n_{2}^{\beta }\left( n_{2}-\beta -1\right) }{\Gamma \left( \beta +2\right) }, \\ \theta _{k}^{\left( \beta \right) } &=&\frac{\left( k-1\right) ^{\beta +1}-2k^{\beta +1}+\left( k+1\right) ^{\beta +1}}{\Gamma \left( \beta +2\right) }\text{ if }k=n_{1}+1,,..,n_{2}-1. \notag \end{eqnarray}$

(5.15)

In the case of the piecewise first model, we have

$\left\{ \begin{matrix} y_{i}^{{{n}_{1}}}={{y}_{i}}\left( 0 \right)+\sum_{j=2}^{{{n}_{1}}}{\left[ \begin{matrix} \frac{23}{12}{{F}_{i}}\left( {{t}_{j}},{{y}_{i}}\left( {{t}_{j}} \right) \right)\Delta t \\ -\frac{4}{3}{{F}_{i}}\left( {{t}_{j-1}},{{y}_{i}}\left( {{t}_{j-1}} \right) \right)\Delta t \\ +\frac{5}{12}{{F}_{i}}\left( {{t}_{j-2}},{{y}_{i}}\left( {{t}_{j-2}} \right) \right)\Delta t \\ \end{matrix} \right]}\ \text{if}\ t\in \left[ 0,{{T}_{1}} \right], \\ \left\{ \begin{matrix} y_{i}^{{{n}_{2}}}=y\left( {{T}_{1}} \right)+\sum_{j={{n}_{1}}+3}^{{{n}_{2}}}{\left[ \begin{matrix} \frac{23}{12}{{F}_{i}}\left( {{t}_{j}},{{y}_{i}}\left( {{t}_{j}} \right) \right)\Delta t \\ -\frac{4}{3}{{F}_{i}}\left( {{t}_{j-1}},{{y}_{i}}\left( {{t}_{j-1}} \right) \right)\Delta t \\ +\frac{5}{12}{{F}_{i}}\left( {{t}_{j-2}},{{y}_{i}}\left( {{t}_{j-2}} \right) \right)\Delta t \\ \end{matrix} \right]}\ \text{if}\ t\in \left[ {{T}_{1}},{{T}_{2}} \right]. \\ +{{\sigma }_{i}}{{y}_{i}}\left( B_{i}^{{{n}_{2}}+1}-B_{i}^{{{n}_{2}}} \right) \\ \end{matrix} \right. \\ \end{matrix} \right.$

(5.16)

For Case 2, we obtain

$\begin{equation} \left\{ \begin{array}{c} \left\{ \begin{array}{c} y_{i}^{n_{1}} = y_{i}\left( 0\right) +\sum_{j = 2}^{n_{1}}\left[ \begin{array}{c} \frac{23}{12}F_{i}\left( t_{j},y_{i}\left( t_{j}\right) \right) \Delta t \\ -\frac{4}{3}F_{i}\left( t_{j-1},y_{i}\left( t_{j-1}\right) \right) \Delta t \\ +\frac{5}{12}F_{i}\left( t_{j-2},y_{i}\left( t_{j-2}\right) \right) \Delta t \end{array} \right] {\rm{ }} \\ +\sigma _{i}\sum_{j = 1}^{n_{1}}y_{j}\left( B_{i}^{j}-B_{i}^{j-1}\right) \end{array} \right. \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ y_{i}^{n_{2}} = y_{i}\left( T_{1}\right) +h^{\alpha }\left( \begin{array}{c} \widetilde{a}_{n_{1}}^{\left( \alpha \right) }F_{i}\left( t_{n_{1}},y_{i}\left( t_{n_{1}}\right) \right) \\ +\sum_{k = 0}^{n_{2}}\widetilde{a}_{n_{1}+k}^{\left( \alpha \right) }F_{i}\left( t_{k},y_{i}\left( t_{k}\right) \right) \end{array} \right) \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right]. \end{array} \right. \end{equation}$

(5.17)

The numerical solutions for short memory are depicted in Figure 5 with the following parameters

$\begin{equation} N = 1000,\mu = 0.01,\beta = 0.6,a = 0.2,\gamma = 0.03, \end{equation}$

(5.18)

Figure 5. Numerical solutions of Covid-19 model with short memory for

$\alpha _{1} = 0.95, \alpha _{2} = 0.75$ .

DownLoad: Full-Size Img PowerPoint

and the following initial conditions

$\begin{eqnarray} S\left( 0\right) & = &1000,E\left( 0\right) = 25,I\left( 50\right) = 22,R\left( 50\right) = 5,S\left( 50\right) = 39 \\ E\left( 50\right) & = &67,I\left( 50\right) = 505,R\left( 50\right) = 390 \end{eqnarray}$

(5.19)

The numerical solutions for Cases 1 and 2 are respectively depicted in Figures 6 and 7 with the following parameters

$\begin{equation} N = 1000,\mu = 0.01,\beta = 0.6,a = 0.2,\gamma = 0.03, \end{equation}$

(5.20)

Figure 6. Numerical solutions of Covid-19 model under the conditions of Case 1

$\sigma _{1} = 0.000005, \sigma _{2} = 0.008, \sigma _{3} = 0.0021, \sigma _{4} = 0.009$ and

$\alpha = 0.7$ .

DownLoad: Full-Size Img PowerPoint

Figure 7. Numerical solutions of Covid-19 model under the conditions of Case 2

$\sigma _{1} = 0.17, \sigma _{2} = 0.25, \sigma _{3} = 0.21, \sigma _{4} = 0.19$ and

$\alpha = 0.7$ .

DownLoad: Full-Size Img PowerPoint

and the following initial conditions

$\begin{eqnarray} S\left( 0\right) & = &1000,E\left( 0\right) = 25,I\left( 50\right) = 22,R\left( 50\right) = 5,S\left( 50\right) = 35 \\ E\left( 50\right) & = &44,I\left( 50\right) = 424,R\left( 50\right) = 527,\alpha = 0.7 \end{eqnarray}$

(5.21)

demonstrates the short memory effect, where the first and second parts present different long tails behaviors characterized by $\alpha _{1}$ and $\alpha _{2}$ ; however, both clearly depict the same process which is the power law process. On the other hand, Figures 6 and 7 show the clear effects of the piecewise differential operators; the first part in Figure 6 shows normal distribution, which is characteristic of the classical differential operator, while the second part clearly shows the effect of randomness. The complete model therefore shows crossover behavior from deterministic to stochastic with no steady state. In Figure 6, the process is different. We start with randomness then end up with long tail behaviors as a result of the power law kernel.

Example 3. We now consider a chaotic problem in particular the 8-wing attractor ^[14]. This model has been studied by several researchers ^[15], the model is given as

$\begin{eqnarray} \overset{\cdot }{x} & = &a\left( y-x\right) +f\left( t\right) yz \\ \overset{\cdot }{y} & = &cx+dy-xz \\ \overset{\cdot }{z} & = &-bz+xy \end{eqnarray}$

(5.22)

where the function $f\left(t\right) = M$ $sgn\left(\sin \left(wt\right) \right) +K.$ The constant $w$ is known to be a switch frequency and $M$ and $K$ are constant parameters.

In the case of short memory, we have

$\begin{eqnarray} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha _{1}}x = a\left( y-x\right) +f\left( t\right) yz \\ _{0}^{C}D_{t}^{\alpha _{1}}y = cx+dy-xz \\ _{0}^{C}D_{t}^{\alpha _{1}}z = -bz+xy \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} _{T_{1}}^{C}D_{t}^{\alpha _{2}}x = a\left( y-x\right) +f\left( t\right) yz \\ _{T_{1}}^{C}D_{t}^{\alpha _{2}}y = cx+dy-xz \\ _{T_{1}}^{C}D_{t}^{\alpha _{2}}z = -bz+xy \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] . \end{eqnarray}$

(5.23)

In the case of piecewise, we can consider three cases. For Case 1, we can write

$\begin{eqnarray} \left\{ \begin{array}{c} _{0}^{ABC}D_{t}^{\alpha }x = a\left( y-x\right) +f\left( t\right) yz \\ _{0}^{ABC}D_{t}^{\alpha }y = cx+dy-xz \\ _{0}^{ABC}D_{t}^{\alpha }z = -bz+xy \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} dx = \left( a\left( y-x\right) +f\left( t\right) yz\right) dt+\sigma _{1}xdB_{1}\left( t\right) \\ dy = \left( cx+dy-xz\right) dt+\sigma _{2}ydB_{2}\left( t\right) \\ dz = \left( -bz+xy\right) dt+\sigma _{3}zdB_{3}\left( t\right) \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] . \end{eqnarray}$

(5.24)

For Case 2, we have

$\begin{eqnarray} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha }x = a\left( y-x\right) +f\left( t\right) yz \\ _{0}^{C}D_{t}^{\alpha }y = cx+dy-xz \\ _{0}^{C}D_{t}^{\alpha }z = -bz+xy \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} dx = \left( a\left( y-x\right) +f\left( t\right) yz\right) dt+\sigma _{1}xdB_{1}\left( t\right) \\ dy = \left( cx+dy-xz\right) dt+\sigma _{2}ydB_{2}\left( t\right) \\ dz = \left( -bz+xy\right) dt+\sigma _{3}zdB_{3}\left( t\right) \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] . \end{eqnarray}$

(5.25)

For Case 3, we can get

$\begin{eqnarray} \left\{ \begin{array}{c} \overset{\cdot }{x} = a\left( y-x\right) +f\left( t\right) yz \\ \overset{\cdot }{y} = cx+dy-xz \\ \overset{\cdot }{z} = -bz+xy \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} dx = \left( a\left( y-x\right) +f\left( t\right) yz\right) dt+\sigma _{1}xdB_{1}\left( t\right) \\ dy = \left( cx+dy-xz\right) dt+\sigma _{2}ydB_{2}\left( t\right) \\ dz = \left( -bz+xy\right) dt+\sigma _{3}zdB_{3}\left( t\right) \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] . \end{eqnarray}$

(5.26)

We shall now present the numerical solution of each case. In the case of short memory, one can use the numerical method based on Lagrange interpolation ^[16]. We can first simplify the model as follows.

$\begin{equation} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha _{1}}M_{i}\left( t\right) = \Pi \left( t,M_{i}\left( t\right) \right) \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ _{T_{1}}^{C}D_{t}^{\alpha _{2}}M_{i}\left( t\right) = \Pi \left( t,M_{i}\left( t\right) \right) \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right] \end{array} \right. {\rm{ }}. \end{equation}$

(5.27)

Thus, applying such method to the above system yields

$\begin{equation} \left\{ \begin{array}{c} M_{i}^{n_{1}} = M_{i}\left( 0\right) +\left[ \begin{array}{c} \frac{\left( \Delta t\right) ^{\alpha _{1}}}{\Gamma \left( \alpha _{1}+2\right) }\sum\limits_{q = 0}^{n_{1}}\Pi \left( t_{q},M_{i}^{q}\right) \\ \times \left[ \begin{array}{c} \left( n_{1}-q+1\right) ^{\alpha _{1}}\left( n_{1}-q+2+\alpha _{1}\right) \\ -\left( n_{1}-q\right) ^{\alpha _{1}}\left( n_{1}-q+2+2\alpha _{1}\right) \end{array} \right] \\ -\frac{\left( \Delta t\right) ^{\alpha _{1}}}{\Gamma \left( \alpha _{1}+2\right) }\sum\limits_{q = 1}^{n_{1}}\Pi \left( t_{q-1},M_{i}^{q-1}\right) \\ \times \left[ \begin{array}{c} \left( n_{1}-q+1\right) ^{\alpha _{1}+1} \\ -\left( n_{1}-q\right) ^{\alpha _{1}}\left( n_{1}-q+1+\alpha _{1}\right) \end{array} \right] \end{array} \right] \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ M_{i}^{n_{2}} = M_{i}\left( T_{1}\right) +\left[ \begin{array}{c} \frac{\left( \Delta t\right) ^{\alpha _{2}}}{\Gamma \left( \alpha _{2}+2\right) }\sum\limits_{k = n_{1}+1}^{n_{2}}\Pi \left( t_{k},M_{i}^{k}\right) \\ \times \left[ \begin{array}{c} \left( n_{2}-q+1\right) ^{\alpha _{2}}\left( n_{2}-q+2+\alpha _{2}\right) \\ -\left( n_{2}-q\right) ^{\alpha _{2}}\left( n_{2}-q+2+2\alpha _{2}\right) \end{array} \right] \\ -\frac{\left( \Delta t\right) ^{\alpha _{2}}}{\Gamma \left( \alpha _{2}+2\right) }\sum\limits_{k = n_{1}+2}^{n_{2}}\Pi \left( t_{k-1},M_{i}^{k-1}\right) \\ \times \left[ \begin{array}{c} \left( n_{2}-q+1\right) ^{\alpha _{2}+1} \\ -\left( n_{2}-q\right) ^{\alpha _{2}}\left( n_{2}-q+1+\alpha _{2}\right) \end{array} \right] \end{array} \right] \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right] \end{array} \right. {\rm{ }}. \end{equation}$

(5.28)

With the piecewise case, we obtain the following numerical solution by using a scheme based on Newton polynomial interpolation ^[17]. Also, we simplify the model as follows.

$\begin{equation} \left\{ \begin{array}{c} _{0}^{ABC}D_{t}^{\alpha _{1}}M_{i}\left( t\right) = \Pi \left( t,M_{i}\left( t\right) \right) \;{\rm{ if }}\;t\in \left[ 0,T_{1}\right] , \\ dM_{i}\left( t\right) = \Pi \left( t,M_{i}\left( t\right) \right) dt+\sigma _{i}M_{i}dB_{i}\left( t\right) \;{\rm{ if }}\;t\in \left[ T_{1},T_{2}\right] \end{array} \right. {\rm{ }}. \end{equation}$

(5.29)

Then applying this method yields

$\begin{equation} \begin{array}{c} M_{i}^{n_{1}} = M_{i}\left( 0\right) +\frac{1-\alpha }{AB\left( \alpha \right) }\Pi \left( t_{n_{1}},M_{i}^{n_{1}}\right) +\frac{\alpha \left( \Delta t\right) ^{\alpha }}{AB\left( \alpha \right) \Gamma \left( \alpha +1\right) } \sum\limits_{k = 2}^{n_{1}}\Pi \left( t_{k-2},M_{i}^{k-2}\right) \\ \times \left\{ \left( n_{1}-k+1\right) ^{\alpha }-\left( n_{1}-k\right) ^{\alpha }\right\} \\ +\frac{\alpha \left( \Delta t\right) ^{\alpha }}{AB\left( \alpha \right) \Gamma \left( \alpha +2\right) }\sum\limits_{k = 2}^{n_{1}}\left[ \Pi \left( t_{k-1},M_{i}^{k-1}\right) -\Pi \left( t_{k-2},M_{i}^{k-2}\right) \right] \\ \times \left\{ \begin{array}{c} \left( n_{1}-k+1\right) ^{\alpha }\left( n_{1}-k+3+2\alpha \right) \\ -\left( n_{1}-k\right) ^{\alpha }\left( n_{1}-k+3+3\alpha \right) \end{array} \right\} \\ +\frac{\alpha \left( \Delta t\right) ^{\alpha }}{2AB\left( \alpha \right) \Gamma \left( \alpha +3\right) }\sum\limits_{k = 2}^{n_{1}}\left[ \Pi \left( t_{k},M_{i}^{k}\right) -2\Pi \left( t_{k-1},M_{i}^{k-1}\right) +\Pi \left( t_{k-2},M_{i}^{k-2}\right) \right] \\ \times \left\{ \begin{array}{c} \left( n_{1}-k+1\right) ^{\alpha }\left[ \begin{array}{c} 2\left( n_{1}-k\right) ^{2}+\left( 3\alpha +10\right) \left( n_{1}-k\right) \\ +2\alpha ^{2}+9\alpha +12 \end{array} \right] \\ -\left( n_{1}-k\right) ^{\alpha }\left[ \begin{array}{c} 2\left( n_{1}-k\right) ^{2}+\left( 5\alpha +10\right) \left( n_{1}-k\right) \\ +6\alpha ^{2}+18\alpha +12 \end{array} \right] \end{array} \right\} ,0\leq t\leq T_{1} \\ \\ M_{i}^{n_{2}+4} = M_{i}^{n_{2}+3}+\sum\limits_{j_{3} = n_{1}+3}^{n_{3}}\left[ \begin{array}{c} \frac{23}{12}\Pi \left( t_{n_{2}+3},M_{i}^{n_{2}+3}\right) -\frac{4}{3}\Pi \left( t_{n_{2}+2},M_{i}^{n_{2}+2}\right) \\ +\frac{5}{12}\Pi \left( t_{n_{2}+1},M_{i}^{n_{2}+1}\right) \end{array} \right] \Delta t \\ +\sigma _{i}M_{i}^{n_{2}}\left( B_{i}^{n_{2}+1}-B_{i}^{n_{2}+1}\right) ,T_{1}\leq t\leq T_{2} \end{array} \end{equation}$

(5.30)

For Case 2, we again apply the scheme with the Newton polynomial to obtain

$\begin{equation} \left\{ \begin{array}{c} M_{i}^{n_{1}} = M_{i}\left( 0\right) +\frac{\left( \Delta t\right) ^{\alpha }}{ \Gamma \left( \alpha +1\right) }\sum\limits_{k = 2}^{n_{1}}\Pi \left( t_{k-2},M_{i}^{k-2}\right) \\ \times \left\{ \left( n_{1}-k+1\right) ^{\alpha }-\left( n_{1}-k\right) ^{\alpha }\right\} \\ +\frac{\left( \Delta t\right) ^{\alpha }}{\Gamma \left( \alpha +2\right) } \sum\limits_{k = 2}^{n_{1}}\left[ \Pi \left( t_{k-1},M_{i}^{k-1}\right) -\Pi \left( t_{k-2},M_{i}^{k-2}\right) \right] , \\ \times \left\{ \begin{array}{c} \left( n_{1}-k+1\right) ^{\alpha }\left( n_{1}-k+3+2\alpha \right) \\ -\left( n_{1}-k\right) ^{\alpha }\left( n_{1}-k+3+3\alpha \right) \end{array} \right\} \\ +\frac{\left( \Delta t\right) ^{\alpha }}{2\Gamma \left( \alpha +3\right) } \sum\limits_{k = 2}^{n_{1}}\left[ \Pi \left( t_{k},M_{i}^{k}\right) -2\Pi \left( t_{k-1},M_{i}^{k-1}\right) +\Pi \left( t_{k-2},M_{i}^{k-2}\right) \right] \\ \times \left\{ \begin{array}{c} \left( n_{1}-k+1\right) ^{\alpha }\left[ \begin{array}{c} 2\left( n_{1}-k\right) ^{2}+\left( 3\alpha +10\right) \left( n_{1}-k\right) \\ +2\alpha ^{2}+9\alpha +12 \end{array} \right] \\ -\left( n_{1}-k\right) ^{\alpha }\left[ \begin{array}{c} 2\left( n_{1}-k\right) ^{2}+\left( 5\alpha +10\right) \left( n_{1}-k\right) \\ +6\alpha ^{2}+18\alpha +12 \end{array} \right] \end{array} \right\} ,0\leq t\leq T_{1} \\ \\ M_{i}^{n_{2}+4} = M_{i}^{n_{2}+3}+\sum\limits_{j_{3} = n_{2}+3}^{n_{3}}\left[ \begin{array}{c} \frac{23}{12}\Pi \left( t_{n_{2}+3},M_{i}^{n_{2}+3}\right) -\frac{4}{3}\Pi \left( t_{n_{2}+2},M_{i}^{n_{2}+2}\right) \\ +\frac{5}{12}\Pi \left( t_{n_{2}+1},M_{i}^{n_{2}+1}\right) \end{array} \right] \Delta t \\ +\sigma _{i}M_{i}\left( C_{n_{2}}\right) \left( B_{i}^{n_{2}+1}-B_{i}^{n_{2}+1}\right) ,T_{1}\leq t\leq T_{2} \end{array} \right. \end{equation}$

(5.31)

where $C_{n_{2}}\in \left[ t_{n_{2}+4}, t_{n_{2}+3}\right]$ . For Case 3, we use the simple Adams-Bashforth methods as follows

$\begin{equation} \left\{ \begin{array}{c} M_{i}^{n_{2}+3} = M_{i}^{n_{2}+2}+\left[ \begin{array}{c} \frac{23}{12}\Pi \left( t_{n_{2}+2},M_{i}^{n_{2}+2}\right) -\frac{4}{3}\Pi \left( t_{n_{2}+1},M_{i}^{n_{2}+1}\right) \\ +\frac{5}{12}\Pi \left( t_{n_{2}},M_{i}^{n_{2}}\right) \end{array} \right] \Delta t,0\leq t\leq T_{1} \\ \\ M_{i}^{n_{2}+4} = M_{i}^{n_{2}+3}+\left[ \begin{array}{c} \frac{23}{12}\Pi \left( t_{n_{2}+3},M_{i}^{n_{2}+3}\right) -\frac{4}{3}\Pi \left( t_{n_{2}+2},M_{i}^{n_{2}+2}\right) \\ +\frac{5}{12}\Pi \left( t_{n_{2}+1},M_{i}^{n_{2}+1}\right) \end{array} \right] \Delta t \\ +\sigma _{i}M_{i}\left( C_{n_{2}}\right) \left( B_{i}^{n_{2}+1}-B_{i}^{n_{2}}\right) ,T_{1}\leq t\leq T_{2} \end{array} \right. \end{equation}$

(5.32)

where $C_{n_{2}}\in \left[ t_{n_{2}+4}, t_{n_{2}+3}\right].$

Numerical solutions are obtained for the following parameters

$\begin{equation} a = 14,b = 43,c = -1,d = 16,M = 9,K = 10,w = \frac{2\pi }{50}. \end{equation}$

(5.33)

The numerical solutions for short memory are depicted in for the initial data $x\left(0\right) = 22.3, y\left(0\right) = 4.9$ and $z\left(0\right) = 2.6.$

Figure 8. Numerical solutions for 8-wing attractor with short memory for

$\alpha _{1} = 0.96$ and

$\alpha _{2} = 0.98$ .

DownLoad: Full-Size Img PowerPoint

For piecewise, we consider the following stochastic-deterministic problem with power-law and Mittag-Leffler kernels:

$\begin{eqnarray} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha }x = a\left( y-x\right) +f\left( t\right) yz+\sigma _{1}xB\prime _{1}\left( t\right) \\ _{0}^{C}D_{t}^{\alpha }y = cx+dy-xz+\sigma _{2}yB\prime _{2}\left( t\right) \\ _{0}^{C}D_{t}^{\alpha }z = -bz+xy+\sigma _{3}zB\prime _{3}\left( t\right) \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} _{{{T}_{1}}}^{ABC}D_{t}^{\alpha }x = a\left( y-x\right) +f\left( t\right) yz \\ _{{{T}_{1}}}^{ABC}D_{t}^{\alpha }y = cx+dy-xz \\ _{{{T}_{1}}}^{ABC}D_{t}^{\alpha }z = -bz+xy \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] \end{eqnarray}$

(5.34)

with the stochastic constants

$\begin{equation} \sigma _{1} = 0.031;\sigma _{2} = 0.035;\sigma _{3} = 0.032. \end{equation}$

(5.35)

The numerical simulation for such a problem can be performed as illustrated in Figure 9 by using the same initial data and parameters.

Figure 9. Stochastic-deterministic 8-wing attractor with piecewise derivative for

$\alpha = 0.99$ .

DownLoad: Full-Size Img PowerPoint

For piecewise, we consider the following stochastic-deterministic problem with power-law and Mittag-Leffler kernels:

$\begin{eqnarray} \left\{ \begin{array}{c} _{0}^{ABC}D_{t}^{\alpha }x = a\left( y-x\right) +f\left( t\right) yz+\sigma _{1}xB\prime _{1}\left( t\right) \\ _{0}^{ABC}D_{t}^{\alpha }y = cx+dy-xz+\sigma _{2}yB\prime _{2}\left( t\right) \\ _{0}^{ABC}D_{t}^{\alpha }z = -bz+xy+\sigma _{3}zB\prime _{3}\left( t\right) \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} _{{{T}_{1}}}^{C}D_{t}^{\alpha }x = a\left( y-x\right) +f\left( t\right) yz \\ _{{{T}_{1}}}^{C}D_{t}^{\alpha }y = cx+dy-xz \\ _{{{T}_{1}}}^{C}D_{t}^{\alpha }z = -bz+xy \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] \end{eqnarray}$

(5.36)

with the following stochastic constants

$\begin{equation} \sigma _{1} = 0.031;\sigma _{2} = 0.035;\sigma _{3} = 0.032. \end{equation}$

(5.37)

The numerical simulation for such a problem can be performed as shown in Figure 10 by using the same initial data and parameters.

Figure 10. Stochastic-deterministic 8-wing attractor with piecewise derivative for

$\alpha = 0.94$ .

DownLoad: Full-Size Img PowerPoint

Also, we consider the following deterministic-stochastic problem with power-law and Mittag-Leffler kernels:

$\begin{eqnarray} \left\{ \begin{array}{c} _{0}^{C}D_{t}^{\alpha }x = a\left( y-x\right) +f\left( t\right) yz \\ _{0}^{C}D_{t}^{\alpha }y = cx+dy-xz \\ _{0}^{C}D_{t}^{\alpha }z = -bz+xy \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} _{{{T}_{1}}}^{ABC}D_{t}^{\alpha }x = a\left( y-x\right) +f\left( t\right) yz+\sigma _{1}xB\prime _{1}\left( t\right) \\ _{{{T}_{1}}}^{ABC}D_{t}^{\alpha }y = cx+dy-xz+\sigma _{2}yB\prime _{2}\left( t\right) \\ _{{{T}_{1}}}^{ABC}D_{t}^{\alpha }z = -bz+xy+\sigma _{3}zB\prime _{3}\left( t\right) \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] \end{eqnarray}$

(5.38)

with the following stochastic constants:

$\begin{equation} \sigma _{1} = 0.02;\sigma _{2} = 0.012;\sigma _{3} = 0.021. \end{equation}$

(5.39)

The numerical solutions for the above problem can be depicted as shown in Figure 11 using same initial data and parameters.

Figure 11. Deterministic-stochastic 8-wing attractor with piecewise derivative for

$\alpha = 0.99$ .

DownLoad: Full-Size Img PowerPoint

Figure 7 shows the results obtained by applying the power-law short memory concept. To show the difference within the interval, we have opted to consider the first part of the interval to be in blue and the second to be in red. However, a quick look at the results shows clearly the effect of a power law, no great change from one interval to another is observed since both depict long-tail behaviors as results of the power-law kernel. In this case, there is no crossover behavior, just a repetition of different long tail behaviors. On the other hand, in Figures 8 and 9, there is a clear change in patterns where the first patterns show deterministic behaviors in particular, long-tails due to power law in Figure 8, and the second part shows randomness, there is, therefore, a clear crossover from power law to randomness. While, in Figure 9, we have two crossovers, the first is due to the Mittag-Leffler kernel that shows a change from stretched exponential to power law and the second is stochastic.

Also, we consider deterministic-stochastic problem with power-law and Mittag-Leffler kernel

$\begin{eqnarray} \left\{ \begin{array}{c} _{0}^{ABC}D_{t}^{\alpha }x = a\left( y-x\right) +f\left( t\right) yz \\ _{0}^{ABC}D_{t}^{\alpha }y = cx+dy-xz \\ _{0}^{ABC}D_{t}^{\alpha }z = -bz+xy \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ 0,T_{1}\right] , \\ \left\{ \begin{array}{c} _{{{T}_{1}}}^{C}D_{t}^{\alpha }x = a\left( y-x\right) +f\left( t\right) yz+\sigma _{1}xB\prime _{1}\left( t\right) \\ _{{{T}_{1}}}^{C}D_{t}^{\alpha }y = cx+dy-xz+\sigma _{2}yB\prime _{2}\left( t\right) \\ _{{{T}_{1}}}^{C}D_{t}^{\alpha }z = -bz+xy+\sigma _{3}zB\prime _{3}\left( t\right) \end{array} \right. \;{\rm{ if }}\;t &\in &\left[ T_{1},T_{2}\right] \end{eqnarray}$

(5.40)

with the following stochastic constants:

$\begin{equation} \sigma _{1} = 0.02;\sigma _{2} = 0.012;\sigma _{3} = 0.021. \end{equation}$

(5.41)

The numerical solutions for the above problem can be depicted as shown in Figure 12 by using the same initial data and parameters.

Figure 12. Deterministic-stochastic 8-Wing attractor with piecewise derivative for

$\alpha = 0.9$ .

DownLoad: Full-Size Img PowerPoint

6. Conclusions

In the last decades, researchers have devoted their attention to better understanding complex realworld problems even on a small scale. They have therefore developed several methods, different differential, and integral operators. To understand the process by which different long tails occur in different intervals, the concept of short memory was introduced. This concept defines power-law derivatives in different intervals and each interval has its own order. This order accounts for the long tail associated with that interval. However, the process is scale-invariant in terms of patterns. On the other hand, because there are many real-world problems that exhibit passages from one process to another, for example, a passage from power law to randomness, the concept of piecewise was introduced. In this work, a detailed analysis was given to show their different fields of applications and show also, when short memory and piecewise concepts can be applied. This paper thus helps researchers to identify what problem is suitable for short memory and piecewise.

Conflict of interest

The authors declare there is no conflict of interest.

Acknowledgments

The authors would like to thank the editorial team and the anonymous reviewers for their critical comments and supportive comments.

Authors' contribution

Mr. Aswin Sugunan and Mr. Rajasekharan Pillai K. conceptualized the research and prepared the original draft. Mr. Aswin Sugunan conducted the data collection and analysis, while Dr. Anice George reviewed and edited the final manuscript.

Conflict of interest

The authors have no conflict of interest to declare.

References

[1]	Sangar S, Dutt V, Thakur R (2019) Economic burden, impoverishment, and coping mechanisms associated with out-of-pocket health expenditure in India: A disaggregated analysis at the state level. Int J Health Plann Manage 34: e301-e313. https://doi.org/10.1002/hpm.2649
[2]	Sato R (2022) Catastrophic health expenditure and its determinants among Nigerian households. Int J Health Econ Manag 22: 459-470. https://doi.org/10.1007/s10754-022-09323-y
[3]	Wagstaff A, Flores G, Hsu J, et al. (2018) Progress on catastrophic health spending in 133 countries: a retrospective observational study. Lancet Glob Health 6: e169-e179. https://doi.org/10.1596/29254
[4]	Ahlin T, Nichter M, Pillai G (2016) Health insurance in India: what do we know and why is ethnographic research needed. Anthropol Med 23: 102-124. https://doi.org/10.1080/13648470.2015.1135787
[5]	Ravi S, Ahluwalia R, Bergkvist S Health and morbidity in India (2004–2014) (2016). Available from: https://www.brookings.edu/wp-content/uploads/2016/12/201612_health-andmorbidity.pdf
[6]	Prinja S, Bahuguna P, Gupta I, et al. (2019) Role of insurance in determining utilization of healthcare and financial risk protection in India. PLoS One 5: e0211793. https://doi.org/10.1371/journal.pone.0211793
[7]	Nandi S, Schneider H, Dixit P (2017) Hospital utilization and out of pocket expenditure in public and private sectors under the universal government health insurance scheme in Chhattisgarh State, India: Lessons for universal health coverage. PLoS One 12: e0187904. https://doi.org/10.1371/journal.pone.0187904
[8]	Rahman MM, Karan A, Rahman MS, et al. (2017) Progress Toward Universal Health Coverage: A Comparative Analysis in 5 South Asian Countries. JAMA Intern Med 177: 1297-1305. https://doi.org/10.1001/jamainternmed.2017.3133
[9]	Volker M, Kanchanachithra M (2021) Longterm effects of health shocks and insurance on rural households consumption and wealth in Vietnam: evidence from panel data. J Public Hlth Dev 19: 1-16.
[10]	World Health OrganizaitonHealth financing for universal coverage. Available from: https://www.who.int/health_financing/topics/voluntary-health-insurance/what-it-is/en/
[11]	Yadav MK, Mohanty PC (2021) Determinants of choice of health insurance: Empirical evidence from responses of Indian households. Int J Health Plann Manage 6: 1809-1829. https://doi.org/10.1002/hpm.3267
[12]	Mulenga J, Mulenga MC, Musonda KMC, et al. (2021) Examining gender differentials and determinants of private health insurance coverage in Zambia. BMC Health Serv Res 21: 1212. https://doi.org/10.1186/s12913-021-07253-y
[13]	Vellakkal S (2013) Impact of Private Health Insurance on Lengths of Hospitalization and Healthcare Expenditure in India: Evidences from a Quasi-Experiment Study. Indian J Econ Dev 1: 24-28.
[14]	Chhabra KR, Sheetz KH, Nuliyalu U, et al. (2020) Out-of-Network Bills for Privately Insured Patients Undergoing Elective Surgery With In-Network Primary Surgeons and Facilities. JAMA 323: 538-547. https://doi.org/10.1001/jama.2019.21463
[15]	Rodriguez-Acevedo AJ, Chan RJ, Olsen CM, et al. (2021) Out-of-pocket medical expenses compared across five years for patients with one of five common cancers in Australia. BMC Cancer 21: 1055. https://doi.org/10.1186/s12885-021-08756-x
[16]	Peng Z, Zhu L (2021) The impacts of health insurance on financial strain for people with chronic diseases. BMC Public Health 21: 1012. https://doi.org/10.1186/s12889-021-11075-2
[17]	Grigorakis N, Floros C, Tsangari H, et al. (2017) Combined social and private health insurance versus catastrophic out of pocket payments for private hospital care in Greece. Int J Health Econ Manag . https://doi.org/10.1007/s10754-016-9203-7
[18]	Rao M, Kadam S, Sathyanarayana TN, et al. (2012) A rapid evaluation of the Rajiv Aarogyasri community health insurance scheme in Andhra Pradesh, India. BMC Proc 6: O4. https://doi.org/10.1186/1753-6561-6-S1-O4
[19]	Sinha RK (2018) Impact of publicly financed health insurance scheme (Rashtriya Swasthya Bima Yojana) from equity and efficiency perspectives. Vikalpa 43: 191-206. https://doi.org/10.1177/0256090918804390
[20]	Wagstaff A, Eozenou P, Smitz M (2020) Out-of-pocket expenditures on Health: A Global stocktake. The World Bank Research Observer 35: 123-157. https://doi.org/10.1093/wbro/lkz009
[21]	Insurance Regulatory and Development Authority Of India.Guidelines on Standard Individual Health Insurance Product. Government of India (2020) . Available from: https://www.irdai.gov.in/admincms/cms/uploadedfiles/Guidelines%20on%20Standard%20Individual%20Health%20Insurance%20Product.pdf.
[22]	Insurance Regulatory and Development Authority Of India.Guidelines on Standard Individual Health Insurance Product. Government of India (2023) . Available from: https://irdai.gov.in/document-detail?documentId=4273788.
[23]	Saksena P, Hsu J, Evans DB (2014) Financial Risk Protection and Universal Health Coverage: Evidence and Measurement Challenges. PLoS Med 11: e1001701. https://doi.org/10.1371/journal.pmed.1001701
[24]	Islam MR, Rahman MS, Islam Z, et al. (2017) Inequalities in financial risk protection in Bangladesh: an assessment of universal health coverage. Int J Equity Health 16: 59. https://doi.org/10.1186/s12939-017-0556-4
[25]	Devadasan N, Seshadri T, Trivedi M, et al. (2013) Promoting universal financial protection: Evidence from the Rashtriya Swasthya Bima Yojana (RSBY) in Gujarat, India. Health Res Policy Syst 11: 29. https://doi.org/10.1186/1478-4505-11-29
[26]	Fan VY, Karan A, Mahal A (2012) State health insurance and out-of-pocket health expenditures in Andhra Pradesh, India. Int J Health Care Finance Econ 12: 189-215. https://doi.org/10.1007/s10754-012-9110-5
[27]	Sood N, Bendavid E, Mukherji A, et al. (2014) Government health insurance for people below poverty line in India: quasi-experimental evaluation of insurance and health outcomes. BMJ 349: g5114. https://doi.org/10.1136/bmj.g5114
[28]	Prinja S, Bahuguna P, Gupta I, et al. (2019) Role of insurance in determining utilization of healthcare and financial risk protection in India. PLoS One 14: e0211793. https://doi.org/10.1371/journal.pone.0211793
[29]	Prinja S, Bahuguna P, Singh MP, et al. (2023) Refining the provider payment system of India's government-funded health insurance programme: an econometric analysis. BMJ Open 13: e076155. https://doi.org/10.1136/bmjopen-2023-076155
[30]	Chen X, Guo D, Tan H, et al. (2022) Can supplementary private health insurance further supplement health. Front Public Health 10: 961019. https://doi.org/10.3389/fpubh.2022.961019
[31]	Sriram S, Khan MM (2020) Effect of health insurance program for the poor on out-of-pocket inpatient care cost in India: evidence from a nationally representative cross-sectional survey. BMC Health Serv Res 20: 839. https://doi.org/10.1186/s12913-020-05692-7
[32]	Reshmi B, Unnikrishnan B, Rajwar E, et al. (2021) Impact of public-funded health insurances in India on health care utilisation and financial risk protection: a systematic review. BMJ Open 11: e050077. https://doi.org/10.1136/bmjopen-2021-050077
[33]	Garg S, Bebarta KK, Tripathi N (2022) Role of publicly funded health insurance in financial protection of the elderly from hospitalisation expenditure in India-findings from the longitudinal aging study. BMC Geriatr 22: 572. https://doi.org/10.1186/s12877-022-03266-2
[34]	Devadasan N, Criel B, Van Damme W, et al. (2007) Indian community health insurance schemes provide partial protection against catastrophic health expenditure. BMC Health Serv Res 7: 43. https://doi.org/10.1186/1472-6963-7-43
[35]	Eze P, Ilechukwu S, Lawani LO (2023) Impact of community-based health insurance in low- and middle-income countries: A systematic review and meta-analysis. PLoS One 18: e0287600. https://doi.org/10.1371/journal.pone.0287600
[36]	Habib SS, Perveen S, Khuwaja HM (2016) The role of micro health insurance in providing financial risk protection in developing countries--a systematic review. BMC Public Health 16: 281. https://doi.org/10.1186/s12889-016-2937-9
[37]	Nair S (2019) A comparative study of the satisfaction level of health insurance claimants of public and private sector general insurance companies. J Inst 17: 33-42.
[38]	Dror DM, Radermacher R, Koren R (2007) Willingness to pay for health insurance among rural and poor persons: field evidence from seven micro health insurance units in India. Health Policy 82: 12-27. https://doi.org/10.1016/j.healthpol.2006.07.011
[39]	Binny DMG (2017) Health insurance in India–Opportunities and challenges. IJLTEMAS 6: 36-43.
[40]	Gambhir RS, Malhi R, Khosla S, et al. (2019) Out-patient coverage: Private sector insurance in India. J Family Med Prim Care 8: 788-792. https://doi.org/10.4103/jfmpc.jfmpc_101_19
[41]	Mathur T, Paul UK, Prasad HN, et al. (2014) Understanding perception and factors influencing private voluntary health insurance policy subscription in the Lucknow region. Int J Health Policy Manag 4: 75-83. https://doi.org/10.15171/ijhpm.2015.08
[42]	Zuhair M, Roy RB (2022) Eliciting relative preferences for the attributes of health insurance schemes among rural consumers in India. Int J Health Econ Manag 22: 443-458. https://doi.org/10.1007/s10754-022-09327-8
[43]	Puth MT, Neuhäuser M, Ruxton GD (2015) Effective use of Spearman's and Kendall's correlation coefficients for association between two measured traits. Animal Behaviour 102: 77-84. https://doi.org/10.1016/j.anbehav.2015.01.010
[44]	Erlangga D, Suhrcke M, Ali S, et al. (2019) The impact of public health insurance on health care utilisation, financial protection and health status in low- and middle-income countries: A systematic review. PLoS One 14: e0219731. https://doi.org/10.1371/journal.pone.0219731
[45]	Sommers BD (2020) Health Insurance Coverage: What Comes After The ACA?. Health Aff 39: 502-508. https://doi.org/10.1377/hlthaff.2019.01416
[46]	Adrion ER, Ryan AM, Seltzer AC, et al. (2016) Out-of-Pocket Spending for Hospitalizations Among Nonelderly Adults. JAMA Intern Med 176: 1325-1332. https://doi.org/10.1001/jamainternmed.2016.3663
[47]	Link CL, McKinlay JB (2010) Only half the problem is being addressed: underinsurance is as big a problem as uninsurance. Int J Health Serv 40: 507-523. https://doi.org/10.2190/HS.40.3.g
[48]	Robertson CT, Yuan A, Zhang W, et al. (2020) Distinguishing moral hazard from access for high-cost healthcare under insurance. PLoS One 15: e0231768. https://doi.org/10.1371/journal.pone.0231768
[49]	Yagi BF, Luster JE, Scherer AM, et al. (2022) Association of Health Insurance Literacy with Health Care Utilization: a Systematic Review. J Gen Intern Med 37: 375-389. https://doi.org/10.1007/s11606-021-06819-0
[50]	Gupta A, Reddy BV, Semwal V, et al. (2017) Financial Burden of Out-of-Pocket Expenditures for Primary Health Care in Hilly Areas of Garhwal Region, Uttarakhand, North India. J Clin Diagn Res 11: LC08-LC11. https://doi.org/10.7860/JCDR/2017/24761.9646
[51]	Ambade M, Sarwal R, Mor N, et al. (2022) Components of Out-of-Pocket Expenditure and Their Relative Contribution to Economic Burden of Diseases in India. JAMA Netw Open 5: e2210040. https://doi.org/10.1001/jamanetworkopen.2022.10040
[52]	Reddy KN, Shah J, Iyer S, et al. (2021) Direct Medical Cost Analysis of Indian COVID-19 Patients Requiring Critical Care Admission. Indian J Crit Care Med 25: 1120-1125. https://doi.org/10.5005/jp-journals-10071-23991
[53]	Deng P, Fu Y, Chen M, et al. (2022) Factors associated with health care utilization and catastrophic health expenditure among cancer patients in China: Evidence from the China health and retirement longitudinal study. Front Public Health 10: 943271. https://doi.org/10.3389/fpubh.2022.943271
[54]	Gustafsson-Wright E, Popławska G, Tanović Z, et al. (2018) The impact of subsidized private health insurance and health facility upgrades on healthcare utilization and spending in rural Nigeria. Int J Health Econ Manag 18: 221-276. https://doi.org/10.1007/s10754-017-9231-y
[55]	Sugunan A, Rajasekharan Pillai K, George A (2023) Effectiveness of interventions to contain out-of-pocket-expenditure in lower-middle income countries: A systematic review and synthesis. Int J Health Plann Manage 38: 918-935. https://doi.org/10.1002/hpm.3642
[56]	Meng Q, Mills A, Wang L, et al. (2019) What can we learn from China's health system reform?. BMJ 365: l2349. https://doi.org/10.1136/bmj.l2349
[57]	Lee DC, Wang J, Shi L, et al. (2022) Health insurance coverage and access to care in China. BMC Health Serv Res 22: 140. https://doi.org/10.1186/s12913-022-07498-1

This article has been cited by:

1.	Rodrigo Moreira, Larissa Ferreira Rodrigues Moreira, Flávio de Oliveira Silva, Brazilian Selic Rate Forecasting with Deep Neural Networks, 2024, 0927-7099, 10.1007/s10614-024-10597-2
2.	Michele Bufalo, Claudia Ceci, Giuseppe Orlando, Addressing the financial impact of natural disasters in the era of climate change, 2024, 73, 10629408, 102152, 10.1016/j.najef.2024.102152
3.	Guo-Hui Yang, Si-Qi Ma, Xiao-Dong Bian, Jiang-Cheng Li, Pierluigi Vellucci, The roles of liquidity and delay in financial markets based on an optimal forecasting model, 2023, 18, 1932-6203, e0290869, 10.1371/journal.pone.0290869
4.	Giacomo Ascione, Michele Bufalo, Giuseppe Orlando, Modeling volatility of disaster-affected populations: A non-homogeneous geometric-skew Brownian motion approach, 2024, 130, 10075704, 107761, 10.1016/j.cnsns.2023.107761

Reader Comments

Your name:*

Email:*
© 2024 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Public Health

3.1 4.8

Metrics

Article views(988) PDF downloads(86) Cited by(0)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

AIMS Public Health

What is the coverage of your health insurance plan? An audit of hospital billing

Related Papers:

Abstract

1. Introduction

2. Motivation for piecewise derivatives

3. Short memory concept

4. Analysis and difference

5. Application to real world problems

6. Conclusions

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

Abstract

1. Introduction

2. Motivation for piecewise derivatives

3. Short memory concept

4. Analysis and difference

5. Application to real world problems

6. Conclusions

Conflict of interest

References

AIMS Public Health

What is the coverage of your health insurance plan? An audit of hospital billing

Related Papers:

Abstract

1. Introduction

2. Motivation for piecewise derivatives

3. Short memory concept

4. Analysis and difference

5. Application to real world problems

6. Conclusions

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog

Abstract

1. Introduction

2. Motivation for piecewise derivatives

3. Short memory concept

4. Analysis and difference

5. Application to real world problems

6. Conclusions

Conflict of interest

References