BV regularity of the adapted entropy solutions for conservation laws with infinitely many spatial discontinuities

1.   Introduction

In this article, we consider the following initial value problem for scalar conservation laws in one space dimension:

It is assumed that the flux function $A(x, \cdot)$ is $C^2$ and uniformly convex, i.e., there exists a positive constant $C$ such that $A_{uu}(x, \cdot) \geq C > 0$ for all $x\in \mathbb{R}$. It is to be noted that as a function of the space variable, the function $A(x, u)$ can have infinitely many spatial discontinuities with possible accumulation points.

Conservation laws with discontinuous flux ^{[2,3,4,5,7,9,11,12,14,15,23,24,25,29,30]} has been a subject of immense interest to the mathematical community, as it is known to model various real-world problems. Examples include, the hydrodynamic limit of interacting particle systems with discontinuous speed parameter ^[13], sedimentation ^[14], petroleum industry and polymer flooding ^[29], two-phase flow in heterogeneous porous medium ^[6], clarifier thickener units used in wastewater treatment plants and mineral processing ^[10], and traffic flow on highways with changing surface conditions ^[15], etc. One of the classical studies of this IVP with the flux having spatial heterogeneities come from the seminal paper of Kruzkov ^[26], which studies its well-posedness for sufficiently smooth flux $A$. However, in practice, the flux function $A$ appearing in the IVP modelling real-life applications, may not be smooth and may have spatial discontinuities. Since the flux contains spatial discontinuities, the Kruzkov entropy condition does not make sense and hence a different notion for uniqueness, incorporating the non-smooth structure of the flux is required. Owing to this, the notion of an adapted entropy solution is used to single out a unique solution for IVPs with non-smooth flux. The notion of adapted entropy condition was introduced by Audusse and Perthame in ^[8] for unimodal and monotone fluxes and later it was generalized in ^[27] to a more general class of fluxes of the type $A(x, u) = g(\beta(x, u))$ with $g$, a continuous function and $\beta$, Caratheodory function (i.e. $\beta$ measurable in the $x$ variable and continuous in the $u$ variable). The existence of the entropy solutions was established via the convergence of numerical approximations in, ^[28] using front tracking approximations for monotone convex fluxes, and in ^[31] using finite volume approximations for possibly non–convex monotone fluxes. Additionally, the convergence of finite volume approximations for unimodal fluxes was studied in ^[18] using a singular mapping technique. In addition, Ghoshal et al. ^[20,21] extended the results for a Locally Lipschitz $g$ and BV spatial $\beta$, using a $\beta-$ TVD (Total Variation Diminishing) property. Further, Ghoshal et al. ^[22] also extended the results using the front tracking approximations for fluxes with flat regions. In this article, we describe the existence and non-existence of total variation bounds on the solution for uniformly convex flux $A(x, \cdot)$.

Total variation plays a pivotal role in the study of hyperbolic conservation laws. In the case of homogeneous flux i.e., $A(x, u) = f(u)$, using the Lax–Oleinik formula, it can be shown that if the flux function is $C^2$ and uniformly convex, i.e., $f''\geq C > 0,$ then the entropy solution $u(t, \cdot)\in {\rm{BV}}_{loc}(\mathbb{R})$ for any initial data $u_0\in L^{\infty}(\mathbb{R}).$ In other words, a $C^2$ uniformly convex flux induces a ${\rm{BV}}$ regularizing effect on the entropy solution. For a locally Lipschitz flux (homogeneous), this result is not true in general. However, front tracking and finite volume approximations indicate that, $t \mapsto {\rm{TV}}(u(t, \cdot){)}$ is non-increasing. Thus, the total variation of the solution at any time $t > 0$ cannot be greater than the total variation of the initial data. On the other hand, for a two flux problem with $A(x, u) = H(-x)g(u)+H(x)f(u),$ where $f$ and $g$ are $C^2$ and convex, the authors in ^[1] construct counterexamples to show that the total variation of the entropy solution (see [1, Def. 2.4] with $A = \theta_g$ and $B = \theta_f$) blows up in finite time, even for ${\rm{BV}}$ initial data.

Furthermore, results produced by the finite volume method ^[9] suggest that the total variation of the entropy solution away from the interface $x = 0$ remains bounded, i.e., $u(t, \cdot) \in {\rm{BV}}_{\mathrm{loc}}(\mathbb{R} \setminus \{0\}).$ Also, the ${\rm{BV}}$ smoothing effect away from the interface for two flux problems with uniformly convex flux is discussed in ^[17]. Surprisingly, if $f(\theta_f) = g(\theta_g),$ the existence of ${\rm{BV}}$ bounds was proved in ^[16] using an explicit formula for the two flux problem obtained in ^[5]. Furthermore, the regularity of the solutions in $BV^s$ space for conservation laws with discontinuous flux were recently investigated in ^[19].

These results can be naturally extended for the case of finitely many discontinuities and hence we have the existence of uniform ${\rm{BV}}$ bounds on the solution.

In the present article, we will show that results on ${\rm{BV}}$ bounds for the case when $F$ is unimodal and has infinitely many spatial discontinuities are completely different from the case of finitely many spatial discontinuities. At this point, we would like to mention that the case where $A(x, \cdot)$ does not contain critical points is relatively simpler and ${\rm{BV}}$ bounds can be easily obtained using finite volume ^[31] and front tracking ^[28] approximations provided $u_0\in {\rm{BV}}(\mathbb{R}).$

Hence, the question of whether a ${\rm{BV}}$ bound on the solution for fluxes having critical points in the $u$-variable and infinitely many spatial discontinuities is interesting and nontrivial. In this article, we provide a sufficient condition on the initial data to obtain a ${\rm{BV}}$ bound for the entropy solution for uniformly convex fluxes. In the later part of this article, we construct two counterexamples to demonstrate that the conditions are essential. Note that the flux $A(x, \cdot)$ considered in the current article has the same minimum for each $x$ and is uniformly convex. By providing two examples in Sections 3.2 and 3.3, we show that the ${\rm{BV}}$-regularity results of ^[16] can not hold when the flux $F$ has infinitely many discontinuities. The example in Section 3.3 further shows that even if we assume uniform convexity of the flux, the ${\rm{TV}}$ (Total Variation) of the entropy solution can blow up. The construction of the example in Section 3.3 is novel and it contains new ideas which differ from previous constructions ^[1,16].

2.   Preliminaries

Throughout this article, we assume that the flux function $A(x, u)$ satisfies the following assumptions.

A-1 The function $x \mapsto A(x, u)$ is measurable, $u \mapsto A(x, u)$ is uniformly convex and there exists $u_M \in {\rm{BV}}(\mathbb{R})$ such that the following holds:

A-2 There exists $(h_1, h_2)\in C^0(\mathbb{R})^2$ such that for all $x\in \mathbb{R},$ we have $h_1(u)\leq A(x, u) \leq h_2(u).$ In addition, $h_i$ for $i = 1, 2$ are strictly decreasing and then increasing functions with $\left| {h_1(\pm \infty)}\right| = + \infty.$

A-3 There exists a continuous function $\eta: \mathbb{R} \rightarrow \mathbb{R}$ and a ${\rm{BV}}$ function $a: \mathbb{R} \rightarrow \mathbb{R}$ such that

Remark 2.1. A typical example of a flux function satisfying the above hypothesis (see ^[8]) is given by

2.1.   Adapted entropy condition and uniqueness

In view of the above hypothesis, we define the following:

Definition 2.1. A function $k: \mathbb{R} \rightarrow \mathbb{R}$ is said to be a stationary state, if $u(x, t) = k(x)$ is the weak solution to the IVP (1.1) and (1.2), with $u_0(x) = k(x)$. For $\alpha > 0$, we work with two types of stationary states namely, $k_{\alpha}^{\pm}: \mathbb{R} \rightarrow \mathbb{R}$ such that $A(x, k^{\pm}_ \alpha(x)) = \alpha$ with $k_ \alpha^{+}(x) \in (u_M(x), \infty)$ and $k_ \alpha^{-}(x) \in (- \infty, u_M(x)).$ On the other hand, for $\alpha = 0,$ we have $k_0^+ = k_0^- = u_M.$ We define $\mathscr{S}_ \alpha$ to be the set of all stationary states corresponding to height $\alpha\geq0$.

Definition 2.2 (Adapted Entropy Condition, ^[8]). A function $u\in L^{\infty}(\mathbb{R} \times [0, T]) \cap C([0, T], L^{1}_{loc}(\mathbb{R}))$ is an adapted entropy solution of the Cauchy problem if it satisfies the following inequality in the sense of distribution:

for $\alpha \geq 0.$ Or equivalently, for all $0\leq\phi \in C_c^{\infty}(\mathbb{R} \times [0, T))$

Theorem 2.1. [Uniqueness ^[8,27]] Let $u, v$ be entropy solutions to the IVP (1.1) and (1.2) with initial data $u_0, v_0 \in L^{\infty}(\mathbb{R}).$ Assume that the flux A(x, u) satisfies the hypotheses (A-1) and (A-2). Then, there exists $M > 0$ such that the following holds,

for $- \infty\leq a < b\leq \infty$ and $t\in [0, T].$

2.2.   Front tracking algorithm and existence

For our BV regularity result established in Theorem 3.1, we employ approximate solutions constructed using a wave front tracking algorithm. The front tracking algorithm can be summarized as follows:

${\bf{Step-I}}$ Approximate the initial data $u_0$ by a piecewise constant function $u^{\delta}_0$ with finitely many discontinuities such that $u_0^ \delta \rightarrow u_0$ in $L^1_{loc}(\mathbb{R})$ as $\delta \rightarrow0$ and

for some $C > 0.$

${\bf{Step-II}}$ Approximate $A(x, u)$ by $A^{\delta}(x, u)$ such that $x\mapsto A^{\delta}(x, \cdot)$ is piecewise constant with finitely many discontinuities and for fixed $x$, the function $u \rightarrow A^{\delta}(x, u)$ is piecewise linear convex such that $A^ \delta(x, u) \rightarrow A(x, u)$ for a.e. $x\in \mathbb{R}.$ In addition, the stationary states $k^{\delta, \pm}_{\alpha}$ corresponding to the flux $A^{\delta}$ should satisfy $k_{\alpha}^{\delta, \pm }(x) \rightarrow k_{\alpha}^{\pm}(x)$ for a.e. $x\in \mathbb{R}$.

${{\bf{Step-III}}}$ Solve the following initial value problem,

and denote the solution by $u^{\delta}$. More specifically, at each discontinuity point of $u^ \delta_0$, and at each discontinuity point of $x \mapsto A^{\delta}(u, x)$, use the Riemann solver to obtain a solution $u^{\delta}(\cdot, \tau)$ until the time $\tau$ at which the a discontinuity (front) of $u^{\delta}$ interacts with other discontinuities of $u^{\delta}$ or with the spatial discontinuities of the flux $A^{\delta}$. Solve the initial value problem (IVP) given by Eq (2.3), at this interaction time $t = \tau$, using $u^{\delta}(\cdot, \tau)$ as the initial data. Repeat this procedure until the next interaction, and continue in this manner.

Theorem 2.2. Suppose $u_0 \in {\rm{BV}}(\mathbb{R}) \cap L^1(\mathbb{R})$ and $A(\cdot, \cdot)$ satisfies the assumptions (A-1)–(A-3). Then, there exists a unique adapted entropy solution to Eq (1.1) corresponding to the initial data $u_0$.

Proof. The proof can be done as in ^[22]. Since $A(x, \cdot)$ is uniformly convex, the proof is even simpler. Due to the strict convexity assumption, the number of fronts does not increase at any interactions (interactions in the interior as well as on the interface). This implies that the front tracking approximations can be continued for all time $t > 0.$ On the other hand, the singular map $\Psi$ is invertible and the TVD property of $\Psi(\cdot, u(t, \cdot))$ gives the desired compactness. Thus, repeating the arguments in ^[22], the entire sequence of front tracking approximations converge to the unique entropy solution. □

3.   Study of BV regularity

This section is devoted to the study of BV regularity of adapted entropy solution. In the next subsection, we introduce a condition on initial data to get a TV bound on entropy solution when the fluxes are uniformly convex. Later, we give two counter-examples to show that neither the additional condition on initial data ($\Lambda(u_0) < \infty$) nor the uniform convexity assumption on flux can be relaxed.

3.1.   Regularity in BV space

To obtain a BV regularity of entropy solutions, we need an assumption on initial data which is stronger than BV. This is introduced below:

Let $b\in L^ \infty(\mathbb{R})$ and $- \infty < x_1 < \cdots < x_{n+1} < \infty$. We define a sequence $\{ \Delta w_j\}_{1\leq j\leq n}$ as follows,

for $1\leq j\leq n$. We define $\Lambda(b)$ as follows,

and the set $X: = \{b\in L^{ \infty}(\mathbb{R}); \, \Lambda(b) < \infty\}$. Since $u_M\in {\rm{BV}}(\mathbb{R})$, we observe that $X\subset {\rm{BV}}(\mathbb{R})$. If $x\mapsto A(x, \cdot)$ is piecewise constant then Eq (3.2) can be simplified in the following way: let $- \infty = z_0 < z_1 < \cdots < z_n < z_{n+1} = \infty$ be discontinuity points of $x\mapsto A(x, u)$. We can write

Remark 3.1. Suppose $u_M\in {\rm{BV}}(\mathbb{R}),$ then $\Lambda(b) < \infty$ implies ${\rm{TV}}(b) < \infty$ but the converse is not true. Furthermore, if $A$ is homogeneous then $\Lambda(b) = {\rm{TV}}(b).$

Theorem 3.1. Let $A(\cdot, \cdot)$ satisfy (A-1)–(A-3). Additionally, we assume that $A(\cdot, x)\in C^2(\mathbb{R})$ and $\partial_{uu}A(\cdot, x)\geq C_1$ for all $x\in \mathbb{R}$ where $C_1 > 0$ does not depend on $x$. Let $u$ be an adapted entropy solution to Eqs (1.1) and (1.2) with initial data $u_0$ such that $\Lambda(u_0) < \infty$ where $\Lambda$ is defined as in Eq (3.2). Then we have

${ where }\; C_2 = \sup\{\|A(\cdot, x)\|_{C^2[-M, M]}, \, x\in \mathbb{R}\}$ and $C_3: = \left(\frac{C_2}{C_1}\right)^{\frac{3}{2}}$.

Before the proof of Theorem 3.1, here we prove a property of uniform convex flux in the following lemma.

Lemma 3.1. For a closed interval $I \subset \mathbb{R},$ let $f, g\in C^2(I)$ such that $f^{ \prime \prime}, g^{ \prime \prime}\in[C_1, C_2]$ for $C_2 > C_1 > 0$. Additionally, assume that $f(\theta_f) = g(\theta_g) = 0$. Then we have

Proof. Suppose $f(u_1) = g(u_2)$ with $u_1 > \theta_f, u_2 > \theta_g$. Now, we observe that

Subsequently, we have

Therefore, we have

Notice that

Hence, we have

□

Proof of Theorem 3.1. We prove the estimate for the approximate solution $u^ \delta$, and then we show that the estimates are uniform over $\delta$. Let $u^ \delta$ be the approximate solution to Eq (1.1) obtained via wave front tracking. Note that there is no affine part on $A(\cdot, x)$ for all $x\in \mathbb{R}$ due to $\partial_{uu}A(x, u)\geq C_1$. Due to uniform convexity no new state arises away from the interface for time $t > 0$. Since there is no linear part in the flux, no rarefaction wave occurs at the interface for $t > 0$. Therefore, for a time $t_0 > 0$ and $x\in \mathbb{R}$, there exists $y(x)\in \mathbb{R}$ such that $A^ \delta(u^ \delta(x, t_0), x) = A^ \delta(u_0^ \delta(y(x)), y(x))$. Suppose $- \infty = x_{0} < x_{1} < \cdots < x_{m} < x_{m+1} = \infty$ are discontinuity points of $x\mapsto A(\cdot, x)$. Let $z_1, z_2\in(x_i, x_{i+1})$ for some $0\leq i\leq m$. Now, there are two cases

1. $y(z_1), y(z_2)\in (x_j, x_{j+1})$ for some $0\leq j\leq m$. Then by Lemma 3.1 we have

2. $y(z_1)\in (x_j, x_{j+1}), y(z_2)\in(x_{k}, x_{k+1})$ for some $0\leq j\neq k\leq m$. Then by Lemma 3.1 and the triangle inequality, we have

Suppose $z\in(x_{i}, x_{i+1})$ for $0\leq i\leq m-1$, then by Lemma 3.1, we have

Combining Eqs (3.3), (3.4) and (3.5) with definition Eq (3.2) of $\Lambda$ we conclude Theorem 3.1. □

3.2.   Counterexample-Ⅰ

In this section, we wish to construct an example of an adapted entropy solution such that $u(\cdot, 1)\notin {\rm{BV}}_{loc}(\mathbb{R})$ for a flux satisfying (A-1)–(A-3) and an initial datum $u_0\in {\rm{BV}}(\mathbb{R})$ with $\Lambda(u_0) < \infty$. We also assume that the flux possesses $C^2$ regularity in the state variable, that is, for a.e. $x\in \mathbb{R}$, $u\mapsto A(x, u)$ is a $C^2$-function (but not necessarily uniformly convex). Note that this result is special for fluxes having infinitely many spatial discontinuities since for a flux $A$ with finitely many discontinuities and satisfying (A-1)–(A-3), we have $TV(u(\cdot, t)) < \infty$ for BV initial data (see ^[16]).

Proposition 3.1. There exists a flux $A$ satisfying (A-1)–(A-3) and an initial datum $u_0$ such that $\Lambda(u_0) < \infty$ such that the corresponding entropy solution to (1.1) has TV blow up at some finite time $T_0 > 0$.

Construction: Let us consider two sequences $\{y_n\}_{n\geq0}$ and $\{z_n\}_{n\geq 1}$ such that they satisfy

Now we consider the following flux,

where $f_n, g_n$ are defined as follows

Note that $u\mapsto A(x, u)$ is a $C^2$-function for a.e. $x\in \mathbb{R}$. Hence (A-1) is satisfied. Observe that $A(x, u)$ satisfies (A-2), with $h_1(u) = u^4, h_2(u) = 2u^4$ and $u_M\equiv0$. Note that (A-3) is verified for $A(x, u)$ with the following choice of $\eta, a$

Loosely speaking, $\left| {(g_n)_+^{-1}(f_n(u))}\right|\geq u^{\frac{1}{2}}n^{-\frac{3}{8}}$ gives a possible blow up in the solution.

We consider an initial datum defined as follows,

We observe that rarefaction wave arises at $x_n^1$ and shock wave is generated at $x_n^2$ with shock speed $\lambda_n$ where $\lambda_n$ is calculated as follows

Assume that at time $t = t_n$ the shock curve hits the interface; therefore, we have $x_n^2+t_n \lambda_n = z_n$. Since the speed of the extreme right characteristic of the rarefaction wave $f^{ \prime}_n(a_n)$ is bigger than $a_n$, we need to give sufficient gap between $x_n^1$ and $x_n^2$ such that before time $t = 1$ it does not hit the shock wave and the interface at $z_n$. Hence, the following inequality must hold:

Now after the shock wave from $x_n^2$ hits the interface at $z_n$ it generates another shock wave from $(z_n, t_n)\in \mathbb{R}\times \mathbb{R}_+$. Note that this new shock wave has states $b_n, 0$ on its left and right respectively where $b_n = (g_n)_+^{-1}(f_n(a_n))$. We want this shock wave not to meet the interface at $y_n$ before time $t = 1$. Hence we must have

where $\xi_n$ is the shock speed between $b_n, 0$, that is,

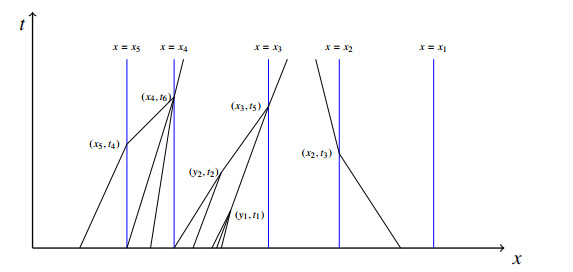
If we are able to choose such $x_n^1, x_n^2, z_n, y_n$ then the solution has the following structure up to time $t = 1$, (see Figure 2)

1. For $t\in[0, t_n]$ we have

where $\lambda_n$ is defined as in Eq (3.8).

2. For $t\in[t_n, 1]$ we have

where $\xi_n$ is defined as in Eq (3.11) and $b_n$ is defined as follows,

Finally, we choose

where $\delta > 0$. Next we choose

Note that

Note that $x_n^2-x_n^1 = f^{ \prime}_n(a_n)$. Hence, we have $z_n-x_n^1 = z_n-x_n^2+x_n^2-x_n^1 > f^{ \prime}(a_n)$ since $z_n-x_n^2 > 0$. Therefore, Eq (3.9) is satisfied. Observe that $y_n-z_n = 2((g_n)_+^{-1}(f_n(a_n)))^{3} = 2\xi_n > \xi_n(1-t_n)$ since $\xi_n > 0$ and $0 < 1-t_n < 1$. Hence, the condition (3.10) is satisfied. Now we note that

Thus, there exists an $y_{ \infty}$ such that $y_n \rightarrow y_{ \infty}$. Set $z^* = y_{ \infty}$ and $y_0 = 0$ in Eq (3.7).

Note that $TV(u_0^n)\leq n^{-1- \delta}$ and $TV(u^n(\cdot, 1))\geq \left| {b_n}\right|$. Observe that

Note if we take an initial datum $u_0{(x)} = \sum\limits_{k = 1}^{ \infty}u_0^k {(x)\mathbb{1}_{(y_{k-1}, y_k)}(x)}$, then $u{(x)} = \sum\limits_{k = 1}^{ \infty}u_k {(x)\mathbb{1}_{(y_{k-1}, y_k)}(x)}$ will be the adapted entropy solution to Eq (1.1). Since $TV(u_0^k)\leq k^{-1- \delta}$ and $\mathrm{supp}(u_0^k), k\geq1$ are mutually disjoint, we have $TV(u_0) < \infty$. We observe that $\mathrm{supp}(u^k(\cdot, 1)), k\geq1$ are mutually disjoint. Therefore, we have,

Now we choose, $\delta\in(0, 4^{-1})$. Then, we have $\frac{7}{8}+\frac{ \delta}{2} < 1$. Hence, $TV(u(\cdot, 1)) = \infty$.

3.3.   Counterexample-Ⅱ

In Section 3.2, we presented an example exhibiting a ${\rm{BV}}$ blow-up of entropy solutions corresponding to an initial datum $u_0$ for which the quantity $\Lambda(u_0)$ is finite but the flux as a function of the state variable is not necessarily uniformly convex. In contrast to the previous section, we now construct an example starting from ${\rm{BV}}$ initial data and a uniformly convex flux such that the entropy solution has a TV blow-up at some finite time $T$. More precisely, we construct a flux and a ${\rm{BV}}$ initial data for which the quantity $\Lambda(u_0)$ is not finite.

Proposition 3.2. There exists a flux $A$ satisfying (A-1)–(A-3) and an initial datum $u_0$ such that $\partial_{uu}A(x, u)\in[C_1, C_2]$ for some $C_2 > C_1 > 0$ and the corresponding entropy solution to Eq (1.1) has TV blow up at some finite time $T_0 > 0$.

Proof. We start by constructing a flux $A$ satisfying conditions (A-1)–(A-3) and the initial datum, for which the above proposition holds true: Let $g(u) = u^2$ and for any $n\in\mathbb{N},$ let

which implies that

and

Hence $f_n\in C^2(\mathbb{R})$ for $n\geq 1$. Note that there exists $n_0\in\mathbb{N}$ such that $f^{ \prime \prime}_n(u)\geq 1$ for $n\geq n_0$. Suppose $\{a_n\}, \{b_n\}$ are two sequences such that $0 < a_{n+1} < b_{n+1} < a_n$. We consider a flux $A$ as follows

We choose $a_n = \frac{1}{n^{2/3}}$ and $b_n = a_n+\frac{1}{n^{2n}}$. We approximate $A$ by $A^N$ as follows

By the choice of $f_n(u) = g(u)$ for $\left| {u}\right|\geq n^{-2/3}$. Consider the following data

Now, we analyze the characteristics. We track the paths traveled by the characteristics emanating from $x = 0, t = 0$ to get structural information about adapted entropy solution to Eq (1.1) corresponding to flux $A^N$ and data $u_0$ as in Eq (3.13).

Let $u^N$ be the adapted entropy solution to Eq (1.1) with flux $A^N$ and data $u_0$. Note that initially a rarefaction arises at $x = 0$ and then it travels through the interfaces, and there is no interaction between waves. We observe that since $n_0\geq 4$, $f_n = g = u^2$ for $u = 1$, no wave arises from interfaces at $t = 0+$ for $x > 0$. Let $L_N:[0, 2] \rightarrow \mathbb{R}$ be the path traveled by the characteristic arising from $x = 0$ with speed $2w_N$ with $w_N: = (N+1)^{-2/3}$.

Note that $L_N$ is a piecewise linear curve originating from $x = 0$ (see Figure 3). We get $L_N(0) = 0$ and wish to estimate $L_N(1)$. Let $v_{N, k}\in[0, \infty)$ be defined such that $g(w_N) = f_m(v_{N, k})$. Suppose $L_N(t)\in [a_k, b_k]$ for $t\in[t_k, t_k']$. Therefore, we have $f^{ \prime}_k(v_{N, k})(t_k'-t_k) = b_k-a_k$. Suppose $t^{ \prime \prime}_k > t_k$ is such that $b_k-a_k = (t^{ \prime \prime}_k-t_k)g^{ \prime}(w_N)$. We obtain

Subsequently, we have

Let $L(\tilde{t}_N) = a_{N/2}$; then we have

Note that $\frac{1}{(N+1)^{2/3}}\geq \frac{1}{2(N/2)^{2/3}}$ for $N\geq n_1$ for some $n_1 > 1$. Subsequently, we have $\frac{k^{2/3}}{(N+1)^{2/3}}\geq \frac{1}{2}$. Now we observe that

Hence, we get $\frac{w_N}{2}\leq v_{N, k}$ for $N/2+1\leq k\leq N$. Since $(1-k^{4/3}u^2)^3\geq 0$ for $\left| {u}\right|\leq k^{-2/3}$ we have $f_k(w_N)\geq f_k(v_{N, k})$, therefore $w_N\geq v_{N, k}$. This shows that

Hence,

Therefore,

Let $u^m$ be the entropy solution to Eq (1.1) with data $u_0$ as in Eq (3.13) and flux $A^m$. It will be now shown that the adapted entropy solution $u^m$ agrees with $u^N$ in some subset of $\mathbb{R}\times[0, 1]$ for sufficiently large $m$. Due to the fact that $f_m = g$ for $u\geq (N+1)^{-2/3}$, then characteristic with speed $2(N+1)^{-2/3}$ travels through $L_N(1)$ for $m\geq N+1$ and we observe the following:

where $D_N$ is defined as (see Figure 3)

We now calculate the variation $\left| {u^N(a_m+, 1)-u^N(a_m{-}, 1)}\right|$, where $u^N$ is the adapted entropy solution to Eq (1.1) corresponding to flux $A^N$ and data $u_0$.

Let the characteristic corresponding to $u = \frac{1}{2m^{2/3}}$, originating from $x = 0$ move through path $Q(t)$. Note that $Q(t)$ is analogous to $L(t)$. By a similar argument as in Eq (3.15), we obtain

Suppose $Q(t)$ hits $x = b_{m-1}$ and $x = a_m$ at time $t = \tilde{t}_1$ and $t = \tilde{t}_2$ respectively. Suppose $u^N(a_m-) = \tilde{w}_N$, then we have,

Since $\tilde{t}_2\geq1$ we get $w_N\leq \tilde{w}_N$. Then we have

Note that

By a similar argument as in Eq (3.14) we have

Hence, from Eq (3.17), we obtain

Then, we get

For sufficiently large $m$, that is there exists an $m_0 > 1$ such that $1-\frac{12B}{m^{2m}}\geq \frac{1}{2}$ for $m\geq m_0$. Therefore, $\tilde{w}_m\leq 2{w}_m$. Hence, we get

Suppose $f_m(\tilde{v}_m) = g(\tilde{w}_m)$. Note that there exists $m_1 > 1$ such that $\left| {\tilde{w}_m-w_m}\right|\leq \frac{1}{6m^{2/3}}$. Then we note that $\frac{1}{3m^{2/3}}\leq \tilde{w}_m\leq \frac{1}{2m^{2/3}}$. Subsequently, we get

Thus, we get $\tilde{w}_m\geq \tilde{v}_m$. Note that

Hence, $1/3\leq \tilde{v}_mm^{2/3}\leq 1/2$. Therefore,

Hence,

Therefore, we obtain for $N\geq 2m+1$,

Finally, we show that $u^m$ converges to an adapted entropy solution to Eq (1.1) for initial data $u_0$ defined as in Eq (3.13) as $m \rightarrow \infty.$ Note that $\left|\left| {u^N-u^m} \right|\right|_{L^1(\mathbb{R}\times[0, 1])}\leq \tilde{B}N^{-2/3}$ for all $m\geq N$. Therefore, $\{u^m\}_{m\geq1}$ is a Cauchy sequence in $L^1(\mathbb{R}\times[0, 1])$. Hence, there exists a $u\in L^1(\mathbb{R}\times[0, 1])$ such that $u^m \rightarrow u$ in $L^1(\mathbb{R}\times[0.1])$. We also note that $A^N(x, \cdot) = A(x, \cdot)$ for $x\in \mathbb{R}\setminus[0, b_N]$. This implies $k^\pm_{ \alpha, N}(x) = k^\pm_ \alpha(x)$ for $x\in \mathbb{R}\setminus[0, b_N]$ and we get $k_{ \alpha, N}^\pm \rightarrow0$ as $N \rightarrow \infty$. Since $u^N$ is an adapted entropy solution, $u^N \rightarrow u$ in $L^1(\mathbb{R}\times[0, T])$, $k^\pm_{ \alpha, N} \rightarrow k^\pm_{ \alpha}, A^N(x, u) \rightarrow A(x, u)$ for a.e. $x\in \mathbb{R}$, we get $u$ as the adapted entropy solution to Eq (1.1) for data $u_0$ as in Eq (3.13) with flux $A(x, u)$. By using Eq (3.16), we note that $u(x, t) = u^N(x, t)$ for $(x, t)\in D^N$. From Eq (3.18) we get

Hence we have $TV(u(\cdot, 1)) = \infty$.

□

Remark 3.2. Let $u_0$ be defined as in Eq (3.13). Due to the finite speed of propagation we can consider a data $\tilde{u}_0 = u_0\chi_{[-M, M]}$ for some large $M > 0$ and the solution gives a TV blow up at time $t = 1$.

Use of AI tools declaration

The authors declare that they have not used Artificial Intelligence (AI) tools in the creation of this article.

Conflict of interest

The authors declare that there are no conflicts of interest.

Acknowledgement

SSG and GV would like to thank the Department of Atomic Energy, Government of India, under project no. 12-R & D-TFR-5.01-0520 for support. SSG acknowledges the Inspire faculty-research grant DST/INSPIRE/04/2016/000237. A part of this work was carried out during the GV's tenure of the ERCIM 'Alain Bensoussan' Fellowship Programme at NTNU.