1.
Introduction
Let Ω⊂Rn (n≥2, possibly n=1971) be a bounded smooth domain and consider the problem
where c∈R is a constant and ν denotes the outward unit normal to ∂Ω. Imposing both Dirichlet and Neumann conditions on ∂Ω makes the problem overdetermined so that, in general, (1.1) has no solution. In a celebrated paper published in 1971, Serrin [15] proved that if (1.1) admits a smooth solution, then Ω must necessarily be a ball. This paper has raised a great interest and, nowadays (half a century later), it has reached almost 600 citations in the Mathscinet. Serrin's original proof combines analytic arguments, such as the Maximum Principle and a refinement of Hopf's boundary Lemma, with geometric techniques such as the moving plane method inspired to the Alexandrov characterization of spheres [1,2]. Starting from [18], several different approaches have been devised as an alternative to Serrin's original proof, see [14] and the references therein for a fairly complete survey.
The moving plane method has been fruitfully used in symmetry results for semilinear elliptic equations, see [4,10] for second order equations and [3] for higher order problems. Let B⊂Rn be the unit ball and consider the semilinear polyharmonic problem under Dirichlet boundary conditions:
Here r=|x| denotes the radial variable and, hence, the outward normal direction to ∂B. The following result, valid for second order equations with m=1, is a restatement of [10,Theorem 1], combined with deep remarks by Spruck [10,Remark 1].
Theorem 1.1. ([4,10]) Let n≥2 and m=1. Assume that
Then, every positive strong solution u∈W2,nloc(B)∩C(¯B) (u>0 in B) of (1.2) is radially symmetric (u=u(r)) and u′(r)<0 for all r∈(0,1). Moreover, if
then every nonnegative and non-trivial strong solution u∈W2,nloc(B)∩C(¯B) (u≥0, u≢0 in B) of (1.2) is radially symmetric (u=u(r)) and u′(r)<0 for all r∈(0,1).
This result was extended in [3,Theorem 1] to the case m≥2, in the following form:
Theorem 1.2. ([3]) Let n≥2 and m≥1. Assume that
Then, every nonnegative and non-trivial strong solution u∈Hm0(B)∩L∞(B) (u≥0, u≢0 in B) of (1.2) is radially symmetric (u=u(r)) and u′(r)<0 for all r∈(0,1).
In its original version, Theorem 1.2 was stated by requiring that the solution u was strictly positive, u>0 in B. But this is not necessary since [9,Theorem 5.1] ensures that if u≥0 is nontrivial, then u>0 (recall that f(u)≥0 in view of (1.5)). Assumption (1.5) is stronger than (1.3) and one may wonder whether Theorem 1.2 also holds under weaker assumptions. A nice example by Sweers [16] shows that, for a smooth and decreasing f, positive radial solutions may not be radially decreasing. Therefore, (1.3) is not enough to obtain the full statement of Theorem 1.1 (radial symmetry and monotonicity) in the higher order case m≥2. This discrepancy of assumptions between the cases m=1 and m≥2 is due to the lack of a maximum principle for polyharmonic operators; see [9,Section 1.2].
To find conditions, different from (1.5), ensuring both radial symmetry and radial monotonicity for (1.2) in the case m≥2 is a challenging problem. The first purpose of this paper is precisely to discuss some conditions on the source f which ensure the radial symmetry of the solutions of (1.2) and their radial monotonicity. In Section 2, particular attention is devoted to the regularity and monotonicity of f: for the biharmonic equation in B⊂Rn, in Theorem 2.3 we exhibit a Hölder-continuous function f for which the radial symmetry of solutions fails, while Proposition 2.4 displays a strictly decreasing and sign-changing f that still ensures the existence of a radially symmetric and strictly decreasing solution.
Contrary to the case of Navier boundary conditions, considered in [17], (1.2) cannot be reduced to a second order system when m≥2. This is why the moving plane procedure used in the proof of Theorem 1.2 was carried on by using fine estimates of the Green function G associated to the polyharmonic operator (−Δ)m, see [3,Section 2]. The second purpose of the present paper is to obtain new properties of the Green function in the so-called conformal dimensions n=2m. In Section 3 we prove that some estimates become explicit identities in conformal dimensions, see formula (3.6). Combined with the inversion in the sphere and the Kelvin transform, this enables us to obtain an elegant symmetry property of the Green function, see Theorem 3.2 and the sketchy representation in Figures 3 and 4. In turn, this result is used in Corollary 3.5 where we present an alternative formula for the computation of the partial derivatives of solutions of (1.2): since the sign of these partial derivatives is the fundamental feature to implement the moving plane procedure, this formula can become useful under suitable assumption on the source f. Indeed, based on this formula, Proposition 3.7 suggests that the radial symmetry and monotonicity of the solutions of (1.2) is ensured if ‖f′‖L∞(R+) is small.
Finally, as an application of Proposition 3.7, in Section 4 we revisit the counterexample by Sweers [16] where radial monotonicity of the solution fails: by appropriately modifying the source f (enforcing the conditions given in Proposition 3.7) we numerically obtain radially symmetric and strictly decreasing solutions of the biharmonic equation in the unit ball of R4 for a decreasing function f with sufficiently small derivative. Throughout this work, some open problems and questions are posed.
2.
Assumptions ensuring radial symmetry and monotonicity
In this section we discuss radial properties (symmetry and monotonicity) of solutions of (1.2). Let us recall the weak formulation of (1.2) within the Sobolev space Hm0(B), which is a Hilbert space if endowed with the scalar product
We denote the induced norm by ‖⋅‖m; in particular, ‖⋅‖0 is the L2(B)-norm. A function u∈Hm0(B) is called a weak solution of (1.2) if f(u)∈H−m(B) (the dual space of Hm0(B)) and (1.2) is satisfied in a weak sense, that is
where ⟨⋅,⋅⟩ stands for the duality product between H−m(B) and Hm0(B). In the present article, however, we will mostly deal with slightly more regular solutions: when f is continuous and u∈Hm0(B)∩L∞(B), we say that u is a strong solution of (1.2) if
the integral exists since u∈L∞(B) and f is continuous. By elliptic regularity, any such strong solution u belongs to C2m−1,α(¯B), for some α∈(0,1), and all partial derivatives of order less than m vanish on ∂B. Moreover, if f is Hölder continuous, then u∈C2m,α(¯B) is a classical solution, see [9]. In the sequel, we always take (at least) f∈C(R;R).
Our first (elementary) result is a restatement of [3,Remark (iv)] and gives a different condition for radial symmetry (but not for radial monotonicity).
Theorem 2.1. Let n≥2 and m≥1. Let λ1 be the first Dirichlet eigenvalue for (−Δ)m in B. If
then there exists at most one strong solution u∈Hm0(B)∩L∞(B) of problem (1.2) which is, moreover, radially symmetric.
Proof. By contradiction, assume that (1.2) admits two strong solutions u1,u2∈Hm0(B)∩L∞(B). Then by (2.1) we have both that
Set w≐u1−u2, subtract these two equations and choose v=w as a test function to obtain
By (2.2) we know that
with strict inequality in a set of positive measure since w≢0 (recall u2≢u1). Therefore, (2.3) yields
which contradicts the Poincaré inequality and proves uniqueness.
Once uniqueness is established, it suffices to remark that if u is a nonradial solution of (1.2), then for any given nontrivial rotation A∈SO(n), also the function uA≐u∘A is a (different) strong solution of (1.2), which contradicts the just proved uniqueness statement.
We have so shown that, if (2.2) holds, then there exists at most a unique solution of (1.2) which is necessarily radially symmetric. This proves the theorem.
Theorem 2.1 states that one cannot expect radial monotonicity under the sole assumption (2.2). Note also that Theorem 2.1 does not require the positivity of the solution and its elementary proof is based on the invariance properties of polyharmonic operators under rotations, a fact that ensures uniqueness. Let us recall that symmetry is also ensured if f′(s)<λ2, for all s∈R (with λ2 being the second Dirichlet eigenvalue for (−Δ)m in B, see [6]), and uniqueness is guaranteed if f∈C(R+;R) is sublinear at 0 and +∞ (see [7]). All this raises a natural question:
Problem 2.2. Is radial symmetry related to uniqueness? Are there examples of multiple radial positive solutions? Except, of course, when f(u)=λ1u!
Some attention also deserves the regularity of f, as this feature is related to the existence of non-radial solutions. The counterexample in [10,Remark 1] shows that Theorem 1.1 (m=1) does not hold if the Lipschitz continuity assumption on f is relaxed to Hölder continuity. We extend this example to the biharmonic equation (m=2), showing that the monotonicity requirement in (1.5) cannot be dropped even in an arbitrarily small interval. We only deal with the biharmonic operator since the amount of computations grows very quickly as m increases.
Theorem 2.3. Let n≥2. For every ε>0 there exists a Hölder-continuous function gε:R+⟶R such that
and such that the problem
admits both
− a radial solution (u=u(r)) in C4(B), nonnegative (but not strictly positive) and radially decreasing (but not strictly radially decreasing): namely, u(r0)=0 and u′(r0)=0 for some r0∈(0,1);
− infinitely many nonnegative nontrivial solutions in C4(B) which are not radially symmetric.
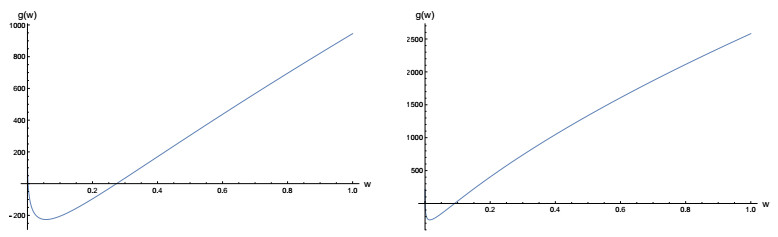
Proof. For any p>4 define the function g:R+⟶R by
Then g∈C0,1−4p([0,∞);R)∩C∞((0,∞);R) and
The quantities between brackets in both the expressions of g(w) and g′(w) can be seen as second order polynomials in the variable w1/p. In particular, there must exist finite numbers M=M(n,p)>0, Γ1=Γ1(n,p)>0, Γ2=Γ2(n,p)∈(0,Γ1) such that
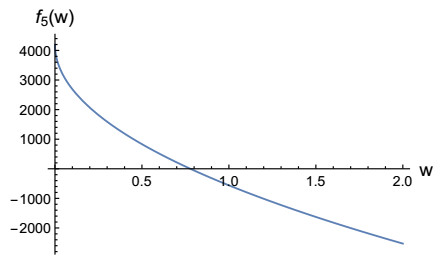
Two plots of g, for given values of p>4 and n≥2, are displayed in Figure 1.
Since m=2, the Eq (1.2) in radial coordinates becomes
where the conditions u′(0)=u‴(0)=0 are needed to ensure the smoothness of u in B, see [8]. By putting u(r)≐(1−r2)p, for r∈[0,1], one sees that such u satisfies u(1)=u′(1)=0 (fulfilling the Dirichlet boundary conditions) and also the equation in (2.6), where g(u(r)) is as in (2.4). Hence,
is a C4(¯B)-positive, radial and strictly radially decreasing solution (u′(r)<0 in (0,1)) of the problem
In order to obtain the statement, we need some rescaling. First we extend u to all Rn by setting
so that u∈C4(Rn) is nonnegative and nontrivial (but not strictly positive), radial and radially decreasing (but not strictly decreasing). We now argue as in [10,p. 220]: take any point x0∈Rn such that |x0|=3 and consider the function ˆu∈C4(Rn) defined as
which is a nonnegative solution of the problem
where B5⊂Rn is the (open) ball of radius 5 centered at the origin of Rn, and g is given by (2.4). Nevertheless, ˆu is not radially symmetric!
Then we rescale the problem as follows. For u=u(r) as in (2.7) and for some γ>0, define v(r)≐u(5r)/γ for r∈[0,1], so that
and v solves
Finally, fix ε>0 and let us construct the function gε in the statement. Choose γ0>0 such that
Then gε≐gγ0 satisfies all the assumptions of the statement and the function v is a radial solution, nonnegative but not strictly positive (since it vanishes in B∖B1/5), and radially decreasing but not strictly radially decreasing (since it is constant in B∖B1/5). Moreover, after rescaling the function ˆu in (2.8), we obtain infinitely many nonnegative nontrivial solutions which are not radially symmetric, one for each x0 and, possibly with multiple bumps.
The next result shows that the monotonicity assumption in (1.5) is not necessary to obtain the statement of Theorem 1.2. For n≥2, consider the function
which is strictly decreasing and sign-changing, see Figure 2 below.
However, we can prove
Proposition 2.4. Let fn be as in (2.9). The unique strong solution of the problem
is given by
and, hence, it is positive, radially symmetric and strictly decreasing in the radial variable.
Proof. The function fn defined in (2.9) satisfies the assumption (2.2) so that, by arguing as in the proof of Theorem 2.1, we infer that (2.10) admits at most one strong solution, which is necessarily radially symmetric.
In radial coordinates, when m=2, the Eq (1.2) becomes (2.6). The fact that u(r)=(1−r4)2, for r∈[0,1], is a (radial) solution follows by noticing that u(1)=u′(1)=0 (fulfilling the Dirichlet boundary conditions), and then, by inserting the expressions of u(r) and fn(u(r)) into (2.6).
3.
A reflection property in conformal dimensions
We introduce here the Green function of the polyharmonic operator (−Δ)m under Dirichlet boundary conditions in B⊂Rn and we determine some of its properties. Let us firstly define
Then for x,y∈B, with x≠y, Boggio [5,p.126] (see also [9,Section 2.6]) gave the following explicit representation of the Green function:
where kmn is a positive constant defined by
see [9,Lemma 2.27], and H:(0,∞)×[0,∞)→R is defined as
The following statement is a direct consequence of Boggio's work [5], elliptic regularity (see [9,Section 2.5]) and the estimates in [12].
Proposition 3.1. Let h∈L∞(B), and let u∈Hm0(B) be a weak solution of (−Δ)mu=h in B under Dirichlet boundary conditions, that is
Then u∈C2m−1,α(¯Ω) and it satisfies
where Dk stands for any partial derivative of order |k|<2m. In particular, u is a strong solution and Dku≡0 on ∂B for |k|≤m−1.
In conformal dimensions n=2m, the Green function admits a simpler representation. Indeed, the change of variables ξ=1z+1 yields
Therefore, by setting κm≐km2m, for every x,y∈B we have
The main result of this section (Theorem 3.2) shows that, in conformal dimensions, the Green function satisfies an elegant reflection property. For this, given the point x=(x1,x′)∈B with x1>0, we define
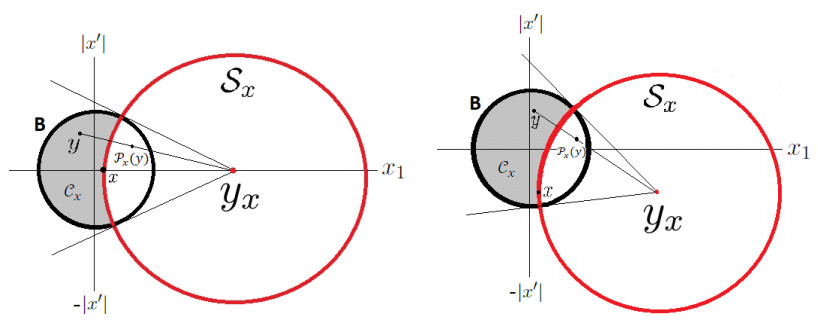
and the set
which is a sphere with center at yx and radius Rx (see the proof of Theorem 3.2 below and also Figure 4). We then denote by Px:Rn∖{yx}⟶Rn the inversion in Sx, given by the expression
Finally, the Kelvin transform of a function h:Cx⟶R with respect to Sx is defined as
Then we prove
Theorem 3.2. For any x=(x1,x′)∈B⊂R2m with x1>0 and any u∈H2m(B)∩Hm0(B) we have
Proof. The following identities hold for the function H defined in (3.3): for all s,t>0 we have
see also [3]. Moreover, for every x,y∈B we have
We then prove the announced symmetry property, namely that the zero level sets of ∂x1G(x,⋅) are spherical caps. Let us denote the zero level set by
By (3.10) and by (3.11) we have
Therefore, ∂x1G(x,y) vanishes if and only if y∈B belongs to the set Sx defined in (3.8). Then we notice that y∈B∩Sx if and only if
Hence, Sx is a sphere with center at yx and radius Rx and the set of negativity of ∂x1G(x,y) is the cap Cx defined in (3.7).
Next, we notice that, by definition of yx and Rx, we have
Then we compute
This computation has two main consequences. First, that the unit sphere ∂B is invariant under the inversion in Sx, that is,
Second, that
We next claim that if Cx is the cap defined in (3.7), then B=Cx∪Px(Cx)∪Lx. To this end, it suffices to show that
Indeed, let y∈Cx, so that Px(y)∈Px(Cx) and |y−yx|>Rx. By definition of Px we have
thus proving that Px(Cx)⊂{y∈B;|y−yx|<Rx}. On the other hand, let y∈B be such that |y−yx|<Rx. Since the function Px is bijective, there exists a point y0∈Rn such that Px(y0)=y. By definition, we then have
implying y0∈Cx, and subsequently, that y∈Px(Cx) and {y∈B;|y−yx|<Rx}⊂Px(Cx).
Since B=Cx∪Px(Cx)∪Lx, by Proposition 3.1, we have
where we used that the Jacobian matrix P′x satisfies
In view of (3.12) and (3.13) we can write
so that
where
To compute I2(x), we first notice that
whereas, for every y∈B we have
After plugging (3.14), (3.17) and (3.18) into I2(x) we get
and, after recalling (3.16), we deduce
A direct computation (see [13,Proposition 7.3]) shows that
which concludes the proof after changing the sign.
Problem 3.3. It is not clear how to derive an expression similar to (3.9) in non-conformal dimensions n≥2, n≠2m. This can be seen after computing
so that the determination of the level sets of ∂x1G(x,⋅) as in (3.12) becomes a delicate task. Is it possible to obtain a representation formula similar to (3.9) in any dimension n≥2?
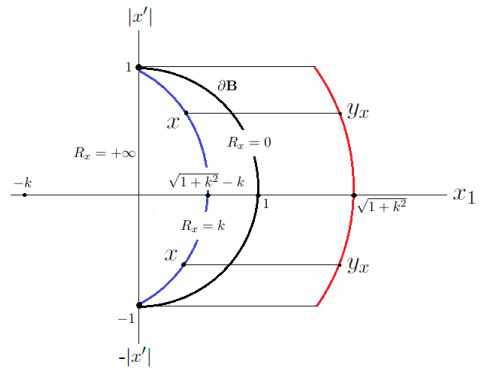
Clearly, a representation formula similar to (3.9) holds for any other directional derivative of u. Note that (3.7) may be rewritten as yx=x+(Rx,0), which implies that yx is an "horizontal translation" of x∈B. Moreover, Rx→0 if x→∂B while Rx→+∞ if x1→0: the limit of the map x↦Rx does not exist when x approaches the equator ∂B∩{x1=0}. The level surfaces of x↦Rx are spheres:
The center of the level-surface sphere is then (x1,x′)=(−k,0) and its radius is √1+k2. For a fixed k>0, when x runs over the level surface Rx=k, the corresponding yx, defined by (3.7), runs over a portion of the sphere centered in the origin with the same radius √1+k2, see Figure 3.
Note also that Cx, the domain of negativity of ∂x1G(x,y), is the intersection between two balls. In Figure 4 we sketch the mutual position of the sphere Sx and the ball B. In particular, we emphasize that
which, again, tells us that yx is exterior to B and it approaches ∂B as x approaches ∂B, while it goes to infinity if x approaches the plane x1=0.
Theorem 3.2 has several relevant consequences. Firstly, we deduce
Corollary 3.4. Let m≥1. For every x∈B⊂R2m such that x1>0, the following identities hold
Proof. We start by noticing that
where n=2m. By induction we then obtain
Consider the polyharmonic version of the original problem (1.1) proposed by Serrin:
A direct application of the binomial expansion yields
that is
Since Um satisfies the Dirichlet boundary conditions in (3.22)2, it is a strong C∞(¯B)-solution of (3.22). Applying identity (3.9) to the function Um and recalling (3.2) (with n=2m), we derive equality (3.20). The identity (3.21) is a straightforward consequence of (3.20), after replacing θ(x,y).
It is remarkable that the right hand side of (3.21) does not depend on x∈B. As a further consequence of Theorem 3.2, we give an alternative formula to compute the partial derivatives of the solutions of (1.2), which should be compared with Proposition 3.1.
Corollary 3.5. Let B⊂R2m be the unit ball. Suppose that f∈C(R;R) and that u∈Hm0(B)∩L∞(B) is a strong solution of (1.2). Then, for every x∈B such that x1>0, the following formula holds:
Formula (3.24) follows directly from (3.9)–(3.19) and suggests the problem:
Problem 3.6. Since the moving plane procedure developed in [3,Section 3] requires that
is it possible to use (3.24) to prove this inequality and to relax assumption (1.5), but still ensuring the statement of Theorem 1.2?
In connection with Problem 3.6 we notice that, by looking at (3.24), it is clear that the overall sign of the integral over Cx is determined by the behavior of the quantity
Fixing x∈B such that x1>0 and u∈Hm0(B), notice that Φx(y)=0 for all y∈∂Cx∩B, while for y∈∂Cx∩∂B (so that Px(y)∈∂B) we have that Φx(y)=0 if f(0)=0, and Φx(y)>0 if f(0)>0.
Under suitable assumptions on the source f, in the next result we give an upper bound for the partial derivative of u (recall that |x|<1!), which shows that ∂x1u(x)<0 for all x∈B located far away from ∂B, with x1≥ε for some ε>0, see Figure 5 for a schematic representation. Therefore, in order to solve Problem 3.6, one should mainly focus the attention on the complement of this region.
To this end, we define
and we state:
Proposition 3.7. Assume that f∈W1,∞(R+;R+) satisfies
and let u∈Hm0(B)∩L∞(B) be a strong solution of (1.2). For any point x=(x1,x′)∈B with x1>0 we have
where Km>0 is defined in (3.21).
Proof. In view of Corollary 3.4, by the Maximum Principle and assumption (3.25), any strong solution u∈Hm0(B)∩L∞(B) of (1.2) satisfies
By embedding theorems and elliptic regularity we also have that
Then, by the Mean Value Theorem, we obtain the inequality
From this we deduce that
thus yielding
and, by using (3.25), we finally obtain
By inserting this bound within (3.24), we get
where
From Corollary 3.4 we infer that
In order to bound I2(x), we notice that
Hence,
From (3.15) and Proposition 3.1, and by proceeding as in the proof of Corollary 3.4, we obtain
After plugging this into (3.28) we deduce that
The conclusion is reached after inserting (3.27) and (3.29) into (3.26).
Proposition 3.7 suggests that the radial symmetry and monotonicity of the solutions of (1.2) can be ensured if ‖f′‖L∞(R+) is small. This assumption is not needed if m=1 (see Theorem 1.1), while for m≥2, the required condition is that f′ be nonnegative (see Theorem 1.2). Therefore, one is led to analyze the cases where the negative part of f′ is small, that is, ‖(f′)−‖L∞(R+) small. This issue is tackled numerically in the next section, for the biharmonic equation in B⊂R4.
4.
On a counterexample by Guido Sweers
As a direct consequence of the Hopf-type lemma by Grunau-Sweers [11,Theorem 3.2] we obtain
Proposition 4.1. Assume that f∈W1,∞(R+;R+) satisfies (3.25), and let u∈Hm0(B)∩L∞(B) be a strong solution of (1.2). Then, there exists γf>0 such that
Guido Sweers [16] provided an explicit example of a non-decreasing radial solution of a linear biharmonic problem in B⊂R2 (equation (1.2) with m=2). In this section we take advantage of his example and give numerical evidence to claim that Proposition 4.1 might be complemented with the statement that γf can be made arbitrarily small (possibly zero) provided that ‖(f′)−‖L∞(R+) is sufficiently small.
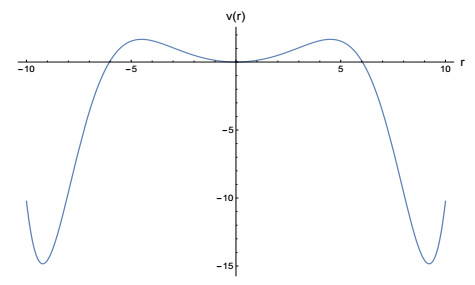
Consider the following radial function defined in R4 (the conformal dimension for the biharmonic operator):
whose plot in the interval [−10,10] is displayed in Figure 6 below.
Since m=2 and n=4, the biharmonic operator in radial coordinates becomes
After noticing that
one readily sees that
Let r0≈9.2218 be the first nonzero local minimum of v; numerically, we find that v(r0)≈−14.8388. Then define the function
which is radially symmetric and strictly positive in [0,1), but not decreasing, in the interval [0,1]; see the left picture in Figure 7 below.
By defining
we observe that f′(w)≡−r40<0, so that f is decreasing and (2.2) is satisfied. By Theorem 2.1 we then know that there exists at most one strong solution of the following problem:
The solution is radially symmetric and a simple computation shows that it coincides with u defined in (4.1). Note that u(0)=|v(r0)| and u(0.5)>16.5>|v(r0)|, so that f(u) is sign-changing in [0,1].
We now modify f in order to reduce ‖(f′)−‖L∞(R+). We take
being γ>0 a variable parameter. Then we consider the problem
and prove the following result:
Proposition 4.2. For every γ>0, the unique strong solution Uγ∈H20(B)∩L∞(B) of (4.2) (which is radially symmetric) is analytic and can be written as
for some coefficients {Ak(γ)}k∈N⊂R that satisfy the following properties:
Moreover, there exists γ0>0 such that, for every γ∈(0,γ0), the function Uγ is positive in [0,1) and strictly decreasing in the radial variable.
Proof. For every γ>0 we notice that the condition (2.2) is satisfied. Then, Theorem 2.1 guarantees the existence of at most one strong solution Uγ∈H20(B)∩L∞(B) to problem (4.2) which is, moreover, radially symmetric and analytic in ¯B. Problem (4.2) in radial coordinates reads:
Upon substitution of (4.3) into (4.4)1 we obtain
which yields the identities
Moreover, the boundary conditions in (4.4)2 imply that
In connection with (3.23) we define
By Corollary (3.4) and by continuous dependence, we have that
in fact, the convergence holds in a stronger norm. Notice that
Thus, by uniform convergence of U_\gamma'' and U_\gamma''' , there exist \delta_{0} \in (0, 1) and \gamma_{0} > 0 such that:
Since U'_{\gamma}(0) = U'_{\gamma}(1) = 0 for every \gamma > 0 , this implies that
Moreover, by uniform convergence of U'_{\gamma} , there exists \gamma_{1} > 0 such that U'_{\gamma}(r) < 0 if r \in [\delta_{0}, 1-\delta_{0}] , for every \gamma \in (0, \gamma_{1}) . Therefore U'_{\gamma} < 0 in (0, 1) whenever \gamma < \min \{ \gamma_{0} \, , \gamma_{1} \} , which also implies that U_{\gamma} is strictly positive in [0, 1) , since U_{\gamma}(1) = 0 .
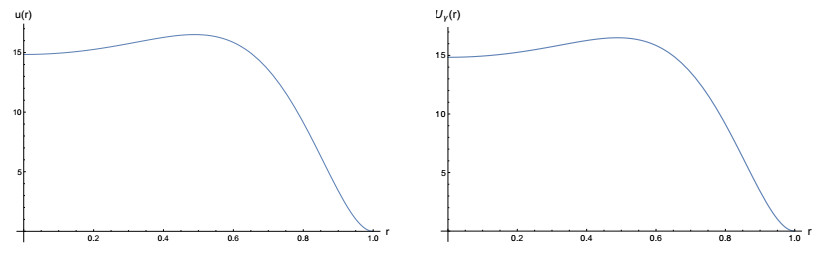
In fact, the function U_{\gamma} is also unique within the class of weak solutions. Proposition 4.2 does not come unexpected, since the solution of (4.4) is closely related to a Bessel function, see [16]. Therefore, the number of critical points of U_{\gamma} is increasing with respect to \gamma and becomes arbitrarily large as \gamma \to \infty , whereas there are no critical points for any sufficiently small \gamma . By taking \gamma = 1 , we numerically obtain the solution displayed in the right picture of Figure 7, which represents the function u in (4.1). Several numerical simulations were performed in order to yield a radially decreasing solution of problem (4.2). The obtained results may be summarized as follows:
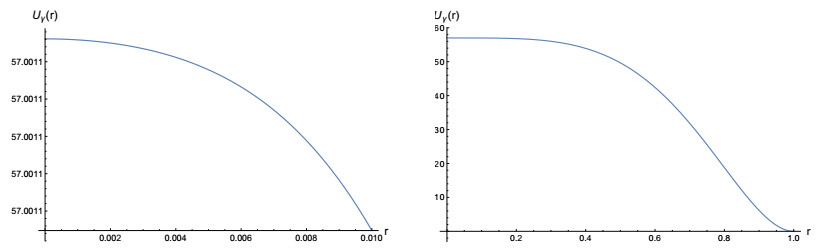
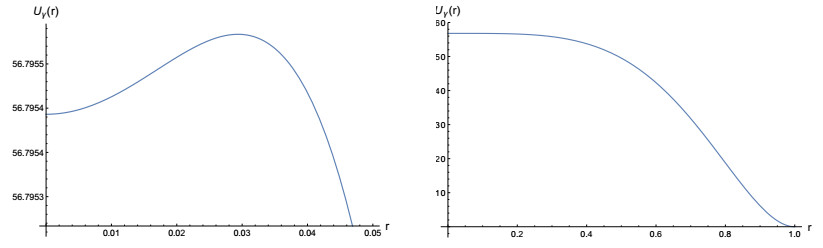
● if \gamma \leq 0.317 , then the associated solution U_{\gamma} of (4.2) is radially symmetric and strictly decreasing in the radial variable in the interval [0, 1] (and therefore, positive), see Figure 8;
● if \gamma \geq 0.318 , then the associated solution U_{\gamma} of (4.2) is radially symmetric but not strictly decreasing in the interval [0, 1] , see Figure 9.
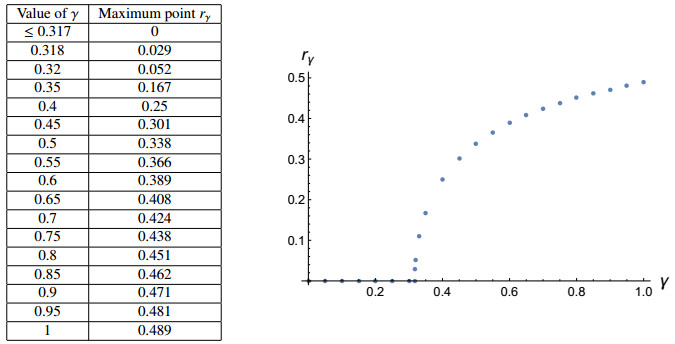
We numerically determined the position of the maximum point r_{\gamma} \in [0, 1] of U_{\gamma} as a function of \gamma \in [0, 1] , see the Table and Figure below. From these data we deduce that the map \gamma \in [0, 1] \mapsto r_{\gamma} is increasing (but not continuous!), giving thus consistency to the discussion following Proposition 4.1. Finally, in connection with the condition (3.25) given in Propositions 3.7 and 4.1, we observe that, for the unique radial solution U_{\gamma} \in H^{2}_{0}({\bf B}) of problem (4.2), the following inequality holds:
From the Table and Figure displayed below we further deduce that
recall that U_{\gamma} is nonnegative in [0, 1] for every \gamma \in [0, 0.317] . Numerical experiments yield the value of \gamma = 0.057 as a threshold for ensuring the positivity of the right-hand side of (4.6), in the sense that
Acknowledgments
The first Author is supported by the PRIN project Direct and inverse problems for partial differential equations: theoretical aspects and applications and by INdAM. The second Author is supported by the Research Programme PRIMUS/19/SCI/01, by the program GJ19-11707Y of the Czech National Grant Agency GA\v{C}R, and by the University Centre UNCE/SCI/023 of the Charles University in Prague.
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad: