1.
Introduction
Human activities have contributed significantly to global warming, and the average global temperature is estimated to be 1.0 ℃ higher than in pre-industrial times [1]. In addition to the global climate crisis, the energy crisis, triggered by the recent Russian invasion of Ukraine, exposes the emergency in overcoming challenges [2]. Faced with this, an urgent response to these concerns can be given through transforming the energy matrix to reduce carbon emissions, a significant cause of climate change. Thus, decarbonizing the energy sector and reducing carbon emissions are the major goals of the energy transformation roadmaps [1].
To corroborate estimates of the reduction of greenhouse gases (GHG), the production and use of hydrogen (H2) plays an important role in the energy transition as a supplementary alternative in energy supply by having characteristics such as low energy loss in its transport, contribute to reducing GHG emissions, be renewable, and be stockable with little or no loss [3,4,5]. Moreover, besides acting in the decarbonization of several sectors, H2 can also act as a zero-emission fuel and be used in generating, co-generating, and supplying of electricity [6,7], representing one of the means for a totally decarbonized future [2].
In this sense, hydrogen fuel cell (HFC) technology is very high on the global political agenda since it represents a low-cost storage option for large amounts of energy for long periods and energy transport over long distances [8,9]. In addition, studies indicate that investments in the entire green H2 value chain over the next 30 years will be about $11.7 trillion [10]. The huge amounts of investments will be fundamental for the technological development and commercial strategies in the H2 area, which requires more research and identification of the most relevant technologies [3], such as the creation of H2 storage devices in a safe, light, and compact form for its consolidation [11].
In this context, the analysis of patent documents related to HFCs is relevant as it allows us to follow technological advances through market behavior indicators and prediction of technological trends [12,13]. However, this prediction, which is preceded by mapping and monitoring of technologies, is possible only through the tracking and analysis of patents, which seeks to extract and organize important data, such as the scope of protection, competitors, leading players, and technological improvements achieved [14,15].
Currently, the most usual analyses performed through patents are [16]: 1st) Analysis as to the history of the number of patent applications over time, in which it is possible to extract information regarding the growth or decrease of interest in a technology; 2nd) analysis as to the history of applications by applicant country, which enables the visualization of which branches of research and technological development the countries have or have been active; 3rd) analysis as to the depositor, which makes it possible to see the companies, institutions and/or individuals that lead the development in a particular technological field; 4th) analysis as to the areas of knowledge, as a way to identify which areas are more prominent in research and development (R&D); and 5th) analysis as to the international patent classification (IPC) that makes it possible to select and filter the documents found, which facilitates access to information.
Thus, the analysis of patent documents is an important key for identifying opportunities to develop new products and processes. The possibility of technological trends generates a competitive gain for companies and allows an improvement in decision-making regarding R&D investments by companies, institutions, and countries [17,18].
In searches carried out in major databases, such as, Scopus and Web of Science, using the terms "hydrogen", "forecasting", "fuel cell", and "patent", along with the boolean operators AND and OR, it was possible to retrieve some studies focused on the analysis of HFCs patents. Some of them deal with the analysis of patents related to the development of fuel cell (FC) technologies [8,19,20,21,22,23,24,25]. However, the cited studies use earlier periods (between 1969 and 2010) and are restricted to a few countries, unlike this study that analyzes applications from several nations in the period from 2001 to 2020.
Other studies already conducted deal specifically with analyzing the technological development of HFCs for vehicles based on the analysis of patents [26,27,28,29,30]. There are also works focused on the evaluation of the technological development of H2 storage materials in FCs [11,12], as well as the technological development, through patent analysis, involving the H2 economy in general [31] and H2 production technologies [32].
In the analysis of trends in FC technology, one can notice the presence of studies focused on evaluating the technological development of biohydrogen FCs [14,33]. Even more specific analyses can be observed, in which the technical and commercial evaluation of the technological development of H2 stations is done through the study of patents [3]. Finally, it was possible to observe studies in which there is a technological evaluation, through patent analysis, of the development of solid oxide fuel cells (SOFCs) [34,35].
In a more comprehensive study, using a broad search for patents, the technological development from the analysis of patent documents about the development of HFCs in the period between 2001 and 2020 was not found. In this context, the guiding question of this research arises: "How is the global technological development of HFCs in the first 20 years of the 21st century?". Thus, we aim to map and analyze patents related to HFC technologies from patent applications between the years 2001 and 2020, and then present, in an organized way, information regarding technological developments in this topic.
This study is structured into five sections: The second section is dedicated to a brief review related to the major aspects of HFC technology. In the third section, the research method is described, as well as the search procedures performed in the patent databases. The fourth section presents the results of the patent search, which are analyzed and discussed in light of the theoretical background. The fifth section presents the conclusions, suggestions, and recommendations for future work.
2.
Hydrogen fuel cells
By definition, a FC can be understood as an electrochemical device that receives fuel on one side (input) and emits electricity on the other (output), a process that can take place for an indefinite period of time as long as the cell is supplied with fuel [36]. HFCs are revolutionizing the way energy is produced [37] and present themselves as a highly viable technology compared with existing technologies. Their use as a substitute for the already known fossil fuels generates a green potential capable of attracting interest in the development of new policies and research by a range of companies and institutions [9] because if H2 is the fuel, the by-products of the reaction will only be water, electricity, and heat [38]. Table 1 presents the five major types of FCs, and each of them has its own characteristics, and it is possible to classify them by the type of electrolyte they use in their reactions [37,39].
2.1. Polymer electrolyte membrane fuel cell
Polymer electrolyte membrane fuel cells (PEMFCs) are a source of alternative energy generation with several applications, such as distributed generation, hybrid cars, and domestic consumption. It is the type of cell that is considered the most common, has a high power density, is capable of fast start-up, and can operate at temperatures between 80 and 100 ℃ for use in automotive industries, power plants, portable generators, as well as military applications. The first PEMFC was created in the year 1839 by William Robert Grove, but no practical use was found. As early as 1950, the General Electric Company started FC development and was contracted for the Gemini mission in 1962 [40,41,42].
In the following years, as early as the 1970s, considerable improvements were implemented with the adoption of the fully fluorinated Nafion membrane. However, PEMFC R&D received only more attention decades later, when innovative methods to reduce the amount of platinum required were developed and refined. The transportation sector stands out as the main application of PEMFCS, due to the impact on the environment, such as GHG emission control [40,41,42].
Most automotive companies work with this type of cell because of its high-power density and excellent dynamic characteristics compared to other types of FCs. Automobile manufacturers such as Toyota, Honda, Hyundai, Daimler, and General Motors have presented plans to commercialize FC vehicles. PEMFCs are the core part of vehicles powered by this type of cell and play a decisive role in the development of such vehicles [40,41,42].
2.2. Alkaline fuel cell
Alkaline fuel cells (AFCs) are an important element in energy conversion and are highly efficient for portable as well as stationary applications. In 1902 and 1904, scholars described FCs using aqueous potassium hydroxide as an electrolyte. The current AFC appeared 63 years after the invention of acid FC by Grove. AFCs are easy to handle, exhibit high electrical efficiency, have excellent suitability for alternating loads, and can be built in small compact modules, which have the potential for combination into large power plants. This type of FC is considered one of the most mature technologies in use since the 1960s by the National Aeronautics and Space Administration (NASA), as they provide both electrical power for onboard systems and potable water. Advanced AFC systems have higher electrical efficiencies, around 70%, in generating electricity, and have optimum reliability when run at low current densities [43,44,45,46,47].
Companies such as Apollo Energy Systems, Gaskatel, Hydrocell, and Independent Power Technologies continue to be active in the development of AFC technology for stationary power generation [47]. It is worth noting that AFCs were the first FCs to successfully enter into operation in view of their generation of energy through H2 for commercial use and show the best performance when operating on pure H2 and oxygen [44].
2.3. Phosphoric acid fuel cell
Phosphoric acid fuel cells (PAFCs) represent one of the FC technologies that have been introduced in several countries, with utility in many applications. More than 500 PAFC plants have been installed and tested since 1970. The leading developers of this type of technology are UTC Fuel Cell, Toshiba, and Fuji Electric. PAFC systems feature high reliability, quality, and efficiency of the power delivered. However, despite presenting excellent technical characteristics and growth of PAFC technology in the previous years from 1990 to 2000, between 2000 and 2003 attention was focused on other types of FC technology [43,44,48].
Already in 2004, the amount of PAFC units commissioned for large stationary applications represented about 40% of the total FC units manufactured, compared to 23% for molten carbonate fuel cells (MCFCs) as well as 20% for the PEMFC type. Possibly, PAFC are the most commercially developed cells that operate at intermediate temperatures and use CHP applications with high energy efficiency. The use of PAFC technology has become interesting in hospitals because the water exhaustion temperature makes it possible to use it in object sterilization processes [43,44,48].
2.4. Molten carbonate fuel cell
In the year 1960, researchers reported on a high-temperature FC that operated for six months, employing an electrolyte comprising a mixture of alkali metal carbonates contained in a magnesium oxide disk. The operation of the cell occurred above the melting point of the carbonates, and the carbonate ion was considered the charge transport medium within the molten electrolyte. Years later, several changes occurred in the materials that make up the MCFC. However, its operating principle has remained unchanged, based on the indirect combination of H2 and oxygen into water via an electron-carrying electrolyte. One of the features that differentiate this type of FC from others is the net transfer of carbon dioxide from the cathode side of the cell to the anode through the electrolyte [44,49,50].
For many years, MCFC technology has been developed in the United States, Japan, Korea, and Europe and has gradually transitioned from a laboratory object to a potential alternative to conventional power generation systems. CHP generation is one area in which MCFC plants can be employed with great prominence because of the high efficiency that can be achieved. The efficiency of MCFC is around 55% and can reach 85% when combined with hybrid CHP cycles. Companies such as Fuel Cell Energy Inc. are investing in technologies that include cogeneration with micro gas turbines that use exhaust gases [44,49,50].
2.5. Solid oxide fuel cell
The first solid ionic conductor, demonstrated at the end of the 19th century, was the "Nernst Glower". In the 1940s, Carl Wagner described the driving process, and the first article about a SOFC was published in a journal in 1962. SOFC technology is the only type of FC that uses true solid electrolytes and has no electrolyte management problems that occur with the other types of FCs. This power generation technology is attractive because of the efficient and clean production of electricity from various fuels. Its main features are solid-state construction and high-temperature operation, which facilitates the direct reforming of hydrocarbons and results in a more flexible fuel selection. SOFC does not require a noble catalyst, which makes it more economical [44,51,52,53,54].
The companies Osaka Gas, Aisin, Kyocera, Chofu, and Toyota have developed small SOFC units with 700 W of power, accompanied by a 90-liter tank to store the hot water that will be used by the cell in its refurbishment process, as well as in residential heating when the cell is installed in a residence [44].
3.
Materials and methods
Regarding the objective, this research is characterized as descriptive and exploratory [55] since the evolution of technologies related to HFCs is described and analyzed. Considering the research approach, this has a quantitative character because it quantifies the results found through variables and indicators, which were analyzed according to the defined objective [56]. As to the analysis method, the research can be classified as inductive since it starts from the examination of the patents found to infer about the world technological development of HFCs in the first 20 years of the 21st century.
Therefore, in order to make the results more comprehensive, the Derwent Innovations Index (DII) was set as the basis for data collection, and the target population chosen for the research are the technologies present in the DII database comprised between 2001 and 2020. The research procedure comprises five steps, as observed in Figure 1.
After delimiting the research objective, the first step was to define the database for retrieving the patent documents. The DII database was selected because it is a complete database that covers patent information collected from 59 patent issuing authorities in the world, classified into four categories (chemical, mechanical engineering, electrical, and electronic), besides having more than 82 million practical inventions and is updated weekly.
In the second step of the research method, the search criteria were defined and the data was collected in the selected database. The searches for patents in the DII database can be conducted using several parameters combined or not, among which it can be mentioned: title, inventor, applicant, patent number, topic, and IPC. To collect the patents for this research, the keywords "hydrogen" and "fuel cell*" were used in the English language in the "topic" field, and the code H01M-008* in the "IPC" field. Between the two selected keywords and between the two defined fields, the inclusive Boolean operator (AND) was used to improve the data retrieval process and to delimit the search only to documents containing these two words, no matter in which order the set of words appears in the text. The truncation element (*) was used in the keyword to retrieve documents that contained variants of the word with the radical cell. The symbol (*) was also used in the IPC code to retrieve documents containing the radical H01M-008.
The topic "field" was selected for patent retrieval since it includes a simultaneous search for the terms used in the patent title and abstract fields. The IPC code H01M-008 refers to the classification of patents related to the field of FCs and their manufacturing methods. Finally, the documents with a priority date between 2001 and 2020 were selected. In the search performed in the DII database on July 2022 using the parameters defined in the search strategy, 22,165 patent documents were retrieved. Furthermore, it is important to highlight that the census method was applied in the search to observe the behavior of the previously defined population since the census' function is to collect, process, and disseminate detailed statistics about a given population [57].
Because the DII database presents only published patent documents, and because there is a delay, which varies from 6 to 18 months, between the filing of a patent and its publication, it was decided not to include the analysis the patents filed between 2021–2022, so that the result presented will be faithful to reality. In the third step, the retrieved data were exported from the DII database through files in.txt format. Each file generated contains information about 1,000 patents, which totaled 23 files. Then, to be processed and organized, the data present in the text files were imported into Microsoft Excel.
In the fourth stage, with all the data available in Excel, it was possible to analyze the patent data related to the major countries that deposited these technologies, the leading companies and institutions that developed the technologies, the major trends for HFCs, and the annual number of patent filings for this technology, among other analyses.
In the fifth step, with the data processed and the graphs generated by Microsoft Office Excel, it was possible to perform a descriptive analysis of the census to obtain information contained in the data collected and, through deductive inference, formulate conclusions regarding the characteristics of the population analyzed. Such information is essential to present the results and make the relevant considerations about the technological development of HFCs in the analyzed period.
4.
Results and discussion
From the documents retrieved from the DII database, this section presents and discusses the results of the patent analysis based on the theoretical background.
4.1. Evaluation of the number of patents filed per year
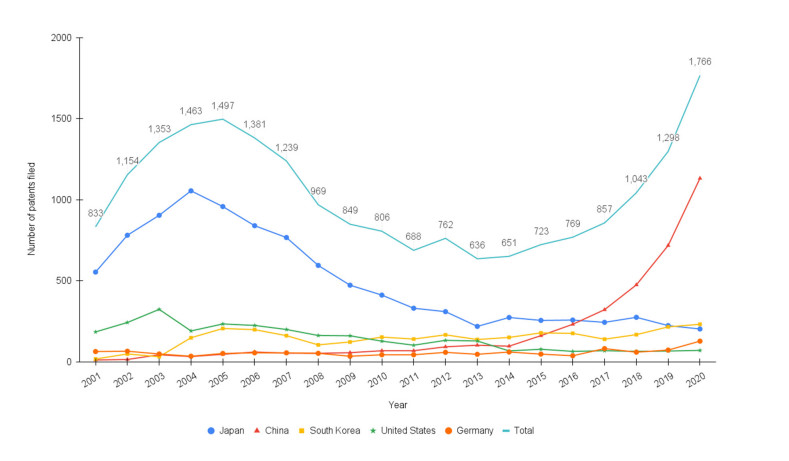
The search methodology adopted enabled the retrieval of 22,165 patent documents filed between 2001 and 2020 in the DII database, as can be seen in Figure 2. In general, a vertiginous growth of patent applications related to HFCs is observed in the first five years of the 21st century, between 2001 and 2005, and some considerations can be made in this context.
It is noteworthy that, in this period, there was a growing demand from governments, companies, and consumers for energy security, energy efficiency, global warming, GHG emissions, and climate change, in addition to the signing of the Kyoto protocol in 1997, which ratified the commitment of countries to reduce GHG emissions [58]. Moreover, this growth can be linked to the strong investment in R&D of FC vehicles, as well as validation and demonstration of the technology, as pointed out by the technology map developed by the government of China [59].
Between 2006 and 2011, there was a drop in the number of patent applications related to HFCs, with an apparent upturn in 2012 but a new drop in the following year, and relative stability in 2014. Such a drop may be related to a possible technological maturation, as well as to the beginning of production and commercialization of the technology in question, as presented in the technology map for HFC vehicles [59].
From 2015 on, the scenario changed and there was a strong growth in the number of patent applications until 2020 for this technology, corroborating the data presented in [2]. The return to growth in patent applications in this area is driven by China and may be an indication of a resumption of research by the industry and institutions, with a view to technological improvement, the development of lower-cost technologies, in addition to the growing pressure from governments and society to reduce carbon dioxide emissions [60], which can be related to the Paris agreement signed in 2015.
Despite the growth of China in the number of patent filings between 2015 and 2020, it was observed through the data collected that the Chinese patent applications in other countries are extremely low, corresponding to only 4% of their total patent applications. Although Japan is losing ground to China in the number of patent filings in the aforementioned period, around 21% of its patent applications are also filed in other countries.
4.2. Major patent filing countries
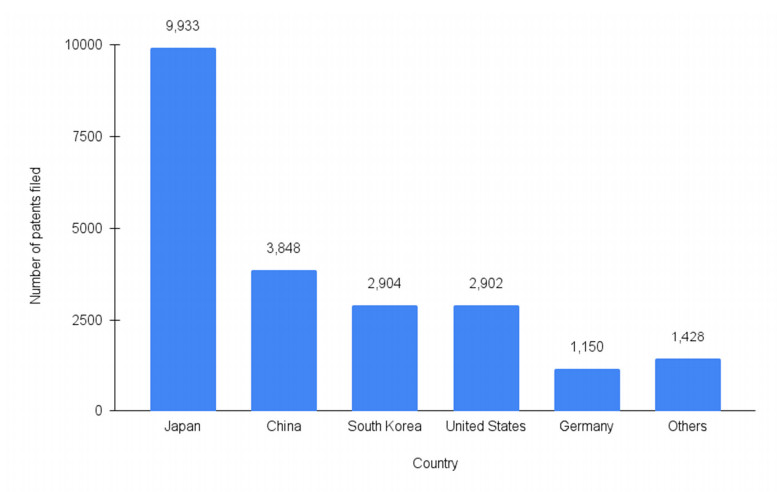
For the analysis of countries that concentrate the majority of patent filings for HFC technology between 2001 and 2020, the study was carried out based on the number of priority patent applications. From the analysis presented in Figure 3, it can be inferred that among the major countries active in the development of technologies for HFCs, there is a dominance by Japan, which holds 44.81% of all patent applications found, followed by China with 17.36% of patents, South Korea with 13.10% of patents, the United States with 13.09% of patents, and Germany with 5.19% of patents, while the other countries together account for 6.44% of total patents in the analyzed period.
The dominance of Japan in R&D for this technology, as observed in Figure 3, can be revealed as a result of the confrontation of the country against the strong dependence on imported fossil fuels, which exposes it to crises, such as the oil crisis of the 1970s, as well as portrays the commitment of Japan to environmental causes. Among them is the reduction of GHG emissions, which strengthens the development of clean and renewable energy technologies [61,62].
However, in a detailed analysis of the participation in patent deposits per year compared to the total number of patents deposited over the analyzed period, it can be seen that Japan, despite being the main holder of HFC technology, has been losing ground and China has been gaining strength in R&D and, consequently, in the protection of this research through patents, as presented in Figure 2. Japan showed a strong and growing pace of research and patenting for the technology analyzed between 2001 and 2004.
China, which showed a profile not so engaged in R&D for the technology analyzed between 2001 and 2014, presented a strong pace of R&D in this technology from 2015 on, with a dizzying growth that stands out, driving, in general, the return of growth in patent applications from that year on, according to Figure 2. Furthermore, the data presented in Figure 2 corroborates the information shown in the annual reports entitled "The Fuel Cell Industry Review" by E4tech, between the years 2017 and 2019, which point to the growing investment in R&D by China in recent years focused on HFCs [60,63,64].
In addition, it is notable that the constant and homogeneous participation of South Korea between 2005 and 2020, without major oscillations in R&D, focused on the technology in question. The representation of the United States was stable in the first ten years of the 21st century, between 2001 and 2010, having a slight decrease in the following years and remaining stable again. Germany had a stable but low representation in terms of HFC technology between 2001 and 2020, as shown in Figures 2 and 3.
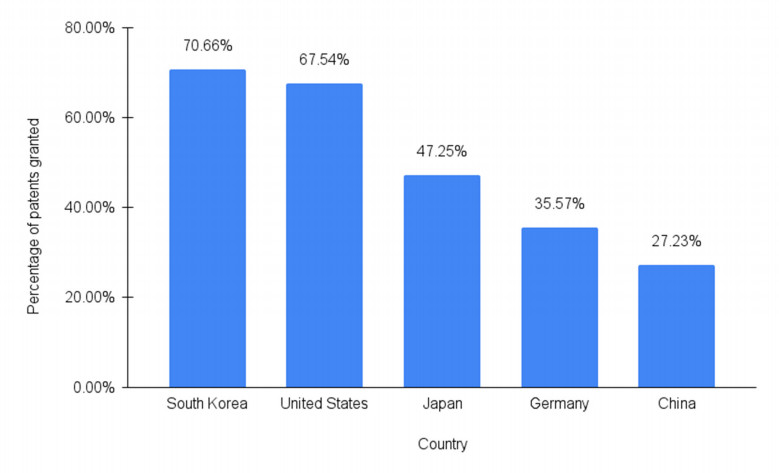
Regarding the percentage of patents granted for HFC technology in the period from 2001 to 2020, it can be seen from the graph in Figure 4 that South Korea has the highest percentage of patents granted, with 70.66% of its patents granted, followed by the United States with 67.54%, Japan with 47.25%, Germany with 35.57%, and China with 27.23%.
In this context, the percentage of success of an invention meeting the patentability requirements and being granted by the office responsible for its analysis will bring greater value to the patent, as well as greater security in its exploitation, considering the right to prevent third parties from using the technology without the necessary license. Furthermore, according to a study carried out by Albuquerque and Macedo [65], the percentage of patents granted has a strong connection with the performance of resources spent on R&D in the country. In this sense, it is possible to observe greater efficiency in investments in R&D, regarding HFC technology, by governments and companies in South Korea and the United States.
4.3. Main patent applicants
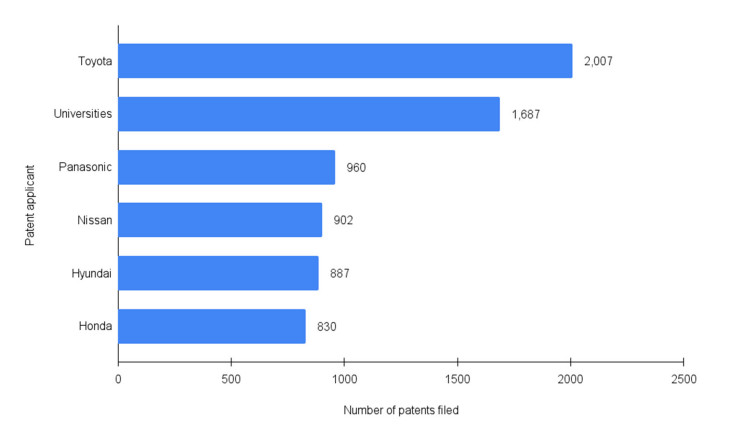
Among the major companies and institutions that filed patents in the area of HFCs between 2001 and 2020, the predominance of Asian companies from the automobile sector is notorious. It is possible to identify the presence of four Japanese companies and one South Korean company, besides the strong presence of universities, as shown in Figure 5. In first place, in the ranking of patent applicants, is Toyota Motor Corporation, with 2,007 applications. Founded in 1937, the Japanese company is dedicated to the technological development of the automotive industry, mainly, and had its first research related to FC vehicles in 1992.
The Japanese Panasonic Corporation ranks second with 960 applications. For over 20 years, the company has been dedicated to H2 energy research and, in 2009, Panasonic was the first company to sell and install residential FCs. Since then, the organization has sought to optimize developments in this technology so that the H2 society can be achieved through initiatives in which H2 can be produced, stored, and used easily, not only for residential use but also in vehicles [66,67].
In third place in the ranking, with 902 patent applications, is the Japanese Nissan Motor Corporation, founded in 1933 and one of the pioneers in automobile manufacturing. Nissan is considered a relatively new player in the HFC area, with research beginning in 1996. In addition, the company introduced its first H2-powered vehicle in 2001, and in 2016, Nissan was highlighted as the first in the market to present a vehicle equipped with a SOFC system using bioethanol [68].
The fourth place in the ranking is occupied by the South Korean company Hyundai Motor, with a total of 887 patent applications. The company was founded in 1967 and is engaged in the manufacture, production, and export of passenger cars, trucks, and commercial vehicles. Hyundai began its research into HFC technologies in 1998 and was one of the first manufacturers to make HFC vehicles commercially available and the first to introduce an SUV with this technology. In 2019, Hyundai Motor and H2 Energy formed a joint venture, Hyundai Hydrogen Mobility, to promote a green H2 ecosystem in Switzerland and other European countries [69].
The Japanese Honda Motor Company, founded in 1948, occupies the fifth position in the ranking, with a total of 830 patent applications, contributing to Japanese sovereignty regarding this technology. Honda, which is active in automobiles, motorcycles, and power products, began its HFC research in the late 1980s as one of the pioneers in research into this technology. As other Japanese companies, the organization is also investing in research for the development of a H2 society [70].
The presence of Asian companies and, more notably, from the automobile sector (as shown in this study), corroborates the data presented in a study conducted by the International Energy Agency [2], which points to the strong presence of Japanese and South Korean companies in the development of FC technologies. Furthermore, it is worth noting the intense R&D activity by universities that, together, totaled 1,687 patent applications between 2001 and 2020. These applications are distributed predominantly among universities in Asian countries and, among the applicants, universities from China with 689 patents, Japan with 326 patents, United States with 255 patents, and South Korea with 209 patents. The relevant presence of universities in Chinese patent applications can be related to the policies of this government in which patent filings are important for the career and promotion of researchers [71].
4.4. Major knowledge areas and international patent classification
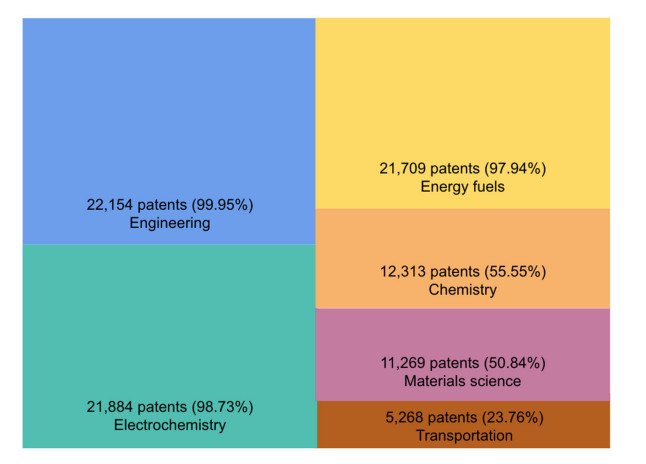
From the data collected in the search conducted in DII regarding patents filed for HFC technology between 2001 and 2020, it is possible to observe and identify the trends presented by the technologies per area of knowledge, according to Figure 6. It can be seen that 99.95% of the patents analyzed are included in the area of engineering, 98.73% are in the area of electrochemistry, 97.94% are in the area of energy fuels, 55.55% are in the area of chemistry, 50.84% are in the area of materials science, and 23.76% are in the area of transportation. There are also patents belonging to the areas of polymer science, computer science and telecommunications, but these are less expressive. It is relevant to mention that the patents may be included in more than one area of knowledge, given that the technologies are sometimes transversal.
In addition to the classification referring to the areas of knowledge described by the DII database, patents receive a universal classification given by the receiving office, the IPC code, which is essential for the quick identification of the technological niche to which that product or process refers. Patents are classified, in general, in more than one IPC code since each one represents a specific fraction of technological information. Table 2 presents an example, in which part of these representations has been described. The distribution of the patents retrieved in the search by IPC covers the areas of electricity, in Section H, and chemistry and metallurgy, in Section C.
As a result of the analysis of data collected in the DII database for HFC patents filed in the period between 2001 and 2020, it was possible to identify three major IPC classification groups in which these patents are distributed, which are: H01M8/00-FC and the manufacture thereof; H01M4/00-electrodes; and C01B3/00-H2, gaseous mixtures containing H2, separation of H2 from mixtures containing it, and purification of H2.
In a deeper analysis, from Table 2, it is possible to observe that the patent documents are mostly focused on: ⅰ) The improvement of the technology, to make it safer, is the case of the documents classified as H01M8/04 (FC with auxiliary arrangements, for example, for control of pressure or for circulation of fluids); ⅱ) FC using solid polymeric membranes, classified as H01M8/10 (FCs with solid electrolytes); ⅲ) pollution reduction, given that there is a predominance of technologies aligned with the reduction of GHG emissions, is the case of documents classified as H01M8/06 (Combination of FCs with means for production of reactants or for treatment of residues).
4.5. Patent analysis per hydrogen fuel cell technology
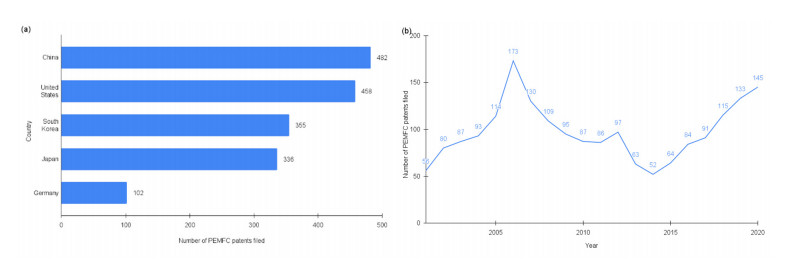
Of the total number of patents filed between 2001 and 2020 for HFC technology in the DII database, 1,954 patent documents refer to PEMFC, 1,289 patents refer to SOFC technology, 483 refer to PAFC technology, 475 refer to AFC, and 359 to MCFC technology. A more detailed analysis shows that the largest number of patent filings for PEMFC technology between 2001 and 2020 came from China, the United States, South Korea, Japan, and Germany, and of the total number of patents related to this technology, almost 50% of these patent applications correspond to documents originating in China and the United States, as shown in Figure 7(a). According to Figure 7(b), a cyclical R&D activity for PEMFC technology occurred between 2001 and 2020, with a decline in the number of patents filed between 2006 and 2014, with an increase in the filing of patents for this technology in the last six years of the analyzed period, from 2015 to 2020.
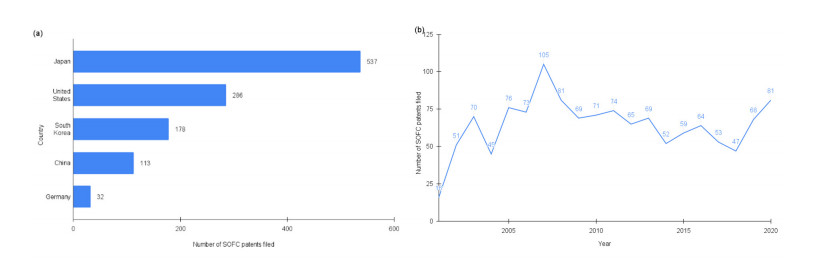
Regarding SOFC patents filed between 2001 and 2020, it is possible to observe from Figure 8(a) that Japan leads the development of this technology with 537 patent documents filed, followed by the United States with 286 patents, South Korea, China, and Germany, with 178,113, and 32 patent documents, respectively. In an analysis within the evaluated time horizon, from 2001 to 2020, an intense R&D engagement for this technology is observed between 2001 and 2007; however, from 2008 onwards, the number of patent applications has declined until 2018, with a discrete return to growth in 2019 and 2020, as shown in Figure 8(b).
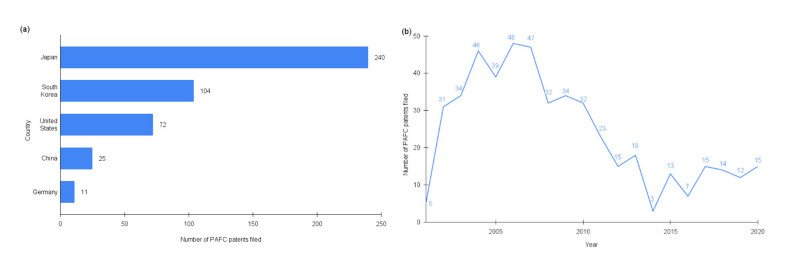
Patents related to PACF technology filed between 2001 and 2020 are strongly dominated by Japan, with a total of 240 patent applications, followed by South Korea, the United States, China, and Germany, with 104, 72, 25, and 11 patents, respectively, as shown in Figure 9(a). Regarding the year-by-year analysis, there was a growing interest in the technology at the beginning of the period analyzed, between 2001 and 2006 as seen in Figure 9(b). However, from 2007, the scenario changes and there is a decline in the intensity of R&D in PAFC, demonstrating a lack of interest in this technology, as corroborated by Sammes et al. [48].
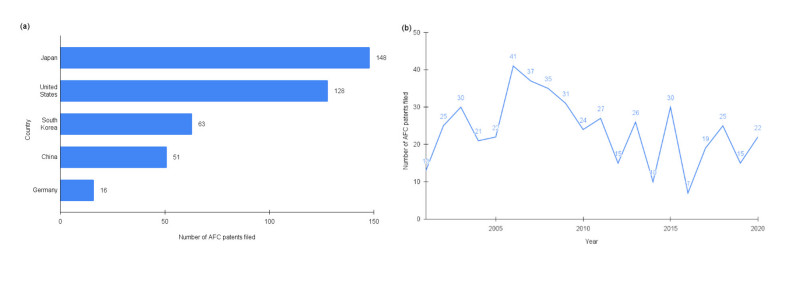
Regarding patents for AFC technology filed between 2001 and 2020, Figure 10(a) shows that more than 58% of applications were made by Japan and the United States, which filed 148 and 128 patents, respectively, followed by South Korea with 63 patents, China with 51 patents, and Germany with 16 patents. Regarding patent filings per year between 2001 and 2020, it can be seen from Figure 10(b) that interest in the technology increased between 2001 and 2005, with a subsequent decline from 2006 onwards, which may indicate a maturity of the technology as discussed in studies [43,44,45,46,47]. Between 2012 and 2020, R&D in this technology continues to be at a low level, in addition to having fluctuated during this period, not maintaining a firm level of R&D.
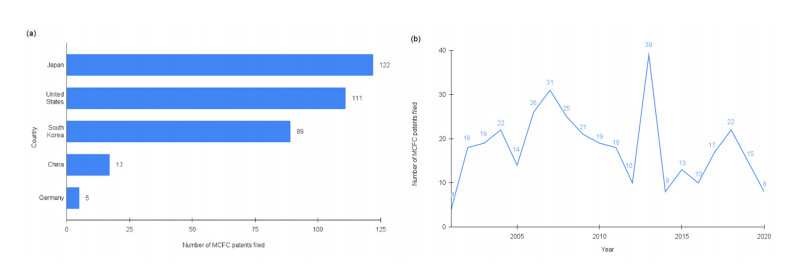
According to Figure 11(a), the patents filed for MCFC technology in the analyzed period, despite presenting a low total, are concentrated in Japan, the United States, and South Korea, which have 122,111, and 89 patent applications, respectively, during the period from 2001 to 2020. These data corroborate the findings of studies carried out by Dicks [49] and Bischoff [50], which state that these are the major countries developing the MCFC technology. China, with 17 patents, and Germany, with 5 patents, are countries that showed lower R&D intensity for this technology in the period analyzed. From 2001 to 2020, there were three peak moments in R&D for MCFC technology as observed in Figure 11(b), which occurred in 2007, 2013, and 2018. However, what can be seen is a low level of R&D throughout the years analyzed for this technology, which requires more investment to become a commercial product [50].
Regarding the major companies that filed HFC patents, their countries of origin, and the technologies adopted by them, it can be observed a predominance of Japan as the country of origin of the main companies active in HFC development, as presented in Table 3. Furthermore, there is a prevalence of PEMFC technology, and this fact can be associated with the attractive properties of the PEMFC technology observed in Table 1, such as low-temperature operation combined with a high-power density, in which it is possible to use it as an energy source in various applications, for example transportation, residential, and portable technologies.
5.
Conclusions
As a disruptive technology with high potential for the decarbonization of the world energy matrix, the use of H2 as clean fuel is becoming closer and closer to reality. The consequence of this process can be seen in the high R&D rates for HFC technology, a fact that reflects the large number of patent applications observed in the period analyzed of this study.
Concerning the guiding question of this research, "How is the global technological development of HFCs in the first 20 years of the 21st century?", the answers were obtained from the technological development evaluation through the analysis of patents documents about HFCs in the DII database from 2001 to 2020. The research in patent databases represents one of the original contributions of this research since it presents an overview of the technological development of HFCs worldwide in the first 20 years of the 21st century and elucidates the trends and guidelines for this sector. This global patent landscape could assist the goals of nations towards decarbonization by developing and applying technologies capable of reducing GHG emissions.
As a result, it was possible to identify and evaluate: ⅰ) The number of patents filed per year, with the identification of peak periods of growth in patent filing; ⅱ) the major countries where patents are filed; ⅲ) the top companies and institutions that filed HFC patents and the major technologies adopted; ⅳ) the principal areas of patent concentration; and ⅴ) the key IPC classification groups in which the patents are distributed.
Concerning the number of patents filed per year (ⅰ), it was possible to observe two peaks in the growth of patent deposits for the technology in question. The first peak was between 2001 and 2005, which may have intrinsic relations to multilateral movements by governments, companies, and society, about mitigating the effects of global warming and climate change, in addition to the growing investment in R&D for HFC powered cars. The second peak was observed between 2015 and 2020, which may represent a movement by the industry for the improvement of technologies already developed to bring more safety and efficiency to their operations and may also be related to the efforts of governments in pursuit of the goals set out in the Paris agreement.
Among the top countries responsible for patent filings for HFC technology (ⅱ), a predominance of Japan is observed, followed by China, South Korea, the United States, and Germany. During the first fifteen years of the analysis, there was a predominance of patent applications from Japan, with a gradual decline in the total number of patents filed from 2006 to 2014. However, between 2015 and 2020, new growth led by China is observed and can be related to the strong investment in R&D for this technology made by this country. Regarding the rate of patent granting for HFC technology between 2001 and 2020, it was found that South Korea and the United States have the highest percentages of granted patents.
Regarding the major depositing companies and institutions (ⅲ), it can be noticed that Asian companies are predominant, with Japan as the country of origin of the significant companies active in HFC development. Furthermore, PEMFC technology is prevalent. In first place is Toyota Motor Corporation, with 2,007 patents, followed by Panasonic (960 patents), Nissan (902 patents), Hyundai (887 patents), and Honda (830 patents). Universities also showed intense R&D activity, with 1,687 patent applications in the analyzed period. Regarding institutions, patent applications for HFC are predominantly distributed among universities in Asian countries, Japan, China, and South Korea.
The major areas of knowledge of these technologies (ⅳ) are engineering, electrochemistry, energy fuels, chemistry, materials science, and transportation. Finally, another relevant factor identified refers to the classification of patents performed from the IPC (ⅴ) and, in this case, it was observed that most patents are arranged in the groups "FC; manufacture thereof" (H01M 8), "electrodes" (H01M 4), and "H2; gaseous mixtures containing H2; separation of H2 from mixtures containing it; purification of H2" (C01B 3). Concerning the focus of technological development of the patents analyzed in the period from 2001 to 2020, it was observed through the data that PEMFC and SOFC technologies are those with the highest R&D intensity.
As a limitation of this study, the fact that not all technology developed was and/or will be patented; therefore, it is likely that there are inventions that were not covered in this research. Nevertheless, technological development investigation through patent analysis is considered an effective way to understand and study advanced technologies, and to predict technological and market trends. Consequently, based on this research, it is suggested that a monitoring of patents be included in the global political agenda to encourage research aimed at decarbonizing vehicles and thus promote a high degree of achievement among countries of the goals of the Kyoto protocol and Paris agreement.
As a suggestion for future research, one can indicate the deepening of the analysis of the patents filed by universities to verify which HFC technologies are more ecologically feasible and, in partnership with the major manufacturers in the sector, develop low-carbon technologies.
Use of AI tools declaration
The authors declare that they have not used Artificial Intelligence (AI) tools in the creation of this article.
Conflict of interest
The authors declare no conflicts of interest.
Author contributions
Lawrence Moura: Methodology, data curation, formal analysis, writing–original draft, writing–review & editing; Mario González: Conceptualization, supervision; Jéssica Silva: Conceptualization, methodology, writing–original draft; Lara Silva: Writing–original draft, figures, tables; Izaac Braga: Conceptualization, figures, tables; Paula Ferreira: Data curation, formal analysis; Priscila Sampaio: Methodology, data curation, formal analysis, writing–review & editing.









 DownLoad:
DownLoad: