1.
Introduction
The consumer of modern society is complex, exigent, critical, and competent. It is impossible to trace a typical consumer's profile because everyone is different from others [1,2,3]. Each one, indeed, asks for safe products but pays attention to the multiple aspects of the products differently from the other people, under their own and specific personality. Many features and aspects of a food product, indeed, can be appreciated, such as naturalness, functionality, low-fat content, intelligent or interactive packaging, social and environmental aspects, ethical aspects, the link with the territory, time-saving service, origin of raw materials, color, form, label information, brand, price, and others [4,5].
Therefore, the food demand is multi-fragmented and changeable and dynamic markets. Focusing on developing sustainable agri-food systems, the consumer has a crucial role, and his ways to influence this process mainly consist of adopting sustainable diets and avoiding food waste.
Although sustainability is a concept that has now entered the shared vision of the populations of advanced countries, it is little studied in terms of food consumption. This is from the perspective of sustainable diets due to the multiplicity of aspects of life dominated by the need to safeguard and save water and energy resources, differentiated waste disposal, the ability to limit the impact of pollution, and so on [6,7]. In this regard, the multidisciplinary aspect of the problem becomes evident in the link between sustainability and food security, related issues in advanced and developing countries. The authors argue that a territory's food security is linked not only to its ability to produce enough food to meet domestic demand but also to its ability to access the five capital assets (natural, social, human, physical, and financial capital), as well as the production of various positive externalities (such as the availability of food crops to regenerate soil organic matter, combat erosion, and protect biodiversity). The establishment of new rules for the management of communal natural resources; the ability of farmers to experiment and solve different problems; the support of marginalized groups or low-level contracts; the achievement of the best health and nutrition for children, and so on), the ability to access technology and knowledge for produce on the territory, to the purchasing power of the population. The challenge for the future is to realize sustainable food systems to reduce the impact on the environment while satisfying the dietary requirements for health without eliminating staple foods (e.g., meat or dairy products) or increasing the cost to the consumer [8]. In this context, Guarnaccia et al. [9] have proposed an exciting framework for implementing a regional sustainable food system in Sicily by analyzing critical environmental and socio-economic indicators and developing a strategic and participatory plan.
This research aims to analyze the link between sustainability and consumption patterns in two study areas-the United Arab Emirates and Sicily-characterized by numerous elements of similarity (from the climatic point of view, in the availability of quality and quantity of water resources; overall sensitivity in adopting organic or sustainable methods of cultivation, high demographic immigration, of a political-economic and tourist nature, with repercussions in the organization of the socio-cultural, territorial working system, etc.) and of diversity (in average incomes per capita, in the availability of financial resources for investments, etc.) [10]. These areas were chosen because they are among the worst-performing countries with high per capita incomes in responding to global challenges on sustainable agriculture, nutritional challenges, combating food waste, food losses, and sustainable eating styles [11].
In the two territorial contexts, the paper has the objective of investigating purchasing behavior to define a sustainable consumption model, the factors conditioning the purchase, and the elements of similarity/dissimilarity on direct the action of public and private stakeholders.
Therefore, the demand for research is to define the intentional behavioral and attitudinal aspects of sustainability that impact the purchase of food products to detect all those elements that can promote sustainable food consumption in the regions under study. While admitting that sustainability is a very articulated concept, especially on the environmental and social level, the researchers deliberately did not focus in particular on one of the possible modes of expression (e.g., organic products, zero kilometers, or respect for the rights of certified workers/children, etc.) because it was inspired by a concept of "transition" that is very much in vogue today in times of economic recovery after the depression of the Covid19 pandemic and the Russia-Ukraine conflict [12].
This work is helpful in the perspective of the "Agenda 2030" of the UN and the commitment undertaken at the international level by the various countries in the direction of greater sustainability of economic and social systems. In the 21st century, the world faces countless complex global challenges, such as climate change mitigation, global health, coping with massive migration, preventing terrorism, ensuring cybersecurity, and preventing misuse of human rights. The responses of many stakeholders to these global challenges require a concerted effort, not only from governments but also from civil society, the private sector, international organizations, private philanthropies, and individuals. Ensuring sustainable consumption and production patterns will be critical to achieving many of the UN's sustainable development goals by 2030 [13].
In this way, sustainability and food security grow into food sovereignty and their ability to promote the restoration, maintenance, and conservation of indigenous food systems and the cultural reproduction of indigenous knowledge of food production, distribution, and nutrition.
2.
A pathway to sustainable consumption, according to the recent literature
The focus on sustainable food consumption has progressively increased with the definition of the new millennium goals on the national and international agenda. For this reason, the literature has been enriched gradually with studies and research on purchasing behaviors and products in different territorial areas became the expression of sustainability [14,15]. However, there is a high polarization of research on organic products among these. In contrast, consumer attitudes and factors that condition purchasing preferences and behavior towards other sustainable products (e.g., fair trade, typical products, products with environmental or green impact certification, animal welfare, etc.), are still little explored overall [16,17,18,19].
This is partly due to the significant spread of organic farming in both rich and developing countries on the planet and partly to the absence of an agreed and "official" definition of the link between products made with different paradigms of sustainability and sustainable consumption, also inspired by way of life [20].
In the first case, an updated reading can be done through empirical evidence collected in two areas of the planet with different degrees of development. In Brazil, for example, where production and sustainable consumption systems are still being developed due to differences in the perception of organic food, a study examined the relationship between the socio-economic and demographic profiles of organic food consumers and their motivations, perceptions, and attitudes. Creating a more consumer-oriented perception, inspiration, and attitude towards organic food and different indications is necessary to improve sustainable food consumption mechanisms in peripheral regions [21].
A different situation was found in Italy, one of the countries in the world with the highest concentration of surface area converted to organic but with a very differentiated consumption of organic products on a territorial and social level. In this case, the theory of planned behavior has shown that the more remarkable aptitude of areas in the Centre-North to consume organic products is linked to experience gained in the past, since family, advice from influential people and friends or society can influence sustainable food choices. While an institutional deficiency can be traced back to the absence of a sustainable food market policy in Italy, not even with legal certification unless it concerns the organic sector [22].
Also, in Italy, a study on identifying key players in the transition to more sustainable food systems shows that consumers of organic products are much more attentive to sustainability in their available food choices and have a more sustainable lifestyle. This sensitivity is positively correlated to the female gender, and young age and food scandals and food safety concerns are strong predictors of the intensity of organic consumption [23,24].
In the second case, there is particular interest in a study of British consumers' priorities towards sustainable food because packaging, food production methods, animal welfare, and local products play an important role in purchasing behavior. Consumers identify these factors as a guide to more sustainable purchasing and, therefore, as a means of supporting this expectation [25].
Regardless of the type of sustainable product, an analysis perspective found in the literature aims to know the antecedents of the purchase intention, helpful in characterizing the behavioral model in purchasing these products. Therefore, a survey was carried out to assess the influence that the ecological well-being, political values, and corporate social responsibility (CSR) of the store have on the willingness of consumers to purchase organic quinoa-based foods [26]. This must be integrated with an analytical perspective in which the ingredients and, therefore, the essence of sustainable food is compared and the role that external factors, such as packaging, have in guiding purchasing choices [27,28].
A survey in Denmark, Finland, Norway, and Sweden has shown that healthy eating patterns, interest in cooking, and supportive environmental policy measures- against socio-demographic factors-are positively correlated with sustainable food consumption. Besides, among all forms of sustainability, local food is the most popular form of sustainable consumption, while the consumption of meat was the most unpopular [29,30].
Sustainable consumption remains a phenomenon to be explained exclusively by socio-demographic factors because consumers have to deal with choices. According to a review of the literature on this topic, the first level of complexity is attributable to aspects such as personality traits, lifestyles, and food-related behavior; among these emerge specific segments defined as "green, " "potential green, " and "non-green" on which to base any empirical investigations [31].
As a result, several psychographic qualities and socio-demographic characteristics explain attitudes toward food consumption. As a result, it appears that convinced sustainable consumers are individuals who believe that their personal purchasing decisions impact global sustainable development, demonstrate greater availability and increase sustainability through their consumption behavior, and are concerned about food quality [32].
In the dynamics of sustainable consumption, there is often a differentiation between declared and actual purchasing behavior, as a not inconsiderable role is played by personal emotions. In this context, a study has shown the role of some inhibitors / behavioral promoters, such as past purchases, premium prices, product availability, and barriers to product variety [33]. A survey in Switzerland, on the other hand, investigated purchasing behavior when comparing organic and conventional products, demonstrating the role that factors such as health, social standards, environmental values, income, and educational level play in consumers' desire to consume more environmentally friendly and healthier foods [34]. Healthy attributes and the time to prepare meals independently to achieve healthier food consumption were relevant for consuming organic products in Italy [35].
Finally, consumers' interest in the choice of sustainable food is supported by family and friends and by the health incentive. At the same time, the price factor contributes only secondarily after the individual is interested in buying sustainable food [36].
3.
Materials and methods
The work aims to fill certain cognitive and interpretative shortcomings in the phenomenon and identify some factors useful for the knowledge of the market of sustainable agri-food products. To this end, the survey was conducted at 6 "farmers' markets" specialized in direct sales, operating in the center and the urban suburbs of Abu Dhabi, Catania, and Palermo.
The choice to limit the detection of the phenomenon understudy to the latter arises from the precise characteristics of sustainable product consumption (concerning preferences in the distribution circuit) and the importance of such distribution in the territory where the analysis was focused. A sample of consumers was obtained at these marketplaces in a "non-probabilistic" method, using a mixed type technique, between October 15 and November 15, 2019, which represents a period when food consumption is not affected by seasonal events (migrations from the city center to tourism, other holidays where prices may increase, such as to affect the quantities demanded, etc.). In the months following the direct consumer survey, the SARS-COVID19 pandemic emergency spread, which profoundly revised the consumption picture. Therefore, the results are not conditioned by seasonal events.
Non-probabilistic sampling, as is known, is used in market research to reduce the time and cost of carrying out research. Similarly, the "mixed" detection technique allows for a leaner work organization. Among the limits, we must remember the impossibility of guaranteeing that all the elements that compose the universe are chosen to form the sample [37,38].
A questionnaire was administered in Sicily (Italy) and the United Arab Emirates (UAE). The chosen sample was about 500 consumers, and the aim was to identify some aspects related to sustainability, such as consumers' sensitivity to sustainability, their behavior, and their opinion or address. The particular questions of the questionnaire were coded and reported in the analysis figures included in the text.
The sample size has been chosen according to the research objectives and the available financial resources. The survey was entrusted to specialized staff and performed using a questionnaire form specially prepared for careful information collection, previously tested on consumers willing to collaborate. The survey was conducted with the full consent of the participants, including the conditions expected by both the researcher and the participants. These included voluntary participation, participant withdrawal at any given time, data protection (guaranteeing anonymity and privacy as required by EU Reg. 2016/679 and Legislative Decree 101 of 10 August 2018), and the dissemination of data in aggregate and not in individual form.
The closed-ended or multiple-choice questions (more alternative options) have allowed the collection of a large amount of qualitative and quantitative information expressed both on an ordinal or nominal scale (consumption frequency, consumed quantities, etc.) or on an interval of intervals or proportional (purchasing methods, socio-economic characteristics, etc.) [39].
The sample size was lowered by 10% by deleting completed forms due to interviewee hesitation on some of the questions (especially personal). In some cases, the reactions were linked to a judgment that considered both the consumer's intensity of specific behaviors and the legitimacy of the conduct.
The associated judgment values range were from 1 (lowest value) to 5 (highest value).
In these cases, for the data analysis, the % weighted value expressed by the following formula was used:
where ni is the number of consumers who answered affirmatively, fi is the weight (or judgment) assigned to the response (from 1 to 5), and the sum nijfij is the total number of answers multiplied by the respective weights.
For each answer, the Weighted mean judgment was also calculated according to the following formula:
where ji is the intensity of judgment expressed and mi the frequency with which it is communicated.
We have applied the Tobit regression to identify the sustainability factors that influence consumer behavior in their purchasing choices [40]. The Tobit regression has been widely used in studies on the consumption of niche or non-niche food products to assess the impact that knowledge and information on health and/or quality aspects have on purchasing choices [41,42,43,44,45].
The two survey areas have many characteristics but an additional income per capita. This has been designated as the dependent variable, whereas the independent variables' coding has been provided in Table 2. The effect of correlations with age, level of education, and study, on the other hand, has been evaluated to complete the analysis, repeating the elaborations. The standard Tobit model can be defined as follows for observation (consumer) that is:
We assumed T = 0 (zero) in this Tobit model, i.e., the data are censored at 0. Thus, we have
where u ~N(0, Σ2), X, and β are vectors of explanatory variables and unknown parameters, respectively. The y* is a latent variable, and y is the purchase of food products. In the present study, we use the functional form of the censored Tobit model as below:
where:
- the list of variables and their coding in the model is shown in Table 1;
- β0, β1-32 and µi are constant regression coefficients and error terms.
The variables extracted from the questionnaire were aimed at defining the general attitude of the person surveyed towards sustainability and the weight of the motives and the factors that determine life choices and food consumption.
All the information collected was treated with multivariate analysis (factor analysis). Variables, results, and statistical significance are detailed in the following paragraphs.
4.
Results and discussions
4.1. Survey areas and socio-economic characteristics of the sample
The two areas under study present many elements of similarity concerning the physical and pedoclimatic environment characteristics but a significant difference in many economic and social aspects.
Sicily is a region with a strong presence of organic farming (equal to 371000 hectares in 2019, representing 25.8% of the area used for agricultural activities; operators are just under 11000, equivalent to 6.3% of farms) [46] and has a per capita income of 17400 EURO (2019 value, expressed in PPA) [47]. In this area, thanks to citizen movements, representatives of civil society, universities, various stakeholders, institutions, there is a movement towards the promotion and recognition of innovation and development models in the areas of food security, the bio-economy, sustainable agriculture, and other related issues (climate action, efficient use of natural resources, safe, clean and efficient energy).
The United Arab Emirates, on the other hand, has made fewer investments in organic farming (47000 hectares in 2018, equivalent to 1.2 percent of the national agricultural area and involving 95 organic producers) [48], but this is in line with the availability of land in this part of the world, given the arid conditions that make the conversion to agriculture difficult, but due to a high per capita income (equal to 42600 euros, expressed in PPA) [49] is being considered.
A sample of consumers was found in the two areas summarized in Table 2.
The sample comprises men (57% in Sicily and 53% in the UAE) aged between 35 and 60 years (more distinctly in Sicily). The level of education is exceptionally high since as many as 43% in Sicily, and 41% in the UAE declared to have a degree equal to a master's degree and/or higher (Ph.D., etc.). The level of education is essential to appreciate aspects of sustainability.
The respondent's household comprises children in 54% in Sicily and 65% in the UAE, and the family bond is formalized by civil and/or religious marriage.
Finally, in line with the disparity in territorial economic development, the representatives of the two areas are polarized in the low-income bracket in Sicily (41% declare an income between 20000 and 39999 EURO/year) and in the high-income bracket in the UAE (over 80000 EURO/year, in 47% of cases).
The data analysis in the table clearly shows that there is a diversity between the areas under study. Therefore, this diversity may help represent further distinctive aspects of purchasing behavior and was accepted. The two areas - beyond what has already been said - have a political and strategic element that unites them and, at the same time, makes them a fascinating object of study: on the one hand, Sicily is among the regions of Italy that have shown the highest conversion to organic farming (organic areas) and has a robust environmental, ethical conscience (expressed through organic movements, organic zero kilometer markets, fair trade purchasing and selling circuits, etc.). On the other hand, the United Arab Emirates, to compensate for the limitations of the physical environment, has opted for sustainable production and consumption models, becoming an exciting case study.
4.2. Consumers by behavioral type
A multivariate analysis of the factors and determinants of consumer behavior was carried out, which was necessary to build up a picture of the importance of the individual sustainability factors.
The procedure results show a close relationship between the factors defined as "active and critical consciousness" (beliefs, opinions, attitudes, subjective norms, sensitivities determined by advice from specialists, friends, and relatives or by the coverage of debated topics in the mass media. While not explicitly dealing with a link between food and sustainability, is used by the consumer to infer a higher or lower level of sustainability in the act of choice) and consumer behavior toward food products with sustainability attributes. Some examples of these factors are "climate change, " "level of knowledge, " "concerns for the future, " "perception of brands, " "restart of consumption after a long crisis period, " etc.
A factorial analysis was carried out using Principal Component Analysis with SPSS software to understand the weight of these aspects. This technique makes it possible to explain and repeat the relationships between empirically connected variables, thanks to the presence of common factors.
To begin, the determinant value (which is non-zero, indicating that there are no linearly dependent variables), the KMO test (equal to 0.79 for Sicily, thus moderate, but 0.85 for the UAE, thus adequate), and the values of the sample adequacy measure for each variable (equal to or greater than 0.80, except in a few cases) were used to evaluate the hypotheses and variables considered, as shown in Table 3.
The number of factors to be used was made using the screen plot of the extracted factors against the corresponding initial eigenvalues. Analysis of the orthogonal rotation loading factor (varimax) of the four main factors in the entire study sample (accounting for approximately 54% and 69% of the cumulative variance explained in Sicily and UAE, respectively) revealed a picture of particular interest [50].
As shown in Table 4, aspects relating to the vision of prospects significantly influenced the consumer's assessment, thus representing the first factor extracted which alone explains over 19% and 24% of the total variance in the two areas.
Among the attributes, "Climate first, " "Health and well-living, " and "I love little cities" were the most important. The interviewees believe that protecting the environment and combating global warming will profoundly impact the economic and productive ecosystem in the two countries. Taking care of physical and psychological well-being, practicing sport, and health check-ups to prevent illnesses will be keywords of future behavior. There is also a return of interest in small towns and shopping in neighborhoods and local shops.
The attributes most related to beliefs in sustainability in the shopping bag together explain more than 14% and 19% of the variance in Sicily and UAE, showing how consumer demands are linked to new values related to health, environment, and affection. Among these are self-care, environment, honesty, tolerance, inclusion, and condition choices to enable the perception of value that unites consumers and brands (embodying values, credibility on sustainability issues, etc.).
Some aspects closely linked to the climate and its changes modify food habits (together responsible for 11% and 13% of the variance); global warming and environmental awareness combined with new actions to contribute to collective demands. This seems to be through new/alternative raw materials and new technologies. The understanding that the unavailability of raw materials due to climate change is becoming problematic. Rising raw material prices threaten to undermine the ecological and digital transition.
Finally, the "food revolution" aspect constitutes the last of the factors extracted and explains only 9% and 12% of the variance extracted in the two areas under study. There is widespread awareness of the importance of reducing meat consumption. Thus, a healthy and balanced consumption trend is emerging, expressing interest in vegetable-based, meat-flavored, high-protein seed-based foods. Not yet vivacious is the willingness to eat food made from insect meals.
4.3. Sensitivity of the territories to sustainability
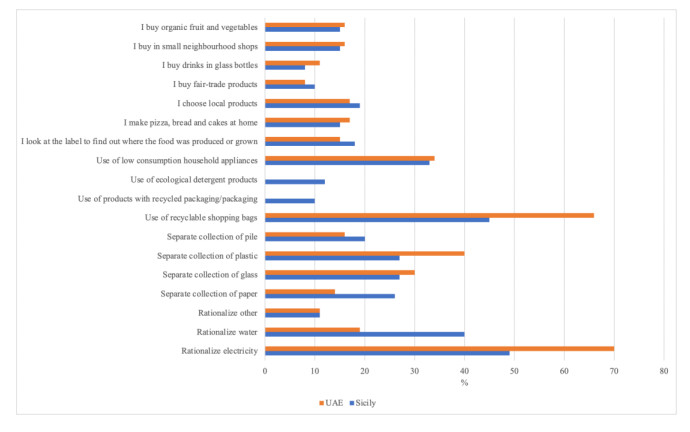
The first aspect analyzed was related to the sensitivity of the territories to the concept of sustainability through the interview with the consumer. These were asked: What consumption do you rationalize? What differentiates in the collection?, Do you use...? when do you make food choices…
Below are the results obtained for both Sicily and UAE.
Figure 1 shows that in the UAE, the rationalization of energy (70%) is more attentive, while in Sicily, the same attention is given to both energy (49%) and water (40%).
In UAE, the predominantly differentiated materials are glass (30%) and plastic (40%), while in Sicily also, batteries (20%) and paper (26%) have about the same attention as glass (27%) and plastic (27%).
In UAE, recycled materials mainly used are shopping bags (66%) and Low consumption household appliances (34%), while in Sicily, there is also a use of Products with contained packaging/packages (10%) and Ecological detergent products (12%).
The choices regarding the question "When do you make food choices" are common both in UAE and in Sicily.
Several studies show a correlation between food consumed in dietary patterns and health status. Diet and nutrition play a crucial role in promoting and maintaining health throughout life, but what we choose to eat and drink has a significant impact on ecosystems and monetary resources [1]. This choice does not necessarily have to become a more substantial burden for the population [51]. Consequently, some studies suggest that a healthy diet with a low environmental impact (therefore more ecological) does not necessarily have to be more expensive. However, the institutions (including organized large-scale retail trade) should take an appropriate communication campaign on the sustainable diet from an economic and environmental point of view [52].
4.4. Address of consumers toward better sustainability
Another aspect analyzed is the opinion of the consumers, of the two different countries, on the actions underway, by the manufacturing companies, for greater diffusion of sustainable products to have an orientation by consumers towards better sustainability. Following are the analyzes related to single answers and the relative intensity of judgment.
Figure 2 compares the UEA and Sicily on the importance attributed to the presence of a logo on the packaging of products able to certify production according to the dictates of sustainability.
A more marked level of trust in the UAE emerges, with a greater concentration of opinions on "they are reliable" and "Sicily, " territory with more significant variability of responses. The result of the research is in line with the international debate on the appropriateness that the sustainability of the food system becomes an integral part of dietary orientation, human health and services, and agriculture, and of the consequent political actions to support it [53,54].
A diversity of opinion also occurs on what sustainability allows to achieve. For the consumer of the UAE, sustainability is considered, above all, a strategic lever of the country's economic development, given the general lack of natural resources that the territory suffers from [8]. On the other hand, the perception of the guarantee of more excellent food and social security appears to be moderate, probably due to a limited ability to link sustainability and resources. On the other hand, Sicily polarises a more significant number of responses on protecting natural resources, mitigating climate change, and food and social security.
More excellent uniformity in the responses between the two areas under study was detected in the necessary actions to promote social and cultural innovation in consumer activities. Significant importance is attributed to the actions for reducing food waste in commercial catering, promoting healthy and sustainable consumption initiatives, and the predisposition of incentives for the consumption of organic products. On the other hand, Divergent was in the position regarding the need to encourage scientific research on consumer behavior.
The value judgment associated with what consumers think, in general, of the information on food packaging to certify respect for the environment and the characteristics of sustainability is always reported in Figure 2. For the UAE, particular importance is attributed to the regulatory aspects of production according to predefined standards and known to the consumer. This aspect recalls other values, such as the product's authenticity, traditionality, and the standardization of the control activity [55]. Control, the adoption of standards, and the support of activities in the areas of origin and counterfeiting, on the other hand, are given a lower value in Sicily. As for what is needed to reassure consumers on the purchase of quality food products (good and safe), two aspects are above all considered. For the UAE, a more excellent value is attributed to the organic certification, while a substantial convergence of the Sicilian consumer has been on the territory's product from the short supply chain.
The weighted judgment value was then calculated for each response and the total average value. The results are shown in Table 5. On the whole, supported assessments emerge for almost all aspects of sustainability expressed by logos and information on packaging and concrete actions that civil society can support to encourage sustainable consumption.
4.5. Brand, packaging, and social innovation in sustainable consumption
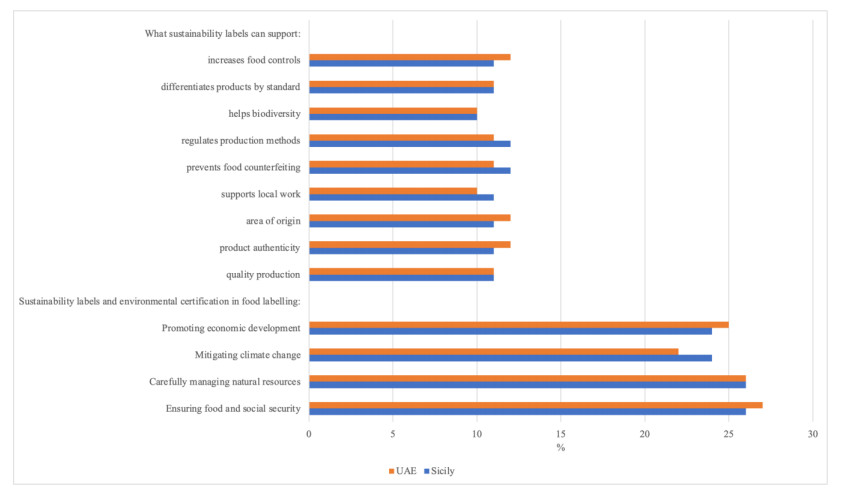
Subsequently, the % weighted value was used for the data analysis. Figure 3 shows the results obtained.
Consumers in both countries have the same opinion that logos of quality sustainability/environmental certification on the packaging of food products are recognizable (21% for UAE, 23% for Sicily), and they help choose the most environmentally friendly products (22% for UAE, 24% for Sicily).
Sustainability can help UAE consumers ensure food and social security (27%), while Sicilian consumers also carefully manage natural resources (26%).
The actions needed to involve society better and promote social innovation in the field of sustainability are common opinions for the consumers of both countries and are: improve the quality of information on organic products (17% for UAE; 18% for Sicily), provide incentives for the purchase of sustainable organic products (16% for UAE; 16% for Sicily), funding research on consumer behavior (17% for UAE; 14% for Sicily), promoting social innovation in the agri-food chain (16% for UAE; 16% for Sicily), strengthen actions to encourage healthier and more sustainable consumption (18% for UAE; 18% for Sicily), strengthen actions to reduce food waste in households and restaurant service industries (16% for UAE; 18% for Sicily).
Also, what think, in general, of the information present on food packaging, which certify respect for the envitonment and the characteristics of sustanibility and what want for to be sure to buy a quality food product (good and safe) are common opnion for the consumers of both countries and are: product quality (11% for UAE and Sicily), authenticity of the product (12% for UAE and a little less for Sicily), area of origin bounded (12% for UAE and a little less for Sicily), encourage work in the production area (10% for UAE and little more for Sicily), avoid buying counterfeit products (11% for UAE and little more for Sicily), regulated production methods (11% for UAE and little more for Sicily) because it is a traditional product (10% for UAE and Sicily). After all, it is a better product than the standard one (11% for UAE; 11% for Sicily) because it is a more checked product (12% for UAE and a little less for Sicily).
The organic brand (49% for UAE; 51% for Sicily) and the territory of origin (51% for UAE; 49% for Sicily) play a relevant role in purchasing in the two territories.
4.6. Consumers' behavior towards sustainable products
Finally, the behavior of consumers from both countries towards "sustainable" products was analyzed. Single answers and the relative intensity of judgment have been studied, and the results are shown in Figures 4 and 5.
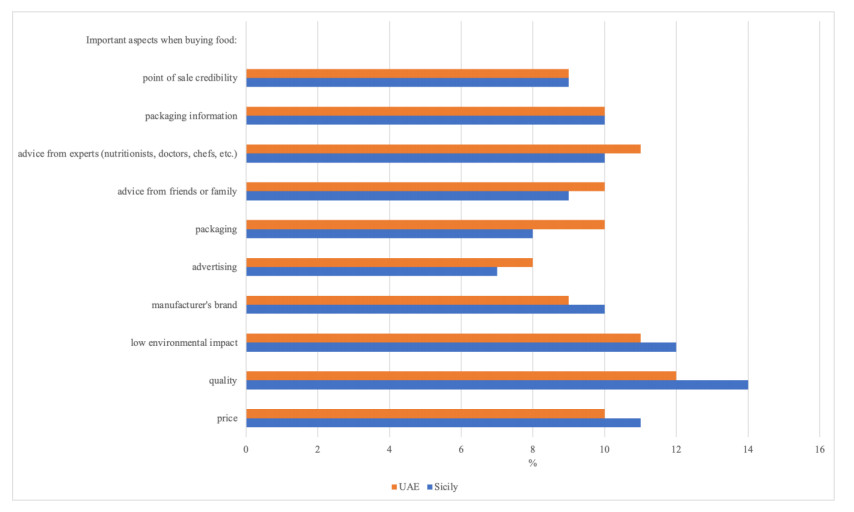
The commercial channel of choice in the UAE was the modern one (e-commerce) and sold to supermarkets and specialized shops, while in Sicily, the discount and the city market are more important. Equal importance - in the two areas - is attributed to the purchase of solidarity groups.
The quality of the product, the promotional message, the information on the label, and the opinion of experts and nutritionists in the UAE are the aspects that are given greater attention when purchasing food goods. In Sicily, quality stands out in other options, but the low environmental impact of the production process also takes on particular importance.
The organic products of consumer interest are, above all, "fruit and vegetable" and "fresh meat and fish" for UAE, unlike in Sicily, where there is a greater variety of situations. The preferred biological products must be connected to the purchase channels because they can influence consumers' choices.
The weighted judgment value was then calculated for each response and the total average value. The results are shown in Table 6.
UAE consumers buy homogeneously across all possible spending channels except for GAS, with a 7% preference. Instead, Sicilian consumers prefer the most classic shopping channels with a low preference for e-commerce (6%).
For UAE and Sicilian consumers, a particular aspect does not prevail when buying food.
As for the type of products purchased, UAE consumers prefer fresh meat and fish (27%) and fruit and vegetables (25%), while Sicilian consumers prefer food business (29%) and fresh meat and fish (20%).
4.7. Tobit Regression model results
The variables used to validate the Tobit model are divided into four behavioral spheres: the motives guiding purchase decisions (which sustainability variable guides the act in general purchasing), the expectations placed on sustainability (the latter's ability to propose concrete solutions to universal problems), the conditions for promoting sustainability that can be achieved in the economy and society (able to raise awareness of the issue), and the conditions for promoting sustainability that can be achieved in the economy and society (able to raise awareness of the issue) (elements that trigger a daily preference for different food products). These were compared to the main socio-economic characteristics found in the literature as factors conditioning the purchase of organic and sustainable products, such as income, age, and educational level, an expression of the respondent's cultural level [20,56].
The Tobit model reveals the existence in the two areas of significant correlations in each of the combinations between dependent and independent variables. It is reliable when evaluating the results, as seen by the varied likelihoods for each regression in Tables 7, 8, and 9.
The models reveal positive correlations between income, age, and educational level and respectively for territoriality of production and products coming from organic farming in proximity, both in Sicily and in the UAE, within the first group of variables.
As far as purchasing behavior is concerned, there is a substantial convergence on the importance of sustainable packaging in the case of food products and expert advice as a reference figure in the representation of the values incorporated in sustainable food.
As regards income (Table 7), on the other hand, the focus on sustainability in both areas also involves the choice of fair-trade products because it is perceived as a tool for achieving higher levels of social security, as well as quality production, in line with what has been found in the literature [57].
Instead, some differences can be seen in the expected impact of climate change, a problem that Sicily is more concerned about than the UAE, whose populations are likely more accustomed to the temperature and seasonal fluctuations; similarly, advertising has little influence in Sicily, unlike the UAEs, where B2C marketing strategies are the lever for meeting consumer expectations and business success.
Concerning age (Table 8), it is possible to observe different positive correlations in the two areas, although with different intensities.
Thus, Sicily prevails the interest in information about the territory of origin on the label, the local origin, the reduction of waste, the environmental impact of processes, the company brand, packaging, and expert advice. All these variables tend to be homogeneously correlated. In the UAE, on the other hand, the impacts on the management of natural resources, economic growth, waste reduction, and expert advice stand out among others in terms of values. This appears to be in line with the country's strong development, which is owing in part to high tourism investment and recently launched institutional campaigns to promote a healthy food culture, beginning with the younger generations, to combat childhood obesity and other health issues caused by the adoption of continental consumption patterns [28,58].
Finally, as far as the interviewee's educational level (Table 9) is concerned, it is possible to detect a homogeneous trend of evaluations on information, suitable packaging, and food waste.
There is a growing awareness of the territory and local economy in Sicily and the cost and quality of sustainable food. In the UAE, on the other hand, there is a growing interest in sustainability to guarantee access to food and food security, as well as in brands and communication campaigns. This is due to the structure of the local distribution system, which is represented by large distribution groups, including multinationals, operating intent. On the one hand, because high investments in the tourism sector have attracted a strong migration flow from neighboring countries, with repercussions on the establishment of a multi-ethnic society and an uncertain and fragile social fabric, and, on the other hand, because of the structure of the local distribution system, which is also represented in the case of organic products by large distribution groups, including multinational.
5.
Conclusions
In advanced countries, rising per capita incomes, combined with other factors and conditions (eating habits, new needs for food security, climate change, sustained migratory flows, and so on) have resulted in a profound shift in agri-food product demand, with a widening of consumer needs, through the demand for differentiated products (at least for quality, certification, safety, and information), and a higher value-added to overcome time constraints [59].
In such a scenario, new competition factors emerge that can determine competitive advantages for countries, such as product quality, price, logistics, traceability, and sustainability of agri-food products [60,61].
When reference is made to the consumption of sustainability products, the focus is mainly on organic farming products [62]. But, many other possible alternatives, from local products to fair trade, sustainability certifications, etc., can be understood within this framework. There is not enough information on how they influence purchasing choices [63].
For these reasons, the research conducted on a sample of consumers in the UAE and Sicily has allowed reaching results of particular interest for scholars and operators of the sector. Up to 500 consumers were interviewed using a questionnaire specifically designed to collect data and information on the characters' perceptions of the concept of sustainability, purchasing habits, consumption opportunities, perceptions of the positive and negative values of organic products, and socio-economic characteristics at various distribution facilities in the futures markets. There are positive correlations between income, age, cultural level, and sensitivity to sustainability, influencing purchasing choices. Some factors are common to the two areas: interest in local products, information, incentives to buy organic products, climate change mitigation, expert advice, and quality. In contrast, others are specific according to the degree of economic development and migration and the territory's social and food issues.
Ultimately, the potential of the assertion of sustainable and organic production and consumption models, considering the changes also taking place on the regulatory level, is linked both to the implementation of policies in favor of supply and to the support and promotion of demand [64,65].
In the first case, structural and infrastructural initiatives must be launched to address some technical-economic territorial and corporate problems in biological production (research and experimentation, also to adequately support the technical management of production in highly critical contexts, such as UAE; analysis of production situations, often fragmented and characterized by a combination of inefficient production factors, organization of supply, to increase the bargaining power of producers, identification of less complex distribution circuits, training and professional qualification of employees; territorial services, and of tangible and intangible resources to support production, etc.). About policies to support demand, adequate information campaigns are necessary for potential buyers and the promotion the quality of these products, the relative health content for a healthy, balanced and sustainable diet.
Finally, in front of sustainable consumption, the adoption of a possible label other than organic can help to reduce the information asymmetry that otherwise exists in the relationship between producer and consumer, resulting in an association focused on traceability and transparency throughout the production cycle (breeding/cultivation, processing, and marketing). The study has multiple implications because the international focus on sustainability has been widely revived in the era of reconstruction from the COVID pandemic.
Therefore, it is of interest to:
● policymakers committed to food sustainability to achieve the UN Sustainable Development Goals (SDGs) and the Paris Agreement targets to combat climate change and accelerate the transition to a low-carbon future;
● local institutions, called upon to find viable solutions to combine nutritional challenges, sustainable diets, and regional development;
● firms and private operators are called upon to find production and distribution solutions capable of providing answers to the expectations of society and consumers, who are increasingly attentive to the dimensions of health, safety, and "green" production. Age, education level, and income have determined different consumer segments towards which companies' marketing actions can be directed.
The main limitations of the study lie in the need to provide further replications to capture more consistent samples and more articulated behavioral patterns according to the different distribution contexts, all questions that need to be answered by future research.
Acknowledgments
This study was carried out thanks to the financial support of the Memorandum of Cultural and Scientific Cooperation between the "ABU DHABI DEVELOPMENT GROUP" (United Arab Emirates) and the University of Catania (Giuseppe Timpanaro, scientific coordinator).
Conflict of interest
The authors declare no conflict of interest.
Author contributions
Conceptualisation G.T. and P.G.; Literature review S.Z.; Qualitative Analysis and Data collection G.T., A.S. and V.T.F.; Methodology G.T. and V.T.F.; Software P.G. and S.Z.; Validation G.T., A.S., P.G. and V.T.F.; Wrote the paper G.T., A.S., P.G. and V.T.F.. All authors contributed to the manuscript revision, read, and approved the submitted and final version.









 DownLoad:
DownLoad: