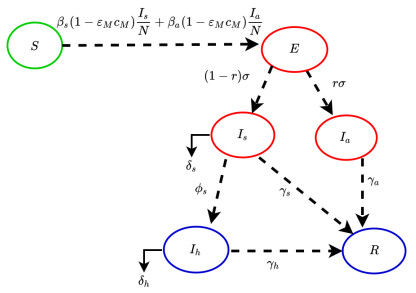
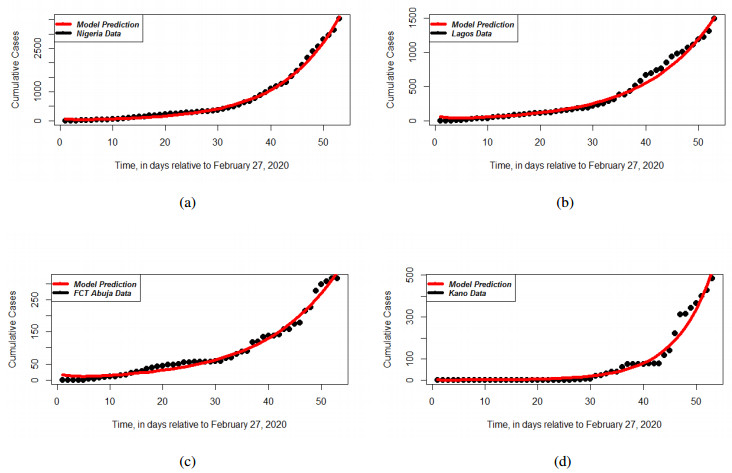
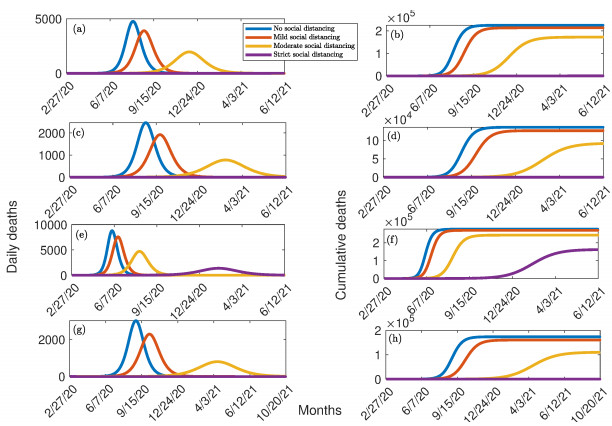
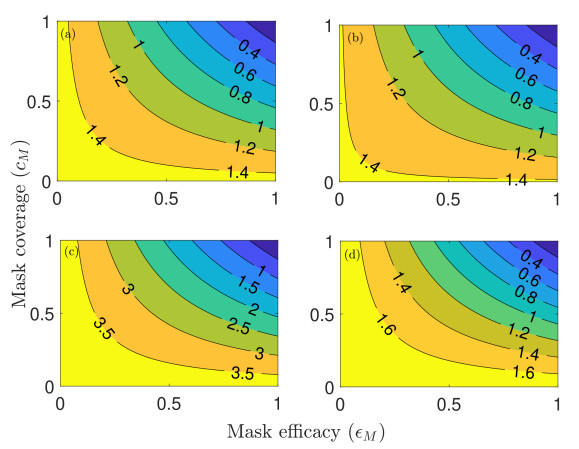
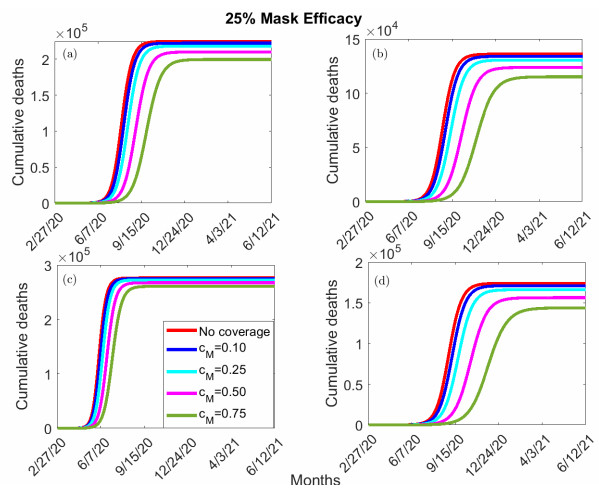
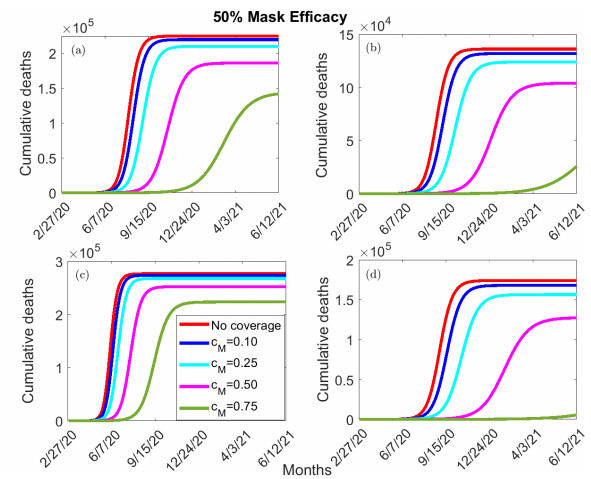
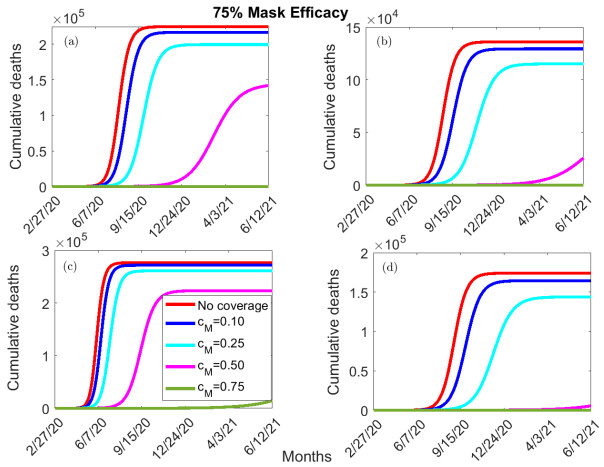
A mathematical model is designed and used to study the transmission dynamics and control of COVID-19 in Nigeria. The model, which was rigorously analysed and parametrized using COVID-19 data published by the Nigeria Centre for Disease Control (NCDC), was used to assess the community-wide impact of various control and mitigation strategies in some jurisdictions within Nigeria (notably the states of Kano and Lagos, and the Federal Capital Territory, Abuja). Numerical simulations of the model showed that COVID-19 can be effectively controlled in Nigeria using moderate levels of social-distancing strategy in the jurisdictions and in the entire nation. Although the use of face masks in public can significantly reduce COVID-19 in Nigeria, its use, as a sole intervention strategy, may fail to lead to a substantial reduction in disease burden. Such substantial reduction is feasible in the jurisdictions (and the entire Nigerian nation) if the public face mask use strategy is complemented with a social-distancing strategy. The community lockdown measures implemented in Nigeria on March 30, 2020 need to be maintained for at least three to four months to lead to the effective containment of COVID-19 outbreaks in the country. Relaxing, or fully lifting, the lockdown measures sooner, in an effort to re-open the economy or the country, may trigger a deadly second wave of the pandemic.
Nomenclatures: A: PV module area (m2); Eg: Energy gap of the semiconductor (eV); FF: Fill factor (%); ID, Io, Iph, Imp, IRS, Isc, Imo: Diode, saturation, photo-generated, maximum power point, reverse saturation, short circuit and module currents (A); k: Boltzmann constant 1.380649 × 10−23 (J K−1); n: Ideality factor; NP, NS: Number of cells in parallel and series; P: Electrical power (kW); q: Electronic charge = 1.602176634 × 10−19 (Coulombs); RS,Rsh: Series and Shunt resistance (Ohm); S: Solar irradiance (W/m2); Sref: Reference solar radiation (1000 W/m2); Tc: Cell temperature(℃); Tref: Reference temperature (25 ℃); V, VT, VMO, Vmp, Voc: Diode, thermal, module, maximum power point, open circuit voltages (V)
Greek Symbols: ƞ: Electrical efficiency(%); α,β,δ,γ: Temperature coefficient of short circuit current, open circuit voltage, fill factor and maximum power (%/℃)
1.
Introduction
The photovoltaic phenomenon is the most important technology that has been used to benefit from sun energy. It was used to convert solar energy into electrical directly through the so-called solar cell [1]. As a result of the development that took place in this field, the researchers have begun to develop and improve the performance of different types of solar cells like multi-junction, perovskite, and quantum [2,3,4,5]. Generally, the output electrical characteristics of PV solar cells are directly affected by the temperature variation. Whereas, the efficiency of the PV cell/module decreased with increasing the temperature [6,7] by 0.5% for each 1 ℃ [8,9]. Therefore, the influence of temperature variation on the behavior of PV cell/module should be described by the indicator of temperature coefficient. Where, the module temperature positively effects on the short circuit current (Isc) and negatively on both open circuit voltage (Voc), maximum power (Pmax) and fill factor (FF) [10]. In indoor conditions, the method of measuring coefficient of temperature is considered inaccurate due to the difference between the characteristics of sun light spectrum and the light that used in simulator of test [11]. In the same manner, inaccuracy of outdoor test occurs because it is not possible to obtain a uniform change in the increasing of sunlight [12]. In open circuit, the temperature coefficient is assumed to be equal to 80–90% of temperature coefficient of maximum power [13] and can be calculated according to the assumption that the energy gap linearly change with the temperature range. While, in maximum power, the temperature coefficient is defined as the summation of temperature coefficient of the Isc, Voc, and FF [14]. The main characteristics of indoor and outdoor measurements can be summarized according to: (1) the PV cell in indoor test is illuminated with solar simulator, and (2) the temperature of the solar cell should be stable with ±2 ℃ and temperature range should be at least 30 ℃ [15,16]. Besides, the oscillating of irradiance in outdoor should be kept within ±2% from the total solar irradiance, the wind speed not exceed 2 m/s, and the PV module temperature should be kept with ±2 ℃ of ambient temperature. According to the literatures review, a number of researchers were presented numerical and experimental studies to analyse the influence of temperature on the PV module performance. The experimental studies were divided between indoor and outdoor measurements. For example, Indra [17] studied the influence of temperature on the output characteristics of the polycrystalline PV module. The outdoor tests show that the maximum power decreased by 17% with changing the PV module temperature from 15 to 60 ℃. Moreover, Amelia et al. [18] investigated the effect of operating temperature on the PV module mono-crystalline type. At first, the manufactured output characteristics of the PV was simulated by PVsyst software. Furthermore, outdoor experiments were investigated using I-V tracer (PROVA 200). In addition, the temperature distribution is determined using a digital thermal camera. The outdoor results showed that a variation of output characteristics of the PV module compared with STCs whereas the output power and the efficiency of the PV module decreases with the increasing of the PV working temperature. Different types of the PV modules were tested indoor and outdoor by [16] to measure the temperature coefficients of Isc and Voc. The results of indoor test showed that a good agreement of the temperature coefficient with the results of the outdoor results. Singh and Ravindra [19] studied the influence of the temperature between 25 to 250 ℃ on the output characteristics of various types of PV modules. Their study was proved that the increasing of temperature lead to decrease each of the Voc, FF and efficiency. According to Jehad Adeeb et al. [20] study, the thin film module has less affected by increasing the temperature with less temperature coefficient about 0.0984% as compared with the other types. Where, the temperature coefficients were recorded 0.109%, for Mono-crystalline and −0.124% for Poly-crystalline. Based on the above literature review, it can be conclude that there is a relationship between the PV temperature and the outputs of the PV module, and can be determine the effects of the temperature on the PV output by indoor and outdoor tests. However, it can be seen that there is a limitation of using numerical analysis to determine the effects of the temperature on the PV outputs. For that, the main objective of this work is to study and analyze the effect of temperature on the output electrical characteristics of polycrystalline PV module using a numerical model validated by experimental test. In the numerical model, the I-V and P-V curves of the PV module were presented under the effect of module temperature ranges from 25 to 65 ℃ and solar irradiance ranges from 200 to 1000 W/m2 using MATLAB software. In addition, the validation of the simulation results are performed with experimental results for the PV module under real weather conditions using I-V tracer (SEAWARD PV200).
2.
Mathematical model
2.1. PV cell/module characteristics
The PV module is a group of solar cells connected in series or in parallel combination. Each cell has approximately 0.5 V. The electrical circuit of PV cell can be represented as shown in Figure 1.
From Shockley equation [22,23], the current and voltage of PV cell in zero illumination can be represented by:
where: V is the voltage across the cell, VT is the thermal voltage (k Tc/q), q is the electronic charge = 1.602176634 × 10−19 coulombs, Tc is the cell temperature (℃), k is the Boltzmann constant = 1.380649 × 10−23 J K−1 and n is the ideality factor of diode.
I0 is the diode saturation current (A) and can be defined as [24]:
where IRS is the reverse saturation current at STCs and Eg is the energy gap of the semiconductor used in the cell.
With the sunlight, the current of the solar cell is offset due to the photo current as [25,26]:
where: Iph is the photo-generated current (A) and expressed as [27]:
where: S is the instantaneous solar irradiance (kW/m2), Sref is the reference solar irradiance (1 kW/m2), Isc is the short circuit current at STCs and α is the temperature coefficient of the Isc.
In case of series resistance, the current generation described by [28]:
where: RS is the series resistance due to resistance of the silicon-bulk and contact material.
In case of parallel and series resistance, the current generation described by:
where: Rsh is the shunt resistor due to inhomogeneity of the surface and loss-current at the solar cell edges.
The voltage of the solar cell can be written as:
Equation 7 can be written in term of PV module as [24]:
In case of PV cells in series:
In case of PV cells in parallel:
where: mo represents the abbreviation of module, NS and Np are the number of cells in series and parallel respectively.
Generally, production power of the PV module can be defined as [29]:
while, the efficiency can be defined as [30]:
where: A is a PV module area.
The fill factor represents the ratio of maximum power to the theoretical [30]:
where Imp and Vmp are the current and voltage at maximum power point.
2.2. Temperature coefficients
The PV module efficiency depends on many types of losses. These losses can be confined as: thermalisation, transmission, emission, and angular mismatch and Carnot losses [10]. The increasing of PV temperature has a negative effect on these types of losses and can be represented by a temperature coefficients [31]. Commonly, the temperature coefficient is measured in indoor conditions and listed in the datasheet of the PV module. It can be classified into: Isc, VOC, FF and Pmax coefficients.
The coefficient of temperature for Isc [10], Voc [32], FF [33,34] and for Pmax [14] can be represented by equations from 13 to 16 respectively.
3.
Experimental details
A polycrystalline PV module made by Fortuner (FRS-165W) was selected in this study to achieve the experimental tests. The PV module is fixed on a steel frame with tilt angle 33o towards the south depending on the latitude of site as shown in Figure 2. The main specifications of the PV module is presented in Table 1. I-V tracer (SEAWARD PV200) was used to measure the output electrical characteristics of the PV module including ISc, VOC, Vmp, Imp, and Pmax. Furthermore, a solar meter (Survey 200R) devise was synchronized with the SEAWARD PV200 to measure the ambient conditions (air temperature and solar radiation) and back temperature of module. All the measured data were stored in a computer and reviewed by solar data logger and then displayed by SolarCert software. The wind speed was measured by a handle anemometer. In addition, an infrared thermal digital camera (visual IR thermometer VT02) was used to image of temperature distribution on the front and back sides of the PV module.
4.
Weather data
Iraq is considered one of the hot and dry countries in the summer season. Where, the maximum temperature reaches almost more than 50 ℃, especially in August month. Four days (1/8/2021, 5/8/2021, 11/8/2021 and 13/8/2021(were selected to measure the parameters of the PV module. As shown in Figure 3, the maximum average ambient temperature was reached about 51 ℃ at noon between (1:00 pm and 2:00 pm). Besides, the average solar radiation was recorded a maximum value about 940 W/m2 at noon as shown in Figure 4. While, the average wind velocity was not exceed 0.5 m/s in the morning at 6:00 am as shown in Figure 5.
5.
Results and discussion
5.1. Numerical results
A MATLAB code was built based on the set of Eqs (1–16) and the technical data of PV module that tabulated in Table 1 to analyse the I-V and P-V curves of the PV module. In addition, the variation of VOC, Isc, Pmax and FF were represented with module temperature to analyze the temperature coefficients. The I-V and P-V curves were represented in ranges of module temperature and solar radiation between (25 to 65) ℃ and (200 to 1000) W/m2. While, the analysis of temperature coefficients were investigated at (600, 800, and 1, 000) W/m2. As shown in Figure 6, the Isc was varied from (9.6 to 9.8) A when the module temperature was changed from (25 to 65) ℃ at constant solar radiation 1000 W/m2. While, the Voc was decreased from (22.05 to 20.2) V resulting in a maximum power changed from (146 to 165) W as shown in Figure 7. It is observed that the increasing the temperature of PV module has critical effect on decreasing the voltage rather than increasing the current. This can be explained as follows: Increasing cell temperature lead to reduce of band gap energy, which indicates to increase the absorbed photons that results in producing more free charge carriers. In other words, lower energy needs to liberate the free charge carrier. This means that the number of flow electrons with more current and low energy will increase (drop in voltage) [30,35].
On the other side, at constant temperature (25 ℃) and solar radiation varied from (200 to 1000) W/m2, the Isc, VOC were varied from (2 to 9.8) A and from (20.1 to 22.05) V respectively resulting in a maximum power range from (31 to 165) W as shown in Figures 8 and 9.
The variation of output characteristics such as: Isc, Voc, Pmax, and FF with the module temperature are plotted in Figures (10–13) for three values of solar radiation (600, 800 and 1, 000) W/m2. As shown in figures, the relationship seems to be a linear model with increasing trend in Isc and decreasing trend in Voc, Pmax, and FF. The temperature coefficients were calculated from the slop of straight lines presented in Table 2. As shown, the temperature coefficient (α) of Isc was obviously changed from 0.058 to 0.091 %/℃ when the solar radiation increased from 600 to 1000 W/m2. While, the temperature coefficient (𝛽) of Voc, (𝜸) of Pmax and (δ) of FF were little changed. As presented in Table 2, the regression equations of module are characterized by a high coefficient of determination (R2) with a range from 0.962 to 0.999. This is an indication that this regression correlation can be used to predict the output electrical characteristics of the PV module.
5.2. Experimental results
5.2.1. Temperature of PV module
The experimental investigation was investigated during four days of August (01/08/2021), (05/08/2021), (11/08/2021) and (13/08/2021) from 6:00 am to 2:00 pm at the center of Middle Technical University-Baghdad, Iraq. The output characteristics of PV module such as: Isc, Imp, Voc, Vmp, Pmax, FF, and electrical efficiency (ƞele) in addition to its back temperature were measured as shown in Figure 14. From figure, the maximum value of PV temperature was recorded about 69 ℃ in 13/08/2021 at 1:00 pm while the minimum temperature was recorded about 37 ℃ in 01/08/2021 at 6:00 am. According to the average value, the PV module temperature was recorded about 37.5 ℃ and 63 ℃ as a minimum and maximum value respectively. The module temperature was varied due to the variation of solar radiation and ambient temperature.
Furthermore, the front and back temperatures of the PV module were imaged using thermal digital camera for one day 01/08/2021 to compare between them as shown in Figure 15. The images were taken every one hour between 8:00 am to 1:00 pm. Generally, the PV back temperature was recorded a higher than the front temperature. Where, the maximum temperature of front and back was recorded 58.9 ℃ and 64.9 ℃ respectively at 11:00 am. This can be explained by the fact that the PV module was installed at average height not exceeding 1m from the ground surface (i.e., it closer to the ground) therefore, the air currents were very low. In addition, more heat will reflect from the ground surface toward the back surface of module.
5.2.2. Output electrical characteristics
Figure 16 shows the variation of module voltage with time. As shown, the Voc was slightly decrease from 20.75 to 20.35 V while, the Vmp was sharply decrease from maximum value 18.55 to 15.9 V due to the higher increase of module temperature. In contrast to this, the Isc and Imp were increased. Where, the Isc was increased from 1.72 to 7.32 A while, Imp was increased from 1.4 to 6.9 A as shown in Figure 17.
As shown in Figure 4, the average solar irradiance was increased from 220 to 920 W/m2 during 6:00 am to 12:00 pm. Therefore, the electrical power was increased from 25.9 to 131.2 W then dropped to 109.7 W at 2:00 pm because of decreasing the solar irradiance to 770 W/m2 as shown in Figure 18. This is accompanied by a decline in the electrical efficiency (ƞele) of the module from 15.6% to 13.3% and decreasing in fill factor from 76% to 71%.
5.3. Comparative of present results with previous studies in literature
The temperature coefficients of current, voltage and maximum power were compared with same studies in literature [10,15,36] as presented in Table 3. The comparison was depended on the same type of PV modules (polycrystalline) that found in the previous studies. The structure of table was built according to technical data of PV module, ambient conditions and temperature coefficients. As presented in Table 3, the temperature coefficient of current (α) was close to the coefficient temperature in [36] at solar radiation 1000 W/m2 while, the coefficients of voltage (𝛽) and power max (𝜸) were recorded greater than those in [36]. In the same manner, all the temperature coefficients in present study recorded less than the coefficients of reference [10]. Otherwise, they recorded greater than the coefficients in reference [15]. On the other side, the linear model with Coefficient of Determination (R2) of the current, voltage and power equations were compared also with [36] at 1000 W/m2 as shown in Table 4. The comparison shows a good agreement between the two studies since the R2 value was very close.
5.
Conclusions
The output electrical characteristics of PV module were investigated by numerical and experimental studies. In addition the temperature coefficients of PV module was analyzed numerically. Based on the analyses of the results, the following conclusions can be drawn:
• The numerical results conclude that the maximum power was recorded 165 W at 1000 W/m2 solar irradiance and 25 ℃ PV module temperature.
• The increasing of module temperature has critical effect on the voltage rather than the current.
• The minimum value of power was recorded about 31 W at 200 W/m2 of solar radiation.
• The temperature coefficient was recorded a maximum value with output power about (−0.26) %/℃. While, the temperature coefficient (𝛽) of Voc, (𝜸) of Pmax and (δ) of FF were little changed.
• The experimental results show that the maximum power was recorded 131.2 W at 920 W/m2 solar radiation.
Acknowledgments
This work was conducted within Middle Technical University and the authors are grateful to the staff of University for their help.
Conflict of interest
The authors demonstrate that there is no conflict of interest regarding this manuscript.










 DownLoad:
DownLoad: