1.
Introduction
Over the last decades, it is more and more evident that the global energy demands are rapidly increasing. This, along with the imminent fossil fuel deficiency, oblige governments and industries to make accelerated efforts toward producing green energy. The main focus is the marine environment, which is a vast source of renewable energy. The Global Offshore Wind Report of 2021 [1] from the Global Wind Energy Council (GWEC) states that from now to 2050, offshore wind is becoming the main medium for global decarbonization, transforming the electricity system in generation and infrastructure, as well as for production of green fuels like hydrogen. Offshore wind is a key technology in net zero scenarios, with fixed-bottom offshore wind being in rapid development until 2030 and floating offshore wind unlocking tremendous potential for fossil fuel displacement from 2030 and beyond.
Even though the majority of offshore wind farms consist of fixed-bottom structures, with the advances of technology into deeper waters, floating wind turbine platforms are capable of becoming more financially profitable due to the extreme abundance of the offshore wind potential [2]. Numerous concepts and projects of floating offshore wind turbines (FOWTs) have been studied and tested, such as the GustoMSC Tri-Floater [3], WindFloat [4], TetraSpar [5], NAUTILUS-10 [6], OC4-DeepCWind and OC5 [7,8]. There are also concepts that aim to integrate other forms of energy, such as wave energy, into a single system, through multi-purpose floating structures, or to integrate multiple wind turbines (WTs) together on a single platform [9]. Although hybrid systems capable of capturing simultaneously wind and wave energy sources are still far from commercial use (only a few concepts [10,11,12] have been reported in the marine sector), numerous studies on hybrid systems have been presented in the literature. Specifically, several wave energy absorption technologies, i.e., attenuators, point absorbers and flap type converters, have been coupled with FOWTs. For example, Bachynski and Moan [13] examined a combined energy system consisting of a wind turbine with three point absorber wave energy converters (WEC) installed on a tension-leg floating structure. Muliawan et al. [14,15] studied a combination of a spar-type floating turbine with Wavebob, i.e., a torus-shaped WEC, while Veigas and Iglesias [16] assessed the performance of a collocated wind and wave farm in Tenerife. Moreover, Michailides et al. [17,18] studied the combination of a semisubmersible wind turbine with three rotating flap-type WECs, and Gao et al. [19] compared the spar torus combination with the latter. Karimirad and Koushan [20] analyzed the dynamic response of a hybrid system based on the Hywind concept and a Wavestar type WEC. Finally, Wang et al. [21,22] assessed the responses of the combination of a spar-type floating wind turbine with a torus-shaped WEC [21] and of a floating wind turbine with three oscillating floater WECs [22].
In addition to the aforementioned wave energy absorption technologies, the Oscillating Water Column (OWC) systems are among the most widely studied solutions for coupled wind and wave energy conversion systems. Particularly, focusing on the last decade, Aubault et al. [23] studied the effect of the Power-Take-Off (PTO) on the inner free surface of an OWC device when it is considered as part of a FOWT system. In addition, Mazarakos et al. [24] presented a coupled hydro-aero-elastic analysis in the frequency and time domains concerning a hybrid TLP supporting a 5 MW WT and encompassing three OWC devices. The latter study was further extended by Mazarakos et al. [25], with scaled-down tank tests to extrapolate the effect of the air turbine type at the top of the OWC chambers to the platform's seakeeping. Perez-Collazo et al. [26] investigated experimentally a Jacket hybrid frame substructure, incorporating a WT and an OWC device, whereas Perez-Collazo et al. [27] examined an offshore wind monopile platform type integrating an OWC, considering regular and irregular waves. Sarmiento et al. [28] validated experimentally the hydrodynamic performance of a semisubmersible hybrid platform, encompassing three OWCs and a 5MW WT, moored with a catenary line system. Michele et al. [29] assessed the interaction effects of an OWC monopile system, supporting a WT, with regular and random waves. It was shown that the characteristics of the oscillating chambers affect the wave power extraction efficiency, whereas the presence of the internal cylindrical pile affects the sloshing eigenfrequencies. Furthermore, Zhou et al. [30] examined the wave loads on a monopile-mounted WT encompassing an OWC using a 3-D time domain numerical model. Recently, a multi-purpose floating TLP concept, named the REFOS platform, consisting of three OWCs and a 10 MW WT, was numerically and experimentally examined. In the latter studies, a hydro-servo-aero-elastic coupled model was developed in the time domain to examine the effect of the WT on the floater's seakeeping, [31,32,33].
Despite the fact that FOWTs are in the path of becoming competitive in the energy market, bearing in mind that they are extensively developed, tested and validated, it is critical to further improve cost-wise factors. The main disadvantage compared to onshore wind farms is that offshore wind turbines are more expensive and difficult to install and maintain, due to the variable and rough sea conditions, construction and operation phases, as well as additional infrastructure regarding the electricity transmission to shore [34]. One of these key factors that necessitate optimization and further research for economy is the mooring system of the structure. In this work, the Renewable Energy Multi-Purpose Floating Offshore System (REFOS) platform [31,32,33] will be utilized for wind and wave energy exploitation in specific locations in the Greek seas. An extreme value analysis will be also conducted in order to determine the designated range of environmental conditions at the installation sites. Additionally, a catenary mooring system will be specified and compared with the initial TLP arrangement in terms of power efficiency, while the energy output will be estimated for the selected locations. The current study can initiate further research and investigation in the field of offshore energy in the Greek Seas, prioritizing the trade-off between power efficiency and financial feasibility for commercial use of moored hybrid floating platforms that produce green energy.
The present manuscript is structured as follows: In Section 2 the wind and wave climate analysis for the potential installation locations in the Greek Seas are presented, whereas Section 3 deals with the hydrodynamic analysis of the floating structure. The analysis is implemented in the frequency domain, involving the solution of the coupled hydro-aero-elastic problem of the floater, the WT, the OWCs and the mooring system. In Section 4 the hydrodynamic behavior of the floating structure is presented in detail, and Section 5 is dedicated to the estimation of the absorbed wind and wave energy by the floating platform. The conclusions are presented in Section 6.
2.
Environmental elements
In this section, apart from the presentation of areas in the Greek seas suitable for offshore wind and wave co-exploitation, an extreme value analysis will be performed on metocean parameters. The results along with the sea-state frequency tables, will enable the definition of the mooring characteristics in ultimate limit states and the energy output estimation of the installed system.
2.1. Site selection and data
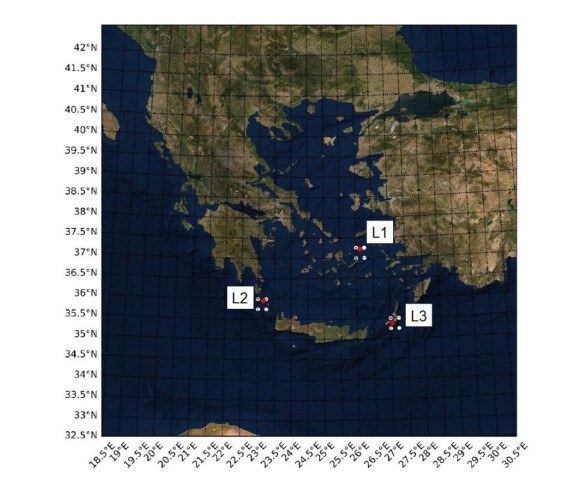
Offshore wind provides a more powerful source of energy than onshore wind. Focusing in the Mediterranean Sea, studies have shown that in terms of Levelized Cost of Energy (LCOE), the lowest values refer to the Gulf of Lion and the Aegean Sea (approximately 95€/MWh), where the wind resource is the best [35]. The offshore wind power potential of the Aegean and Ionian Seas has been analytically studied by Soukissian et al. [36], including annual and inter-annual variability characteristics using a 15-year hindcast of wind data. It is concluded that offshore deep-water locations, especially in the Aegean Sea, are characterized by high values of offshore wind resources (mean annual wind power density up to 885 W/m2 in the central Aegean and up to 584 W/m2 in the Ionian Sea). Due to the fact that the main concept of the present paper is to assess a multi-purpose floating structure in order to capture both wind and wave energy, the temporal relations between the two phenomena are also critical. Such study for the Greek Seas has been presented by Kardakaris et al. [37], in order to assess the complementarity and synergy between wind and waves and depict the most promising areas using EMODnet bathymetry [38]. In this context, three offshore locations were selected at approximately 200 m of water depth (Figure 1). The first location (L1) is east of Cyclades complex and south of Ikaria Isl. [37.220° N, 26.115° E] (at 230 m water depth), the second one (L2) is between Kythira Isl. and Antikythera Isl. [35.964° N, 23.160° E] (at 208m water depth), and the third site (L3) is located between Kasos Isl. and Karpathos Isl. [35.378° N, 27.038° E] (at 246 m water depth).
In this work, 20 years (2000-2019) of the ERA5 reanalysis wind and wave datasets [39] with a time step of one hour have been utilized for the Greek Seas (defined by a rectangle with the top left corner at 42° N, 19° E and bottom right corner at 33° N, 30° E). For the significant wave height and the wave energy period, the data are provided on a 0.50° × 0.50° spatial grid, while for the wind speed, the data are available at 100 m height (i.e., at a typical wind turbine hub height) on a 0.25° × 0.25° spatial grid. The ERA5 reanalysis dataset, produced by the European Centre for Medium-Range Weather Forecasts (ECMWF), combines vast amounts of historical observations into global estimates using advanced modeling and data assimilation systems [39]. Further works regarding sensitivity testing between the ERA5 wind and wave data and in-situ measurements can be found in [40,41,42,43].
2.2. Data preparation and sea-state tables
The ERA5 reanalysis dataset was spatially co-located with the selected locations via the nearby grid point values by using a simple form of inverse squared distance weighting interpolation function based on the values of the four nearest grid points. Denoting by x1, x2, x3 and x4 the respective variables (wind or wave parameters) at the four grid points surrounding the selected location and by r1, r2, r3 and r4 the corresponding distances from that location, the requested data for each variable at the specific site can be estimated as follows:
Regarding the wave data, the ERA5 reanalysis dataset refers to the wave energy period Te. Under the JONSWAP spectrum with a peak enhancement factor γ = 3.3, Tp and Te are approximately related as follows:
In Tables 1-3 the sea-state occurrences for locations L1, L2 and L3 are respectively presented.
2.3. Extreme value analysis (EVA)
In general, design parameters corresponding to environmental loads implied by wind, waves, currents, ice, etc. are used to evaluate the resistance of an offshore structure in the ultimate limit state, whereas the accurate estimation of design values greatly facilitates the analysis of different serviceability limit states and fatigue. In this respect, a bivariate EVA of wind and waves can produce 50- or 100-year return forces that would be experienced by a typical offshore system. Regarding EVA methods, the most widely used are the Block Maxima (BM) and the Peaks-Over-Threshold (POT). The latter will be used in this work for the EVA of the wave parameters for the examined locations, since the available sample size of annual maxima is rather poor (20 years) [44,45]. More applications regarding the estimation of metocean extremes can be found in [46,47,48]. A similar study has been conducted by Devis-Morales et al. [49] for the offshore Colombian Caribbean Sea, where a 24-year dataset of Cross-Calibrated Multi-Platform (CCMP) winds and a 35-year dataset of ERA-Interim significant wave heights were analyzed via BM method, POT method and the Method of Independent Storm (MIS). Further applications of the POT method can be found both regionally, e.g., with Hs data of Figueira da Foz, Portugal [50], and globally for ocean wind speed and Hs, estimating 100-year return periods from a 46-year ERA-40 dataset [51]. POT method has been also used for estimation of long-term trends in the frequency and intensity of severe storm waves [52], as well as for developing extremes' modeling, capturing both short- and long-range correlations with a fitted autoregressive fractionally integrated moving average (ARFIMA) model [53].
2.3.1. Peaks-over-threshold method and model diagnostics
In the present work, the Peaks-Over-Threshold method is utilized. For a detailed description of the method, see Coles [54]. The main challenge in the POT method is to choose a threshold u that balances bias and variance. Too low a threshold is likely to violate the asymptotic basis of the model, leading to bias, whereas too high a threshold will generate few excesses with which the model can be estimated, leading to high variance. In this context, two approaches are available for threshold selection: One is an exploratory technique carried out prior to model estimation; the other is an assessment of the stability of parameter estimates, based on the fitting of models across a range of different thresholds [55]. Regarding the first approach, theory suggests that when the generalized Pareto distribution is appropriate, the sample mean of the threshold excesses of u changes linearly with u. This leads to the mean residual life (MRL) plot, which constitutes the locus of points
where x(1) , …, x(nu) consist of the nu observations that exceed u, and xmax is the largest of the x(i). Above a threshold u0 at which the generalized Pareto distribution provides a valid approximation to the excess distribution, the MRL plot should be approximately linear in u. In the second approach for threshold selection, the aim is to estimate the model at a range of thresholds and to look for stability of parameter estimates. This argument suggests plotting both σ∗=σu−ξu (modified scale) and ξ (shape) estimates of the generalized Pareto distribution against u, together with confidence intervals for each of these quantities, and selecting u0 as the lowest value of u for which the estimates remain near-constant. The parameters of the generalized Pareto distribution can be estimated by the Maximum Likelihood (ML) method.
In offshore and coastal engineering applications, the concept of return period and design value is chosen as common practice to adequately cover the ultimate limit state scenarios. The formal definition of the return period implies that the n− year design value is expected to be exceeded on average once during the next n years. The period of n years is called the return period, associated with the design value [54]. In this case, the N -year return level zN is estimated through the following relation:
Where ζu denotes the exceedance probability or the proportion of data above a threshold u, and ny denotes the exceedance observations per year. When ξ=0, equation (4) simplifies as follows:
The quality of the fitted generalized Pareto model can be assessed by probability (PP) and quantile (QQ) plots. For a threshold u, threshold excesses y(1) ≤…≤ y(n) and an estimated model ˆH, the probability plot consists of the pairs
where
while the quantile plot constitutes the locus of points
where
provided that ˆξ ≠0. If ˆξ = 0 the equations (7) and (9) are modified, accordingly, as follows:
Provided that both plots depict a very close relation between theoretical and sample quantities, the model diagnostics are completed, and the model is adequately identified.
2.3.2. EVA results
After the necessary modifications in the datasets described in section 2.2 and the methodology presented in (2.3.1), an EVA on significant wave height (Hs), peak wave period (Tp) and wind speed (Uw) at 100 m above sea level was performed for the three examined locations via a Python script based on [55].
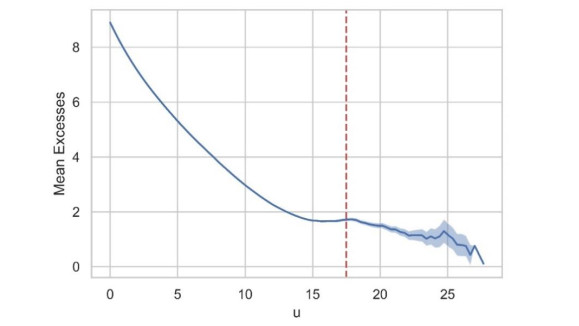
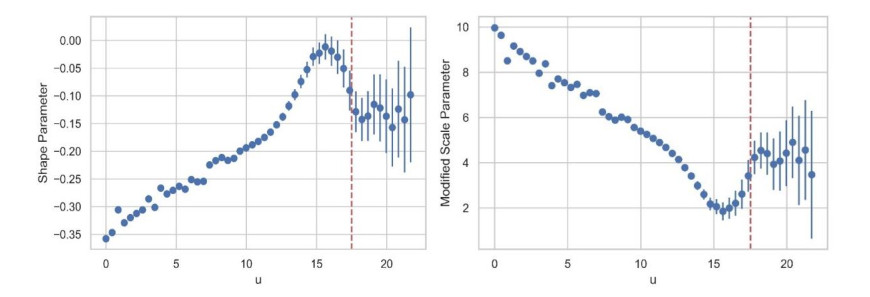
The first step of the analysis is to select an appropriate threshold level. For that purpose, the MRL plot (Figure 2) and the shape (ξ) and modified scale (σ∗) stability plots (Figure 3) were used. Τhe threshold u should be located at the end of the linear part of the curve in the MRL plot and on the constant part of both stability plots [54]. Regarding the first plot, it is linear approximately up to u ≈ 13, and then it curves and returns to linearity for u between 18 and 22. Based on this behavior, a threshold of 15, 18, 20 or 22 can be potentially selected. However, when stability plots are taken into account, the error bars above the threshold u = 18 become significant, suggesting an increased variance in this interval. Although a fair selection is u = 15, as it meets all the aforementioned requirements, a slightly higher threshold was selected (u = 17.5) after trials, as it did not negate the variance prerequisites and provided more consistent results, as will be discussed below. Besides, such approach is in favor of safety when designing a project, since EVA results are utilized for robustness in ultimate limit states. A similar procedure was followed for all locations, and the resulting thresholds are shown in Table 4.
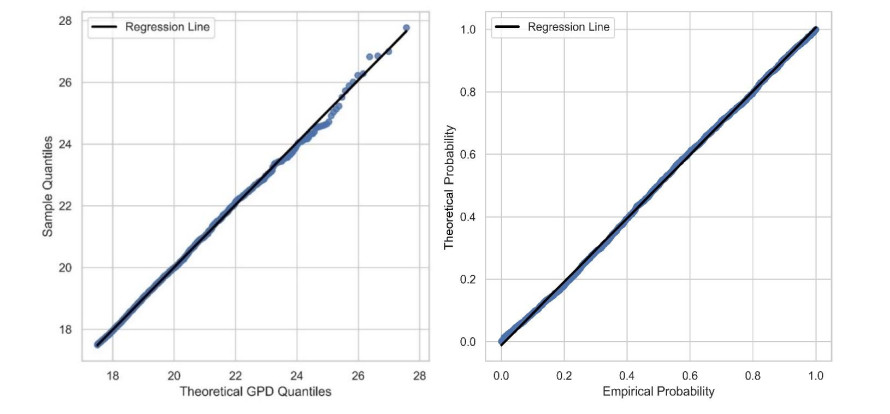
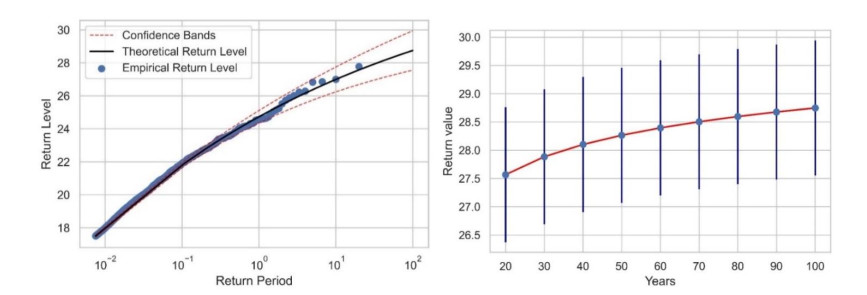
Having selected the thresholds, diagnostic plots for the fitted generalized Pareto model are shown in Figures 4 and 5. None of the plots gives any real cause for concern about the quality of the fitted model, which supports the bias-variance trade-off. Furthermore, the confidence bands at the return level plot are rather narrow.
The extracted return values of wind speed at location L1 for 20-100 year return periods are shown in the right panel of Figure 5, along with the corresponding error bars. All the relevant results are also summarized in Tables 5-7. For location L3, the return levels of the wave parameters are smaller compared to the locations L2 and L3. Regarding wind speed, location L1 exhibits the largest design values compared to L2 and L3, as location L1 is characterized by strong winter and summer winds (Etesian winds).
3.
Multi-purpose floating platform and mooring system
In order to exploit both offshore wind and wave energy via an individual structure, the Renewable Energy Floating Offshore System (REFOS) [31,32,33] is selected to be installed at the three examined locations presented in the previous section. The REFOS platform consists of a main cylindrical column supporting the DTU 10 MW reference wind turbine (RWT) [56] and three oscillating water column (OWC) WECs in a triangular configuration placed at the corners of the platform. Each OWC comprises an internal offset vertical cylinder and an external coaxial cylindrical shell. In the annulus area formed between the two solids, oscillations of the water column are developed. Consequently, the produced air volume flow generates an air turbine, located at the chamber's top, which is coupled to an electric generator (see Figure 6). The initial design ensures station-keeping via three lines spread symmetrically about the structure's vertical axis, which are located at the bottom of the OWCs' offset columns. In Table 8 the geometric characteristics of the examined platform are summarized, whereas its mooring properties are presented in Table 9.
3.1. Hydrodynamic formulation
The detailed hydrodynamic problem has been reported extensively in [31,32,33]. Nevertheless, for completeness, a short outline of the relevant formulation is presented below.
The floating platform is considered to be excited by a plane periodic wave of amplitude H/2, wave frequency ω and wave number k. The water depth, which is assumed as constant, is d=200 m. Small amplitude, inviscid, incompressible and irrotational flow are assumed so that linear potential theory can be employed. A global Cartesian co-ordinate system O-XYZ with origin on the seabed and its vertical axis directed positive upwards is used. The system coincides with the vertical axis of the central cylindrical body, which supports the WT. Also, four local cylindrical co-ordinate systems (rq,θq,zq), q = 1, 2, 3, 4 are located on the seabed with their vertical axes pointing upwards. The latter coincides with the vertical axis of q solid, q= 1, 2, 3, 4 (i.e., three OWCs and one central cylindrical body). The fluid flow around each q body, defined by Φq(rq,θq,zq,t)=Re{φq(rq,θq,zq)e−iωt}, can be written as
where φqD denotes the diffraction potential around the q body when the latter is considered fixed in the wave impact, with inner atmospheric air pressure head. The φqpj stands for the motion radiation potential around the q body, due to the forced oscillation of the p body in the j direction, with unit velocity amplitude ˙ξpj0. Here, the air pressure inside the OWC chambers is also assumed equal to the atmospheric. The term φqpP denotes the pressure radiation potential around the q body, due to unit time harmonic oscillating pressure head pp0 in the p OWC device. Here, the bodies q and p are considered fixed to the wave train.
The velocity potentials φqD(q=1,..,4),φqpj(q,p=1,…,4;j=1,…,6),φqpp(q=1,…,4;p=1,2,3) have to satisfy the Laplace equation in the fluid domain and the following boundary conditions.
On the seabed (z=0),
On the mean wetted surface,
At the outer free surface of each body (z=200),
At the inner free surface of each device (z=200),
In Equation (14), the term ∂()/∂→nq stands for the derivative in the direction of the outward unit normal vector →nq to the mean body's wetted surface. The term npj denotes the generalized normal components defined as →np=(np1,np2,np3) and →rp×→np=(np4,np5,np6), where →rp is the position vector with respect to the origin of the coordinate system. Finally, a radiation condition should be fulfilled, which states that the propagating disturbances must be outgoing.
The velocity potentials φqD,φqpj,φqpp are evaluated through the method of matched axisymmetric eigenfunction expansions. Hence, the fluid field around each solid can be subdivided into coaxial ring-shaped regions in which different expressions of the velocity potential can be established. These velocity potential solutions are matched by the continuity requirements of the hydrodynamic pressure and radial velocity along vertical boundaries of adjacent fluid regions and the fulfillment of the kinematic boundary condition at the bodies' vertical walls. Regarding the wave interaction phenomena between the array's members and the incoming waves, the multiple scattering approach has been applied. The latter is based on the superposition of the incident wave potential and various orders of propagating and evanescent modes that are scattered and radiated by the array members. The method of matched axisymmetric eigenfunction expansions and the multiple scattering approach have been thoroughly presented in [57,58,59], so they are no further elaborated here.
Having calculated the velocity potentials in all fluid regions, the time dependent volume flow Qq(rq,θq,zq,t)=Re{qq(rq,θq,zq)e−iωt}, produced by the oscillating internal water surface in the q device, q= 1, 2, 3, equals
where Sqi is the cross sectional area of the inner water surface inside the OWC device q.
In our case, a Wells-type air turbine is assumed to be placed in each device's duct between the chamber and the outer atmosphere since it rotates in one direction regardless of the direction of the air flow. The pneumatic admittance of the air turbine is considered equal to the one by [60], i.e., Λq=343.848m5/(kNs). For simplicity, the total volume flow qq in the q device is proportional to the chamber air pressure [58]:
In Equation 18, the term Λq attains the same value regardless of the examined device q.
Finally, the absorbed power, Pq, of each q OWC device of the array, via Equation 18, can be written as [61]
3.2. Mooring system
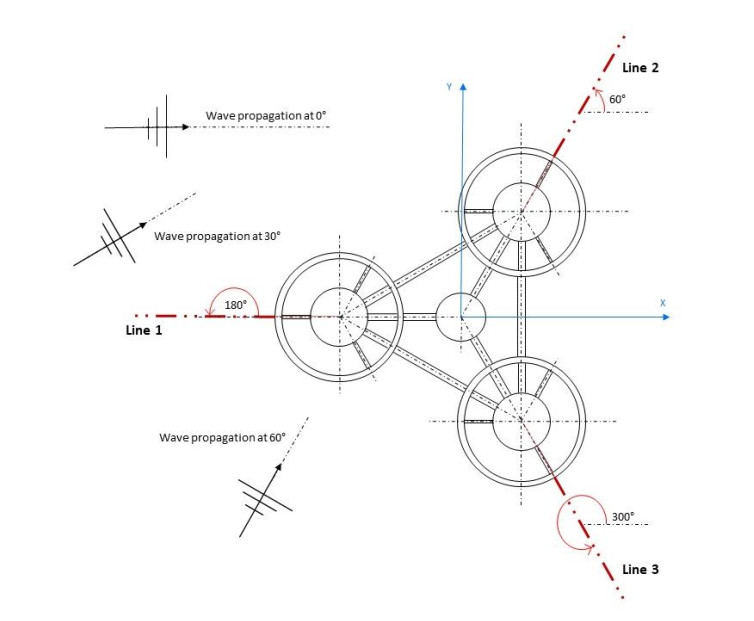
In this section, the characteristics of the mooring system of the REFOS platform are determined. Initializing the procedure, it is necessary to decide the mooring system configuration, regarding the number of lines as well as their directions. In the present work, a catenary mooring system is selected for the REFOS structure. The system is assumed to consist of 3 lines, one attached to each inner concentric cylinder of each OWC device (see Figure 7). The fairlead coordinates as well as the line angles with respect to the global coordinate system (i.e., originating from the sea surface and the center of the central cylindrical body supporting the WT) are as follows.
Line 1: (x, y, z, α) = (−28.87 m, 0 m, −20 m, 180 deg)
Line 2: (x, y, z, α) = (14.43 m, 25 m, −20 m, 60 deg)
Line 3: (x, y, z, α) = (14.43 m, −25 m, −20 m, 300 deg)
In terms of materials, a studless chain of Class R3 is used for the mooring lines. The installation depth was considered the same with the TLP concept (about 200 m) for comparison purposes. Moreover, for the maximum offset criterion δx, it holds that δx/D = 6%. Here, D stands for the distance of the fairlead from the seabed, i.e., D = 180 m. The line characteristics and the resulting horizontal pretension are shown in Table 10.
Last but not least, the stiffness matrix with respect to the global coordinate system was estimated and is shown in Table 11.
3.3. Coupled hydro-aero-elastic formulation
In order to investigate the dynamic equilibrium of the forces acting on the REFOS structure as well as to determine its total responses, the following system of differential equations of motion, describing the coupled hydro-aero-elastic problem in the frequency domain, was formulated, taking also into account the catenary mooring system that is applied instead of the TLP arrangement.
Here, Ai,j , Bi,j are the hydrodynamic mass and potential damping coefficients of the platform [31]; fTi and fTP,i are the exciting and the pressure hydrodynamic forces acting on the platform [31]; Ξj0 is the motion component of the REFOS system in the j-th direction with respect to a global co-ordinate system; Mi, j is the platform's mass matrix; CHi,j is the platform's hydrostatic restoring stiffness matrix. Regarding the contribution of the wind turbine, the mass matrix MWTi,j includes the WT inertia (including the gyroscopic effects due to rotation), the term BWTi,j denotes the damping matrix that includes the WT damping due to rotation and aerodynamics, and finally, its stiffness matrix Ci, jWT includes the contributions from both aerodynamics and gravity [31]. The remaining terms Bi, jm and Ci, jm are the mooring system's damping matrix and restoring stiffness matrix, respectively, with respect to the global co-ordinate system as well. The reader is referred to [31,32,33] for analytical representations of the above coefficients, as well as for a detailed presentation of the coupled hydro-aero-elastic formulation.
4.
Calculations and results
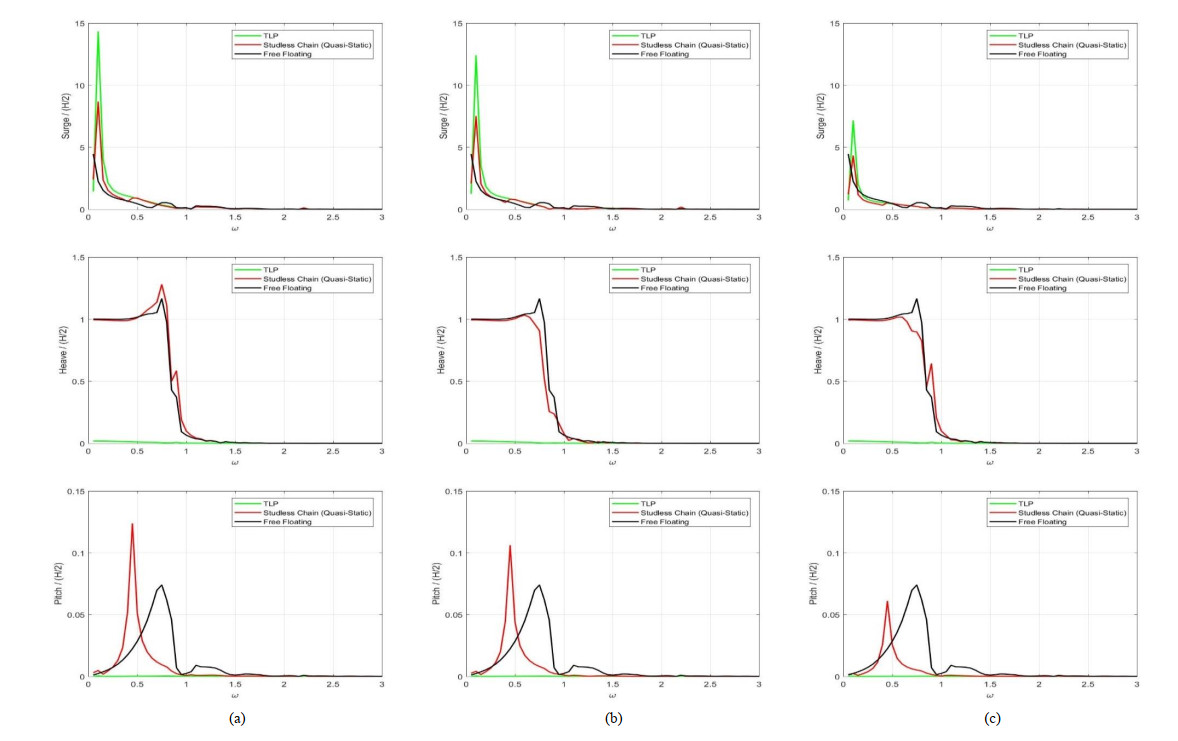
The hydrodynamic analysis of the REFOS components was conducted using the HAMVAB software [25,31,63]. The latter was preferred in the present contribution against other available numerical tools since, by keeping the same accuracy with them [64,65], it represents an efficient tool in the early design phases of such floating structures without requiring much RAM or computational time (i.e., central computational unit (CPU) time for each wave frequency is about 56 s). Setting the new data in the input files of the program along with all the respective specifications of the mooring lines, the analysis was performed in the frequency domain at a range of ω = [0.05, 3] rad/s with a step of 0.05 rad/s, as well as for three different wave directions, i.e., 0 deg, 30 deg and 60 deg, with respect to the global coordinate system (see Figure 7). For comparison purposes with the quasi-static analysis, a free-floating case was also investigated, whereas TLP case data were already available [31,32,33]. The motions of the floater can be expressed in terms of the response amplitude operator (RAO). The dynamic modeling enables the evaluation of the dynamic tension at the top of each line and subsequently the mooring line damping and the mooring restoring coefficients. To perform such calculations demands an iterative procedure, where RAOs need to converge to some extent of precision, re-evaluating the dynamic tensions of the lines. From this, the mooring system's damping matrix and restoring stiffness matrix can be derived, consequently resulting in the sought-for matrices for each investigated frequency [66].
In Figures 8 and 9, all cases are compared referring to the three wave directions respectively. The figures display the RAOs of surge, heave and pitch motions and rotations, along with the pressure inside each OWC device. Regarding motion RAOs (see Figure 8), higher values in surge motion are depicted in the lower frequency range. Concerning the maximization of the surge RAO of the moored platform, this can be traced back to the mooring restoring stiffness, which imposes a resonance location in the surge motion at ω≈0.1 rad/s. In the heave direction, the RAO motions of the free floating and moored with catenary lines structures start their variation from unity for ω tending to zero. However, this is not the case for the TLP case, since the appeared small responses are expected, as by definition the mooring system is more rigid. Catenary system responses are also shown to be close to the ones of the free-floating case, except for pitch motion, where the peak of the figure is shifted in lower values of ω. As far as the effect of the wave direction on the motions of the structure is concerned, it can be seen from the figures that the wave heading angle does not seem to affect the platform motions since the RAOs in the examined directions attain a similar variation pattern regardless the wave direction angle.
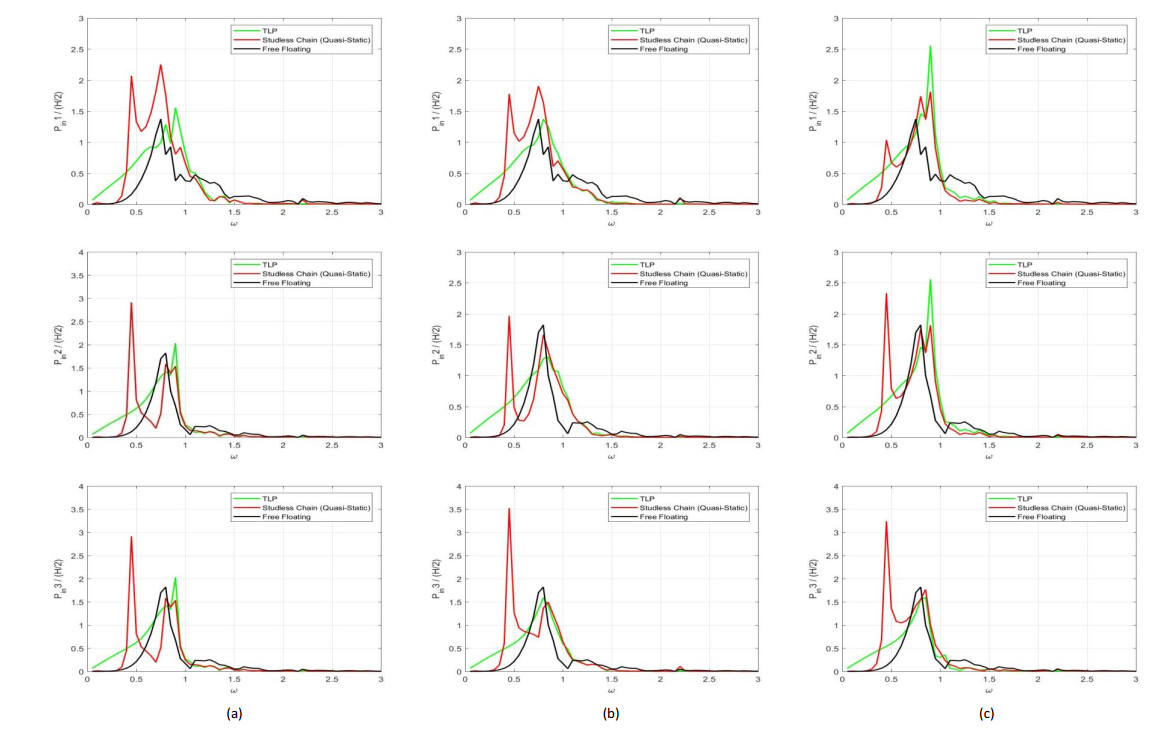
In Figure 9 the inner air pressure head of the three OWCs is presented for the examined wave heading angles. The results indicate that the angle of the wave train and the location of the OWC to the wave impact affect the values of pq0,q=1,2,3. It is shown that higher pressure values are always developed in the windward OWCs; however, depending on the wave direction, equivalent results occur in the leeward ones. Furthermore, it can be seen that pq0 attains a peculiar behavior at the neighborhood of ω≈0.8 rad/s regardless of the wave direction angle, the examined device and the mooring type. This ω value corresponds to the frequency where the pumping resonance of the water column inside the oscillating chamber occurs. Also, the inner air pressure of the OWCs in the catenary mooring case attains a maximum at ω≈0.45 rad/s. This can be traced back to the pitch motion of the structure, which maximizes also at the same wave frequency.
For investigating the dynamic effects, a spring and damping constant were required to perform the dynamic analysis of the mooring system. Regarding the examined frequencies, the range [0.95, 1.15] rad/s was investigated, as it corresponds to frequently observed sea-states. Table 12 presents the surge and heave responses (RAOs), Ξj0,j=1,3, as well as the absorbed wave energy by the OWCs, Pq,q=1,2,3. It can be observed that the heave responses (Heave) of the TLP hybrid structure attain the least values compared to the quasi-static and dynamic cases. Also, the absorbed wave energy (Eabs) from the TLP structure is higher than the corresponding values of the other two examined mooring cases, for ω = 0.95 rad/s and ω = 1 rad/s. On the other hand, the rest of the examined frequencies show increased Pq values that surpass the respective ones from the TLP case. This can be traced back to the dynamic effect of the moorings, which seems to be beneficial for the air pressure head inside the OWCs, and consequently to the absorbed wave power.
5.
Energy output estimation
In this section, the amount of the offshore wind and wave energy produced via the discussed multi-purpose platform will be estimated, comparing also the alternative mooring systems in terms of efficiency. During its operational phase, the installed wind turbine, i.e., the DTU 10 MW RWT, will be producing energy continuously except for the maintenance periods and during the hours with wind conditions outside the operating range. Offshore sites are characterized by strong and steady wind regime, so a high energy output is anticipated. The power curve of the DTU 10MW RWT as well as the rest of the specifications can be found in [67]. The cut-in—cut-out range is [4,25] m/s, and the rated speed is 11.4 m/s, while the manufacturer states that the rated power is 10640 kW.
As far as wave energy converters are concerned, it is necessary to evaluate the produced energy depending on the installation site sea-state conditions. From a mathematical scope, a simple approximate analytical solution has been derived for the efficiency of wave energy absorption of an OWC wave energy device [68]. However, since moored structures are discussed in this work, the influence of the mooring system cannot be neglected, as it affects the structure's responses. Such work, comparing a TLP and a catenary mooring system towards the OWC's efficiency, has proven the former as the most efficient case in the entire band of the examined wave frequencies [69]. Although the benefits of this higher efficiency are very important, they are mitigated due to the high construction and installation costs of the TLP moorings, which set the motivation for further investigation on more economic solutions.
5.1. Offshore wind energy
As already mentioned, the produced energy will be calculated regarding the three examined locations mentioned in Section 2. However, the wind speed timeseries refer to 100 m asl, while the hub height of the DTU 10 MW RWT is 119 m. Thus, the data were adjusted accordingly given that
where uh2 (m/s) is the calculated wind speed at height h2 (m), uh1 (m/s) is the known wind speed at height h1 (m), and z0 (m) is the roughness length equal to 0.0002 m for neutral atmospheric conditions.
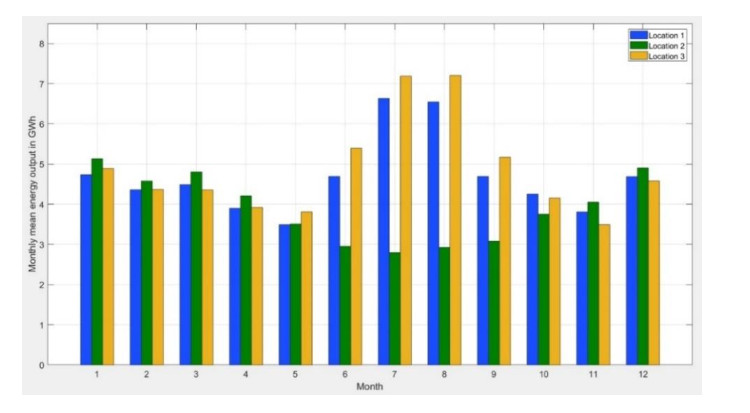
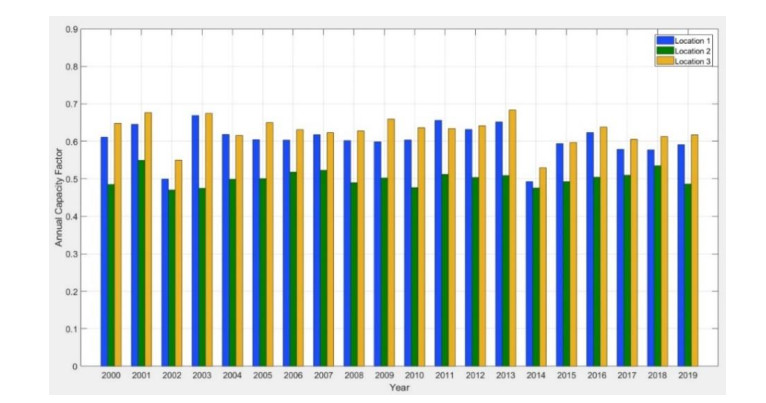
The mean annual energy as well as the monthly mean energy can be estimated, analyzing the 20-year datasets. For the former one, each year is analyzed separately, while for the latter, a monthly clustering is needed. Lastly, due to the fact that the timeseries are in 1-hour timesteps, the sum of the power values that correspond to the wind speed data based on the power curve will result in the sought-for energy. Τhe results are shown in Figures 10 and 11. Regarding the three locations, the annual energy output is relatively higher in locations L1 and L3 than in location L2, while the same feature is observed in the summer period at the respective monthly mean values (6.5-7 GWh on average in July and August). The exact opposite phenomenon can be seen to occur in the winter period, yet at a much lower intensity. The aforementioned observations verify and prove the presence of the strong Etesian winds in the Aegean Sea, which mostly affect locations L1 and L3 in our case. As far as energy output is concerned, these locations produce 56.30 GWh/y and 58.55 GWh/y on average, respectively, while for L2 the production is limited to 46.70 GWh/y. In general, seasonal periodicity is clearly shown in the monthly results, while similar conclusions for inter-annual cycles require longer datasets. Moreover, the capacity factor (CF) is calculated for every site and year (Figure 12). The capacity factor is the ratio of the actual energy produced in a given period to the hypothetical maximum possible, i.e., full time operation of the WT at the rated power. In our case, full time at rated power equals 365 d × 24 h × 10640 kW = 93206400 kWh/y, and the results correspond to the produced energy depicted in Figure 10. Regarding the discussed locations, L1 and L3 appear to be really promising sites with CFs of 60.4% and 62.8% on average, respectively, while L2 is also a very reliable choice, with a CF of 50.1%. Such CF values are relatively much higher than the ones that appear in a typical onshore project, yet in these sites there is a very high frequency of occurrence of wind speeds in the operational WT range of [4,25] m/s (86.5% for L1, 81.3% for L2 and 87.5% for L3). Consequently, the intense wind climate in these offshore locations may render such projects reliable and promising for financially feasible production of green energy.
5.2. Wave energy
Installing the REFOS platform at the investigated installation sites, OWC devices will produce energy depending on the wave climate and the structure's responses. In order to estimate the absorbed energy from the devices, the JONSWAP spectrum, provided by DNV [70], and absorbed energy results, Pq, for each device should be utilized. Regarding the JONSWAP spectrum, SJ(ω), which corresponds to developing sea-states in fetch limited situations, it is formulated via the Pierson-Moskowitz spectrum that describes fully developed seas. Subsequently, the absorbed power per sea-state (spectrum) can be calculated via Equation 22. At this stage, the results are independent of location yet depend on the mooring system and wave direction:
where Pq is defined in Equation (19).
The absorbed energy per sea-state is estimated by multiplying the tables of sea-state occurrences with the ones of the absorbed power and by dividing all the cell values with the 20 years of the examined data, in order to derive the mean annual absorbed energy per sea-state, per location and for each mooring system case. By adding all cell values, the total mean annual wave energy absorbed from the OWC devices is calculated for every case, and the final results are presented in Table 13. Clearly, location L2, which is characterized by the greater fetch lengths and sea-state conditions, has the highest wave energy production. Comparing the other two installation sites, location L3 exhibits the lowest wave energy, due to the topography of the area. As far as the mooring system is concerned, the TLP design is characterized by the highest efficiency, as it is by definition the most rigid solution. However, the catenary mooring system also presents high energy production. Comparing all cases in terms of the wave direction, peak values appear when the excitation wave propagates at 60 deg, while the opposite is observed for the 30 deg wave.
As far as the results of the dynamic analysis are concerned, wave energy calculations were performed regarding two wave frequencies: ω = 0.95 rad/s and ω = 1.15 rad/s. These correspond to the [6,7] (s) and [5,6] (s) peak wave period classes, respectively. The latter classes are characterized by rather high frequency of occurrences based on the analysis in Section 2.2. Table 14 presents the results from the dynamic analysis along with the respective ones from TLP and quasi-static approach for comparison purposes. It is observed that peak values of the wave energy for the TLP case attain higher values regardless of the examined location and the investigated sea-states. However, for a more comprehensive overview of the produced wave energy, dynamic results of every frequency are necessary to yield the complete power tables for every installation site.
6.
Conclusions and discussion
Offshore wind is characterized as the main medium for generating green energy in the near future [1]. The integration of technologies to exploit ocean wave energy potential is also in the spotlight for more effective offshore power plants. In this work, a multi-purpose hybrid (wind and wave energy) floating platform with two different mooring systems has been assessed at three locations of the Greek Seas. In this respect, the environmental conditions of the examined areas have been analyzed along with an extreme value analysis of the wind and sea states based on the Peak over Threshold method. The respective results were accounted for in the catenary mooring line system specification, which was also investigated in terms of power efficiency, performing both quasi-static and dynamic analyses. Dominant regarding the energy potential and preferred among others due to bathymetry, the selected locations were evaluated through their offshore wind and wave energy potential output.
In the frame of the POT method, it is shown that higher threshold selection than the one suggested from theory gave improved results based on the relevant diagnostic plots. All selections did not negate variance prerequisites and supported the bias-variance trade-off. Moreover, regarding return levels, the lowest wave design values correspond to location L3, while the highest wind speed design values were provided for location L1, due to the strong winter and summer (Etesian) winds as well as the lack of near highland. As far as the ultimate limit state analysis is concerned, the results of EVA were not critical for the floating system. The maximum 100-year return values of the examined variables were around 30 m/s and 7 m for wind speed and significant wave height, respectively, at L1 and 12.5 s for peak wave period at L2. Assuming an extreme sea-state of [Hs - Tp] = [7-12.5], the structure did not show any cause for concern.
Concerning the energy output, higher offshore wind energy is capable of being produced in locations L1 and L3, due to the strong wind climate all year round. Location L2 is also a very promising site and showed a relatively high capacity factor, yet it presented the highest wave energy production, due to the greater fetch and swells that characterize the area. Regarding wave direction, the 60 deg wave gave the highest wave energy outputs overall, while the lowest results were derived from the wave propagating at 30 deg. Comparing the two main mooring systems in terms of efficiency, TLP represents the best-case scenario. Expected results were also determined with regard to the quasi-static and dynamic analyses. The dynamic approach presented higher peak wave energy production in the respective investigated sea-states. However, all frequency results are necessary to yield the complete power tables for every installation site and gain a more comprehensive overview of the energy status.
Considering all of the aforementioned facts regarding the research conducted for the purpose of this work, it can be concluded that catenary mooring systems are a very competitive solution for multi-purpose floating structures and floating wind turbines in general. Their performance in station-keeping as well as in ultimate limit states promises a reliable and robust design along with construction and installation financial feasibility. Such projects can become profitable, taking into account the decreased overall costs that lead to a lower levelized cost of energy (LCOE). Catenary mooring systems should also be studied regarding the dynamic responses of the lines, especially integrating more phenomena in the models such as vortex-induced vibrations (VIVs), seabed interactions and internal damping due to friction between the chain links, as well as avoiding snap loads and fatigue. The additional effects of mooring induced damping further decrease motions, which is expected to increase the efficiency of the OWCs. Further assessments, such as those described in Duan et al. [71] and Russo et al. [72], should also be performed regarding the offshore wind turbine performance, as applied coupled motions tilt the rotor and alter the turbine's operation. Moreover, fatigue analyses and anchor design should be applied as well, while optimization of the air turbines of the OWCs would further contribute to making multi-purpose floating structures competitive in the energy market for offshore wind and wave co-exploitation.
Author contributions
Conceptualization, T.H.S. and D.N.K.; methodology, T.H.S. and D.N.K.; software, K.K.; validation, K.K.; formal analysis, T.H.S., D.N.K. and K.K.; investigation, K.K.; writing—original draft preparation, K.K.; writing—review and editing, T.H.S., D.N.K. and K.K. All authors have read and agreed to the published version of the manuscript.
Data availability statement
The ERA5 reanalysis dataset has been obtained from https://cds.climate.copernicus.eu (accessed on 3 March 2021).
Acknowledgments
The authors wish to express their appreciation to Professor Emeritus Spyridon Mavrakos, coordinator of the REFOS project [73], who provided the required data for the TLP moored floating structure.
Conflicts of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad: