Stabilization of wind farm integrated transmission system with input delay

1.   Introduction

In recent years the problems of control and stabilisation for thermoelastic systems have been studied intensively. We refer for instance to [22], [42] in the context of controllability, and to [14], [18], [23], [24], [35] for stabilization, among others.

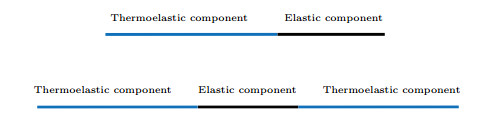
A closely related interesting issue is the asymptotic behaviour of a system consisting of two different materials joined together at the interface, one being purely elastic and the other thermoelastic (see Figure 1). In these systems, other than the intrinsic coupling effects of thermal and elastic components, typical in thermoelasticity, the thermoelastic and purely elastic rods are also coupled through the interface.

Exponential and polynomial decay properties for this kind of systems were proved by Rivera et al. in [12], [28], [31] and Messaoudi et al. in [30], using the energy multiplier method. Han and Xu in [15] got a sharp polynomial decay rate for a thermoelastic transmission problem with joint mass, based on a detailed spectral analysis and resolvent operator estimates. We also refer to [29] for the analysis of the large time behaviour of transmission problems of multi-dimensional thermoelasticity.

In this work, we consider similar transmission problems in multi-connected networks. More precisely, we are interested in the large time behaviour of star-shaped networks constituted by coupled thermoelastic and purely elastic rods (see Figure 2).

The aim of this work is to give a complete analysis on the large time behaviour of these systems proving exponential, polynomial and slow decay rates. The optimality of these results is also discussed.

In order to present the problems under consideration more precisely some notations are needed. We denote by $e_j,\; j=1,2,\cdots,n$ the segments occupying the intervals $(0,\ell_j),\; \ell_j\geq 0$, respectively. Denote by $x=0$ the common node of this star-shaped network. Assume that the edges $j=1,2,...,N_1\; (0 <N_1< N)$ in the network are constituted by thermoelastic rods, given by the following equations:

and the other edges in the network are all purely elastic ones given by

Here and in the sequel $u_j(x,t),\; j=1,2,\cdots,N$ denote the displacements of the rods at time $t$, and $\theta_k(x,t),\; k=1,2,\cdots,N_1$ the temperature difference with respect to a fixed reference temperature.

Assume that the exterior nodes of the network are all clamped, and the displacements and temperatures are all continuous at the common node. There is no heat exchange between thermoelastic components and purely elastic ones and the balance of forces at the common node is fulfilled. Thus, the boundary and transmission conditions read as follows:

with initial conditions

Overall the thermoelastic-elastic network system under consideration reads as follows:

Remark 1. The transmission problem in Figure 1 can be considered as a special case of the above network ($N=2,\; N_1=1$).

The natural energy of this system is as follows

and a direct calculation yields the dissipation law

Hence, the energy of system (5) is decreasing. Moreover, from (6), it is easy to see that the dissipation mechanism only acts in the thermoelastic rods. This motivates the problem of whether or not the dissipation is strong enough to make the total energy of the network decay to zero, and with which rate.

In this paper, the large time behaviour of system (5) is mainly discussed based on frequency domain analysis ([5], [6], [13], [19], [25], [26] and [34]). In [37], Shel showed the exponential stability of networks of thermoelastic and elastic materials for some special cases by similar methods. However, it was assumed that there was no heat exchange between the thermoelastic rods connected at the common nodes. In this paper, we allow for the heat exchange between thermoelastic rods at common nodes, which is a natural assumption.

By estimating the resolvent operator along the imaginary axis and employing multiplier techniques, we get a necessary and sufficient condition for system (5) to decay uniformly exponentially, namely that there is no more than one purely elastic rod entering in the network. If this condition fails, the system lacks exponential decay and we further show that the decay rate of the networks can not be faster than $t^{-1}$. Moreover, for a very special case that there are two purely elastic rods involved in the network, the optimal polynomial decay result is obtained and for the general case that there are more purely elastic rods involved, a nearly optimal polynomial decay result is also derived under certain Diophantine approximation conditions, that refer to the lengths of the purely elastic rods involved in the network.

To discuss the sharpness of slow decay rates it is useful to get explicit information on the spectrum of the system, and compare its real and imaginary parts (see [6] and [41]). However, spectra of PDE networks are often difficult to calculate. Thus, we prove the optimality by estimating the norm of the resolvent operator along the imaginary axis (see [1]). But resolvent estimates are hard to be achieved due to the thermoelastic coupling. Thus, we employ diagonalisation argument to deal with the resolvent problem. This allows building explicit approximations of solutions ensuring that the polynomial decay rates we get are nearly optimal.

The rest of the paper is organised as follows. In section 2, the main result of this paper is given. Section 3 is devoted to show the well-posedness and strong asymptotic stability of the system (5). In section 4, we prove the exponential and nearly optimal slow decay rates, under different conditions. Section 5 is devoted to discuss some more general slow decay rates for system (5). Especially, for the special case $N-N_1=2$, the condition to achieve optimal decay of the network is obtained. In section 6, the numerical simulations of the dynamical behaviour of system (5) are presented.

The results in this paper contribute to the understanding of the decay properties of wave and thermoelastic wave networks, a topic in which important issues are still to be understood.

There has been an extensive literature on other closely related issues such as the large time behaviour and controllability properties of elastic networks with node and boundary feedback controls. We refer, among others (the present list of references is by no means complete), to Lagnese et al.[21] for the modelling and control of elastic networks; Ammari et al. [2], [3] and [4] and Nicaise et al. [32] for stabilisation problems on networks of wave and Euler-Bernoulli beams with star-shaped and tree-shaped configurations; Dáger and Zuazua [8], [9] and [10] for boundary controllability of wave networks; Xu et al. [16], [17] and [40] for the stabilisation and spectral properties of the wave networks.

2.   Main results

This section is devoted to state the main result of this paper.

As we will see later, system (5) can be rewritten as an abstract Cauchy problem in an appropriate Hilbert space $\mathcal{H}$:

where $U(t)=( u, u_t, \theta)^T$ and $U(0)=( u^{(0)}, u^{(1)}, \theta^{(0)})^T\in \mathcal{H}$ are given.

The problem of whether the energy of solutions tends to zero as time goes to infinity or not has a simple answer:

Theorem 2.1. Operator $\mathcal{A}$ generates a $C_0$ semigroup of contractions on $\mathcal{H}$. Moreover, the energy of the system (5) decays to zero as $t\to\infty$ if and only if one of the following two conditions is fulfilled,

1). $N-N_1=1$;

2). $N-N_1\geq 2$ and $\ell_i/\ell_j\notin Q,\; i,j=N_1+1, N_1+2, \cdots,N,\; i\neq j$.

We now obtain explicit decay rates for network (5).

It is well known that if all the components in the network are thermoelastic, that is $N=N_1$, the energy of the system decays exponentially to zero.

In fact, in Propositions 1 and 2 in section 4, we obtain the following necessary and sufficient condition for the exponential decay.

Theorem 2.2. The energy of system (5) decays to zero exponentially if and only if $N-N_1\leq 1$, that is, if no more than one purely elastic undamped rod is involved in the network.

Accordingly, when $N-N_1>1$, one can only expect slow decay rate for network (5). In order to address this issue we need the following definition from [36] and [11].

Definition 2.3. ([36], [11]) Real numbers $\ell_1, \ell_2, \cdots, \ell_m$ are said to verify the conditions $(S)$, if $\ell_1,\ell_2,\cdots, \ell_m$ are linearly independent over the field $Q$ of rational numbers; and the ratios $\ell_i/\ell_j$ are algebraic numbers for $i, j=1,2,\cdots,m$.

The notation (S) for this condition was introduced in [11] to refer to the fundamental contribution by Schmidt [36], that defined this class of irrational numbers for the simultaneous approximation by rational ones. It should be noted that the condition (S) denotes a narrow class of irrationals, since the set of algebraic numbers is countable and has Lebesgue measure zero.

By a detailed frequency domain analysis, we have the following explicit polynomial decay rate for system (5).

Theorem 2.4. When $N-N_1>1$, The following estimation always holds.

Thus, we can not expect a decay rate which is beyond first order polynomial. Furthermore, if $\ell_{N_1+1},\; \ell_{N_1+2},\; \cdots, \ell_{N}\; (N-N_1>1)$ satisfy the conditions $(S)$, then for any $\epsilon>0$, there always exists a constant $C_\epsilon>0$ such that the energy of network (5) satisfies

for all $(u^{(0)}, u^{(1)},\theta^{(0)})\in\mathcal{D}(\mathcal{A})$. Thus, this decay rate is nearly sharp in the sense of (8).

Remark 2. Using the method of proof of Theorem 2.4, we can obtain more general slow decay rates (polynomial, logarithmic or arbitrarily slow decay), which will be presented in section 5. Especially, for a very special case that there are two purely elastic rods involved in the network ($N-N_1=2$), we show that the network can achieve the optimal decay rate $t^{-1}$ if the mutual ratio of the lengths of the purely elastic rods belongs to the set of irrational numbers having a continuous fraction expansion $[a_0, a_1, ..., a_n, ...]$ with bounded $(a_n)$.

Remark 3. The optimality result in (8) is well-known for wave-like equations with velocity damping in the case of one single string with damping on an internal point, which is equivalent to a simple star-like network constituted only by two strings ([20], [11]).

Here we show the same lower bound on the decay rate for the more general system involving thermoelastic rods. However, for general cases that more than two purely elastic rods entering in the networks, it is still an open problem to find the condition to guarantee achieving a sharp polynomial decay rate.

3.   Well-posedness and strong stability

This section is devoted to show the well-posedness of network (5) by the semigroup theory and prove Theorem 2.1, with a necessary and sufficient condition for strong stability of this system.

Let us first introduce an appropriate Hilbert space setting for the well-posedness of the system.

For $n \ge 1$, define

Set the state space $\mathcal{H}$ as follows:

equipped with inner product:

for $W=(u, w, \theta), \widetilde{W}=(\widetilde{u}, \widetilde{w}, \widetilde{\theta})\in \mathcal{H}$.

$(\mathcal{H}, \|\cdot\|_{\mathcal{H}})$ is a Hilbert space.

Then, define the system operator $\mathcal{A}$ in $\mathcal{H}$ as follows:

where $\alpha={\rm{diag}}(\alpha_1,\alpha_2,\cdots,\alpha_{N_1}),\; \beta={\rm{diag}}(\beta_1,\beta_2,\cdots,\beta_{N_1})$, $I_{N\times N_1}= [I_{N_1}, 0]^T$ and $I_{N_1}$ is the $N_1-$unit matrix with domain

Thus, system (5) can be rewritten as the evolution equation (7) in $\mathcal{H}$.

It is easy to check that $\mathcal{A}$ is dissipative in $\mathcal{H}$. Moreover, $\mathcal{A}$ is injective and surjective and hence $0\in\rho(\mathcal{A})$. Then, by Lummer-Phillips theorem (see [33]), $\mathcal{A}$ generates a $C_0$ semigroup of contractions $S(t)$ on $\mathcal{H}$.

Now, we focus on proving the strong stability property of the system in Theorem 2.1. The proof by contradiction is mainly used here.

Proof of Theorem 2.1.

Sufficiency. If the strong stability of system (5) does not hold, then by the Lyubich-Phóng strong stability theorem (see [27]), there exists at least one $\widetilde{\lambda}=i\widetilde{\sigma}\in\sigma(\mathcal{A}),\, \widetilde{\sigma}\in\mathbb{R},\widetilde{\sigma}\neq 0$ on the imaginary axis. Assume that

is an eigenvector of $\mathcal{A}$ corresponding to such $\widetilde{\lambda}$. We get

which yields $\theta_k= \theta_{k,x}=0,\; k=1,2,\cdots,N_1.$ Then by the boundary and transmission conditions in (5), $u_{j}(x),\; j=1,2,\cdots,N$ satisfy the following equations:

If $N-N_1=1$, it is easy to get $( u, \widetilde{\lambda} u,\theta)=0$, which contradicts the fact that $(u, \widetilde{\lambda} u,\theta)=0$ is an eigenvector.

If $N-N_1\geq 2$, we get by direct calculation,

which satisfy

Since $( u,\widetilde{\lambda} u, \theta)$ is the eigenvector corresponding to $\widetilde{\lambda}$, then there are at least $c_{j_1},\; c_{j_2}$ for some $j_1,\; j_2\geq N_1+1$ such that

Hence, $\sinh\widetilde{\lambda}\ell_{j_1}=\sinh\widetilde{\lambda}\ell_{j_2}=0.$ Thus, $\ell_{j_1}/\ell_{j_2}\in Q$, which is in contradiction with condition (2) in Theorem 2.1.

Necessity. If there exist $i_0,\; j_0$ satisfying $N_1+1\leq i_0, j_0\leq N,\; \ell_{i_0}/{\ell_{j_0}}=p/q,$ with $p, q$ nonzero integers, we get easily that $\left( (\widehat{u}_j(x))_{j=1}^N, \widetilde{\lambda}(\widehat{u}_j(x))_{j=1}^N,( 0 )_{k=1}^{N_1} \right)$ is an eigenvector corresponding to $\widetilde{\lambda}= iq\pi/\ell_{j_0}= ip\pi/\ell_{i_0},$ in which

This contradicts the strong stability property of system (5). Therefore, $\ell_i/\ell_j\notin Q,\; i,j=N_1+1, N_1+2,\cdots,N,\; i\neq j$. The proof is complete.

Remark 4. For the proof of "Sufficiency", we also can use the unique continuation property for wave networks in Dager and Zuazua [11] (See Corollary 5.28, p.135). Indeed, (10), in the absence of thermal components, corresponds to the eigenproblem associated with the pure wave system and, according to the results in [11], its unique solution is the trivial one, which contradicts that $\left( (u_j)_{j=1}^N,\widetilde{\lambda}(u_j)_{j=1}^N, (\theta_k)_{k=1}^{N_1}\right)^T$ is an eigenvector. Then the desired result follows.

4.   Decay rates

This section is devoted to achieve explicit decay rates of the total energy of solutions of system (5). The exponential decay and slow decay rates are deduced under different assumptions of the various components of the network.

4.1.   Exponential decay rate: Case $N-N_1=1$

In this subsection, we analyse the decay rate of network (5) when $N-N_1=1$, namely, there is only one purely elastic rod involved in the network. To do this, let us introduce the following lemma as described in [13], [19], [25] and [34].

Lemma 4.1. Let $(S(t))_{t\geq 0}$ be a $C_0$ semigroup on a Hilbert space $\mathcal{H}$ generated by $\mathcal{A}$. Then the semigroup is exponentially stable if and only if

and

We are then in conditions to prove the following proposition, which is one of the main statements in Theorem 2.1:

Proposition 1. When $N-N_1=1$, the energy of system (5) decays exponentially to zero.

Proof. It is sufficient to show that the conditions in Lemma 4.1 are fulfilled. It should be noted that although the idea of this proof is similar to the one in [37], some different multipliers are employed to get certain estimates so as to deal with the transmission conditions in the present paper.

By the argument of the proof of Theorem 2.1, it is easy to see that, in the present case, there is no eigenvalue of $\mathcal{A}$ on the imaginary axis, that is, the condition (11) in Lemma 4.1 holds. Now we proceed to prove condition (12) and to deduce the exponential decay rate.

If condition (12) is not fulfilled, then there exists a sequence of real numbers $\sigma_n$ such that the corresponding resolvents $T_n=(i\sigma_n I-\mathcal{A})^{-1}$ satisfy $\|T_n\|_{\mathcal{H}}\to\infty, n\to\infty.$ By Banach-Steinhaus theorem, there exists $F\in\mathcal{H}$ such that

Thus,

So there exists a sequence $\Phi^n=(U^n, V^n, \Theta^n)\in\mathcal{D}(\mathcal{A})$, with $\|\Phi^n\|_\mathcal{H}=1$, where $U^n=(u_j^n)_{j=1}^N,\; V^n=(v_j^n)_{j=1}^N,\; \Theta^n=(\theta_j^n)_{j=1}^{N-1}$, and a sequence $\sigma_n\in\mathbb{R}$ with $\sigma_n\to \infty$ such that

namely,

Note that $((i\sigma_n-\mathcal{A})\Phi^n, \Phi^n)_{\mathcal{H}}=-\sum_{j=1}^{N-1}\limits\frac{\alpha_j}{\beta_j} \int_0^{\ell_j}|\theta^n_{j,x}|^2dx \to 0.$ Thus,

and hence by Poincaré inequality, we get $\theta^n_{j}\to 0,$ in $L^2(0, \ell_j),\; j=1,2,\cdots,N-1.$ Moreover, by the Gagliardo-Nirenberg inequality (see [25]), we get $\|\theta^n_{j}\|_{L^\infty}\leq d_1\|\theta^n_{j,x}\|^{\frac{1}{2}} \|\theta^n_{j}\|^{\frac{1}{2}}+d_2{\|\theta^n_{j}\|}\to 0,$ which implies that

Removing $\theta_{j,x}^n$ in (14) and substituting (13) into (14), we get

and taking the inner product of (19) with $xu^n_{j,x}$ in $L^2(0, \ell_j)$, we have

Note that

Hence, $u^n_{j,x}(\ell_j),\; j=1,2,\cdots,N-1$ are bounded. Similarly, taking the inner product of (19) with $(x-\ell_j) u^n_{j,x}$ in $L^2(0, \ell_j)$ yields

Hence,

Thus, $u^n_{j,x}(0)$ and $\sigma_n u^n_{j}(0)$ are bounded.

Dividing (15) by $i\sigma_n$, together with (13), we get $-\theta^n_{j,xx}/(i\sigma_n)+\beta_j u^n_{j,x}\to 0,\; j=1,2,\cdots, N-1.$ Hence, $\theta^n_{j,xx}/i\sigma_n$ is bounded in $L^2(0,\ell_j)$. Then taking the $L^2-$product of the above with $u^n_{j,x}$ yields

Integrating the above by parts, we have

Note that dividing (14) by $i\sigma_n$, we obtain the boundedness of $u^n_{j,xx}/(i\sigma_n)$ in $L^2(0,\ell_j)$. Thus,

By the Gagliardo-Nirenberg inequality,

we have ${\theta^n_{j,x}(\ell_j)}/(\sqrt{i\sigma_n}),\;{\theta^n_{j,x}(0)}/{(\sqrt{i\sigma_n})} \to 0,\; j=1,2,\cdots,N-1$. By the boundedness of $u^n_{j,x}(\ell_j)$ and $u^n_{j,x}(0)$, together with (22), we have

Thus, $u_j^n\to 0,\; in \; H^1(0,\ell_j), j=1,2,\cdots,N-1.$ Dividing (14) by $i\sigma_n$ and taking the product of the obtained identity with $v^n_j$ from $0$ to $\ell_j$ yields

and hence $(v^n_j,v^n_j)-(u^n_{j,xx}/i\sigma_n,i\sigma u_j^n)\to 0, j=1,2,\cdots,N-1.$

Integrating by parts, we have

Note that $u_j^n(0)\to 0, \; j=1,2,\cdots,N-1,$ due to $u^n_j,\; u^n_{j,x}\to 0,\; in\; L^2(0, \ell_j),\; j=1,2,\cdots,N-1$ and Gagliardo-Nirenberg inequality. Hence, due to the boundedness of $u_{j,x}^n(0)$,

Then from (20), we have

On the other hand, on the segment $(0, \ell_N)$, $u^n_N(0),\; \sigma_n u^n_{N}(0)\to 0$ due to (18), (27) and the transmission condition at $x=0$.

Taking the inner product of (16) with $(x-\ell_N)u^n_{N,x}$, we have

Note that

Thus,

Hence,

Thus, by (17), (24) and (26), we get $\Phi^n\to 0,\; in\; \mathcal{H},$ which is in contradiction with $\|\Phi^n\|=1$. The desired result follows.

4.2.   Lack of exponential decay: Case $N-N_1>1$

In this subsection, we shall show that if more than one purely elastic undamped rod is involved in the network, the exponential decay rate does not hold.

Proposition 2. If $N-N_1>1$ the network (5) lacks the property of exponential decay.

Proof. Note that from Lemma 4.1, it is sufficient to show that the norm of the resolvent operator of system (5) along the imaginary axis is necessarily unbounded when $N-N_1>1$.

To do it we consider the resolvent problem

where $U=((u_j)_{j=1}^N, (v_j)_{j=1}^N, (\theta_j)_{j=1}^{N_1}),\; F=((f_j)_{j=1}^N, (g_j)_{j=1}^N, (\eta_j)_{j=1}^{N_1}),$ which is equivalent to

Choose $f_j=0,\; j=1,2,\cdots,N,$ and $g_j=\eta_j=0,\; j=1,2\cdots,N_1$. Then we have

Using explicit representation formulas we get

In order to calculate $u_j,\; \theta_j,\; j=1,2,\cdots,N_1$, let us consider the following coupled system.

that is,

We rewrite (35) in the vector form

where $Y_j(x)=(u_j,\frac{u_{j,x}}{\lambda}, \theta_j, \frac{\theta_{j,x}}{\lambda})^T, A_j= \left[\begin{array}{cccc} 0&\lambda&0&0\\ \lambda&0&0&\alpha_j\\ 0&0&0&\lambda\\ 0&\beta_j\lambda&1&0 \end{array} \right].$

Set $d_{j,1}=\frac{\lambda[\lambda+\alpha_j\beta_j+1+\sqrt{(\lambda+\alpha_j\beta_j+1)^2-4\lambda}]}{2}, d_{j,2}=\frac{\lambda[\lambda+\alpha_j\beta_j+1-\sqrt{(\lambda+\alpha_j\beta_j+1)^2-4\lambda}]}{2}.$ In order to diagonalize the matrix $A_j$, we employ the transformation $Y_j:=P_jZ_j, j=1,2,\cdots,N_1,$ where

and

We then have $\frac{d Z_j(x)}{d x}=P_j^{-1}A_jP_j Z_j=\left[\begin{array}{cccc}\sqrt{d_{j,1}}&0&0&0\\ 0&-\sqrt{d_{j,1}}&0&0\\ 0&0&\sqrt{d_{j,2}}&0\\ 0&0&0&-\sqrt{d_{j,2}}\end{array}\right]Z_j.$

In this way we obtain the following system

and

By the boundary and transmission conditions in (5), together with (34), we get the following estimate (the technical details are given as in Appendix):

Choosing $\sigma_n\ell_{N_1+1}=n\pi,\; n\to+\infty$, then $\sin\sigma_n\ell_{N_1+1}=0$. Since the irrational numbers always can be approximated by rational ones, we can find a subsequence $\sigma_{n_k}$, such that

In fact, note that there is always a sequence of rational numbers $\frac{p_m^n}{q_m^n},\; p_m^n, q_m^n \in \mathbb{Z}^+,\; n=1,2,\cdots$ such that $|\frac{\ell_m}{\ell_{N_1+1}}-\frac{p_m^n}{q_m^n}|\to0,\; m=N_1+2,N_1+3, \cdots,N, n\to\infty.$ Thus choose $n_k= \prod_{m=N_1+2}^{N}\limits q_m^k$. Then we have

Set $g_{N_1+1}^{n_k}(s):=\sin\sigma_{n_k}(\ell_{N_1+1}-s), g_j^{n_k}(s)=0, j=N_1+2,N_1+3,\cdots,N$. Then we have

Thus, from (37), we get

Hence, by (34), we have

due to the fact that $\sin\sigma_{n_k}\ell_j\to 0, j=N_1+2,N_1+3,\cdots,N.$ Note that $v_j^{n_k}=i\sigma_{n_k}u^{n_k}_{j}(x)$. Therefore,

Summarising the developments above we find a sequence $(\sigma_n, F^n)$, where $F^n\in \mathcal{H}$ and $\|F^n\|_{\mathcal{H}}$ is bounded, satisfying

which implies the lack of exponential decay rate of system (5). The proof is complete.

4.3.   Lower bounds on the polynomial decay rate

This subsection is devoted to get the lower bounds on the polynomial decay rate as stated in Theorem 2.4.

We need the following result from [6] (see also [26]).

Lemma 4.2. A $C_0$ semigroup $e^{t\mathcal{A}}$ of contractions on a Hilbert space satisfies

for some constant $C>0$, if and only if the following conditions hold:

$1).\;\; \{i\beta| \beta\in\mathbb{R}\}\subset\rho(\mathcal{A})$;

$2).\;\; \limsup_{|\beta|\to\infty}\limits\frac{1}{\beta^{\ell}}\|(i\beta-\mathcal{A})^{-1}\|<\infty.$

Proposition 3. The decay rate of the energy of system (5) can be, at most, polynomial of order $t^{-1}$.

Proof. From Lemma 4.2, it is sufficient to show that there exists at least one sequence $(\sigma_n, F^n)$ such that $\sigma_n\to+\infty,\; n\to\infty$ and

where $F^n\in \mathcal{H}$ and $\|F^n\|_{\mathcal{H}}$ is bounded, $\widetilde{C}$ being some positive constant.

For simplicity, we consider the case $N-N_1=2$, that is, there are two purely elastic rods involved in the network. Based on the proof of Proposition 2, we choose the sequence $(\sigma_{n_k},F_{n_k})$ satisfying (38) in the following three steps.

Step 1). Choose $\sigma_n=\frac{1}{\ell_{N_1+1}+\ell_{N_1+2}} n\pi$. Thus we get $\sin\sigma_n(\ell_{N_1+1}+\ell_{N_1+2})=0$.

Since

we have

Step 2). Choose $f_j=0,\; j=1,2,\cdots,N,$ $g_j=\eta_j=0,\; j=1,2\cdots,N_1$ and $g_{N_1+1}^{n}(s)=\sin\sigma_{n}(\ell_{N_1+1}-s), g_{N_1+2}^n (s)=0$ in (32).

Substituting the above into (37), we get

Note that

and

Hence,

Step 3). Note that we have chosen in Step 1) that $\sigma_n=\frac{1}{\ell_{N_1+1}+\ell_{N_1+2}} n\pi$ and thus

By Dirichlet theorem, we can always find an infinite subsequence $n_k\in \mathbb{Z}$ such that

and hence $|\sin\sigma_{n_k}\ell_{N_1+2}|=|\sin (\frac{\ell_{N_1+2}}{\ell_{N_1+1}+\ell_{N_1+2}}n_k\pi)|< \frac{\pi}{n_k}.$

Thus, there exists a positive constant $C$

Note that from (33) and (34), we get $v_{N_1+2}^{n_k}(x)=i\sigma_{n_k}u^{n_k}_{N_1+2}(x)$ and

Hence, by (41), there exists a positive constant $\widehat{C}$ satisfying

Summarizing above, we have found a sequence $(\sigma_{n_k}, F_{n_k})$, where

in which $f_j=0,\; j=1,2,\cdots,N,$ $g_j=\eta_j=0,\; j=1,2\cdots,N_1$ and $g_{N_1+1}^{n}(s)=\sin\sigma_{n}(\ell_{N_1+1}-s), g_{N_1+2}^n (s)=0$, such that

where $\widetilde{C}>0$ is some constant. The proof is complete.

Remark 5. For general case $N-N_1>2$, We can prove that the networks also satisfy (38) by modifying the above construction slightly. Indeed, choose $g_{N_1+1}^{n}(s)=\sin\sigma_{n}(\ell_{N_1+1}-s), g_{j}^n (s)=0,\; N \geq j>N_1+1$ in step 2), and choose $n_k\in \mathbb{Z}$ such that $|||\frac{\ell_{N_1+2}}{\ell_{N_1+1}+\ell_{N_1+2}}n_k|||< \frac{1}{n_k},$ and $\sin\sigma_{n_k}\ell_j \nrightarrow 0,\; N\geq j>N_1+2,\; k\to\infty$ in step 3). Thus, by the same discussion as above, the desired result follows.

4.4.   Explicit polynomial decay rate: Case $N-N_1>1$ (Proof of Theorem 2.4)

In subsection 4.2 we proved that if there are more than one purely elastic rods involved in the network (5), the system can not achieve the exponential decay. We have also shown that the decay rate can be, at most, polynomial of order $t^{-1}$.

The mutual-irrationality of the radii of $\ell_j,\; j=N_1+1,N_1+2,\cdots,N$ in Theorem 2.1 is a sufficient and necessary condition for strong stability. So, for the rest of this section, we always assume this condition to be fulfilled.

Now, we prove effective and explicit polynomial decay results when $N-N_1>1$, and $\ell_j,\; j=N_1+1,N_1+2,\cdots,N$ satisfy the conditions (S) in Definition 2.3.

Proof of Theorem 2.4. Similarly to the proof of Theorem 2.2, we argue by contradiction, on the basis of Lemma 4.2.

Let $\Phi_n$ be a sequence such that $\Phi^n=(U^n, V^n, \Theta^n)\in\mathcal{D}(\mathcal{A})$, with $\|\Phi^n\|_\mathcal{H}=1$, where $U^n=(u_j^n)_{j=1}^N,\; V^n=(v_j^n)_{j=1}^N,\; \Theta^n=(\theta_j^n)_{j=1}^{N_1}$, and a sequence $\sigma_n\in\mathbb{R}$ with $\sigma_n\to \infty$ such that

where $\epsilon$ is given as in Theorem 2.4. Hence,

and

Note that $(\sigma_n^{2(1\!+\!\epsilon)}(i\sigma_n\!-\!\mathcal{A})\Phi^n, \Phi^n)_{\mathcal{H}}\!=\!-\sum_{j=1}^{N_1}\alpha_j\beta_j \int_0^{\ell_j}|\sigma_n^{(1\!+\!\epsilon)}\theta^n_{j,x}|^2dx\!\to\! 0.$

Hence,

Thus, by Poincaré inequality,

Similar to the proof of Theorem 2.2, we can get that

Thus, by the transmission conditions at the common node, we get

Then we will prove that $\Phi^n\to 0$ in $\mathcal{H}$, which leads to a contradiction. To do this, we discuss it by the following two cases:

Case 1). Assume that $\sin \sigma_n\ell_j\neq0,\; j=N_1+1, N_1+2,\cdots,N$.

By (42), (45), we can easily get that $u_{j,xx}^n+\sigma_n^2 u_j=-\frac{g_j^n+i\sigma_nf_j^n}{ \sigma_n^{2(1+\epsilon)}}.$

Thus a direct calculation yields

Hence,

It is easy to show that

Set

When $\ell_j,\; j= N_1+1,N_1+2,\cdots,N$ satisfy the conditions $(S)$ as given in Definition 2.3, by Corollary A.10 in [11], we get

For any given sequence $\sigma_n$, assume that there exists $j_0^n$($N_1+1 \leq j^{n}_0\leq N$) such that

Hence, for any $j\neq j^n_0,\; N_1+1\leq j\leq N$, we have

which implies that

Therefore, by (49) and (52) together with (51), we get that

Then by the transmission condition (50), we obtain that

Taking the inner product of (45) with $(x-\ell_j)u^n_{j,x},\; j=N_1+1,\; N_1+2,\cdots,N$, respectively,

We have

Hence,

Therefore,

Thus, by (47) and (59), we have obtained

which contradicts $\|\Phi^n\|_\mathcal{H}=1$.

Now let us continue to consider the second step:

Case 2). Assume that there exists $j_0,\; N_1+1\leq j_{0}\leq N$ such that $\sin \sigma_n\ell_{j_0}=0$.

Note that there exists at most one $j_0^n,\; N_1+1\leq j_0\leq N$ satisfying $\sin \sigma_n\ell_{j_0}=0$ due to the irrationality of the mutual ratii between $\ell_j,\; N_1+1\leq j\leq N$. In this case,

where $\gamma_n$ is given as in (53). Thus, by the same arguments as in $Step\; 1)$, we can get

which together with the transmission condition (50), implies

Then by (57)-(59) in $Step \; 1)$, we get

Hence, the same contradiction holds as in $Step \; 1)$.

Therefore, by Lemma 4.2, we get the polynomial decay rate of system (5), that is

Note that in Proposition 3, we have proved that the polynomial decay order of the energy of system (5) is at most $t^{-1}$. Hence, $t^{-\frac{1}{1+\epsilon}}$ is the nearly sharp. The proof of Theorem 2.4 is complete.

5.   More general slow decay rates

In the last section, we have shown that, when $N-N_1>1$, namely, when the network (5) involves more than one purely elastic rod, the system can not achieve the exponential decay rate. Then we further derived the nearly optimal polynomial decay rate, if the conditions $(S)$ in Definition 2.3 are fulfilled.

In fact, the discussion of the proof of Theorem 2.4 (see subsection 4.3), shows that the slow decay rate of the system is determined by $\gamma_n$ given as (53), which depends on the property of the lengths of the purely elastic rods entering in the network. Hence, other more general slow decay rates can be obtained when different conditions on $\ell_j,\; j=N_1+1,N_1+2,\cdots,N$ are imposed.

To do this, let us introduce the following definition on the irrational sets (see p. 209 in [11]), which is deduced from [7] (see Theorem Ⅰ, p. 120).

Definition 5.1. (Theorem [7], [11]) 1. Set $B_\epsilon$: for all $\epsilon>0$ there exists a set $B_\epsilon\subset\mathbb{R}$, such that the Lebesgue measure of $\mathbb{R}\setminus B_\epsilon$ vanishes, and a constant $C_\epsilon>0$ for which, if $\xi\in B_\epsilon$, then $|||\xi m|||\geq \frac{C_\epsilon}{m^{1+\epsilon}}$ for all integer number $m$. All the algebraic irrational numbers belong to $B_\epsilon$.

2. Set $\mathcal{F}$: the set of all real numbers $\rho$ such that $\rho\notin Q$ and so that its expansion as a continued fraction $[0, a_1, a_2,\cdots, a_n, \cdots]$ is such that $(a_n)$ is bounded. In particular $\mathcal{F}$ is contained in the sets $B_\epsilon$ for every $\epsilon>0$. (see p. 209 in [11] for more details). It contains all quadratic algebraic irrational numbers and also some transcendental numbers. This set $\mathcal{F}$ has Lebesgue measure zero and is not denumerable.

We have the following result:

Corollary 1. For any $(u^{(0)}, u^{(1)},\theta^{(0)})\in \mathcal{D}(\mathcal{A})$, there always exists a constant $C>0$ such that the energy of network (5) satisfies

where $\widetilde{s}$ is given as follows:

$\bullet$ if $\frac{\ell_i}{\ell_j}\in B_\epsilon,\; i, j=N_1\!+\!1, N_1\!+\!2,\cdots,N, i\neq j$, then $\widetilde{s}\!=\!{N-N_1-1+\epsilon}$;

$\bullet$ if $\frac{\ell_i}{\ell_j}\in \mathcal{F},\; i, j=N_1+1, N_1+2,\cdots,N,\; i\neq j$, then $\widetilde{s}=N-N_1-1$.

Proof. Since the proof is similar to the one of Theorem 2.4, we only give a sketch of it.

If the decay rate is not fulfilled, there exists a sequence $\Phi^n=(U^n, V^n, \Theta^n)\in\mathcal{D}(\mathcal{A})$, with $\|\Phi^n\|_\mathcal{H}=1$, where $U^n=(u_j^n)_{j=1}^N,\;V^n=(v_j^n)_{j=1}^N,\; \Theta^n=(\theta_j^n)_{j=1}^{N_1}$, and a sequence $\sigma_n\in\mathbb{R}$ with $\sigma_n\to \infty$ such that

where $\widetilde{s}$ is given as in Corollary 1.

By Diophantine approximation (see [11]), different estimates can be gotten for $\gamma_n$, when the irrational numbers $\ell_i/\ell_j$ belong to the sets $B_\varepsilon$ and $\mathcal{F}$ (as in as in Definition (5.1), respectively. By Corollary A. 10 in [11] (see also [39]), we get that

(1) if $\frac{\ell_i}{\ell_j}\in B_\epsilon,\; i, j=N_1+1, N_1+2,\cdots,N,\; i\neq j$, then $\gamma_n\geq c_\epsilon/\sigma_n^{N-N_1-1+\epsilon},\; n\geq 1,\; \epsilon>0$;

(2) if $\frac{\ell_i}{\ell_j}\in \mathcal{F},\; i, j=N_1+1, N_1+2,\cdots,N,\; i\neq j$, then $\gamma_n\geq c/\sigma_n^{N-N_1-1},\; n\geq 1$.

Thus, proceeding as in the discussion of the proof of Theorem 2.4, finally we can get

This contradicts $\|\Phi^n\|_\mathcal{H}=1$. Hence, the desired result follows.

Remark 6. By Corollary 1, it is easy to see that when $N-N_1=2$, that is there are two purely elastic rods in the network, the system can achieve optimal polynomial decay rate $t^{-1}$, if $\frac{\ell_{N_1+1}}{\ell_N}\in\mathcal{F}$.

More generally, by the similar proof as the one for Theorem 2.4, together with the so called $M_{\log}-$Theorem in [5], we obtain the general slow decay rate of system (5) as follows.

Corollary 2. Set $M_{\log}(s)=M(s)(\log(1+M(s))+\log(1+s)),$ where $M(s): \mathbb{R}^+\rightarrow (0,\infty)$ is continuous and increasing.

If

where

then

where $M_{log}^{-1}(\cdot)$ is the inverse function of $M_{log}$.

Especially, if $M(s)\leq ce^{as},\; a, c>0$, then the network (5) achieves logarithmic decay rate.

Proof. We can still use the proof of Theorem 2.4 to derive

which together with $M_{\log}-$Theorem in Batty and Duyckaerts [5] yields (61).

Remark 7. From Corollary 2, we see that in order to obtain an explicit decay rate, it is very important to estimate the lower bound of $\gamma_n$. Some techniques such as Diophantine approximations can be used to estimate it. However, it is still open to get a sharp estimate for it.

6.   Numerical simulations

This section is devoted to present some numerical simulations on the dynamical behaviour of system (5) to support the results obtained above.

The backward Euler method in time (time step: $dt=0.01$) and the Chebyshev spectral method in space (spatial grid size $S=40$) were employed, in a MatLab environment (see [38]).

For simplicity, we assume that the star-shaped network consists of three edges, the lengths of which are given as

The following cases are considered:

Case A. $N_1=3$ (Three thermoelastic rods)

In this case, all the three edges of the network are constituted by thermoelastic ones. First, choose the initial conditions as follows:

and the parameters in system (5):

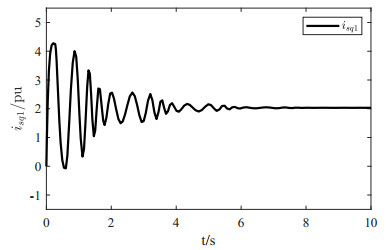
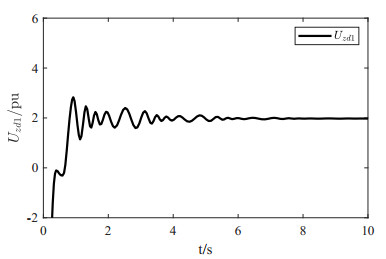
The dynamical behaviour of $u_j,\; \theta_j,\; j=1,2,3$ in the time interval $[0,100]$ is given as in Figure A-1, 2, 3, 4, 5, 6. We observe the exponential decay rate of system (5).

Case B. $N_1=0$ (Three purely elastic rods)

In this case, the network consists of purely elastic rods, that is, no thermal-damping dissipates energy. The initial conditions are the same as in Case A. Solutions are plotted in Figure B-1, 2, 3, which confirms the conservative character of the system.

Case C. $N_1=2$ (Two thermoelastic rods and one purely elastic one)

In this case, the network is constituted by two thermoelastic rods and one purely elastic one. The initial conditions and parameters in system (5) are chosen as (62) and (63). We get the dynamical behaviour in Figure C-1, 2, 3, 4, 5.

In these figures, we can see that the behaviour of each $u_j,\; j=1,2,3$ and $\theta_j,\; j=1,2$ are convergent to zero very fast. This shows numerically that the energy of system (5) decays to zero exponentially, which is consistent with the result on exponential decay rate in previous sections.

Case D. $N_1=1$ (One thermoelastic rod and two purely elastic rods)

In this case, the network consists of one thermoelastic rod and two purely elastic ones. The lengths of rods are still given as $\ell_1=1,\; \ell_2=2,\; \ell_3=1$. Simulations are plotted in Figure D-1, 2, 3, 4. We observe the lack of decay as predicted by the theory.

Case E. $N_1=1,\; \ell_2=\sqrt{2}$ (One thermoelastic rod and two purely elastic ones)

In this case, the network is still constituted by one thermoelastic rod and two purely elastic ones. This time the length $\ell_2=\sqrt{2}$, which leads to $\frac{\ell_2}{\ell_3}=\sqrt{2}\notin Q$.

In Figure E-1, 2, 3, 4, we observe a very slow decay rate. It implies that the energy of this system decays to zero but lacks exponential growth rate.

Moreover, we presented Figure F-1, 2 to compare the decay rate of the energy for each case. Figure F-1 shows the dynamical behaviours of the logarithmic scale of the energy for Case A, B and C. Figure F-2 shows the dynamical behaviours of the energies for Case D and E. From these two figures, we can clearly see the behaviours of energies for each case respect time, which are consistent with our theoretical results obtained in this paper.

Appendix A.   Proof of (37)

This appendix is devoted to show how to get (37).

Note that $\theta_j(0)=\theta_k(0), u_j(0)=u_k(0)$ due to the continuity at the common node in system (5).

Thus, by (36), for $j=1,2,\cdots,N_1$,

Thus, by the boundary condition $u_j(\ell_j)=\theta_j(\ell_j)=0,\;j=1,2,\cdots,N_1$, we get

Then a direct calculation yields, for $j=1,2,\cdots,N_1$,

where

and

Then by the transmission condition $\sum_{j=1}^{N_1}\limits\frac{\alpha_j}{\beta_j}\theta_{j,x}(0)=0$, we get

which implies that

Hence by (A.1),

On the other hand, by (34),

Then by the transmission condition $\sum_{j=1}^N\limits u_{j,x}(0)=\sum_{j=1}^{N_1}\limits\alpha_j\theta_j(0)$, together with (A.3) and (A.4), we have

Hence,

As $\sigma\to\infty$, we have the following estimation:

Hence,

and

Substituting the above into (A.1), we get that

Then by (A.6),

Thus, (37) has been obtained.