In this paper, the reproducing kernel Hilbert space method had been extended to model a numerical solution with two-point temporal boundary conditions for the fractional derivative in the Caputo sense, convergent analysis and error bounds are discussed to verify the theoretical results. Numerical examples are given to illustrate the accuracy and efficiency of the presented approach.
1.
Introduction
In recent years, the study of fractional derivatives has been an important topic. It has been used to model many phenomena in numerous fields such as science and engineering. There are many interpretations for fractional derivatives, such as the definition of Caputo [1], the definition of Riemann-Liouville [2], the definition of Grunwald-Letnikov [3], and most recently, Conformable [4], Atangana-Baleanu [5], Wallström [6], Jumarie [7], Klimek [8] and others.
In practice, where quantitative results are needed for given real-world problems, numerically approximate solutions can often be demonstrably better, more reliable, more detailed, efficient and cost-effective than analytical ones for certain fractional structures. A number of studies [9,10,11,12,13,14] were therefore involved in developing approaches for providing estimated solutions. One of these approaches is the Hilbert space kernel reproduction (RKHS) method used for the first time by S. Zaremba for the harmonic and biharmonic functions at the beginning of the 20th century to find solutions for boundary value problems (BVPs).
The RKHS precede the Dirac delta function in many ways, among which we mention providing an important structure for random distribution of multi-round data and, providing accurate approximation of multi-dimensional general functions and the possibility to pick any point in the integration interval.
The RKHS algorithm has been successfully applied to various fields of numerical analysis, computational mathematics, probability and statistics [15,16], biology [17], quantum mechanics and wave mechanics [18]. Therefore wide range of research works have been directed to its applications in various stochastic categories [19], and defined problems involving operator equations [20], partial differential equations [21,22], integrative equations [23,24], and differential integration equations [24,25,26,27,28,29]. In addition, many studies have focused in recent years on the use of the RKHS method as a framework for seeking approximate numerical solutions to different problems [30,31,32,33,34,35,36,37,38,39].
Moreover, the numerical solution of the different groups of BVP can be found in [40,41,42]. The two-point BVPs has a strong interest in applied mathematics, this kind of problems arise directly from mathematical models or by turning partial differential equations into ordinary differential equations. As this type of problems does not have an exact solution, many special techniques have been used to solve it, including the shooting method [43,44], the collocation method [45,46], the finite difference method [47,48], and the quasilinearization method [49,50]. The continuous genetic algorithm approach was used to solve these schemes in [51,52,53].
The present paper is structured as follows: in Section 2, we set out some basic concepts and results from fractional calculus theory. In Section 3, the iterative form of the reproducing kernel algorithm is used to build and measure the solution of the fractional differential method with temporal two points. In Section 4 and 5, the convergence and error estimator are discussed to provide a number of numerical results to demonstrate the efficiency and accuracy of the reproducing kernel Hilbert space method. At last in section 6, a conclusion of the results is made.
2.
Preliminaries
In applied mathematics and mathematical analysis, there are several definitions of fractional derivatives, Riemann-Liouville and Caputo are the most popular of all [54]. In this section, we list some of these definitions in addition to reproducing kernel spaces on finite domain [t0,tf].
Definition 2.1. [55] Let n∈R+. The operator Jnt0 defined on L1[t0,tf] by
for t0≤x≤tf, is called the Riemann-Liouville fractional integral operator of order n. For n=0, we set J0t0:=I, the identity operator.
Definition 2.2. [55] Let n∈R+ and m=[n]. The operator Dnt0 defined by
is called the Riemann-Liouville fractional differential operator of order n. For n=0, we set D0t0:=I, the identity operator.
Definition 2.3. [55] Let α∈R+ and n−1<α<n. The operator Dα∗t0 defined by
for t0≤x≤tf, is called the Caputo differential operator of order α.
Definition 2.4. [35] Let M be nonempty set, the function K:M×M⟶C is a reproducing kernel of the Hilbert space H if the following conditions are met:
(1) K(.,t)∈M,∀t∈M,
(2) the reproducing property: ∀t∈M,∀z∈H:⟨z(.),K(.,t)⟩=z(t).
The second condition means that the value of z at the point t is reproduced by the inner product of z with K.
Note: The reproducing kernel is unique, symmetric and positive definite.
Definition 2.5. L2[t0,tf]={ϑ|∫tft0ϑ2(t)dt<∞}.
Definition 2.6. The space W12[t0,tf] is defined as:
The inner product and its norm are given by:
Definition 2.7. The space W22[t0,tf] is defined by:
W22[t0,tf]={ϑ|ϑ,ϑ′areabsolutelycontinuousrealvaluefunctions,ϑ″∈L2[t0,tf],ϑ(t0)=0}.
The inner product and its norm are given by:
Definition 2.8. W32[t0,tf]={ϑ|ϑ,ϑ′,ϑ″areabsolutelycontinuous,ϑ(3)∈L2[t0,tf],ϑ(t0)=0,ϑ(tf)=0}.
The inner product and its norm in W32[t0,tf] are given by:
Remark 2.1. The Hilbert space Wm2[t0,tf] is called a reproducing kernel if for any fixed t∈[t0,tf], ∃Kt(s)∈Wm2[t0,tf] such that ⟨ϑ(s),Kt(s)⟩Wm2=ϑ(t) for any ϑ(s)∈Wm2[t0,tf] and s∈[t0,tf].
Remark 2.2.
(1) In [56], W12 is RKHS and its reproducing kernel is:
(2) In [57], W22 is RKHS and its reproducing kernel is:
3.
Reproducing kernel Hilbert space method (RKHSM)
In this section, we develop an iterative method for constructing and calculating fractional differential equations with a temporal two-point solution. In order to emphasize the idea, we start by considering the general form of the BVP:
Subject to BC's:
where:
δ, β ∈R, and Dα denotes the Caputo fractional derivative of order α and
We use the RKHS method to obtain a solution of BVPs (3.1) and (3.2) based on the following methodology:
● To attain a problem with homogenous BC's, we first assume that: Y(t0)=γ, (γ arbitrary) and
We get:
Subject to:
● Then, we construct the reproducing kernel space W22[t0,tf] in which each function satisfies the homogeneous boundary conditions of (3.5) using the space W12[t0,tf].
Take Kt(τ) and Rt(τ) to be the reproducing kernel functions of the spaces W22[t0,tf] and W12[t0,tf] respectively.
● Next, we define the invertible bounded linear operator L:W22[t0,tf]⟶W12[t0,tf] such that:
The BVPs (3.4), (3.5) can therefore be transformed to the following form:
Where U(t) and V(t) are in W22[t0,tf] and F,G∈W12[t0,tf].
Applying Riemann-Liouville fractional integral operator Jαt0 to both sides using U(t0)=0 and V(t0)=0, we get:
Thus, we can notice that: LU(t)=U(t), and so the BVPs are transformed to the equivalent form:
● When choosing a countable dense set {ti}∞i=1 from [t0,tf] for the reproducing kernel of the space W22[t0,tf], we define a complete system on W22[t0,tf] as: Ψi(t)=L∗Φi(t) where Φi(t)=Rti(τ), and L∗ is the adjoint operator of L.
Lemma 3.1. Ψi(t) can be written on the following form:
Proof. It is clear that:
● The orthonormal function system {¯Ψηi(t)}∞i=1, η=1,2 of the space W22[t0,tf] can be derived from Gram-Schmidt orthogonalization process of {Ψηi(t)}∞i=1 as follows:
where Bηik are positive orthogonalization coefficients such that:
Cηik given by: ⟨Ψηi,Ψηk⟩W22.
Theorem 3.1. If the operator L is invertible i.e: L−1 exist, and if {ti}∞i=1 is dense on [t0,tf], then {Ψηi}∞i=1, η=1,2 is the complete function system of the space W22[t0,tf].
Proof. For each fixed U(t),V(t)∈W22[t0,tf], let ⟨U(t),Ψ1i(t)⟩=0,and ⟨V(t),Ψ2i(t)⟩=0,i=1,2,... that is:
since {ti}∞i=1 is dense on [t0,tf] then LU(t)=0, and LV(t)=0 it follows that U(t)=0,V(t)=0 since L−1 exist and U(t),V(t) are continuous.
Theorem 3.2. For each U(t),V(t)∈W22[t0,tf] the series
are convergent in the sense of the norm of W22[t0,tf]. In contrast if {ti}∞i=1 is dense subset on [t0,tf] then the solutions of (3.8) given by:
Proof. Let U(t),V(t)∈W22[t0,tf] be the solutions of (3.8), since U(t),V(t)∈W22[t0,tf], and ∑∞i=1⟨U(t),¯Ψ1i(t)⟩W22[t0,tf]¯Ψ1i(t) and ∑∞i=1⟨V(t),¯Ψ2i(t)⟩W22[t0,tf]¯Ψ2i(t) represent the Fourier series expansion about normal orthogonal system {¯Ψηi(t)}∞i=1, η=1,2, and W22[t0,tf] is Hilbert space, then the series ∑∞i=1⟨U(t),¯Ψ1i(t)⟩W22[t0,tf]¯Ψ1i(t),∑∞i=1⟨V(t),¯Ψ2i(t)⟩W22[t0,tf]¯Ψ2i(t) are convergent in the sense of ‖.‖W22[t0,tf]. In contrast, according to the orthogonal basis {¯Ψηi(t)}∞i=1, we have:
The same for finding V(t):
The theorem is proved.
Since W22 is Hilbert space we get:
∑∞i=1∑ik=1B1ik⟨LU(t),Φ1k(t)⟩W12¯Ψ1i(t)<∞ and ∑∞i=1∑ik=1B2ik⟨LV(t),Φ2k(t)⟩W12¯Ψ2i(t)<∞.
Hence:
are convergent in the sense of ‖.‖W22 and (3.11) represents the numerical solution of (3.8).
Remark 3.1.
(1) If the system (3.7) is linear, then the exact solutions can be found directly from (3.10).
(2) If the system (3.7) is non linear, then the exact and numerical solutions can be obtained by:
where:
We use the known quantities ληi,η=1,2 to approximate the unknowns Aηi,η=1,2 as follows: we put t1=t0 and set U0(t1)=U(t1),V0(t1)=V(t1) then U0(t1)=V0(t1)=0 from the conditions of (3.8), and define the n-term approximation to U(t),V(t) by:
where the coefficient ληi(η=1,2,i=1,2,...,n), are presented as follows:
and so:
We can guarantee that the approximations Un(t),Vn(t) satisfies the conditions enjoined by (3.7) through the iterative process of (3.16).
4.
Error estimation and convergence
In this section, we present some convergence theories to emphasize that the approximate solution we got is close to the exact solution. Indeed, this finding is very powerful and efficient to RKHS theory and its applications.
Lemma 4.1. ‖Un(t)‖∞n=1, and ‖Vn(t)‖∞n=1 are monotone increasing in the sense of the norm of ‖.‖2W22.
Proof. Since ‖¯Ψηi(t)‖∞i=1,η=1,2 are the complete orthonormal systems in the space W22[t0,tf] then we have:
Thus ‖Un(t)‖W22,‖Vn(t)‖W22 are monotone increasing.
Lemma 4.2. As n→∞, the approximate solutions Un(t),Vn(t) and its derivatives U′n(t),V′n(t) are uniformly convergent to the exact solutions U(t),V(t) and its derivatives U′(t),V′(t) respectively.
Proof. For any t∈[t0,tf]:
and
if ‖Un(t)−U(t)‖W22⟶0,‖Vn(t)−V(t)‖W22⟶0 as n→∞, then the approximate solutions U(i)n(t),V(i)n(t) are uniformly converges to the exact solutions U(i)(t),V(i)(t)i=1,2 respectively.
Theorem 4.1. If
and F(t,U(t),V(t)),G(t,U(t),V(t)) are continuous in [t0,tf], then:
Proof. For the first part, we will prove that:
it is easy to see that:
{|Un−1(tn)−U(t)|=|Un−1(tn)−Un−1(t)+Un−1(t)−U(t)|≤|Un−1(tn)−Un−1(t)|+|Un−1(t)−U(t)|,|Vn−1(tn)−V(t)|=|Vn−1(tn)−Vn−1(t)+Vn−1(t)−V(t)|≤|Vn−1(tn)−Vn−1(t)|+|Vn−1(t)−V(t)|,
by reproducing property of Kt(τ) we have:
and
thus
{|Un−1(tn)−Un−1(t)|=|⟨Un−1(τ),Ktn(τ)−Kt(τ)⟩W22|≤‖Un−1(τ)‖W22‖Ktn(τ)−Kt(τ)‖W22,|Vn−1(tn)−Vn−1(t)|=|⟨Vn−1(τ),Ktn(τ)−Kt(τ)⟩W22|≤‖Vn−1(τ)‖W22‖Ktn(τ)−Kt(τ)‖W22,
and from the symmetric property of Kt(τ) we get:
hence: |Un−1(tn)−Un−1(t)|⟶0 as tn→t.
By lemma (4.2)
thus:
Therefore
in the sense of the ‖.‖W22 as tn⟶t and n⟶∞ for any t∈[t0,tf].
Moreover, since F and G are continuous, we obtain:
Theorem 4.2. Suppose that ‖Un‖W22 and ‖Vn‖W22 are bounded in Eq (3.14), if {ti}∞i=1 is dense on [t0,tf], then the approximate solutions Un(t), Vn(t) in Eq (3.14) convergent to the exact solutions U(t),V(t) of Eq (3.7) in the space W22[t0,tf] and U(t),V(t) given by (3.12).
Proof. We first start by proving the convergence of Un(t) and Vn(t) from Eq (3.14) we conclude that:
by orthogonality of {¯Ψηi(t)}∞i=1,(η)=1,2 we get:
‖Un(t)‖W22,‖Vn(t)‖W22 are monotone increasing by Lemma (2). From the assymption that ‖Un(t)‖W22,‖Vn(t)‖W22 are bounded, ‖Un(t)‖W22,‖Vn(t)‖W22 are convergent as n→∞, then ∃c,d constants such that
if m>n using
further that
so:
since W22[t0,tf] is complete, ∃U(t),V(t) in W22[t0,tf] such that
in the sense of the norm of W22[t0,tf].
Now, we prove that U(t),V(t) are solutions of Eq (3.7). Since {ti}∞i=1 is dense on [t0,tf],∀t∈[t0,tf],∃ subsequence {tnj} such that tnj⟶j→∞t. From lemma (3) and (4) in [25] we have:
let j goes to ∞, by theorem (4.1) and the continuity of F and G we have:
that is U(t),V(t) are solutions of Eq (3.7).
Theorem 4.3. Let ξn=|Un(t)−U(t)|, ξ′n=|Vn(t)−V(t)|, where: Un(t),Vn(t),U(t),V(t) denote the approximate and the exact solutions respectively, then the sequences of numbers {ξn},{ξ′n} are decreasing in the sense of the norm ‖.‖W22 and ξn⟶n→∞0,ξ′n⟶n→∞0.
Proof. From the extension form of U(t),V(t) and Un(t),Vn(t) in Eqs (3.12), (3.14) and (3.15) we can write:
and
Clearly: ‖ξn‖∞n=1,‖ξ′n‖∞n=1 are decreasing in a sense of ‖.‖W22 from theorem (3.2) the series ∑∞i=1λ1i¯Ψ1i(t),∑∞i=1λ2i¯Ψ2i(t) are convergent, thus ‖ξn‖W22⟶0,‖ξ′n‖W22⟶0 as n⟶∞.
Theorem 4.4. The approximate solutions Un(t),Vn(t) of (3.7) converge to its exact solutions U(t),V(t) with not less than the second order convergence. That is: |Un−U|≤Mk2 and |Vn−V|≤Nk2, where k=tf−t0n.
Proof. See [36].
5.
Numerical examples and algorithm
Numerical examples are conducted in order to verify the accuracy of this method. Computations are performed using Mathematica 11.0.
Algorithm 1: Use the following stages to approximate the solutions of BVPs (3.4) and (3.5) based on RKHS method.
● Stage A: Fixed t∈[t0,tf] and set τ∈[t0,tf]
for i=1,...,n do the following stages:
- stage 1: set ti=t0+(tf−t0)in;
- stage 2: if τ≤t let
else let
- stage 3: For η=1,2;
set
Output the orthogonal functions system Ψηi(t).
● Stage B: Obtain the orthogonalization coefficients Bηij as follows:
For η=1,2;
For i=1,...,n;
For j=1,...,i set Cηik=⟨Ψηi,Ψηj⟩W22 and B11=1Sqrt(Cη11).
Output Cηij and B11.
● Stage C: For η=1,2;
For i=1,...,n, set Bηii=(‖Ψηi‖2W22−∑i−1k=1(Cηik)2)−12;
else if j≠i set Bηij=−(∑i−1k=1CηikBηkj).(‖Ψηi‖2W22−∑i−1k=1(Cηik)2)−12.
Output the orthogonalization coefficients Bηij.
● Stage D: For η=1,2;
For i=1,...,n set ¯Ψηi(t)=∑ik=1BηikΨηi(t).
Output the orthonormal functions system ¯Ψηi(t).
Stage E: Set t1=0 and choose U0(t1)=0,V0(t1)=0;
For η=1,2;
For i=1 set
For i=2,3,...,n set
set
Outcome the numerical solutions Un(t),Vn(t).
Then we implement the above algorithm using numerical simulations. We arrange the resulting data in tables and graphs for examples discussed on [t0,tf] as follows:
Example 5.1. Consider the following system:
subject to:
with exact solution when \alpha = 1 is:
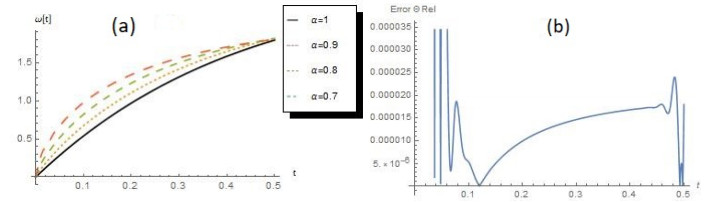
After the initial conditions have been homogenised and algorithm 1 used, apply t_i = \frac{0.5i}{n} , \overline{i = 1, n}\; and n = 40 , the tables 1 and 2 describe the exact solutions of \omega(t) and \Theta(t) and approximate solutions for different values of \alpha .
Graphs of the approximate solutions of \omega(t) are plotted in Figure 1 (a), for different values of \alpha . It is obvious from Figure 1 (a) that the approximate solutions are in reasonable alignment with the exact solution when \alpha = 1 and the solutions are continuously based on a fractional derivative. The graph in Figure 1 (b) represent the absolute errors of \theta(t) .
Example 5.2. Consider the following system:
with conditions:
when \alpha = 1 the exact solution is:
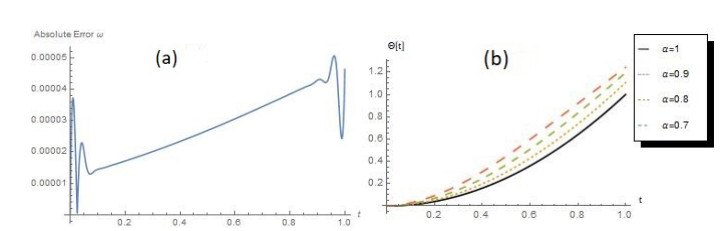
After homogenizing the initial conditions and using algorithm 1, apply t_i = \frac{i}{n} , \overline{i = 1, n}\; and n = 35 , the tables 3 and 4 describe the exact solutions of \omega(t) and \Theta(t) and approximate solutions for different values of \alpha .
Graphs of the approximate solutions of \theta(t) are plotted in Figure 2 (b) for different values of \alpha . The graph in Figure 2 (a) represent the absolute errors of \omega(t) .
Example 5.3. Consider the following fractional system:
subject to:
with exact solution:
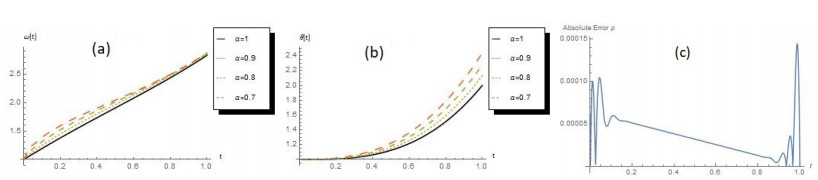
After the initial conditions have been homogenised and algorithm 1 used, apply t_i = \frac{i}{n} , \overline{i = 1, n}\; and n = 30 , the tables 5-7 describe the exact solutions of \omega(t) , \Theta(t) and \rho and approximate solutions for different values of \alpha .
Graphs of the approximate solutions of \omega(t) and \theta(t) are plotted in Figure 3 (a), Figure 3 (b) for different values of \alpha . The graph in Figure 3 (c) represent the absolute errors of \rho(t) .
Now, we consider the following tables where the RKHS method has been applied in order to give numerical approximations with other values of n, and then compare it with finite difference and collocation methods.
6.
Conclusions
In this article, we effectively utilize the RKHSM to develop an approximate solution of differential fractional equations with temporal two-point BVP. The results of examples demonstrate reliability and consistency of the method. In the future, we recommend further research on the RKHS method, as solving the temporal two-point boundary value problems with the conformable and the Atangana-Baleanu derivatives. We expect to achieve better results and good approximations for the solutions.
Conflict of interest
The authors state that they have no conflict of interest. All authors have worked in an equal sense to find these results.







 DownLoad:
DownLoad: