Water resources management versus the world

1.   Introduction

Water resources management has traditionally focused on satisfying human-based needs and demands more than environmental water needs. Its focus is to service multiple goals from storing water to securing water supply to the removal and treatment of wastewater, and responding to flooding and stormwater both as risks and occurrences [1,2]. Thus, water resources management has been defined as "… controlling the supply, distribution, use and disposal of water to achieve specific objectives…" [3]. Water, in this context, is either a resource for human use or a problem disrupting human production and activities.

In her opening sentence of the Forewordto the UN-Water's 2018 World Water Development Report, the Director-General of UNESCO, makes what can be read as a plea or a call to arms: "We need new solutions to managing water resources to offset the raising challenges to water security from population growth and climate change" [4]. This statement implies that some organisations and people may be losing faith in the effectiveness and ability of traditional human-centric approaches to water management to achieve sustainable water resources goals [2]. Some support to this view is provided by [1] in pointing out that uncertainty surrounds the fate of water resources management to be able to adapt or effectively respond to changes in water availability. For example, some uncertainty derives from rainfall-runoff modelling and the relationship to stream flows and, thus, the availability of water [1]. Natural variability in stream flows across catchments plays a further role in creating uncertainty [5].

The diversity and intensity of threats and pressures that water resources are exposed to, for example, climate change [6], underlies a loss of faith in traditional water resources management approaches to achieve sustainable water availability. Increasing temperatures and drought intensities associated with climate change trends are shaping several negative impacts affecting water availability from, for example, enhanced evaporation of soil moisture, increased evapotranspiration from vegetation, reduced runoff and stream flows [7–9]. Although evaporative processes return water to atmospheric storage and the hydrological cycle [8,10,11] there is no guarantee it will return as rainfall to where it came from. Nonetheless, these processes are important for local environments and ecosystems linked within place-based hydrological cycles and, thus, water availability and a holistic place-based management of water [11,12].

The effect of changing climates and rainfall patterns cumulatively intensify the risks to maintaining water availability as they influence deviations from expected rainfall and drying patterns, for example, wet areas becoming wetter and dry areas becoming drier [7,13]. Furthermore, human-driven threats from growing populations and urbanisation, and agricultural production related to food security issues are intensifying the pressures on water management to achieve sustainable water availability due to increased water demands [4]. The multiple threats to water resources are predicted to intensify throughout the coming decades thereby worsening water availability and scarcity conditions leading to further declining availability of water resources [14]. In fact, the UN-Water's 2018 World Water Development Report identifies declining water availability as a current and real occurrence in many countries globally [4]. This is supported by [8] in a study which revealed that 187 of the 405 basins investigated suffered severe water scarcity for up to 9 months of the year, while a further 14 basin suffered severe water scarcity for 11 or 12 months of the year.

The linking of social and economic benefits to sustainable water resources management systems illustrates the continuing human-centric focus of water management [4]. In this context, a human-centric system traditional water resources management focused on achieving social and economic outcomes from the use of water can be said to be a threat to itself. Water resources management from this human-centric perspective attempts to "force" water to fit human ideals of water as only a resource to be exploited. In short, traditional water management is a "water processing" system functioning to service only human demands. Thus, water is a resource to be exploited to service human social and economic systems. The environment in this relationship remains merely the source for resources for human use as reflected in trade-offs made, for example, biasing agricultural irrigation water demands over environmental water demands [15,16].

Retaining a human-centric approach as a business-as-usual model for water resources management in terms of securing water for human use and urban sanitation services is unlikely to secure sustainable water availability for the future given the current threats from growing populations and urbanisation and climate change and the predicted trends of increasing frequencies and intensities or drought and flooding events [4,9,17]. Sustainable water availability relative to water demands is a significant environmental and social issue for the twenty-first century [9]. Thus, if the traditional approach to water management derived from entrenched human-centric perspectives of water associated with economic development and agricultural production [15] is not re-evaluated relative to the expected intensification of diverse human and climate change impacts, the availability of water as historically known may become a mere memory.

The notion that water resources may not be available to service human demands as it has been in the past is a key challenge water resources management approaches need to confront. Many water resources management decisions have been based on historical rainfall records which may suggest that water is abundant as rainfall will remain regular [18,19]. The thinking here is that while there will be periods of rainfall deficit and drought, "normal" rainfall patterns will return and replenish water flows and storage systems. This is based on the concept of "stationarity": "…hat natural systems fluctuate within an unchanging envelope of variability…" [8]. Hence, some predictability can be associated with rainfall patterns. However, [19] further argue that due to the apparent death of stationarity conditions and, thus, the emergence of non-stationarity conditions where natural systems may fluctuate beyond the historical range of variability, from preceding human actions and climate change impacting on the stationarity of planetary systems' natural processes, new extreme climatic trends are occurring. If non-stationarity is an ongoing trend and no adequate response to achieve sustainable water availability emerges as a key goal for water resources management, future environmental and human health and well-being is expected to be dire if not catastrophic [20]. If water is truly considered or accepted as a public good for the benefit of all, including the environment and ecosystems, then water needs to be acknowledged as a key stakeholder in its management and not traded off against continuing economic development aspirations. Without water, economic activities, including agricultural production, and society more broadly, will not progress [20]. Consequently, it is no longer a viable option to wait for a return to stationarity planetary conditions of regular and predictable rainfall patterns [21,22]. This scenario points to the importance of transitioning water resources management to a holistic water-centric focused system in which water is acknowledged as a key stakeholder and sustainable water availability is a key goal and outcome.

Sustainable water availability is defined here as when the availability of water is maintained within the limit of "peak water" [23] for meeting the consumptive needs of water users. Peak water is when available freshwater resources begin to become significantly stressed and reach their maximum limit of availability due to water extraction exceeding replenishment and natural water flows being significantly reduced leading to environmental and ecological damage [20,23]. The limit of peak water for the purposes of sustainable water availability may not exceed 20% of total streamflow and runoff as calculated by [8]. In short, [8] calculate the availability of fresh (blue) water for human consumption from natural runoff is 20% of total monthly runoff. Under [8] performance metrics, total water scarcity/unavailability is reached when all available water (i.e., 20% of natural runoff) is consumed. The remaining 80% is the presumed flow to meet environmental demands. In short, consuming more than 20% of natural runoff will impact the environment including the functioning and health of diverse ecosystems. However, [8] note that the 20% metric for natural runoff within a catchment is a precautionary standard to avoid over-consumption of water resources which will lead to significant water availability deficits for environmental requirements and, consequently, declining environmental and ecosystem health and functioning. Thus, maintaining sustainable water availability requires that ongoing water consumption does not exceed 20% of natural monthly runoff. These monthly performance metrics can underpin a water-centric focused water resources management system with the central goal of achieving and maintaining sustainable water availability. Such a water-centric approach means that water demands, and consumption, will need to be flexible and adaptive to the variability of place-based natural runoff flows and the influences of changing climates and rainfall patterns. It is clear from [8] analysis that if additional water is diverted from environmental flows to meet increasing human demand and consumption, the risk of increasingly negative impacts on environmental and ecosystem health and, consequently, human health and well-being intensifies.

In response to developing sustainable water availability as a key water resources management outcome and the UNESCO Director-General's plea, as identified above, it is time to transition from the current human-centric water resources management towards a holistic water-centric focused water management system in which water is acknowledged as a key stakeholder in its management with sustainable water availability being a key goal and crucial outcome. Several approaches can be implemented to enhance a holistic water-centric focused water management system, including adopting nature-based solutions (NBS) [4,24,25], water soft paths [26], and green infrastructure [27,28] as strategies to assist in the realisation of sustainable water resources management outcomes.

This conceptual paper discusses the possible development of a holistic water-centric focused water resources management to meet the challenge of developing sustainable water availability derived from blending NBS, water soft paths, and green infrastructure as a response to human and climate change threats to water resources. NBS, water soft paths, and green infrastructure are discussed as potential components of a water-centric focused water resources management system in the next section. This is followed by a discussion conceptualising the development of a holistic catchment-wide water-centric focused water resources management system. Policy implications are then considered prior to brief conclusions closing this paper

2.   Nature-based solutions, water soft paths, and green infrastructure: components of a holistic water-centric focused water management system

Water availability is under threat from climate change impacts influencing changing rainfall patterns and increasing drought intensities [6,7,13]. There are global trends in declining rainfall and increasing drought frequencies and intensities throughout the 21^st Century potentially influencing a decrease in water availability [29]. The severe impacts of droughts on water availability is evidenced by recent events in South Africa [30] and in Australia through the millennium drought [31]. These trends in changing climates and rainfall patterns along with pressures from diverse human-based threats as population and urbanisation growth, and agricultural production influencing increases in water demands, provide a rationale for concentrating the goal of water resources management on achieving sustainable water availability within a new water-centric focused water resources management system.

Further concern relates directly to whether current water management systems being focused on maintaining water storage and supply can effectively achieve a goal of sustainable water availability under increasing urban, agricultural, and climate change threats [2]. In response to these concerns, a holistic water-centric focused water resources management perspective is proposed and presented in the following sections. NBS, water soft paths, and green infrastructure are innovative perspectives gaining momentum as water resources management strategies [2,4,28]. It is argued that adopting NBS, water soft paths, and green infrastructure strategies and technologies in conjunction with water-centric approaches to catchment and landscape management can provide a holistic approach to achieving sustainable water availability.

2.1.   Nature-based solutions

NBS are strategies based on natural processes or mimic natural processes operating in nature which are implemented in urban environments to augment water management responses to, for example, stormwaters and wastewaters [25,32,33]. Examples of NBS includes "constructed wetlands and evapotranspirative willow systems" [32] and are considered effective economical and cost-effective means to treat wastewaters towards recycling treated water through a water-centric water resource management system. Constructed wetlands in urban areas are intended to mimic natural wetlands functions in the treatment of wastewaters. Evapotranspirative willow systems are constructed watertight beds containing soil in which chosen willow duplicates are grown. These systems treat wastewater and produce biomass for energy production [32,34].

A NBS perspective as linked to biomimicking utilises the natural processes of place as "blue-prints" to design effective and holistic water management approaches, for example, developing constructed wetlands for treating stormwater and wastewater [33,35]. It is argued that mimicking natural processes of nature can be effective and efficient responses to reduce threats to water resources management systems [36]. In this context, nature is a teacher from which humans can learn. Learning from nature provides opportunities for understanding the limits and variability of natural resources, including water availability. Such learning opportunities may facilitate human systems becoming more flexible in response to water resource limits and variability and highlights the importance of positioning water as a key stakeholder in a water-centric focused water management system with an established goal of achieving sustainable water availability. That is, human systems need to "fit" within the water resource limits and variabilities rather than human systems trying to "fit" water resources into constructed management system parameters. For as [37] state, "… humans are an indivisible part of nature…" which strongly positions human systems functionality within the limits and variability of nature's resources.

NBS need to be considered and implemented in the knowledge that they are long-term strategies, not temporary or short-term technical quick fixes. As [37] point out, "expanding the focus from nature-based solutions to deeper conceptualisations of nature will shift the focus from more shallow to deeper leverage points." That is, the implementation of NBS within water-centric focused water management approaches, for example, redefines the relationship between the human and nonhuman systems as collaborative.

In linking NBS with water management, [34] define NBS as involving "… the planned and deliberate use of ecosystem services to improve water quantity and quality and to increase resilience to climate change." Underpinning an increasing interest in implementing NBS as water management approaches is that in mimicking natural processes of nature, they are multi-functional. For example, NBS provide filtering and treatment services to improve quality through constructed wetlands which can also provide biomass for energy. Furthermore, NBS act to reduce the impacts of flooding as vegetation and forests slow and trap the flow of floodwaters leading to increased infiltration and further recharging of groundwater storage [2,34]. However, NBS are not replacements for traditional grey water infrastructure but rather are implemented alongside current infrastructure whereby each approach acts in collaboration with the other [36,38].

2.2.   Water soft paths

A water-centric water resources management system also incorporates water soft path approaches, for example, recycling grey water from laundries, showers, and baths to irrigate lawns and gardens [26,39]. Other water soft path related strategies include implementing "vacuum" toilets which capture black wastewater and/or "waterless dry toilets" and waste separation technologies that separate urine and solid waste which require minimal change to new toilets incorporating waste separation technologies and "drying pans" [32]. Rainfall harvesting in urban areas is a further soft path option for urban spaces and contributes to achieving sustainable water availability. These approaches and strategies increase the conservation of water thereby reducing pressures to attain additional sources of supply, for example, groundwater, and pressures to invest in further grey infrastructure [39].

A water soft path element of water management acknowledges the limits of water availability and, consequently, promotes water conservation and saving and recycling as water-centric goals to reduce water supply pressures. In short, a water soft path approach aims to decrease the demand for water rather than increasing the demand for new water supply sources. Decreasing the demand for water refers to finding alternative ways to complete tasks without water or at least reducing the amount of water required, for example, using vacuum or composting toilets [26,39]. Furthermore, a water soft path approach associated with demand management challenges the notion of the necessity for water to complete all tasks. For example, why is potable water used to remove waste? In this context, a water soft path approach is complementary to and with NBS and, thus, a water-centric water management approach with the goal of achieving sustainable water availability through water conservation.

Furthermore, through water soft path approaches alternative "sources" of water, for example, from water savings and recycling, may reduce overall pressure on 20% of monthly water availability allocated for human consumptive needs as discussed previously [8,26]. However, there needs to be a distinction between needs and wants/demands for water. A need being related to essential services including potable water uses, for example, hydration and food preparation, and for personal hygiene. Essential sanitation services for the removal of wastewaters can be accomplished with lower quality of water than potable quality. Further demands above the essential needs are considered "wants". Many of these "water wants" may be serviced by recycled water in which quality is matched to purpose [26,39]. For example, irrigation of urban public green spaces and household gardens and lawns can be serviced with recycled water of lower quality as can several agricultural demands for water [39]. A further benefit from this solution is that as the water infiltrates into the soil it can increase vegetation and crop productivity or return to stream flows or groundwater storage.

2.3.   Green infrastructure

There is some overlap of green infrastructure approaches and NBS. For example, both claim the use of open public green spaces, planting trees along streets, creating green walls and rooftop gardens and vertical (wall) gardens as strategies [4,36,38,40]. Further examples of green infrastructure approaches include riparian conservation as flood buffers, conservation of natural wetlands to facilitate water treatment processes to improve quality, increasing green spaces with urban areas, and increasing the area of permeable urban surfaces [28]. Using vegetation, for example, grass areas and trees, as green infrastructure to diffuse floodwaters and increase opportunities for water storage to occur through infiltration are further benefits of biomimicking natural processes within a water-centric and holistic community-wide water management system [33,41].

Within a green infrastructure approach to water resources management, ecosystems, for example wetlands and grasslands, can be conceptualised as effective natural water infrastructure. They improve water quality and act to mitigate flood risks. From an economic perspective, ecosystems provide goods and services which enhance the development of human society, for example, a supply of clean water. However, although these are human-centric perspectives, there is an emerging realisation that maintaining healthy ecosystems simultaneously provides effective natural water management options. As such, green infrastructure can be considered as important water management investments [27]. As the [27] states in relation to water management, "leaving natural systems out of the planning process can be costly." Thus, investing in diverse ecosystems as strategies within water resources management has natural resources, economic, and societal benefits.

3.   Discussion: conceptualising a holistic water-centric focused water resources management system

As [42] argue, a transformational change in how the environment and the natural systems which underpin human existence are valued must drive the development of more sustainable human-environment interactions and relationships. In the context of water resources management one such transformational change in values can be that water is acknowledged as a key stakeholder and active agent influencing human affairs.

In line with water being an active agent in human affairs, [43] define water as a "master variable" underpinning and supporting the existence of ecosystems and humans. Others refer to water in this regard as the "bloodstream" of the environment [44] underpinning and supporting all life, human and nonhuman [45]. Given these strong conceptualisations, water needs to be held in the highest reverence and offered the highest safeguards and protection possible for it to continue underpinning environmental and human life and well-being. The wide-spread acknowledgement of water being the bloodstream of the environment and human existence, an effective water-centric focused water resources management approach must be considered as an imperative for environmental and human sustainability. A business-as-usual human-centric approach to water management can no longer be considered as effective or efficient under the expected consequences of changing climatic and environmental conditions and limits.

Many responses taken to develop sustainable water management systems are human-centric focused implemented primarily to reduce or counteract human vulnerabilities to increasing drought and flood frequencies and intensities while attempting to maintain a business-as-usual relationship with natural resources [43]. Consequently, little focus is directed by water resources management towards reducing the vulnerabilities of water resources to changing climatic conditions and human exploitation. Focusing on sustainable water availability as a key goal of water-centric driven water resources management approaches is a repositioning of attitudes towards reducing the vulnerabilities of water resources and human society to the impacts of changing climates and increasing human-based water demands. As noted by [43] "water availability and variability will define how the terrestrial biosphere adapts…" leading to a potential redistribution of ecosystems which in turn will impact society's relationships with the environment as a source of resources for productive and consumptive purposes. Furthermore, reductions in water availability and ecosystems will limit the adaptive capacities of communities and society to changing climatic impacts across spatial and temporal scales. Consequently, NBS/green infrastructure and water soft path strategies need to be implemented within a catchment- and community-wide vision for developing a water-centric focused water management system which targets sustainable water availability as its key goal and outcome [36].

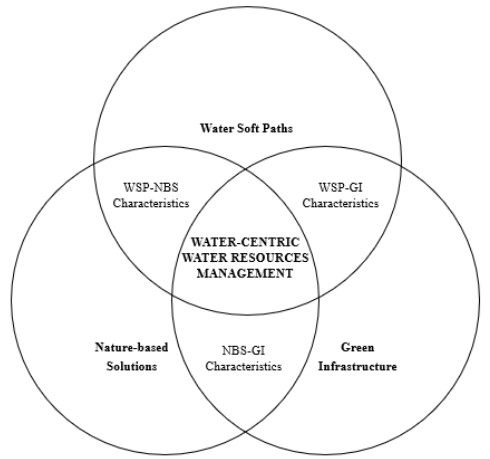
Figure 1 presents a very simplistic model of a water-centric water management system developed from the interconnected implementation of NBS, green infrastructure, and water soft paths. Within this system there are shared water-centric characteristics between NBS, green infrastructure, and water soft paths emphasising water as a key stakeholder, for example, conservation, storage, and quality. Shared characteristics between the interconnected approaches may include, water conservation/water storage (WSP-GI), matching water quality to use/improving water quality (WSP-NBS), and increased urban and catchment-wide vegetated (green) areas (NBS-GI).

Biomimicking strategies [33] implemented as NBS and green infrastructure, for example, constructed wetlands and evapotranspirative willow systems, illustrate the value of understanding the natural processes of places and ecosystems as possible "blue-prints" for guiding the implementation of NBS within a water-centric water resources management system [46]. The inclusion of ecosystems within holistic and water-centric focused water management approaches acts as "in situ" green/water infrastructure [46]. As water infrastructure, natural ecosystems along with NBS provide fresh and clean water through the filtering and treatment of polluted waters, and flood protection. Furthermore, through a greater use of natural ecosystems as green/water infrastructure a reduction in the cost of constructing grey infrastructure can be made [46].

It must also be noted that achieving an effective water-centric focused water resources management system is interdependent on other challenges to water being addressed simultaneously, for example, the management of catchments, landscapes, including land use, and drought impacts. In this context, river and floodplain management are elements of a water-centric water resources management system in terms of runoff as "a measure of sustainable water availability" [29] and the processes of river flows and floodplains, for example, through water storage capacities of soils and hydrological processes [12]. River and floodplain management can be, furthermore, supported with the implementation of NBS and natural ecosystems as green/water infrastructure [46] underpinning a water-centric water management system in terms of achieving sustainable water availability. Alternatively, without appropriate river and floodplain management collaborating with NBS and green/water infrastructure strategies sustainable water availability is under threat and, thus, becomes an uncertain outcome [46].

A water-centric focused water resources management approach will address these issues by positioning water as the key stakeholder interconnecting whole catchment, hydrological, and geomorphic contexts, including retention of water in soils leading to healthier soil structure and vegetation cover [12]. Furthermore, sustainable water availability, as a long-term goal of an effective water-centric water resources management approach, maintains sustainable river flows which in turn sustains the environment and provides an opportunity to meet the water demands of all users, including the environment and ecosystems, within the whole catchment [1,12]. However, even within a holistic water-centric focused water resources management system, water extractions from rivers need to occur within the catchment's sustainable water availability limits and variability as impacted and shaped by its hydrological connectivity to climate change and human consumptive uses of water [8,12]. The concern for water availability, in this context, is as more and more water is used, less and less water will be available to meet future social, economic development, and environmental demands. In this and similar situations making trade-offs to satisfy further or growing human water demands may impact the health of the environment and its ecosystems and, consequently, in the longer term, impact human health and well-being due to a reliance on ecosystem goods and service which underpin communities and society [45,47]. The bottom line is that water allocations for additional economic and/or production, including agriculture, and other non-potable, uses needs to be assessed within the place-based "peak water" limits and variability of catchment-wide sustainable water availability [23,45].

In response to declining urban water availability due to climate change impacts, [43] argue that human systems and society need to be reshaped in line with a recognition that water is a master variable that underpins human and societal interactions with the environment. An efficient and effective urban water management system can be developed to service the community's water needs. This includes matching water quality with purpose of use, for example, using low quality water for removing waste. This will increase the availability of potable water for drinking and industries requiring higher quality of water in their production processes. So, the questions a water soft path approach may ask are: why use water of high quality for a particular function when water of a lower quality can be as suitable? Are there alternative ways water can be used in particular functions? [39].

Within urban spaces sustainable urban design systems and water sensitive urban design [49] are components of a holistic water-centric water management approach addressing wastewater, stormwater, and sanitation issues [39]. These challenges increase as urban areas increase to accommodate population growth and migration trends to metropolitan areas while striving to generate circular cities whereby water conservation and recycling are important strategies for achieving sustainable water availability [35,39]. In urban areas there are multiple under-utilised areas, for example, rooftops, in which NBS and green infrastructures can be implemented in collaboration with water soft paths contributing to a holistic water-centric focused water management strategy [35].

Avoiding the collapse of natural water system from the impacts of climate change must be a priority for effective water management systems [43]. This points to the notion that water needs to be acknowledged as a key stakeholder in a water-centric focused water resources management system [4]. Hence, the importance of shifting to a water-centric focused approach which emphasises sustainable water availability as the key goal and required outcome. Maintaining sustainable water availability will reduce the risk of system, natural and human, failures. This includes adopting water soft path strategies to enhance water conservation, savings, and recycling, as well as water sensitive technologies for example water-less toilets [26].

4.   Policy implications for water-centric focused water resources management systems

Appropriate legislation needs to provide guidance on transitioning to an effective water-centric focused water resources management system in which a key goal is achieving sustainable water availability. Thus, water resources and nature more broadly need to be re-evaluated beyond their economic values towards developing a more coexistent relationship between nature and humanity [33]. It is in this context that policy and regulatory approaches will need to provide frameworks for implementing NBS and water soft path strategies promoting and supporting behavioural change leading to water conservation and recycling. In short, appropriate policies will need to "… cultivate and negotiate relations with the material world" [50].

Furthermore, advancing appropriate policies and regulatory frameworks which support and guide a water-centric focus towards holistic sustainable water availability provides opportunities to interconnect and coordinate with broader catchment and land management systems in a more focused and synergetic water-centric management approach to underpin a goal of achieving place-based long-term sustainable water availability [4,51]. The command-and-control model for supplying water to households and businesses is no longer appropriate under changing environmental conditions. Such a model is not flexible enough to adapt to varying river flows dynamics and the uncertainty of water availability under changing climate and rainfall patterns associated with predicted trends in developing non-stationarity planetary conditions [11,19,22,52]. Due to changing climates and rainfall patterns under non-stationarity conditions, the old water paradigm focused on water supply through the building of physical infrastructure needs to be re-thought. No longer can a reliance on stable and regular (predictable) climate and rainfall regimes be used as a baseline for achieving sustainable water availability [21]. Hence, the natural processes of place need to be acknowledged in policy as playing a significant role in the provision of water and other natural resources that human social and economic systems rely on [52]. This is a significant challenge for water and catchment management policies, and society broadly, to recognise the agency of place and matter and its impacts on water resources management systems. The recognition of nature's agency and its impact on humanity is, simultaneously, recognition of nature's political status in changing human practices and behaviours [53].

Retaining the status quo with the infrastructure-based old water paradigm will not increase the effectiveness of water resources management practices. Attempting to "balance" social and economic needs with environmental and ecological needs has been tried in the past with little success as demonstrated by the ongoing environmental impacts and the increasing threats from climate change to society and natural resources [37]. A possible reason for unsuccessful attempts to balance social, economic, and environmental and ecological needs with the management of water resources is that trade-offs are required. As economic development is a powerful focus for many governments losing any perceived ability to develop the economy further, trade-offs will be biased towards business-as-usual economic development goals at a cost the environment is expected to pay [37,52]. Within the context of balancing human needs with environmental and ecological needs, [37] argued that "the time has come for humans to give back to nature…" No, humans need to go beyond "giving back". It is more a time for humans to place nature and its resources, for example, water, above concepts of commercialism. For without water, for example, economic production and development cannot progress. Water needs to be held in higher esteem and reverence to human needs.

Shifting to a water-centric focused water management system where water is acknowledged as a key stakeholder in its own management will be a challenge for public policy and regulatory frameworks to provide appropriate and efficient institutional support across all aspects of water resources management and governance. Such a shift in water resources management will need the governmental leadership at all levels in the provision of appropriate environmental and water policies and regulatory frameworks for effective water-centric resources management and water governance broadly [51,54]. This requires a broad re-thinking of the regulatory and policy domains governing catchment and river management, water allocations and water management itself to avoid potential jurisdictional conflicts between local and central governments [54]. However, it will be dependent of the will and ability of governments to shift from an anthropocentric dominated ideology to a water-centric driven water management and water governance models where water is acknowledged as a central stakeholder [4,54,55]. This is a significant challenge due to the embedded social and cultural designation of natural entities of nature as mere resources for consumption in social and economic systems of production linked to market orientated agendas and ongoing economic development associated with, for example, mining and agricultural sectors [18,42,48]. As noted by [20], "the water problem is predominantly a political one in which democratic politics can in fact work to achieve outcomes other than … a choice between destruction and despair..." Although [20] goes on to affirm that "politics offers the possibility of thinking and acting in such a way that it can actually change how the world works", the current neoliberal market-based model is a powerful opponent of change. Thus, politics may be seen to offer possibilities, but this may be all it can offer, possibilities without hope of actualisation.

A further challenge facing the implementation of NBS as with water soft paths strategies is that they need to be injected into current policies and further entrenched in future policy initiatives and regulatory frameworks. Current water management policies are, however, primarily focused on maintaining current grey water infrastructure. Consequently, current water policies will have little short- or long-term effect in addressing the impacts of climate change on water resources due to a business-as-usual market-based stance on economic development and use of natural resources [18]. Shifting to more "greener" options or water-centric approaches such as NBS and water soft paths are faced with significant challenges [34]. That is, NBS will reassessed in relation to alternative approaches, for example, the expansion of grey water infrastructure. Against such proposals, NBS and water soft paths run the risk of being assessed as being "unproven" strategies and, therefore, will not attract political or community support and/or funding investment [34,36]. However, for any serious shift away from traditional human-centric supply focused to a water-centric focused water resources management system with a goal of achieving sustainable water availability, institutional frameworks, policies, and incentives are required to scaffold the implementation of strategies and technologies from households to business and industries. And here lays a significant barrier to innovation in water resources management. Governments do not lead but follow and, thus, fail to be innovative [18].

5.   Conclusions

Traditional water resources management systems maintaining a business-as-usual approach driven by an anthropocentric perspective focused on water supply is inadequate to address the ongoing threats it faces from increased water demands from urban and population growth, increasing agricultural production to meet future food security, and the impacts of climate change, including increasing frequencies and intensities of droughts and flooding. It is in this sense that current water resources management is a threat to its own sustainability. Hence, the increasing significance of transitioning from a human-centric water management system to a water-centric focused goal of achieving sustainable water availability.

A water-centric focus which acknowledges water as a key stakeholder with a goal of achieving sustainable water availability, rather than merely supply management, provides an alternative framework for water resources management confronting diverse threats to sustainable water availability. Furthermore, the importance of achieving sustainable water availability being a key goal of a water-centric focused water management system is that it is a crucial component for the environment and ecosystems and of hydrological cycle providing clean water for human consumptive purposes.

In transitioning to and developing water-centric focused water management approaches, implementing NBS, green infrastructure, and water soft paths technologies as blended strategies provide innovative holistic water management opportunities. Implementing these strategies as a combined water management perspective simultaneously positions water as a central key stakeholder of water resources management and sustainable water availability as a key goal and outcome. Water soft paths can balance supply and demand pressures through solutions for water conservation and saving and recycling while NBS and green infrastructure can assist in treating and recovering water resources from wastewater and stormwaters. Furthermore, achieving catchment-wide sustainable water availability can provide a buffer to the impacts of climate change, extreme drought conditions, and ongoing hydrological variabilities. Importantly, however, a catchment-wide and urban water-centric focused water management approach allows for the development of beneficial human-environment relationships forged through water. Providing opportunities for water to remain connected to the environment is beneficial to the environment and humans as the environment and its ecosystems provide goods and services that underpin human health and life. The bottom line is, without sustainable water availability there does not seem to be must sense, common or otherwise, in the concept and practice of water resources management. Therefore, it is recommended for government leadership, through appropriate legislation and policy directions focused on water as a key stakeholder, to drive new, flexible, and innovative approaches to water-centric urban water management design and planning.

6.   Limitations and future research perspectives

One of the limitations of this paper is that it is a conceptual rethinking focused on water resources management rather than water governance models. However, a link between water-centric water resources management and water governance is indicated when discussing the challenges for governments to develop policy and regulatory frameworks to encompass water-centric approaches for the management and governance of water resources. As a conceptual paper a further limitation is the lack of empirical evidence provided. However, these limitations do provide opportunities for further research. For example, research to build relevant knowledge concerning the effectiveness of a blended NBS, green infrastructure, and water soft paths approach in developing catchment-wide and urban-based effective and efficient water-centric focused water resources management systems is necessary. A further research opportunity may concern what potential is there for water resources management and water governance to provide a conjoined water-centric focus in the management and governance of urban and catchment waters? Yet another opportunity is an assessment of the economic value of a water-centric focus in the management and governance of water relative to current water management and governance models. The results of this research will better inform appropriate water-centric environmental, catchment, and water resources management policies towards sustainable water availability.

Acknowledgements

I would like to thank Dr. Peter Hastings and two anonymous reviews for constructive comments on a previous draft.

Conflicts of interest

The author declares no conflict of interest.