Low-frequency relationship between money growth and inflation in Turkey

Huseyin Tastan; Sercin Sahin; Huseyin Tastan; Sercin Sahin

doi:10.3934/QFE.2020005

Quantitative Finance and Economics

2020, Volume 4, Issue 1: 91-120. doi: 10.3934/QFE.2020005

Previous Article Next Article

Research article

Low-frequency relationship between money growth and inflation in Turkey

Huseyin Tastan ,
Sercin Sahin ^,

Department of Economics, Yildiz Technical University, Istanbul, Turkey

Received: 01 January 2020 Accepted: 17 February 2020 Published: 20 February 2020
JEL Codes: E31, E51, C49

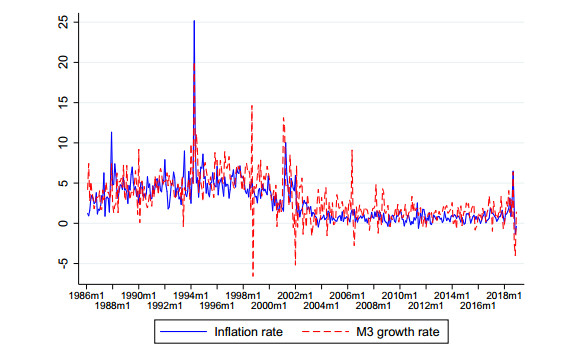
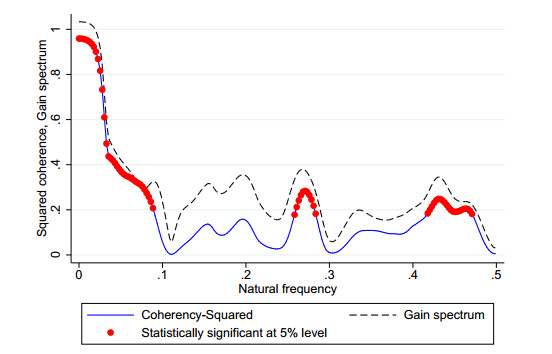
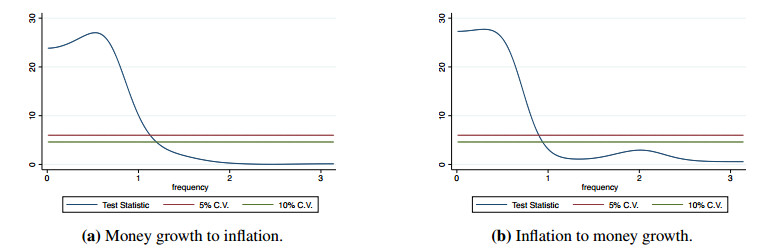
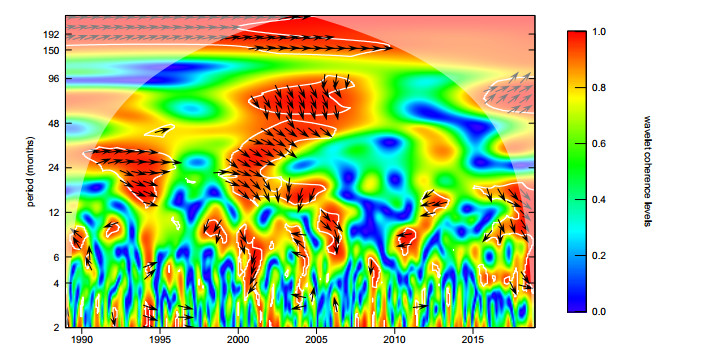
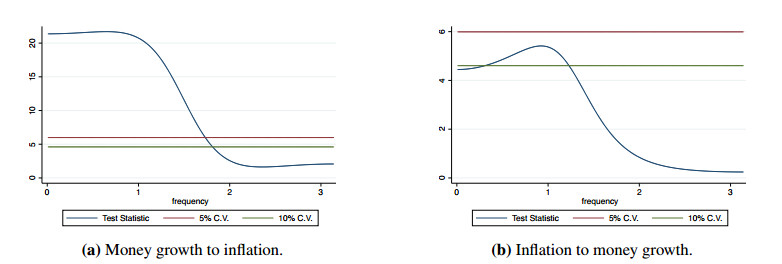
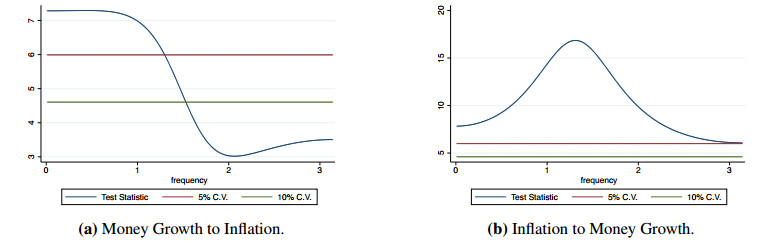
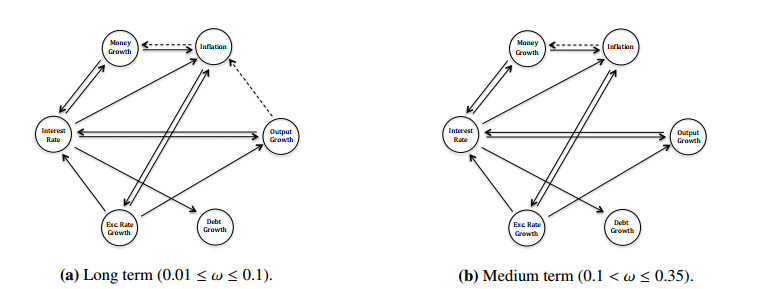
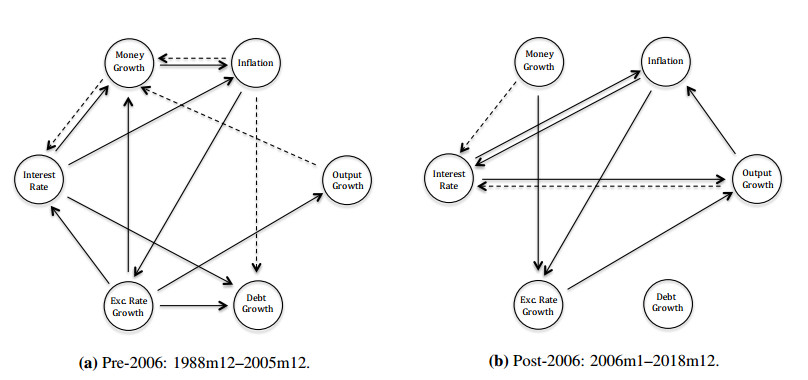
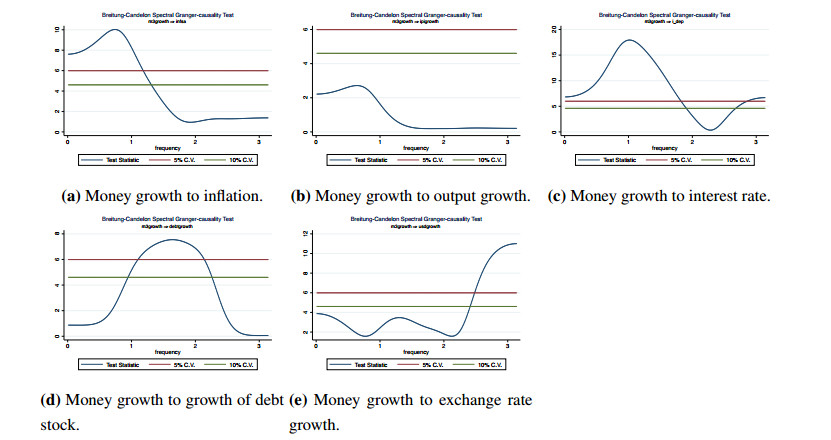
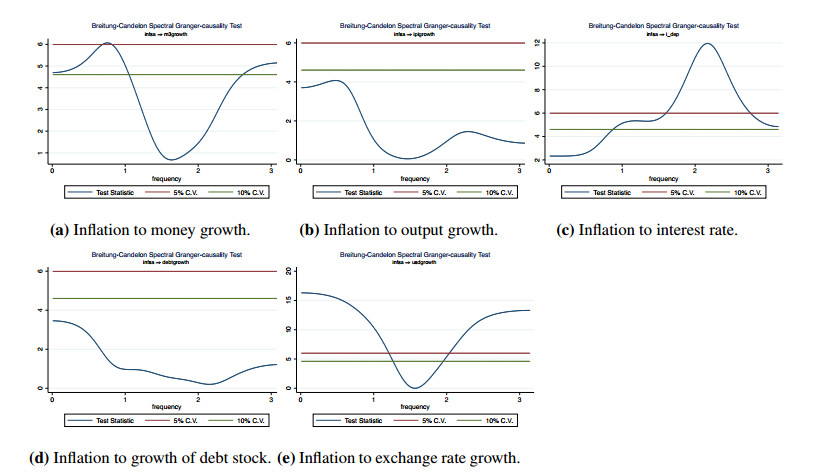
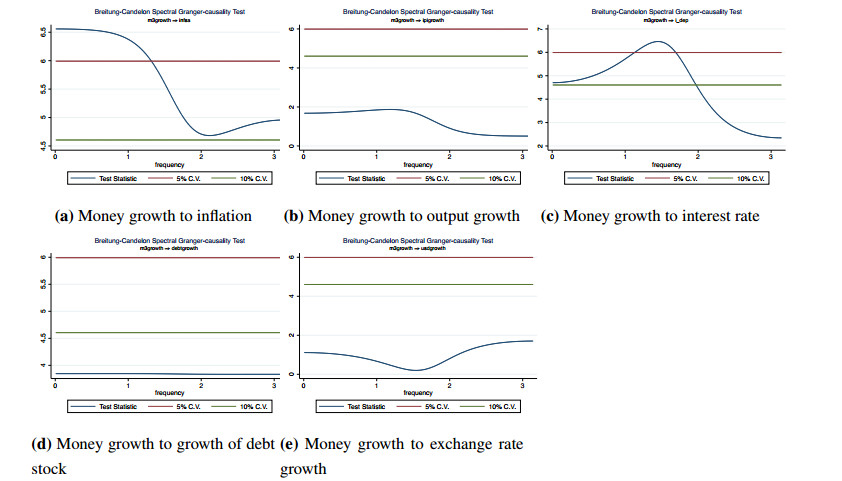
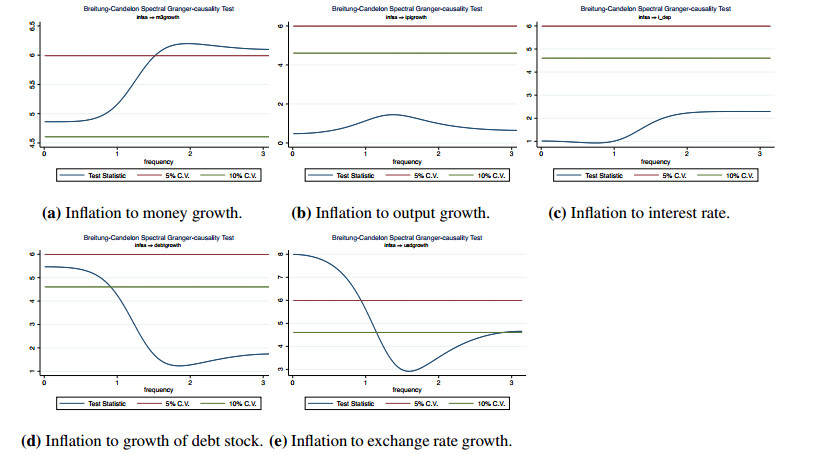
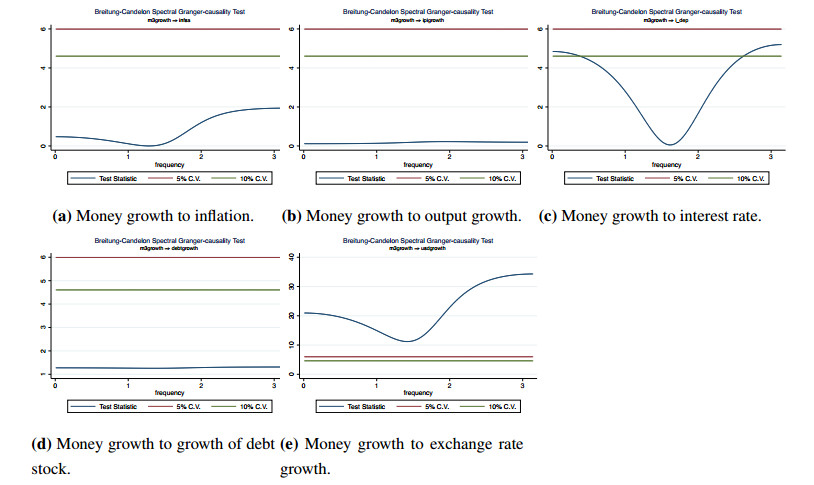
This paper examines the long-run and medium-run predictive relationship between money growth and inflation in Turkey for the period 1986m1–2018m12, using frequency-domain methods. For the full sample, the measures of spectral coherence and gain spectrum suggest a one-to-one relationship, and the frequency domain decomposition of the Granger causality test indicates a bidirectional predictive relationship between the two variables at zero frequency. As suggested by the wavelet coherence, we also analyzed the two subperiods before and after 2006 separately. Our results suggest that while both variables have predictive power for each other in the second subperiod, only money growth helps predict inflation in the first one. In order to prevent spurious results, the analysis is rerun in a multivariate Vector Autoregression (VAR) system, where output growth, interest rate, exchange rate growth, and domestic debt growth are included as additional variables. We observe that while money growth has predictive power for inflation in the first subperiod, this relationship disappears in the second one. We argue that the change in the relationship between the two variables at low frequencies after 2006 is primarily a result of the decrease in fiscal dominance of the government, the CBRT's switch to the inflation targeting regime, and the CBRT's "unconventional monetary policy framework".

Keywords:

Citation: Huseyin Tastan, Sercin Sahin. Low-frequency relationship between money growth and inflation in Turkey[J]. Quantitative Finance and Economics, 2020, 4(1): 91-120. doi: 10.3934/QFE.2020005

Related Papers:

[1]	P. Pirmohabbati, A. H. Refahi Sheikhani, H. Saberi Najafi, A. Abdolahzadeh Ziabari . Numerical solution of full fractional Duffing equations with Cubic-Quintic-Heptic nonlinearities. AIMS Mathematics, 2020, 5(2): 1621-1641. doi: 10.3934/math.2020110
[2]	SAIRA, Wenxiu Ma, Suliman Khan . An efficient numerical method for highly oscillatory logarithmic-algebraic singular integrals. AIMS Mathematics, 2025, 10(3): 4899-4914. doi: 10.3934/math.2025224
[3]	Kai Wang, Guicang Zhang . Curve construction based on quartic Bernstein-like basis. AIMS Mathematics, 2020, 5(5): 5344-5363. doi: 10.3934/math.2020343
[4]	Taher S. Hassan, Amir Abdel Menaem, Hasan Nihal Zaidi, Khalid Alenzi, Bassant M. El-Matary . Improved Kneser-type oscillation criterion for half-linear dynamic equations on time scales. AIMS Mathematics, 2024, 9(10): 29425-29438. doi: 10.3934/math.20241426
[5]	Dexin Meng . Wronskian-type determinant solutions of the nonlocal derivative nonlinear Schrödinger equation. AIMS Mathematics, 2025, 10(2): 2652-2667. doi: 10.3934/math.2025124
[6]	Samia BiBi, Md Yushalify Misro, Muhammad Abbas . Smooth path planning via cubic GHT-Bézier spiral curves based on shortest distance, bending energy and curvature variation energy. AIMS Mathematics, 2021, 6(8): 8625-8641. doi: 10.3934/math.2021501
[7]	Chunli Li, Wenchang Chu . Remarkable series concerning $\binom{3n}{n}$ and harmonic numbers in numerators. AIMS Mathematics, 2024, 9(7): 17234-17258. doi: 10.3934/math.2024837
[8]	Beatriz Campos, Alicia Cordero, Juan R. Torregrosa, Pura Vindel . Dynamical analysis of an iterative method with memory on a family of third-degree polynomials. AIMS Mathematics, 2022, 7(4): 6445-6466. doi: 10.3934/math.2022359
[9]	A. Palanisamy, J. Alzabut, V. Muthulakshmi, S. S. Santra, K. Nonlaopon . Oscillation results for a fractional partial differential system with damping and forcing terms. AIMS Mathematics, 2023, 8(2): 4261-4279. doi: 10.3934/math.2023212
[10]	Tongzhu Li, Ruiyang Lin . Classification of Möbius homogeneous curves in $\mathbb{R}^4$ . AIMS Mathematics, 2024, 9(8): 23027-23046. doi: 10.3934/math.20241119

Abstract

1. Introduction

We consider the following family of nonlinear oscillators

$\begin{equation} y_{zz}+k(y)y_{z}^{3}+h(y)y_{z}^{2}+f(y)y_{z}+g(y) = 0, \end{equation}$

(1.1)

where $k$ , $h$ , $f\neq0$ and $g\neq0$ are arbitrary sufficiently smooth functions. Particular members of (1.1) are used for the description of various processes in physics, mechanics and so on and they also appear as invariant reductions of nonlinear partial differential equations ^[1,2,3].

Integrability of (1.1) was studied in a number of works ^{[4,5,6,7,8,9,10,11,12,13,14,15,16]}. In particular, in ^[15] linearization of (1.1) via the following generalized nonlocal transformations

$\begin{equation} w = F(y), \quad d\zeta = (G_{1}(y)y_{z}+G_{2}(y))dz. \end{equation}$

(1.2)

was considered. However, equivalence problems with respect to transformations (1.2) for (1.1) and its integrable nonlinear subcases have not been studied previously. Therefore, in this work we deal with the equivalence problem for (1.1) and its integrable subcase from the Painlevé-Gambier classification. Namely, we construct an equivalence criterion for (1.1) and a non-canonical form of Ince Ⅶ equation ^[17,18]. As a result, we obtain two new integrable subfamilies of (1.1). What is more, we demonstrate that for any equation from (1.1) that satisfy one of these equivalence criteria one can construct an autonomous first integral in the parametric form. Notice that we use Ince Ⅶ equation because it is one of the simplest integrable members of (1.1) with known general solution and known classification of invariant curves.

Moreover, we show that transformations (1.2) preserve autonomous invariant curves for equations from (1.1). Since the considered non-canonical form of Ince Ⅶ equation admits two irreducible polynomial invariant curves, we obtain that any equation from (1.1), which is equivalent to it, also admits two invariant curves. These invariant curves can be used for constructing an integrating factor for equations from (1.1) that are equivalent to Ince Ⅶ equation. If this integrating factor is Darboux one, then the corresponding equation is Liouvillian integrable ^[19]. This demonstrates the connection between nonlocal equivalence approach and Darboux integrability theory and its generalizations, which has been recently discussed for a less general class of nonlocal transformations in ^[20,21,22].

The rest of this work is organized as follows. In the next Section we present an equivalence criterion for (1.1) and a non-canonical form of the Ince Ⅶ equation. In addition, we show how to construct an autonomous first integral for an equation from (1.1) satisfying this equivalence criterion. We also demonstrate that transformations (1.2) preserve autonomous invariant curves for (1.1). In Section 3 we provide two examples of integrable equations from (1.1) and construct their parametric first integrals, invariant curves and integrating factors. In the last Section we briefly discuss and summarize our results.

2. Main results

We begin with the equivalence criterion between (1.1) and a non-canonical form of the Ince Ⅶ equation, that is ^[17,18]

$\begin{equation} w_{\zeta\zeta}+3w_{\zeta}+\epsilon w^{3}+2w = 0. \end{equation}$

(2.1)

Here $\epsilon\neq0$ is an arbitrary parameter, which can be set, without loss of generality, to be equal to $\pm 1$ .

The general solution of (1.1) is

$\begin{equation} w = {\rm e}^{-(\zeta-\zeta_{0})}\rm{cn}\left\{\sqrt{\epsilon}({\rm e}^{-(\zeta-\zeta_{0})}-C_{1}),\frac{1}{\sqrt{2}}\right\}. \end{equation}$

(2.2)

Here $\zeta_{0}$ and $C_{1}$ are arbitrary constants and $\rm{cn}$ is the Jacobian elliptic cosine. Expression (2.2) will be used below for constructing autonomous parametric first integrals for members of (1.1).

The equivalence criterion between (1.1) and (2.1) can be formulated as follows:

Theorem 2.1. Equation (1.1) is equivalent to (2.1) if and only if either

$\begin{equation} \begin{gathered} (I)\quad 25515lgp^{2}q_{y}+2352980l^{10}+\left(3430q-6667920p^{3}\right) l^{5}\\ -14580qp^{3}-10q^{2}-76545lgqpp_{y} = 0, \end{gathered} \end{equation}$

(2.3)

$\begin{equation} \begin{gathered} (II)\quad 343l^{5}-972p^{3} = 0, \end{gathered} \end{equation}$

(2.4)

holds. Here

$\begin{equation} \begin{gathered} l = 9(fg_{y}-gf_{y}+fgh-3kg^{2})-2f^{3}, \quad p = gl_{y}-3lg_{y}+l(f^{2}-3gh),\\ q = 25515g_{y}lp^{2}-5103lgpp_{y}+686l^{5}-8505p^{2}\left( f^{2}-3gh \right) l+6561p^{3}. \end{gathered} \end{equation}$

(2.5)

The expression for $G_{2}$ in each case is either

$\begin{equation} (I) \quad G_{2} = \frac {126 l^{2}qp^{2}}{470596 l^{10}- \left( 1333584 p^{3}+1372 q \right) l^{5}+q^{2}}, \end{equation}$

(2.6)

$\begin{equation} (II) \quad G_{2}^{2} = -\frac{49l^{3}G_{2}+9p^{2}}{189pl}. \end{equation}$

(2.7)

In all cases the functions $F$ and $G_{1}$ are given by

$\begin{equation} F^{2} = \frac{l}{81\epsilon G_{2}^{3}}, \quad G_{1} = \frac{G_{2}(f-3G_{2})}{3g}. \end{equation}$

(2.8)

Proof. We begin with the necessary conditions. Substituting (1.2) into (2.1) we get

$\begin{equation} y_{zz}+k(y)y_{z}^{3}+h(y)y_{z}^{2}+f(y)y_{z}+g(y) = 0, \end{equation}$

(2.9)

where

$\begin{equation} \begin{gathered} k = \frac{FG_{1}^{3}(\epsilon F^{2}+2)+3G_{1}^{2}F_{y}+G_{1}F_{yy}-F_{y}G_{1,y}}{G_{2}F_{y}}, \\ h = \frac{G_{2}F_{yy}+(6G_{1}G_{2}-G_{2,y})F_{y}+3FG_{2}G_{1}^{2}(\epsilon F^{2}+2)}{G_{2}F_{y}},\\ f = \frac{3G_{2}(F_{y}+FG_{1}(\epsilon F^{2}+2))}{F_{y}}, \quad g = \frac{FG_{2}^{2}(\epsilon F^{2}+2)}{F_{y}}. \end{gathered} \end{equation}$

(2.10)

As a consequence, we obtain that (1.1) can be transformed into (2.1) if it is of the form (2.9) (or (1.1)).

Conversely, if the functions $F$ , $G_{1}$ and $G_{2}$ satisfy (2.10) for some values of $k$ , $h$ , $f$ and $g$ , then (1.1) can be mapped into (2.1) via (1.2). Thus, we see that the compatibility conditions for (2.10) as an overdertmined system of equations for $F$ , $G_{1}$ and $G_{2}$ result in the necessary and sufficient conditions for (1.1) to be equivalent to (2.1) via (1.2).

To obtain the compatibility conditions, we simplify system (2.10) as follows. Using the last two equations from (2.10) we find the expression for $G_{1}$ given in (2.8). Then, with the help of this relation, from (2.10) we find that

$\begin{equation} 81\epsilon F^{2}G_{2}^{3}-l = 0, \end{equation}$

(2.11)

and

$\begin{equation} \begin{gathered} 567lG_{2}^{3}+(243lgh-81lf^{2}-81gl_{y}+243lg_{y})G_{2}-7l^{2} = 0,\\ 243lgG_{2,y}+324lG_{2}^{3}-81gl_{y}G_{2}+2l^{2} = 0, \end{gathered} \end{equation}$

(2.12)

Here $l$ is given by (2.5).

As a result, we need to find compatibility conditions only for (2.12). In order to find the generic case of this compatibility conditions, we differentiate the first equation twice and find the expression for $G_{2}^{2}$ and condition (2.3). Differentiating the first equation from (2.12) for the third time, we obtain (2.6). Further differentiation does not lead to any new compatibility conditions. Particular case (2.4) can be treated in the similar way.

Finally, we remark that the cases of $l = 0$ , $p = 0$ and $q = 0$ result in the degeneration of transformations (1.2). This completes the proof.

As an immediate corollary of Theorem 2.1 we get

Corollary 2.1. If coefficients of an equation from (1.1) satisfy either (2.3) or (2.4), then an autonomous first integral of this equation can be presented in the parametric form as follows:

$\begin{equation} y = F^{-1}(w), \quad y_{z} = \frac{G_{2}w_{\zeta}}{F_{y}-G_{1}w_{\zeta}}\,. \end{equation}$

(2.13)

Here $w$ is the general solution of (2.1) given by (2.2). Notice also that, formally, (2.13) contains two arbitrary constants, namely $\zeta_{0}$ and $C_{1}$ . However, without loss of generality, one of them can be set equal to zero.

Now we demonstrate that transformations (1.2) preserve autonomous invariant curves for equations from (1.1).

First, we need to introduce the definition of an invariant curve for (1.1). We recall that Eq (1.1) can be transformed into an equivalent dynamical system

$\begin{equation} y_{z} = P, \quad u_{z} = Q, \quad P = u, \quad Q = -k u^{3}-h u^{2}-f u -g. \end{equation}$

(2.14)

A smooth function $H(y, u)$ is called an invariant curve of (2.14) (or, equivalently, of (1.1)), if it is a nontrivial solution of ^[19]

$\begin{equation} PH_{y}+QH_{u} = \lambda H, \end{equation}$

(2.15)

for some value of the function $\lambda$ , which is called the cofactor of $H$ .

Second, we need to introduce the equation that is equivalent to (1.1) via (1.2). Substituting (1.2) into (1.1) we get

$\begin{equation} w_{\zeta\zeta}+\tilde{k}w_{\zeta}^{3}+\tilde{h}w_{\zeta}^{2}+\tilde{f}w_{\zeta}+\tilde{g} = 0, \end{equation}$

(2.16)

where

$\begin{equation} \begin{gathered} \tilde{k} = \frac{kG_{2}^{3}-gG_{1}^{3}+(G_{1,y}-hG_{1})G_{2}^{2}+(fG_{1}-G_{2,y})G_{1}G_{2}}{F_{y}^{2}G_{2}^{2}},\\ \tilde{h} = \frac{(hF_{y}-F_{yy})G_{2}^{2}-(2fG_{1}-G_{2,y})G_{2}F_{y}+3gG_{1}^{2}F_{y}}{F_{y}^{2}G_{2}^{2}},\\ \tilde{f} = \frac{fG_{2}-3gG_{1}}{G_{2}^{2}}, \quad \tilde{g} = \frac{gF_{y}}{G_{2}^{2}}. \end{gathered} \end{equation}$

(2.17)

An invariant curve for (2.16) can be defined in the same way as that for (1.1). Notice that, further, we will denote $w_{\zeta}$ as $v$ .

Theorem 2.2. Suppose that either (1.1) possess an invariant curve $H(y, u)$ with the cofactor $\lambda(y, u)$ or (2.16) possess an invariant curve $\widetilde{H}(w, v)$ with the cofactor $\tilde{\lambda}(w, v)$ . Then, the other equation also has an invariant curve and the corresponding invariant curves and cofactors are connected via

$\begin{equation} \begin{gathered} H(y,u) = \widetilde{H}\left(F,\frac{F_{y}u}{G_{1}u+G_{2}}\right), \quad \lambda(y,u) = (G_{1}u+G_{2})\tilde{\lambda}\left(F,\frac{F_{y}u}{G_{1}u+G_{2}}\right). \end{gathered} \end{equation}$

(2.18)

Proof. Suppose that $\widetilde{H}(w, v)$ is an invariant curve for (2.16) with the cofactor $\widetilde{\lambda}(w, v)$ . Then it satisfies

$\begin{equation} v\widetilde{H}_{w}+(-\tilde{k} v^{3}-\tilde{h} v^{2}-\tilde{f} v -\tilde{g})\widetilde{H}_{v} = \widetilde{\lambda}\widetilde{H}. \end{equation}$

(2.19)

Substituting (1.2) into (2.19) we get

$\begin{equation} uH_{y}+(-k u^{3}-h u^{2}-f u-g)H = (G_{1}u+G_{2}) \tilde{\lambda}\left(F,\frac{F_{y}u}{G_{1}u+G_{2}}\right) H . \end{equation}$

(2.20)

This completes the proof.

As an immediate consequence of Theorem 2.2 we have that transformations (1.2) preserve autonomous first integrals admitted by members of (1.1), since they are invariant curves with zero cofactors.

Another corollary of Theorem 2.2 is that any equation from (1.1) that is connected to (2.1) admits two invariant curves that correspond to irreducible polynomial invariant curves of (2.1). This invariant curves of (2.1) and the corresponding cofactors are the following (see, ^[23] formulas (3.18) and (3.19) taking into account scaling transformations)

$\begin{equation} \widetilde{H} = \pm i \sqrt{-2\epsilon}(v+w)+w^{2}, \quad \tilde{\lambda} = \pm \sqrt{-2\epsilon}w-2. \end{equation}$

(2.21)

Therefore, we have that the following statement holds:

Corollary 2.2. If coefficients of an equation from (1.1) satisfy either (2.3) or (2.4), then is admits the following invariant curves with the corresponding cofactors

$\begin{equation} H = \pm i \sqrt{-2\epsilon}\left(\frac{F_{y}u}{G_{1}u+G_{2}}+F\right)+F^{2}, \quad \lambda = \left(G_{1}u+G_{2}\right)\left(\pm \sqrt{-2\epsilon}F-2\right). \end{equation}$

(2.22)

Let us remark that connections between (2.1) and non-autonomous variants of (1.1) can be considered via a non-autonomous generalization of transformations (1.2). However, one of two nonlocally related equations should be autonomous since otherwise nonlocal transformations do not map a differential equation into a differential equation ^[5].

In this Section we have obtained the equivalence criterion between (1.1) and (2.1), that defines two new completely integrable subfamilies of (1.1). We have also demonstrated that members of these subfamilies posses an autonomous parametric first integral and two autonomous invariant curves.

3. Examples

In this Section we provide two examples of integrable equations from (1.1) satisfying integrability conditions from Theorem 2.1.

Example 1. One can show that the coefficients of the following cubic oscillator

$\begin{equation} y_{zz}-\frac{12\epsilon \mu y}{(\epsilon \mu^{2}y^{4}+2)^{2}}y_{z}^{3}-6\mu y y_{z}+2\mu^{2}y^{3}(\epsilon\mu^{2}y^{4}+2) = 0, \end{equation}$

(3.1)

satisfy condition (2.3) from Theorem 2.1. Consequently, Eq (3.1) is completely integrable and its general solution can be obtained from (2.2) by inverting transformations (1.2). However, it is more convenient to use Corollary 2.1 and present the autonomous first integral of (3.1) in the parametric form as follows:

$\begin{equation} y = \pm\sqrt{\frac{w}{\mu}}, \quad y_{z} = \frac{w(\epsilon w^{2}+2) w_{\zeta}}{2w_{\zeta}+w(\epsilon w^{2}+2)}, \end{equation}$

(3.2)

where $w$ is given by (2.2), $\zeta$ is considered as a parameter and $\zeta_{0}$ , without loss of generality, can be set equal to zero. As a result, we see that (3.1) is integrable since it has an autonomous first integral.

Moreover, using Corollary 2.2 one can find invariant curves admitted by (3.1)

$\begin{equation} \begin{gathered} H_{1,2} = \frac{y^{4}\left[(\sqrt{2}\pm \sqrt{-\epsilon}\mu y^{2})^{2}(\sqrt{2}\mp\sqrt{-\epsilon}\mu y^{2})+2(\epsilon\mu y^{2}\mp\sqrt{-2\epsilon})u\right]}{2\mu^{2}y^{2}(\epsilon\mu^{2}y^{4}+2)-4u},\\ \lambda_{1,2} = \pm\frac{2(\mu y^{2}(\epsilon\mu^{2}y^{4}+2)-2u)(\sqrt{-2\epsilon}\mu y^{2}\mp 2)}{y(\epsilon\mu^{2}y^{4}+2)} \end{gathered} \end{equation}$

(3.3)

With the help of the standard technique of the Darboux integrability theory ^[19], it is easy to find the corresponding Darboux integrating factor of (3.1)

$\begin{equation} M = \frac{(\epsilon \mu^{2}y^{4}+2)^{\frac{9}{4}}}{(2\epsilon u^{2}+(\epsilon\mu^{2}y^{4}+2)^{2})^{\frac{3}{4}}(\mu y^{2}(\epsilon \mu^{2}y^{4}+2)-2u)^{\frac{3}{2}}}. \end{equation}$

(3.4)

Consequently, equation is (3.1) Liouvillian integrable.

Example 2. Consider the Liénard (1, 9) equation

$\begin{equation} y_{zz}+(b_{i}y^{i}){y_{z}}+a_{j}y^{j} = 0, \quad i = 0,\ldots 4, \quad j = 0,\ldots,9. \end{equation}$

(3.5)

Here summation over repeated indices is assumed. One can show that this equation is equivalent to (2.1) if it is of the form

$\begin{equation} y_{zz}-9(y+\mu)(y+3\mu)^{3}y_{z}+2y(2y+3\mu)(y+3\mu)^{7} = 0, \end{equation}$

(3.6)

where $\mu$ is an arbitrary constant.

With the help of Corollary 2.1 one can present the first integral of (3.6) in the parametric form as follows:

$\begin{equation} y = \frac{3\sqrt{-2\epsilon}\mu w}{2-\sqrt{-2\epsilon}w}, \quad y_{z} = \frac{7776 \sqrt{2} \epsilon \mu^{5} w w_{\zeta}}{(\sqrt{-2\epsilon}w-2)^{5}(2\sqrt{-\epsilon}w_{\zeta}+(\sqrt{2}\epsilon w +2\sqrt{-\epsilon})w)}, \end{equation}$

(3.7)

where $w$ is given by (2.2). Thus, one can see that (3.5) is completely integrable due to the existence of this parametric autonomous first integral.

Using Corollary 2.2 we find two invariant curves of (3.6):

$\begin{equation} H_{1} = \frac{y^{2}[(2y+3\mu)(y+3\mu)^{4}-2u)]}{(y+3\mu)^{2}[(y+3\mu)^{4}y-u]}, \quad \lambda_{1} = \frac{6\mu(u-y(y+3\mu)^{4})}{y(y+3\mu)}, \end{equation}$

(3.8)

and

$\begin{equation} H_{2} = \frac{y^{2}(y+3\mu)^{2}}{y(y+3\mu)^{4}-u}, \quad \lambda_{2} = \frac{2(2y+3\mu)(u-2y(y+3\mu)^{4})}{y(y+3\mu)}. \end{equation}$

(3.9)

The corresponding Darboux integrating factor is

$\begin{equation} M = \left[y(y+3\mu)^{4}-u\right]^{-\frac{3}{2}}\left[(2y+3\mu)(y+3\mu)^{4}-2u\right]^{-\frac{3}{4}}. \end{equation}$

(3.10)

As a consequence, we see that Eq (3.6) is Liouvillian integrable.

Therefore, we see that equations considered in Examples 1 and 2 are completely integrable from two points of view. First, they possess autonomous parametric first integrals. Second, they have Darboux integrating factors.

4. Conclusions and discussion

In this work we have considered the equivalence problem between family of Eqs (1.1) and its integrable member (2.1), with equivalence transformations given by generalized nonlocal transformations (1.2). We construct the corresponding equivalence criterion in the explicit form, which leads to two new integrable subfamilies of (1.1). We have demonstrated that one can explicitly construct a parametric autonomous first integral for each equation that is equivalent to (2.1) via (1.2). We have also shown that transformations (1.2) preserve autonomous invariant curves for (1.1). As a consequence, we have obtained that equations from the obtained integrable subfamilies posses two autonomous invariant curves, which corresponds to the irreducible polynomial invariant curves of (2.1). This fact demonstrate a connection between nonlocal equivalence approach and Darboux and Liouvillian integrability approach. We have illustrate our results by two examples of integrable equations from (1.1).

Acknowledgments

The author was partially supported by Russian Science Foundation grant 19-71-10003.

Conflict of interest

The author declares no conflict of interest in this paper.

References

[1]	Agenor P, Bayraktar N (2008) Contracting models of the phillips curve: Empirical estimates for middle income countries. Centre for Growth and Business Cycle Research Discussion Paper Series No: 094.
[2]	Akcay OC, Alper CE, Ozmucur S (1996) Budget deficit, money supply and inflation: Evidence from low and high frequency data for turkey. Bogazici University Department of Economics Research Papers ISS/EC-1996-12.
[3]	Assenmacher-Wesche K, Gerlach S (2008a) Interpreting euro area inflation at high and low frequencies. Eur Econ Rev 52: 964-986.
[4]	Assenmacher-Wesche K, Gerlach S (2008b) Money growth, output gaps and inflation at low and high frequency: Spectral estimates for switzerland. J Econ Dyn Control 32: 411-435.
[5]	Baser-Andic S, Kucuk H, Ogunc F (2014) Inflation dynamics in turkey: In pursuit of a domestic cost measure. Central Bank of the Republic of Turkey Working Papers No.14/20.
[6]	Bastav L (2015) Turkish inflation dynamics: New keynesian phillips curve (2000-2013). Iktisat Isletme ve Finans 30: 57-80.
[7]	Beck G, Wieland V (2010) Money in monetary policy design: Monetary cross-checking in the newkeynesian model. ECB Working Papers No.1191.
[8]	Bloomfield P (2000) Fourier Analysis of Time Series (1st ed. ed.), John Wiley and Sons.
[9]	Breitung J, Candelon B (2006) Testing for short- and long-run causality: A frequency-domain approach. J Econometrics 132: 363-378. doi: 10.1016/j.jeconom.2005.02.004
[10]	Breitung J, Schreiber S (2018) Assessing causality and delay within a frequency band. Econometrics Stat 6: 57-73. doi: 10.1016/j.ecosta.2017.04.005
[11]	Catik A, Martin C (2008) Relative price variability and the phillips curve: Evidence from turkey. Ege University Working Papers in Economics No:08/07.
[12]	Celasun O (2006) Sticky inflation and the real effects of exchange rate based stabilization. J Int Econ 70: 115-139. doi: 10.1016/j.jinteco.2005.05.014
[13]	Cespedes L, Ochoa M, Soto C (2005) The new keynesian phillips curve in an emerging market economy: The case of chile. Central Bank of Chile Working Paper No:355.
[14]	DeGrauwe P, Polan M (2005) Is inflation always and everywhere a monetary phenomenon? Scand J Econ 107: 239-259. doi: 10.1111/j.1467-9442.2005.00406.x
[15]	ECB (1999) The stability-oriented monetary policy strategy of the eurosystem. ECB Monthly Bulletin January, 39-50.
[16]	ECB (2003) The outcome of the ecb's evaluation of its monetary policy strategy. ECB Monthly Bulletin June, 79-92.
[17]	Eichler M (2007) Granger causality and path diagrams for multivariate time series. J Econometrics 137: 334-353. doi: 10.1016/j.jeconom.2005.06.032
[18]	Enders W, Lee J (2012) The flexible fourier form and dickey-fuller type unit root tests. Econ Lett 117: 196-199. doi: 10.1016/j.econlet.2012.04.081
[19]	Friedman M (1963) Inflation: Causes and Consequences, New York: Asia Publishing House.
[20]	Friedman M, Schwartz AJ (1983) Monetary Trends in the United States and the United Kingdom: Their Relations to Income, Prices, and Interest Rates, University of Chicago Press.
[21]	Gali J, Gertler M (1999) Inflation dynamics: A structural econometrics analysis. J Monetary Econ 44: 195-222. doi: 10.1016/S0304-3932(99)00023-9
[22]	Gal J, Gertler M, Lopez-Salido J (2001) European inflation dynamics. Eur Econ Rev 45: 1237-1270. doi: 10.1016/S0014-2921(00)00105-7
[23]	Gallegati M, Giri F, Fratianni MU (2019) Money growth and inflation: International historical evidence on high inflation episodes for developed countries. Bank Finland Res Discussion Pap 1.
[24]	Gerlach S (2002) The ecb's two pillars. Mimeo.
[25]	Gerlach-Kristen P (2006) A two-pillar phillips curve for switzerland. Deutsche Bundesbank Discussion Paper No 36/2004.
[26]	Geweke J (1982) Measurement of linear dependence and feedback between multiple time series. J Am Stat Assoc 77: 424-438.
[27]	Geweke J (1984) Measures of conditional linear dependence and feedback between time series. J Am Stat Assoc 79: 907-915. doi: 10.1080/01621459.1984.10477110
[28]	Granger C (1969) Investigating causal relations by econometric models and cross-spectral methods. Econometrica 37: 424-438. doi: 10.2307/1912791
[29]	Hosoya Y (1991) The decomposition and measurement of the interdependence between second-order stationary processes. Probab Theory Relat Fields 88: 429-444. doi: 10.1007/BF01192551
[30]	Hosoya Y (2001) Elimination of third-series effect and defining partial measures of causality. J Time Ser Anal 22: 537-554. doi: 10.1111/1467-9892.00240
[31]	Jaeger A (2003) The ecb's money pillar: An assessment. IMF Working Paper WP/03/82.
[32]	Janssen N, Nolan C, Thomas R (2002) Money, debt and prices in the united kingdom, 1705?1996. Economica 69: 461-479.
[33]	Jiang C, Chang T, Li XL (2015) Money growth and inflation in china: New evidence from a wavelet analysis. Int Rev Econ Financ 33: 249-261. doi: 10.1016/j.iref.2014.10.005
[34]	Jordan T, Peytrignet M, Rich G (2001) The role of m3 in the policy analysis of the swiss national bank. Monetary Analysis: Tools and Applications 2001: 47-62.
[35]	Kara H (2015) Interest rate corridor and the monetary policy stance. TCMB Research Notes in Economics, No:2015-13.
[36]	Karaçimen E (2014) Financialization in turkey: The case of consumer debt. J Balkan Near Eastern Stud 16: 161-180. doi: 10.1080/19448953.2014.910393
[37]	Lim CH, Papi L (1997) An econometric analysis of the determinants of inflation in turkey. IMF Working Paper WP/97/10.
[38]	Lucas R (1976) Econometric policy evaluation: a critique. Carnegie-Rochester Conference Series on Public Policy 1: 19-46. doi: 10.1016/S0167-2231(76)80003-6
[39]	Lucas R (1980) Two illustrations of the quantity theory of money. Am Econ Rev 70: 1005-1014.
[40]	McCallum B (1984) On low-frequency estimates of long-run relationships in macroeconomics. J Monetary Econ 14: 3-14. doi: 10.1016/0304-3932(84)90023-0
[41]	Nelson E (2003) The future of monetary aggregates in monetary policy analysis. J Monetary Econ 50: 1029-1059. doi: 10.1016/S0304-3932(03)00063-1
[42]	Neumann M (2003) The european central bank's first pillar reassessed. University of Bonn.
[43]	Neumann M, Greiber C (2004) Inflation and core money growth in the euro area. Deutsche Bundesbank Discussion Paper No 36/2004.
[44]	Phillips A (1958) The relation beteen unemployment and the rate of chage in money wages in the united kingdom, 1861-1957. Economica 25: 283-299.
[45]	Ramos-Francia M, Torres A (2008) Inflation dynamics in mexico: A characterization using the new phillips curve North Am J Econ Financ 19: 274-289.
[46]	Rehman M (2010) Money-inflation relationship: Band spectrum analysis approach. Pak J Appl Econ 20: 67-76.
[47]	Roberts J (1995) New keynesian economics and the phillips curve. J Money Credit Bank 27: 975-984. doi: 10.2307/2077783
[48]	Rua A (2012) Money growth and inflation in the euro area: A time-frequency view. Oxford Bulletin Econ Stat 74: 875-885. doi: 10.1111/j.1468-0084.2011.00680.x
[49]	Samuelson P, Solow R (1960) Analytical aspects of anti-inflation policy. Am Econ Rev 50: 177-194.
[50]	Sasongko G, Huruta AD (2018) Monetary policy and the causality between inflation and money supply in indonesia. Bus Theory Pract 19: 80-87. doi: 10.3846/btp.2018.09
[51]	Saz G (2011) The turkish phillips curve experience and the new keynesian phillips curve: A conceptualization and application of a novel measure for marginal costs. Int J Financ Econ 63.
[52]	Schreiber S (2009) Low-frequency determinants of inflation in the euro area. IMK-Macroeconomic Policy Institute Working paper-6/2009.
[53]	Su CW, Fan JJ, Chang HL, et al. (2016) Is there causal relationship between money supply growth and inflation in china? evidence from quantity theory of money. Rev Dev Econ 20: 702-719.
[54]	Svensson L (2002) Monetary policy in times of low inflation. In: Federal Reserve Bank of Kansas City.
[55]	Tastan H (2015) Testing for spectral granger causality. Stata J 15: 1157-1166. doi: 10.1177/1536867X1501500411
[56]	Taylor J (1993) Discretion versus policy rules in practice. Carnegie-Rochester Conference Series on Public Policy, 195-204. doi: 10.1016/0167-2231(93)90009-L
[57]	Tekin-Koru A, Ozmen E (2003) Budget deficits, money growth and inflation: The turkish evidence. AppL Econ 35: 591-596. doi: 10.1080/0003684022000025440
[58]	Teles P, Uhlig H, Valle e Azevedo J (2016) Is quantity theory still alive? Econ J 126: 442-464. doi: 10.1111/ecoj.12336
[59]	Terzi N (2015) Financial inclusion and turkey. Acad J Interdiscip Stud 4: 269.
[60]	Us V (2004) Inflation dynamics and monetary policy strategy: Some prospects for the turkish economy. J Policy Model 26: 1003-1013. doi: 10.1016/j.jpolmod.2004.07.001
[61]	von Hagen J, Hofmann B (2003) Monetary policy orientation in times of low inflation. Conference on Monetary Policy under Low Inflation, Federal Reserve Bank of Cleveland.
[62]	Whiteman C (1984) Lucas on the quantity theory: hypothesis testing without theory. Am Econ Rev 74: 742-749.
[63]	Yazgan ME, Yilmazkuday H (2005) Inflation dynamics of turkey: A structural estimation. Stud Nonlinear Dyn Econometrics 9: 1-13.
[64]	Yildirim N, Tastan H (2012) Capital flows and economic growth across spectral frequencies: Evidence from turkey. Panoeconomicus 4: 441-462. doi: 10.2298/PAN1204441Y

This article has been cited by:

1.	Dmitry I. Sinelshchikov, Linearizabiliy and Lax representations for cubic autonomous and non-autonomous nonlinear oscillators, 2023, 01672789, 133721, 10.1016/j.physd.2023.133721
2.	Jaume Giné, Xavier Santallusia, Integrability via algebraic changes of variables, 2024, 184, 09600779, 115026, 10.1016/j.chaos.2024.115026
3.	Meryem Belattar, Rachid Cheurfa, Ahmed Bendjeddou, Paulo Santana, A class of nonlinear oscillators with non-autonomous first integrals and algebraic limit cycles, 2023, 14173875, 1, 10.14232/ejqtde.2023.1.50
4.	Jaume Giné, Dmitry Sinelshchikov, Integrability of Oscillators and Transcendental Invariant Curves, 2025, 24, 1575-5460, 10.1007/s12346-024-01182-x

Reader Comments

Your name:*

Email:*
© 2020 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)