This paper shows how gamma oscillations can be combined with neural population models and dynamic causal modeling (DCM) to distinguish among alternative hypotheses regarding cortical excitability and microstructure. This approach exploits inter-subject variability and trial-specific effects associated with modulations in the peak frequency of gamma oscillations. Neural field models are used to evaluate model evidence and obtain parameter estimates using invasive and non-invasive gamma recordings. Our overview comprises two parts: in the first part, we use neural fields to simulate neural activity and distinguish the effects of post synaptic filtering on predicted responses in terms of synaptic rate constants that correspond to different timescales and distinct neurotransmitters. We focus on model predictions of conductance and convolution based field models and show that these can yield spectral responses that are sensitive to biophysical properties of local cortical circuits like synaptic kinetics and filtering; we also consider two different mechanisms for this filtering: a nonlinear mechanism involving specific conductances and a linear convolution of afferent firing rates producing post synaptic potentials. In the second part of this paper, we use neural fields quantitatively—to fit empirical data recorded during visual stimulation. We present two studies of spectral responses obtained from the visual cortex during visual perception experiments: in the first study, MEG data were acquired during a task designed to show how activity in the gamma band is related to visual perception, while in the second study, we exploited high density electrocorticographic (ECoG) data to study the effect of varying stimulus contrast on cortical excitability and gamma peak frequency.
1.
Introduction
The investigation of partially ordered algebraic systems was initiated by Conrad[16,17]. Particularly, an ordered semigroup (also called a partially ordered semigroup or a po-semigroup) is a well-known example of the partially ordered algebraic systems, which is a semigroup together with a compatible partial order. The notion of ideals and filters on ordered semigroups play a significant role in studying the structure of ordered semigroups, especially the decompositions of ordered semigroups see [2,23,34], and there are many applications in various branches of mathematics and computer science. Many mathematicians have studied different aspects of ideals and filters on ordered semigroups. For examples, see [2,22,23,24,25,34].
The theory of algebraic hyperstructures have been originated by Marty[28] in 1934 when he studied hypergroups, which are considered as a generalization of groups, by using hyperoperations. Later on, several kinds of hyperstructures were studied by many mathematicians, for instance, see [4,5,6,7,19,20,26]. In 2011, Davvaz and Heidari[18] applied the notion of ordered semigroups to hyperstructures and presented a new class of hyperstructures called ordered semihypergroups. Such hyperstructure can be considered as an extension and a generalization of ordered semigroups. The relationships between ordered semihypergroups and ordered semigroups were described by Davvaz et al.[11]. Some properties of hyperideals on ordered semihypergroups were investigated by Changphas and Davvaz[3]. Next, Tang et al.[32] introduced the concept of a (right, left) hyperfilter on ordered semihypergroups and discussed their related properties. Omidi and Davvaz[29] generalized the concept of the relation $ \mathcal{N} $ on ordered semigroups into ordered semihypergroups and proved some remarkable results. Note that, in an ordered semihypergroup $ S $, the relation $ \mathcal{N} $ on $ S $ is defined by $ \mathcal{N}: = \left\lbrace (x, y) \in S\times S : N(x) = N(y)\right\rbrace $ where $ N(x) $ is the hyperfilter generated by the element $ x $ of $ S $. Kehayopulu[21] proved that the relation $ \mathcal{N} $ is the least semilattice congruence on semihypergroups. Meanwhile, Kehayopulu illustrate by counterexample that $ \mathcal{N} $ is not the least semilattice congruence on ordered semihypergroups in general. The author introduced the concept of complete semilattice congruence on ordered semihypergroups and proved that $ \mathcal{N} $ is the smallest complete semilattice congruence. Recently, Tang and Davvaz[31] studied the relation $ \mathcal{N} $ and Green's relations, especially the relations $ \mathcal{L, R} $ and $ \mathcal{J} $, on ordered semihypergroups. They showed that every (respectively, right, left) hyperideal can be decomposable into its ($ \mathcal{R} $-, $ \mathcal{L} $-)$ \mathcal{J} $-classes and posed a problem: " Can every prime hyperideal be decomposable into its $ \mathcal{N} $-classes?"
On the other hand, the investigation of algebraic hyperstructures have been widely expanded. In 2006, Davvaz and Vougiouklis[14] applied the notion of hypergroups to $ n $-ary algebras and presented the notion of $ n $-ary hypergroups for $ n \geq 2 $. Leoreanu-Fotea and Davvaz [27] studied some binary relations on $ n $-ary hypergroups. Anvariyeh and Momeni [1] investigated the interesting properties of $ n $-ary relations on $ n $-ary hypergroups. In 2009, Davvaz et al.[13] introduced a new class of hyperstructure called $ n $-ary semihypergroups. This new class can be considered as a natural extension of semigroups, semihypergroups, ternary semigroups, ternary semihypergroups and $ n $-ary semigroups. The interesting results of $ n $-ary semigroups and $ n $-ary semihypergroups, the readers can be referred to [8,12,13,15,30]. Recently, Daengsaen and Leeratanavalee [9] applied the theory of $ n $-ary algebras to ordered semihypergroups and introduced the notion of ordered $ n $-ary semihypergroups for $ n \geq 2 $. Such new notion is a natural generalization of ordered semigroups, ordered semihypergroups, ordered ternary semigroups and ordered ternary semihypergroups. Also, they characterized several kinds of regularities of ordered $ n $-ary semihypergroups. In this paper, we attempt to study the notion of hyperfilters on ordered $ n $-ary semihypergroups. In particular, we introduce the concept of $ j $-hyperfilters, for all positive integers $ 1 \leq j \leq n $ and $ n\geq 2 $, of ordered $ n $-ary semihypergroups and use such concept to construct the relation $ \mathcal{N} $ for studying the properties of semilattice congruence on ordered $ n $-ary semihypergroups where $ n \geq 2 $.
The present paper is organized as follows. In Section 2, we recall some basic notions and elementary results of algebraic hyperstructures. In Section 3, we introduce the concept of $ j $-hyperfilters, which is a generalization of left and right hyperfilters of ordered semihypergroups and discuss the connection between $ j $-hyperfilters and completely prime $ j $-hyperideals on ordered $ n $-ary semihypergroups where $ n \geq 2 $. In Section 4, we investigate some properties of the relation $ \mathcal{N} $ on $ n $-ary semihypergroups. We illustrate by counterexample that $ \mathcal{N} $ does not necessarily to be the least semilattice congruence on $ n $-ary semihypergroup, where $ n\geq 3 $, in general. At the meantime, we provide a sufficient condition that makes the previous result true. In Section 5, we introduce the concept of a complete $ * $-semilattice (strongly) regular relation on ordered $ n $-ary semihypergroups, where $ n \geq 3 $, and prove that $ \mathcal{N} $ is the smallest complete $ * $-semilattice strongly regular relation. In the last section, we give an example to show that a prime hyperideal of ordered $ n $-ary semihypergroups cannot be decomposable into its $ \mathcal{N} $-classes in general. However, we provide some special condition for prime hyperideals that lead to be decomposable into its $ \mathcal{N} $-classes. As an application of our results, the related problem posed by Tang and Davvaz is solved.
2.
Preliminaries
In this section, we recall some elementary results of algebraic hyperstructures. For more detail, we refer to [8,9,12,13,14].
Let $ S $ be a nonempty set and let $ \mathcal{P}^*(S) $ denote the set of all nonempty subsets of $ S $. A mapping $ f:\underbrace{S \times \cdots \times S}_{S {\text{ appears}\; n \geq 2\; \text{times}}} \to \mathcal{P}^*(S) $ is called an $ n $-ary hyperoperation. A structure $ (S, f) $ is called an $ n $-ary hypergroupoid [14]. Throughout this paper, we use the symbol $ a_j^k $ to denote a sequence of elements $ a_j, a_{j+1}, ..., a_k $ of $ S $. For the case $ k < j $, $ a_j^k $ is the empty symbol. Then $ f(a_1, a_2, ..., a_n) = f(a_1^n) $ and
In case $ a_1 = ... = a_j = a $ and $ c_{k+1} = ... = c_n = c $, we write the second expression in the form $ f(a^j, b_{j+1}^k, c^{n-k}) $. For the symbols of a sequence of subsets of $ S $, we denote analogously. For $ X_1, ..., X_n \in \mathcal{P}^*(S) $, we define
If $ X_1 = \{a_1\} $, then we write $ f(\{a_1\}, X_2^n) $ as $ f(a_1, X_2^n) $ and analogously in other cases. In case $ X_1 = ... = X_j = Y $ and $ X_{j+1} = ... = X_n = Z $, we write $ f(X_1^n) $ as $ f(Y^j, Z^{n-j}) $. The hyperoperation $ f $ is associative[13] if
hold for all $ a_1^{2n-1} \in S $ and for all $ 1 < j \leq n $. In this case, the $ n $-ary hypergroupoid $ (S, f) $ is called an $ n $-ary semihypergroup (also called an $ n $-ary hypersemigroup)[13].
An ordered $ n $-ary semihypergroup $ (S, f, \leq) $ (also called a partially ordered $ n $-ary semihypergroup or a po-$ n $-ary semihypergroup)[9] is an $ n $-ary semihypergroup $ (S, f) $ and a partially ordered set $ (S, \leq) $ such that a partial order $ \leq $ is compatible with $ f $. Indeed, for any $ a, b \in S $,
for all $ c_1^n \in S $ and for all $ 1 \leq j \leq n $. Note that, for any $ X, Y \in \mathcal{P}^*(S) $, $ X \preceq Y $ means for every $ x \in X $ there exists $ y \in Y $ such that $ x \leq y $. If $ H $ is an $ n $-ary subsemihypergroup of an ordered $ n $-ary semihypergroup $ (S, f, \leq) $, i.e $ f(H^n) \subseteq H $, then $ (H, f, \leq) $ is an ordered $ n $-ary semihypergroup.
Definition 2.1. [9] Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup. For any positive integer $ 1 \leq j \leq n $ and $ n\geq 2 $, a nonempty subset $ I $ of $ S $ is called a $ j $-hyperideal of $ S $ if it satisfies the following two conditions:
$ \rm(I1) \; I $ is a $ j $-hyperideal of an $ n $-ary semihypergroup $ (S, f) $, i.e., $ f(a_1^{j-1}, b, a_{j+1}^n) \subseteq I $ for all $ b \in I $ and $ a_1^{j-1}, a_{j+1}^n \in S $.
$ \rm(I2) $ If $ b \in I $ and $ c \in S $ such that $ c \leq b $ then $ c \in I $.
If $ I $ is a $ j $-hyperideal, for all $ 1 \leq j\leq n $, then $ I $ is said to be a hyperideal.
Here, $ 1 $-hyperideal ($ n $-hyperideal) is called a right hyperideal (left hyperideal), respectively. Throughout this paper, we let $ S $ stand for an ordered $ n $-ary semihypergroup $ (S, f, \leq) $. By applying Lemma 4 in [8], the following lemma is obtained.
Lemma 2.2. [8] For any positive integer $ 1 \leq j \leq n $, let $ \{I_k : k \in \mathcal{J}\} $ be a collection of $ j $-hyperideals (hyperideals) of $ S $. Then $ \bigcup\limits_{k \in \mathcal{J}}I_k $ is a $ j $-hyperideal (hyperideal) of $ S $ and $ \bigcap\limits_{k \in \mathcal{J}}I_k $ is also a $ j $-hyperideal (hyperideal) of $ S $ if $ \bigcap\limits_{k \in \mathcal{J}}I_k \neq \emptyset $.
A nonempty subset $ P $ of $ S $ is called prime[31] if, for every $ a_1^n \in S $, $ f(a_1^n) \subseteq P $ implies $ a_k \in P $ for some $ 1\leq k \leq n $. Equivalently, if $ a_1^n \notin P $ then $ f(a_1^n) \nsubseteq P $.
$ P $ is called completely prime[32] if, for every $ a_1^n \in S $, $ f(a_1^n) \cap P \neq \emptyset $ implies $ a_k \in P $ for some $ 1 \leq k \leq n $. Equivalently, if $ a_1^n \notin P $ then $ f(a_1^n) \cap P = \emptyset $.
A ($ j $-)hyperideal $ I $ of $ S $ is called a prime ($ j $-)hyperideal (completely prime ($ j $-)hyperideal) of $ S $ if $ I $ is a prime (completely prime) subset of $ S $. Clearly, every completely prime ($ j $-)hyperideal of $ S $ is a prime ($ j $-)hyperideal of $ S $.
Example 2.3. Let $ \mathbb{Z} $ be the set of all integers. Define $ f:\mathbb{Z}^n \to \mathcal{P}^*(\mathbb{Z}) $ by
for all $ x_1^n \in \mathbb{Z} $. Then $ (\mathbb{Z}, f, \leq) $ is an ordered $ n $-ary semihypergroup where $ \leq $ is a usual partial order on $ \mathbb{Z} $. Let $ \mathbb{N} $ be the set of all natural numbers. Then $ (\mathbb{N}, f, \leq) $ is an ordered $ n $-ary subsemihypergroup of $ \mathbb{Z} $.
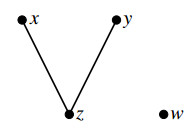
Example 2.4. Let $ S = \{w, x, y, z\} $. Define $ f:S^n \to \mathcal{P}^*(S) $ by $ f(x_1^n) = ((...(x_1 \circ x_2) \circ...) \circ x_n) $, for all $ x_1^n \in S $, where $ \circ $ is defined by the following table.
By applying Example 2 in [31], $ (S, f) $ is an $ n $-ary semihypergroup. Define a partial order $ \leq $ on $ S $ as follows:
Such relation can be presented by the following diagram.
Then $ (S, f, \leq) $ is an ordered $ n $-ary semihypergroup. It is easy to verify that the sets $ A_1 = \{x, z\} $, $ A_2 = \{y, z\} $, $ A_3 = \{w, x, z\} $ and $ A_4 = \{x, y, z\} $ are all proper $ 2 $-hyperideals of $ S $. Then we obtain the results as follows:
(1) $ A_1 $ and $ A_4 $ are not prime since there exists $ w \in S\setminus A_k $ such that $ f(w^n) \subseteq A_k $ for all $ k = 1, 4 $. It follows that $ A_1, A_4 $ are also not completely prime.
(2) $ A_2 $ is prime since $ f(x_1^n) \nsubseteq A_2 $ for all $ x_1^n \in S\setminus A_2 $. But, it is not completely prime since there exists $ w \in S\setminus A_2 $ such that $ f(w^n) \cap A_2 \neq \emptyset $.
(3) $ A_3 $ is completely prime since $ f(x_1^n) \cap A_3 = \emptyset $ for all $ x_1^n \in S\setminus A_3 $. It follows that $ A_3 $ is prime.
3.
Hyperfilters on ordered $ n $-ary semihypergroups
In this section, we introduce the concept of $ j $-hyperfilters on ordered $ n $-ary semihypergroups, which generalizes the notion of right and left hyperfilters on ordered semihypergroups[32], and investigate their related properties.
Definition 3.1. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup. For any positive integer $ 1 \leq j \leq n $ and $ n\geq 2 $, an $ n $-ary subsemihypergroup $ K $ of $ S $ is said to be a $ j $-hyperfilter of $ S $ if it satisfies the following two conditions:
$ \rm(F1) \; K $ is a $ j $-hyperfilter of an $ n $-ary semihypergroup $ (S, f) $, i.e., for all $ a_1^n \in S $, $ f(a_1^n) \cap K \neq \emptyset $ implies $ a_j \in K $.
$ \rm(F2) $ If for any $ a \in K $ and $ b \in S $ such that $ a \leq b $ then $ b \in K $.
If $ K $ is a $ j $-hyperfilter, for all $ 1 \leq j\leq n $, then $ K $ is called a hyperfilter.
Here, $ 1 $-hyperfilter ($ n $-hyperfilter) is said to be a right hyperfilter (left hyperfilter, respectively). For any $ a \in S $ and $ 1 \leq j \leq n $, we denote by $ N^j(a) $($ N(a) $) the $ j $-hyperfilter (hyperfilter) of $ S $ generated by $ a $ and called the principal $ j $-hyperfilter (hyperfilter) generated by $ a $. A $ j $-hyperfilter (hyperfilter) $ K $ of $ S $ is said to be proper if $ K \neq S $. Clearly, Definition 3.1 coincides with Definition 3.1 given in [32] if $ n = 2 $.
Example 3.2. Let $ S = \{w, x, y, z \} $. Define $ f:S^n \to \mathcal{P}^*(S) $ by $ f(x_1^n) = ((...(x_1 \circ x_2) \circ...) \circ x_n) $, for all $ x_1^n \in S $, where $ \circ $ is defined by the following table
and a partial order $ \leq $ is defined as follows:
Such relation can be presented by the following diagram.
By applying Example 3.3 in [33], $ (S, f, \leq) $ is an ordered $ n $-ary semihypergroup. Clearly, the sets $ \{w, x\} $ and $ S $ are all $ 1 $-hyperfilters of $ S $.
Proposition 3.3. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n \geq 2 $. For any $ 1 \leq j \leq n $, let $ K_1, K_2 $ be two $ j $-hyperfilters of $ S $. Then $ K_1 \cap K_2 $ is a $ j $-hyperfilter of $ S $ if $ K_1 \cap K_2 \neq \emptyset $.
Proof. Let $ a_1^n \in K_1 \cap K_2 $. Then $ a_1^n \in K_i $ for all $ i = 1, 2 $. Since $ K_i $ is an $ n $-ary subsemihypergroup, we obtain $ f(a_1^n) \subseteq K_1 \cap K_2 $. Thus $ K_1 \cap K_2 $ is an $ n $-ary semihypergroup of $ S $. Next, let $ b_1^n \in S $ and $ c \in f(b_1^n) \cap (K_1 \cap K_2) $. Then $ c \in f(b_1^n) \cap K_i $ for all $ i = 1, 2 $. Since $ K_i $ is a $ j $-hyperfilter of $ S $, we have $ b_j \in K_i $ for all $ i = 1, 2 $. So $ b_j \in K_1 \cap K_2 $. Finally, let $ a \in K_1 \cap K_2 $ such that $ a \leq b \in S $. Since $ K_i $ is a $ j $-hyperfilter, we obtain $ b \in K_i $ for all $ i = 1, 2 $. So $ b \in K_1 \cap K_2 $. Therefore $ K_1 \cap K_2 $ is a $ j $-hyperfilter of $ S $.
By Proposition 3.3, we obtain the following result.
Corollary 3.4. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n \geq 2 $ and $ K_1, K_2 $ be two hyperfilters of $ S $. $ K_1 \cap K_2 $ is a hyperfilter of $ S $ if $ K_1 \cap K_2 \neq \emptyset $.
Proposition 3.3 and Corollary 3.4 show that the intersection of finitely many $ j $-hyperfilters(hyperfilters) of $ S $ is always a $ j $-hyperfilter(hyperfilter) if their intersection is nonempty. In 2015, Tang et al.[32] showed that the union of two hyperfilters of an ordered semihypergroup $ H $ (for a special case $ n = 2 $) is not necessarily a hyperfilter of $ H $ (see Example 3.5 in [32]). Using the idea of Lemma 3.6 in [32], we obtain the results as follows.
Proposition 3.5. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n \geq 3 $. For any $ 1 < j < n $, let $ K_1, K_2 $ be two $ j $-hyperfilters of $ S $. Then $ K_1 \cup K_2 $ is a $ j $-hyperfilter of $ S $ if and only if $ K_1 \subseteq K_2 $ or $ K_2 \subseteq K_1 $.
Proof. Now, let $ j $ be a fixed positive integer with $ 1 < j < n $ and $ n \geq 3 $. Let $ K_1 \cup K_2 $ be a $ j $-hyperfilter of $ S $. Assume that $ K_1 \nsubseteq K_2 $ and $ K_2 \nsubseteq K_1 $. There are $ a, b \in K_1 \cup K_2 $ such that $ a \in K_1, a \notin K_2, b \notin K_1 $ and $ b \in K_2 $. Since $ K_1 \cup K_2 $ is an $ n $-ary subsemihypergroup, we have $ f(a^{j-1}, b^{n-j+1}) \subseteq K_1 \cup K_2 $. If $ f(a^{j-1}, b^{n-j+1}) \cap K_1 \neq \emptyset $ then, since $ K_1 $ is a $ j $-hyperfilter of $ S $, we obtain $ b \in K_1 $. It is impossible. So $ f(a^{j-1}, b^{n-j+1}) \cap K_1 = \emptyset $. This implies that $ f(a^{j-1}, b^{n-j+1}) \subseteq K_2 $. Since $ K_2 $ is an $ n $-ary subsemihypergroup, we obtain $ f(b^{j-1}, f(a^{j-1}, b^{n-j+1}), b^{n-j}) \subseteq K_2 $. Then
Since $ K_2 $ is a $ j $-hyperfilter, we obtain $ a \in K_2 $. It is impossible. Thus $ K_1 \subseteq K_2 $ or $ K_2 \subseteq K_1 $. Conversely, if $ K_1 \subseteq K_2 $ or $ K_2 \subseteq K_1 $ then $ K_1 \cup K_2 $ is a $ j $-hyperfilter of $ S $.
Corollary 3.6. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n \geq 2 $ and $ K_1, K_2 $ be two hyperfilters of $ S $. Then $ K_1 \cup K_2 $ is a hyperfilter of $ S $ if and only if $ K_1 \subseteq K_2 $ or $ K_2 \subseteq K_1 $.
Theorem 3.7. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n \geq 2 $ and $ K \in \mathcal{P}^*(S) $. For any $ 1 \leq j\leq n $, the following assertions are equivalent.
$ \rm(i) \; K $ is a $ j $-hyperfilter of $ S $.
$ \rm(ii) \; S\setminus K = \emptyset $ or $ S\setminus K $ is a completely prime $ j $-hyperideal of $ S $.
Proof. (i) $ \Longrightarrow $ (ii) Let $ K $ be a $ j $-hyperfilter of $ S $. Suppose that $ S\setminus K \neq \emptyset $. We show that $ S\setminus K $ is a completely prime $ j $-hyperideal of $ S $. First of all, we show that $ K $ is a $ j $-hyperideal of $ S $. Let $ a \in S\setminus K $ and $ b_1^{j-1}, b_{j+1}^n \in S $. If $ f(b_1^{j-1}, a, b_{j+1}^n) \cap K \neq \emptyset $ then, since $ K $ is a $ j $-hyperfilter, we get $ a \in K $. It is impossible. So $ f(b_1^{j-1}, a, b_{j+1}^n) \cap K = \emptyset $. It implies that $ f(b_1^{j-1}, a, b_{j+1}^n) \subseteq S\setminus K $. Next, let $ x\in S $ and $ y \in S\setminus K $ such that $ x \leq y $. If $ x \notin S\setminus K $ then $ x \in K $. By Definition 3.1 (F2), we have $ y \in K $. It is impossible. Hence $ x \in S\setminus K $. Thus $ S\setminus K $ is a $ j $-hyperideal of $ S $. Next, we show that $ S\setminus K $ is completely prime. Let $ a_1^n \in S $ such that $ f(a_1^n) \cap (S\setminus K) \neq \emptyset $. Assume that $ a_i \notin S\setminus K $ for all $ i = 1, ..., n $. Then $ a_i \in K $ for all $ i = 1, ..., n $. Since $ K $ is an $ n $-ary subsemihypergroup, we obtain $ f(a_1^n)\subseteq K $. It implies that $ f(a_1^n) \cap (S\setminus K) = \emptyset $, which is impossible. Hence $ a_i \in S\setminus K $ for some $ i = 1, ..., n $. Thus $ S\setminus K $ is a completely prime $ j $-hyperideal of $ S $.
(ii) $ \Longrightarrow $ (i) If $ S\setminus K = \emptyset $ then $ K = S $ is a $ j $-hyperfilter of $ S $. Now, suppose that $ S\setminus K $ is a completely prime $ j $-hyperideal of $ S $. We will show that $ K $ is a $ j $-hyperfilter of $ S $. First of all, let $ a_1^n \in K $. If $ f(a_1^n) \nsubseteq K $ then $ f(a_1^n) \cap (S\setminus K) \neq \emptyset $. Since $ S\setminus K $ is completely prime, we obtain $ a_i \in S\setminus K $ for some $ i = 1, ..., n $. It is impossible. Thus $ f(a_1^n) \subseteq K $. Next, let $ b_1^n \in S $ and $ f(b_1^n) \cap K \neq \emptyset $. If $ b_j \notin K $ then $ b_j \in S\setminus K $. Since $ S\setminus K $ is a $ j $-hyperideal, we obtain $ f(b_1^n) \subseteq f(S^{j-1}, b_j, S^{n-j})\subseteq f(S^{j-1}, (S\setminus K), S^{n-j}) \subseteq S\setminus K $. This is impossible. So $ b_j \in K $. Finally, let $ x \in K $ and $ y \in S $ such that $ x \leq y $. If $ y \notin K $ then $ y \in S\setminus K $. Since $ S\setminus K $ is a $ j $-hyperideal, by Definition 2.1 (I2), we get $ x \in S \setminus K $. It is impossible. So $ y \in K $. Therefore $ K $ is a $ j $-hyperfilter of $ S $.
Using the similar proof of the previous theorem, we obtain the result as follows.
Corollary 3.8. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n \geq 2 $ and $ K \in \mathcal{P}^*(S) $. Then the following assertions are equivalent.
$ \rm(i) \; K $ is a hyperfilter of $ S $.
$ \rm(ii) \; S\setminus K = \emptyset $ or $ S\setminus K $ is a completely prime hyperideal of $ S $.
Example 3.9. From the ordered $ n $-ary semihypergroup $ (S, f, \leq) $ given in Example 3.2, we know that the set $ \{w, x\} $ is a proper $ 1 $-hyperfilter of $ S $. By Theorem 3.7, we have $ \{y, z\} = S\setminus \{w, x\} $ is a completely prime $ 1 $-hyperideal of $ S $. In fact, one can verify that a proper completely prime $ 1 $-hyperideal of $ S $ is $ \{y, z\} $ since $ f(x_1^n) \cap \{y, z\} = \emptyset $ for all $ x_1^n \in S\setminus \{y, z\} $.
Lemma 3.10. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n \geq 2 $ and $ a, b \in S $. For any $ 1 \leq j \leq n $, the following assertions hold:
$ \rm(i) $ If $ b \in N^j(a) $ then $ N^j(b) \subseteq N^j(a) $.
$ \rm(ii) $ If $ a \leq b $ then $ N^j(b) \subseteq N^j(a) $.
$ \rm(iii) $ If $ a \leq b $ then $ N^j(a) \setminus N^j(b) $ is a completely prime $ j $-hyperideal of $ N^j(a) $ where $ N^j(a) \setminus N^j(b) \neq \emptyset $.
Proof. (i) Let $ b \in N^j(a) $. Since $ N^j(b) $ is the smallest $ j $-hyperfilter of $ S $ containing $ b $, we have $ N^j(b) \subseteq N^j(a) $.
(ii) Let $ a \leq b $. Since $ N^j(a) $ is a $ j $-hyperfilter of $ S $ and $ a \in N^j(a) $, by Definition 3.1 (F2), we obtain $ b \in N^j(a) $. By (i), we have $ N^j(b) \subseteq N^j(a) $.
(iii) Let $ a \leq b $. Then $ N^j(b) \subseteq N^j(a) $. By Theorem 3.7, we have $ N^j(a) \setminus N^j(b) = \emptyset $ or $ N^j(a) \setminus N^j(b) $ is a completely prime $ j $-hyperideal of $ N^j(a) $. Thus $ N^j(a) \setminus N^j(b) $ is a completely prime $ j $-hyperideal of $ N^j(a) $ where $ N^j(a) \setminus N^j(b)\neq \emptyset $.
Remark 3.11. Lemma 3.10 is still true if the principal $ j $-hyperfilters $ N^j(a) $ and $ N^j(b) $ are replaced by the principal hyperfilters $ N(a) $ and $ N(b) $, respectively. Moreover, the results on this section also hold on $ n $-ary semihypergroups $ (S, f) $.
4.
Semilattice strongly regular relations on $ n $-ary semihypergroups
In this section, we introduce the concept of semilattice (strongly) regular relations on $ n $-ary semihypergroups and investigate their related properties.
Let $ (S, f) $ be an $ n $-ary semihypergroup with $ n\geq 2 $ and $ \rho $ be a relation on $ S $. For any two elements $ A, B \in \mathcal{P}^*(S) $, we write
$ A \bar{\rho} B $ if and only if for any $ a \in A $ there exists $ b \in B $ such that $ a \rho b $ and for all $ b^\prime \in B $ there exists $ a^\prime \in A $ such that $ a^\prime \rho b^\prime $,
$ A \bar{\bar{\rho}} B $ if and only if $ a \rho b $ for all $ a \in A $ and $ b \in B $, see [10].
For any $ 1\leq j\leq n $, an equivalence relation $ \rho $ is called $ j $-regular relation (strongly $ j $-regular relation) if, for every $ a, b, x_1^n \in S $,
Here, $ \rho $ is said to be a regular relation (strongly regular relation) if it is a $ j $-regular relation (strongly $ j $-regular relation) for all $ 1\leq j \leq n $.
Lemma 4.1. Let $ (S, f) $ be an $ n $-ary semihypergroup with $ n\geq 2 $. For any $ A_1^n, B_1^n \in \mathcal{P}^*(S) $, the following statements hold:
$ \rm(i) $ If $ \rho $ is regular and $ A_k \bar{\rho} B_k $, for all $ k = 1, ..., n $, then $ f(A_1^n) \bar{\rho} f(A_1^n) $.
$ \rm(ii) $ If $ \rho $ is strongly regular and $ A_k \bar{\bar{\rho}} B_k $, for all $ k = 1, ..., n $, then $ f(A_1^n) \bar{\bar{\rho}} f(A_1^n) $.
Proof. The proof is straightforward.
Definition 4.2. Let $ (S, f) $ be an $ n $-ary semihypergroup with $ n\geq 2 $. A regular relation (strongly regular relation) $ \rho $ on $ S $ is said to be a semilattice regular relation (semilattice strongly regular relation) if
for all $ x, x_1^n \in S $ and for all $ \alpha \in S_n $ where $ S_n $ is the set of all permutations on $ \{1, 2, ..., n\} $.
Note that any semilattice strongly regular relation, semilattice equivalence relation[29] and semilattice congruence[21] on semihypergroups (for case $ n = 2 $) are the same.
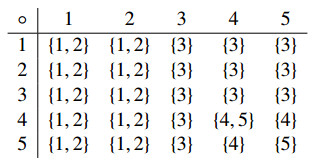
Example 4.3. Let $ S = \{1, 2, 3, 4, 5 \} $. Define $ f:S\times S\times S \to \mathcal{P}^*(S) $ by $ f(x_1, x_2, x_3) = (x_1 \circ x_2) \circ x_3 $, for all $ x_1, x_2, x_3 \in S $, where $ \circ $ is defined by the following table.
By applying Example 3.2 in [32], $ (S, f) $ is a ternary semihypergroup (an $ 3 $-ary semihypergroup). Let
It is easy to show that $ \sigma_1, \sigma_2 $ are equivalence relations on $ S $. Furthermore, one can verify that $ \sigma_2 $ is a semilattice strongly regular relation on $ S $. On the other hand, $ \sigma_1 $ is not a semilattice strongly regular relation on $ S $ because we have $ f(4, 4, 4) = \{4, 5\} $ but $ (4, 5) \notin \sigma_1 $, so $ \sigma_1 $ does not satisfy with the condition $ 4\ \overline{\overline{\sigma_1}} f(4, 4, 4) $ in Definition 4.2.
For any $ K\in \mathcal{P}^*(S) $, we write $ \delta_K : = \left\lbrace (a, b) \in S \times S : a, b \in K\ \text{ or}\ a, b \notin K\right\rbrace. $ Clearly, $ \delta_K $ is an equivalence relation on $ S $.
Theorem 4.4. Let $ (S, f) $ be an $ n $-ary semihypergroup with $ n\geq 2 $ and $ K $ be a completely prime hyperideal of $ S $. Then $ \delta_K $ is a semilattice strongly regular relation on $ S $.
Proof. Let $ K $ be a completely prime hyperideal of $ S $.
(1) We show that $ \delta_K $ is a strongly regular relation. Firstly, let $ j $ be a fixed positive integer with $ 1 \leq j \leq n $. Let $ a, b, x_1^{j-1}, x_{j+1}^n \in S $ such that $ a \delta_K b $. To show that $ f(x_1^{j-1}, a, x_{j+1}^n) \overline{\overline{\delta_K}} f(x_1^{j-1}, b, x_{j+1}^n) $, let $ u \in f(x_1^{j-1}, a, x_{j+1}^n) $ and $ v \in f(x_1^{j-1}, b, x_{j+1}^n) $. Since $ a \delta_K b $, we have $ a, b \in K $ or $ a, b \notin K $.
Case 1.1: $ a, b \in K $. Since $ K $ is a $ j $-hyperideal, we get $ u \in f(x_1^{j-1}, a, x_{j+1}^n) \subseteq K $ and $ v \in f(x_1^{j-1}, b, x_{j+1}^n) \subseteq K $. So $ u \delta_K v $.
Case 1.2: $ a, b \notin K $. Then we have 2 subcases to be considered as follows.
Case 1.2.1: There exists $ x_i \in K $ for some $ i = 1, ..., j-1, j+1, ..., n $. Since $ K $ is an $ i $-hyperideal, we obtain $ u \in f(x_1^{j-1}, a, x_{j+1}^n) \subseteq K $ and $ v \in f(x_1^{j-1}, b, x_{j+1}^n) \subseteq K $. So $ u \delta_K v $.
Case 1.2.2: $ x_i \notin K $ for all $ i = 1, ..., j-1, j+1, ..., n $. Then $ f(x_1^{j-1}, a, x_{j+1}^n) \cap K = \emptyset $ and $ f(x_1^{j-1}, b, x_{j+1}^n) \cap K = \emptyset $. In fact, if $ f(x_1^{j-1}, a, x_{j+1}^n) \cap K \neq \emptyset $ then, since $ K $ is completely prime, we obtain $ x_i \in K $ for some $ i = 1, ..., n $ (we write $ x_i = a $ if $ i = j $), which is a contradiction. Similarly, if $ f(x_1^{j-1}, b, x_{j+1}^n) \cap K \neq \emptyset $ then we also get a contradiction. Thus $ f(x_1^{j-1}, a, x_{j+1}^n) \cap K = \emptyset $ and $ f(x_1^{j-1}, b, x_{j+1}^n) \cap K = \emptyset $. Hence $ u \in f(x_1^{j-1}, a, x_{j+1}^n) \subseteq S\setminus K $ and $ v \in f(x_1^{j-1}, b, x_{j+1}^n) \subseteq S\setminus K $. It follows that $ u\delta_K v $. From Case 1.1 and 1.2, we conclude that $ f(x_1^{j-1}, a, x_{j+1}^n) \overline{\overline{\delta_K}} f(x_1^{j-1}, b, x_{j+1}^n) $ and so $ \delta_K $ is a strongly $ j $-regular relation. For arbitrary positive integer $ j $, we obtain $ \delta_K $ is a strongly regular relation.
(2) We show that $ \delta_K $ is semilattice. Let $ a, a_1^n \in S $ and $ \alpha \in S_n $. Firstly, we show that $ a \overline{\overline{\delta_K}} f(a^n) $. Let $ u \in f(a^n) $. We consider two cases as follows.
Case 2.1: $ a \in K $. Since $ K $ is a hyperideal of $ S $, we have $ u \in f(a^n) \subseteq K $. So $ a \delta_K u $.
Case 2.2: $ a \notin K $. Then $ f(a^n) \cap K = \emptyset $. In fact, if $ f(a^n) \cap K \neq \emptyset $ then, since $ K $ is completely prime, we have $ a \in K $. It is impossible. So $ f(a^n) \cap K = \emptyset $. It follows $ u \notin K $. So $ a \delta_K u $. From Cases 2.1 and 2.2, we conclude that $ a \overline{\overline{\delta_K}} f(a^n) $. Next, we show that $ f(a_1^n) \overline{\overline{\delta_K}} f(a_{\alpha(1)}^{\alpha(n)}) $. Let $ u \in f(a_1^n) $ and $ v \in f(a_{\alpha(1)}^{\alpha(n)}) $. We consider two cases as follows.
Case 1: $ f(a_1^n) \cap K \neq \emptyset $. Since $ K $ is completely prime, we obtain $ a_i \in K $ for some $ i = 1, ..., n $. Since $ \alpha $ is bijective, there is $ i^\prime \in \{1, ..., n\} $ such that $ \alpha(i^\prime) = i $. Since $ K $ is an $ i^\prime $-hyperideal and an $ i $-hyperideal, we obtain $ f(a_{\alpha(1)}^{\alpha(n)}) = f(a_{\alpha(1)}, ..., a_{\alpha(i^\prime)}, ...., a_{\alpha(n)}) \subseteq K $ and $ f(a_1^n) = f(a_1, ..., a_i, ..., a_n) \subseteq K $. Thus $ u, v \in K $ and it follows that $ u\delta_Kv $.
Case 2: $ f(a_1^n) \cap K = \emptyset $. Then $ f(a_{\alpha(1)}^{\alpha(n)})\cap K = \emptyset $. Indeed, if $ f(a_{\alpha(1)}^{\alpha(n)})\cap K \neq \emptyset $ then, since $ K $ is completely prime, we have $ a_{\alpha(i^\prime)} \in K $ for some $ i^\prime \in \{1, ..., n\} $. We set $ i = \alpha(i^\prime) $. Since $ K $ is an $ i $-hyperideal, we obtain $ f(a_1^n) = f(a_1, ..., a_i, ..., a_n) \subseteq K $. It is impossible. Thus $ f(a_{\alpha(1)}^{\alpha(n)})\cap K = \emptyset $. Hence $ u \in f(a_1^n) \subseteq S\setminus K $ and $ v \in f(a_{\alpha(1)}^{\alpha(n)})\subseteq S\setminus K $ and it follows that $ u \delta_K v $. From Cases 1 and 2, we conclude that $ f(a_1^n) \overline{\overline{\delta_K}} f(a_{\alpha(1)}^{\alpha(n)}) $ and the proof is complete.
For any positive integer $ 1\leq j\leq n $ and $ n\geq 2 $, let $ \mathcal{N}_j $ ($ \mathcal{N} $) denote the relation on an (ordered) $ n $-ary semihypergroup $ S $ which is defined by
Clearly, $ \mathcal{N}_j $ and $ \mathcal{N} $ are equivalence relations on $ S $. For any $ a \in S $, we denote by $ (a)_{\mathcal{N}_j} $ the $ \mathcal{N}_j $-class containing $ a $ and the set $ S/\mathcal{N}_j: = \left\lbrace (a)_{\mathcal{N}_j} : a \in S \right\rbrace $. For the relation $ \mathcal{N} $, we define analogously.
Theorem 4.5. Let $ (S, f) $ be an $ n $-ary semihypergroup with $ n\geq 2 $. Then the following statements hold:
$ \rm(i) \; \mathcal{N} $ is a semilattice strongly regular relation.
$ \rm(ii) \; \mathcal{N} = \bigcap \left\lbrace \delta_K : K\in \mathcal{CPI(S)} \right\rbrace, $
where $ \mathcal{CPI(S)} $ is the set of all completely prime hyperideals of $ S $.
Proof. (i) First of all, we show that $ \mathcal{N} $ is a strongly regular relation on $ S $. Let $ a, b, x_1^n \in S $ such that $ a \mathcal{N} b $. Then $ N(a) = N(b) $. For any fixed positive integer $ j $ with $ 1 \leq j \leq n $, let $ u \in f(x_1^{j-1}, a, x_{j+1}^n) $ and $ v \in f(x_1^{j-1}, b, x_{j+1}^n) $. Since $ f(x_1^{j-1}, a, x_{j+1}^n) \cap N(u) \neq \emptyset $ and $ N(u) $ is a hyperfilter, by Definition 3.1 (F1), we obtain $ a, x_1^{j-1}, x_{j+1}^n \in N(u) $. By Lemma 3.10, we obtain $ N(a) \subseteq N(u) $. Since $ b \in N(b) = N(a) $, we get $ b \in N(u) $. Since $ b, x_1^{j-1}, x_{j+1}^n \in N(u) $ and $ N(u) $ is an $ n $-ary subsemihypergroup, we obtain $ v \in f(x_1^{j-1}, b, x_{j+1}^n) \subseteq N(u) $. By Lemma 3.10, we have $ N(v) \subseteq N(u) $. Using the similar previous process, we also obtain that $ N(u) \subseteq N(v) $. So $ N(u) = N(v) $ and it follows that $ u \mathcal{N} v $. Hence $ f(x_1^{j-1}, a, x_{j+1}^n) \overline{\overline{\mathcal{N}}} f(x_1^{j-1}, b, x_{j+1}^n) $. So $ \mathcal{N} $ is a strongly regular relation on $ S $. Next, we show that $ \mathcal{N} $ is a semilattice strongly regular relation on $ S $. Let $ a, a_1^n \in S $ and $ \alpha \in S_n $. To show that $ a \overline{\overline{\mathcal{N}}} f(a^n) $, let $ u \in f(a^n) $. Then $ f(a^n) \cap N(u) \neq \emptyset $. By Definition 3.1 (F1), we obtain $ a \in N(u) $. By Lemma 3.10, we get $ N(a) \subseteq N(u) $. Since $ N(a) $ is an $ n $-ary subsemihypergroup and $ a \in N(a) $, we obtain $ u \in f(a^n) \subseteq N(a) $. By Lemma 3.10, we have $ N(u) \subseteq N(a) $. So $ N(a) = N(u) $ and it implies that $ a \overline{\overline{\mathcal{N}}} f(a^n) $. Finally, we show that $ f(a_1^n) \overline{\overline{\mathcal{N}}} f(a_{\alpha(1)}^{\alpha(n)}) $. Let $ u \in f(a_1^n) $ and $ v \in f(a_{\alpha(1)}^{\alpha(n)}) $. Since $ f(a_1^n) \cap N(u) \neq \emptyset $, by Definition 3.1 (F1), we obtain $ a_1^n \in N(u) $. It follows that $ v \in f(a_1^n) \subseteq N(u) $ and so $ N(v) \subseteq N(u) $. Conversely, since $ f(a_{\alpha(1)}^{\alpha(n)}) \cap N(v) \neq \emptyset $, by using the same process, we have $ N(u) \subseteq N(v) $. So $ N(u) = N(v) $ and it follows that $ u \mathcal{N} v $. Hence $ f(a_1^n) \overline{\overline{\mathcal{N}}} f(a_{\alpha(1)}^{\alpha(n)}) $. Thus $ \mathcal{N} $ is a semilattice strongly regular relation on $ S $.
(ii) Firstly, we will show that $ \mathcal{N} \subseteq \bigcap\left\lbrace \delta_K : K \in \mathcal{CPI(S)} \right\rbrace $. Let $ (x, y) \in \mathcal{N} $. Then $ N(x) = N(y) $. Assume that $ (x, y) \notin \delta_K $ for some $ K \in \mathcal{CPI(S)} $. Then there exist two cases:
Case 1.1: $ x \in K $ and $ y \notin K $. Then $ y \in S\setminus K \subseteq S $. Since $ S\setminus(S\setminus K) = K $ is completely prime, by Corollary 3.8, we have $ S\setminus K $ is a hyperfilter of $ S $. Since $ y \in S\setminus K $, by Lemma 3.10, we obtain $ x\in N(x) = N(y) \subseteq S\setminus K $. It is impossible.
Case 1.2: $ x \notin K $ and $ y \in K $. Using the similar proof as in Case 1.1, we also get a contradiction. From Cases 1.1 and 1.2, we conclude that $ (x, y) \in \delta_K $ for all $ K\in \mathcal{CPI(S)} $. Thus $ \mathcal{N} \subseteq \bigcap \left\lbrace \delta_K : K\in \mathcal{CPI(S)} \right\rbrace $. Conversely, let $ (x, y)\in \delta_K $ for all $ K \in \mathcal{CPI(S)} $. Assume that $ (x, y) \notin \mathcal{N} $. Then there exist 2 cases, i.e. $ x \notin N(y) $ or $ y \notin N(x) $. Indeed, if $ x \in N(y) $ and $ y \in N(x) $ then, by Lemma 3.10, we get $ N(x) \subseteq N(y) $ and $ N(y)\subseteq N(x) $. Consequently, $ N(x) = N(y) $ and so $ (x, y) \in \mathcal{N} $. This is a contradiction. Thus $ x \notin N(y) $ or $ y \notin N(x) $.
Case 2.1: $ x \notin N(y) $. Then $ x \in S\setminus N(y) $. Since $ N(y) $ is a hyperfilter and $ S\setminus N(y) \neq \emptyset $, by Corollary 3.8, we have $ S\setminus N(y) $ is a completely prime hyperideal. Since $ x \in S\setminus N(y) $ and $ y\notin S\setminus N(y) $, it follows that $ (x, y) \notin \delta_{S\setminus N(y)} $. This is a contradiction.
Case 2.2: $ y \notin N(x) $. Using the similar proof as in Case 2.1, we get a contradiction. Hence $ (x, y) \in \mathcal{N} $. Therefore $ \bigcap \left\lbrace \delta_K : K\in \mathcal{CPI(S)} \right\rbrace \subseteq \mathcal{N} $ and the proof is complete.
As we have known from [21] that the relation $ \mathcal{N} $ is the smallest semilattice strongly regular relation on semihypergroups. However, we illustrate by the following example that the similar result in $ n $-ary semihypergroups, for $ n\geq 3 $, is not necessary true.
Example 4.6. Let $ S = \{1, -1, i, -i\} $. Then $ (S, \cdot) $ is a commutative semigroup under the usual multiplication $ \cdot $ over complex numbers. Define $ f:S\times S\times S \to \mathcal{P^*}(S) $ by $ f(x, y, z) = \{x\cdot y\cdot z\} $. Then $ (S, f) $ is a ternary semihypergroup. Clearly, $ \mathcal{CPI(S)} = \{S\} $. In fact, suppose that $ A $ is an arbitrary hyperideal of $ S $.
If $ 1 \in A $ then $ S = f(S, 1, 1) \subseteq f(S, S, 1) \subseteq A $.
If $ -1 \in A $ then $ S = f(S, -1, -1) \subseteq f(S, S, -1) \subseteq A $.
If $ i \in A $ then $ S = f(S, -i, i) \subseteq f(S, S, i) \subseteq A $.
If $ -i \in A $ then $ S = f(S, i, -i) \subseteq f(S, S, -i) \subseteq A $.
Consequently, $ S = A $. By Theorem 4.5(ii), we have
$ \mathcal{N} = \delta_S = \{(1, 1), (1, -1), (1, i), (1, -i), (-1, 1), (-1, -1), (-1, i), (-1, -i), $
$ (i, 1), (i, -1), (i, i), (i, -i), (-i, 1), (-i, -1), (-i, i), (-i, -i)\}. $
Next, let
It is not difficult to show that $ \rho $ is a strongly regular relation on $ S $. Furthermore, we have
Since $ (S, \cdot) $ is commutative, we get $ f(x_1^3) = f(x_{\alpha(1)}^{\alpha(3)}) $ and so $ f(x_1^3)\ \bar{\bar{\rho}} f(x_{\alpha(1)}^{\alpha(3)}) $ for all $ \alpha \in S_3 $. By Definition 4.2, we conclude that $ \rho $ is a semilattice strongly regular relation on $ S $ and $ \rho \subseteq \mathcal{N} $.
By the previous example, we observe that the relation $ \mathcal{N} $ is not necessary the smallest semilattice strongly regular relation on $ n $-ary semihypergroups, for $ n\geq 3 $, in general. To provide a sufficient condition that makes the above conclusion true, we need to introduce the following definition.
Definition 4.7. Let $ (S, f) $ be an $ n $-ary semihypergroup with $ n\geq 3 $. A semilattice regular relation (semilattice strongly regular relation) $ \rho $ on $ S $ is called a $ * $-semilattice regular relation ($ * $-semilattice strongly regular relation) if for every $ x, y \in S $,
Example 4.8. Consider a ternary semihypergroup $ (S, f) $ given in Example 4.6. Obviously, $ -i\ \bar{\bar{\rho}}\ f(-i, -1, -1) = \{-i\} $. Since $ f(-i, -i, -1) = \{1\} $ and $ (-i, 1) \notin \rho $, we conclude that $ \rho $ is not a $ * $-semilattice strongly regular relation on $ S $.
However, the relation $ \mathcal{N} $ is always a $ * $-semilattice strongly regular relation on $ n $-ary semihypergroups where $ n\geq 3 $.
Proposition 4.9. Let $ (S, f) $ be an $ n $-ary semihypergroup with $ n\geq 3 $. Then the following assertions hold:
$ \rm(i) $ If $ K $ is a completely prime hyperideal of $ S $, then $ \delta_K $ is a $ * $-semilattice strongly regular relation on $ S $.
$ \rm(ii) $ The relation $ \mathcal{N} $ is a $ * $-semilattice strongly regular relation on $ S $.
Proof. From Theorems 4.4 and 4.5, we obtain that $ \delta_K $ and $ \mathcal{N} $ are semilattice strongly regular relations on $ S $.
(i) Let $ x, y \in S $ be such that $ x \overline{\overline{\delta_K}} f(x, y^{n-1}) $. To show that $ x \overline{\overline{\delta_K}} f(x^{n-1}, y) $, let $ a \in f(x^{n-1}, y) $. We consider two cases as follows:
Case 1: $ x \notin K $. Since $ x \overline{\overline{\delta_K}} f(x, y^{n-1}) $, by the definition of $ \delta_K $, we have $ f(x, y^{n-1}) \cap K = \emptyset $. It follows that $ f(x, y^{n-1}) \cap (S\setminus K) \neq\emptyset $. Since $ K $ is a completely prime hyperideal of $ S $, by Corollary 3.8, we have $ S\setminus K $ is a hyperfilter of $ S $. By (F1) in Definition 3.1, we have $ x, y \in S\setminus K $. Since $ S\setminus K $ is an $ n $-ary subsemihypergroup of $ S $, we obtain $ a \in f(x^{n-1}, y) \subseteq S\setminus K $. By the definition of $ \delta_{K} $, we have $ x \delta_{K} a $. Thus $ x \overline{\overline{\delta_K}} f(x^{n-1}, y) $.
Case 2: $ x \in K $. Since $ K $ is a hyperideal of $ S $, by (I1) in Definition 2.1, we obtain $ a \in f(x^{n-1}, y) \subseteq K $. Consequently, $ x \delta_{K} a $ and so $ x \overline{\overline{\delta_K}} f(x^{n-1}, y) $. From Cases 1 and 2, we conclude that $ \delta_K $ is a $ * $-semilattice strongly regular relation on $ S $.
(ii) Let $ x, y \in S $ with $ x \overline{\overline{\mathcal{N}}} f(x, y^{n-1}) $. Then $ N(x) = N(b) $ for all $ b \in f(x, y^{n-1}) $. To show that $ x \overline{\overline{\mathcal{N}}} f(x^{n-1}, y) $, let $ c \in f(x^{n-1}, y) $. Since $ b \in f(x, y^{n-1})\cap N(x) $, by (F1) in Definition 3.1, we have $ x, y \in N(x) $. Since $ N(x) $ is an $ n $-ary semihypergroup, we have $ c \in f(x^{n-1}, y) \subseteq N(x) $. By Lemma 3.10, we get $ N(c) \subseteq N(x) $. Conversely, since $ f(x^{n-1}, y) \cap N(c)\neq \emptyset $, by (F1) in Definition 3.1, we have $ x \in N(c) $. So $ N(x) \subseteq N(c) $. Consequently, $ N(x) = N(c) $ and it follows that $ x \mathcal{N} c $ for all $ c \in f(x^{n-1}, y) $. Therefore $ x \overline{\overline{\mathcal{N}}} f(x^{n-1}, y) $ and the proof is complete.
Theorem 4.10. Let $ (S, f) $ be an $ n $-ary semihypergroup with $ n\geq 3 $. Let $ \rho $ be a $ * $-semilattice strongly regular relation on $ S $. Then there exists a collection $ \mathcal{C} $ of proper completely prime hyperideals of $ S $ such that
Proof. Let $ x \in S $. Setting
Since $ \rho $ is a $ * $-semilattice strongly regular relation, we obtain $ x \bar{\bar{\rho}} f(x^n) $. So $ x \in F_x $. Firstly, we show that $ F_x $ is a hyperfilter of $ S $.
(1) We show that $ F_x $ is an $ n $-ary subsemihypergroup of $ S $. Let $ y_1^n \in F_x $. Then $ f(y_1^n) \subseteq F_x $. Indeed, let $ u \in f(y_1^n) $. We will show that $ u \in F_x $, i.e., $ x \bar{\bar{\rho}} f(x^{n-1}, u) $. Let $ v \in f(x^{n-1}, u) $. Since $ y_k \in F_x $ for all $ k = 1, ..., n $, we have $ x \bar{\bar{\rho}} f(x^{n-1}, y_k) $ for all $ k = 1, ..., n $. Since $ \rho $ is a $ * $-semilattice strongly regular relation on $ S $, by associativity and Lemma 4.1, we obtain
Hence $ x \bar{\bar{\rho}} f(x^{n-1}, f(y_1^n)) $. Since $ v \in f(x^{n-1}, u) \subseteq f(x^{n-1}, f(y_1^n)) $, we have $ x \rho v $. So $ x \bar{\bar{\rho}} f(x^{n-1}, u) $ and it implies that $ u \in F_x $. Thus $ f(y_1^n) \subseteq F_x $.
(2) We show that $ F_x $ satisfies (F1) in Definition 3.1. Let $ y_1^n \in S $ such that $ f(y_1^n) \cap F_x \neq \emptyset $. Then there exists $ v \in f(y_1^n) $ and $ v \in F_x $. Then $ x \bar{\bar{\rho}} f(x^{n-1}, v) $. First of all, we show that $ x\bar{\bar{\rho}} f(x^{n-1}, f(y_1^n)) $. Let $ a \in f(x^{n-1}, f(y_1^{n})) $. Then $ a \in f(x^{n-1}, d) $ for some $ d \in f(y_1^n) $. Since $ f(y_1^n) \bar{\bar{\rho}} f(y_1^n) $ and $ v, d \in f(y_1^n) $, we have $ v \rho d $. Since $ \rho $ is a strongly regular relation, we obtain $ f(x^{n-1}, v)\bar{\bar{\rho}} f(x^{n-1}, d) $. Since $ x \bar{\bar{\rho}} f(x^{n-1}, v) $, we obtain $ x \bar{\bar{\rho}} f(x^{n-1}, d) $. Since $ a \in f(x^{n-1}, d) $, we have $ x\rho a $. Thus $ x \bar{\bar{\rho}} f(x^{n-1}, f(y_1^n)) $. Next, we show that $ y_k \in F_x $ for all $ k = 1, ..., n $. For any $ 1\leq k \leq n $, we have
Since $ \rho $ is a strongly regular relation, we obtain
Since $ \rho $ is a $ * $-semilattice strongly regular relation on $ S $, we have $ x \bar{\bar{\rho}} f(x^{n-1}, y_k) $. So $ y_k \in F_x $ for all $ k = 1, 2, ..., n $. This follows that $ F_x $ satisfies the condition (F1) in Definition 3.1. By (1) and (2), we conclude that $ F_x $ is a hyperfilter of $ S $. By Corollary 3.8, we have $ S\setminus F_x = \emptyset $ or $ S\setminus F_x $ is a completely prime hyperideal of $ S $. If $ S\setminus F_x = S $ then, since $ F_x \subseteq S $, we have $ F_x = \emptyset $. It is impossible. Thus $ S\setminus F_x $ is a proper completely prime hyperideal of $ S $. Define
Next, we show that $ \rho = \bigcap\limits_{x\in S} \delta_{S\setminus F_x} $. Let $ (a, b) \in \rho $ and $ x \in S $. We consider the following two cases.
Case 1: $ a \notin S\setminus F_x $. Then $ a \in F_x $. We obtain $ x \bar{\bar{\rho}} f(x^{n-1}, a) $. Since $ (a, b) \in \rho $, we obtain $ f(x^{n-1}, a) \bar{\bar{\rho}} f(x^{n-1}, b) $. Then $ x \bar{\bar{\rho}} f(x^{n-1}, b) $ and so $ b \in F_x $. It follows that $ b \notin S\setminus F_x $. Thus $ (a, b) \in \delta_{S\setminus F_x} $.
Case 2: $ a \in S\setminus F_x $. If $ b \notin S\setminus F_x $ then, using the similar proof as in Case 1, we have $ a \notin S\setminus F_x $, which is a contradiction. So $ b \in S\setminus F_x $. It follows that $ (a, b) \in \delta_{S\setminus F_x} $. Thus $ \rho \subseteq \bigcap\limits_{x\in S}\delta_{S\setminus F_x} $. Conversely, let $ (a, b) \in \bigcap\limits_{x\in S}\delta_{S \setminus F_x} $. Then $ (a, b) \in \delta_{S\setminus F_a} $. Since $ a \in F_a $, we have $ a \notin S\setminus F_a $. Since $ (a, b) \in \delta_{S\setminus F_a} $, we have $ b \notin S\setminus F_a $. So $ b \in F_a $. By the definition of $ F_a $, we have $ a \bar{\bar{\rho}} f(a^{n-1}, b) $. On the other hand, by using the similar process, we have $ b\bar{\bar{\rho}} f(b^{n-1}, a) $. Then
It implies that $ (a, b) \in \rho $. Therefore $ \bigcap\limits_{x\in S}\delta_{S\setminus F_x} \subseteq \rho $ and the proof is complete.
Corollary 4.11. Let $ (S, f) $ be an $ n $-ary semihypergroup with $ n\geq 3 $. Then the relation $ \mathcal{N} $ is the least $ * $-semilattice strongly regular relation on $ S $.
Proof. Let $ \rho $ be a $ * $-semilattice strongly regular relation on $ S $. By Theorem 4.10, there exists a collection $ \mathcal{C} $ of proper completely prime hyperideals of $ S $ such that $ \rho = \bigcap\limits_{K\in \mathcal{C}}\delta_K $. By Theorem 4.5, we have $ \mathcal{N} = \bigcap\limits_{K\in \mathcal{CPI(S)}}\delta_K $. Since $ \mathcal{C} \subseteq \mathcal{CPI(S)} $, we obtain that
5.
Complete $ * $-semilattice strongly regular relations
As we have known from [21] that the relation $ \mathcal{N} $ is not the smallest semilattice congruence on ordered semihypergroups in general. The following example show that the result is also true in case of the $ * $-semilattice strongly regular relation on ordered $ n $-ary semihypergroups with $ n \geq 3 $.
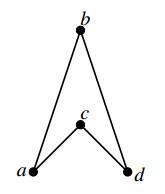
Example 5.1. Let $ S = \{a, b, c, d \} $. Define $ f:S\times S\times S \to \mathcal{P}^*(S) $ by $ f(x_1, x_2, x_3) = (x_1 \circ x_2) \circ x_3 $, for all $ x_1, x_2, x_3 \in S $, where $ \circ $ is defined by the following table.
By applying Example 1 in [31], $ (S, f) $ is a ternary semihypergroup. Define a partial order $ \leq $ on $ S $ by
Such relation can be presented by the following diagram.
Then $ (S, f, \leq) $ is an ordered ternary semihypergroup. Moreover, we have $ N(b) = \{b\} $ and $ N(a) = S = N(c) = N(d) $. It follows that
It is not difficult to show that
is a $ * $-semilattice strongly regular relation on $ S $ and $ \rho \subseteq \mathcal{N} $.
Next, we introduce the concept of complete $ * $-semilattice (strongly) regular relations on ordered $ n $-ary semihypergroups, where $ n\geq 3 $, that generalizes Definition 4.1 in [21].
Definition 5.2. Let $ S $ be an ordered $ n $-ary semihypergroup with $ n\geq 3 $. A $ * $-semilattice regular relation ($ * $-semilattice strongly regular relation) $ \rho $ on $ S $ is said to be complete if for every $ x, y \in S $,
Note that Theorem 4.4, Theorem 4.5 and Proposition 4.9 are also true for ordered $ n $-ary semihypergroups where $ n\geq 3 $.
Proposition 5.3. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n\geq 3 $. Then the relation $ \mathcal{N} $ is a complete $ * $-semilattice strongly regular relation on $ S $.
Proof. Firstly, let $ x, y \in S $ such that $ x \leq y $. We show that $ x \overline{\overline{\mathcal{N}}} f(x^{n-1}, y) $. Let $ a \in f(x^{n-1}, y) $. Since $ x \leq y $, by Lemma 3.10, we have $ y \in N(y) \subseteq N(x) $. Then $ x, y \in N(x) $. Since $ N(x) $ is an $ n $-ary subsemihypergroup, we obtain $ a \in f(x^{n-1}, y) \subseteq N(x) $. Hence $ N(a) \subseteq N(x) $. Conversely, since $ f(x^{n-1}, y) \cap N(a) \neq \emptyset $, by (F1), we obtain $ x \in N(a) $. By Lemma 3.10, we get $ N(x) \subseteq N(a) $. So $ N(x) = N(a) $. It follows that $ x \mathcal{N} a $. Thus $ x \overline{\overline{\mathcal{N}}} f(x^{n-1}, y) $.
Theorem 5.4. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n\geq 3 $. Let $ \rho $ be a complete $ * $-semilattice strongly regular relation on $ S $. Then there exists a collection $ \mathcal{C} $ of proper completely prime hyperideals of $ S $ such that
Proof. Let $ x \in S $. Define
From Theorem 4.10, it remains to show that the set $ F_x $ satisfies (F2) in Definition 3.1. Let $ y \in F_x $ and $ z \in S $ such that $ y \leq z $. Since $ y \in F_x $, we have $ x \bar{\bar{\rho}} f(x^{n-1}, y) $. Since $ y\leq z $ and $ \rho $ is a complete $ * $-semilattice strongly regular relation on $ S $, we have $ y \bar{\bar{\rho}} f(y^{n-1}, z) $. Since $ \rho $ is a $ * $-semilattice strongly regular relation on $ S $, we have $ y \bar{\bar{\rho}} f(y^n) $. Next, we consider
Consequently, $ z \in F_x $ and the proof is complete.
Corollary 5.5. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n \geq 3 $. Then $ \mathcal{N} $ is the smallest complete $ * $-semilattice strongly regular relation on $ S $.
Proof. The proof is similar to Corollary 4.11.
Example 5.6. Consider the ternary semihypergroup $ (S, f) $ given in Example 5.1. Define a partial order $ \leq $ on $ S $ by
Such relation can be presented by the following diagram.
By applying Example 1 in [31], $ (S, f, \leq) $ is an ordered ternary semihypergroup. There are 4 complete $ * $-semilattice strongly regular relations as follows:
Furthermore, we have $ N(b) = \{b\} $, $ N(c) = \{c\} $ and $ N(a) = N(d) = S $. It follows that $ \mathcal{N} : = \{(a, a), (a, d), (b, b), (c, c), (d, a), (d, d)\} = \sigma_1 $. Obviously, $ \mathcal{N} $ is the smallest complete $ * $-semilattice strongly regular relation on $ S $.
6.
Decomposition of (completely) prime hyperideals of ordered $ n $-ary semihypergroups
In this section, we show that every completely prime hyperideal on an ordered $ n $-ary semihypergroups can be decomposable into its $ \mathcal{N} $-classes. But the result is not true in the case of prime hyperideals, see Example 6.6. This leads to answer the open problem given in [31]. However, we will provide a sufficient condition that makes the above conclusion true for case of prime hyperideals. Note that Theorems 4.4 and 4.5 are also true for every ordered $ n $-ary semihypergroup with $ n\geq 2 $.
Theorem 6.1. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n\geq 2 $. Let $ K $ be a completely prime hyperideal of $ S $. Then
Proof. Firstly, we show that $ \bigcup\left\lbrace (a)_{\mathcal{N}} : a \in K \right\rbrace \subseteq K $. Let $ b \in (a)_{\mathcal{N}} $ for some $ a \in K $. By Theorem 4.5, we have $ (a, b) \in \mathcal{N} = \bigcap \left\lbrace\delta_A : A \in \mathcal{CPI(S)}\right\rbrace $. So $ (a, b) \in \delta_K $. Since $ a\in K $, we get $ b \in K $. Thus $ \bigcup\left\lbrace (a)_{\mathcal{N}} : a \in K \right\rbrace \subseteq K $. As we have known that $ a \in (a)_{\mathcal{N}} $ for all $ a \in K $, so $ K \subseteq \bigcup \{(a)_{\mathcal{N}} : a\in K\} $. Therefore $ K = \bigcup\left\lbrace (a)_{\mathcal{N}} : a \in K \right\rbrace $.
To solve the problem raised by Tang and Davvaz in [31], we firstly prove the following lemmas.
Lemma 6.2. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n\geq 2 $. Let $ K $ be a nonempty subset of $ S $ satisfying the following property:
For any $ 1 \leq j \leq n $, if a nonempty subset $ S\setminus K $ is a prime $ j $-hyperideal of $ S $ then $ K $ is a $ j $-hyperfilter of $ S $.
Proof. The proof is similar to the proof of the converse of Theorem 3.7 by applying the property $ (\odot) $.
Remark 6.3. For case $ n = 2 $, we obtain that the notion of prime hyperideal on $ S $ satisfying the property $ (\odot) $ coincides with the notion of prime hyperideal on ordered semihypergroups introduced by Kehayopulu (see Definition 2.5 in [21]).
The following results, Lemma 6.4 and Theorem 6.5, generalize Proposition 2.5 and Theorem 2.10 in [21].
Lemma 6.4. Let $ (S, f, \leq) $ be an ordered $ n $-ary semihypergroup with $ n\geq 2 $. Then the following statements hold:
$ \rm(i) $ For any $ K\in \mathcal{P}^*(S) $ satisfying the property $ (\odot) $, $ K $ is a hyperfilter of $ S $ if and only if either $ S\setminus K = \emptyset $ or $ S\setminus K $ is a prime hyperideal of $ S $.
$ \rm(ii) \; \mathcal{N} = \bigcap \left\lbrace \delta_{K}: K \in \mathcal{PH(S)} \right\rbrace $ where $ \mathcal{PH(S)} $ is the set of all prime hyperideals of $ S $ satisfying the property $ (\odot) $.
Proof. As we have known that every completely prime hyperideal of $ S $ is always a prime hyperideal of $ S $. By Corollary 3.8 and Lemma 6.2, the proof of (i) is complete. The proof of (ii) is similar to the proof of Theorem 4.5(ii) by applying (i).
Using the similar proof of Theorem 6.1, by using Lemma 6.4(ii), we obtain the result as follows.
Theorem 6.5. Let $ (S, f\leq) $ be an ordered $ n $-ary semihypergroup with $ n\geq 2 $. Let $ K $ be a prime hyperideal of $ S $ satisfying the property $ (\odot) $. Then $ K = \bigcup\left\lbrace (a)_{\mathcal{N}} : a \in K\right\rbrace $.
The following example shows that a prime hyperideal of $ S $ that does not satisfy the property $ (\odot) $ cannot be decomposable into its $ \mathcal{N} $-classes.
Example 6.6. From the ordered $ n $-ary semihypergroup $ (S, f, \leq) $ given in Example 2.4, we obtain that the sets $ A_2 = \{y, z\} $ and $ A_3 = \{w, x, z\} $ are prime $ 2 $-hyperideals of $ S $. Furthermore, $ A_2, A_3 $ are also prime hyperideals of $ S $. Clearly, $ A_3 $ satisfies the property $ (\odot) $ but $ A_2 $ does not satisfy the property $ (\odot) $ since there exists $ w \in S $ such that $ f(w^n) \nsubseteq A_2 $ and $ f(w^n) \nsubseteq S\setminus A_2 $. On the other hand, we can verify that $ N(w) = N(x) = N(z) = S $ and $ N(y) = \{y\} $. Hence
Then $ (w)_{\mathcal{N}} = \{w, x, z\} = (x)_{\mathcal{N}} = (z)_{\mathcal{N}} $ and $ (y)_{\mathcal{N}} = \{y\} $. Clearly,
and
Remark 6.7. If we set $ n = 2 $ then the problem given in [31] is answered by Theorem 6.5 and Example 6.6.
7.
Conclusions
In this paper, we introduced the concept of $ j $-hyperfilters of ordered $ n $-ary semihypergroups, where a positive integer $ 1\leq j\leq n $ and $ n\geq 2 $, and discussed their related properties. Such notion can be considered as a generalization of left and right hyperfilters of ordered semihypergroups. The interesting properties of $ j $-hyperfilters by means of completely prime $ j $-hyperideals were established. Furthermore, we defined and investigated the semilattice strongly regular relation $ \mathcal{N} $ on ordered $ n $-ary semihypergroups by the hyperfilters. In particular, we illustrated by counterexample that the relation $ \mathcal{N} $ is not necessary the least semilattice strongly regular relation on ordered $ n $-ary semihypergroups where $ n\geq 3 $. Meanwhile, we introduced the concept of complete $ * $-semilattice strongly regular relations on ordered $ n $-ary semihypergroups, where $ n\geq 3 $, and proved that $ \mathcal{N} $ is the smallest complete $ * $-semilattice strongly regular relation. Finally, we provided some necessary conditions to show that every prime hyperideal can be decomposable into its $ \mathcal{N} $-classes. This result answers the related problem posed by Tang and Davvaz in [31].
Acknowledgments
The authors are highly grateful to the referees for their valuable comments and suggestions for improving the article. This research was supported by Chiang Mai University, Chiang Mai 50200, Thailand.
Conflict of interest
The authors declare no conflict of interest.










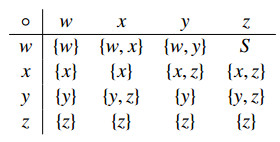

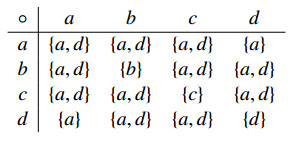








 DownLoad:
DownLoad: