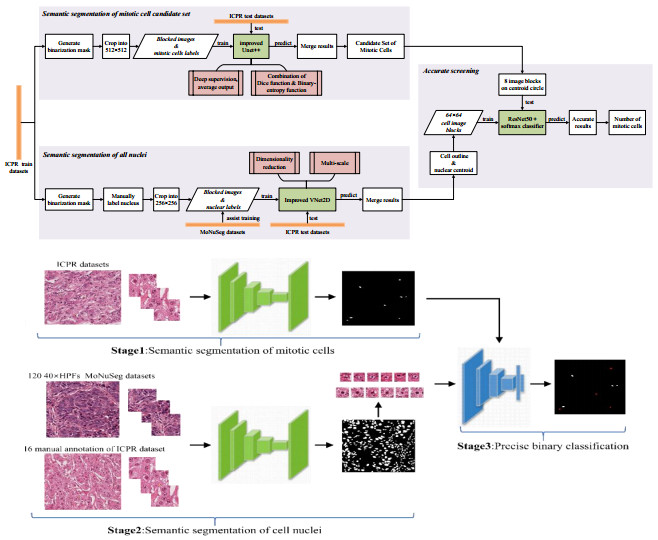
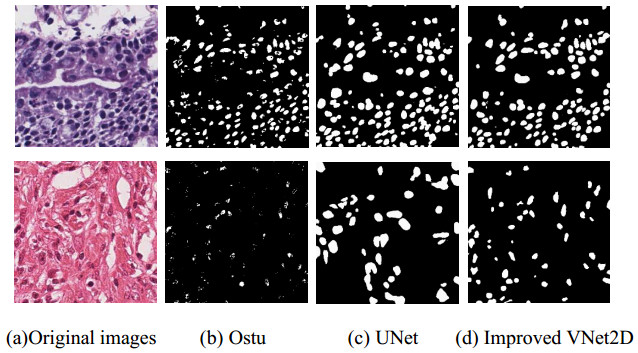
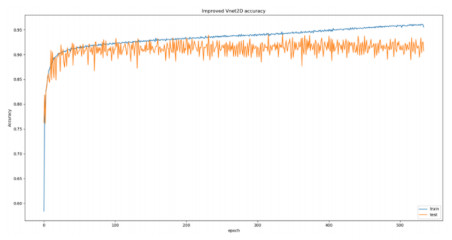
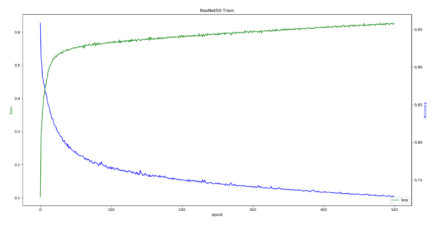
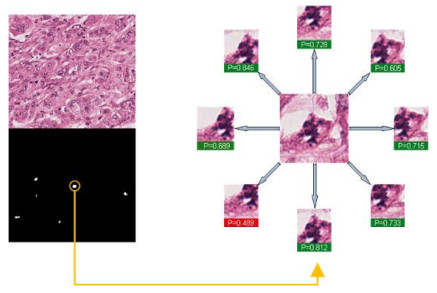
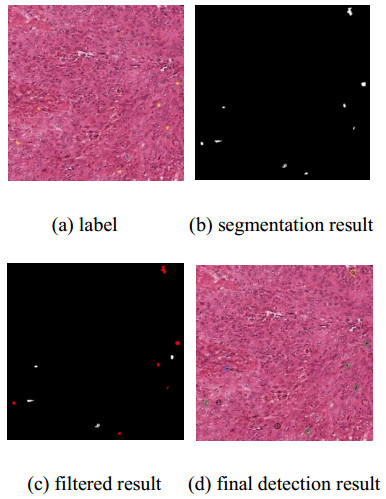
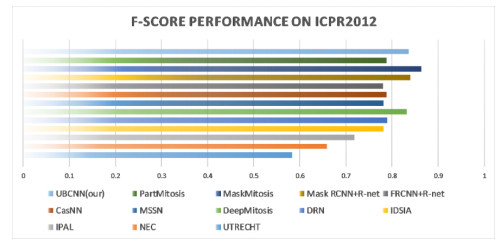
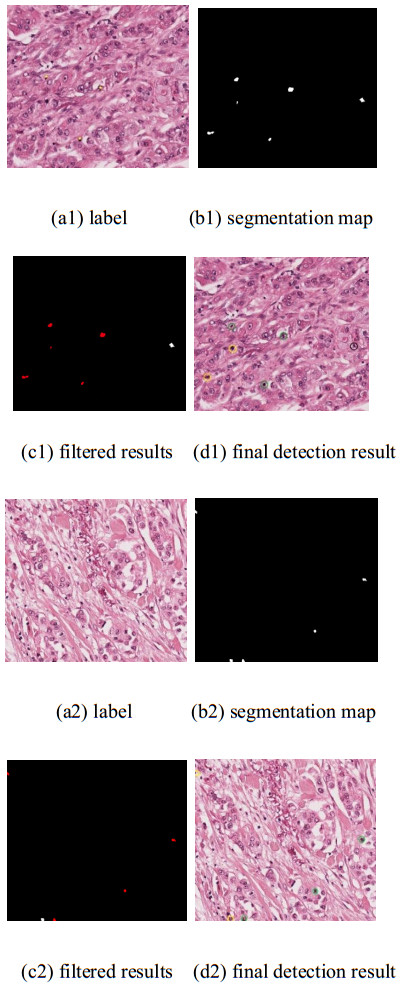
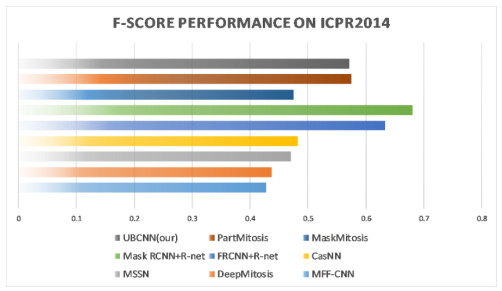
The number of mitotic tumor cells detected in each slide is one of the key indicators of breast cancer prognosis. However, accurate mitotic cell counts are still a difficult problem for pathologists and related experts. Traditional methods use manual design algorithms to extract features of mitotic cells, and most methods rely on sliding windows to achieve pixel-level classification through deep learning. However, the complex background and high resolution of pathological images make the above methods time-consuming and ineffective. In order to solve the above problems, we propose a new cascaded convolutional neural network UBCNN (cascaded CNN based on UNet), which consists of three parts: semantic segmentation and classification to detect mitosis. First, we use an improved UNet ++ segmentation network to locate the candidate set of mitotic targets. Secondly, an adequately labeled cell nucleus data set is sent to an improved two-dimensional VNet network, and the cell nucleus is located by means of semantic segmentation to obtain accurate image blocks of mitotic and non-mitotic cells. Finally, the obtained cell image block is used to train a convolutional neural network to achieve binary classification, and the candidate set area is screened to retain the final result of mitosis cells. This paper verifies the detection effect of the above-mentioned cascade detection algorithm on the ICPR 2012 and 2014 mitosis automatic detection competition data sets. The evaluation indicators include accuracy, recall and F-score. Our cascade detection algorithm based on segmentation and classification reached 0.831 on the ICPR 2012 data set and 0.576 on the ICPR 2014 data set. Compared with other existing algorithms, the detection effect was improved, which was very competitive.
1.
Introduction
Greene [1] mentioned in his book "The Elegance of the Universe" that the 11th dimension appears when the Heterotic-E matching constant is greater than 1 but not less than 1. In this case, it may be thought that the 11th dimension is a different topology. I think this may be a discrete topology. So, is a topology possible in which different topologies of different dimensions can exist? In this context, for a topological space in which different topologies can be written in different parameters, it should be looked at whether a set is created in which different sets can be written in different parameters. So, let us first analyze Molodtsov's soft set [2] which makes it possible to write different sets with different parameters.
Molodtsov [2] defined a soft set and gave some properties about it. For this, he thought that there are many uncertainties to solve complicated problems such as in sociology, economics, engineering, medical science, environment problems, statistics, etc. There is no deal to solve them successfully. However, there are some theories such as vague sets theory [3], fuzzy sets [4], probability, intuitionistic fuzzy sets [5], rough sets [6], interval mathematics [7], etc., but these studies have their own complexities. Also, you can see the latest study examples based on them [8,9,10,11,12,13].
Maji et al. [14] defined soft subset, soft superset, soft equality and they gave some operators such as intersection, union of two soft sets and complement of a soft set. They presented some properties about them. But some properties of them are false. So, Yang [15] gave counterexample about some of them. Also, Ali et al. [16] gave counter example about the others. Then they redefined operations of soft sets. Then the other researchers pointed out these false about soft operators and they gave some new definitions about soft operators of soft sets [17,18,19,20,21,22,23,24,25]. These are so valuable studies.
Up to now, there are many studies on the soft sets and their operations, also, taking universal set of parameters E is finite and countable because of the definition of the soft set. There are many soft sets and their operators defined. As first, in the soft set defined by Molodtsov [2] and developed by Maji et al. [14] the union of any soft set and its complement need not be a universal set. So, this situation makes a lot of deficiencies like a problem about complement of any soft set. To overcome this problem, researchers see two different ways. One way is using fixed parameter set in soft sets like Shabir and Naz's work [26]. They defined a lot of concept about a soft set and its topology. They used same parameter sets in their soft sets. This situation limits the soft topology. As a result of the fixed parameter, a soft point could be defined with fixed parameter. As a result of this, all soft sets have same values in all their parameters [27,28,29,30]. Some researchers tried to overcome this situation by defining another soft point [17,24,27,31]. You can see the latest study examples based on Shabir and Naz's work [32,33,34,35,36,37,38].
The other way is to redefine soft set and its operations. As first, Çağman and Enginoğlu [19] redefined soft set and its operations. This study is so valuable. But anyone could not take uncountable or infinite universal parameter set in practice. Because it is not pointed out what will we do with parameter sets between two soft sets exactly while using soft operators. You can see the latest study examples based on Çağman and Enginoglu's work [32,36,37,38,39,40]. Çağman et al. [41] defined a soft topology. They use different parameter sets in their soft sets. But all of them are finite because of the definition of soft operations. Then, Zhu and Wen [25] redefined a soft set and gave operations of it. Also, they pointed out what will we do with parameters set between two soft sets while using soft operators. But it is so complicated and as a result, cause same problems. You can see the latest study examples based on Zhu and Wen's work [42,43,44].
A similar situation drew attention of Fatimah et al. in [45]. They said that in their study all soft and hybrid soft sets used so far binary operation (either 0 or 1) or else real numbers between 0 and 1. So they defined a new soft set and it is called an N-Soft set. They used n parameter in their study, n∈N, is natural numbers. Riaz et al. defined an N-Soft topology in [42]. Therefore, we can say that they use set of parameters E as infinite and countable. They use initial universe X as finite and countable. But in fact, in real life or in space they do not have to be finite and countable.
In order to overcome all the problems mentioned about above and to define a more comprehensive and a more generalized soft set, I defined an amply soft set. I named this soft set as an amply soft set, together with its operations, in order to eliminate the complexity by selecting the ones that are suitable for a certain purpose among the previously defined soft sets and the operations between them and redefining otherwise. Amply soft sets use any kind of universal parameter set and initial universe (such as finite or infinite, countable or uncountable). Also, I introduced subset, superset, equality, empty set, and whole set about amply soft sets. And I gave operations such as union, intersection, difference of two amply soft sets and complement of an amply soft set. Then three different amply soft point such as amply soft whole point, amply soft point and monad point are defined. Also examples related taking universal set as uncountable are given.
I defined a new soft topology, and it is called as a PAS topology. The PAS topology allows to write different elements of classical topologies in its each parameter sets. The classical topologies may be finite, infinite, countable or uncountable. This situation removes all of the boundaries in a soft topology and cause it to spread over larger areas. A PAS topology is a special case of an AS topology. For this purpose, I defined a new soft topology, and it is called as an amply soft topology or briefly an AS topology. I introduce AS open sets, AS closed sets, interior and closure of an AS set and subspace of any AS topological space. I gave parametric separation axioms which are different from Ti separation axioms. Ti questions the relationship between the elements of space itself while Pi questions the strength of the connection between their parameters.
2.
Materials and methods
These terminologies are used hereafter in the paper: X denotes an initial universe, E denotes a universal set of parameters; A,B,C are subsets of E.
Definition 1 Let P(X) denote the power set of X. If F:E→P(X) is a mapping given by
then F with A is called as an amply soft set over X and it is denoted by F∗A. We can say an AS set instead of an amply soft set for briefness.
Example 1 Let X={x1,x2,x3,x4} be a universal set, E={e1,e2,e3,e4,e5} a set of parameters and A={e1,e2,e4,e5} a subset of E. Let F:A→P(X) be the mapping given by F(e1)={x1,x2},F(e2)={x2,x4},F(e4)={x3},F(e5)={x3}. Then we can show it looks like the following:
Definition 2 Let F∗A and G∗B be two amply soft sets over X.F∗A is subset of G∗B, denoted by F∗A˜⊆G∗B, if F(e)⊆G(e), for all e∈A.
Definition 3 Let F∗A and G∗B be two amply soft sets over X.F∗A is superset of G∗B, denoted by
Definition 4 Let F∗A and G∗B be two amply soft sets over X. If F∗A is subset of G∗B and G∗B is subset of F∗A also, then F∗A and G∗B are said to be an equal and denoted by F∗A≅G∗B.
Definition 5 An amply soft set F∗A over X is said to be an empty amply soft set denoted by ˜∅ if F(e)=∅ for all e∈E.
Definition 6 An amply soft set F∗E over X is said to be an absolute amply soft set denoted by ˜X if for all e∈E,F(e)=X.
Definition 7 The union of two amply soft sets of F∗A and G∗B over a common universe X is the amply soft set H∗C, where C=A∪B and for all e∈E,
We can write F∗A˜∪G∗B≅F∪G∗A∪B≅H∗C.
Definition 8 The intersection H∗C of two amply soft sets F∗A and G∗B over a common universe X denoted by F∗A˜∩G∗B is defined as C=A∩B and for all e∈E,
We can write F∗A˜∩G∗B≅F∩G∗A∩B≅H∗C.
Definition 9 The difference H∗A of two amply soft sets F∗A and G∗B over X is denoted by F∗A˜∖G∗B and it is defined as
We can write F∗A˜∖G∗B≅F∖G∗A≅H∗A.
Definition 10 Let F∗A be an amply soft set over ˜X. The complement of an amply soft set F∗A over X is denoted by (F∗A)~′≅F'∗E where F':E→P(X) a mapping is defined as F'(e)=X−F(e) for all e∈E.
Note that, F':E→P(X) is a mapping given by
Example 2 Let X={h1,h2,h3,h4,h5},E={e1,e2,e3,e4,e5} and its subsets A={e1,e2,e3},B={e1,e3,e4},C={e1,e4} and F∗A,G∗B,H∗C are amply soft sets over X defined as follows respectively,
Then,
1. F∗A˜∪G∗B≅F∪G∗A∪B
2. F∗A˜∩G∗B≅F∩G∗A∩B≅{{h1}{e1},{h1,h2}{e3}}.
3. F∗A˜∖G∗B≅F∖G∗A≅{{h2}{e1},{h2,h3,h4}{e2},{h5}{e3}}.
4. G∗B˜∖F∗A≅G∖F∗B≅{{h4}{e1,e3},{h3,h5}{e4}}.
5. (F∗A)~′≅F'∗E≅{{h3,h4,h5}{e1},{h1,h5}{e2},{h3,h4}{e3},X{e4,e5}}.
6. 6. (G∗B)~′≅G'∗E≅{{h2,h3,h5}{e1},{h3,h5}{e3},{h1,h2,h4}{e4},X{e2,e5}}.
Proposition 1 Let F∗A,G∗B and H∗C be amply soft sets over X;A,B,C⊆E. Then the followings are hold.
1. F∗A˜∪F∗A≅F∗A,
2. F∗A˜∩F∗A≅F∗A,
3. F∗A˜∪˜∅≅F∗A,
4. F∗A˜∩˜∅≅˜∅,
5. F∗A˜∪˜X≅˜X,
6. F∗A˜∩˜X≅F∗A,
7. F∗A˜∪G∗B≅G∗B˜∪F∗A,
8. F∗A˜∩G∗B≅G∗B˜∩F∗A,
9. (F∗A˜∪G∗B)˜∪H∗C≅F∗A˜∪(G∗B˜∪H∗C),
10. (F∗A˜∩G∗B)˜∩H∗C≅F∗A˜∩(G∗B˜∩H∗C),
11. F∗A˜∩(G∗B˜∪H∗C)≅(F∗A˜∩G∗B)˜∪(F∗A˜∩H∗C),
12. F∗A˜∪(G∗B˜∩H∗C)≅(F∗A˜∪G∗B)˜∩(F∗A˜∪H∗C).
13. ((F∗A)~′)~′≅F∗A
14. (F∗A˜∪G∗B)~′≅(F∗A)~′˜∩(G∗B)~′
15. (F∗A˜∩G∗B)~′≅(F∗A)~′˜∪(G∗B)~′
Proof. Let F∗A and G∗B be amply soft sets over X;A,B⊆E.
1. F∗A defined as from the Definition 1,
So
Then,
2. It can be shown like above.
3. F∗A defined as from the Definition 1,
And ˜∅ defined as from the Definition 5, F(e)=∅ for all e∈E.
So
Then,
4. It can be shown like above.
5. F∗A defined as from the Definition 1,
And ˜X defined as from the Definition 6, F(e)=X for all e∈E.
So
Then,
6. F∗A defined as from the Definition 1,
And ˜X defined as from the Definition 6, F(e)=X for all e∈E.
So
Then,
7. F∗A˜∪G∗B=F∪G∗A∪B. Then from the Definition 7, for all e∈E
So any one can see that clearly, F∪G(e)=G∪F(e) for all e∈E.
Then,
8. F∗A˜∩G∗B≅F∩G∗A∩B. Then from the Definition 8, for all e∈E
So any one can see that clearly, F∩G(e)=G∩F(e) for all e∈E.
Then,
The others can be shown like aboves.
Let F∗A and G∗B be amply soft sets over X,A,B⊂E.
(F∗A)~′ is defined as
from the Definition 10. Then ((F∗A)~′)~′≅(F'∗E)~′=(F')'∗E defined as
Then, it means from the Definition 1,
The others can be shown like aboves.
Example 3 Let us consider the amply soft sets F∗A,G∗B,H∗C over X on the Example 2.
1. Let us see if (F∗A∪G∗B)∪H∗C=F∗A∪(G∗B∪H∗C).
Then,
Then,
So,
2. Let us see if (F∗A)˜∩G∗B)˜∩H∗C≅F∗A˜∩(G∗B˜∩H∗C).
Then,
Then,
So,
3. Let us see if F∗A˜∩(G∗B˜∪H∗C)≅(F∗A˜∩G∗B)˜∪(F∗A˜∩H∗C).
Then,
Then,
So,
4. Let us see if F∗A˜∪(G∗B˜∩H∗C)≅(F∗A˜∪G∗B)˜∩(F∗A˜∪H∗C).
Then,
⇐):
So,
5. Let us see if (F∗A)~′)~′≅F∗A.
(F∗A)~′ is showed in matter 5 on the Example 2. Then,
and it is equal
Then
6. Let us see if (F∗A˜∪G∗B)~′≅(F∗A)~′˜∩(G∗B)~′.
F∗A˜∪G∗B≅F∪G∗A∪B is showed in matter 1 on the Example 2. Then,
(F∗A)~′ is showed as in matter 5 on the Example 2 and (G∗B)~′ is showed as in matter 6 on the Example 2. Then,
So,
7. Let us see if (F∗A˜∩G∗B)~′≅(F∗A)~′˜∪(G∗B)~′.
F∗A˜∩G∗B is showed as in matter 2 on the Example 2. Then,
(F∗A)~′ is showed as in matter 5 on the Example 2. And (G∗B)~′ is showed as in matter 6 on the Example 2. Then,
So,
The following example, we take E as an uncountable parameter.
Example 4 Let X={x1,x2,x3,x4,x5,x6,x7,x8,x9,x10}be set of walnut types, E be a parameter set of resistance in percent against to walnut illness; F and G, denotes different types of walnut illnesses, be mappings from E to P(X); E=[0,100]⊂R and A=(60,90], B=(80,100]⊂E, R is set of real numbers.
And let F∗A be an amply soft set for walnut types resistancing in percent against to a type of walnut illness, is defined by
and
G∗B be an amply soft set for walnut types resistancing in percent against to another type of walnut illness, is defined by
Then we can find the followings:
1. F∗(60,90]˜∪G∗(75,95]≅F∪G∗
(60,95]≅{{x1,x3,x7,x8,x9,x10}(60,70],{x1,x7,x9,x10}(70,75],
{x1,x2,x3,x7,x9,x10}(75,80],{x1,x2,x3,x9,x10}(80,85],{x1,x2,x9,x10}(85,90],{x1,x2}(90,95]}.
2. F∗(60,90]˜∩G∗(75,95]≅F∩G∗(75,90]={{x1,x10}(75,85],{x1}(85,90]}.
3. F∗(60,90]˜∖G∗(75,95]≅F∖G∗(60,90]≅{{x1,x3,x7,x8,x9,x10}(60,70],{x1,x7,x9,x10}(70,75],{x7,x9}(75,80],{x9}(80,85],{x9,x10}(85,90]}.
4. G∗(75,95]˜∖F∗(60,90]≅G∖F∗(75,95]≅{{x2,x3}(75,85],{x2}(85,90],{x1,x2}(90,95]}.
5. (F∗(60,90])~′≅F'∗[0,100]≅{{x2,x4,x5,x6}(60,70],{x2,x3,x4,x5,x6,x8}(70,80],{x2,x3,x4,x5,x6,x7,x8}(80,90],X[0,60]∪(95,100]}.
6. (G∗(75,95])~′≅G'∗[0,100]≅{{x4,x5,x6,x7,x8,x9}(75,85],{x3,x4,x5,x6,x7,x8,x9,x10}(85,95],X[0,75]∪(95,100]}.
The following example, we take E and X as an uncountable.
Example 5 Let X=R,E=(0,10]⊂R,F∗(2,5] and G∗(3,8] be amply soft sets, defined as follows respectively, over X, R is a set of real numbers.
Then we can find the following:
1. F∗(2,5]˜∪G∗(3,8]≅F∪G∗(2,8]≅{[10,15](2,3],[7,15](3,4],[7,12]∪{13}(4,5],[7,12](5,7],(0,100)(7,8]}
2. F∗(2,5]˜∩G∗(3,8]≅F∩G∗(3,5]≅{[10,12](3,4]}
3. F∗(2,5]˜∖G∗(3,8]≅F∖G∗(2,5]≅{[10,15](2,3],(12,15](3,4],{13}(4,5]}
4. G∗(3,8]˜∖F∗(2,5]≅G∖F∗(3,8]≅{[7,10)(3,4],[7,12](4,7],(0,100)(7,8]}
5. (F∗(2,5])~′≅F'∗(0,10]≅{R−[10,15](2,4],R−{13}(4,5],R(0,10]−(2,5]}
6. (G∗(3,8])~′≅G'∗(0,10]≅{R−[7,12](3,7],R−(0,100)(7,8],R(0,10]−(3,8]}
Definition 11 Let F∗E be an amply soft set over X and x∈X. If F∗E defined as F(e)={x} for all e∈E, then F∗E is called as an amply soft whole point and it is denoted by ˜x.
Definition 12 Let F∗Abe an amply soft set over X and x∈X. If F∗A defined as F(e)={x} for all e∈A, then F∗A is called as an amply soft point and it is denoted by x.
Definition 13 Let {a}⊂E and let F∗{a}be an amply soft set over X,x∈X. If F∗{a} defined as F(a)={x}, then F∗{a} is called a monad point and it is denoted by xa.
Definition 14 Let F∗Ebe an amply soft set over X and x∈X. We say that ˜x˜∈F∗E read as amply soft whole point ˜x belongs to the amply soft set F∗E if x∈F(e)for all e∈E.
Definition 15 Let F∗E be an amply soft set over X and x∈X. We say that ˜x˜∉F∗E if x∉F(e) for some e∈E.
Definition 16 Let F∗A be an amply soft set over X,x∈X. We say that x˜∈F∗A read as amply soft point x belongs to the amply soft set F∗A if x∈F(e) for all e∈A.
Definition 17 Let F∗A be an amply soft set over X and x∈X. We say that x˜∉F∗A if x∉F(e) for some e∈A.
Definition 18 Let a∈A and F∗A be an amply soft set over X,x∈X. We say that xa˜∈F∗A read as monad point x belongs to the amply soft set F∗A if x∈F(a).
Definition 19 Let a∈E and F∗{a} be an amply soft set over X,x∈X. We say that xa˜∉F∗{a} if x∉F(a).
Proposition 2 Let F∗A and G∗B be amply soft sets over X,x∈X. If x˜∈F∗A and x˜∈G∗Bthen x˜∈F∗A˜∪G∗B.
Proof. It is obvious from the Definition 7 and the Definition 16.
Proposition 3 Let F∗A and G∗Bbe amply soft sets over X,x∈X. If x˜∈F∗A,x˜∈G∗B and F∗A˜∩G∗B˜≠˜∅ then x˜∈F∗A˜∩G∗B.
Proof. It is obvious from the Definition 8 and the Definition 16.
Proposition 4 Let F∗A and G∗B be amply soft sets over X,x∈X,x∈F∗A and x˜∈G∗B. If A∪B=E, then ˜x˜∈F∗A˜∪G∗B.
Proof. It is obvious from the Proposition 2 and the Definition 14.
Example 6 Let us consider the Example 2.
We can see that clearly, h1˜∉F∗A,h1˜∈H∗C,h2˜∈F∗A,h2˜∈H∗C
1. F∗A˜∩H∗C≅F∩H∗A∩C=F∩H∗{e1}={{h1,h2}{e1}}.
So we can see that clearly, h1˜∈F∗A˜∩H∗C,h2˜∈F∗A˜∩H∗C.
2. F∗A˜∪H∗C≅F∪H∗A∪C≅F∪H∗{e1,e2,e3,e4}≅{{h1,h2}{e1},{h2,h3,h4}{e2},{h1,h2,h5}{e3},{h1,h2,h3}{e4}}.
So we can see that clearly, h1˜∉F∗A˜∪H∗C and h2˜∈F∗A˜∪H∗C.
Proposition 5 Let F∗A be an amply soft set over X and a∈A. If monad points xa˜∈F∗A for all a∈A then x˜∈F∗A.
Proof. It is obvious from the Definition 18 and the Definition 12.
Proposition 6 Let F∗E be an amply soft set over X. If monad points xa˜∈F∗E for all a∈E then ˜x˜∈F∗E.
Proof. It is obvious from the Definition 18 and the Definition 11.
Example 7 Let us consider the Example 2.
Monad points h2e1,h2e2,h2e3˜∈F∗A. Then we can see that clearly, h2˜∈F∗A.
3.
AS and PAS topologies
Definition 20 Let ˜τ be the collection of amply soft sets over X, then ˜τ is said to be an amply soft topology (or briefly AS topology) on ˜X if,
1. ˜∅,˜X belong to ˜τ
2. The union of any number of amply soft sets in ˜τ belongs to ˜τ
3. The intersection of any two amply soft sets in ˜τ belongs to ˜τ.
The triplet (˜X,˜τ,E) is called as an amply soft topological space over ˜X.
We will use AS topological space ˜X instead of amply soft topological space (˜X,˜τ,E) for shortly.
Definition 21 Let (˜X,˜τ,E) be an AS topological space, then the members of ˜τ are said to be AS open sets in an AS topological space ˜X.
Definition 22 Let (˜X,˜τ,E) be an AS topological space. An amply soft set F∗A over ˜X is said to be an AS closed set in an AS topological space ˜X, if its complement (F∗A)~′ belongs to ˜τ.
Proposition 7 Let (˜X,˜τ,E) be an AS topological space. Then
1. ˜∅,˜X are AS closed sets in AS topological space ˜X,
2. The intersection of any number of AS closed sets is an AS closed set in an AS topological space ˜X,
3. The union of any two AS closed sets is an AS closed set in an AS topological space ˜X.
Proposition 8 Let (˜X,˜τ,E) be an AS topological space. Then the collection τe={F(e)|F∗E˜∈˜τ} for each e∈E, defines topologies on X.
Proof. Let (˜X,˜τ,E) be an AS topological space.
1. Because (˜X,˜τ,E) is an AS topological space; ˜∅,˜X˜∈˜τ from the Definition 20.
a. Since ˜X˜∈˜τ, there exist an AS open set F∗E such that F(e)=X for all e∈E from the Definition 6. And we can see that clearly, X∈τe for all e∈E.
b. Since ˜∅∈˜τ, there exist an AS open set G∗E such that G(e)=∅ for all e∈E from the Definition 5. And we can see that clearly, ∅∈τe for all e∈E.
2. Because (˜X,˜τ,E) is an AS topological space, the union of any number of amply soft sets in ˜τ belongs to ˜τ from the Definition 20. Then from the Definition 7, the union of any number of sets in τe belongs to τe for all e∈E.
3. Because (˜X,˜τ,E) is an AS topological space, the intersection of any two amply soft sets in ˜τ belongs to ˜τ from the Definition 20. Then from the Definition 8, the intersection of any two sets in τe belongs to τe for all e∈E.
So τe={F(e)|(F,E)˜∈˜τ} for each e∈E, defines topologies on X.
Example 8 Let X={x,y,z} be a universal set, E={e1,e2,e3} a parameter set and
such that every members an amply soft set over X.
We can see that clearly ˜τ is an AS topology from the Definition 20. And so, we can see that
and
are topologies on X.
Also,
Let F∗E≅{{z}{e1},{x,z}{e2},X{e3}} and G∗E≅{X{e1},{x}{e2},{x,y}{e3}} be amply soft sets over X. We can see that clearly these amply soft sets are AS closed sets on ˜τ.
1. We want to learn whether the union of these AS closed sets is an AS closed set.
F∗E˜∪G∗E≅F∪G∗E={X{e1,e3},{x,z}{e2}}. And its complement is (F∪G∗E)~′≅(F∪G)'∗E≅{{y}{e2}}. It is an AS open set that we can see it clearly in ˜τ. From the Definition 22, the union of F∗E and G∗E is an AS closed set.
2. We want to learn whether the intersection of these AS closed sets is an AS closed set.
F∗E˜∩G∗E≅F∩G∗E≅{{z}{e1},{x}{e2},{x,y}{e3}}. And its complement is (F∩G∗E)~′≅(F∩G)~′∗E≅{{x,y}{e1},{y,z}{e2},{z}{e3}}.
It is an AS open set that we can see it clearly in ˜τ. From the Definition 22, the intersection of F∗E and G∗E is an AS closed set.
Definition 23 Let (˜X,˜τ1,E) and (˜X,˜τ2,E) be two AS topological space on ˜X. Then the followings are hold.
1. If ˜τ2˜⊆˜τ1 then ˜τ1 is finer then ˜τ2,
2. If ˜τ2˜⊆˜τ1 then ˜τ2 is coarser then ˜τ1.
Definition 24 Let (˜X,˜τ,E) be an AS topological space and ˜β˜⊆˜τ. If every element of ˜τ can be written as any union of elements of ˜β, then ˜β is called as an AS basis for the AS topology ˜τ. Then we can say that each element of ˜β is an AS basis element.
Example 9 Let us consider the AS topology on the Example 8. Then
is an AS basis for the AS topology ˜τ.
Definition 25 Let n∈N, en∈E. And let (X,τn) be topological spaces over same initial universe X. And let f be mapping from τn to τen for all e∈E. Then ˜β≅{τen} is an AS basis for an AS topology ˜τ. We can say that it is called as an AS topology produced by classical topologies and for shortly a PAS topology.
Example 10 Let X={x,y,z},E={e1,e2,e3} and (X,τ1),(X,τ2),(X,τ3) are topological spaces over X such that τ1={∅,X,{x,y},{x,z},{x}},τ2={∅,X,{y},{y,z}},τ3={∅,X,{y},{z},{y,z}}.
Let n∈{1,2,3},f is mapping from τn to τen for all e∈E. We take ˜β≅{τe1,τe2,τe3} with helping f, for produce AS topology over X.
Then, we can define PAS topology ˜τ over ˜X. For this we have to take any union of element of ˜β from the Definition 24. So;
Hence, we establish PAS topological space (˜X,˜τ,E) over ˜X from the AS basis ˜β≅{τe1,τe2,τe3}.
Proposition 9 Any PAS topological space (˜X,~τ1,E) is finer then the AS topological space (˜X,~τ2,E) over same universe ˜X.
Example 11 Let us consider the PAS topology on the Example 10 and consider the AS topology ˜τ over same universe on Example 8. We can see that clearly, any element of the AS topology is subset of the PAS topology. So we can say that PAS topological space (˜X,~τ1,E) is finer then AS topological space (˜X,~τ2,E) over same universe ˜X.
Proposition 10 Let n∈N,en∈E,(X,τn) be topological spaces over same initial universe X,βn be basis for each τn and let f1 be mapping from βn to βen for all e∈E,~β1≅{βen} be an AS basis for an AS topology ˜τ1. And let ~β2≅{τen} be an AS basis for an AS topology ˜τ2 such that f2 be mapping from τn to τen for all e∈E. Then PAS topological space (˜X,˜τ1,E) is finer then PAS topological space (˜X,˜τ2,E).
Definition 26 Let (˜X,˜τ,E) be an AS topological space and F∗A be an AS set over ˜X. Then the collection,
is called a subspace AS topology on F∗A. Hence, (F∗A,~τF∗A,A) is called as an AS topological subspace of (˜X,˜τ,E).
Definition 27 Let (X,τ,E) be an AS topological space and F∗A be an AS set over ˜X. Then the interior of F∗A denoted by (F∗A)˜° is defined as a union of all AS open subsets of F∗A.
Note that this, (F∗A)˜° is the biggest AS open set that is contained by F∗A.
Example 12 Let us consider the AS topology (˜X,˜τ,E) on the Example 8. And F∗A≅{{x,y}{e1},{z}{e2}} be an AS set over X such that A={e1,e2}⊂E. Then,
Example 13 Let us consider the PAS topology (X,˜τ,E) on the Example 10 and F∗A≅{{x,y}{e1},{z}{e2}} be an AS set over X such that A={e1,e2}⊂E. Then,
Proposition 11 Let F∗A be an AS set on X and let τ1 be a PAS topological space and τ2 be an AS topological space on X. Then, (F∗A)˜° on ˜τ2 is contained by (F∗A)˜° on ˜τ1.
Proof. It is obvious from the Proposition 9 and the Definition 27.
Definition 28 Let (X,τ,E) be an AS topological space and F∗A be an AS set over ˜X. Then the closure of F∗A denoted by ¯F∗A is defined as the intersection of all AS closed supersets of F∗A. Note that this, ¯F∗A is the smallest AS closed set is containing F∗A.
Example 14 Let us consider the AS topology (˜X,˜τ,E) on the Example 8 and F∗A≅{{x,y}{e1},{z}{e2}} be an AS set over ˜X such that A={e1,e2}⊂E. Then,
Example 15 Let us consider the PAS topology (˜X,˜τ,E) on the Example 10 and F∗A≅{{x,y}{e1},{z}{e2}} be an AS set over X such that A={e1,e2}⊂E. Then,
Proposition 12 Let F∗A be an AS set, ˜τ1 be a PAS topological space and ˜τ2 be an AS topological space on ˜X. Then, ¯F∗A on ˜τ2 is containing ¯F∗A on ˜τ1.
Proof. It is obvious from the Proposition 9 and the Definition 28.
Note that these separation axioms defined as parametric separations in below are different from Ti separation axioms. Ti questions the relationship between the elements of space itself while Pi questions the strength of the connection between their parameters.
Definition 29 Let (˜X,˜τ,E) be an AS topological space and a,b∈E such that a≠b, if there exist x∈X and. AS open sets F∗A and G∗B such that xa˜∈F∗A and xb˜∉F∗A; or xb˜∈G∗B and xa˜∉G∗B, then (˜X,˜τ,E) is called as a P0 space.
Example 16 Let us consider the AS topology (˜X,˜τ,E) on the Example 8.
For e1,e2∈E, there exist x∈X and AS open set {{x,y}{e1},{y}{e2}} such that xe1˜∈{{x,y}{e1},{y}{e2}} and xe2˜∉{{x,y}{e1},{y}{e2}}.
For e1,e3∈E, there exist y∈X and AS open set {{x,z}{e1},{y}{e3}} such that ye3˜∈{{x,z}{e1},{y}{e3}} and ye1˜∉{{x,z}{e1},{y}{e3}}.
For e2,e3∈E, there exist y∈X and AS open set {{y,z}{e2},{z}{e3}} such that ye2˜∈{{y,z}{e2},{z}{e3}} and ye3˜∉{{y,z}{e2},{z}{e3}}.
So (˜X,˜τ,E) is a P0 space from the Definition 29.
Definition 30 Let (˜X,˜τ,E) be an AS topological space and a,b∈E such that a≠b. If there exist x∈X and AS open sets F∗A and G∗B such that xa˜∈F∗A and xb˜∉F∗A; and xb˜∈G∗B and xa˜∉G∗B, then (˜X,˜τ,E) is called as a P1 space.
Example 17 Let us consider the AS topology (˜X,˜τ,E) on the Example 8.
For e1,e2∈E there exist z∈X and AS open sets {{x,z}{e1},{y}{e3}} and {{y,z}{e2},{z}{e3}} such that ze1˜∈{{x,z}{e1},{y}{e3}} and ze2˜∉{{x,z}{e1},{y}{e3}}; and ze2˜∈{{y,z}{e2},{z}{e3}} and ze1˜∉{{y,z}{e2},{z}{e3}}.
For e1,e3∈E there exist y∈X and AS open sets {{x,z}{e1},{y}{e3}} and {{x,y}{e1},{y}{e2}} such that ye3˜∈{{x,z}{e1},{y}{e3}} and ye1˜∉{{x,z}{e1},{y}{e3}}; and ye1˜∈{{x,y}{e1},{y}{e2}} and ye3˜∉{{x,y}{e1},{y}{e2}}.
For e2,e3∈E there exist y∈X and AS open sets {{y,z}{e2},{z}{e3}} and {{x,z}{e1},{y}{e3}} such that ye2˜∈{{y,z}{e2},{z}{e3}} and ye3˜∉{{y,z}{e2},{z}{e3}} and ye3˜∈{{x,z}{e1},{y}{e3}} and ye2˜∉{{x,z}{e1},{y}{e3}}.
So (˜X,˜τ,E) is a P1 space from the Definition 30.
Theorem 1 Let (˜X,˜τ,E) be a P1 space, then it is also a P0 space.
Proof. It is obvious from the Definition 30 and the Definition 29.
Definition 31 Let (˜X,˜τ,E) be an AS topological space and a,b∈E such that a≠b. If there exist x∈X and AS open sets F∗A and G∗B such that xa˜∈F∗A, xb˜∈G∗B and F∗A˜∩G∗B≅˜∅, then (˜X,˜τ,E) is called as a P2 space.
Example 18 Let us consider the AS topology (˜X,˜τ,E) on the Example 8.
For e1,e2∈E, there exist z∈X and AS open sets {{x,z}{e1},{y}{e3}} and {{y,z}{e2},{z}{e3}} such that ze1˜∈{{x,z}{e1},{y}{e3}},ze2˜∈{{y,z}{e2},{z}{e3}} and
But for e1,e3∈E, there aren't any exist x∈X and AS open sets F∗A and G∗B such that xe1˜∈F∗A,xe3˜∈G∗B and F∗A˜∩G∗B≅˜∅.
So (X,τ,E) is not a P2 space.
Theorem 2 Let (˜X,˜τ,E) be a P2 space, then it is also a P1 space.
Proof. Let (\widetilde{{\bf{X}} }, {\widetilde{\mathit{τ }}}, {\bf{E}}) be a {\bf{P}}_{{2}} space and {\bf{a}}, {\bf{b}}\in {\bf{E}} such that {\bf{a}}\ne {\bf{b}} . Then there exist {\bf{x}}\in {\bf{X}} and AS open sets {\bf{F}}{\bf{*}}{\bf{A}} and {\bf{G}}{\bf{*}}{\bf{B}} such that {{\bf{x}}}_{{\bf{a}}}\widetilde{ \in }{\bf{F}}{\bf{*}}{\bf{A}}, {{\bf{x}}}_{{\bf{b}}}\in {\bf{G}}{\bf{*}}{\bf{B}} and {\bf{F}}{\bf{*}}{\bf{A}}\widetilde{\cap} {\bf{G}}{\bf{*}}{\bf{B}} = \widetilde{\emptyset } from the Definition 31. Because of {\bf{F}}{\bf{*}}{\bf{A}}\widetilde{\cap} {\bf{G}}{\bf{*}}{\bf{B}} \cong \widetilde{\emptyset } , there exist AS open sets {\bf{F}}{\bf{*}}{\bf{A}} and {\bf{G}}{\bf{*}}{\bf{B}} clearly that {{\bf{x}}}_{{\bf{a}}}\widetilde{ \in }{\bf{F}}{\bf{*}}{\bf{A}}, {{\bf{x}}}_{{\bf{b}}}\widetilde{\notin}{\bf{F}}{\bf{*}}{\bf{A}} and {{\bf{x}}}_{{\bf{b}}}\widetilde{ \in }{\bf{G}}{\bf{*}}{\bf{B}}, {{\bf{x}}}_{{\bf{a}}}\widetilde{\notin}{\bf{G}}{\bf{*}}{\bf{B}} . This is true for all {\bf{x}}\in {\bf{X}} and then, it is a {\bf{P}}_{{1}} space from the Definition 30.
Theorem 3 Any PAS topological space (\tilde X, \widetilde{ \tau }, E) is a P_{{2}} space.
Proof. Let (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) be a PAS topological space, \boldsymbol{n}\in \mathbb{N} , {\boldsymbol{e}}_{\boldsymbol{n}}\in \boldsymbol{E} . There exist topological spaces (\boldsymbol{X}, {\boldsymbol{\tau }}_{\boldsymbol{n}}) over same initial universe \boldsymbol{X} . And \boldsymbol{f} be mapping from {\boldsymbol{\tau }}_{\boldsymbol{n}}\boldsymbol{t}\boldsymbol{o}{\boldsymbol{\tau }}_{{\boldsymbol{e}}_{\boldsymbol{n}}} for all e \in \boldsymbol{E}; \widetilde{\boldsymbol{\beta } } = \left\{{\boldsymbol{\tau }}_{{\boldsymbol{e}}_{\boldsymbol{n}}}\right\} be an AS basis for a PAS topology \widetilde{ \boldsymbol{\tau } } from the Definition 25. For \boldsymbol{e}_{{1}}, \boldsymbol{e}_{{2}}\in \boldsymbol{E} such that \boldsymbol{e}_{{1}}\ne \boldsymbol{e}_{{2}}. Therefore, there exist AS open sets \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\} and \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\} such that {\boldsymbol{x}}_{\boldsymbol{e}_{{1}}}\widetilde{ \in }\left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\}, {\boldsymbol{x}}_{\boldsymbol{e}_{{2}}}\widetilde{ \in }\left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\} and \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\}\widetilde{\cap} \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\} \cong \widetilde{\emptyset} . This is true for all \boldsymbol{x}\in \boldsymbol{X} and then, (\boldsymbol{X}, \boldsymbol{\tau }, \boldsymbol{E}) is a \boldsymbol{P}_{{2}} space from the Definition 31.
Example 19 Let us consider the PAS topology (\tilde X, \widetilde{ \tau }, E) on the Example 10. It is clearly seen that (\tilde X, \widetilde{ \tau }, E) is a P_{{2}} space from the Definition 31.
Example 20 Let e_{{1}}, e_{{2}}\in E, x\in X and (X, {\tau }_{e_{{1}}}) and (X, {\tau }_{e_{{2}}}) be indiscrete topological spaces over same universe X . And let (\tilde X, \widetilde{ \tau }, E) be their PAS topology. So; \widetilde{ \tau } = \{\widetilde{\emptyset}, \tilde X, \{{X}_{\{e_{{1}}\}}\}, \{{X}_{\{e_{{2}}\}}\left\}\right\} is an AS topological space over \tilde X .
Therefore there exist AS open sets \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\} and \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\} such that {\boldsymbol{x}}_{{\bf{e}}_{{1}}}\widetilde{ \in }\left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\}, \; {\boldsymbol{x}}_{{\bf{e}}_{{2}}}\widetilde{ \in }\left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\} and \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\}\widetilde{\cap} \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\} \cong \widetilde{\emptyset}. So (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is a \boldsymbol{P}_{{2}} space from the Definition 31.
Definition 32 Let (\tilde X, \widetilde{ \tau }, E) be an AS topological space, H*C be an AS closed set, a\in E, x\in X such that {x}_{a}\widetilde{\notin}H*C . If there exist x\in X and AS open sets F*A and G*B such that {x}_{a}\widetilde{ \in }F*A, H*C\widetilde{\subseteq}G*B and F*A\widetilde{\cap} G*B \cong \widetilde{\emptyset} then (\tilde X, \widetilde{ \tau }, E) is called as a Halime space.
Definition 33 Let (\tilde X, \widetilde{ \tau }, E) be an AS topological space. Then it is said to be a P_{{3}} space if it is both a Halime space and a P_{{1}} space.
Example 21 Let us consider the AS topology (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) on the Example 8. Let us see if it is Halime space.
For \boldsymbol{e}_{{1}}\in \boldsymbol{E} , choose {\boldsymbol{x}}_{\boldsymbol{e}_{{1}}}, there exist AS closed sets \boldsymbol{H}\boldsymbol{*}\boldsymbol{C} such as \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}} , {\boldsymbol{X}}_{\{\boldsymbol{e}_{{3}}\}}\} , \{{\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} , \{{\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}, \boldsymbol{e}_{{3}}\}}\} , \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{3}}\}}\} such that {\boldsymbol{x}}_{\boldsymbol{e}_{{1}}}\notin \boldsymbol{H}\boldsymbol{*}\boldsymbol{C}. And AS open set of super set of each \boldsymbol{H}\boldsymbol{*}\boldsymbol{C} is only \mathit{\boldsymbol{\tilde X}} . So there is not any exist AS open set \boldsymbol{F}\boldsymbol{*}\boldsymbol{A} such that {\boldsymbol{x}}_{\boldsymbol{e}_{{1}}}\widetilde{ \in }\boldsymbol{F}\boldsymbol{*}\boldsymbol{A} and \boldsymbol{F}\boldsymbol{*}\boldsymbol{A}\widetilde{\cap} \mathit{\boldsymbol{\tilde X}} \cong \widetilde{\emptyset}.
This situation is similarly for {\boldsymbol{y}}_{\boldsymbol{e}_{{1}}} and {\boldsymbol{z}}_{\boldsymbol{e}_{{1}}} . So (\boldsymbol{X}, \boldsymbol{\tau }, \boldsymbol{E}) is not a Halime space.
Example 22 Let X = \{x, y, z\} be a universal set, E = \{e_{{1}}, e_{{2}}, e_{{3}}\} be a parameter set,
\widetilde{ \tau } = \{\widetilde{\emptyset}, \tilde X, \{{\{x, y\}}_{\{e_{{1}}\}}, {\{x, z\}}_{\{e_{{2}}\}}, {\{y, z\}}_{\{e_{{3}}\}}\}, \{{\left\{z\right\}}_{\{e_{{1}}\}}, {\left\{y\right\}}_{\{e_{{2}}\}}, {\left\{x\right\}}_{\{e_{{3}}\}}\left\}\right\} such that every members an amply soft set E\to P\left(X\right) . Then (\tilde X, \widetilde{ \tau }, E) is an AS topological space. Let us see if it is a P_{{3}} space.
Firstly, let us see if it is a Halime space.
For \boldsymbol{e}_{{1}}\in \boldsymbol{E} , choose {\boldsymbol{x}}_{{\bf{e}}_{{1}}} and for an AS closed set \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\} such that {\boldsymbol{x}}_{{\bf{e}}_{{1}}}\widetilde{\notin}\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} . There exists an AS open set \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} such that \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\left\}\widetilde{\subseteq}\right\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\left\}\right\} . And there exists an AS open set \{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} such that {\boldsymbol{x}}_{{\bf{e}}_{{1}}}\widetilde{ \in }\{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} and \{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\}\widetilde{\cap} \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} \cong \widetilde{\emptyset} .
For \boldsymbol{e}_{{2}}\in \boldsymbol{E} , choose {\boldsymbol{x}}_{\boldsymbol{e}_{{2}}} and for an AS closed set \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} such that {\boldsymbol{x}}_{\boldsymbol{e}_{{2}}}\widetilde{\notin}\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} . There exists an AS open set \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} such that \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\left\}\widetilde{\subseteq}\right\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\left\}\right\} . And there exists an AS open set \{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} such that {\boldsymbol{x}}_{\boldsymbol{e}_{{2}}}\widetilde{ \in }\{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} and \{{\left\{\boldsymbol{x}, \boldsymbol{y}\right\}}_{\left\{{\boldsymbol{e}}^{1}\right\}}, {\left\{\boldsymbol{x}, \boldsymbol{z}\right\}}_{\left\{{\boldsymbol{e}}^{2}\right\}}, {\left\{\boldsymbol{y}, \boldsymbol{z}\right\}}_{\left\{{\boldsymbol{e}}^{3}\right\}}\}\widetilde{\cap} \{{\left\{\boldsymbol{z}\right\}}_{\left\{{\boldsymbol{e}}^{1}\right\}}, {\left\{\boldsymbol{y}\right\}}_{\left\{{\boldsymbol{e}}^{2}\right\}}, {\left\{\boldsymbol{x}\right\}}_{\left\{{\boldsymbol{e}}^{3}\right\}}\}\} \cong \widetilde{\emptyset}.
For \boldsymbol{e}_{{3}}\in \boldsymbol{E} , choose {\boldsymbol{z}}_{\boldsymbol{e}_{{3}}} and for an AS closed set \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} such that {\boldsymbol{z}}_{\boldsymbol{e}_{{3}}}\widetilde{\notin}\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\}. There exists an AS open set \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} such that \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\left\}\widetilde{\subseteq}\right\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\left\}\right\} . And there exists an AS open set \{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} such that {\boldsymbol{z}}_{\boldsymbol{e}_{{3}}}\widetilde{ \in }\{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} and \{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\}\widetilde{\cap} \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} \cong \widetilde{\emptyset},
So (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is a Halime space from the Definition 32.
Finally, let us see if it is a \boldsymbol{P}_{{1}} space.
For \boldsymbol{e}_{{1}}, \boldsymbol{e}_{{2}}\in \boldsymbol{E} , there exist \boldsymbol{y}\in \boldsymbol{X} and AS open sets \{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} and \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} such that {\boldsymbol{y}}_{\boldsymbol{e}_{{1}}}\widetilde{ \in }\{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} and {\boldsymbol{y}}_{\boldsymbol{e}_{{2}}}\widetilde{\notin}\{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} ; and {\boldsymbol{y}}_{\boldsymbol{e}_{{2}}}\in \boldsymbol{ }\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} and {\boldsymbol{y}}_{\boldsymbol{e}_{{1}}}\widetilde{\notin}\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\}.
For \boldsymbol{e}_{{1}}, \boldsymbol{e}_{{3}}\in \boldsymbol{E} , there exist \boldsymbol{x}\in \boldsymbol{X} and AS open sets \{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} and \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} such that {\boldsymbol{x}}_{\boldsymbol{e}_{{1}}}\widetilde{ \in }\{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} and {\boldsymbol{x}}_{\boldsymbol{e}_{{3}}}\widetilde{\notin}\{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} ; and {\boldsymbol{x}}_{\boldsymbol{e}_{{3}}}\widetilde{ \in }\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} and {\boldsymbol{x}}_{\boldsymbol{e}_{{1}}}\widetilde{\notin}\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} .
For \boldsymbol{e}_{{2}}, \boldsymbol{e}_{{3}}\in \boldsymbol{E} , there exist \boldsymbol{y}\in \boldsymbol{X} and AS open sets \{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} and \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} such that {\boldsymbol{y}}_{\boldsymbol{e}_{{3}}}\widetilde{ \in }\{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} and {\boldsymbol{y}}_{\boldsymbol{e}_{{2}}}\widetilde{\notin}\{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} ; and {\boldsymbol{y}}_{\boldsymbol{e}_{{2}}}\widetilde{ \in }\{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} and {\boldsymbol{y}}_{\boldsymbol{e}_{{3}}}\notin \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} .
Therefore, (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is a \boldsymbol{P}_{{1}} space from the Definition 19. (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is a \boldsymbol{P}_{{3}} space because it is both a Halime space and a \boldsymbol{P}_{{1}} space.
Theorem 4 Let (\tilde X, \widetilde{ \tau }, E) be a P_{{3}} space, then it is also a P_{{2}} space.
Proof. Let (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) be a \boldsymbol{P}_{{3}} space. Then it is both a \boldsymbol{P}_{{1}} and a Halime space from the Definition 33.
Because \widetilde{\boldsymbol{X} }, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is a Halime space, for an AS closed set \boldsymbol{H}\boldsymbol{*}\boldsymbol{C} and \boldsymbol{a}\in \boldsymbol{E}, \boldsymbol{ }\boldsymbol{x}\in \boldsymbol{X} such that {\boldsymbol{x}}_{\boldsymbol{a}}\widetilde{\notin}\boldsymbol{H}\boldsymbol{*}\boldsymbol{C} , there exist AS open sets \boldsymbol{F}\boldsymbol{*}\boldsymbol{A} and \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} such that {\boldsymbol{x}}_{\boldsymbol{a}}\widetilde{ \in }\boldsymbol{F}\boldsymbol{*}\boldsymbol{A}, \boldsymbol{ }\boldsymbol{H}\boldsymbol{*}\boldsymbol{C}\widetilde{\subseteq}\boldsymbol{G}\boldsymbol{*}\boldsymbol{B} and \boldsymbol{F}\boldsymbol{*}\boldsymbol{A}\widetilde{\cap} \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} \cong \widetilde{\emptyset} . So from last equality it is seen that {\boldsymbol{x}}_{\boldsymbol{a}}\widetilde{ \in }\boldsymbol{F}\boldsymbol{*}\boldsymbol{A}, {\boldsymbol{x}}_{\boldsymbol{a}}\widetilde{\notin}\boldsymbol{G}\boldsymbol{*}\boldsymbol{B} . Because (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is also a P_{{1}} space and \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} is an AS open set, there exist {\boldsymbol{x}}_{\boldsymbol{b}}\widetilde{ \in }\boldsymbol{G}\boldsymbol{*}\boldsymbol{B} such that \boldsymbol{b}\in \boldsymbol{E} . And now on, We can see that clearly, there exist AS open sets \boldsymbol{F}\boldsymbol{*}\boldsymbol{A} and \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} such that {\boldsymbol{x}}_{\boldsymbol{a}}\widetilde{ \in }\boldsymbol{F}\boldsymbol{*}\boldsymbol{A}, {\boldsymbol{x}}_{\boldsymbol{b}}\widetilde{ \in }\boldsymbol{G}\boldsymbol{*}\boldsymbol{B} and \boldsymbol{F}\boldsymbol{*}\boldsymbol{A}\widetilde{\cap} \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} \cong \widetilde{\emptyset} so (\boldsymbol{X}, \boldsymbol{\tau }, \boldsymbol{E}) is a \boldsymbol{P}_{{2}} space, from the Definition 31.
Definition 34 Let (\tilde X, \widetilde{ \tau }, E) be an AS topological space, H*C and K*D be AS closed sets such that H*C\widetilde{\cap} K*D = \widetilde{\emptyset} . If there exist AS open sets F*A and G*B such that H*C\widetilde{\subseteq}F*A, K*D\widetilde{\subseteq}G* B and F*A\widetilde{\cap} G*B \cong \widetilde{\emptyset} then (\tilde X, \widetilde{ \tau }, E) is called as an Orhan space.
Definition 35 Let (\tilde X, \widetilde{ \tau }, E) be an AS topological space. Then it is said to be a P_{{4}} space if it is both an Orhan space and a P_{{1}} space.
Example 23 Let us consider the AS topology (\tilde X, \widetilde{ \tau }, E) on the Example 22. Let us see if it is a P_{{4}} space.
\{{\{\boldsymbol{x}, \boldsymbol{y}\}}_{\{\boldsymbol{e}_{{1}}\}}, {\{\boldsymbol{x}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{2}}\}}, {\{\boldsymbol{y}, \boldsymbol{z}\}}_{\{\boldsymbol{e}_{{3}}\}}\} and \{{\left\{\boldsymbol{z}\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {\left\{\boldsymbol{y}\right\}}_{\{\boldsymbol{e}_{{2}}\}}, {\left\{\boldsymbol{x}\right\}}_{\{\boldsymbol{e}_{{3}}\}}\}\} are disjoint AS closed sets on it. And we know that these are also disjoint AS open sets. So (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is an Orhan Space. And also (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is a \boldsymbol{P}_{{1}} space from the Example 22, so (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is a \boldsymbol{P}_{{4}} space from the Definition 35.
Example 24 Let us consider the PAS topology on the Example 20. Let us see if it is a P_{{4}} space.
Firstly, let's see if it is an Orhan space. There exist AS closed sets \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\} and \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\} such that \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\}\widetilde{\cap} \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\} \cong \widetilde{\emptyset}. And we know that \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\} and \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\} are also AS open sets such that \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\}\widetilde{\subseteq}\left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\}, \boldsymbol{ }\left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\left\}\widetilde{\subseteq}\right\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\left\}\right\} and \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\}\widetilde{\cap} \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\} \cong \widetilde{\emptyset} . So (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is an Orhan space.
Finally, let's see if it is a \boldsymbol{P}_{{1}} space.
There exist AS open sets \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\} and \left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\} such that {\boldsymbol{x}}_{{\bf{e}}_{{1}}}\widetilde{ \in }\left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\} , {\boldsymbol{x}}_{{{\bf{e}}}_{2}}\widetilde{\notin}\left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{1}}\}}\right\} and {\boldsymbol{x}}_{{\bf{e}}_{{2}}}\widetilde{ \in }\left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\}, \; {\boldsymbol{x}}_{{{\bf{e}}}_{1}}\widetilde{\notin}\left\{{\boldsymbol{X}}_{\{\boldsymbol{e}_{{2}}\}}\right\}. So (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is a \boldsymbol{P}_{{1}} space. Because (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is both an Orhan space and a \boldsymbol{P}_{{1}} space, it is a \boldsymbol{P}_{{4}} space from the Definition 35.
Proposition 13 Any PAS topological space (\tilde X, \widetilde{ \tau }, E) may not be a P_{{4}} space.
Example 25 Let \mathbb{R} be real numbers, E = \{e_{{1}}, e_{{2}}\} be a universal parameter set, (\mathbb{R}, \tau _{{1}}) a discrete topological space and (\mathbb{R}, \tau _{{2}}) a finite complement topological space and from the Definition 25, their PAS topology over \widetilde{\mathbb{R}} \; (\widetilde{\mathbb{R}}, \widetilde{ \tau }, E).
Now let us see if \widetilde{\mathbb{R}} is a \boldsymbol{P}_{{4}} space.
Firstly, we can say that the PAS topology is a \boldsymbol{P}_{{1}} space because of the Theorem 3 and the Theorem 2. Let us see it,
For \boldsymbol{e}_{{1}}, \boldsymbol{ }\boldsymbol{e}_{{2}}; there exist \bf 2\in \mathbb{R} and \{{\left\{\bf 2\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {(\mathbb{R}-\{\mathrm{\bf 2, 3}, \bf 5\left\}\right)}_{\{\boldsymbol{e}_{{2}}\}}\}, \boldsymbol{ }\boldsymbol{ }\{{\left\{\bf 3\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {(\mathbb{R}-\{\mathrm{\bf 3, 5}\left\}\right)}_{\{\boldsymbol{e}_{{2}}\}}\}\widetilde{ \in }\widetilde{ \boldsymbol{\tau } } such that {\bf 2}_{\boldsymbol{e}_{{1}}}\widetilde{ \in }\{{\left\{\bf 2\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {(\mathbb{R}-\{\mathrm{\bf 2, 3}, \bf 5\left\}\right)}_{\{\boldsymbol{e}_{{2}}\}}\} and {\bf 2}_{\boldsymbol{e}_{{2}}}\widetilde{\notin}\{{\left\{\bf 2\right\}}_{\{\boldsymbol{e}_{{1}}\}}, {(\mathbb{R}-\{\mathrm{\bf 2, 3}, \bf 5\left\}\right)}_{\{\boldsymbol{e}_{{2}}\}}\} ; {\bf 2}_{\boldsymbol{e}_{{2}}}\widetilde{ \in }\{{\left\{\bf 3\right\}}_{\{\boldsymbol{e}_{{1}}\}}, (\mathbb{R}-{3, 5}){e2}} and 2{e1}∉{{3}{e1}, (\mathbb{R}-{3, 5}){e2}}. Therefore, it is clearly seen that (\widetilde{\mathbb{R}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is a \boldsymbol{P}_{{1}} space from the Definition 30.
Finally, let us see if \widetilde{\mathbb{R}} is an Orhan space. Let \boldsymbol{U}, \boldsymbol{V}\widetilde{\subseteq}\mathbb{R} be finite sets. Then we can choose \left\{{\boldsymbol{U}}_{\{\boldsymbol{e}_{{2}}\}}\right\} and \left\{{\boldsymbol{V}}_{\{\boldsymbol{e}_{{2}}\}}\right\} AS closed sets such that \left\{{\boldsymbol{U}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\widetilde{\cap} \left\{{\boldsymbol{V}}_{\{\boldsymbol{e}_{{2}}\}}\right\} \cong \widetilde{\emptyset} But for \boldsymbol{A}, \boldsymbol{B}\subseteq \boldsymbol{E} , we can't find any AS open sets \boldsymbol{F}\boldsymbol{*}\boldsymbol{A} and \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} such that \left\{{\boldsymbol{U}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\widetilde{\subseteq}\boldsymbol{F}\boldsymbol{*}\boldsymbol{A}, \boldsymbol{ }\boldsymbol{ }\left\{{\boldsymbol{V}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\widetilde{\subseteq}\boldsymbol{G}\boldsymbol{*}\boldsymbol{B} and \boldsymbol{F}\boldsymbol{*}\boldsymbol{A}\widetilde{\cap} \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} \cong \widetilde{\emptyset}. For this purpose, suppose that, there exist \left\{{(\boldsymbol{R}-\boldsymbol{U})}_{\{\boldsymbol{e}_{{2}}\}}\right\}, \boldsymbol{ }\boldsymbol{ }\left\{{(\boldsymbol{R}-\boldsymbol{V})}_{\{\boldsymbol{e}_{{2}}\}}\right\} be AS open sets such that \left\{{\boldsymbol{V}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\widetilde{\subseteq}\left\{{(\boldsymbol{R}-\boldsymbol{U})}_{\{\boldsymbol{e}_{{2}}\}}\right\} , \left\{{\boldsymbol{U}}_{\{\boldsymbol{e}_{{2}}\}}\right\}\widetilde{\subseteq}\left\{{(\boldsymbol{R}-\boldsymbol{V})}_{\{\boldsymbol{e}_{{2}}\}}\right\} and \left\{(\boldsymbol{R}-\boldsymbol{U})_{\left\{\boldsymbol{e}_{2}\right\}}\right\} \widetilde{\cap}\{(\boldsymbol{R}- V){e2}} = Ø. Therefore, we can obtain U∪V = \mathbb{R}. This result contradicts the finite selection of \boldsymbol{U} and \boldsymbol{V} . Hence, (\widetilde{\mathbb{R}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is not an Orhan space and so it is not a \boldsymbol{P}_{{4}} space.
Theorem 5 Let (\tilde X, \widetilde{ \tau }, E) be a P_{{4}} space, then it is also a P_{{3}} space.
Proof. Let (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) be a \boldsymbol{P}_{{4}} space. Then it is both a \boldsymbol{P}_{{1}} and an Orhan space from the Definition 35. Because (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is an Orhan space, for AS closed sets \boldsymbol{H}\boldsymbol{*}\boldsymbol{C} and \boldsymbol{K}\boldsymbol{*}\boldsymbol{D} such that \boldsymbol{H}\boldsymbol{*}\boldsymbol{C}\widetilde{\cap} \boldsymbol{K}\boldsymbol{*}\boldsymbol{D} \cong \widetilde{\emptyset}, there exist AS open sets \boldsymbol{F}\boldsymbol{*}\boldsymbol{A} and \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} such that \boldsymbol{H}\boldsymbol{*}\boldsymbol{C}\widetilde{\subseteq}\boldsymbol{F}\boldsymbol{*}\boldsymbol{A}, \boldsymbol{ }\boldsymbol{K}\boldsymbol{*}\boldsymbol{D}\widetilde{\subseteq}\boldsymbol{G}\boldsymbol{*}\boldsymbol{B} and \boldsymbol{F}\boldsymbol{*}\boldsymbol{A}\cap \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} = {\emptyset } . For this,
1. Let AS closed set \boldsymbol{H}\boldsymbol{*}\boldsymbol{C} = {\emptyset }, then for \boldsymbol{x}\in \boldsymbol{X}, \boldsymbol{a}\in \boldsymbol{E}; {\boldsymbol{x}}_{\boldsymbol{a}}\widetilde{\notin}\boldsymbol{H}\boldsymbol{*}\boldsymbol{C} and for {\boldsymbol{x}}_{\boldsymbol{a}}\widetilde{ \in }\boldsymbol{G}\boldsymbol{*}\boldsymbol{B} , there exist \boldsymbol{F}\boldsymbol{*}\boldsymbol{A} and \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} such that \boldsymbol{H}\boldsymbol{*}\boldsymbol{C}\widetilde{\subseteq}\boldsymbol{F}\boldsymbol{*}\boldsymbol{A}, \boldsymbol{ }\boldsymbol{K}\boldsymbol{*}\boldsymbol{D}\widetilde{\subseteq}\boldsymbol{G}\boldsymbol{*}\boldsymbol{B} and \boldsymbol{F}\boldsymbol{*}\boldsymbol{A}\widetilde{\cap} \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} \cong \widetilde{\emptyset}, so (\boldsymbol{X}, \boldsymbol{\tau }, \boldsymbol{E}) is a Halime space from the Definition 32.
2. Let AS closed set \boldsymbol{H}\boldsymbol{*}\boldsymbol{C}\widetilde{\ne}\widetilde{\emptyset}, then there exist {\boldsymbol{x}}_{\boldsymbol{a}}\widetilde{ \in }\boldsymbol{H}\boldsymbol{*}\boldsymbol{C} and then there exist AS closed set \boldsymbol{K}\boldsymbol{*}\boldsymbol{D} such that {\boldsymbol{x}}_{\boldsymbol{a}}\widetilde{\notin}\boldsymbol{K}\boldsymbol{*}\boldsymbol{D} because \boldsymbol{H}\boldsymbol{*}\boldsymbol{C}\widetilde{\cap} \boldsymbol{K}\boldsymbol{*}\boldsymbol{D} \cong \widetilde{\emptyset}. Then (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is an Orhan space, so there exist AS open sets \boldsymbol{F}\boldsymbol{*}\boldsymbol{A} and \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} such that \boldsymbol{H}\boldsymbol{*}\boldsymbol{C}\widetilde{\subseteq}\boldsymbol{F}\boldsymbol{*}\boldsymbol{A} , \boldsymbol{K}\boldsymbol{*}\boldsymbol{D}\widetilde{\subseteq}\boldsymbol{G}\boldsymbol{*}\boldsymbol{B} and \boldsymbol{F}\boldsymbol{*}\boldsymbol{A}\widetilde{\cap} \boldsymbol{G}\boldsymbol{*}\boldsymbol{B} \cong \widetilde{\emptyset}. Therefore (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is also a Halime space. And since (\mathit{\boldsymbol{\tilde X}}, \widetilde{ \boldsymbol{\tau } }, \boldsymbol{E}) is both a Halime and a \boldsymbol{P}_{{1}} space, it is a \boldsymbol{P}_{{3}} space from the Definition 33.
Corolary 1 Any AS topological space (\tilde X, \widetilde{ \tau }, E) is a P_{{4}} space \Rightarrow P_{{3}} space \Rightarrow P_{{2}} space \Rightarrow P_{{1}} space \Rightarrow P_{{0}} space.
Proof. It is clearly seen that from the Theorem 5, the Theorem 4, the Theorem 2 and the Theorem 1 respectively.
4.
Conclusions
I defined an amply soft set. Amply soft sets use any kind of universal parameter set and initial universe (such as finite or infinite, countable or uncountable). Also, I introduced subset, superset, equality, empty set, and whole set about amply soft sets. And I gave operations such as union, intersection, difference of two amply soft sets and complement of an amply soft set. Then three different amply soft point such as amply soft whole point, amply soft point, and monad point were defined. Also examples related taking universal set as uncountable were given.
I defined a new soft topology, and it is called as a PAS topology. The PAS topology allows to write different elements of classical topologies in its each parameter sets. The classical topologies may be finite, infinite, countable or uncountable. This situation removes all of the boundaries in a soft topology and cause it to spread over larger areas. A PAS topology is a special case of an AS topology. For this purpose, I defined a new soft topology, and it is called as an amply soft topology or briefly an AS topology. I introduce AS open sets, AS closed sets, interior and closure of an AS set and subspace of any AS topological space. I gave parametric separation axioms which are different from Ti separation axioms. Ti questions the relationship between the elements of space itself while Pi questions the strength of the connection between their parameters.
In our next study, we will touch on the concept of a monad metrizable space [46] which we took the preliminary step in this study. A space concept in monad metrizable space will gain a new perspective. I will compute distance in real space between different topologies or metric spaces in different dimensions. First of all, I will define passing points between different topologies. And I will define monad metric.
Conflict of interest
The author declares that there is no conflict of interest regarding the publication of this paper.















 DownLoad:
DownLoad: