|
[1]
|
P. Gabriel, S. P. Garbett, V. Quaranta, D. R. Tyson, G. F. Webb, The contribution of age structure to cell population responses to targeted therapeutics, J. Theor. Biol., 311 (2012), 19-27.
|
|
[2]
|
S. S. Hamed, R. M. Straubinger, W. J. Jusko, Pharmacodynamic modeling of cell cycle and apoptotic effects of gemcitabine on pancreatic adenocarcinoma cells, Cancer Chemother Pharmacol, 72 (2013), 553-563.
|
|
[3]
|
X. Miao, G. Koch, S. Ait-Oudhia, R. M. Straubinger, W. J. Jusko, Pharmacodynamic modeling of cell cycle effects for gemcitabine and trabected in combinations in pancreatic cancer cells, Front. Pharmacol., 7 (2016), 421.
|
|
[4]
|
J. A. Alberts Bruce, Molecular Biology of the Cell: a Problems Approach, 4th edition, Garland Science, New York, NY, 2002.
|
|
[5]
|
E. S. Wenzel, A. T. Singh, Cell-cycle checkpoints and aneuploidy on the path to cancer, In Vivo, 32 (2018), 1-5.
|
|
[6]
|
A. Zetterberg, O. Larsson, K. G. Wiman, What is the restriction point?, Curr. Opin. Cell Biol., 7 (1995), 835-842.
|
|
[7]
|
C. Schwarz, A. Johnson, M. K. oivomägi, E. Zatulovskiy, C. J. Kravitz, A. Doncic, et al., A precise Cdk activity threshold determines passage through the restriction point, Mol. Cell, 69 (2018), 253-264.
|
|
[8]
|
A. Zilman, V. Ganusov, A. Perelson, Stochastic models of lymphocyte proliferation and death, PLoS One, 5 (2010), e12775.
|
|
[9]
|
A. V. Gett, P. D. Hodgkin, A cellular calculus for signal integration by T cells, Nature, 1 (2000), 239-244.
|
|
[10]
|
K. Léon, J. Faro, J. Caneiro, A general mathematical framework to model generation structure in a population of asynchronously dividing cells, J. Theor. Biol., 229 (2004), 455-476.
|
|
[11]
|
R. Callard, P. Hodgkin, Modeling T- and B-cell growth and differentiation, Immunol. Rev., 216 (2007), 119-129.
|
|
[12]
|
J. A. Smith, L. Martin, Do cells cycle?, Proc. Natl. Acad. Sci. U.S.A., 70 (1973), 1263-1267.
|
|
[13]
|
A. Golubev, Exponentially modified Gaussian (EMG) relevance to distributions related to cell proliferation and differentiation, J. Theor. Biol., 262 (2010), 257-266.
|
|
[14]
|
A. Golubev, Genes at work in random bouts, BioEssays, 34 (2012), 311-319.
|
|
[15]
|
A. Golubev, Applications and implications of the exponentially modified gamma distribution as a model for time variabilities related to cell proliferation and gene expression, J. Theor. Biol., 393 (2016), 203-217.
|
|
[16]
|
S. J. Cain, P. C. Chau, Transition probability cell cycle model part I-balanced growth, J. Theor. Biol., 185 (1997), 55-67.
|
|
[17]
|
S. Svetina, B. Žekš, Transition probability model of the cell cycle exhibiting the age-distribution for cells in the indeterministic state of the cell cycle, in Biomathematics and Cell Kinetics (eds. A. J. Valleron and P. D. M. MacDonald), Elsevier/North-Holland Biomedical Press, New York, 1978, 71-82.
|
|
[18]
|
S. Cooper, The continuum model: statistical implications, J. Theor. Biol., 94 (1982), 783-800.
|
|
[19]
|
S. Banerjee, K. Lo, M. K. Daddysman, A. Selewa, T. Kuntz, A. R. Dinner, et al., Biphasic growth dynamics during Caulobacter crescentus division, bioRxiv, (2017), 047589.
|
|
[20]
|
Z. W. Jones, R. Leander, V. Quaranta, L. A. Harris, D. R. Tyson, A drift-diffusion checkpoint model predicts a highly variable and growth-factor-sensitive portion of the cell cycle g1 phase, PLOS ONE, 13 (2018), 1-20.
|
|
[21]
|
J. Folks, R. S. Chikara, The inverse Gaussian distribution and its statistical application-a review, J. R. Statist. Soc. B, 40 (1978), 263-289.
|
|
[22]
|
O. Angulo, J. López-Marcos, Numerical integration of nonlinear size-structured population equations, Ecol. Model., 133 (2000), 3-14.
|
|
[23]
|
S. C. Tate, S. Cai, R. T. Ajamie, T. Burke, R. P. Beckmann, E. M. Chan, et al., Semi-mechanistic pharmacokinetic/pharmacodynamic modeling of the antitumor activity of LY2835219, a new cyclin-dependent kinase 4/6 inhibitor, in mice bearing human tumor xenografts, Clin. Cancer Res., 20 (2014), 3763-3774.
|
|
[24]
|
R. R. Goldberg, Methods of Real Analysis, John Wiley & Sons, Hoboken, NJ, 1976.
|
|
[25]
|
K. Ito, F. Kappel, G. Peichel, A fully discretized approximation scheme for size-structured population models, SIAM J. Numer. Anal., 28 (1991), 923-954.
|
|
[26]
|
C. C. Pugh, Undergraduate texts in mathematics, in Real Mathematical Analysis (eds. S. Axler, F. Gehring and K. Ribet), vol. 19, Springer International Publishing, New York, NY, 2003.
|
|
[27]
|
Wikipedia, Leibniz integral rule, 2019. Available from: https://en.wikipedia.org/wiki/Leibniz_integral_rule.
|













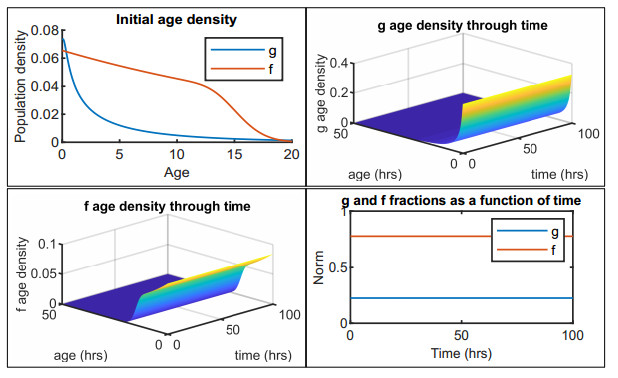
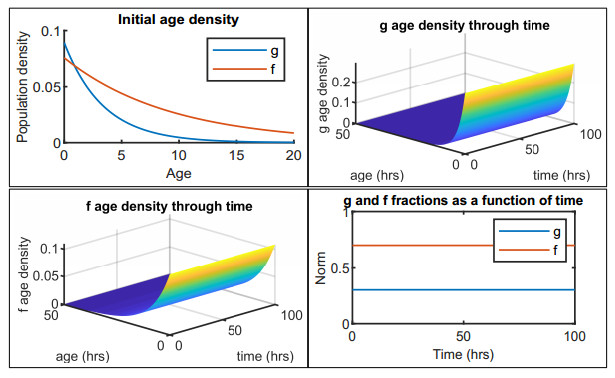
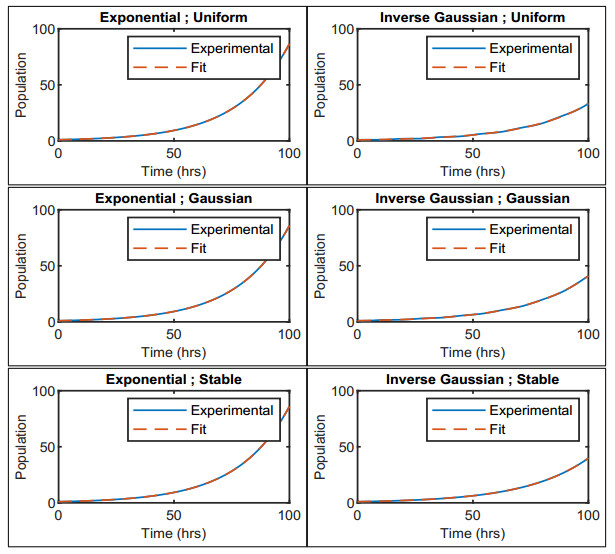

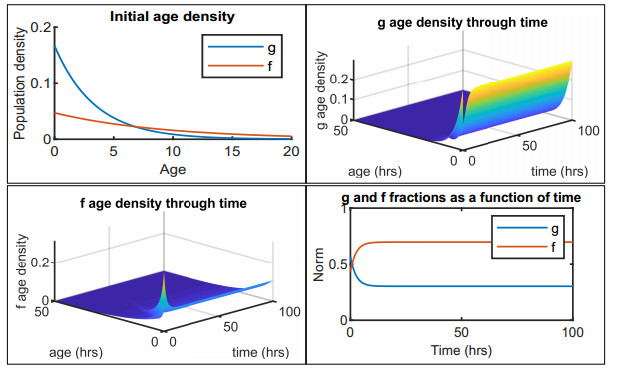


 DownLoad:
DownLoad: