Biochemically failing metastatic prostate cancer is typically treated with androgen ablation. However, due to the emergence of castration-resistant cells that can survive in low androgen concentrations, such therapy eventually fails. Here, we develop a partial differential equation model of the growth and response to treatment of prostate cancer that has metastasized to the bone. Existence and uniqueness results are derived for the resulting free boundary problem. In particular, existence and uniqueness of solutions for all time are proven for the radially symmetric case. Finally, numerical simulations of a tumor growing in 2-dimensions with radial symmetry are carried in order to evaluate the therapeutic potential of different treatment strategies. These simulations are able to reproduce a variety of clinically observed responses to treatment, and suggest treatment strategies that may result in tumor remission, underscoring our model's potential to make a significant contribution in the field of prostate cancer therapeutics.
1.
Introduction
Biological dispersal means that organisms move from one location to another within a habitat. This phenomenon has an important impact on population dynamics, disease and distribution of species [1,2,3,4]. It is well known that the interactions between dispersal and spatial heterogeneity could create very interesting phenomena. Moreover, a number of ecologists and evolutionary biologists have been interested in this issue for many years.
Over the last few decades, researchers from both biology and mathematics have used some specific mathematical models to describe population dynamics in spatial ecology and evolution. On one hand, taking into account the effects of random perturbations, some researchers investigated some models which described more realistically ecosystems through stochastic approaches, see [5,6,7,8,9]. On the other hand, some researchers considered the impacts of the random diffusion on the population dynamics and used the reaction-diffusion equations to model population dynamics. Among these models, the two species Lotka-Volterra competition-diffusion system perhaps is the most salient example. The following classical Lotka-Volterra competition-diffusion system
models two competing species. Here $ u(x, t) $ and $ v(x, t) $ represent the densities of two species at location $ x $ and time $ t $. $ d_{1} $ and $ d_{2} $ are random diffusion rates of species $ u $ and $ v $, respectively, which are therefore assumed to be non-negative. The habitat $ \Omega $ is a bounded domain in $ \mathbb{R}^{N} $ with smooth boundary $ \partial\Omega $. $ \partial_{\nu} = \nu\cdot\nabla $, where $ \nu $ denotes the unit outer normal vector on $ \partial\Omega $ and the no-flux boundary condition means that no individual can move in or out through the boundary of the habitat. $ m $ represents their common intrinsic growth rate which also reflects the environmental richness of the resources at location $ x $. $ b $ and $ c $ represent inter-specific competition coefficients with $ (b, c) \in (0, 1)\times(0, 1) $ and both intra-specific competition coefficients are normalized to 1.
Let $ g $ belong to $ \mathcal{C}^{\gamma}(\overline{\Omega}) $ for some $ \gamma $ satisfying $ 0 < \gamma < 1 $ with $ \int_{\Omega}gdx\geq0 $ and $ g\not\equiv0 $. It is well known that there is a unique positive solution to the following problem
which is denoted by $ \varpi_{d, g} $ (see, e.g., [1]). This implies that system (1.1) admits two semi-trivial steady states, denoted by $ (\omega_{d_{1}}, 0) $ and $ (0, \omega_{d_{2}}) $, for every $ d_1 > 0 $ and $ d_2 > 0 $.
For system (1.1), when $ b = c = 1 $, one well-known and widely accepted result is due to Hastings [10]. It was shown by Hastings [10] that the species with slower diffusion rate has advantage against its competitor with larger diffusion rate, regardless of the initial values, which is contrary to the case of $ m\equiv $ constant. When $ m\equiv $ constant, there is a compact global attractor consisting of a continuum of steady states $ \{((1-s)m, sm)|s\in[0, 1]\} $ connecting the two semi-trivial steady states to system (1.1) for every $ d_{1}, d_{2} > 0 $.
In an attempt to understand this phenomenon, Lou [11] adopted the weak competition approach to study the system (1.1). Lou [11] verified that there exists a critical value for competition coefficient $ c_{*} $ in $ (0, 1) $ such that (ⅰ) for every $ c $ less than this critical value, if $ d_{1}, d_{2} > 0 $, the steady state $ (\varpi_{d_{1}}, 0) $ is unstable; (ⅱ) for every $ c $ satisfying $ c_{*} < c < 1 $, there exists $ \bar{d_{1}} > 0 $ and $ \bar{d_{2}} > 0 $ such that $ (\varpi_{d_{1}}, 0) $ is unstable. Meanwhile, when $ c $ is in $ (c_{*}, 1) $, there exists a constant $ b_{*} $ in $ (0, 1] $ such that for some $ (d_{1}, d_{2}) $ in $ (0, +\infty)\times(0, +\infty) $, $ (\varpi_{d_{1}}, 0) $ is globally asymptotically stable provided that $ b $ satisfies $ 0 < b\leq b_{*} $. He and Ni [12] made a great breakthrough on the estimate of linear stability of any coexistence stead state, and based on this, they provided a complete classification on all possible long time dynamical behaviors of system (1.1). We refer to [13,14,15,16] for more investigations.
In addition to random dispersal, it is quite reasonable to suppose that species could take directed movements due to certain external environment force or self-propelling in some environments. On one hand, species will move downstream due to the unidirectional water flow in a river [17,18,19]. On the other hand, the individuals are very smart so that they can sense and follow gradients in resource distribution [20,21,22,23] which can be modeled by the following competition-diffusion-advection system
where $ d_1 $ and $ d_2 $ are random diffusion rates of species $ u $ and $ v $, respectively. $ \alpha $ and $ \beta $ measure the speed of movement upward along the gradient of resources. $ m_{1} $ and $ m_{2} $ are intrinsic growth rates of $ u $ and $ v $, respectively. The boundary condition means that the environment is isolated. This system has been frequently used as a standard model to study the evolution of conditional dispersal and a number of related works have been done, see, e.g., [24,25,26] for $ \alpha, \beta > 0 $, [27,28,29] for $ \alpha > 0 = \beta $ when $ m_{1} = m_{2} $ and $ b = c = 1 $. For more investigations, we refer to [30,31,32,33,34].
Recently, Wang [21] studied system (1.3), and he established $ \Sigma_{u} $ and $ \Sigma_{v} $ (which can be seen in the following definition) for $ m_1 $ and $ m_2 $ are non-constant under $ d_{1}, d_{2}, \alpha $ and $ \beta $ satisfying certain conditions and $ (b, c) $ in $ (0, 1]\times(0, 1] $. Xu and Jiang [22] investigated the dynamics of system (1.3) on the condition that $ m_1 $ is not a constant, $ m_{2} = \overline{m} $ is a constant ($ \nabla\overline{m}\equiv0 $) and $ b = c = 1 $. In this case, advection rate $ \beta $ has no effect on system (1.3). They showed that there exists a critical value for the diffusion rate $ d_{2}^{*} $ in $ (0, +\infty) $, and their results can be interpreted biologically in the following statements:
$ (i) $ The species $ u $ can always invade when the species $ v $ is rare for every $ d_1, d_2 > 0 $, and $ \alpha\geq0 $.
$ (ii) $ For $ \alpha/d_{1}\leq1/\max_{\overline{\Omega}}m_{1} $, the species $ v $ can invade when the species $ u $ is rare, and the two species will coexist if $ d_{2} $ is larger than $ d_{2}^{*} $.
$ (iii) $ If $ m_{1} $ is positive in $ \overline{\Omega} $ and $ \alpha/d_{1}\geq1/\min_{\overline{\Omega}}m_{1} $, then the two species always coexist for every $ d_{2} > 0 $. In this case, the species $ u $ has relatively strong advection, and then it leaves sufficient habitat for $ v $ to evolve.
How does the inter-specific competition coefficients $ b $ and $ c $ affect the dynamics of system (1.3) when $ m_1 $ is not a constant and $ m_2 $ is constant? What values of the inter-specific competition coefficient $ b $ and $ c $ are favorable for the coexistence of the two species? In this paper, we shall discuss these topics in detail. Throughout this paper, the basic assumption on $ b $ and $ c $ includes part of the range $ bc\leq1 $, which contains the weak competition case ($ 0 < b, c < 1 $) as well as $ b = c = 1 $. At first, we make the following assumption.
$ {\bf{(M)}} \; m_{1} $ belongs to $ \mathcal{C}^{2+\gamma}(\overline{\Omega}) $ for some $ \gamma $ satisfying $ 0 < \gamma < 1 $, and $ m_{2} = \overline{m} $ is a non-negative constant, $ m_{1}\not\equiv m_{2} $. Moreover,
Assumption $ {\bf{(M)}} $, biologically, implies that the total resources for two competitors are fixed at exactly the same level. Due to the assumption that $ m_2 $ is a non-negative constant, it holds $ \nabla m_2 = 0 $, then the advective rate of species $ v $ (i.e., $ \beta $) has no effect on the dynamics of system (1.3). Moreover, throughout this paper, we assume that $ d_1, d_2 > 0, d_1\neq d_2 $ and $ \alpha > 0 $.
Besides the assumption $ {\bf{(M)}} $, we define $ \omega: = \alpha/d_{1}. $
Then system (1.3) becomes
We assume that the initial data $ u_0, v_0 $ of system (1.4) are non-negative and not identically zero, then by maximum principle [35], we can obtain $ u > 0, v > 0 $. Under the assumption $ {\bf{(M)}} $, for all $ d_{1}, d_{2} > 0 $ and $ \alpha > 0 $, there exist two semi-trivial steady states to system (1.4) (see [1,36]), denoted by $ (\bar{u}_{d_{1}, \omega, m_{1}}, 0) $ and $ (0, \overline{m}) $ respectively, where $ \bar{u}_{d_{1}, \omega, m_{1}} $ is the unique positive solution ([37]) of
and $ \overline{m} $ is the unique positive solution of
To study the dynamics of system (1.4), we should study the stability of semi-trivial steady states $ (\bar{u}, 0) $ and $ (0, \overline{m}) $. The stability of $ (\bar{u}_{d_{1}, \omega, m_{1}}, 0) $ is determined by the principal eigenvalue of the following linear problem
which is denoted by $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) $. The stability of $ (0, \overline{m}) $ is determined by the principal eigenvalue of the following linear problem
which is denoted by $ \lambda_{1}(d_{1}, \omega, m_{1}-b\overline{m}) $.
Moreover, $ (\bar{u}, 0) $ is linearly stable if $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}) > 0 $ and it is unstable if $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}) < 0 $. Similarly, $ (0, \overline{m}) $ is linearly stable if $ \lambda_{1}(d_{1}, \omega, m_{1}-b\overline{m}) > 0 $ and it is unstable if $ \lambda_{1}(d_{1}, \omega, m_{1}-b\overline{m}) < 0 $.
To describe the first result, according to [12], for every $ d_{1} > 0, d_{2} > 0 $ and $ \omega \leq 1/\max_{\overline{\Omega}}m_{1} $, we first introduce several notations:
$ \Sigma_{u} : = \{(d_{1}, d_{2}) \in \Gamma: (\bar{u}_{d_{1}, \omega, m_{1}}, 0) $ is linearly stable$\}$,
$ \Sigma_{v} : = \{(d_{1}, d_{2}) \in \Gamma: (0, \overline{m}) $ is linearly stable$\}$,
$ \Sigma_{-} : = \{(d_{1}, d_{2}) \in \Gamma: $ both $ (\bar{u}_{d_{1}, \omega, m_{1}}, 0) $ and $ (0, \overline{m}) $ are linearly unstable$\}$,
where $ \Gamma : = \mathbb{R}^{+}\times \mathbb{R}^{+}\; \mathrm{and}\; \mathbb{R}^{+} = (0, \infty). $
Define
and
In particular, if $ {\bf{(M)}} $ holds, then
To characterize the set $ \Sigma_{u} $ in terms of $ c > 0 $, for each $ c > 0 $ and $ \omega \leq 1/\max_{\overline{\Omega}}m_{1} $, we define
where
In fact, we have the following equivalent descriptions:
The first result is as follows.
Theorem 1.1. Suppose that $ {\bf{(M)}} $ holds and suppose $ \omega \leq 1/\max_{\overline{\Omega}}m_{1} $. Let $ L_{u} $ and $ S_{u} $ be defined as in Eq (1.9), and let $ (b, c) $ be in $ \Xi $. Then we have $ \Sigma_{v} = \emptyset $, and the following results hold:
$ (i) $
where $ d_{2}^{*}(d_{1}, \omega) $ is defined as follows:
$ I_{c}, I_{c}^{0} $ and $ I_{c}^{1} $ are defined in Eqs (1.10) and (1.11);
$ (ii) $ there exists at least one stable positive coexistence steady state for system (1.4) if $ (d_1, d_2) \in\Sigma_{-} $, where
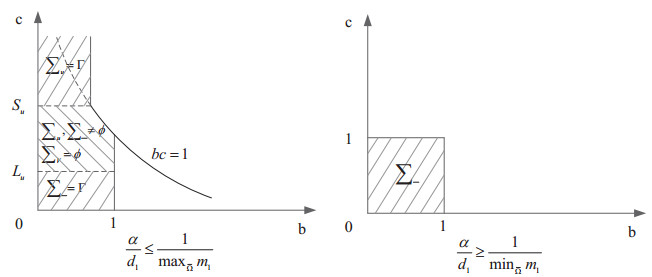
Remark 1.1. In Theorem 1.1, we consider the case of $ \omega \leq 1/\max_{\overline{\Omega}}m_{1} $. We divides the region of $ bc $-plane discussed in Theorem 1.1 into three parts. Species $ v $ can invade the space occupied by the species $ u $ so that would coexist with species $ u $ when $ c\leq L_u $ and $ 0 < b\leq1 $. When $ c $ takes on the intermediate value, that is $ L_u < c < S_u $, whether the two species will coexist or not is dependent on the values of random diffusion rates $ d_1, d_2 $ and the ratio $ \omega $. This result generalizes (Theorem 1.2 [22]) in which the authors discussed the case where the inter-specific competition coefficients $ b $ and $ c $ were $ 1 $. If $ c\geq S_u $, then $ (\bar{u}_{d_{1}, \omega, m_{1}}, 0) $ is linearly stable. These results are similar to the non-advection case (Theorem 3.3 [12]). It means that in an advective environment, as long as the ratio of advection rate to random diffusion rate is appropriately small, advection contributes to dispersal much less than random diffusion, and a sufficient amount of diffusive movement can counterbalance the advection.
When $ \omega\geq 1/\min_{\overline{\Omega}}m_{1} $, the following result holds.
Theorem 1.2. Suppose that $ {\bf{(M)}} $ holds, and $ m_{1} $ is positive for all $ x $ in $ \overline{\Omega} $. If $ \omega \geq 1/\min_{\overline{\Omega}}m_{1} $, then there exists at least one stable positive coexistence steady state for system (1.4) provided that $ (b, c)\in(0, 1]\times(0, 1] $.
Remark 1.2. Theorem 1.2 implies that, when $ \alpha/d_{1}\leq 1/\max_{\overline{\Omega}}m_{1} $, for every $ (b, c)\in(0, 1]\times(0, 1] $ and $ d_2 > 0 $, species $ v $ can invade the space occupied by the species $ u $, thereby the two species could coexist. This result generalizes (Theorem 1.5 [22]) for $ b = c = 1 $ case. From a biological point of view, if the ratio of advection rate $ \alpha $ to random diffusion rate $ d_1 $ of species $ u $ is appropriately large, advection plays a dominant role in dispersal for species $ u $. Species $ v $ adopts random diffusion strategy. Due to the advection, the species $ u $ concentrates at somewhere richer in resources, therefore, there leaves sufficient resources for species $ v $ to survival. Thereby, the two species coexist.
Figure 1 illustrates the region which is part of $ bc $-plane considered in Theorems 1.1 and 1.2.
The rest of this paper is organized as follows. In Section 2, we present some preliminary results which will be used in verifying our results. Section 3 is devoted to establishing our main results. We give a short discussion in Section 4.
2.
Preliminaries
Since system (1.4) generates a monotone dynamical system, the potential population dynamics, to a large extent, can be determined by the qualitative properties of its steady states. For a monotone dynamical system, we have the following conclusions (see, e.g., (Proposition 9.1 and Theorem 9.2 [38]):
$ (I) $. If both semi-trivial steady states of a monotone dynamical system are unstable, then there is at least one locally stable coexistence steady state;
$ (II) $. If a monotone dynamical system has no coexistence steady state, then one of the semi-trivial steady state is unstable and the other is globally asymptotically stable.
Let $ \mu_{1}(h) $ denote the unique nonzero principal eigenvalue of
where $ h\not\equiv $ constant and could change sign.
The following lemma collects some important properties of $ \mu_{1}(h) $. The proof can be found in [39,40,41].
Lemma 2.1. The problem (2.1) admits a nonzero principal eigenvalue $ \mu_{1} = \mu_{1}(h) $ if and only if $ \int_{\Omega}hdx\neq 0 $ and $ h $ changes sign. More precisely, if $ h $ changes sign, then
$ (i) \; \int_{\Omega}hdx = 0 \Leftrightarrow 0 $ is the only principal eigenvalue;
$ (ii) \; \int_{\Omega}hdx > 0 \Leftrightarrow \mu_{1}(h) < 0 $;
$ (iii) \; \int_{\Omega}hdx < 0 \Leftrightarrow \mu_{1}(h) > 0 $;
$ (iv) \; \mu_{1}(h_{1}) > \mu_{1}(h_{2}) $ if $ h_{1} \leq h_{2} $ a.e., and $ h_{1} $ and $ h_{2} $ both change sign;
$ (v) \; \mu_{1}(h) $ is continuous in $ h $; more precisely, $ \mu_{1}(h_{l})\rightarrow h $ in $ L^{\infty}(\Omega) $.
In order to analyze the principal eigenvalue of problem (1.7), for convenience, we consider the following more general form of eigenvalue problem:
Denote the principal eigenvalue of problem (2.2) by $ \lambda_{1}(d, h) $. By the variational approach, $ \lambda_{1}(d, h) $ can be characterized by
The following lemma collects some important properties of $ \lambda_{1}(d, h) $ as well as the connection between $ \lambda_{1}(d, h) $ and $ \mu_{1}(h) $ (see [40]).
Lemma 2.2. The first eigenvalue $ \lambda_{1}(d, h) $ of problem (2.2) depends smoothly on $ d > 0 $ and continuously on $ h \in L^{\infty}(\Omega) $. Moreover, it has the following properties:
$ (i) \; \int_{\Omega}hdx \geq 0 \Rightarrow \lambda_{1}(d, h) < 0 $ for all $ d > 0 $;
$ (ii) $
$ (iii) \; \lambda_{1}(d, h) < \lambda_{1}(d, k) $ if $ h \geq k $ and $ h\not\equiv k $. In particular $ \lambda_{1}(d, h) > 0 $ if $ h\geq(\not\equiv)0 $.
To study the stability of the semi-trivial steady state $ (0, \overline{m}) $, it is needed to introduce the following linear eigenvalue problem
Denote the principal eigenvalue of problem (2.4) by $ \lambda_{1}(d, \omega, h) $. Then $ \lambda_{1}(d, \omega, h) $ can be expressed by the following variational formula (see, e.g., [1])
where $ h\in C(\overline{\Omega}) $ is not a constant. Then we obtain the following result [1].
Lemma 2.3. Let $ \lambda_{1}(d, \omega, h) $ be the principal eigenvalue of problem (2.4). Suppose that $ h $ is a continuous function on $ \overline{\Omega} $ and $ h $ is not a constant. Then we have the following result
and the equality holds only if $ h_{1}\equiv h_{2} $.
The proofs of our results depend heavily on the following result.
Lemma 2.4. Assume that $ m\in C^{2}(\overline{\Omega}) $, $ m\not\equiv constant $ and $ \int_{\Omega}mdx\geq0 $ ($ m $ may change sign). Then there exists a unique positive solution to the steady state problem
which is denoted by $ \bar{u} $. Moreover,
$ (i) $ if $ \alpha/d \leq 1/\max_{\overline{\Omega}}m $, then $ \int_{\Omega}\bar{u}dx > \int_{\Omega}mdx $;
$ (ii) $ if $ m > 0 $ in $ \overline{\Omega} $, and $ \alpha/d \geq 1/\min_{\overline{\Omega}}m $, then $ \int_{\Omega}\bar{u}dx < \int_{\Omega}mdx $.
Proof. The existence and uniqueness of positive solution $ \bar{u} $ is well-known [36]. For the proof of (ⅰ) see Lemma 3.1 in [22]. For the proof of (ⅱ), see Lemma 4.1 in [24]. For completeness, we provide a proof of (ⅰ).
If $ \alpha/d \leq 1/\max_{\overline{\Omega}}m $, set
Then $ \eta $ satisfies
Let $ \eta(x^{*}) = \max_{\overline{\Omega}}\eta $. Then we can always choose $ x^{*}\in\Omega $. If $ x^{*}\in\partial\Omega $, then $ \eta(x) < \eta(x^{*}) $ for all $ x\in\Omega $. By the Hopf boundary lemma ([42]), $ \frac{\partial\eta}{\partial\nu}(x^{*}) > 0 $. This contradicts the boundary condition $ \partial_{\nu}\eta = 0 $ on $ \partial\Omega $, which implies that $ x^{*} $ can be chosen in $ \Omega $. For such $ x^{*} \in \Omega $, it holds that $ \Delta\eta(x^{*})\leq0, \nabla\eta(x^{*}) = 0 $. Thus, by the equation of $ \eta $, we have $ \bar{u}(x^{*})\leq m(x^{*}) $. Therefore,
where the last inequality follows from $ ye^{-\frac{\alpha}{d}y} \leq d/\alpha e $. It follows that $ \eta(x) \leq d/\alpha e $ for every $ x\in \overline{\Omega} $. Then due to $ \alpha/d \leq 1/\max_{\overline{\Omega}}m $, we have
Define a function $ f(y) = ye^{-\frac{\alpha}{d}}y $, $ y \in [0, d/\alpha] $. Since $ f'(y) > 0 $ for $ y \in [0, d/\alpha] $, $ f $ has an inverse function, denoted by $ s $. It is obvious that $ s $ is defined in $ [0, d/\alpha e] $, and its range is $ [0, d/\alpha] $. Hence, for each $ x\in \overline{\Omega} $, $ f(\bar{u}(x)) $ and $ s(\eta(x)) $ is well-defined by Eqs (2.7) and (2.8).
Dividing system (2.6) by $ s(\eta) $ and integrating in $ \Omega $, we obtain
which can be written as
Next we prove the following inequality
If $ \bar{u}(x) < m(x) $, we have $ f(\bar{u}(x)) = \bar{u}(x)e^{-\frac{\alpha}{d}\bar{u}(x)} > \bar{u}(x)e^{-\frac{\alpha}{d}m(x)} = \eta(x) $, then by the strictly monotone increasing property of $ s $, it holds that $ \bar{u}(x) > s(\eta(x)) $, and then Eq (2.9) follows.
If $ \bar{u}(x) \geq m(x) $, we have $ f(\bar{u}(x)) = \bar{u}(x)e^{-\frac{\alpha}{d}\bar{u}(x)}\leq \bar{u}(x)e^{-\frac{\alpha}{d}m(x)} = \eta(x) $. It follows that $ \bar{u}(x)\leq s(\eta(x)) $, and Eq (2.9) holds either in this case.
Hence, we obtain $ \int_{\Omega}\bar{u}dx > \int_{\Omega}mdx $ if $ \alpha/d \leq 1/\max_{\overline{\Omega}}m $.
3.
Proof of the main results
Firstly, we prove the unstability of $ (0, \overline{m}) $ for every $ d_{1}, d_{2} > 0 $, and $ \omega > 0 $ by employing a method similar to that used in [22].
Lemma 3.1. Suppose that $ {\bf{(M)}} $ holds. Then for every $ d_{1}, d_{2} > 0 $ and $ \omega > 0 $, $ (0, \overline{m}) $ is unstable provided $ b $ in $ (0, 1] $ and $ c > 0 $.
Proof. Let $ \lambda_{1}(d_{1}, \omega, m_{1}-b\overline{m}) $ be the principal eigenvalue of problem (1.8) and let $ \psi > 0 $ be the corresponding eigenfunction. Then
Consider
Set $ \phi = e^{-\omega m_{1}}\psi $. Then $ \phi $ satisfies
Dividing Eq (3.3) by $ \psi $ and integrating in $ \Omega $, we have
Define
then $ \Omega = \Omega^{+}\bigcup\Omega^{-} $, and
It follows from Eqs (3.4) and (3.5) that $ \lambda_{1}(d_{1}, \omega, m_{1}-\overline{m}) < 0 $. In view of Lemma 2.3, we derive
which implies that $ (0, \overline{m}) $ is linearly unstable.
Proof of Theorem 1.1. According to Lemma 3.1, it holds that $ \Sigma_{v} = \emptyset $. Next we give the proof of Eq (1.13) in two steps.
$ {\bf{Step\; 1.}} \; (d_1, d_2)\in\Sigma_{u} $ indicates that $ d_{1}\in I_{c} $.
From Eq (1.12), we know
Suppose that $ d_{1}\not\in I_{c} $, where $ I_{c} $ is defined in Eq (1.10). Then $ \int_{\Omega}(\overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}})dx\geq0 $. By Lemma 2.2 (ⅰ), $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}})\leq0 $ for all $ d_{2} > 0 $, i.e., $ (d_{1}, d_{2})\not\in\Sigma_{u} $. Hence $ (d_{1}, d_{2})\in\Sigma_{u} $ implies that $ d_{1}\in I_{c} $.
$ {\bf{Step\; 2.}} $ Characterize the set $ I_{c} $ for all $ c > 0 $ in detail.
If $ c\leq L_{u} $, by the definition of $ L_u $ in Eq (1.9), $ \int_{\Omega}(\overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}})dx\geq0 $ for all $ d_1 > 0 $. Hence $ I_c = \emptyset $ and $ \Sigma_u = \emptyset $. Moreover,
Indeed, if $ d_1^{'}\in I_{c}^{1}\neq\emptyset $, then $ L_u < c $ and for some $ x_{0}\in\overline{\Omega} $, it follows that
where $ \omega_{'} = \alpha/d_1^{'} $. Hence $ I_{c}^{1}\neq\emptyset $ gives rise to $ L_u < c < S_u $.
On the other hand, if $ L_u < c < S_u $, then there exists some $ d_1^{''} > 0\; (\omega^{''} = \alpha/d_1^{''}) $ and $ y_0\in\overline{\Omega} $ such that
i.e., $ d_1^{''}\in I_c^{1}\neq\emptyset $. This finishes the proof of Eq (3.6).
We now claim that $ I_c $ admits the following decomposition:
To finish the proof of Eq (3.7), it suffices to show that if $ S_u < +\infty $ and $ c\geq S_u $, then $ I_c = I_{c}^{0} = \mathbb{R}^{+} $. By the definition of $ S_u $ in Eq (1.9), we deduce that
Hence to show that $ I_c = I_{c}^{0} = \mathbb{R}^{+} $, it suffices to show that $ \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}\not\equiv0 $ for $ c\geq S_u $. Since $ \overline{m} $ is a constant, this is obviously true if $ c > S_u $. This finishes the proof of Eq (3.7).
Therefore
i.e., $ \Sigma_{u} = \Gamma. $
Now assume that $ L_{u} < c < S_{u} $ and $ d_{1}\in I_{c} $. If $ d_{1}\in I_{c}^{0} $, by Lemma 2.2 (ⅲ), $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) > 0 $, then for any $ d_{1}\in I_{c}^{0} $, $ (\bar{u}_{d_{1}, \omega, m_{1}}, 0) $ is linearly stable. If $ d_{1}\in I_{c}^{1} $, then $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) > 0 $ for all $ d_2 > 1/\mu_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) $ by Lemma 2.2 (ⅱ). Thus after defining
we obtain that, when $ L_{u} < c < S_{u} $, $ (d_1, d_2)\in\Sigma_{u} $ if and only if $ d_1\in I_c^{1}, d_2 > 1/\mu_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) $ or $ d_1\in I_c^{0}, d_2 > 0 $.
Finally, we prove Eq (1.14).
If $ c\leq L_u $, then $ \int_{\Omega}(\overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}})dx\geq0 $ for all $ d_{1} > 0 $. Hence by Lemma 2.2 (i), $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}})\leq0 $ for all $ (d_1, d_2)\in\Gamma $ and $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) = 0 $ if and only if $ c = L_u $ and $ \overline{m}\equiv L_u \bar{u}_{d_{1}, \omega, m_{1}} $. Due to the fact that $ \overline{m} $ is a constant, it is easy to see that the equality $ \overline{m}\equiv L_u \bar{u}_{d_{1}, \omega, m_{1}} $ is not true, which implies that for all $ (d_1, d_2)\in\Gamma, \; \lambda_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) < 0 $, i.e., $ (\bar{u}_{d_{1}, \omega, m_{1}}, 0) $ is unstable. If $ c\geq S_u $, by Eq (3.8), $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) > 0 $, then for all $ (d_1, d_2)\in\Gamma $, $ (\bar{u}_{d_{1}, \omega, m_{1}}, 0) $ is linearly stable. If $ L_u < c < S_u $, for $ d_1\in I_{c}^{0} $, $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) > 0 $ for all $ d_2 > 0 $. For $ d_1\in I_{c}^{1} $, $ \lambda_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) < 0 $ for all $ d_2 < 1/\mu_{1}(d_{2}, \overline{m}-c\bar{u}_{d_{1}, \omega, m_{1}}) $. Then
By the theory of monotone dynamical system, we derive that system (1.4) has at least one coexistence steady state for $ \Sigma_{-} $. This finishes the proof of Theorem 1.1.
Proof of Theorem 1.2. If $ {\bf{(M)}} $ holds, $ m_{1} > 0 $ in $ \overline{\Omega} $, and $ \omega \geq 1/\min_{\overline{\Omega}}m_{1} $, then by Lemma 2.4, the unique positive solution $ \bar{u}_{d_{1}, \omega, m_{1}} $ of Eq (1.9) satisfies $ \int_{\Omega}\bar{u}_{d_{1}, \omega, m_{1}}dx < \int_{\Omega}m_{1}dx $.
Indeed, by the theory of monotone dynamical system, we only need to prove that $ (\bar{u}_{d_{1}, \omega, m_{1}}, 0) $ is unstable. It is sufficient to show that the principal eigenvalue $ \lambda_{1} $ of the problem
is negative.
Consider the following principal eigenvalue problem
Dividing system (3.10) by $ \psi $, integrating in $ \Omega $, we obtain
Since $ \omega\geq 1/\min_{\overline{\Omega}}m_{1}(x) $, it holds that $ \int_{\Omega}(\bar{u}_{d_{1}, \omega, m_{1}}-\overline{m})dx = \int_{\Omega}(\bar{u}_{d_{1}, \omega, m_{1}}-m_{1})dx < 0 $. Then $ \lambda_{1}(d_{2}, \overline{m}-\bar{u}) < 0 $. From Lemma 2.2 (ⅲ)
for $ c\in (0, 1] $.
Then $ (\bar{u}_{d_{1}, \omega, m_{1}}, 0) $ is unstable. From the proof of Lemma 3.1, we know that $ (0, \overline{m}) $ is unstable for $ (b, c)\in(0, 1]\times(0, 1] $ and by the theory of monotone system, system (1.4) has at least one stable positive coexistence steady state.
4.
Discussion
We study the dynamics of a Lotka-Volterra competition-diffusion-advection model. The two competing species $ u $ and $ v $ adopt the dispersal strategies of a combination of random dispersal and biased movement upward along the resource gradient $ \nabla m_{i} $ (i = 1, 2) (the species are very smart and they will move toward regions richer in resources). In this paper, it is assumed that the two species have the same level of total resources but the species $ v $ adopts the homogeneous distribution and its competitor, the species $ u $, adopts heterogenous distribution. This means that $ \nabla m_{2}\equiv0 $ which implies that the species $ v $ only experiences random dispersal but has no biased movement.
Based on the above assumptions, we mainly study the effect of the inter-specific competition coefficients on the dynamics of system (1.4). From Lemma 3.1, $ (0, \overline{m}) $ is unstable for each one of the following conditions: $ d_{1}, d_{2} > 0 $, $ \alpha\geq0 $, and $ 0 < b\leq1, 0 < c $. This phenomenon indicates that the homogeneous distribution is disadvantageous for $ v $, therefore the species $ u $ can always invade the species $ v $. To study the coexistence regime for system (1.4), we discuss two cases. For the case of $ \alpha/d_{1}\geq 1/\min_{\overline{\Omega}}m_{1} $, the species $ u $ and $ v $ will coexist for every $ d_{2} > 0 $ and $ (b, c)\in(0, 1]\times(0, 1] $, see Theorem 1.1. From a biologic perspective, this case can be explained noting that the species $ u $ is characterized by a relatively strong advection, and then it leaves sufficient habitat for $ v $ to evolve. This result generalizes that in [22] for $ b = c = 1 $. For the case of $ \alpha/d_{1}\leq 1/\max_{\overline{\Omega}}m_{1} $, whether $ u $ and $ v $ will coexist depends on the values of $ d_1, d_2 $ and $ c $. In order to discuss this case, we define $ S_u, L_u $ and $ I_c $. We find that if $ c\leq L_u $, for all $ d_1 > 0 $ and $ d_2 > 0 $ the two species coexist. When $ c $ takes on the intermediate values, that is, $ L_u < c < S_u $, whether the two species will coexist or not is dependent on the values of $ d_1, d_2 $ and $ \omega $. If $ c\geq S_u $, species $ v $ can not displace species $ v $. These results are obtained in Theorem 1.2 which is more complex than Theorem 1.3 in [22].
For $ \alpha/d_{1} $ in the interval $ (1/\max_{\overline{\Omega}}m_{1}, 1/\min_{\overline{\Omega}}m_{1}) $, the dynamics of system (1.4) becomes more complicated. Moreover, for a general form of system (1.3), the dynamical behavior is known to be very limited and this case is far away from a complete understanding.
Acknowledgments
The authors express their gratitude to the anonymous reviewers and editors for their valuable comments and suggestions which led to the improvement of the original manuscript.
This work was partially supported by NNSF of China (No. 11971185).
Conflict of interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad:


