1. Introduction
The Ligand Epitope Antigen Presentation System (LEAPS) is unique as a platform for developing T cell vaccines that convert a peptide containing single and multiple T cell epitopes into an immunogen and simultaneously directs the nature of the subsequent immune response [1,2] to either a Th1 or Th2/Treg directed response. LEAPS vaccines act as both antigen and adjuvant. We reviewed [3,4,5,6] and compared the LEAPS approach to other vaccine technologies and conjugates (including Ii-Key, PaDRe, SLP, TRL, as well as DNA vaccines) that promote T cell activation but LEAPS is unique in its ability to define the nature of the subsequent response without concurrent administration of a cytokine, its gene, or other response determining adjuvant. This review will focus on J-LEAPS vaccines because these vaccines elicit their response by promoting the maturation of precursors into dendritic cells that produce Interleukin (IL) IL12p70 and promote Th1 responses (DC1 cells) (see later). This review of J-LEAPS vaccines describes newer applications of the technology.
LEAPS vaccines have been developed and tested in animal models and elicit protection against lethal challenge with herpes simplex type 1 (HSV-1) [7,8] and influenza A [9] viruses, elicit relevant immune responses to human immunodeficiency virus (HIV) [10,11] and tuberculosis [1,2] prevent the establishment of tumors and the spread of HER-2/neu tumor cells [12] and stop the progression of autoimmune diseases such as experimental autoimmune myocarditis [13] and rheumatoid arthritis (RA) [14,15,16]. Several of these vaccines are awaiting the opportunity to be tested in humans.
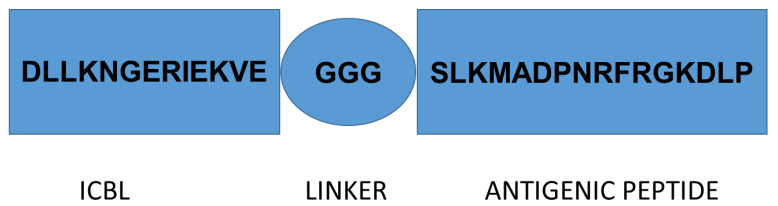
The antigenic peptides incorporated into LEAPS vaccines can be as small as 8 amino acids or as large as 32 amino acids but must contain at least one T cell epitope [9]. Peptides as small as 8 amino acids are usually poor or not immunogens but are effective in LEAPS vaccines. The peptides to be incorporated into a LEAPS vaccine are identified in the literature or through in silico predictions to contain T cell epitopes important for eliciting T cell associated protective or therapeutic responses and have chemical properties conducive to synthesis, stability and delivery. Attachment to either of two immune cell binding ligands (ICBL) (approximately 13 amino acids) through a triglycine linker [1,2,12] (Figure 1 and Table 1) creates the LEAPS vaccine and covalent attachment is required for activity. The J-ICBL is a peptide from the beta-2 microglobulin component of the MHC Ⅰ molecule (DLLKNGERIEKVE, aa 38–50) [17] and the G-ICBL is a peptide from the beta chain of the MHC Ⅱ molecule (NGQEEKAGVVSTGLI, aa 135–149) [18,19,20]. The DerG (DGQEEKAGVVSTGLI) ICBL is functional and preferred to the G-ICBL because it is more stable. These ICBLs were chosen because they interact with receptors on leukocytes and mutations within these peptide sequences or antibodies that bind to these sequences prevent the onset of immune responses. The ICBL itself is a poor immunogen and does not elicit an antibody response without conjugation to a large carrier such as keyhole limpet hemocyanin (KLH). The LEAPS vaccines are administered in an oil-in-water adjuvant emulsion for depot release. Most LEAPS vaccines have been tested with the Montanide ISA 51 adjuvant [7,14,21,22] but they have also been tested with MPL (Lipid A) and Novasomes [3]. Alum and similar adjuvants are not effective [10].
Table 1. Peptides incorporated into J-LEAPS vaccines.
| Peptide |
Source |
Sequence |
| INFECTIOUS DISEASE VACCINES |
|
|
| Herpes simplex virus type 1 |
|
|
| H1 |
ICP27 322–332 |
LYRTFAGNPRA |
| gB |
Glycoprotein B 498–505 |
SSIEFARL |
| gD |
Glycoprotein D 8–23 |
SLKMADPNRFRGKDLP |
| Mycobacterium tuberculosis |
|
|
| M |
38 kDa protein 350–369 |
DQVHFQPLPPAVVKLSDAL |
| HIV-1 |
|
|
| H |
HGP-30 p17 protein 85–115 |
YSVHQRIDVKDTKEALEKIEEEQNKSKKKA |
| mHGP30 |
HGP-30 p17 protein 82–112 |
ATLYSVHQRIDVKDTKEALEKIEEEQN |
| Influenza |
|
|
| M2e |
M2 envelope protein 2–24 |
SLLTEVETPIRNEWGCRCNDSSD |
| NP |
Nucleoprotein 144–158 |
NDATYQRTRALVRTG |
| HA1 |
Hemagglutinin 38–53 |
LKSTQNAIDEITNKVN |
| HA2 |
Hemagglutinin 1–13 |
GLFGAIAGFIEGG |
| THERAPIES |
|
|
| Breast Cancer |
|
|
| HER |
Rat HER-2/neu 66–74 |
TYVPANASL |
| Rheumatoid Arthritis |
|
|
| CEL-2000 |
Human Collagen type 2 254–273 |
TGGKPGIAGFKGEQGPKGEP |
| Myocarditis |
|
|
| My-1 |
Murine Cardiac Myosin 334–352 |
DSAFDVLSFTAEEKAGVYK |
The G-and DerG-ICBLs are not the topic of this review and will be mentioned only briefly. The G and DerG-ICBL are structurally related peptides that interact with the CD4 molecule present on a major subclass of T cells but also on other cells, e.g. certain classes of monocytes, macrophages, dendritic cells, and mast cells. The G-and DerG-ICBLs promote immunomodulatory regulatory CD4+ CD25+ FoxP3+ T cell (Treg) and Th2 type responses via CD4+ T cells and not DCs [14,15]. Konig and colleagues demonstrated that the G-peptide binds to CD4 and at high concentrations it inhibits T cell responses while at more physiological concentrations, the peptide enhances T cell responses through promoting changes in calcium flux and cyclic AMP levels [20]. Antibody production by G (or DerG)-LEAPS vaccines requires an antigenic boost with protein antigen and in the mouse this results in a secondary Th2 antibody response indicated by the presence of IgG1 and IgG3 but lack of IgM [1,2]. A DerG-LEAPS vaccine is therapeutic in a proteoglycan-induced mouse model for rheumatoid arthritis (RA) [14,15].
2. J-LEAPS Vaccines
The initial J-LEAPS vaccines incorporated a peptide from the 38 kDa protein of M. tuberculosis (peptide 350–369)
[1,2]; a peptide from the p17 HGP-30 protein of human immunodeficiency virus-1 (HIV) [10,11] (peptide 85–115) or a region of the p41 envelope portion (peptide 603–620) of HIV [1]. Subsequent studies demonstrated that successful J-LEAPS vaccines contain a CD8 T cell epitope [9]. Providing a protein boost to mice following immunization with either of these vaccines produced a secondary immune response dominated by the IgG2a subtype. This indicated that the J-LEAPS vaccines elicit a primary Th1 focused response. Th1 responses are usually pro-inflammatory and activate macrophages for more effective antimicrobial activity towards intracellular microbes, activate T cells to produce more interferon gamma (IFNγ) to reinforce the response, and activate cytolytic CD8 T cells. In contrast, attachment of the same antigenic peptides to the G-ICBL or DerG-ICBL instead of the J-ICBL induced Th2 related antibody responses upon protein boost and Treg responses [1,2,14]. Hints towards the mechanism of action of the J-ICBL were obtained in studies performed with the JgD anti-HSV vaccine which incorporates peptide 8–23 from the herpes simplex virus Type 1 (HSV-1) glycoprotein D (gD) [4] and with the JH vaccine that incorporates peptide 85–115 from the HIV p17 protein [10,11]. Temporal antibody ablation studies showed that CD8 bearing cells are important to initiate the response to JgD and CD4-bearing cells, CD8 bearing cells and IFNγ were important for delivering protection from lethal challenge with HSV-1 [8]. In mice, CD8 is expressed on T cells but also on a dendritic cell type 1 (DC1) subset [22] which is especially adept at cross presentation of antigen and important for activating CD8 T cells. Treatment of immune-naive uninfected mice with JgD or JH caused the production and presence in serum of IL12p70 by the 4th day post immunization [22]. IL17 and IL12p70 were detectable in serum on the 10th day, followed by waning of IL17 and an increase in IFNγ on the 20th day after immunization. Interestingly, serum levels of tumor necrosis factor alpha (TNFα) did not increase in response to JgD treatment. IL12p70 is produced by DC1 cells, a subset of DCs that promote Th1 responses [23]. As described below, treatment of precursor cells from mouse bone marrow or human monocytes with JgD or JH promoted their maturation to become DC1s.
3. Prophylactic J-LEAPS Peptide Vaccines
The proof of concept for J-LEAPS vaccines was obtained by demonstration of protection from lethal challenge with herpes simplex virus type 1 (HSV-1) after immunization with antigen specific J-LEAPS vaccines. G-LEAPS vaccines either had no effect or exacerbated the infection as would be predicted following induction of a Th2 type of response. The initial study utilized a vaccine that incorporated an 11 amino acid CD8 T cell epitope from the intracellular viral protein ICP27 (JH1) [7]. Antibody production to this epitope or protein would not be protective. Vaccination of Balb/C mice elicited protection from lethal challenge with minimal morbidity and mortality and generated delayed type hypersensitivity (DTH) responses to the viral antigens but did not generate an antibody response either upon immunization or challenge by infectious virus. These studies indicated success of the JH1 vaccine and that a T cell response was elicited which was sufficient for protection. Similar protections were elicited towards the 8 amino acid major CD8 T cell epitope (SSIEFARL) from glycoprotein B (gB) of HSV-1 recognized by C57/BL6 mice [4]. Again, no antibody was produced prior to challenge by infectious virus. Heteroconjugates of the H1 or gB or gD peptides with the G-ICBL did not confer protective immunity [4,8].
The most in depth vaccine studies were performed with JgD [4,8,22]. As with the other anti-HSV vaccines, this vaccine elicited protection against morbidity and mortality. Antibody was only detected when there was minor breakthrough disease signs indicative of virus production which provided the requisite protein boost. Elicitation of protection was observed in multiple strains of mice including four different inbred and three different outbred strains [8].
4. J-LEAPS Dendritic Cell Vaccines
The J-ICBL promotes interactions between the LEAPS vaccine and dendritic cell precursors and promotes their maturation into DC1s [22,24]. Treatment of mouse bone marrow cells with JgD or JH generated cells with the mature DC1 phenotype within 48 hours [22]. The conversion occurred whether the bone marrow cells were not pretreated or pretreated with GM-CSF alone or GM-CSF and IL4 according to standard protocols for generating DCs. Similarly, treatment of purified human monocytes obtained from peripheral blood with JgD or JH after pretreatment with GM-CSF plus IL4 or not, also generated DC1s with a similar phenotype to the mouse DC1s [24]. These cells are referred to as JgD-muDCs and JH-muDCs from the mouse and JgD-huDC and JH-huDC from humans. JH-DCs and JgD-DCs from human and mouse produced IL12p70 and DC1 related chemokines but unlike DC1s produced by other means, did not produce TNFα. The JgD-muDCs and JH-muDCs were able to induce the Th1 cytokines, IL2 and IFNγ, from na ve mouse spleen cells [22] and the JgD-huDCs and JH-huDCs were able to elicit these cytokines from human peripheral blood T cells [6,24]. In the converse of this experiment, a cytokine response (IL2 or IFNγ) was not elicited by T cells treated with JgD or JH for 48 hours followed by washing away free peptide prior to mixing with monocytes. Demonstration that JgD and JH elicit similar reactions in human and mouse dendritic cell precursors provides basis for developing J-LEAPS vaccines for humans.
The strongest proof that the JgD peptide promotes maturation of precursors to DC1s was obtained by the antigen specific protection from lethal HSV-1 challenge elicited after adoptive transfer of carefully washed, ex vivo generated JgD-muDCs into otherwise immuno-naive mice [22]. Mice receiving JgD-muDCs were protected whereas mice receiving JH-muDCs were not. Bone marrow cells treated with the J-LEAPS peptide for 48h were administered on two occasions 14 days apart and then one week later the mice received a lethal challenge with HSV-1. All the mice receiving JgD-muDCs survived with only very minor disease signs observed in 2 out of 7 mice. The antigen specificity of the response was demonstrated by the deaths of all mice receiving JH-muDCs, cells generated with the HIV vaccine.
In similar studies by Boonnak et al. [9], J-LEAPS muDCs, matured by J-LEAPS vaccines containing appropriate CTL protective influenza A epitopes, prevented morbidity and mortality when administered as a treatment after lethal challenge with influenza A. Bone marrow cells pre-incubated in GM-CSF were treated with J-LEAPS peptides that incorporated 15 to 30 amino acid—long peptides derived from influenza virus NP, M2e, or HA proteins. The treatment caused maturation of the cells to become DC1s. The NP and M2e peptides contained CD8 T cell epitopes while the HA peptide contained CD4 T and B cell epitopes. The mice that were treated with J-NP-muDCs, or J-M2e-muDCs or a mixture of DCs matured with all four of the influenza peptides (J-NP, J-M2e, J-HA1, J-HA2) as late as 48 h after lethal viral challenge maintained normal weight and viability whereas those receiving LEAPS-muDCs prepared with only the J-HA1, J-HA2 or the unrelated JH vaccine (vaccine for HIV) died within 9 days. Influenza virus replication and TNFα, IL6 and IL1α inflammatory cytokine levels in the lungs were reduced while IL12p70 and IFNγ levels were increased by the successful treatments indicating rapid modulation of the T cell cytokine response to infection. These studies confirmed the ability of J-LEAPS vaccines to promote the maturation of DC1s. Adoptive transfer of the J-LEAPS-DCs after virus challenge provided therapy for the on-going disease, limiting production and spread of the virus and providing antigen specific immunomodulation of the cytokine storm that exacerbates the disease. Unlike conventional immunization, as with the J-LEAPS peptides, the preformed and antigen presenting J-LEAPS-DCs were able to elicit the therapeutic response very quickly and rapidly enough to induce protections after influenza challenge to protect the animals from disease.
5. Antitumor LEAPS Vaccines
Therapeutic immune responses to tumors depend upon a strong CD8 cytotoxic T lymphocyte (CTL) response as part of a Th1 and Tc1 response [25] similar to those elicited by J-LEAPS vaccines. The CTLs driven by IL12 and Th1 and Tc1 responses are more sensitive to antigen than T cells induced by other responses [26,27,28,29] and IL12 promotes other responses that support anti-tumor activities [28,29]. As for the antiviral vaccines, anti-tumor vaccines can be designed by attaching a CTL epitope from a tumor antigen to the J-ICBL. Appropriate tumor antigens and epitopes from these antigens for inclusion in J-LEAPS vaccines can be obtained from studies described in the literature or from in silico analysis of tumor proteins.
As a proof of concept oncology study, a J-LEAPS vaccine for HER-2/neu breast cancer (J-HER) was designed and tested in a mouse model [12]. The HER-2/neu cell surface receptor is overexpressed in many breast cancer tumors and the tumor cells require the function of the protein [30,31]. CTL epitopes have been identified within HER-2/neu for mouse and human immune systems [32,33]. J-HER incorporates a prominent CTL eliciting minimal epitope (aa 66–74: TYVPANASL) for BALB/c mice [32,33]. The vaccine was administered as an emulsion with Montanide ISA-51 in an oil in water emulsion. The vaccine was tested in mice challenged with TUBO cells, a tumor cell line [34] originally obtained from a spontaneous mammary gland tumor that arose in genetically altered BALB/c mice that overexpress HER-2/neu.
When administered as a prophylactic vaccination before tumor challenge, J-HER protected against tumor development for at least 48 days. When administered after the tumor challenge, vaccination with J-HER made a large difference in the health and survival of the tumor bearing mice by controlling tumor spread even though the primary tumors continued to grow. The ability of the vaccine to elicit this type of therapeutic response suggests that a J-HER vaccine would be useful as a neoadjuvant therapy administered prior to surgery to initiate responses against tumor recurrence or metastasis. A HER-2/neu protein based dendritic cell vaccine has shown efficacy in this capacity [29,35].
The success of the J-HER pilot study indicates the potential of J-LEAPS tumor vaccines. J-LEAPS tumor vaccines can be prepared as minimal epitope peptides, tandem epitope peptides or mixtures of peptides. These vaccines can be readily synthesized to include different CD8 T cell tumor antigen epitopes. The J-LEAPS vaccines can be customized to contain several tumor antigen peptides to optimize tumor therapy or to personalize treatment for individuals with different HLA types. The J-LEAPS vaccines can be administered as peptides or as J-LEAPS DCs.
As stated by Palucka and Banchareau [26], the goal of cancer immunotherapy is to elicit tumor-specific CD8+ T cell mediated immune responses that will be sufficiently robust and long-lasting to generate durable tumor regression and/or eradication. They and others [36] utilize ex-vivo generated and antigen primed DCs for tumor therapy. Key to the success of these therapies is the proper maturation of precursors to become DC1s capable of activating the appropriate anti-tumor response and the choice of the optimal tumor antigen to be presented to the CD8 T cells [36]. Dendreon developed the first FDA approved anti-tumor Antigen Presenting Cell (APC) vaccine (sipuleucel-T) for prostate cancer [37]. Autologous peripheral-blood mononuclear cells from patients were treated with a conjugate of prostatic acid phosphatase and GM-CSF to promote maturation of cells and presentation of the tumor antigen upon re-infusion into the patient. The cells were then reinfused into the patient on three occasions. The sipuleucel-T generated APC cell population contained a mixture of cells including DCs but was not optimized for DC1s. Czerniecki et al. [29,35] and others [36] have focused their efforts on the use of antigen loaded DC1s as immunotherapy. This ensures treatment with the optimal antigen presenting cell expressing appropriate tumor antigens to elicit CD8 T cell responses.
DC1 cells capable of presenting relevant tumor antigenic epitopes can be designed using J-LEAPS peptides for use as autologous cell vaccines. Using the JgD-muLEAPS-DC and influenza-mu-LEAPS-DC as models, the tumor-peptide-LEAPS-DCs can be prepared within 48 hours of leukopheresis of the patient's monocytes (and probably less than 24 hours) and be ready to be infused as adoptive transfer therapy. Procedures could be developed for on-site maturation of the J-LEAPS-DCs allowing rapid generation of a customized autologous DC vaccine that delivers the appropriate antigen specific response.
Both J-LEAPS peptide and J-LEAPS-DC anti-tumor vaccines can readily be developed to customize the treatment of the cancer patient. Each approach has its advantages and disadvantages. Studies with the anti-viral J-LEAPS peptide vaccines indicate that DC1s are elicited in the body after immunization to mediate therapy but elicitation of a sufficient T cell response for therapy takes time. The J-LEAPS-DCs act quickly and can be injected into the draining lymph node of the tumor to focus the response but require extensive and expensive cell fractionation and tissue culture procedures prior to its use in treating the patient with concerns for the viability, sterility, means of delivery and function of the cells. Both approaches await further trials.
6. Therapeutic LEAPS Vaccines for Autoimmune Diseases
Th17 responses are a major driving force for rheumatoid arthritis (RA) and psoriasis as evidenced by the efficacy of therapeutic ablation of Th17 responses with antibodies to TNFα, IL17 or IL23 [38]. Unfortunately, these potent therapies compromise host protections provided by the Th17 response for tuberculosis and other infections. J-LEAPS vaccines offer a therapeutic option. The ability of LEAPS vaccines to promote the maturation of precursors into DC1s which promote a Th1 response defined by production of IFNγ but not TNFα and the ability of J-influenza-DCs to modulate the pathogenic inflammatory responses induced by influenza infection, suggest their use for immunomodulation of autoimmune diseases driven by Th17 proinflammatory responses. IFNγ inhibits Th17 responses [39]. Unlike the antibody ablation therapies, the LEAPS vaccine therapy would be focused on the antigen specific T cells that are promoting the disease rather than the entire cytokine driven immune response.
In a mouse model for experimental autoimmune myocarditis, treatment with a J-LEAPS vaccine incorporating an epitope from murine cardiac myosin (J-My1) prevented the development of the disease [13]. Similarly, the LEAPS vaccine, CEL-2000 (a conjugate composed of the J-ICBL and an immunodominant type Ⅱ collagen (CII) epitope) was therapeutic when administered after the onset of disease symptoms in the collagen induced arthritis (CIA) mouse model for rheumatoid arthritis, a Th17 driven disease [16]. Treatment with CEL-2000 caused a reduction in serum levels of pro-inflammatory IL17, IL6 and TNFα while increasing IL12, IFNγ and IL10 levels. IL10 is a regulatory cytokine produced by regulatory T cells, B cells and even some Th1 cells [40].
Although useful for Th17 driven autoimmune responses, a J-LEAPS vaccine was not effective in a mouse model for rheumatoid arthritis driven by Th1 proinflammatory responses to proteoglycan [14]. Therapy was provided by a derG-LEAPS vaccine incorporating an epitope from proteoglycan. LEAPS vaccines provide a choice of appropriate immunomodulatory therapy once the cytokine nature of the inflammatory response driving autoimmune disease is known. A J-LEAPS vaccine is appropriate for Th17 proinflammatory autoimmune diseases whereas a derG-LEAPS vaccine would be appropriate for Th1 proinflammatory autoimmune diseases.
7. Future Potential for J-LEAPS Vaccines
J-LEAPS vaccines and J-LEAPS-DCs provide the opportunity to custom design vaccines that elicit appropriate protective and therapeutic responses to disease related peptides. As demonstrated for influenza, J-LEAPS-DCs provide the additional advantage of initiating rapid, effective, protective therapeutic responses. All challenge studies with J-LEAPS vaccines to date have been limited to mice. However, both JgD and JH demonstrated their ability to induce responses in human cells that parallel those in mice by converting human monocytes into functional dendritic cells capable of inducing allotypic responses [25]. These studies showed the potential for application of J-LEAPS technology to human diseases.
A human J-LEAPS vaccine could consist of a single heteroconjugate peptide containing one or more epitopes or a mixture of J-LEAPS vaccine peptides. Customized or mixtures of vaccines can be developed to accommodate the heterogeneity in human histocompatibility molecules and disease related antigens. Many of the protective antigenic peptide sequences for intracellular microbial and viral infections and tumors are already known and J-LEAPS peptides can be readily synthesized under GMP conditions to produce vaccines for these diseases. For autoimmune diseases, J-LEAPS therapeutic vaccines can be utilized after the patient's disease is demonstrated to be driven by Th17 responses. In vitro assays to test these elements were developed for mouse spleen cells [14] but can be readily adapted to use for a human patient's peripheral blood cells to provide the potential for personalized medicine.
Despite great potential, funding limitations have precluded the jump to clinical trials for J-LEAPS vaccines. Studies are about to be initiated on a DerG-LEAPS vaccine for RA (CEL-4000) [14] to prepare this vaccine for first in man testing.
8. Conclusion
The J-LEAPS vaccine platform provides the means to custom design peptide vaccines that promote the maturation of dendritic cells to deliver interferon gamma driven CD8 T cell responses. The resultant immune responses are sufficient to elicit protections against lethal herpes simplex virus and likely other viral and intracellular infections, cytolytic therapy for cancer and immunomodulatory therapy for Th17 driven autoimmune diseases. Ex-vivo derived DC1s can be prepared with the J-LEAPS vaccine peptides to provide a more rapid acting and focused response. In addition to prophylaxis, the J-LEAPS peptide and J-LEAPS-DC vaccines provide therapy by promoting appropriate anti-tumor responses and immunomodulation of pathogenic inflammatory responses. The J-LEAPS vaccines interact with human dendritic cell precursors and promote similar responses with human cells as with mouse cells suggesting the efficacy of J-LEAPS vaccines in humans. The J-LEAPS vaccines can be customized to include appropriate epitopes, exclude inhibitory or regulatory epitopes, and to accommodate different MHC backgrounds. In addition, mixtures of peptides can be used to prepare J-LEAPS vaccines which can be used to optimize or expand their spectrum and efficacy.
Acknowledgements
All the studies presented in this review have been published and funding sources acknowledged in those publications. We would like to thank our collaborators for their contributions to the development of the J-LEAPS technology.
Conflict of Interest
All authors declare that they have no conflict of interest in this paper.









 DownLoad:
DownLoad: 

