Estimation techniques utilizing dual auxiliary variables in stratified two-phase sampling

1.   Introduction

Fractional Calculus (FC) is the subject dealing with the derivative and integration of non-integer orders. In ^[1], the researchers generalize the classical diffusion and wave equations to different physical processes, such as slow diffusion, classical diffusion, diffusion-wave hybrid, and the classical wave equation. Differential equations (DEs) are valuable tools for describing critical natural phenomena such as phase transition, electrochemistry, electromagnetism, filtration, acoustics, cosmology, biochemistry, and the dynamics of biological groups ^[2]. The present idea of DEs has been further extended by using different derivatives operators with fractional orders. The mathematical models with fractional derivatives and integrations are more accurate and adequate than ordinary models^[3]. There are various applications of fractional calculus in applied sciences like Poisson-Nerst Planck diffusion ^[4], earth quack nonlinear oscillation ^[5], air foil, chaos theory ^[6], fluid traffic ^[7], Financial ^[8], Zener ^[9], Electrodynamics ^[10], Cancer chemotherapy ^[11], Hepatitis B Virus ^[12], Tuberculosis ^[13], Pine wilt disease ^[14], Diabetes ^[15] and many other various applications in applied sciences ^[16,17]. The applications that have been mentioned above-attracted researchers to the subject and developed various sophisticated mathematical models in terms of fractional integrals and derivatives. These models are further analyzed and solved by using some numerical and analytical techniques such as the functional constraint's method ^[18], the iterated pseudo-spectral method ^[19], reduced differential transforms algorithm (RDTA)^[20], q-homotopy analysis Shehu transform algorithm (q-HASTA) ^[20], predictor-corrector algorithm ^[21], Adams-Bashforth-Moulton algorithm ^[22], and the numerical method for DEs in fractional order: based on the definition of Grunwald-Letnikov (GL) fractional derivative ^[22].

One of the most effective tools for researchers to simulate physical phenomena in nature, including fluid dynamics, mathematical biology, quantum physics, linear optics, and chemical kinetics, are considered to be fractional partial differential equations (FPDEs) ^[23]. Other physical phenomena are accurately modeled by FPDEs, such as the analytical solution of a coupled system of non-linear PDEs is presented in ^[24]; the solution of non-linear ODEs, which is previously achieved in ^[25]; non-linear PDEs, which is presented in^[26]; fractional telegraph equations which are presented in ^[27], the unsteady fractional flow of a polytrophic gas model which is introduced in ^[28], and the fractional Fokker-Plank equation and the Schrödinger equation can be found in ^[29].

Many researchers have tried hard to find the solutions of these FPDES through numerous techniques such as Homotopy perturbation transform method (HPTM) ^[30], Homotopy perturbation method (HPM) ^[31], Homotopy analysis method (HAM) ^[32], the Finite differences method^[33], the multiple exponential function algorithms and variational iteration method (VIM) ^[34]. Moreover, Feng's first integral method^[35], Abazari and Ganji provide the reduce differential transform method (RDTM) for PDEs ^[36], Mehshless method (MM) ^[37], Laplace Adomian decomposition method (LADM)^[38], and modified homotopy perturbation method (MHPM) ^[39].

The Riemann-Liouville (R-L) integral ^[40], Caputo ^[41,42], Caputo-Fabrizio^[43] and Atangana-Baleanu^[44] have been developed using the various fractional derivative operators (FDOs) to provide a precise meaning to the derivative with the optimal order of the derivative. These fractional derivative operators differ fundamentally from one another in that they each have a variety of kernels from which to choose depending on the demands of a given application. "Be aware that these three definitions of fractional derivatives have advantages and disadvantages. An arbitrary function does not need to be continuous at the origin or differentiable in order to take the RL fractional derivative. The fact that the RL derivative of a constant is not zero is one limitation in the RL's capacity for simulating real-world phenomena. On the other hand, the Caputo fractional derivative has the advantage of agreeing with the usual initial and boundary conditions included in the formulation of the equation. The disadvantage of Caputo's derivative is that we must first find the function's derivative in order to estimate the fractional derivative of a function in the Caputo sense. Atangana-Baleanu derivative tries to address some of the drawbacks from earlier. Besides, the fractional order derivative based on the AB operator is defined using limits rather than integrals. The details can be found in ^{[41,42,43,44,45]}."

In this study, we used three different FDOs: the Caputo fractional differential operator (C-FDO), the Caputo-Fabrizio fractional differential operator (C-FFDO), and the Atangana-Baleanu fractional differential operator (A-BFDO) to achieve the analytical solutions of the non-linear fractional order 3D Navier Stokes equations with $g_1 = g_2 = g_3 = 0$. The approximate analytical solution for time-fractional non-homogeneous Cauchy equation ^[20,46] are obtained with the help of a new iterative transform method (NITM)^[47]. NITM is an analytical technique in which the solution is obtained by an infinite series with higher convergent components. The accuracy of the proposed method is shown by graphs and tables with various fractional operators. The structure of this research article is presented as follows: in Section 2, we discuss some preliminary concepts. In Sections 3 and 4, we discuss the procedure of NITM for FPDEs and the system of FPDEs restrictively. The numerical results are discussed in Section 5. Section 6 is the conclusion section.

2.   Basic definitions

In this part of the paper, the preliminary concepts and important definitions are presented, which are very useful for the continuation of this research work.

Definition 2.1. The Caputo fractional derivative operator (C-FDO) is given as ^[48]

Definition 2.2. The Laplace transform (LT) of C-FDO is given as ^[48]

Definition 2.3. The formulation of the Caputo-Fabrizio fractional differential operator (C-FFDO) is ^[43]

the function $\mathbb{B}(\delta)$ is the normalization function depending on $\delta\ni \mathbb{B}(0) = \mathbb{B}(1) = 1$.

Definition 2.4. The LT of the C-FFDO is define as ^[43]

In particular, we have

Definition 2.5. The Mittag-leffler function was introduced in 1903^[49], and is given as

Definition 2.6. The Atangana-Baleanu fractional derivative operator (ABFDO) is defined as ^[50]

where $\mathbb{B}(\cdot)$ is a normalization function such that $\mathbb{B}(0) = \mathbb{B}(1) = 1$ and ${E}_{\delta}$ is the Mittag-leffler function defined in Definition 2.5.

Definition 2.7. The LT of the ABFDO is define as^[50]

Definition 2.8. The Aboodh transform (AT) is define by ^[51]

Theorem 1. The AT of C-FDO is given as ^[51]

Theorem 2. The AT of C-FFDO is defined as ^[52]

Theorem 3. If U(s) is the AT of Atangana-Baleanu-Caputo operator then ^[53]

Proofs. The proofs of these Theorems 1–3 can be found in ^[51,52,53].

3.   NITM procedure for FPDEs

To understand the basic methodology of the NITM within three different fractional derivative operators (FDOs), we consider the following three different cases.

3.1.   NITM solution within C-FDO^[47]

Let us consider a general non-homogenous FPDEs within C-FDO of the form,

having initial condition

In Eq (3.1) ${\mathbb{L}}$ is linear and ${{\bf N}}$ is the non-linear operator, while $f({{{\xi_o}}}, {\hat{t}})$ is a source term.

Applying} the AT to Eq (3.1), we obtain

where

Taking the inverse AT on Eq (3.3), we obtain

Next, we apply the New Iterative Method present in ^[54], we obtain an infinite series solution

Since ${\mathbb{L}}$ is linear

The nonlinear operator ${{\bf N}}$ becomes

In view of Eqs (3.3–3.5) and Eq (3.6) is equivalent to

Next, we consider the following recurrence relation,

The NITM series form solution is given by

3.2.   NITM solution within ABO ^[47]

We have a general non-homogenous AB-FDE of the form

having initial condition

In Eq (3.12) ${\mathbb{L}}$ is linear and ${{\bf N}}$ is the non-linear operator, while $f({{{\xi_o}}}, {\hat{t}})$ is a source term.

Taking the AT to Eq (3.12), we get

where

Taking the inverse AT on Eq (3.14), we obtain

where the source term is denoted by $\vartheta({{{\xi_o}}}, {\hat{t}})$. Next we apply New Iterative Method introduced in ^[54], we obtain an infinite series solution

Since ${\mathbb{L}}$ is linear

The nonlinear term ${{\bf N}}$ is decomposed by

In view of Eqs (3.15–3.17) and Eq (3.18) is equivalent to,

furthermore, consider the recursive relation of the following form,

The NITM approximate solution is given by

3.3.   NITM solution within C-FFDO ^[47]

We have a general non-homogenous FPDEs of the form

having initial condition

where $\mathfrak{D}_{\hat{t}}^{\delta+m}$ is C-FFDO and ${\mathbb{L}}$ and ${{\bf N}}$ are the linear and non-linear operator respectively, while $f({{{\xi_o}}}, {\hat{t}})$ is a source term.

Taking the AT to Eq (3.23), we obtain

where

Taking the inverse AT to Eq (3.25), we obtain

where the source term is denoted by $\vartheta({{{\xi_o}}}, {\hat{t}})$. Next, we apply the New Iterative Method introduced in ^[54], we obtain an infinite series solution

Since ${\mathbb{L}}$ is linear

The nonlinear term ${{\bf N}}$ is decomposed by

In view of Eqs (3.26–3.28) and Eq (3.29) is equivalent to

Next, consider the recursive relation of the following form,

The NITM approximate solution is given by

4.   NITM for system of FPDEs

We give the following methods to study the NITM process.

4.1.   NITM solution within CO^[47]

Consider a general system of non-homogenous Caputo type FPDEs of the form

having initial condition

In Eq (4.1) $f_1({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$, $f_2({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$ and $f_3({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$, are the source terms and ${\mathbb{L}}$ and ${{\bf N}}$ are the linear and non-linear operator respectively, while $\mathfrak{D}_{\hat{t}}^{\delta+m}$ is a Caputo type fractional derivative operator.

Taking the AT of Eq (4.1), we have

where

Taking the inverse AT on Eq (4.3), we obtain

Now, the source terms for the given system are presented as $\vartheta_1({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$, $\vartheta_2({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$ and $\vartheta_3({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$ respectively.

Next, applying the New Iterative Method introduced in ^[54] and considering the solution of an infinite series form,

Since ${\mathbb{L}}$ is linear

and the nonlinear term ${{\bf N}}$ is decomposed as

In view of Eqs (4.4–4.6) and Eq (4.7), is equivalent to

furthermore, we obtained a recursive relation of the following form

The NITM approximate solution is given by

4.2.   NITM solution within ABO^[47]

We have a general non-homogenous system of C-FFDE of the form

having initial condition

In Eq (4.12) $f_1({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$, $f_2({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$ and $f_3({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$, are the source terms, ${\mathbb{L}}$ and ${{\bf N}}$ are the linear and non-linear operator respectively, while $\mathfrak{D}_{\hat{t}}^{\delta+m}$ is Atangana-Baleanu type fractional derivative operator.

Applying the AT to Eq (4.12), we have

where

Taking the inverse AT to Eq (4.14), we obtain

where the source term is denoted by $\vartheta({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$. Further we apply NIM introduced in ^[54]. We consider the solution as an infinite series given as

Since ${\mathbb{L}}$ is linear

The nonlinear operator ${{\bf N}}$ is decomposed as

In view of Eqs (4.15–4.17) and Eq (4.18) is equivalent to

considering the following recursive relation

The NITM approximate solution is given by

4.3.   NITM solution within C-FFDO ^[47]

Let us consider a general non-homogenous system of FPDEs in terms of C-FPDO,

having initial condition

In Eq (4.23) $f_1({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$, $f_2({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$ and $f_3({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$, are the source terms, ${\mathbb{L}}$ and ${{\bf N}}$ are the linear and non-linear operator respectively, while $\mathfrak{D}_{\hat{t}}^{\delta+m}$ is the Caputo-Fabrizio type fractional derivative operator.

Applying the AT to Eq (4.23), we have

where

Taking the inverse AT on Eq (4.25), we obtain

where the source terms are denoted by $\vartheta_1({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$, $\vartheta_2({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$ and $\vartheta_3({{{\xi_o}}}, {{{\xi_1}}}, {{{\xi_2}}}, {\hat{t}})$. Further we apply NIM introduced in ^[48].We consider the solution as an infinite series given as

Since ${\mathbb{L}}$ is linear

The nonlinear operator ${{\bf N}}$ is decomposed as

In view of Eqs (4.26–4.28) and Eq (4.29) is equivalent to

considering the following recursive relation

The NITM approximate solution is given by

5.   Numerical results

In this section, we will find the analytical solutions of a non-homogeneous, time-fractional Cauchy equation with different fractional derivative operators.

5.1.   Example

The non-homogeneous time-fractional Cauchy equation is ^[20,46]

having initial condition

The exact solution is given as ^[20,46]

5.1.1.   NITM solution within C-FDO

Applying AT to Eq (5.1), we obtain

applying the inverse AT on Eq (5.2), we have

so the iterative scheme is

put n = 0 in Eq (5.4), we have

Put n = 1 in Eq (5.4), we have

The approximate NITM solution using the Caputo operator with three terms iterations

When the series goes to infinity term then we obtain the following solution

which is the the exact solution of the Eq (5.1). The exact solution are also yield in VIM ^[46], q-HASTA and RDTA ^[20].

5.1.2.   The NITM within A-BFDO

Applying AT to Eq (5.1), we obtain

applying the inverse AT on Eq (5.5), we have

so the iterative scheme of Eq (5.6), is

put n = 0 in Eq (5.7)

Put n = 1 in Eq (5.7), we have

The NITM solution with three terms approximation within Atangana-Baleanu operator is

5.1.3.   The NITM solution with Caputo Fabrizio operator

Applying AT to Eq (5.1), we obtain

taking the inverse AT on Eq (5.8), we have

so the iterative scheme of Eq (5.9), is

put n = 0 in Eq (5.10)

Put n = 1 in Eq (5.10),

The NITM solution with three terms approximation within Caputo Fabrizio operator is

the series form approximate solution to three term iterations is given as

5.2.   Example

Consider fractional order 3D Navier Stokes equation of the form^[38]

having initial condition

The exact solution of the Eq (5.12), is

where

Case 1: The solution of system (5.12), using NITM within C-FDO.

Applying AT on both sides of Eq (5.12), we obtain

applying the inverse AT on Eq (5.13), we have

so the iterative scheme is

put n = 0 in Eq (5.15), we have

Put n = 1 in Eq (5.15), we have

after simplification we get,

The approximate NITM solution using the Caputo operator with three terms iterations

Case 2: NITM solution of system (5.12) within AB operator.

ApplyAT to both sides of Eq (5.12), we get

applying the inverse AT on Eq (5.16), we have

so the iterative scheme is

put n = 0 in Eq (5.18), we have

Put n = 1 in Eq (5.18), we have

The NITM solution with three terms approximation within the Atangana-Baleanu operator is

Case 3: The solution of system(5.12), using NITM with a Caputo Fabrizio operator.

Applying AT to Eq (5.12), we obtain

applying the inverse AT on Eq (5.19), we have

so the iterative scheme is

put n = 0 in Eq (5.21) we have

Put n = 1 in Eq (5.21), we have

The NITM solution with three terms approximation within Caputo Fabrizio operator is

the series form solution is obtain by

6.   Results and discussion

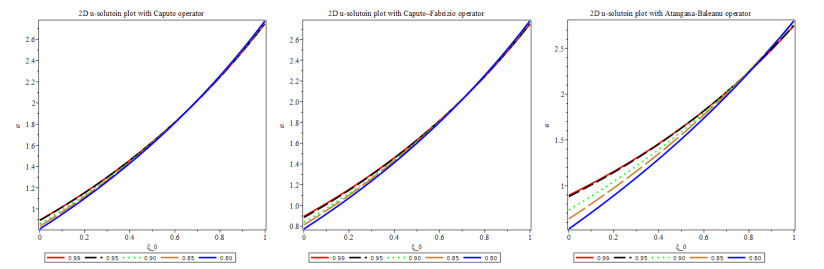
We used an approximate analytical scheme, and the obtained solution is displayed through graphs and tables. Figure 1 shows the comparison 2D solution plots of the example (5.1) for different fractional order $\delta$ and with different fractional operators. Figures 2–4 are the solution plots for the N.S. system (5.2) at different fractional operators and various fractional order $\delta$ respectively. Figure 5 is the compression 2D-solution plots of exact and approximate solutions for the N.S. system (5.2). Table 1 is the comparison table of example (5.1) between the exact solution and approximate solution at $\delta = 1$ and with three different operators. Table 2 shows the numerical simulation of example (5.1) at different fractional orders, space and time levels while the derivative is used in the Caputo sense. Tables 3–5 represent a numerical simulation of example (5.2) at different fractional operators, orders, space, and time levels for ${u}$, $\mu$, and $\nu$ respectively. Graphs and tables show the accuracy of the proposed techniques. All the numerical simulations are done by Maple code on maple 2023.

7.   Conclusions

In the present paper, the fractional views of the three-dimensional Navier-Stokes and non-homogeneous equations are examined by using the new iterative transform method (NITM). The fractional derivatives are replaced with different operators such as Atangana Baleanu, Caputo, and Caputo Fabrizio operators. The nonlinear terms in each problem are represented by the Jafaari polynomial, which has direct implementation over the entire problem. The solutions are obtained with the singular and non-singular kernels of the operators and confirm their effectiveness in the simulation of every problem. It is observed that the solutions under different operators are identical and verify the valuable dynamics of the suggested problems. Considering the benefits of the present operators, the expansion will be greatly appreciated in order to add new operators and approaches in the future. The current approach can be extended to solve other fractional problems due to its simple and straightforward implementation.

Nomenclature

Acknowledgements

The authors acknowledge the financial support provided by the Center of Excellence in Theoretical and Computational Science (TaCS-CoE), KMUTT. Moreover, this research project is supported by Thailand Science Research and Innovation (TSRI) Basic Research Fund: Fiscal year 2023 under project number FRB660073/0164.

Authors contribution

Qasim khan (Methodology, Software, Conceptualization, & Writing original draft);

Anthony Suen (Supervision, Writing-review);

Hassan Khan (Conceptualization, Draft Writing);

Poom Kummam (Funding, Draft Writing).

Conflict of interest

The authors declare there are no conflicts of interest.