A robust study of the transmission dynamics of malaria through non-local and non-singular kernel

1.   Introduction

It is reported that malaria is a life-threatening vector-borne infection transfer through mosquitoes. Female Anopheles mosquitoes carrying the disease bite humans and spread the disease to people. On the other hand, there are alternative ways to get malaria, including blood transfusions from people who have been exposed to the plasmodium parasite and pregnant women who transmit the disease to their offspring through the placenta ^[1]. In 2018, there were 405 thousand fatalities globally due to malaria, according to the World Health Organization ^[2]. Only a half-degree Celsius rise in temperature has the potential to boost mosquito populations by 30% to 100% ^[3]. Malaria may spread more swiftly at hotter temperatures because mosquitoes and the parasites that cause it can grow more quickly. But if the temperature rises too much, neither the malaria parasite nor the insects can live. The spread of malaria is also impacted by fixed water, such as dams, which serve as mosquito breeding grounds. Increased larval reproduction brought on by increased rainfall results in an increase in the number of malaria-carrying mosquitoes ^[4].

Asymptomatic malaria is frequently disregarded in mathematical models, which contributes to one of the difficulties of malaria ^[5,6,7]. It is well-known that asymptomatic patients are not identified and treated while still transmitting the illness, carrier cases can have a considerable influence on the dynamics of the disease ^[8,9,10]. Sometimes, the importance and significance of asymptomatic cases are discussed by different researchers in the literature ^[11,12,13]. Persons can be portrayed as partially immune individuals who are asymptomatically infected since asymptomatic infections frequently arise as a result of partial immunity to malaria brought on by repeated exposures ^[14,15]. By applying mathematical modelling approaches, several epidemic models have been utilised to evaluate the effects of malaria management tactics on its transmission ^[15,16]. The authors in ^[17], formulated an epidemic model of malaria in order to analyse the impact of distinct factors. In ^[18], the researchers introduced age age-structured models that simulates the transmission pathways between hosts and vectors in an endemic situation is developed in order to better understand how the development of immunity affects reported epidemiological trends. Here, by including both symptomatic and asymptomatic cases of the disease, and we want to comprehend how asymptomatic malaria patients influence the dynamics of the illness. An individual may get several hundred infected mosquito bites annually in areas with significant malaria transmission ^[19,20]. There is a danger of superinfection in these areas because mosquitoes frequently transfer Plasmodium sporozoites to people who already have the parasites from a prior infection. Super-infections have also been noted to occur substantially more frequently in asymptomatic carriers than in clinical cases ^[20]. The main objective of this work is to take into account the effect of asymptomatic carriers and reinfection that is rarely included in the dynamics of malaria, the potential for a superinfection to cause asymptomatic people to become symptomatic.

There are several malaria prevention and treatment options available today ^[21]. These prevention strategies include giving malaria patients medication, using insect repellents, using bednets coated with an insecticide, spraying with insecticides, and more. Disease control strategies have a large impact, and as a result, they have been extensively researched using mathematical models ^[22,23]. Some of the most often discussed issues in malaria management relate to the efficacy and ideal timing of certain drugs ^[24] as well as general approaches to therapy ^[25,26]. Once malaria has been contracted, one of the major ways to battle it is by the use of medicine. This helps to minimise morbidity and mortality as well as the spread of the disease. Use of bed nets is another widely researched preventative measure. The most effective malaria preventive strategy that lowers population-level infections is probably the use of bed-nets. Numerous authors conducted research on significant problems pertaining to the usage and efficiency of bed nets ^[26]. The relevance of creating time-dependent optimum control techniques, which are intended to reduce the negative impact of the infection on daily life as well as the expense of implementing the controls, has also been acknowledged by mathematical models in addition to time-independent treatment strategies. For malaria, optimal control can be achieved with any of the available preventive strategies, although it is most frequently achieved with the application of bed-nets ^[27,28], therapy ^[29,30], and occasionally even with immunisation, which isn't yet a widely used malaria control measure ^[15]. The authors utilized an optimum control for bed-net use and an optimal control for therapy in this study. They investigated how the asymptomatic class and optimum control interact. The control measures introduced in ^[31] are effective, however, our main target is to conceptualize the role of asymptomatic carriers and index of memory in the transmission of malaria.

Our model is more sophisticated than this one because it allows for the superinfection of asymptomatic people. This trait significantly affects the dynamics of the disease and the effectiveness of control methods. Furthermore, memory is an important factor in the transmission route of vector-borne infections ^[32,33] and fractional derivatives have the ability to represent the role of memory in the mathematical model of biological phenomena. Moreover, numerous real-world problems are effectively represented in the fields of biology, economics, mathematics, physics, and other disciplines through fractional derivatives ^[34,35]. In the literature, new fractional operators are introduced and efficiently used by researchers ^[36,37]. These novel operators illustrate the dynamics of real-word problem with non-local and non-singular kernel. The Atangana-Baleanu (ABC) fractional nonlocal operator used in this study is a particular case of the new generalized Hattaf fractional (GHF) operator presented in ^[38,39]. In order to achieve more precise results and investigate the role of memory in the infection's transmission pathway, we therefore concentrated on the dynamics of malarial transmission within the fractional framework.

The article is structured as: The foundational results and theory of fractional calculus are introduced in Section 2. To provide a more accurate picture of the malaria transmission dynamics, we set up a compartmental model in Section 3 with reinfection, asymptomatic carriers and index of memory. The recommended dynamics of malaria is examined for basic analytic results in Section 3 of this work. In Section 4, the uniqueness and existence of the solution is interrogated in detail. Section 5 derives appropriate conditions for Ulam-Hyers stability. In Section 6, we offered a numerical method for resolving the recommended system of malaria, and we numerically examined the dynamics of malaria as a function of various parameters. At the end, the conclusion and ending remarks are included in Section 7.

2.   Fractional dynamics of malaria

In evaluation of malaria dynamics, the host population is classified into five groups: susceptible $S_h(t)$, exposed $E_h(t)$, infected ($I_h(t)$, asymptomatic $A_h(t)$, and temporarily immune $R_h(t)$. At time $t$, there are three groups of mosquitoes: susceptible $S_v(t),$ exposed $E_v(t),$ and infected $I_v(t))$ vectors. Further, we have the following

and

In areas where malaria is prevalent, the average lifespan of people is $\frac{1}{\mu_h}$, where $\mu_h$ is the human population's natural death rate. Similar to humans, mosquitoes have an average lifespan of $\frac{1}{\mu_v}$, where $\mu_v$ represents the natural death rate of mosquitoes. The symbols $\Lambda_h$ and $\Lambda_v$ are the rate of recruitment of hosts and vectors, respectively. New births and immigration serve as sources of new human recruitment.

Malaria can be transmitted by an asymptomatic person, albeit frequently to a lesser level than by a sick person. In the next step, the forces of infection are defined by $\lambda_{h}$ and $\lambda_{v}$ for hosts and vectors, given by

The average number of mosquito bites per person is multiplied by the probability that an infectious human virus will pass to a mosquito that is susceptible to it to determine the input factor $\beta_h$, which indicates the effective contact rate of hosts. Similar to that, $\beta_v$, also known as the effective contact rate of vectors, is determined by adding the mosquito population density to the risk of transmission per bite. Here, S shows how asymptomatic individuals' infectivity differs from that of individuals in the symptomatic class $I_h$, either by growing or by declining (or increasing). Under the aforementioned assumptions, the transmission dynamics of malaria is given by

with

and

The interaction of humans and mosquitoes are modeled in the above equations to understand how the subgroups of these population change with time and to check how the infection spread in the society. One of the most recently popular fields of mathematics is the area of fractional derivatives, which deals with real and complex order derivatives and integrals. Despite the fact that it is as old as the field of ordinary calculus, this type of calculus has piqued the interest of researchers from a variety of professions due to the astonishing outcomes obtained when some of these scholars applied fractional operators to describe real-world difficulties. To more properly address the transmission phenomenon, we characterize the dynamics of malaria using Caputo-derivatives as follows

where $^{ABC}_0 D_t ^{\zeta}$ symbolizes Liouville-Caputo's derivative and $\zeta$ is the fractional order. In this formulation, the order of the operator is indicated by $\zeta$. One can easily prove that the solution of the recommended model of malaria are positive and bounded.

3.   Fundamental results

Condensed form of the notations shown below will be utilised

and

We define a Banach space for further analysis of the system. When $0 \leq t \leq T < \infty$, $W = [0, T]$ and $F = B(w, R_8)$ are used to define the Banach space, moreover, the supremum norm is as

with

and

Definition 3.1. Assume that $\sigma \in H^{1}(0, T)$ and $\varphi \in (0, 1]$. Then, for a function $\sigma$, according to the following definition, the left ABC derivative of order $\varphi$ with a lower constraint of 0 is

where $0 < \varphi \leq 1$ and $N(0) = N(1) = 1$ are satisfied by the normalisation function $N(\varphi)$, which is defined as $N(\varphi) = \frac{\varphi}{2-\varphi}$. The Mittag-Leffler function described by the series is also known as $\mathfrak{E}$.

where $\Gamma(.)$ in the above indicates gamma function and $Re (\varphi) > 0$.

Definition 3.2. Assume that $\sigma \in L^{1}(0, T)$ and $\varphi \in (0, 1]$. Then, for a function $\sigma$, the left ABC integral having zero lower limit of order $\varphi$ is defined by

Definition 3.3. Let $\sigma(t)$ be a given function, them Laplace transform for ABC framework is given by

Lemma 3.1. The solution of the below mentioned problem

for $\varphi \in(0, 1]$ is

Definition 3.4. Assume $\mathcal{F}$ is a Banach space and take the operator $\prod: \mathcal{F} \rightarrow \mathcal{F}$, If there is a constant $k > 0$ in a manner that

in which the Lipschitz constant is indicated by $\prod$. The word $"\prod"$ is a contraction if $\kappa < 1$.

Theorem 3.1. In case $\prod: F \rightarrow F$ is a contraction mapping where $F$ is a Banach space. Then, one can find a fixed point of $\prod$.

Theorem 3.2. Assume the Banach space $F$ have a non-empty, convex and closed subsect $P$. Also consider that $\mathfrak{E}$ and $\mathfrak{F}$ translate $P$ into $F$ and that

(i). $\mathfrak{E}u+\mathfrak{F}v\in P$ for all $\Upsilon_{1}, \Upsilon_{2} \in M$;

(ii). $\mathfrak{E}$ is continuous and compact;

(iii). The contraction mapping is $\mathfrak{F}$. Then, $\Upsilon \in P$ exists in a manner that $\mathfrak{E}\Upsilon + \mathfrak{F}\Upsilon = \Upsilon$.

3.1.   Analysis of the model

An epidemiologically significant threshold value that assesses the potential for a disease to infect a population is the basic reproduction number $\mathcal{R}_0$. First, we determine the infection-free steady-state $\mathcal{E}_{0} = (\frac{\Lambda_{h}}{\mu_{h}}, 0, 0, 0, 0, \frac{\Lambda_{v}}{\mu_{v}}, 0, 0)$. The reproduction parameter through next generation matrix technique can be determined as

From the above, we can easily determine $\mathcal{F}\mathcal{V}^{-1}$, given by

and

where

The spectral radius of $\mathcal{F}\mathcal{V}^{-1}$ is the reproduction value given by

Theorem 3.3. In the case of $\mathcal{R}_0 < 1$, the infection-free steady-state of system (2.2) is locally asymptotically stable and unstable in other cases.

Proof. To prove the local stability of DFE for $\mathcal{R}_0 < 1$, we utilize the well-known Jacobian matrix method at DFE as follows

The first eigenvalue from the above is $-\mu_h$ which is negative, further, we have the following

The second and third eigenvalues from the above are $-(\mu_h+\gamma_h)$ and $-\mu_v$. These eigenvalues are negative and the rest can be deduced from the below sub-matrix

The characteristic equation of the above sub-matrix $J_{2}$ is given by

Here, we utilize the criteria of Routh-Hurwitz. It is easy to show that the coefficient $A_i, i = 1, 2, \dots 5$ satisfies the conditions of Routh-Hurwitz for $\mathcal{R}_0 < 1$. This implies that the real part of all eigenvalues are negative and the infection-free steady-state is locally asymptotically stable and unstable in other cases.

4.   Investigation of the solutions

Here, using the fixed-point method, we discuss the existence and uniqueness results for the model (2.2). The recommended model (2.2) can be represented as

where

We can express system (2.2) as

where

Here, the transposition operation is indicated by the sign $A^{T}$. Application of Lemma 3.1 lead to the system (4.3) to the following

Using the Lipschitzian premise for existence uniqueness and some growth conditions as:

(H1) One can find $\mu_{\mathcal{H}}$ and $\eta_{\mathcal{H}}$ in a manner that

(H2) One can find a constant $L_{\mathcal{H}} > 0$ in a manner that

Theorem 4.1. Assume that the hypothesis H1 and H2 holds true. Furthermore, if $\frac{1-\varphi}{N(\varphi)}L_\mathcal{H}$, $\theta_1 < 1$, there exists at least one solution of the integral Eq (4.5) equivalent of the model (2.2) given by

Proof. Consider $\mathbb{B_{\lambda}} = { \Upsilon\in F : ||\Upsilon|| \leq \lambda}$ is closed convex set with $\lambda \geq \frac{\theta_{2}}{1-\theta_{1}}$, where

We define the operators $\prod_{1} and \prod_{2}$ as

where $F = \Pi_{1}+\Pi_{2}$. We now provide the evidence in the following stages:

Step 1. $\Pi_{1}\Upsilon_{1}+\Pi_{2} \in \mathbb{B_{\varphi}}$, for $\Pi_{1}, \Pi_{2} \in \mathbb{B_{\varphi}}$.

Clearly, the Eqs (4.6) and (4.7) combine with the hypothesis H1 provides the following

This confirms that $\Pi_{1}\Upsilon_{1}+\Pi_{2}\Upsilon_{2} \in \mathbb{B_{\lambda}}$.

Step 2. We show that $\Pi_{1}$ is contraction.

Assume that $\Upsilon, \Upsilon^{\Upsilon} \in \mathbb{B_{\lambda}}$. Then the hypothesis H2 implies that

As $\frac{1-\varphi}{N(\varphi)}L_{\mathcal{H}} < 1$, the contraction of the mapping $\Pi_{1}$ is proved.

Step 3. We demonstrate the relative compactness of $\Pi_2$.

We demonstrate that $\Pi_2$ is continuous, uniformly bounded, and equicontinuous in order to support this claim. The continuity of $\Upsilon(t)$ insures the continuity of $\Pi_{2}\Upsilon(t)$. Further, we consider $\Upsilon \in\mathbb{B_{\lambda}}$, then

Hence, $\Pi_{2}$ is uniformly bounded on $\mathbb{B_{\lambda}}$. In the next step, we will prove that $\Pi_{2}$ is equicontinuous. Let $\Upsilon \in \mathbb{B_{\lambda}}$ and $t_{1}, t_{2} \in [0, T]$ in a manner that $t_{1} < t_{2}$, then

The right-hand side of the aforementioned inequality goes toward zero as $t_1\rightarrow t_2$. As a consequences of this, $\Pi_2$ is totally continuous and reasonably compact according to the Arzela-Ascoli theorem. The Eq (4.5) therefore has at least one solution according to Theorem 3.2 which implies that there exists at least one solution of the recommended model.

Theorem 4.2. The uniqueness of the solution of (2.2) has been insured through the uniqueness of the solution of the integral Eq (4.5) through the hypothesis H1 if

Proof. To prove the theorem, we choose $\Pi:F\rightarrow F$ given by

Further, take $\Upsilon$ and $\Upsilon^{*}$ in F and the time $0 \leq t \leq T$. Then, we have

As a result of (4.12), $\Pi$ is decreasing. Thus, there is only one solution to the integral Eq (4.5) which implies that there exists a unique solution of the model (2.2) of malaria.

5.   Ulam-Hyers stability

Ulam was the one who first proposed the idea of Ulam stability ^[40,41]. The stability mentioned above has then been examined for different fractional operators in several research works; we mention some of them here like ^[42,43,44]. Furthermore, as stability is necessary for an approximate solution, we attempt to achieve Ulam type stability for the model (2.2) by applying nonlinear functional analysis.

Definition 5.1. Assume there is a $\lambda > 0$ and if the $\widetilde{\Upsilon} \in F$ is greater than zero for some $\epsilon > 0$ with

then, the recommended system (2.2) of malaria is $U-H$ stable. Afterwards, $\Upsilon \in F$ exists satisfying the model (2.2) with the below condition for initial values

such that

where

Remark 5.1. Suppose $g \in C[0, T]$ in a manner that $g(0) = 0$ and small perturbation combine with the below

(a). For $\epsilon > 0$, $|g(t)| \leq \epsilon$, for $t \in [0, T]$.

(b). At $t \in [0, T]$, then

in which $g(t) = (g_{1}(t), g_{2}(t), g_{3}(t), g_{4}(t), g_{5}(t), g_{6}(t), g_{7}(t), g_{8}(t))^{T}$.

Lemma 5.1. the solution to the problem

verifies the below

in which $\widetilde{\Upsilon_{g}}(t)$ is a solution of (5.4), $\widetilde{\Upsilon}(t)$ is fulfills (5.1-a) with $\kappa: = \left(\frac{\Gamma(\alpha)(1-\varphi)+T^{\varphi}}{N(\varphi)\Gamma(\varphi)}\right).$

Proof. The answer to (5.4) is provided by Remark 5.1 and Lemma 3.1.

Also, we have

Remark 5.1 implies that

Theorem 5.1. In accordance with the assumptions of Theorem 4.2, the suggested model (2.2) of malaria will be $U-H$ stable.

Proof. Assume $\widetilde{\Upsilon}\in F$ be the solution of (5.1-a) and $\Upsilon \in F$ be the singular solution of the system (2.2-a) with the following

Then, we have

Lemma 5.1 and hypothesis H1 implies that

Further, Remark 5.1 give us

which implies

Due to $\theta_{3} < 1$. For $\lambda = \frac{2\kappa}{1-\theta_{3}}$, we get $||\widetilde{\Upsilon}-\Upsilon||\leq\lambda\epsilon$ and as a result of this, the suggested model (2.2) of malaria is $U-H$ stable.

6.   Numerical scheme

Here, we provide numerical method for the solution of our system (2.2) of malaria infection. The proposed approach is then used to acquire the numerical simulations. We use the modified fractional version of the Adams-Baschforth approach to achieve this purpose.

First, the model (2.2) is transformed into integral equations applying the initial conditions and fractional integral operator as

which gives

In the next step, we proceed with the first equation of model (6.2) in order to obtain an iterative approach as follows:

Choose $t = t_{s+1}$, for $s = 0, 1, 2, \dots.$ We have

The function $P_{1}(\xi, S_{B}(\xi))$ is now approximated on the interval $[t_{m}, t_{m+1}]$ as seen by the following interpolation polynomial:

which implies

In the following, we compute the integrals $I_{m-1, \varphi}$ and $I_{m, \varphi}$ as

Put $t_{m} = mh$ we get

and

Substituting (6.7) and (6.8) into (6.4), we get

In the same way

and

The above numerical scheme is utilized to highlight the solution pathways of the recommended system of malaria. Several numerical scheme have been developed for different fractional operators ^[45,46]. Here, we mainly focussed to identify the key factors of the system through numerical findings. However, the convergent, stability of the proposed method and comparison analysis with other numerical methods will be considered in the future work. Several simulations are carried out to identify the role of input factors on the system of malaria infection. The values of input parameters are assumed for numerical purposes while the value of state-variables are taken to be $S_h(0) = 1200, E_h(0) = 150, I_h(0) = 130, A_h(0) = 80, R_h(0) = 100, S_v(0) = 1000, E_v(0) = 300$ and $I_v(0) = 200$.

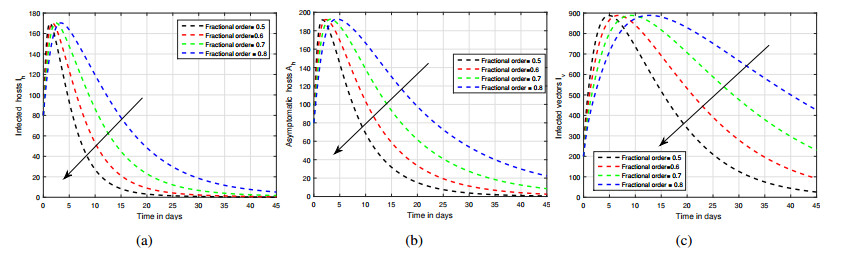
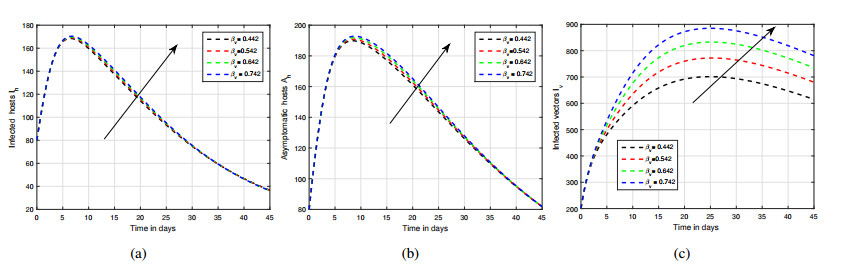
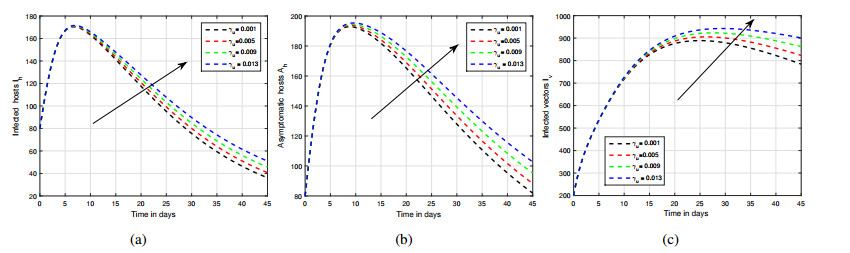
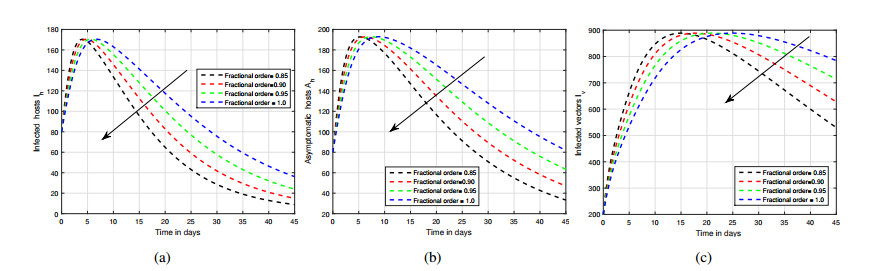
The impact of memory on the time series of malaria infection was visually depicted in the first simulation. Different memory values were used to represent the plot of the affected individuals of both the classes illustrated in Figures 1 and 2. We observed that lower value of memory decrease the level of infection. The policymakers are advised to use this parameter since it appears to be beneficial at reducing infection. In second simulation represent in Figure 3, the impact of the carrier fraction on the dynamics has been visualized. It can be seen that the increase of this value increase the infection in the society. Therefore, this parameter is dangerous and can spread the infection of malaria to uninfected regions. The effect of input factor $\beta_v$ has been conceptualized in third simulations in Figure 4. We assumed different values of $\beta_v$ and showed its impact on the dynamics of the system. The role of the immunity-losing rate has been visualised in final simulation illustrated in Figure 5. The results indicate that this parameter is also sensitive and can make the control of the infection difficult. On the basis of our numerical results, we highlighted the most critical scenarios of the system for the control and prevention of the malaria infection in the society.

7.   Concluding remarks

In this work, we structured the dynamics of malaria with asymptomatic carriers and reinfection after recovery through non-integer derivative. This paper aims to study in detail a malaria epidemic model which estimates the transmissibility of malaria infection using a nonsingular fractional derivative. We investigated the stead-states and obtained basic reproduction number of the system. We have shown that the infection-free steady-state is locally stable for $\mathcal{R}_0 < 1$ and vice versa. The well-known Krasnoselskii and Banach fixed-point theorems has been used to ensure the existence and uniqueness of the considered model. In addition to this, several stability outcomes of the Ulam type have been established. We simulated the outcomes corresponding to various values of input parameters using the fractional Adams-Bashforth approach. The role of input factors of the recommended system has been visualized. From the simulation, we deduced that the control of fractional parameter reduced the level of infection in the society. The parameter $\alpha$ also contribute significantly and is dangerous in the sense to increase the risk of infection. The results that have been shown may aid in a more thorough knowledge of the current situation and in the development of preventative measures that will minimise infection. In the future work, we will validate our model of malaria infection with real data on availability. We will also represent the recommended model of malaria with time delay to show the influence of incubation and maturation delay on the dynamics of malaria.

Acknowledgement

This research work was funded by Institutional Fund Projects under grant IFPIP: 1282-130-1443. The authors gratefully acknowledge technical and financial support provided by the Ministry of Education and King Abdulaziz University, DSR, Jeddah, Saudi Arabia.

Conflict of interest

The authors declare no conflict of interest.