Analytical and numerical negative boundedness of fractional differences with Mittag–Leffler kernel

1.   Introduction

Define the sets $\mathbb{N}_{a}$ and $\mathbb{N}_{a}^{b}$, for any $a, b \in \mathbb{R}$ with $b-a$ a nonnegative integer, to be $\mathbb{N}_{a}: = \lbrace a, a+1, a+2, \dots \rbrace$ and $\mathbb{N}_{a}^{b}: = \lbrace a, a+1, a+2, \dots, b \rbrace$, respectively. It worth recalling that the nabla (or backward) difference of a function $\mathrm{g}\ : \ \mathbb{N}_a\rightarrow \mathbb{R}$ can be expressed as follows

As is known, there is a strong correlation between the sign of the nabla operator and whether $\mathrm{g}$ is either monotone increasing or monotone decreasing. For example, if $(\nabla\mathrm{g})({t})\ge0$, then $\mathrm{g}$ is increasing function on $\mathbb{N}_{a}$.

In recent years a nonlocal version of the discrete calculus has been proposed. This nonlocal version is known as the "discrete fractional calculus", a research area popularized by the seminal papers of Atici and Eloe ^[9,10,11] in the late 2000s and then further extended by the subsequent work of Lizama ^[25]. One of the reasons for the interest in the discrete fractional calculus is its emerging applications in biological mathematics – see, for example, the recent work of Atici, et al. ^[7,8], in which the authors apply discrete fractional calculus to the modeling of tumors.

To understand the nonlocal nature of the fractional calculus, consider a commonly utilized version of the discrete fractional difference – namely, for $\nu > 0$ the $\nu$-th order Riemann-Liouville difference, which for a function $\mathrm{g}\ : \ \mathbb{N}_a\rightarrow \mathbb{R}$ is denoted by $_a^{{\rm{RL}}}\Delta ^{\nu}\mathrm{g}$ and defined pointwise by

where a positive integer $N$ satisfying $N-1 < \nu\le N$. The key fact about (1.1) is that it is nonlocal, very much unlike the integer-order difference mentioned in the first paragraph of this section. Consequently, the relationships between the sign of $\big(_a^{{\rm{RL}}}\Delta ^{\nu}\mathrm{g}\big)(t)$ and the monotone behavior of $g$ are quite muddled and complex. This question was initially investigated by Dahal and Goodrich ^[13] in 2014, and then subsequently investigated by many authors including Abdeljawad and Baleanu ^[1], Bravo, Lizama, and Rueda ^[12], Goodrich and Jonnalagadda ^[16], Goodrich and Lizama ^[18,19], Goodrich and Muellner ^[22], and Jia, Erbe, and Peterson ^[15,24].

Very recently Goodrich, Lyons, and Velcsov ^[20] together with Jonnalagadda ^[17] and Scapellato ^[21] demonstrated that a function can increase (under certain conditions) even if its fractional difference is negative. This is something that plainly cannot happen in the integer-order case. And this phenomenon provides further evidence of the highly complicating nature of the nonlocal structure of fractional-order difference operators. It also has serious implications for the use of fractional calculus in modeling since one of the most important uses of calculus in modeling is to identify where functions are increasing or decreasing.

So, all in all, there is a large body of evidence that nonlocal discrete operators behave in ways that are very complicated, particularly as concerns their ability to detect the qualitative behaviors of the functions on which they operate. At the same time, there are a variety of definitions for discrete fractional differences and sums. Therefore, it is relevant to determine whether these aberrant behaviors are exhibited by all such nonlocal difference operators – or only some of them.

Consequently, in this brief note, we propose to continue investigating these questions in the specific context of the fractional difference with exponential-type kernels (see Definitions 2.1 and 2.2). In particular, we demonstrate that, as with other definitions of the fractional difference, the type of difference studied here can be negative even though the function on which it acts is increasing, and this observation confirms that fractional differences with Mittag-Leffler kernels exhibit the same sort of aberrant behavior as other nonlocal difference operators. This complements not only the already mentioned reference ^[17] but also builds upon earlier work by Abdeljawad, Al-Mdallal, and Hajji ^[6]. And it continues to demonstrate some of the surprising properties of this class of discrete nonlocal operators. To see the development of fractional calculus with Mittag-Leffler kernels we advise the readers to see the recently published articles ^[28,29].

2.   Essential tools and main results

In this section, we begin with recalling the necessary fundamental discrete operators for our main results briefly. The interest reader may visit the monograph ^[23] by Goodrich and Peterson and recently published articles ^[1,2,3,26] for additional information and mathematical background regarding the discrete fractional calculus.

The first and well-known definition in this article is the discrete Mittag-Leffler functions. We then provide the definition of the discrete fractional difference defined using the Mittag-Leffler kernel on the set $\mathbb{N}_{a}$. The discrete Mittag-Leffler function of $2-$parameters is given by (see ^[27]):

for $\lambda\in\mathbb{R}$ such that $1 > |\lambda|$, and $\nu, \beta, {t}\in\mathbb{C}$ such that $\mathop{{\rm{Re}}}\nolimits(\nu) > 0$. It is essential to see that ${t}^{\overline{\nu}}$ is the rising function and given as follows

for $\nu\in\mathbb{R}$ and ${t}\in\mathbb{R}$ apart from the elements $\{\ldots, -2, -1, 0\}$. As a special case of the above definition, the discrete Mittag-Leffler function of $1-$parameter is given as follows

Remark 2.1. Considering Remark 1 in ^[4], we can obtain the following for $\lambda_{1} = -\frac{\nu-1}{2-\nu}$ and $1 < \nu < \; \frac{3}{2}:$

● $\mathtt{E}_{\overline{{\nu-1}}}(\lambda_{1}, 0) = 1$,

● $\mathtt{E}_{\overline{{\nu-1}}}(\lambda_{1}, 1) = 2-\nu$,

● $\mathtt{E}_{\overline{{\nu-1}}}(\lambda_{1}, 2) = \nu\, (2-\nu)^{2}$,

● $\mathtt{E}_{\overline{{\nu-1}}}(\lambda_{1}, 3) = \frac{2-\nu}{2}\left[(\nu-1)^3(2\nu-3)-3(\nu-1)^2+2\right]$,

● $0 < \mathtt{E}_{\overline{{\nu-1}}}(\lambda_{1}, {t}) < 1$ for each $1 < \nu < \frac{3}{2}$ and ${t} = 1, 2, 3, \cdots$. At the same time, we have that $\mathtt{E}_{\overline{{\nu-1}}}(\lambda_{1}, {t})$ is monotonically decreasing for each $1 < \nu < \frac{3}{2}$ and ${t} = 0, 1, 2, \cdots$.

Definition 2.1. (see [1,Definition 2.24]) Let $0 < \nu < \frac{1}{2}$ and $\lambda_{0} = -\frac{\nu}{1-\nu}$. Then, the discrete fractional difference operators with Mittag-Leffler kernels of order $\nu$ denoted by $_a^{{\rm{ABC}}}\nabla^{\nu} \mathrm{g}$ and $_a^{{\rm{ABR}}}\nabla^{\nu} \mathrm{g}$, respectively, defined by

and

for each $t\in \mathbb{N}_{a+1}$. Here the function $\nu\mapsto\mathrm{H}(\nu)$ is a normalization constant satisfying $0 < \mathrm{H}(\nu)$.

Definition 2.2. (see ^[5]) For $\mathrm{g}:\mathbb{N}_{a-\aleph}\to\mathbb{R}$ with $\aleph < \nu\leq \aleph+\frac{1}{2}$ and $\aleph\in\mathbb{N}_{0}$, Then, the discrete fractional difference operators with Mittag-Leffler kernels of order $\nu$, respectively, are defined by

and

for $t\in\mathbb{N}_{a+1}$. Here $\lambda_{\aleph} = -\frac{\nu-\aleph}{\aleph+1-\nu}$.

The following is the essential lemma which brings us to the main results.

Lemma 2.1. Let the function $\mathrm{g}$ be defined on $\mathbb{N}_{a}$ and $1 < \nu < \frac{3}{2}$. Then we have that

for each $t\in\mathbb{N}_{a+3}$.

Proof. From Definitions 2.1 and 2.2, the following can be deduced for $1 < \nu < \frac{3}{2}:$

for each $t\in\mathbb{N}_{a+3}$, and hence the proof is complete.

The first main result we present, Theorem 2.1, demonstrates that $\left(_a^{{\rm{ABR}}}\nabla^{\nu} \mathrm{g}\right) (a+3)$ can be negative even though $(\nabla\mathrm{g})(a+3) > 0$ – cf., [14,Theorem 3.1].

Theorem 2.1. Let the function $\mathrm{g}$ be defined on $\mathbb{N}_{a}$, and let $1 < \nu < 1.5$ and $\varepsilon > 0$. Assume that

If $\big(\nabla\mathrm{g}\big)(a+1)\ge0$, $\bigl(\nabla \mathrm{g}\bigr)(a+2) \geq 0$, and $\frac{1}{2}(\nu-1)^{2}\bigl(2\nu^{2}-5\nu+2\bigr) < -\varepsilon,$ then $\left(\nabla \mathrm{g}\right)(a+3) \geq 0.$

Proof. Due to Lemma 2.1 and the condition (2.1) we get

for each $t\in \mathbb{N}_{a+3}$. Setting $t = a+3$ in (2.2), yields

Since $\left(\nabla \mathrm{g}\right) (a+2) \geq 0$ by assumption, it follows that

Also, we know that $\bigl(\nabla \mathrm{g}\bigr)(a+1)\ge0$. So, we can use the inequalities (2.2) and (2.3) to deduce that $\left(\nabla \mathrm{g}\right)(a+3) \geq 0$, using especially that

by the assumption given in the statement of the theorem, and this ends the proof.

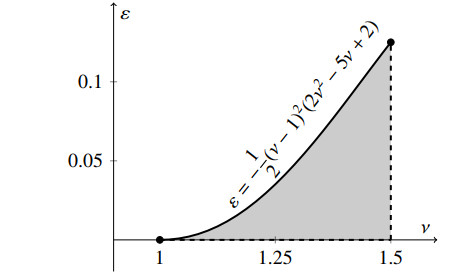
Remark 2.2. Figure 1 shows the graph of $\nu\mapsto-\frac{1}{2}(\nu-1)^{2}\bigl(2\nu^{2}-5\nu+2\bigr)$ for $\nu\in\left(1, \frac{3}{2}\right)$. Observe that in order for Theorem 2.1 to be applied it must hold that $\varepsilon\in\left(0, -\frac{1}{2}(\nu-1)^{2}\bigl(2\nu^{2}-5\nu+2\bigr)\right)$ for a fixed $\nu\in\left(1, \frac{3}{2}\right)$. This admissible region for $\varepsilon$ is shown by the light grey region in the figure.

Now, define the set $\mathscr{F}_{\mathrm{k}, \varepsilon}\subseteq\left(1, \frac{3}{2}\right)$ as follows:

Lemma 2.2 proves that the nested collection $\left\lbrace\mathscr{F}_{\mathrm{k}, \varepsilon} \right\rbrace _{\mathrm{k} = a+1}^{\infty}$ is decreasing (whenever $\varepsilon > 0$). This is a phenomenon that has been observed in similar contexts (e.g., [14,Lemma 3.3], [20,Lemma 3.2], [21,Lemma 3.2]).

Lemma 2.2. Let $1 < \nu < \frac{3}{2}$. Then for each $\varepsilon > 0$ and $\mathrm{k}\in\mathbb{N}_{a+3}$ we have that $\mathscr{F}_{\mathrm{k}+1, \varepsilon} \subseteq \mathscr{F}_{\mathrm{k}, \varepsilon}$.

Proof. Let $\varepsilon > 0$ and $\nu \in \mathscr{F}_{\mathrm{k}+1, \varepsilon}$ be arbitrary for some fixed $\mathrm{k}\in\mathbb{N}_{a+3}$. Then we have

Since $\mathtt{E}_{\overline{{\nu-1, \nu-1}}}(\lambda_{1}, \mathrm{k}+1-a)$ is decreasing for each $\mathrm{k}\in\mathbb{N}_{a+3}$ (see ^[4]), $1 < \nu < \frac{3}{2}$, and $\lambda_{1} < 0$, we have

This implies that $\nu \in \mathscr{F}_{\mathrm{k}, \varepsilon}$, and, therefore, $\mathscr{F}_{\mathrm{k}+1, \varepsilon} \subseteq \mathscr{F}_{\mathrm{k}, \varepsilon}$. Thus, we have accomplished the result.

Theorem 2.1 and Lemma 2.2 now lead to the following corollary, which is the principal analytical result of this note – cf., [14,Corollary 3.4]. Corollary 2.1 asserts that the same pathological behavior observed with other discrete fractional differences carries over to the Mittag-Leffler kernel setting.

Corollary 2.1. Let the assumptions of Theorem 2.1 be fulfilled together with

for each $\nu\in\left(1, \frac{3}{2}\right)$, $t\in\mathbb{N}_{a+3}^{s}$ and some fixed $s\in\mathbb{N}_{a+3}$. Now, if we assume that $\big(\nabla\mathrm{g}\big)(a+1)\ge0$, $\bigl(\nabla \mathrm{g}\bigr)(a+2)\geq 0$, and $\nu \in \mathscr{F}_{s, \varepsilon}$, then we have $\left(\nabla \mathrm{g}\right)(t) \geq 0,$ for all $t\in\mathbb{N}_{a+1}^{s}$.

Proof. Due to the assumption that $\nu \in \mathscr{F}_{s, \varepsilon}$ and Lemma 2.2, we have

This leads to

for each $\mathrm{k}\in\mathbb{N}_{a+3}^{s}$.

We now can proceed by induction to complete the proof as follows. At first, for $t = a+3$ we can obtain $\left(\nabla \mathrm{g}\right)(a+3) \geq 0$ immediately as in Theorem 2.1 with the help of inequalities (2.4) and (2.5) just as in the proof of Theorem 2.1. Accordingly, we can continue and inductively iterate inequality (2.2) to get $\left(\nabla \mathrm{g}\right)(t) \geq 0,$ for all $t\in\mathbb{N}_{a+2}^{s}$ as requested. Note that in this last step we are using the fact that $\mathtt{E}_{\overline{\nu-1}}(\lambda_{1}, t-r+1)-\mathtt{E}_{\overline{\nu-1}}(\lambda_{1}, t-r)\geq 0,$ for each $(r, t)\in\mathbb{N}_{a+2}^{t-1}\times\mathbb{N}_{a+3}^{s}$, which is true since the partial function $t\mapsto\mathtt{E}_{\overline{\nu-1}}(\lambda_{1}, t)$ is decreasing – see Remark 2.1. Thus, we have completed the proof.

We next provide an example in order to demonstrate the application of the preceding ideas.

Example 2.1. Considering Lemma 2.1 with $t:= a+3$:

For $a = 0$, it follows that

If we take $\nu = 1.99, \mathrm{g}(0) = 0.01, \mathrm{g}(1) = 1.01, \mathrm{g}(2) = 1.001, \mathrm{g}(3) = 1.005$, and $\epsilon = 0.002$, we have

Note that $\left(_0^{{\rm{ABR}}}\nabla^{1.99} \mathrm{g}\right)(3) < 0$. Yet, as Theorem 2.1 correctly predicts, it, nonetheless, holds that $(\nabla \mathrm{g})(1) > 0$. Thus, the collection of functions to which Theorem 2.1 applies is non-void.

We conclude this note by providing a brief numerical analysis of the set $\mathscr{F}_{\mathrm{k}, \varepsilon}$, which plays a key role in Corollary 2.1; throughout we take $a = 0$ purely for convenience. Let us first consider Figure 2 above. This is a heat map, which identifies the cardinality of the set $\big\{k\in\mathbb{N}\ : \ \nu\in\mathscr{F}_{k, \varepsilon}\big\}$. It is worth mentioning that the warmer colors (i.e., oranges and reds) are associated to higher cardinality values as indicated by the vertical sidebar in Figure 2. We see that there is a concentration of larger cardinalities in a roughly triangular region as indicated in the figure. The largest cardinalities seem to be concentrated for $1.07\lessapprox\nu\lessapprox1.10$ and $\varepsilon > 0$ close to zero; this implies that the analytical results presented earlier (i.e., Corollary 2.1) should be valid for the greatest number of time steps ${t}$ when $\nu$ and $\varepsilon$ are in this region of the $(\nu, \varepsilon)$-parameter space.

On the other hand, Figures 3–6 plot the interval of $k$ values such that $\mathscr{F}_{k, \varepsilon}\neq\varnothing$ for different choices of both $\nu$ and $\varepsilon$. Consistent with the heat map in Figure 2, we see that there is a relative maximum when $\nu$ is away from the boundary values $\nu = 1$ and $\nu = 1.5$, though the precise value depends on the value of $\varepsilon$. In particular, as $\varepsilon\to0^+$, the maximum seems to approach about $1.07$, just as indicated by Figure 2. In addition, we see that the length of the intervals drops off sharply both as $\nu\to1^+$ and as $\nu\to1.5^-$ – again, precisely as depicted in Figure 2.

Finally, the data contained in Figures 2–6 is not entirely dissimilar from the observations in [14,Figures 1–4], which analyzed the Riemann-Liouville fractional nabla difference. In each of the Mittag-Leffler kernel and the Riemann-Liouville settings the $\nu$-values for which the respective monotonicity-type theorems – i.e., Corollary 2.1 and [14,Corollary 3.4] – seem to be most applicable are apparently concentrated for $\nu$ close to $1$. A possible, albeit non-rigorous, explanation for this common observation is that when $\nu\approx1$ the fractional difference is "more like" the first-order difference, which is closely connected to monotonicity. But we do not have a precise analytical explanation for this numerical observation, and we hope that this sort of curiosity provides motivation to analyze further these types of fractional difference operators in the future. Nonetheless, the results of this note show that this is a common feature across multiple types of fractional difference operators

.

3.   Conclusions

In this brief note we have demonstrated that the fractional difference with Mittag-Leffler kernel behaves in an aberrant manner, similar to that of other classes of nonlocal difference operators. In particular, we have shown that such a difference acting on a function can be negative even if the function on which it acts is increasing. This sort of unusual behavior is not possible when considering a local difference operator, but it seems to be an almost defining feature of nonlocal discrete operators as the results of this note demonstrate.

Acknowledgements

This research was supported by Taif University Researchers Supporting Project (No. TURSP-2020/155), Taif University, Taif, Saudi Arabia.

Conflicts of interest

The authors declare that they have no conflicts interests.