An extension of mathematical model for severity of rice blast disease

Saharat Tabonglek; Amir Khan; Usa Wannasingha Humphries; Saharat Tabonglek; Amir Khan; Usa Wannasingha Humphries

doi:10.3934/math.2023125

AIMS Mathematics

2023, Volume 8, Issue 1: 2419-2434. doi: 10.3934/math.2023125

Previous Article Next Article

Research article

An extension of mathematical model for severity of rice blast disease

Department of Mathematics, Faculty of Science, King Mongkut's University of Technology Thonburi, Bangkok 10140, Thailand

Received: 22 August 2022 Revised: 14 October 2022 Accepted: 19 October 2022 Published: 02 November 2022
MSC : 92D25, 92D30

This paper aims to extend the spore dispersal model to the Healthy-Latent-Infectious-Removed (HLIR) epidemic model for assessing the severity of rice blast disease. The model was solved by the Finite Difference Method (FDM). The results of the model were compared to data from the Prachinburi Rice Research Center (PRRC) on the severity of rice blast disease. Because of a small error, the comparison results showed good agreement between the PRRC data and the simulation by looking at the value of Willmott's index of agreement ( $d$ ). The first bed $d$ was 0.7166, while the second bed $d$ was 0.6421, indicating the model's performance. Furthermore, the optimal parameter, the fraction of spores deposited on the crop, was determined to be 0.173 and 0.016 for beds 1 and 2, respectively. The model can simulate and analyze rice blast outbreaks for educational purposes in future preparedness planning.

Keywords:

Citation: Saharat Tabonglek, Amir Khan, Usa Wannasingha Humphries. An extension of mathematical model for severity of rice blast disease[J]. AIMS Mathematics, 2023, 8(1): 2419-2434. doi: 10.3934/math.2023125

Related Papers:

[1]	Samia Bushnaq, Sajjad Ali, Kamal Shah, Muhammad Arif . Approximate solutions to nonlinear fractional order partial differential equations arising in ion-acoustic waves. AIMS Mathematics, 2019, 4(3): 721-739. doi: 10.3934/math.2019.3.721
[2]	M. Ali Akbar, Norhashidah Hj. Mohd. Ali, M. Tarikul Islam . Multiple closed form solutions to some fractional order nonlinear evolution equations in physics and plasma physics. AIMS Mathematics, 2019, 4(3): 397-411. doi: 10.3934/math.2019.3.397
[3]	M. Hafiz Uddin, M. Ali Akbar, Md. Ashrafuzzaman Khan, Md. Abdul Haque . New exact solitary wave solutions to the space-time fractional differential equations with conformable derivative. AIMS Mathematics, 2019, 4(2): 199-214. doi: 10.3934/math.2019.2.199
[4]	Mamta Kapoor, Nehad Ali Shah, Wajaree Weera . Analytical solution of time-fractional Schrödinger equations via Shehu Adomian Decomposition Method. AIMS Mathematics, 2022, 7(10): 19562-19596. doi: 10.3934/math.20221074
[5]	Rasool Shah, Abd-Allah Hyder, Naveed Iqbal, Thongchai Botmart . Fractional view evaluation system of Schrödinger-KdV equation by a comparative analysis. AIMS Mathematics, 2022, 7(11): 19846-19864. doi: 10.3934/math.20221087
[6]	Azzh Saad Alshehry, Humaira Yasmin, Rasool Shah, Roman Ullah, Asfandyar Khan . Numerical simulation and analysis of fractional-order Phi-Four equation. AIMS Mathematics, 2023, 8(11): 27175-27199. doi: 10.3934/math.20231390
[7]	Rehana Ashraf, Saima Rashid, Fahd Jarad, Ali Althobaiti . Numerical solutions of fuzzy equal width models via generalized fuzzy fractional derivative operators. AIMS Mathematics, 2022, 7(2): 2695-2728. doi: 10.3934/math.2022152
[8]	Yunmei Zhao, Yinghui He, Huizhang Yang . The two variable (φ/φ, 1/φ)-expansion method for solving the time-fractional partial differential equations. AIMS Mathematics, 2020, 5(5): 4121-4135. doi: 10.3934/math.2020264
[9]	Aslı Alkan, Halil Anaç . The novel numerical solutions for time-fractional Fornberg-Whitham equation by using fractional natural transform decomposition method. AIMS Mathematics, 2024, 9(9): 25333-25359. doi: 10.3934/math.20241237
[10]	Naher Mohammed A. Alsafri, Hamad Zogan . Probing the diversity of kink solitons in nonlinear generalised Zakharov-Kuznetsov-Benjamin-Bona-Mahony dynamical model. AIMS Mathematics, 2024, 9(12): 34886-34905. doi: 10.3934/math.20241661

Abstract

1. Introduction

Fractional partial differential equations (PDEs) have gained prominence and recognition in recent years, owing to their verified applicability in a wide range of relatively diverse domains of science and engineering. For instance, considering the nonlinear oscillation of fractional derivatives can be employed to model earthquakes, fractional derivatives in a fluid-dynamic traffic model can be leveraged to alleviate the deficiency caused by the assumption of a continuous flow of traffic. Researchers including Coimbra, Davison and Essex, Riesz, Riemann and Liouville, Hadamard, Weyl, Jumarie, Caputo and Fabrizio, Atangana and Baleanu, Grünwald and Letnikov, Liouville and Caputo have proposed a variety of fractional operator formulations and conceptions. On the other hand, the Liouville-Caputo is the finest fractional filter. Furthermore, fractional PDEs are used to model a variety of physical phenomena, including chemical reaction and population dynamics, virology, image processing, bifurcation, thermodynamics, Levy statistics, porous media, physics, and engineering problems, (see [1-7]).

The Shehu transform (ST) was recently highlighted by Maitama and Zhao [] as an interesting integral transformation. A modification of the Laplace and Sumudu transformation is the ST. However, we can retrieve the Laplace transform by replacing $\varpi = 1$ in ST. This approach can be used to compress complex non-linear PDEs into simpler equations.

The comprehensive evaluation of numerous advanced asymptotic approaches for the exploration of solitary solutions of nonlinear PDEs, and DEs has been presented, see [9-16]. For instance, the Adomian decomposition method (ADM) [17] for obtaining seven order Sawada-Kotera equations, pseudo-spectral method (PSM) [18] for finding the numerical solution of the Laxs 7th-order KdV equation, q-homotopy analysis method (q-HAM) for finding the convergence of special PDES [19], Lie symmetry analysis (LSA) [20] for dealing with the conservation laws and exact solutions of the seventh-order time fractional Sawada-Kotera-Ito equation, Laguerre wavelets collocation method (LWCM) and Haar wavelet for the numerical solution of the Benjamina-Bona-Mohany equations [21], a new Legendre Wavelets decomposition method (NLWDM) for solving PDEs [22], discrete Adomian decomposition method (DADM) [23] for constructing numerical solution of time fractional Navier-Stokes equation.

The ZKE was built in two dimensions to demonstrate nonlinear complex phenomena such as isotope waves in a massively magnetic flux uncompressed plasma [24,25].The SDM will be implemented to develop the major objectives of this research. The time-fractional ZKE is stated as:

$\begin{eqnarray} \mathcal{D}_{\zeta}^{\delta}\mathcal{U}+a_{1}(\mathcal{U}^{\eta_{1}})_{\phi}+b_{1}(\mathcal{U}^{\eta_{2}})_{\phi\phi\phi}+b_{1}(\mathcal{U}^{\eta_{3}})_{\zeta\zeta \phi} = 0, \end{eqnarray}$

(1.1)

where $\mathcal{U} = \mathcal{U}(\phi, \psi, \zeta)$ and $\mathcal{D}_{\zeta}^{\delta}$ is the Caputo fractional derivative with order $\delta, \; 0 < \delta\leq1,$ $a_{1}$ and $b_{1}$ are arbitrary constants and $\eta_{i}, \; i = 1, 2, 3$ are integers and $\eta_{i}\neq0\; (i = 1, 2, 3)$ that demonstrates the characteristics of physical phenomena such as ion acoustic waves in a plasma consisting of cold ions and hot isothermal electrons in the framework of a balanced magnetic flux ([26,27]). In [25], for example, the ZKEs were used to investigate shallowly nonlinear isotope ripples in significantly magnetism impaired plasma in three dimensions.

In spite of the incredible improvement, the Adomian decomposition method (ADM) was contemplated by Gorge Adomian in 1980. The ADM, for example, has been effectively defined in numerous analytical structures of PDEs, especially in Burger's equation [28], time-fractional Kawahara equation [29], fuzzy heat-like and wave-like equations [30] and Lane-Emden-Fowler type equations [31]. The ADM was found to be strongly associated with a plethora of integral transforms, including ARA, Shehu, Fourier, Aboodh, Laplace and others. Presently, modified Laplace ADM [32] has been utilized to effectively resolve Volterra integral equations employing the noted numerical formulation, discrete ADM [23] has been used to solve the time-fractional Navier-Stokes equation, and Laplace ADM [33] has been considered to identify the approximate results of a fractional system of epidemic structures of a vector-borne disease, and so on.

Several of the aforesaid approaches have the disadvantage of being always stratified and necessitating a significant amount of algorithmic effort. To minimize the computing complexity and intricacy, we suggested the Shehu decomposition method (SDM), which is a composition of the ST and the decomposition method for solving the time-fractional ZKE, which is the main motivation for this research. The projected technique develops a convergent series as a solution. SDM has fewer parameters than other analytical methods. It is the preferred approach because it does not require discretion or linearization.

In this study, we first provided a fractional ZKE, followed by a description of the SDM, and then, a comparison characterization of the SDM presented with the existing methods. The graphical representations were then thoroughly explained in relation to the ZK problem. We presented an algorithm for SDM, discussed its estimation accuracy, and then showed two examples that demonstrate the effectiveness and stability of a novel approach so that their obtained simulations can be analyzed. Finally, as a part of our concluding remarks, we discussed the accumulated facts of our findings.

2. Prelude

In order to perform our research, we require various terminologies and postulate outcomes from the literature.

Definition 2.1. ([]) Shehu transform (ST) for a mapping $\mathcal{U}(\zeta)$ containing exponential order defined on the set of mappings is described as follows:

$\begin{eqnarray} \mathbf{S} = \Big\{\mathcal{U}(\zeta)|\exists\mathcal{P}, p_{1}, p_{2} > 0, \big\vert\mathcal{U}(\zeta)\big\vert < \mathcal{P}\exp(|\zeta|/p_{\jmath}), \; if\; \zeta\in(-1)^{\jmath}\times[0, \infty), \jmath = 1, 2;\big(\mathcal{P}, p_{1}, p_{2} > 0\big)\Big\}, \end{eqnarray}$

(2.1)

where $\mathcal{U}(\zeta)$ is denoted by $\mathbf{S}\big[\mathcal{U}(\zeta)\big] = \mathcal{S}(\xi, \varpi),$ is stated as

$\begin{eqnarray} \mathbf{S}\big[\mathcal{U}(\zeta)\big] = \int\limits_{0}^{\infty}\mathcal{U}(\zeta)\exp\Big(-\frac{\xi}{\varpi}\zeta\Big)d\zeta = \mathcal{S}(\xi, \varpi), \; \zeta\leq0, \; \varpi\in[\kappa_{1}, \kappa_{2}]. \end{eqnarray}$

(2.2)

The following is an example of a supportive ST:

$\begin{eqnarray} \mathbf{S}\big[\zeta^{\delta}\big] = \int\limits_{0}^{\infty}\exp\Big(-\frac{\xi}{\varpi}\zeta\Big)\zeta^{\delta}d\zeta = \Gamma(\delta+1)\Big(\frac{\varpi}{\xi}\Big)^{\delta+1}. \end{eqnarray}$

(2.3)

Definition 2.2. ([]) The inverse ST of a function $\mathcal{U}(\zeta)$ is described as

$\begin{eqnarray} \mathbf{S}^{-1}\Big[\Big(\frac{\varpi}{\xi}\Big)^{m\delta+1}\Big] = \frac{\zeta^{m\delta}}{\Gamma(m\delta+1)}, \; \; \Re(\delta) > 0, \; \; and \; m > 0. \end{eqnarray}$

(2.4)

Lemma 2.3. Consider ST of $\mathcal{U}_{1}(\zeta)$ and $\mathcal{U}_{2}(\zeta)$ are $\mathcal{P}(\xi, \varpi)$ and $\mathcal{Q}(\xi, \varpi),$ respectively [8],

$\begin{eqnarray} \mathbf{S}\big[\gamma_{1}\mathcal{U}_{1}(\zeta)+\gamma_{2}\mathcal{U}_{2}(\zeta)\big]&& = \mathbf{S}\big[\gamma_{1}\mathcal{U}_{1}(\zeta)\big]+\mathbf{S}\big[\gamma_{2}\mathcal{U}_{2}(\zeta)\big]\\&& = \gamma_{1}\mathcal{P}(\xi, \varpi)+\gamma_{2}\mathcal{Q}(\xi, \varpi), \end{eqnarray}$

(2.5)

where $\gamma_{1}$ and $\gamma_{2}$ are unspecified terms.

Lemma 2.4. ([])For order $\delta > 0,$ the Caputo fractional derivative (CFD) of ST is defined as

$\begin{eqnarray} \mathbf{S}\big[\mathcal{D}_{\zeta}^{\delta}\mathcal{U}(\zeta)\big] = \Big(\frac{\xi}{\varpi}\Big)^{\delta}\mathbf{S}\big[\mathcal{U}(\phi, \zeta)\big]-\sum\limits_{\kappa = 0}^{m-1}\Big(\frac{\xi}{\varpi}\Big)^{\delta-\kappa-1}\mathcal{U}^{(\kappa)}(\phi, 0), \; m-1\leq\delta\leq m, \; m\in\mathbb{N}. \end{eqnarray}$

(2.6)

3. Description of the SDM

Considering the nonlinear partial differential equation:

$\begin{eqnarray} \mathcal{D}_{\zeta}^{\delta}\mathcal{U}(\phi, \zeta)+\mathcal{L}\mathcal{U}(\phi, \zeta)+\check{\mathcal{N}}\mathcal{U}(\phi, \zeta) = \mathcal{F}(\phi, \zeta), \; \zeta > 0, \; 0 < \delta\leq1, \end{eqnarray}$

(3.1)

subject to the condition

$\begin{eqnarray} \mathcal{U}(\phi, 0) = \mathcal{G}(\phi), \end{eqnarray}$

(3.2)

where $\mathcal{D}_{\zeta}^{\delta} = \frac{\partial^{\delta}\mathcal{U}(\phi, \zeta)}{\partial \zeta^{\delta}}$ indicates the CFD with $0 < \delta\leq1$ while $\mathcal{L}$ and $\check{\mathcal{N}}$ are linear/nonlinear factors and the source term refers to $\mathcal{F}(\phi, \zeta).$

Implementing the ST to (3.1), and we attain

$\begin{eqnarray*} \mathbf{S}\big[\mathcal{D}_{\zeta}^{\delta}\mathcal{U}(\phi, \zeta)+\mathcal{L}\mathcal{U}(\phi, \zeta)+\check{\mathcal{N}}\mathcal{U}(\phi, \zeta)\big] = \mathbf{S}\big[\mathcal{F}(\phi, \zeta)\big]. \end{eqnarray*}$

Applying the differentiation property of ST, yields

$\begin{eqnarray} &&\frac{\xi^{\delta}}{\varpi^{\delta}}\mathcal{U}(\xi, \varpi) = \sum\limits_{\kappa = 0}^{m-1}\Big(\frac{\xi}{\varpi}\Big)^{\delta-\kappa-1}\mathcal{U}^{(\kappa)}(0)+\mathbf{S}\big[\mathcal{L}\mathcal{U}(\phi, \zeta)+\check{\mathcal{N}}\mathcal{U}(\phi, \zeta)\big]+\mathbf{S}\big[\mathcal{F}(\phi, \zeta)\big]. \end{eqnarray}$

(3.3)

Th inverse ST of (3.3) provides

$\begin{eqnarray} \mathcal{U}(\phi, \zeta) = \mathbf{S}^{-1}\bigg[\sum\limits_{\kappa = 0}^{m-1}\Big(\frac{\xi}{\varpi}\Big)^{\delta-\kappa-1}\mathcal{U}^{(\kappa)}(0)+\frac{\varpi^{\delta}}{\xi^{\delta}}\mathbf{S}\big[\mathcal{F}(\phi, \zeta)\big]\bigg]-\mathbf{S}^{-1}\bigg[\frac{\varpi^{\delta}}{\xi^{\delta}}\mathbf{S}\big[\mathcal{L}\mathcal{U}(\phi, \zeta)+\check{\mathcal{N}}\mathcal{U}(\phi, \zeta)\big]\bigg]. \end{eqnarray}$

(3.4)

The infinite series representation of SDM is denoted by the mapping $\mathcal{U}(\phi, \zeta)$ as follows:

$\begin{eqnarray} \mathcal{U}(\phi, \zeta) = \sum\limits_{m = 0}^{\infty}\mathcal{U}_{m}(\phi, \zeta). \end{eqnarray}$

(3.5)

Thus, the nonlinearity ${\check{\mathcal{N}}}(\phi, \zeta)$ can be estimated by the ADM represented as

$\begin{eqnarray} {\check{\mathcal{N}}}\mathcal{U}(\phi, \zeta) = \sum\limits_{m = 0}^{\infty}\tilde{A}_{m}(\mathcal{U}_{0}, \mathcal{U}_{1}, ...), \; m = 0, 1, ...\; , \end{eqnarray}$

(3.6)

where

$\tilde{A}_{m}(\mathcal{U}_{0}, \mathcal{U}_{1}, ...) = \frac{1}{m!}\bigg[\frac{d^{m}}{d\lambda^{m}}{\check{\mathcal{N}}}\bigg(\sum\limits_{\jmath = 0}^{\infty}\lambda^{\jmath}\mathcal{U}_{\jmath}\bigg)\bigg]_{\lambda = 0}, \; \; m > 0.$

Substituting (3.5) and (3.6) into (3.4), we have

$\begin{eqnarray} \sum\limits_{m = 0}^{\infty}\mathcal{U}_{m}(\phi, \zeta)&& = \mathcal{G}(\phi)+\tilde{\mathcal{G}}(\phi)-\mathbf{S}^{-1}\bigg[\frac{\varpi^{\delta}}{\xi^{\delta}}\mathbf{S}\big[\mathcal{L}\mathcal{U}(\phi, \zeta)+\sum\limits_{m = 0}^{\infty}\tilde{A}_{m}\big]\bigg]. \end{eqnarray}$

(3.7)

Consequently, the following is the recursive methodology for (3.7):

$\begin{eqnarray} \mathcal{U}_{0}(\phi, \zeta)&& = \mathcal{G}(\phi)+\tilde{\mathcal{G}}(\phi), \; \; m = 0, \\\mathcal{U}_{m+1}(\phi, \zeta)&& = -\mathbf{S}^{-1}\bigg[\frac{\varpi^{\delta}}{\xi^{\delta}}\mathbf{S}\big[\mathcal{L}\big(\mathcal{U}_{m}(\phi, \zeta)\big)+\sum\limits_{m = 0}^{\infty}\tilde{A}_{m}\big]\bigg], \; \; m\geq1. \end{eqnarray}$

(3.8)

4. Illustrative examples

Example 4.1. Assume the following time-dependent fractional-order Zakharov-Kuznetsov equation:

$\begin{eqnarray} \mathcal{D}_{\zeta}^{\delta}\mathcal{U}+\frac{\partial\mathcal{U}^{2}}{\partial \phi}+\frac{1}{8}\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{2}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{2}}{\partial \phi^{3}}\Big] = 0, \end{eqnarray}$

(4.1)

subject to the initial condition

$\begin{eqnarray} \mathcal{U}(\phi, \psi, 0) = \frac{4}{3}\theta\sinh^{2}(\phi+\psi), \end{eqnarray}$

(4.2)

where $\theta$ is an arbitrary constant.

Proof. Applying the ST on both sides of (4.1), we find

$\begin{eqnarray} &&\mathbf{S}\Big[\frac{\partial^{\delta}\mathcal{U}}{\partial\zeta^{\delta}}\Big] = -\mathbf{S}\bigg[\frac{\partial\mathcal{U}^{2}}{\partial \phi}+\frac{1}{8}\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{2}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{2}}{\partial \phi^{3}}\Big]\bigg], \\&& \Big(\frac{\xi}{\varpi}\Big)^{\delta}\mathbf{S}\big[\mathcal{U}(\phi, \psi, \zeta)\big]-\sum\limits_{\kappa = 0}^{n_{1}-1} \Big(\frac{\xi}{\varpi}\Big)^{\delta-\kappa-1}\frac{\partial^{\kappa}\mathcal{U}^{(\kappa)}(\phi, 0)}{\partial\zeta^{\kappa}} = -\mathbf{S}\bigg[\frac{\partial\mathcal{U}^{2}}{\partial \phi}+\frac{1}{8}\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{2}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{2}}{\partial \phi^{3}}\Big]\bigg]. \end{eqnarray}$

(4.3)

Employing the inverse ST, we have

$\begin{eqnarray} \mathcal{U}(\phi, \psi, \zeta) = \mathbf{S}^{-1}\Bigg[ \Big(\frac{\varpi}{\xi}\Big)^{\delta}\sum\limits_{\kappa = 0}^{n_{1}-1} \Big(\frac{\xi}{\varpi}\Big)^{\delta-\kappa-1}\frac{\partial^{\kappa}\mathcal{U}^{(\kappa)}(\phi, 0)}{\partial\zeta^{\kappa}}-\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[\frac{\partial\mathcal{U}^{2}}{\partial \phi}+\frac{1}{8}\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{2}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{2}}{\partial \phi^{3}}\Big]\bigg]\Bigg]. \end{eqnarray}$

(4.4)

It follows that

$\begin{eqnarray} &&\mathcal{U}(\phi, \psi, \zeta) = \mathbf{S}^{-1}\bigg[\frac{\varpi}{\xi}{\mathcal{U}(\phi, \psi, 0)}\bigg]-\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[\frac{\partial\mathcal{U}^{2}}{\partial \phi}+\frac{1}{8}\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{2}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{2}}{\partial \phi^{3}}\Big]\bigg]\Bigg], \\&&\mathcal{U}(\phi, \psi, \zeta) = \mathbf{S}^{-1}\bigg[\frac{4}{3}\frac{\varpi}{\xi}{\theta\sinh^{2}(\phi+\psi)}\bigg]-\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[\frac{\partial\mathcal{U}^{2}}{\partial \phi}+\frac{1}{8}\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{2}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{2}}{\partial \phi^{3}}\Big]\bigg]\Bigg]. \end{eqnarray}$

(4.5)

Utilizing the Shehu's decomposition method, we get

$\begin{eqnarray} \sum\limits_{\jmath = 0}^{\infty}\mathcal{U}_{\jmath}(\phi, \psi, \zeta) = \frac{4}{3}\theta\sinh^{2}(\phi+\psi)-\mathbf{S}^{-1}\Bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[\check{\mathcal{N}}(\mathcal{U})_{\phi}+\frac{1}{8}\Big[\check{\mathcal{N}}(\mathcal{U})_{\phi\phi\phi}+\check{\mathcal{N}}(\mathcal{U})_{\phi\psi\psi}\Big]\bigg]\Bigg], \end{eqnarray}$

(4.6)

where $\check{\mathcal{N}}(\mathcal{U})$ is the Admoian's polynomial describing nonlinear term appearing in the above mentioned equations.

$\begin{eqnarray} \check{\mathcal{N}}(\mathcal{U}) = \mathcal{U}^{2} = \sum\limits_{\jmath = 0}^{\infty}\mathcal{H}_{\jmath}(\mathcal{U}). \end{eqnarray}$

(4.7)

First few Admoian's polynomials are presented as follows:

$\begin{eqnarray*} \mathcal{H}_{0}&& = \mathcal{U}_{0}^{2}, \nonumber\\ \mathcal{H}_{1}&& = 2\mathcal{U}_{0}\mathcal{U}_{1}, \nonumber\\ \mathcal{H}_{2}&& = 2\mathcal{U}_{0}\mathcal{U}_{2}+\mathcal{U}_{1}^{2}, \nonumber\\ \mathcal{U}_{0}(\phi, \psi, \zeta)&& = \frac{4}{3}\theta\sinh^{2}(\phi+\psi), \nonumber\\ \mathcal{U}_{\jmath+1}(\phi, \psi, \zeta)&& = -\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[\bigg(\sum\limits_{\jmath = 0}^{\infty}\mathcal{H}_{\jmath}(\mathcal{U})\bigg)_{\phi}+\frac{1}{8}\bigg(\sum\limits_{\jmath = 0}^{\infty}\mathcal{H}_{\jmath}(\mathcal{U})\bigg)_{\phi\phi\phi}+\frac{1}{8}\bigg(\sum\limits_{\jmath = 0}^{\infty}\mathcal{H}_{\jmath}(\mathcal{U})\bigg)_{\phi\psi\psi}\bigg], \end{eqnarray*}$

for $\jmath = 0, 1, 2, ...$

$\begin{eqnarray*} \mathcal{U}_{1}(\phi, \psi, \zeta)&& = -\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[(\mathcal{U}_{0}^{2})_{\phi}+\frac{1}{8}(\mathcal{U}_{0}^{2})_{\phi\phi\phi}+\frac{1}{8}(\mathcal{U}_{0}^{2})_{\phi\psi\psi}\bigg]\Bigg]\nonumber\\&& = \bigg(-\frac{224}{9}\theta^{2}\sinh^{2}(\phi+\psi)\cosh(\phi+\psi)-\frac{32}{3}\theta^{2}\sinh(\phi+\psi)\cosh^{3}(\phi+\psi)\bigg)\mathbf{S}^{-1}\Big(\Big(\frac{\varpi}{\xi}\Big)^{\delta+1}\Big)\nonumber\\&& = \bigg(-\frac{224}{9}\theta^{2}\sinh^{2}(\phi+\psi)\cosh(\phi+\psi)-\frac{32}{3}\theta^{2}\sinh(\phi+\psi)\cosh^{3}(\phi+\psi)\bigg)\frac{\zeta^{\delta}}{\Gamma(\delta+1)}. \end{eqnarray*}$

Accordingly, we can derive the remaining terms as follows

$\begin{eqnarray} \mathcal{U}_{2}(\phi, \psi, \zeta)&& = -\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[(2\mathcal{U}_{0}\mathcal{U}_{1})_{\phi}+\frac{1}{8}(2\mathcal{U}_{0}\mathcal{U}_{1})_{\phi\phi\phi}+\frac{1}{8}(2\mathcal{U}_{0}\mathcal{U}_{1})_{\phi\psi\psi}\bigg]\Bigg]\\&& = \frac{128}{27}\theta^{3}\Big(1200\cosh^{6}(\phi+\psi)-2080\cosh^{4}(\phi+\psi)\\&&\quad+968\cosh^{2}(\phi+\psi)-79\Big)\frac{\zeta^{2\delta}}{\Gamma(2\delta+1)}, \end{eqnarray}$

(4.8)

$\begin{eqnarray} \mathcal{U}_{3}(\phi, \psi, \zeta)&& = -\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[(2\mathcal{U}_{0}\mathcal{U}_{2}+\mathcal{U}_{1}^{2})_{\phi}+\frac{1}{8}(2\mathcal{U}_{0}\mathcal{U}_{2}+\mathcal{U}_{1}^{2})_{\phi\phi\phi}+\frac{1}{8}(2\mathcal{U}_{0}\mathcal{U}_{2}+\mathcal{U}_{1}^{2})_{\phi\psi\psi}\bigg]\Bigg]\\&& = -\frac{2048}{81}\theta^{4}\sinh(\phi+\psi)\cosh(\phi+\psi)\Big(88, 4000\cosh^{6}(\phi+\psi)-160,200\cosh^{4}(\phi+\psi)\\&&\quad+85,170\cosh^{2}(\phi+\psi)-11,903\Big)\frac{\zeta^{3\delta}}{\Gamma(3\delta+1)}. \end{eqnarray}$

(4.9)

The approximate-analytical SDM solution is

$\begin{eqnarray} \mathcal{U}(\phi, \psi, \zeta)&& = \mathcal{U}_{0}(\phi, \psi, \zeta)+\mathcal{U}_{1}(\phi, \psi, \zeta)+\mathcal{U}_{2}(\phi, \psi, \zeta)+\mathcal{U}_{3}(\phi, \psi, \zeta)+..., \\\mathcal{U}(\phi, \psi, \zeta)&& = \frac{4}{3}\theta\sinh^{2}(\phi+\psi)-\bigg(\frac{224}{9}\theta^{2}\sinh^{2}(\phi+\psi)\cosh(\phi+\psi)\\&&\quad+\frac{32}{3}\theta^{2}\sinh(\phi+\psi)\cosh^{3}(\phi+\psi)\bigg)\frac{\zeta^{\delta}}{\Gamma(\delta+1)}+\frac{128}{27}\theta^{3}\Big(1200\cosh^{6}(\phi+\psi)\\&&\quad-2080\cosh^{4}(\phi+\psi)+968\cosh^{2}(\phi+\psi)-79\Big)\frac{\zeta^{2\delta}}{\Gamma(2\delta+1)}\\&&\quad-\frac{2048}{81}\theta^{4}\sinh(\phi+\psi)\cosh(\phi+\psi)\Big(88, 4000\cosh^{6}(\phi+\psi)-160,200\cosh^{4}(\phi+\psi)\\&&\quad+85,170\cosh^{2}(\phi+\psi)-11,903\Big)\frac{\zeta^{3\delta}}{\Gamma(3\delta+1)}+...\; . \end{eqnarray}$

(4.10)

The exact solution for $\delta = 1$ is presented by

$\begin{eqnarray} \mathcal{U}(\phi, \psi, \zeta) = \frac{4}{3}\theta\sinh^{2}(\phi+\psi-\theta\zeta). \end{eqnarray}$

(4.11)

and show the comparison results for exact, SDM, and absolute error of $\mathcal{U}_{abs} = \|\mathcal{U}^{E}-\mathcal{U}^{SDM}\|$ solution for (4.1), when $\theta = 0001$ and for various fractional orders $\delta = 0.67, \; 0.75, \; 1.$ It can be seen that the proposed method closely corresponds the exact, VIM [34], VIA [35] and RPSM [35].

Table 1. Exact

$(\mathcal{U}_{E})$ and SDM-approximate

$(\mathcal{U}_{SDM})$ solution with absolute error

$(\mathcal{U}_{abs})$ in comparison derived by VIM

$(\mathcal{U}_{VIM})$ [34], PIA

$(\mathcal{U}_{PIA})$ [35] and RPSM

$(\mathcal{U}_{RPSM})$ [35] for Example 4.1 at

$\theta = 0.001,$

$\delta = 1, \; 0.67$ and 0.75.

$\phi$	$\psi$	$\zeta$	$\mathcal{U}_{E}$	$\mathcal{U}_{SDM}$	$\mathcal{U}_{abs}$	$\mathcal{U}_{\delta=0.67}$	$\mathcal{U}_{\delta=0.75}$	$\mathcal{U}_{VIM}$ [34]
0.1	0.1	0.2	5.394 $\times10^{-5}$	5.331 $\times10^{-5}$	6.313 $\times10^{-7}$	5.341 $\times10^{-5}$	5.328 $\times10^{-5}$	5.356 $\times10^{-5}$
		0.3	7.668 $\times10^{-4}$	7.562 $\times10^{-4}$	1.055 $\times10^{-5}$	7.488 $\times10^{-4}$	7.507 $\times10^{-4}$	7.570 $\times10^{-4}$
		0.4	5.383 $\times10^{-5}$	5.308 $\times10^{-5}$	7.541 $\times10^{-7}$	5.419 $\times10^{-5}$	5.375 $\times10^{-5}$	5.410 $\times10^{-5}$
0.6	0.6	0.2	7.668 $\times10^{-4}$	7.562 $\times10^{-4}$	1.055 $\times10^{-5}$	7.488 $\times10^{-4}$	7.507 $\times10^{-4}$	7.570 $\times10^{-4}$
		0.3	7.665 $\times10^{-4}$	7.513 $\times10^{-4}$	1.522 $\times10^{-5}$	7.447 $\times10^{-4}$	7.461 $\times10^{-4}$	7.531 $\times10^{-4}$
		0.4	7.663 $\times10^{-4}$	7.468 $\times10^{-4}$	1.948 $\times10^{-5}$	7.417 $\times10^{-4}$	7.425 $\times10^{-4}$	7.501 $\times10^{-4}$
0.9	0.9	0.2	1.840 $\times10^{-3}$	1.801 $\times10^{-3}$	3.993 $\times10^{-5}$	1.772 $\times10^{-3}$	1.779 $\times10^{-3}$	1.803 $\times10^{-3}$
		0.3	1.740 $\times10^{-3}$	1.882 $\times10^{-3}$	5.803 $\times10^{-5}$	1.755 $\times10^{-3}$	1.761 $\times10^{-3}$	1.788 $\times10^{-3}$
		0.4	1.840 $\times10^{-3}$	1.765 $\times10^{-3}$	7.487 $\times10^{-5}$	1.743 $\times10^{-3}$	1.747 $\times10^{-3}$	1.775 $\times10^{-3}$

| Show Table

DownLoad: CSV

Table 2. Other comparison of the projected scheme with PIA and RPSM for Example 4.1 at

$\theta = 0.001$ having different fractional-order

$\delta = 0.67$ and

$\delta = 0.75$ .

$\phi$	$\psi$	$\zeta$	$\mathcal{U}_{PIA}$ [35]	$\mathcal{U}_{RPSM}$ [35]	$\mathcal{U}_{PIA}$ [35]	$\mathcal{U}_{RPSM}$ [35]
0.1	0.1	0.2	5.3307 $\times10^{-5}$	6.3129 $\times10^{-7}$	5.3285 $\times10^{-5}$	5.3562 $\times10^{-5}$
		0.3	5.28631 $\times10^{-5}$	5.28410 $\times10^{-5}$	5.29757 $\times10^{-5}$	5.29675 $\times10^{-5}$
		0.4	5.25777 $\times10^{-5}$	5.25897 $\times10^{-5}$	5.27039 $\times10^{-5}$	5.27119 $\times10^{-5}$
0.6	0.6	0.2	2.95493 $\times10^{-3}$	2.95185 $\times10^{-3}$	2.96356 $\times10^{-3}$	2.96251 $\times10^{-3}$
		0.3	2.92662 $\times10^{-3}$	2.92709 $\times10^{-3}$	2.93717 $\times10^{-3}$	2.93780 $\times10^{-3}$
		0.4	2.90307 $\times10^{-3}$	2.90522 $\times10^{-3}$	2.91448 $\times10^{-3}$	2.91561 $\times10^{-3}$
0.9	0.9	0.2	1.06822 $\times10^{-2}$	1.05522 $\times10^{-2}$	1.07716 $\times10^{-2}$	2.91561 $\times10^{-2}$
		0.3	1.04487 $\times10^{-2}$	1.01199 $\times10^{-2}$	1.05488 $\times10^{-2}$	1.03695 $\times10^{-2}$
		0.4	9.02777 $\times10^{-2}$	9.60606 $\times10^{-2}$	1.03736 $\times10^{-2}$	9.96743 $\times10^{-2}$

| Show Table

DownLoad: CSV

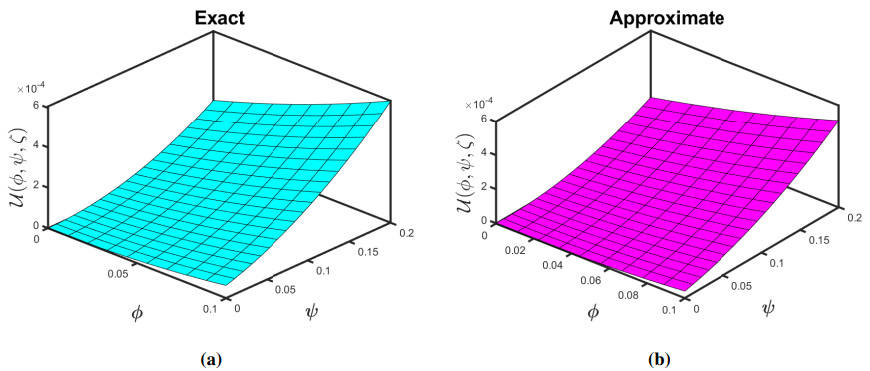
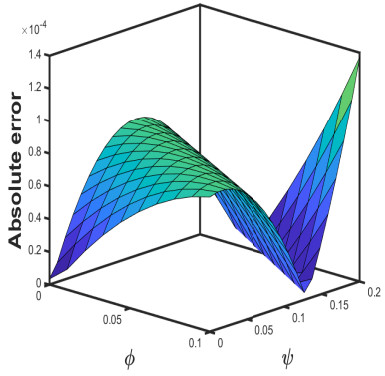
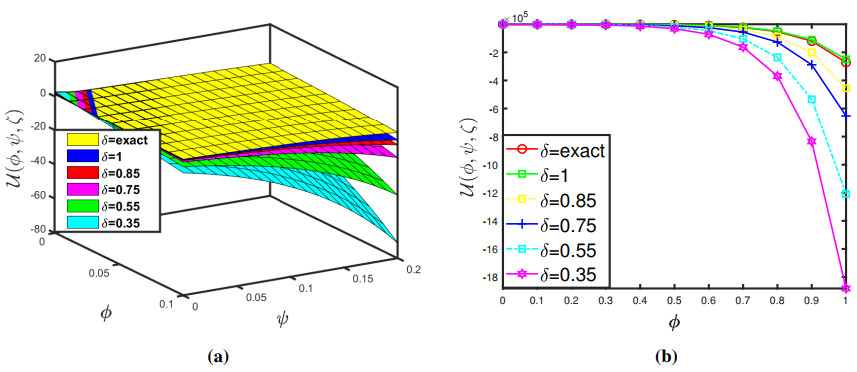
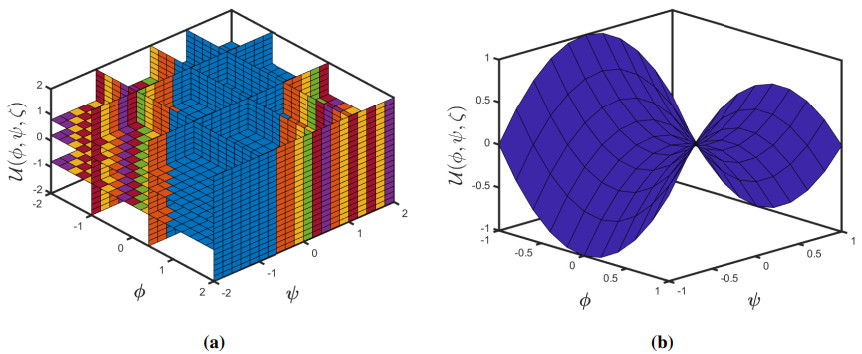
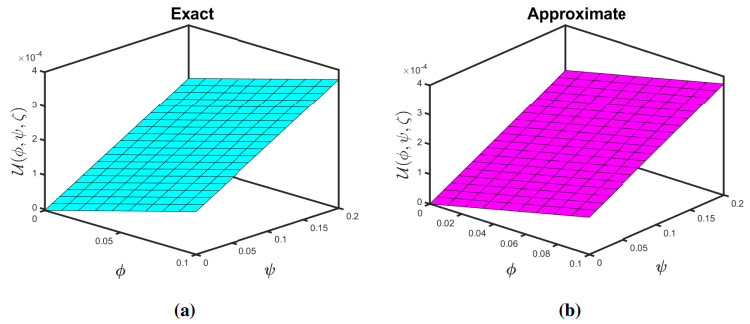
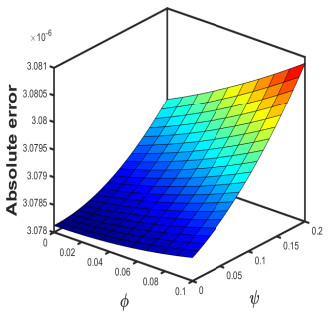
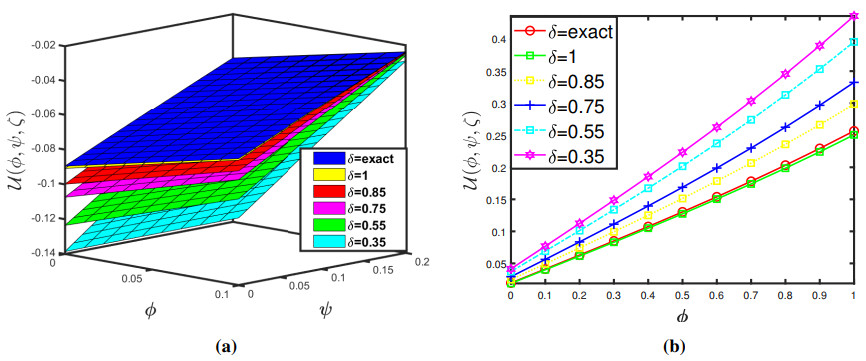
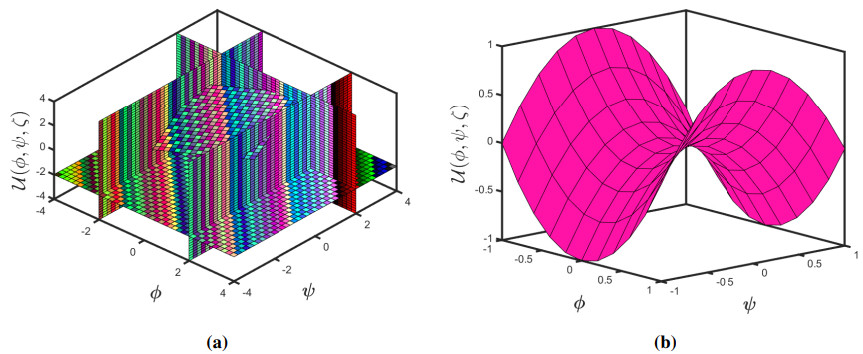
Taking $\theta = 0.005$ and $\delta = 1$ , we exhibit the approximate-analytical solution of the fractional KZEs equation up to 4 components in (a and b). Furthermore, we establish absolute errors at $\delta = 1$ for the exact-approximate solutions in the accompanying . Also, we have seen how different fractional orders perform in surface plots and 2D plots in and some $\delta_{1}\delta_{2}-slice$ (a and b) solutions are presented in 4 when $\theta = 0.005$ and $\zeta = 0.5$ . As a result of this behaviour, we might conclude that the approximation solution tends to be a precise solution. Accordingly, as the iteration increases, the absolute inaccuracy decreases. Consequently, as the number of terms grows, the SDM findings approach the exact result.

Figure 1. Numerical behavior of exact and approximate solution to the

$\mathcal{U}(\phi, \psi, \zeta)$ for Example 4.1 when the parameters are

$\theta = 0.0005, \delta = 1,$ and

$\zeta = 0.5$ .

DownLoad: Full-Size Img PowerPoint

Figure 2. Surface representation of

$\mathcal{U}(\phi, \psi, \zeta)$ absolute error plot for Example 4.1 at

$\theta = 0.005$ and

$\delta = 1$ .

DownLoad: Full-Size Img PowerPoint

Figure 3. Numerical behavior of different fractional orders to the function

$\mathcal{U}(\phi, \psi, \zeta)$ for Example 4.1 when the parameters are

$\theta = 0.05,$ and

$\zeta = 0.9$ .

DownLoad: Full-Size Img PowerPoint

The graphs in Figures 1–4 assist us to comprehend the behaviour of fractional orders when space and time scale variables fluctuate. Additionally, the findings of this study will aid scientists connected to pattern formation theory, optical designs, or mathematical modelling in comprehending the structural phenomena of the ANOVA-test. Furthermore, the efficiency of the projected method can be boosted by getting additional approximate solution expressions.

Figure 4. Numerical behavior of

$\delta_{1}\delta_{2}$ -slice solution to the

$\mathcal{U}(\phi, \psi, \zeta)$ for Example 4.1 (a) exact and (b) approximate when the parameters are

$\theta = 0.0005, \delta = 1,$ and

$\zeta = 0.5$ .

DownLoad: Full-Size Img PowerPoint

Example 4.2. Assume the following time-dependent fractional-order Zakharov-Kuznetsov equation:

$\begin{eqnarray} \mathcal{D}_{\zeta}^{\delta}\mathcal{U}+\frac{\partial\mathcal{U}^{3}}{\partial \phi}+2\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{3}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{3}}{\partial \phi^{3}}\Big] = 0, \end{eqnarray}$

(4.12)

subject to the initial condition

$\begin{eqnarray} \mathcal{U}(\phi, \psi, 0) = \frac{3}{2}\theta\sinh\Big[\frac{1}{6}(\phi+\psi)\Big], \end{eqnarray}$

(4.13)

where $\theta$ is an arbitrary constant.

Proof. Applying the ST on both sides of (4.12), we find

$\begin{eqnarray} &&\mathbf{S}\Big[\frac{\partial^{\delta}\mathcal{U}}{\partial\zeta^{\delta}}\Big] = -\mathbf{S}\bigg[\frac{\partial\mathcal{U}^{3}}{\partial \phi}+2\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{3}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{3}}{\partial \phi^{3}}\Big]\bigg], \\&& \Big(\frac{\xi}{\varpi}\Big)^{\delta}\mathbf{S}\big[\mathcal{U}(\phi, \psi, \zeta)\big]-\sum\limits_{\kappa = 0}^{n_{1}-1} \Big(\frac{\xi}{\varpi}\Big)^{\delta-\kappa-1}\frac{\partial^{\kappa}\mathcal{U}^{(\kappa)}(\phi, 0)}{\partial\zeta^{\kappa}} = -\mathbf{S}\bigg[\frac{\partial\mathcal{U}^{3}}{\partial \phi}+2\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{3}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{3}}{\partial \phi^{3}}\Big]\bigg]. \end{eqnarray}$

(4.14)

Employing the inverse ST, we have

$\begin{eqnarray} \mathcal{U}(\phi, \psi, \zeta) = \mathbf{S}^{-1}\Bigg[ \Big(\frac{\varpi}{\xi}\Big)^{\delta}\sum\limits_{\kappa = 0}^{n_{1}-1} \Big(\frac{\xi}{\varpi}\Big)^{\delta-\kappa-1}\frac{\partial^{\kappa}\mathcal{U}^{(\kappa)}(\phi, 0)}{\partial\zeta^{\kappa}}-\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[\frac{\partial\mathcal{U}^{3}}{\partial \phi}+2\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{3}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{3}}{\partial \phi^{3}}\Big]\bigg]\Bigg]. \end{eqnarray}$

(4.15)

It follows that

$\begin{eqnarray} &&\mathcal{U}(\phi, \psi, \zeta) = \mathbf{S}^{-1}\bigg[\frac{\varpi}{\xi}{\mathcal{U}(\phi, \psi, 0)}\bigg]-\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[\frac{\partial\mathcal{U}^{3}}{\partial \phi}+2\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{3}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{3}}{\partial \phi^{3}}\Big]\bigg]\Bigg], \\&&\mathcal{U}(\phi, \psi, \zeta) = \mathbf{S}^{-1}\bigg[\frac{3}{2}\frac{\varpi}{\xi}{\theta\sinh\big[\frac{1}{6}(\phi+\psi)\big]}\bigg]-\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[\frac{\partial\mathcal{U}^{3}}{\partial \phi}+2\Big[\frac{\partial}{\partial \phi}\Big(\frac{\partial^{2}\mathcal{U}^{3}}{\partial \psi^{2}}\Big)+\frac{\partial^{3}\mathcal{U}^{3}}{\partial \phi^{3}}\Big]\bigg]\Bigg]. \end{eqnarray}$

(4.16)

Utilizing the Shehu's decomposition method, we get

$\begin{eqnarray} \sum\limits_{\jmath = 0}^{\infty}\mathcal{U}_{\jmath}(\phi, \psi, \zeta) = \frac{3}{2}\theta\sinh\big[\frac{1}{6}(\phi+\psi)\big]-\mathbf{S}^{-1}\Bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[\check{\mathcal{N}}(\mathcal{U})_{\phi}+\frac{1}{8}\Big[\check{\mathcal{N}}(\mathcal{U})_{\phi\phi\phi}+\check{\mathcal{N}}(\mathcal{U})_{\phi\psi\psi}\Big]\bigg]\Bigg], \end{eqnarray}$

(4.17)

where $\check{\mathcal{N}}(\mathcal{U})$ is the Admoian's polynomial describing nonlinear term appearing in the above mentioned equations.

$\begin{eqnarray} \check{\mathcal{N}}(\mathcal{U}) = \mathcal{U}^{3} = \sum\limits_{\jmath = 0}^{\infty}\mathcal{G}_{\jmath}(\mathcal{U}). \end{eqnarray}$

(4.18)

First few Admoian's polynomials are presented as follows:

$\begin{eqnarray*} \mathcal{G}_{0}&& = \mathcal{U}_{0}^{3}, \nonumber\\ \mathcal{G}_{1}&& = 3\mathcal{U}_{0}^{2}\mathcal{U}_{1}, \nonumber\\ \mathcal{G}_{2}&& = 3\mathcal{U}_{0}^{2}\mathcal{U}_{2}+3\mathcal{U}_{0}^{2}\mathcal{U}_{1}^{2}, \nonumber\\ \mathcal{U}_{0}(\phi, \psi, \zeta)&& = \frac{3}{2}\theta\sinh\Big[\frac{1}{6}(\phi+\psi)\Big], \nonumber\\ \mathcal{U}_{\jmath+1}(\phi, \psi, \zeta)&& = -\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[\bigg(\sum\limits_{\jmath = 0}^{\infty}\mathcal{G}_{\jmath}(\mathcal{U})\bigg)_{\phi}+2\bigg(\sum\limits_{\jmath = 0}^{\infty}\mathcal{G}_{\jmath}(\mathcal{U})\bigg)_{\phi\phi\phi}+2\bigg(\sum\limits_{\jmath = 0}^{\infty}\mathcal{G}_{\jmath}(\mathcal{U})\bigg)_{\phi\psi\psi}\bigg], \end{eqnarray*}$

for $\jmath = 0, 1, 2, ...$

$\begin{eqnarray*} \mathcal{U}_{1}(\phi, \psi, \zeta)&& = -\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[(\mathcal{U}_{0}^{3})_{\phi}+2(\mathcal{U}_{0}^{3})_{\phi\phi\phi}+2(\mathcal{U}_{0}^{3})_{\phi\psi\psi}\bigg]\Bigg]\nonumber\\&& = \bigg(-3\theta^{3}\sinh^{2}\Big[\frac{1}{6}(\phi+\psi)\Big]\cosh\Big[\frac{1}{6}(\phi+\psi)\Big]+\frac{3}{8}\theta^{3}\cosh^{3}\Big[\frac{1}{6}(\phi+\psi)\Big]\bigg)\mathbf{S}^{-1}\Big(\Big(\frac{\varpi}{\xi}\Big)^{\delta+1}\Big)\nonumber\\&& = \bigg(-3\theta^{3}\sinh^{2}\Big[\frac{1}{6}(\phi+\psi)\Big]\cosh\Big[\frac{1}{6}(\phi+\psi)\Big]+\frac{3}{8}\theta^{3}\cosh^{3}\Big[\frac{1}{6}(\phi+\psi)\Big]\bigg)\frac{\zeta^{\delta}}{\Gamma(\delta+1)}. \end{eqnarray*}$

Accordingly, we can derive the remaining terms as follows

$\begin{eqnarray*} \mathcal{U}_{2}(\phi, \psi, \zeta)&& = -\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[(3\mathcal{U}_{0}^{2}\mathcal{U}_{1})_{\phi}+2(3\mathcal{U}_{0}^{2}\mathcal{U}_{1})_{\phi\phi\phi}+2(3\mathcal{U}_{0}^{2}\mathcal{U}_{1})_{\phi\psi\psi}\bigg]\Bigg]\nonumber\\&& = \frac{3}{32}\theta^{5}\sinh\Big[\frac{1}{6}(\phi+\psi)\Big]\bigg[765\cosh^{4}\Big[\frac{1}{6}(\phi+\psi)\Big]\nonumber\\&&\quad-729\cosh^{2}\Big[\frac{1}{6}(\phi+\psi)\Big]+91\bigg]\frac{\zeta^{2\delta}}{\Gamma(2\delta+1)}, \end{eqnarray*}$

$\begin{eqnarray*} \mathcal{U}_{3}(\phi, \psi, \zeta)&& = -\mathbf{S}^{-1}\bigg[\Big(\frac{\varpi}{\xi}\Big)^{\delta}\mathbf{S}\bigg[(3\mathcal{U}_{0}^{2}\mathcal{U}_{2}+3\mathcal{U}_{0}^{2}\mathcal{U}_{1}^{2})_{\phi}\nonumber\\&&\quad+2(3\mathcal{U}_{0}^{2}\mathcal{U}_{2}+3\mathcal{U}_{0}^{2}\mathcal{U}_{1}^{2})_{\phi\phi\phi}+2(3\mathcal{U}_{0}^{2}\mathcal{U}_{2}+3\mathcal{U}_{0}^{2}\mathcal{U}_{1}^{2})_{\phi\psi\psi}\bigg]\Bigg]\nonumber\\&& = -\frac{3}{128}\cosh\Big[\frac{1}{6}(\phi+\psi)\Big]\bigg[171,738\cosh^{6}\Big[\frac{1}{6}(\phi+\psi)\Big]-349,884\cosh^{4}\Big[\frac{1}{6}(\phi+\psi)\Big]\nonumber\\&&\quad+215,496\cosh^{2}\Big[\frac{1}{6}(\phi+\psi)\Big]-36,907\bigg]\frac{\zeta^{3\delta}}{\Gamma(3\delta+1)}. \end{eqnarray*}$

The approximate-analytical SDM solution is

$\begin{eqnarray} \mathcal{U}(\phi, \psi, \zeta)&& = \mathcal{U}_{0}(\phi, \psi, \zeta)+\mathcal{U}_{1}(\phi, \psi, \zeta)+\mathcal{U}_{2}(\phi, \psi, \zeta)+\mathcal{U}_{3}(\phi, \psi, \zeta)+..., \\\mathcal{U}(\phi, \psi, \zeta)&& = \frac{3}{2}\theta\sinh\Big[\frac{1}{6}(\phi+\psi)\Big]-\bigg(3\theta^{3}\sinh^{2}\Big[\frac{1}{6}(\phi+\psi)\Big]\cosh\Big[\frac{1}{6}(\phi+\psi)\Big]\\&&\quad+\frac{3}{8}\theta^{3}\cosh^{3}\Big[\frac{1}{6}(\phi+\psi)\Big]\bigg)\frac{\zeta^{\delta}}{\Gamma(\delta+1)}\\&&\quad+\frac{3}{32}\theta^{5}\sinh\Big[\frac{1}{6}(\phi+\psi)\Big]\bigg[765\cosh^{4}\Big[\frac{1}{6}(\phi+\psi)\Big]-729\cosh^{2}\Big[\frac{1}{6}(\phi+\psi)\Big]\\&&\quad+91\bigg]\frac{\zeta^{2\delta}}{\Gamma(2\delta+1)}-\frac{3}{128}\cosh\Big[\frac{1}{6}(\phi+\psi)\Big]\bigg[171,738\cosh^{6}\Big[\frac{1}{6}(\phi+\psi)\Big]\\&&\quad-349,884\cosh^{4}\Big[\frac{1}{6}(\phi+\psi)\Big]\\&&\quad+215,496\cosh^{2}\Big[\frac{1}{6}(\phi+\psi)\Big]-36,907\bigg]\frac{\zeta^{3\delta}}{\Gamma(3\delta+1)}+...\; . \end{eqnarray}$

(4.19)

The exact solution for $\delta = 1$ is presented by

$\begin{eqnarray} \mathcal{U}(\phi, \psi, \zeta) = \frac{3}{2}\theta\sinh\Big[\frac{1}{6}(\phi+\psi-\theta\zeta)\Big]. \end{eqnarray}$

(4.20)

show the comparison results for exact, SDM, and absolute error of $\mathcal{U}_{abs} = \|\mathcal{U}^{E}-\mathcal{U}^{SDM}\|$ solution for (4.12), when $\theta = 0001$ and for various fractional orders $\delta = 0.67, \; 0.75, \; 1.$ It can be seen that the proposed method closely matches the exact, and VIM [34].

Table 3. Exact

$(\mathcal{U}_{E})$ and SDM-approximate

$(\mathcal{U}_{SDM})$ solution with absolute error

$(\mathcal{U}_{abs})$ in comparison derived by VIM

$(\mathcal{U}_{VIM})$ [34], for Example 4.2 at

$\theta = 0.001,$

$\delta = 1, \; 0.67$ and 0.75.

$\phi$	$\psi$	$\zeta$	$\mathcal{U}_{E}$	$\mathcal{U}_{SDM}$	$\mathcal{U}_{abs}$	$\mathcal{U}_{0.67}$	$\mathcal{U}_{0.75}$	$\mathcal{U}_{VIM}$
0.1	0.1	0.2	4.996 $\times10^{-5}$	5.001 $\times10^{-5}$	4.988 $\times10^{-8}$	5.001 $\times10^{-5}$	5.001 $\times10^{-5}$	5.001 $\times10^{-5}$
		0.3	4.993 $\times10^{-5}$	5.001 $\times10^{-5}$	7.481 $\times10^{-8}$	5.001 $\times10^{-5}$	5.001 $\times10^{-5}$	5.001 $\times10^{-5}$
		0.4	4.991 $\times10^{-5}$	5.001 $\times10^{-5}$	9.975 $\times10^{-8}$	5.001 $\times10^{-5}$	5.001 $\times10^{-5}$	5.001 $\times10^{-5}$
0.6	0.6	0.2	3.020 $\times10^{-4}$	3.020 $\times10^{-4}$	5.079 $\times10^{-8}$	3.020 $\times10^{-4}$	3.020 $\times10^{-4}$	3.020 $\times10^{-4}$
		0.3	3.019 $\times10^{-4}$	3.020 $\times10^{-4}$	7.619 $\times10^{-8}$	3.020 $\times10^{-4}$	3.020 $\times10^{-4}$	3.020 $\times10^{-4}$
		0.4	3.019 $\times10^{-4}$	3.020 $\times10^{-4}$	1.016 $\times10^{-7}$	3.020 $\times10^{-4}$	3.020 $\times10^{-4}$	3.020 $\times10^{-4}$
0.9	0.9	0.2	4.567 $\times10^{-4}$	4.568 $\times10^{-4}$	5.198 $\times10^{-8}$	4.568 $\times10^{-4}$	4.568 $\times10^{-4}$	4.568 $\times10^{-4}$
		0.3	4.567 $\times10^{-4}$	4.568 $\times10^{-4}$	7.797 $\times10^{-7}$	4.568 $\times10^{-4}$	4.568 $\times10^{-4}$	4.568 $\times10^{-4}$
		0.4	4.567 $\times10^{-4}$	4.568 $\times10^{-4}$	1.040 $\times10^{-7}$	4.568 $\times10^{-4}$	4.568 $\times10^{-4}$	4.568 $\times10^{-4}$

| Show Table

DownLoad: CSV

Taking $\theta = 0.0005$ and $\delta = 1$ , we exhibit the approximate-analytical solution of the fractional KZEs equation up to 4 components in (a and b), respectively. Furthermore, we established absolute errors at different values of $\delta$ for the exact-approximate solutions in the accompanying Figure 6. Also, we have seen how different fractional orders perform in 2D and 3D plots in in (a and b) behaves. Also, denotes the $\delta_{1}\delta_{2}$ -slice solutions for the exact and approximate solutions (a and b), respectively. As a result of this behaviour, we might conclude that the approximation solution tends to actual solution. Accordingly, as iteration increases, the absolute inaccuracy decreases. Consequently, as the iterations expands, the SDM findings approaches the exact result. The graphs in Figures 5–8 assist us to comprehend the behaviour of fractional orders when space and time scale variables fluctuate. Additionally, the findings of this study will aid scientists connecting in pattern formation theory, optical designs, or mathematical modelling in comprehending the structural phenomena of the ANOVA-test. Furthermore, the efficiency of the projected method can be boosted by getting additional approximate solution expressions.

Figure 5. Numerical behavior of exact and approximate solution to the

$\mathcal{U}(\phi, \psi, \zeta)$ for Example 4.2 when the parameters are

$\theta = 0.0005, \delta = 1,$ and

$\zeta = 0.5$ .

DownLoad: Full-Size Img PowerPoint

Figure 6. Surface representation of

$\mathcal{U}(\phi, \psi, \zeta)$ absolute error plot for Example 4.2 at

$\theta = 0.005$ and

$\delta = 1$ .

DownLoad: Full-Size Img PowerPoint

Figure 7. Numerical behavior of

$\delta_{1}\delta_{2}$ -slice solution to the

$\mathcal{U}(\phi, \psi, \zeta)$ for Example 4.2 (a) exact and (b) approximate when the parameters are

$\theta = 0.0005, \delta = 1,$ and

$\zeta = 0.5$ .

DownLoad: Full-Size Img PowerPoint

Figure 8. Numerical behavior of

$\delta_{1}\delta_{2}$ -slice solution to the

$\mathcal{U}(\phi, \psi, \zeta)$ for Example 4.1 (a) exact and (b) approximate when the parameters are

$\theta = 0.0005, \delta = 1,$ and

$\zeta = 0.5$ .

DownLoad: Full-Size Img PowerPoint

5. Conclusions

In this paper, the Shehu decomposition method (SDM) is effectively implemented for solving nonlinear time-fractional ZKEs. The proposed findings illustrate that there is a strong correlation between the projected method and the closed form solutions. Moreover, the governed approach is reliable and pragmatic for solving other diverse linear and nonlinear PDEs appearing in various disciplines of physics and mathematics. However, this methodology does not necessitate the condition matrix, Lagrange multiplier, or costly integration calculations, so the findings are noise-free, which addresses the drawbacks of earlier techniques. It is worth mentioning that the proposed methods are pragmatic analytical tools for identifying approximate-analytical solutions to complicated nonlinear PDEs. Moreover, we deduce that this approach will be used to deal with other non-linear fractional order systems of equations that are extremely complex.

Acknowledgments

This research was supported by Taif University Research Supporting Project Number (TURSP-2020/96), Taif University, Taif, Saudi Arabia.

Conflict of interest

The authors declare that they have no conflict of interest.

References

[1]	D. O. TeBeest, C. Guerber, M. Ditmore, 2007, Rice blast, The Plant Health Instructor. https://doi.org/10.1094/PHI-I-2007-0313-07. Reviewed 2012.
[2]	N. L. Suriani, D. N. Suprapta, N. Nazir, N. M. S. Parwanayoni, A. A. K. Darmadi, D. A. Dewi, et al., A mixture of piper leaves extracts and rhizobacteria for sustainable plant growth promotion and bio-control of blast pathogen of organic bali rice, Sustainability, 12 (2020), 8490. https://doi.org/10.3390/su12208490 doi: 10.3390/su12208490
[3]	M. E. Jarroudi, H. Karjoun, L. Kouadio, M. E. Jarroudi, Mathematical modelling of non-local spore dispersion of wind-borne pathogens causing fungal diseases, Appl. Math. Comput., 376 (2020), 1–11. https://doi.org/10.1016/j.amc.2020.125107 doi: 10.1016/j.amc.2020.125107
[4]	B. Hau, C. J. de Vallavieille-Pope, Wind-dispersed diseases, In: The epidemiology of plant diseases, Netherlands: Springer, 2006.
[5]	S. Kirtphaiboon, U. Humphries, A. Khan, A. Yusuf, Model of rice blast disease under tropical climate conditions, Chaos Soliton. Fract., 143 (2021), 1–8. https://doi.org/10.1016/j.chaos.2020.110530 doi: 10.1016/j.chaos.2020.110530
[6]	A. S. Kapoor, R. Prasad, G. Sood, Forecasting of rice blast in Kangra district of Himachal Pradesh, Indian Phytopathol., 57 (2004), 440–445.
[7]	W. Li, J. Ji, L. Huang, Dynamics of a controlled discontinuous computer worm system, Proc. Amer. Math. Soc., 148 (2020), 4389–4403. https://doi.org/10.1090/proc/15095 doi: 10.1090/proc/15095
[8]	W. Li, J. Ji, L. Huang, Z. Guo, Global dynamics of a controlled discontinuous diffusive SIR epidemic system, Appl. Math. Lett., 121 (2021), 107420. https://doi.org/10.1016/j.aml.2021.107420 doi: 10.1016/j.aml.2021.107420
[9]	S. Tabonglek, U. W. Humphries, A. Khan, Mathematical model for rice blast disease caused by spore dispersion affected from climate factors, Symmetry, 14 (2022), 1131. https://doi.org/10.3390/sym14061131 doi: 10.3390/sym14061131
[10]	J. B. Burie, A. Calonnec, M. Langlais, Modeling of the invasion of a fungal disease over a vineyard, Model. Simu. Sci. Eng. Tec., 2 (2008), 11–21. https://doi.org/10.1007/978-0-8176-4556-4_2 doi: 10.1007/978-0-8176-4556-4_2
[11]	C. J. Willmott, S. G. Ackleson, R. E. Davis, J. J. Feddema, K. M. Klink, D. R. Legates, et al., Statistics for the evaluation and comparison of models, J. Geophys. Res. Oceans, 90 (1985), 8995–9005. https://doi.org/10.1029/JC090iC05p08995 doi: 10.1029/JC090iC05p08995
[12]	M. H. Ali, I. Abustan, A new novel index for evaluating model performance, J. Nat. Resour. Dev., 4 (2021), 1–9. https://doi.org/10.5027/jnrd.v4i0.01 doi: 10.5027/jnrd.v4i0.01
[13]	F. van den Bosch, J. A. J. Metz, J. C. Zadoks, Pandemics of focal plant disease, a model, Phytopathology, 89 (1999), 495–505. https://doi.org/10.1094/PHYTO.1999.89.6.495 doi: 10.1094/PHYTO.1999.89.6.495
[14]	S. Lee, C. Masclaux-Daubresse, Current understanding of leaf senescence in rice, Int. J. Mol. Sci., 22 (2021), 1–19. https://doi.org/10.3390/ijms22094515 doi: 10.3390/ijms22094515
[15]	S. Bregaglio, P. Titone, G. Cappelli, L. Tamborini, G. Mongiano, R. Confalonieri, Coupling a generic model to the WARM rice simulator to assess leaf and panicle blast impact in a temperature climate, Eur. J. Agron., 76 (2016), 107–117. https://doi.org/10.1016/j.eja.2016.02.009 doi: 10.1016/j.eja.2016.02.009
[16]	T. Gilet, L. Bourouiba, Fluid fragmentation shapes rain-induced foliar disease transmission, J. Roy. Soc. Interface, 12 (2015), 1–12. https://doi.org/10.1098/rsif.2014.1092 doi: 10.1098/rsif.2014.1092
[17]	O. Singh, J. Bathula, D. K. Singh, Rice blast modeling and forecasting, Int. J. Chem. Stud., 7 (2019), 2788–2799.
[18]	S. Savary, A. Nelson, L. Willocquet, I. Pangga, J. Aunario, Modeling and mapping potential epidemics of rice disease globally, Crop Prot., 34 (2012), 6–17. https://doi.org/10.1016/j.cropro.2011.11.009 doi: 10.1016/j.cropro.2011.11.009

This article has been cited by:

1.	Mostafa M.A. Khater, Thongchai Botmart, Unidirectional shallow water wave model; Computational simulations, 2022, 42, 22113797, 106010, 10.1016/j.rinp.2022.106010
2.	Alina Alb Lupas, Characteristics of a Subclass of Analytic Functions Introduced by Using a Fractional Integral Operator, 2022, 8, 2409-5761, 75, 10.15377/2409-5761.2021.08.5
3.	Maysaa Al Qurashi, Saima Rashid, Sobia Sultana, Fahd Jarad, Abdullah M. Alsharif, Fractional-order partial differential equations describing propagation of shallow water waves depending on power and Mittag-Leffler memory, 2022, 7, 2473-6988, 12587, 10.3934/math.2022697
4.	Mostafa M. A. Khater, Computational Traveling Wave Solutions of the Nonlinear Rangwala–Rao Model Arising in Electric Field, 2022, 10, 2227-7390, 4658, 10.3390/math10244658
5.	Vediyappan Govindan, Samad Noeiaghdam, Unai Fernandez-Gamiz, Sagar Ningonda Sankeshwari, R. Arulprakasam, Bing Zhao Li, Shehu Integral Transform and Hyers-Ulam Stability of nth order Linear Differential Equations, 2022, 18, 24682276, e01427, 10.1016/j.sciaf.2022.e01427
6.	Rashid Nawaz, Nicholas Fewster-Young, Nek Muhammad Katbar, Nasir Ali, Laiq Zada, Rabha W. Ibrahim, Wasim Jamshed, Haifa Alqahtani, Numerical inspection of (3 + 1)- perturbed Zakharov–Kuznetsov equation via fractional variational iteration method with Caputo fractional derivative, 2024, 85, 1040-7790, 1162, 10.1080/10407790.2023.2262123
7.	M. L. Rupa, K. Aruna, K. Raghavendar, Insights into the time Fractional Belousov-Zhabotinsky System Arises in Thermodynamics, 2024, 63, 1572-9575, 10.1007/s10773-024-05770-0
8.	Saumya Ranjan Jena, Itishree Sahu, A novel approach for numerical treatment of traveling wave solution of ion acoustic waves as a fractional nonlinear evolution equation on Shehu transform environment, 2023, 98, 0031-8949, 085231, 10.1088/1402-4896/ace6de
9.	Yong Zhang, Graham E. Fogg, HongGuang Sun, Donald M. Reeves, Roseanna M. Neupauer, Wei Wei, Adjoint subordination to calculate backward travel time probability of pollutants in water with various velocity resolutions, 2024, 28, 1607-7938, 179, 10.5194/hess-28-179-2024

Reader Comments

Your name:*

Email:*
© 2023 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)