1.
Introduction
The Sustainable Development Goals (SDGs) were launched in 2015 as part of the 2030 Agenda for Sustainable Development adopted by the United Nations (UN). This plan of action was based on 17 SDGs designed to address the global challenges of climate change, social inequality and environmental degradation. These objectives call for worldwide action involving civil society, public administrations and companies to achieve shared and sustainable prosperity. These goals were not specifically intended to be targets for businesses; nonetheless, businesses play a key role in the Sustainable Development Agenda. The promulgation of the SDG concept encourages companies to take an active and effective role by integrating sustainability strategies into their business operations (Muñoz-Torres et al., 2019). To this end, various initiatives, such as the SDG Compass Guide (UN Global Compact, 2015), SDG Ambition (UN Global Compact, 2020) and SDG Essentials for Business (WBCSD, 2021), among others, have been designed to guide firms in their commitment to SDGs.
These initiatives implies the integration of SDGs into business strategies, although the adoption of these new strategies suppose some organizational challenges (Vildåsen, 2018; van Tulder, 2018). Some authors claim that the efforts made by firms toward the adoption of SDGs will translate into higher levels of organizational performance (Morioka et al., 2018; Elalfy et al., 2021; Diaz-Sarachaga, 2021; Khaled et al., 2021). Others, such as van Zanten and van Tulder (2018), express doubts as to the ability of isolated organizations to meet certain SDG requirements. Some academics, such as Li and Wu (2017) and Lassala et al. (2021) go as far as to assert that SDG adoption involves long implementation periods that could hinder organizational performance. Thus, the analysis of the relationship between firms' SDG commitment and organizational performance has so far proved inconclusive. These controversial results could be due to the omission of certain variables potentially affecting this interaction.
The institutional characteristics of the business environment, in particular, could influence the process of adopting sustainability practices associated with the SDGs. Buhr and Freedman (2001), Delmas and Toffel (2008), Chen and Bouvain (2009), Vormedal and Ruud (2009) and Jensen and Berg (2012) had already pointed out, in this regard, that the country-specific institutional context affects corporate behavior and the adoption of sustainability practices by defining the "rules of the game." The institutional context of a society is shaped by cultural factors, norms and values, which determine how companies work and behave (Salem and Ayadi, 2022). Doupnik and Salter (1995, p.191) had already stated that "culture is determined by environmental factors modified by external influences. Culture, in term, have institutional consequences in the form of legal system, political system and nature of capital markets." Even so, previous studies have ignored the role of these factors in the adoption of the SDG, focusing instead on organizational characteristics in firm samples drawn largely from institutionally homogeneous economies (e.g., Rosati and Faria, 2019a, 2019b). This leads us to question whether cultural factors, as an expression of the institutional context in which the organization is operating, might affect the interaction between SDG adoption and organizational performance levels.
This study has then two aims: to analyze the interaction between SDG commitment and organizational performance and to examine how this interaction is influenced by cultural factors. To achieve these objectives, we accessed data for a sample of 3,420 companies representing 30 countries and covering the period from 2015 to 2020. This study makes several contributions to the existing literature. First, it emphasizes the significance of cultural factors in shaping the adoption of the SDGs in private firms, expanding the understanding of the contextual influences on organizations' sustainability practices. Second, by highlighting the relevance of institutional theory, we contribute to a better comprehension of the mechanisms through which external factors, based on the cultural context, determine organizations' commitment toward sustainability goals. Additionally, the study advocates for a multi-theory approach that combines firm-specific characteristics and institutional factors to provide a comprehensive overview of organizations' engagement with the SDGs. This approach enhances the understanding of the complex interplay between internal and external factors in influencing sustainability practices. Finally, we underscore the importance of considering the broader socio-cultural environment in which organizations operate when designing and implementing sustainability initiatives. This latter contribution is especially relevant for practitioners, particularly managers, who need to create an efficient organization-environment balance that is adapted to institutional conditions. Public administrations should also promote international initiatives, given that some multinational companies might commit to SDGs in their immediate environment while ignoring the impact of their activities further afield.
The structure of the paper is as follows. Section 2 presents a literature review and our hypotheses. Section 3 reports the data, the variables and the statistical techniques used to test the proposed hypotheses, and the results are given in Section 4. Section 5 discusses the results, and the final section provides the conclusions.
2.
Literature review and working hypotheses
SDGs were originally conceived as a guide for the consideration of governments, lawmakers and regulators when designing public policies (Stevens and Kanie, 2016; Biermann et al., 2017). The collaboration of the private sector is key in SDG implementation (Florini and Pauli, 2018; Vazquez-Brust et al., 2020). This is a challenge for private businesses who are called upon to alter their organizational decision-making processes in order to achieve goals that were never designed to fit business perspectives (Scheyvens et al., 2016; Frey and Sabbatino, 2018; Gneiting and Mhlanga, 2021). To facilitate SDG adoption, the United Nations(2015, 2021) published the SDG Compass, among other documents, as a road map for businesses. Santos and Bastos (2020) highlight that SDG adoption would benefit private companies by revealing business opportunities, promoting stakeholder relationships, stabilizing societies and markets and facilitating the initiation of corporate sustainability practices.
There are various theories to account for the advantages of SDG adoption. Arguing from the agency theory perspective, García-Meca and Martínez-Ferrero (2021) underline that "SDG addressing can have financial effects due to the fact that value relevant disclosure improves performance by reducing information asymmetry." Lower information asymmetry would mean fewer conflicts of interests between shareholders, other stakeholders and the management, thereby curbing opportunism (Naciti, 2019; Buallay, 2019). Stakeholder theory has also been put forward as a possible theoretical framework to explain the potential advantages of SDG adoption, taking into account the mutual influences of agents and organizations (Freeman, 1984). Khaled et al. (2021) claims that companies commit to SDGs as a way to meet stakeholder interests, which would imply that, by meeting the direct and indirect needs of stakeholders, organizations could improve their performance and probability of survival, while protecting social objectives and development (Erin et al., 2022). Meanwhile, legitimacy theory provides a complementary framework to explain SDG engagement in business. Suchman (1995) defines legitimacy as "a generalised perception or assumption that the actions of an entity are desirable, proper, or appropriate within some socially constructed system of norms, values, beliefs, and definitions." In a quest for legitimacy, some companies adopt SDGs as a way to raise their performance and improve, maintain or repair their image among stakeholders (Faisal et al., 2015; Erin et al., 2020, 2022). These are, however, internal development approaches, whereby the organization tries to reduce internal conflict by promoting stakeholder relationships, enhancing corporate image and building legitimacy.
Cultural factors, permeating the institutional context, can also contribute to SDG adoption. In Hofstede's (2001) conceptualization of cultural factors, he defines cultural factors as "a set of values, in the sense of broad tendencies to prefer certain states of affairs over others." Hofstede's cultural factors are based on six dimensions: a) Power distance, b) Individualism, c) Masculinity, d) Uncertainty avoidance, e) Long-term orientation and f) Indulgence1. Institutional theory, postulated by DiMaggio and Powell (1983), Meyer and Rowan (1991) and Scott (2013), highlights that corporate decision-making is shaped by the social norms, rules and values prevailing in the business operating environment. SDGs could therefore be identified as a cultural paradigm shift based on the new rules emerging from current environmental and social demands (Chu et al., 2018; Berrone et al., 2013). Pizzi et al.(2020, 2021) provided evidence that the SDG commitment decision depends on certain contextual factors, which could condition the organization's view of the issue. Companies will tend to homogenize their behavior with that of others in order to meet contextual demands and boost their legitimacy (Powell and DiMaggio, 1984). Institutional theory identifies this process as isomorphism, which is a response to internal and external pressures leading to the homogenization of organizations operating in the same context (Hawley, 1968). Two types of isomorphism have been identified: competitive and institutional (Meyer, 1979). Competitive isomorphism is that which occurs in a context of market competition, niche change and business fitness measures, where organizations will tend to adopt similar practices in addressing certain market challenges (Hannan and Freeman, 1977). Institutional isomorphism, meanwhile, drives companies, under the influence of coercive, mimetic and normative pressures, to imitate others in a bid to boost their legitimacy. Thus, SDG commitment may be driven both by a company's idiosyncratic sustainability standards (competitive isomorphism) and its surrounding cultural framework (institutional isomorphism), such that both internal and external factors intervene.
1 Power distance expresses the degree to which the less powerful members of a society accept and expect that power is distributed unequally. Individualism can be defined as a preference for a loosely-knit social framework in which individuals are expected to take care only of themselves and their immediate families. The Masculinity side of its dimension represents a preference in society for achievement, heroism, assertiveness and material rewards for success. Society at large is more competitive. Its opposite, Femininity, stands for a preference for cooperation, modesty, caring for the weak and quality of life. The Uncertainty Avoidance dimension expresses the degree to which the members of a society feel uncomfortable with uncertainty and ambiguity. Long-Term Orientation assesses links with one's own past while dealing with the challenges of the present and the future. Finally, Indulgence represents a society that allows relatively free gratification of basic and natural human drives related to enjoying life and having fun (Hofstede, 2011). Additionally, we have also included the level of development in the SDGs achieved by the different countries.
Previous literature has shown inconclusive results regarding the relationship between SDG commitment and organizational performance (Muhmad and Muhamad, 2021). Morioka et al. (2018), Elalfy et al. (2021), Diaz-Sarachaga (2021) and Khaled et al. (2021) provide evidence of positive interaction between the two. This could be explained by improvements in resource-use efficiency (Muhmad and Muhamad, 2021) together with greater diversification of funding sources (Zabala-Aguayo and Ślusarczyk, 2020). However, Li and Wu (2017) and Lassala et al. (2021) warn that these positive effects are not immediate and that companies may even see efficiency and productivity losses during the implementation period. Other authors even cast doubt on the "actionability" of SDGs, which were not specifically designed for the business context (van Zanten and van Tulder, 2018). According to these authors, the actionability of the SDGs means that the fulfilment of some SDG objectives requires collaboration with other economic agents. Indeed, the excessive generality of some SDGs could result in a lack of specificity in firms' SDG-related policies, with explicit performance-related effects.
These contradictory results could be due to omitted variables. Indeed, previous studies draw their conclusions from different contexts: Li and Wu (2017) focused on a sample of Chinese companies, and Diaz-Sarachaga (2021) focused on Spanish ones. The cultural differences between these two contexts could account for the observed disparity of signs. Elalfy et al. (2021) highlight, in this respect, the possible influence of institutional and regional factors in SDG adoption. Diaz-Sarachaga (2021) points out that the identification of conditions for SDG attainment remains a pending issue for researchers. Thus, although internal pressures appear to motivate SDG adoption as a way to increase efficiency levels, the reported outcomes for organizational performance differ across study contexts. Pizzi et al. (2021) conducted an analysis of the determinants influencing business contribution to the 2030 Agenda. Their findings indicate that the adoption of the SDGs is influenced by firm-level, governance-level and report-level determinants. However, they also suggest the need for future research to assess the impact of cultural-specific characteristics on firms. Indeed, few studies have explored the impact of cultural factors on the interaction between commitment to the SDGs and organizational performance. Using the Hofstede's cultural factors, Rosati and Faria (2019a) found that organizations reporting on SDGs are more likely to operate in countries characterized by indulgent, egalitarian, short-term-oriented and individualistic cultures. Similarly, Pizzi et al. (2022) highlight that companies operating in institutional contexts with a long-term orientation and a balanced level of indulgence and restraint are more inclined to disclose their contributions to the SDGs. Ordonez-Ponce (2023) also note that certain cultural dimensions, such as individualism, uncertainty avoidance, long-term orientation and indulgence, positively influence sustainability, whereas power distance and masculinity have a negative impact. Based on the findings of these previous studies, all cultural factors, except power distance and masculinity, will have a positive impact on the commitment toward the SDGs and the influence on organizational performance.
There are different reasons that justify this positive effect. The cultural context could explain inter-organizational differences as being driven by the external pressures of institutional isomorphism (Hák et al., 2016; Schramade, 2017; Rosati and Faria, 2019a; Pizzi et al., 2020, 2021). These authors conclude that the organizational context determines both degrees of engagement with SDGs and levels of organizational performance. In the same vein, Blodgett et al. (2001) and Prexl and Signitzer (2008) highlight a clear connection between organizational and managerial norms and values and those prevailing in the surrounding environment. However, previous studies have some limitations. Some studies focus solely on a macro-level analysis without considering the role of cultural factors at the firm level. Others concentrate on the level of voluntary information disclosure related to the SDGs without examining the interaction between cultural factors, commitment to the SDGs and organizational performance. To test this interaction, we propose the following working hypotheses:
H1: SDG engagement has an impact on organizational performance.
H2: Cultural factors influence the interaction of SDG engagement with organizational performance.
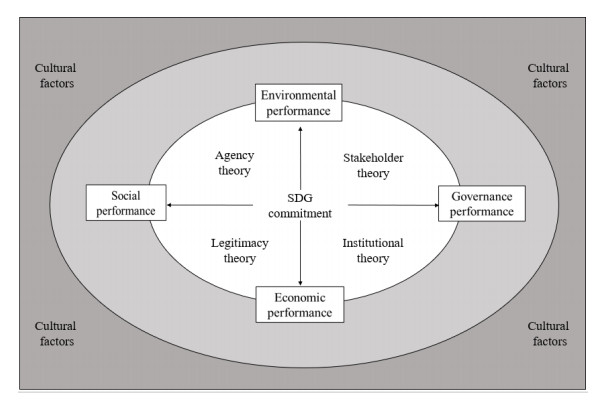
The rejection of the first hypothesis implies that commitment with SDGs has no effect in terms of organizational performance, possibly because of actionability issues, as proposed by van Zanten and van Tulder (2018). Non-rejection, on the other hand, would mean that SDG engagement affects business performance, although the impact could be moderated by cultural factors, as postulated by the second hypothesis. In this case, differences in cultural pressures could result in different degrees of SDG commitment. Figure 1 depicts interactions between SDG engagement, organizational performance and cultural factors based on the exposed theoretical frameworks.
3.
Methodology
3.1. Sample
To test the proposed working hypotheses, we accessed data for the years 2015 to 2020 on a sample of 3,420 members of the RobecoSAM (S & P Sustainability2) universe, which is made up of companies that contribute to the targets as formulated in the UN Sustainable Development Goals (SDGs) (RobecoSAM, 2022). The sample covers 30 countries, with a plurality located in the United States (21.43%), followed by Japan (11.11%), China (9.21%), the United Kingdom (7.69%), France (3.83%), India (3.68%), Australia (3.30%) and Germany (3.01%).3 The sample period starts in 2015, the year of the initial SDG proposal (United Nations, 2015). Table 1 shows the main details of the study sample.
2 The RobecoSAM (S&P Sustainability) universe has been retrieved from https://www.spglobal.com/esg/csa/.
3 The remainder belong to South Korea (2.81%), Hong Kong (2.57%), Brazil (2.46%), Canada (2.11%), South Africa (2.02%), Sweden (1.93%), Switzerland (1.81%), the Netherlands (1.64%) and others (19.39%).
Data provided by EIKON-Refinitiv reveal that the sample companies show quite modest ESG scores, with only 8.38% scoring higher than 81 points. The majority of these companies maintain positive economic return rates, some showing rates of over 10%. Firm size in this sample is high in terms of total assets and leverage, and the dominant sectors, based on the Thomson Reuters Business Classification (TRBC), are technology (14.18%), consumer cyclicals (13.48%), financials (14.12%) and industrials (14.06%). These firms operate in a diversity of institutional environments, with a prevalence of strong SDG commitment (98.00%). The data for the study variables were retrieved from the EIKON-Refinitiv and ORBIS databases and the Hofstede Centre (2022)4. Figure 2 shows the distribution of the studied sample by country, activity sector and cultural factors.
4 Data Availability: The database and the covariance matrix can be found at https://doi.org/10.5281/zenodo.79760XX.
3.2. Main variables
3.2.1. Sustainable Development Goal engagement
Findings by Jacob (2017), Lior et al. (2018) and Khaled et al. (2021), among others, evidence a diversity of systems for reporting corporate SDG commitment levels. The United Nations (2021) through the SDG compass initiative accounted for a total number of 1,553 indicators that companies can use to report their progress toward the SDGs. Under the Global Reporting Initiative proposal, this set of indicators is reduced to 362 indicators, which is also a large number. Recently, Khaled et al (2021) have summarized this proposal in 48 indicators, linking the SDG compass, the GRI measurements and the indicators provided by EIKON Refinitiv. The selection of the SDG engagement, based on Refinitiv EIKON, has been used by other authors, such as Sierra et al. (2022), van Zanten & Huij (2022) and Zampone et al. (2023). This database uses the following central question to guide the measurement of the dependent variable: "Which data points reflect the SDGs reported in corporate sustainability reports (or equivalent) according to SDG Compass?" According to all these authors, Refinitiv Eikon data collects information about different aspects of SDGs, whose values are reflected in various data points. Using 17 dichotomous items, one for each SDG, this agency evaluates firms' contributions to each of the 17 SDGs defined by the United Nations.
3.3.2. Organizational performance
The environmental, social, governance and economic aspects of a business are encompassed in what is known as organizational performance (Richard et al., 2009), for which we use two indicators. One is the ESG score from EIKON-Refinitiv which serves as an indicator of environmental, social and governance performance (EIKON-Refinitiv, 2019). According to López-Arceiz et al. (2020), the ESG score constitutes an indicator oriented toward accountability, which is especially relevant in the context of the stakeholder management associated with the SDGs. The other, also used by following Ortas and Moneva (2011), is the return on assets, an accounting proxy for economic performance. According to these authors, "accounting-based measures reflect the organization's internal efficiency, " being a suitable proxy when the effects of an internal decision, as is the case with SDG engagement, are analyzed.
3.2.3. Cultural factors
The inclusion of cultural factors enables us to analyze the contextual determinants of SDG engagement and organizational performance. For this, we propose the use of the cultural values based on Hofstede's classification (2011), which identifies six indicators: power distance, individualism, masculinity, uncertainty avoidance, long-term orientation and indulgence. Hofstede's values have been used in several papers (Nurunnabi, 2015; Lee and Herold, 2018; López-Arceiz et al., 2018, 2020). In addition to these, we use country-level SDG scores retrieved from EIKON-Refinitiv based on the indicators constructed by the Organisation for Economic Cooperation and Development (2017) as a proxy for the institutional context of companies adopting SDGs. According to McArthur and Rasmussen (2019), these indicators could serve as a tool for tracking progress within countries, relative to each society's own needs on each goal.
3.2.4. Control variables
This study includes the following control variables: size, leverage and activity. In relation to size, García-Meca and Martínez-Ferrero (2021) claim that "larger firms can benefit from increased firm performance by enjoying better scaled economies and having greater pools of resources." We take the natural log of total assets as a firm-size indicator (SZ) (López-Arceiz et al., 2018). The level of leverage (LEV) has also been positively associated with the adoption of sustainability practices (Buallay, 2019). We have included this variable as it has been observed that managers tend to disclose more ESG information when facing increased scrutiny from financial institutions (Atan et al., 2018). Additionally, the specific activity sector of companies can impact their adoption of the SDGs, with firms in environmentally impactful sectors potentially facing greater difficulties in aligning with the SDGs (Khaled et al., 2021). We use the Thomson Reuters Business Classification (TRBC) for activity sectors: energy, basic materials, industrials, consumer cyclicals, consumer non-cyclicals, financials, healthcare, technology, telecommunication services and utilities.
Appendix Ⅰ describes the variables presented in this section.
3.3. Statistical techniques
Given the aim of this study, we begin with a descriptive analysis of the indicators in terms of their first- and second-order moments. We then estimate the correlation matrix for the different indicators. After this preliminary analysis, we test the relationship between SDG commitment and sustainable performance in our sample firms using simultaneous equation modeling. The choice of statistical methodology responds to the size of the sample, the absence of multivariate normality and the temporal dependence between the observations (López-Arceiz et al., 2017). Equations (1–2) summarize the specified model.
ESGit and ROAit represent the ESG score and economic return of the i-th company in the period t, respectively. The term SDGwit considers the company commitment toward the w-th Sustainable Development Goal of the i-th company in period t. The Controlit term introduces organizational size, leverage and activity as control variables. Finally, α, β, γ and δ are the parameters of the estimated model, while ε is the random error term.
After estimating the basic model, we specify another including the cultural factors defined in the previous section (3–4):
The term Cultit represents the cultural factors (power distance, independence, masculinity, uncertainty avoidance, long-term orientation and indulgence, as well as country-level SDG achievement). Finally, we specify a model including lagged variables, as per Equations (5–6). Lagged variables are necessary in the context of innovative sustainable practices as their effect can only be observed in the long run (Bradley et al., 2010). Additionally, from a methodological viewpoint, lagged variables enable us to avoid endogeneity, simultaneity and reverse causality problems (Anzola-Román et al., 2023).
The subindex t-k denotes the lagged variables representing SDG commitment in previous periods (k = 1, k = 2). The analysis is then performed using a fixed effect estimator for panel data and SPSS 25.0, EQS 6.3 and Stata 16.0 software. Figure 3 represents the path diagram that enables us to test the proposed working hypotheses.
4.
Results
Table 2 shows the averages of the studied variables for the companies that composed the sample, allocated by country. Particularly, we have included the variables related to the SDG engagement, organizational performance, size, leverage and the cultural factors.
The general observation is that these firms have low SDG commitment (mean < 0.300). The highest SDG levels of commitment are to SDG 13 – Climate action and SDG 12 – Responsible consumption and production, and the lowest levels are to SDG 14 – Life below water and SDG 2 – Zero hunger. We also note high levels of organizational performance in terms of both ESG score and return on assets. Nevertheless, there are differences among countries. For instance, in relation to SDG 3 – Health and welfare, some countries (e.g., France and Sweden) have a score higher than 0.150, while others present lower values, such as the United States (0.088) and Australia (0.096). These differences could be explained by cultural factors. We detect that some societies in Nordic countries present a clear tendency to promote femininity values under low uncertainty tolerance and in an indulgent context. This description contrasts with other societies in continental countries where masculinity, risk tolerance and low indulgence are common. This preliminary evidence would suggest that cultural factors could influence the interaction between SDG engagement and organizational performance.
The correlation matrix for the variables of interest is shown in Table 3. The study variables have been grouped in four categories: SDG engagement, organizational performance, control variables and cultural factors.
The correlation matrix reveals a positive correlation among the different SDGs (p-value < 0.010). The observable negative impact of SDG commitment on economic return (p-value < 0.010) suggests a trade-off between SDG commitment and economic return, although this notion could be nuanced by the heterogeneity of organizations within the RobecoSAM (S & P Sustainability) universe. The correlation matrix also shows a positive interaction between engagement with SDGs and ESG score (p-value < 0.010). This result appears to indicate that SDG engagement promotes high levels of sustainable performance, despite yielding low economic return, at least in the short-term. The correlation matrix also shows that long-term orientation and uncertainty tolerance have positive associations with the various interactions analyzed (p-value < 0.010). Additionally, power distance and masculinity are found to have a negative impact on commitment toward the SDGs (p-value < 0.010). The cultural factors also influence performance levels, particularly in relation to the ESG score, indicating their potential significant effect on the studied interactions. For instance, in relation to some SDGs oriented toward environmental issues, such as SDG 14 – Life below water, this result could be explained by the positive impact of the sustainable practices adopted by the company and the expenses associated with an investment that could decrease the economic return, especially in the short run. Nevertheless, this result is also affected by some cultural factors (avoidance uncertainty and long-term orientation), which could potentiate this interaction.
Table 4 shows the results of the proposed regressions including parameter estimates of the organizational performance variables regressed on SDG commitment. We also include the cultural factors as a moderating variable.
There is a positive interaction between commitment to SDGs and ESG scores [(0.143; 0.263), p-value < 0.010]. In particular, we note commitment to SDG 13 – Climate action (0.263, p-value < 0.010), SDG 8 – Decent work and economic growth (0.255, p-value < 0.010) and SDG 5 – Gender equality (0.237, p-value < 0.010). However, the sign of the interactions between SDGs and economic return is negative [(−0.055; −0.008), p-value < 0.010]. This effect is particularly pronounced in the cases of SDG 11 – Sustainable cities and communities (−0.055, p-value < 0.010), SDG 7 – Affordable and clean energy (−0.048, p-value < 0.010) and SDG8 – Decent work and economic growth (−0.048, p-value < 0.010). Thus, we are unable to reject H1, given that SDG engagement does have an impact on organizational performance. It should be noted, however, that the sign of the effect varies with the specified indicator. This result is in accordance with Feng et al. (2021) and Muhmad and Muhamad (2021), who provided evidence that commitment to the SDGs contributes to improving corporate sustainability practices by effectively balancing environmental, social and economic impacts. However, Ma et al. (2017), Zhang and Chen (2017), Li and Wu (2017), Ahmad and Buniamin (2021) and Lassala et al. (2021) suggest that pursuing certain SDGs could potentially have negative effects on economic performance, as the resources invested may not yield immediate returns. Our findings support a positive association between SDG commitment and higher levels of sustainability performance, while also indicating a potential trade-off in terms of economic returns. These results could be conditioned by the cultural environment surrounding the organization.
In particular, we find evidence to show that cultural factors have a catalytic effect on SDG commitment. Two of them, namely, individualism and long-term orientation, are found, in general terms, to enhance the impact of SDGs on organizational performance. More specifically, the positive effect of SDG commitment is stronger in highly individualistic environments ([(0.022; 0.242), p-value < 0.010]. This effect is weaker ([−0.136;−0.002], p-value < 0.100) in societies promoting masculinity, understood asheroism, assertiveness and material rewards for success. We can also highlight the positive effect of long-term orientation ([0.044; 0.139], p-value < 0.010) in terms of both ESG attainment and economic return. These results contrast with those found for other factors, such as indulgence, uncertainty and power distance. Indulgent societies, which allow relatively free gratification of human needs, tend also to promote commitment toward SDG targets (p-value < 0.010), albeit with some exceptions: namely, SDG 5 – Gender equality (−0.023, p-value < 0.010) and SDG 11 – Sustainable cities and communities (−0.027, p-value < 0.010). Societies deciding to take a bet on SDG implementation amid high levels of uncertainty ([0.018; 0.088]; p-value < 0.010), appear to lose out in terms of economic return ([−0.052; −0.010]; p-value < 0.100), while the outcome is better when the same decision is taken under high power distance ([0.016; 0.079]; p-value < 0.010). Finally, the observed negative signs ([−0.253; −0.022]; p-value < 0.010) indicate that SDG engagement generates a substitution effect at country level. However, further analysis of the observed positive ESG scores reveals some exceptions. Thus, we find a degree of alignment and complementarity in SDG 1 – no poverty, SDG 2 – zero hunger, SDG 4 – quality education, SDG 11 – sustainable cities and communities, SDG 12 – responsible consumption and production and SDG 15 – life on land. Therefore, cultural factors can influence SDG attainment in different directions. Individualism, femininity values contrary to masculinity, long-term orientation and indulgence tend to promote SDG commitment at the business level, thereby enhancing organizational performance. Power distance, uncertainty and country-level engagement in SDGs yield diverse outcomes, depending both on the indicator used to measure organizational performance and on the SDG being pursued. These results are in line with Rosati and Faria (2019a), Pizzi et al. (2022) and Ordonez-Ponce (2023), who suggest that organizations reporting on SDGs are influenced by cultural factors. Our study extends this result and shows that some cultural dimensions have a positive influence on the relationship between the commitment toward the SDGs and the levels of organizational performance. Consequently, we cannot reject H2, as cultural factors condition the abovementioned interaction.
4.1. Robustness
This section presents some additional analysis we have performed to make our results robust. Tables 5 and 6 contain the estimations for the models with lagged variables. Lagged variables are crucial for studying innovative sustainable practices, addressing long-term effects and some methodological issues. Table 5 shows the results assuming that commitment in period t−1 affects organizational performance in period t. Table 6 contains estimates of the effects of SDG commitment in period t−2 on organizational performance in period t. While all the estimates include the control variables, the tables show only the moderator effects5. The first column shows the total effect of the company's SDG commitment.
5 The full regression estimates are available upon request.
In Table 5, it can be seen that the above reported results still hold for lagged SDG commitment. First, in relation to H1, we observe positive/negative effects of SDG commitment on ESG score (p-value < 0.010) and economic return (p-value < 0.010), respectively. Second, with respect to H2, individualism, femininity values contrary to masculinity, long-term orientation and indulgence continue to intensify the positive effect of SDG commitment on organizational performance. Nevertheless, we can highlight that, although the signs of the effects remain the same, their intensities are lower. A contrast in the results emerges in Table 6. With SDG commitment lagged by two periods, the impact of SDG engagement on economic return becomes positive (p-value < 0.010). The moderator effect on the ESG score disappears (p-value > 0.100), and that on economic return persists (p-value < 0.010) and even turns positive. Therefore, individualism, masculinity, long-term orientation and indulgence maintain their effects. Nevertheless, it is still worth noting the sign of masculinity, which would indicate that the underlying issue of material rewards behind this cultural value increases its impact over time. Additionally, this gradual but increasing positive effect in the long-term appears to be aligned with long-run commitment to SDGs. Depending on the institutional context, therefore, businesses might see a temporary decline in their economic performance before achieving long-term return gains. These findings align with those presented in the previous section. Therefore, despite its negative short-term effect, engagement with SDGs improves ESG scores and long-term economic returns, all of which are influenced by the characteristics of the cultural environment surrounding the organization.
Moreover, in Appendix Ⅱ, we provide the estimations for the basic model including the control variables. The results are confirmed, but it can also be seen that size is negatively associated with ESG score [(−0.186; −0.178); p-value < 0.010]. Meanwhile, leverage is associated positively with ESG score [(0.081; 0.098); p-value < 0.010] and negatively with economic return [(−0.036; −0.030); p-value < 0.010]. Finally, we note that the considered activities perform better than the basic category. This is particularly true for energy [(0.093; 0.179); p-value < 0.010] and healthcare [(0.054; 0.137); p-value < 0.010].
5.
Discussion
The results of this research show that there is a significant interaction between the commitment toward SDGs and the levels of organizational performance. ESG scores increase, while economic returns decrease temporarily, before improving in the longer term. These interactions are influenced by the organization's institutional context. In particular, factors such as individualism, absence of masculinity, long-term orientation and indulgence tend to exert a positive influence on the potential value of SDG commitment for organizational performance.
Previous literature on these issues has come up with controversial results (Khaled et al., 2021). Some authors, such as van Zanten and van Tulder (2018) and Ramos et al. (2022) claim that SDG commitment has no power over organizational performance because SDG targets are not set to be accomplished directly by private companies. Problems with actionability could adversely affect the organizational performance of firms engaging in the implementation of SDGs. Ma et al. (2017), Zhang and Chen (2017), Li and Wu (2017) and Ahmad and Buniamin (2021) show a negative relationship, whereby investment in SDGs compromises organizational performance levels. This result contrasts with Lassala et al. (2021), Muhmad and Muhamad (2021) and Feng et al. (2021), who associate commitment to SDGs with high levels of organizational performance. In this sense, the pursuit of SDGs would mean implementing advanced sustainability practices at the corporate level, thereby leveling out the environmental, social and economic impacts generated by the organization.
Our results show that SDGs have actionability potential for private firms, albeit in varying degrees. We can also note that commitment to SDGs improves ESG scores and also long-term economic returns, despite a short-term trade-off. Theoretically, this could be explained as being due to the improvement in the accessibility of economic, human and financial resources that comes with SDG commitment (Nicolò et al., 2022). The origins of this delayed positive interaction could also lie in social pressures motivating organizations to align themselves with their stakeholders (Calabrese et al., 2021). In this respect, SDG adoption would legitimize firms by making them appear more committed and socially conscious (Kücükgül et al., 2022). Finally, the negative sign on short-term returns might be explained in terms of opportunity costs. Short-term expenditures could require organizations to reduce their investment in assets with better short-term return potential (Wang et al., 2018). Nevertheless, this interaction could be sensitive to institutional and cultural influences on corporate approaches to sustainability practices and SDG adoption (Gaziulusoy and Brezet, 2015; Durugbo and Amankwah‐Amoah, 2019; van den Heiligenberg et al., 2022).
The results show that the characteristics of some countries could enhance the organizational performance effects related to the adoption of the SDGs. Some cultural characteristics of the institutional environment, particularly those relating to high levels of individualism, long-term orientation, femininity values contrary to masculinity and indulgence, strengthen the abovementioned positive effects. The level of SDG commitment within a society also shows a substitution effect. These results align with those of authors such as Hák et al. (2016), Schramade (2017), Rosati and Faria (2019a) and Pizzi et al.(2020, 2021), who provided evidence that institutional factors can affect the adoption of sustainability practices. We add to their findings with evidence to show that these factors can also affect commitment toward SDGs, in some cases not only by promoting their adoption but also by enhancing their positive impact on organizational performance, especially over longer periods.
These cultural factors, however, could describe a type of society with specific characteristics that promote the adoption of SDGs. Using the six factors provided by Hofstede's center (2022), the European countries that would fit the described profile are Austria, Belgium, Luxembourg, Switzerland and the United Kingdom. They can therefore be said to provide the most suitable environments for promoting corporate SDG adoption, in contrast with some Asian and Latin American environments, which undermine its potential benefits, a possibility that Naomi and Akbar (2021) attribute to the high levels of corruption and underdevelopment in those areas of the world. A similar conclusion is reached by Campagnolo et al. (2018), who show some Asian and Latin American countries as ranking among the lowest for SDG promotion in a list topped by European countries.
We must highlight that Hofstede's dimensions present some limitations, such as an oversimplification of cultural differences, inconsistencies between categories, provision of a static model and a lack of comparability (Signorini et al., 2009). Despite these limitations, this proposal also presents some advantages. On the one hand, they are the result of the cultural context where an organization is working. In this sense, Bertelsmann (2018) considers that culture and cultural context are the results of the religion, language and history of every country. However, these dimensions cannot be easily operationalized, but they can be behind the configuration of the Hofstede model. On the other hand, Hofstede's dimensions constitute an attempt to quantify the cultural development of a society. This property also enhances the usability of Hofstede's dimensions even if we compare with the proposal of some international organizations. For instance, Eurostat (2023), in the survey related to "Quality of Life in Europe, " highlights that "the gaps between EU Member States were probably related to cultural factors." Nevertheless, this organization does not define the meaning of cultural factors, which are associated with the socio-demographic structure of the population. The Global UN (2012) project also considers that cultural factors contribute to sustainable development as it promotes more effective interventions of public administrations and governments that lead to achieving economic, social, environmental and governance aims. The lack of operationalization of cultural factors poses a challenge in research, yet international bodies recognize their influence on organizational behavior and the adoption of sustainability practices. In the context of the SDGs, their adoption is contingent upon the institutional context, leading to varying outcomes in organizational performance, thereby explaining the contradictory findings in the existing literature.
6.
Conclusions
The aim of this study was to analyze the interaction between corporate SDG commitment and organizational performance and examine the cultural factors involved. The results obtained show that, by committing to SDGs, companies are able to modify their organizational performance, with specific benefits including higher ESG scores and greater long-term economic returns. However, these interaction effects are conditioned by cultural and institutional factors affecting the corporate environment. According to our observations, the positive forces in this respect are individualism, femininity values, long-term orientation and indulgence.
The above findings have various implications. Our academic contribution is evidence of the influence of cultural factors in the adoption of SDGs by private firms. In committing to SDGs, companies are led by isomorphic pressures, as argued by institutional theory. By examining cultural factors, we complement the findings of previous research, which has traditionally focused on organizational characteristics. We also highlight the role of SDG adoption as a legitimacy-seeking strategy, whereby firms attempt to improve their image with stakeholders. Consequently, success in this respect depends not only on the effort made by the organization but also on the cultural context in which it is operating. Among the implications for practitioners and lawmakers, one is the need for managers to adapt their corporate strategies and actions to the prevailing cultural and institutional environment, especially if it presents barriers to SDG integration. Another has to do with the observed adverse effect of SDG adoption on short-term economic returns, which could compromise a firm's financial strength. Finally, our results emphasize the need for public administrations and regulators to raise awareness of today's social and environmental challenges and promote closer collaboration with private companies. The latter requires context-specific social and environmental policies, especially in societies where cultural and institutional factors might prove a hindrance to SDG adoption.
This study has limitations requiring attention in future research. One is that it focuses on multinational and transnational companies operating in developed and emerging economies, which are potentially better endowed with resources for committing toward the SDGs and under greater pressure from financial markets and regulators to achieve SDG goals. We also leave it for future research to determine in what circumstances SDG adoption stems more from greenwashing than from true commitment and to explore additional institutional factors, such as the recent changes in social and environmental regulations, which could affect SDG adoption. Another limitation requiring attention is the lack of exhaustiveness resulting from the use of a single indicator of sustainability performance. Thus, our study leaves several avenues open for the development of an integrated theory to overcome current research gaps.
Use of AI tools declaration
The author declares that he has not used the Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
We gratefully acknowledge financial support from grant PID2019-104304GB-I00, grant PID2019-104304GB-I00 funded by MCIN/AEI/ 10.13039/501100011033 and grant TED2021-131216B-I00 funded by MCIN/AEI/10.13039/501100011033 and European Union "NextGenerationEU"/PRTR.
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad:





