On three dimensional fractal dynamics with fractional inputs and applications

1.   Introduction

The Cauchy transform of a measure in the plane is a useful tool for geometric measure theory ^[1,2,3], and it has also important applications in solving integral equations ^[4,5]. If the measure is a self-similar measure, the Cauchy transform of it has very rich fractal behavior. Stricharz et. al. ^[6] initiated the study of the Cauchy transform $F(z) = \int_{K}(z-\omega)^{-1}d\mu(\omega)$ of a self-similar measure $\mu$ with compact support $K$, and they proved that $F$ has a Holder continuous extension over $K$ and showed how to compute the Laurent expansion of $F$ in the complement of a disk containing $K$. Soon afterwards, more analytic and geometric properties of $F$ were given by Dong and Lau ^{[7,8,9,10,11,12]}: for example, the asymptotic behavior of the Laurent coefficients of $F$ and the region of starlikeness of $F$. They also gave estimates for the Taylor coefficients of the Cauchy transforms of some special Hausdorff measures ^[13,14]. For the special case that $K$ is the Sierpinski gasket, and $\mu$ is the normalized Hausdorff measure on $K$, Dong and Lau ^[8,9,10,11] carried out a detailed study of the properties of the mapping of the Cauchy transform on $K$ and investigated some open problems proposed in ^[6]. Away from $K$, $F$ is well-behaved, but the image of $F$ is chaotic near the boundary of $K$ and is difficult to catch [see 6, 7, 12]. In this paper, we will consider the properties of the function spaces of $F(z)$ near the Sierpinski gasket.

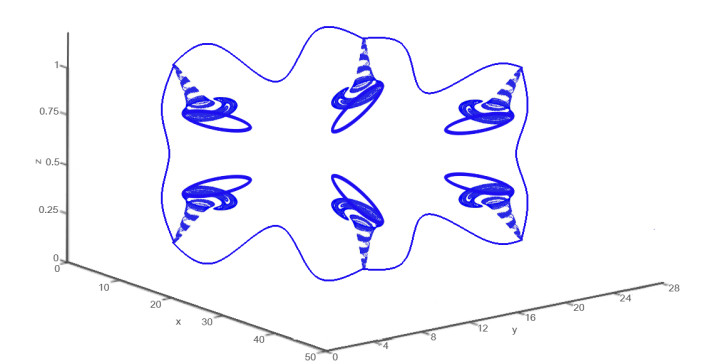
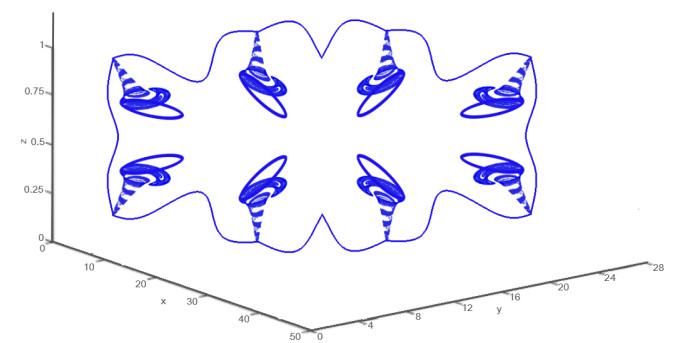
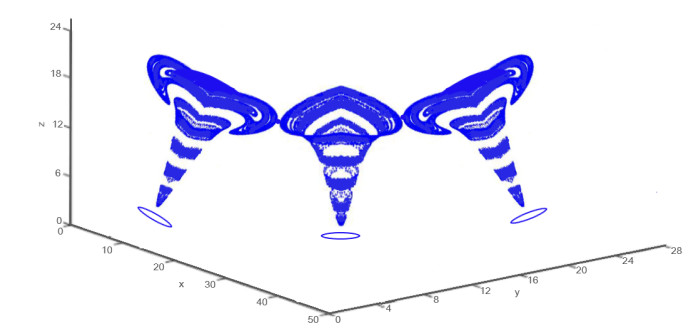
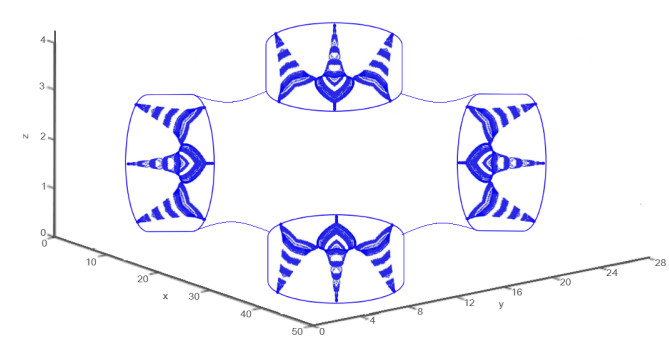
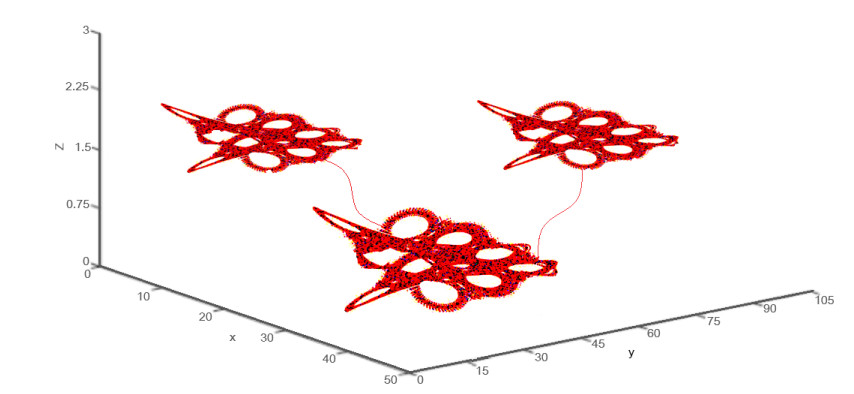
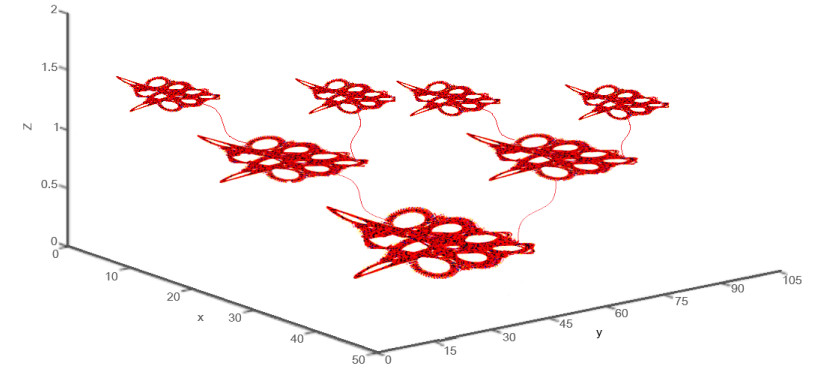
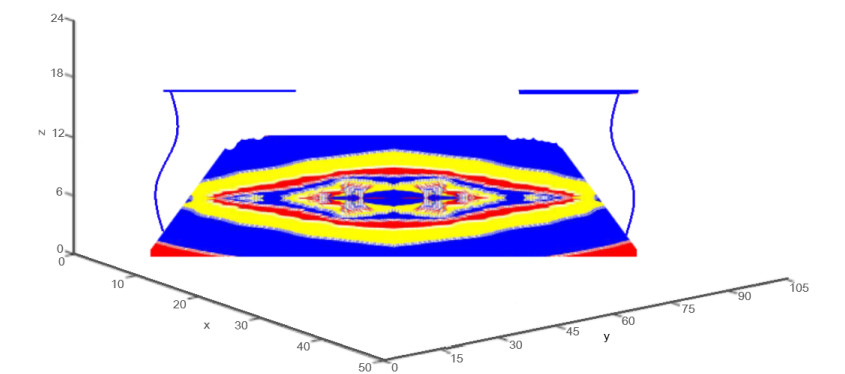
Let $S_j(z) = \varepsilon_j +(z-\varepsilon_j)/2$ be an iterated function system, where $\varepsilon_j = e^{2j\pi i/3}$ for $j = 0, 1, 2$. The attractor $K$ of $\{S_j\}_{j = 0}^2$ is just the Sierpinski gasket (Figure 1). It is well known that $K$ is a compact set, $\mathbb{C}\setminus K$ is a multiply connected domain, and the Hausdorff dimension of $K$ is $\alpha = \log3/\log2$. We denote unbounded connected region of $\mathbb{C}\setminus K$ by $\triangle_0$ and the triangular connected region of $\mathbb{C}\setminus K$ by $\triangle_n(n\geq 1)$. Then, $\mathbb{C}\setminus K = \cup_{n = 0}^\infty\triangle_n$.

Let $\mu$ be the uniform self-similar measure on $K$, i.e., $\mu$ is the restriction of the $\alpha$ -Hausdorff measure on $K$ normalized to a probability measure. With slight abusing of notation, we let $\mathcal{H}^\alpha$ be the Hausdorff measure normalized on $K$. From the basic property of the Hausdorff measure ^[15], for $E\subset\mathbb{C}$, we have $\mathcal{H}^\alpha(\phi(E)) = \mathcal{H}^\alpha(E)$, where $\phi$ can be the complex conjugation or the rotation of $e^{i\theta}$. Also, for any $n\in\mathbb{Z}, \, \mathcal{H}^{\alpha}(2^nE) = 2^{\alpha n}\mathcal{H}(E)$. The Cauchy transform of $\mu = \mathcal{H}^{\alpha} \vert_K$ is

Our main consideration is on the dyadic points of $\partial\triangle_0$. With fixed $k$, for $1\leq m\leq 2^k-1$, let

These are the dyadic points on the line segment joining the two vertices $\varepsilon_1$ and $\varepsilon_2.$ The dyadic points on the other two sides of $\partial\triangle_0$ can be obtained by $z_{k, m}$ multiplied by $\varepsilon_j, j = 1, 2$. It suffices to consider $z_{k, m}$ since $\varepsilon_jF(\varepsilon_jz) = F(z), \, j = 0, 1, 2.$

The paper is organized as follows. In Section 2, we introduce some necessary results and notations. In Section 3, we give an $H^p$ space property of $F(1/z)$ on $\vert z \vert < 1$. In the final section, we study the multiplier property of $F(1/z)$ on $\vert z \vert < 1$.

2.   Preliminaries

In this section, we first give some necessary notations and propositions firstly. Let $T = e^{\pi i}(K-1)$ be a relocation of the Sierpinski gasket $K$. The new vertices are at $0, \, \sqrt3e^{\pi i/6}, \, \sqrt3e^{-\pi i/6}$. Set $S_jK = K_j, \, j = 0, 1, 2$. Let $T_j = e^{\pi i}(K_j-1), \, j = 0, 1, 2,$ denote the three triangular components of $T$ containing the respective vertices. We define the "Sierpinski cones" of $T$ (Figure 2) as $A_0 = \bigcup_{n\in\mathbb{Z}}2^n(T_1\cup T_2).$ For $\ell = 1, \cdots, 5$, let $A_\ell = e^{\ell\pi i/3}A_0$, and

It is easy to check that $H_\ell(2z) = 2^{\alpha-2}H_\ell(z)$ by the scaling property of Hausdorff measure. In the sequel, we need the following propositions.

Proposition 2.1. ^[9] There exists some constant $C > 0$ such that,

Proposition 2.2. ^[9] For $0 < \rho < 1$, there exists some constant $C > 0$ which depends on $\rho$ such that for $\vert\arg z \vert < 5\pi/6$ and $0 < \vert z \vert\leq\rho\sqrt 3$,

For the details of the proof of the above two propositions, we can see ^[10].

3.   $H^p$ property of $F(\frac1{z})$ on $\vert z \vert < 1$

In this section, we consider the function space property of $F'(\frac1{z})$ on $\mathbb D = \{z: \vert z \vert < 1\}$. The Hardy space $H^p$ consists of analytic functions $f$ in $\mathbb D$ such that

Theorem 3.1. Let $g(z) = F(\frac 1{z})$ for $z\in\mathbb{D}$. Then, $g'(z)\in H^p$ for $0 < p < \frac 1{2-\alpha}$ and $g'\notin H^p$ for $p\geq\frac 1{2-\alpha}$, where $\alpha$ is the Hausdorff dimension of $K$.

Remark Similarly, we may prove that $g^{(k)}(z)\in H^p$ for $0 < p < \frac 1{k+1-\alpha}$ and $g^{(k)}(z)\notin H^p$ for $p\geq\frac 1{k+1-\alpha}$.

Proof. Note that $g(z)$ is analytic in ${\mathbb D}$, and $g'(e^{i\theta})$ exists for $\theta\notin\{ 0, 2\pi/3, 4\pi/3\}$. By Theorem 2.6 in [16, p. 21], we only need to prove $g'(e^{i\theta}) \in L^p$ for $0 < p < 1/(2-\alpha),$ and $g'(e^{i\theta})\notin L^p$ for $p\geq 1/(2-\alpha)$.

For $-\pi/3\leq\theta < 0$, let $z = e^{i\theta}$ and $z^* = \rho e^{-i\theta}\in \partial\triangle_0,$ where $\rho > 0$. By the sine rule, we have

From Proposition 2.1, there exists some constant $C > 0$ such that

Notice that $\mathcal{H}^\alpha$ and $K$ are symmetric with respect to the real-axis. Then, $g'(\bar{z}) = \overline{g'(z)}$, and $\int_{-\pi/3}^{\pi/3}{ \vert g'(e^{i\theta}) \vert}^pd\theta = 2 \int_{-\pi/3}^0{ \vert g'(e^{i\theta}) \vert}^pd\theta.$ Hence, for $0 < p < 1/(2-\alpha)$,

The above inequality gives $g'(e^{i\theta})\in L^p$ for $0 < p < 1/(2-\alpha)$.

Next, we will prove $g'(e^{i\theta})\notin L^{\frac1{2-\alpha}}$. For $0 < t\leq\sqrt {3}/2$ and $\vert\theta\mid < 5\pi/6$, from Proposition 2.2, we obtain

where the positive integer $N$ satisfies $1/2\leq 2^Nt < 1$. For $0 < t < 1$, let $1+te^{i\theta} = e^{i\varphi}$. Then,

Since $F'(e^{i\varphi}) = -e^{-2i\varphi} g'(e^{-i\varphi)})$ and $H_3(2z) = 2^{\alpha-2}H_3(z)$, we have

by using (3.2). Define $\beta = \arcsin(t/2)$ and $b = b(t): = 2^{N}t$. Noting that $b = b(t)\in [\frac {1}{2}, 1)$ and $i(e^{i\beta}-1) = -2\sin(\beta/2)e^{i\beta/2}$, we see that

To estimate $\varepsilon(t)$, we set $E_1 = -T_1, \, E_2 = -T_2$. Then,

With consideration of geometric factors, for $b\in[1/2, 1)$, $n\geq 1$ and $w\in E_1\cup E_2$, the two inequalities $\vert w-2^{-n}bi\mid\geq 3/4$ and $\vert bi-2^{-n}w\mid\geq \sqrt 3 b/2$ hold. Hence,

By $\sin\beta = t/2$, it is easy to check that $\sin(\beta/2) = \sqrt{1-\sqrt{1-t^2/4}}\Big/\sqrt 2 < t/3$ for small $t > 0$. This shows that we can find constants $C_2 > 0$ and $\delta > 0$ such that

From (3.4)–(3.6), we know that

where $C$ is a positive constant. This implies that, for $0 < t\leq\delta,$ we have

Let the positive integer $N_0$ satisfy $2^{-N}\leq\delta$ for all $N\geq N_0$. Note that $\varphi '(t)\geq c_1 > 0$ for $0 < t\leq\delta$. According to (3.7), we obtain

We can check that $H_3(z)$ is non-constant analytic in $\vert\arg z \vert < 5\pi/6$. This gives $c_2 > 0$. Noting that $\varphi(2^{-N-1})\rightarrow 0^+$ as $N\rightarrow \infty$, we have

By using ${(a+b)}^p\leq2^p(a^p+b^p)$ for $a > 0, b > 0$ and $p > 0$, we have

which implies that $g'(z)\notin H^p$ for $p\geq 1/(2-\alpha)$.

4.   Multiplier property of $F(\frac1{z})$ on $\vert z \vert < 1$

In this section, we consider the multiplier property of $g(z)$. Let $\Lambda$ denote the set of complex-valued Borel measures on $\mathbb{T} = \{z: \vert z \vert = 1\}$, let $k_\lambda(z) = (1-z)^{-\lambda}$ for $\lambda > 0$, and $k_\lambda(z) = \log\frac1{1-z}+1$ for $\lambda = 0$. Here, we choose the branch of $k_\lambda(z)$ which equals $1$ when $z = 0$. Let $\mathfrak{F}_\lambda$ denote the family of functions $h$ for which there exists $\mu\in\Lambda$ such that

Each $\mathfrak{F}_\lambda$ is a Banach space with respect to the norm defined by

where $\parallel\mu\parallel$ denotes the total variation of the measure $\mu$. The spaces $\mathfrak{F}_\lambda$ were introduced in ^[17,18], and some roperties of functions in $\mathfrak{F}_\lambda$ were obtained in ^[19,20].

An analytic function $\upsilon(z)$ in ${\mathbb D}$ is called a multiplier of $\mathfrak{F}_\lambda$ provided that $\upsilon(z)h(z)\in \mathfrak{F}_\lambda$ for all $h\in \mathfrak{F}_\lambda$. Let $\mathcal{M}_\lambda$ denote the set of all multipliers of $\mathfrak{F}_\lambda$. $\mathcal{M}_\lambda$ is a Banach space with respect to the norm defined by

The family $\mathcal{M}_\lambda$ has been studied in ^[19,20,21]. In this section, we will consider the multiplier property of $g(z) = F(1/z)$ with respect to $\mathfrak{F}_\lambda$.

Theorem 4.1. For each $\beta\geq 0$, $g(z)\in \mathcal{M}_\beta$. For any small $\varepsilon > 0$, $g'(z)\in\mathfrak{F}_{2-\alpha+\varepsilon}$ and $g'(z)\notin\mathfrak{F}_{2-\alpha-\varepsilon}$, where $\alpha$ is the Hausdorff dimension of $K$.

Proof. Since $g'\in H^p$ for some $p > 1$, we have $g\in\mathcal{M}_\beta$ for each $\beta\geq 0$ by Theorem 3.1 in [21, p. 621]. It follows from the remark of Theorem 3.1 that $g''(z)\in H^{1/(3-\alpha)}\subset H^{1/(3-\alpha+\varepsilon)}$. Together with Theorem 3 in [17, p. 116], we see that $H^{1/p}\subset\mathfrak{F}_p$ for $p\geq 1$. Hence, $g''\in \mathfrak{F}_{3-\alpha+\varepsilon}$. Note that $f\in \mathfrak{F}_\lambda$ if and only if $f'\in \mathfrak{F}_{\lambda+1}$ [17, p. 112]. Consequently $g'(z)\in\mathfrak{F}_{2-\alpha+\varepsilon}$ follows. From [7, p. 70], we obtain that $g(z) = z+\sum_{n = 1}^{\infty}a_{3n+1}z^{3n+1}$ for $\vert z \vert < 1$, and $c_1n^{-\alpha}\leq a_{3n+1}\leq c_2n^{-\alpha}$ for $n\geq 1,$ with constants $c_1 > 0$ and $c_2 > 0$. Assume that $g'(z)\in \mathfrak{F}_{2-\alpha-\varepsilon}$. Since every complex measure on $\mathbb{T}$ is of bounded variation, it follows easily that there exists some constant $c > 0$ such that $\vert (3n+1)a_{3n+1} \vert\leq cn^{1-\alpha-\varepsilon}, \, n\geq 1.$ This is a contradiction. Then, the result follows.

In view of Theorem 4.1, an interesting question is to determine if $g'(z)\in\mathfrak{F}_{2-\alpha}$, which is equivalent to ([17, p. 115]).

where $d_n(\lambda) = \frac{\Gamma(n+\lambda)}{\Gamma(n+1)\Gamma(\lambda)}$ is defined by $(1-x)^{-\lambda} = \sum_{n = 0}^\infty d_n(\lambda)x^n$. It follows from Stirling's formula that $d_n(\lambda)(n+1)^{1-\lambda} = \Gamma(\lambda)^{-1}+c(\lambda)(n+1)^{-1}+O((n+1)^{-2})$. Then,

where $\vert c_n \vert\leq C(n+1)^{-2}$. If we substitute this into (4.2), then we have

Since $\vert d_n(\alpha+1)\int_K\omega^nd\mathcal{H}^\alpha(\omega) \vert\leq C$ by ^[7], it follows that $h_2(z)\in H^2$ and $h_3(z)\in H^\infty$, which imply $c_1h_2(z)+h_3(z)\in \mathfrak{F}_1$ as $H^\infty\subset H^2\subset H^1\subset\mathfrak{F}_1$. Consequently,

In view of (4.3), by ^[18], we know that $g'(z)\in\mathfrak{F}_{2-\alpha}$ if and only if $\int_0^zh_1(t)dt\in\mathfrak{F}_0.$ This leads us to consider

Theorem 4.2. $f_\varepsilon\in\mathcal{M}_\beta$ for each $\beta\geq 0$ if $\varepsilon > 0$, and $f_0(z)\notin \mathcal{M}_\beta$ for each $\beta\geq 0$.

Proof. For the first assertion, we only need to show $f'_\varepsilon(z)\in H^p$ for some $p > 1$ by Theorem 3.1 in [21, p. 621]. Noting that $(1-x)^{-\lambda} = \sum_{n = 0}^\infty d_n(\lambda)x^n$ for $\vert x \vert < 1$, it follows easily by the H$\ddot{ \rm{o}}$lder inequality that

It follows from Proposition 4.2 in ^[7] that $\int_K \vert\omega \vert^{n}d\mathcal{H}^\alpha(\omega)\leq Cn^{-\alpha}$. Combining this with $d_n(\lambda)\sim\Gamma(\lambda)^{-1}n^{\lambda-1}$($n\rightarrow \infty$), we get that

Notice that $p(\alpha+1-\varepsilon)-2-\alpha\rightarrow -\varepsilon-1$ as $p\rightarrow 1$, we can choose $p > 1$ such that $p(\alpha+1-\varepsilon)-2-\alpha < -1-\varepsilon/2$. Hence, $f'_\varepsilon\in H^p$ for $p > 1$.

For the second assertion, it is sufficient to prove that $f_0(z)$ is unbounded in $\mathbb{D}$. By Theorem 5.2 in ^[7], we get that $\int_K \omega^nd\mathcal{H}^\alpha(\omega) = 0$ for $n\neq 3k$, and there exists some constant $c_1 > 0$ such that $\int_K\omega^{3k}d\mathcal{H}^\alpha(\omega)\geq c_1k^{-\alpha}$ for all $k\geq 1$. Note that $d_n(\alpha)\geq c_2n^{\alpha-1}$ for some constant $c_2 > 0$ and all $n\geq 1$. It follows that there exists some constant $c_3 > 0$ such that

Although we can not prove $f_0(z) = \int_0^zh_1(t)dt\in\mathfrak{F}_0$(or $g'(z)\in\mathfrak{F}_{2-\alpha}$), yet we can prove $f_0(z)\in \rm{BMOA}$, which consists of all functions $f\in H^1$ satisfying

where the supremum is taken over all arcs $I\subset\mathbb{T}$ with $\vert I \vert = \int_I \vert d\zeta \vert$ and $f_I = \vert I \vert^{-1}\int_If(\zeta) \vert d\zeta \vert$. It should be noted that $\mathfrak{F}_0\subset \rm{BMOA}\subset H^p$ for all $p > 0$ [21, p. 617].

Theorem 4.3. $f_0(z)\in \rm{BMOA}$.

Proof. We first prove that there exists some positive constant $C$ such that

It is equivalent to prove that $p(z): = (1-z^3)f'_0(z)$ is bounded for $\vert z \vert < 1$. It is easy check that $p(z)$ is continue on $\{z:\, \vert z \vert\leq 1/2\}$. Hence, $\max_{ \vert z \vert\leq 1/2} \vert p(z) \vert < \infty$. Next, we prove $p(z)$ is bounded for $1/2 < \vert z \vert < 1$. Let $\Omega = \{re^{i\theta}:1/2 < r < 1, \, -\pi/3\leq\theta\leq 0\}$. For $z\in\Omega$, let $d = {\rm{dist}}(z^{-1}, K)$. Obviously, $d > 0$ as $1 < \vert z \vert^{-1} < 2$. Noting that $p(e^{2\pi i/3}z) = p(z)$, $\vert p(\bar z) \vert = \vert p(z) \vert$, and we can check that there exists some positive constant $C_1$ such that

With consideration of geometry, we find that there exists some constant $C_2 > 0$ such that $d = {\rm{dist}}(z^{-1}, K)\geq C_2 \vert 1-z \vert$ for $z\in\Omega$. Hence,

Note that $\vert p(e^{2\pi i/3}z) \vert = \vert p(z) \vert$, $\vert p(\bar z) \vert = \vert p(z) \vert$. We obtain that $p(z)$ is bounded for $1/2 < \vert z \vert < 1$, and (4.5) follows.

It is known that an analytic function $\psi(z)$ on ${\mathbb D}$ belongs to BMOA if and only if $\vert\psi'(z) \vert^2(1- \vert z \vert^2)dxdy/\pi$ is a Carleson measure [22, p. 240]. By using (4.5), we have $\vert f'_0(z) \vert\leq C \vert 1-z^3 \vert^{-1}$. Hence, for any small sector $S_h(\theta_0) = \{re^{i\theta}: 1-h\leq r < 1, \vert \theta-\theta_0 \vert\leq h\}$,

This shows that $\vert f'_0(z) \vert^2(1- \vert z \vert^2)dxdy/\pi$ is a Carleson measure, and the result follows.

Acknowledgments

This work was supported by the NNSF of China (Grant No. 12101219) and the Hunan Provincial NSF (Grant No. 2022JJ40141). Also, the authors are grateful to Professor Xin-Han Dong for his guidance to complete this paper.

Conflict of interest

The authors declare no conflicts of interest.