Exact solutions of a class of nonlinear dispersive long wave systems via Feng's first integral method

1.   Introduction

The nonlinear dispersive long wave equations (DLWEs) are a class of important nonlinear partial differential equations (PDEs), which have widely arisen in oceanic water waves ^[1], fluid or plasma mechanics ^[2], dynamics ^[3] and other fields ^{[4,5,6,7,8,9,10]}. The solitary wave solutions of the DLWEs can be used to describe nonlinear wave phenomena such as dispersion, dissipation, diffusion, reaction, convection, etc. As far as we know, there exist some methods to find exact solutions of DLWEs, it is concluded that: the Bácklund transformations method and the Hirota bilinear method ^[1,2], the bifurcation method of planar dynamical systems ^[3], the modified Clarkson and Kruskal's (CK's) direct method ^[4], the variable separation approach ^[5], etc.

In this paper, we consider the following (2+1)-dimensional nonlinear dispersive long wave system:

where $u(x, y, t)$ is the horizontal velocity, $v(x, y, t)$ represents the wave altitude above the undisturbed water surface, $t$ denotes the time, and $(x, y)$ stands for the spreading plane. In fact, the system $(1.1)$ can be used to solve nonlinear evolutional problems in two spatial dimensions by inverse spectral transform, which was first obtained by Boiti ^[6] as a compatibility condition for a weak Lax pair. It is a hot topic to find the traveling wave solution of nonlinear partial differential equations (PDEs). Zhou ^[3] utilized dynamical systems and the numerical simulation method to study dynamical behaviors of travelling waves. Lou ^[7] gained nine types of two-dimensional partial differential equation and thirteen types of the ordinary differential equation with the help of the direct and nonclassical method. Zhang ^[8] obtained a kind of special multisoliton-like solutions of the system by using the Bácklund transform. Yomba ^[9] got some new soliton-like solutions in virtue of a modified extending tanh method. Elgarayhi ^[10] employed Jacobi elliptic functions to construct periodic wave solutions of the system (1.1). Recently, Zhu and Xia $et$ $al.$ studied the exact solutions of the Klein-Gordon equation and Hirota-Satsuma coupled KdV system ^[11] and Ginzburg-Landau equation ^[12] via method of the bifurcation theory of planar dynamical system. Zhang and Xia $et$ $al.$ also employed dynamical system method to obtain the exact solutions of the generalized combined double sinh-cosh-Gordon equation ^[13] and the fractional-order and integer-order Biswas-Milovic equation ^[14].

Different from them, we use Feng's first integral method ^[15] to obtain the exact solutions of the system (1.1). In fact, Feng's first integral method was initially proposed by Feng ^[16] which is based on the ring theory of commutative algebra. This powerful method has been widely applied to solve many PDEs and further developed by many authors ^{[17,18,19,20,21,22,23]}. Particularly, if $x = y$, the system (1.1) can be reduced to (1+1)-dimensional nonlinear dispersive long wave systems as follows:

where $u(x, t)$ and $v(x, t)$ stand for the horizontal velocity and height of water waves respectively. The system (1.2) can be used to describe the evolvement of the horizontal velocity composition $u(x, t)$ and height $v(x, t)$ of water waves which are spreading at an infinite narrow channel of finite constant depth in both directions ^[1,5].

The rest of this paper is organized as follows: Section 2, we introduce the steps of first integral method for the nonlinear partial differential systems. In section 3, we obtain eight groups of solutions for the (1+1)-dimensional and (2+1)-dimensional nonlinear dispersive long wave system respectively via Feng's first integral method. In section 4, we make a short summary.

2.   The steps of first integral method

Hosseini ^[22] and El-Sabbagh ^[23] had summarized the steps of Feng's first integral method for the PDEs. Here, we apply it to the system (1.2) as follows:

Step I. Utilize traveling wave transformations $u(x, t) = \phi(\xi)$ and $v(x, t) = \varphi(\xi)$, where $\xi = x-ct$, we can change (1.2) into an ordinary differential system as follows:

where "$'$" and "$''$" etc denote the derivatives about the same variable $\xi$.

Step II. By some proper mathematical calculation, we try to transform the system (2.1) into a second-order ordinary differential equation. Here, we can integrate the first equation of (2.1) once and let the integral constant be 0 and integrate it once. We have:

where $k_1$ is an integral constant. Integrate the second equation of (2.1) once, we have:

where $k_2$ is an integral constant. We rewrite (2.2) as $\varphi = c\phi-\frac{1}{2}\phi^2+k_1$ and substitute it into (2.3), we have:

Step III. With the introduction of new variables $X = \phi(\xi)$ and $Y = \phi'(\xi) = X'$, (2.4) can be changed into a system of ODEs as the following forms:

Step IV. We try to seek one first integration to (2.5) which reduces (2.4) to a first-order integrable ordinary differential equation by the Division Theorem which is on the basis of ring theory of commutative algebra. Finally, we can establish an exact solution to (1.2) by means of solving the resulting first-order integrable differential equation.

Division Theorem ^[24]: Suppose that $P(w, z)$ and $Q(w, z)$ are polynomials of two variables $w$ and $z$ and $P(w, z)$ is irreducible in $C[w, z]$. If $Q(w, z)$ vanishes at all zero points of $P(w, z)$, then there exists a polynomial $R(w, z)$ in $C[w, z]$ such that

Remark 2.1 If the system (1.2) is instead of a common partial differential system, the above train of thought and specific solving steps are the same (see ^[22,23]). Moreover, if the independent variables of (1.2) are $x, y$ and $t$ even more, the steps to resolve the system are similar.

3.   The exact solutions of the nonlinear dispersive long wave system

In this section, we try to seek the exact solutions for the (1+1)-dimensional and (2+1)-dimensional nonlinear dispersive long wave system via Feng's first integral method which was described in Sect. 2.

3.1.   Exact solutions to the (1+1)-dimensional nonlinear dispersive long wave system

By the steps of Feng's first integral method which were described in Sect. 2, we utilize the Division Theorem to seek the first integration of (2.5) now. Assume that $X = X(\xi)$ and $Y = Y(\xi)$ are the nontrivial solutions to (2.5) and $P(X, Y) = \sum_{i = 0}^m s_i(X)Y^i$ is an irreducible polynomial in $C[X, Y]$ such that:

where $s_i(X), i = 0, 1, 2, ..., m$ are polynomials of $X$, which $s_m(X)\neq 0$. In this case, we generally take $m = 1$ or $m = 2$. Equation (3.1) is also called the first integration to (2.5). According to the Division Theorem, there exists a polynomial $Q(X, Y) = g(X) + h(X)Y \in C[X, Y]$ such that:

Case 1: We suppose $m = 1$ in (3.1) and equate the coefficients of $Y^i, i = 0, 1$ on both sides of (3.2), that we can obtain a group of equations as follows:

As $s_i(X), i = 0, 1$ are polynomials of $X$, then from the first equation of (3.3), we can conclude that $s_1(X)$ is a constant and $h(X) = 0$. Without loss of generality, we take $s_1(X) = 1$ and substitute it into the second and third equations of (3.3), then balance the degrees of $g(X)$ and $s_0(X)$, that we can conclude that $\deg(g(X)) = 1$. Thus, we can assume $g(X) = A_1X + A_0$, where $A_1\neq0$, $A_1$ and $A_0$ are undetermined constants, then from the second equation of (3.3), we obtain:

where $B$ is an integral constant. We substitute $s_0(X), s_1(X)$ and $g(X)$ into the third equation of (3.3) and equate the coefficient of each power of $X$ to zero, which yields:

Solving (3.5), which leads to:

and

where $c$ and $B$ are arbitrary constants. By substituting (3.6), (3.7) into (3.1) and considering $Y = X'$, we can derive two first-order ordinary differential equations for $X'$ as follows:

and

By solving (3.8) and (3.9) respectively and considering $X(\xi) = \phi(\xi)$, $u(x, t) = \phi (\xi)$, $v(x, t) = \varphi(\xi)$, $\xi = x-ct$ and $\varphi = c\phi-\frac{1}{2}\phi^2+k_1$, we obtain four groups of solutions for (1.2) as follows:

where $c$ and $B$ are arbitrary constants, $2B-c^2 > 0$ and $C_1$ is an integral constant.

where $c$ and $B$ are arbitrary constants, $2B-c^2 < 0$ and $C_2$ is an integral constant.

where $c$ and $B$ are arbitrary constants, $2B+c^2 < 0$ and $C_3$ is an integral constant.

where $c$ and $B$ are arbitrary constants, $2B+c^2 > 0$ and $C_4$ is an integral constant.

Remark 3.1 We have got four groups of solitary wave solutions for (1.2) via Feng's first integral method when $m = 1$ in (3.1).

Case 2: Suppose $m = 2$ in (3.1) and equalize the coefficients of $Y^i, i = 0, 1, 2$ on both sides of (3.2), we obtain a group of equations as follows:

Because $s_i(X), i = 0, 1, 2$ are polynomials of $X$, then from the first equation of (3.14) we can conclude that $s_2(X)$ is a constant and $h(X) = 0$. Without loss of generality, we take $s_2(X) = 1$ and substitute it into the second and third and fourth equations of (3.14), then balance the degrees of $g(X)$ and $s_1(X)$ as well as $s_0(X)$ and $s_1(X)$, we can conclude that $\deg(g(X)) = 1$. Thus, we assume $g(X) = A_1X + A_0$, where $A_1\neq0$, $A_1$ and $A_0$ are undetermined constants, then from the second equation of (3.14), we have:

where $B$ is an integral constant. Then we substitute $s_2(X), s_1(X)$ and $g(X)$ into the third equation of (3.14). We have:

We substitute $s_0(X), s_1(X)$, $s_2(X)$ and $g(X)$ into the fourth equation of (3.14) and equate the coefficient of each power of $X$ to zero, that we can obtain a system of equations made up of $A_1, A_0, B, B_1, c, k_1$ and $k_2$. By solving this system, that we obtain two groups of solutions as follows:

and

where $c$ and $B$ are arbitrary constants. We substitute (3.17) and (3.18) into (3.15) and (3.16) respectively, that we can obtain the expressions for $s_0(X), s_1(X)$ and $s_2(X)$, then we plug these expressions into (3.1) and consider $Y = X'$, that we can derive two second-order equations for $X'$ as follows:

and

Solve (3.19) and (3.20) respectively, we obtain the following two first-order ordinary differential equations for $X'$:

and

Solve (3.21) and (3.22) respectively and consider $X(\xi) = \phi (\xi)$, $u(x, t) = \phi (\xi)$, $v(x, t) = \varphi(\xi)$, $\xi = x-ct$ and $\varphi = c\phi-\frac{1}{2}\phi^2+k_1$, we obtain four groups of solutions for (1.2) as follows:

where $c$ and $B$ are arbitrary constants, $B-c^2 > 0$ and $C_5$ is an integral constant.

where $c$ and $B$ are arbitrary constants, $B-c^2 < 0$ and $C_6$ is an integral constant.

where $c$ and $B$ are arbitrary constants, $B+c^2 < 0$ and $C_7$ is an integral constant.

where $c$ and $B$ are arbitrary constants, $B+c^2 > 0$ and $C_8$ is an integral constant.

Remark 3.2 We have got four groups of solitary wave solutions for (1.2) via Feng's first integral method when $m = 2$ in (3.1). All exact solutions of the (1+1)-dimensional nonlinear dispersive long wave system (1.2) have been obtained.

Now, we plot the three-dimensional graphs of the solutions (3.10) and (3.11) respectively (see the following Figures 1–4), the graphs of the rest of three groups of solutions (3.12), (3.23) and (3.25) are similar to (3.10), while the graphs of the rest of other three groups of solutions (3.13), (3.24) and (3.26) are similar to (3.11). We illustrate the figures as follows:

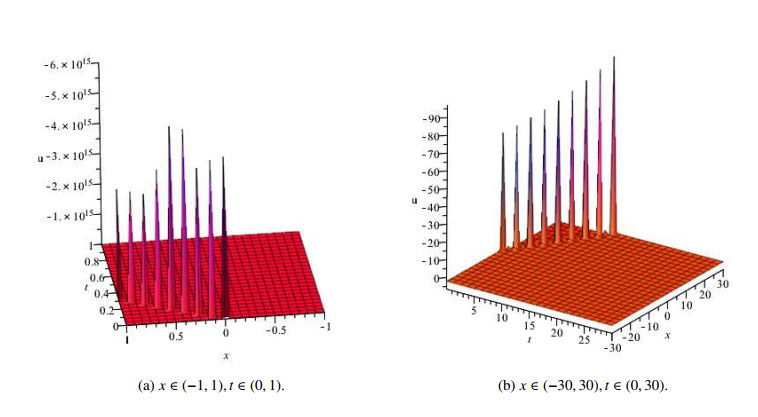
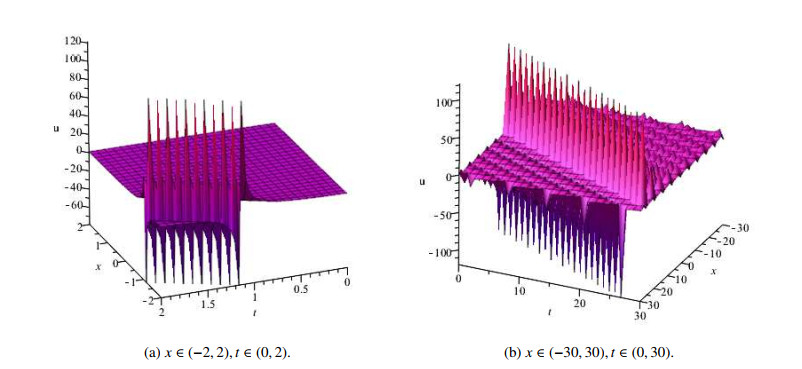
(1) Figure 1(a) displays the three-dimensional graph of the solution $u(x, t)$ of (3.10) with eligible parameters "$c = 1, B = 1, C_1 = 0$ '' and small variable intervals "$x \in(-2, 2), t \in(0, 2)$''. Figure 1(b) shows the three-dimensional graph of the solution $u(x, t)$ of (3.10) which takes the same eligible parameters and larger variable intervals "$x \in(-30, 30), t \in(0, 30)$''. The process of drawing and simulation shows that this is a class of solitary wave solution and the number and size of the solitons change greatly as the interval changes.

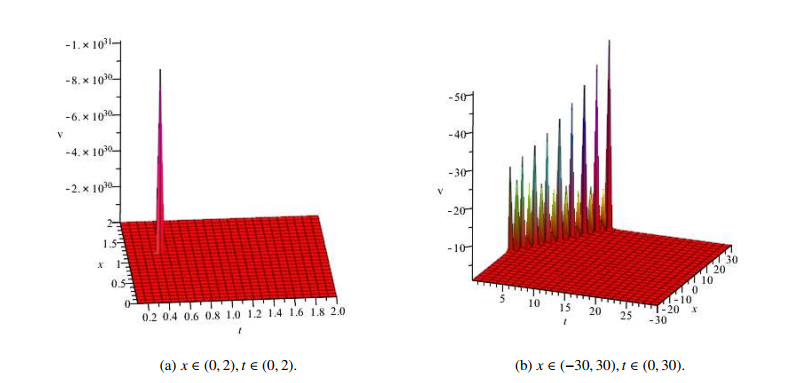
(2) Figure 2 displays the three-dimensional graph of the solution $v(x, t)$ of (3.10) with $c = 1, B = 1$ and $C_1 = 0$. Let "$x\in(-2, 2), t\in(0, 2)$'' in Figure 2(a), while the larger variable intervals "$x\in(-30, 30), t\in(0, 30)$'' is taken in Figure 2(b). The process of drawing and simulation shows that it is a distorted wave solution if we take a smaller variable interval, while it is a class of solitary wave solution if we take a larger variable interval. The number and size of these solitons change greatly as the interval changes.

(3) Figures 3(a) and 3(b) display the three-dimensional graphs of the solution $u(x, t)$ of (3.11) with eligible parameters $c = 3, B = 4$ and $C_2 = 0$ while Figures 4(a) and 4(b) display the three-dimensional graphs of the solution $v(x, t)$ of (3.11) with eligible parameters $c = 3, B = 4$ and $C_2 = 0$. The process of drawing and simulation shows that they are also a class of solitary wave solutions and the number and size of the solitons change greatly as the intervals change.

3.2.   Exact solutions to the (2+1)-dimensional nonlinear dispersive long wave system

By using the travel wave transformations $u(x, t) = \phi(\xi)$ and $v(x, t) = \varphi(\xi)$, where $\xi = x+ay-ct$, we change (1.1) into an ordinary differential system as follows:

Integrate the first equation of (3.27) once and let the integral constant be equal to 0 and integrate it once. We have:

where $k_3$ is an integral constant. Integrate the second equation of (3.27) once, we obtain:

where $k_4$ is an integral constant. We rewrite (3.28) as $\varphi = ac\phi-\frac{a}{2}\phi^2+k_3$ and substitute it into (3.29), we have:

With the introduction of new variables $\phi(\xi) = X(\xi)$ and $X'(\xi) = Y(\xi)$, (3.30) converts to a system of ODEs:

We utilize the Division Theorem to seek the first integration to (3.31) now. Assume that $X = X(\xi)$ and $Y = Y(\xi)$ are the nontrivial solutions to (3.31) and $P(X, Y) = \sum_{i = 0}^m s_i(X)Y^i$ is an irreducible polynomial in $C[X, Y]$ such that:

where $s_i(X), i = 0, 1, 2, ..., m$ are polynomials of $X$, which $s_m(X)\neq 0$. In this case, we generally take $m = 1$ or $m = 2$. Equation (3.32) is also referred to as the first integration to (3.31). According to Division Theorem ^[24], there exists a polynomial $Q(X, Y) = g(X) + h(X)Y \in C[X, Y]$ such that:

Case 3: Assume $m = 1$ in (3.32) and equate the coefficients of $Y^i, i = 0, 1$ on both sides of (3.33), we derive a groups of equations as follows:

Because $s_i(X), i = 0, 1$ are polynomials of $X$, then from the first equation of (3.34), we can conclude that $s_1(X)$ is a constant and $h(X) = 0$. Without loss of generality, we take $s_1(X) = 1$ and substitute it into the second and third equations of (3.34), then balance the degrees of $g(X)$ and $s_0(X)$, we can conclude that $\deg(g(X)) = 1$. Thus, we assume $g(X) = A_1X + A_0$, where $A_1\neq0, A_1$ and $A_0$ are undetermined constants, then from the second equation of (3.34), we have:

where $B$ is an integral constant. We substitute $s_0(X), s_1(X)$ and $g(X)$ into the third equation of (3.34) and equate the coefficient of each power of $X$ to zero that we obtain a system of equations made up of $a, \; A_1, \; A_0, \; B, \; c, \; k_3$ and $k_4.$ By solving this system, we obtain two groups of solutions as follows:

and

where $a, \; c$ and $B$ are arbitrary constants. Substitute (3.36) and (3.37) into (3.32) and consider $Y = X'$, we derive two first-order ordinary differential equations for $X'$ as follows:

and

Now, by solving Eqs (3.38) and (3.39) respectively and considering $X(\xi) = \phi(\xi)$, $u(x, t) = \phi (\xi)$, $v(x, t) = \varphi(\xi)$, $\xi = x+ay-ct$ and $\varphi = ac\phi-\frac{a}{2}\phi^2+k_3$, we obtain four groups of solutions for (1.1) as follows:

where $a, c$ and $B$ are arbitrary constants, $2B-c^2 > 0$ and $C_9$ is an integral constant.

where $a, c$ and $B$ are arbitrary constants, $2B-c^2 < 0$ and $C_{10}$ is an integral constant.

where $a, c$ and $B$ are arbitrary constants, $2B+c^2 < 0$ and $C_{11}$ is an integral constant.

where $a, c$ and $B$ are arbitrary constants, $2B+c^2 > 0$ and $C_{12}$ is an integral constant.

Remark 3.3 We have got four groups of solitary wave solutions for (1.1) via Feng's first integral method when $m = 1$ in (3.32).

Case 4: Suppose $m = 2$ in (3.32) and equate the coefficients of $Y^i, i = 0, 1, 2$ on both sides of (3.33), we obtain equations as follows:

Since $s_i(X), i = 0, 1, 2$ are polynomials of $X$, then from the first equation of (3.44), we can conclude that $s_2(X)$ is a constant and $h(X) = 0$. For simplicity, we take $s_2(X) = 1$ and substitute it into the second and third and forth equations of (3.44), then balance the degrees of $g(X)$ and $s_1(X)$ as well as $s_0(X)$ and $s_1(X)$, we can conclude that $\deg(g(X)) = 1$. Thus, we can assume $g(X) = A_1X + A_0$, where $A_1\neq0$, $A_1$ and $A_0$ are undetermined constants, from the second equation of (3.44), we have:

where $B$ is an integral constant. Then we substitute $s_2(X), s_1(X)$ and $g(X)$ into the third equation of (3.44), we obtain:

Now we substitute $s_0(X), s_1(X)$, $s_2(X)$ and $g(X)$ into the fourth equation of (3.44) and equate the coefficient of each power of $X$ to zero, that we can derive a system of equations made up of $a, \; A_1, \; A_0, \; B, \; B_1, \; c, \; k_3$ and $k_4$. By solving this system, we obtain two groups of solutions as follows:

and

where $a, c$ and $B$ are arbitrary constants.

Now we substitute (3.47), (3.48) into (3.45) and (3.46) respectively and obtain the expressions for $s_0(X), \; s_1(X)$ and $s_2(X)$, then plug them into (3.32) and consider $Y = X'$, that we can derive two equations for $X'$:

and

Solve (3.49) and (3.50) respectively, we obtain the following two first-order ordinary differential equations:

and

Now, resolve (3.51) and (3.52) respectively and consider $X(\xi) = \phi (\xi)$, $u(x, t) = \phi (\xi)$, $v(x, t) = \varphi(\xi)$, $\xi = x+ay-ct$ and $\varphi = ac\phi-\frac{a}{2}\phi^2+k_3$, that we obtain four groups of solutions for (1.1) as follows:

where $a, c$ and $B$ are arbitrary constants, $B-c^2 > 0$ and $C_{13}$ is an integral constant.

where $a, c$ and $B$ are arbitrary constants, $B-c^2 < 0$ and $C_{14}$ is an integral constant.

where $a, c$ and $B$ are arbitrary constants, $B+c^2 < 0$ and $C_{15}$ is an integral constant.

where $a, c$ and $B$ are arbitrary constants, $B+c^2 > 0$ and $C_{16}$ is an integral constant.

Remark 3.4 We have got four groups of solitary wave solutions for (1.1) via Feng's first integral method when $m = 2$ in (3.32). Now, all exact solutions of the (2+1)-dimensional nonlinear dispersive long wave system (1.1) have been obtained.

Now, we plot the three-dimensional graphs of the solution (3.55) and (3.56) respectively (see the following Figures 5–8). The rest of three groups of graphs for (3.40), (3.42) and (3.53) are similar to (3.55), while the rest of other three groups of graphs for (3.41), (3.43) and (3.54) are similar to (3.56). We illustrate the figures as follows:

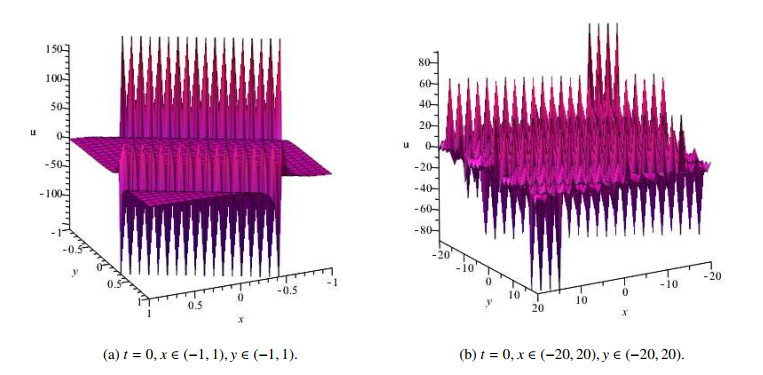
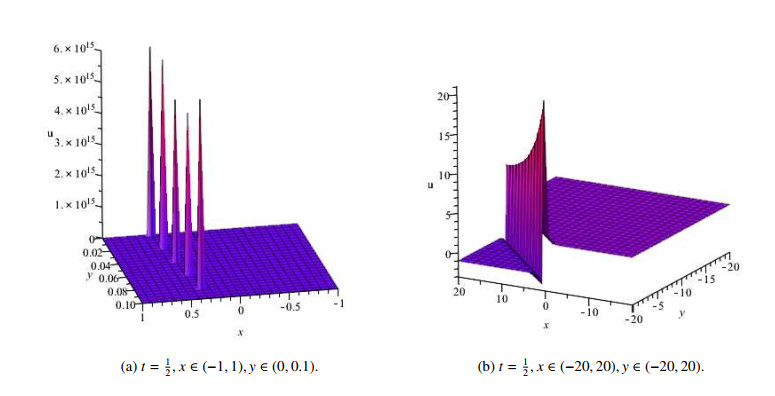
(1) Figure 5(a) displays the three-dimensional graph of the solution $u(x, y, t)$ of (3.55) with $c = 1, B = -5, a = 2, C_{15} = 0, t = 0$ and small variable intervals $x\in(-1, 1), \; y\in(-1, 1)$. Figure 5(b) shows the three-dimensional graph of the solution $u(x, y, t)$ of (3.55) with same parameters and larger variable intervals $x\in(-20, 20), \; y\in(-20, 20)$. The process of drawing and simulation shows that this is a class of solitary wave solution and the number and size of these solitons change greatly as the interval changes. (Remark: Here we let $t$ as a constant, while the case is similar to Figure 1 if we take $x$ (or $y$) as a constant and $y$ (or $x$) and $t$ are variable. The cases for Figures 6–8 are the same).

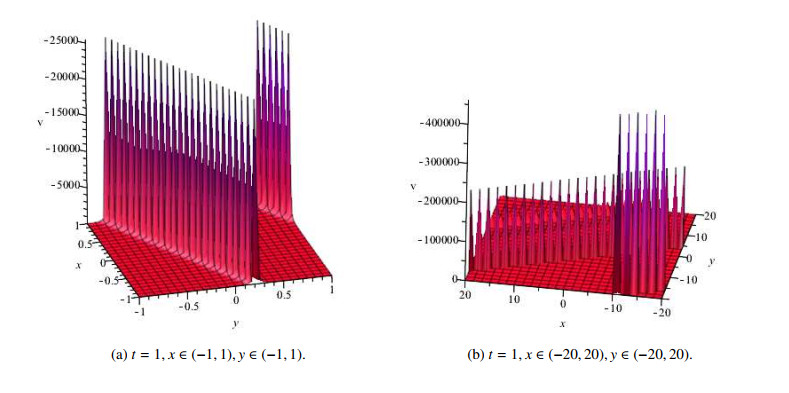
(2) Figure 6 displays the three-dimensional graph of the solution $v(x, y, t)$ of (3.55) with $c = 1, B = -5, a = 2, C_{15} = 0$ and $t = 1$. Let $x\in(-1, 1), \; y\in(-1, 1)$ in Figure 6(a), while the larger variable interval $x\in(-20, 20), \; y\in(-20, 20)$ is taken in Figure 6(b). The process of drawing and simulation shows that it is also a class of solitary wave solution and the number and size of the solitons change greatly as the interval changes.

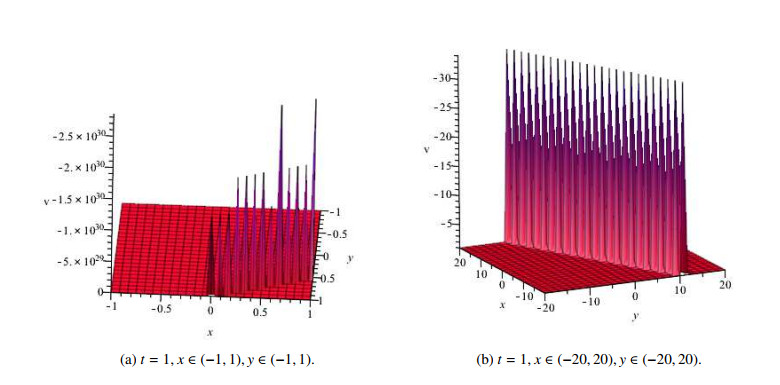
(3) Figures 7(a) and 7(b) display the three-dimensional graphs of the solution $u(x, y, t)$ of (3.56) with $c = 1, B = 3, a = 2, C_{16} = 0$ and $t = \frac{1}{2}$, while Figures 8(a) and 8(b) show the three-dimensional graphs of the solution $v(x, y, t)$ of (3.56) with $c = 2, B = 5, a = 2, C_{16} = 0$ and $t = 1$. The process of drawing and simulation shows that they are also a class of solitary wave solutions and the number and size of the solitons change greatly as the intervals change.

4.   Conclusions

In this paper, Feng's first integral method was successfully applied to solve the (1+1)-dimensional and (2+1)-dimensional nonlinear dispersive long wave systems. We gain eight groups of exact solutions for the (1+1)-dimensional and (2+1)-dimensional nonlinear dispersive long wave system respectively via Feng's first integral method. We find that there exist some similarities in the expressions of the solutions of (1+1)-dimensional and (2+1)-dimensional DLWEs but there exists some differences in their dimensions for their respective solutions. While system (1.1) and system (1.2) have different physical significance due to their dimensions. We plot their figures typically to illustrate the features of the results. The process of drawing and simulation shows that the solutions of system (1.1) and (1.2) all belong to solitary wave solutions except that we take a minimum interval that it belongs to a distorted wave solution for the example Figure 2(a). The number and size of their solitons change greatly as the intervals change. There exist some problems that subject to be resolved in this paper, for example, if we take $m = 3$ or larger number in (3.1) and (3.32), do the system (1.1) and (1.2) exist solutions or can we find out? This can be the direction of our future work.

Acknowledgments

This paper was supported by Natural Science Foundation of Fujian Province under Grant (No. 2017J01769), Science and Technology Project of Education Department of Fujian Province (No. JA15512), and Senior talent introduction program (No. YJ201802). We give thanks to Professor Y. H. Xia also his series of papers ^{[11,12,13,14]} which gave a lot of enlightenments for this paper.

Conflict of interest

The authors declare that there is no conflict of interest regarding publication of this paper.