Solutions to (3)–(7)–(8) in the
In this paper, we study the following kind of Schrödinger-Poisson system in R2
{−Δu+V(x)u+ϕu=K(x)f(u), x∈R2,Δϕ=u2, x∈R2,
where f∈C(R,R), V(x) and K(x) are both axially symmetric functions. By constructing a new variational framework and using some new analytic techniques, we obtain an axially symmetric solution for the above planar system. Our result improves and extends the existing works.
Citation: Qiongfen Zhang, Kai Chen, Shuqin Liu, Jinmei Fan. Existence of axially symmetric solutions for a kind of planar Schrödinger-Poisson system[J]. AIMS Mathematics, 2021, 6(7): 7833-7844. doi: 10.3934/math.2021455
| [1] | Qi Luo, Ryan Weightman, Sean T. McQuade, Mateo Díaz, Emmanuel Trélat, William Barbour, Dan Work, Samitha Samaranayake, Benedetto Piccoli . Optimization of vaccination for COVID-19 in the midst of a pandemic. Networks and Heterogeneous Media, 2022, 17(3): 443-466. doi: 10.3934/nhm.2022016 |
| [2] | Prateek Kunwar, Oleksandr Markovichenko, Monique Chyba, Yuriy Mileyko, Alice Koniges, Thomas Lee . A study of computational and conceptual complexities of compartment and agent based models. Networks and Heterogeneous Media, 2022, 17(3): 359-384. doi: 10.3934/nhm.2022011 |
| [3] | Xia Li, Chuntian Wang, Hao Li, Andrea L. Bertozzi . A martingale formulation for stochastic compartmental susceptible-infected-recovered (SIR) models to analyze finite size effects in COVID-19 case studies. Networks and Heterogeneous Media, 2022, 17(3): 311-331. doi: 10.3934/nhm.2022009 |
| [4] | Eiman, Kamal Shah, Muhammad Sarwar, Thabet Abdeljawad . On rotavirus infectious disease model using piecewise modified ABC fractional order derivative. Networks and Heterogeneous Media, 2024, 19(1): 214-234. doi: 10.3934/nhm.2024010 |
| [5] | Ryan Weightman, Temitope Akinode, Benedetto Piccoli . Optimal control of pandemics via a sociodemographic model of non-pharmaceutical interventions. Networks and Heterogeneous Media, 2024, 19(2): 500-525. doi: 10.3934/nhm.2024022 |
| [6] | Guillaume Cantin, Cristiana J. Silva, Arnaud Banos . Mathematical analysis of a hybrid model: Impacts of individual behaviors on the spreading of an epidemic. Networks and Heterogeneous Media, 2022, 17(3): 333-357. doi: 10.3934/nhm.2022010 |
| [7] | Xavier Blanc, Claude Le Bris, Frédéric Legoll, Tony Lelièvre . Beyond multiscale and multiphysics: Multimaths for model coupling. Networks and Heterogeneous Media, 2010, 5(3): 423-460. doi: 10.3934/nhm.2010.5.423 |
| [8] | Richard Carney, Monique Chyba, Taylor Klotz . Using hybrid automata to model mitigation of global disease spread via travel restriction. Networks and Heterogeneous Media, 2024, 19(1): 324-354. doi: 10.3934/nhm.2024015 |
| [9] | Yimamu Maimaiti, Zunyou Lv, Ahmadjan Muhammadhaji, Wang Zhang . Analyzing vegetation pattern formation through a time-ordered fractional vegetation-sand model: A spatiotemporal dynamic approach. Networks and Heterogeneous Media, 2024, 19(3): 1286-1308. doi: 10.3934/nhm.2024055 |
| [10] | Xia Li, Andrea L. Bertozzi, P. Jeffrey Brantingham, Yevgeniy Vorobeychik . Optimal policy for control of epidemics with constrained time intervals and region-based interactions. Networks and Heterogeneous Media, 2024, 19(2): 867-886. doi: 10.3934/nhm.2024039 |
In this paper, we study the following kind of Schrödinger-Poisson system in R2
{−Δu+V(x)u+ϕu=K(x)f(u), x∈R2,Δϕ=u2, x∈R2,
where f∈C(R,R), V(x) and K(x) are both axially symmetric functions. By constructing a new variational framework and using some new analytic techniques, we obtain an axially symmetric solution for the above planar system. Our result improves and extends the existing works.
In this paper we propose an epidemiological compartmental model where the efficacy time of vaccinations, i.e., the time a dosed individual needs to become immune, plays a key role. Furthermore, we also account for the concurrent effect of different vaccines, differing, for instance, in the time they need to provide immunization. The role of age can also be accounted for, letting vaccines' efficacy and immunization times depend on age, as well as on the choice of the vaccine type. These features are well known to be relevant in the present Covid-19 pandemic.
Compartmental models are a formidable tool in the description of a variety of real situations. The techniques above suggest a general framework able to introduce a specific dynamic evolution within compartments. In these models, each individual is considered to be of one compartment at any given time, its evolution consisting in passing from one compartment to another one, based on the structure and on the parameters of the model. In other words, the global dynamics consist in individuals entering the system (e.g., newborns), others leaving the system (e.g., casualties) and, during the evolution, passing from one compartment to another (e.g., getting ill, being vaccinated, recovering,
In the context of epidemiological models describing the spread of infectious diseases, the SIR model, named after its three compartments (Susceptible, Infected and Recovered), is the traditional prototype. This epidemic model dates back to 1927, see [16], less than a decade after the 1918 influenza pandemic. In what is probably its simplest form [22,§10.2], a prototype SIR model reads
| S→I→R↓{˙S=−ρIS˙I=ρIS−(ϑ+μ)I˙R=ϑI | (1) |
where
The popularity of (1) is due partly to its simplicity, which allows to describe the disease behavior by estimating a small number of parameters, and partly to its being amenable to a variety of extensions. The dynamics of the SIR epidemic model are widely considered, here we recall for instance [1,4,21,25], or [14,Chapter 6], [22,Chapter 10], and [23,§1.5.1].
The literature on SIR-type models, typically containing additional compartments, is indeed huge. For instance, SEIR and SEIRS-type models contain also the compartment E (Exposed) where infected individuals spend a latent/incubation period prior to become infective, see [12,13,18,19] and the references therein. Vaccination campaigns and effects are described in SVIR-type models which consider individuals that get vaccinated, see [9,17,20] or also the different approach in [5].
Recall also, for instance, the SIHR model proposed in [6], where infected individuals are either Infective (I) or Hospitalized (H), the former ones spreading the disease, while the latter ones being isolated, typically hospitalized or in quarantine, thus taking into account lockdown effects.
Since 2020, with the Covid-19 pandemic, the development and use of these models boomed. The need to explicitly introduce the immunization time of a vaccine and the concurrent use of different vaccines lead us to introduce a dynamics within compartments. This machinery allows to fix a priori the time an individual spends in a compartment, as in the case of the immunization time of a vaccine, see §2, allowing also this time to be age dependent, as in §3. This general framework is sufficiently flexible to account for the simultaneous adoption of different vaccines, as in model (4).
In other instances, it might be appropriate to smoothen the change of status related to the change of compartment, an example being the SEIR model as modified in §4. Indeed, pass from (15) to (16) allows to account for a somewhat continuous evolution from exposed to infective. In all these examples, key statistics, such as the basic reproduction number, are naturally extended to these new frameworks keeping their original meaning.
Worth mentioning is the recent SIDARTHE model from [11], consisting of
In §6, the SIHR model is modified to comprehend spatial (geographic) movements. The introduction of the age structure, where advisable, is in general possible, as shown in §7. These two steps, namely the introduction of space and age structures, are indeed doable in all the examples discussed.
Remark that the present framework indeed provides an extension to the current compartmental modeling habits. In fact, suitable choices of various functions or parameters allow to trivialize intra–compartmental dynamics, thus recovering known models.
Pandemic dynamics can clearly be described through many other tools. For instance, the recent work [2] proposes a multiscale approach and discusses the current kinetic literature on the subject. Cellular automata are used for instance in [10] in a probabilistic setting, still with a compartmental structure, see [10,Figure 1 (b)]. The literature also offers essays correlating pandemics to other human activities, primarily to economy, see for instance [8].
In the effective application of the models discussed below, a key issue is parameters' estimation. We defer for instance to [24] and to [27] for two entirely different approaches.
From the analytic point of view, a rigorous abstract approach to these classes of models is possible but at the cost of a quite intricate PDE based formalism, see a first attempt in [7]. Therefore, the sequel is devoted to show that intra-compartmental dynamics can both refine usual models, and also take into account new features, not captured by standard compartmental models.
The model presented in [20], here slightly modified, amounts to this extension of (1):
| V↗↓↘S→I→R↓{˙S=−ρSIS−p(t,S)˙V=p(t,S)−ρVIV−ϑVV˙I=(ρSS+ρVV)I−(ϑI+μ)I˙R=ϑII+ϑVV. | (2) |
Here,
Before introducing the immunization time in (2), we remark that setting
The effect of doses, as is well known, is not immediate. On the contrary, the time
| V(0)→V(T∗)↗↓↘S→I→R↓{˙S=−ρSIS−p(t,S)∂tV+∂τV=−ρVIV˙I=(ρSS+∫T∗0ρVV)I−ϑI−μI˙R=ϑI+V(t,T∗)V(t,0)=p(t,S(t)). | (3) |
Here, the number of individuals at time
In connection with the present Covid-19 pandemic, we remark that model (3) can be easily extended to the case of different, say
| Vℓ(0)→Vℓ(Tℓ∗)ℓ=1,…,m↗↓↘S→I→R↓{˙S=−ρSIS−m∑ℓ=1pℓ(t,S)∂tVℓ+∂τVℓ=−ρVℓIVℓ˙I=(ρSS+m∑ℓ=1∫Tℓ∗0ρVℓVℓ)I−(ϑ+μ)I˙R=ϑI+m∑ℓ=1Vℓ(t,Tℓ∗)Vℓ(t,0)=pℓ(t,S(t)). | (4) |
Note that, as is to be expected, according to (4) the total number of individuals is affected only by mortality, in the sense that
| ddt(S(t)+m∑ℓ=1∫Tℓ∗0Vℓ(t,τ)dτ+I(t)+R(t))=−μI(t). |
A parameter often used to describe the tendency of the pandemic is the (time dependent) basic reproduction number
| Ro(t)=ρSS(t)+m∑ℓ=1∫Tℓ∗0ρVℓ(τ)Vℓ(t,τ)dτϑ+μ, | (5) |
so that the increasing in the infected individuals at time
| ddtI(t)≷0⟺Ro(t)≷1. | (6) |
The above equivalence holds also in the case of the parameters
At first sight, the numerator in the right hand side of (5) is misleading, for it apparently implies that higher values of
A direct comparison between (2) and (3), though suggestive, is inevitably highly arbitrary. Clearly,
Thus, below, to investigate the role of
1
| ρS=5×10−4ϑ=1×10−3μ=1×10−4ρV(τ)=ρS√1−τT∗p(t,S)=0.5χ[10,+∞[(t)χR+(S) | (7) |
and initial data
| So=99.9,Vo(τ)≡0,Io=0.1,Ro=0. | (8) |
We consider
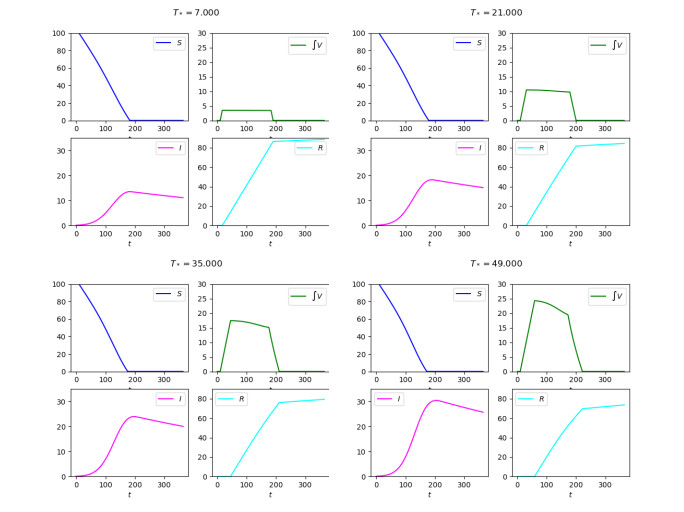
Times necessary for the vaccination to provide immunity and corresponding casualties according to model (3)–(7)–(8). The initial total population is
| 1 | 7 | 14 | 21 | 28 | 35 | 42 | 49 | |
| Deaths: | 0.28 | 0.32 | 0.37 | 0.43 | 0.49 | 0.56 | 0.63 | 0.70 |
The relevant differences in the diagram of the map
In Figure 1, the differences in the
The evolutions in the
The flexibility introduced by dynamics internal to the dosed (
| p(t,S)=0.5χ[10,30]∪[120,+∞[(t)χR+(S). | (9) |
Then, the number of casualties sharply grows with respect to the number of casualties in (3)–(7)–(8), as clearly shown when comparing Table 1 with Table 2.
Times necessary for the vaccination to provide immunity and corresponding casualties, according to model (3)–(7)–(8), in the case vaccinations are suspended as detailed in (9). The initial total population is
| 1 | 7 | 14 | 21 | 28 | 35 | 42 | 49 | |
| Deaths: | 1.11 | 1.18 | 1.25 | 1.32 | 1.38 | 1.43 | 1.48 | 1.53 |
The diagrams in Figure 2 also confirm the negative effect in a suspension of the vaccination campaign.
As a further example, we consider the problem of choosing between two different vaccines. We integrate (4) with
| T1∗=7ρV1(τ)=ρS√1−τT1∗p1(t,S)=ωχ[20,+∞[(t)χR+(S)T2∗=35ρV2(τ)=0.2ρS√1−τT2∗p2(t,S)=(0.5−ω)χ[20,+∞[(t)χR+(S), | (10) |
with the parameter
The insertion of an intra–compartmental dynamics is not limited to ODE models. As it is well known, age differences often have a role in the spreading of diseases. Therefore, we introduce the following extension of (3):
| {∂tS+∂aS=−∫R+ρ(t,a,α)I(t,α)dαS−p(t,a,S)∂tV+∂aV+∂τV=−ρVIV∂tI+∂aI=∫R+ρ(t,a,α)I(t,α)dαS+∫R+∫T∗0ρV(t,a,τ,α)V(t,α,τ)dαdτI−ϑI−μI∂tR+∂aR=ϑI+V(t,a,T∗), | (11) |
Vaccination entering also as boundary datum in the evolution of the
| V(t,a,0)=p(t,a,S(t,a)). | (12) |
In (11)–(12), we use the same symbols as in (3), allowing the various parameters to depend also on age. For instance
System (11) needs to be supplied with initial and boundary data, such as
| {S(0,a)=So(a)V(0,a,τ)=Vo(a,τ)I(0,a)=Io(a)R(0,a)=Ro(a){S(t,0)=Sb(t)V(t,0,τ)=0I(t,0)=0R(t,0)=0 | (13) |
where, as it is realistic, we set to
Note that setting all data and parameters in (11)–(12)–(13) constant in
Extending the analogous property valid for system (3), note that the total number of individuals varies not only due to mortality but also due to the natality
| ddt∫R+(S(t,a)+∫T∗0V(t,a,τ)dτ+I(t,a)+R(t,a))da=−∫R+μ(t,a)I(t,a)da+Sb(t). |
The present model realistically allows infection to be propagated across different ages. Therefore, we can only have an index
| Ro(t)=∫R+∫R+(ρ(t,α,a)S(t,α)+∫T∗0ρV(t,a,τ,α)V(t,α,τ)dτ)dαI(t,a)da∫R+(ϑ(t,a)+μ(t,a))I(t,a)da |
we have the following extension of (6):
| ddt∫R+I(t,a)da≷0⟺Ro(t)≷1. | (14) |
Remark that also model (11) can easily be extended to the case of different vaccines having different full efficacy times through the introduction of different vaccinated populations
The use of model (11)–(12)–(13) allows to tackle the key issue of optimizing the use of the available vaccines on the basis of their different efficacy on different age classes.
The description provided by (1) is often too approximate. For instance, it might be necessary to distinguish between exposed (
| S→E→I→R↓↓{˙S=−ρIS˙E=ρIS−(ϑE+μE+κ)E˙I=κE−(ϑI+μI)I˙R=ϑEE+ϑII, | (15) |
where we introduced the term
Assume now that individuals infected at time
| S→I(0)→I(τ)→→R↓{˙S=−∫R+ρ(τ)I(t,τ)dτS∂tI+∂τI=−ϑ(τ)I−μ(τ)I˙R=∫ϑ(τ)I(t,τ)dτI(t,0)=∫R+ρ(τ)I(t,τ)dτS(t). | (16) |
Observe that the last line above is in fact a boundary condition, prescribing how many individuals get infected at time
Remark that the dependence of
Observe that, as is to be expected, the variation in the total number of individuals resulting from (16) is due exclusively to mortality. Indeed
| ddt(S(t)+∫R+I(t,τ)dτ+R(t))=−∫R+μ(τ)I(t,τ)dτ. | (17) |
Within the framework of (16), an explicit expression for the basic reproduction number
| Ro(t)=∫R+ρ(τ)I(t,τ)dτS(t)∫R+(ϑ(τ)+μ(τ))I(t,τ)dτ |
and it is immediate to see that its exceeding unity is equivalent to the increase of the total number of infected individuals:
| ddt(∫R+I(t,τ)dτ)≷0⟺Ro(t)≷1. | (18) |
It goes without saying that suitable choices of initial data and parameters in (16) allow to recover within (16) the solutions of (1). Here we only provide a quick example of a comparison among solutions to (1), (15) and (16), with data and parameters in (1) being an average of those in (15), while the choices in (16) are an interpolation of those in (15), as detailed in (19).
| (1)(15)(16)ρ=0.1ρ=0.1ρ(τ)=0.1(1−e−0.2τ)ϑ=3(ϑE,ϑI)=(7,1)ϑ(τ)={7τ<21τ≥2μ=0.5(μE,μI)=(0.1,0.9)μ(τ)=0.9−0.8e−2τκ=5So=10So=10So=10Io=6.(Eo,Io)=(1,5)Io(τ)={0.5τ∈[0,2[2.5τ∈[2,4]Ro=0Ro=0Ro=0. | (19) |
Figure 4 shows sample integrations of (1), (15) and (16). In the situation considered, according to mortality, the mixed ODE–PDE system (16) can be seen in some senses in the middle between the other two classical purely ODE based compartmental models (1) and (15). Intra-compartmental dynamics can thus recover the dynamics described through standard compartmental models.

Above, the integrations of (1) and (15), below on the left that of (16) (19). The rightmost diagram on the second line displays the total number of living individuals in the three cases, showing that, with respect to mortality, the ODE–PDE model (16) can be seen in some senses in the middle between the ODE models (1) and (15)
.On the other hand, other scenarios can hardly be recovered through the use of only standard compartments. Indeed, for instance, it can be reasonable to assume that infected individuals are most infective in given time intervals after infection where, in general, these intervals depend on the specific disease under consideration. Such a situation, within Model (16), is easily described and we consider, for instance, the following choices:
| ρ(τ)=ˉρte−α(t−ˉT)2 with ρ=0.1 and (i)α=1.0,ˉT=10.0,(ii)α=1.0,ˉT=4.0,(iii)α=0.25,ˉT=4.0. | (20) |
The sample choices (20) differ in the time
The results are summarized in Figure 5. The differences in the evolutions prescribed by (16) corresponding to the choices (20) are very intuitive, Case

Above, from left to right, the integrations of Case
We now consider the SIDARTHE model introduced in [11]. It consists of
Populations in model (21) from [11]
.| Susceptible | healthy | can be infected | ||
| Infected | asymptomatic | infective | undetected | |
| Diagnosed | asymptomatic | infective | detected | |
| Ailing | symptomatic | infective | undetected | |
| Recognized | symptomatic | infective | detected | |
| Threatened | acutely symptomatic | infected | detected | |
| Healed | healthy | immune | ||
| Extinct |
Its block diagram and the corresponding system of ordinary differential equations read:
| S→I→D↙↓↓↘H←A→R↘↑↘↓↘↓↑E←T→↓↑↓←←←←←←←{˙S=−αIS−γAS−βDS−δRS˙I=αIS+γAS+βDS+δRS−(ε+ζ+λ)I˙D=εI−(η+ρ)D˙A=ζI−(ϑ+μ+κ)A˙R=ηD+ϑA−(ν+ξ)R˙T=μA+νR−(σ+τ)T˙H=λI+ρD+κA+ξR+σT˙E=τT. | (21) |
We refer to [11] for the specific meaning of each constant and for the motivations of this model.
Here, we note that both the evolutions
| (22) |
Introduce now the populations
| S→I(0)→I(s)→→R(0)→R(s)→→E↘↙ ⟶H⟵ | (23) |
| {˙S=−∫α(s)I(t,s)dsS−∫δ(s)R(t,s)dsS∂tI+∂sI=∫α(s)I(t,s)dsS+∫δ(s)R(t,s)dsS−∫ε(s)I(t,s)ds−∫ϑ(s)I(t,s)ds∂tR+∂sR=∫ϑ(s)I(t,s)s−∫η(s)R(t,s)ds˙H=∫ε(s)Ids˙E=∫η(s)R(t,s)ds, | (24) |
All integrals being computed over
Note also that in model (24) the explicit expression of the basic reproduction number is immediately at hand. Indeed, defining
| Ro(t)=∫(α(s)I(t,s)+δ(s)R(t,s))dsS(t)∫(ε(s)+ϑ(s))I(t,s)ds |
the key property (18) still holds.
As a further example of intra-compartmental dynamics we consider movements. Indeed, as a prototype for the description of the role of quarantine in the spreading of a pandemic, consider the model [6,Formula (9)]
| S→I↘↓RH↗{∂tS+μSS=−ρIS∂tI+μII=ρIS−κI−ϑI∂tH+μHH=κI−ηH∂tR+μRR=ϑI+ηH, | (25) |
where we used the standard notation for the
In this connection, it can be relevant to take into consideration events that provoked relevant gatherings of crowds, a well known example being the Atalanta vs. Valencia football match played in Milan (Italy) on February 19th, 2020, see [26]. To this aim, following [6], we introduce a geographical movement as follows
| S(x)↺→I(x)↺↘↓R(x)↺H↗{∂tS+div(vSS)+μSS=−ρIS∂tI+div(vII)+μII=ρIS−κI−ϑI∂tH+μHH=κI−ηH∂tR+div(vRR)+μRR=ϑI+ηH. | (26) |
Above, all populations are also space dependent, so that, for instance,
Introducing age structure in the SIS model [15], see also [3,Formula 10.1], we get
| S⟷I{∂tS+∂aS=−ρIS+γI∂tI+∂aI=ρIS−γI. | (27) |
In this model, when infected individuals recover, they get back to being susceptible at a rate governed by the, possibly age dependent, parameter
| S⟶⟶I↖↙R(0)→R(T∗){∂tS+∂aS=−ρIS+R(t,a,T∗(a))∂tI+∂aI=ρIS−γI∂tR+∂aR+∂τR=0R(t,a,0)=γI(t,a). | (28) |
Note however that the very simple nature of the third equation allows to rewrite (28) as a system of
| {∂tS+∂aS=−ρIS+γI(t−T∗(a),a−T∗(a))∂tI+∂aI=ρIS−γI(t,a). | (29) |
The form (28) is more prone to extensions than (29), since it allows to specify various evolutions of the
From the analytic point of view, we remark that (28) provides a first minimal example of a "junction" in
| [1] |
S. Chen, X. Tang, On the planar Schrödinger-Poisson system with the axially symmetric potential, J. Differ. Equations, 268 (2020), 945–976. doi: 10.1016/j.jde.2019.08.036

|
| [2] |
R. Benguria, H. Brezis, E. Lieb, The Thomas-Fermi-von Weizsäcker theory of atoms and molecules, Comm. Math. Phys., 79 (1981), 167–180. doi: 10.1007/BF01942059

|
| [3] |
A. Paredes, D. Olivieri, M. Humberto, From optics to dark matter: A review on nonlinear Schrödinger-Poisson systems, Physica D, 403 (2020), 132301. doi: 10.1016/j.physd.2019.132301

|
| [4] | J. Chen, S. Chen, X. Tang, Ground state solutions for asymptotically periodic Schrödinger-Poisson systems in R2, Electron. J. Differ. Equations, 192 (2018), 1–18. |
| [5] | X. Tang, Non-Nehari manifold method for asymptotically periodic Schrödinger equation, Sci. China Math., 58 (2015), 715–728. |
| [6] | F. Bernini, D. Mugnai, On a logarithmic Hartree equation, Adv. Nonlinear Anal., 9 (2020), 850–865. |
| [7] |
S. Cingolani, T. Weth, On the planar Schrödinger-Poisson system, Ann. Inst. Henri Poincaré, Anal. Non Linéaire, 33 (2016), 169–197. doi: 10.1016/j.anihpc.2014.09.008

|
| [8] |
M. Du, T. Weth, Ground states and high energy solutions of the planar Schrödinger-Poisson system, Nonlinearity, 30 (2017), 3492–3515. doi: 10.1088/1361-6544/aa7eac

|
| [9] |
S. Chen, J. Shi, X. Tang, Ground state solutions of Nehari-Pohozaev type for the planar Schrödinger-Poisson system with general nonlinearity, Discrete Contin. Dyn. Syst., Ser. A, 39 (2019), 5867–5889. doi: 10.3934/dcds.2019257

|
| [10] | S. Chen, X. Tang, Existence of ground state solutions for the planar axially symmetric Schrödinger-Poisson system, Discrete Contin. Dyn. Syst., Ser. B, 24 (2019), 4685–4702. |
| [11] |
L. Wen, S. Chen, V. D. Rădulescu, Axially symmetric solutions of Schrödinger-Poisson system with zero mass potential in R2, Appl. Math. Lett., 104 (2020), 106244. doi: 10.1016/j.aml.2020.106244

|
| [12] |
Z. Chen, W. Zou, Ground states for a system of Schrödinger equations with critical exponent, J. Func. Anal., 262 (2012), 3091–3107. doi: 10.1016/j.jfa.2012.01.001

|
| [13] | S. Chen, X. Tang, Berestycki-Lions conditions on ground state solutions for a nonlinear Schrödinger equation with variable potentials, Adv. Nonlinear Anal., 9 (2020), 496–515. |
| [14] |
J. Peng, S. Chen, X. Tang, Semiclassical solutions for linearly coupled Schrödinger equations without compactness, Complex Var. Elliptic Equations, 64 (2019), 548–556. doi: 10.1080/17476933.2018.1450395

|
| [15] |
X. Tang, New super-quadratic conditions for asymptotically periodic Schrödinger equations, Can. Math. Bull., 60 (2017), 422–435. doi: 10.4153/CMB-2016-090-2

|
| [16] |
X. Tang, X. Lin, J. Yu, Nontrivial solutions for Schrödinger equation with local super-quadratic conditions, J. Dyn. Differ. Equations, 31 (2019), 369–383. doi: 10.1007/s10884-018-9662-2

|
| [17] | G. Che, H. Chen, Existence of multiple nontrivial solutions for a class of quasilinear Schrödinger equations on RN, Bull. Belg. Math. Soc. Simon Stevin, 25 (2018), 39–53. |
| [18] |
X. Tang, Non-Nehari manifold method for asymptotically linear Schrödinger equation, J. Aust. Math. Soc., 98 (2015), 104–116. doi: 10.1017/S144678871400041X

|
| [19] | B. Sirakov, Standing wave solutions of the nonlinear Schrödinger equations in RN, Ann. Mat. Pura Appl., 183 (2002), 73–83. |
| [20] | J. Fan, Y. Jiang, Q. Zhang, Semiclassical Solutions for a Kind of Coupled Schrödinger Equations, Adv. Math. Phys., 2020 (2020), 4378691. |
| [21] |
X. Zhang, Existence and multiplicity of solutions for a class of elliptic boundary value problems, J. Math. Anal. Appl., 410 (2014), 213–226. doi: 10.1016/j.jmaa.2013.08.001

|
| [22] |
Q. Zhang, C. Gan, T. Xiao, Z. Jia, Some results of nontrivial solutions for Klein-Gordon-Maxwell systems with local super-quadratic conditions, J. Geom. Anal., 31 (2021), 5372–5394. doi: 10.1007/s12220-020-00483-2

|
| [23] | L. Wang, X. Zhang, H. Fang, Multiplicity of solutions for a class of quasilinear elliptic systems in Orlicz-Sobolev spaces, Taiwan. J. Math., 21 (2017), 881–912. |
| [24] | Q. Zhang, C. Gan, T. Xiao, Z. Jia, An improved result for Klein-Gordon-Maxwell systems with steep potential well, Math. Methods Appl. Sci., 2020 (2020). |
| [25] | E. Lieb, M. Loss, Analysis, 2nd Ed., Graduate Studies in Mathematics, Vol. 14, American Mathematical Society, Providence, RI, 2001. |
| 1. | Rinaldo M. Colombo, Mauro Garavello, Infectious Disease Spreading Fought by Multiple Vaccines Having a Prescribed Time Effect, 2023, 71, 0001-5342, 10.1007/s10441-022-09452-4 | |
| 2. | Lihong Sun, Qiang He, Yueyang Teng, Qi Zhao, Xin Yan, Xingwei Wang, A complex network-based vaccination strategy for infectious diseases, 2023, 136, 15684946, 110081, 10.1016/j.asoc.2023.110081 | |
| 3. | R.M. Colombo, M. Garavello, M. Tandy, On the coupling of well posed differential models, 2023, 232, 0362546X, 113290, 10.1016/j.na.2023.113290 | |
| 4. | Rinaldo M. Colombo, Mauro Garavello, Francesca Marcellini, Elena Rossi, 2024, Chapter 28, 978-3-031-55263-2, 325, 10.1007/978-3-031-55264-9_28 |
Times necessary for the vaccination to provide immunity and corresponding casualties according to model (3)–(7)–(8). The initial total population is
| 1 | 7 | 14 | 21 | 28 | 35 | 42 | 49 | |
| Deaths: | 0.28 | 0.32 | 0.37 | 0.43 | 0.49 | 0.56 | 0.63 | 0.70 |
Times necessary for the vaccination to provide immunity and corresponding casualties, according to model (3)–(7)–(8), in the case vaccinations are suspended as detailed in (9). The initial total population is
| 1 | 7 | 14 | 21 | 28 | 35 | 42 | 49 | |
| Deaths: | 1.11 | 1.18 | 1.25 | 1.32 | 1.38 | 1.43 | 1.48 | 1.53 |
Populations in model (21) from [11]
.| Susceptible | healthy | can be infected | ||
| Infected | asymptomatic | infective | undetected | |
| Diagnosed | asymptomatic | infective | detected | |
| Ailing | symptomatic | infective | undetected | |
| Recognized | symptomatic | infective | detected | |
| Threatened | acutely symptomatic | infected | detected | |
| Healed | healthy | immune | ||
| Extinct |
| 1 | 7 | 14 | 21 | 28 | 35 | 42 | 49 | |
| Deaths: | 0.28 | 0.32 | 0.37 | 0.43 | 0.49 | 0.56 | 0.63 | 0.70 |
| 1 | 7 | 14 | 21 | 28 | 35 | 42 | 49 | |
| Deaths: | 1.11 | 1.18 | 1.25 | 1.32 | 1.38 | 1.43 | 1.48 | 1.53 |
| Susceptible | healthy | can be infected | ||
| Infected | asymptomatic | infective | undetected | |
| Diagnosed | asymptomatic | infective | detected | |
| Ailing | symptomatic | infective | undetected | |
| Recognized | symptomatic | infective | detected | |
| Threatened | acutely symptomatic | infected | detected | |
| Healed | healthy | immune | ||
| Extinct |