1.
Introduction
Modern complex dynamical systems can give rise to systems which have nonsmooth dynamics wherein the discontinuities in the system dynamics are intentionally introduced as part of the controller architecture to achieve hierarchical system stabilization [12,13,14,18,19]. In particular, the use of hierarchical embedded control subsystems within the feedback control system that possess a multiechelon hierarchical hybrid control architecture characterized by continuous-time dynamics at the lower levels of the hierarchy and discrete-time dynamics at the higher levels of the hierarchy. The lower-level units directly interact with the dynamical system to be controlled while the higher-level units receive information from the lower-level units as inputs and provide (possibly discrete) output commands, which serve to coordinate and reconcile the (sometimes competing) actions of the lower-level units [13,18]. The mathematical descriptions of many of these controlled dynamical systems can be characterized by hybrid systems [8,9,13] and impulsive differential equations [2,3,4,5,13,21,23,31,32].
Impulsive dynamical systems can be viewed as a subclass of hybrid systems and consist of three elements—namely, a continuous-time differential equation, which governs the motion of the dynamical system between impulsive or resetting events; a difference equation, which governs the way the system states are instantaneously changed when a resetting event occurs; and a criterion for determining when the states of the system are to be reset. Unlike continuous-time and discrete-time dynamical systems, which require specific system dynamics structures (e.g., non-Lipschitzian vector fields [6] and nilpotent system matrices [15]) to hold for achieving finite convergence, a unique feature of impulsive dynamical systems is that it may be possible to reset the system states to an equilibrium state achieving finite-time convergence without requiring non-Lipschitzian system dynamics.
Finite-time convergence to a Lyapunov stable equilibrium, that is, finite time stability, was first addressed by Roxin [30] and rigorously studied in [6,7] for continuous-time, time-invariant systems with continuous but non-Lipschitzian dynamics using continuous Lyapunov functions. Extensions of finite-time stability to continuous-time, time-varying nonlinear dynamical systems are presented in [17,27], whereas extensions of finite-time stability to discrete-time autonomous dynamical systems were recently reported in [15,16]. With the notable exception of [29], finite time stability of impulsive dynamical systems has not been studied in the literature.
In this paper, we develop sufficient conditions for finite time stability of nonlinear impulsive dynamical systems. Specifically, we merge the results of [6,15,16,29] to develop new sufficient Lyapunov conditions for finite time stability of impulsive dynamical systems involving a scalar differential inequality on the Lyapunov function for the continuous-time dynamics as well as a scalar difference inequality and a minimum operator on the Lyapunov function for the discrete-time resetting dynamics. Furthermore, using our proposed finite time stability results, we present a finite time hybrid stabilization framework, that is, the problem of finding universal state feedback hybrid control laws that guarantee finite-time stability of the closed-loop system, for impulsive dynamical systems. Finally, to show the utility of the proposed hybrid stabilization framework we apply our results on several numerical examples involving network impulsive dynamical systems.
2.
Mathematical preliminaries
In this section, we establish notation and definitions needed for developing the main results of this paper. Let R denote the set of real numbers, R+ denote the set of positive real numbers, ¯R+ denote the set of nonnegative numbers, Rn denote the set of n×1 real column vectors, Rn×m denote the set of n×m real matrices, Z denote the set of integers, Z+ denote the set of positive integers, ¯Z+ denote the set of nonnegative integers, (⋅)T denote transpose, and (⋅)† denote the Moore-Penrose generalized inverse. We write Bε(x) for the open ball centered at x with radius ε, ‖⋅‖ for the Euclidean vector norm in Rn, V′(x) for the Fréchet derivative of V at x, and ΔV(x)≜V(f(x))−V(x) for the difference operator of V:Rn→R at x for a given f(x). Furthermore, we write ¯S and ∂S to denote the closure and boundary of the set S⊂Rn, respectively.
Consider the nonlinear state-dependent impulsive dynamical system G given by
where, for every t≥0, x(t)∈D⊆Rn, D is an open set with 0∈D, Δx(t)≜x(t+)−x(t), where x(t+)≜x(t)+fd(x(t))=lim, f_{{\rm{c}}}: \mathcal{D} \to \mathbb{R}^n is continuous and satisfies f_{{\rm{c}}}(0) = 0 , f_{{\rm{d}}}:\mathcal{D}\to \mathbb{R}^n is continuous, and \mathcal{Z} \subset \mathcal{D} is the resetting set. Note that x_{\rm{e}}\in\mathcal{D} is an equilibrium point of (2.1) and (2.2) if and only if f_{\rm{c}}(x_{\rm{e}}) = 0 and f_{\rm{d}}(x_{\rm{e}}) = 0 . We refer to the differential equation (2.1) as the continuous-time dynamics, and we refer to the difference equation (2.2) as the resetting law.
A function x:\mathcal{I}_{x_{0}}\to\mathcal{D} is a solution to the impulsive dynamical system (2.1) and (2.2) on the interval \mathcal{I}_{x_{0}}\subseteq\mathbb{R} with initial condition x(0) = x_{0} , where \mathcal{I}_{x_{0}} denotes the maximal interval of existence of a solution to (2.1) and (2.2), if x(\cdot) is left-continuous and x(t) satisfies (2.1) and (2.2) for all t\in\mathcal{I}_{x_{0}} . For further discussion on solutions to impulsive differential equations, see [4,13,21,26,31].
For a particular trajectory x(t) , we let t_{k}\triangleq\tau_{k}(x_{0}) denote the k th instant of time at which x(t) intersects \mathcal{Z} , and we call the times t_{k} the resetting times. Thus, the trajectory of (2.1) and (2.2) from the initial condition x(0) = x_{0} is given by \psi(t, x_{0}) for 0 < t\leq t_{1} , where \psi(\cdot, \cdot) denotes the solution to the continuous-time dynamics (2.1). If the trajectory reaches a state x_{1}\triangleq x(t_{1}) satisfying x_{1}\in\mathcal{Z} , then the state is instantaneously transferred to x_{1}^{+}\triangleq x_{1}+f_{\rm{d}}(x_{1}) according to the resetting law (2.2). The trajectory x(t) , t_{1} < t\leq t_{2} , is then given by \psi(t-t_{1}, x_{1}^{+}) , and so on. Our convention here is that the solution x(t) of (2.1) and (2.2) is left continuous, that is, it is continuous everywhere except at the resetting times t_{k} , and x_{k}\triangleq x(t_{k}) = \lim_{\varepsilon\rightarrow 0^{+}} x(t_{k}-\varepsilon) and x_{k}^{+}\triangleq x(t_{k})+f_{\rm{d}}(x(t_{k})) = \lim_{\varepsilon \rightarrow 0^{+}}x(t_{k}+\varepsilon) for k = 1, 2, \ldots .
To ensure well-posedness of the solutions to (2.1) and (2.2), we make the following additional assumptions [13].
Assumption 2.1. If x\in\overline{\mathcal{Z}}\backslash\mathcal{Z} , then there exists \varepsilon > 0 such that, for all 0 < \delta < \varepsilon , \psi(\delta, x)\notin\mathcal{Z} .
Assumption 2.2. If x\in\mathcal{Z} , then x+f_{\rm{d}}(x)\notin\mathcal{Z} .
Assumption 2.1 ensures that if a trajectory reaches the closure of \mathcal{Z} at a point that does not belong to \mathcal{Z} , then the trajectory must be directed away from \mathcal{Z} ; that is, a trajectory cannot enter \mathcal{Z} through a point that belongs to the closure of \mathcal{Z} but not to \mathcal{Z} . Furthermore, Assumption 2.2 ensures that when a trajectory intersects the resetting set \mathcal{Z} , it instantaneously exits \mathcal{Z} . Finally, we note that if x_{0}\in\mathcal{Z} , then the system initially resets to x_{0}^{+} = x_{0}+f_{\rm{d}}(x_{0})\not\in\mathcal{Z} , which serves as the initial condition for the continuous-time dynamics (2.1).
It follows from Assumptions 2.1 and 2.2 that for a particular initial condition, the resetting times t_{k} = \tau_{k}(x_{0}) are distinct and well defined [13]. Since the resetting set \mathcal{Z} is a subset of the state space and is independent of time, impulsive dynamical systems of the form (2.1) and (2.2) are time-invariant systems. These systems are called state-dependent impulsive dynamical systems [13]. Since the resetting times are well defined and distinct, and since the solution to (2.1) exists, it follows that the solution of the impulsive dynamical system (2.1) and (2.2) also exists over a forward time interval. For details on the existence and uniqueness of solutions of impulsive dynamical systems in forward time see [4,21,31].
It is important to note that the analysis of impulsive dynamical systems can be quite involved. In particular, such systems can exhibit Zenoness and beating, as well as confluence, wherein solutions exhibit infinitely many resettings in a finite time, encounter the same resetting surface a finite or infinite number of times in zero time, and coincide after a certain point in time. In this paper, we allow for the possibility of confluence and Zeno solutions; however, Assumption 2.2 precludes the possibility of beating. Furthermore, since not every bounded solution of an impulsive dynamical system over a forward time interval can be extended to infinity due to Zeno solutions, we assume that existence and uniqueness of solutions are satisfied in forward time. For details see [2,4,21].
Remark 2.1. Let x^{*}\in\mathcal{D} satisfy f_{\rm{d}}(x^{*}) = 0 . Then x^{*}\notin\mathcal{Z} . To see this, suppose x^{*}\in\mathcal{Z} . Then x^{*}+f_{\rm{d}}(x^{*}) = x^{*}\in\mathcal{Z} , which contradicts the assumption that if x\in\mathcal{Z} , then x+f_{\rm{d}}(x)\not\in \mathcal{Z} . Hence, if x = 0 is an equilibrium point of (2.1) and (2.2), then 0\notin\mathcal{Z} . Hence, if x = x^* is an equilibrium point of (2.1) and (2.2), then x^* \not\in \mathcal{Z} .
3.
Finite time stability of impulsive dynamical systems
In this section, we present sufficient conditions for finite time stability of the nonlinear impulsive dynamical systems. The following definition introduces the notion of finite time stability for impulsive dynamical systems. For this definition, we denote the trajectory or solution curve of (2.1) and (2.2) satisfying x(0) = x by s(\cdot, x) or s^{x}(\cdot) .
Definition 3.1. Consider the nonlinear impulsive dynamical system \mathcal{G} given by (2.1) and (2.2). The zero solution x(t)\equiv 0 to (2.1) and (2.2) is finite time stable if there exist an open neighborhood \mathcal{N}\subseteq\mathcal{D} of the origin and a function T:\mathcal{N}\backslash\{0\}\rightarrow(0, \infty) , called the settling-time function, such that the following statements hold:
i) Finite time convergence. For every x\in\mathcal{N}\backslash\{0\} , s^{x}(t) is defined on [0, T(x)) , s^{x}(t)\in\mathcal{N}\backslash\{0\} for all t\in[0, T(x)) , and \lim_{t\rightarrow T^+(x)}s(t, x) = 0 .
ii) Lyapunov stability. For every \varepsilon > 0 there exists \delta > 0 such that \mathcal{B}_{\delta}(0)\subset\mathcal{N} and, for every x\in\mathcal{B}_{\delta}(0)\backslash\{0\} , s(t, x)\in\mathcal{B}_{\varepsilon}(0) for all t\in[0, T(x)) .
The zero solution x(t)\equiv 0 to (2.1) and (2.2) is globally finite time stable if it is finite time stable with \mathcal{N} = \mathcal{D} = \mathbb{R}^{n} .
Remark 3.1. The notion of finite time stability introduced here is different from the same term discussed in [1,20,25]. Specifically, the term finite time stability discussed in [1,20,25] deals with systems whose operation is constrained to a fixed finite interval of time and requires bounds on the system state variables.
Note that if the zero solution x(t)\equiv 0 to (2.1) and (2.2) is finite time stable, then it is asymptotically stable, and hence, finite time stability is a stronger notion than asymptotic stability. Next, we show that if the zero solution x(t)\equiv 0 to (2.1) and (2.2) is finite time stable, then (2.1) and (2.2) has a solution s(\cdot, \cdot) defined on \mathbb{R}_+ \times \mathcal{N} , and s(t, x) = 0 for all t > T(x) , x\in \mathcal{N} , where T(0) \triangleq 0 .
Proposition 3.1. Consider the nonlinear impulsive dynamical system (2.1) and (2.2). Assume that the zero solution x(t)\equiv 0 to (2.1) and (2.2) is finite time stable and let \mathcal{N} \subseteq \mathcal{D} and T: \mathcal{N}\backslash\{0\}\to(0, \infty) be as in Definition 3.1. Then, (2.1) and (2.2) has a solution s(\cdot, \cdot) defined on \mathbb{R}_+ \times \mathcal{N} , and s(t, x) = 0 for all t > T(x) , x\in \mathcal{N} , where T(0) \triangleq 0 .
Proof. For the case where the finite time convergence of the zero solution x(t)\equiv 0 to (2.1) and (2.2) occurs through the continuous dynamics (2.1), the proof is similar to the proof of Proposition 2.3 in [6], and hence, s(t, x) is defined on \mathbb{R}_+ and satisfies s(t, x) = 0 on [T(x), \infty) for every x \in \mathcal{N} . In general, however, for impulsive dynamical systems it may be possible to reset the states to the origin for some k = m \in \mathbb{Z}_+ . In this case, since x(t) \equiv 0 is an equilibrium point of (2.1) and (2.2), it follows that s(t, x_0) = 0 , t > \tau_{m}(x_0) = T(x_0) . Moreover, it follows from Assumption 2.2 (see Remark 2.1) that 0 \notin \mathcal{Z} , and hence, beating is precluded. Therefore, (2.1) and (2.2) has a solution s(\cdot, \cdot) defined on \mathbb{R}_+ \times \mathcal{N} , and s(t, x) = 0 for all t > T(x) , x\in \mathcal{N} , where T(0) \triangleq 0 .
The following result provides sufficient conditions for finite time stability of impulsive systems using a Lyapunov function involving a scalar differential inequality on the continuous-time dynamics.
Theorem 3.1. Consider the nonlinear impulsive dynamical system \mathcal{G} given by (2.1) and (2.2). Assume there exists a continuously differentiable function V:\mathcal{D}\rightarrow\overline{\mathbb{R}}_{+} satisfying V(0) = 0 , V(x) > 0 , x\in\mathcal{D} , x\neq 0 , and
where \gamma > 0 and \alpha\in (0, 1) . Then the zero solution x(t)\equiv 0 to (2.1) and (2.2) is finite time stable. Moreover, there exists an open neighborhood \mathcal{N} of the origin and a settling-time function T: \mathcal{N} \to \mathbb{R}_+ such that
If, in addition, \mathcal{D} = \mathbb{R}^{n} and V(\cdot) is radially unbounded, then the zero solution x(t)\equiv 0 to (2.1) and (2.2) is globally finite time stable.
Proof. Note that it follows from Theorem 2.1 of [13] that the zero solution to (2.1) and (2.2) is asymptotically stable. Thus, it remains to be shown that for all initial conditions in some neighborhood \mathcal{N}\subseteq\mathcal{D} of the origin the trajectories of (2.1) and (2.2) converge to the origin in finite time. Since the system (2.1) and (2.2) is asymptotically stable, it follows that there exists \delta > 0 such that for all x_0\in\mathcal{B}_{\delta}(0)\subset\mathcal{D} the trajectory s(t, x_0)\rightarrow 0 as t\rightarrow\infty . Next, we separately consider the cases when the trajectories of (2.1) and (2.2) have a finite and infinite number of resettings.
Assume that for some x_0\in\mathcal{B}_{\delta}(0) the trajectory s(t, x_0) , t\geq 0 , exhibits a finite number of resettings with resetting times \tau_{k}(x_0) , k = 1, \ldots, m . If s(\tau^{+}_{m}(x_0), x_0) = 0 , then since f_{\rm{c}}(0) = 0 it follows that s(t, x_0) = 0 , t\geq \tau_{m}(x_0) , which implies that s(t, x_0) , t\geq t_0 , converges to the origin in finite time with a settling-time function T(x_0) = \tau_{m}(x_0) . Furthermore, note that \tau_{1}(x^{+}_{k}) \leq T_{\rm{c}}(x^{+}_{k}) , k = 0, 1, \ldots, m-1 , since (2.1) and (2.2) exhibits a finite number of resettings, where T_{\rm{c}}(\cdot) is the settling-time function for (2.1) and (2.2) with \mathcal{Z} = \varnothing . Now, for \mathcal{Z} = \varnothing , it was shown in [6] that the continuous-time dynamics are finite time stable and
and hence, since \tau_{1}(x_0) < T_{\rm{c}}(x_0) , it follows that
Thus, since V(x+f_{\rm{d}}(x))\leq V(x) , x\in\mathcal{Z} , it follows from (3.5) that
Similarly, it follows from (3.4) that, for y = x^{+}_{2} ,
Recursively repeating this procedure for k = 3, 4, \ldots, m , it follows that, with \tau_{1}(x^{+}_{0}) = \tau_{1}(x_0) ,
Thus,
Alternatively, if s(\tau^{+}_{m}(x_0), x_0)\neq 0 , then, for all t > \tau_{m}(x_0) , the continuous-time dynamics are active and it follows from (3.1) and Theorem 4.2 of [6] that the trajectory s(t, s(\tau^{+}_{m}(x_0), x_0)) , t\geq 0 , converges to the origin in finite time given by \frac{1}{\gamma(1-\alpha)}[V(s(\tau^{+}_{m}(x_0), x_0))]^{1-\alpha} . In this case, it follows from (3.9) that the settling-time function for s(t, x_0) , t\geq 0 , is given by
where T(x_m^+) \leq \frac{1}{\gamma(1-\alpha)}(V(x_m^+))^{1-\alpha} is the settling-time function for the piece of the trajectory s(t, s(\tau_m^+(x_0), x_0)) , t \geq \tau_m^+(x_0) , where \lim_{t \to T(x_m^+) }s(t, s(\tau^{+}_{m}(x_0), x_0)) = 0 .
Next, assume that for some x_0\in\mathcal{B}_{\delta}(0) the trajectory s(t, x_0) , t\geq 0 , exhibits an infinite number of resettings with the resetting times \tau_{k}(x_0) , k = 0, 1, \ldots , where \tau_{0}(x_0) \triangleq 0 . Let x^{+}_{k} \triangleq s(\tau^{+}_{k}(x_0), x_0) , k = 0, 1, \ldots , where x^{+}_{0} \triangleq x_0 , and note that since (2.1) and (2.2) is asymptotically stable it follows that \tau_{1}(x^{+}_{k})\rightarrow 0 as k\rightarrow\infty . Now, note that since x(t)\equiv 0 is asymptotically stable, it follows that \lim_{k\rightarrow\infty}\tau_{1}(x^{+}_{k}) = 0 . Hence, it follows from (3.8), with m\rightarrow\infty , that
Thus,
which implies that the trajectory s(\cdot, x_0) is Zeno [13] and converges to the origin in finite time with an infinite number of resettings, that is, s(t, x_0)\rightarrow 0 as t\rightarrow T(x_0) .
Finally, suppose, ad absurdum, that s(t', x_0)\neq 0 for some t' > T(x_0) , x_0\in\mathcal{B}_{\delta}(0) . Then, since V(\cdot) is positive definite, V(s(t', x_0)) = \beta > 0 . Furthermore, since s(t, x_0)\rightarrow 0 as t\rightarrow T(x_0) , there exists t'' < T(x_0) such that V(s(t'', x_0)) < \beta . Now, since V(s(t, x_0)) is a decreasing function of time, it follows that for t'' < T(x_0) < t' ,
which leads to a contradiction. Hence, s(t, x_0) = 0 , t\geq T(x_0) , x_0\in\mathcal{B}_{\delta}(0) , which implies convergence in finite time with \mathcal{N}\triangleq\mathcal{B}_{\delta}(0) . This completes the proof of finite time stability.
Finally, if \mathcal{D} = \mathbb{R}^{n} and V(\cdot) is radially unbounded, then global finite time stability follows using standard arguments. See, for instance, [13].
Next, we provide a sufficient condition for finite time stability of impulsive systems using a Lyapunov function involving a scalar difference inequality and a minimum operator on the resetting dynamics.
Theorem 3.2. Consider the nonlinear impulsive dynamical system \mathcal{G} given by (2.1) and (2.2). Assume there exists a continuously differentiable function V:\mathcal{D}\rightarrow\overline{\mathbb{R}}_{+} satisfying V(0) = 0 , V(x) > 0 , x\in\mathcal{D} , x\neq 0 , and
where c > 0 and \{x\in \mathcal{D}\backslash \{0\}:V(x) < d\} \subseteq \mathcal{Z} , d \in (0, c] . Then the zero solution x(t)\equiv 0 to (2.1) and (2.2) is finite time stable. Moreover, the possibility of Zeno solutions is precluded. If, in addition, \mathcal{D} = \mathbb{R}^{n} and V(\cdot) is radially unbounded, then the zero solution x(t)\equiv 0 to (2.1) and (2.2) is globally finite time stable.
Proof. Note that it follows from Theorem 2.1 of [13] that the zero solution to (2.1) and (2.2) is asymptotically stable. Thus, it remains to be shown that for all initial conditions in some neighborhood \mathcal{N}\subseteq\mathcal{D} of the origin the trajectories of (2.1) and (2.2) converge to the origin in finite time. Since the system (2.1) and (2.2) is asymptotically stable, it follows that there exists \delta > 0 such that for all x_0\in\mathcal{B}_{\delta}(0)\subset\mathcal{D} the trajectory s(t, x_0)\rightarrow 0 as t\rightarrow\infty . Note that since s(t, x_0)\rightarrow 0 as t \rightarrow \infty , there exists t = t' such that V(s(t', x_0)) \leq c . Now, suppose there exists t = t'' > t' such that x(t'') = s(t'', x_0)\in \mathcal{Z} and V(x(t'')) \geq d . In this case, if V(x) \leq c , x \in \mathcal{Z} , then V(x+f_{\rm d}(x)) = 0 , and hence, V(x^+(t'')) = 0 , which implies convergence in finite time with \mathcal{N}\triangleq\mathcal{B}_{\delta}(0) . Alternatively, suppose there exists t = t' such that x(t') \in \mathcal{Z} and V(x(t')) < d . In this case, since \{x\in \mathcal{D}\backslash\{0\}:V(x) < d\} \subseteq \mathcal{Z} , d \in (0, c] , it follows that V(x+f_{\rm d}(x)) = 0 , which implies convergence in finite time with \mathcal{N}\triangleq\mathcal{B}_{\delta}(0) . This completes the proof of finite time stability.
Next, we separately consider the cases when the trajectories of (2.1) and (2.2) have a finite and infinite number of resettings. Assume that for some x_0\in\mathcal{B}_{\delta}(0) the trajectory s(t, x_0) , t\geq 0 , exhibits a finite number of resettings with resetting times \tau_{k}(x_0) , k = 1, \ldots, m . It follows from \{x\in \mathcal{D}\backslash\{0\}:V(x) < d\} \subseteq \mathcal{Z} , d \in (0, c] , and (3.15) that s(\tau^{+}_{m}(x_0), x_0) = 0 , and hence, since f_{\rm{c}}(0) = 0 it follows that s(t, x_0) = 0 , t\geq \tau_{m}(x_0) , which implies that s(t, x_0) , t\geq 0 , converges to the origin in finite time with a settling-time function T(x_0) = \tau_{m}(x_0) .
Alternatively, suppose, ad absurdum, that for some x_0\in\mathcal{B}_{\delta}(0) the trajectory s(t, x_0) , t\geq 0 , exhibits an infinite number of resettings with the resetting times \tau_{k}(x_0) , k = 0, 1, \ldots , where \tau_{0}(x_0) \triangleq 0 . Let x^{+}_{k} \triangleq s(\tau^{+}_{k}(x_0), x_0) , k = 0, 1, \ldots , where x^{+}_{0} \triangleq x_0 , and note that since (2.1) and (2.2) is asymptotically stable it follows that \tau_{1}(x^{+}_{k})\rightarrow 0 as k\rightarrow\infty . It follows from (3.14) and (3.15) that
and hence, there exists m > 0 such that V(x_m^+) = 0 , which leads to a contradiction. Thus, the trajectories of (2.1) and (2.2) with (3.14) and (3.15) can only have a finite number of resettings, and hence, the possibility of Zeno solutions is precluded.
Finally, if \mathcal{D} = \mathbb{R}^{n} and V(\cdot) is radially unbounded, then global finite time stability follows using standard arguments.
Finally, we merge the hypothesis of Theorems 3.1 and 3.2 to develop a stronger Lyapunov finite time stability theorem for impulsive dynamical systems using a Lyapunov function involving a scalar differential and difference inequality on both the continuous-time dynamics and discrete-time resetting dynamics.
Theorem 3.3. Consider the nonlinear impulsive dynamical system \mathcal{G} given by (2.1) and (2.2). Assume there exists a continuously differentiable function V:\mathcal{D}\rightarrow\overline{\mathbb{R}}_{+} satisfying V(0) = 0 , V(x) > 0 , x\in\mathcal{D} , x\neq 0 , and
where \gamma > 0 , \alpha\in (0, 1) , and c > 0 . Then the zero solution x(t)\equiv 0 to (2.1) and (2.2) is finite time stable. Moreover, the possibility of Zeno solutions is precluded. Furthermore, there exists an open neighborhood \mathcal{N} of the origin and a settling-time function T: \mathcal{N} \to \mathbb{R}_+ such that
If, in addition, \mathcal{D} = \mathbb{R}^{n} and V(\cdot) is radially unbounded, then the zero solution x(t)\equiv 0 to (2.1) and (2.2) is globally finite time stable.
Proof. The proof follows as a direct consequence of Theorems 3.1 and 3.2.
Remark 3.2. Note that Theorems 3.2 and 3.3 also hold for the case where (3.15) and (3.18) are replaced by
where c > 0 and \alpha \in (0, 1) . For details of this fact, see Theorem 4.2 of [15]. A similar remark holds for Theorems 4.2 and 4.3 below.
4.
Finite time stabilization of impulsive dynamical systems
In this section, we use the finite time stability results developed in Section 3 to design hybrid finite time stabilizing controllers for nonlinear affine in the control impulsive dynamical systems. Consider the controlled nonlinear impulsive dynamical system given by
where f_{\rm{c}}:\mathbb{R}^{n}\rightarrow\mathbb{R}^{n} satisfying f_{\rm{c}}(0) = 0 and G_{\rm{c}}:\mathbb{R}^{n}\rightarrow\mathbb{R}^{n\times m_{\rm{c}}} are continuous functions, f_{\rm{d}}:\mathbb{R}^{n}\rightarrow\mathbb{R}^{n} and G_{\rm{d}}:\mathbb{R}^{n}\rightarrow\mathbb{R}^{n\times m_{\rm{d}}} are continuous, u_{\rm{c}}(t)\in\mathbb{R}^{m_{\rm{c}}} , t\geq t_0 , and u_{\rm{d}}(t_k)\in\mathbb{R}^{m_{\rm{d}}} , k\in\overline{\mathbb{Z}}_{+} . For the statement of the following results, \mathbb{N}^n denotes the set of n\times n nonnegative definite matrix functions.
Theorem 4.1. Consider the controlled nonlinear impulsive dynamical system given by (4.1) and (4.2). If there exist a continuously differentiable function V:\mathbb{R}^{n}\rightarrow\overline{\mathbb{R}}_{+} and continuous functions P_{1u}:\mathbb{R}^{n}\rightarrow\mathbb{R}^{1\times m_{\rm{d}}} , P_{2u}:\mathbb{R}^{n}\rightarrow\mathbb{N}^{m_{\rm{d}}} , and L: \mathbb{R}^n \to \mathbb{R}^{1\times m_d} such that V(\cdot) is positive definite and
where \gamma > 0 , \alpha\in (0, 1) , and \mathcal{R} \triangleq \{x\in\mathbb{R}^{n}, \, x\notin\mathcal{Z}\, :\, V'(x)G_{\rm{c}}(x) = 0\} , then the nonlinear impulsive dynamical system (4.1) and (4.2) with the hybrid feedback control law (u_{\rm{c}}, u_{\rm{d}}) = (\phi_{\rm{c}}(\cdot), \phi_{\rm{d}}(\cdot)) given by
and
where \alpha(x) \triangleq V'(x)f_{\rm{c}}(x), \, x\in\mathbb{R}^{n} , \beta(x)\triangleq G_{\rm{c}}^{\rm{T}}(x)V'^{\rm{T}}(x), \, x\in\mathbb{R}^{n} , w_{\gamma}(V(x))\triangleq -\gamma(V(x))^{\alpha} , x\in\mathbb{R}^{n} , and c_{0} > 0 , is finite time stable.
Proof. Note that between resettings the time derivative of V(\cdot) along the trajectories of (4.1), with u_{\rm{c}} = \phi_{\rm{c}}(x) , x\in\mathbb{R}^{n} , given by (4.6), is given by
In addition, using (4.3) and (4.5), the difference of V(\cdot) at the resetting instants, with u_{\rm{d}} = \phi_{\rm{d}}(x) , x\in\mathcal{Z} , given by (4.7), is given by
Hence, it follows from Theorem 3.1 that the zero solution x(t)\equiv 0 to (4.1) and (4.2) with u_{\rm{c}} = \phi_{\rm{c}}(x) , x\notin\mathcal{Z} , given by (4.6) and u_{\rm{d}} = \phi_{\rm{d}}(x) , x\in\mathcal{Z} , given by (4.7), is finite time stable, which proves the result.
Theorem 4.2. Consider the controlled nonlinear impulsive dynamical system given by (4.1) and (4.2). If there exist a continuously differentiable function V:\mathbb{R}^{n}\rightarrow\overline{\mathbb{R}}_{+} and continuous functions P_{1u}:\mathbb{R}^{n}\rightarrow\mathbb{R}^{1\times m_{\rm{d}}} , P_{2u}:\mathbb{R}^{n}\rightarrow\mathbb{N}^{m_{\rm{d}}} , and L: \mathbb{R}^n \to \mathbb{R}^{1\times m_d} such that V(\cdot) is positive definite, (4.3) holds, and
where c > 0 , \alpha\in (0, 1) , \{x\in \mathcal{D}\backslash\{0\}:V(x) < d\} \subseteq \mathcal{Z} , d\in(0, c] , and \mathcal{R} \triangleq \{x\in\mathbb{R}^{n}, \, x\notin\mathcal{Z}\, :\, V'(x)G_{\rm{c}}(x) = 0\} , then the nonlinear impulsive dynamical system (4.1) and (4.2) with the hybrid feedback control law (u_{\rm{c}}, u_{\rm{d}}) = (\phi_{\rm{c}}(\cdot), \phi_{\rm{d}}(\cdot)) given by
and
where \alpha(x) \triangleq V'(x)f_{\rm{c}}(x), \, x\in\mathbb{R}^{n} , and \beta(x)\triangleq G_{\rm{c}}^{\rm{T}}(x)V'^{\rm{T}}(x) , x\in\mathbb{R}^{n} , is finite time stable.
Proof. Note that between resettings the time derivative of V(\cdot) along the trajectories of (4.1), with u_{\rm{c}} = \phi_{\rm{c}}(x) , x\in\mathbb{R}^{n} , given by (4.12), is given by
In addition, using (4.3) and (4.11), the difference of V(\cdot) at the resetting instants, with u_{\rm{d}} = \phi_{\rm{d}}(x) , x\in\mathcal{Z} , given by (4.13), is given by
Hence, it follows from Theorem 3.2 that the zero solution x(t)\equiv 0 to (4.1) and (4.2) with u_{\rm{c}} = \phi_{\rm{c}}(x) , x\notin\mathcal{Z} , given by (4.12) and u_{\rm{d}} = \phi_{\rm{d}}(x) , x\in\mathcal{Z} , given by (4.13), is finite time stable, which proves the result.
Theorem 4.3. Consider the controlled nonlinear impulsive dynamical system given by (4.1) and (4.2). If there exist a continuously differentiable function V:\mathbb{R}^{n}\rightarrow\overline{\mathbb{R}}_{+} and continuous functions P_{1u}:\mathbb{R}^{n}\rightarrow\mathbb{R}^{1\times m_{\rm{d}}} , P_{2u}:\mathbb{R}^{n}\rightarrow\mathbb{N}^{m_{\rm{d}}} , and L: \mathbb{R}^n \to \mathbb{R}^{1\times m_d} such that V(\cdot) is positive definite, (4.3) holds, and
where \gamma > 0 , \alpha\in (0, 1) , c > 0 , and \mathcal{R} \triangleq \{x\in\mathbb{R}^{n}, \, x\notin\mathcal{Z}\, :\, V'(x)G_{\rm{c}}(x) = 0\} , then the nonlinear impulsive dynamical system (4.1) and (4.2) with the hybrid feedback control law (u_{\rm{c}}, u_{\rm{d}}) = (\phi_{\rm{c}}(\cdot), \phi_{\rm{d}}(\cdot)) given by
and
where \alpha(x) \triangleq V'(x)f_{\rm{c}}(x), \, x\in\mathbb{R}^{n} , \beta(x)\triangleq G_{\rm{c}}^{\rm{T}}(x)V'^{\rm{T}}(x), \, x\in\mathbb{R}^{n} , w_{\gamma}(V(x))\triangleq -\gamma(V(x))^{\alpha} , x\in\mathbb{R}^{n} , and c_{0} > 0 , is finite time stable.
Proof. Note that between resettings the time derivative of V(\cdot) along the trajectories of (4.1), with u_{\rm{c}} = \phi_{\rm{c}}(x) , x\in\mathbb{R}^{n} , given by (4.19), is given by
In addition, using (4.3) and (4.18), the difference of V(\cdot) at the resetting instants, with u_{\rm{d}} = \phi_{\rm{d}}(x) , x\in\mathcal{Z} , given by (4.20), is given by
Hence, it follows from Theorem 3.3 that the zero solution x(t)\equiv 0 to (4.1) and (4.2) with u_{\rm{c}} = \phi_{\rm{c}}(x) , x\notin\mathcal{Z} , given by (4.19) and u_{\rm{d}} = \phi_{\rm{d}}(x) , x\in\mathcal{Z} , given by (4.20), is finite time stable, which proves the result.
Since f_{{\rm{c}}}(\cdot) and G_{{\rm{c}}}(\cdot) are continuous and V(\cdot) is continuously differentiable, it follows that \alpha(x) and \beta(x) , x\in\mathbb{R}^{n} , are continuous functions, and hence, \phi_{{\rm{c}}}(x) given by (4.6), (4.12), and (4.19) are continuous for all x\in\mathbb{R}^{n} if either \beta(x)\neq 0 or \alpha(x)-w_{\gamma}(V(x)) < 0 . Hence, the feedback control laws given by (4.6), (4.12), and (4.19) are continuous everywhere except for the origin. The following result provides necessary and sufficient conditions under which the feedback control laws given by (4.6), (4.12), and (4.19) are guaranteed to be continuous at the origin in addition to being continuous everywhere else.
Proposition 4.1. The feedback control laws \phi_{\rm{c}}(x) given by (4.6) and (4.19) are continuous on \mathbb{R}^{n} if and only if for every \varepsilon > 0 , there exists \delta > 0 such that for all 0 < \|x\| < \delta there exists u_{{\rm{c}}}\in\mathbb{R}^{m_{{\rm{c}}}} such that \|u_{{\rm{c}}}\| < \varepsilon and \alpha(x)+\beta^{\rm{T}}(x)u_{{\rm{c}}} < w_\gamma(V(x)) .
Proof. The proof is similar to the proof of Proposition 5.1 in [28] and, hence, is omitted.
Proposition 4.2. The feedback control law \phi_{\rm{c}}(x) given by (4.12) is continuous on \mathbb{R}^{n} if and only if for every \varepsilon > 0 , there exists \delta > 0 such that for all 0 < \|x\| < \delta there exists u_{{\rm{c}}}\in\mathbb{R}^{m_{{\rm{c}}}} such that \|u_{{\rm{c}}}\| < \varepsilon and \alpha(x)+\beta^{\rm{T}}(x)u_{{\rm{c}}} < 0 .
Proof. The proof is similar to the proof of Theorem 6.8 in [11] and, hence, is omitted.
Remark 4.1. If the conditions of Proposition 4.1 (respectively, Proposition 4.2) are satisfied, then the feedback control laws \phi_{\rm{c}}(x) given by (4.6) and (4.19) (respectively, (4.12)) are continuous on \mathbb{R}^{n} . However, it is important to note that for a particular trajectory x(t) , t\geq 0 , of (4.1) and (4.2), \phi_{\rm{c}}(x(t)) is left-continuous on [0, \infty) and is continuous everywhere on [0, \infty) except on an unbounded closed discrete set of times when the resettings occur for x(t) , t\geq 0 .
5.
Finite time stabilizing hybrid control for impulsive network systems
In this section, we apply the hybrid control frameworks developed in Section 4 to the control of nonlinear impulsive network systems. Specifically, we consider the network dynamical system \mathcal{G} involving information exchange between n interconnected nodes or subsystems, where x_i:[0, \infty)\rightarrow{\mathbb{R}} denotes the information of the i th subsystem, u_{{\rm{c}}i}:[0, \infty)\rightarrow\mathbb{R} denotes the control input to the i th subsystem, \sigma_{{\rm{c}}ij}:{\mathbb{R}}^{n}\rightarrow\mathbb{R} , i\neq j , i, j = 1, \ldots, n , denotes the instantaneous rate of information transfer from the j th subsystem to the i th subsystem between resettings, \sigma_{{\rm{d}}ij}:\mathbb{R}^{n}\rightarrow \mathbb{R} , i\neq j , i, j = 1, \ldots, n , denotes the amount of information transferred from the j th subsystem to the i th subsystem at the resetting instant, and \mathcal{Z}\subset{\mathbb{R}}^{n} is a resetting set for the impulsive network system \mathcal{G} .
An information balance for each subsystem \mathcal{G}_{i} , i = 1, \ldots n , representing a multiagent system yields [10,13]
where \mathcal{Z}_i \subset \mathbb{R} , i \in \{1, \dots, n\} , or, equivalently, in vector form
where x(t) = [x_{1}(t), \ldots, x_{n}(t)]^{\rm{T}} , t\geq t_0 , f_{{\rm{c}}i}(x) = \sum_{j = 1, j\neq i}^{n}\phi_{{\rm{c}}ij}(x_i, x_j) , where \phi_{{\rm{c}}ij}(x_i, x_j)\triangleq \sigma_{{\rm{c}}ij}(x_i, x_j)-\sigma_{{\rm{c}}ji}(x_i, x_j) , i\neq j , i, j = 1, \ldots, n , denotes the net information transfer from the j th subsystem to the i th subsystem between resettings, G_{\rm{c}}(x) = {\rm diag} [G_{{\rm{c}}1}(x_1), \ldots, G_{{\rm{c}}n}(x_n)] , x\in{\mathbb{R}}^{n} , G_{{\rm{c}}i}:\mathbb{R}\rightarrow\mathbb{R} , i = 1, \ldots, n , is such that x_i G_{{\rm c}i}(x_i) = 0 if and only if x_i = 0 for all i = 1, \dots, n , G_{\rm{d}}(x) = {\rm diag} [G_{{\rm{d}}1}(x), \ldots, G_{{\rm{d}}n}(x)] , x\in\mathcal{Z} , G_{{\rm{d}}i}:\mathbb{R}^{n}\rightarrow\mathbb{R} , i = 1, \ldots, n , u_{\rm{c}}(t)\in\mathbb{R}^{n}, \, t\geq t_0 , u_{\rm{d}}(t_k)\in\mathbb{R}^{n} , k\in{\mathbb{Z}} , f_{{\rm{d}}i}(x) = \sum_{j = 1, j\neq i}^{n}\phi_{{\rm{d}}ij}(x_i, x_j) , where \phi_{{\rm{d}}ij}(x_i, x_j)\triangleq \sigma_{{\rm{d}}ij}(x_i, x_j)-\sigma_{{\rm{d}}ji}(x_i, x_j) , x_i\in\mathcal{Z}_i , i\neq j , i, j = 1, \ldots, n , denotes the net amount of information transferred from the j th subsystem to the i th subsystem at the instant of resetting, and \mathcal{Z} = \cup_{i = 1}^n \{x\in \mathbb{R}^n:x_i\in \mathcal{Z}_i\} .
Here, we assume that \sigma_{{\rm{c}}ij}(0) = 0 , i\neq j , i, j = 1, \ldots, n , and u_{\rm{c}} = [u_{{\rm{c}}1}, \ldots, u_{{\rm{c}}n}]^{\rm{T}}:\mathbb{R}\rightarrow\mathbb{R}^{n} is such that u_{{\rm{c}}i}:\mathbb{R}\rightarrow\mathbb{R} , i = 1, \ldots, n , are bounded piecewise continuous functions of time. Furthermore, we assume that for i, j = 1, \dots, n , (x_i-x_j)\phi_{{\rm{c}}ij}(x_i, x_j) \leq 0 , x \notin \mathcal{Z} , (x_i-x_j)\phi_{{\rm{d}}ij}(x_i, x_j) \leq 0 , x \in \mathcal{Z} , and (\Delta x_i - \Delta x_j)/(x_i-x_j) \geq -1 , x_i \neq x_j , x \in \mathcal{Z} . The first and second assumptions imply that information flows from information rich subsystems to information poor subsystems between resettings as well as across resetting events and in reminiscent of the second law of thermodynamics, whereas the third assumption implies that for any pair of connected subsystems the information difference across resetting events is monotonic. For further details of these assumptions, see [10].
For the dynamical system \mathcal{G} given by (5.3) and (5.4) we construct feedback stabilizing controllers using Theorems 4.1–4.3. Specifically, consider the Lyapunov function candidate V(x) = x^ {\rm{T}} x , x\in{\mathbb{R}}^{n} and note that V(0) = 0 and V(x) > 0 , x\neq 0 , x\in{\mathbb{R}}^{n} . It follows from (4.3) that
Furthermore, note that \mathcal{R}\triangleq\{x\in{\mathbb{R}}^{n}, \, x\notin\mathcal{Z}: \, V'(x)G_{{\rm{c}}}(x) = 0\} = \{x\in{\mathbb{R}}^{n}, \, x\notin\mathcal{Z}:\, x_i G_{{\rm c}i}(x_i) = 0, \, i = 1, \dots, n\} = \{0\} .
First, we use Theorem 4.1 to construct a finite-time stabilizing hybrid feedback controller for the dynamical system \mathcal{G} given by (5.3) and (5.4). Note that with \alpha(x) = V'(x) f_{\rm c}(x) = 2 x^ {\rm{T}} f_{\rm c}(x) = 2 \sum_{i = 1}^{n} \sum_{j = 1, j\neq i}^{n} x_i\phi_{{\rm{c}}ij}(x_i, x_j) , \beta(x) = G^ {\rm{T}}_{\rm c} (x) V'^ {\rm{T}}(x) = 2 G^ {\rm{T}}_{\rm c}(x) x , \gamma = 1 , \alpha = 0.5 , and c_0 > 0 , a finite-time stabilizing hybrid feedback controller (4.6) and (4.7) is given by
and, with L(x) = -2[x+f_{{\rm d}}(x)]^ {\rm{T}} G_{\rm d}(x) ,
Since \mathcal{R} = \{0\} , (4.4) is trivially satisfied. Moreover, it follows from assumptions (x_i-x_j)\phi_{{\rm{d}}ij}(x_i, x_j) \leq 0 , x \in \mathcal{Z} , and (\Delta x_i - \Delta x_j)/(x_i-x_j) \geq -1 , x_i \neq x_j , i, j = 1, \dots, n , x \in \mathcal{Z} , that
and hence, (4.5) is satisfied. Thus, it follows from Theorem 4.1 that the closed-loop system (5.3) and (5.4) with hybrid feedback control law (5.7) and (5.8) is finite time stable.
Next, we use Theorem 4.2 to construct a finite-time stabilizing hybrid feedback controller for the dynamical system \mathcal{G} given by (5.3) and (5.4). The finite time stabilizing hybrid feedback controller (4.12) and (4.13) is given by
and, with L(x) = [L_1(x), \dots, L_n(x)] , L_i: \mathbb{R}\to \mathbb{R} , i = 1, \dots, n , L_i(x) = -2G_{{\rm d}i}(x)[x_i- {\rm sign} (x_i) \min\{|x_i|, c\}] , where {\rm sign}(x_i) \triangleq x_i/|x_i| , x_i \neq 0 , {\rm sign}(0) \triangleq 0 ,
where {\rm \textbf{min}} \{x, c\} = [{\rm sign} (x_1) \min\{|x_1|, c\}, \dots, {\rm sign} (x_n)\min\{|x_n|, c\}]^ {\rm{T}} . Since \mathcal{R} = \{0\} , (4.10) is trivially satisfied. Moreover, note that
and hence, (4.11) is satisfied. Thus, it follows from Theorem 4.2 that the closed-loop system (5.3) and (5.4) with hybrid feedback control law (5.10) and (5.11) is finite time stable.
Finally, we use Theorem 4.3 to construct a finite-time stabilizing hybrid feedback controller for (5.3) and (5.4) using (4.19) and (4.20), given by
and, with L(x) = [L_1(x) \dots L_n(x)] , L_i: \mathbb{R}\to \mathbb{R} , i = 1, \dots, n , L_i(x) = -2G_{{\rm d}i}(x)[x_i- {\rm sign} (x_i) \min\{|x_i|, c\}] ,
Since \mathcal{R} = \{0\} , (4.17) is trivially satisfied. Moreover, it follows from (5.12) that (4.18) is satisfied. Thus, it follows from Theorem 4.3 that the closed-loop system (5.3) and (5.4) with hybrid feedback control law (5.13) and (5.14) is finite time stable.
For the following simulations we consider (5.3) and (5.4) with \sigma_{{\rm{c}}ij}(x_i, x_j) = \sigma_{{\rm{c}}ij} x_{j} , \sigma_{{\rm{d}}ij}(x_i, x_j) = \sigma_{{\rm{d}}ij}x_{j} , G_{{\rm{c}}i}(x_i) = {\rm sign}(x_i) |x_i|^{-1/4} , and G_{{\rm d}i} = {\rm sign}(x_i) |x_i|^{1/2} , where \sigma_{{\rm{c}}ij}\geq 0 , i\neq j , i, j = 1, \ldots, n , \sigma_{{\rm{d}}ij} \geq 0 , i, j = 1, \dots, n . To show that the conditions of Proposition 4.1 are satisfied with control law (5.7) and w_{\gamma}(V(x)) \triangleq -\gamma (V(x))^\alpha , let u_c = -\frac{1}{2}[|x_1|^{1/4}, \dots, |x_n|^{1/4}]^ {\rm{T}} and note that it follows from the assumption (x_i-x_j)\phi_{{\rm{c}}ij}(x_i, x_j) \leq 0 , i, j = 1, \dots, n , x \notin \mathcal{Z} , that
Thus, for every \varepsilon > 0 there exists \delta > 0 , such that for all 0 < \|x\| < \delta there exists u_{{\rm{c}}}\in\mathbb{R}^{m_{{\rm{c}}}} such that \|u_{{\rm{c}}}\| < \varepsilon and \alpha(x)+\beta^{\rm{T}}(x)u_{{\rm{c}}} < w_{\gamma}(V(x)) , and hence, the continuous-time feedback control law (5.7) is continuous on {\mathbb{R}}^{n} . Similarly, it follows from (5.15) that the conditions of Proposition 4.2 (respectively, Proposition 4.1) are satisfied with control law (5.10) (respectively, (5.13)), and hence, the continuous-time feedback control law (5.10) (respectively, (5.13)) is continuous on {\mathbb{R}}^{n} .
We set n = 2 , \sigma_{{\rm{c}}12} = 1.5 , \sigma_{{\rm{c}}21} = 1.5 , \sigma_{{\rm{d}}12} = 0.25 , \sigma_{{\rm{d}}21} = 0.25 , c_{0} = 1 , c = 0.5 , and \alpha = 0.5 , with the initial condition x_0 = [8, 4]^{\rm{T}} . To construct the feedback control law (5.8), let L: \mathbb{R}^2 \to \mathbb{R}^{1\times 2} be given by
Next, to construct the feedback control law (5.11) and (5.14), let L: \mathbb{R}^2 \to \mathbb{R}^{1\times 2} be given by
where c > 0 . For our simulations we consider several different resetting sets.
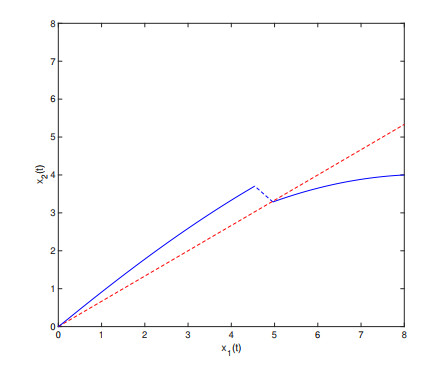
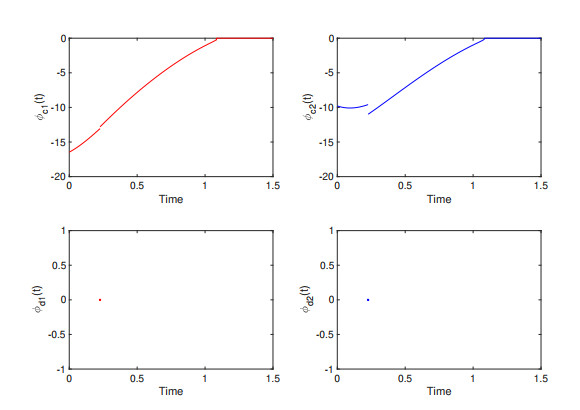
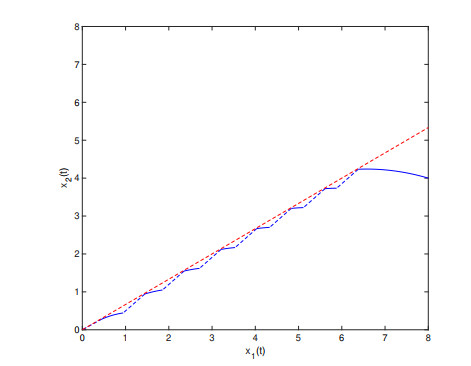
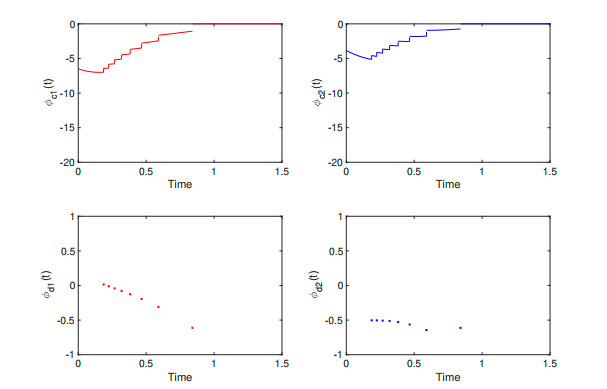
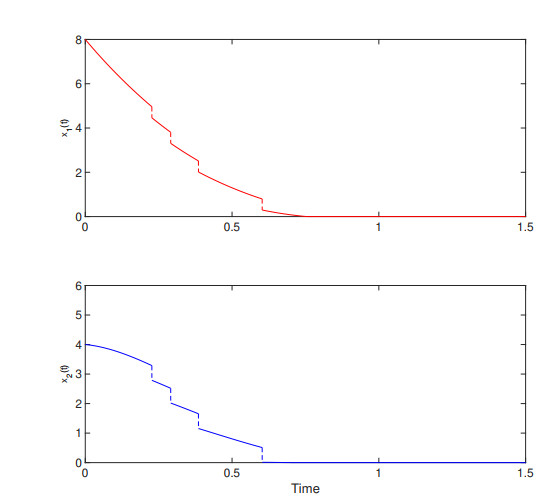
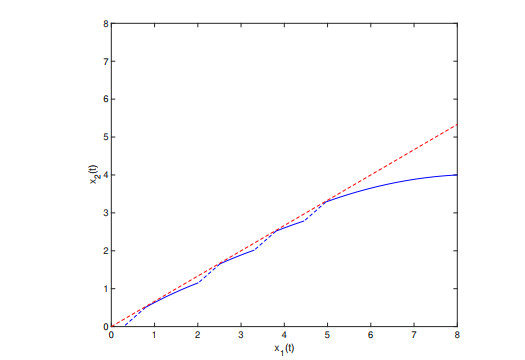
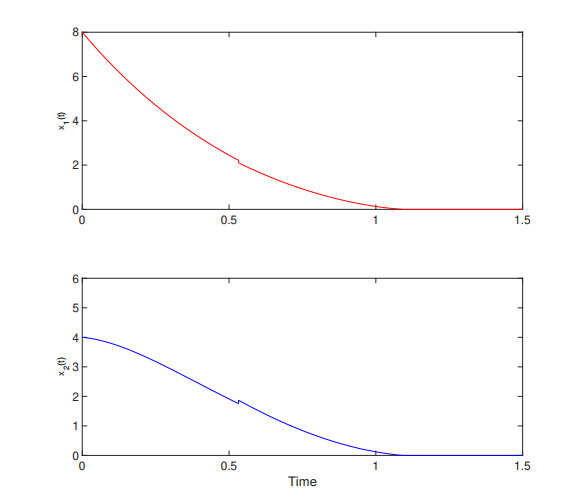
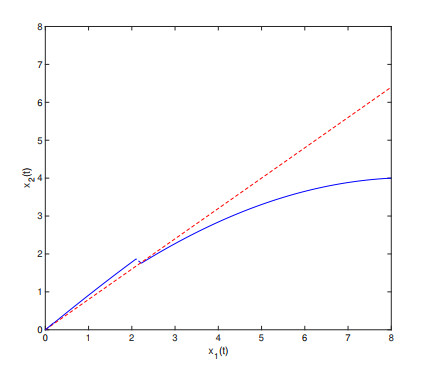
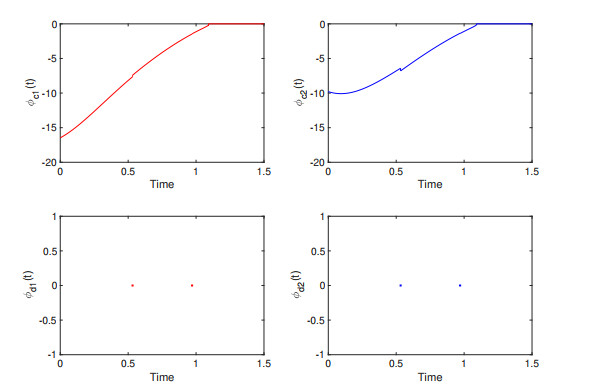
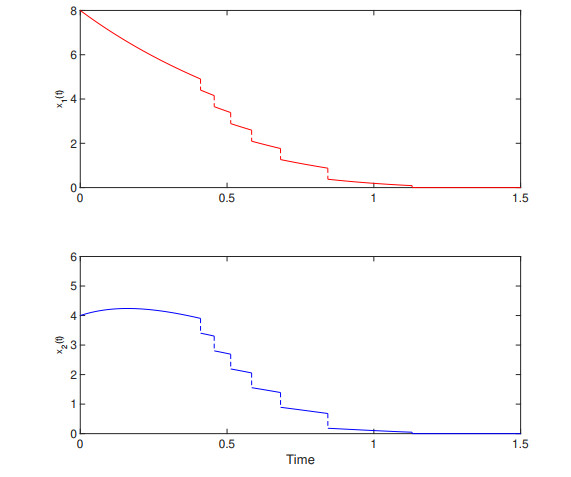
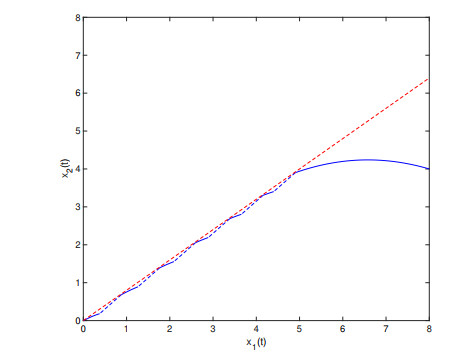
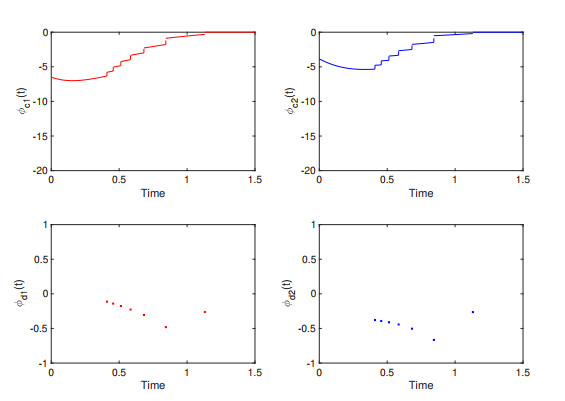
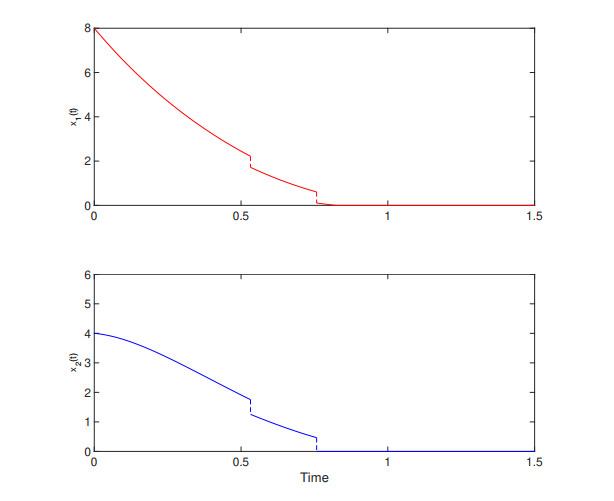
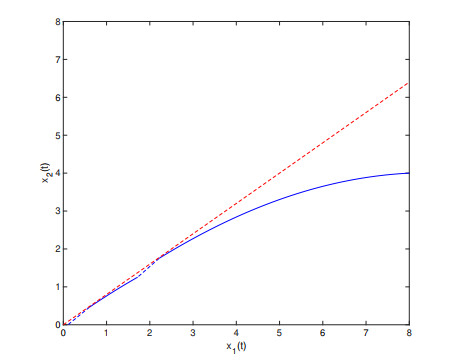
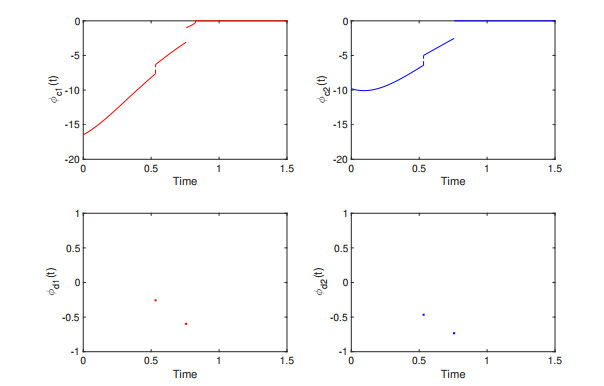
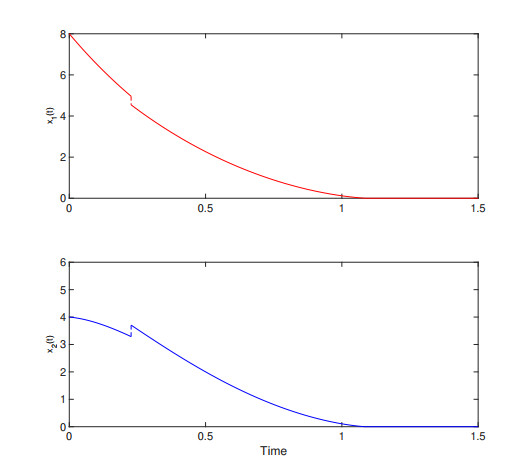
For the first simulation, consider \mathcal{Z}_1 \triangleq \{x_1 \backslash\{0\}: x_1 - \frac{3}{2} x_2 = 0\} and \mathcal{Z}_2 \triangleq \{x_2\backslash\{0\}: x_2 - \frac{2}{3}x_1 = 0\} . Figure 1 shows the states of the closed-loop system with the feedback control law (5.7) and (5.8) versus time, Figure 2 shows the phase portrait of the closed-loop system, where the dashed line denotes the resetting set \mathcal{Z}_{\rm I} \triangleq \mathcal{Z}_1 \cup \mathcal{Z}_2 = \{x\in\mathbb{R}^{2}\backslash\{0\}:\, 3 x_2- 2x_1 = 0\} , and Figure 3 shows the control signals as a function of time. Next, Figure 4 shows the states of the closed-loop system with the feedback control law (5.10) and (5.11) versus time, Figure 5 shows the phase portrait of the closed-loop system, where the dashed line denotes the resetting set \mathcal{Z}_{\rm I}^\prime \triangleq\{x\in\mathbb{R}^{2}\backslash\{0\}:\, 3 x_2 - 2 x_1 = 0\} \cup \{x\in \mathbb{R}^2\backslash\{0\}: V(x) < 0.1\} , and Figure 6 shows the control signals as a function of time. Finally, Figure 7 shows the states of the closed-loop system with the feedback control law (5.13) and (5.14) versus time, Figure 8 shows the phase portrait of the closed-loop system, where the dashed line denotes the resetting set \mathcal{Z}_{\rm I} = \{x\in\mathbb{R}^{2}\backslash\{0\}:\, 3 x_2- 2x_1 = 0\} , and Figure 9 shows the control signals as a function of time.
For the next simulation, consider \mathcal{Z}_1 \triangleq \{x_1 \backslash\{0\}: x_1 - \frac{5}{4} x_2 = 0\} and \mathcal{Z}_2 \triangleq \{x_2\backslash\{0\}: x_2 - \frac{4}{5}x_1 = 0\} . Figure 10 shows the states of the closed-loop system with the feedback control law (5.7) and (5.8) versus time, Figure 11 shows the phase portrait of the closed-loop system, where the dashed line denotes the resetting set \mathcal{Z}_{\rm II} \triangleq \mathcal{Z}_1 \cup \mathcal{Z}_2 = \{x\in\mathbb{R}^{2}\backslash\{0\}:\, 5 x_2-4 x_1 = 0\} , and Figure 12 shows the control signals as a function of time. Next, Figure 13 shows the states of the closed-loop system with the feedback control law (5.10) and (5.11) versus time, Figure 14 shows the phase portrait of the closed-loop system, where the dashed line denotes the resetting set \mathcal{Z}_{\rm II}^\prime \triangleq\{x\in\mathbb{R}^{2}\backslash\{0\}:\, 5x_2-4x_1 = 0\} \cup \{x\in \mathbb{R}^2\backslash\{0\}: V(x) < 0.1\} , and Figure 15 shows the control signals as a function of time. Finally, Figure 16 shows the states of the closed-loop system with the feedback control law (5.13) and (5.14) versus time, Figure 17 shows the phase portrait of the closed-loop system, where the dashed line denotes the resetting set \mathcal{Z}_{\rm II} = \{x\in\mathbb{R}^{2}\backslash\{0\}:\, 5x_2-4x_1 = 0\} , and Figure 18 shows the control signals as a function of time.
The achieved settling times of (5.3) and (5.4) with the different resetting sets and hybrid feedback control laws are given in Table 1. Note that the hybrid feedback control law (5.10) and (5.11) does not always outperform (5.7) and (5.8); its performance depends on the resetting set and initial condition. However, note that the hybrid feedback control law (5.13) and (5.14) always outperforms the other two hybrid controller architectures.
6.
Conclusions
The notion of finite time stability for impulsive dynamical systems originally developed in [29] was extended to develop new Lyapunov theorems for finite time stability of hybrid systems involving a scalar differential inequality on the Lyapunov function for the continuous-time dynamics as well as a scalar difference inequality and a minimum operator on the Lyapunov function for the discrete-time resetting dynamics. These results were then used to develop universal finite-time stabilizing hybrid controllers for nonlinear impulsive dynamical systems. Finally, the results were used to design hybrid feedback stabilizers for several illustrative numerical examples involving network impulsive dynamical systems. Future research will explore merging the results of [22,24] with the results in this paper to obtain finite time Lyapunov stability theorems for hybrid systems with time delay.
Acknowledgments
This work was supported in part by the Air Force Office of Scientific Research under Grant FA9550-20-1-0038.









 DownLoad:
DownLoad: