The optimal problems for torsional rigidity

1.   Introduction

The theory of bifurcations is crucial in understanding qualitative properties of differential systems depending on one or more parameters. Bifurcations of codimension one and two are well-addressed in the literature ^[1,2,3] when they are non-degenerate, but many open problems emerge when they become degenerate ^[4] or the codimension is beyond 2.

The classical normal form of the double-Hopf bifurcation in differential systems of dimension 4 is based on six generic conditions (HH.0)–(HH.5) ^[1]. Some of the pioneering papers reporting results on non-degenerate double-Hopf bifurcation are references ^[5,6,7,8].

If one or more generic conditions fail to be satisfied, a degeneracy arises and, thus, the generic results are not valid anymore. In this work we study two degenerate double-Hopf bifurcations. The degeneracy we refer at is related to generic conditions which are necessary in obtaining normal forms.

Another added value of our results is that we discovered new bifurcation diagrams and new phase portraits and, thus, new properties of double-Hopf bifurcation which, to our knowledge, were not previously reported in the literature. The results emerged from the analysis of a new system which appeared due to degeneracy.

Our study is performed in a general framework and produces generic results which can be applied in particular systems exhibiting this bifurcation. The double-Hopf bifurcation (degenerate or not) can be often met in practical models based on differential systems and having a large number of equations (at least four) ^{[4,9,10,11,12]}. Interesting results on discrete time systems undergoing double-Hopf bifurcations are presented in ^[11]. Thus, understanding better this bifurcation and obtaining new generic properties of it is desirable and important for particular models describing real-world phenomena. This is the purpose of the present work.

The paper is organized as follows. In Section 2 the classical normal form obtained in ^[1] using six generic conditions (HH.0)–(HH.5) is presented and a new normal form up to order 3 in polar coordinates is obtained as two of the generic conditions, namely (HH.1) and (HH.3) fail. In Section 3, the dynamics and bifurcation of this normal form, around the origin, is analyzed as the condition (HH.3) is not satisfied, but (HH.1) is valid, while in Section 4 a similar study is done when the condition (HH.1) is not fulfilled, but (HH.3) is satisfied. In Section 5 some conclusions and the relationship between the dynamics of the 2D amplitude system and the dynamics of the 4D truncated normal form are given.

2.   About the double-Hopf bifurcation

Consider a differential system of the form

with $ f $ smooth, $ \dot{x} = \frac{dx}{dt} $, and assume that $ x = 0 $ is an equilibrium point of the system for all $ \alpha $ with $ \left\vert \alpha \right\vert = \sqrt{\alpha _{1}^{2}+\alpha _{1}^{2}} $ small enough, that is, $ f\left(0, \alpha \right) \equiv 0; $ $ x = 0 $ stands for $ x = (0, 0, 0, 0) $ and $ \alpha = 0 $ for $ \alpha = (0, 0). $ The system (2.1) can be written as

where $ F\left(x, \alpha \right) = O\left(\left\vert x\right\vert ^{2}\right) $ is a smooth function denoting Taylor rest with terms of order at least $ 2. $

Assume the matrix $ A(\alpha) $ has two pairs of simple complex-conjugate eigenvalues $ \lambda _{1}, $ $ \overline{\lambda }_{1}, $ $ \lambda _{2}, $ $ \overline{\lambda }_{2}, $

for all sufficiently small $ \left\vert \alpha \right\vert, $ where $ \mu _{1, 2}\left(\alpha \right) $ and $ \omega _{1, 2}\left(\alpha \right) $ are smooth functions of $ \alpha $ such that $ \mu _{1}\left(0\right) = \mu _{2}\left(0\right) = 0, $ respectively, $ \omega _{1}\left(0\right) = \omega _{10} > 0 $ and $ \omega _{2}\left(0\right) = \omega _{20} > 0. $ When these conditions are satisfied, the origin of system (2.1) is called a double-Hopf or Hopf-Hopf singularity, and, under supplementary conditions, a double-Hopf bifurcation occurs at $ \alpha = 0 $.

In complex coordinates, the system (2.2) can be further reduced to the form

where

and

Other two equations are the conjugates of system $ \left(2.3\right) $ and are omitted in what follows.

A first normal form corresponding to system(2.3) is described in the following lemma, reported in ^[1].

Lemma 2.1. Assume that the following conditions take place ^[1]

Then, there exists a locally defined, smooth and smoothlyparameter-dependent, invertible transformation of the complex variables thatreduces system $ (2.3) $ for all sufficiently small $ \left\vert \alpha\right\vert $ into the following form:

where $ w_{1, 2} $ are complex-valued functions, $ \left\Vert \left(w_{1}, \overline{w}_{1}, w_{2}, \overline{w}_{2}\right) \right\Vert ^{2} = \left\vert w_{1}\right\vert ^{2}+\left\vert w_{2}\right\vert ^{2} $.

In system (2.4) we used the following notations: $ a_{1}+ib_{1} = G_{2100}\left(\alpha \right), $ $ a_{2}+ib_{2} = G_{1011}\left(\alpha \right), $ $ a_{3}+ib_{3} = G_{3200}\left(\alpha \right), $ $ a_{4}+ib_{4} = G_{2111}\left(\alpha \right) $ and $ a_{5}+ib_{5} = G_{1022}\left(\alpha \right), $ respectively, $ c_{1}+id_{1} = H_{1110}\left(\alpha \right), $ $ c_{2}+id_{2} = H_{0021}\left(\alpha \right), $ $ c_{3}+id_{3} = H_{2210}\left(\alpha \right), $ $ c_{4}+id_{4} = H_{1121}\left(\alpha \right) $ and $ c_{5}+id_{5} = H_{0032}\left(\alpha \right), $ where $ a_{i} = a_{i}\left(\alpha \right), $ $ b_{i} = b_{i}\left(\alpha \right), $ $ c_{i} = c_{i}\left(\alpha \right) $ and $ d_{i} = d_{i}\left(\alpha \right), $ $ i = \overline{1, 5}, $ are real smooth functions of $ \alpha $ which depend on the coefficients $ g_{ijkl}\left(\alpha \right) $ and $ h_{ijkl}\left(\alpha \right). $ The coefficients of third order terms in system (2.4) are given explicitly in ^[1].

The system (2.4) has been transformed further in ^[1] to a normal form based on six generic conditions.

In polar coordinates $ w_{1} = r_{1}e^{i\varphi _{1}} $ and $ w_{2} = r_{2}e^{i \varphi _{2}}, $ the system (2.4) becomes

where the real functions $ \Phi _{k}, $ $ \Psi _{k} $ are smooth functions of their arguments and are $ 2\pi $-periodic in $ \varphi _{j}, $ $ \Phi _{k} = O\left(\left(r_{1}^{2}+r_{2}^{2}\right) ^{3}\right) $, $ \Psi _{k}\left(0, 0, \varphi _{1}, \varphi _{2}\right) = 0 $.

The main dynamics of the system (2.5) is given by its first two equations

the other two describing only the rate of rotation of an orbit, and, because $ \omega _{1, 2}\left(0\right) \neq 0, $ they can be approximated by

Changing to $ \rho _{i} = r_{i}^{2}, $ $ i = 1, 2, $ and truncating the higher order terms, system (2.6) leads to the planar system

referred as the amplitude equations; $ \dot{\rho}_{i} = \frac{d\rho _{i}}{dt}, $ $ i = 1, 2. $

In the hypotheses

in ^[1], the system (2.4) has been transformed further into the following normal form

where the real functions $ \Phi _{k}, $ $ \Psi _{k} $ are smooth functions of their arguments and are $ 2\pi $-periodic in $ \varphi _{j}, $ $ \Phi _{k} = O\left(\left(r_{1}^{2}+r_{2}^{2}\right) ^{3}\right) $, $ \Psi _{k}\left(0, 0, \varphi _{1}, \varphi _{2}\right) = 0. $

Consequently, the truncated amplitude system obtained in ^[1] reads:

This system is analyzed in two cases, the "simple case" as $ p_{11}(0)p_{22}(0) > 0 $ and the "difficult case" as $ p_{11}(0)p_{22}(0) < 0 $.

The study of the generic Hopf-Hopf bifurcation is also done in ^[2], starting with the amplitude system (2.6), truncated up to third order terms, in the nondegeneracy hypotheses

and

In this paper we study the degenerate Hopf-Hopf bifurcation when conditions (HH.1) and (HH.3) are not satisfied. In order to do this, we derive a new normal form for the amplitude system.

Theorem 2.2. Assume that the following three generic conditions are satisfied:

$\textrm{(HH.2)}$ $ a_{2}\left(0\right) = Re\left[ G_{1011}\left(0\right) \right] \neq0, $

$\textrm{(HH.4)}$ $ c_{2}\left(0\right) = Re\left[ H_{0021}\left(0\right) \right] \neq0, $

$\textrm{(HH.5)}$ the map $ \alpha \mapsto \left(\mu _{1}\left(\alpha \right), \mu_{2}\left(\alpha \right) \right) $ is regular at $ \alpha = 0. $

Then, system $ (2.6) $ is locally topologically equivalent around theorigin $ O $, for all sufficiently small $ \left\vert \alpha \right\vert $, tothe following system

where

and

with $ n_{2}\left(\mu \right) = -\frac{a_{5}\left(\mu \right) }{a_{2}\left(\mu\right) } $ and $ n_{1}\left(\mu \right) = -\frac{c_{4}\left(\mu \right)+c_{1}\left(\mu \right) n_{2}\left(\mu \right) }{c_{2}\left(\mu \right) }, $ where $ p_{ij} = p_{ij}\left(\mu \right) $ for $ i = 1, 2 $ and $ j = \overline{1, 5}, $ are well-defined and smooth functions for all $ \left\vert \mu\right\vert $ small enough.

Proof. We rescale the time by

where $ n_{i} = n_{i}\left(\alpha \right), $ $ i = \overline{1, 2}, $ are smooth functions which will be determined later. Then, system (2.6) is orbitally equivalent to the system

where $ p_{11} = a_{1}+\mu _{1}n_{1}, $ $ p_{12} = a_{2}+\mu _{1}n_{2}, $ $ p_{13} = a_{3}+a_{1}n_{1} $, $ p_{14} = a_{4}+a_{1}n_{2}+a_{2}n_{1}, $ and $ p_{15} = a_{5}+a_{2}n_{2} $, respectively $ p_{21} = c_{1}+\mu _{2}n_{1}, $ $ p_{22} = c_{2}+\mu _{2}n_{2}, $ $ p_{23} = c_{3}+c_{1}n_{1}, $ $ p_{24} = c_{4}+c_{1}n_{2}+c_{2}n_{1} $ and $ p_{25} = c_{5}+c_{2}n_{2}. $

Since $ a_{2}\left(0\right) \neq 0 $ and $ c_{2}\left(0\right) \neq 0, $ one can nullify the coefficients $ p_{15} $ and $ p_{24} $ by taking $ n_{2}\left(\alpha \right) = -\frac{a_{5}\left(\alpha \right) }{a_{2}\left(\alpha \right) } $ and $ n_{1}\left(\alpha \right) = -\frac{c_{4}\left(\alpha \right) +c_{1}\left(\alpha \right) n_{2}\left(\alpha \right) }{c_{2}\left(\alpha \right) }. $ Notice that $ n_{1}\left(\alpha \right) $ and $ n_{2}\left(\alpha \right) $ are well-defined for all $ \left\vert \alpha \right\vert $ small enough, and so are $ p_{ij}\left(\alpha \right). $

Assume the map $ \alpha \mapsto \left(\mu _{1}\left(\alpha \right), \mu _{2}\left(\alpha \right) \right) $ is regular at $ \alpha = 0, $ that is,

From the Inverse Function Theorem this condition ensures that for any $ \mu _{1}, \mu _{2}\in \mathbb{R} $ with $ \left\vert \mu \right\vert = \sqrt{\mu _{1}^{2}+\mu _{2}^{2}} $ small enough, there exist $ \alpha _{1} = \alpha _{1}\left(\mu \right) $ and $ \alpha _{2} = \alpha _{2}\left(\mu \right) $ which are obtained locally from the system

This implies that, one can consider further $ \mu = \left(\mu _{1}, \mu _{2}\right) $ as the parameter of the system. Thus, $ \omega _{1, 2} = \omega _{1, 2}\left(\mu \right), $ $ a_{i} = a_{i}\left(\mu \right), $ $ b_{i} = b_{i}\left(\mu \right), $ $ c_{i} = c_{i}\left(\mu \right), $ $ d_{i} = d_{i}\left(\mu \right), $ $ p_{ij} = p_{ij}\left(\mu \right), $ $ i = 1, 2, $ $ j = \overline{1, 5}. $ Since $ \mu _{1, 2}\left(0\right) = 0, $ the properties $ \omega _{1}\left(0\right) = \omega _{10} > 0 $ and $ \omega _{2}\left(0\right) = \omega _{20} > 0 $ are preserved. The theorem is proved.

Remark that the coefficients $ p_{ij} $ in system (2.13) are not the same as the ones in system (2.10), but the values $ p_{11}\left(0\right), $ $ p_{12}\left(0\right), $ $ p_{21}\left(0\right), $ $ p_{22}\left(0\right) $ are the same as in system (2.8).

The truncated 4D system of system (2.13) reads

where $ p_{12}(0)p_{22}(0)\neq 0, $ because $ p_{12}(0) = a_{2}(0)\neq 0, \; \ $from (HH.2) and $ p_{22}(0) = c_{2}\left(0\right) \neq 0, $ from (HH.4).

We aim to tackle in this work the normal form system (2.13), truncated up to third order terms, when $ a_{2}\left(0\right) c_{2}\left(0\right) > 0. $

Assume $ p_{12}\left(0\right) = a_{2}\left(0\right) < 0 $ and $ p_{22}\left(0\right) = c_{2}\left(0\right) < 0. $ Make the changes

The transformation $ \left(\rho _{1}, \rho _{2}\right) \longmapsto \left(\xi _{1}, \xi _{2}\right) $ is well defined for all $ \left\vert \mu \right\vert $ small enough, because $ p_{12}\left(0\right) \neq 0 $ and $ p_{22}\left(0\right) \neq 0 $ are satisfied from (HH.2) and (HH.4), and it is nonsingular as $ a_{2}\left(0\right) c_{2}\left(0\right) \neq 0. $ Since $ \frac{d\xi _{1}}{dt} = -p_{12}\left(\mu \right) \frac{d\rho _{1}}{ d\tau }\frac{d\tau }{dt} $ and $ \frac{d\xi _{2}}{dt} = -p_{22}\left(\mu \right) \frac{d\rho _{2}}{d\tau }\frac{d\tau }{dt}, $ the form system (2.13) in its lowest terms in $ \left(\xi _{1}, \xi _{2}\right) $, known as the truncated form, becomes $ \ $

where $ \theta \left(\mu \right) = \frac{p_{11}}{p_{12}}\left(\mu \right), $ $ \gamma \left(\mu \right) = \frac{p_{12}}{p_{22}}\left(\mu \right), $ $ M\left(\mu \right) = \frac{p_{14}}{p_{12}p_{22}}\left(\mu \right), $ $ N\left(\mu \right) = \frac{p_{13}}{p_{12}^{2}}\left(\mu \right), $ $ \delta \left(\mu \right) = \frac{p_{21}}{p_{12}}\left(\mu \right), $ $ S\left(\mu \right) = \frac{p_{23}}{p_{12}^{2}}\left(\mu \right) $ and $ P\left(\mu \right) = \frac{p_{25}}{p_{22}^{2}}\left(\mu \right) $. However, in what follows, some of these expressions are needed only at $ \mu = 0.\ $

The dot over quantities stands now for the derivatives with respect to the new time. Denote also by $ f $ the smooth vector field associated with system (2.16).

Remark 1. Due to the transformation system (2.15), the stability of an equilibrium point in the 2D system (2.16) is preserved in the 4D system (2.14); see also ^[1].

Remark 2. 1) Notice that $ \theta (0) = \frac{a_{1}\left(0\right) }{ a_{2}\left(0\right) }, $ $ \gamma (0) = \frac{a_{2}\left(0\right) }{ c_{2}\left(0\right) } $ and $ \delta (0) = \frac{c_{1}\left(0\right) }{ a_{2}\left(0\right) } $ are well-defined from (HH.2), (HH.4). Moreover, $ \gamma (0) > 0 $ while $ \theta (0)\delta (0) $ can be $ 0. $

2) When $ p_{12}\left(0\right) > 0 $ and $ p_{22}\left(0\right) > 0, $ the change $ \xi _{1} = p_{12}\left(\mu \right) \rho _{1}, $ $ \xi _{2} = p_{22}\left(\mu \right) \rho _{2}, $ $ t = -2\tau $ lead to

that can be reduced to system (2.16).

As $ \rho _{i} = r_{i}^{2}\geq 0, $ $ i = 1, 2, $ taking into account system (2.15) and the fact that $ p_{12}\left(0\right) < 0, $ $ p_{22}\left(0\right) < 0, $ it follows that the system (2.16) must be studied only on the set (the first quadrant)

Thus, only the equilibria of system (2.16) situated in $ D $ will be analyzed.

Remark 3. The lines $ \xi _{1} = 0 $ and $ \xi _{2} = 0 $ are invariant curves for system (2.16), thus the set $ D $ is invariant with respect to the dynamical system associated to system (2.16).

In the following we analyze the truncated amplitude system (2.16) in two cases when the Hopf-Hopf bifurcation degenerates, namely (ⅰ) $ \delta \left(0\right) = 0, $ $ \theta (0)\neq 0; $ (ⅱ) $ \theta \left(0\right) = 0, $ $ \delta (0)\neq 0. $

Remind that system (2.16) which is analyzed in the next two sections was obtained in the hypotheses (HH.0), (HH.2), (HH.4), (HH.5), and, as $ p_{12}(0) < 0 $, $ p_{22}(0) < 0 $, we have $ \gamma(0) > 0 $.

In addition, our study is done for $ \mu $ in a neighborhood of the origin $ V_{\varepsilon } $ given by $ \left\vert \mu \right\vert < \varepsilon $, for $ \varepsilon > 0 $ sufficiently small.

As all the coefficients are smooth functions depending on the parameter $ \mu $, for $ \mu \in V_{\varepsilon } $ we can write

and similar for the other coefficients. In all the expressions below only the significant lower order terms in $ \mu_1, \mu_2 $ will be considered, as necessary. Also, in order to save symbols, if, for instance we have $ N(0)\ne 0 $ we shall denote $ N = N(0), $ thus, close to $ \mu = 0 $, we have $ N(\mu) = N+O(|\mu |), $ and so on for the other coefficients.

3.   Analysis of system (2.16) when $ \delta (0)=0 $ and $ \theta (0)\neq 0 $

Assume that $ \delta (0) = 0, $ $ \theta (0)\neq 0. $ This means that condition (HH.3) is not satisfied, but (HH.1) is valid.

Then $ \delta (\mu) = \delta _{1}\mu _{1}+\delta _{2}\mu _{2}+O(\left\vert \mu \right\vert ^{2}), $ with $ \delta _{1} = \frac{ \partial \delta }{\partial \mu _{1}}\left(0\right), $ $ \delta _{2} = \frac{ \partial \delta }{\partial \mu _{2}}\left(0\right). $ One of the equilibria of system (2.16) is $ E_{0} = \left(0, 0\right). $ Two more equilibria

where $ \theta = \theta(0) $, bifurcate from $ E_{0} $ as soon as $ \mu _{1}\neq 0, $ respectively, $ \mu _{2}\neq 0 $, i.e. at the bifurcation lines

and

respectively. Their stability is described by Lemma 3.1.

Lemma 3.1. (1) The eigenvalues of $ E_{0} $ are $ \mu _{1} $ and $ \mu _{2}. $

(2) The eigenvalues of $ E_{1} $ are $ -\mu _{1}+O\left(\mu _{1}^{2}\right) $and $ \left(\mu _{2}+\frac{S-\delta _{1}\theta }{\theta ^{2}}\mu_{1}^{2}\right) \left(1+O\left(\mu _{1}\right) \right) $, thus, whenever $ E_{1} $ lies in $ D, $ $ E_{1} $ is either (i) a saddle as $ \mu _{1}\left(\mu_{2}+\frac{S-\delta _{1}\theta }{\theta ^{2}}\mu _{1}^{2}\right) > 0, $ (ii)an unstable node as $ \mu _{2}+\frac{S-\delta _{1}\theta }{\theta ^{2}}\mu_{1}^{2} > 0, \mu _{1} < 0, \ $(iii) a stable node as $ \mu _{2}+\frac{S-\delta_{1}\theta }{\theta ^{2}}\mu _{1}^{2} < 0, \mu _{1} > 0. $

(3) The eigenvalues of $ E_{2} $ are $ \mu _{1}-\gamma \mu _{2}+O(\mu _{2}^{2}) $and $ -\mu _{2}+O(\mu _{2}^{2}). $ Therefore, for $ \left\vert \mu \right\vert $sufficiently small, $ \ $ $ E_{2} $ is (i) a saddle as $ \mu _{1}-\gamma \mu_{2} > 0, $ (ii) a stable node as $ \mu _{1}-\gamma \mu _{2} < 0, $ whenever itexists in $ D. $

On the other hand, system (2.16) has the nontrivial equilibrium $ E_{3} = \left(\xi _{1}^{\ast }, \xi _{2}^{\ast }\right), $ with

The associated eigenvalues satisfy

When $ \theta > 0, $ $ E_{3} $ lies in $ D $ for parameters in the region

It is easy to see that for $ \mu \in R_{1}, $ with $ \left\vert \mu \right\vert $ sufficiently small, we have $ \lambda _{1}\lambda _{2} > 0 $ and the curve $ \lambda _{1}+\lambda _{2} = 0 $ does not cross region $ R_{1}, $ thus $ \lambda _{1}+\lambda _{2} < 0, $ and $ E_{3} $ is stable.

When $ \theta < 0, $ $ E_{3} $ lies in $ D $ for parameters in region

By system (3.1), it follows $ \lambda _{1}\lambda _{2} < 0, $ for sufficiently small $ \left\vert \mu \right\vert. $ Thus, $ E_{3} $ is a saddle. The following result is proved.

Lemma 3.2. If $ \theta < 0, $ then $ E_{3} $ is a saddle, while if $ \theta > 0, $ the equilibrium $ E_{3} $ is a hyperbolic attractor, for allsufficiently small $ \left\vert \mu \right\vert, $ for which $ E_{3} $ lies in $ D. $

Consequently there can be no Hopf bifurcation at $ E_{3}. $

As the term $ \delta _{2} $ does not influence the topological type of equilibria, we may restrict our attention only to the $ \left(\theta, \delta _{1}\right) - $ plane.

Regions $ R_{1} $ and $ R_{2} $ are delimitated by the curves

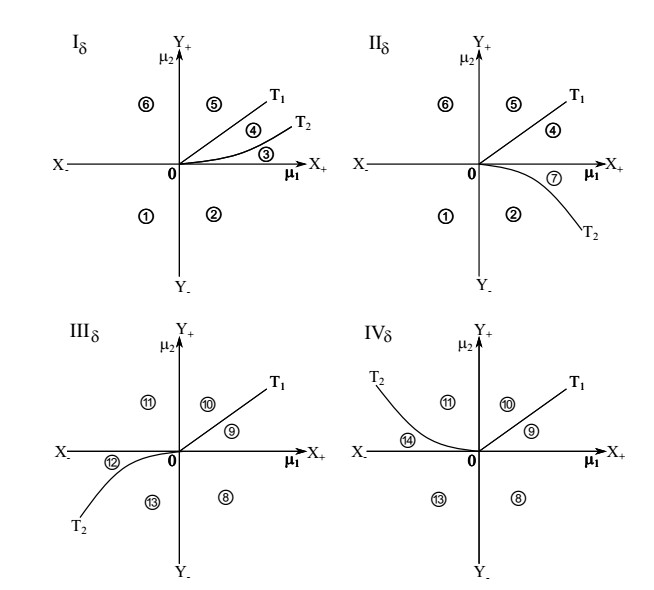
We notice that $ E_{3} $ collides with $ E_{2} $ on $ T_{1}, $ and it collides with $ E_{1} $ on $ T_{2}. $

Proposition 3.3. System $ (2.16) $ experiences the following transcritical bifurcations:

(i) at the point $ E_{0} $ as the parameter $ \mu _{1} $ varies through thebifurcation value $ \mu _{1} = 0, $ for a fixed $ \mu _{2}\neq 0 $ (when $ E_{0} = E_{1}); $

(ii) at the point $ E_{0} $ as the parameter $ \mu _{2} $ varies through thebifurcation value $ \mu _{2} = 0, $ for a fixed $ \mu _{1}\neq 0 $ (when $ E_{0} = E_{2}); $

(iii) at the point $ E_{1} $ as the parameter $ (\mu _{1}, \mu _{2}) $ crossesthe curve $ T_{2} $ (when $ E_{1} = E_{3}); $

(iv) at the point $ E_{2} $ as the parameter $ (\mu _{1}, \mu _{2}) $ crosses thecurve $ T_{1} $ (when $ E_{2} = E_{3}). $

Proof. We apply Sotomayor Theorem (^[13,14], to prove these statements.

(ⅰ) The Jacobian matrix $ Df(E_{0}, \mu _{0}) $ at $ \mu _{0} = \left(0, \mu _{2}\right), $ $ \mu _{2}\neq 0, $ has a zero eigenvalue with the right eigenvector $ v = \left(1, 0\right) ^{T} $ and the left eigenvector $ w = \left(1, 0\right) ^{T}. $ It follows

thus the transcritical bifurcation conditions are satisfied.

(ⅱ) The Jacobian matrix $ Df(E_{0}, \mu _{0}) $ at $ \mu _{0} = \left(\mu _{1}, 0\right), $ $ \mu _{1}\neq 0, $ has a zero eigenvalue with the right eigenvector $ v = \left(0, 1\right) ^{T} $ and the left eigenvector $ w = \left(0, 1\right) ^{T}. $ It follows

ensuring the existence of a transcritical bifurcation.

(ⅲ) Consider $ \mu _{0}\in T_{2}, $ $ \mu _{1}\neq 0, $ and $ \mu _{2} $ as a bifurcation parameter. We find that $ v = (-\gamma, \theta)^{T} $ and $ w = (0, 1)^{T} $ are right and left eigenvectors of the Jacobian matrix $ Df(E_{1}, \mu _{0}), $ respectively, corresponding to the zero eigenvalue, and

consequently, for sufficiently small $ \left\vert \mu \right\vert, \ $the conditions are satisfied.

(ⅳ) Finally, consider $ \mu _{0}\in T_{1}, $ $ \mu _{2}\neq 0, $ and $ \mu _{1} $ as a bifurcation parameter, thus $ \mu _{0} = (\gamma \mu _{2}, \mu _{2}) $. We find the eigenvectors $ v = (1, -\delta)^{T} $ and $ w = (1, 0)^{T}, $ and

for sufficiently small $ \left\vert \mu \right\vert. $

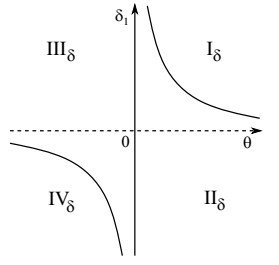
For a fixed $ \gamma > 0, $ and $ S > 0, $ the curves $ S-\delta _{1}\theta = 0, $ $ \theta = 0, $ determine four regions in the $ \left(\theta, \delta _{1}\right) - $ plane, illustrated in Figure 1, corresponding to the following cases:

Ⅰ$ _{\delta } $: $ \theta > 0, $ $ S-\delta _{1}\theta < 0; $

Ⅱ$ _{\delta } $: $ \theta > 0, \ $ $ S-\delta _{1}\theta > 0; $

Ⅲ$ _{\delta } $: $ \theta < 0, \ $ $ S-\delta _{1}\theta > 0; $

Ⅳ$ _{\delta } $: $ \theta < 0, \ $ $ S-\delta _{1}\theta < 0. $

For each region Ⅰ$ _{\delta } $-Ⅳ$ _{\delta }, $ in the parametric portraits in the $ \left(\mu _{1}, \mu _{2}\right) $- plane, the parameter strata are determined by the origin and the bifurcation curves $ X_{-}, $ $ X_{+}, $ $ Y_{-}, $ $ Y_{+}, $ $ T_{1}, $ and $ T_{2}. $ Consequently, the following result is obtained.

Theorem 3.4. For all $ \gamma > 0, S > 0, $ in the $ \left(\theta, \delta_{1}\right) - $ plane, the bifurcation curves consist of

The four parameter portraits for $ (\theta, $ $ \delta _{1}) $ in regions Ⅰ$ _{\delta } $, Ⅱ$ _{\delta } $, Ⅲ$ _{\delta } $, Ⅳ$ _{\delta } $ are shown in Figure 2. The 14 generic phase portraits are given in Figure 3.

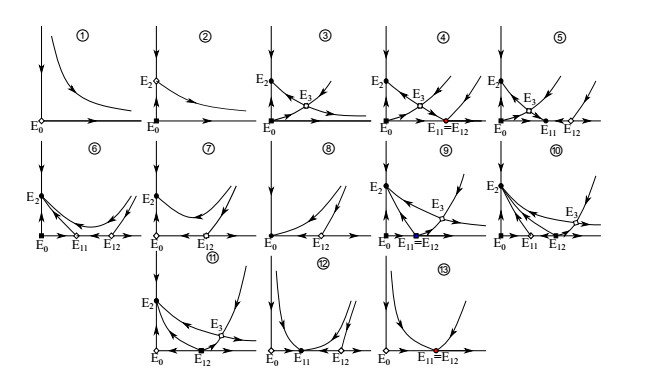
In Figure 3 we used the following markers to emphasize the topological type of the equilibria: a black disc for an attractor, a black square for a repeller and a diamond for a saddle point. All of these phase portraits are also found in the nondegenerate double-Hopf bifurcation case ^[1,2]. As $ S\leq 0, $ a similar study can be done.

Remark 4. As proved in Proposition 3.3, each of the curves $ X_{-}, $ $ X_{+}, $ $ Y_{-}, $ $ Y_{+}, $ $ T_{1}, $ $ T_{2} $ consists of values of transcritical bifurcation (for which two of the four equilibria collide and change topological type), separating two parameter strata, one where both equilibria involved in the transcritical bifurcation lie on $ D $ and one where only one of them lies on $ D. $ Although for parameters on a bifurcation curve the corresponding equilibrium is a saddle-node, the generic phase portrait is equivalent to the one of the stratum where only one of the two equilibria is in $ D. $

4.   Analysis of system (2.16) when $ \theta (0)=0 $ and $ \delta (0) \neq 0 $

Assume $ \theta (0) = 0, $ $ \delta (0)\neq 0. $ In this case the condition (HH.1) is not satisfied, and (HH.3) is valid. Then $ \theta (\mu) = \theta _{1}\mu _{1}+\theta _{2}\mu _{2}+O(\left\vert \mu \right\vert ^{2}). $

In this case, two trivial equilibria of system (2.16) are $ E_{0} = \left(0, 0\right), $ with the eigenvalues $ \mu _{1} $ and $ \mu _{2}, $ and $ E_{2} = \left(0, \mu _{2}+O\left(\mu _{2}^{2}\right) \right) $ with eigenvalues $ \mu _{1}-\gamma \mu _{2}+O(\left\vert \mu\right\vert ^2) $ and $ -\mu _{2} +P\mu _{2}^{2}(1+O(\left\vert \mu\right\vert)) $. Remark that these equilibria keep the same form as in the case treated in the previous section, and their stability remains as described by Lemma 3.1.

As $ \xi _{2} = 0, $ the equation giving the equilibrium points reads:

Assume that $ N(0)\neq 0, $ thus $ N\left(\mu \right) \neq 0 $ for sufficiently small $ \left\vert \mu \right\vert. $ If $ \Delta (\mu) = \theta ^{2}(\mu)-4N(\mu)\mu _{1}\geq 0, $ Eq (4.1) has two real solutions

Thus, as $ \mu _{1}N > 0, $ two equilibrium points, $ E_{11} = \left(\xi _{11}, 0\right) $ and $ E_{12} = \left(\xi _{12}, 0\right), $ are lying on the positive $ \xi _{1}- $axis, provided that $ \left(\mu _{1}, \mu _{2}\right) \in R_{3}, $ where $ R_{3} $ is a region in the parametric plane given by

Obviously, as $ \Delta \left(\mu \right) = 0, $ we have $ E_{11} = E_{12} = \frac{ \theta }{2N}. $

As $ \mu _{1}N < 0, $ we have $ \xi _{11} < 0 < \xi _{12} $, thus, only the equilibrium $ E_{12} $ lies in D for parameters in region

As $ \mu _{1} = 0, $ we have $ E_{11} = E_{0} $ if $ \theta _{2}\mu _{2}N > 0, $ while $ E_{12} = E_{0} $ if $ \theta _{2}\mu _{2}N < 0. $ Using the Implicit Functions Theorem, we find that, for sufficiently small $ \left\vert \mu \right\vert, $ $ \Delta \left(\mu \right) = 0 $ for parameters on the curve, denoted also by $ \Delta, $ given as

The eigenvalues of $ E_{11} = \left(\xi _{11}, 0\right) $ satisfy $ \lambda _{1}^{E_{11}} = -\xi _{11}\sqrt{\Delta \left(\mu \right) }\leq 0, $ and

For $ E_{12} = \left(\xi _{12}, 0\right) $ we have $ \lambda _{1}^{E_{12}} = \xi _{12}\sqrt{\Delta \left(\mu \right) }\geq 0 $ and

An important relation for studying the behavior of $ E_{11} $ and $ E_{12} $ is

Note that as $ \lambda _{2}^{E_{11}}\lambda _{2}^{E_{12}} = 0, $ we get

Lemma 4.1. As the parameter $ \mu $ crosses the curve $ \Delta, $ asaddle-node bifurcation takes place. In addition, if $ N > 0, $ for parameter sufficiently small, close to the curve $ \Delta, $ we have:

(i) $ E_{11} $ is an attractor and $ E_{12} $ is a saddle as $ \mu _{2}\left(2N-\delta \theta _{2}\right) < 0; $

(ii) $ E_{11} $ is a saddle and $ E_{12} $ is a repeller as $ \mu _{2}\left(2N-\delta \theta _{2}\right) > 0. $

Proof. Consider $ \mu _{0}\in \Delta. $ Then $ \xi _{11} = \xi _{12} = \frac{ \theta _{2}}{2N}\mu _{2}+O(\mu _{2}^{2}), $ and the eigenvalues of equilibrium $ E_{11} $ are $ \lambda _{1} = 0 $, $ \lambda _{2} = \frac{2N-\delta \theta _{2}}{2N}\mu _{2}+O(\mu _{2}^{2}). $ The Jacobian matrix $ Df(E_{11}, \mu _{0}), $ has for the zero eigenvalue the right eigenvector $ v = \left(1, 0\right) ^{T} $ and the left eigenvector $ w = \left(2N-\delta \theta _{2}, \gamma \theta _{2}\right) ^{T}. $ It follows

for sufficiently small $ \left\vert \mu \right\vert. $ Thus, according to Sotomayor Theorem, a saddle-node bifurcation takes place.

For parameters close to the bifurcation curve we have $ sign(\lambda _{1}^{E_{11}}) = -sign(N), $ $ sign(\lambda _{1}^{E_{12}}) = sign(N), $ and $ sign(\lambda _{2}^{E_{11}}) = sign(\lambda _{2}^{E_{12}}) = sign(\frac{2N-\delta \theta _{2}}{2N}\mu _{2}). $ Consequently, if $ N > 0, $ the equilibrium $ E_{11} $ is an attractor and $ E_{12} $ is a saddle as $ \mu _{2}\left(2N-\delta \theta _{2}\right) < 0, $ while as $ \mu _{2}\left(2N-\delta \theta _{2}\right) > 0, $ the equilibrium $ E_{11} $ is a saddle and $ E_{12} $ is a repeller.

Denote by

and

As a consequence of Lemma 4.1, for a parameter $ \mu $ in $ \Delta _{+}, $ sufficiently small, the equilibrium $ E_{11}( = E_{12}) $ is a saddle-node, with one attractive direction and three repelling directions, while for a parameter $ \mu $ in $ \Delta _{-}, $ the equilibrium $ E_{11} $ is a saddle-node, with one repelling direction and three attractive ones.

Consider the curves

Proposition 4.2. The following statements hold:

(i) for $ \mu \in Y_{+}^{\prime }, $ equilibria $ \ E_{0} $ and $ E_{11} $coincide; when parameters cross this curve, a transcritical bifurcationtakes place, and $ E_{0} $ and $ E_{11} $ interchange the topological type;

(ii) for $ \mu \in Y_{-}^{\prime }, $ equilibria $ \ E_{0} $ and $ E_{12}\ $coincide and are saddle-nodes. A transcritical bifurcation takes place whenparameters are crossing the curve $ Y_{-}^{\prime }, $ and $ E_{0} $ and $ E_{12} $interchange the topological type.

(iii) for $ \mu \in \left\{ \left(\mu _{1}, \mu _{2}\right), \mu _{2} = 0, \mu_{1}\neq 0\right\}, $ equilibria $ \ E_{0} $ and $ E_{2}\ $coincide and atranscritical bifurcation takes place when parameters are crossing thiscurve.

Proof. As in Proposition 3.3, we apply Sotomayor Theorem (^[13]) to prove these statements.

(ⅰ) Consider $ \mu _{0}\in Y_{+}^{\prime }. $ As $ \mu _{1} = 0 $ and $ \theta _{2}\mu _{2}N > 0, $ it follows that $ E_{11} = E_{0} $. The Jacobian matrix $ Df(E_{0}, \mu _{0}) $ at $ \mu _{0} = \left(0, \mu _{2}\right), $ $ \mu _{2}\neq 0, $ has a zero eigenvalue with the right eigenvector $ v = \left(1, 0\right) ^{T} $ and the left eigenvector $ w = \left(1, 0\right) ^{T}. $ It follows

for sufficiently small $ \left\vert \mu \right\vert, $ thus the transcritical bifurcation conditions are satisfied.

(ⅱ) Consider $ \mu _{0}\in Y_{-}^{\prime }. $ As $ \mu _{1} = 0 $ and $ \theta _{2}\mu _{2}N < 0, $ it follows that $ \xi _{12} = 0, $ thus $ E_{12} = E_{0} $. We obtain the same values for the quantities involved in the transcritical bifurcation conditions as in case (i).

(ⅲ) The Jacobian matrix $ Df(E_{0}, \mu _{0}) $ at $ \mu _{0} = \left(\mu _{1}, 0\right), $ $ \mu _{1}\neq 0, $ has a zero eigenvalue with the right eigenvector $ v = \left(0, 1\right) ^{T} $ and the left eigenvector $ w = \left(0, 1\right) ^{T}. $ It follows

ensuring the existence of a transcritical bifurcation.

For $ E_{3} = \left(\xi _{1}^{\ast }, \xi _{2}^{\ast }\right), $ solution of system

we obtain

Thus, $ E_{3} $ is a nontrivial equilibrium for system (2.16) provided

Denote by

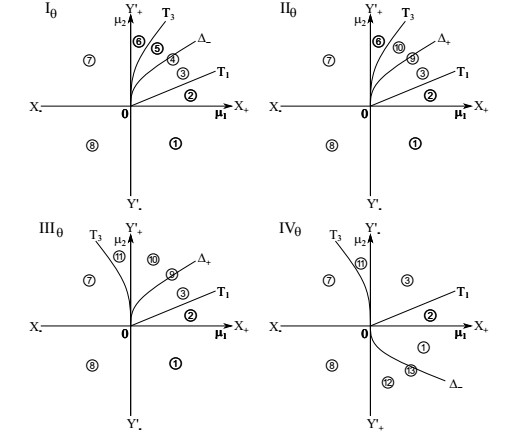
the parameter curve for which $ \xi _{2}^{\ast } = 0. $ Thus $ R_{5} $ is delimited by $ T_{1} $ and $ T_{3} $.

The eigenvalues of $ E_{3} $ satisfy

where $ p(\mu) = -\delta ^{2}\mu _{1}\left(1+O\left(\left\vert \mu \right\vert \right) \right) +k_{1}\mu _{2}^{2}\left(1+O\left(\left\vert \mu \right\vert \right) \right) $ and $ k_{1} = \left(\theta _{2}\delta -N\right) +\gamma \left(2N-\theta _{2}\delta \right). $

Applying Implicit Functions Theorem to the implicit equation $ p\left(\mu _{1}, \mu _{2}\right) = 0, $ the following lemma is obtained.

Lemma 4.3. If $ (\theta _{2}\delta -N)+\gamma (2N-\theta _{2}\delta)\neq0, $ there exists a neighborhood $ U $ of $ 0 $ in the parameter plane such that $ p\left(\mu \right) = 0 $ in $ U $ iff $ \mu \in U\cap H, $ where

for $ \left\vert \mu \right\vert $ sufficiently small.

As a consequence we obtain the following result concerning the topological type of the nontrivial equilibrium $ E_{3}. $

Lemma 4.4. Assume $ N > 0. $ For sufficiently small $ \left\vert \mu\right\vert, $ the following statements hold:

(1) If $ \delta > 0, $ then $ E_{3} $ is a saddle.

(2) If $ \delta < 0, $ $ \theta _{2}\delta -2N\geq 0, $ then $ E_{3} $ is ahyperbolic attractor.

(3) If $ \delta < 0, $ $ \theta _{2}\delta -2N < 0, $ then the equilibrium $ E_{3} $is a hyperbolic repeller as $ \delta ^{2}\mu _{1} < [\gamma (2N-\theta_{2}\delta)+(\theta _{2}\delta -N)]\mu _{2}^{2}, $ and a hyperbolicattractor for the other parameters in $ R_{5}\backslash H, $

A similar result can be obtained if $ N < 0. $ We explore next the local bifurcations at the equilibrium $ E_{3}. $

Proposition 4.5. The following statements hold:

(i) For $ \mu _{0}\in T_{1}, $ equilibria $ E_{2} $ and $ E_{3} $ coincide. Whenparameter $ \mu $ crosses $ T_{1} $ a transcritical bifurcation takes place at $ E_{3}.$

(ii) For $ \mu _{0}\in T_{3}, $ $ E_{3} $ coincides either with $ E_{11} $ if $ \frac{2N-\theta _{2}\delta }{2N\delta }\mu _{2} < 0, $ or with $ E_{12} $ if $ \frac{2N-\theta _{2}\delta }{2N\delta }\mu _{2} > 0. $

(iii) Assume $ 2N-\delta \theta _{2}\neq 0, $ and $ \frac{\partial \delta }{\partial \mu _{1}}\neq 0. $ Then, when parameter $ \mu $ crosses $ T_{3}, $ atranscritical bifurcation takes place at $ E_{3}. $

Proof. (ⅰ) Consider $ \mu _{0}\in T_{1}, $ $ \mu _{0} = (\gamma \mu _{2}+O(\mu _{2}^{2}), \mu _{2}), $ with $ \mu _{2} > 0. $ Applying Sotomayor theorem, we obtain the right eigenvector $ v = \left(1, -\delta \right) ^{T} $ and the left eigenvector $ w = \left(1, 0\right) ^{T}, \ $and

for $ \left\vert \mu _{0}\right\vert $ sufficiently small. Thus the transcritical bifurcation conditions are satisfied.

(ⅱ) Consider $ \mu _{0}\in T_{3}, $ sufficiently small. Then $ \mu _{0} = \left(\frac{\theta _{2}\delta -N}{\delta ^{2}}\mu _{2}^{2}+O(\mu _{2}^{3}), \mu _{2}\right), $ $ \mu _{2}\neq 0. $ We obtain $ \xi _{1}^{\ast } = \frac{1}{\delta }\mu _{2}+O(\mu _{2}^{2})\neq 0, $ $ \xi _{2}^{\ast } = 0, $ and

consequently, $ E_{3} = E_{12} $ if $ \frac{2N-\theta _{2}\delta }{2N\delta }\mu _{2} > 0, $ and $ E_{3} = E_{11} $ if $ \frac{2N-\theta _{2}\delta }{2N\delta }\mu _{2} < 0. $

(ⅲ) Consider $ \mu _{0}\in T_{3}, $ sufficiently small. In order to prove the existence of a transcritical bifurcation, we choose $ \mu _{1} $ as the bifurcation parameter. Write $ \delta $ as

The Jacobian matrix $ Df(E_{3}, \mu _{0}) $ has a zero eigenvalue with the right eigenvector

and the left eigenvector $ w = \left(0, 1\right) ^{T}. $ It follows

for sufficiently small $ \left\vert \mu \right\vert, $ ensuring the existence of a transcritical bifurcation.

From Lemma 4.4 it follows that system (2.16) may exhibit a Hopf bifurcation at $ E_{3} $.

Remark 5. The above analysis shows that the term $ \theta _{1} $ does not influence the topological type of the equilibria, and only the coefficients $ \gamma, \delta, \theta _{2} $ and $ N $ are significant, for sufficiently small $ \left\vert \mu \right\vert. $

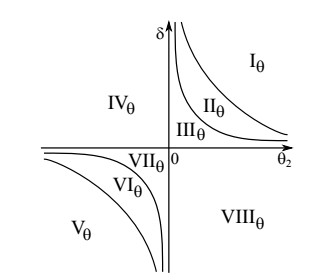
For a fixed $ \gamma > 0, $ and $ N > 0, $ the curves $ \delta = 0, $ $ \theta _{2} = 0, \theta _{2}\delta -N = 0, $ $ \theta _{2}\delta -2N = 0\ $determine eight regions in the $ \left(\theta _{2}, \delta \right) - $ plane (see Figure 4), corresponding to the following cases:

Ⅰ$ _{\theta } $: $ \theta _{2} > 0, \delta > 0, $ $ \theta _{2}\delta -2N > 0; $

Ⅱ$ _{\theta } $: $ \theta _{2} > 0, \delta > 0, \ \theta _{2}\delta -N > 0, $ $ \theta _{2}\delta -2N < 0; $

Ⅲ$ _{\theta } $: $ \theta _{2} > 0, \delta > 0, $ $ \theta _{2}\delta -N < 0; $

Ⅳ$ _{\theta } $: $ \theta _{2} < 0, \delta > 0, $ $ \theta _{2}\delta -N < 0; $

Ⅴ$ _{\theta } $: $ \theta _{2} < 0, \delta < 0, $ $ \theta _{2}\delta -2N > 0; $

Ⅵ$ _{\theta } $: $ \theta _{2} < 0, \delta < 0, $ $ \theta _{2}\delta -N > 0, \theta _{2}\delta -2N < 0; $

Ⅶ$ _{\theta } $: $ \theta _{2} < 0, \delta < 0, $ $ \theta _{2}\delta -N < 0; $

Ⅷ$ _{\theta } $: $ \theta _{2} > 0, \delta < 0, $ $ \theta _{2}\delta -N < 0. $

Theorem 4.6. For all $ \gamma > 0, N > 0, $ respectively, $ (\theta _{2}, \delta) $in regions I$ _{\theta } $, II$ _{\theta } $, III$ _{\theta } $, IV$ _{\theta } $, V$ _{\theta } $ in the $ \left(\theta _{2}, \delta \right) - $ plane, thebifurcation curves consist of

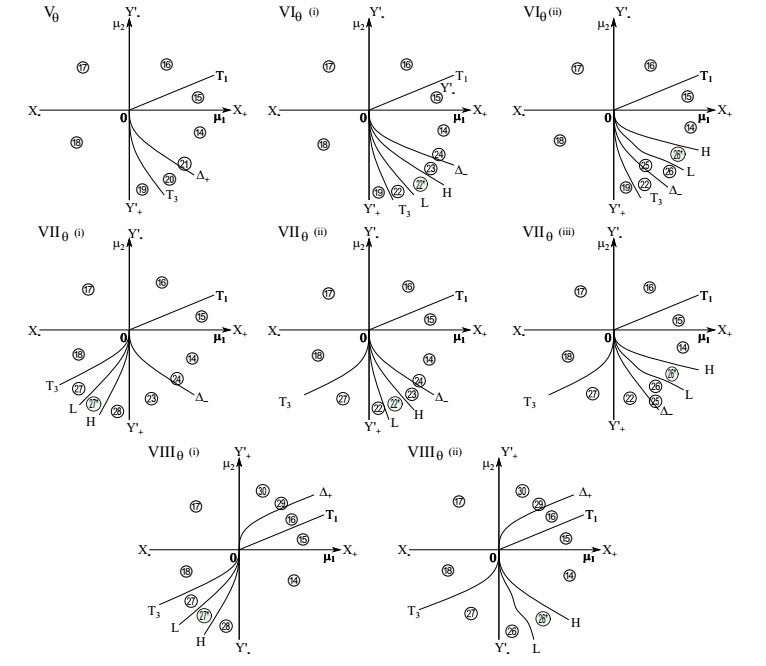
The generic parameter portraits are shown in Figures 5 and 7, and the corresponding generic phase portraits in Figures 6 and 8.

A Hopf bifurcation may occur when $ \left(\mu _{1}, \mu _{2}\right) $ crosses the curve $ H $ and $ \left(\theta _{2}, \delta \right) $ is situated in regions VI$ _{\theta } $, VII$ _{\theta } $, VIII$ _{\theta }. $ In the case when the first Lyapunov is non-zero, a limit cycle surrounding the equilibrium $ E_{3} $ appear. This cycle is stable if the Hopf bifurcation is supercritical (the first Lyapunov coefficient is negative) or is unstable if the Hopf bifurcation is subcritical (i.e., the first Lyapunov coefficient is positive). As the first Lyapunov coefficient is zero, the equilibrium $ E_{3} $ may be a nonlinear centre.

Remark that, as the parameters move away from $ H, $ towards the region containing the limit cycle generated by the Hopf bifurcation, the cycle may disappear, either by transforming into a homoclinic loop or by becoming too large, and no longer inside the visible neighborhood of the origin. In such cases there should exist a bifurcation curve $ L $ originating at $ \mu = 0, $ along which those situations may occur. The position of the curve $ L $ depends on the type of the Hopf bifurcation. These situations also appear in the "difficult case" in the nondegenerate double-Hopf bifurcation. The existence and location of the curve $ L $ can be proved following the lines in ^[1,2].

Theorem 4.7. For all $ \gamma > 0, N > 0, $ and $ (\theta _{2}, \delta) $ in regionsVI$ _{\theta } $, VII$ _{\theta } $, VIII$ _{\theta } $ in the $ \left(\theta_{2}, \delta \right) - $ plane, the bifurcation curves consist of

The generic parameter portraits are shown in Figure 7, in the hypothesis that the Lyapunov coefficient is negative. The corresponding generic phase portraits are given in Figure 8. Many of the phase portraits in Figures 6 and 8 cannot be found in the nondegenerate double-Hopf bifurcation.

The effect of adding higher-order terms to the truncated normal form system (2.16) and the correspondence between the properties of the 2D amplitude system (2.16) and the 4D system (2.1), have been described, for example, in ^{[1,2,15,16,17,18]}. However, the study of nonsymmetric general perturbations of the truncated normal forms is far from complete, as pointed out in ^[1].

Thus, we remark that the study of adding higher order terms to the truncated normal form is a complex open problem which is outside the aim of this article.

5.   Conclusions

The classical normal form of the Hopf-Hopf bifurcation, in differential systems of dimension four and having minimum two independent parameters, is based on six generic conditions (2.8).

In this work we have studied two degenerate Hopf-Hopf bifurcations, namely:

1) the case when the generic condition (HH.3) in system (2.8) is not fulfilled, treated in Section 3;

2) the case when the generic condition (HH.1) in system (2.8) is not fulfilled, treated in Section 4.

In our study we have obtained new bifurcation diagrams and new phase portraits which were not previously reported in generic studies on double-Hopf bifurcation. Such an example is a saddle-node bifurcation in the amplitude system which corresponds to a fold bifurcation of cycles in the four dimensional system and which is present only in the degenerate case $ \theta = 0 $.

The results describing the dynamics, contribute to a better understanding of the behavior of a system presenting a Hopf-Hopf singularity.

Finally, we summarize in the Table 1 the correspondence of equilibria, cycles and bifurcations between the 2D amplitude system (2.16) and the 4D normal form system (2.14):

Acknowledgments

This research was supported by Horizon2020-2017-RISE-777911 project.

Conflict of interest

The authors declare there is no conflicts of interest.