This work extends the concept of mate functions in nearrings to seminearrings and discusses the properties of mate functions. We obtain a complete characterisation of mate functions in a seminearring R. We show that every mate function φ of R gives rise to a mutual mate function for R. We derive a necessary and sufficient condition for a seminearring to possess a unique mutual mate function. We also obtain a necessary and sufficient condition for a seminearring to be a seminearfield vis-a-vis the behaviour of its mate functions.
1.
Introduction
In the literature seminearrings first appeared in Van Hoorn and Van Rootselaar, 1967[1]. Indeed, they have studied some fundamental properties of seminearrings and introduced various notions of ideals. Later, Albert Hoogewijs studied embeddings and I-congruences of seminearrings[2]. Weinert has made some significant contributions to seminearrings[3,4,5] and also studied non-associative seminearrings towards the contributions to seminearfields and seminearrings as ordered algebras. The theory was further enhanced by S.A. Huq[6], Javed Ahsan[7] and Tim Boykett[8]. The role of seminearring structure applied in many places of theoretical computer science, viz. algebra communicating processes, theory of automata and also seen in semigroup mapping and reversible computation models. The concept of regularity in rings was first introduced by Von Neumann[9]. Beidleman[10] and Ligh Steve[11] extended this concepts to nearrings. Each of these theories has its inherent difficulties. Thereafter Suryanarayanan[12] implemented the notion of mate functions in order to deal the regularity structure of nearring in a very easy way. Finally, Javed Ahsan proposed the study of regularity structure in seminearrings. Now it is quite natural for us to extend the concepts of mate function to seminearrings and handle the regularity structure in a simple manner. Moreover it is a useful device for studying certain lattices.
This paper comprises six sections. We review some basic results and definitions about seminearrings in Section 2. In Section 3, we give examples and study the properties of mate function in detail. A complete characterisation is obtained in Section 4 for a mapping ϕ from R to R as a mate function of R. Section 5, we also present the notion of mutual mate function of R. A necessary and sufficient condition for a seminearring R to possess a unique mutual mate function is obtained in the last section.
2.
Preliminaries
We consider some basic definitions related to seminearrings which are used in subsequent sections. By a seminearring we say that an algebraic system (R,+,.), where
(ⅰ) (R,+) is a semigroup
(ⅱ) (R,.) is a semigroup and
(ⅲ) (a+x)n=an+xn, ∀ a,x,n∈R (i.e. right distributite law).
In view of axiom (iii), what is defined above may more precisely a right seminearring. The theory runs parallel for a left seminearring, which is defined by replacing a(x+n)=ax+an ∀ a,x,n∈R (i.e. left distributite law) in axiom (iii)[13]. According [1], seminearrings is the common generalization of nearrings and semirings. R is a right(left) absorbing zero if r.0=0(0.r=0) holds for all r∈R. All along this paper, R always denotes a right seminearring with zero absorbing.
Let (Γ,+) be a semigroup with identity 0. Then the following sets of mappings from Γ to Γ are seminearrings under pointwise addition and composition of mappings.
(ⅰ) the set M(Γ) of all self-maps of Γ
(ⅱ) M0(Γ)={ϕ:Γ→Γ|ϕ(0)=0}
(ⅲ) Mc(Γ)={ϕ:Γ→Γ|ϕisaconstantmap}.
A seminearring R is called regular if for all h∈R there exists y∈R such that h=hyh [9]. A seminearring R is left[right] normal if for each a∈R, we have a∈Ra[a∈aR] and normal if it is both left and right normal[14]. A right seminearfield is a system (R,+,.) such that
(ⅰ) (R,+) is a semigroup
(ⅱ) (R∗,.) is a group (where R∗=R−{0}) and
(ⅲ) (l+m)n=ln+mn ∀ l,m,n∈R[3,8].
An element a∈R is called
(ⅰ) nilpotent, if an=0 for some integer n≥1
(ⅱ) idempotent, if a2=a (The set of all idempotents of R will be denoted by E)[14].
Suppose R has any non empty subset A, then
(ⅰ) C(A)= {x∈R/xa=axforalla∈A}
(ⅱ) C(R) is called the center of R
(ⅲ) when E⊆C(R), then we say that the idempotents are central[14].
A seminearring homomorphism between two right seminearrings R and R′ is a map ϕ:R→R′ satisfying ϕ(a+b)=ϕ(a)+ϕ(b) and ϕ(ab)=ϕ(a)ϕ(b) for all a,b∈R [14]. A semigroup (Γ,+) with zero is an R−semigroup if the composition is (x,γ)↣xγ of R×Γ→Γ such that
(ⅰ) (m+n)γ=mγ+nγ
(ⅱ) (mn)γ=m(nγ) and
(ⅲ) 0γ=0 for all m,n∈R,γ∈Γ.
It is clear that Γ is an R−semigroup with R=M(Γ). Also, the semigroup (R,+) of a seminearring (R,+,.) is an R−semigroup. A mapping h:A→B between R−semigroups A and B is a R-homomorphism if for every a,a1∈A and x∈R, h(a+a1)=h(a)+h(a1) and h(xa)=xh(a)[15,16]. A non empty subset A of a seminearring R is called a right (left) ideal if (i) for all x,y∈A, x+y∈A and (ii) for all x∈A and r∈R, xr(rx)∈A. The word ideal will always mean a subset of R which is both a left and a right ideal of R[14]. If A, B are the non empty subsets of a seminearring R, then AB will denote the set of all finite sums of the form ∑akbk with ak∈A, bk∈B. In particular, for each a∈R, aR(Ra) will denote the set of all finite sums of the form ∑axk(∑xka) with xk∈R. Since R is right distributive, it follows that Ra={xa:x∈R}. Clearly aR(Ra) is a right (left) ideal of R. Moreover, the ideal aR(Ra) is called as principal right(left) ideal in particular, if the non empty set is singleton {a} then aR(Ra) is respectively the principal right(left) ideal generated by an element a[14]. A function f:A→A (that is, a unary operation on the set A) is called an involution if f(f(x))=x holds for all x∈A.
3.
Mate functions
Let ϕ be a mapping from R into R in which v=vϕ(v)v holds for every v∈R. Then ϕ is a mate function for R and ϕ(v) is called a mate of v. In fact R admits mate functions iff it is regular.
Since R has one mate function, it has more, because every element of R can serve as a mate of zero 0, that is identity element of (R,+).
We furnish below an example of a seminearring admits mate functions.
Example 3.1. The seminearring (R,+,.) constructed on the semigroups (R,+) and (R,.) with
R={0,h1,h2,h3,h4} is given by:
This seminearring (R,+,.) admits mate functions. The maps ϕ and g from R into R defined by ϕ(0)=0, ϕ(h1)=h1, ϕ(h2)=h2, ϕ(h3)=h3, ϕ(h4)=h4 and g(0)=h1, g(h1)=h4, g(h2)=h1, g(h3)=h1, g(h4)=h3 respectively are mate functions for R. It can be verified that this seminearring has exactly twenty mate functions.
Proposition 3.1. If R is a left (right) normal seminearring then R has a mate function ϕ.
Proof. The fact that ϕ is a mate function of R, ∀ v∈R, v=vϕ(v)v∈Rv. It is clear that R is a left normal.
Similarly R is aslo a right normal. Hence the result follows.
Remark 3.1. By Proposition 3.1, it is clear that if R is to admit mate functions then for all v∈R, v∈vR and v∈Rv - both a right and a left normal conditions hold good i.e. R is a normal seminearring. We give an example to show that a normal seminearring need not admit mate functions.
Example 3.2. For instance in the seminearring (R,+,.) constructed on the semigroups (R,+) and (R,.) with R={0,h1,h2,h3} is defined as follows:
This seminearring is a normal seminearring without a mate function - here 'h1' has no mate.
Proposition 3.2. Suppose ϕ is a mate of R. Then for each v∈R,
(ⅰ) ϕ(v)v and vϕ(v) are idempotents.
(ⅱ) Rv=Rϕ(v)v and vϕ(v)R=vR.
Proof. The proof is straightforward.
Theorem 3.1. If R admits mate functions then any homomorphic image R′ of R also admits mate functions.
Proof. Suppose that R admit a mate function ϕ and g:R→R′ is a seminearring epimorphism. We define h:R′→R′ as follows. For v′∈R′, h(v′)=(g∘ϕ)(v) where v∈R is such that g(v)=v′. It is clear that v′h(v′)v′=g(v)(g∘ϕ)(v)g(v)=g(v)g(ϕ(v))g(v)=g(vϕ(v)v)=g(v) (since ϕ is a mate function of R) = v′. Consequently h serves as a mate function for R′.
Proposition 3.3. Each ideal (left and right) A of R is idempotent whenever ϕ is a mate of R.
Proof. If A of R is a left ideal then RA⊆A. Therefore A2=AA⊆RA⊆A. Also for any a in A, a=aϕ(a)a=a(ϕ(a)a)∈A(RA)⊆AA=A2 and hence A is idempotent. In the sequel, we shall demonstrate that each right ideal A of R is idempotent as well.
Proposition 3.4. Let ϕ be a mate function for the seminearring R. If E⊆C(R) then,
(ⅰ) vϕ(v)=(vϕ(v))r=vrϕ(v)r and
(ⅱ) ϕ(v)v=(ϕ(v)v)r=ϕ(v)rvr, ∀ v∈R and for all positive integers r.
Proof. (i) We first notice that vϕ(v)∈E (by Proposition 3.2). As E⊆C(R) we have vϕ(v)=(vϕ(v))2=vϕ(v)(vϕ(v))=v2ϕ(v)2 continuing in the same vein we get (vϕ(v))=(vϕ(v))r=vr(ϕ(v)r for all positive integers r.
(ii) This follows directly from the proof of (i).
The next Lemma is also valid for a seminearring R.
Lemma 3.1. ([17]) Let R be a seminearring. Then the following are equivalent.
(i) R has no nonzero nilpotent elements.
(ii) If v∈R such that v2=0 implies v=0.
4.
Equivalent conditions for mate functions
Now we will have the necessary and sufficient conditions for a map ϕ:R→R to be a mate function of R.
Theorem 4.1. Suppose that R is a seminearring and that ϕ is a map of R into itself. Then the following are equivalent.
(ⅰ) ϕ is a mate of R.
(ⅱ) ϕ(a)a is an idempotent and Ra=Rϕ(a)a ∀ a∈R.
(ⅲ) For every pair of principal left ideals Ra, Rb and for every R-homomorphism h:Ra→Rb, h(va)=vah(ϕ(a)a) for all v in R.
Proof. (i)⇒(ii) It followed by Proposition 3.2.
(ii)⇒(iii) Since Ra=Rϕ(a)a, we observe that for every v∈R, there is some y∈R such that va=yϕ(a)a. For a, b in R we consider an R-homomorphism h:Ra→ Rb.
Obviously we have h(va)=h(yϕ(a)a) (where y corresponds to v as already indicated) =h(yϕ(a)aϕ(a)a) (since ϕ(a)a∈E)=yϕ(a)ah(ϕ(a)a) (since by R-homomorphism) = vah(ϕ(a)a) and (iii) follows.
(iii)⇒(i) Let us take b=a, h to be the identity R-homomorphism and v such that va=a (this is possible since R is a left normal seminearring). Clearly then, we have a=va=h(va)=vah(ϕ(a)a)=(va)ϕ(a)a (since h is identity homomorphism) = aϕ(a)a. Hence ϕ is a mate function and (i) follows.
We furnish below another characterisation of mate functions.
Theorem 4.2. A map ϕ from right normal seminearring R→R is a mate function of R iff aϕ(a)∈E and aR=aϕ(a)R for each a∈R.
Proof. The proof of the only if part follows from Proposition 3.2. As for the first part (the if part), we can see that as R is a right normal seminearring and as aR=aϕ(a)R for each a in R, there is some v in R where in a=aϕ(a)v. Since aϕ(a)∈E, we have aϕ(a)a=aϕ(a)aϕ(a)v=(aϕ(a))2v=aϕ(a)v=a. The required outcome now follows.
Corollary 4.2.1. Let ϕ be a map of a normal seminearring R into itself. Then the following are equivalent.
(ⅰ) ϕ is a mate of R.
(ⅱ) ϕ(a)a is an idempotent and Ra=Rϕ(a)a for every a in R.
(ⅲ) aϕ(a) is an idempotent and aR=aϕ(a)R for every a in R.
(ⅳ) For every pair of principal left ideals Ra, Rb and for every R-homomorphism h:Ra→Rb, h(va)=va h(ϕ(a)a) ∀ v∈R.
Proof. Follows from Theorems 4.1 and 4.2.
5.
Mutual mate functions
The definition of mate function it does not require that ϕ(a) = ϕ(a)aϕ(a) ∀ a∈R. In other words, though ϕ(a) serves as a mate of a, a itself need not serve as a mate of ϕ(a) – more so under the same mate function ϕ. But we shall show that if R admits a mate function ϕ, then ϕ gives rise to a mate function g, possibly different from ϕ itself, such that a and g(a) are mates of each other. Before that we have the following:
Definition 5.1. A mate function ϕ of R is known as a mutual mate function if ϕ(a) = ϕ(a)aϕ(a) ∀ a∈R. We refer to each of a and ϕ(a) as a mutual mate of each other. If a mutual mate function ϕ happens to be an involution then ϕ is called an involutary mate function of R.
Remark 5.1. For a mate function ϕ of R to be a mutual mate function of R, we just demand that a and ϕ(a) are mutual mates for every a in R. The element a need not be the mate of ϕ(a) under the same ϕ.
It is obvious that every involutary mate function is a mutual mate function.
Lemma 5.1. Suppose that R admits a mate function ϕ. Then it has a mutual mate function λ.
Proof. Let us define λ:R→ R such that λ(i)=ϕ(i)iϕ(i) ∀ i∈R. Clearly then, we have ∀ i∈R, iλ(i)i=i(ϕ(i)iϕ(i))i=(iϕ(i)i)ϕ(i)i=i(ϕ(i)i)2=iϕ(i)i(sinceϕ(i)i∈E)=i and this guarantees that λ is a mate function of R. Also λ(i)iλ(i)=ϕ(i)((iϕ(i)i)ϕ(i)i)ϕ(i)=ϕ(i)(iϕ(i)i)ϕ(i)=ϕ(i)iϕ(i)=λ(i).
This guarantees that λ is a mutual mate function of R.
Remark 5.2. If ϕ is a mutual mate function of R, then apart from the condition (ⅱ) and (ⅲ) of Corollary 4.2.1, we have
(ⅰ) ϕ(a)R=ϕ(a)aR and
(ⅱ) Rϕ(a)=Raϕ(a) for every a in R.
These two results can be established as in the proof of Proposition 3.2(ⅱ).
We recall that a seminearring R is a nil seminearring if every element of R is nilpotent i.e ∀ a∈R, there exists k so ak=0 (where k is a positive integer). For such a seminearring, we have the following:
Theorem 5.1. Suppose R is a nil seminearring with a mate function ϕ, let us define g:R→R by g(a)=ϕ(a)[aϕ(a)+ak−1] for every a in R, where k (depending on a) is some definite integer >1 such that ak=0. Then g is a mate of R. If ϕ is a mutual mate of R, then so is g.
Proof. For every a in R, we have ag(a)a=aϕ(a)[aϕ(a)+ak−1]a=aϕ(a)(aϕ(a)a)=aϕ(a)a=a.
This guarantees that g is a mate function.
Suppose ϕ is a mutual mate of R. Then for every a∈R, g(a)ag(a)=ϕ(a)[aϕ(a)+ak−1]ag(a)=ϕ(a)[aϕ(a)a]g(a)=ϕ(a)ag(a)=ϕ(a)a(ϕ(a)[aϕ(a)+ak−1]=ϕ(a)[aϕ(a)+ak−1]=g(a).
Therefore g is a mutual mate function of R.
Remark 5.3. If R is a seminearring with a mutual mate function ϕ and if a2=0 for some a in R, then the element ϕ(a)[aϕ(a)+a] is a mutual mate of a.
6.
Seminearring with a unique mutual mate function
In this section we derive a necessary and sufficient condition for a seminearring R to admit a unique mutual mate and give a characterisation of a seminearfield. Lemma 5.1 shows the existence of a mutual mate function for R whenever it admits a mate function. It is quite natural for us to probe into the possibilities for R to have a unique mutual mate function. The following result gives a complete characterisation of such a seminearring:
Theorem 6.1. Let R admit mate functions. Then R possesses a unique mutual mate function iff E⊆C(R).
Proof. As for the first part (the if part), we suppose that ϕ is the unique mutual mate function of R. Clearly then, ϕ is involutory as both a and ϕ(ϕ(a)) serve as mutual mate of ϕ(a) for all a in R. Also ϕ fixes every element of E. It is then clear that for all a, y in E, both yϕ(ay) and ϕ(ay)a serve as mutual mates of ay.
The uniqueness of ϕ (as the mutual mate function of R) demands that these mutual mates of ay must be identical with ϕ(ay). It is then easy to observe that, (ϕ(ay))2=(ϕ(ay)a)(yϕ(ay))=ϕ(ay)ayϕ(ay)=ϕ(ay).
This forces ϕ(ay)∈E. But since ϕ is involutory and since it fixes every idempotent, ay=ϕ(ϕ(ay))=ϕ(ay)∈E. This guarantees that (E,.) is a subsemigroup of (R,.). We make use of this result to observe that ϕ(ya)(=ya) also can serve as a mutual mate of ay for all a, y in E. Again from the uniqueness of ϕ, we get ay=ϕ(ay)=ϕ(ya)=ya and the only if part follows.
For the if part, we first observe that Lemma 5.1 shows the existence of a mutual mate ϕ of R. If possible, let g be another mutual mate function of R. To prove g=ϕ, we freely make use of the following:
(i) the assumption that E⊆C(E) and
(ii) the result that for every a in R and for every mate function ϕ of R, both ϕ(a)a and aϕ(a)∈E. We have for all a in R, g(a)=g(a)ag(a)=g(a)(aϕ(a)a)g(a)=(ϕ(a)a)(g(a)ag(a))=ϕ(a)ag(a)=ϕ(a)(ag(a))=(ϕ(a)aϕ(a)ag(a)=ϕ(a)aϕ(a)=ϕ(a). This guarantees that ϕ is unique as the mutual mate function for R.
Lemma 6.1. Suppose vy=0 for some v, y in an arbitrary seminearring R. Then (yv)2R=0 and in particular (yv)k=0 for every k≥2.
Proof. Now vy=0⇒(yv)2=yvyv=y(vy)v=y0v=0. Hence for all n in R, we have (yv)2n=0n=0. This yields (yv)2R=0. Thus (yv)2(yv)k−2=0 by taking n=(yv)k−2 where k is an integer ≥2. Thus (yv)k=0. The required result follows.
Theorem 6.2. Suppose that R has a mutual mate function ϕ. Then the following are true.
(ⅰ) ϕ has the reversal law i.e. if k is any positive integer then for v1,v2,…vk∈R, we have ϕ(v1,v2,…vk)=ϕ(vk)ϕ(vk−1)…ϕ(v1).
(ⅱ) ϕ(ak)=(ϕ(a))k for any positive integer k and for any a in R.
(ⅲ) R has no nonzero nilpotent elements.
Proof. (i) Let us prove this by simple induction on the number of elements k. When k=1, the result holds trivially. We shall assume that the result holds for any set of k elements of R. Let v1,v2,…vk∈R and let v=v1,v2,…vk for convenience.
Now by assumption, ϕ(v)=ϕ(v1,v2,…vk)=ϕ(vk)…ϕ(v2)ϕ(v1). Let y be any element of R to get the desired result by simple induction, we need only to prove that, ϕ(vy)=ϕ(y)ϕ(v). (For this, we make use of Theorem 4.1 and Theorem 6.1).
Now vy=(vϕ(v)v)(yϕ(y)y)=v(ϕ(v)v)(yϕ(y))y=v(yϕ(y))(ϕ(v)v)y=vyϕ(y)ϕ(v)vy. Also ϕ(y)ϕ(v)=(ϕ(y)yϕ(y))(ϕ(v)vϕ(v))=ϕ(y)(yϕ(y))(ϕ(v)v)ϕ(v)=ϕ(y)(ϕ(v)v)(yϕ(y))ϕ(v)=ϕ(y)ϕ(v)vyϕ(y)ϕ(v). This guarantees that ϕ(y)ϕ(v) is a mutual mate of vy.
Since ϕ(vy) is the unique mutual mate of vy, we must have, ϕ(vy)=ϕ(y)ϕ(v) and the result follows.
(ii) This follows by taking a=v1=⋯=vk in (ⅰ).
(iii) Suppose v2=0 for some v in R. By Lemma 3.1 we need only prove that v=0.
Since v2=0, we have 0=ϕ(0)=ϕ(v2)=(ϕ(v))2 by (ⅱ). Clearly then, we have from the uniqueness of ϕ, ϕ(v)=ϕ(v)[vϕ(v)+v] (by Remark 5.3 which guarantees that the right hand side of this equality is also a mutual mate of v).
Hence 0=ϕ(v2)=(ϕ(v))2=ϕ(v)(vϕ(v)+v)ϕ(v)=ϕ(v)(v(ϕ(v))2+vϕ(v))=ϕ(v)(0+vϕ(v))=ϕ(v). This forces ϕ(v)=0 whenever v2=0. But as ϕ is involutory (from the proof of Theorem 6.1), we have ϕ(ϕ(v))=ϕ(0)=0 which, in turn, implies that v=0. The result follows by Lemma 3.1
Theorem 6.3. Suppose R admit mate functions. R is a seminearfield iff all possible mate functions of R agree in R∗.
Proof. The 'onlyif' part is obvious. For the 'if' part, let ϕ be any mate function of R. If ve=0 for some v∈R and for some e in E∗, it is clear that both e and v+ϕ(e) serve as mates of e. This forces v=0 and as such none of the non-zero idempotent is a right zero divisor. It follows that every e in E∗ is a right identity. For every v in R∗, both vϕ(v) and ϕ(v)v serve as mates of e in E∗. This guarantees that vϕ(v)=e=ϕ(v)v for all v in R∗ and for all e in E∗. Hence ev=v=ve. This holds even when v=0. These facts force E∗={e} where e is the two sided identity of (R,.). The desired result now follows since ϕ(v) serves as the inverse of v for every v in R∗.
Acknowledgments
The authors would like to express our appreciation to the anonymous referees for the comprehensive reading of this paper and their valuable comments and suggestions.
Conflict of interest
The authors declare there is no conflict of interest.









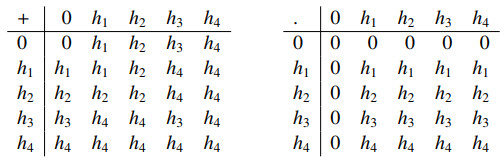
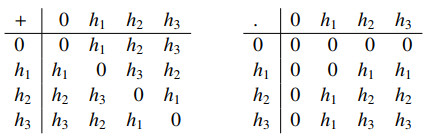




 DownLoad:
DownLoad: