1. Introduction
Theoretical limit of hardening of the material can be achieved by elimination of all defects and achieving perfect single crystal structure, or, on the contrary, by increasing the density of defects.
However, the defects exert both strengthening and softening influence. One can get a sample with no impurity atoms, but to get material without dislocations is not possible.
Dislocations appear during the formation and growth of crystals and are characterized by a “critical” density, when the crystal strength has a minimum value. At a very small specific quantity of defects metal strength should be high. Such material has not been received yet, except for whiskers. Therefore, high-strength materials can be obtained on the basis of “nanowhiskers” or nanoparticles (nanomaterials).
Covalent compounds based on light elements have a complex of useful performance properties and provide a basis for creation of ceramic materials of various purpose. The paper studies nanoceramic materials with cubic structure—a diamond, SiC, BN, AlN.
As is known, nanomaterials differ from traditional ones in that their forming or constituent structural units (nano-elements) have nanometer level size at least in one direction. Nanomaterials, being “massive” materials (bulk materials), behave like traditional materials. However, the level of their properties will be determined by constituent nanoelements’ properties. Thus, it is reasonable to mark out their distinctive characteristics.
An important type of three-dimensional nanostructured material is a compact or consolidated polycrystalline with nanometer-sized grains, its entire volume is filled with nanograins, grains have no free surface, and there are only grain boundaries—interfaces. Interfaces formation and “disappearance” of nanoparticles(nanograins) surface—this is what differ fundamentally three-dimensional compact nanomaterials from nanocrystalline powders of different agglomeration ranges, composed of the same-sized particles as compact nanostructured material.
2. The Theory, Models and Research Methods
The objective of this study—building (based on a priori pseudopotential method, with taking into account the role of interface between nano-elements) of analytical model which willproperly describes the mechanical properties of nanoceramics.
For this purpose, we need some intermediate models describing mechanical properties of the object in going from multidimensional material to a nanoparticle.
2.1. Theoretical Strength at Uniaxial Deformation of Multidimensional Materials
An anisotropic single crystal under loadings deforms easier in certain crystallographic directions [1,2]. For the investigated materials with cubic structure such directions are space diagonals of elementary cell. Atomic planes, perpendicular to diagonals, are close-packed. Therefore, it is reasonable to describe diamond-like materials’ structure in hexagonal axes, having chosen a spatial cube diagonal [111] for a coordinate axis OZ. Then the planes {111}, being octahedron planes, will have indexes 001 (i.e., basic planes).
In this case, the structure of a diamond and diamond-like materials will be described by three-layer alternation of planes (001) like AA′BB′CC′ where planes A,B,C will consist of atoms of one grade (C,B,Al,Si), and planes A′,B′,C′ will consist of atoms of another one (C,N). The distance between two close packed atomic planes A and A′ will be equal to c/4, and between A′ and B will be equal to c/12 (c=a0√3, a0: cubic lattice parameter). The elementary cell of diamond-like materials in hexagonal axes consists of 6 atoms which are in planes AA′BB′CC′.
In the work based on pseudopotential method (through paired interatomic potentials) interlayer interaction energy has been calculated, with the help of which we have calculated theoretical strength of diamond-like materials in uniaxial deformation along the direction [111].
On the other hand, the theory of pseudo-potential can be formulated in terms of pair potential only when ions are redistributed at constant average density of material. According to calculation data, the change of average atom volume for investigated materials turned out to be small (ΔΩ/Ω0≈0,0098), which made it possible to neglect a change of average material density and calculate theoretical strength of these materials on the basis of interatomic potentials.
We have calculated energy of interaction between close-packed atomic planes. Close-packed atomic planes are spaced apart at a distance h (c/4 or c/12) and are shifted relative to each other at zero or vector ρ. According to results of calculation the interaction energy (Φ0(c/4, 0)) of atomic planes of A, A' and A', B types (interaction energy: Φ(c/12, ρ)) differ ten times [3].
With known values of interaction energy of atomic layers strain tensor is determined by ratio [1].
where ez is unit strain.
Theoretical strength inside the layers (interaction energy: Φ(c/12, ρ)) is five times higher than strength between layers (interaction energy Φ0(c/4, 0)). In brittle materials having cubic structure and being subjected to uniaxial tension in the direction of [111], microcracks appear first of all between atomic planes {111}, being at distance c/4.
The general view of functional dependence of strain on interplane distance is the same for investigated diamond-like materials. The curve stress-strain has a characteristic peak with a sharp decline at compression which is connected with possible phase transition. At high loadings the phase transition of diamond-like materials in metal condition [4] is possible.
The calculated values of theoretical strength for corresponding tension strain and compression strain are shown in Table 1.
Table 1. The values of theoretical strength (σ1max, σ2max) and corresponding strain (−e111*, e111**) under compression and tension.
| Crystal | Compression | | Tension | |
| σ1max(GPa) | −e111* | σ2max(GPa) | e111** |
| C | 204.40 | 0.0975 | 139.07 | 0.1456 |
| BN | 175.40 | 0.0978 | 119.24 | 0.1458 |
| SiC | 147.56 | 0.0984 | 103.12 | 0.1459 |
| AlN | 88.26 | 0.0998 | 59.05 | 0.1460 |
As Table 1shows, at uniaxial tension or compression, the deformation corresponding to ultimate strength of diamond-like materials, for given class of materials has almost the same value (about 0.1 at compression and 0.146 at tension).
Thus, when describing the crystal structures of diamond-like materials in hexagonal axes basic close-packed atomic planes (perpendicular to diagonal), are separated by a distance c/4 and c/12 (c:crystal lattice parameter, which coincides with the spatial diagonal of a cubic lattice). Atomic planes separated by a distance c/12 are more strongly bound than planes spaced at a distance of c/4. As a result, at uniaxial deformation, the material breaks down into bi-layers representing strongly bound atomic planes.
2.2. Nanoparticles having Diamond-like Structure
The supposition that the external surfaces of the nanoparticles have energy -Ф0/2 (the surface of a nanoparticle has positive energy) is fundamental in the calculation of mechanical characteristics of nanoparticles in the form of a nanoplate or a nanotimber.
Interplanar interactionenergy within a crystal always has a negative sign, the surface energy is negative toward infinitely remote point, but as compared with the energy inside the crystal—positive. In exceptional cases, for some materials the surface energy as compared to the internal energy can have a negative sign, which is explained by strong interaction of the surface with the surrounding particles of the medium. For simplicity we assume that a nanoparticle is in vacuum. The surface of the nanoparticle is its weak point. Outer surface influence on the ions and electrons inner state has been evaluated with the help of averaging of neighboring layers’ energy.
The interaction energy of atomic planes in i-layer of infinite nanoplate with taking into account the influence of 2 free surfaces having the energy −Φ1/2 and −Φ2/2 (energy Φi has a negative sign, Ф1 = Ф2 = Ф0) is written as[5]
|
Φi=Φ0−Φ112i+1−Φ212j+2−i−1j⋅2j+1(Φ1+Φ2)
|
(2)
|
where j is the number of layers in the nanoparticle.
The last term in Eq. (1) provides the law of conservation of the total energy of the system with a limited number of atomic planes. Equation (2) can be used to calculate the interlayer interaction energy both for bulk samples and nanoplates. In case of infinite nano timber (basal plane of a nanoparticle has sizes Na and Ma, and the number of them is uncountable, N, M—whole numbers), we consider the energy of the side surfaces, a—parameter of the basal plane of the crystal lattice of diamond-like nanoparticles in the hexagonal axes.
According to the statement of the problem we calculate the mechanical properties under uniaxial loading, using the energy of interaction between structural units perpendicular to the loading axis. The side surfaces are parallel to the axis of deformation, that is, they are perpendicular to the other space diagonal ([111]). Therefore, the interaction energy between the sides atoms located on the adjacent planes is desired surface energy Φ0/2. Then, the energy of interaction between atomic layers can be represented as
|
ΦS=(N1Φ0+N2ΦB)/(N1+N2)
|
(3)
|
where N1 is the number of atoms on the planes inside the nanotimber, N2 is the number of edge atoms of these planes, ΦB is the energy of interaction between the edge atoms in neighboring layers.
To determine the average value of the nanoplate energy we summarize Eq. (2) for all layers and divide into a number of layers j. As a result, we obtain [6] for nanoplates with infinite base plane.
The number of layers j = 3d/c, where d is the nanoplate thickness. For an infinite nanotimber from Eq. (3) we obtain
where N (N = N1 + N2) can be determined from the value of the base plane area; S=d2=a2√3⋅N2, here a is the base plane parameter.
For a limited nanotimber or nanoplate the average energy of interaction between atomic layers, respectively, will be
In view of formula (1) the average strength of a nanoplate with thickness d is
where σ0 is theoretical strength of bulk diamond-like crystal in the direction of [111]. Since nano dimension is expressed only in one direction, coefficient КP = c/3 depends only on parameter c.
In case of an infinite nano timber we have
where KS≈6a4√3 depends on the lattice parameters on the base plane, where the size limit is registered (nano dimension).
In case of a nanoparticle
Formulas (7-9) essentially express Hall-Petch’s law, not for polycrystalline material but for ideal crystals in going from unlimited volume to a nanoplate, whisker (nanotimber) or nanoparticle. As a result, from first principles, we have obtained the inverse Hall Patch’s law, where dependence on the size of a nanoplate, whisker or nanoparticles is presented in the form of d−1 instead of d−1/2. Hall-Petch coefficients Kp, Ks and KΩ depend on the lattice parameters.
2.3. Constructing Nanomaterials of Nanoparticles
Suppose the nanomaterialconsists of nanoparticles in the form of bounded nano timbers having a diamond-like structure. Let us choose a representative nano timber in the material. Suppose its base atomic planes are perpendicular to the axis of strain. It is thought that the side surfaces of the representative nano timber are perpendicular to the base planes and the interface boundaries of nanoparticles are quasicoherent.
Having selected the representative nano timber we proceed to taking account of the surrounding nano timbers’ influence. Given the representative nano timber geometric shape we assume that on latteral sides it must be surrounded by neighboring nano timbers, basal planes of which form the angles αi(i=1,2,3,4) with similar planes of the selected nano timber. And in the chosen direction of deformation the representative nano timber (on the top and on the bottom) is in contact with nano timbers whose basal planes form angles βi(i=1,2) with representative nano timber planes.
The nano timber surface, parallel to the basal planes, with account of neighboring nano timbers has the energy equal to
|
(−Φ02−Φ02cos(βi))/2;i=1,2
|
(10)
|
When joining with the side nano timbers the energy of interaction between boundary lines of atoms belonging to representative nano timber will be written as
|
(−Φ02−Φ02cos(αi))/2;i=1,2,3,4
|
(11)
|
If in formulas (4-6) substitute Φ0 from expressions (10) and (11) then we obtain an average energy of interaction between close-packed atomic planes of bounded nano timber in the material. Given certain values of layers interaction energy we determine the strength by means of relation (1).
As a result, for the nanoparticle of infinite nano timber type in nanomaterial, strength will be (taking into account the side neighbors only)
|
σS≈σ0(1−5+∑icos(αi)/4N)
|
(12)
|
For nanoplate having unlimited basal plane we have (there are nanoplates closing the top and the bottom)
|
ˉσP≈σ0(1−2+cos(β1)+cos(β2)8j)
|
(13)
|
Here, σ0 istheoretical strength of perfect crystals.
The average value of the bounded nano timber strength will be
|
ˉσΩ≈σ0(1−2+cos(β1)+cos(β2)8j)⋅(1−5+∑icos(αi)/4N)
|
(14)
|
Substituting values j and N we obtain
where
|
k∗Ω≈a4√3(5+∑icos(αi)/4)+c⋅(2+∑icos(βi))/24
|
(16)
|
If we average the cosine of angles which change in the range 0≤αl;βi≤900, then we get the Hall-Petch coefficient for nanoceramics
Relation (15) presents inverse Hall-Petch law for nanoceramics with regard to nanoparticles’ interface. The calculated values of parameters (a, c) of FCP lattice for BN are: а = 0.2565 and с = 0.6424 nm.
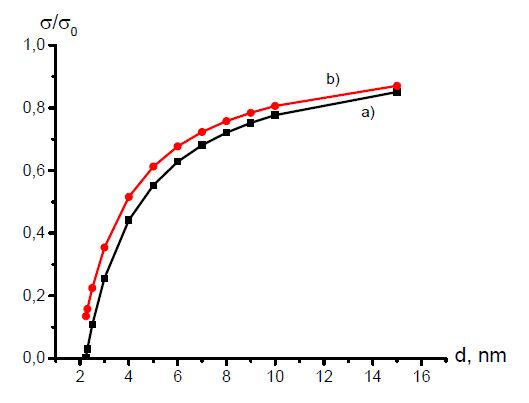
Maximum strength of crystals, nanoparticles and nanoceramics has been calculated by means of formulas (9, 15, 17). For nanoparticles and nanoceramics we have shown the dependence of the ultimate strength on the size of nanoparticles for BN (Figure 1).
As the nanoparticles’ size increases the strength also increases, tending to ideal crystal strength. Nanoparticle strength is always less than the strength of nanoceramics which consists of nanoparticles of the same size. Coefficient kΩ = 2.231 for nanoparticles and kΩ = 1.937 for nanoparticles with regard to interactions with surrounding ones. There is a minimum size of nanoparticles, below which the physical sense of strength is lost. This size is determined by crystal lattice parameters of the material. In case of BN, this limit is 2 nm for a nanoparticle and 2.24 m for nanoceramics.
3. Conclusions
1. The direct Hall-Petch’s law is not applicable to nanoparticles. The inverse Hall-Petch’s law determines the maximum strength of the materials having ideal structure with regard to linear size of the particle. Reducing the size of a real crystal (with defects) we can obtain almost ideal structure. For these nanocrystals only inverse Hall-Petch’s law will operate, which considers nanocrystals’ outside face as their only defect.
2. In inverse Hall-Petch’s law, obtained from quantum mechanical calculations, dependence of nanocrystal strength on its size is represented as d−1 instead of d−1/2.
3. Nanoceramics arecharacterized by higher maximum strength than its constituent nanoparticles.
Conflict of Interest
The authors declare that there is no conflict of interest regarding the publication of this manuscript.









 DownLoad:
DownLoad: