Stable isotope ratios and speleothem chronology from a high-elevation alpine cave, southern San Juan Mountains, Colorado (USA): Evidence for substantial deglaciation as early as 13.5 ka

Ray Kenny; Ray Kenny

doi:10.3934/geosci.2019.1.41

AIMS Geosciences

2019, Volume 5, Issue 1: 41-65. doi: 10.3934/geosci.2019.1.41

Previous Article Next Article

Research article

Stable isotope ratios and speleothem chronology from a high-elevation alpine cave, southern San Juan Mountains, Colorado (USA): Evidence for substantial deglaciation as early as 13.5 ka

Ray Kenny ^,

Research Geologist, Durango, CO-81301, USA

Received: 10 November 2018 Accepted: 07 March 2019 Published: 27 March 2019

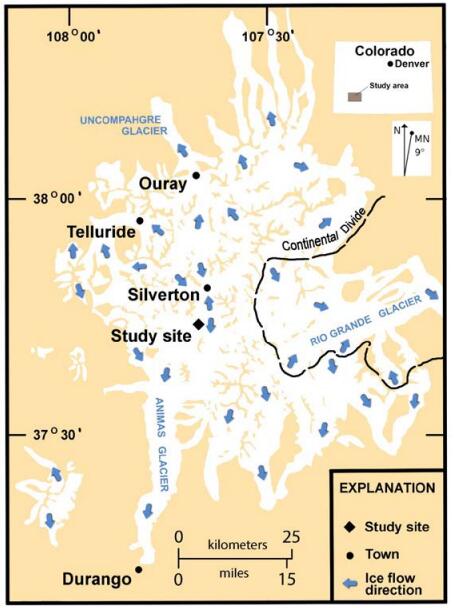
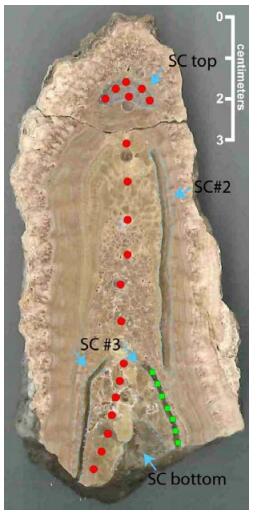
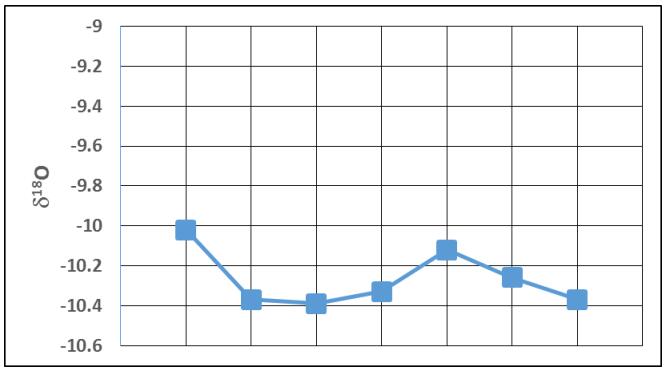
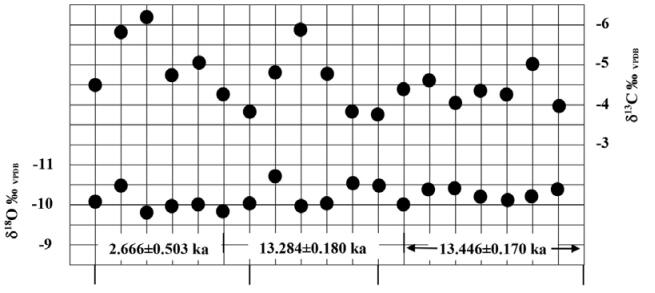
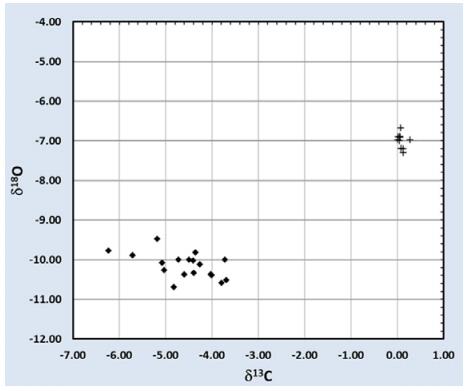
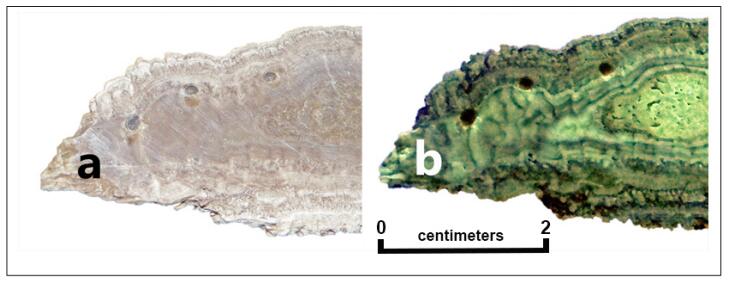
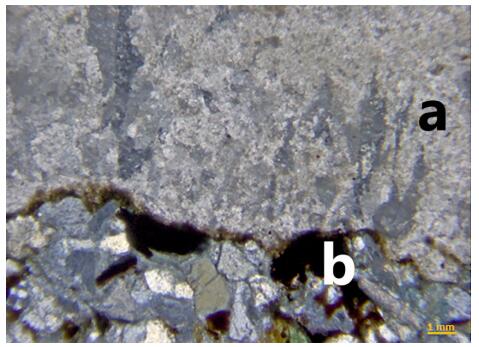
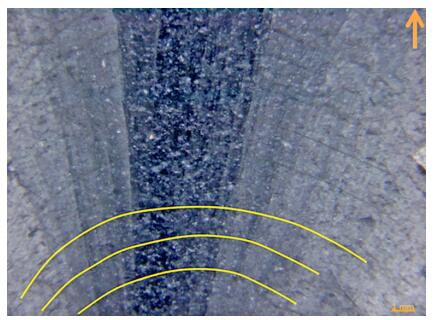
Deglaciation ages for valley glaciers from the last glacial maximum are difficult to obtain with precision. The unequal or non-uniform loss of glacial ice cover (spatial heterogeneity) during glacial retreat results in exposure of considerable land surface area prior to complete deglaciation. New U-series dates from an 11cm long stalagmite in a shallow, high elevation (3242 m) cave in the southern San Juan Mountains of southwestern Colorado (USA), indicate that substantial, high-elevation glacial ice ablation occurred prior to 13.5 ka. The stalagmite dates compare well with other deglaciation studies in central and southern Colorado that show deglaciation occurred between ca. 13 ka to 15 ka. Low δ¹³C values from 13.5 ka speleothem growth bands, relative to the δ¹³C value of the host carbonate, suggest that alpine plant and soil cover was established at this location, aspect, and elevation prior to 13.5 ka. Calcite luminescence in the 13.5 ka growth bands indicates the presence of humic and fulvic acids released by the roots of living plants and decomposition of vegetative matter. The carbon isotope ratios, calcite luminescence, and U-series dates suggest that the southern San Juan Mountains, at this high elevation site and southern aspect were substantially ice-free prior to 13.5 ka.

Keywords:

Citation: Ray Kenny. Stable isotope ratios and speleothem chronology from a high-elevation alpine cave, southern San Juan Mountains, Colorado (USA): Evidence for substantial deglaciation as early as 13.5 ka[J]. AIMS Geosciences, 2019, 5(1): 41-65. doi: 10.3934/geosci.2019.1.41

Related Papers:

[1]	Jin Gao, Lihua Dai . Anti-periodic synchronization of quaternion-valued high-order Hopfield neural networks with delays. AIMS Mathematics, 2022, 7(8): 14051-14075. doi: 10.3934/math.2022775
[2]	Yuehong Zhang, Zhiying Li, Wangdong Jiang, Wei Liu . The stability of anti-periodic solutions for fractional-order inertial BAM neural networks with time-delays. AIMS Mathematics, 2023, 8(3): 6176-6190. doi: 10.3934/math.2023312
[3]	Xiaofang Meng, Yongkun Li . Pseudo almost periodic solutions for quaternion-valued high-order Hopfield neural networks with time-varying delays and leakage delays on time scales. AIMS Mathematics, 2021, 6(9): 10070-10091. doi: 10.3934/math.2021585
[4]	Nina Huo, Bing Li, Yongkun Li . Global exponential stability and existence of almost periodic solutions in distribution for Clifford-valued stochastic high-order Hopfield neural networks with time-varying delays. AIMS Mathematics, 2022, 7(3): 3653-3679. doi: 10.3934/math.2022202
[5]	Huizhen Qu, Jianwen Zhou . $S$ -asymptotically $\omega$ -periodic dynamics in a fractional-order dual inertial neural networks with time-varying lags. AIMS Mathematics, 2022, 7(2): 2782-2809. doi: 10.3934/math.2022154
[6]	Dan-Ning Xu, Zhi-Ying Li . Mittag-Leffler stabilization of anti-periodic solutions for fractional-order neural networks with time-varying delays. AIMS Mathematics, 2023, 8(1): 1610-1619. doi: 10.3934/math.2023081
[7]	Ailing Li, Xinlu Ye . Finite-time anti-synchronization for delayed inertial neural networks via the fractional and polynomial controllers of time variable. AIMS Mathematics, 2021, 6(8): 8173-8190. doi: 10.3934/math.2021473
[8]	Huahai Qiu, Li Wan, Zhigang Zhou, Qunjiao Zhang, Qinghua Zhou . Global exponential periodicity of nonlinear neural networks with multiple time-varying delays. AIMS Mathematics, 2023, 8(5): 12472-12485. doi: 10.3934/math.2023626
[9]	Yanshou Dong, Junfang Zhao, Xu Miao, Ming Kang . Piecewise pseudo almost periodic solutions of interval general BAM neural networks with mixed time-varying delays and impulsive perturbations. AIMS Mathematics, 2023, 8(9): 21828-21855. doi: 10.3934/math.20231113
[10]	Xiaojin Guo, Chuangxia Huang, Jinde Cao . Nonnegative periodicity on high-order proportional delayed cellular neural networks involving $D$ operator. AIMS Mathematics, 2021, 6(3): 2228-2243. doi: 10.3934/math.2021135

Abstract

1. Introduction

Due to the engineering backgrounds and strong biological significance, Babcock and Westervelt ^[1,2] introduced an inertial term into the traditional multidirectional associative memory neural networks, and established a class of second order delay differential equations, which was called as the famous delayed inertial neural networks model. Arising from problems in different applied sciences such as mathematical physics, control theory, biology in different situations, nonlinear vibration, mechanics, electromagnetic theory and other related fields, the periodic oscillation is an important qualitative property of nonlinear differential equations ^{[3,4,5,6,7,8,9]}. Consequently, assuming that the activation functions are bounded and employing reduced-order variable substitution which convert the inertial systems into the first order differential equations, the authors in ^[10,11] and ^[12] have respectively gained the existence and stability of anti-periodic solution and periodic solution for addressed inertial neural networks models. Manifestly, the above transformation will raise the dimension in the inertial neural networks system, then some new parameters need to be introduced. This will increase huge amount of computation and be attained hard in practice ^[13,14]. For the above reasons, most recently, avoiding the reduced order method, the authors in ^[15] and ^[16] respectively developed some non-reduced order methods to establish the existence and stability of periodic solutions for inertial neural networks with time-varying delays.

It has been recognized that, in neural networks dynamics touching the communication, economics, biology or ecology areas, the relevant state variables are often considered as proteins and molecules, light intensity levels or electric charge, and they are naturally anti-periodic ^[17,18,19]. Such neural networks systems are often regarded as anti-periodic systems. Therefore, the convergence analysis and stability on the anti-periodic solutions in various neural networks systems with delays have attracted the interest of many researchers and some excellent results are reported in ^{[20,21,22,23,24,25,26,27]}. In particular, the anti-periodicity on inertial quaternion-valued high-order Hopfield neural networks with state-dependent delays has been established in ^[28] by employing reduced-order variable substitution. However, few researchers have utilized the non-reduced order methods to explore such topics on the following high-order inertial Hopfield neural networks involving time-varying delays:

$\begin{eqnarray*} &&x_{i}''(t)\\& = &-\bar{a}_{i}(t)x_{i}'(t)-\bar{b}_{i}(t)x_{i}(t)+\sum\limits_{j = 1}^{n}\bar{c}_{ij}(t)A_{j}(x_{j}(t)) +\sum\limits_{j = 1}^{n}\bar{d}_{ij}(t)B_{j}(x_{j}(t- q_{ij}( t)) ) \\&&+ \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}\theta_{ijl}(t)Q_{j}(x_{j}( t-\eta_{ijl} (t) ))Q_{l}(x_{l}( t-\xi_{ijl}( t) ))+J_{i}(t), \ t\geq 0, \end{eqnarray*}$

(1.1)

associating with initial value conditions:

$\begin{align} x_{i}(s) = \varphi_{i}(s), \ x_{i}'(s) = \psi_{i}(s), \ -\tau_{i} \leq s \leq 0, \ \varphi_{i}, \ \psi_{i}\in C([-\tau_{i} , 0], \mathbb{R}), \end{align}$

(1.2)

where $\sum\limits_{j = 1}^{n}\bar{c}_{ij}(t)A_{j}(x_{j}(t)), \ \sum\limits_{j = 1}^{n}\bar{d}_{ij}(t)B_{j}(x_{j}(t- q_{ij}(t)))$ and $\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}\theta_{ijl}(t)Q_{j}(x_{j}(t-\eta_{ijl} (t)))Q_{l}(x_{l}(t-\xi_{ijl}(t)))$ are respectively the first-order term and the second-order term of the neural network, $A_{j}, \ B_{j}$ and $Q_{j}$ are the nonlinear activation functions, $\tau_{i} = \max\limits_{1\leq l, j\leq n}\{\sup\limits_{t\in \mathbb{R}}q_{ij}(t), \ \sup\limits_{t\in \mathbb{R}}\eta_{ijl}(t), \sup\limits_{t\in \mathbb{R}}\xi_{ijl}(t) \},$ $J_{i}, \bar{c}_{ij}, \bar{d}_{ij}, \theta_{ijl}, \bar{a}_{i}, \bar{b}_{i} :\mathbb{R}\rightarrow \mathbb{R}$ and $q_{ij}, \ \eta_{ijl}, \ \xi_{ijl} :\mathbb{R}\rightarrow \mathbb{R}^+$ are bounded and continuous functions, $\bar{a}_{i}, \bar{b}_{i}, q_{ij}, \ \eta_{ijl}, \ \xi_{ijl}$ are periodic functions with period $T > 0$ , the input term $J_{i}$ is $T$ -anti-periodic $(J_{i}(t+T) = -J_{i}(t) \ \mbox{for all} \ t\in \mathbb{R})$ , and $i, j, l\in D: = \{1, 2, \cdots, n\}$ .

Motivated by the above arguments, in this paper, without adopting the reduced order method, we propose a novel approach involving differential inequality techniques coupled with Lyapunov function method to demonstrate the existence and global exponential stability of anti-periodic solutions for system (1.1). Particularly, our results are new and supplement some corresponding ones of the existing literature ^{[19,20,21,22,23,24,25,26,27,28]}. In a nutshell, the contributions of this paper can be summarized as follows. 1) A class of anti-periodic high-order inertial Hopfield neural networks involving time-varying delays are proposed; 2) Under some appropriate anti-periodic assumptions, all solutions and their derivatives in the proposed neural networks model are guaranteed to converge to the anti-periodic solution and its derivative, respectively; 3) Numerical results including comparisons are presented to verify the obtained theoretical results.

The remaining parts of this paper are organized as follows. In Section 2, we make some preparations. In Section 3, the existence and the global exponential stability of the anti-periodic solution are stated and demonstrated. Section 4 shows numerical examples. Conclusions are drawn in Section 5.

2. Preliminaries

To study the existence and uniqueness of anti-periodic solutions to system (1.1), we first require the following assumptions and some key lemmas:

Assumptions:

$(F_{1})$ For $i, j, l \in D,$ $A_{j}(u), B_{j}(u), Q_{j}(u)$ are all non-decreasing functions with $A_{j}(0) = B_{j}(0) = Q_{j}(0) = 0$ , and there are nonnegative constants $L^{A}_{j}$ , $L^{B}_{j}$ , $L^{Q}_{j}$ and $M^{Q}_{j}$ such that

$|A_{j}(u )-A_{j}(v )|\leq L^{A} _{j}|u -v |, \ |B_{j}(u )-B_{j}(v )| \leq L^{B}_{j}|u -v |, \ |Q_{j}(u )-Q_{j}(v )| \leq L^{Q}_{j}|u -v |, \$

$|Q_j(u)| \leq M_{j}^{Q}, \ \bar{c}_{ij}(t+ T )A_j(u) = -\bar{c}_{ij}(t)A_j(-u), \ \ \bar{d}_{ij}(t+T)B_j(u) = -\bar{d}_{ij}(t)B_j(-u),$

and

$\ \theta_{ijl}(t+T)Q_j(u)Q_l(v) = -\theta_{ijl}(t)Q_j(-u)Q_l(-v),$

$\mbox{for all} \ u, \ v \in \mathbb{ R}.$

$(F_{2})$ There are constants $\beta_{i} > 0$ and $\alpha_{i}\geq 0, \gamma_{i}\geq 0$ obeying

$\begin{align} E_{i} (t) \lt 0, \ \ \ 4E_{i}(t)G_{i }(t) \gt H_{i}^{2}(t), \ \forall t\in \mathbb{R}, \ i\in D, \end{align}$

(2.1)

where

$\left. \begin{array}{ll} E_{i}(t) = \alpha_{i}\gamma_{i}-\bar{a}_{i}(t)\alpha_{i}^{2} + \frac{1}{2}\alpha_{i}^{2}\sum\limits_{j = 1}^{n}(|\bar{c}_{ij}(t)|L^{A}_{j} +|\bar{d}_{ij}(t)|L^{B}_{j})\\ \ \ \ \ \ \ \ \ \ +\frac{1}{2} \alpha_{i}^{2}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}+L^{Q}_{j}M^{Q}_{l}), \\ G_{i} (t) = -\bar{b}_{i}(t)\alpha_{i}\gamma_{i} +\frac{1}{2}\sum\limits_{j = 1}^{n}(|\bar{c}_{ij}(t)|L^{A}_{j}+|\bar{d}_{ij}(t)|L^{B}_{j} )|\alpha_{i}\gamma_{i}| \\ \ \ \ \ \ \ \ \ \ +\frac{1}{2}\sum\limits_{j = 1}^{n}\alpha_{j}^{2}(|\bar{c}_{ji}(t)|L^{A}_{i} + \bar{d}_{ji}^{+} L^{B}_{i}\frac{1}{1-\dot{q} _{ji} ^{+}} )\\ \ \ \ \ \ \ \ \ \ +\frac{1}{2}\sum\limits_{j = 1}^{n}(|\bar{c}_{ji}(t)|L^{A}_{i} + \bar{d}_{ji}^{+} L^{B}_{i}\frac{1}{1-\dot{q} _{ji} ^{+}} ) |\alpha_{j}\gamma_{j}|\\ \ \ \ \ \ \ \ \ \ +\frac{1}{2} \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\alpha_{i}\gamma_{i}||\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}+L^{Q}_{j}M^{Q}_{l}) \\ \ \ \ \ \ \ \ \ \ +\frac{1}{2} \sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|) \theta_{lji} ^{+} M^{Q}_{j}L^{Q}_{i} \frac{1}{1-\dot{\xi} _{lji} ^{+}} \\ \ \ \ \ \ \ \ \ \ +\frac{1}{2} \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|) \theta_{jil} ^{+}L^{Q}_{i}M^{Q}_{l} \frac{1}{1-\dot{\eta} _{jil} ^{+}} ) , \end{array}\right.$

$\left. \begin{array}{ll} H_{i}(t) = \beta_{i}+\gamma_{i}^{2} -\bar{a}_{i}(t)\alpha_{i}\gamma_{i}-\bar{b}_{i}(t)\alpha_{i}^{2}, \ \dot{q}_{ij}^{+} = \sup\limits_{t\in \mathbb{R}}q'_{ij}(t), \\ \dot{\eta}_{ijl}^{+} = \sup\limits_{t\in \mathbb{R}}\eta'_{ijl}(t), \ \dot{\xi}_{ijl}^{+} = \sup\limits_{t\in \mathbb{R}}\xi'_{ijl}(t), \ \ q_{ij}^{+} = \sup\limits_{t\in \mathbb{R}}q_{ij}(t), \ \eta_{ijl}^{+} = \sup\limits_{t\in \mathbb{R}}\eta_{ijl}(t), \\ \xi_{ijl}^{+} = \sup\limits_{t\in \mathbb{R}}\xi_{ijl}(t), \ \bar{c}_{ij}^{+} = \sup\limits_{t\in \mathbb{R}}|\bar{c}_{ij}(t)| , \ \bar{d}_{ij}^{+} = \sup\limits_{t\in \mathbb{R}}|\bar{d}_{ij}(t)|, \ i,j, l \in D . \end{array}\right.$

$(F_{3})$ For $i, j, l\in D,$ $q_{ij}$ , $\eta_{ijl}$ and $\xi_{ijl}$ are continuously differentiable, $q_{ij}'(t) = \dot{q_{ij}} (t) < 1$ , $\eta_{ijl}'(t) = \dot{\eta_{ijl} }(t) < 1$ and $\xi_{ijl}'(t) = \dot{\xi_{ijl}} (t) < 1$ for all $t\in \mathbb{R}$ .

We will adopt the following notations:

$\theta_{ijl}^{+} = \max\limits_{t\in [0, T]}|\theta_{ijl}(t)|, i, j, l \in D.$

Remark 2.1. Since (1.1) can be converted into the first order functional differential equations. In view of $(F_{1})$ and (^[29], p176, Theorem 5.4), one can see that all solutions of $(1.1)$ and (1.2) exist on $[0, \ +\infty).$

Lemma 2.1. Under $(F_{1})$ , $(F_{2})$ and $(F_{3})$ , label $x (t) = (x_{1}(t), x_{2}(t), \cdots, x_{n}(t))$ and $y (t) = (y_{1}(t), y_{2}(t), \cdots, y_{n}(t))$ as two solutions of system (1.1) satisfying

$\begin{align} x_{i}(s) = \varphi^{x}_{i}(s), \ x_{i}'(s) = \psi^{x}_{i}(s) , \ y_{i}(s) = \varphi^{y}_{i}(s),\ y_{i}'(s) = \psi^{y}_{i}(s), \end{align}$

(2.2)

where $-\tau_{i} \leq s \leq 0, \ i\in D, \ \varphi^{x}_{i}, \psi^{x}_{i}, \varphi^{y}_{i}, \psi^{y}_{i}\in C([-\tau_{i}, 0], \mathbb{R}).$ Then, there are two positive constants $\lambda$ and $M = M(\varphi^{x}, \psi ^{x}, \varphi^{y}, \psi ^{y})$ such that

$| x_{i}(t)- y_{i}(t)|\leq M e^{ -\lambda t}, \ \ \ | x_{i}'(t)- y_{i}'(t)|\leq M e^{- \lambda t}, \ \mbox{for all} \ t\geq 0, \ i\in D.$

Proof. Denote $x (t) = (x_{1}(t), x_{2}(t), \cdots, x_{n}(t))$ and $y (t) = (y_{1}(t), y_{2}(t), \cdots, y_{n}(t))$ as two solutions of (1.1) and (1.2). Let $w_{i}(t) = y_{i}(t)-x_{i}(t)$ , then

$\begin{eqnarray*} w_{i}''(t) & = &-\bar{a}_{i}(t) w_{i}'(t) -\bar{b}_{i}(t)w_{i}(t)\\&&+\sum\limits_{j = 1}^{n}\bar{c}_{ij}(t)\widetilde{A}_{j}(w_{j}(t)) +\sum\limits_{j = 1}^{n}\bar{d}_{ij}(t)\widetilde{B}_{j}(w_{j}(t-q_{ij}(t)))\\&& + \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}\theta_{ijl}(t)[Q_{j}(y_{j}( t-\eta_{ijl} (t) ))Q_{l}(y_{l}( t-\xi_{ijl} (t) ))-Q_{j}(y_{j}( t-\eta_{ijl} (t) ))\\&& \times Q_{l}(x_{l}( t-\xi_{ijl} (t) )) +Q_{j}(y_{j}(t- \eta_{ijl} (t) ))Q_{l}(x_{l}( t-\xi_{ijl} (t) )) \\&& -Q_{j}(x_{j}( t-\eta_{ijl} (t) ))Q_{l}(x_{l}( t-\xi_{ijl} (t) ))] , \end{eqnarray*}$

(2.3)

$\mbox{where } \ i, j\in D,$ $\widetilde{A}_{j}(w_{j}(t)) = A_{j}(y_{j}(t))- A_{j}(x_{j}(t))$ and

$\widetilde{B}_{j}(w_{j}(t-q_{ij}(t))) = B_{j}(y_{j}(t-q_{ij}(t))) - B_{j}(x_{j}(t-q_{ij}(t))).$

According to $(F_{2})$ and the periodicity in (1.1), one can select a constant $\lambda > 0$ such that

$\begin{align} E_{i}^{\lambda}(t) \lt 0, \ \ \ 4E_{i}^{\lambda}(t)G_{i }^{\lambda}(t) \gt (H_{i}^{\lambda}(t))^{2} , \ \forall t\in \mathbb{R}, \end{align}$

(2.4)

where

$\left\{\begin{array}{ll} E_{i} ^{\lambda}(t) = \lambda\alpha_{i}^{2}+\alpha_{i}\gamma_{i}-\bar{a}_{i}(t)\alpha_{i}^{2} + \frac{1}{2}\alpha_{i}^{2}\sum\limits_{j = 1}^{n}(|\bar{c}_{ij}(t)|L^{A}_{j} +|\bar{d}_{ij}(t)|L^{B}_{j})\\ \ \ \ \ \ \ \ \ \ +\frac{1}{2} \alpha_{i}^{2}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}+L^{Q}_{j}M^{Q}_{l}), \\ G_{i} ^{\lambda}(t) = -\bar{b}_{i}(t)\alpha_{i}\gamma_{i}+\lambda\beta_{i}+\lambda\gamma_{i}^{2} +\frac{1}{2}\sum\limits_{j = 1}^{n}(|\bar{c}_{ij}(t)|L^{A}_{j}+|\bar{d}_{ij}(t)|L^{B}_{j} )|\alpha_{i}\gamma_{i}| \\ \ \ \ \ \ \ \ \ \ +\frac{1}{2}\sum\limits_{j = 1}^{n}\alpha_{j}^{2}(|\bar{c}_{ji}(t)|L^{A}_{i} + \bar{d}_{ji}^{+} L^{B}_{i}\frac{1}{1-\dot{q} _{ji} ^{+}}e^{2\lambda q_{ji}^{+}} )\\ \ \ \ \ \ \ \ \ \ +\frac{1}{2}\sum\limits_{j = 1}^{n}(|\bar{c}_{ji}(t)|L^{A}_{i} + \bar{d}_{ji}^{+} L^{B}_{i}\frac{1}{1-\dot{q} _{ji} ^{+}}e^{2\lambda q_{ji}^{+}}) |\alpha_{j}\gamma_{j}|\\ \ \ \ \ \ \ \ \ \ +\frac{1}{2} \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\alpha_{i}\gamma_{i}||\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}+L^{Q}_{j}M^{Q}_{l}) \\ \ \ \ \ \ \ \ \ \ +\frac{1}{2} \sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|) \theta_{lji} ^{+} M^{Q}_{j}L^{Q}_{i} e^{2\lambda \xi_{lji} ^{+}} \frac{1}{1-\dot{\xi} _{lji} ^{+}} \\ \ \ \ \ \ \ \ \ \ +\frac{1}{2} \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|) \theta_{jil} ^{+}L^{Q}_{i}M^{Q}_{l} e^{2\lambda \eta_{jil} ^{+}} \frac{1}{1-\dot{\eta} _{jil} ^{+}} ) , \\ H_{i}^{\lambda}(t) = \beta_{i}+\gamma_{i}^{2}+2\lambda \alpha_{i}\gamma_{i} -\bar{a}_{i}(t)\alpha_{i}\gamma_{i}-\bar{b}_{i}(t)\alpha_{i}^{2},\ \ \ \ i,j\in D . \end{array}\right.$

Define the Lyapunov function by setting

$\begin{eqnarray*} K(t)& = & \frac{1}{2}\sum\limits_{i = 1}^{n}\beta_{i}w_{i}^{2}(t)e^{2\lambda t}+\frac{1}{2}\sum\limits_{i = 1}^{n}(\alpha_{i} w _{i}'(t)+\gamma_{i}w_{i}(t))^{2}e^{2\lambda t} \\&&+\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2} \bar{d}_{ij} ^{+}+|\alpha_{i}\gamma_{i}|\bar{d}_{ij} ^{+})e^{ 2\lambda q_{ij}^{+} }L^{B}_{j}\int_{t-q _{ij}(t)}^{t}w_{j}^{2}(s)\frac{1}{1-\dot{q} _{ij} ^{+}}e^{2\lambda s}ds \\&&+\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|) \theta_{lji} ^{+} M^{Q}_{j}L^{Q}_{i} e^{2\lambda \xi_{lji} ^{+}} \int_{t-\xi_{lji}( t) }^{t}w_{i}^{2}(s)\frac{1}{1-\dot{\xi} _{lji} ^{+}}e^{2\lambda s}ds \\&& +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|) \theta_{jil} ^{+}L^{Q}_{i}M^{Q}_{l} e^{2\lambda \eta_{jil} ^{+}} \int_{t-\eta_{jil}( t) }^{t}w_{i}^{2}(s)\frac{1}{1-\dot{\eta} _{jil} ^{+}}e^{2\lambda s}ds . \end{eqnarray*}$

Straightforward computation yields that

$K ' (t) = 2\lambda[ \frac{1}{2}\sum\limits_{i = 1}^{n}\beta_{i}w_{i}^{2}(t)e^{2\lambda t}+\frac{1}{2}\sum\limits_{i = 1}^{n}(\alpha_{i} w '_{i}(t)+\gamma_{i}w_{i}(t))^{2}e^{2\lambda t}]\\ \;\;\;+ \sum\limits_{i = 1}^{n}\beta_{i}w_{i}(t) w '_{i}(t)e^{2\lambda t}+\sum\limits_{i = 1}^{n}(\alpha_{i} w '_{i}(t)+\gamma_{i}w_{i}(t)) (\alpha_{i} w ''_{i}(t)+\gamma_{i} w '_{i}(t))e^{2\lambda t}\\ \;\;\;+\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}\bar{d}_{ij} ^{+}+|\alpha_{i}\gamma_{i}|\bar{d}_{ij} ^{+})e^{ 2\lambda q_{ij}^{+} }\frac{1}{1-\dot{q} _{ij} ^{+}}L^{B}_{j} \\ \;\;\; \times [w_{j}^{2}(t)e^{2\lambda t}-w_{j}^{2}(t-q_{ij}(t))e^{2\lambda (t-q_{ij}(t))}(1- q'_{ij}(t))] \\ \;\;\; +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|) \theta_{lji} ^{+} M^{Q}_{j}L^{Q}_{i} e^{2\lambda \xi_{lji} ^{+}}\frac{1}{1-\dot{\xi} _{lji} ^{+}} \\ \;\;\; \times [w_{i}^{2}(t)e^{2\lambda t}- w_{i}^{2}(t-\xi_{lji}( t))e^{2\lambda (t-\xi_{lji}( t))}(1- \xi_{lji}'( t))]\\ \;\;\; +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|) \theta_{jil} ^{+}L^{Q}_{i}M^{Q}_{l} e^{2\lambda \eta_{jil} ^{+}} \frac{1}{1-\dot{\eta} _{jil} ^{+}} \\ \;\;\; \times [w_{i}^{2}(t)e^{2\lambda t}-w_{i}^{2}(t-\eta_{jil}( t))e^{2\lambda (t-\eta_{jil}( t))}(1-\eta_{jil}'( t))]$

$\\ = 2\lambda[ \frac{1}{2}\sum\limits_{i = 1}^{n}\beta_{i}w_{i}^{2}(t)e^{2\lambda t}+\frac{1}{2}\sum\limits_{i = 1}^{n}(\alpha_{i}w '_{i}(t)+\gamma_{i}w_{i}(t))^{2}e^{2\lambda t}]\\ \;\;\;+\sum\limits_{i = 1}^{n}(\beta_{i}+\gamma_{i}^{2})w_{i}(t)w '_{i}(t)e^{2\lambda t}+\sum\limits_{i = 1}^{n}\alpha_{i}(\alpha_{i}w '_{i}(t)+\gamma_{i}w_{i}(t))e^{2\lambda t}\\ \;\;\;\times [-\bar{a}_{i}(t)w '_{i}(t)-\bar{b}_{i}(t)w_{i}(t)+\sum\limits_{j = 1}^{n}\bar{c}_{ij}(t)\widetilde{A}_{j}(w_{j}(t)) +\sum\limits_{j = 1}^{n}\bar{d}_{ij}(t)\widetilde{B}_{j}(w_{j}(t-q_{ij}(t))) \\ \;\;\;+ \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}\theta_{ijl}(t)(Q_{j}(y_{j}(t- \eta_{ijl} (t) ))Q_{l}(y_{l}( t-\xi_{ijl} (t) )) \\ \;\;\; -Q_{j}(y_{j}(t- \eta_{ijl} (t) ))Q_{l}(x_{l}( t-\xi_{ijl} (t) ))+Q_{j}(y_{j}( t-\eta_{ijl}( t) ))Q_{l}(x_{l}( t-\xi_{ijl} (t) ))\\ \;\;\; -Q_{j}(x_{j}( t-\eta_{ijl}( t )))Q_{l}(x_{l}(t- \xi_{ijl} (t ))))] +\sum\limits_{i = 1}^{n}\alpha_{i}\gamma_{i}(w '_{i} (t))^{2}e^{2\lambda t}\\ \ \;\;\;+\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}\bar{d}_{ij} ^{+}+|\alpha_{i}\gamma_{i}|\bar{d}_{ij} ^{+})e^{ 2\lambda q_{ij}^{+} }L^{B}_{j} \\ \;\;\; \times [w_{j}^{2}(t)\frac{1}{1-\dot{q} _{ij} ^{+}}e^{2\lambda t}-w_{j}^{2}(t-q_{ij}(t))e^{2\lambda (t-q_{ij}(t))}\frac{ 1- q'_{ij}(t)}{1-\dot{q} _{ij} ^{+}} ]\\ \;\;\; +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|) \theta_{lji} ^{+} M^{Q}_{j}L^{Q}_{i} e^{2\lambda \xi_{lji} ^{+}} \\ \;\;\; \times [w_{i}^{2}(t)\frac{1}{1-\dot{\xi} _{lji} ^{+}} e^{2\lambda t}- w_{i}^{2}(t-\xi_{lji}( t))e^{2\lambda (t-\xi_{lji}( t))} \frac{1- \xi_{lji}'( t) }{1-\dot{\xi} _{lji} ^{+}}] \\ \;\;\; +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|) \theta_{jil} ^{+}L^{Q}_{i}M^{Q}_{l} e^{2\lambda \eta_{jil} ^{+}} \\ \;\;\; \times [w_{i}^{2}(t) \frac{1}{1-\dot{\eta} _{jil} ^{+}}e^{2\lambda t}-w_{i}^{2}(t-\eta_{jil}( t))e^{2\lambda (t-\eta_{jil}( t))} \frac{1-\eta_{jil}'( t)}{1-\dot{\eta} _{jil} ^{+}} ]$

$\\ \leq e^{2\lambda t}\{\sum\limits_{i = 1}^{n}(\beta_{i}+\gamma_{i}^{2}+2\lambda \alpha_{i}\gamma_{i} -\bar{a}_{i}(t)\alpha_{i}\gamma_{i}-\bar{b}_{i}(t)\alpha_{i}^{2})w_{i}(t)w '_{i}(t) \\ \;\;\;+\sum\limits_{i = 1}^{n}(\lambda\alpha_{i}^{2}+\alpha_{i}\gamma_{i}-\bar{a}_{i}(t)\alpha_{i}^{2})(w '_{i}(t))^{2}-\sum\limits_{i = 1}^{n}(\bar{b}_{i}(t)\alpha_{i}\gamma_{i}-\lambda\beta_{i}-\lambda\gamma_{i}^{2})w_{i}^{2}(t) \\ \;\;\;+\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}\bar{d}_{ij} ^{+}+|\alpha_{i}\gamma_{i}|\bar{d}_{ij} ^{+})\frac{1}{1-\dot{q} _{ij} ^{+}}e^{2 \lambda q_{ij}^{+} }L^{B}_{j}w_{j}^{2}(t) \\ \;\;\; +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|) \theta_{lji} ^{+} M^{Q}_{j}L^{Q}_{i} e^{2\lambda \xi_{lji} ^{+}} w_{i}^{2}(t)\frac{1}{1-\dot{\xi} _{lji} ^{+}} \\ \;\;\; +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|) \theta_{jil} ^{+}L^{Q}_{i}M^{Q}_{l} e^{2\lambda \eta_{jil} ^{+}} w_{i}^{2}(t) \frac{1}{1-\dot{\eta} _{jil} ^{+}} \\ \;\;\;-\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}\bar{d}_{ij} ^{+}+|\alpha_{i}\gamma_{i}|\bar{d}_{ij} ^{+}) L^{B}_{j} w_{j}^{2}(t-q_{ij}(t)) \\ \;\;\; -\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|) \theta_{lji} ^{+} M^{Q}_{j}L^{Q}_{i} w_{i}^{2}(t-\xi_{lji}( t)) \\ \;\;\;- \frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|) \theta_{jil} ^{+}L^{Q}_{i}M^{Q}_{l} w_{i}^{2}(t-\eta_{jil}( t)) \\ \;\;\;+\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}| w '_{i}(t)|+|\alpha_{i}\gamma_{i}||w_{i}(t)|)|\bar{c}_{ij}(t)| |\widetilde{A}_{j}(w_{j}(t))|\\ \;\;\;+\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}| w '_{i}(t)|+|\alpha_{i}\gamma_{i}||w_{i}(t)|)|\bar{d}_{ij}(t)| |\widetilde{B}_{j}(w_{j}(t-q_{ij}(t)))|\}\\ \;\;\; +\sum\limits_{i = 1}^{n}(\alpha_{i}^{2}| w '_{i}(t)|+|\alpha_{i}\gamma_{i}||w_{i}(t)|)\\ \;\;\;\times \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}|w_{l}(t- \xi_{ijl} (t) ) | +L^{Q}_{j}|w_{j}( t-\eta_{ijl} (t) ) |M^{Q}_{l})\}$

$\\ = e^{2\lambda t}\{\sum\limits_{i = 1}^{n}(\beta_{i}+\gamma_{i}^{2}+2\lambda \alpha_{i}\gamma_{i} -\bar{a}_{i}(t)\alpha_{i}\gamma_{i}-\bar{b}_{i}(t)\alpha_{i}^{2})w_{i}(t) w '_{i}(t) \\ \;\;\; +\sum\limits_{i = 1}^{n}(\lambda\alpha_{i}^{2}+\alpha_{i}\gamma_{i}-\bar{a}_{i}(t)\alpha_{i}^{2}) (w '_{i}(t))^{2} \\ \;\;\; +\sum\limits_{i = 1}^{n}[-\bar{b}_{i}(t)\alpha_{i}\gamma_{i}+\lambda\beta_{i}+\lambda\gamma_{i}^{2} +\frac{1}{2}\sum\limits_{j = 1}^{n}(\alpha_{j}^{2} \bar{d}_{ji} ^{+}+|\alpha_{j}\gamma_{j}|\bar{d}_{ji} ^{+})\frac{1}{1-\dot{q} _{ji} ^{+}}e^{ 2\lambda q_{ji}^{+} }L^{B}_{i}\\ \;\;\; +\frac{1}{2} \sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|) \theta_{lji} ^{+} M^{Q}_{j}L^{Q}_{i} e^{2\lambda \xi_{lji} ^{+}} \frac{1}{1-\dot{\xi} _{lji} ^{+}} \\ \;\;\; +\frac{1}{2} \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|) \theta_{jil} ^{+}L^{Q}_{i}M^{Q}_{l} e^{2\lambda \eta_{jil} ^{+}} \frac{1}{1-\dot{\eta} _{jil} ^{+}} ]w_{i}^{2}(t) \\ \;\;\;-\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}\bar{d}_{ij} ^{+}+|\alpha_{i}\gamma_{i}|\bar{d}_{ij} ^{+}) L^{B}_{j} w_{j}^{2}(t-q_{ij}(t)) \\ \;\;\; -\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|) \theta_{lji} ^{+} M^{Q}_{j}L^{Q}_{i} w_{i}^{2}(t-\xi_{lji}( t)) \\ \;\;\;- \frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|) \theta_{jil} ^{+}L^{Q}_{i}M^{Q}_{l} w_{i}^{2}(t-\eta_{jil}( t)) \\ \;\;\;+\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}| w '_{i}(t)|+|\alpha_{i}\gamma_{i}||w_{i}(t)|)|\bar{c}_{ij}(t)| |\widetilde{A}_{j}(w_{j}(t))| \\ \;\;\;+\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}| w '_{i}(t)|+|\alpha_{i}\gamma_{i}||w_{i}(t)|)|\bar{d}_{ij}(t)| |\widetilde{B}_{j}(w_{j}(t-q_{ij}(t)))|\}\\ \;\;\; +\sum\limits_{i = 1}^{n}(\alpha_{i}^{2}| w '_{i}(t)|+|\alpha_{i}\gamma_{i}||w_{i}(t)|)\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\theta_{ijl}(t)|\\ \;\;\;\times (M^{Q}_{j}L^{Q}_{l}|w_{l}(t- \xi_{ijl} (t) ) | +L^{Q}_{j}|w_{j}( t-\eta_{ijl} (t) ) |M^{Q}_{l})\}, \ \ \forall t \in [0, +\infty).$

(2.5)

It follows from $(F_{1})$ and $PQ \leq \frac{1}{2} (P^{2} + Q^{2}) (P, Q\in \mathbb{R})$ that

$\begin{eqnarray*} &&\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}| w '_{i}(t)|+|\alpha_{i}\gamma_{i}||w_{i}(t)|)|\bar{c}_{ij}(t)| |\widetilde{A}_{j}(w_{j}(t ))|\\ &\leq&\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\alpha_{i}^{2}|\bar{c}_{ij}(t)|L^{A}_{j}( (w '_{i}(t))^{2}+w_{j}^{2}(t)) \\ &&+\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}|\alpha_{i}\gamma_{i}||\bar{c}_{ij}(t)|L^{A}_{j}(w_{i}^{2}(t) +w_{j}^{2}(t))\\ & = &\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\alpha_{i}^{2}|\bar{c}_{ij}(t)|L^{A}_{j} (w '_{i}(t))^{2} \\ &&+\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(|\alpha_{i}\gamma_{i}||\bar{c}_{ij}(t)|L^{A}_{j} +\alpha_{j}^{2}|\bar{c}_{ji}(t)|L^{A}_{i}+|\alpha_{j}\gamma_{j}||\bar{c}_{ji}(t)|L^{A}_{i})w_{i}^{2}(t), \end{eqnarray*}$

$\begin{eqnarray*} &&\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}| w '_{i}(t)|+|\alpha_{i}\gamma_{i}||w_{i}(t)|)|\bar{d}_{ij}(t)| |\widetilde{B}_{j}(w_{j}(t-q_{ij}(t)))|\\ &\leq&\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\alpha_{i}^{2}|\bar{d}_{ij}(t)|L^{B}_{j}( (w' _{i}(t))^{2}+w_{j}^{2}(t-q_{ij}(t)))\\ &&+\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}|\alpha_{i}\gamma_{i}||\bar{d}_{ij}(t)| L^{B}_{j}(w_{i}^{2}(t)+w_{j}^{2}(t-q_{ij}(t))) \\& = & \frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\alpha_{i}^{2}|\bar{d}_{ij}(t)|L^{B}_{j} (w '_{i}(t))^{2} +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}|\alpha_{i}\gamma_{i}||\bar{d}_{ij}(t)|L^{B}_{j}w_{i}^{2}(t) \\ &&+\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{i}^{2}|\bar{d}_{ij}(t)|L^{B}_{j}+|\alpha_{i}\gamma_{i}||\bar{d}_{ij}(t)|L^{B}_{j})w_{j}^{2}(t-q_{ij}(t)), \end{eqnarray*}$

and

$\begin{eqnarray*} && \sum\limits_{i = 1}^{n}(\alpha_{i}^{2}| w '_{i}(t)|+|\alpha_{i}\gamma_{i}||w_{i}(t)|)\\&&\times \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}|w_{l}( t-\xi_{ijl} (t) ) | +L^{Q}_{j}|w_{j}(t- \eta_{ijl} (t) ) |M^{Q}_{l}) \\ &\leq&\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}\alpha_{i}^{2}|\theta_{ijl}(t)|M^{Q}_{j}L^{Q}_{l}( (w' _{i}(t))^{2}+w_{l}^{2}(t-\xi_{ijl} (t) )) \\&& +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\alpha_{i}\gamma_{i}||\theta_{ijl}(t)|M^{Q}_{j}L^{Q}_{l} ( (w _{i}(t))^{2}+w_{l}^{2}(t-\xi_{ijl} (t) ))\\&& + \frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}\alpha_{i}^{2}|\theta_{ijl}(t)|L^{Q}_{j}M^{Q}_{l}( (w' _{i}(t))^{2}+w_{j}^{2}(t-\eta_{ijl}( t) )) \\&& +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\alpha_{i}\gamma_{i}||\theta_{ijl}(t)|L^{Q}_{j}M^{Q}_{l} ( (w _{i}(t))^{2}+w_{j}^{2}(t-\eta_{ijl} (t) )) \\& = & \frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}\alpha_{i}^{2}|\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}+L^{Q}_{j}M^{Q}_{l}) (w' _{i}(t))^{2} \\&& +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\alpha_{i}\gamma_{i}||\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}+L^{Q}_{j}M^{Q}_{l}) (w _{i}(t))^{2}\\&& +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{i}^{2}+|\alpha_{i}\gamma_{i}|)|\theta_{ijl}(t)|M^{Q}_{j}L^{Q}_{l} w_{l}^{2}(t-\xi_{ijl} (t) ) \\&& +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{i}^{2}+|\alpha_{i}\gamma_{i}|)|\theta_{ijl}(t)|L^{Q}_{j}M^{Q}_{l} w_{j}^{2}(t-\eta_{ijl} (t) ) \\ & = & \frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}\alpha_{i}^{2}|\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}+L^{Q}_{j}M^{Q}_{l}) (w' _{i}(t))^{2} \\&& +\frac{1}{2}\sum\limits_{i = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\alpha_{i}\gamma_{i}||\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}+L^{Q}_{j}M^{Q}_{l}) (w _{i}(t))^{2}\\&& +\frac{1}{2}\sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}\sum\limits_{i = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|)|\theta_{lji}(t)|M^{Q}_{j}L^{Q}_{i} w_{i}^{2}(t-\xi_{lji} (t) ) \\&& +\frac{1}{2}\sum\limits_{j = 1}^{n}\sum\limits_{i = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|)|\theta_{jil}(t)|L^{Q}_{i}M^{Q}_{l} w_{i}^{2}(t-\eta_{jil} (t) ) , \end{eqnarray*}$

which, together with (2.4) and (2.5), entails that

$\begin{eqnarray*} K'(t)&\leq&e^{2\lambda t}\{\sum\limits_{i = 1}^{n}(\beta_{i}+\gamma_{i}^{2}+2\lambda \alpha_{i}\gamma_{i} -\bar{a}_{i}(t)\alpha_{i}\gamma_{i}-\bar{b}_{i}(t)\alpha_{i}^{2})w_{i}(t) w '_{i}(t) \\ &&+\sum\limits_{i = 1}^{n}[\lambda\alpha_{i}^{2}+\alpha_{i}\gamma_{i}-\bar{a}_{i}(t)\alpha_{i}^{2} + \frac{1}{2}\alpha_{i}^{2}\sum\limits_{j = 1}^{n}(|\bar{c}_{ij}(t)|L^{A}_{j} +|\bar{d}_{ij}(t)|L^{B}_{j})\\&& +\frac{1}{2} \alpha_{i}^{2}\sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}+L^{Q}_{j}M^{Q}_{l})](w '_{i}(t))^{2} \\ &&+\sum\limits_{i = 1}^{n}[-\bar{b}_{i}(t)\alpha_{i}\gamma_{i}+\lambda\beta_{i}+\lambda\gamma_{i}^{2} +\frac{1}{2}\sum\limits_{j = 1}^{n}(|\bar{c}_{ij}(t)|L^{A}_{j}+|\bar{d}_{ij}(t)|L^{B}_{j} )|\alpha_{i}\gamma_{i}| \\ &&+\frac{1}{2}\sum\limits_{j = 1}^{n}\alpha_{j}^{2}(|\bar{c}_{ji}(t)|L^{A}_{i} + \bar{d}_{ji}^{+} L^{B}_{i}\frac{1}{1-\dot{q} _{ji} ^{+}}e^{2\lambda q_{ji}^{+}} ) \\ && +\frac{1}{2}\sum\limits_{j = 1}^{n}(|\bar{c}_{ji}(t)|L^{A}_{i} + \bar{d}_{ji}^{+} L^{B}_{i}\frac{1}{1-\dot{q} _{ji} ^{+}}e^{2\lambda q_{ji}^{+}}) |\alpha_{j}\gamma_{j}|\\&& +\frac{1}{2} \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}|\alpha_{i}\gamma_{i}||\theta_{ijl}(t)|(M^{Q}_{j}L^{Q}_{l}+L^{Q}_{j}M^{Q}_{l}) \\&& +\frac{1}{2} \sum\limits_{l = 1}^{n}\sum\limits_{j = 1}^{n}(\alpha_{l}^{2}+|\alpha_{l}\gamma_{l}|) \theta_{lji} ^{+} M^{Q}_{j}L^{Q}_{i} e^{2\lambda \xi_{lji} ^{+}} \frac{1}{1-\dot{\xi} _{lji} ^{+}} \\&& +\frac{1}{2} \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}(\alpha_{j}^{2}+|\alpha_{j}\gamma_{j}|) \theta_{jil} ^{+}L^{Q}_{i}M^{Q}_{l} e^{2\lambda \eta_{jil} ^{+}} \frac{1}{1-\dot{\eta} _{jil} ^{+}} ) ]w_{i}^{2}(t)\}\\ & = & e^{2\lambda t}\{\sum\limits_{i = 1}^{n}(E^{\lambda}_{i} (t)(w '_{i}(t))^{2}+G^{\lambda}_{i}(t) w_{i}^{2}(t)+H^{\lambda}_{i}(t)w_{i}(t) w '_{i}(t))\}\\ & = &e^{2\lambda t}\{ \sum\limits_{i = 1}^{n}E_{i}^{\lambda}(t)( w '_{i}(t)+\frac{H_{i}^{\lambda}(t)}{2E_{i}^{\lambda}(t)}w_{i}(t))^{2} +\sum\limits_{i = 1}^{n}(G_{i}^{\lambda}(t)-\frac{(H^{\lambda}_{i}(t)) ^{2}}{4E_{i}^{\lambda}(t)}) w_{i}^{2}(t)\} \\ &\leq&0, \ \ \forall t \in [0, +\infty). \end{eqnarray*}$

This indicates that $K (t)\leq K (0)$ for all $t \in [0, +\infty)$ , and

$\frac{1}{2}\sum\limits_{i = 1}^{n}\beta_{i}w_{i}^{2}(t)e^{2\lambda t}+\frac{1}{2}\sum\limits_{i = 1}^{n}(\alpha_{i} w '_{i}(t)+\gamma_{i}w_{i}(t))^{2}e^{2\lambda t} \leq K (0), \ t \in [0, +\infty).$

Note that

$(\alpha_{i} w'_{i}(t) e^{ \lambda t}+\gamma_{i}w_{i}(t)e^{ \lambda t})^{2} = (\alpha_{i}w '_{i}(t)+\gamma_{i}w_{i}(t))^{2}e^{2\lambda t}$

and

$\alpha_{i}| w '_{i}(t)| e^{ \lambda t}\leq |\alpha_{i} w'_{i}(t) e^{ \lambda t}+\gamma_{i}w_{i}(t)e^{ \lambda t}|+| \gamma_{i}w_{i}(t)e^{ \lambda t}|,$

one can find a constant $M > 0$ such that

$| w '_{i}(t)|\leq M e^{ -\lambda t}, \ \ \ | w_{i}(t)|\leq M e^{- \lambda t}, \ \ t\geq 0, \ i\in D ,$

which proves Lemma 2.1.

Remark 2.2. Under the assumptions adopted in Lemma 2.1, if $y (t)$ is an equilibrium point or a periodic solution of (1.1), one can see $y (t)$ is globally exponentially stable. Moreover, the definition of global exponential stability can be also seen in ^[13,16].

3. Anti-periodicity of system (1.1)

Now, we set out the main result of this paper as follows.

Theorem 3.1. Under assumptions $(F_{1})$ – $(F_{3})$ , system (1.1) possesses a global exponential stable $T$ -anti-periodic solution.

Proof. Denote $\kappa(t) = (\kappa_{1}(t), \kappa_{2}(t), \cdots, \ \kappa_{n}(t))$ be a solution of system (1.1) satisfying:

$\begin{align} \kappa_{i}(s) = \varphi^{\kappa}_{i}(s), \ \ \kappa_{i}'(s) = \psi^{\kappa}_{i}(s), \ -\tau_{i} \leq s \leq 0, \ \varphi^{\kappa}_{i}, \psi^{\kappa}_{i}\in C([ -\tau_{i} , 0], \mathbb{R}), \ i\in D. \end{align}$

(3.1)

With the aid of $(F_{1})$ , one can see that

$\bar{a}_{i}(t+T) = \bar{a}_{i}(t ), \ \ \bar{b}_{i}(t+T) = \bar{b}_{i}(t ), \ \ q_{ij}(t+T) = q_{ij}(t ) ,$

$\eta_{ijl}(t+T) = \eta_{ijl}(t ) , \ \ \xi_{ijl}(t+T) = \xi_{ijl}(t ), \ \ J_{i}(t+T) = -J_{i}(t ),$

$(-1)^{m+1}\bar{c}_{ij}(t+ (m+1)T)A_{j}(\kappa_{j}(t+ (m+1)T )) = \bar{c}_{ij}(t)A_{j}((-1)^{m+1}\kappa_{j}(t+ (m+1)T )),$

$(-1)^{m+1}\bar{d}_{ij}(t+ (m+1)T)B_{j}(\kappa_{j}( t+ (m+1)T-q_{ij}(t )) ) = \bar{d}_{ij}(t)B_{j}((-1)^{m+1}\kappa_{j}( t+ (m+1)T-q_{ij}(t )) ),$

and

$(-1)^{m+1}\theta_{ijl}(t+ (m+1)T) Q_{j}(\kappa_{j}(t+ (m+1)T- \eta_{ijl} (t ) )) Q_{l}( \kappa_{l}( t+ (m+1)T-\xi_{ijl} (t ) ))$

$= \theta_{ijl}(t) Q_{j}((-1)^{m+1}\kappa_{j}(t+ (m+1)T- \eta_{ijl} (t ) )) Q_{l}((-1)^{m+1}\kappa_{l}( t+ (m+1)T-\xi_{ijl} (t ) )),$

where $\ t\in \mathbb{R}$ and $i, j, l\in D$ .

Consequently, for any nonnegative integer $m,$

$\begin{eqnarray*} \ \ \ \ && ((-1)^{m+1}\kappa_{i} (t + (m+1) T))'' \\& = &-\bar{a}_{i}(t)((-1)^{m+1}\kappa_{i} (t + (m+1) T))'-\bar{b}_{i}(t)((-1)^{m+1}\kappa_{i}(t+ (m+1) T)) \\&&+\sum\limits_{j = 1}^{n}\bar{c}_{ij}(t)A_{j}((-1)^{m+1}\kappa_{j}(t+ (m+1)T )) \\&& +\sum\limits_{j = 1}^{n}\bar{d}_{ij}(t)B_{j}((-1)^{m+1}\kappa_{j}( t+ (m+1)T-q_{ij}(t )) )+ \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}\theta_{ijl}(t) \\&& \times Q_{j}((-1)^{m+1}\kappa_{j}(t+ (m+1)T- \eta_{ijl} (t ) )) \\&& \times Q_{l}((-1)^{m+1}\kappa_{l}( t+ (m+1)T-\xi_{ijl} (t ) ))\\&& +J_{i}(t) , \ \mbox{for all} \ i\in D, t+(m+1)T\geq 0. \end{eqnarray*}$

(3.2)

Clearly, $(-1)^{m+1} \kappa (t + (m+1)T) \ (t+(m+1)T\geq 0)$ satisfies (1.1), and $v(t) = -\kappa (t + T)$ is a solution of system (1.1) involving initial values:

$\varphi^{v}_{i}(s) = -\kappa_{i}(s+T), \psi^{v}_{i}(s) = -\kappa_{i}'(s+T), \ \mbox{for all} \ s\in [ -\tau_{i}, 0], i\in D.$

Thus, with the aid of Lemma 2.1, we can pick a constant $M = M(\varphi^{\kappa}, \psi ^{\kappa}, \varphi^{v}, \psi ^{v})$ satisfying

$| \kappa_{i}(t)- v_{i}(t)|\leq M e^{-\lambda t} , \ \ \ | \kappa_{i}'(t)- v_{i}'(t)|\leq M e^{-\lambda t} ,\ \ \mbox{for all} \ t\geq 0, i\in D.$

Hence,

$\begin{align} \left. \begin{array}{ll} | (-1)^{p } \kappa_{i}(t+pT)- (-1)^{p+1}\kappa _{i}(t+(p+1)T)|\\ = | \kappa_{i}(t+pT )- v_{i}(t+pT)| \leq M e^{-\lambda (t+pT)} , \\ | ((-1)^{p }\kappa_{i}(t+pT))'- ((-1)^{p+1}\kappa_{i}(t+(p+1)T))'|\\ = | \kappa_{i}'(t+pT)- v_{i}'(t+pT)| \leq M e^{-\lambda (t+pT)} , \end{array} \right \} \ \forall i\in D, \ t+pT\geq 0. \end{align}$

(3.3)

Consequently,

$(-1)^{m+1}\kappa_{i}(t+(m+1)T) = \kappa_{i} (t ) +\sum\limits_{p = 0}^{m }[ (-1)^{p+1}\kappa _{i}(t + (p+1)T)- (-1)^{p }\kappa_{i} (t + pT)] \ (i\in D)$

and

$\begin{eqnarray*} &&((-1)^{m+1}\kappa_{i}(t+(m+1)T))'\\& = & \kappa_{i} '(t ) +\sum\limits_{p = 0}^{m }[ ((-1)^{p+1}\kappa _{i}(t + (p+1)T))'- ((-1)^{p }\kappa_{i} (t + pT))'] \ (i\in D). \end{eqnarray*}$

Therefore, (3.3) suggests that there exists a continuous differentiable function $y (t) = (y_{1} (t), y_{2} (t), \cdots, y_{n} (t))$ such that $\{ (-1)^{m }\kappa (t + mT)\}_{m\geq 1}$ and $\{ ((-1)^{m }\kappa (t + mT))'\}_{m\geq 1}$ are uniformly convergent to $y (t)$ and $y'(t)$ on any compact set of $\mathbb{R}$ , respectively.

Moreover,

$y(t+T) = \lim\limits_{m\rightarrow +\infty}(-1)^{m }\kappa (t +T+ m T) = -\lim\limits_{(m+1)\rightarrow +\infty}(-1)^{m +1} \kappa (t + (m +1)T) = -y(t )$

involves that $y(t)$ is $T -$ anti-periodic on $\mathbb{R}$ . It follows from ( $F_1$ )-( $F_3$ ) and the continuity on (3.2) that $\{(\kappa'' (t + (m+1)T) \}_{m\geq 1}$ uniformly converges to a continuous function on any compact set of $\mathbb{R}$ . Furthermore, for any compact set of $\mathbb{R}$ , setting $m \longrightarrow+\infty$ , we obtain

$\begin{eqnarray*} y_{i}''(t)& = &-\bar{a}_{i}(t)y_{i}'(t)-\bar{b}_{i}(t)y_{i}(t) +\sum\limits_{j = 1}^{n}\bar{c}_{ij}(t)A_{j}(y_{j}(t)) +\sum\limits_{j = 1}^{n}\bar{d}_{ij}(t)B_{j}(y_{j}(t- q_{ij}( t)) )\\&& + \sum\limits_{j = 1}^{n}\sum\limits_{l = 1}^{n}\theta_{ijl}(t)Q_{j}(y_{j}( t-\eta_{ijl} (t) ))Q_{l}(y_{l}( t-\xi_{ijl}( t) ))+J_{i}(t),, i\in D, \end{eqnarray*}$

which involves that $y(t)$ is a $T -$ anti-periodic solution of (1.1). Again from Lemma 2.1, we gain that $y(t)$ is globally exponentially stable. This finishes the proof of Theorem 3.1.

Remark 3.1. For inertial neural networks without high-order terms respectively, suppose

$\begin{align} |\bar{a}_{i}-\bar{b}_{i} | \lt 2, \ A_{i} \ \mbox{ and }\ B_{i} \ \mbox{are bounded,} \ i\in D, \end{align}$

(3.4)

and

$\begin{align} |\bar{a}_{i}-\bar{b}_{i}+1| \lt 1, i\in D, \end{align}$

(3.5)

the authors gained the existence and stability on periodic solutions in ^[10,11] and anti-periodic solutions in ^[12]. Moreover, the reduced-order method was crucial in ^[10,11,12] when anti-periodicity and periodicity of second-order inertial neural networks were considered. However, (3.4) and (3.5) have been abandoned in Theorem 3.1 and the reduced-order method has been substituted in this paper. Therefore, our results on anti-periodicity of high-order inertial Hopfield neural networks are new and supplemental in nature.

4. Examples and numerical simulations

Example 4.1. Let $n = 2$ , and consider a class of high-order inertial Hopfield neural networks in the form of

$\begin{align} \left\{ \begin{array}{ll} x_{1}''(t) \\ = -14.92 x_{1}'(t) -27.89x_{1}(t)+ 2.28(\sin t) A_{1}(x_{1}(t))\\ \ \ \ + 2.19 (\cos t) A_{2}(x_{2}(t)) \\ \ \ \ -0.84 (\cos 2t) B_{1}(x_{1}( t-0.2 \sin^{2} t ))+2.41 (\cos 2t )B_{2}(x_{2}( t-0.3 \sin^{2} t ))\\ \ \ \ +4 (\sin 2t)Q_{1}(x_{1}(t-0.4 \sin^{2} t )) Q_{2}(x_{2}(t-0.5 \sin^{2} t ))+55\sin t , \\ x_{2}''(t) \\ = -15.11 x_{2}'(t) -31.05x_{2}(t)- 1.88 (\sin t) A_{1}(x_{1}(t))\\ \ \ \ - 2.33 (\cos t) A_{2}(x_{2}(t)) \\ \ \ \ -2.18 (\sin 2t) B_{1}(x_{1}( t-0.2 \cos^{2} t ))+3.18(\cos 2t) B_{2}(x_{2}( t-0.3 \cos^{2} t ))\\ \ \ \ +3.8 (\sin 2t)Q_{1}(x_{1}( t-0.4 \cos^{2} t )) Q_{2}(x_{2}( t-0.5 \cos^{2} t ))+48\sin t , \end{array} \right. \end{align}$

(4.1)

where $t\geq 0, \ A_{1}(u) = A_{2}(u) = \frac{1}{35} |u |, \ B_{1}(u) = B_{2}(u) = \frac{1}{48} u, \ Q_{1}(u) = Q_{2}(u) = \frac{1}{55} \arctan u.$

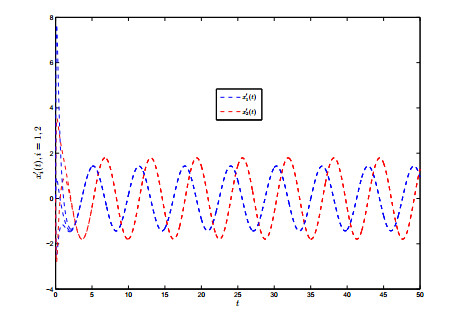
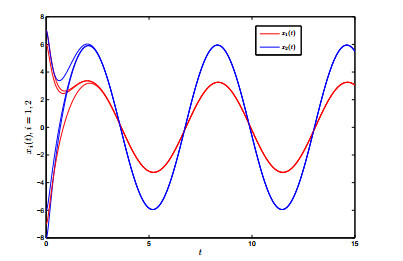
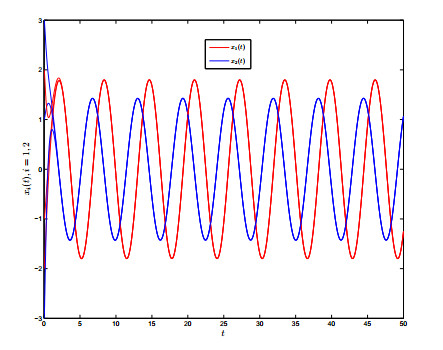
Using a direct calculation, one can check that (4.1) satisfies $(2.4)$ and $(F_{1})-(F_{3})$ . Applying Theorem 3.1, it is obvious that system (4.1) has a globally exponentially stable $\pi$ -anti-periodic solution. Simulations reflect that the theoretical anti-periodicity is in sympathy with the numerically observed behavior (Figures 1 and 2).

Figure 1. Numerical solutions

$x(t)$ to system (4.1) with initial values:

$(\varphi_1(s), \varphi_2(s), \psi_1(s), \psi_2(s))\equiv (-1, 3, 0, 0), (-2, 1, 0, 0), (2, -3, 0, 0), s\in[-5, 0]$ .

DownLoad: Full-Size Img PowerPoint

Figure 2. Numerical solutions

$x'(t)$ to system (4.1) with initial value

$(\varphi_1(s), \varphi_2(s), \psi_1(s), \psi_2(s)) \equiv (-1, 3, 0, 0), (-2, 1, 0, 0), (2, -3, 0, 0), s\in[-5, 0]$ .

DownLoad: Full-Size Img PowerPoint

Example 4.2. Regard the following high-order inertial Hopfield neural networks involving time-varying delays and coefficients:

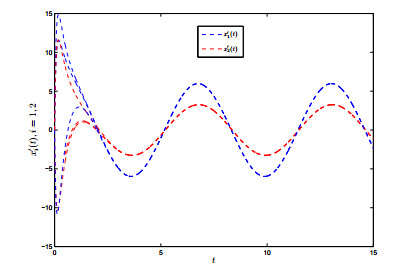
$\begin{align} \left\{ \begin{array}{ll} x_{1}''(t) \\ = -(14+0.9 |\sin t|) x_{1}'(t) -(27+0.8|\cos t|)x_{1}(t)+ 2.28(\sin t) A_{1}(x_{1}(t))\\ \ \ \ + 2.19 (\cos t) A_{2}(x_{2}(t)) \\ \ \ \ -0.84 (\cos 2t) B_{1}(x_{1}( t-0.2 \sin^2 t ))+2.41 (\cos 2t )B_{2}(x_{2}( t-0.3 \sin^2 t ))\\ \ \ \ +4 (\sin t)Q_{1}(x_{1}(t-0.4 \sin^2 t)) Q_{2}(x_{2}(t-0.5 \sin^2 t))+100\sin t , \\ x_{2}''(t) \\ = -(15+0.1 |\cos t|) x_{2}'(t) -(31+0.1|\sin t|)x_{2}(t)- 1.88 (\sin t) A_{1}(x_{1}(t))\\ \ \ \ - 2.33 (\cos t) A_{2}(x_{2}(t)) \\ \ \ \ -2.18 (\sin 2t) B_{1}(x_{1}( t-0.2 \cos^2 t ))+3.18(\cos 2t) B_{2}(x_{2}( t-0.3 \cos^2 t ))\\ \ \ \ +3.8 (\sin t)Q_{1}(x_{1}( t-0.4 \cos^2 t)) Q_{2}(x_{2}( t-0.5 \cos^2 t))+200\sin t , \end{array} \right. \end{align}$

(4.2)

where $t\geq 0, \ A_{1}(u) = A_{2}(u) = \frac{1}{35} |u |, \ B_{1}(u) = B_{2}(u) = \frac{1}{48} u, \ Q_{1}(u) = Q_{2}(u) = \frac{1}{110} (|x+1| -|x-1|).$ Then, by Theorem 3.1, one can find that all solutions of networks (4.2) are convergent to a $\pi$ -anti-periodic solution (See Figures 3 and 4).

Figure 3. Numerical solutions

$x(t)$ to system (4.2) with initial values:

$(\varphi_1(s), \varphi_2(s), \psi_1(s), \psi_2(s)) \equiv (6, -8, 0, 0), (7, -6, 0, 0), (-7, 7, 0, 0), s\in[-0.5, 0]$ .

DownLoad: Full-Size Img PowerPoint

Figure 4. Numerical solutions

$x'(t)$ to system (4.2) with initial value

$(\varphi_1(s), \varphi_2(s), \psi_1(s), \psi_2(s)) \equiv (6, -8, 0, 0), (7, -6, 0, 0), (-7, 7, 0, 0), s\in[-0.5, 0]$ .

DownLoad: Full-Size Img PowerPoint

Remark 4.1. From the –, one can see that the solution is similar to sinusoidal oscillation, and there exists a $\pi$ -anti-periodic solution satisfying $x (t+\pi) = -x (t)$ . To the author's knowledge, the anti-periodicity on high-order inertial Hopfield neural networks involving time-varying delays has never been touched by using the non-reduced order method. Manifestly, the assumptions (3.4) and (3.5) adopted in ^[10,11] are invalid in systems (4.1) and (4.2). In addition, the most recently papers ^[10,11] only considered the polynomial power stability of some proportional time-delay systems, but not involved the exponential power stability of the addressed systems. And the results in ^{[35,36,37,38,39,40,41,42,43,44,45,46,47,48,49,50,51,52,53,54,55,56,57,58,59,60,61,62,63,64,65,66,67}^,68,69,^{70,71,72,73,74,} ^{75,76,77,78,79,80,81,82]} have not touched on the anti-periodicity of inertial neural networks. This entails that the corresponding conclusions in ^{[10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34,35,36,37,38,39,40,}^{41,42,43,44,45,46,47,48,} ^{49,50,51,52,53,54,55,56}^,57,58,^{59,60,61,62,63,64,65,66,67,68,69,70,71,72,73,74,75,76}^{,77,78,79,80,81,82]} and the references cited therein can not be applied to show the anti-periodic convergence for systems (4.1) and (4.2).

5. Conclusion

In this paper, abandoning the reduced order method, we apply inequality techniques and Lyapunov function method to establish the existence and global exponential stability of anti-periodic solutions for a class of high-order inertial Hopfield neural networks involving time-varying delays and anti-periodic environments. The obtained results are essentially new and complement some recently published results. The method proposed in this article furnishes a possible approach for studying anti-periodic on other types high-order inertial neural networks such as shunting inhibitory cellular neural networks, BAM neural networks, Cohen-Grossberg neural networks and so on.

Acknowledgments

The authors would like to express the sincere appreciation to the editor and reviewers for their helpful comments in improving the presentation and quality of the paper.

Conflict of interest

The authors confirm that they have no conflict of interest.

References

[1]	Andrews JT, Carrara PE, King FB, et al. (1975) Holocene environmental changes in the Alpine Zone, Northern San Juan Mountains, Colorado: Evidence from Bog stratigraphy and palynology. Quat Res 5: 173–197. doi: 10.1016/0033-5894(75)90022-8
[2]	Petersen KL, Mehringer PJ (1976) Postglacial timberline fluctuations, La Plata Mountains, Southwestern Colorado. Arct Alp Res 8: 275–288. doi: 10.2307/1550475
[3]	Carrara PE, Mode WN, Rubin M, et al. (1984) Deglaciation and postglacial timberline in the San Juan Mountains, Colorado. Quat Res 21: 42–55. doi: 10.1016/0033-5894(84)90088-7
[4]	Friedman I, Carrara P, Gleason J (1988) Isotopic evidence of Holocene climatic change in the San Juan Mountains, Colorado. Quat Res 30: 350–353. doi: 10.1016/0033-5894(88)90010-5
[5]	Elias SA, Carrara PE, Toolin LJ, et al. (1991) Revised age of deglaciation of Lake Emma based on new radiocarbon and macrofossil analysis: Quat Res 36: 307–321.
[6]	Gillam ML (1998) Late Cenozoic geology and soils of the Lower Animas River Valley, Colorado and New Mexico: Boulder. University of Colorado, 477.
[7]	Benson L, Madole R, Landis G, et al. (2005) New data for late Pleistocene Pinedale glaciation from southwestern Colorado. Quat Sci Rev 24: 49–65. doi: 10.1016/j.quascirev.2004.07.018
[8]	Toney JL, Anderson RS (2006) A postglacial palaeoecological record from the San Juan Mountains of Colorado USA: fire, climate and vegetation history. Holocene 16: 505–517. doi: 10.1191/0959683606hl946rp
[9]	Guido ZS, Ward DJ, Anderson RS (2007) Pacing the post-Glacial Maximum demise of the Animas Valley glacier and the San Juan Mountain ice cap, Colorado. Geology 35: 739–742. doi: 10.1130/G23596A.1
[10]	Ward DJ, Anderson RS, Guido ZS, et al. (2009) Numerical modeling of cosmogenic deglaciation records, Front Range and San Juan mountains, Colorado. J Geophys Res Earth Surface 114: F01026.
[11]	Mason C, Ruleman CA, Kenny R (2011) Rate and timing of deglaciation using ¹⁰Be cosmogenic nuclide surface exposure dating, Mt. Massive Wilderness, Colorado, USA. Geol Soc Am Abst Prog 43: 65.
[12]	Young NE, Briner JP, Leonard EM, et al. (2011) Assessing climatic and nonclimatic forcing of Pinedale glaciation and deglaciation in the western United States. Geology 39: 171–174. doi: 10.1130/G31527.1
[13]	Dorale JA, Edwards RL, Ito E, et al. (1998) Climate and vegetation history of the midcontinent from 75 to 25 ka: a speleothem record from Crevice Cave, Missouri, USA. Science 282: 1871–1874.
[14]	Dorale JA, Edwards RL, Alexander EC Jr, et al. (2001) Uranium-series dating of speleothems: Current techniques, limits, and applications. In: Sasowsky ID, Mylroi JE (eds), Study of Cave Sediments: Physical and Chemical records of palaeoclimate, Newark: Kluwar/Plenum, 177–197.
[15]	Richards DA, Dorale JA (2003) Uranium-series chronology and environmental applications of speleothem. Rev Mineral Geochem 52: 407–460. doi: 10.2113/0520407
[16]	Asmerom Y (2009) Speleothems, In: Gornitz V (ed), Encyclopedia of Paleoclimatology and ancient environments, The Netherlands, Springer, 916–918.
[17]	Spötl C, Mangini A, Richards DA (2006) Chronology and paleoenvironment of Marine Isotope Stage 3 from two high-elevation speleothems, Austrian Alps. Quat Sci Rev 25: 1127–1136. doi: 10.1016/j.quascirev.2005.10.006
[18]	Hendy CH (1971) The isotopic geochemistry of speleothems, I. The calculation of the effects of different modes of formation on the isotopic composition of speleothems and their applicability as paleoclimatic indicators. Geochem Cosmochem Acta 35: 801–824.
[19]	Spötl C, Mangini A (2002) Stalagmite from the Austrian Alps reveals Dansgaard-Oeschger events during isotope stage 3: Implications for the absolute chronology of Greenland ice cores. Earth Planet Sci Lettr 203: 507–518. doi: 10.1016/S0012-821X(02)00837-3
[20]	Moseley GE, Spötl C, Svensson A, et al. (2014) Multi-speleothem record reveals tightly coupled climate between central Europe and Greenland during Marine Isotope Stage 3. Geology 42: 1043–1046. doi: 10.1130/G36063.1
[21]	Dykoski CA, Edwards RL, Cheng H, et al. (2005) A high-resolution, absolute-dated Holocene and deglacial Asian monsoon record from Dongge Cave, China. Earth Planet Sci Lett 233: 71–86. doi: 10.1016/j.epsl.2005.01.036
[22]	Neff U, Burns SJ, Mangini A, et al. (2001) Strong coherence between solar variability and the monsoon in Oman between 9 and 6 kyr ago. Nature 411: 290–294. doi: 10.1038/35077048
[23]	Berstad IM, Lundberg J, Lauritzen SE, et al. (2002) Comparison of the climate during Marine Isotope Stage 9 and 11 inferred from a speleothem isotope record from northern Norway. Quat Res 58: 361–371. doi: 10.1006/qres.2002.2387
[24]	Wang Y, Cheng H, Edwards RE, et al. (2005) The Holocene Asian monsoon: links to solar changes and North Atlantic climate. Science 308: 854–857. doi: 10.1126/science.1106296
[25]	Mangini A, Spötl C, Verdes P (2005) Reconstruction of temperature in the Central Alps during the past 2000 years from a δ¹⁸O stalagmite record. Earth Planet Sci Lettr 235: 741–751. doi: 10.1016/j.epsl.2005.05.010
[26]	Genty D, Blamart D, Ouahdi R, et al. (2003) Precise dating of Dansgaard-Oeschger climate oscillations in western Europe from stalagmite data. Nature 421: 833–837. doi: 10.1038/nature01391
[27]	Denniston RF, González LA, Asmerom Y, et al. (2000) Speleothem records of early and late Holocene vegetation dynamics in the Ozark Highlands, USA. Quat Int 67: 21–27. doi: 10.1016/S1040-6182(00)00005-7
[28]	McDermott F (2004) Palaeo-climate reconstruction from stable isotope variations in speleothems: A review. Quat Sci Rev 23: 901–918. doi: 10.1016/j.quascirev.2003.06.021
[29]	Atwood WW, Mather KF (1932) Physiography and Quaternary geology of the San Juan Mountains, Colorado. Washington, D.C.: USGS Prof Paper 166: 176.
[30]	Porter SC, Pierce KL, Hamilton TD (1983) Late Wisconsin mountain glaciation in the western United States, In: Porter SC (ed), Late Quaternary environments of the United States, v. 1, The late Pleistocene, Minneapolis: U of Minnesota Press: 1–111.
[31]	Benson L, Madole R, Phillips W, et al. (2004) The probable importance of snow and sediment shielding on cosmogenic ages of north-central Colorado Pinedale and pre-Pinedale moraines. Quat Sci Rev 23: 193–206. doi: 10.1016/j.quascirev.2003.07.002
[32]	Medville D (2001) The exploration and survey of Surprise Cave. NSS News 59: 288–289.
[33]	Atkinson TC (1983) Growth mechanisms of speleothems in Castleguard Cave, Columbia Icefields, Alberta, Canada. Arct Alp Res 15: 523–536. doi: 10.2307/1551238
[34]	Rangwala I (2008). 20th Century Climate Change: Chapter 5, The San Juan Mountains in Southwest Colorado: Investigating long term trends in climate and hydrological variables and explaining the causes for a rapid climate change in the region between 1985–2005: New Jersey, Rutgers University, 35.
[35]	Ray AJ, Barsugli JJ, Averyt KB (2008) Climate change in Colorado: A synthesis to support water resources management and adaptation: Western Water assessment for Colorado Water Conservation, Paper 53. Available from: http://cwcb.state.co.us/Home/ClimateChange/ClimateChangeInColoradoReport/
[36]	Mote PW, Hamlet AF, Clark M, et al. (2005) Declining mountain snowpack in western North America. Bull Am Meteorol Soc 86: 39–49. doi: 10.1175/BAMS-86-1-39
[37]	Clow D (2008) Changes in the Timing of Snowmelt in Colorado. In: Presentation at the 50th Annual Convention of the Colorado Water Congress, January: 23–25.
[38]	Carrara PE (2011) Deglaciation and Postglacial treeline fluctuation in the Northern San Juan Mountains, Colorado. Washington, D.C.: USGS Prof Paper 1782: 48.
[39]	Musgrove M, Banner JL, Mack LE, et al. (2001) Geochronology of late Pleistocene to Holocene speleothems from central Texas: Implications for regional paleoclimate. GSA Bull 113: 1532–1543. doi: 10.1130/0016-7606(2001)113<1532:GOLPTH>2.0.CO;2
[40]	Fanditis J, Ehhalt DH (1970) Variations of the carbon and oxygen isotopic composition in stalagmites and stalactites: Evidence of non-equilibrium isotopic fractionation. Earth Planet Sci Lett 10: 136–144. doi: 10.1016/0012-821X(70)90075-0
[41]	Gascoyne M, Schwarcz HP, Ford DC (1980) A palaeotemperature record for the mid-Wisconsin in Vancouver Island. Nature 285: 474–476. doi: 10.1038/285474a0
[42]	Polyak VJ, Asmerom Y (2001) Late Holocene climate and cultural changes in the southwestern United States. Science 294: 148–151. doi: 10.1126/science.1062771
[43]	Gilson JR, Macarthney E (1954) Luminescence of speleothems from Devon, UK: the presence of organic activators (abs). Ashford Speleo Soc J 6: 8–11.
[44]	Lauritzen SE, Ford DC, Schwarcz HP (1986) Humic substances in speleothems matrix-paleoclimatic significance. Proceedings of 9th International Congress of Speleology, Barcelona, 2: 77–79.
[45]	Shopov YY (2001) Luminescence of cave minerals. Bull Venezuelan Speleo Soc 35: 27–33.
[46]	White WB, Brennan ES (1989) Luminescence of speleothems due to fulvic acid and other activators. Proceedings of 10th International Congress of Speleology, Budapest 1: 212–214.
[47]	Shopov YY (1997) Luminescence of cave minerals. In: Hill C, Forti P (eds), Cave Minerals of the World, 2^nd Ed, Huntsville, Alabama: National speleological society, 244–248.
[48]	Ford DC (1997) Dating and Paleo-environmental studies of speleothems. In: Hil C, Forti P (eds), Cave Minerals of the World, 2^nd Ed, Huntsville, Alabama: National speleological society: 271–284.
[49]	McGarry SF, Baker A (2000) Organic acid fluorescence: applications to speleothem palaeoenvironmental reconstruction. Quat Sci Rev 19: 1087–1101. doi: 10.1016/S0277-3791(99)00087-6
[50]	Thiagarajan N, Subhas AV, Southon JR, et al. (2014) Abrupt pre-Bølling–Allerød warming and circulation changes in the deep ocean. Nature 511: 75–78. doi: 10.1038/nature13472
[51]	Feng W, Hardt BF, Banner JL, et al. (2014) Changing amounts and sources of moisture in the U.S. southwest since the Last Glacial Maximum in response to global climate change. Earth Planet Sci Lett 401: 47–56.
[52]	Cheng H, Edwards RL, Broecker WS, et al. (2009) Ice age terminations. Science 326: 248–252. doi: 10.1126/science.1177840
[53]	Denton GH, Anderson RF, Toggweiler JR, et al. (2010) The last glacial termination. Science 328: 1652–1656. doi: 10.1126/science.1184119
[54]	Broecker WS, Denton GH (1990) The role of ocean-atmosphere reorganizations in glacial cycles. Quat Sci Rev 9: 305–341. doi: 10.1016/0277-3791(90)90026-7
[55]	Barrows TT, Juggins S, De Deckker P, et al. (2007) Paleoceanography 22: PA2215.
[56]	Asmerom Y, Polyak V, Burns S, et al. (2007) Solar forcing of Holocene climate; new insights from a speleothem record, Southwestern United States. Geology 35: 1–4. doi: 10.1130/G22865A.1
[57]	Lachniet MS, Denniston RF, Asmerom Y, et al. (2014) Orbital control of western North America atmospheric circulation and climate over two glacial cycles. Nat Commun 5: 3805. doi: 10.1038/ncomms4805
[58]	Rasmussen SO, Andersen KK, Svensson AM, et al. (2006) A new Greenland ice core chronology for the last glacial termination. J Geophys Res Atmos 111: 6.
[59]	Linge H, Baker A, Andersson C, et al. (2009) Variability in luminescent lamination and initial ²³⁰Th/²³²Th activity ratios in a late Holocene stalagmite from northern Norway. Quat Geochronol 4: 181–192. doi: 10.1016/j.quageo.2009.01.009
[60]	Rajendran CP, Sanwal J, Morell KD, et al. (2016) Stalagmite growth perturbations from the Kumaun Himalaya as potential earthquake recorders. J Seismol 20: 579–594. doi: 10.1007/s10950-015-9545-5
[61]	Railsback LB, Akers PD, Wang L, et al. (2013) Layer-bounding surfaces in stalagmites as keys to better paleoclimatological histories and chronologies. Int J Speleol 42: 167–180. doi: 10.5038/1827-806X.42.3.1
[62]	Stock GM, Granger DE, Sasowsky ID, et al. (2005) Comparison of U–Th, paleomagnetism, and cosmogenic burial methods for dating caves: implications for landscape evolution studies. Earth Planet Sci Lett 236: 388–403. doi: 10.1016/j.epsl.2005.04.024
[63]	Polyak VJ, Asmerom Y (2001) Late Holocene climate and cultural changes in the southwestern United States. Science 294: 148–151. doi: 10.1126/science.1062771
[64]	Asmerom Y, Polyak VJ, Burns SJ (2010) Variable winter moisture in the southwestern United States linked to rapid glacial climate shifts. Nat Geosci 3: 114–117. doi: 10.1038/ngeo754
[65]	Harmon RS, Ford DC, Schwarcz HP (1977) Interglacial chronology of the Rocky and Mackenzie Mountains based upon ²³⁰Th–²³⁴U dating of calcite speleothems. Can J Earth Sci 14: 2543–2552. doi: 10.1139/e77-220
[66]	Fairchild IJ, Baker A (2012) Speleothem Science. Chichester, Wiley-Blackwell: 432.
[67]	Steffensen JP, Andersen KK, Bigler M, et al. (2008) High-Resolution Greenland Ice Core Data Show Abrupt Climate Change Happens in Few Years. Science 321: 680–684. doi: 10.1126/science.1157707
[68]	Kenny R, Neet KE (1993) Upper Pennsylvanian-Permian (Naco Group) paleosols (north-central Arizona): field and isotopic evidence. Geoderma 58: 131–148. doi: 10.1016/0016-7061(93)90038-M
[69]	Kenny R, Knauth LP (2001) Stable isotope variations in the Neoproterozoic Beck Spring Dolomite and Mesoproterozoic Mescal Limestone paleokarst: Implications for life on land in the Precambrian. GSA Bull 113: 650–658. doi: 10.1130/0016-7606(2001)113<0650:SIVITN>2.0.CO;2
[70]	Allan JR, Matthews RK (1982) Isotopic signatures associated with early meteoric diagenesis. Sedimentology 29: 797–817. doi: 10.1111/j.1365-3091.1982.tb00085.x
[71]	Beeunas MA, Knauth LP (1985) Preserved stable isotope signature of subaerial diagenesis in the 1.2-b.y. Mescal Limestone, central Arizona: Implications for the timing and development of a terrestrial plant cover. GSA Bull 96: 737–745.
[72]	Dreybrodt W (1999) Chemical kinetic, speleothem growth and climate. Boreas 28: 347–356. doi: 10.1080/030094899422073
[73]	Frisia S, Borsato A, Fairchild IJ, et al. (2000) Calcite fabrics, growth mechanisms, and environments of formation in speleothems from the Italian Alps and southwestern Ireland. J Sed Res 70: 1183–1196. doi: 10.1306/022900701183
[74]	Onac B (1997) Crystallography of Speleothems, In: Hill C, Forti P (eds.) Cave Minerals of the World, 2^nd Ed, Huntsville, Alabama: National Speleological Society, 230–235.
[75]	Kendall AC, Broughton PL (1978) Origin of fabrics in speleothems composed of columnar calcite crystals. J Sed Res 48: 519–538.
[76]	Gonzales LA, Carpenter SJ, Lohmann KC (1992) Inorganic calcite morphology: roles of fluid chemistry and fluid flow. J Sed Petrol 62: 383–399.
[77]	Fountain AG, Walder JS (1998) Water flow through temperate glaciers. Rev Geophys 36: 299–328. doi: 10.1029/97RG03579
[78]	Hock R, Jansson P, Braun LN (2005) Modelling the response of mountain glacier discharge to climate warming, In: Huber UM, Bugmann HKM, Reasoner MA (eds.) Global change and mountain regions (A state of knowledge overview), Springer, Dordrecht, 243–252.
[79]	Hodson A, Anesio AM, Tranter M, et al. (2008) Glacial ecosystems. Ecol Monogr 78: 41–67. doi: 10.1890/07-0187.1
[80]	Bates B, Kundzewicz ZW, Wu S, et al. (2008) Climate change and water. Technical paper of the Intergovernmental Panel on Climate Change, Geneva: IPCC Secretariat, 210.
[81]	Lacelle D (2007) Environmental setting, (micro) morphologies and stable C–O isotope composition of cold climate carbonate precipitates-a review and evaluation of their potential as paleoclimatic proxies. Quat Sci Rev 26: 1670–1689. doi: 10.1016/j.quascirev.2007.03.011
[82]	Turgeon S, Lundberg J (2001) Chronology of discontinuities and petrology of speleothems as paleoclimatic indicators of the Klamath Mountains, southwest Oregon, USA. Carb Evap 16: 153–167. doi: 10.1007/BF03175833
[83]	Frisia S (2015) Microstratigraphic logging of calcite fabrics in speleothems as tool for palaeoclimate studies. Int J Speleo 44: 1–16.
[84]	Brook GA, Railsback LB, Cooke HJ, et al. (1992) Annual Growth Layers in a Stalagmite from Drotsky's Cave, Ngamiland: Relationships between layer thickness and precipitation. Botsw Notes Rec 24: 151–63.
[85]	Burns SJ, Fleitmann D, Mudelsee M, et al. (2002) A 780-year annually resolved record of Indian Ocean monsoon precipitation from a speleothem from south Oman. J Geophys Res 107: 4434. doi: 10.1029/2001JD001281
[86]	Baker A, Smart PL, Edwards RL, et al. (1993) Annual growth banding in cave stalagmite. Nature 304: 518–520.
[87]	Fleitmann D, Burns SJ, Neff U, et al. (2004) Palaeoclimate interpretation of high-resolution oxygen isotope profiles derived from annually laminated speleothems from southern Oman. Quat Sci Rev 23: 935–945. doi: 10.1016/j.quascirev.2003.06.019
[88]	Klaar MJ, Kidd C, Malone E, et al. (2015) Vegetation succession in deglaciated landscapes: implications for sediment and landscape stability. Earth Surf Process Landf 40: 1088–1100. doi: 10.1002/esp.3691
[89]	Morris PJ, Swindles GT, Valdes PJ, et al. (2018) Global peatland initiation driven by regionally asynchronous warming. Proc Natl Acad Sci USA 115: 4851–4856. doi: 10.1073/pnas.1717838115
[90]	Lawrence DB, Schoenike RE, Quispel A, et al. (1967) The role of Dryas drummondii in vegetation development following ice recession at Glacier Bay, Alaska, with special reference to its nitrogen fixation by root nodules. J Ecol: 793–813.
[91]	Lawrence DB (1958) Glaciers and vegetation in south-eastern Alaska. Am Sci 46: 138A–122.
[92]	Milner AM, Fastie CL, Chapin FS, et al. (2007) Interactions and linkages among ecosystems during landscape evolution. BioSci 57: 237–247. doi: 10.1641/B570307
[93]	Prach K, Rachlewicz G (2012) Succession of vascular plants in front of retreating glaciers in central Spitsbergen. Polish Polar Res 33: 319–328. doi: 10.2478/v10183-012-0022-3
[94]	Mizuno K (2005) Glacial fluctuation and vegetation succession on Tyndall Glacier, Mt Kenya. Mtn Res Develop 25(1): 68–76.
[95]	Schildgen T (2000) Fire and ice: Geomorphic history of Middle Boulder Creek as determined by isotopic dating techniques. CO Front Range: Williamstown, Massachusetts, Williams College: 30.
[96]	Johnsen SJ, Clausen HB, Dansgaard W, et al. (1997) The δ18O record along the Greenland Ice Core Project deep ice core and the problem of possible Eemian climatic instability. J Geophys Res (Oceans) 102: 26397–26410. doi: 10.1029/97JC00167
[97]	Hostetler SW, Clark PU (1997) Climatic controls of western US glaciers at the last glacial maximum. Quat Sci Rev 16: 505–511. doi: 10.1016/S0277-3791(96)00116-3
[98]	Oviatt CG, Currey DR, Sack D (1992) Radiocarbon chronology of Lake Bonneville, eastern Great Basin, USA. Palaeogeogr Palaeoclimatol Palaeoecol 99: 225–241. doi: 10.1016/0031-0182(92)90017-Y
[99]	Butler D (1986) Pinedale deglaciation and subsequent Holocene environmental changes and geomorphic responses in the central Lemhi Mountains, Idaho, USA. Géogr Phys Quat 40: 39–46.
[100]	Thackray GD, Lundeen KA, Borgert JA (2004) Latest Pleistocene alpine glacier advances in the Sawtooth Mountains, Idaho, USA: reflections of midlatitude moisture transport at the close of the last glaciation. Geology 32: 225–228. doi: 10.1130/G20174.1
[101]	Serrano E, González-Trueba JJ, Pellitero R, et al. (2013) Quaternary glacial evolution in the Central Cantabrian Mountains (northern Spain). Geomorphology 196: 65–82. doi: 10.1016/j.geomorph.2012.05.001
[102]	Smedley RK, Glasser NF, Duller GAT (2016) Luminescence dating of glacial advances at Lago Buenos Aires (∼46 S), Patagonia. Quat Sci Rev 134: 59–73. doi: 10.1016/j.quascirev.2015.12.010
[103]	Friele PA, Clague JJ (2002) Younger Dryas readvance in Squamish river valley, southern Coast mountains, British Columbia. Quat Sci Rev 21: 1925–1933. doi: 10.1016/S0277-3791(02)00081-1

This article has been cited by:

1.	Xin Long, Novel stability criteria on a patch structure Nicholson’s blowflies model with multiple pairs of time-varying delays, 2020, 5, 2473-6988, 7387, 10.3934/math.2020473
2.	Luogen Yao, Qian Cao, Anti-periodicity on high-order inertial Hopfield neural networks involving mixed delays, 2020, 2020, 1029-242X, 10.1186/s13660-020-02444-3
3.	Qian Cao, Xin Long, New convergence on inertial neural networks with time-varying delays and continuously distributed delays, 2020, 5, 2473-6988, 5955, 10.3934/math.2020381
4.	Ramazan Yazgan, Salsabil Hajjaji, Farouk Chérif, Weighted Pseudo Almost-Automorphic Solutions of Quaternion-Valued RNNs With Mixed Delays, 2023, 55, 1370-4621, 423, 10.1007/s11063-022-10890-x
5.	Yanli Xu, Periodic dynamic on a class of neutral-type Rayleigh equations incorporating D operators, 2023, 0952-813X, 1, 10.1080/0952813X.2023.2165720
6.	M. Sai Pravallika, S. Vasavi, S. P. Vighneshwar, Prediction of temperature anomaly in Indian Ocean based on autoregressive long short-term memory neural network, 2022, 34, 0941-0643, 7537, 10.1007/s00521-021-06878-8
7.	Weiping Fan, Anti-periodic stability on a class of neutral-type Rayleigh equations involving D operators, 2022, 0952-813X, 1, 10.1080/0952813X.2022.2153275
8.	Xiaojin Guo, Chuangxia Huang, Zhichun Yang, Jiping Zhang, Jinde Cao, Stability Analysis of High-order Proportional Delayed Cellular Neural Networks with D Operators, 2022, 20, 1598-6446, 660, 10.1007/s12555-020-0902-y
9.	Zhenjie Wang, Wenxia Cui, Wenbin Jin, Finite-time synchronization for fuzzy inertial cellular neural networks with time-varying delays via integral inequality, 2021, 41, 10641246, 3653, 10.3233/JIFS-211065
10.	Jian Zhang, Ancheng Chang, Gang Yang, Periodicity on Neutral-Type Inertial Neural Networks Incorporating Multiple Delays, 2021, 13, 2073-8994, 2231, 10.3390/sym13112231
11.	Chaouki Aouiti, Mahjouba Ben Rezeg, Impulsive multidirectional associative memory neural networks: New results, 2021, 14, 1793-5245, 10.1142/S1793524521500601
12.	Xiaojin Guo, Chuangxia Huang, Jinde Cao, Nonnegative periodicity on high-order proportional delayed cellular neural networks involving $D$ operator, 2020, 6, 2473-6988, 2228, 10.3934/math.2021135
13.	Ankit Kumar, Subir Das, Vijay K. Yadav, Jinde Cao, Chuangxia Huang, Synchronizations of fuzzy cellular neural networks with proportional time-delay, 2021, 6, 2473-6988, 10620, 10.3934/math.2021617
14.	Weiping Fan, Periodic Stability on a Class of D-Operator-Based Neutral-Type Rayleigh Equations Accompanying Mixed Delays, 2022, 1370-4621, 10.1007/s11063-022-11033-y
15.	Bing Hao, Tianwei Zhang, Stability analysis and synchronized control of fuzzy Mittag-Leffler discrete-time genetic regulatory networks with time delays, 2022, 43, 10641246, 587, 10.3233/JIFS-212361
16.	Yuehong Zhang, Zhiying Li, Wangdong Jiang, Wei Liu, The stability of anti-periodic solutions for fractional-order inertial BAM neural networks with time-delays, 2023, 8, 2473-6988, 6176, 10.3934/math.2023312
17.	Jin Gao, Lihua Dai, Anti-periodic synchronization of quaternion-valued high-order Hopfield neural networks with delays, 2022, 7, 2473-6988, 14051, 10.3934/math.2022775
18.	Chengjie Chen, Fuhong Min, Yunzhen Zhang, Han Bao, ReLU-type Hopfield neural network with analog hardware implementation, 2023, 167, 09600779, 113068, 10.1016/j.chaos.2022.113068
19.	Lilun Zhang, Le Li, Chuangxia Huang, Positive stability analysis of pseudo almost periodic solutions for HDCNNs accompanying $D$ operator, 2022, 15, 1937-1632, 1651, 10.3934/dcdss.2021160
20.	Xianhui Zhang, Le Li, Changchun Bao, Jian Zhang, Anti-periodicity on inertial Cohen-Grossberg neural networks involving distributed delays, 2023, 0952-813X, 1, 10.1080/0952813X.2023.2196976
21.	Mei Xu, Honghui Yin, Bo Du, Periodic Solution Problems for a Class of Hebbian-Type Networks with Time-Varying Delays, 2023, 15, 2073-8994, 1985, 10.3390/sym15111985
22.	Chenyang Li, Kit Ian Kou, Yanlin Zhang, Yang Liu, Advancements in exponential synchronization and encryption techniques: Quaternion-Valued Artificial Neural Networks with two-sided coefficients, 2024, 08936080, 106982, 10.1016/j.neunet.2024.106982

Reader Comments

Your name:*

Email:*
© 2019 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)