An improved coupled PDE system applied to the inverse image denoising problem

1.   Introduction

Endometriosis which is an estrogen-dependent disease is defined by the presence of endometrial tissue outside the uterine cavity [1]. The disease affects approximately 10% of women of reproductive age and 20% to 50% of women with infertility or chronic pelvic pain [2]. Being a steroid hormone dependent disease, some of the risk factors for its development are early menarche, late on-set menopause and other conditions that cause extended estrogen exposure [3]. Although the etiology of endometriosis remains unclear, a number of studies have supported that endometriosis is a multifactorial disease with possible causes being genetic, hormonal, immunological and environmental [4]–[6]. Laparoscopy which is the gold standard for the diagnosis of endometriosis is invasive and expensive [1]. Blood biomarkers could be an alternative to the diagnostic surgery because they are minimally invasive, allows repeated measurements, readily available, provides a rapid result, cost effective and highly suitable for high-throughput measurements [7].

Genetic predisposition due to certain susceptible genes plays an important role in pathogenesis of endometriosis [4],[6],[8]. Recent genetic studies have revealed an association between the development of endometriosis and the polymorphisms of several sex steroid genes [5],[9]. Genetic factors have been shown to increase the susceptibility to endometriosis hence complicating the disease etiology and pathogenesis [10],[11]. A genetic alteration of the endometrial cells influencing their tendency to implant may be hereditary, as a heritable component to the disease has been established [12]–[14]. Endometriosis is an estrogen dependent disorder [15] and any hormonal alteration may influence the ability of endometrial cells to proliferate, attach to the mesothelium and/or evade immune mediated clearance. Although many candidate genes are involved initially in the pathogenesis of endometriosis, including genes involved in hormone receptors [16], there has been a conflict in the results from those studies which lack replication in independent populations. Identification of genetic polymorphisms could be used as genetic biomarkers for endometriosis [17].

The dysregulation of estrogen and progesterone receptor-ligand signaling is one of the most credited hypotheses about a possible cause of endometriosis [5]. In humans and other primates, estrogen and progesterone play a significant role in the pathogenesis of the disease by promoting endometriotic tissue survival, maintenance, and differentiation [18],[19]. As previously reported, genetic mutations in Eostrogen 1 (ESR1) may lead to aberrant gene expression and may be involved in pathogenesis of endometriosis [20]. The progesterone gene polymorphisms seem to damage receptor-ligand functionality in the target tissue. Progesterone resistance which is due to a significant reduction of progesterone receptors (PGRs) in endometriosis patients is manifested by selective molecular abnormalities [18]. The influence of ESR1 and progesterone receptor (PGR) polymorphisms have been previously studied in different female populations but there is no consensus in the results from all the studies [21]–[23].

Due to inconsistent results in different populations, this study sought to evaluate genetic variants in ESR 1 and PGR in olive baboons induced with endometriosis. The intraperitoneal inoculation with autologous menstrual endometrium in baboons results in the formation of endometriotic lesions with histological and morphological characteristics similar to those in women [24],[25].

2.   Materials and methods

2.1.   Animals

The study which involved ten adult female olive baboons (P. anubis) was carried out in the Institute of Primate Research (IPR). All the animals (average mean weight of 15.2 kg) were trapped in the wild and maintained in quarantine for 3 months. To ensure they were disease free, the baboons were screened for common pathogens, simian T-lymphotropic virus-1, and simian immunodeficiency virus. They were housed indoors with natural lighting in group cages and fed on commercial food pellets with fruits and vegetable supplementation three times a week and water ad libitum. During the surgical procedures, the baboons were anesthetized with a mixture of ketamine (Anesketin, 15 mg/kg; Eurovet NV/SA, Heusden-Zolder, Belgium) and xylazine (Bomazine 2%, 2 mg/kg; Bomac Laboratories Ltd, Auckland, New Zealand) administered intramuscularly for induction, and 1–2% halothane (Halothane; Nicholas Piramal India Ltd, Andhra Pradesh, India) with N2O/O2 (70%/30%) for maintenance. The animals received antibiotics for 1 week after surgery (Clamoxyl LA; Pfizer, Paris, France), and their pain was controlled with ibuprofen (Ketofen; Merial, Lyon, France) for 3 days.

2.2.   Induction of experimental endometriosis

Anaesthesia and laparoscopies were carried out as described previously [26]. Screening video laparoscopy during the mid-luteal phase (approximately 25^th day of the cycle) was performed on the baboons to confirm absence of endometriosis. The animals were then allowed to recover for one menstrual cycle as previously described [27]. The induction laparoscopy in ten baboons (n = 10) was performed on the first or second day of menstruation [27]. Briefly, 1 gram of menstrual endometrium was harvested on days 1–2 after the onset of the next menses, by transcervical uterine curettage from each animal and minced through an 18 – gauge needle. The menstrual endometrium was autologously seeded onto ectopic sites (uterosacral ligaments, uterovesical fold, pouch of douglas, ovaries) as previously described [28].

2.3.   Sample collection, preparation and DNA extraction

Blood samples were obtained prior to the disease induction (baseline) and then on days 25 and 50 Post Induction (PI). Thirty samples from the ten baboons were used for the study. Using a sterile needle (Macaque 23–25 G; Baboon 21–23 G), 4ml of peripheral blood was drawn from each baboon and collected in Ethylenediaminetetraacetic acid (EDTA) tubes. The collected blood was centrifuged at 3000 g for 10 min at 4°C, 30 min to 1 hour after sampling. The plasma samples were frozen in aliquots of 500 µl at −80°C until further analysis. Genomic DNA was extracted from plasma using QIAamp DNA blood mini kit (QIAGEN, Germany) according to the manufacturer's instructions. The DNA concentration and purity were measured using an absorbance ratio of 260/280 nm by NanoDrop (Thermo Scientific, NanoDrop 2000). The ratio of the absorbance at 260 and 280 nm (OD260/OD280) was used to assess the purity of extracted DNA where the ratio of 1.8 was generally accepted.

2.4.   Genotyping and DNA sequencing

Genomic DNA from the plasma samples was amplified using gene specific primers for ESR1 and PGR genes. The sequences of the polymerase chain reaction (PCR) primer sets and cycling conditions are shown in Table 1. PCR was carried out with a final volume of 20 µl. Briefly, 2 µl of genomic DNA was added to 18 µl Thermo Scientific Dream Taq Hot Start Green PCR Master Mix (2×). The HotStar Taq® master mix (2×) comprised of 2.5 units HotStarTaq DNA polymerase (1×), 1x PCR buffer and 200 µM of each dNTP. We used a DNA-free control to check for contamination. PCR products were visualized by GelRed staining on a 2% agarose gel. All the visible DNA bands were excised on an Ultra Slim Blue Light Trans illuminator (Maestrogen). The DNA was extracted from the gels using the QIAquick^R gel extraction kit (Qiagen) according to the manufacturer's instructions. The DNA concentration and purity were measured using an absorbance ratio of 260/280 nm by NanoDrop (Thermo Scientific, NanoDrop 2000). The identities of the PCR products were verified by sequencing using the ABI 3500 XL Genetic Analyzer for sanger sequencing (Inqaba Biotechnical Industries (Pty) Ltd, Pretoria, South Africa).

2.5.   DNA sequence analysis

The sequenced samples were analyzed using the Bioedit software and BLAST tool from NCBI. The electropherograms were visualized using Bioedit software tool where consensus sequences were generated. Single nucleotide polymorphisms were detected as sequence differences in multiple alignments using Clustalw [29]. The SNPs were identified as transitions or transversions in coding and non-coding regions of the DNA sequence. This was followed by amino acid sequence analysis to check for amino acid changes as a result of the nucleotide substitutions.

2.6.   Ethical statement

This study was approved by the Institutional Scientific Evaluation and Review Committee and the Animal Care and Use Committee of the Institute of Primate Research (IPR) Nairobi – Kenya.

3.   Results

3.1.   Gene amplification

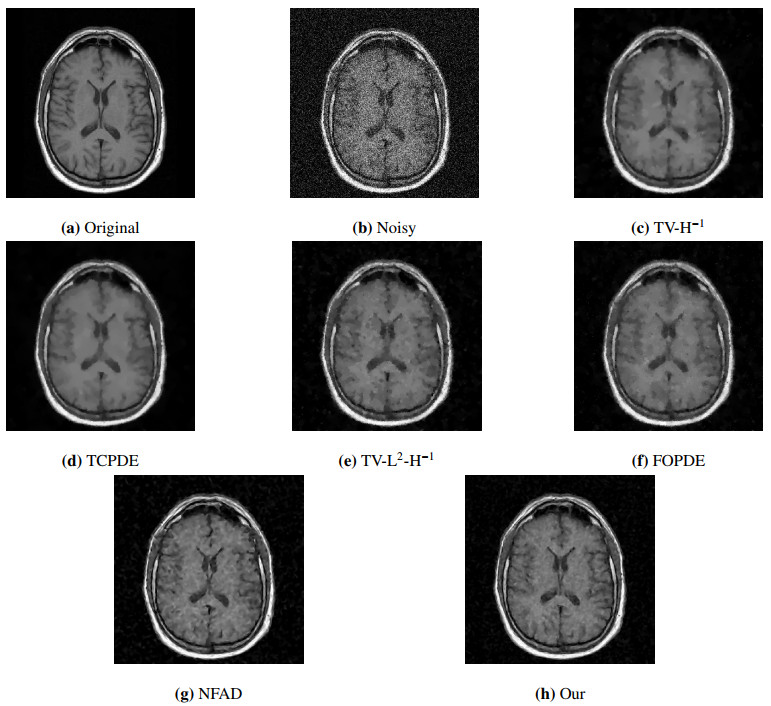
In total, 30 samples were amplified using gene specific primers which gave reliable amplification with PCR products of size 966 bp for ESR 1 and 180 bp for PGR genes (Table 1). A total of 14 positive amplicons for ESR 1 gene and 17 positive amplicons for PGR were sequenced (Figure 1).

Table 1.  Primers used for gene analysis.

Gene	Primer sequences (5′–3′)	Annealing Temperature	PCR program (40 cycles)	Size
ESR 1	F: CTGCCACCCTATCTGTATC	57°C	95°C 3 min, 95°C 30 sec, 57°C 30 sec, 72°C 60 sec	966 bp
	R: ACCCTGGCGTCGATTATCT
PGR	F: TTCGAAACTTACATATTGATGACCA	59°C	95°C 3 min, 95°C 30 sec, 59°C 30 sec, 72°C 60 sec	180 bp
	R: CACTTAAAATAACAAAAACAACAAAAG

---

 | Show Table

DownLoad: CSV

3.2.   Genomic positions of single nucleotide polymorphisms (SNPs)

The single nucleotide polymorphisms in ESR 1 gene were more than SNPs in PGR gene (Figures 2 and 3). For the ESR 1 gene, most of the point mutations occurred in most of the samples as shown in Table 2. In PGR gene, there were only two point mutations in which one of the SNPs (T<->C) occurred only in one sample (Figure 3 and Table 2). The genomic positions for each SNP in the two genes are indicated in Figures 2 and 3. Sequences 1–5 and 12–14 are diseased samples collected on day 25 PI while sequences 6–11 are diseased samples collected on day 50PI (ESR 1, Figure 2). Sequences 1–8 and 9–17 are diseased samples collected on days 25 and 50 PI respectively (PGR, Figure 3).

3.3.   Types of polymorphisms and effects of the SNPs on the amino acids

The transition substitutions were more predominant than transversions (79.5% vs 20.5%) for all sequenced DNA fragments. In ESR 1 gene, transitions C↔T and A↔G are over-represented with 43.6% and 35.9% of the total substitutions respectively while transversions G↔T and A↔C occurred at 15.4% and 5.1% (Table 2, Figure 4). In PGR gene, transitions T↔C occurred at 5.8% while A->G was present in all the sequenced DNA fragments (Table 2). Most of the point mutations resulted to an amino acid change although some of them had no effect on the amino acid as shown in Table 2. There were no differences on the type of point mutations between days 25 and 50 PI.

Table 2.  Distribution of SNPs in ESR 1 and PGR according to genotypes and their effects on the amino acids.

Genes	Point mutations	Position	Amino acid change	SNP frequency
ESR 1	A->G	A1G	S1G	25.6%
		A170G	Q57R
		A200G	STOP67W
		A252G	No change
		A349G	T117A
		A547G	M183C
		A716G	K239R
		A783G	No change
		A798G	No change
		A817G	N273D
	G->A	G283A	E95K	10.3%
		G516A	No change
		G613A	V205I
		G648A	No change
	C->T	C3T	No change	15.4%
		C244T	No change
		C355T	L119F
		C414T	No change
		C561T	No change
		C840T	No change
	T->C	T65C	L22P	28.2%
		T80C	F27S
		T154C	W52R
		T159C	No change
		T213C	No change
		T394C	STOP132R
		T553C	C185R
		T572C	I191T
		T594C	No change
		T656C	I219T
		T662C	L221P
	G->T	G77T	S26I	5.1%
		G439T	E47STOP
	T->G	T143G	F48C	10.3%
		T152G	V51G
		T162G	C54W
		T470G	I157S
	A->C	A230C	Y77S	5.1%
		A338C	Y113S
PGR	T->C	T46C	S16P	5.8%
	A->G	A138G	No change	100%

---

 | Show Table

DownLoad: CSV

Figure 1.  Gel analysis of ESR 1 & PGR genes. (A) PCR amplification of ESR 1 using gene-specific primers. Lanes 3, 5, 6, 8, 9, 23–25, 31 & 32 are controls, Lanes 11, 22, 33 are NTCs and lanes 1, 2, 4, 10, 12 (25 dpi), 13–22 (50 dpi), 26–30 (25 dpi) are diseased samples. (B) PCR amplification of PGR using gene-specific primers. Lanes 1–10 (25 dpi), 12–21 (50 dpi), 23–32 (Controls) represents diseased samples while 11, 22 & 33 are NTCs.

DownLoad: Full-Size Img PowerPoint

Figure 2.  Analysis of SNPs in the DNA sequence of ESR 1 gene. The polymorphism in the ESR 1 gene is represented by multiple sequence alignment in Bioedit software.

DownLoad: Full-Size Img PowerPoint

Figure 3.  Analysis of SNPs in the DNA sequence of PGR gene. The polymorphism in the PGR gene is represented by multiple sequence alignment in Bioedit software.

DownLoad: Full-Size Img PowerPoint

Figure 4.  Distribution of transitions and trans versions among SNPs in ESR 1 gene.

DownLoad: Full-Size Img PowerPoint

4.   Discussion

Several studies have shown an association between genetic factors and development of endometriosis although the results have been inconsistent across different world populations [30] and therefore several replication studies are required in order for the genetic basis of endometriosis to be clarified. Single nucleotide polymorphisms can generate synonymous or non-synonymous mutations if present in coding regions of genes, or lead to alterations of the gene product if present in intronic or intergenic regions [31]. Due to genetic predisposition towards endometriosis, studies to identify genomic variants involved in the pathogenesis of endometriosis are necessary. Genetic variations existing in ESR 1 and PGR genes could be explored as genetic biomarkers for endometriosis.

We investigated genomic variants in ESR 1 and PGR genes which are involved in steroidogenesis and sex hormone receptorial activity. Our study revealed presence of both non-synonymous and synonymous mutations in both ESR 1 and PGR genes. Twenty-six SNPs in ESR 1 gene resulted to a change in amino acid while thirteen had no effect on the amino acid (Table 2). Due to the change in amino acid which results to altered protein function, these non-synonymous mutations in ESR 1 gene could be involved in the pathogenesis of endometriosis as previously reported [5],[16]. From our findings, there were more point mutations in ESR 1 gene than PGR gene.

The C<->T and G<->A point mutations accounted for the highest SNP frequency (43.6% and 35.9% respectively). These results indicate that the transition substitutions were more predominant than transversions in the ESR 1 gene (79.5% vs 20.5%). These transition substitutions have also been previously reported in women with endometriosis [32]. From the results, the T<->C SNP in PGR gene occurred only in one sample out of the seventeen sequenced samples hence this result is unreliable. The A>G mutation on the PGR gene which occurred in all the sequenced DNA fragments did not result to a change in the amino acid sequence. There was no difference on the type of point mutations between the two disease time points. This could be because the two disease time points (days 25 & 50PI) both represent the early stages of endometriosis as previously established in other studies [34],[35].

Results from this study reveal high correlation between the genomic variants in the non-human primates and in women with endometriosis as previously reported [35]. The staging of endometriosis in the baboons was done using revised American Fertility Society (rAFS) scoring system [36] after modification to baboon size [28]. Our study had several limitations which include small sample size, inclusion of only minimal and mild stages of disease, and collection of control tissues only at a single time point before disease induction and not at consecutive time points for exact matching. Despite these limitations, we identified several polymorphisms in the ESR 1 gene which have previously been identified in women patients with endometriosis.

5.   Conclusions

The current study identified several ESR 1 genomic variants in samples from baboons with induced endometriosis. These results, together with numerous previous studies, suggest that ESR1 gene polymorphisms could be involved in pathogenesis of endometriosis. The PGR point mutations identified from this study were synonymous mutations. Functional studies are needed to elucidate the possible effect of these ESR1 non-synonymous mutations on ESR1 expression which eventually affects the function of the sex steroids.