Initial boundary value problem for a inhomogeneous pseudo-parabolic equation

1.   Introduction

In this paper, we consider the following initial-boundary value problem

and its corresponding steady-state problem

where $\Omega\subset \mathbb{R}^n$ ($n\geq1$ is an integer) is a bounded domain with boundary $\partial \Omega$ and $u_0\in H_0^1( \Omega)$; the parameters $p$ and $\sigma$ satisfy

(1) was called homogeneous (inhomogeneous) pseudo-parabolic equation when $\sigma = 0$ ($\sigma\neq0$). The concept "pseudo-parabolic" was proposed by Showalter and Ting in 1970 in the paper [20], where the linear case was considered. Pseudo-parabolic equations describe a variety of important physical processes, such as the seepage of homogeneous fluids through a fissured rock [1], the unidirectional propagation of nonlinear, dispersive, long waves [2,23], and the aggregation of populations [17].

The homogeneous problem, i.e. $\sigma = 0$, was studied in [3,4,5,7,9,10,13,15,16,21,24,25,26,27,28,29]. Especially, for the Cauchy problem (i.e. $\Omega = \mathbb{R}^n$ and there is no boundary condition), Cao et al. [4] showed the critical Fujita exponent $p_c$ (which was firstly introduced by Fujita in [8]) is $1+2/n,$ i.e. if $1<p\leq p_c$, then any nontrivial solution blows up in finite time, while global solutions exist if $p>p_c$. In [28], Yang et al. proved that for $p>p_c$, there is a secondary critical exponent $\alpha_c = {2}/{(p-1)}$ such that the solution blows up in finite time for $u_0$ behaving like $|x|^{- \alpha}$ at {$|x|\rightarrow\infty$} if $\alpha = (0, \alpha_c)$; and there are global solutions for for $u_0$ behaving like $|x|^{- \alpha}$ at {$|x|\rightarrow\infty$} if $\alpha = ( \alpha_c,n)$. For the zero Dirichlet boundary problem in a bounded domain $\Omega$, in [13,25,26], the authors studied the properties of global existence and blow-up by potential well method (which was firstly introduced by Sattinger [19] and Payne and Sattinger [18], then developed by Liu and Zhao in [14]), and they showed the global existence, blow-up and asymptotic behavior of solutions with initial energy at subcritical, critical and supercritical energy level. The results of [13,25,26] were extended by Luo [15] and Xu and Zhou [24] by studying the lifespan (i.e. the upper bound of the blow-up time) of the blowing-up solutions. Recently, Xu et al. [27] and Han [9] extended the previous studies by considering the problem with general nonlinearity.

Li and Du [12] studied the Cauchy problem of equation in (1) with $\sigma>0$. They got the critical Fujita exponent ($p_c$) and second critical exponent ($\alpha_c$) by the integral representation and comparison principle. The main results obtained in [12] are as follows:

(1) If $1<p\leq p_c: = 1+(2+\sigma)/n$, then every nontrivial solution blows up in finite time.

(2) If $p>p_c$, the distribution of the initial data has effect on the blow-up phenomena. More precisely, if $u_0\in\Phi_ \alpha$ and $0< \alpha< \alpha_c: = (2+\sigma)/(p-1)$ or $u_0$ is large enough, then the solution blows up in finite time; if $u_0 = \mu\phi(x)$, $\phi\in \Phi^ \alpha$ with $\alpha_c< \alpha<n$, $0<\mu<\mu_1$, then the solution exists globally, where $\mu_1$ is some positive constant,

and

Here $BC( \mathbb{R}^n)$ is the set of bounded continuous functions in $\mathbb{R}^n$.

In view of the above introductions, we find that

(1) for Cauchy problem in $\mathbb{R}^n$, only the case $\sigma\geq0$ was studied;

(2) for zero Dirichlet problem in a bounded domain $\Omega$, only the case $\sigma = 0$ was studied.

The difficulty of allowing $\sigma$ to be less than $0$ is the term $|x|^\sigma$ become infinity at $x = 0$. In this paper, we consider the problem in a bounded domain $\Omega$ with zero Dirichlet boundary condition, i.e. problem (1), and the parameters satisfies (3), which allows $\sigma$ to be less than $0$. To overcome the singularity of $|x|^\sigma$ at $x = 0$, we use potential well method by introducing the $|x|^\sigma$ weighed-$L^{p+1}( \Omega)$ space and assume there is a lower bound of $\sigma$, i.e,

for $n\geq3$.

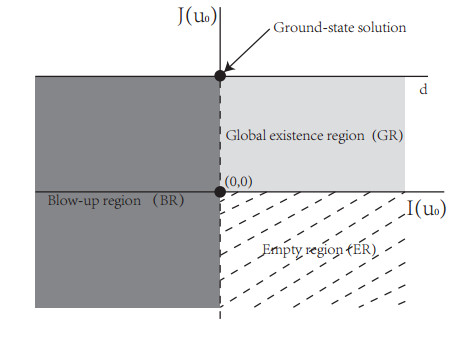
The main results of this paper can be summarized as follows: Let $J$ and $I$ be the functionals given in (12) and (13), respectively; $d$ be the mountain-pass level given in (14); $S_\rho$ and $S^\rho$ be the sets defined in (20).

(1) (the case $J(u_0)\leq d$, see Fig. 1) If $u_0\in H_0^1( \Omega)$ such that $(I(u_0),J(u_0))$ is in the dark gray region (BR), then the solution blows up in finite time; if $u_0\in H_0^1( \Omega)$ such that $(I(u_0),J(u_0))$ is in the light gray region (GR), then the solution exists globally; if $u_0\in H_0^1( \Omega)$ such that $(I(u_0),J(u_0)) = (0,d)$, then $u_0$ is a ground-state solution and (1) admits a global solution $u\equiv u_0$; there is no $u_0\in H_0^1( \Omega)$ such that $(I(u_0),J(u_0))$ is in the dotted part (ER).

(2) (the case $J(u_0)>d$) If $u_0\in S_\rho$ for some $\rho\geq J(u_0)>d$, then the solution exists globally and goes to $0$ in $H_0^1( \Omega)$ as times goes to infinity; if $u_0\in S^\rho$ for some $\rho\geq J(u_0)>d$, then the solution blows up in finite time.

(3) (arbitrary initial energy level) For any $M\in \mathbb{R}$, there exits a $u_0\in H_0^1( \Omega))$ satisfying $J(u_0) = M$ such that the corresponding solution blows up in finite time.

(4) Moreover, under suitable assumptions, we show the exponential decay of global solutions and lifespan (i.e. the upper bound of blow-up time) of the blowing-up solutions.

The organizations of the remain part of this paper are as follows. In Section 2, we introduce the notations used in this paper and the main results of this paper; in Section 3, we give some preliminaries which will be used in the proofs; in Section 4, we give the proofs of the main results.

2.   Notations and main results

Throughout this paper we denote the norm of $L^{\gamma}( \Omega)$ for $1\leq\gamma\leq \infty$ by $\|\cdot\|_{L^\gamma}$. That is, for any $\phi\in L^{\gamma}( \Omega)$,

We denote the $|x|^\sigma$-weighted $L^{p+1}( \Omega)$ space by $L^{p+1}_{\sigma}( \Omega)$, which is defined as

where

By standard arguments as the space $L^{p+1}( \Omega)$, one can see $L^{p+1}_{\sigma}( \Omega)$ is a Banach space with the norm $\|\cdot\|_{L_\sigma^{p+1}}$.

We denote the inner product of $H_0^1( \Omega)$ by $(\cdot,\cdot)_{H_0^1}$, i.e.,

The norm of $H_0^1( \Omega)$ is denoted by $\|\cdot\|_{H_0^1}$, i.e.,

An equivalent norm of $H_0^1( \Omega)$ is $\|\nabla(\cdot)\|_{L^2}$, and by Poincaré's inequality, we have

where $\lambda_1$ is the first eigenvalue of $-\Delta$ with zero Dirichlet boundary condition, i.e,

Moreover, by Theorem 3.2, we have

Then we let $C_{p\sigma}$ as the optimal constant of the embedding $H_0^1( \Omega)\hookrightarrow L^{p+1}_{\sigma}( \Omega)$, i.e.,

We define two functionals $J$ and $I$ on $H_0^1( \Omega)$ by

and

By (3) and (10), we know that $J$ and $I$ are well-defined on $H_0^1( \Omega)$.

We denote the mountain-pass level $d$ by

where $N$ is the Nehari manifold, which is defined as

By Theorem 3.3, we have

where $C_{p\sigma}$ is the positive constant given in (11).

For $\rho\in \mathbb{R}$, we define the sub-level set $J^\rho$ of $J$ as

Then, we define the set $N^\rho: = N\cap J^\rho$. In view of (15), (12), (17), we get

For $\rho>d$, we define two constants

and two sets

Remark 1. There are two remarks on the above definitions.

(1) By the definitions of $N^\rho$, $\lambda_\rho$ and $\Lambda_\rho$, it is easy to see $\lambda_\rho$ is non-increasing with respect to $\rho$ and $\Lambda_\rho$ is non-decreasing with respect to $\rho$.

(2) By Theorem 3.4, we have

Then the sets $S_\rho$ and $S^\rho$ are both nonempty. In fact, for any $\phi\in H_0^1( \Omega)\setminus\{0\}$ and $s>0$,

So,

In this paper we consider weak solutions to problem (1), local existence of which can be obtained by Galerkin's method (see for example [22,Chapter II,Sections 3 and 4]) and a standard limit process and the details are omitted.

Definition 2.1. Assume $u_0\in H_0^1( \Omega)$ and (3) holds. Let $T>0$ be a constant. A function $u = u(x,t)$ is called a weak solution of problem (1) on $\Omega\times[0,T]$ if $u(\cdot,t)\in L^\infty(0,T;H_0^1( \Omega))$, $u_t(\cdot,t)\in L^2(0,T;H_0^1( \Omega))$ and the following equality

holds for any $v\in H_0^1( \Omega)$ and a.e. $t\in[0,T]$. Moreover,

Remark 2. There are some remarks on the above definition.

(1) Since $u(\cdot,t)\in L^\infty(0,T;H_0^1( \Omega))\hookrightarrow L^2(0,T;H_0^1( \Omega))$, $u_t(\cdot,t)\in L^2(0,T; H_0^1( \Omega))$, we have $u\in H^1(0,T; H_0^1( \Omega))$. According to [6], $u\in C([0,T]; H_0^1( \Omega))$, then (23) makes sense. Moreover, by (10), all terms in (22) make sense for $u\in C([0,T];H_0^1( \Omega))$ and $u_t\in L^2(0,T;H_0^1( \Omega))$.

(2) Denote by ${T_{\max}}$ the maximal existence of $u$, then $u(\cdot,t)\in L^\infty(0,T;H_0^1( \Omega))\cap C([0,T];H_0^1( \Omega))$, $u_t(\cdot,t)\in L^2(0,T;H_0^1( \Omega))$ for any $T< {T_{\max}}$.

(3) Taking $v = u$ in (22), we get

where $\|\cdot\|_{H_0^1}$ is defined in (7) and $I$ is defined in (13).

(4) Taking $v = u_t$ in (22), we get

where $J$ is defined in (12).

Definition 2.2. Assume (3) holds. A function $u\in H_0^1( \Omega)$ is called a weak solution of (2) if

holds for any $v\in H_0^1( \Omega)$.

Remark 3. There are some remarks to the above definition.

(1) By (10), we know all the terms in (26) are well-defined.

(2) If we denote by $\Phi$ the set of weak solutions to (2), then by the definitions of $J$ in (12) and $N$ in (15), we have

where $J'(\phi) = 0$ in $H^{-1}( \Omega)$ means $\left\langle J'(\phi),\psi \right\rangle = 0$ for all $\psi\in H_0^1$ and $\left\langle\cdot,\cdot \right\rangle$ means the dual product between $H^{-1}( \Omega)$ and $H_0^1( \Omega)$.

With the set $\Phi$ defined above, we can defined the ground-state solution to (2).

Definition 2.3. Assume (3) holds. A function $u\in H_0^1( \Omega)$ is called a ground-state solution of (2) if $u\in \Phi\setminus\{0\}$ and

With the above preparations, now we can state the main results of this paper. Firstly, we consider the case $J(u_0)\leq d$. By the sign of $I(u_0)$, we can classify the discussions into three cases:

(1) $J(u_0)\leq d$, $I(u_0)>0$ (see Theorem 2.4);

(2) $J(u_0)\leq d$, $I(u_0)<0$ (see Theorem 2.5);

(3) $J(u_0)\leq d$, $I(u_0) = 0$. In this case, by the definition of $d$ in (14), we have $u_0 = 0$ or $J(u_0) = d$ and $I(u_0) = 0$. In Theorem 2.6, we will show problem (1) admits a global solution $u(\cdot,t)\equiv u_0$.

Theorem 2.4. Assume (3) holds and $u = u(x,t)$ is a weak solution to (1) with $u_0\in V$, then $u$ exists globally and

where

In, in addition, $J(u_0)<d$, we have the following decay estimate:

Remark 4. Since $u_0\in V$, we have $I(u_0)>0$. Then it follows from the definitions of $J$ in (12) and $I$ in (13) that

So the equality (28) makes sense.

Theorem 2.5. Assume (3) holds and $u = u(x,t)$ is a weak solution to (1) with $u_0\in W$. Then ${T_{\max}}<\infty$ and $u$ blows up in finite time in the sense of

where

and ${T_{\max}}$ is the maximal existence time of $u$. If, in addition, $J(u_0)<d$, then

Remark 5. There are two remarks.

(1) If $J(\phi)<0$, then we can easily get from the definitions of $J$ and $I$ in (12) and (13) respectively that $I(\phi)<0$. So we have $\phi\in W$ if $J(\phi)<0$.

(2) The sets $V$ and $W$ defined in (29) and (31) respectively are both nonempty. In fact for any $\phi\in H_0^1( \Omega)\setminus\{0\}$, we let

Then (see Fig. 2)

(a) $f(0) = f(s_4^*) = 0$, $f(s)$ is strictly increasing for $s\in(0,s_3^*)$, strictly decreasing for $s\in(s_3^*,\infty)$, $\lim_{s\uparrow\infty}f(s) = -\infty$, and

(b) $g(0) = g(s_3^*) = 0$, $g(s)$ is strictly increasing for $s\in(0,s_1^*)$, strictly decreasing for $s\in(s_1^*,\infty)$, $\lim_{s\uparrow\infty}g(s) = -\infty$, and

(c) $f(s)<g(s)$ for $0<s<s_2^*$, $f(s)>g(s)$ for $s>s_2^*$, and

where

So, $\{s\phi:s_1^*<s<s_3^*\}\subset V$, $\{s\phi: s_3^*<s<\infty\}\subset W$.

Theorem 2.6. Assume (3) holds and $u = u(x,t)$ is a weak solution to (1) with $u_0\in G$. Then problem (1) admits a global solution $u(\cdot,t)\equiv u_0(\cdot)$, where

Remark 6. There are two remarks on the above theorem.

(1) Unlike Remark 5, it is not easy to show $G\neq\emptyset$. In fact, if we use the arguments as in Remark 5, we only have $J(s_3^*\phi)\leq d$ and $I(s_3^*\phi) = 0$ (see Fig. 2 and (33)). In Theorem 2.7, we will use minimizing sequence argument to show $G\neq\emptyset$.

(2) To prove the above Theorem, we only need to show $G$ is the set of the ground-state solution of (2), which is done in Theorem 2.7.

Theorem 2.7. Assume (3) holds and let $G$ be the set defined in (34), then $G\neq\emptyset$ and $G$ is the set of the ground-state solution of (2).

Secondly, we consider the case $J(u_0)>d$, and we have the following theorem.

Theorem 2.8. Assume (3) holds and the initial value $u_0\in H_0^1( \Omega)$ satisfying $J(u_0)>d$.

(i): If $u_0\in S_\rho$ with $\rho\geq J(u_0)$, then problem (1) admits a global weak solution $u = u(x,t)$ and $\|u(\cdot,t)\|_{H_0^1}\downarrow 0$ as $t\uparrow\infty$.

(ii): If $u_0\in S^\rho$ with $\rho\geq J(u_0)$, then the weak solution $u = u(x,t)$ of problem (1) blows up in finite time.

Here $S_\rho$ and $S^\rho$ are the two sets defined in (20).

Next, we show the solution of the problem (1) can blow up at arbitrary initial energy level (Theorem 2.10). To this end, we firstly introduce the following theorem.

Theorem 2.9. Assume (3) holds and $u = u(x,t)$ is a weak solution to (1) with $u_0\in \hat W$. Then

and $u$ blows up in finite time in the sense of

where

and ${T_{\max}}$ is the maximal existence time of $u$.

By using the above theorem, we get the following theorem.

Theorem 2.10. For any $M\in \mathbb{R}$, there exists $u_0\in H_0^1( \Omega)$ satisfying $J(u_0) = M$ such that the corresponding weak solution $u = u(x,t)$ of problem (1) blows up in finite time.

3.   Preliminaries

The following lemma can be found in [11].

Lemma 3.1. Suppose that $0<T\leq\infty$ and suppose a nonnegative function $F(t)\in C^2[0,T)$ satisfies

for some constant $\gamma>0$. If $F(0)>0$, $F'(0)>0$, then

and $F(t)\uparrow\infty$ as $t\uparrow T$.

Theorem 3.2. Assume $p$ and $\sigma$ satisfy (3). Then $H_0^1( \Omega)\hookrightarrow L^{p+1}_{\sigma}( \Omega)$ continuously and compactly.

Proof. Since $\Omega\subset \mathbb{R}^n$ is a bounded domain, there exists a ball $B(0,R): = \{x\in \mathbb{R}^n:|x| = \sqrt{x_1^2+\cdots x_n^2}<R\}\supset \Omega$.

We divide the proof into three cases. We will use the notation $a\lesssim b$ which means there exits a positive constant $C$ such that $a\leq Cb$.

Case 1. $\sigma\geq0$. By the assumption on $p$ in (3), one can see

Then we have, for any $u\in H_0^1( \Omega)$,

which, together with (37), implies $H_0^1( \Omega)\hookrightarrow L^{p+1}_{\sigma}( \Omega)$ continuously and compactly.

Case 2. $-n<\sigma<0$ and $n = 1$ or $2$. We can choose $r\in\left(1,-\frac{n}{\sigma}\right)$. Then by Hölder's inequality and

for any $u\in H_0^1( \Omega)$, we have

which, together with (38), implies $H_0^1( \Omega)\hookrightarrow L^{p+1}_{\sigma}( \Omega)$ continuously and compactly.

Case 3. $\frac{(p+1)(n-2)}{2}-n<\sigma<0$ and $n\geq 3$. Then there exists a constant $r>1$ such that

By the second inequality of the above inequalities, we have

So,

Then by Hölder's inequality, for any $u\in H_0^1( \Omega)$, we have

which, together with (39), implies $H_0^1( \Omega)\hookrightarrow L^{p+1}_{\sigma}( \Omega)$ continuously and compactly. Here $\omega_{n-1}$ denotes the surface area of the unit ball in $\mathbb{R}^n$.

Theorem 3.3. Assume $p$ and $\sigma$ satisfy (3). Let $d$ be the constant defined in (14), then

where $C_{p\sigma}$ is the positive constant defined in (11).

Proof. Firstly, we show

where $N$ is the set defined in (15) and

By the definition of $N$ in (15) and $s_\phi^*$ in (41), one can easily see that $s_\phi^* = 1$ if $\phi\in N$ and $s_\phi^*\phi\in N$ for any $\phi\in H_0^1( \Omega)\setminus\{0\}$.

On one hand, since $N\subset H_0^1( \Omega)$ and $s_\phi^* = 1$ for $\phi\in N$, we have

On the other hand, since $\{s_\phi^*\phi: \phi\in H_0^1( \Omega)\setminus\{0\}\}\subset N$, we have

Then (40) follows from the above two inequalities.

By (40), the definition of $d$ in (14), the definition of $J$ in (12), and the definition of $C_{p\sigma}$ in (11), we have

Theorem 3.4. Assume (3) holds. Let $\lambda_\rho$ and $\Lambda_\rho$ be the two constants defined in (19). Here $\rho>d$ is a constant. Then

Proof. Let $\rho>d$ and $N^\rho$ be the set defined in (18). By the definitions of $\lambda_\rho$ and $\Lambda_\rho$ in (19), it is obvious that

Since $N^\rho\subset N$, it follows from the definitions of $d$, $J$, $I$ in (14), (12), (13), respectively, that

which implies

On the other hand, by (8) and (18), we have

Combining the above two inequalities with (43), we get (42), the proof is complete.

Theorem 3.5. Assume (3) holds and $u = u(x,t)$ is a weak solution to (1). Then the sets $W$ and $V$, defined in (31) and (29) respectively, are both variant for $u$, i.e., $u(\cdot,t)\in W$ ($u(\cdot,t)\in V$) for $0\leq t< {T_{\max}}$ when $u_0\in W$ ($u_0\in V$), where ${T_{\max}}$ is the maximal existence time of $u$.

Proof. We only prove the invariance of $W$ since the proof of the invariance of $V$ is similar.

For any $\phi\in W$, since $I(\phi)<0$, it follows from the definition of $I$ (see (13)) and (11) that

which implies

Let $u(x,t)$ be the weak solution of problem (1) with $u_0\in W$. Since $I(u_0)<0$ and $u\in C([0, {T_{\max}}), H_0^1( \Omega))$, there exists a constant $\varepsilon>0$ small enough such that

Then by (24), $\frac{d}{dt}\|u(\cdot,t)\|_{H_0^1}^2>0$ for $t\in[0, \varepsilon]$, and then by (25) and $J(u_0)\leq d$, we get

We argument by contradiction. Since $u(\cdot,t)\in C([0, {T_{\max}}),H_0^1( \Omega))$, if the conclusion is not true, then there exists a $t_0\in(0, {T_{\max}})$ such that $u(\cdot,t)\in W$ for $0\leq t<t_0$, but $I(u(\cdot,t_0)) = 0$ and

(note (25) and (46), $J(u(\cdot,t_0)) = d$ cannot happen). By (44), we have $u(\cdot,t)\in C([0, {T_{\max}}),H_0^1( \Omega))$ and $u(\cdot,t_0)\in \overline{W}$, then

which, together with $I(u(\cdot,t_0)) = 0$, implies $u(\cdot,t_0)\in N$. Then it follows from the definition of $d$ in (14) that

which contradicts (47). So the conclusion holds.

Theorem 3.6. Assume (3) holds and $u = u(x,t)$ is a weak solution to (1) with $u_0\in W$. Then

where $W$ is defined in (31) and ${T_{\max}}$ is the maximal existence time of $u$.

Proof. Let $N^-: = \{\phi\in H_0^1( \Omega):I(\phi)<0\}$. Then by Theorem 3.5, $u(\cdot,t)\in N^-$ for $0\leq t< {T_{\max}}$.

By the proof in Theorem 3.3,

where we have used $I(u(\cdot,t))<0$ in the last inequality. Since $I(u(\cdot,t))<0$, we get from (41) that

Then

and (48) follows from the above inequality.

Theorem 3.7. Assume (3) holds and $u = u(x,t)$ is a weak solution to (1) with $u_0\in\hat W$. Then $I(u(\cdot,t))<0$ for $0\leq t< {T_{\max}}$, where ${T_{\max}}$ is the maximal existence time of $u$ and $\hat W$ is defined in (36)

Proof. Firstly, we show $I(u_0)<0$. In fact, by the definition of $J$ in (12), $u_0\in\hat W$, and (8), we get

which implies

Secondly, we prove $I(u(\cdot,t))<0$ for $0<t< {T_{\max}}$. In fact, if it is not true, in view of $u\in C([0, {T_{\max}}),H_0^1( \Omega))$, there must exist a $t_0\in(0, {T_{\max}})$ such that $I(u(\cdot,t))<0$ for $t\in[0,t_0)$ but $I(u(\cdot,t_0)) = 0$. Then by (24), we get $\|u(\cdot,t_0)\|_{H_0^1}^2> \|u_0\|_{H_0^1}^2$, which, together with $u_0\in\hat W$ and (8), implies

On the other hand, by (24), (12), (13) and $I(u(\cdot,t_0)) = 0$, we get

which contradicts (49). The proof is complete.

4.   Proofs of the main results

Proof of Theorem 2.4. Let $u = u(x,t)$ be a weak solution to (1) with $u_0\in V$ and ${T_{\max}}$ be its maximal existence time. By Theorem 3.5, $u(\cdot,t)\in V$ for $0\leq t< {T_{\max}}$, which implies $I(u(\cdot,t))>0$ for $0\leq t< {T_{\max}}$. Then it follows from (25), (12) and (13) that

which implies $u$ exists globally (i.e. ${T_{\max}} = \infty$) and

Next, we prove $\|u(\cdot,t)\|_{H_0^1}$ decays exponentially, if in addition, $J(u_0)<d$. By (24), (13), (11), (50), (16) we have

which leads to

The proof is complete.

Proof of Theorem 2.5. Let $u = u(x,t)$ be a weak solution to (1) with $u_0\in W$ and ${T_{\max}}$ be its maximal existence time.

Firstly, we consider the case $J(u_0)<d$ and $I(u_0)<0$. By Theorem 3.5, $u(\cdot,t)\in W$ for $0\leq t< {T_{\max}}$. Let

For any $T^*\in(0, {T_{\max}})$, $\beta>0$ and $\alpha>0$, we let

Then

and (by (24), (12), (13), (48), (25))

Since $I(u(\cdot,t))<0$, it follow from (24) and the first equality of (54) that

Then

By (6), Schwartz's inequality and Hölder's inequality, we have

which, together with the definition of $F(t)$, implies

Then it follows from (54) and the above inequality that

In view of (55), (56), and (57), we have

If we take $\beta$ small enough such that

then $F(t)F''(t)-\frac{p+1}{2}(F'(t))^2\geq0$. Then, it follows from Lemma 3.1 that

Then for

we get

Minimizing the above inequality for $\alpha$ satisfying (59), we get

Minimizing the above inequality for $\beta$ satisfying (58), we get

By the arbitrariness of $T^*< {T_{\max}}$ it follows that

Secondly, we consider the case $J(u_0) = d$ and $I(u_0)<0$. By the proof of Theorem 3.5, there exists a $t_0>0$ small enough such that $J(u(\cdot,t_0))<d$ and $I(u(\cdot,t_0))<0$. Then it follows from the above proof that $u$ will blow up in finite time. The proof is complete.

Proof of Theorems 2.6 and 2.7. Since Theorem 2.6 follows from Theorem 2.7 directly, we only need to prove Theorem 2.7.

Firstly, we show $G\neq\emptyset$. By the definition of $d$ in (14), we get

Then a minimizing sequence $\{\phi_k\}_{k = 1}^\infty\subset N$ exists such that

which implies $\{\phi_k\}_{k = 1}^\infty$ is bounded in $H_0^1( \Omega)$. Since $H_0^1( \Omega)$ is reflexive and $H_0^1( \Omega)\hookrightarrow L_\sigma^{p+1}$ continuously and compactly (see (10)), there exists $\varphi\in H_0^1( \Omega)$ such that

(1) $\phi_k\rightharpoonup \varphi$ in $H_0^1( \Omega)$ weakly;

(2) $\phi_k\rightarrow \varphi$ in $L_\sigma^{p+1}( \Omega)$ strongly.

Now, in view of $\|\nabla(\cdot)\|_{L^2}$ is weakly lower continuous in $H_0^1( \Omega)$, taking $\liminf_{k\uparrow\infty}$ in the equality $\|\nabla\phi_k\|_{L^2}^2 = \|\phi_k\|_{L_\sigma^{p+1}}^{p+1}$ (sine $\phi_k\in N$), we get

We claim

In fact, if the claim is not true, then by (61),

By the proof of Theorem 3.3, we know that $s^*_\varphi\varphi\in N$, which, together with the definition of $d$ in (14), implies

where

On the other hand, since $s^*_\varphi\varphi\in N$, we get from the definitions of $J$ in (12) and $I$ in (13), $s^*_\varphi\in(0,1)$, $\|\nabla(\cdot)\|_{L^2}$ is weakly lower continuous in $H_0^1( \Omega)$, (60) that

which contradicts to (63). So the claim is true, i.e.

which, together with $H_0^1( \Omega)$ is uniformly convex and $\phi_k\rightharpoonup \varphi$ in $H_0^1( \Omega)$ weakly, implies $\phi_k\rightarrow \varphi$ strongly in $H_0^1( \Omega)$. Then by (60), $J(\varphi) = d$, which, together with (62) and the definition of $G$ in (34), implies $\varphi\in G$, i.e., $G\neq\emptyset$.

Second, we prove $G\subset\Phi$, where $\Phi$ is the set defined in (27). For any $\varphi\in G$, we need to show $\varphi\in\Phi$, i.e. $\varphi$ satisfies (26). Fix any $v\in H_0^1( \Omega)$ and $s\in(- \varepsilon, \varepsilon)$, where $\varepsilon>0$ is a small constant such that $\|\varphi+sv\|_{L_\sigma^{p+1}}^{p+1}>0$ for $s\in(- \varepsilon, \varepsilon)$. Let

Then $I(\tau(s)(\varphi+sv)) = 0$. So by the definition of $N$ in (15), the set

is a curve on $N$, which passes $\varphi$ when $s = 0$. The function $\tau(s)$ is differentiable and

where

Since (62), we get $\tau(0) = 1$ and

Let

Since $\tau(s)(\varphi+sv)\in N$ for $s\in(- \varepsilon, \varepsilon)$, $\tau(s)(\varphi+sv)|_{s = 0} = \varphi$, $\varrho(0) = J(\varphi) = d$, it follows from the definition of $d$ that $\varrho(s)$ $(s\in(- \varepsilon, \varepsilon))$ achieves its minimum at $s = 0$, then $\varrho'(0) = 0$. So,

So, $\varphi\in\Phi$, i.e. $G\subset \Phi$. Moreover, we have $G\subset (\Phi\setminus\{0\})$ since $\varphi\neq0$ for any $\varphi\in G$.

Finally, in view of Definition 2.3 and $J(\varphi) = d$ $(\forall \varphi\in G)$, to complete the proof, we only need to show

In fact, by the above proof and (27), we have $G\subset\Phi\setminus\{0\}\subset N$. Then, in view of the definition of $d$ in (14), i.e.,

and $J(\varphi) = d$ for any $\varphi\in G$, we get (66). The proof is complete.

Proof of Theorem 2.8. Let $u = u(x,t)$ be the solution of problem (1) with initial value $u_0$ satisfying $J(u_0)>d$. We denote by ${T_{\max}}$ the maximal existence of $u$. If $u$ is global, i.e. ${T_{\max}} = \infty$, we denote by

the $\omega$-limit set of $u_0$.

(i) Assume $u_0\in S_\rho = \{\phi\in H_0^1( \Omega):\|\phi\|_{H_0^1}\leq \lambda_\rho, I(\phi)>0\}$ (see (20)) with $\rho\geq J(u_0)$. Without loss of generality, we assume $u(\cdot,t)\not = 0$ for $0\leq t< {T_{\max}}$. In fact it there exists a $t_0$ such that $u(\cdot,t_0) = 0$, then it is easy to see the function $v$ defined as

is a global weak solution of problem (1), and the proof is complete.

We claim that

Since $I(u_0)>0$, if the claim is not true, there exists a $t_0\in(0, {T_{\max}})$ such that

and

which together with the definition of $N$ in (15) and the assumption that $u(\cdot,t)\neq0$ for $0\leq t< {T_{\max}}$, implies $u(\cdot,t_0)\in N$. Moreover, by using (68), similar to the proof of (46), we have $J(u(\cdot,t_0))<J(u_0)$, i.e. $u(\cdot,t_0)\in J^{J(u_0)}$ (see (17)). Then $u(\cdot,t_0)\in N^{J(u_0)}$ (since $N^{J(u_0)} = N\cap J^{J(u_0)}$) and then $\|u(\cdot,t_0)\|_{H_0^1}\geq \lambda_{J(u_0)}$ (see (19)). By monotonicity (see Remark 1) and $\rho\geq J(u_0)$, we get

On the other hand, it follows from (24), (68) and $u_0\in S_\rho$ that

which contradicts (70). So (67) is true. Then by (24) again, we get

which implies $u$ exists globally, i.e. ${T_{\max}} = \infty$.

By (24) and (67), $\|u(\cdot,t)\|_{H_0^1}$ is strictly decreasing for $0\leq t<\infty$, so a constant $c\in[0,\|u_0\|_{H_0^1})$ exists such that

Taking $t\uparrow\infty$ in (24), we get

Note that $I(u(\cdot,s))>0$ for $0\leq s<\infty$, so, for any sequence $\{t_n\}$ satisfying $t_n\uparrow\infty$ as $n\uparrow\infty$, if the limit $\lim_{n\uparrow\infty}I(u(\cdot,t_n))$ exists, it must hold

Let $\omega$ be an arbitrary element in $\omega(u_0)$. Then there exists a sequence $\{t_n\}$ satisfying $t_n\uparrow\infty$ as $n\uparrow\infty$ such that

Then by (71), we get

As the above, one can easily see

which implies $\omega\notin N^{J(u_0)}$. In fact, if $\omega \in N^{J(u_0)}$, by (19), $\lambda_{J(u_0)}\leq \|\omega\|_{H_0^1}$, a contradiction. Since $N^{J(u_0)} = N\cap J^{u_0}$ and $\omega\in J^{J(u_0)}$, we get $\omega\notin N$. Therefore, by the definition of $N$ in (15) and (73), $\omega = 0$, then it follows from $\|u(\cdot,t)\|_{H_0^1}$ is strictly decreasing and (72) that

(ⅱ) Assume $u_0\in S^\rho = \{\phi\in H_0^1( \Omega):\|\phi\|_{H_0^1}\geq\Lambda_\rho, I(\phi)<0\}$ (see (20)) with $\rho\geq J(u_0)$. We claim that

Since $I(u_0)<0$, if the claim is not true, there exists a $t_0\in(0, {T_{\max}})$ such that

and

Since (75), by (44) and $u\in C([0, {T_{\max}}),H_0^1( \Omega))$, we get

which, together with the definition of $N$ in (15), implies $u(\cdot,t_0)\in N$. Moreover, by using (75), similar to the proof of (46), we have $J(u(\cdot,t_0))<J(u_0)$, i.e. $u(\cdot,t_0)\in J^{J(u_0)}$ (see (17)). Then $u(\cdot,t_0)\in N^{J(u_0)}$ (since $N^{J(u_0)} = N\cap J^{J(u_0)}$) and then $\|u(\cdot,t_0)\|_{H_0^1}\leq \Lambda_{J(u_0)}$ (see (19)). By monotonicity (see Remark 1) and $\rho\geq J(u_0)$, we get

On the other hand, it follows from (24), (75) and $u_0\in S^\rho$ that

which contradicts (77). So (74) is true.

Suppose by contradiction that $u$ does not blow up in finite time, i.e. ${T_{\max}} = \infty$. By (24) and (74), $\|u(\cdot,t)\|_{H_0^1}$ is strictly increasing for $0\leq t<\infty$. If the limit $\lim_{t\uparrow\infty}\|u(t)\|_{H_0^1}$ exists, i.e. there exists a constant $\tilde c\in[\|u_0\|_{H_0^1},\infty)$ such that

Taking $t\uparrow\infty$ in (24), we get

Note $-I(u(\cdot,s))>0$ for $0\leq s<\infty$, so, for any sequence $\{t_n\}$ satisfying $t_n\uparrow\infty$ as $n\uparrow\infty$, if the limit $\lim_{n\uparrow\infty}I(u(\cdot,t_n))$ exists, it must hold

Let $\omega$ be an arbitrary element in $\omega(u_0)$. Then there exists a sequence $\{t_n\}$ satisfying $t_n\uparrow\infty$ as $n\uparrow\infty$ such that

Since $\|u(\cdot,t)\|_{H_0^1}$ is strictly increasing, $\lim_{t\uparrow\infty}\|u(\cdot,t)\|_{H_0^1}$ exists and

Then by (78), we get

By (24), (25) and (74), one can easily see

which implies $\omega\notin N^{J(u_0)}$. In fact, if $\omega \in N^{J(u_0)}$, by (19), $\Lambda_{J(u_0)}\geq \|\omega\|_{H_0^1}$, a contradiction. Since $N^{J(u_0)} = N\cap J^{u_0}$ and $\omega\in J^{J(u_0)}$, we get $\omega\notin N$. Therefore, by the definition of $N$ in (15) and (80), $\omega = 0$. However, this contradicts $\|\omega\|_{H_0^1}>\Lambda_{J(u_0)}>0$. So $u$ blows up in finite time. The proof is complete.

Proof of Theorem 2.9. Let $u = u(x,t)$ be a weak solution of (1) with $u_0\in\hat W$ and ${T_{\max}}$ be its maximal existence time, where $\hat W$ is defined in (36). By Theorem 3.7, we know that $I(u(\cdot,t))<0$ for $0\leq t< {T_{\max}}$. Then by (8) and (24), we get

The remain proofs are similar to the proof of Theorem 2.9. For any $T^*\in(0, {T_{\max}})$, $\beta>0$ and $\alpha>0$, we consider the functional $F(t)$ again (see (52)). We also have (53), (54), but there are some differences in (55), in fact, by (81) and (25), we have

We also have (56) and (57). Then it follows from (56), (57) and (82) that

If we take $\beta$ small enough such that

then $F(t)F''(t)-\frac{p+1}{2}(F'(t))^2\geq0$. Then, it follows from Lemma 3.1 that

Then for

we get

Minimizing the above inequality for $\alpha$ satisfying (84), we get

Minimizing the above inequality for $\beta$ satisfying (58), we get

By the arbitrariness of $T^*< {T_{\max}}$ it follows that

Proof of Theorem 2.10. For any $M\in \mathbb{R}$, let $\Omega_1\subset \Omega$ and $\Omega_2\subset \Omega$ be two arbitrary disjoint open domains. Let $\psi\in H_0^1( \Omega_2)\setminus\{0\}$, extending $\psi$ to $\Omega$ by letting $\psi = 0$ in $\Omega\setminus \Omega_2$, then $\psi\in H_0^1( \Omega)$. We choose $\alpha$ large enough such that

For such $\alpha$ and $\psi$, we take a $\phi\in H_0^1( \Omega_1)\setminus\{0\}$ (which is extended to $\Omega$ by letting $\phi = 0$ in $\Omega\setminus \Omega_1$ i.e. $\phi\in H_0^1( \Omega)$) such that

where (see Remark 5)

which can be done since

and $\phi$ can be chosen such that $\|\nabla \phi\|_{L^2}\gg\|\phi\|_{L_\sigma^{p+1}}$.

By Remark 5 again,

By (87) and (86), we can choose $s\in[0,\infty)$ such that $v: = s\phi$ satisfies $J(v) = M-J( \alpha \psi)$. Letting $u_0: = v+ \alpha \psi\in H_0^1( \Omega)$, since $\Omega_1$ and $\Omega_2$ are disjoint, we get

and (note (85))

Let $u = u(x,t)$ be the weak solution of problem (1) with initial value $u_0$ given above. Then by Theorem 2.9, $u$ blows up in finite time.