1. Introduction

The present paper is devoted to the following differential system, which is composed by a fourth order differential equation and a second order one. Namely,

$\begin{align}\label{sys} \begin{cases} (M+2m\xi)y_{tt} = -EIy_{xxxx}+H_0\left(\frac{2y_x}{\xi^2}+\frac{3 s'(y_x^2+\ell^2\theta_x^2)}{\xi^4}\right)_x-\frac{EA}{L_c}\left[\int_0^L{\frac{y_x^2+\ell^2\theta_x^2}{\xi^3}}\right]\frac{s''}{\xi^3}\\ ~~~~~~~~~~~~~~~~~~~~~~~~~~~~~-\frac{2EA}{L_c}\left[\int_0^L{\frac{s''y}{\xi^3}}\right]\left(\frac{s'}{\xi}-\frac{y_x}{\xi^3}\right)_x+\frac{2EA\ell^2}{L_c}\left[\int_0^L{\frac{s''\theta}{\xi^3}}\right] \left(\frac{\theta_x}{\xi^3}\right)_x, \qquad x\in(0, L), t>0, \\ \left(\frac{M}{3}+2m\xi\right)\theta_{tt} = \frac{GK}{\ell^2}\theta_{xx}+2H_0\left(\frac{\theta_x}{\xi^2}+\frac{3 s'y_x\theta_x}{\xi^4}\right)_x-\frac{2EA}{L_c}\left[\int_0^L{\frac{y_x\theta_x}{\xi^3}}\right]\frac{s''}{\xi^3}\\ ~~~~~~~~~~~~~~~~~~~~~~~~~~~~~-\frac{2EA}{L_c}\left[\int_0^L{\frac{s''\theta}{\xi^3}}\right]\left(\frac{s'}{\xi}-\frac{y_x}{\xi^3}\right)_x+\frac{2EA}{L_c}\left[\int_0^L{\frac{s''y}{\xi^3}}\right] \left(\frac{\theta_x}{\xi^3}\right)_x, \qquad ~~~x\in(0, L), t>0. \end{cases} \end{align}$

(1.1)

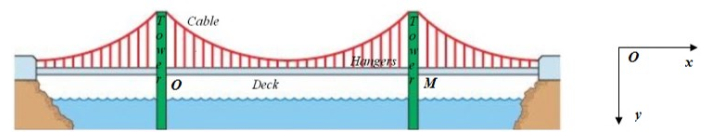
The system (1.1), suggested by Arioli-Gazzola ^[2], aims to model the main span of a suspension bridge. In ^[2], the authors considered torsional instability through several numerical results, see ^[8,9] for some more recent results on torsional instability. Here we give a simple sketch for suspension bridges in Figure 1. The deck, which is viewed as a thin rectangular plate, is hooked to a large number of hangers. At their upper endpoints, the hangers are connected with the main cables, which are sustained by the towers. We assume that the origin of the coordinate system is at the point $O$ and that the positive vertical axis is oriental downwards, see Fig. 1.

In system (1.1), $L$ is the length of the deck between the two towers and $2\ell$ is its width, $y = y(x, t)$ is the vertical (downwards) displacement of the longitudinal midline of the deck, $\theta = \theta(x, t)$ is the torsional angle of the deck. The position of the cables at rest is denoted by $-s = -s(x) < 0$ (the positive direction is vertical downwards). Hence, the function

$\xi = \xi(x): = \sqrt{1+s'(x)^2}$

represents the length of the cable at rest. For the other parameters $M, m, E, I, H_0, L_c, A, G, K$ which are positive constants, one can find their physical meanings in [2,Section 4.4].

As in Arioli-Gazzola ^[1], the degenerate plate (modeling the deck of the main span of the bridge) is assumed to be hinged at its two short edges where the cross sections between the towers cannot rotate. Hence, the boundary conditions associated to (1.1) are

$y(0, t) = y(L, t) = y_{xx}(0, t) = y_{xx}(L, t) = \theta(0, t) = \theta(L, t) = 0, \qquad \forall t>0.$

(1.2)

In order to consider an initial value problem, we introduce the following conditions:

$y(x, 0) = y^0, \quad y_t(x, 0) = y^1, \quad \theta(x, 0) = \theta^0, \quad \theta_t(x, 0) = \theta^1, \qquad \forall x\in (0, L).$

(1.3)

The system (1.1) is suggested, recently, by Arioli-Gazzola ^[2] for describing the dynamics of a suspension bridge. In that article, they made several numerical experiments for the nonlinear model (1.1) and gave a new explanation for the torsional instability in suspension bridge, but they did not fully study the system (1.1) from the theoretical point of view. Hence, the aim of this paper is to solve the initial-boundary value problem (1.1)-(1.2)-(1.3) theoretically. For simplicity, we write them as follows

$\begin{align}\label{linpy} \begin{cases} y_{tt}+\Phi y_{xxxx} = h_1(y, \theta), \quad &x\in(0, L), t>0, \\ \theta_{tt}-\Psi_y(x, t)\theta_{xx} = h_2(y, \theta), \quad &x\in(0, L), t>0, \\ y(0, t) = y(L, t) = y_{xx}(0, t) = y_{xx}(L, t) = \theta(0, t) = \theta(L, t) = 0, \quad &t>0, \\ y(x, 0) = y^0, \quad y_t(x, 0) = y^1, \quad \theta(x, 0) = \theta^0, \quad \theta_t(x, 0) = \theta^1, \quad & x\in (0, L), \end{cases} \end{align}$

(1.4)

where $\Phi = \Phi(x) = \frac{EI}{M+2m\xi}$, $\Psi_y(x, t) = \left(\frac{M}{3}+2m\xi\right)^{-1}\left(\frac{GK}{\ell^2}+\frac{2H_0}{\xi^2}+\frac{6H_0s'y_x}{\xi^4}+\frac{2AE}{\xi^3L_c}\int_0^L{\frac{s''y}{\xi^3}}\right)$ and the two operators $h_1(\cdot, \cdot)$, $h_2(\cdot, \cdot)$: $H^4(0, L)\times H^3(0, L)\mapsto L^2(0, L)$ are given by

$\begin{align*} h_1(y, \theta) = &\frac{H_0}{M+2m\xi}\left(\frac{2y_x}{\xi^2}+\frac{3 s'(y_x^2+\ell^2\theta_x^2)}{\xi^4}\right)_x-\frac{EA}{(M+2m\xi) L_c}\left[\int_0^L{\frac{y_x^2+\ell^2\theta_x^2}{\xi^3}}\right]\frac{s''}{\xi^3}\\ &-\frac{2EA}{(M+2m\xi)L_c}\left[\int_0^L{\frac{s''y}{\xi^3}}\right]\left(\frac{s'}{\xi}-\frac{y_x}{\xi^3}\right)_x+\frac{2EA\ell^2}{(M+2m\xi)L_c}\left[\int_0^L{\frac{s''\theta}{\xi^3}}\right] \left(\frac{\theta_x}{\xi^3}\right)_x, \\ h_2(y, \theta) = &\left(\frac{M}{3}+2m\xi\right)^{-1}\left[\left(\frac{2H_0}{\xi^2}+\frac{6 H_0 s'y_x}{\xi^4}+\frac{2AE}{\xi^3L_c}\left[\int_0^L{\frac{s''y}{\xi^3}}\right]\right)_x\theta_x-\frac{2EA}{L_c}\left[\int_0^L{\frac{y_x\theta_x}{\xi^3}}\right]\frac{s''}{\xi^3}\right]\\ &-\left(\frac{M}{3}+2m\xi\right)^{-1}\frac{2EA}{L_c}\left[\int_0^L{\frac{s''\theta}{\xi^3}}\right]\left(\frac{s'}{\xi}-\frac{y_x}{\xi^3}\right)_x. \end{align*}$

The second order differential equation in (1.4) is of mixed type and the unpleasant terms $h_1(y, \theta)$, $h_2(y, \theta)$ are nonlinear and nonlocal. Moreover, the term $h_1(y, \theta)$ contains the second order derivatives of $\theta$ in $x$ whereas $h_2(y, \theta)$ includes the couplings such as $y_x\theta_x$ and the second order derivatives of $y$ in $x$. These peculiarities show that (1.4) is a nonstandard problem and it appears quite challenging to prove existence of a solution. Here we restrict to the case where the second order equation in (1.4) is a "hyperbolic" type one, i.e. $\Psi_y(x, t)>0$ and then we investigate the system (1.4) by the Galerkin method as the researchers study this type of (nonlinear) hyperbolic equations or systems, see for instance ^{[1,3,10,11,12]} and references therein. However, note that the complicated terms $h_1(y, \theta)$ and $h_2(y, \theta)$ include the second order derivatives of $y, \theta$, we may obtain solutions by the Galerkin method only if the initial data in higher regularity functional spaces such as $H^4\times H^3$. Moreover, the terms $h_1(y, \theta)$ and $h_2(y, \theta)$ are somewhat different from the cases, such as Ball ^[3] or Temam ^[11] and references therein we met before, it seems out of reach to obtain existence results by applying their methods directly.

In this paper we follow a different path and tackle the problem (1.4) in some high regularity functional spaces. We first solve a correspond linear problem and we obtain existence and uniqueness results. Then we show that the original system admits a local solution by applying the Contraction Mapping principle and the iterative method.

Let

$\begin{align*} H^2_*& = H^2_*(0, L): = \{u\in H^2(0, L);u(x) = 0 \mbox{ on }\{0, L\}\}, \\ H^3_*& = H^3_*(0, L): = \{u\in H^3(0, L);u(x) = u''(x) = 0 \mbox{ on }\{0, L\}\}, \\ H^4_*& = H^4_*(0, L): = \{u\in H^4(0, L);u(x) = u''(x) = 0 \mbox{ on }\{0, L\}\}, \end{align*}$

which are complete subspaces of the Hilbert spaces $H^2(0, L)$, $H^3(0, L)$ and $H^4(0, L)$ respectively. Denote

$Y: = C^0([0, T];H^4_*(0, L))\cap C^1([0, T];H^2_*(0, L))\cap C^2([0, T];L^2(0, L));$

$\Theta: = C^0([0, T];H^3_*(0, L))\cap C^1([0, T];H^2_*(0, L))\cap C^2([0, T];L^2(0, L)).$

We say that $(y, \theta)\in Y\times \Theta$ is a strong solution of (1.4) if it fulfills the initial conditions and if the following equations are satisfied for every $t\in [0, T]$:

$y_{tt}+\Phi y_{xxxx} = h_1(y, \theta), \quad \theta_{tt}-\Psi_y(x, t)\theta_{xx} = h_2(y, \theta).$

Then we prove

Theorem 1.1. Assume that $s\in C^4(0, L)\cap C^3([0, L])$. Let $y^0\in H_*^4$, $\theta^0\in H_*^3$ and $y^1, \theta^1\in H_*^2$. Then for a small $T>0$ there exists a strong solution $(y, \theta)$ of (1.4). Moreover,

$\theta\in C^2([0, T];H_0^1(0, L)).$

Remark 1.2. The component $\theta$ of $(y, \theta)$ is a classical solution to the second order equation.

By the Sobolev embedding theorem, we have $\theta_{xx}\in C^0([0, T]\times[0, L])$, and then $\theta_{tt}\in C^0([0, T]\times[0, L])$. Hence, $\theta\in C^2([0, L]\times [0, T])$.

Remark 1.3. The existence of global solutions of (1.4) is still open.

According to [2,Section 2.2], where the authors analyzed all the energies of the bridge, we can write the total energy of the structure for $t\geq 0$ as follows

$\begin{align}\label{er} E(t) = &\frac{M}{2}\int_0^L{\left[y_t^2+\frac{\ell^2\theta_t^2}{3}\right]}dx+m\int_0^L{\left[y_t^2+\ell^2\theta_t^2\right]\xi}dx+\frac{EI}{2}\int_0^L{y_{xx}^2}dx+\frac{GK}{2}\int_0^L{\theta_x^2}dx\nonumber\\ &-(Mg+2mg)\int_0^L{y}dx-2H_0\int_0^L{s'y_x}dx+H_0\int_0^L{\left[\frac{y_x^2+\ell^2\theta_x^2}{\xi^2}\right]}dx\nonumber\\ &+\frac{AE}{L_c}\left[\left(\int_0^L{\frac{s'y_x}{\xi}}dx\right)^2+\left(\int_0^L{\frac{\ell s'\theta_x}{\xi}}dx\right)^2\right]+H_0\int_0^L{\left[\frac{s'y_x(y_x^2+3\ell^2\theta_x^2)}{\xi^4}\right]}dx\nonumber\\ &-\frac{AE}{L_c}\int_0^L{\frac{s'y_x}{\xi}}dx\int_0^L{\left[\frac{y_x^2+\ell^2\theta_x^2}{\xi^3}\right]}dx-\frac{AE}{L_c}\int_0^L{\frac{\ell s'\theta_x}{\xi}}dx\int_0^L{\frac{\ell y_x\theta_x}{\xi^3}}dx. \end{align}$

(1.5)

Although the energy is conserved, we cannot guarantee that the solution of (1.4) is global in time. The reason is that the functions $s'y_x$, $s'\theta_x$ and $y_x\theta_x$ may have indefinite sign, one does not know if the sum of the last three terms in (1.5) is positive or negative. If the sum has the negative sign, then it can happen that it goes to $-\infty$ at some time whereas the sum of the remainder terms in (1.5) goes to $+\infty$ even if the energy still remains constant. In that case the solution may blow up in finite time.

---

2. Preliminaries

On the Hilbert space $L^2(0, L)$, we denote the standard scalar product and norm by

$(u, v)_2: = \int_0^L{uv}dx;\quad \|u\|_2: = \left(\int_0^L{u^2}dx\right)^{1/2}, \qquad \forall u, v\in L^2(0, L).$

Then the scalar products, the norms of the Hilbert spaces $H_0^1(0, L)$ and $H^2_*(0, L)$ can be defined, respectively, by

$\begin{align*}(u, v)_{H_0^1}&: = (u', v')_2, \quad \|u\|_{H_0^1}: = \|u'\|_2, \qquad \forall u, v\in H_0^1(0, L);\\ (u, v)_{H_*^2}&: = (u'', v'')_2, \quad \|u\|_{H_*^2}: = \|u''\|_2, \qquad \forall u, v\in H^2_*(0, L).\end{align*}$

Recalling that the spaces $H^2_*$, $H^3_*$, $H^4_*$ in Section 1, we denote the duality pairings between $H^2_*$, $H^3_*$, $H^4_*$ and their dual spaces, respectively, by $\langle\cdot, \cdot\rangle_{H^2}$, $\langle\cdot, \cdot\rangle_{H^3}$ and $\langle\cdot, \cdot\rangle_{H^4}$. Given a bounded function $W = W(x)>0$, we define the weighted scalar products and the corresponding weighted norms on these spaces, respectively, by

$\begin{align*} (u, v)_{H^2_W}&: = (\sqrt{W}u'', \sqrt{W}v'')_2, \quad\|u\|_{H^2_W}: = \|\sqrt{W} u''\|_2, \qquad \forall u, v \in H^2_*(0, L);\\ (u, v)_{H^3_W}&: = (\sqrt{W}u''', \sqrt{W}v''')_2, \quad \|u\|_{H^3_W}: = \|\sqrt{W} u'''\|_2, \qquad \forall u, v \in H^3_*(0, L);\\ (u, v)_{H^4_W}&: = (\sqrt{W}u'''', \sqrt{W}v'''')_2, \quad \|u\|_{H^4_W}: = \|\sqrt{W} u''''\|_2, \qquad \forall u, v \in H^4_*(0, L). \end{align*}$

We remark that $W = \Phi, \Psi_y(x, t), K$ and $F(t)$ in the sequel.

Assume that $s = s(x)\in C^4(0, L)\cap C^3([0, L])$ and let $\|s'\|_{0} = \max_{x\in [0, L]}|s'|$. For any $T>0$, we define a bounded set $S$ by

$S: = \left\{y\in C^0([0, T];H_*^4(0, L)): \sup\limits_{t\in [0, T]}\|y\|_{H_\Phi^4}^2\leq \Lambda^2\right\},$

where $\Lambda$ satisfies

$0<\Lambda<\frac{M+6m}{M+6m\sqrt{1+\|s'\|_{0}^2}}\left[\frac{2H_0}{1+\|s'\|_{0}}+\frac{GK}{\ell^2}\right]\left[6H_0\|s'\|_{0}L^{5/2}+\frac{2AE}{L_c}L^{7/2}\right]^{-1}.$

(2.1)

For any $T>0$, let

$\overline{Y}: = C^0([0, T];H_*^4(0, L)) \cap C^1([0, T];H_*^2(0, L)), \\ \overline{\Theta}: = C^0([0, T];H_*^3(0, L))\cap C^1([0, T];H_*^2(0, L)),$

(2.2)

then $\overline{Y}\times \overline{\Theta}$ is a Banach space when it endowed with the norm

$\|(y, \theta)\|_{\overline{Y}\times \overline{\Theta}}: = \left(\sup\limits_{t\in[0, T]}\|y(t)\|_{H_\Phi^4}^2+\sup\limits_{t\in[0, T]}\|y_t(t)\|_{H_*^2}^2+\sup\limits_{t\in[0, T]}\|\theta(t)\|_{H_{\Psi_y(x, t)}^3}^2+\sup\limits_{t\in[0, T]}\|\theta_t(t)\|_{H_*^2}^2\right)^{1/2}.$

Assume that $V\subset L^2$ is a Hilbert space endowed with the scalar product $(\cdot, \cdot)_V$. We denote its dual space by $V'$ and the duality pairing by $\langle\cdot, \cdot\rangle_V$. Given a bilinear continuously symmetric form $a(\cdot, \cdot)$ on $V$, one can associate with $a(\cdot, \cdot)$ a linear operator $\mathcal{A}$ from $V$ into $V'$ defined by

$\langle \mathcal{A}u, v\rangle_V = a(u, v), \qquad \forall u, v\in V,$

which is self-adjoint. We denote by $\mathcal{A}^{-1}$ its inverse which is also self-adjoint.

Let $H$ be another Hilbert space satisfying $V\subset H$ and we restrict to the case where the injection of $V$ in $H$ is compact. In this case $\mathcal{A}^{-1}$ can be considered as a self-adjoint compact operator in $H$. Then we are able to use the elementary spectral theory of self-adjoint compact operators in Hilbert space (see for instance ^[7]) and we infer that there exists a complete orthonormal family $\{e_k\}_{k\in \mathbb{N}}$ of $H$ such that

$\mathcal{A}^{-1}e_k = \mu_k e_k, \quad e_k = \mu_k\mathcal{A}e_k \qquad \forall k\in\mathbb{N},$

where $\{\mu_k\}_{k\in \mathbb{N}}$ is decreasing and goes to 0. The family $\{e_k\}_{k\in \mathbb{N}}$ is also orthogonal for $a(\cdot, \cdot)$ in $V$.

Problem 1. Assume that $0 < K = K(x)$ is a bounded function. We consider the following linear problem in $H_*^4(0, L)$

$\begin{align*} \begin{cases} y = \lambda Ky_{xxxx}, \quad &x\in (0, L), \\ y(x) = y_{xx}(x) = 0, \quad &x\in \{0, L\}. \end{cases} \end{align*}$

Let $\mathcal{A} = K \frac{d^4}{dx^4}$. Then from the above argument there exist a basis $\{\epsilon_k\}_{k\in \mathbb{N}}$ of $H_*^4(0, L)$ (which ia also a basis of $H_*^2(0, L)$) and a positive sequence $\{\lambda_k\}$ such that

$(\epsilon_k, \epsilon_k)_{H_*^2} = \lambda_k (\epsilon_k, \epsilon_k)_{H_K^4}.$

Problem 2. Given $T>0$, for every $t\in [0, T]$, let $0 < F(t) = F(x, t)$ be a bounded function in $x$. For every $t\in [0, T]$, we consider the problem

$\begin{align*} \begin{cases} -\theta = \mu F(t)\theta_{xx}, \quad &x\in (0, L), \\ \theta(x) = 0, \quad &x\in \{0, L\}. \end{cases} \end{align*}$

Assume that $\mathcal{A} = F(t)\frac{d^2}{dx^2}$ for every $t\in [0, T]$. Then there exists a sequence of functions $\{\mu_i(t)\}$ and a complete orthonormal family $\{e_i\}_{i\in \mathbb{N}}$ of $H_*^2(0, L)$ and $H_0^1(0, L)$ such that

$(e_i, e_i)_{H_0^1} = \mu_i(t) (e_i, e_i)_{H_{F(t)}^2}.$

Problem 3. For every $t\in [0, T]$, we consider the linear problem in $H_*^3(0, L)$

$\begin{align*} \begin{cases} -\theta_{x} = \nu F(t)\theta_{xxx}, \quad &x\in (0, L), \\ \theta(x) = 0, \quad &x\in \{0, L\}. \end{cases} \end{align*}$

In fact, one can similarly obtain that a basis denoted by $\{u_k\}_{k\in \mathbb{N}}$ of the Hilbert spaces $H_*^3(0, L)$ and $H_*^2(0, L)$ satisfies

$(u_k, u_k)_{H_*^2} = \nu_i(t) (u_k, u_k)_{H_{F(t)}^3}.$

In the sequel, we will use the Gronwall-type inequality, which can be deduced from ^[4] or [5,Lemma A.5/p.157].

Proposition 1. Let $\psi\in C^1(\mathbb{R}_+)$ be such that $\psi(0) = 0$, $0\leq \psi'(t)\leq C_1+C_2\sqrt{\psi(t)}$ (with $C_1, C_2>0$) for all $t>0$. Then

$\psi(t)\leq \frac{(C_1+C_2)^2}{4}t^2+(C_1+C_2)t\qquad \forall t>0.$

Now we deal with several linear problems. For any $T>0$, assume that $g = g(x, t)\in C^0([0, T]; L^2(0, L))$ and $g_t\in C^0([0, T]; L^2(0, L))$, we consider

$\begin{align}\label{y} \begin{cases} y_{tt}+Ky_{xxxx} = g, \quad &x\in (0, L), ~t>0, \\ y(x, t) = y_{xx}(x, t) = 0, \quad &x\in \{0, L\}, ~t>0, \\ y(x, 0) = y^0, \quad y_t(x, 0) = y^1, \quad & x\in (0, L), \end{cases} \end{align}$

(2.3)

where $K>0$ is a bounded function as in Problem 1. Then we prove

Lemma 2.1. Assume that $y^0\in H_*^4(0, L)$ and $y^1\in H_*^2(0, L)$. Then for any $T>0$ the problem (2.3) admits a unique strong solution

$y\in \overline{Y}\cap C^2([0, T];L^2(0, L)),$

where $\overline{Y}$ is defined in (2.2).

Proof. Assume that $\{\epsilon_k\}$ is a basis of $H_*^4(0, L)$, and also is a basis of $H_*^2(0, L)$, see Problem 1. Let $\|\epsilon_k\|_{H_K^4} = 1$, then $\|\epsilon_k\|_{H_*^2} = \sqrt{\lambda_k} \downarrow 0$. For any $n\geq 1$, denote $\mathcal{E}_n: = {\rm{span}}\{\epsilon_1, \epsilon_2, ..., \epsilon_n\}$ and we put

$(y^0)_n: = \sum\limits_{k = 1}^n(y^0, \epsilon_k)_{H_K^4}\epsilon_k\to y^0\mbox{ in } H_*^4(0, L), \qquad (y^1)_n: = \sum\limits_{k = 1}^n(y^1, \epsilon_k)_{H_*^2}\epsilon_k\to y^1 \mbox{ in } H_*^2(0, L).$

(2.4)

Then we look for

$y_n = \sum\limits_{k = 1}^ny_n^k(t)\epsilon_k,$

which solves the following variational problem

$((y_n)_{tt}, \varphi)_{H_*^2}+(y_n, \varphi)_{H_K^4} = (g, \varphi_{xxxx})_2, \qquad \forall \varphi\in \mathcal{E}_n, t>0.$

(2.5)

Taking $\varphi = \epsilon_k$ ($k = 1, 2, ..., n$) in the equation (2.5), we obtain $n$ equations

$\lambda_k(y_n^k)_{tt}+y_n^k(t) = (g, (\epsilon_k)_{xxxx})_2.$

(2.6)

According to the theory of linear ODE's, one can find a unique solution $y_n^k(t)$ to (2.6) for all $t\in [0, T]$ and the initial conditions

$y_n^k(0) = (y^0, \epsilon_k)_{H_K^4}, \qquad (y_n^k)'(0) = (y^1, \epsilon_k)_{H^2}.$

Hence, there exists a unique solution $y_n(x, t)$ of (2.5).

For any fixed $T>0$, let $\varphi = (y_n)_{t}$ in the equation (2.5) and integrating on $(0, t)$ with $t < T$, then

$\begin{align*}\|(y_n)_t\|_{H_*^2}^2+\|y_n\|_{H_K^4}^2& = \|(y^1)_n\|_{H_*^2}^2+\|(y^0)_n\|_{H_K^4}^2+2\int_0^t{\int_0^L{(y_n)_{xxxxt}g}dx}ds\\ & = \|(y^1)_n\|_{H_*^2}^2+\|(y^0)_n\|_{H_K^4}^2+2((y_n)_{xxxx}(s), g(s))_2|_0^t-2\int_0^t{\int_0^L{(y_n)_{xxxx}g_t}dx}ds.\end{align*}$

Since $g\in C^0([0, T]; L^2(0, L))$ and $g_t\in C^0([0, T]; L^2(0, L))$, there exists a constant $\sigma\in (0, 1)$ such that (by the Hölder inequality and the Young inequality)

$2\left|((y_n)_{xxxx}(s), g(s))_2|_0^t-\int_0^t{\int_0^L{(y_n)_{xxxx}g_t}dx}ds\right|\leq C+ \sigma \|y_n\|_{H_K^4}^2+C_T\left(\int_0^t{\|y_n(s)\|_{H_K^4}^2}d s\right)^{1/2}.$

Hence,

$\|(y_n)_t\|_{H_*^2}^2+(1-\sigma)\|y_n\|_{H_K^4}^2\leq C+C_T\left(\int_0^t{\|y_n(s)\|_{H_K^4}^2}d s\right)^{1/2}.$

(2.7)

Then by Proposition 1, (2.7) can be written as

$\|(y_n)_t\|_{H_*^2}^2+(1-\sigma)\|y_n\|_{H_K^4}^2\leq C+C_T, \quad \forall t\in (0, T),$

(2.8)

where $C$ and $C_T$ are independent of $n$. Hence, the components $y_n^k$ do not depend on $n$, that is,

$y_n = y_n(x, t) = \sum\limits_{k = 1}^n y^k(t)\epsilon_k.$

Now we prove that $\{y_n\}$ admits a strongly convergent subsequence in a suitable sense. Let $m>n\geq 1$ and define

$y_{m, n}: = y_m(x, t)-y_n(x, t) = \sum\limits_{k = n+1}^m y^k(t)\epsilon_k .$

Hence,

$y_{m, n}(x, 0) = (y^0)_{m}-(y^0)_{n}, \quad (y_{m, n})_t(x, 0) = (y^1)_{m}-(y^1)_{n}.$

Subtracting the two equations (2.5) (with $n$ and $m$) and taking $\varphi = (y_{m, n})_{t}$, we obtain by using the orthogonality of the $\{\epsilon_k\}$ and by integrating over $(0, t)$ with $t < T$

$\|(y_{m, n})_{t}\|_{H_*^2}^2+\|y_{m, n}\|_{H_K^4}^2 = \|(y^1)_m-(y^1)_n\|_{H_*^2}^2+\|(y^0)_m-(y^0)_n\|_{H_K^4}^2\to 0, \quad \mbox{ by} (2.4).$

Therefore, $\{y_n\}$ is a Cauchy sequence in $\overline{Y}$. By completeness of these spaces we conclude that there exists $y\in \overline{Y}$ such that

$y_n\to y\quad \mbox{in } \overline{Y}\quad \mbox{ as }n\to \infty.$

From the equation in (2.3), we also have the additional regularity $y\in C^2([0, T]; L^2(0, L))$.

Finally, arguing by contradiction and assuming that there are two solutions, we subtract the two linear equations for the two solutions and we obtain a homogeneous linear problem; then one can easily show that the two solutions are identical.

For any $T>0$, let $f = f(x, t)\in C^0([0, T]; L^2(0, L))$ and $f_t\in C^0([0, T]; L^2(0, L))$. We consider another linear problem

$\begin{align}\label{theta} \begin{cases} \theta_{tt}-F(t)\theta_{xx} = f, \quad &x\in (0, L), ~t\in (0, T), \\ \theta(x, t) = 0, \quad &x\in \{0, L\}, ~t\in (0, T), \\ \theta(x, 0) = \theta^0, \quad \theta_t(x, 0) = \theta^1, \quad & x\in (0, L), \end{cases} \end{align}$

(2.9)

where $F(t) = F(x, t)>0$ satisfies $F(t)$ and $F_t(t)$ are bounded. Then we prove

Lemma 2.2. Assume that $\theta^0\in H_*^2(0, L)$ and $\theta^1\in H_0^1(0, L)$. Then for a small $T>0$ the problem (2.9) admits a unique solution

$\theta\in C^0([0, T];H_*^2(0, L))\cap C^1([0, T];H_0^1(0, L))\cap C^2([0, T];L^2(0, L)).$

Proof. Assume that $\{e_i\}$ is a basis of the spaces $H_*^2(0, L)$ and $H_0^1(0, L)$ with $\|e_i\|_{H_{F(t)}^2} = F_i(t)>0$ for every $t\in [0, T]$. Then $\|e_i\|_{H_0^1} = \sqrt{\mu_i(t)}F_i(t) = \alpha_i$ with $\alpha_i\downarrow 0$ as $i\to +\infty$.

For any $n\geq 1$, denote a subset $E_n: = {\rm{span}}\{e_1, e_2, ..., e_n\}$ and let

$(\theta^0)_n = \sum\limits_{i = 1}^{n}(\theta^0, e_i)_{H_{F(0)}^2}e_i\to \theta^0\quad \mbox{ in }H_*^2(0, L)\qquad (\theta^1)_n = \sum\limits_{i = 1}^{n}(\theta^0, e_i)_{H_0^1}e_i\to \theta^1\quad \mbox{ in }H_0^1(0, L).$

(2.10)

Then we look for $\theta_n$ in the form of

$\theta_n = \sum\limits_{i = 1}^n\theta_n^i(t)e_i,$

which solves the following approximating problem

$(\theta_n)_{tt}-F(t) (\theta_n)_{xx} = f, \qquad x\in (0, L), t>0.$

(2.11)

Testing the equation (2.11) with $\varphi = -(e_i)_{xx}$ ($i = 1, 2, ..., n$) and integrating over $(0, L)$, we obtain $n$ equations

$\label{thelin1}\alpha_i^2(\theta_n^i(t))_{tt}+F_i^2(t)\theta_n^i(t) = -(f, (e_i)_{xx})_2.$

(2.12)

According to the method of successive approximations (see Coddington-Levinson ^[6]), one can find a unique solution $\theta_n^i(t)$ to (2.12) in $[0, T]$ with $T>0$ small, and hence there exists a unique solution $\theta_n(x, t)$ of (2.11) with the initial conditions

$\theta_n^i(0) = (\theta^0, e_i)_{H_{F(0)}^2}, \qquad (\theta_n^i)'(0) = (\theta^1, e_i)_{H_0^1}.$

Then for this fixed $T>0$, testing the equation (2.11) with $\varphi = -(\theta_n)_{xxt}$ and integrating on $(0, L)\times(0, t)$ with $t < T$, we have

$\begin{align*}\|(\theta_n)_t\|_{H_0^1}^2+\|\theta_n\|_{H_{F(t)}^2}^2 = &\|(\theta^1)_{n}\|_{H_0^1}^2+\|(\theta^0)_{n}\|_{H_{F(0)}^2}^2-2(f(t), (\theta_n)_{xx}(t))_2+2(f(0), (\theta_n)_{xx}(0))_2\\ &+2\int_0^t{((\theta_n)_{xx}, f_t)_2}d\tau+\int_0^t{((\theta_n)_{xx}^2, F_t)_2}d\tau.\end{align*}$

Since $F, F_t$ are bounded and $f, f_t \in C^0([0, T]; L^2(0, L))$, by the Hölder inequality and the Young inequality, there exists $\delta\in (0, 1)$ such that

$\|(\theta_n)_t\|_{H_0^1}^2+\delta\|\theta_n\|_{H_{F(t)}^2}^2\leq C+C\int_0^t{\|\theta_n(\tau)\|_{H_{F(\tau)}^2}^2}d \tau\leq C+CT \sup\limits_{t\in [0, T]}\|\theta_n\|_{H_{F(t)}^2}^2.$

For a smaller $T>0$ if necessary, it follows that

$\sup\limits_{t\in [0, T]}\|(\theta_n)_t\|_{H_0^1}^2+\sup\limits_{t\in [0, T]}\|\theta_n\|_{H_{F(t)}^2}^2\leq C_T, \quad \forall t\in (0, T),$

where $C_T$ is independent of $n$ and we can write

$\theta_n = \sum\limits_{i = 1}^n\theta^i(t)e_i.$

Let $m>n\geq 1$ and define

$\theta_{m, n}: = \theta_m(x, t)-\theta_n(x, t) = \sum\limits_{i = n+1}^m \theta^i(t)e_i,$

and then

$\theta_{m, n}(x, 0) = (\theta^0)_{m}-(\theta^0)_{n}, \quad (\theta_{m, n})_t(x, 0) = (\theta^1)_{m}-(\theta^1)_{n}.$

Subtracting the two equations (2.11) (with $n$ and $m$) and testing the difference equation with $\varphi = -(\theta_{m, n})_{xxt}$, we obtain by integrating over $(0, L)\times(0, t)$ with $t < T$

$\begin{align*} \|(\theta_{m, n})_{t}\|_{H_0^1}^2+\|\theta_{m, n}\|_{H_{F(t)}^2}^2& = C_{m, n}+\int_0^t{(F_t, (\theta_{m, n})_{xx}^2)_2}ds\\ &\leq C_{m, n}+ c\int_0^t{ \|\theta_{m, n}(s)\|_{H_{F(s)}^2}^2}ds\leq C_{m, n}+ cT\sup\limits_{t\in [0, T]}\|\theta_{m, n}\|_{H_{F(t)}^2}^2, \end{align*}$

where $C_{m, n} = \|(\theta^1)_m-(\theta^1)_n\|_{H^1}^2+\|(\theta^0)_m-(\theta^0)_n\|_{H_{F(0)}^2}^2$. Hence, for a smaller $T>0$ if necessary, we have

$\sup\limits_{t\in [0, T]}\|(\theta_{m, n})_{t}\|_{H_0^1}^2+\sup\limits_{t\in [0, T]}\|\theta_{m, n}\|_{H_{F(t)}^2}^2\leq (1-cT) C_{m, n}\to 0, \quad \mbox{ by} (2.10).$

Therefore, $\{\theta_n\}$ is a Cauchy sequence in $C^0([0, T]; H_*^2(0, L)) \cap C^1([0, T]; H_0^1(0, L))$. By completeness of these spaces we conclude that there exists $\theta\in C^0([0, T]; H_*^2(0, L)) \cap C^1([0, T]; H_0^1(0, L))$ such that

$\theta_n\to \theta\quad \mbox{in } C^0([0, T];H_*^2(0, L)) \cap C^1([0, T];H_0^1(0, L))\quad \mbox{ as }n\to \infty.$

From the equation in (2.9), one can prove that $\theta_{tt}\in C^0([0, T]; L^2(0, L))$ and hence $\theta\in C^2([0, T]; L^2(0, L))$. By contradiction one can get the uniqueness result and we finish the proof.

Furthermore, if $F(x, t)\in C^2([0, L])\times C^1([0, T])$, $f\in C^0([0, T]; H^2(0, L))$, $f_t\in C^0([0, T]; L^2(0, L))$, then we have

Lemma 2.3. Assume that $\theta^0\in H_*^3(0, L)$ and $\theta^1\in H_*^2(0, L)$. Then for a small $T>0$ the unique solution $\theta$ of the problem (2.9) satisfies

$\theta\in \overline{\Theta}\cap C^2([0, T];H_0^1(0, L)),$

where $\overline{\Theta}$ is defined in (2.2).

Proof. Assume that $\{u_k\}$ is the basis of $H_*^2(0, L)$ and $H_*^3(0, L)$. Then the solutions $\theta_n$ of the problem (2.11) can be written in the form of

$\theta_n = \sum\limits_{k = 1}^n\vartheta_n^k(t)u_k.$

Let

$(\theta^0)_n: = \sum\limits_{k = 1}^n(\theta^0, u_k)_{H_{F(0)}^3}u_k, \qquad (\theta^1)_n: = \sum\limits_{k = 1}^n(\theta^1, u_k)_{H_*^2}u_k,$

so that

$(\theta^0)_n\to \theta^0\mbox{ in }H_*^3(0, L), \qquad (\theta^1)_n\to \theta^1\mbox{ in }H_*^2(0, L)\quad \mbox{ as }n\to \infty.$

(2.13)

Differentiating (2.11) with respect to $x$, we have

$(\theta_n)_{xtt}-F(t) (\theta_n)_{xxx} = F_x(t) (\theta_n)_{xx}+f_x, \qquad x\in (0, L), t>0.$

(2.14)

For the fixed $T>0$ in Lemma 2.2, testing (2.14) with $-(\theta_n)_{xxxt}$ and integrating over $(0, L)\times(0, t)$ with $t < T$, we are led to

$\begin{align*}\|(\theta_n)_{t}\|_{H_*^2}^2+\|\theta_n\|_{H_{F(t)}^3}^2 = &\|(\theta^1)_n\|_{H_*^2}^2+\|(\theta^0)_n\|_{H_{F(0)}^3}^2-2\int_0^t{(F_x(s)(\theta_n)_{xx}, (\theta_n)_{xxxt})_2}ds\\ &+\int_0^t{(F_t, (\theta_n)_{xxx}^2)_2}ds-2\int_0^t{(f_x, (\theta_n)_{xxxt})_2}ds.\end{align*}$

Now we estimate the three nonlocal terms. Since $F(x, t)\in C^2([0, L])\times C^1([0, T])$, we have

$\int_0^t{(F_t, (\theta_n)_{xxx}^2)_2}ds\leq C\int_0^t{\|\theta_n(s)\|_{H_{F(s)}^3}^2}ds$

and

$\begin{align*} \int_0^t{(F_x(s)(\theta_n)_{xx}, (\theta_n)_{xxxt})_2}ds& = -\int_0^t{(F_{xx}(s)(\theta_n)_{xx}, (\theta_n)_{xxt})_2}ds-\int_0^t{(F_x(s)(\theta_n)_{xxx}, (\theta_n)_{xxt})_2}ds\\ &\leq C\int_0^t{\|\theta_n(s)\|_{H_*^2}\|(\theta_n)_{t}(s)\|_{H_*^2}}ds+C\int_0^t{\|\theta_n(s)\|_{H_{F(s)}^3}\|(\theta_n)_{t}(s)\|_{H_*^2}}ds\\ &\leq C\int_0^t{\|\theta_n(s)\|_{H_{F(s)}^3}^2}ds+C\int_0^t{\|(\theta_n)_{t}(s)\|_{H_*^2}^2}ds. \end{align*}$

The condition $f\in C^0([0, T]; H^2(0, L))$ allows us to estimate

$\begin{align*} \int_0^t{(f_x, (\theta_n)_{xxxt})_2}ds = -\int_0^t{(f_{xx}, (\theta_n)_{xxt})_2}ds&\leq C\left(\int_0^t{\|f_{xx}(s)\|_2^2}ds\right)^{1/2}\left(\int_0^t{\|(\theta_n)_{t}(s)\|_{H_*^2}^2}ds\right)^{1/2}\\ &\leq C+\int_0^t{\|(\theta_n)_{t}(s)\|_{H_*^2}^2}ds. \end{align*}$

Hence, we have

$\|(\theta_n)_{t}\|_{H_*^2}^2+\|\theta_n\|_{H_{F(t)}^3}^2\leq C+C\int_0^t{\|\theta_n(s)\|_{H_{F(s)}^3}^2}ds+C\int_0^t{\|(\theta_n)_{t}(s)\|_{H_*^2}^2}ds.$

For a smaller $T>0$ if necessary, one gets

$\sup\limits_{t\in [0, T]}\|(\theta_n)_{t}\|_{H_*^2}^2+\sup\limits_{t\in [0, T]}\|\theta_n\|_{H_{F(t)}^3}^2\leq C, \quad C\mbox{ is independent of } n.$

Then there exists a unique $\theta\in \overline{\Theta}$ (by following the procedure in the proof of Lemma 2.2) such that

$\theta_n\to \theta\quad \mbox{in } \overline{\Theta}\quad \mbox{ as }n\to \infty.$

From the equation in (2.9), one can prove that $\theta_{tt}\in C^0([0, T]; H_0^1(0, L))$ and then $\theta\in C^2([0, T]; H_0^1(0, L))$. The proof is finished.

With the results above and according to Ball ^[3], where the author considered some nonlinear (nonlocal) hyperbolic problems, we are able to prove

Theorem 2.4. Assume that $s\in C^4(0, L)\cap C^3([0, L])$ and $q(x, t)\in S$. Let $y^0\in H_*^4$, $\theta^0\in H_*^3$ and $y^1, \theta^1\in H_*^2$. If $T>0$ is small enough, then for any $(z, \eta)\in \overline{Y}\times \overline{\Theta}$ there exists a unique solution $(y, \theta)\in \overline{Y}\times \overline{\Theta}$ of the problem

$\begin{align}\label{linp1} \begin{cases} y_{tt}+\Phi y_{xxxx} = h_1(z, \eta), \quad &x\in(0, L), t>0, \\ \theta_{tt}-\Psi_{q}(x, t)\theta_{xx} = h_2(z, \eta), \quad &x\in(0, L), t>0, \\ y(0, t) = y(L, t) = y_{xx}(0, t) = y_{xx}(L, t) = \theta(0, t) = \theta(L, t) = 0, \quad &t>0, \\ y(x, 0) = y^0, \quad y_t(x, 0) = y^1, \quad \theta(x, 0) = \theta^0, \quad \theta_t(x, 0) = \theta^1, \quad & x\in (0, L). \end{cases} \end{align}$

(2.15)

Proof. The condition $q(x, t)\in S$ leads to the function $\Psi_{q}(x, t)>0$ and

$\Psi_{q}(x, t)\in C^2([0, L])\times C^0([0, T]).$

Moreover, $(z, \eta)\in \overline{Y}\times \overline{\Theta}$, then we have

$h_1(z, \eta)\in C^0([0, T]; L^2(0, L)), \quad h_2(z, \eta)\in C^0([0, T];H^2(0, L))$

and

$(h_1(z, \eta))_t, (h_2(z, \eta))_t\in C^0([0, T]; L^2(0, L)).$

Hence, the statements of Theorem 2.4 follows by Lemmas 2.1, 2.2 and 2.3.

---

3. Proof of Theorem 1.1

This section is devoted to the proof of Theorem 1.1. First we define a subset of $\overline{Y}\times \overline{\Theta}$ (see (2.2)) by

$Q: = \{(y, \theta)\in \overline{Y}\times \overline{\Theta}: \|(y, \theta)\|_{\overline{Y}\times \overline{\Theta}}^2\leq \gamma \Lambda^2 \mbox{ and (1.3) holds}\},$

where $\Lambda$ satisfies (2.1) and $\gamma\in (0, 1)$. For any $(z, \eta)\in Q\subset \overline{Y}\times \overline{\Theta}$, the unique solution $(y, \theta)$ of (2.15) allows us to define a map $\Gamma: Q\to \overline{Y}\times \overline{\Theta}$ by

$(y, \theta) = \Gamma(z, \eta), \qquad (z, \eta)\in Q.$

Let $y^0\in H_*^4$, $\theta^0\in H_*^3$, $y^1, \theta^1\in H_*^2$ and $h(0) = h_1(y(x, 0), \theta(x, 0))\in L^2(0, L)$ satisfy

$\|y^0\|_{H_\Phi^4}^2+\|y^1\|_{H_*^2}^2+\|\theta^0\|_{H_{\Psi_{q}(x, 0)}^3}^2+\|\theta^1\|_{H_*^2}^2+2\|h(0)\|_{2}\|y^0\|_{H_\Phi^4}+16\|h(0)\|_{2}^2\leq \rho\Lambda^2,$

(3.1)

where $0 < \rho < \gamma/T_0$ with $T_0>2$. Then we prove

Lemma 3.1. Assume that (3.1) holds. Then if $T>0$ is sufficiently small, $\Gamma(Q)\subset Q$.

Proof. Given any $(z, \eta)\in Q$, the solution $(y, \theta)$ satisfies

$\begin{align*}\|y\|_{H_\Phi^4}^2+\|y_t\|_{H_*^2}^2+&\|\theta\|_{H_{\Psi_{q}(x, t)}^3}^2+\|\theta_t\|_{H_*^2}^2 = \|y^0\|_{H_\Phi^4}^2+\|y^1\|_{H_*^2}^2+\|\theta^0\|_{H_{\Psi_{q}(x, 0)}^3}^2+\|\theta^1\|_{H_*^2}^2\\ &+\int_0^t{({(\Psi_{q}(x, t)})_t, \theta_{xxx}^2)_2}ds-2\int_0^t{((\Psi_{q}(x, t))_x\theta_{xx}, \theta_{xxxt})_2}ds\\ &-2\int_0^t{((h_2(z, \eta))_x, \theta_{xxxt})_2}ds +2\int_0^t{\int_0^L{(y)_{xxxxt}h_1(z, \eta)}dx}ds.\end{align*}$

Now we estimate the nonlocal terms. Since $\Psi_{q}(x, t)\in C^2([0, L])\times C^1([0, T])$, we have

$\begin{align*} \int_0^t{({(\Psi_{q}(x, t)})_t, \theta_{xxx}^2)_2}ds&\leq c\int_0^t{\|\theta\|_{H_{\Psi_{q}(x, s)}^3}^2}ds\\ &\leq cT\sup\limits_{t\in[0, T]}\|\theta(t)\|_{H_{\Psi_{q}(x, t)}^3}^2\leq c_1T \|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}^2 \end{align*}$

and

$\begin{align*} 2\int_0^t{((\Psi_{q}(x, t))_x\theta_{xx}, \theta_{xxxt})_2}ds = &-2\int_0^t{((\Psi_{q}(x, t))_{xx}\theta_{xx}, \theta_{xxt})_2}ds\\&-2\int_0^t{((\Psi_{q}(x, t))_x\theta_{xxx}, \theta_{xxt})_2}ds\\ \leq& cT(\sup\limits_{t\in[0, T]}\|\theta(t)\|_{H_{\Psi_{q}(x, t)}^3}^2+\sup\limits_{t\in[0, T]}\|\theta_t(t)\|_{H_*^2}^2)\\ \leq& c_2T \|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}^2. \end{align*}$

The condition $h_2(z, \eta)\in C^0([0, T]; H^2(0, L))$ allows us to obtain by the Young inequality

$\begin{align*} 2\int_0^t{((h_2(z, \eta))_x, \theta_{xxxt})_2}ds& = -2\int_0^t{((h_2(z, \eta))_{xx}, \theta_{xxt})_2}ds\\ &\leq CT+\frac{1}{4}\sup\limits_{t\in[0, T]}\|\theta_t(t)\|_{H_*^2}^2 \leq CT+\frac{1}{4} \|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}^2; \end{align*}$

Since $(z, \eta)\in Q$ implies that $h_1(z, \eta)\in C^1([0, T])$ for every $x\in (0, L)$, it follows that

$|h_1(z(x, t), \eta(x, t))| = |h(t)|\leq |h(0)|+C_hT, \quad \mbox{ for every }t\in [0, T].$

Hence,

$\begin{align*} 2&\int_0^t{\int_0^L{(y)_{xxxxt}h_1(z, \eta)}dx}ds\\ & = 2(y_{xxxx}(t), h_1(z, \eta))_2-2(y_{xxxx}(0), h(0))_2-2\int_0^t{\int_0^L{(h_1(z, \eta))_t y_{xxxx}}dx}ds\\ &\leq 2\|h_1(z, \eta)\|_{2} \|y(t)\|_{H_\Phi^4}+2\|h(0)\|_{2}\|y^0\|_{H_\Phi^4}+ CT+\frac{1}{8}\sup\limits_{t\in[0, T]}\|y(t)\|_{H_\Phi^4}^2\\ &\leq \frac{1}{4}\sup\limits_{t\in[0, T]}\|y(t)\|_{H_\Phi^4}^2+16(\|h(0)\|_{2}^2+(C_hT)^2L)+CT+2\|h(0)\|_{2}\|y^0\|_{H_\Phi^4}\\ &\leq\frac{1}{4}\|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}^2+ 16\|h(0)\|_{2}^2+CT^2+CT+2\|h(0)\|_{2}\|y^0\|_{H_\Phi^4}. \end{align*}$

Then we deduce that

$\left(\frac{1}{2}-(c_1+c_2)T\right)\|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}^2\leq \rho \Lambda^2+CT^2+CT.$

Let $T\leq \frac{T_0-2}{2(c_1+c_2)T_0}$ with $T_0>2$, then

$\|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}^2\leq \rho T_0 \Lambda^2+T_0(CT^2+CT).$

If $T>0$ is smaller enough, then

$\|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}^2\leq \gamma\Lambda^2,$

which proves that $\Gamma(Q)\subset Q$.

Next, we show that the map $\Gamma$ is contractive for sufficiently small $T$.

Lemma 3.2. Assume that (3.1) holds. If $T>0$ is sufficiently small, then $\Gamma$ is a contractive map.

Proof. Let $(z^a, \eta^a)$, $(z^b, \eta^b)$ be two different elements in $Q$ and let $(y^a, \theta^a) = \Gamma(z^a, \eta^a)$, $(y^b, \theta^b) = \Gamma(z^b, \eta^b)$ and we denote

$z = z^a-z^b, \quad \eta = \eta^a-\eta^b, \quad y = y^a-y^b, \quad \theta = \theta^a-\theta^b,$

which yields that $z(x, 0) = \eta(x, 0) = y(x, 0) = \theta(x, 0) = 0$, and hence,

$h_1(z^a(x, 0), \eta^a(x, 0))-h_1(z^b(x, 0), \eta^b(x, 0)) = 0.$

(3.2)

Differentiating the second equation in (2.15) with respect to $x$ both side and then subtracting the two problems (2.15) for $(y^a, \theta^a)$ and $(y^b, \theta^b)$, we get a system

$\begin{align}\label{linp2} \begin{cases} y_{tt}+\Phi y_{xxxx} = h_1(z^a, \eta^a)-h_1(z^b, \eta^b), \quad &x\in(0, L), t>0, \\ \theta_{xtt}-\Psi_{q}(x, t)\theta_{xxx} = (\Psi_{q}(x, t))_x\theta_{xx}+(h_2(z^a, \eta^a))_x-(h_2(z^b, \eta^b))_x, \quad &x\in(0, L), t>0, \\ y(0, t) = y(L, t) = y_{xx}(0, t) = y_{xx}(L, t) = \theta(0, t) = \theta(L, t) = 0, \quad &t>0, \\ y(x, 0) = 0, \quad y_t(x, 0) = 0, \quad \theta(x, 0) = 0, \quad \theta_t(x, 0) = 0, \quad & x\in (0, L). \end{cases} \end{align}$

(3.3)

Multiplying (3.3) by $(y_{xxxxt}, -\theta_{xxxt})$ and integrating over $(0, L)\times (0, t)$ with $t < T$, one has

$\begin{align*} \|y\|_{H_\Phi^4}^2+\|y_t\|_{H_*^2}^2+\|\theta\|_{H_{\Psi_{q}(x, t)}^3}^2+\|\theta_t\|_{H_*^2}^2 = &2\int_0^t{\int_0^L{(h_1(z^a, \eta^a)-h_1(z^b, \eta^b))y_{xxxxt}}dx}ds\\ &+\int_0^t{\int_0^L{[(\Psi_{q}(x, t))_t\theta_{xxx}^2-2(\Psi_{q}(x, t))_x\theta_{xx}\theta_{xxxt}]}dx}ds\\ &-2\int_0^t{\int_0^L{[h_2(z^a, \eta^a)-h_2(z^b, \eta^b)]_x\theta_{xxxt}}dx}ds\\ : = &I+II-III. \end{align*}$

Since $h_1(z, \eta)\in C^1([0, T]; L^2(0, L))$ and $h_1(z(\cdot, t), \eta(\cdot, t))\in C^1([0, T])$, we have

$\|h_1(z^a, \eta^a)-h_1(z^b, \eta^b)\|_2\leq C T\sup\limits_{t\in [0, T]}\|[h_1(z^a, \eta^a)-h_1(z^b, \eta^b)]_t\|_2 \leq CT\|(z(t), \eta(t))\|_{\overline{Y}\times \overline{\Theta}}.$

Hence, from (3.2) we obtain

$\begin{align*} I = &2(y_{xxxx}(t), [h_1(z^a, \eta^a)-h_1(z^b, \eta^b)]|_0^t)_2-2\int_0^t{\int_0^L{(h_1(z^a, \eta^a)-h_1(z^b, \eta^b))_ty_{xxxx}}dx}ds\\ \leq & C T\sup\limits_{t\in [0, T]}\|[h_1(z^a, \eta^a)-h_1(z^b, \eta^b)]_t\|_2\|(y(t)\|_{H_\Phi^4}+CT \|(y(t)\|_{H_\Phi^4}\|(z(t), \eta(t))\|_{Y\times\Theta}\\ \leq & CT \|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}\|(z(t), \eta(t))\|_{\overline{Y}\times \overline{\Theta}}. \end{align*}$

Since $\Psi_{q}(x, t)\in C^2([0, L])\times C^1([0, T])$, we have

$\begin{align*} II = &\int_0^t{\int_0^L{(\Psi_{q}(x, t))_t\theta_{xxx}^2}dx}ds-2\int_0^t{\int_0^L{(\Psi_{q}(x, t))_{xx}\theta_{xx}\theta_{xxt}}dx}ds\\ &-2\int_0^t{\int_0^L{(\Psi_{q}(x, t))_{x}\theta_{xxx}\theta_{xxt}}dx}ds\\ \leq &cT(\sup\limits_{t\in[0, T]}\|\theta(t)\|_{H_{\Psi_{q}(x, t)}^3}^2+\sup\limits_{t\in[0, T]}\|\theta_t(t)\|_{H_*^2}^2)\leq cT \|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}^2. \end{align*}$

Since $h_2(z, \eta)\in C^0([0, T]; H^2(0, L)), (h_2(z, \eta))_t\in C^0([0, T]\times L^2(0, L))$, it yields that

$III = -2\int_0^t{\int_0^L{(h_2(z^a, \eta^a)-h_2(z^b, \eta^b))_{xx}\theta_{xxt}}dx}ds\leq CT \|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}\|(z(t), \eta(t))\|_{\overline{Y}\times \overline{\Theta}}.$

Therefore,

$\left(1-cT\right)\|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}^2\leq CT \|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}\|(z(t), \eta(t))\|_{\overline{Y}\times \overline{\Theta}}.$

Then if $T>0$ is small enough, we have

$\|(y(t), \theta(t))\|_{\overline{Y}\times \overline{\Theta}}< \alpha \|(z(t), \eta(t))\|_{\overline{Y}\times \overline{\Theta}}, \mbox{ with }0<\alpha<1$

and we finish the proof.

Then by the Contraction Mapping principle, there exists a unique local solution to (2.15) with $h_1(y, \theta)$ and $h_2(y, \theta)$ in place of $h_1(z, \eta)$ and $h_2(z, \eta)$.

Now we prove the existence result of (1.4). For any given $q_0\in S$, there exists a unique solution $(y_1, \theta_1)\subset Q$ of the problem (1.4) with $\Psi_y(x, t) = \Psi_{q_0}(x, t)$. Moreover, $y_1\in S$. Let $\Psi_y(x, t) = \Psi_{y_1}(x, t)$ in (1.4), then the problem (1.4) with $\Psi_y(x, t) = \Psi_{y_{1}}(x, t)$ has a unique solution which is denoted by $(y_2, \theta_2)$. Repeating this process, we obtain that the problem (1.4) with $\Psi_y(x, t) = \Psi_{y_{n-1}}(x, t)$ has a unique solution $(y_n, \theta_n)$ ($n\geq 1$).

Since $(y_n, \theta_n)\in Q$, we have that $(y_n, \theta_n)$ is bounded in $C^2([0, T]; L^2(0, L))\times C^2([0, T]; H_0^1(0, L))$ from the system (1.4). Hence, there exists $(y, \theta)\in Q\cap (C^2([0, T]; L^2(0, L))\times C^2([0, T]; H_0^1(0, L)))$ such that for every $t\in [0, T]$

$(y_n, \theta_n)\rightharpoonup (y, \theta), \quad \mbox{ weakly in }H_*^4(0, L)\times H_*^3(0, L),$

$((y_n)_{tt}, (\theta_n)_{tt})\rightharpoonup (y_{tt}, \theta_{tt}), \quad \mbox{ weakly in } L^2(0, L)\times H_0^1(0, L),$

which implies that for every $t\in [0, T]$

$(y_n)_{xxxx}\rightharpoonup y_{xxxx}, \quad \mbox{ weakly in } L^2(0, L)$

and for every $t\in [0, T]$

$h_1(y_n, \theta_n)\to h_1(y, \theta), \quad h_2(y_n, \theta_n)\to h_2(y, \theta), \quad \Psi_{y_n}(x, t)\to\Psi_{y}(x, t), \quad \mbox{ strongly in } L^2(0, L).$

Moreover, for every $t\in [0, T]$, we have $(\theta_n)_{xx}\to\theta_{xx}$ strongly in $L^2(0, L)$, then it yields that

$\begin{align*}\|\Psi_{y_{n-1}}(x, t)(\theta_n)_{xx}-\Psi_y(x, t) \theta_{xx}\|_2& = \|(\Psi_{y_{n-1}}(x, t)-\Psi_y(x, t))(\theta_n)_{xx}\|_2+\|\Psi_y(x, t)((\theta_n)_{xx}-\theta_{xx})\|_2\\ &\leq C\|\Psi_{y_{n-1}}(x, t)-\Psi_y(x, t)\|_2+C\|(\theta_n)_{xx}-\theta_{xx}\|_2\to 0. \end{align*}$

Then $(y, \theta)\in \overline{Y}\times \overline{\Theta}$ satisfies the two equations of (1.4) in $L^2(0, L)$ for every $t\in [0, T]$ and we claim that $(y, \theta)$ is a solution of the problem (1.4) and we finish the proof.

---

Acknowledgments

The author is grateful to his colleagues for several remarks which lead to an improvement of the present paper. This work is supported by Gaocengci Rencai Zizhu Xiangmu of Hebei Province: CL201701 and partially supported by National Natural Science Foundation of China: 11701162.

---

Conflict of interest

The author declares no conflict of interest.

---