On the fractional model of Fokker-Planck equations with two different operator

1.   Introduction

The studies on the fractional calculus during the last few decades have gained importance in many areas ^[1,2,3,4]. Besides, fractional derivatives are important for the definition of recollection and hereditary features of different necessities and behaviour. This is the advantage of fractional differential equations in reappear well-known integer order problems.

Recently, some scientists have been interested in improving new definition of fractional derivative. These derivative definitions change from Riemann-Liouville derivative to the Caputo-Fabrizio derivative introduced by Caputo and Fabrizio ^{[5,6,7,8,9,10,11,12,13]}. They are claimed that the new derivative has interesting properties than the former derivatives. Their derivative does not run into a any singularity, thus a new fractional order derivative without a singular kernel can efficiently describe the effect of memory and also able to portray material heterogeneities and structures in different cases, which are physically symbolized by distinction or variation of the average.

In this paper, we apply the LHAM to find analytical approximated solution for fractional Fokker-Planck equations using in case of every two fractional operators. LHAM is a combining of the homotopy analysis method projected by Liao and the Laplace transform ^[14,15]. Some writers have projected various systems for fractional partial differential equations with every two fractional operators. In ^[16], Dehghan practised the HAM to solve fractional partial differential equations with in case of Liouville-Caputo. In ^[17], is studied a fractional differential equation with a changeble coefficient. Jafari in ^[18] applied the HAM in order to solve the high orderly fractional differential equation analized by Diethelmand Ford ^[19]. In ^[20], is produced a mathematical analysis of an example studied the Caputo-Fabrizio fractional derivative, where analytical and calculation advances are finded. Morales-Delgado et al. ^[21] presented LHAM to supply a new solutions in case of every two fractional operators.

The aim in this work is to establish approximate solutions of the fractional model of Fokker-Planck equations (FPEs) with space-time fractional derivatives as follows ^[22]:

with the initial state $v(x, 0) = h(x)$.

$\Phi (x, t, v)$ and $\Omega (x, t, v)$ are drift and diffusion coefficients, $\alpha$ and $\beta$ are parameters that definite the order of time-space fractional derivatives, severally. For $\alpha = 1$, $\beta = 1$, Eq. (1.1) is a classical FPE. These equations are used in the pattern of divergent diffusion techniques. In ^[22], q-homotopy analysis transform method is used to obtain analytical solutions for Eqs. (1.1), stochastic expression and computer model of fractional FPE representing divergent diffusion is analysed in ^[23] and in ^[24,25] approximated solutions are obtained by using Monte Carlo technique, exact solutions for fractional FPE has been determined by using various methods, for example Laplace transform method ^[26], Homotopy perturbation method (HPM) ^[27], Homotopy perturbation transform method (HPTM) ^[28], Adomian decomposition method (ADM) ^[29], Finite element method ^[30] and Residual power series method ^[31] and more ^[32,33,34]. But fractional Fokker-Planck equation has not been analysed via LHAM.

In the section 2 of this article, some basic definitions related to in case of every two fractional operators. In section 3, LHAM is applied to obtain the solution of the fractional FPEs and some tables and graphical outcomes are contained to show the reliability and efficiency of the technique. Finally, in section 4, consequences are introduced.

2.   Materials and method

We first represent the main definitions and several properties of the fractional calculus theory ^[2] in this part.

Definition 2.1. The Riemann-Liouville fractional integral operator of order $\alpha (\alpha \ge 0)$ is defined as

Definition 2.2. The Caputo fractional derivatives of order $\alpha$ is defined as

where ${D^m}$ is the classical differential operator of order $m$.

For the Caputo derivative we have

The efficacy of this definition is confined to functions $v$ such that ${v^{(m)}}$$\in {L_1}(a, b).$

If ${v^{(m)}} \in {L_1}({{\rm{R}}^ + })$ and if ${v^{(m)}}(t)$ is of exponential order ${v_m}$, with ${v_m} > 0$, $\forall m = 0, 1, 2, ..., n - 1$, the form advised in the sources ^[11] as follows,

for $Re(z) > l,$$l = \max\{ {v_m}:m = 0, 1, 2, ..., n - 1\}.$

Then,

Therefore, in Eq. (2.2) if transformations happen as follows;

${(t - z)^{m - \alpha - 1}}$$\to \exp(- \alpha (t - z)/(1 - \alpha))$ and $\frac{1}{{\Gamma (m - \alpha)}}$$\to$$\frac{{(2 - \alpha)M(\alpha)}}{{2(1 - \alpha)}},$ the new definition of fractional operator is expressed by Caputo and Fabrizio ^[5,8];

Definition 2.3. Let $v \in {H^1}(a, b)$, the new fractional Caputo derivative is defined as;

$M(\alpha)$ is a standardization function that $M(0) = M(1) = 1$ ^[5]. Then equation (2.5) does not have singularities at $t = z.$

But, if $v \notin {H^1}(a, b)$, equation (2.5) can be rewrited as;

Definition 2.4. The fractional integral of order of v is defined by

where $0 < \alpha < 1$.

Remark ^[6]. According to the definition 2.4, the fractional integral of Caputo type of function of order $0 < \alpha < 1$ is an medial between function $v$ and its integral of order one.

Thus,

The above formulation gives an expressed for

Therefore, in ^[6] is rewrote the recent fractional Caputo derivative as follow:

Theorem 1. (see ^[5,6] for proof) If the function v(z) as

in the new fractional Caputo derivative, we write

Definition 2.5. After above definition (2.3), if $\alpha \in (0, 1]$ and, we can define the Laplace transform in case of C-F ^[5,8]:

From equation (2.9),

3.   Laplace homotopy analysis method for fractional differential equation

where $(x, t) \in [0, 1] \times [0, \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\smile}$}}{T}],$$n - 1 < \alpha \leqslant n,$

and

then,

where L[$v(x, t)](z) = \Psi (x, z),$ therefore

Then, we can write,

where $\Psi (x, z) = L[v(x, t)],$$\tilde \sigma (x, z) = L[\sigma (x, t)],$ and

We can obtain the solution of equation (3.6) as follows,

substituting (3.7) into (3.6), we obtain

from the coefficients of powers of $p$,

and when $p \to 1$, equation (3.9) gives the approximate solution of (3.5) and (3.6), and as follows,

if we apply the inverse of the Laplace transform of (3.10), we achieve the approximate solution of equation (3.11),

For the operator of Caputo-Fabrizio

where $(x, t) \in [0, 1] \times [0, \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\smile}$}}{T}],$$m - 1 < \alpha + n \leqslant m,$ the initial positions are

and

in case of the Caputo-Fabrizio fractional derivative, we can write

where $L[v(x, t)](z) = \Psi (x, z),$ then equation (3.14),

Then, we can construct the following homotopy for the above equation:

where $\Psi (x, z) = L[v(x, t)],$ $\tilde \sigma (x, z) = L[\sigma (x, t)],$ and

Then,

Eq. (3.18) is the solution of eq. (3.17).

Substituting eq.(3.18) into (3.17), we obtain,

from the coefficients of powers of $p$,

and when $z \to 1$, equation (3.19) yields the approximate solution of (3.16) and (3.17),

if we apply the inverse of the Laplace transform for (3.20), we find solution of Eq. (3.12),

Let us define

where, ${S_n}(x, t)$ is the $n$ th partial sum of the infinite series of approximate solution ^[29], then the relative error $RE(\%)$ is calculated as $RE(\%) = \left| {\frac{{{S_n}(x, t) - {v_{exact}}(x, t)}}{{{v_{exact}}(x, t)}}} \right| \times 100.$

4.   Examples

In this part, some examples are solved using every two fractional operators in order to demonstrate the effectiveness of the LHAM, in addition the convergence and stability of the method are discussed.

Case 1.

We think that the linear time-fractional FPE in the Liouville-Caputo sense as follows:

By the initial condition

The exact solution for (4.1) for $\alpha = 1$ is $v(x, t) = x{e^t}$ ^[22].

Now, using the LHAM, we have

the approximate solution is

we have

and, when $n \to \infty$,

For $\alpha = 1$,

This solution is the same as exact solution for (4.1) equation.

Case 2.

We think that the linear time-fractional FPE in case of the Caputo-Fabrizio as follows:

by the initial condition

The exact solution for (4.7) for $\alpha = 1$ is $v(x, t) = x{e^t}$ ^[22].

Now, using the LHAM, we have

the approximate solution is

we have

and, when $n \to \infty$,

For $\alpha = 1,$

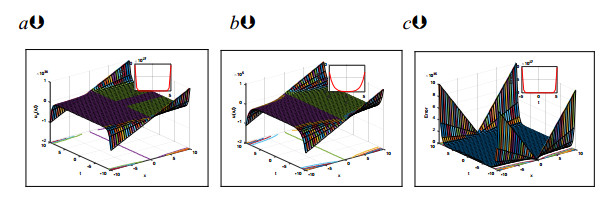
In Figures 1 and 2, we drawn 3D and 2D graphics of exact solution, absolute error and approximate solution for $\alpha = 1.$ ${v_{LHAM}}(x, t)$ approximate solution obtained in ten iterations. We consider that better results can be achieved if the number of iterations is increased.

In Table 1, we organize table of series solutions ${v_k}(x, t)$ for $k = 10.$ Comparison among numerical solutions with admitted consequences is made.These results found by using Laplace homotopy analysis method, residual power series method ^[31], q-homotopy analysis transform method ^[22] and homotopy perturbation transform method ^[28].

In Table 2, we made comparison among exact and series solutions ${v_k}(x, t)$ for $k = 10.$ We obtained approximate solution ${v_{LHAM}}$ in case of the Caputo-Fabrizio.

5.   Conclusion

In this study the LHAM has utilized in order to find approximate analytical solution of time-fractional Fokker-Planck equation in case of the Liouville-Caputo and the Caputo-Fabrizio. We have compared the approximate solutions received in the sight of LHAM with those outcomes received from the exact analytical solutions. This operation indicates an accurate understanding between the LHAM and exact outcomes. It is clear that the LHAM gives correct and convergent series solutions applying only a few iterates in every two fractional derivative. Because the Laplace transform permits one in many positions to get over the deficiency chiefly produced by unsatisfied boundary or initial conditions, the LHAM is a strong method that requires inferior calculation time and this method is much useful than the HPM.

Acknowledgments

We would like to Express our thanks to the an onymous referees who help us improved this paper.

Conflict of interest

The authors declare no conflict of interest in this paper.