The mitotic checkpoint complex (MCC): looking back and forth after 15 years

Song-Tao Liu; Hang Zhang; Song-Tao Liu; Hang Zhang

doi:10.3934/molsci.2016.4.597

AIMS Molecular Science

2016, Volume 3, Issue 4: 597-634. doi: 10.3934/molsci.2016.4.597

Previous Article Next Article

Review Topical Sections

The mitotic checkpoint complex (MCC): looking back and forth after 15 years

Song-Tao Liu ^,,
Hang Zhang

Department of Biological Sciences, University of Toledo, 2801 West Bancroft St., Toledo, OH 43606, USA

Received: 06 September 2016 Accepted: 20 October 2016 Published: 24 October 2016

The mitotic checkpoint is a specialized signal transduction pathway that contributes to the fidelity of chromosome segregation. The signaling of the checkpoint originates from defective kinetochore-microtubule interactions and leads to formation of the mitotic checkpoint complex (MCC), a highly potent inhibitor of the Anaphase Promoting Complex/Cyclosome (APC/C)—the E3 ubiquitin ligase essential for anaphase onset. Many important questions concerning the MCC and its interaction with APC/C have been intensively investigated and debated in the past 15 years, such as the exact composition of the MCC, how it is assembled during a cell cycle, how it inhibits APC/C, and how the MCC is disassembled to allow APC/C activation. These efforts have culminated in recently reported structure models for human MCC:APC/C supra-complexes at near-atomic resolution that shed light on multiple aspects of the mitotic checkpoint mechanisms. However, confusing statements regarding the MCC are still scattered in the literature, making it difficult for students and scientists alike to obtain a clear picture of MCC composition, structure, function and dynamics. This review will comb through some of the most popular concepts or misconceptions about the MCC, discuss our current understandings, present a synthesized model on regulation of CDC20 ubiquitination, and suggest a few future endeavors and cautions for next phase of MCC research.
- mitotic checkpoint; mitotic checkpoint complex; anaphase promoting complex/cyclosome; ubiquitin ligase; BUBR1; MAD2; CDC20; chromosome segregation
Citation: Song-Tao Liu, Hang Zhang. The mitotic checkpoint complex (MCC): looking back and forth after 15 years[J]. AIMS Molecular Science, 2016, 3(4): 597-634. doi: 10.3934/molsci.2016.4.597

Related Papers:

Abstract

The mitotic checkpoint is a specialized signal transduction pathway that contributes to the fidelity of chromosome segregation. The signaling of the checkpoint originates from defective kinetochore-microtubule interactions and leads to formation of the mitotic checkpoint complex (MCC), a highly potent inhibitor of the Anaphase Promoting Complex/Cyclosome (APC/C)—the E3 ubiquitin ligase essential for anaphase onset. Many important questions concerning the MCC and its interaction with APC/C have been intensively investigated and debated in the past 15 years, such as the exact composition of the MCC, how it is assembled during a cell cycle, how it inhibits APC/C, and how the MCC is disassembled to allow APC/C activation. These efforts have culminated in recently reported structure models for human MCC:APC/C supra-complexes at near-atomic resolution that shed light on multiple aspects of the mitotic checkpoint mechanisms. However, confusing statements regarding the MCC are still scattered in the literature, making it difficult for students and scientists alike to obtain a clear picture of MCC composition, structure, function and dynamics. This review will comb through some of the most popular concepts or misconceptions about the MCC, discuss our current understandings, present a synthesized model on regulation of CDC20 ubiquitination, and suggest a few future endeavors and cautions for next phase of MCC research.

References

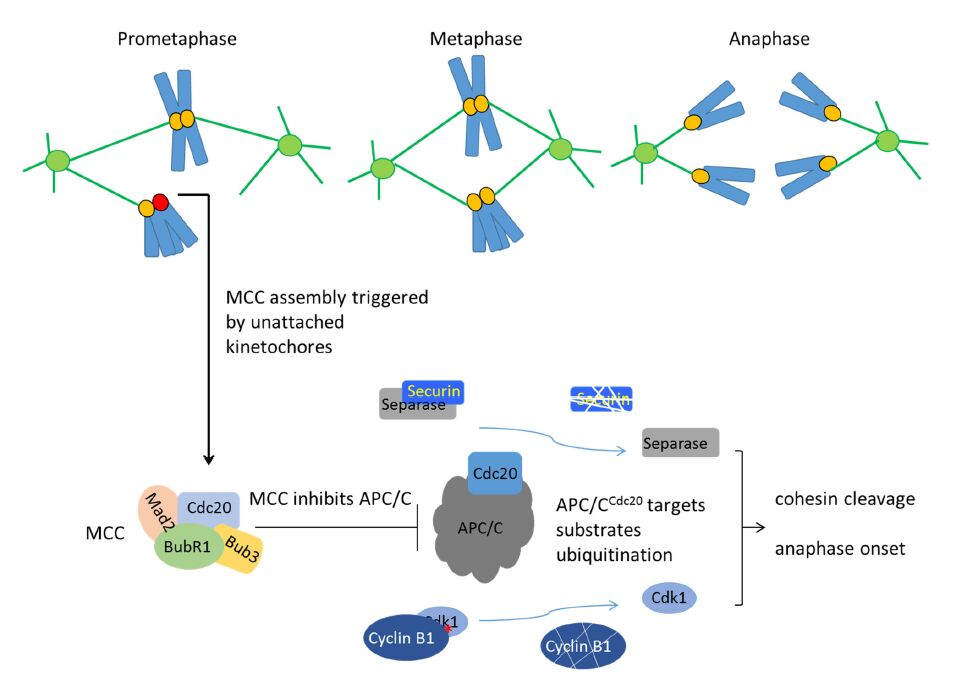
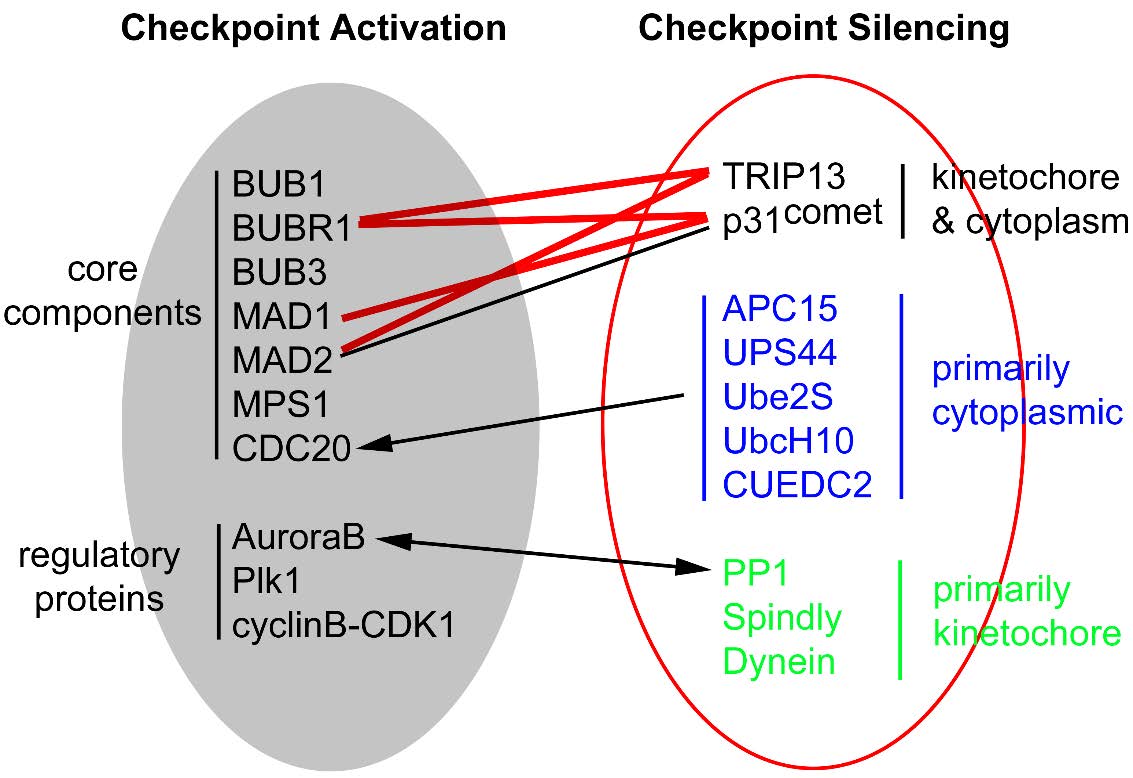
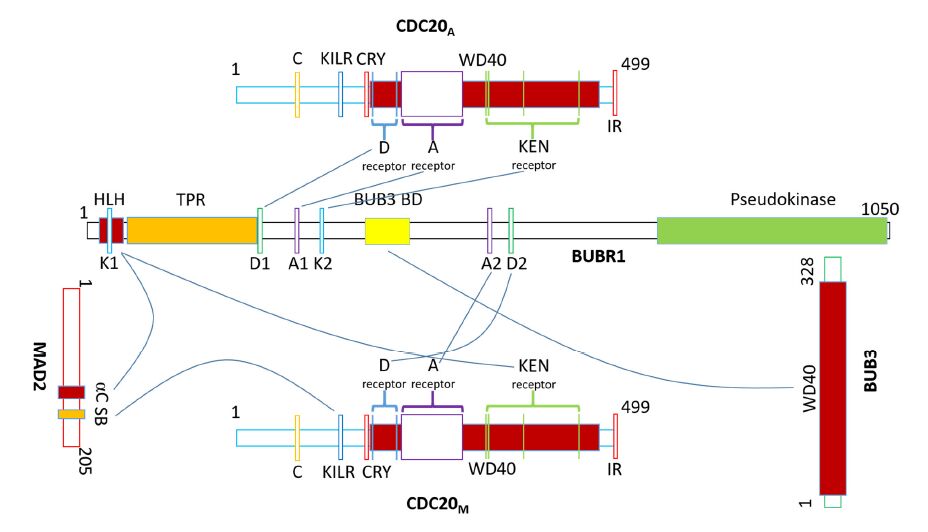
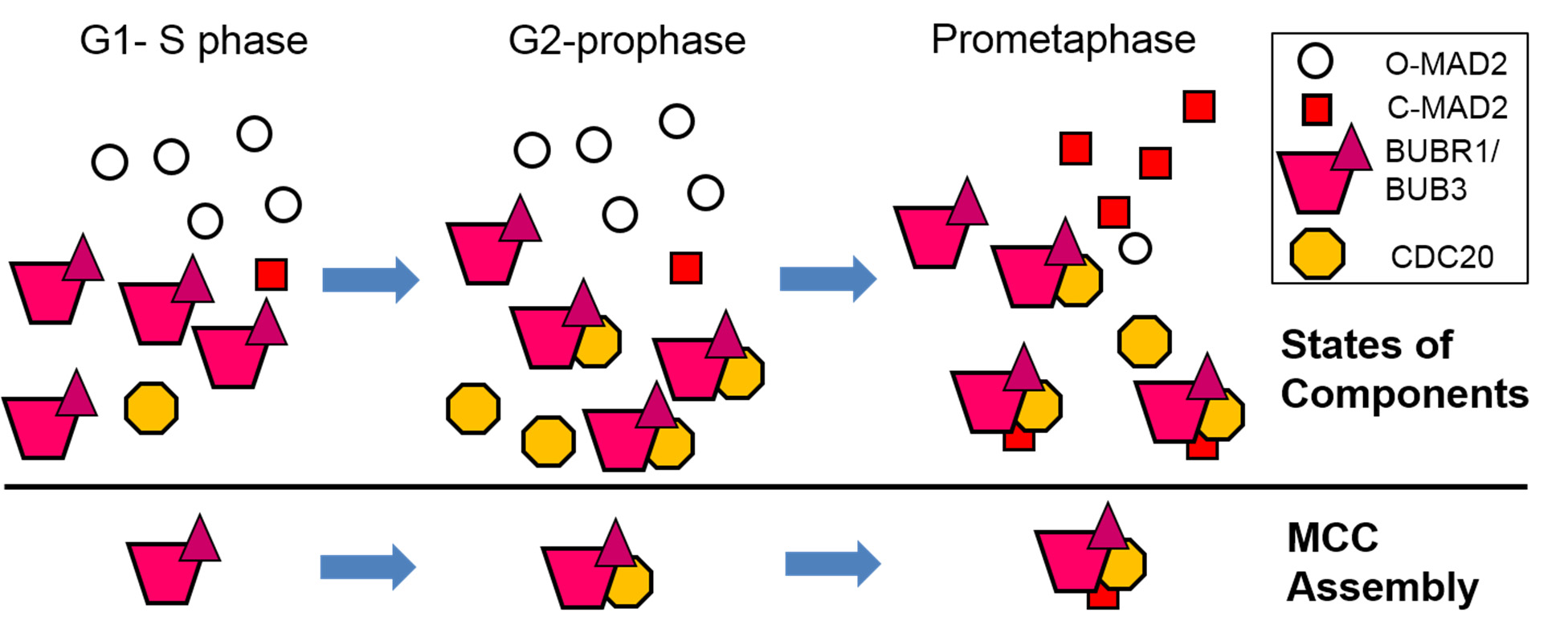
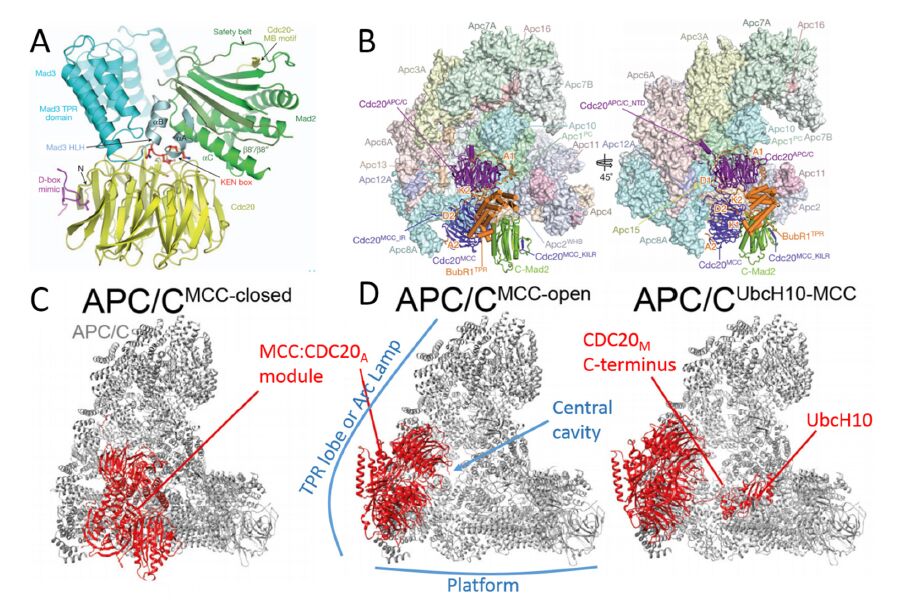
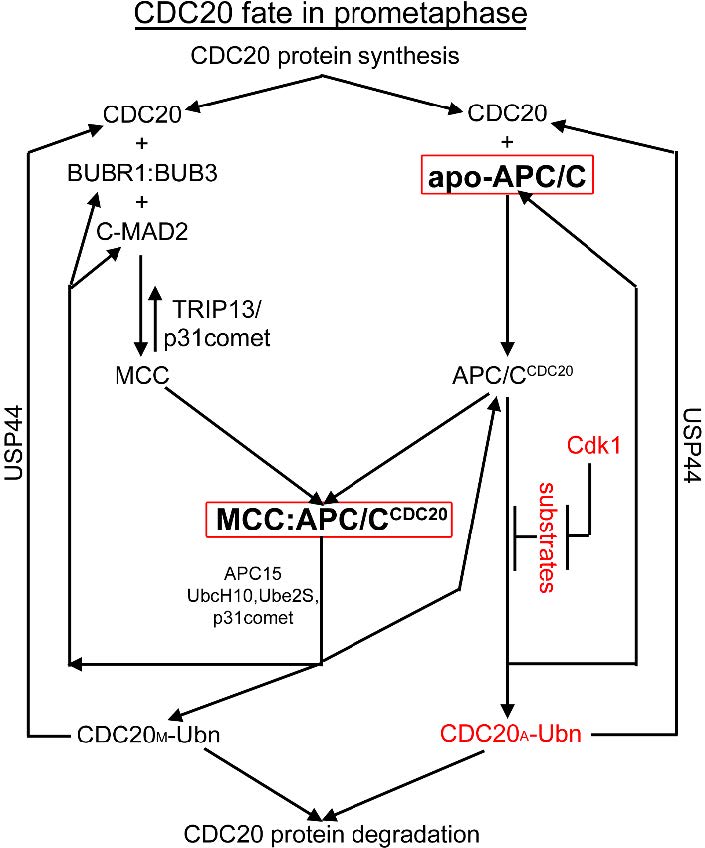
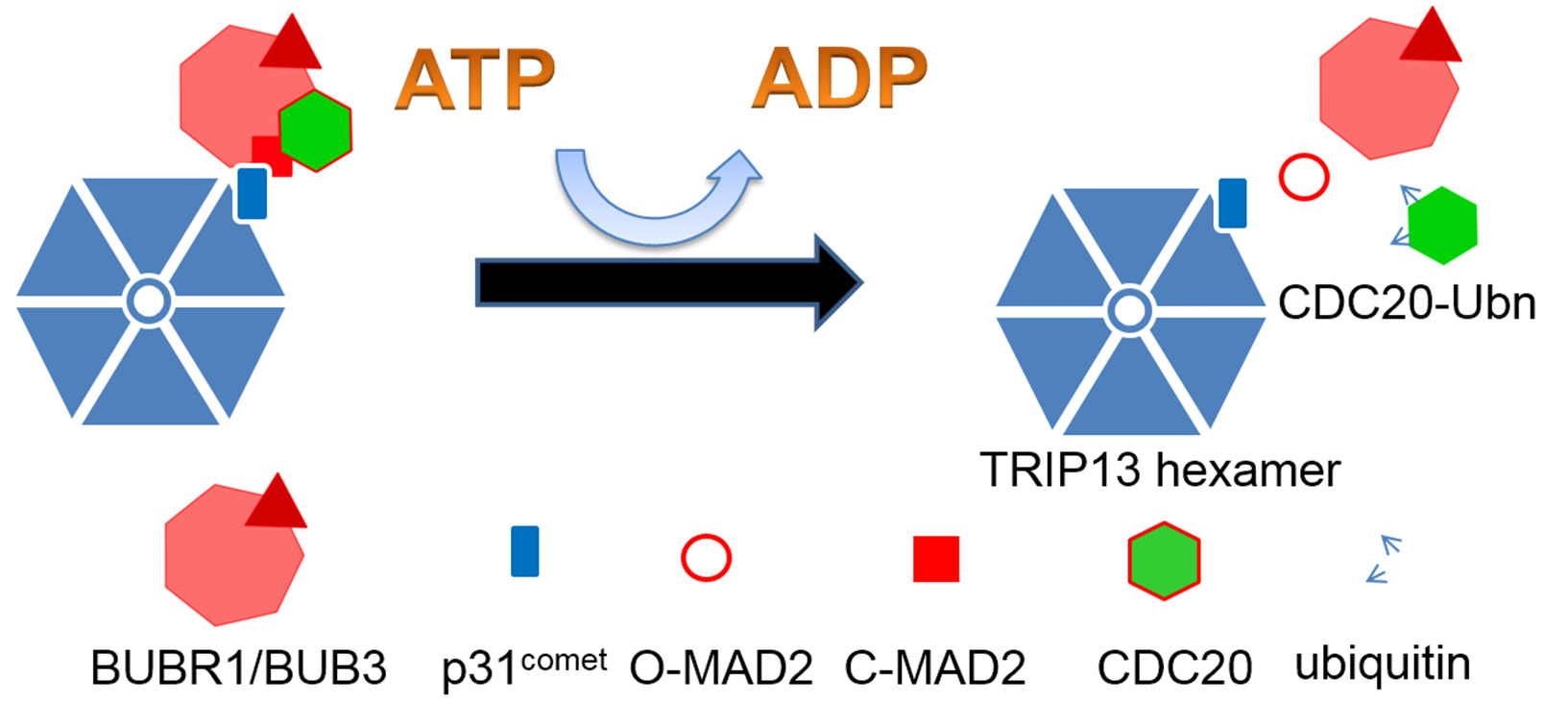
[1]	Hartwell LH, Weinert TA (1989) Checkpoints: controls that ensure the order of cell cycle events. Science 246: 629-634. doi: 10.1126/science.2683079
[2]	Li R, Murray AW (1991) Feedback control of mitosis in budding yeast. Cell 66: 519-531. doi: 10.1016/0092-8674(81)90015-5
[3]	Hoyt MA, Totis L, Roberts BT (1991) S. cerevisiae genes required for cell cycle arrest in response to loss of microtubule function. Cell 66: 507-517.
[4]	Sudakin V, Ganoth D, Dahan A, et al. (1995) The cyclosome, a large complex containing cyclin-selective ubiquitin ligase activity, targets cyclins for destruction at the end of mitosis. Mol Biol Cell 6: 185-197. doi: 10.1091/mbc.6.2.185
[5]	King RW, Peters JM, Tugendreich S, et al. (1995) A 20S complex containing CDC27 and CDC16 catalyzes the mitosis-specific conjugation of ubiquitin to cyclin B. Cell 81: 279-288. doi: 10.1016/0092-8674(95)90338-0
[6]	Irniger S, Piatti S, Michaelis C, et al. (1995) Genes involved in sister chromatid separation are needed for B-type cyclin proteolysis in budding yeast. Cell 81: 269-278. doi: 10.1016/0092-8674(95)90337-2
[7]	Tugendreich S, Tomkiel J, Earnshaw W, et al. (1995) CDC27Hs colocalizes with CDC16Hs to the centrosome and mitotic spindle and is essential for the metaphase to anaphase transition. Cell 81: 261-268. doi: 10.1016/0092-8674(95)90336-4
[8]	Cohen-Fix O, Peters JM, Kirschner MW, et al. (1996) Anaphase initiation in Saccharomyces cerevisiae is controlled by the APC-dependent degradation of the anaphase inhibitor Pds1p. Genes Dev 10: 3081-3093. doi: 10.1101/gad.10.24.3081
[9]	Funabiki H, Kumada K, Yanagida M (1996) Fission yeast Cut1 and Cut2 are essential for sister chromatid separation, concentrate along the metaphase spindle and form large complexes. EMBO J 15: 6617-6628.
[10]	Hwang LH, Lau LF, Smith DL, et al. (1998) Budding yeast Cdc20: a target of the spindle checkpoint. Science 279: 1041-1044. doi: 10.1126/science.279.5353.1041
[11]	He X, Patterson TE, Sazer S (1997) The Schizosaccharomyces pombe spindle checkpoint protein mad2p blocks anaphase and genetically interacts with the anaphase-promoting complex. Proc Natl Acad Sci U S A 94: 7965-7970. doi: 10.1073/pnas.94.15.7965
[12]	Li Y, Gorbea C, Mahaffey D, et al. (1997) MAD2 associates with the cyclosome/anaphase-promoting complex and inhibits its activity. Proc Natl Acad Sci U S A 94: 12431-12436. doi: 10.1073/pnas.94.23.12431
[13]	Fang G, Yu H, Kirschner MW (1998) The checkpoint protein MAD2 and the mitotic regulator CDC20 form a ternary complex with the anaphase-promoting complex to control anaphase initiation. Genes Dev 12: 1871-1883. doi: 10.1101/gad.12.12.1871
[14]	Sudakin V, Chan GK, Yen TJ (2001) Checkpoint inhibition of the APC/C in HeLa cells is mediated by a complex of BUBR1, BUB3, CDC20, and MAD2. J Cell Biol 154: 925-936. doi: 10.1083/jcb.200102093
[15]	Hardwick KG, Johnston RC, Smith DL, et al. (2000) MAD3 encodes a novel component of the spindle checkpoint which interacts with Bub3p, Cdc20p, and Mad2p. J Cell Biol 148: 871-882. doi: 10.1083/jcb.148.5.871
[16]	Millband DN, Hardwick KG (2002) Fission yeast Mad3p is required for Mad2p to inhibit the anaphase-promoting complex and localizes to kinetochores in a Bub1p-, Bub3p-, and Mph1p-dependent manner. Mol Cell Biol 22: 2728-2742. doi: 10.1128/MCB.22.8.2728-2742.2002
[17]	Fraschini R, Beretta A, Sironi L, et al. (2001) Bub3 interaction with Mad2, Mad3 and Cdc20 is mediated by WD40 repeats and does not require intact kinetochores. Embo J 20: 6648-6659. doi: 10.1093/emboj/20.23.6648
[18]	Chen RH (2002) BubR1 is essential for kinetochore localization of other spindle checkpoint proteins and its phosphorylation requires Mad1. J Cell Biol 158: 487-496. doi: 10.1083/jcb.200204048
[19]	Chao WC, Kulkarni K, Zhang Z, et al. (2012) Structure of the mitotic checkpoint complex. Nature 484: 208-213. doi: 10.1038/nature10896
[20]	Alfieri C, Chang L, Zhang Z, et al. (2016) Molecular basis of APC/C regulation by the spindle assembly checkpoint. Nature 536: 431-436. doi: 10.1038/nature19083
[21]	Yamaguchi M, VanderLinden R, Weissmann F, et al. (2016) Cryo-EM of Mitotic Checkpoint Complex-Bound APC/C Reveals Reciprocal and Conformational Regulation of Ubiquitin Ligation. Mol Cell 63: 593-607. doi: 10.1016/j.molcel.2016.07.003
[22]	Sczaniecka M, Feoktistova A, May KM, et al. (2008) The spindle checkpoint functions of Mad3 and Mad2 depend on a Mad3 KEN box-mediated interaction with Cdc20-anaphase-promoting complex (APC/C). J Biol Chem 283: 23039-23047. doi: 10.1074/jbc.M803594200
[23]	Musacchio A (2015) The Molecular Biology of Spindle Assembly Checkpoint Signaling Dynamics. Curr Biol 25: R1002-1018. doi: 10.1016/j.cub.2015.08.051
[24]	Jia L, Kim S, Yu H (2013) Tracking spindle checkpoint signals from kinetochores to APC/C. Trends Biochem Sci 38 302-311.
[25]	London N, Biggins S (2014) Signalling dynamics in the spindle checkpoint response. Nat Rev Mol Cell Biol 15: 736-747. doi: 10.1038/nrm3888
[26]	Foley EA, Kapoor TM (2013) Microtubule attachment and spindle assembly checkpoint signalling at the kinetochore. Nat Rev Mol Cell Biol 14: 25-37.
[27]	Lara-Gonzalez P, Westhorpe FG, Taylor SS (2012) The spindle assembly checkpoint. Curr Biol 22: R966-980. doi: 10.1016/j.cub.2012.10.006
[28]	Stukenberg PT, Burke DJ (2008) The role of the kinetochore in spindle checkpoint signaling. In: De Wulf P, Earnshaw, W.C., eds., editor. The Kinetochore: from Molecular Discoveries to Cancer Therapy. New York: Springer.
[29]	Rieder CL, Cole RW, Khodjakov A, et al. (1995) The checkpoint delaying anaphase in response to chromosome monoorientation is mediated by an inhibitory signal produced by unattached kinetochores. J Cell Biol 130: 941-948. doi: 10.1083/jcb.130.4.941
[30]	Li X, Nicklas RB (1995) Mitotic forces control a cell-cycle checkpoint. Nature 373: 630-632. doi: 10.1038/373630a0
[31]	Weiss E, Winey M (1996) The Saccharomyces cerevisiae spindle pole body duplication gene MPS1 is part of a mitotic checkpoint. J Cell Biol 132: 111-123. doi: 10.1083/jcb.132.1.111
[32]	Suijkerbuijk SJ, van Dam TJ, Karagoz GE, et al. (2012) The vertebrate mitotic checkpoint protein BUBR1 is an unusual pseudokinase. Dev Cell 22: 1321-1329. doi: 10.1016/j.devcel.2012.03.009
[33]	Vleugel M, Hoogendoorn E, Snel B, et al. (2012) Evolution and function of the mitotic checkpoint. Dev Cell 23: 239-250. doi: 10.1016/j.devcel.2012.06.013
[34]	Guo Y, Kim C, Ahmad S, et al. (2012) CENP-E--dependent BubR1 autophosphorylation enhances chromosome alignment and the mitotic checkpoint. J Cell Biol 198: 205-217. doi: 10.1083/jcb.201202152
[35]	Musacchio A, Salmon ED (2007) The spindle-assembly checkpoint in space and time. Nat Rev Mol Cell Biol 8: 379-393.
[36]	Zachos G, Black EJ, Walker M, et al. (2007) Chk1 is required for spindle checkpoint function. Dev Cell 12: 247-260. doi: 10.1016/j.devcel.2007.01.003
[37]	Chan GK, Jablonski SA, Starr DA, et al. (2000) Human Zw10 and ROD are mitotic checkpoint proteins that bind to kinetochores. Nat Cell Biol 2: 944-947. doi: 10.1038/35046598
[38]	Yao X, Abrieu A, Zheng Y, et al. (2000) CENP-E forms a link between attachment of spindle microtubules to kinetochores and the mitotic checkpoint. Nat Cell Biol 2: 484-491. doi: 10.1038/35019518
[39]	Williams BC, Li Z, Liu S, et al. (2003) Zwilch, a New Component of the ZW10/ROD Complex Required for Kinetochore Functions. Mol Biol Cell 14: 1379-1391. doi: 10.1091/mbc.E02-09-0624
[40]	Montembault E, Dutertre S, Prigent C, et al. (2007) PRP4 is a spindle assembly checkpoint protein required for MPS1, MAD1, and MAD2 localization to the kinetochores. J Cell Biol 179: 601-609. doi: 10.1083/jcb.200703133
[41]	Santaguida S, Vernieri C, Villa F, et al. (2011) Evidence that Aurora B is implicated in spindle checkpoint signalling independently of error correction. Embo J 30: 1508-1519. doi: 10.1038/emboj.2011.70
[42]	Xia G, Luo X, Habu T, et al. (2004) Conformation-specific binding of p31(comet) antagonizes the function of Mad2 in the spindle checkpoint. Embo J 23: 3133-3143. doi: 10.1038/sj.emboj.7600322
[43]	Habu T, Kim SH, Weinstein J, et al. (2002) Identification of a MAD2-binding protein, CMT2, and its role in mitosis. Embo J 21: 6419-6428. doi: 10.1093/emboj/cdf659
[44]	Tipton AR, Wang K, Oladimeji P, et al. (2012) Identification of novel mitosis regulators through data mining with human centromere/kinetochore proteins as group queries. BMC Cell Biol 13: 15. doi: 10.1186/1471-2121-13-15
[45]	Wang K, Sturt-Gillespie B, Hittle JC, et al. (2014) Thyroid hormone receptor interacting protein 13 (TRIP13) AAA-ATPase is a novel mitotic checkpoint-silencing protein. J Biol Chem 289: 23928-23937. doi: 10.1074/jbc.M114.585315
[46]	Eytan E, Wang K, Miniowitz-Shemtov S, et al. (2014) Disassembly of mitotic checkpoint complexes by the joint action of the AAA-ATPase TRIP13 and p31(comet). Proc Natl Acad Sci U S A 111: 12019-12024. doi: 10.1073/pnas.1412901111
[47]	Gao YF, Li T, Chang Y, et al. (2011) Cdk1-phosphorylated CUEDC2 promotes spindle checkpoint inactivation and chromosomal instability. Nat Cell Biol 13: 924-933. doi: 10.1038/ncb2287
[48]	Mansfeld J, Collin P, Collins MO, et al. (2011) APC15 drives the turnover of MCC-CDC20 to make the spindle assembly checkpoint responsive to kinetochore attachment. Nat Cell Biol 13: 1234-1243. doi: 10.1038/ncb2347
[49]	Foster SA, Morgan DO (2012) The APC/C subunit Mnd2/Apc15 promotes Cdc20 autoubiquitination and spindle assembly checkpoint inactivation. Mol Cell 47: 921-932. doi: 10.1016/j.molcel.2012.07.031
[50]	Uzunova K, Dye BT, Schutz H, et al. (2012) APC15 mediates CDC20 autoubiquitylation by APC/C(MCC) and disassembly of the mitotic checkpoint complex. Nat Struct Mol Biol 19: 1116-1123. doi: 10.1038/nsmb.2412
[51]	Reddy SK, Rape M, Margansky WA, et al. (2007) Ubiquitination by the anaphase-promoting complex drives spindle checkpoint inactivation. Nature 446: 921-925. doi: 10.1038/nature05734
[52]	Stegmeier F, Rape M, Draviam VM, et al. (2007) Anaphase initiation is regulated by antagonistic ubiquitination and deubiquitination activities. Nature 446: 876-881. doi: 10.1038/nature05694
[53]	Garnett MJ, Mansfeld J, Godwin C, et al. (2009) UBE2S elongates ubiquitin chains on APC/C substrates to promote mitotic exit. Nat Cell Biol 11: 1363-1369. doi: 10.1038/ncb1983
[54]	Griffis ER, Stuurman N, Vale RD (2007) Spindly, a novel protein essential for silencing the spindle assembly checkpoint, recruits dynein to the kinetochore. J Cell Biol 177: 1005-1015. doi: 10.1083/jcb.200702062
[55]	Liu D, Vleugel M, Backer CB, et al. (2010) Regulated targeting of protein phosphatase 1 to the outer kinetochore by KNL1 opposes Aurora B kinase. J Cell Biol 188: 809-820. doi: 10.1083/jcb.201001006
[56]	Rosenberg JS, Cross FR, Funabiki H (2011) KNL1/Spc105 recruits PP1 to silence the spindle assembly checkpoint. Curr Biol 21: 942-947. doi: 10.1016/j.cub.2011.04.011
[57]	Howell BJ, McEwen BF, Canman JC, et al. (2001) Cytoplasmic dynein/dynactin drives kinetochore protein transport to the spindle poles and has a role in mitotic spindle checkpoint inactivation. J Cell Biol 155: 1159-1172. doi: 10.1083/jcb.200105093
[58]	Foley EA, Maldonado M, Kapoor TM (2011) Formation of stable attachments between kinetochores and microtubules depends on the B56-PP2A phosphatase. Nat Cell Biol 13: 1265-1271. doi: 10.1038/ncb2327
[59]	Suijkerbuijk SJ, Vleugel M, Teixeira A, et al. (2012) Integration of kinase and phosphatase activities by BUBR1 ensures formation of stable kinetochore-microtubule attachments. Dev Cell 23: 745-755. doi: 10.1016/j.devcel.2012.09.005
[60]	Pinsky BA, Biggins S (2005) The spindle checkpoint: tension versus attachment. Trends Cell Biol 15: 486-493. doi: 10.1016/j.tcb.2005.07.005
[61]	King JM, Nicklas RB (2000) Tension on chromosomes increases the number of kinetochore microtubules but only within limits. J Cell Sci 113 Pt 21: 3815-3823.
[62]	Wilson L, Jordan MA (1995) Microtubule dynamics: taking aim at a moving target. Chem Biol 2: 569-573. doi: 10.1016/1074-5521(95)90119-1
[63]	Wilson L, Panda D, Jordan MA (1999) Modulation of microtubule dynamics by drugs: a paradigm for the actions of cellular regulators. Cell Struct Funct 24: 329-335. doi: 10.1247/csf.24.329
[64]	Skoufias DA, Andreassen PR, Lacroix FB, et al. (2001) Mammalian mad2 and bub1/bubR1 recognize distinct spindle-attachment and kinetochore-tension checkpoints. Proc Natl Acad Sci U S A 98: 4492-4497. doi: 10.1073/pnas.081076898
[65]	Kapoor TM, Mayer TU, Coughlin ML, et al. (2000) Probing spindle assembly mechanisms with monastrol, a small molecule inhibitor of the mitotic kinesin, Eg5. J Cell Biol 150: 975-988. doi: 10.1083/jcb.150.5.975
[66]	Collin P, Nashchekina O, Walker R, et al. (2013) The spindle assembly checkpoint works like a rheostat rather than a toggle switch. Nat Cell Biol 15: 1378-1385. doi: 10.1038/ncb2855
[67]	Dick AE, Gerlich DW (2013) Kinetic framework of spindle assembly checkpoint signalling. Nat Cell Biol 15: 1370-1377. doi: 10.1038/ncb2842
[68]	Chen RH, Waters JC, Salmon ED, et al. (1996) Association of spindle assembly checkpoint component XMAD2 with unattached kinetochores. Science 274: 242-246. doi: 10.1126/science.274.5285.242
[69]	Li Y, Benezra R (1996) Identification of a human mitotic checkpoint gene: hsMAD2. Science 274: 246-248. doi: 10.1126/science.274.5285.246
[70]	Mao Y, Abrieu A, Cleveland DW (2003) Activating and silencing the mitotic checkpoint through CENP-E-dependent activation/inactivation of BubR1. Cell 114: 87-98. doi: 10.1016/S0092-8674(03)00475-6
[71]	Ji Z, Gao H, Yu H (2015) CELL DIVISION CYCLE. Kinetochore attachment sensed by competitive Mps1 and microtubule binding to Ndc80C. Science 348: 1260-1264.
[72]	Hiruma Y, Sacristan C, Pachis ST, et al. (2015) CELL DIVISION CYCLE. Competition between MPS1 and microtubules at kinetochores regulates spindle checkpoint signaling. Science 348: 1264-1267.
[73]	Aravamudhan P, Goldfarb AA, Joglekar AP (2015) The kinetochore encodes a mechanical switch to disrupt spindle assembly checkpoint signalling. Nat Cell Biol 17: 868-879. doi: 10.1038/ncb3179
[74]	Luo X, Yu H (2008) Protein metamorphosis: the two-state behavior of Mad2. Structure 16: 1616-1625. doi: 10.1016/j.str.2008.10.002
[75]	Mapelli M, Musacchio A (2007) MAD contortions: conformational dimerization boosts spindle checkpoint signaling. Curr Opin Struct Biol 17: 716-725. doi: 10.1016/j.sbi.2007.08.011
[76]	Peters JM (2006) The anaphase promoting complex/cyclosome: a machine designed to destroy. Nat Rev Mol Cell Biol 7: 644-656.
[77]	Barford D (2011) Structural insights into anaphase-promoting complex function and mechanism. Philos Trans R Soc Lond B Biol Sci 366: 3605-3624. doi: 10.1098/rstb.2011.0069
[78]	Chang L, Barford D (2014) Insights into the anaphase-promoting complex: a molecular machine that regulates mitosis. Curr Opin Struct Biol 29: 1-9.
[79]	Qiao R, Weissmann F, Yamaguchi M, et al. (2016) Mechanism of APC/CCDC20 activation by mitotic phosphorylation. Proc Natl Acad Sci U S A 113: E2570-2578. doi: 10.1073/pnas.1604929113
[80]	Zhang S, Chang L, Alfieri C, et al. (2016) Molecular mechanism of APC/C activation by mitotic phosphorylation. Nature 533: 260-264. doi: 10.1038/nature17973
[81]	Steen JA, Steen H, Georgi A, et al. (2008) Different phosphorylation states of the anaphase promoting complex in response to antimitotic drugs: a quantitative proteomic analysis. Proc Natl Acad Sci U S A 105: 6069-6074. doi: 10.1073/pnas.0709807104
[82]	Fujimitsu K, Grimaldi M, Yamano H (2016) Cyclin-dependent kinase 1-dependent activation of APC/C ubiquitin ligase. Science 352: 1121-1124. doi: 10.1126/science.aad3925
[83]	Kimata Y, Baxter JE, Fry AM, et al. (2008) A role for the Fizzy/Cdc20 family of proteins in activation of the APC/C distinct from substrate recruitment. Mol Cell 32: 576-583. doi: 10.1016/j.molcel.2008.09.023
[84]	Glotzer M, Murray AW, Kirschner MW (1991) Cyclin is degraded by the ubiquitin pathway. Nature 349: 132-138. doi: 10.1038/349132a0
[85]	Pfleger CM, Kirschner MW (2000) The KEN box: an APC recognition signal distinct from the D box targeted by Cdh1. Genes Dev 14: 655-665.
[86]	Di Fiore B, Davey NE, Hagting A, et al. (2015) The ABBA motif binds APC/C activators and is shared by APC/C substrates and regulators. Dev Cell 32: 358-372. doi: 10.1016/j.devcel.2015.01.003
[87]	Reis A, Levasseur M, Chang HY, et al. (2006) The CRY box: a second APCcdh1-dependent degron in mammalian cdc20. EMBO Rep 7: 1040-1045. doi: 10.1038/sj.embor.7400772
[88]	Burton JL, Xiong Y, Solomon MJ (2011) Mechanisms of pseudosubstrate inhibition of the anaphase promoting complex by Acm1. EMBO J 30: 1818-1829. doi: 10.1038/emboj.2011.90
[89]	Diaz-Martinez LA, Tian W, Li B, et al. (2015) The Cdc20-binding Phe box of the spindle checkpoint protein BubR1 maintains the mitotic checkpoint complex during mitosis. J Biol Chem 290: 2431-2443. doi: 10.1074/jbc.M114.616490
[90]	Lischetti T, Zhang G, Sedgwick GG, et al. (2014) The internal Cdc20 binding site in BubR1 facilitates both spindle assembly checkpoint signalling and silencing. Nat Commun 5: 5563. doi: 10.1038/ncomms6563
[91]	Elowe S, Dulla K, Uldschmid A, et al. (2010) Uncoupling of the spindle-checkpoint and chromosome-congression functions of BubR1. J Cell Sci 123: 84-94. doi: 10.1242/jcs.056507
[92]	Morgan DO (2016) Cell division: Mitotic regulation comes into focus. Nature 536: 407-408. doi: 10.1038/nature19423
[93]	Tipton AR, Wang K, Link L, et al. (2011) BUBR1 and Closed MAD2 (C-MAD2) Interact Directly to Assemble a Functional Mitotic Checkpoint Complex. J Biol Chem 286: 21173-21179. doi: 10.1074/jbc.M111.238543
[94]	Izawa D, Pines J (2015) The mitotic checkpoint complex binds a second CDC20 to inhibit active APC/C. Nature 517: 631-634.
[95]	Lara-Gonzalez P, Scott MI, Diez M, et al. (2011) BubR1 blocks substrate recruitment to the APC/C in a KEN-box-dependent manner. J Cell Sci 124: 4332-4345. doi: 10.1242/jcs.094763
[96]	Larsen NA, Al-Bassam J, Wei RR, et al. (2007) Structural analysis of Bub3 interactions in the mitotic spindle checkpoint. Proc Natl Acad Sci U S A 104: 1201-1206. doi: 10.1073/pnas.0610358104
[97]	Larsen NA, Harrison SC (2004) Crystal structure of the spindle assembly checkpoint protein Bub3. J Mol Biol 344: 885-892. doi: 10.1016/j.jmb.2004.09.094
[98]	Primorac I, Weir JR, Chiroli E, et al. (2013) Bub3 reads phosphorylated MELT repeats to promote spindle assembly checkpoint signaling. Elife 2: e01030.
[99]	Overlack K, Primorac I, Vleugel M, et al. (2015) A molecular basis for the differential roles of Bub1 and BubR1 in the spindle assembly checkpoint. Elife 4: e05269.
[100]	Taylor SS, Ha E, McKeon F (1998) The human homologue of Bub3 is required for kinetochore localization of Bub1 and a Mad3/Bub1-related protein kinase. J Cell Biol 142: 1-11. doi: 10.1083/jcb.142.1.1
[101]	Yu H (2007) Cdc20: a WD40 activator for a cell cycle degradation machine. Mol Cell 27: 3-16. doi: 10.1016/j.molcel.2007.06.009
[102]	Visintin R, Prinz S, Amon A (1997) CDC20 and CDH1: a family of substrate-specific activators of APC-dependent proteolysis. Science 278: 460-463. doi: 10.1126/science.278.5337.460
[103]	Schwab M, Lutum AS, Seufert W (1997) Yeast Hct1 is a regulator of Clb2 cyclin proteolysis. Cell 90: 683-693. doi: 10.1016/S0092-8674(00)80529-2
[104]	Tian W, Li B, Warrington R, et al. (2012) Structural analysis of human Cdc20 supports multisite degron recognition by APC/C. Proc Natl Acad Sci U S A 109: 18419-18424. doi: 10.1073/pnas.1213438109
[105]	He J, Chao WC, Zhang Z, et al. (2013) Insights into degron recognition by APC/C coactivators from the structure of an Acm1-Cdh1 complex. Mol Cell 50: 649-660. doi: 10.1016/j.molcel.2013.04.024
[106]	Chang L, Zhang Z, Yang J, et al. (2014) Molecular architecture and mechanism of the anaphase-promoting complex. Nature 513: 388-393. doi: 10.1038/nature13543
[107]	Buschhorn BA, Petzold G, Galova M, et al. (2011) Substrate binding on the APC/C occurs between the coactivator Cdh1 and the processivity factor Doc1. Nat Struct Mol Biol 18: 6-13. doi: 10.1038/nsmb.1979
[108]	da Fonseca PC, Kong EH, Zhang Z, et al. (2011) Structures of APC/C(Cdh1) with substrates identify Cdh1 and Apc10 as the D-box co-receptor. Nature 470: 274-278. doi: 10.1038/nature09625
[109]	Izawa D, Pines J (2011) How APC/C-Cdc20 changes its substrate specificity in mitosis. Nat Cell Biol 13: 223-233. doi: 10.1038/ncb2165
[110]	Schwab M, Neutzner M, Mocker D, et al. (2001) Yeast Hct1 recognizes the mitotic cyclin Clb2 and other substrates of the ubiquitin ligase APC. Embo J 20: 5165-5175. doi: 10.1093/emboj/20.18.5165
[111]	Vodermaier HC, Gieffers C, Maurer-Stroh S, et al. (2003) TPR subunits of the anaphase-promoting complex mediate binding to the activator protein CDH1. Curr Biol 13: 1459-1468. doi: 10.1016/S0960-9822(03)00581-5
[112]	Luo X, Tang Z, Rizo J, et al. (2002) The Mad2 spindle checkpoint protein undergoes similar major conformational changes upon binding to either Mad1 or Cdc20. Mol Cell 9: 59-71. doi: 10.1016/S1097-2765(01)00435-X
[113]	Izawa D, Pines J (2012) Mad2 and the APC/C compete for the same site on Cdc20 to ensure proper chromosome segregation. J Cell Biol 199: 27-37. doi: 10.1083/jcb.201205170
[114]	Mondal G, Baral RN, Roychoudhury S (2006) A new Mad2-interacting domain of Cdc20 is critical for the function of Mad2-Cdc20 complex in the spindle assembly checkpoint. Biochem J 396: 243-253. doi: 10.1042/BJ20051914
[115]	Aravind L, Koonin EV (1998) The HORMA domain: a common structural denominator in mitotic checkpoints, chromosome synapsis and DNA repair. Trends Biochem Sci 23: 284-286.
[116]	Luo X, Tang Z, Xia G, et al. (2004) The Mad2 spindle checkpoint protein has two distinct natively folded states. Nat Struct Mol Biol 11: 338-345. doi: 10.1038/nsmb748
[117]	Sironi L, Mapelli M, Knapp S, et al. (2002) Crystal structure of the tetrameric Mad1-Mad2 core complex: implications of a 'safety belt' binding mechanism for the spindle checkpoint. Embo J 21: 2496-2506. doi: 10.1093/emboj/21.10.2496
[118]	Sironi L, Melixetian M, Faretta M, et al. (2001) Mad2 binding to Mad1 and Cdc20, rather than oligomerization, is required for the spindle checkpoint. Embo J 20: 6371-6382. doi: 10.1093/emboj/20.22.6371
[119]	Luo X, Fang G, Coldiron M, et al. (2000) Structure of the Mad2 spindle assembly checkpoint protein and its interaction with Cdc20. Nat Struct Biol 7: 224-229. doi: 10.1038/73338
[120]	Orth M, Mayer B, Rehm K, et al. (2011) Shugoshin is a Mad1/Cdc20-like interactor of Mad2. Embo J 30: 2868-2880. doi: 10.1038/emboj.2011.187
[121]	Lee SH, McCormick F, Saya H (2010) Mad2 inhibits the mitotic kinesin MKlp2. J Cell Biol 191: 1069-1077. doi: 10.1083/jcb.201003095
[122]	Schibler A, Koutelou E, Tomida J, et al. (2016) Histone H3K4 methylation regulates deactivation of the spindle assembly checkpoint through direct binding of Mad2. Genes Dev 30: 1187-1197.
[123]	Mapelli M, Massimiliano L, Santaguida S, et al. (2007) The Mad2 conformational dimer: structure and implications for the spindle assembly checkpoint. Cell 131: 730-743. doi: 10.1016/j.cell.2007.08.049
[124]	Yang M, Li B, Tomchick DR, et al. (2007) p31comet blocks Mad2 activation through structural mimicry. Cell 131: 744-755. doi: 10.1016/j.cell.2007.08.048
[125]	Yang M, Li B, Liu CJ, et al. (2008) Insights into mad2 regulation in the spindle checkpoint revealed by the crystal structure of the symmetric mad2 dimer. PLoS Biol 6: e50. doi: 10.1371/journal.pbio.0060050
[126]	Skinner JJ, Wood S, Shorter J, et al. (2008) The Mad2 partial unfolding model: regulating mitosis through Mad2 conformational switching. J Cell Biol 183: 761-768. doi: 10.1083/jcb.200808122
[127]	De Antoni A, Pearson CG, Cimini D, et al. (2005) The Mad1/Mad2 complex as a template for Mad2 activation in the spindle assembly checkpoint. Curr Biol 15: 214-225. doi: 10.1016/j.cub.2005.01.038
[128]	Campbell MS, Chan GK, Yen TJ (2001) Mitotic checkpoint proteins HsMAD1 and HsMAD2 are associated with nuclear pore complexes in interphase. J Cell Sci 114: 953-963.
[129]	Chen RH, Brady DM, Smith D, et al. (1999) The spindle checkpoint of budding yeast depends on a tight complex between the Mad1 and Mad2 proteins. Mol Biol Cell 10: 2607-2618. doi: 10.1091/mbc.10.8.2607
[130]	Martin-Lluesma S, Stucke VM, Nigg EA (2002) Role of hec1 in spindle checkpoint signaling and kinetochore recruitment of mad1/mad2. Science 297: 2267-2270. doi: 10.1126/science.1075596
[131]	Hewitt L, Tighe A, Santaguida S, et al. (2010) Sustained Mps1 activity is required in mitosis to recruit O-Mad2 to the Mad1-C-Mad2 core complex. J Cell Biol 190: 25-34. doi: 10.1083/jcb.201002133
[132]	Tipton AR, Ji W, Sturt-Gillespie B, et al. (2013) Monopolar Spindle 1 (MPS1) Kinase Promotes Production of Closed MAD2 (C-MAD2) Conformer and Assembly of the Mitotic Checkpoint Complex. J Biol Chem 288: 35149-35158. doi: 10.1074/jbc.M113.522375
[133]	Liu ST, Chan GK, Hittle JC, et al. (2003) Human MPS1 Kinase Is Required for Mitotic Arrest Induced by the Loss of CENP-E from Kinetochores. Mol Biol Cell 14: 1638-1651. doi: 10.1091/mbc.02-05-0074
[134]	Liu ST, Hittle JC, Jablonski SA, et al. (2003) Human CENP-I specifies localization of CENP-F, MAD1 and MAD2 to kinetochores and is essential for mitosis. Nat Cell Biol 5: 341-345. doi: 10.1038/ncb953
[135]	Liu ST, Rattner JB, Jablonski SA, et al. (2006) Mapping the assembly pathways that specify formation of the trilaminar kinetochore plates in human cells. J Cell Biol 175: 41-53. doi: 10.1083/jcb.200606020
[136]	Tipton AR, Tipton M, Yen T, et al. (2011) Closed MAD2 (C-MAD2) is selectively incorporated into the mitotic checkpoint complex (MCC). Cell Cycle 10: 3740-3750. doi: 10.4161/cc.10.21.17919
[137]	Tang Z, Bharadwaj R, Li B, et al. (2001) Mad2-Independent inhibition of APCCdc20 by the mitotic checkpoint protein BubR1. Dev Cell 1: 227-237. doi: 10.1016/S1534-5807(01)00019-3
[138]	Fang G (2002) Checkpoint protein BubR1 acts synergistically with Mad2 to inhibit anaphase-promoting complex. Mol Biol Cell 13: 755-766. doi: 10.1091/mbc.01-09-0437
[139]	Kulukian A, Han JS, Cleveland DW (2009) Unattached kinetochores catalyze production of an anaphase inhibitor that requires a Mad2 template to prime Cdc20 for BubR1 binding. Dev Cell 16: 105-117. doi: 10.1016/j.devcel.2008.11.005
[140]	Han JS, Holland AJ, Fachinetti D, et al. (2013) Catalytic Assembly of the Mitotic Checkpoint Inhibitor BubR1-Cdc20 by a Mad2-Induced Functional Switch in Cdc20. Mol Cell 51: 92-104. doi: 10.1016/j.molcel.2013.05.019
[141]	Westhorpe FG, Tighe A, Lara-Gonzalez P, et al. (2011) p31comet-mediated extraction of Mad2 from the MCC promotes efficient mitotic exit. J Cell Sci 124: 3905-3916. doi: 10.1242/jcs.093286
[142]	Miller JJ, Summers MK, Hansen DV, et al. (2006) Emi1 stably binds and inhibits the anaphase-promoting complex/cyclosome as a pseudosubstrate inhibitor. Genes Dev 20: 2410-2420. doi: 10.1101/gad.1454006
[143]	Eytan E, Braunstein I, Ganoth D, et al. (2008) Two different mitotic checkpoint inhibitors of the anaphase-promoting complex/cyclosome antagonize the action of the activator Cdc20. Proc Natl Acad Sci U S A 105: 9181-9185. doi: 10.1073/pnas.0804069105
[144]	Poddar A, Stukenberg PT, Burke DJ (2005) Two complexes of spindle checkpoint proteins containing Cdc20 and Mad2 assemble during mitosis independently of the kinetochore in Saccharomyces cerevisiae. Eukaryot Cell 4: 867-878. doi: 10.1128/EC.4.5.867-878.2005
[145]	Weinstein J (1997) Cell cycle-regulated expression, phosphorylation, and degradation of p55Cdc. A mammalian homolog of CDC20/Fizzy/slp1. J Biol Chem 272: 28501-28511.
[146]	Wolthuis R, Clay-Farrace L, van Zon W, et al. (2008) Cdc20 and Cks direct the spindle checkpoint-independent destruction of cyclin A. Mol Cell 30: 290-302. doi: 10.1016/j.molcel.2008.02.027
[147]	Malureanu LA, Jeganathan KB, Hamada M, et al. (2009) BubR1 N terminus acts as a soluble inhibitor of cyclin B degradation by APC/C(Cdc20) in interphase. Dev Cell 16: 118-131. doi: 10.1016/j.devcel.2008.11.004
[148]	Ma HT, Poon RY (2011) Orderly inactivation of the key checkpoint protein mitotic arrest deficient 2 (MAD2) during mitotic progression. J Biol Chem 286: 13052-13059. doi: 10.1074/jbc.M110.201897
[149]	Michel LS, Liberal V, Chatterjee A, et al. (2001) MAD2 haplo-insufficiency causes premature anaphase and chromosome instability in mammalian cells. Nature 409: 355-359. doi: 10.1038/35053094
[150]	Hernando E, Nahle Z, Juan G, et al. (2004) Rb inactivation promotes genomic instability by uncoupling cell cycle progression from mitotic control. Nature 430: 797-802. doi: 10.1038/nature02820
[151]	Schvartzman JM, Duijf PH, Sotillo R, et al. (2011) Mad2 is a critical mediator of the chromosome instability observed upon Rb and p53 pathway inhibition. Cancer Cell 19: 701-714. doi: 10.1016/j.ccr.2011.04.017
[152]	Herzog F, Primorac I, Dube P, et al. (2009) Structure of the anaphase-promoting complex/cyclosome interacting with a mitotic checkpoint complex. Science 323: 1477-1481. doi: 10.1126/science.1163300
[153]	Kramer ER, Scheuringer N, Podtelejnikov AV, et al. (2000) Mitotic regulation of the APC activator proteins CDC20 and CDH1. Mol Biol Cell 11: 1555-1569. doi: 10.1091/mbc.11.5.1555
[154]	Simonetta M, Manzoni R, Mosca R, et al. (2009) The influence of catalysis on mad2 activation dynamics. PLoS Biol 7: e10.
[155]	Nilsson J, Yekezare M, Minshull J, et al. (2008) The APC/C maintains the spindle assembly checkpoint by targeting Cdc20 for destruction. Nat Cell Biol 10: 1411-1420. doi: 10.1038/ncb1799
[156]	Kallio M, Weinstein J, Daum JR, et al. (1998) Mammalian p55CDC mediates association of the spindle checkpoint protein Mad2 with the cyclosome/anaphase-promoting complex, and is involved in regulating anaphase onset and late mitotic events. J Cell Biol 141: 1393-1406. doi: 10.1083/jcb.141.6.1393
[157]	Diaz-Martinez LA, Yu H (2007) Running on a treadmill: dynamic inhibition of APC/C by the spindle checkpoint. Cell Div 2: 23. doi: 10.1186/1747-1028-2-23
[158]	Fava LL, Kaulich M, Nigg EA, et al. (2011) Probing the in vivo function of Mad1:C-Mad2 in the spindle assembly checkpoint. Embo J 30: 3322-3336. doi: 10.1038/emboj.2011.239
[159]	Sedgwick GG, Larsen MS, Lischetti T, et al. (2016) Conformation-specific anti-Mad2 monoclonal antibodies for the dissection of checkpoint signaling. MAbs 8: 689-697. doi: 10.1080/19420862.2016.1160988
[160]	Davenport J, Harris LD, Goorha R (2006) Spindle checkpoint function requires Mad2-dependent Cdc20 binding to the Mad3 homology domain of BubR1. Exp Cell Res 312: 1831-1842. doi: 10.1016/j.yexcr.2006.02.018
[161]	Murray AW, Kirschner MW (1989) Dominoes and clocks: the union of two views of the cell cycle. Science 246: 614-621. doi: 10.1126/science.2683077
[162]	Meraldi P, Draviam VM, Sorger PK (2004) Timing and checkpoints in the regulation of mitotic progression. Dev Cell 7: 45-60. doi: 10.1016/j.devcel.2004.06.006
[163]	Waters JC, Chen RH, Murray AW, et al. (1998) Localization of Mad2 to kinetochores depends on microtubule attachment, not tension. J Cell Biol 141: 1181-1191. doi: 10.1083/jcb.141.5.1181
[164]	Hauf S, Cole RW, LaTerra S, et al. (2003) The small molecule Hesperadin reveals a role for Aurora B in correcting kinetochore-microtubule attachment and in maintaining the spindle assembly checkpoint. J Cell Biol 161: 281-294. doi: 10.1083/jcb.200208092
[165]	Canman JC, Sharma N, Straight A, et al. (2002) Anaphase onset does not require the microtubule-dependent depletion of kinetochore and centromere-binding proteins. J Cell Sci 115: 3787-3795. doi: 10.1242/jcs.00057
[166]	Ma HT, Chan YY, Chen X, et al. (2012) Depletion of p31comet protein promotes sensitivity to antimitotic drugs. J Biol Chem 287: 21561-21569. doi: 10.1074/jbc.M112.364356
[167]	Brown NG, VanderLinden R, Watson ER, et al. (2016) Dual RING E3 Architectures Regulate Multiubiquitination and Ubiquitin Chain Elongation by APC/C. Cell 165: 1440-1453. doi: 10.1016/j.cell.2016.05.037
[168]	Brown NG, Watson ER, Weissmann F, et al. (2014) Mechanism of polyubiquitination by human anaphase-promoting complex: RING repurposing for ubiquitin chain assembly. Mol Cell 56: 246-260. doi: 10.1016/j.molcel.2014.09.009
[169]	Primorac I, Musacchio A (2013) Panta rhei: the APC/C at steady state. J Cell Biol 201: 177-189. doi: 10.1083/jcb.201301130
[170]	Jin L, Williamson A, Banerjee S, et al. (2008) Mechanism of ubiquitin-chain formation by the human anaphase-promoting complex. Cell 133: 653-665. doi: 10.1016/j.cell.2008.04.012
[171]	Williamson A, Wickliffe KE, Mellone BG, et al. (2009) Identification of a physiological E2 module for the human anaphase-promoting complex. Proc Natl Acad Sci U S A 106: 18213-18218. doi: 10.1073/pnas.0907887106
[172]	Dimova NV, Hathaway NA, Lee BH, et al. (2012) APC/C-mediated multiple monoubiquitylation provides an alternative degradation signal for cyclin B1. Nat Cell Biol 14: 168-176. doi: 10.1038/ncb2425
[173]	Meyer HJ, Rape M (2014) Enhanced protein degradation by branched ubiquitin chains. Cell 157: 910-921. doi: 10.1016/j.cell.2014.03.037
[174]	Tang Z, Li B, Bharadwaj R, et al. (2001) APC2 Cullin protein and APC11 RING protein comprise the minimal ubiquitin ligase module of the anaphase-promoting complex. Mol Biol Cell 12: 3839-3851. doi: 10.1091/mbc.12.12.3839
[175]	Brown NG, VanderLinden R, Watson ER, et al. (2015) RING E3 mechanism for ubiquitin ligation to a disordered substrate visualized for human anaphase-promoting complex. Proc Natl Acad Sci U S A 112: 5272-5279. doi: 10.1073/pnas.1504161112
[176]	Fang G, Yu H, Kirschner MW (1998) Direct binding of CDC20 protein family members activates the anaphase-promoting complex in mitosis and G1. Mol Cell 2: 163-171. doi: 10.1016/S1097-2765(00)80126-4
[177]	Chang L, Zhang Z, Yang J, et al. (2015) Atomic structure of the APC/C and its mechanism of protein ubiquitination. Nature 522: 450-454. doi: 10.1038/nature14471
[178]	Kelly A, Wickliffe KE, Song L, et al. (2014) Ubiquitin chain elongation requires E3-dependent tracking of the emerging conjugate. Mol Cell 56: 232-245. doi: 10.1016/j.molcel.2014.09.010
[179]	Braunstein I, Miniowitz S, Moshe Y, et al. (2007) Inhibitory factors associated with anaphase-promoting complex/cylosome in mitotic checkpoint. Proc Natl Acad Sci U S A 104: 4870-4875. doi: 10.1073/pnas.0700523104
[180]	Miniowitz-Shemtov S, Teichner A, Sitry-Shevah D, et al. (2010) ATP is required for the release of the anaphase-promoting complex/cyclosome from inhibition by the mitotic checkpoint. Proc Natl Acad Sci U S A 107: 5351-5356. doi: 10.1073/pnas.1001875107
[181]	Pan J, Chen RH (2004) Spindle checkpoint regulates Cdc20p stability in Saccharomyces cerevisiae. Genes Dev 18: 1439-1451. doi: 10.1101/gad.1184204
[182]	Foe IT, Foster SA, Cheung SK, et al. (2011) Ubiquitination of Cdc20 by the APC occurs through an intramolecular mechanism. Curr Biol 21: 1870-1877. doi: 10.1016/j.cub.2011.09.051
[183]	Ge S, Skaar JR, Pagano M (2009) APC/C- and Mad2-mediated degradation of Cdc20 during spindle checkpoint activation. Cell Cycle 8: 167-171. doi: 10.4161/cc.8.1.7606
[184]	Jia L, Li B, Warrington RT, et al. (2011) Defining pathways of spindle checkpoint silencing: functional redundancy between Cdc20 ubiquitination and p31(comet). Mol Biol Cell 22: 4227-4235. doi: 10.1091/mbc.E11-05-0389
[185]	Varetti G, Guida C, Santaguida S, et al. (2011) Homeostatic control of mitotic arrest. Mol Cell 44: 710-720. doi: 10.1016/j.molcel.2011.11.014
[186]	King EM, van der Sar SJ, Hardwick KG (2007) Mad3 KEN boxes mediate both Cdc20 and Mad3 turnover, and are critical for the spindle checkpoint. PLoS ONE 2: e342. doi: 10.1371/journal.pone.0000342
[187]	Zhang Y, Foreman O, Wigle DA, et al. (2012) USP44 regulates centrosome positioning to prevent aneuploidy and suppress tumorigenesis. J Clin Invest 122: 4362-4374. doi: 10.1172/JCI63084
[188]	Zhang Y, van Deursen J, Galardy PJ (2011) Overexpression of ubiquitin specific protease 44 (USP44) induces chromosomal instability and is frequently observed in human T-cell leukemia. PLoS One 6: e23389. doi: 10.1371/journal.pone.0023389
[189]	Kim S, Yu H (2011) Mutual regulation between the spindle checkpoint and APC/C. Semin Cell Dev Biol 22: 551-558. doi: 10.1016/j.semcdb.2011.03.008
[190]	Zeng X, Sigoillot F, Gaur S, et al. (2010) Pharmacologic inhibition of the anaphase-promoting complex induces a spindle checkpoint-dependent mitotic arrest in the absence of spindle damage. Cancer Cell 18: 382-395. doi: 10.1016/j.ccr.2010.08.010
[191]	Bezler A, Gonczy P (2010) Mutual antagonism between the anaphase promoting complex and the spindle assembly checkpoint contributes to mitotic timing in Caenorhabditis elegans. Genetics 186: 1271-1283. doi: 10.1534/genetics.110.123133
[192]	Chesnel F, Bazile F, Pascal A, et al. (2006) Cyclin B dissociation from CDK1 precedes its degradation upon MPF inactivation in mitotic extracts of Xenopus laevis embryos. Cell Cycle 5: 1687-1698. doi: 10.4161/cc.5.15.3123
[193]	Holt LJ, Krutchinsky AN, Morgan DO (2008) Positive feedback sharpens the anaphase switch. Nature 454: 353-357. doi: 10.1038/nature07050
[194]	Ye Q, Rosenberg SC, Moeller A, et al. (2015) TRIP13 is a protein-remodeling AAA+ ATPase that catalyzes MAD2 conformation switching. Elife 4.
[195]	Vader G (2015) Pch2(TRIP13): controlling cell division through regulation of HORMA domains. Chromosoma 124: 333-339. doi: 10.1007/s00412-015-0516-y
[196]	Musacchio A (2015) Closing the Mad2 cycle. Elife 4.
[197]	Verdugo A, Vinod PK, Tyson JJ, et al. (2013) Molecular mechanisms creating bistable switches at cell cycle transitions. Open Biol 3: 120179. doi: 10.1098/rsob.120179
[198]	Hauf S (2013) The spindle assembly checkpoint: progress and persistent puzzles. Biochem Soc Trans 41: 1755-1760. doi: 10.1042/BST20130240
[199]	Hagan RS, Manak MS, Buch HK, et al. (2011) p31(comet) acts to ensure timely spindle checkpoint silencing subsequent to kinetochore attachment. Mol Biol Cell 22: 4236-4246. doi: 10.1091/mbc.E11-03-0216
[200]	Teichner A, Eytan E, Sitry-Shevah D, et al. (2011) p31comet promotes disassembly of the mitotic checkpoint complex in an ATP-dependent process. Proc Natl Acad Sci U S A 108: 3187-3192. doi: 10.1073/pnas.1100023108
[201]	Miniowitz-Shemtov S, Eytan E, Ganoth D, et al. (2012) Role of phosphorylation of Cdc20 in p31(comet)-stimulated disassembly of the mitotic checkpoint complex. Proc Natl Acad Sci U S A 109: 8056-8060. doi: 10.1073/pnas.1204081109
[202]	Carter SL, Eklund AC, Kohane IS, et al. (2006) A signature of chromosomal instability inferred from gene expression profiles predicts clinical outcome in multiple human cancers. Nat Genet 38: 1043-1048. doi: 10.1038/ng1861
[203]	Martin KJ, Patrick DR, Bissell MJ, et al. (2008) Prognostic breast cancer signature identified from 3D culture model accurately predicts clinical outcome across independent datasets. PLoS ONE 3: e2994. doi: 10.1371/journal.pone.0002994
[204]	Rhodes DR, Yu J, Shanker K, et al. (2004) Large-scale meta-analysis of cancer microarray data identifies common transcriptional profiles of neoplastic transformation and progression. Proc Natl Acad Sci U S A 101: 9309-9314. doi: 10.1073/pnas.0401994101
[205]	Neuwald AF, Aravind L, Spouge JL, et al. (1999) AAA+: A class of chaperone-like ATPases associated with the assembly, operation, and disassembly of protein complexes. Genome Res 9: 27-43.
[206]	Ma HT, Poon RY (2016) TRIP13 Regulates Both the Activation and Inactivation of the Spindle-Assembly Checkpoint. Cell Rep 14: 1086-1099. doi: 10.1016/j.celrep.2016.01.001
[207]	Nelson CR, Hwang T, Chen PH, et al. (2015) TRIP13PCH-2 promotes Mad2 localization to unattached kinetochores in the spindle checkpoint response. J Cell Biol 211: 503-516. doi: 10.1083/jcb.201505114
[208]	Hardwick KG, Shah JV (2010) Spindle checkpoint silencing: ensuring rapid and concerted anaphase onset. F1000 Biol Rep 2: 55.
[209]	Vanoosthuyse V, Hardwick KG (2009) Overcoming inhibition in the spindle checkpoint. Genes Dev 23: 2799-2805. doi: 10.1101/gad.1882109
[210]	Chan YW, Fava LL, Uldschmid A, et al. (2009) Mitotic control of kinetochore-associated dynein and spindle orientation by human Spindly. J Cell Biol 185: 859-874. doi: 10.1083/jcb.200812167
[211]	Gassmann R, Holland AJ, Varma D, et al. (2010) Removal of Spindly from microtubule-attached kinetochores controls spindle checkpoint silencing in human cells. Genes Dev 24: 957-971. doi: 10.1101/gad.1886810
[212]	Meadows JC, Shepperd LA, Vanoosthuyse V, et al. (2011) Spindle checkpoint silencing requires association of PP1 to both Spc7 and kinesin-8 motors. Dev Cell 20: 739-750. doi: 10.1016/j.devcel.2011.05.008
[213]	Porter IM, Schleicher K, Porter M, et al. (2013) Bod1 regulates protein phosphatase 2A at mitotic kinetochores. Nat Commun 4: 2677.
[214]	Pinsky BA, Kotwaliwale CV, Tatsutani SY, et al. (2006) Glc7/protein phosphatase 1 regulatory subunits can oppose the Ipl1/aurora protein kinase by redistributing Glc7. Mol Cell Biol 26: 2648-2660. doi: 10.1128/MCB.26.7.2648-2660.2006
[215]	Vanoosthuyse V, Meadows JC, van der Sar SJ, et al. (2009) Bub3p facilitates spindle checkpoint silencing in fission yeast. Mol Biol Cell 20: 5096-5105. doi: 10.1091/mbc.E09-09-0762
[216]	Kim T, Moyle MW, Lara-Gonzalez P, et al. (2015) Kinetochore-localized BUB-1/BUB-3 complex promotes anaphase onset in C. elegans. J Cell Biol 209: 507-517. doi: 10.1083/jcb.201412035
[217]	Yang Y, Lacefield S (2016) Bub3 activation and inhibition of the APC/C. Cell Cycle 15: 1-2. doi: 10.1080/15384101.2015.1106746
[218]	Windecker H, Langegger M, Heinrich S, et al. (2009) Bub1 and Bub3 promote the conversion from monopolar to bipolar chromosome attachment independently of shugoshin. EMBO Rep 10: 1022-1028. doi: 10.1038/embor.2009.183
[219]	Yang Y, Tsuchiya D, Lacefield S (2015) Bub3 promotes Cdc20-dependent activation of the APC/C in S. cerevisiae. J Cell Biol 209: 519-527. doi: 10.1083/jcb.201412036
[220]	Han JS, Vitre B, Fachinetti D, et al. (2014) Bimodal activation of BubR1 by Bub3 sustains mitotic checkpoint signaling. Proc Natl Acad Sci U S A 111: E4185-4193. doi: 10.1073/pnas.1416277111
[221]	Kim S, Sun H, Ball HL, et al. (2010) Phosphorylation of the spindle checkpoint protein Mad2 regulates its conformational transition. Proc Natl Acad Sci U S A 107: 19772-19777. doi: 10.1073/pnas.1009000107
[222]	Wassmann K, Liberal V, Benezra R (2003) Mad2 phosphorylation regulates its association with Mad1 and the APC/C. Embo J 22: 797-806. doi: 10.1093/emboj/cdg071
[223]	Elowe S, Hummer S, Uldschmid A, et al. (2007) Tension-sensitive Plk1 phosphorylation on BubR1 regulates the stability of kinetochore microtubule interactions. Genes Dev 21: 2205-2219. doi: 10.1101/gad.436007
[224]	Matsumura S, Toyoshima F, Nishida E (2007) Polo-like kinase 1 facilitates chromosome alignment during prometaphase through BubR1. J Biol Chem 282: 15217-15227. doi: 10.1074/jbc.M611053200
[225]	Chan GK, Jablonski SA, Sudakin V, et al. (1999) Human BUBR1 is a mitotic checkpoint kinase that monitors CENP-E functions at kinetochores and binds the cyclosome/APC. J Cell Biol 146: 941-954. doi: 10.1083/jcb.146.5.941
[226]	Dou Z, von Schubert C, Korner R, et al. (2011) Quantitative mass spectrometry analysis reveals similar substrate consensus motif for human Mps1 kinase and Plk1. PLoS ONE 6: e18793. doi: 10.1371/journal.pone.0018793
[227]	Huang H, Hittle J, Zappacosta F, et al. (2008) Phosphorylation sites in BubR1 that regulate kinetochore attachment, tension, and mitotic exit. J Cell Biol 183: 667-680. doi: 10.1083/jcb.200805163
[228]	Mao Y, Desai A, Cleveland DW (2005) Microtubule capture by CENP-E silences BubR1-dependent mitotic checkpoint signaling. J Cell Biol 170: 873-880. doi: 10.1083/jcb.200505040
[229]	Elowe S (2011) Bub1 and BubR1: at the interface between chromosome attachment and the spindle checkpoint. Mol Cell Biol 31: 3085-3093. doi: 10.1128/MCB.05326-11
[230]	Bolanos-Garcia VM, Blundell TL (2011) BUB1 and BUBR1: multifaceted kinases of the cell cycle. Trends Biochem Sci 36: 141-150. doi: 10.1016/j.tibs.2010.08.004
[231]	Chung E, Chen RH (2003) Phosphorylation of Cdc20 is required for its inhibition by the spindle checkpoint. Nat Cell Biol 5: 748-753. doi: 10.1038/ncb1022
[232]	Kallio M, Mustalahti T, Yen TJ, et al. (1998) Immunolocalization of alpha-tubulin, gamma-tubulin, and CENP-E in male rat and male mouse meiotic divisions: pathway of meiosis I spindle formation in mammalian spermatocytes. Dev Biol 195: 29-37. doi: 10.1006/dbio.1997.8822
[233]	Wu H, Lan Z, Li W, et al. (2000) p55CDC/hCDC20 is associated with BUBR1 and may be a downstream target of the spindle checkpoint kinase. Oncogene 19: 4557-4562. doi: 10.1038/sj.onc.1203803
[234]	Zich J, Hardwick KG (2009) Getting down to the phosphorylated 'nuts and bolts' of spindle checkpoint signalling. Trends Biochem Sci.
[235]	Hornbeck PV, Zhang B, Murray B, et al. (2015) PhosphoSitePlus, 2014: mutations, PTMs and recalibrations. Nucleic Acids Res 43: D512-520. doi: 10.1093/nar/gku1267
[236]	Kim M, Murphy K, Liu F, et al. (2005) Caspase-mediated specific cleavage of BubR1 is a determinant of mitotic progression. Mol Cell Biol 25: 9232-9248. doi: 10.1128/MCB.25.21.9232-9248.2005
[237]	Baek KH, Shin HJ, Jeong SJ, et al. (2005) Caspases-dependent cleavage of mitotic checkpoint proteins in response to microtubule inhibitor. Oncol Res 15: 161-168.
[238]	Choi E, Choe H, Min J, et al. (2009) BubR1 acetylation at prometaphase is required for modulating APC/C activity and timing of mitosis. Embo J 28: 2077-2089. doi: 10.1038/emboj.2009.123
[239]	Park I, Lee HO, Choi E, et al. (2013) Loss of BubR1 acetylation causes defects in spindle assembly checkpoint signaling and promotes tumor formation. J Cell Biol 202: 295-309. doi: 10.1083/jcb.201210099
[240]	Yang F, Hu L, Chen C, et al. (2012) BubR1 is modified by sumoylation during mitotic progression. J Biol Chem 287: 4875-4882. doi: 10.1074/jbc.M111.318261
[241]	Yang F, Huang Y, Dai W (2012) Sumoylated BubR1 plays an important role in chromosome segregation and mitotic timing. Cell Cycle 11: 797-806. doi: 10.4161/cc.11.4.19307
[242]	Doncic A, Ben-Jacob E, Barkai N (2005) Evaluating putative mechanisms of the mitotic spindle checkpoint. Proc Natl Acad Sci U S A 102: 6332-6337. doi: 10.1073/pnas.0409142102
[243]	Sear RP, Howard M (2006) Modeling dual pathways for the metazoan spindle assembly checkpoint. Proc Natl Acad Sci U S A 103: 16758-16763. doi: 10.1073/pnas.0603174103
[244]	Mistry HB, MacCallum DE, Jackson RC, et al. (2008) Modeling the temporal evolution of the spindle assembly checkpoint and role of Aurora B kinase. Proc Natl Acad Sci U S A 105: 20215-20220. doi: 10.1073/pnas.0810706106
[245]	Chen J, Liu J (2014) Spatial-temporal model for silencing of the mitotic spindle assembly checkpoint. Nat Commun 5: 4795. doi: 10.1038/ncomms5795
[246]	Chen J, Liu J (2016) Spindle Size Scaling Contributes to Robust Silencing of Mitotic Spindle Assembly Checkpoint. Biophys J 111: 1064-1077. doi: 10.1016/j.bpj.2016.07.039
[247]	Ibrahim B (2015) Toward a systems-level view of mitotic checkpoints. Prog Biophys Mol Biol 117: 217-224. doi: 10.1016/j.pbiomolbio.2015.02.005
[248]	Ibrahim B (2015) Spindle assembly checkpoint is sufficient for complete Cdc20 sequestering in mitotic control. Comput Struct Biotechnol J 13: 320-328. doi: 10.1016/j.csbj.2015.03.006
[249]	Ibrahim B, Dittrich P, Diekmann S, et al. (2008) Mad2 binding is not sufficient for complete Cdc20 sequestering in mitotic transition control (an in silico study). Biophys Chem 134: 93-100. doi: 10.1016/j.bpc.2008.01.007
[250]	Ibrahim B, Schmitt E, Dittrich P, et al. (2009) In silico study of kinetochore control, amplification, and inhibition effects in MCC assembly. Biosystems 95: 35-50. doi: 10.1016/j.biosystems.2008.06.007
[251]	Ciliberto A, Shah JV (2009) A quantitative systems view of the spindle assembly checkpoint. Embo J 28: 2162-2173. doi: 10.1038/emboj.2009.186
[252]	Burton JL, Solomon MJ (2007) Mad3p, a pseudosubstrate inhibitor of APCCdc20 in the spindle assembly checkpoint. Genes Dev 21: 655-667. doi: 10.1101/gad.1511107
[253]	D'Arcy S, Davies OR, Blundell TL, et al. (2010) Defining the molecular basis of BubR1 kinetochore interactions and APC/C-CDC20 inhibition. J Biol Chem 285: 14764-14776. doi: 10.1074/jbc.M109.082016
[254]	Bolanos-Garcia VM, Lischetti T, Matak-Vinkovic D, et al. (2011) Structure of a Blinkin-BUBR1 complex reveals an interaction crucial for kinetochore-mitotic checkpoint regulation via an unanticipated binding Site. Structure 19: 1691-1700. doi: 10.1016/j.str.2011.09.017
[255]	Krenn V, Wehenkel A, Li X, et al. (2012) Structural analysis reveals features of the spindle checkpoint kinase Bub1-kinetochore subunit Knl1 interaction. J Cell Biol 196: 451-467. doi: 10.1083/jcb.201110013
[256]	Harris L, Davenport J, Neale G, et al. (2005) The mitotic checkpoint gene BubR1 has two distinct functions in mitosis. Exp Cell Res 308: 85-100. doi: 10.1016/j.yexcr.2005.03.036
[257]	Zhang Y, Lees E (2001) Identification of an overlapping binding domain on Cdc20 for Mad2 and anaphase-promoting complex: model for spindle checkpoint regulation. Mol Cell Biol 21: 5190-5199. doi: 10.1128/MCB.21.15.5190-5199.2001
[258]	Sethi N, Monteagudo MC, Koshland D, et al. (1991) The CDC20 gene product of Saccharomyces cerevisiae, a beta-transducin homolog, is required for a subset of microtubule-dependent cellular processes. Mol Cell Biol 11: 5592-5602. doi: 10.1128/MCB.11.11.5592
[259]	Rosenberg SC, Corbett KD (2015) The multifaceted roles of the HORMA domain in cellular signaling. J Cell Biol 211: 745-755. doi: 10.1083/jcb.201509076
[260]	Mapelli M, Filipp FV, Rancati G, et al. (2006) Determinants of conformational dimerization of Mad2 and its inhibition by p31comet. Embo J 25: 1273-1284 doi: 10.1038/sj.emboj.7601033

Reader Comments

Your name:*

Email:*
© 2016 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)