Relation between DNA damage measured by comet assay and <em>OGG1 </em>Ser326Cys polymorphism in antineoplastic drugs biomonitoring

Carina Ladeira; Susana Viegas; Mário Pádua; Elisabete Carolino; Manuel C. Gomes; Miguel Brito; Carina Ladeira; Susana Viegas; Mário Pádua; Elisabete Carolino; Manuel C. Gomes; Miguel Brito

doi:10.3934/genet.2015.3.204

AIMS Genetics

2015, Volume 2, Issue 3: 204-218. doi: 10.3934/genet.2015.3.204

Previous Article Next Article

Research article Special Issues

Relation between DNA damage measured by comet assay and OGG1 Ser326Cys polymorphism in antineoplastic drugs biomonitoring

1.
Environment and Health Research Group, Escola Superior de Tecnologia da Saúde de Lisboa-IPL, Portugal;
2.
Grupo de Investigação em Genética e Metabolismo, Escola Superior de Tecnologia da Saúde de Lisboa-IPL, Portugal;
3.
Centro de Investigação e Estudos em Saúde Pública, Escola Nacional de Saúde Pública, ENSP, Universidade Nova de Lisboa, Portugal;
4.
Faculdade de Ciências, Universidade de Lisboa, Portugal

Received: 16 April 2015 Accepted: 11 August 2015 Published: 25 January 2015

Antineoplastic drugs are hazardous chemical agents used mostly in the treatment of patients with cancer, however health professionals that handle and administer these drugs can become exposed and develop DNA damage. Comet assay is a standard method for assessing DNA damage in human biomonitoring and, combined with formamidopyrimidine DNA glycosylase (FPG) enzyme, it specifically detects DNA oxidative damage.
The aim of this study was to investigate genotoxic effects in workers occupationally exposed to cytostatics (n = 46), as compared to a control group with no exposure (n = 46) at two Portuguese hospitals, by means of the alkaline comet assay. The potential of the OGG1 Ser326Cys polymorphism as a susceptibility biomarker was also investigated. Exposure was evaluated by investigating the contamination of surfaces and genotoxic assessment was done by alkaline comet assay in peripheral blood lymphocytes. OGG1 Ser326Cys (rs1052133) polymorphism was studied by Real Time PCR.
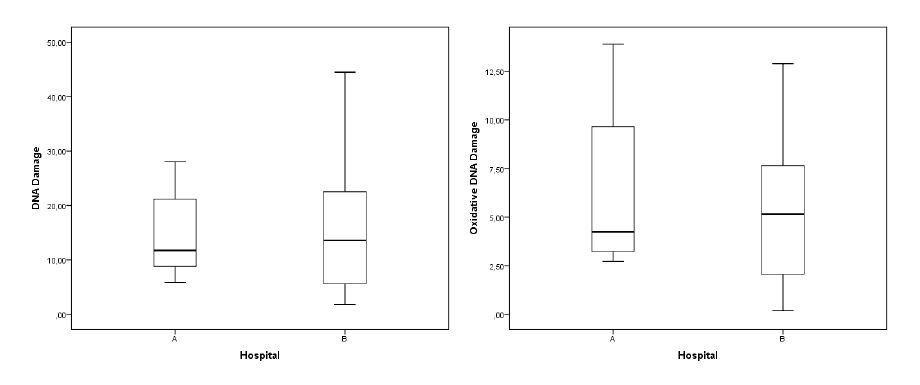
As for exposure assessment, there were 121 (37%) positive samples out of a total of 327 samples analysed from both hospitals. No statistically significant differences (Mann-Whitney test, p > 0.05) were found between subjects with and without exposure, regarding DNA damage and oxidative DNA damage, nevertheless the exposed group exhibited higher values. Moreover, there was no consistent trend regarding the variation of both biomarkers as assessed by comet assay with OGG1 polymorphism.
Our study was not statistically significant regarding occupational exposure to antineoplastic drugs and genetic damage assessed by comet assay. However, health professionals should be monitored for risk behaviour, in order to ensure that safety measures are applied and protection devices are used correctly.

Keywords:

Citation: Carina Ladeira, Susana Viegas, Mário Pádua, Elisabete Carolino, Manuel C. Gomes, Miguel Brito. Relation between DNA damage measured by comet assay and OGG1 Ser326Cys polymorphism in antineoplastic drugs biomonitoring[J]. AIMS Genetics, 2015, 2(3): 204-218. doi: 10.3934/genet.2015.3.204

Related Papers:

[1]	Panpan Jia, Jizhu Nan, Yongsheng Ma . Separating invariants for certain representations of the elementary Abelian $ p $-groups of rank two. AIMS Mathematics, 2024, 9(9): 25603-25618. doi: 10.3934/math.20241250
[2]	Hengbin Zhang . Automorphism group of the commuting graph of $ 2\times 2 $ matrix ring over $ \mathbb{Z}_{p^{s}} $. AIMS Mathematics, 2021, 6(11): 12650-12659. doi: 10.3934/math.2021729
[3]	Huaqing Gong, Shilin Yang . The representation ring of a non-pointed bialgebra. AIMS Mathematics, 2025, 10(3): 5110-5123. doi: 10.3934/math.2025234
[4]	Hicham Saber, Tariq Alraqad, Rashid Abu-Dawwas . On graded $ s $-prime submodules. AIMS Mathematics, 2021, 6(3): 2510-2524. doi: 10.3934/math.2021152
[5]	Fatma Zehra Uzekmek, Elif Segah Oztas, Mehmet Ozen . $ (\theta_i, \lambda) $-constacyclic codes and DNA codes over $ \mathbb{Z}_{4}+u\mathbb{Z}_{4}+u^{2}\mathbb{Z}_{4} $. AIMS Mathematics, 2024, 9(10): 27908-27929. doi: 10.3934/math.20241355
[6]	Pengcheng Ji, Jialei Chen, Fengxia Gao . Projective class ring of a restricted quantum group $ \overline{U}_{q}(\mathfrak{sl}^{}_2) $. AIMS Mathematics, 2023, 8(9): 19933-19949. doi: 10.3934/math.20231016*
[7]	Dong Su, Shilin Yang . Automorphism groups of representation rings of the weak Sweedler Hopf algebras. AIMS Mathematics, 2022, 7(2): 2318-2330. doi: 10.3934/math.2022131
[8]	Junyong Zhao . On the number of unit solutions of cubic congruence modulo $ n $. AIMS Mathematics, 2021, 6(12): 13515-13524. doi: 10.3934/math.2021784
[9]	Fareeha Jamal, Muhammad Imran . Distance spectrum of some zero divisor graphs. AIMS Mathematics, 2024, 9(9): 23979-23996. doi: 10.3934/math.20241166
[10]	Nikken Prima Puspita, Indah Emilia Wijayanti . Bi-clean and clean Hopf modules. AIMS Mathematics, 2022, 7(10): 18784-18792. doi: 10.3934/math.20221033

Abstract

1. Introduction

Tensor categories should be thought as counterparts of rings in the world of categories ^[1,2,3], i.e., the categorification of groups and rings ^[4,5,6]. They are ubiquitous in noncommutative algebra and representation theory. Tensor categories were introduced by Bénabou ^[7] in 1963 and Lane ^[8] as "categories with multiplication", and its related theories are now widely used in many fields of mathematics, including algebraic geometry ^[9], algebraic topology ^[10], number theory ^[11], operator algebraic theory ^[12], etc. The theory of tensor categories is also seen as a development following from that of Hopf algebras and their representation theory ^[13,14]. As an important invariant in the theory of tensor categories, the concept of a $ \mathbb{Z}_+ $-ring can be traced back to Lusztig's work ^[15] in 1987. Later, in ^[16,17], the notion of a $ \mathbb{Z}_+ $-module over a $ \mathbb{Z}_+ $-ring was introduced. Module categories over multitensor categories were first considered in ^[4,18], and then the notion of an indecomposable module category was introduced in ^[17]. As a categorification of irreducible $ \mathbb{Z}_+ $-modules, it is interesting to classify indecomposable exact module categories over a given tensor category. In this process, it is often necessary to first classify all irreducible $ \mathbb{Z}_+ $-modules over the Grothendieck ring of a given tensor category.

Typical examples of $ \mathbb{Z}_+ $-rings are the representation rings of Hopf algebras ^{[19,20,21,22,23,24]}. Another example is the Grothendieck rings of tensor categories ^{[25,26,27,28]}. It is natural to consider the classification of all irreducible $ \mathbb{Z}_+ $-modules over them. For example, Etingof and Khovanov classified irreducible $ \mathbb{Z}_+ $-modules over the group ring $ \mathbb ZG $, and showed that indecomposable $ \mathbb{Z}_+ $-modules over the representation ring of $ {\rm SU}(2) $, under certain conditions, correspond to affine and infinite Dynkin diagrams ^[16]. Also, there is a lot of related research in the context of near-group fusion categories. For instance, Tambara and Yamagami classified semisimple tensor categories with fusion rules of self-duality for finite abelian groups. Evans, Gannon, and Izumi have contributed to the classification of the near-group $ C^{*} $-categories ^[29,30]. Yuan et al. ^[31] studied irreducible $ \mathbb{Z}_+ $-modules of the near-group fusion ring $ K\left(\mathbb Z_3, 3\right) $ and so on.

In this paper, we explore the problem of classifying irreducible based modules of rank up to 5 over the complex representation ring $ r(S_4) $, and then discuss their categorification. Furthermore, we overcome the technical difficulty of solving a series of non-negative integer equations using MATLAB. In contrast with the representation ring of $ S_3 $, $ r(S_n) $ is no longer a near-group fusion ring when $ n > 3 $, and the classification of irreducible $ \mathbb{Z}_+ $-modules over general $ r(S_n) $ seems to be a hopeless task. Hence, our paper attempts to classify irreducible based modules for the non-near-group fusion ring $ r(S_4) $. In fact, the fusion rule of $ r(S_n) $ is already a highly nontrivial open problem in combinatorics, namely counting the multiplicities of irreducible components of the tensor product of any two irreducible complex representations of $ S_n $ (so called the Kronecker coefficients).

The paper is organized as follows. In Section 2, we recall some basic definitions and propositions. In Section 3, we discuss the irreducible based modules of rank up to 5 over $ r(S_4) $ and give the classification of all these based modules (Propositions 3.1–3.5). In Section 4, we first show that any $ \mathbb{Z}_+ $-module over the representation ring $ r(G) $ of a finite group $ G $ categorified by a module category over the representation category $ {\rm Rep}(G) $ should be a based module (Theorem 4.2), and then determine which irreducible based modules over $ r(S_4) $ can be categorified (Theorem 4.12).

2. Preliminaries

Throughout this paper, all rings are assumed to be associative with unit 1. Let $ \mathbb{Z}_+ $ denote the set of nonnegative integers. First, we recall the definitions of $ \mathbb{Z}_+ $-rings and $ \mathbb{Z}_+ $-modules. For more details about these concepts, readers can refer to ^[17,32].

2.1. $ \mathbb{Z}_+ $-rings and $ \mathbb{Z}_+ $-modules

In this section, we first recall some definitions, and then we exhibit a class of identities for transposed Poisson $ n $-Lie algebras.

Definition 2.1. Let $ A $ be a ring which is free as a $ \mathbb{Z} $-module:

(i) A $ \mathbb{Z}_+ $-basis of $ A $ is a basis

$ B = \left \{ b_{i} \right \} _{i\in I}, $

such that

$ b_{i}b_{j} = \sum\limits_{k\in I} c_{ij}^{k} b_{k}, $

where $ c_{ij}^{k}\in \mathbb{Z}_+ $.

(ii) A $ \mathbb{Z}_+ $-ring is a ring with a fixed $ \mathbb{Z}_+ $-basis and with unit 1 being a non-negative linear combination of the basis elements.

(iii) A $ \mathbb{Z}_+ $-ring is unital if the unit $ 1 $ is one of its basis elements.

Definition 2.2. Let $ A $ be a $ \mathbb{Z}_+ $-ring with basis $ \left \{ b_{i} \right \}_{i\in I} $. A $ \mathbb{Z}_+ $-module over $ A $ is an $ A $-module $ M $ with a fixed $ \mathbb{Z} $-basis $ \left \{ m_{l} \right \} _{l\in L} $ such that all the structure constants $ a_{il}^{k} $, defined by the equality

$ b_{i} m_{l} = \sum\limits_{k} a_{il}^{k} m_{k} $

are non-negative integers.

A $ \mathbb{Z}_+ $-module has the following equivalent definition referring to [32, Section 3.4].

Definition 2.3. Let $ A $ be a $ \mathbb{Z}_+ $-ring with basis $ \left \{ b_{i} \right \}_{i\in I} $. A $ \mathbb{Z}_+ $-module $ M $ over $ A $ means an assignment where each basis $ b_{i} $ in $ A $ is in one-to-one correspondence with a non-negative integer square matrix $ M_i $ such that $ M $ forms a representation of $ A $:

$ M_{i} M_{j} = \sum\limits_{k\in I} c_{ij}^{k} M_{k}, \; \; \forall \space i, j, k\in I, $

where the unit of $ A $ corresponds to the identity matrix. The rank of a $ \mathbb{Z}_+ $-module $ M $ is equal to the order of the matrix $ M_{i} $.

Definition 2.4. (i) Two $ \mathbb{Z}_+ $-modules $ M_{1}, \, M_{2} $ over $ A $ with bases $ {\left \{ m_{i}^{1} \right \} }_{i\in L_{1}}, {\left \{ m_{j}^{2} \right \} }_{j\in L_{2}} $ are equivalent if and only if there exists a bijection $ \phi $: $ L_{1}\to L_{2} $ such that the induced $ \mathbb{Z} $-linear map $ \tilde{\phi} $ of abelian groups $ M_{1}, \, M_{2} $ defined by

$ \tilde{\phi} (m_{i}^{1} ) = m_{{\phi} (i)}^{2} $

is an isomorphism of $ A $-modules. In other words, for $ a\in A $, let $ a_{M_{1} } $ and $ a_{M_{2} } $ be the matrices with respect to the bases $ {\left \{ m_{i}^{1} \right \} }_{i\in L_{1}} $ and $ {\left \{ m_{j}^{2} \right \} }_{j\in L_{2}} $, respectively. Then, two $ \mathbb{Z}_+ $-modules $ M_{1} $, $ M_{2} $ of rank $ n $ are equivalent if and only if there exists an $ n\times n $ permutation matrix $ P $ such that

$ a_{M_{2}} = Pa_{M_{1}}P^{-1}, \; \; \; \forall \space a\in A. $

(ii) The direct sum of two $ \mathbb{Z}_+ $-modules $ M_{1}, \, M_{2} $ over $ A $ is the module $ M_{1}\oplus M_{2} $ over $ A $ whose basis is the union of the bases of $ M_{1} $ and $ M_{2} $.

(iii) A $ \mathbb{Z}_+ $-module $ M $ over $ A $ is indecomposable if it is not equivalent to a nontrivial direct sum of $ \mathbb{Z}_+ $-modules.

(iv) A $ \mathbb{Z}_+ $-submodule of a $ \mathbb{Z}_+ $-module $ M $ over $ A $ with basis $ \left \{ m_{l} \right \}_{l\in L} $ is a subset $ J\subset L $ such that the abelian subgroup of $ M $ generated by $ {\left \{ m_{j} \right \} }_{j\in J} $ is an $ A $-submodule.

(v) A $ \mathbb{Z}_+ $-module $ M $ over $ A $ is irreducible if any $ \mathbb{Z}_+ $-submodule of $ M $ is 0 or $ M $. In other words, the $ \mathbb{Z} $-span of any proper subset of the basis of $ M $ is not an $ A $-submodule.

2.2. Based rings and based modules

Let $ A $ be a $ \mathbb{Z}_+ $-ring with basis $ \left \{ b_{i} \right \}_{i\in I} $, and let $ I_{0} $ be the set of $ i\in I $ such that $ b_{i} $ occurs in the decomposition of 1. Let $ \tau $: $ A\to \mathbb Z $ denote the group homomorphism defined by

$ \tau (b_{i} ) =

$\begin{cases}1, & \text{ if } \ i\in I_{0} , \\0, & \text{ if } \ i\notin I_{0} .\end{cases}$ $

Definition 2.5. A $ \mathbb{Z}_+ $-ring with basis $ \left \{ b_{i} \right \}_{i\in I} $ is called a based ring if there exists an involution $ i \mapsto i^{*} $ of $ I $ such that the induced map

$ a = \sum\limits_{i\in I} a_{i} b_{i}\mapsto {}a^{*} = \sum\limits_{i\in I} a_{i} b_{i^*}, \; \; \; a_{i}\in \mathbb{Z}, $

is an anti-involution of the ring $ A $, and

$ \tau (b_{i}b_{j} ) =

$\begin{cases}1, & { if } \ i = j^{*} , \\0, & { if } \ i\ne j^{*} .\end{cases}$ $

A fusion ring is a unital based ring of finite rank.

Definition 2.6. A based module over a based ring $ A $ with basis $ \left \{ b_{i} \right \}_{i\in I} $ is a $ \mathbb{Z}_+ $-module $ M $ with basis $ \left \{ m_{l} \right \}_{l\in L} $ over $ A $ such that

$ a_{il}^{k} = a_{i^{\ast } k}^{l} , $

where $ a_{il}^{k} $ are defined as in Definition 2.2.

Let $ A $ be a unital $ \mathbb{Z}_+ $-ring of finite rank with basis $ \left \{ b_{i} \right \}_{i\in I} $, and let $ M $ be a $ \mathbb{Z}_+ $-module over $ A $ with $ \mathbb{Z} $-basis $ \left \{ m_{l} \right \} _{l\in L} $. Take

$ b = \sum\limits_{i\in I}b_i. $

For any fixed $ m_{l_0} $, the $ \mathbb{Z}_+ $-submodule of $ M $ generated by $ m_{l_0} $ is the $ \mathbb{Z} $-span of $ \left \{m_k\right\}_{k\in Y} $, where the set $ Y $ consists of $ k\in L $ such that $ m_k $ is a summand of $ bm_{l_0} $. Also, we need the following facts.

Proposition 2.1. [32, Proposition 3.4.6] Let $ A $ be a based ring of finite rank over $ \mathbb{Z} $. Then there exist only finitely many irreducible $ \mathbb{Z}_+ $-modules over $ A $.

Proposition 2.2. [17, Lemma 2.1] Let $ M $ be a based module over a based ring $ A $. If $ M $ is decomposable as a $ \mathbb{Z}_+ $-module over $ A $, then $ M $ is irreducible as a $ \mathbb{Z}_+ $-module over $ A $.

As a result, any $ \mathbb{Z}_+ $-module of finite rank over a fusion ring is completely reducible, and then only irreducible $ \mathbb{Z}_+ $-modules need to be classified.

In general, the rank of an irreducible $ \mathbb{Z}_+ $-module over a fusion ring $ A $ may be larger than the rank of $ A $; e.g., $ A = r(D_5) $ for the dihedral group $ D_5 $ ([33, Remark 1]). In this paper, we explore which irreducible based modules over $ r(S_4) $ can be categorified by indecomposable exact module categories over the representation category $ {\rm Rep}(S_4) $. Since all these module categories are of rank not greater than 5, we only deal with based modules of rank up to 5 correspondingly.

3. Irreducible based modules over $ r(S_4) $

In this section, we will classify the irreducible based modules over the complex representation ring $ r(S_4) $ of $ S_4 $ up to equivalence. $ r(S_4) $ is a commutative fusion ring having a $ \mathbb{Z}_+ $-basis $ \left \{ 1, V_{\psi}, V_{\rho _{1}}, V_{\rho _{2}}, V_{\rho _{3}} \right \} $ with the fusion rule.

$\begin{equation} \begin{array}{c} V_{\psi}^2 = 1, \quad V_{\psi}V_{\rho _{1}} = V_{\rho _{1}}, \quad V_{\psi}V_{\rho _{2}} = V_{\rho _{3}}, \quad V_{\rho _{1}}^2 = 1+V_{\psi}+V_{\rho _{1}}, \\[.5em] V_{\rho_1 }V_{\rho_2 } = V_{\rho_2 }+V_{\rho_3}, \quad V_{\rho _{2}}^2 = 1+ V_{\rho _{1}}+ V_{\rho _{2}}+ V_{\rho _{3}}, \end{array} \end{equation}$ $

(3.1)

where $ 1 $, $ V_{\psi} $, and $ V_{\rho _{1}} $ denote the trivial representation, sign representation, and 2-dimensional irreducible representation, respectively, while $ V_{\rho_2} $ stands for the 3-dimensional standard representation and $ V_{\rho_3} $ denotes its conjugate representation. Then we have the following Table 1.

Table 1. The complex character table of $ S_4 $.

	$ (1) $	$ (12) $	$ (123) $	$ (1234) $	$ (12)(34) $
$ \chi_1 $	$ 1 $	$ 1 $	$ 1 $	$ 1 $	$ 1 $
$ \chi_\psi $	$ 1 $	$ -1 $	$ 1 $	$ -1 $	$ 1 $
$ \chi_{\rho_1} $	$ 2 $	$ 0 $	$ -1 $	$ 0 $	$ 2 $
$ \chi_{\rho_2} $	$ 3 $	$ 1 $	$ 0 $	$ -1 $	$ -1 $
$ \chi_{\rho_3} $	$ 3 $	$ -1 $	$ 0 $	$ 1 $	$ -1 $

| Show Table

DownLoad: CSV

Let $ M $ be a based module of $ r(S_4) $ with the basis $ \left \{ m_{l} \right \} _{l\in L} $. Let $ T, \, Q, \, U $, and $ W $ be the matrices representing the action of $ V_{\psi}, \, V_{\rho _{1}}, \, V_{\rho _{2}} $, and $ V_{\rho _{3}} $ on $ M $ respectively. They are all symmetric matrices with nonnegative integer entries by Definition 2.6. Let $ E $ be the identity matrix. By the fusion rule of $ r(S_4) $, we have

$\begin{align} T^2& = E, \end{align}$ $

(3.2)

$\begin{align} TQ& = QT = Q, \end{align}$ $

(3.3)

$\begin{align} TU& = UT = W, \end{align}$ $

(3.4)

$\begin{align} Q^2& = E+T+Q, \end{align}$ $

(3.5)

$\begin{align} QU& = U+TU, \end{align}$ $

(3.6)

$\begin{align} U^2& = E+Q+U+TU. \end{align}$ $

(3.7)

In particular, since $ T^2 = E $ and $ T $ has nonnegative integer entries, we know that $ T $ is a symmetric permutation matrix.

Convention 3.1. Let $ P_n $ be the group of $ n\times n $ permutation matrices. Since there is naturally a group isomorphism between $ S_n $ and $ P_n $, we will use the cycle notation of permutations to represent permutation matrices.

3.1. Irreducible based modules of rank $ \le 3 $ over $ r(S_4) $

We define a $ \mathbb{Z}_+ $-module $ M_{1, 1} $ of rank 1 over $ r(S_4) $ by letting

$\begin{equation} V_{\psi} \mapsto 1, \quad V_{\rho _{1}}\mapsto 2, \quad V_{\rho _{2}}\mapsto 3, \quad V_{\rho _{3}}\mapsto 3. \end{equation}$ $

(3.8)

Proposition 3.1. Any irreducible based module of rank $ 1 $ over $ r(S_4) $ is equivalent to $ M_{1, 1} $.

Proof. Note that any integral fusion ring $ A $ has the unique character $ {\rm FPdim} $: $ A\to \mathbb{Z} $, which takes non-negative values on the $ \mathbb{Z}_+ $-basis, so there exists a unique $ \mathbb{Z}_+ $-module $ M $ of rank 1 over it. Clearly, such $ M $ is a based module. Now this argument is available for the situation $ A = r(S_4) $. □

Next, we consider irreducible based modules of rank $ 2, 3 $. According to the fusion rule of $ r(S_4) $ given in (3.1), it is sufficient to only list the representation matrices of $ V_{\psi} $, $ V_{\rho _{1}} $, and $ V_{\rho _{2}} $ acting on them. For simplicity, we choose to present our result for the cases of small rank 2 and 3 directly, and then analyze the cases of higher rank 4 and 5 with details.

Proposition 3.2. Let $ M $ be an irreducible based module of rank $ 2 $ over $ r(S_4) $. Then $ M $ is equivalent to one of the based modules $ M_{2, i}, \, 1\leq i\leq 3 $, listed in Table 2.

Table 2. Inequivalent irreducible based modules of rank 2 over $ r(S_4) $.

	$ V_{\psi} $	$ V_{\rho _{1}} $	$ V_{\rho _{2}} $
$ M_{2, 1} $	$ $\begin{pmatrix} 1&0\\0&1\end{pmatrix}$ $	$ $\begin{pmatrix} 2&0\\0&2\end{pmatrix}$ $	$ $\begin{pmatrix} 1&2\\2&1\end{pmatrix}$ $
$ M_{2, 2} $	$ $\begin{pmatrix} 0&1\\1&0\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1\\1&1\end{pmatrix}$ $	$ $\begin{pmatrix} 2&1\\1&2\end{pmatrix}$ $
$ M_{2, 3} $	$ $\begin{pmatrix} 0&1\\1&0\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1\\1&1\end{pmatrix}$ $	$ $\begin{pmatrix} 1&2\\2&1\end{pmatrix}$ $

| Show Table

DownLoad: CSV

Proposition 3.3. Let $ M $ be an irreducible based module of rank $ 3 $ over $ r(S_4) $. Then $ M $ is equivalent to one of the based modules $ M_{3, i}, \, 1\leq i\leq 3 $, listed in Table 3.

Table 3. Inequivalent irreducible based modules of rank 3 over $ r(S_4) $.

	$ V_{\psi} $	$ V_{\rho _{1}} $	$ V_{\rho _{2}} $
$ M_{3, 1} $	$ $\begin{pmatrix} 1&0&0\\0&1&0\\0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1\\1&0&1\\1&1&0\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&1\\1&1&1\\1&1&1\\\end{pmatrix}$ $
$ M_{3, 2} $	$ $\begin{pmatrix} 0&1&0\\1&0&0\\0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&1\\0&0&1\\1&1&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&0&1\\0&1&1\\1&1&2\\\end{pmatrix}$ $
$ M_{3, 3} $	$ $\begin{pmatrix} 0&1&0\\1&0&0\\0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&1\\0&0&1\\1&1&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1\\1&0&1\\1&1&2\\\end{pmatrix}$ $

| Show Table

DownLoad: CSV

3.2. Irreducible based modules of rank $ 4, \, 5 $ over $ r(S_4) $

Proposition 3.4. Let $ M $ be an irreducible based module of rank $ 4 $ over $ r(S_4) $. Then $ M $ is equivalent to one of the based modules $ M_{4, i}, \, 1\leq i\leq 7 $, listed in Table 4.

Table 4. Inequivalent irreducible based modules of rank 4 over $ r(S_4) $.

	$ V_{\psi} $	$ V_{\rho _{1}} $	$ V_{\rho _{2}} $
$ M_{4, 1} $	$ $\begin{pmatrix} 1&0&0&0\\0&1&0&0\\0&0&1&0\\0&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&1&1\\0&2&0&0\\1&0&0&1\\1&0&1&0\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&0&0\\1&2&1&1\\0&1&0&0\\0&1&0&0\\\end{pmatrix}$ $
$ M_{4, 2} $	$ $\begin{pmatrix} 1&0&0&0\\0&1&0&0\\0&0&1&0\\0&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 2&0&0&0\\0&2&0&0\\0&0&2&0\\0&0&0&2\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1&1\\1&0&1&1\\1&1&0&1\\1&1&1&0\\\end{pmatrix}$ $
$ M_{4, 3} $	$ $\begin{pmatrix} 0&1&0&0\\1&0&0&0\\0&0&1&0\\0&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0\\1&1&0&0\\0&0&2&0\\0&0&0&2\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&0&1&1\\0&1&1&1\\1&1&0&1\\1&1&1&0\\\end{pmatrix}$ $
$ M_{4, 4} $	$ $\begin{pmatrix} 0&1&0&0\\1&0&0&0\\0&0&1&0\\0&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0\\1&1&0&0\\0&0&2&0\\0&0&0&2\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1&1\\1&0&1&1\\1&1&0&1\\1&1&1&0\\\end{pmatrix}$ $
$M_{4, 5} $	$ $\begin{pmatrix} 0&1&0&0\\1&0&0&0\\0&0&0&1\\0&0&1&0\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0\\1&1&0&0\\0&0&1&1\\0&0&1&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1&1\\1&0&1&1\\1&1&0&1\\1&1&1&0\\\end{pmatrix}$ $
$ M_{4, 6} $	$ $\begin{pmatrix} 0&1&0&0\\1&0&0&0\\0&0&0&1\\0&0&1&0\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0\\1&1&0&0\\0&0&1&1\\0&0&1&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1&1\\1&0&1&1\\1&1&1&0\\1&1&0&1\\\end{pmatrix}$ $
$ M_{4, 7} $	$ $\begin{pmatrix} 0&1&0&0\\1&0&0&0\\0&0&0&1\\0&0&1&0\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0\\1&1&0&0\\0&0&1&1\\0&0&1&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&0&1&1\\0&1&1&1\\1&1&1&0\\1&1&0&1\\\end{pmatrix}$ $

| Show Table

DownLoad: CSV

Proof. Before giving its detailed proof, we provide the following proof outline first.

$ ({\rm{i}}) $ The symmetric group $ S_4 $ has 3 conjugacy classes of permutations of order $ \le 2 $, so there are 3 representatives for matrix $ T $ up to conjugation as follows:

$\begin{equation*} T_1 = E_4, \quad T_2 = (12), \quad T_3 = (12)(34). \end{equation*}$ $

Consequently, we can take $ T = T_r $ for some $ r = 1, 2, 3 $ as the representation matrix of $ V_\psi $ for the based module $ M $ up to equivalence.

$ ({\rm{ii}}) $ Use MATLAB to search all solutions of the representation matrices $ Q $ and $ U $ in the group of nonnegative integer matrix Eqs (3.3)–(3.7) by constraint satisfaction.

$ ({\rm{iii}}) $ Distinguish all conjugacy classes of tuples $ (T, Q, U) $ without simultaneous block decomposition. They correspond to the equivalence classes of irreducible based modules over $ r(S_4) $.

□

Proof. Let $ M $ be a based module of rank 4 over $ r(S_4) $, with the action of $ r(S_4) $ on it given by

$\begin{equation} V_{\psi} \mapsto T, \quad V_{\rho _{1}}\mapsto Q = (a_{ij})_{1\leq i, j\leq4} , \quad V_{\rho _{2}}\mapsto U = (b_{ij})_{1\leq i, j\leq4}, \quad V_{\rho _{3}}\mapsto W = TU, \nonumber \end{equation}$ $

where $ a_{ij} = a_{ji} $, $ b_{ij} = b_{ji} $.

The symmetric group $ S_4 $ has two conjugacy classes of permutations of order 2. One conjugacy class of 6 permutations includes $ (12) $, and the other one of 3 permutations includes $ (12)(34) $. As previously seen, $ T $ is the unit or an element of order 2 in $ P_4 $, so we have 10 candidates for $ T $, and each of them is conjugate to one of the following 3 matrices:

$\begin{equation*} T_1 = E_4, \quad T_2 = (12) , \quad T_3 = (12)(34) . \end{equation*}$ $

Hence, for the based module $ M $ determined by the pair $ (T, Q, U) $, there exists a $ 4\times 4 $ permutation matrix $ P $ such that

$ T' = PTP^{-1} $

is one of the above $ T_r $'s $ (1\leq r\leq 3) $. Correspondingly, let

$ Q' = PQP^{-1} , \; \; \; U' = PUP^{-1}. $

Then we get a based module $ M' $ determined by the pair $ (T', Q', U') $ and equivalent to $ M $ as based modules by Definition 2.4 (ⅰ). So, we have reduced the proof to the situation when $ T = T_r $.

Case 1. $ T = T_1 = E_4 $.

Since $ Q $ satisfies Eq (3.5), we obtain the following system of integer equations:

$\begin{equation*} \left\{\begin{array}{l} a_{11}^2+a_{12}^2+a_{13}^2+a_{14}^2 = 2+a_{11}, \\ a_{11}a_{12}+a_{12}a_{22}+a_{13}a_{23}+a_{14}a_{24} = a_{12}, \\ a_{11}a_{13}+a_{12}a_{23}+a_{13}a_{33}+a_{14}a_{34} = a_{13}, \\ a_{11}a_{14}+a_{12}a_{24}+a_{13}a_{34}+a_{14}a_{44} = a_{14}, \\ a_{12}^2+a_{22}^2+a_{23}^2+a_{24}^2 = 2+a_{22}, \\ a_{12}a_{13}+a_{22}a_{23}+a_{23}a_{33}+a_{24}a_{34} = a_{23}, \\ a_{12}a_{14}+a_{22}a_{24}+a_{23}a_{34}+a_{24}a_{44} = a_{24}, \\ a_{13}^2+a_{23}^2+a_{33}^2+a_{34}^2 = 2+a_{33}, \\ a_{13}a_{14}+a_{23}a_{24}+a_{33}a_{34}+a_{34}a_{44} = a_{34}, \\ a_{14}^2+a_{24}^2+a_{34}^2+a_{44}^2 = 2+a_{44}. \end{array}\right. \end{equation*}$ $

We use MATLAB to figure out all the solutions of $ Q $ as follows:

$\begin{array}{l} Q_1 = \begin{pmatrix} 0&0&1&1 \\ 0&2&0&0 \\ 1&0&0&1 \\ 1&0&1&0 \end{pmatrix}, \quad Q_2 = \begin{pmatrix} 0&1&0&1 \\ 1&0&0&1 \\ 0&0&2&0 \\ 1&1&0&0 \end{pmatrix}, \quad Q_3 = \begin{pmatrix} 0&1&1&0 \\ 1&0&1&0 \\ 1&1&0&0 \\ 0&0&0&2 \end{pmatrix}, \\[2em] Q_4 = \begin{pmatrix} 2&0&0&0 \\ 0&0&1&1 \\ 0&1&0&1 \\ 0&1&1&0 \end{pmatrix}, \quad Q_5 = \begin{pmatrix} 2&0&0&0 \\ 0&2&0&0 \\ 0&0&2&0 \\ 0&0&0&2 \end{pmatrix}. \end{array}$ $

Next, we calculate $ U $ after taking $ Q $ as one $ Q_k $ $ \left (1\le k\le5 \right) $.

Case 1.1. $ Q = Q_1 $.

Since $ U $ satisfies Eq (3.6), we get

$\begin{equation*} \begin{split} b_{12}& = b_{23} = b_{24}, \\ b_{11}& = b_{13} = b_{14} = b_{33} = b_{34} = b_{44}. \end{split} \end{equation*}$ $

Then, by Eq (3.7), we have

$\begin{equation*} \left\{\begin{array}{l} 3b_{11}^2+b_{12}^2 = 2b_{11}+1, \\ 3b_{11}b_{12}+b_{12}b_{22} = 2b_{12}, \\ 3b_{12}^2+b_{22}^2 = 2b_{22}+3. \end{array}\right. \end{equation*}$ $

The solutions of $ U $ given by MATLAB are as follows:

$\begin{equation*} U_1 = \begin{pmatrix} 0&1&0&0 \\ 1&2&1&1 \\ 0&1&0&0 \\ 0&1&0&0 \end{pmatrix}, \quad U_2 = \begin{pmatrix} 1&0&1&1 \\ 0&3&0&0 \\ 1&0&1&1 \\ 1&0&1&1 \end{pmatrix}. \end{equation*}$ $

It is easy to check that the based module determined by $ \left (T_1, Q_1, U_1\right) $ is an irreducible based module denoted as $ M_{4, 1} $, while the based module determined by $ \left (T_1, Q_1, U_2\right) $ is reducible.

Note that there exists a permutation matrix $ P = (14)(23) $ such that

$ PQ_1P^{-1} = Q_2. $

Let

$ U'_1 = PU_1P^{-1}. $

There is an irreducible based module $ N' $ determined by the pair $ \big (T_1, Q_2, U'_1\big) $ and equivalent to $ M_{4, 1} $ by Definition 2.4 (ⅰ). Conversely, any irreducible based module with representation matrices $ T_1 $ and $ Q_2 $ is equivalent to $ M_{4, 1} $. The same analysis tells us that irreducible based modules with representation matrices $ T_1 $ and $ Q_3 $ (or $ Q_4 $) are also equivalent to $ M_{4, 1} $.

Case 1.2. $ Q = Q_5 $.

Since $ U $ satisfies Eqs (3.6) and (3.7), we get a system of integer equations as follows:

$\begin{equation*} \left\{\begin{array}{l} b_{11}^2+b_{12}^2+b_{13}^2+b_{14}^2 = 2b_{11}+3, \\ b_{11}b_{12}+b_{12}b_{22}+b_{13}b_{23}+b_{14}b_{24} = 2b_{12}, \\ b_{11}b_{13}+b_{12}b_{23}+b_{13}b_{33}+b_{14}b_{34} = 2b_{13}, \\ b_{11}b_{14}+b_{12}b_{24}+b_{13}b_{34}+b_{14}b_{44} = 2b_{14}, \\ b_{12}^2+b_{22}^2+b_{23}^2+b_{24}^2 = 2b_{22}+3, \\ b_{12}b_{13}+b_{22}b_{23}+b_{23}b_{33}+b_{24}b_{34} = 2b_{23}, \\ b_{12}b_{14}+b_{22}b_{24}+b_{23}b_{34}+b_{24}b_{44} = 2b_{24}, \\ b_{13}^2+b_{23}^2+b_{33}^2+b_{34}^2 = 2b_{33}+3, \\ b_{13}b_{14}+b_{23}b_{24}+b_{33}b_{34}+b_{34}b_{44} = 2b_{34}, \\ b_{14}^2+b_{24}^2+b_{34}^2+b_{44}^2 = 2b_{44}+3. \\ \end{array}\right. \end{equation*}$ $

Thus, the solutions of $ U $ by MATLAB are as follows:

$\begin{align*} &U_1 = \begin{pmatrix} 0&1&1&1 \\ 1&0&1&1 \\ 1&1&0&1 \\ 1&1&1&0\end{pmatrix}, \quad U_2 = \begin{pmatrix} 1&0&0&2 \\ 0&1&2&0 \\ 0&2&1&0 \\ 2&0&0&1\end{pmatrix}, \quad U_3 = \begin{pmatrix}1&0&2&0 \\ 0&1&0&2 \\ 2&0&1&0 \\ 0&2&0&1\end{pmatrix}, \quad U_4 = \begin{pmatrix}1&2&0&0 \\ 2&1&0&0 \\ 0&0&1&2 \\ 0&0&2&1\end{pmatrix}, \\[2em] &U_5 = \begin{pmatrix} 1&0&2&0 \\ 0&3&0&0 \\ 2&0&1&0 \\ 0&0&0&3\end{pmatrix}, \quad U_6 = \begin{pmatrix} 1&0&0&2 \\ 0&3&0&0 \\ 0&0&3&0 \\ 2&0&0&1\end{pmatrix}, \quad U_7 = \begin{pmatrix}1&2&0&0 \\ 2&1&0&0 \\ 0&0&3&0 \\ 0&0&0&3\end{pmatrix}, \quad U_8 = \begin{pmatrix}3&0&0&0 \\ 0&3&0&0 \\ 0&0&1&2 \\ 0&0&2&1\end{pmatrix}, \\ &U_9 = \begin{pmatrix} 3&0&0&0 \\ 0&1&2&0 \\ 0&2&1&0 \\ 0&0&0&3\end{pmatrix}, \quad U_{10} = \begin{pmatrix} 3&0&0&0 \\ 0&1&0&2 \\ 0&0&3&0 \\ 0&2&0&1\end{pmatrix}, \quad U_{11} = \begin{pmatrix}3&0&0&0 \\ 0&3&0&0 \\ 0&0&3&0 \\ 0&0&0&3\end{pmatrix}. \end{align*}$ $

Since $ T_1 $ and $ Q_5 $ are diagonal and the solutions $ U_t $ $ \left (2\le t\le11 \right) $ are block diagonal with at least two blocks, only the based module determined by $ (T_1, Q_5, U_1) $ is irreducible, denoted as $ M_{4, 2} $.

Case 2. $ T = T_2 = (12) $.

Since $ Q $ satisfies Eq (3.3), we get

$\begin{equation*} Q = \begin{pmatrix} a_{11}&a_{11}&a_{13}&a_{14} \\ a_{11}&a_{11}&a_{13}&a_{14}\\ a_{13}&a_{13}&a_{33}&a_{34} \\ a_{14}&a_{14}&a_{34}&a_{44} \end{pmatrix}. \end{equation*}$ $

Since $ Q $ also satisfies Eq (3.5), we have the following system of integer equations:

$\begin{equation*} \left\{\begin{array}{l} 2a_{11}^2+a_{13}^2+a_{14}^2 = a_{11}+1, \\ 2a_{11}a_{13}+a_{13}a_{33}+a_{14}a_{34} = a_{13}, \\ 2a_{11}a_{14}+a_{13}a_{34}+a_{14}a_{44} = a_{14}, \\ 2a_{13}^2+a_{33}^2+a_{34}^2 = a_{33}+2, \\ 2a_{13}a_{14}+a_{33}a_{34}+a_{34}a_{44} = a_{34}, \\ 2a_{14}^2+a_{34}^2+a_{44}^2 = a_{44}+2. \\ \end{array}\right. \end{equation*}$ $

Hence, the solutions of $ Q $ by MATLAB are as follows:

$\begin{array}{l} Q_1 = \begin{pmatrix} 0&0&0&1 \\ 0&0&0&1 \\ 0&0&2&0 \\ 1&1&0&1\end{pmatrix}, \quad Q_2 = \begin{pmatrix} 0&0&1&0 \\ 0&0&1&0 \\ 1&1&1&0 \\ 0&0&0&2\end{pmatrix}, \quad Q_3 = \begin{pmatrix}1&1&0&0 \\ 1&1&0&0 \\ 0&0&2&0 \\ 0&0&0&2\end{pmatrix}. \end{array}$ $

Since $ U $ satisfies Eq (3.4), we get

$\begin{equation*} U = \begin{pmatrix} b_{11}&b_{12}&b_{13}&b_{14}\\ b_{12}&b_{11}&b_{13}&b_{14}\\ b_{13}&b_{13}&b_{33}&b_{34} \\ b_{14}&b_{14}&b_{34}&b_{44} \end{pmatrix}. \end{equation*}$ $

Next, we calculate $ U $ after taking $ Q $ as one $ Q_k $ $ \left (1\le k\le3 \right) $.

Case 2.1. $ Q = Q_1 $.

Since $ U $ satisfies Eqs (3.6) and (3.7), the solutions of $ U $ given by MATLAB are as follows:

$\begin{array}{l} U_1 = \begin{pmatrix} 0&1&0&1 \\ 1&0&0&1 \\ 0&0&3&0 \\ 1&1&0&2\end{pmatrix}, \quad U_2 = \begin{pmatrix} 1&0&0&1 \\ 0&1&0&1 \\ 0&0&3&0 \\ 1&1&0&2\end{pmatrix}. \end{array}$ $

Since $ T_2 $, $ Q_1 $ and all the solutions $ U_t $ for $ t = 1, 2 $ are block diagonal with at least two blocks, the based modules determined by each pair $ (T_2, Q_1, U_t) $ are reducible.

Note that there exists a permutation matrix $ P = (12)(34) $ such that

$ PQ_1P^{-1} = Q_2. $

Let

$ U'_t = PU_tP^{-1}. $

Then each based module $ N_t $ determined by the pair $ \left (T_2, Q_2, U'_t\right) $ is reducible. Namely, any based module with representation matrices $ T_2 $ and $ Q_2 $ is reducible.

Case 2.2. $ Q = Q_3 $.

Since $ U $ satisfies Eqs (3.6) and (3.7), we have

$\begin{array}{l} U_1 = \begin{pmatrix} 0&1&1&1 \\ 1&0&1&1 \\ 1&1&0&1 \\ 1&1&1&0\end{pmatrix}, \quad U_2 = \begin{pmatrix} 1&0&1&1 \\ 0&1&1&1 \\ 1&1&0&1 \\ 1&1&1&0\end{pmatrix}, \quad U_3 = \begin{pmatrix}1&2&0&0 \\ 2&1&0&0 \\ 0&0&1&2 \\ 0&0&2&1\end{pmatrix}, \\[2em] U_4 = \begin{pmatrix}1&2&0&0 \\ 2&1&0&0 \\ 0&0&3&0 \\ 0&0&0&3\end{pmatrix}, \quad U_5 = \begin{pmatrix} 2&1&0&0 \\ 1&2&0&0 \\ 0&0&1&2 \\ 0&0&2&1\end{pmatrix}, \quad U_6 = \begin{pmatrix} 2&1&0&0 \\ 1&2&0&0 \\ 0&0&3&0 \\ 0&0&0&3\end{pmatrix}. \end{array}$ $

Since $ T_2 $, $ Q_3 $ and the solutions $ U_s $ $ (3\le s\le 6) $ are block diagonal with at least two blocks, only the based module determined by $ (T_2, Q_3, U_1) $ and $ (T_2, Q_3, U_2) $ are irreducible, denoted as $ M_{4, 3} $ and $ M_{4, 4} $, respectively. It is easy to check that $ M_{4, 3} $ and $ M_{4, 4} $ are inequivalent based modules.

Case 3. $ T = T_3 = (12)(34) $.

Since $ Q $ satisfies Eq (3.3), we get

$\begin{equation*} Q = \begin{pmatrix} a_{11}&a_{11}&a_{13}&a_{13} \\ a_{11}&a_{11}&a_{13}&a_{13}\\ a_{13}&a_{13}&a_{33}&a_{33} \\ a_{13}&a_{13}&a_{33}&a_{33} \end{pmatrix}. \end{equation*}$ $

Then, by Eq (3.5), we have the following system of integer equations:

$\begin{equation*} \left\{\begin{array}{l} 2a_{11}^2+2a_{13}^2 = a_{11}+1, \\ 2a_{11}a_{13}+2a_{13}a_{33} = a_{13}, \\ 2a_{13}^2+2a_{33}^2 = a_{33}+1. \end{array}\right. \end{equation*}$ $

$ Q $ has the following unique solution:

$\begin{equation*} Q_1 = \begin{pmatrix} 1&1&0&0\\ 1&1&0&0\\ 0&0&1&1\\ 0&0&1&1 \end{pmatrix}. \end{equation*}$ $

Since $ U $ satisfies Eq (3.4), we get

$\begin{equation*} U = \begin{pmatrix} b_{11}&b_{12}&b_{13}&b_{14}\\ b_{12}&b_{11}&b_{14}&b_{13}\\ b_{13}&b_{14}&b_{33}&b_{34} \\ b_{14}&b_{13}&b_{34}&b_{33} \end{pmatrix}. \end{equation*}$ $

Since $ U $ also satisfies Eqs (3.6) and (3.7), we obtain the solutions of $ U $ by MATLAB as follows:

$\begin{array}{l} U_1 = \begin{pmatrix} 0&1&1&1 \\ 1&0&1&1 \\ 1&1&0&1 \\ 1&1&1&0\end{pmatrix}, \quad U_2 = \begin{pmatrix} 0&1&1&1 \\ 1&0&1&1 \\ 1&1&1&0 \\ 1&1&0&1\end{pmatrix}, \quad U_3 = \begin{pmatrix}1&0&1&1 \\ 0&1&1&1 \\ 1&1&1&0 \\ 1&1&0&1\end{pmatrix}, \quad U_4 = \begin{pmatrix}1&0&1&1\\ 0&1&1&1 \\ 1&1&0&1 \\ 1&1&1&0\end{pmatrix}, \\[2em] U_5 = \begin{pmatrix} 1&2&0&0 \\ 2&1&0&0 \\ 0&0&1&2 \\ 0&0&2&1\end{pmatrix}, \quad U_6 = \begin{pmatrix} 1&2&0&0 \\ 2&1&0&0 \\ 0&0&2&1 \\ 0&0&1&2\end{pmatrix}, \quad U_7 = \begin{pmatrix}2&1&0&0 \\ 1&2&0&0 \\ 0&0&1&2 \\ 0&0&2&1\end{pmatrix}, \quad U_8 = \begin{pmatrix}2&1&0&0 \\ 1&2&0&0 \\ 0&0&2&1 \\ 0&0&1&2\end{pmatrix}. \end{array}$ $

Clearly, $ T_3 $, $ Q_1 $ and the solutions $ U_s $ are block diagonal with at least two blocks, but the based module determined by the pair $ \left (T_3, Q_1, U_t\right) $ is irreducible, denoted as $ M_{4, s} $, where $ 5\le s \le8, 1\le t\le4 $. Define the $ \mathbb Z $-module isomorphism $ \phi $: $ M_{4, 6}\to M_{4, 8} $ by

$\begin{equation*} \phi(v_1^1) = v_4^2, \quad \phi(v_2^1) = v_3^2, \quad \phi(v_3^1) = v_2^2, \quad\phi(v_4^1) = v_1^2. \end{equation*}$ $

It is easy to see that $ M_{4, 6} $ is equivalent to $ M_{4, 8} $ as based modules over $ r(S_4) $ under $ \phi $. Then, we can check that $ \left \{ M_{4, s} \right \} _{5\le s \le7} $ are inequivalent irreducible based modules. □

Finally, we construct two based modules $ M_{5, i}\ \left (i = 1, 2 \right) $ over $ r(S_4) $ with the actions of $ r(S_4) $ on them presented in Table 5.

Table 5. Inequivalent irreducible based modules of rank 5 over $ r(S_4) $.

	$ V_{\psi} $	$ V_{\rho _{1}} $	$ V_{\rho _{2}} $
$ M_{5, 1} $	$ $\begin{pmatrix} 0&1&0&0&0\\ 1&0&0&0&0\\ 0&0&0&1&0\\ 0&0&1&0&0\\ 0&0&0&0&1\\ \end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&0&0&1\\ 0&0&0&0&1\\ 0&0&1&1&0\\ 0&0&1&1&0\\ 1&1&0&0&1\\ \end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&0&1&0\\ 0&0&1&0&0\\ 0&1&1&1&1\\ 1&0&1&1&1\\ 0&0&1&1&0\\ \end{pmatrix}$ $
$ M_{5, 2} $	$ $\begin{pmatrix} 0&1&0&0&0\\ 1&0&0&0&0\\ 0&0&0&1&0\\ 0&0&1&0&0\\ 0&0&0&0&1\\ \end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0&0\\ 1&1&0&0&0\\ 0&0&1&1&0\\ 0&0&1&1&0\\ 0&0&0&0&2\\ \end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&0&1&1\\ 0&0&1&0&1\\ 0&1&0&0&1\\ 1&0&0&0&1\\ 1&1&1&1&1\\ \end{pmatrix}$ $

| Show Table

DownLoad: CSV

Proposition 3.5. Let $ M $ be an irreducible based module of rank $ 5 $ over $ r(S_4) $. Then $ M $ is equivalent to one of the based modules $ M_{5, i}\, (i = 1, 2) $, listed in Table 5.

Proof. Let $ M $ be a based module of rank 5 over $ r(S_4) $, with the action of $ r(S_4) $ on it given by

$\begin{equation*} V_{\psi} \mapsto T, \quad V_{\rho _{1}}\mapsto Q = (a_{ij})_{1\leq i, j\leq5} , \quad V_{\rho _{2}}\mapsto U = (b_{ij})_{1\leq i, j\leq5}, \quad V_{\rho _{3}}\mapsto W = TU, \end{equation*}$ $

where $ a_{ij} = a_{ji} $, $ b_{ij} = b_{ji} $.

First, by a similar argument applied in the case of rank 4, we only need to deal with one of the following 3 cases for $ T $:

$\begin{equation*} T_1 = E_5, \quad T_2 = (12) , \quad T_3 = (12)(34) . \end{equation*}$ $

Case 1. $ T = T_1 = E_5 $.

There are 11 solutions of $ Q $ satisfying Eq (3.5), but only two conjugacy classes by permutation matrices with their representatives given as follows:

$\begin{equation*} Q_1 = \begin{pmatrix} 0&1&1&0&0 \\ 1&0&1&0&0 \\ 1&1&0&0&0 \\ 0&0&0&2&0 \\ 0&0&0&0&2 \end{pmatrix}, \quad Q_2 = \begin{pmatrix} 2&0&0&0&0 \\ 0&2&0&0&0 \\ 0&0&2&0&0 \\ 0&0&0&2&0 \\ 0&0&0&0&2 \end{pmatrix}. \end{equation*}$ $

Next, we calculate $ U $ after taking $ Q $ as one $ Q_k $ $ \left (k = 1, 2 \right) $.

Case 1.1. $ Q = Q_1 $.

There are 4 solutions of $ U $ satisfying Eqs (3.6) and (3.7) as follows:

$\begin{equation*} U_1 = \begin{pmatrix} 0&0&0&1&0 \\ 0&0&0&1&0 \\ 0&0&0&1&0 \\ 1&1&1&2&0 \\ 0&0&0&0&3 \end{pmatrix}, \ \ U_2 = \begin{pmatrix} 0&0&0&0&1 \\ 0&0&0&0&1 \\ 0&0&0&0&1 \\ 0&0&0&3&0 \\ 1&1&1&0&2 \end{pmatrix}, \ \ U_3 = \begin{pmatrix} 1&1&1&0&0 \\ 1&1&1&0&0 \\ 1&1&1&0&0 \\ 0&0&0&3&0 \\ 0&0&0&0&3 \\ \end{pmatrix}, \ \ U_4 = \begin{pmatrix} 1&1&1&0&0 \\ 1&1&1&0&0 \\ 1&1&1&0&0 \\ 0&0&0&1&2 \\ 0&0&0&2&1 \end{pmatrix}. \end{equation*}$ $

Case 1.2. $ Q = Q_2 $.

There are 31 solutions of $ U $ satisfying Eqs (3.6) and (3.7), but only 4 conjugacy classes by permutation matrices and their representatives as follows:

$\begin{equation*} U_1 = \begin{pmatrix} 0&1&1&1&0 \\ 1&0&1&1&0 \\ 1&1&0&1&0 \\ 1&1&1&0&0 \\ 0&0&0&0&3 \end{pmatrix}, \ \ U_2 = \begin{pmatrix} 1&0&0&2&0 \\ 0&1&2&0&0 \\ 0&2&1&0&0 \\ 2&0&0&1&0 \\ 0&0&0&0&3 \end{pmatrix}, \ \ U_3 = \begin{pmatrix} 1&2&0&0&0 \\ 2&1&0&0&0 \\ 0&0&3&0&0 \\ 0&0&0&3&0 \\ 0&0&0&0&3 \\ \end{pmatrix}, \ \ U_4 = \begin{pmatrix} 3&0&0&0&0 \\ 0&3&0&0&0 \\ 0&0&3&0&0 \\ 0&0&0&3&0 \\ 0&0&0&0&3 \end{pmatrix}. \end{equation*}$ $

Each pair $ (T_1, Q_k, U_r) $ above determines a based module, but is not irreducible for any $ 1\le r\le4 $.

Case 2. $ T = T_2 = (12) $.

There are 5 solutions of $ Q $ satisfying Eq (3.5), but only 3 conjugacy classes with the following representatives:

$\begin{equation*} Q_1 = \begin{pmatrix} 0&0&0&0&1 \\ 0&0&0&0&1 \\ 0&0&2&0&0 \\ 0&0&0&2&0 \\ 1&1&0&0&1 \end{pmatrix}, \quad Q_2 = \begin{pmatrix} 1&1&0&0&0 \\ 1&1&0&0&0 \\ 0&0&0&1&1 \\ 0&0&1&0&1 \\ 0&0&1&1&0 \end{pmatrix}, \quad Q_3 = \begin{pmatrix} 1&1&0&0&0 \\ 1&1&0&0&0 \\ 0&0&2&0&0 \\ 0&0&0&2&0 \\ 0&0&0&0&2 \end{pmatrix}. \end{equation*}$ $

Next, we calculate $ U $ after choosing $ Q $.

Case 2.1. $ Q = Q_1 $.

There are 4 solutions of $ U $ satisfying Eqs (3.6) and (3.7) as follows:

$\begin{equation*} U_1 = \begin{pmatrix} 0&1&0&0&1 \\ 1&0&0&0&1 \\ 0&0&1&2&0 \\ 0&0&2&1&0 \\ 1&1&0&0&2 \end{pmatrix}, \ \ U_2 = \begin{pmatrix} 0&1&0&0&1 \\ 1&0&0&0&1 \\ 0&0&3&0&0 \\ 0&0&0&3&0 \\ 1&1&0&0&2 \end{pmatrix}, \ \ U_3 = \begin{pmatrix} 1&0&0&0&1 \\ 0&1&0&0&1 \\ 0&0&1&2&0 \\ 0&0&2&1&0 \\ 1&1&0&0&2 \\ \end{pmatrix}, \ \ U_4 = \begin{pmatrix} 1&0&0&0&1 \\ 0&1&0&0&1 \\ 0&0&3&0&0 \\ 0&0&0&3&0 \\ 1&1&0&0&2 \end{pmatrix}. \end{equation*}$ $

Case 2.2. $ Q = Q_2 $.

There are 2 solutions of $ U $ satisfying Eqs (3.6) and (3.7) as follows:

$\begin{equation*} U_1 = \begin{pmatrix} 1&2&0&0&0 \\ 2&1&0&0&0 \\ 0&0&1&1&1 \\ 0&0&1&1&1 \\ 0&0&1&1&1 \end{pmatrix}, \ \ U_2 = \begin{pmatrix} 2&1&0&0&0 \\ 1&2&0&0&0 \\ 0&0&1&1&1 \\ 0&0&1&1&1 \\ 0&0&1&1&1 \end{pmatrix}. \end{equation*}$ $

Case 2.3. $ Q = Q_3 $.

There are 14 solutions of $ U $ satisfying Eqs (3.6) and (3.7), but only 6 conjugacy classes by permutation matrices with their representatives given as follows:

$\begin{array}{l} U_1 = \begin{pmatrix} 0&1&1&1&0 \\ 1&0&1&1&0 \\ 1&1&0&1&0 \\ 1&1&1&0&0 \\ 0&0&0&0&3 \end{pmatrix}, \quad U_2 = \begin{pmatrix} 1&0&1&1&0 \\ 0&1&1&1&0 \\ 1&1&0&1&0 \\ 1&1&1&0&0 \\ 0&0&0&0&3 \end{pmatrix}, \quad U_3 = \begin{pmatrix} 1&2&0&0&0 \\ 2&1&0&0&0 \\ 0&0&1&2&0 \\ 0&0&2&1&0 \\ 0&0&0&0&3 \end{pmatrix}, \\[3em] U_4 = \begin{pmatrix} 2&1&0&0&0 \\ 1&2&0&0&0 \\ 0&0&1&2&0 \\ 0&0&2&1&0 \\ 0&0&0&0&3 \end{pmatrix}, \quad U_5 = \begin{pmatrix} 1&2&0&0&0 \\ 2&1&0&0&0 \\ 0&0&3&0&0 \\ 0&0&0&3&0 \\ 0&0&0&0&3 \\ \end{pmatrix}, \quad U_6 = \begin{pmatrix} 2&1&0&0&0 \\ 1&2&0&0&0 \\ 0&0&3&0&0 \\ 0&0&0&3&0 \\ 0&0&0&0&3 \end{pmatrix}. \end{array}$ $

Through analysis, all based modules derived from Case 2 are reducible.

Case 3. $ T = T_3 = (12)(34) $.

There are 3 solutions of $ Q $ satisfying Eq (3.5) as follows:

$\begin{equation*} Q_1 = \begin{pmatrix} 0&0&0&0&1 \\ 0&0&0&0&1 \\ 0&0&1&1&0 \\ 0&0&1&1&0 \\ 1&1&0&0&1 \end{pmatrix}, \quad Q_2 = \begin{pmatrix} 1&1&0&0&0 \\ 1&1&0&0&0 \\ 0&0&1&1&0 \\ 0&0&1&1&0 \\ 0&0&0&0&2 \end{pmatrix}, \quad Q_3 = \begin{pmatrix} 1&1&0&0&0 \\ 1&1&0&0&0 \\ 0&0&0&0&1 \\ 0&0&0&0&1 \\ 0&0&1&1&1 \end{pmatrix}. \end{equation*}$ $

Next, we calculate $ U $ after fixing $ Q $.

Case 3.1. $ Q = Q_1 $.

There are 6 solutions of $ U $ satisfying Eqs (3.6) and (3.7) as follows:

$\begin{array}{l} U_1 = \begin{pmatrix} 0&0&0&1&0 \\ 0&0&1&0&0 \\ 0&1&1&1&1 \\ 1&0&1&1&1 \\ 0&0&1&1&0 \end{pmatrix}, \quad U_2 = \begin{pmatrix} 0&0&1&0&0 \\ 0&0&0&1&0 \\ 1&0&1&1&1 \\ 0&1&1&1&1 \\ 0&0&1&1&0 \end{pmatrix}, \quad U_3 = \begin{pmatrix} 0&1&0&0&1 \\ 1&0&0&0&1 \\ 0&0&1&2&0 \\ 0&0&2&1&0 \\ 1&1&0&0&2 \end{pmatrix}, \\[3em] U_4 = \begin{pmatrix} 0&1&0&0&1 \\ 1&0&0&0&1 \\ 0&0&2&1&0 \\ 0&0&1&2&0 \\ 1&1&0&0&2 \end{pmatrix}, \quad U_5 = \begin{pmatrix} 1&0&0&0&1 \\ 0&1&0&0&1 \\ 0&0&1&2&0 \\ 0&0&2&1&0 \\ 1&1&0&0&2 \\ \end{pmatrix}, \quad U_6 = \begin{pmatrix} 1&0&0&0&1 \\ 0&1&0&0&1 \\ 0&0&2&1&0 \\ 0&0&1&2&0 \\ 1&1&0&0&2 \end{pmatrix}. \end{array}$ $

Each pair $ (T_3, Q_1, U_r) $ $ \left (1\le r\le6 \right) $ above determines a based module, but only the based modules with representation matrices $ U_1 $ and $ U_2 $ are irreducible. Such two irreducible based modules are denoted by $ M_{5, 1} $ and $ M'_{5, 1} $, with the corresponding $ \mathbb Z $-basis $ \left \{ v_1^k, v_2^k, v_3^k, v_4^k, v_5^k\right \} $ for $ k = 1, 2 $, respectively. Define the $ \mathbb Z $-module isomorphism $ \phi $: $ M_{5, 1}\to M'_{5, 1} $ by

$\begin{equation*} \phi(v_s^1) = v_s^2, \quad \phi(v_3^1) = v_4^2, \quad \phi(v_4^1) = v_3^2, \quad s = 1, 2, 5. \end{equation*}$ $

Then it is easy to see that $ M_{5, 1} $ is equivalent to $ M'_{5, 1} $ as based modules over $ r(S_4) $ under $ \phi $.

Case 3.2. $ Q = Q_2 $.

There are 10 solutions of $ U $ satisfying Eqs (3.6) and (3.7), but only 7 conjugacy classes with their representatives given as follows:

$\begin{array}{l} U_1 = \begin{pmatrix} 0&0&0&1&1 \\ 0&0&1&0&1 \\ 0&1&0&0&1 \\ 1&0&0&0&1 \\ 1&1&1&1&1 \end{pmatrix}, \ \ U_2 = \begin{pmatrix} 0&1&1&1&0 \\ 1&0&1&1&0 \\ 1&1&0&1&0 \\ 1&1&1&0&0 \\ 0&0&0&0&3 \\ \end{pmatrix}, \ \ U_3 = \begin{pmatrix} 0&1&1&1&0 \\ 1&0&1&1&0 \\ 1&1&1&0&0 \\ 1&1&0&1&0 \\ 0&0&0&0&3 \\ \end{pmatrix}, \ \ U_4 = \begin{pmatrix} 1&0&1&1&0 \\ 0&1&1&1&0 \\ 1&1&1&0&0 \\ 1&1&0&1&0 \\ 0&0&0&0&3 \\ \end{pmatrix}, \end{array}$ $

$\begin{array}{l} U_5 = \begin{pmatrix} 1&2&0&0&0 \\ 2&1&0&0&0 \\ 0&0&1&2&0 \\ 0&0&2&1&0 \\ 0&0&0&0&3 \end{pmatrix}, \ \ U_6 = \begin{pmatrix} 1&2&0&0&0 \\ 2&1&0&0&0 \\ 0&0&2&1&0 \\ 0&0&1&2&0 \\ 0&0&0&0&3 \end{pmatrix}, \ \ U_7 = \begin{pmatrix} 2&1&0&0&0 \\ 1&2&0&0&0 \\ 0&0&2&1&0 \\ 0&0&1&2&0 \\ 0&0&0&0&3 \end{pmatrix}. \end{array}$ $

Each pair $ (T_3, Q_2, U_t) $ $ \left (2\le t\le7 \right) $ above determines a based module, but only the based module with representation matrix $ U_1 $ is irreducible. We denote it by $ M_{5, 2} $.

Also, the based modules obtained by taking $ Q = Q_3 $ are equivalent to the based module $ M_{5, 1} $ found in Case 3.1. □

4. Categorified based modules by module categories over $ { Rep}(S_4) $

In this section, we will apply the knowledge of module categories over the complex representation category of a finite group to find which based modules over $ r(S_4) $ can be categorified by module categories over the representation category $ {\rm Rep}(S_4) $ of $ S_4 $. For the details about module categories over tensor categories, see, e.g., [32, Section 7].

First, we recall the required result for the upcoming discussion. For any finite group $ G $, the second cohomology group $ H^2(G, {{\mathbb C}}^*) $ is known to be a finite abelian group called the Schur multiplier and classifies central extensions of $ G $. The notion of a universal central extension of a finite group was first investigated by Schur in ^[34].

Let $ {\rm Rep}(G, \alpha) $ denote the semisimple abelian category of projective representations of $ G $ with the multiplier $ \alpha\in Z^2(G, {{\mathbb C}}^*) $. Equivalently, $ {\rm Rep}(G, \alpha) $ is the representation category of the twisted group algebra $ {{\mathbb C}} G_\alpha $ of $ G $ with multiplication

$ g\cdot_\alpha h = \alpha(g, h)gh, \quad g, h\in G. $

In particular,

$ {\rm Rep}(G, \alpha) = {\rm Rep}(G), $

when taking $ \alpha = 1 $.

Let $ \alpha \in Z^2(G, {{\mathbb C}}^*) $ represent an element of order $ d $ in $ H^2(G, {{\mathbb C}}^*) $. Define

$ {\rm Rep}^\alpha(G) = \bigoplus \limits_{j = 0}^{d-1}{\rm Rep}(G, \alpha^j). $

According to the result in ^[35], we know that $ {\rm Rep}^\alpha(G) $ becomes a fusion category with the tensor product of two projective representations in $ {\rm Rep}(G, \alpha^i) $ and $ {\rm Rep}(G, \alpha^j) $ respectively lying in $ {\rm Rep}(G, \alpha^{i+j}) $, and the dual object in $ {\rm Rep}(G, \alpha^i) $ lying in $ {\rm Rep}(G, \alpha^{d-i}) $. Correspondingly, we have the fusion ring

$\begin{equation} r^\alpha(G) = \bigoplus \limits_{j = 0}^{d-1}r(G, \alpha^j). \end{equation}$ $

(4.1)

Now let $ H $ be a subgroup of $ G $ and $ \alpha \in Z^2(H, {{\mathbb C}}^*) $. The category $ {\rm Rep}(H, \alpha) $ is a module category over $ {\rm Rep}(G) $ by applying the restriction functor $ {\rm Res}_H^{G} $: $ {\rm Rep}(G)\to {\rm Rep}(H) $.

Theorem 4.1. [17, Theorem 3.2] The indecomposable exact module categories over the representation category $ {\rm Rep}(G) $ are of the form $ {\rm Rep}(H, \alpha) $ and are classified by conjugacy classes of pairs $ (H, [\alpha]) $.

Consequently, by [32, Proposition 7.7.2], we know the following:

Proposition 4.1. The Grothendieck group

$ r(H, \alpha) = {\rm Gr}({\rm Rep}(H, \alpha)) $

is an irreducible $ \mathbb{Z}_+ $-module over $ r(G) $.

Next, we show that any $ \mathbb{Z}_+ $-module over the complex representation ring $ r(G) $ of a finite group $ G $ categorified in this way is a based module.

Theorem 4.2. Let $ G $ be a finite group, $ H $ a subgroup of $ G $, and $ \alpha \in Z^2(H, {{\mathbb C}}^*) $. The $ \mathbb{Z}_+ $-module $ r(H, \alpha) $ over $ r(G) $ is a based module.

Proof. Let $ \left \{ {\psi}_i \right \} _{i\in I} $ be the $ \mathbb{Z}_+ $-basis of $ r(G) $. Take $ r^\alpha(H) $ defined in Eq (4.1) as a $ \mathbb{Z}_+ $-module over $ r(G) $ with the $ \mathbb{Z} $-basis $ \left \{ {\chi}_k \right \} _{k\in J} $ such that

$ \psi_i.\chi_k = \sum\limits_l a_{ik}^l \chi_l, \quad a_{ik}^l\in \mathbb{Z}_+. $

On the other hand, we write the fusion rule of the fusion ring $ r^\alpha(H) $ as follows:

$\begin{equation*} \chi_i\chi_j = \sum\limits_{k = 1}^{s} n_{ij}^k\chi_k, \quad n_{ij}^k\in \mathbb{Z}_+. \end{equation*}$ $

Since the number $ n_{ij}^{k^*} $ is invariant under cyclic permutations of $ i, j, k $, we have

$ n_{ij}^k = n_{k^*i}^{j^*} = n_{i^*k}^{j}. $

By the restriction rule, we interpret $ r(G) $ as a subring of $ r^\alpha(H) $ and write down

$ \psi_i = \sum\limits_j r_{ij} \chi_j, \quad r_{ij}\in \mathbb{Z}_+. $

Then

$ \psi_i.\chi_k = \sum\limits_j r_{ij} \chi_j\chi_k = \sum\limits_{j, \, l} r_{ij}n_{jk}^l \chi_l. $

By comparing the coefficients, we see that

$ a_{ik}^l = \sum\limits_{j} r_{ij}n_{jk}^l = \sum\limits_{j} r_{ij}n_{j^*l}^k = \sum\limits_{j} r_{i^*j^*}n_{j^*l}^k = \sum\limits_{j} r_{i^*j}n_{jl}^k = a_{i^*l}^k, $

so $ r^\alpha(H) $ is a based module over $ r(G) $, and $ r(H, \alpha) $ is clearly a based submodule of $ r^\alpha(H) $. Equivalently, any $ \mathbb{Z}_+ $-module over $ r(G) $ categorified by a module category $ {\rm Rep}(H, \alpha) $ over $ {\rm Rep}(G) $ must be a based module. □

By Theorem 4.2, we only need to focus on those inequivalent irreducible based modules $ M_{i, j} $ over $ r(S_4) $ collected in Section 3, each of which is possibly categorified by a module category $ {\rm Rep}(H, \alpha) $ for some $ H < S_4 $ and $ \alpha \in Z^2(H, {{\mathbb C}}^*) $.

All the non-isomorphic subgroups of the symmetric group $ S_4 $ are as follows:

$ ({\rm{i}}) $ The symmetric group $ S_3 $;

$ ({\rm{ii}}) $ The cyclic groups $ \mathbb Z_i, \, 1\leq i\leq 4 $;

$ ({\rm{iii}}) $ The Klein 4-group $ K_4 $;

$ ({\rm{iv}}) $ The alternating group $ A_4 $;

$ ({\rm{v}}) $ The dihedral group $ D_4 $;

$ ({\rm{vi}}) $ The symmetric group $ S_4 $ itself.

Correspondingly, the Schur multipliers we consider here are given as follows (see e.g., ^[36]):

$ H^2(\mathbb Z_n, {{\mathbb C}}^*)\cong H^2(S_3, {{\mathbb C}}^*)\cong 0, \ n\geq 1, \quad \ H^2(K_4, {{\mathbb C}}^*)\cong H^2(D_4, {{\mathbb C}}^*)\cong H^2(A_4, {{\mathbb C}}^*)\cong H^2(S_4, {{\mathbb C}}^*)\cong\mathbb Z_2. $

As a result, we only need to consider the following two situations:

(1) Module category $ {\rm Rep}(H) $ for any subgroup $ H < S_4 $;

(2) Module category $ {\rm Rep}(H, \alpha) $ for any subgroup $ H < S_4 $ and nontrivial twist $ \alpha \in Z^2(H, {{\mathbb C}}^*) $.

4.1. The module categories over $ { Rep}(S_4) $ with trivial twists

(ⅰ) First, we consider the representation category $ {\rm Rep}(S_3) $ as a module category over $ {\rm Rep}(S_4) $.

Theorem 4.3. $ r(S_3) = {\rm Gr}({\rm Rep}(S_3)) $ is an irreducible based module over $ r(S_4) = {\rm Gr}({\rm Rep}(S_4)) $ equivalent to the based module $ M_{3, 2} $ in Table 3.

Proof. According to the branching rule of symmetric groups (see e.g., [37, Theorem 2.8.3]), we have the following restriction rules:

$\begin{align*} {\rm Res}_{S_3}^{S_4} \left ( 1 \right ) = 1, \; \; \; {\rm Res}_{S_3}^{S_4} \left ( V_{\psi} \right ) = \chi, \; \; \; {\rm Res}_{S_3}^{S_4} \left ( V_{\rho_{1}} \right ) = V, \; \; \; {\rm Res}_{S_3}^{S_4} \left ( V_{\rho_{2}} \right ) = 1+V, \; \; \; {\rm Res}_{S_3}^{S_4} \left ( V_{\rho_{3}} \right ) = \chi+V, \end{align*}$ $

where $ \chi $ and $ V $ denote the sign representation and the standard representation in $ {\rm Rep}({S_3}) $, respectively. Hence, we get the representation matrices of basis elements of $ r(S_4) $ acting on $ r({S_3}) $ as follows:

$\begin{align*} 1 \mapsto E_3, \quad V_{\psi} \mapsto \begin{pmatrix}0& 1 &0 \\1&0 &0 \\0&0 &1\end{pmatrix}, \quad V_{\rho _{1}}\mapsto \begin{pmatrix}0& 0 &1 \\0& 0 &1 \\1& 1&1 \end{pmatrix}, \quad V_{\rho _{2}}\mapsto \begin{pmatrix}1& 0 &1 \\0& 1 &1 \\1& 1&2 \end{pmatrix}, \quad V_{\rho _{3}}\mapsto \begin{pmatrix}0& 1 &1 \\1& 0 &1 \\1& 1&2 \end{pmatrix}. \end{align*}$ $

We see that $ r(S_3) $ is an irreducible based module $ M_{3, 2} $ according to Table 3. In other words, the based module $ M_{3, 2} $ can be categorified by the module category $ {\rm Rep}({S_3}) $ over $ {\rm Rep}(S_4) $. □

Remark 4.1. Since the roles of the standard representation and its dual in $ r(S_4) $ are symmetric, we can exchange the notations $ V_{\rho_{2}} $ and $ V_{\rho_{3}} $ for them to get the following restriction rules instead:

$\begin{equation*} {\rm Res}_{S_3}^{S_4} \left ( V_{\rho_{2}} \right ) = \chi+V, \quad {\rm Res}_{S_3}^{S_4} \left ( V_{\rho_{3}} \right ) = 1+V. \end{equation*}$ $

Therefore, we get another action of $ r(S_4) $ on $ r({S_3}) $ such that $ r(S_3) $ is an irreducible based module over $ r(S_4) $ equivalent to the based module $ M_{3, 3} $ according to Table 3. In other words, the based module $ M_{3, 3} $ can also be categorified by the module category $ {\rm Rep}({S_3}) $ over $ {\rm Rep}(S_4) $.

(ⅱ) Second, we consider $ {\rm Rep}(\mathbb{Z}_4) $ as a module category over $ {\rm Rep}(S_4) $.

Theorem 4.4. $ r(\mathbb{Z}_4) = {\rm Gr}({\rm Rep}(\mathbb{Z}_4)) $ is an irreducible based module over $ r(S_4) $ equivalent to the based module $ M_{4, 5} $ in Table 4.

Proof. Let

$ \mathbb{Z}_4 = \left \{ 1, g, g^2, g^3 \right \} $

be the cyclic group of order $ 4 $, with four non-isomorphic 1-dimensional irreducible representations denoted by $ U_i, \, i = 0, 1, 2, 3 $. Let $ U_0 = 1 $ represent the trivial representation, and

$\begin{equation*} \chi_{U_1}(g) = \sqrt{-1} , \quad \chi_{U_2}(g) = -1, \quad\chi_{U_3}(g) = -\sqrt{-1}. \end{equation*}$ $

On the other hand, we consider $ \mathbb{Z}_4 $ as the subgroup of $ S_4 $ generated by $ g = (1234) $. Then, by the character table of $ S_4 $ (Table 1), we have

$\begin{align*} \chi_\psi(g^i)& = (-1)^i, \quad \chi_{\rho_1}(g^i) = 1+(-1)^i, \\ \chi_{\rho_2}(g^i)& = (-1)^i+(\sqrt{-1})^i+(-\sqrt{-1})^i, \\ \chi_{\rho_3}(g^i)& = 1+(\sqrt{-1})^i+(-\sqrt{-1})^i. \end{align*}$ $

So, the restriction rule of $ r(S_4) $ on $ r(\mathbb{Z}_4) $ is given as follows:

$\begin{align*} {\rm Res}_{\mathbb{Z}_4}^{S_4} \left ( 1 \right )& = 1, \quad {\rm Res}_{\mathbb{Z}_4}^{S_4} \left ( V_{\psi} \right ) = U_2, \quad {\rm Res}_{\mathbb{Z}_4}^{S_4} \left ( V_{\rho_{1}} \right ) = 1+U_2, \\ {\rm Res}_{\mathbb{Z}_4}^{S_4} \left ( V_{\rho_{2}} \right )& = U_1+U_2+U_3, \quad\quad{\rm Res}_{\mathbb{Z}_4}^{S_4} \left ( V_{\rho_{3}} \right ) = 1+U_1+U_3. \end{align*}$ $

Then, we get the representation matrices of basis elements of $ r(S_4) $ acting on $ r(\mathbb{Z}_4) $ as follows:

$\begin{align*} &1 \mapsto E_4, \space V_{\psi} \mapsto \begin{pmatrix}0& 0 &1 &0\\0& 0 &0&1\\1& 0&0&0\\0&1&0&0\end{pmatrix}, \space V_{\rho _{1}}\mapsto \begin{pmatrix}1&0&1&0\\0&1&0&1\\1&0&1&0\\0&1&0&1\end{pmatrix}, \space &V_{\rho _{2}}\mapsto \begin{pmatrix}0&1&1&1\\1&0&1&1\\1&1&0&1\\1&1&1&0 \end{pmatrix}, \space V_{\rho_{3}}\mapsto \begin{pmatrix}1&1&0&1\\1&1&1&0\\0&1&1&1\\1&0&1&1\end{pmatrix}. \end{align*}$ $

Let $ \left \{ w_i\right \}_{1\leq i\leq 4} $ be the stated $ \mathbb{Z} $-basis of $ M_{4, 5} $, and define a $ \mathbb{Z} $-linear map $ \varphi $: $ M_{4, 5}\to r(\mathbb{Z}_4) $ by

$\begin{equation*} \varphi (w_1) = U_3, \quad \varphi (w_2) = U_1, \quad \varphi (w_3) = U_2, \quad\varphi (w_4) = 1. \end{equation*}$ $

Then, it is easy to check that $ \varphi $ is an isomorphism of $ r(S_{4}) $-modules, so $ M_{4, 5} $ is equivalent to $ r(\mathbb{Z}_4) $ as based modules by Definition 2.4 (ⅰ). In other words, the based module $ M_{4, 5} $ can be categorified by the module category $ {\rm Rep}(\mathbb{Z}_4) $ over $ {\rm Rep}(S_4) $. □

Remark 4.2. By the same argument as in Remark 4.1, $ V_{\rho _{2}} $ and $ V_{\rho _{3}} $ can be required to satisfy the following restriction rules instead:

$\begin{equation*} {\rm Res}_{\mathbb{Z}_4}^{S_4} \left ( V_{\rho_{2}} \right ) = 1+U_1+U_3, \quad{\rm Res}_{\mathbb{Z}_4}^{S_4} \left ( V_{\rho_{3}} \right ) = U_1+U_2+U_3. \end{equation*}$ $

Therefore, we get another action of $ r(S_4) $ on $ r({\mathbb{Z}_4}) $ such that $ r(\mathbb{Z}_4) $ is an irreducible based module over $ r(S_4) $ equivalent to the based module $ M_{4, 7} $ according to Table 4. In other words, the based module $ M_{4, 7} $ can also be categorified by the module category $ {\rm Rep}(\mathbb{Z}_4) $ over $ {\rm Rep}(S_4) $.

Also, one can similarly check that the module category $ {\rm Rep}(\mathbb{Z}_2) $ over $ {\rm Rep}(S_4) $ categorifies the based modules $ M_{2, 2} $ and $ M_{2, 3} $, while $ {\rm Rep}(\mathbb{Z}_3) $ over $ {\rm Rep}(S_4) $ categorifies the based module $ M_{3, 1} $.

(ⅲ) Now we consider $ {\rm Rep}(K_4) $ as a module category over $ {\rm Rep}(S_4) $.

Theorem 4.5. $ r(K_4) = {\rm Gr}({\rm Rep}(K_4)) $ is an irreducible based module over $ r(S_4) $ equivalent to the based module $ M_{4, 7} $ in Table 4.

Proof. We consider $ K_4 $ as the subgroup of $ S_4 $ generated by $ (12) $ and $ (34) $, and it has four non-isomorphic 1-dimensional irreducible representations $ Y_0 = 1 $ and $ Y_1, Y_2, Y_3 $ such that

$\begin{align*} &\chi_{Y_1}((12)) = -1, \quad \chi_{Y_1}((34)) = 1;\quad\; \; \chi_{Y_2}((12)) = 1, \\ &\chi_{Y_2}((34)) = -1;\quad \chi_{Y_3}((12)) = -1, \quad \chi_{Y_3}((34)) = -1. \end{align*}$ $

On the other hand, by the character table of $ S_4 $ (Table 1), we have

$\begin{align*} &\chi_\psi((12)) = \chi_\psi((34)) = -1, \quad \chi_\psi((12)(34)) = 1;\\ &\chi_{\rho_1}((12)) = \chi_{\rho_1}((34)) = 0, \quad \chi_{\rho_1}((12)(34)) = 2;\\ &\chi_{\rho_2}((12)) = \chi_{\rho_2}((34)) = 1, \quad \chi_{\rho_2}((12)(34)) = -1;\\ &\chi_{\rho_3}((12)) = \chi_{\rho_3}((34)) = -1, \quad \chi_{\rho_3}((12)(34)) = -1. \end{align*}$ $

So, we have the following restriction rules:

$\begin{align*} {\rm Res}_{K_4}^{S_4} \left ( 1 \right )& = 1, \quad {\rm Res}_{K_4}^{S_4} \left ( V_{\psi} \right ) = Y_3, \quad {\rm Res}_{K_4}^{S_4} \left ( V_{\rho_{1}} \right ) = 1+Y_3, \\ {\rm Res}_{K_4}^{S_4} \left ( V_{\rho_{2}} \right )& = 1+Y_1+Y_2, \quad\quad {\rm Res}_{K_4}^{S_4} \left ( V_{\rho_{3}} \right ) = Y_1+Y_2+Y_3. \end{align*}$ $

Then we get the representation matrices of basis elements of $ r(S_4) $ acting on $ r(K_4) $ as follows:

$\begin{align*} &1 \mapsto E_4, \quad V_{\psi} \mapsto \begin{pmatrix}0& 0 &0 &1\\0& 0 &1&0 \\0&1&0&0\\1&0&0&0\end{pmatrix}, \quad V_{\rho _{1}}\mapsto \begin{pmatrix}1&0&0&1\\0&1&1&0\\0&1&1&0\\1&0&0&1 \end{pmatrix}, \\ &V_{\rho _{2}}\mapsto \begin{pmatrix}1&1&1&0\\1&1&0&1\\1&0&1&1\\0&1&1&1\end{pmatrix}, \quad\quad V_{\rho_{3}}\mapsto \begin{pmatrix}0&1&1&1\\1&0&1&1\\1&1&0&1\\1&1&1&0 \end{pmatrix}. \end{align*}$ $

Let $ \left \{ w_i\right \}_{1\leq i\leq 4} $ be the stated $ \mathbb{Z} $-basis of $ M_{4, 7} $ listed in Table 4. Then

$\begin{equation*} w_1\mapsto Y_2, \quad w_2\mapsto Y_1, \quad w_3\mapsto 1, \quad w_4\mapsto Y_3, \end{equation*}$ $

defines an equivalence of $ \mathbb{Z}_+ $-modules between $ M_{4, 7} $ and $ r(K_4) $. In other words, the irreducible based module $ M_{4, 7} $ can be categorified by the module category $ {\rm Rep}(K_4) $ over $ {\rm Rep}(S_4) $. □

Remark 4.3. In a manner analogous to the argument in Remark 4.1, it follows that the irreducible based module $ M_{4, 5} $ can also be categorified by the module category $ {\rm Rep}(K_4) $ over $ {\rm Rep}(S_4) $.

(ⅳ) We consider $ {\rm Rep}(A_4) $ as a module category over $ {\rm Rep}(S_4) $.

Theorem 4.6. $ r(A_4) = {\rm Gr}({\rm Rep}(A_4)) $ is an irreducible based module over $ r(S_4) $ equivalent to the based module $ M_{4, 1} $ in Table 4.

Proof. We know that $ A_4 $ has three non-isomorphic 1-dimensional irreducible representations and one 3-dimensional irreducible representation, denoted by $ N_0, N_1, N_2 $, and $ N_3 $, respectively, where $ N_0 = 1 $ represents the trivial representation, and

$\begin{align*} &\chi_{N_1}((123)) = \omega , \quad \chi_{N_1}((12)(34)) = 1;\quad \chi_{N_2}((123)) = \omega^2, \quad \chi_{N_2}((12)(34)) = 1;\\ &\chi_{N_3}((123)) = \chi_{N_3}((132)) = 0, \quad\ \chi_{N_3}((12)(34)) = -1, \quad\ \omega = \frac{-1+\sqrt{-3} }{2}. \end{align*}$ $

On the other hand, the character table of $ S_4 $ (Table 1) tells us that

$\begin{align*} &\chi_\psi((123)) = 1, \quad\; \; \chi_\psi((12)(34)) = 1;\quad \chi_{\rho_1}((123)) = -1, \quad \chi_{\rho_1}((12)(34)) = 2;\\ &\chi_{\rho_2}((123)) = 0, \quad \chi_{\rho_2}((12)(34)) = -1;\quad \chi_{\rho_3}((123)) = 0, \quad \chi_{\rho_3}((12)(34)) = -1. \end{align*}$ $

So, we have the following restriction rules:

$\begin{align*} {\rm Res}_{A_4}^{S_4} \left ( 1 \right ) = {\rm Res}_{A_4}^{S_4} \left ( V_{\psi} \right ) = 1, \quad {\rm Res}_{A_4}^{S_4} \left ( V_{\rho_{1}} \right ) = N_1+N_2, \quad {\rm Res}_{A_4}^{S_4} \left ( V_{\rho_{2}} \right ) = {\rm Res}_{A_4}^{S_4} \left ( V_{\rho_{3}} \right ) = N_3. \end{align*}$ $

Hence, we get the representation matrices of basis elements of $ r(S_4) $ acting on $ r(A_4) $ as follows:

$\begin{align*} 1 \mapsto E_4, \quad V_{\psi} \mapsto E_4, \quad V_{\rho _{1}}\mapsto \begin{pmatrix}0& 1 &1 &0\\1& 0 &1&0 \\1& 1&0&0\\0&0&0&2 \end{pmatrix}, \quad V_{\rho _{2}}, V_{\rho_{3}}\mapsto \begin{pmatrix}0& 0 &0 &1\\0& 0 &0 &1\\0& 0 &0 &1\\1&1&1&2 \end{pmatrix}. \end{align*}$ $

Then, $ r(A_4) $ is an irreducible based module over $ r(S_4) $ equivalent to $ M_{4, 1} $ listed in Table 4. In other words, the irreducible based module $ M_{4, 1} $ can be categorified by the module category $ {\rm Rep}(A_4) $ over $ {\rm Rep}(S_4) $. □

(ⅴ) Next, we consider $ {\rm Rep}(D_4) $ as a module category over $ {\rm Rep}(S_4) $.

Theorem 4.7. $ r(D_4) = {\rm Gr}({\rm Rep}(D_4)) $ is an irreducible based module over $ r(S_4) $ equivalent to the based module $ M_{5, 2} $ in Table 5.

Proof. The dihedral group

$ D_4 = \langle r, s\, |\, r^4 = s^2 = (rs)^2 = 1\rangle $

has four 1-dimensional irreducible representations and one 2-dimensional irreducible representation up to isomorphism, denoted by $ W_0, W_1, W_2, W_3 $, and $ W_4 $, respectively. Let $ W_0 = 1 $ stand for the trivial representation, and

$\begin{align*} &\chi_{W_1}(r) = 1 , \quad\; \; \chi_{W_1}(s) = -1; \quad\chi_{W_2}(r) = -1, \quad \chi_{W_2}(s) = 1;\\ &\chi_{W_3}(r) = -1, \quad\chi_{W_3}(s) = -1; \quad \chi_{W_4}(r) = \chi_{W_4}(s) = \chi_{W_4}(rs) = 0. \end{align*}$ $

On the other hand, we consider $ D_4 $ as the subgroup of $ S_4 $ by taking $ r = (1234) $ and $ s = (12)(34) $. Then $ rs = (13) $. By the character table of $ S_4 $ (Table 1), we have

$\begin{align*} &\chi_\psi((1234)) = -1, \quad \chi_\psi((12)(34)) = 1, \quad \chi_\psi((13)) = -1;\\ &\chi_{\rho_1}((1234)) = 0, \quad \chi_{\rho_1}((12)(34)) = 2, \quad \chi_{\rho_1}((13)) = 0;\\ &\chi_{\rho_2}((1234)) = -1, \quad \chi_{\rho_2}((12)(34)) = -1, \quad \chi_{\rho_2}((13)) = 1;\\ &\chi_{\rho_3}((1234)) = 1, \quad \chi_{\rho_3}((12)(34)) = -1, \quad \chi_{\rho_3}((13)) = -1. \end{align*}$ $

So, we have the following restriction rules:

$\begin{align*} {\rm Res}_{D_4}^{S_4} \left ( 1 \right )& = 1, \quad {\rm Res}_{D_4}^{S_4} \left ( V_{\psi} \right ) = W_2, \quad {\rm Res}_{D_4}^{S_4} \left ( V_{\rho_{1}} \right ) = 1+W_2, \\ {\rm Res}_{D_4}^{S_4} \left ( V_{\rho_{2}} \right )& = W_3+W_4, \quad\quad {\rm Res}_{D_4}^{S_4} \left ( V_{\rho_{3}} \right ) = W_1+W_4. \end{align*}$ $

Then we get the representation matrices of basis elements of $ r(S_4) $ acting on $ r(D_4) $ as follows:

$\begin{align*} &1 \mapsto E_5, \quad V_{\psi} \mapsto \begin{pmatrix}0& 0 &1 &0&0\\0& 0 &0&1&0 \\1& 0&0&0&0\\0&1&0&0&0\\0&0&0&0&1 \end{pmatrix}, \quad V_{\rho _{1}}\mapsto \begin{pmatrix}1&0&1&0&0\\0&1&0&1&0\\1&0&1&0&0\\0&1&0&1&0\\0&0&0&0&2 \end{pmatrix}, \\ &\; \; \; \; \; \; V_{\rho _{2}}\mapsto \begin{pmatrix}0&0&0&1&1\\0&0&1&0&1\\0&1&0&0&1\\1&0&0&0&1\\1&1&1&1&1 \end{pmatrix}, \quad V_{\rho_{3}}\mapsto \begin{pmatrix}0&1&0&0&1\\1&0&0&0&1\\0&0&0&1&1\\0&0&1&0&1\\1&1&1&1&1 \end{pmatrix}. \end{align*}$ $

Then $ r(D_4) $ is an irreducible based module over $ r(S_4) $ equivalent to $ M_{5, 2} $ listed in Table 5. In other words, the irreducible based module $ M_{5, 2} $ can be categorified by the module category $ {\rm Rep}(D_4) $ over $ {\rm Rep}(S_4) $. □

(ⅵ) Finally, we consider $ {\rm Rep}(S_4) $ as a module category over itself.

Theorem 4.8. The regular $ \mathbb{Z}_+ $-module $ r(S_4) $ over itself is equivalent to the irreducible based module $ M_{5, 1} $ in Table 5.

Proof. Let $ r(S_4) $ be the regular $ \mathbb{Z}_+ $-module over itself with the $ \mathbb{Z} $-basis $ \left \{ 1, V_{\psi}, V_{\rho_{1}}, V_{\rho_{2}}, V_{\rho_{3}} \right \} $, and the action of $ r(S_4) $ on it is given as follows:

$\begin{align*} &1 \mapsto E_5, \quad V_{\psi} \mapsto \begin{pmatrix} 0&1&0&0&0 \\ 1&0&0&0&0 \\ 0&0&1&0&0 \\ 0&0&0&0&1 \\ 0&0&0&1&0 \end{pmatrix}, \quad V_{\rho _{1}}\mapsto \begin{pmatrix} 0&0&1&0&0 \\ 0&0&1&0&0 \\ 1&1&1&0&0 \\ 0&0&0&1&1 \\ 0&0&0&1&1 \end{pmatrix}, \\ &\; \; \; \; \; \; \; V_{\rho _{2}}\mapsto \begin{pmatrix} 0&0&0&1&0 \\ 0&0&0&0&1 \\ 0&0&0&1&1 \\ 1&0&1&1&1 \\ 0&1&1&1&1 \end{pmatrix}, \quad V_{\rho _{3}}\mapsto \begin{pmatrix} 0&0&0&0&1 \\ 0&0&0&1&0 \\ 0&0&0&1&1 \\ 0&1&1&1&1 \\ 1&0&1&1&1 \end{pmatrix}. \end{align*}$ $

Then, the regular $ \mathbb{Z}_+ $-module $ r(S_4) $ over itself is equivalent to $ M_{5, 1} $ listed in Table 5. In other words, the irreducible based module $ M_{5, 1} $ over $ r(S_4) $ can be categorified by the module category $ {\rm Rep}(S_4) $ over itself. □

Remark 4.4. Following the argument presented in Remark 4.1, if we exchange the notations $ V_{\rho _{2}} $ and $ V_{\rho _{3}} $ with their restriction rules given in the proof of Theorems 4.7 and 4.8, we see that $ r(D_4) $ and $ r(S_4) $ are still equivalent to $ M_{5, 2} $ and $ M_{5, 1} $, respectively.

4.2. The module categories over $ { Rep}(S_4) $ with nontrivial twists

Lastly, we consider the module category $ {\rm Rep}(H, \alpha) $ over $ {\rm Rep}(S_4) $, where $ H $ is a subgroup of $ S_4 $ with $ \alpha $ representing the unique nontrivial cohomological class in $ H^2(H, {{\mathbb C}}^*) $. All non-isomorphic irreducible projective representations of $ H $ with the multiplier $ \alpha $ form a $ \mathbb Z $-basis of $ r(H, \alpha) $, whose cardinality is the number of $ \alpha $-regular conjugacy classes by [38, Theorem 6.1.1].

First, we consider the twisted group algebra of $ K_4 $. There is only one irreducible projective representation with respect to $ \alpha $ up to isomorphism, see, e.g., [39, Appendix D.1]. Hence, $ r(K_4, \alpha) $ is a based module of rank $ 1 $ over $ r(S_4) $ equivalent to $ M_{1, 1} $ defined in (3.8). Namely, the based module $ M_{1, 1} $ can also be categorified by $ {\rm Rep}(K_4, \alpha) $.

Second, we consider the twisted group algebra of $ D_4 $.

Theorem 4.9. $ r(D_4, \alpha) = {\rm Gr}({\rm Rep}(D_4, \alpha)) $ is an irreducible based module over $ r(S_4) $ equivalent to the based module $ M_{2, 3} $ in Table 2.

Proof. Let

$ D_4 = \langle r, s\, |\, r^4 = s^2 = (rs)^2 = 1\rangle . $

Let $ \alpha\in Z^2(D_4, {{\mathbb C}}^*) $ be the 2-cocycle defined by

$\begin{equation} \alpha (r^is^j, r^{i'}s^{j'}) = (\sqrt{-1})^{ji'}.\nonumber \end{equation}$ $

Here, $ i, i'\in {\left \{ 0, 1, 2, 3 \right \} }, \, j, j'\in\left \{ {0, 1} \right \} $. As shown in [40, Section 3.7], this is a unitary 2-cocycle representing the unique non-trivial cohomological class in $ H^2(D_4, {{\mathbb C}}^*) $. According to [35, Section 3], there exist two (2-dimensional) non-isomorphic irreducible projective representations of $ D_4 $ with respect to $ \alpha $, which are given by

$\begin{equation} \begin{aligned} \pi_{l}: D_4 & \rightarrow \mathrm{GL}_{2}(\mathbb{C}), \\ r^{i} s^{j} & \mapsto A_{l}^{i} B^{j}, \end{aligned}\nonumber \end{equation}$ $

where

$ A_l =

$\begin{pmatrix}(\sqrt{-1})^l &0 \\ 0&(\sqrt{-1})^{1-l}\end{pmatrix}$ , \; \; \; B =

$\begin{pmatrix}0 &1 \\1 &0\end{pmatrix}$ , \; \; \; l = 1, 2. $

Also, for irreducible representations $ W_0 $–$ W_4 $ of $ D_4 $ mentioned in the proof of Theorem 4.7, we have

$\begin{equation} W_0\otimes \pi_l = W_1\otimes\pi_l = \pi_l, \quad W_2\otimes \pi_l = W_3\otimes \pi_l = \pi_{3-l}, \quad W_4\otimes \pi_l = \pi_1+\pi_2.\nonumber \end{equation}$ $

Next, using the previous restriction rule of $ r(S_4) $ on $ r(D_4) $, we get the representation matrices of basis elements of $ r(S_4) $ acting on $ r(D_4, \alpha) $ as follows:

$\begin{equation} \begin{aligned} 1 \mapsto E_2, \quad V_{\psi} \mapsto \begin{pmatrix} 0 &1 \\ 1&0 \end{pmatrix}, \quad V_{\rho_1} \mapsto \begin{pmatrix} 1 &1 \\ 1&1 \end{pmatrix}, \quad V_{\rho_2} \mapsto \begin{pmatrix} 1&2 \\ 2&1 \end{pmatrix}, \quad V_{\rho_3} \mapsto \begin{pmatrix} 2&1 \\ 1&2 \end{pmatrix}.\nonumber \end{aligned} \end{equation}$ $

Then, $ r(D_4, \alpha) $ is an irreducible based module over $ r(S_4) $ equivalent to $ M_{2, 3} $ listed in Table 2. In other words, the irreducible based module $ M_{2, 3} $ can be categorified by the module category $ {\rm Rep}(D_4, \alpha) $ over $ {\rm Rep}(S_4) $. □

Remark 4.5. As discussed in Remark 4.1, it follows that the irreducible based module $ M_{2, 2} $ can also be categorified by the module category $ {\rm Rep}(D_4, \alpha) $ over $ {\rm Rep}(S_4) $.

Next, we consider the twisted group algebras of $ A_4 $ and $ S_4 $. By [38, Theorem 6.1.1], $ A_4 $ has three (2-dimensional) non-isomorphic irreducible projective representations, denoted as $ V_{\gamma _{1}}, V_{\gamma _{2}} $, and $ V_{\gamma _{3}} $, respectively. Similarly, $ S_4 $ has two (2-dimensional) non-isomorphic irreducible projective representations $ V_{\xi_1}, V_{\xi_2} $, and one (4-dimensional) irreducible projective representation $ V_{\xi_3} $. We give the character table for projective representations of $ A_4 $ and $ S_4 $ in Tables 6 and 7, respectively, where primes are used to differentiate between the two classes splitting from a single conjugacy class of $ A_4 $ in its double cover $ \tilde A_4 $, and the same applies to $ S_4 $; subscripts distinguish between the two classes splitting from the conjugacy classes $ (31)^{'} $ and $ (31)^{''} $ in the double cover $ \tilde S_4 $ of $ S_4 $, respectively. For more details, see [41, Section 4].

Table 6. The character table for irreducible projective representations of $ A_4 $.

	$ (1^4)^{'} $	$ (1^4)^{''} $	$ (2^2) $	$ (31)^{'}_1 $	$ (31)^{''}_1 $	$ (31)^{'}_2 $	$ (31)^{''}_2 $
$ \chi_{\gamma _{1}} $	$ 2 $	$ -2 $	$ 0 $	$ 1 $	$ -1 $	$ 1 $	$ -1 $
$ \chi_{\gamma _{2}} $	$ 2 $	$ -2 $	$ 0 $	$ \omega $	$ -\omega $	$ \omega^2 $	$ -\omega^2 $
$ \chi_{\gamma _{3}} $	$ 2 $	$ -2 $	$ 0 $	$ \omega^2 $	$ -\omega^2 $	$ \omega $	$ -\omega $

| Show Table

DownLoad: CSV

Table 7. The character table for irreducible projective representations of $ S_4 $.

	$ (1^4)^{'} $	$ (1^4)^{''} $	$ (2 1^2) $	$ (2^2) $	$ (3 1)^{'} $	$ (31)^{''} $	$ (4)^{'} $	$ (4)^{''} $
$ \chi_{\xi_1} $	$ 2 $	$ -2 $	$ 0 $	$ 0 $	$ 1 $	$ -1 $	$ \sqrt{2} $	$ -\sqrt{2} $
$ \chi_{\xi_2} $	$ 2 $	$ -2 $	$ 0 $	$ 0 $	$ 1 $	$ -1 $	$ -\sqrt{2} $	$ \sqrt{2} $
$ \chi_{\xi_3} $	$ 4 $	$ -4 $	$ 0 $	$ 0 $	$ -1 $	$ 1 $	$ 0 $	$ 0 $

| Show Table

DownLoad: CSV

In Table 6, we denote

$ \omega = e^{2\pi\sqrt{-1}/3} = \frac{-1+\sqrt{-3}}{2}. $

Then we have the following theorems.

Theorem 4.10. $ r(A_4, \alpha) = {\rm Gr}({\rm Rep}(A_4, \alpha)) $ is an irreducible based module over $ r(S_4) $ equivalent to the based module $ M_{3, 1} $ in Table 3.

Proof. For the irreducible representations $ N_0, N_1, N_2 $, and $ N_3 $ of $ A_4 $ mentioned in the proof of Theorem 4.6, we obtain the following tensor product rule in $ r^\alpha(A_4) $ by computing the values of products of characters:

$\begin{align*} &N_0 \otimes V_{\gamma_i } = V_{\gamma_{i} }, \quad N_1 \otimes V_{\gamma_j } = V_{\gamma_{j+1} }, \quad N_1 \otimes V_{\gamma_3 } = V_{\gamma_{1} };\quad N_2 \otimes V_{\gamma_1 } = V_{\gamma_{3} }, \\ & N_2 \otimes V_{\gamma_2 } = V_{\gamma_{1} }, \quad\ N_2 \otimes V_{\gamma_3 } = V_{\gamma_{2} };\quad\ N_3 \otimes V_{\gamma_i } = V_{\gamma_{1} }+V_{\gamma_{2} }+V_{\gamma_{3} }, \end{align*}$ $

where $ i = 1, 2, 3 $, $ j = 1, 2 $. Next, by combining this with the previous restriction rule of $ r(S_4) $ on $ r(A_4) $, we obtain

$ 1, V_{\psi} \mapsto E_3, \quad V_{\rho_1} \mapsto

$\begin{pmatrix} 0 &1 &1\\ 1 &0 &1\\ 1 &1 &0 \end{pmatrix}$ , \quad V_{\rho_2}, V_{\rho_3} \mapsto

$\begin{pmatrix} 1 &1 &1\\ 1 &1 &1\\ 1 &1 &1 \end{pmatrix}$ . $

Then $ r(A_4, \alpha) $ is an irreducible based module over $ r(S_4) $ equivalent to $ M_{3, 1} $ listed in Table 3. In other words, the irreducible based module $ M_{3, 1} $ can be categorified by $ {\rm Rep}(A_4, \alpha) $. □

Theorem 4.11. $ r(S_4, \alpha) = {\rm Gr}({\rm Rep}(S_4, \alpha)) $ is an irreducible based module over $ r(S_4) $ equivalent to the based module $ M_{3, 3} $ in Table 3.

Proof. Let $ \alpha $ be a nontrivial 2-cocycle in $ Z^2(S_4, {{\mathbb C}}^*) $ (see e.g., [42, Section 3.2.4]). By checking products of characters, we get the following tensor product rule in $ r^\alpha(S_4) $:

$\begin{align*} 1 \otimes V_{\xi_i } = V_{\xi_{i} };\; \; \; V_\psi \otimes V_{\xi_j } = V_{\xi_{3-j} }, \; \; \; V_\psi \otimes V_{\xi_3 } = V_{\xi_{3} };\; \; \; V_{\rho_1} \otimes V_{\xi_j } = V_{\xi_{3} }, \; \; \; V_{\rho_1} \otimes V_{\xi_3 } = V_{\xi_{1} }+ V_{\xi_{2} }+V_{\xi_{3} };\\ V_{\rho_2} \otimes V_{\xi_j } = V_{\xi_{3-j} }+ V_{\xi_{3} }, \; \; \; V_{\rho_2} \otimes V_{\xi_3 } = V_{\rho_3} \otimes V_{\xi_3 } = V_{\xi_{1} }+ V_{\xi_{2} }+2V_{\xi_{3} };\; \; \; V_{\rho_3} \otimes V_{\xi_j } = V_{\xi_{j} }+ V_{\xi_{3} };\; \; \; \; \; \end{align*}$ $

where $ i = 1, 2, 3 $, $ j = 1, 2 $. Thus, we get

$ 1 \mapsto E_3, \quad V_{\psi} \mapsto

$\begin{pmatrix} 0 &1 &0\\ 1 &0 &0\\ 0 &0 &1 \end{pmatrix}$ , \quad V_{\rho_1} \mapsto

$\begin{pmatrix} 0 &0 &1\\ 0 &0 &1\\ 1 &1 &1 \end{pmatrix}$ , \quad V_{\rho_2} \mapsto

$\begin{pmatrix} 0 &1 &1\\ 1 &0 &1\\ 1 &1 &2 \end{pmatrix}$ , \quad V_{\rho_3} \mapsto

$\begin{pmatrix} 1 &0 &1\\ 0 &1 &1\\ 1 &1 &2 \end{pmatrix}$ . $

Then $ r(S_4, \alpha) $ is an irreducible based module over $ r(S_4) $ equivalent to $ M_{3, 3} $ listed in Table 3. In other words, the irreducible based module $ M_{3, 3} $ over $ r(S_4) $ can be categorified by $ {\rm Rep}(S_4, \alpha) $. □

In summary, we have the following classification theorem.

Theorem 4.12. The inequivalent irreducible based modules over $ r(S_4) $ are

$ M_{1, 1}, \; \; \; {\left \{ M_{2, i}\right \} } _{i = 1, 2, 3} , \; \; \; {\left \{ M_{3, j}\right \} } _{j = 1, 2, 3} , \; \; \; {\left \{ M_{4, s}\right \} } _{1\le s\le 7} \; \; \; \mathit{\text{and}}\; \; \; {\left \{ M_{5, t}\right \} } _{t = 1, 2}, $

among which

$ M_{1, 1} , \; \; \; {\left \{ M_{2, i}\right \} } _{i = 2, 3} , \; \; \; {\left \{ M_{3, j}\right \} } _{j = 1, 2, 3}, \; \; \; {\left \{ M_{4, s}\right \} } _{s = 1, 5, 7} \; \; \; \mathit{\text{and}}\; \; \; {\left \{ M_{5, t}\right \} } _{t = 1, 2} $

can be categorified by module categories over $ {\rm Rep}(S_4) $; see Table 8.

Table 8. Inequivalent irreducible based modules over $ r(S_4) $.

		$ V_{\psi} $	$ V_{\rho _{1}} $	$ V_{\rho _{2}} $	Categorification
Rank 1	$ M_{1, 1} $	$ 1 $	$ 2 $	$ 3 $	$ {\rm Rep}(\mathbb Z_1) $, $ {\rm Rep}(K_4, \alpha) $
Rank 2	$ M_{2, 1} $	$ $\begin{pmatrix} 1&0\\0&1\end{pmatrix}$ $	$ $\begin{pmatrix} 2&0\\0&2\end{pmatrix}$ $	$ $\begin{pmatrix} 1&2\\2&1\end{pmatrix}$ $	No
	$ M_{2, 2} $	$ $\begin{pmatrix} 0&1\\1&0\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1\\1&1\end{pmatrix}$ $	$ $\begin{pmatrix} 2&1\\1&2\end{pmatrix}$ $	$ {\rm Rep}(\mathbb Z_2) $, $ {\rm Rep}(D_4, \alpha) $
	$ M_{2, 3} $	$ $\begin{pmatrix} 0&1\\1&0\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1\\1&1\end{pmatrix}$ $	$ $\begin{pmatrix} 1&2\\2&1\end{pmatrix}$ $	$ {\rm Rep}(\mathbb Z_2) $, $ {\rm Rep}(D_4, \alpha) $
Rank 3	$ M_{3, 1} $	$ $\begin{pmatrix} 1&0&0\\0&1&0\\0&0&1\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1\\1&0&1\\1&1&0\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&1\\1&1&1\\1&1&1\end{pmatrix}$ $	$ {\rm Rep}(\mathbb Z_3) $, $ {\rm Rep}(A_4, \alpha) $
	$ M_{3, 2} $	$ $\begin{pmatrix} 0&1&0\\1&0&0\\0&0&1\end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&1\\0&0&1\\1&1&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&0&1\\0&1&1\\1&1&2\\\end{pmatrix}$ $	$ {\rm Rep}(S_3) $
	$ M_{3, 3} $	$ $\begin{pmatrix} 0&1&0\\1&0&0\\0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&1\\0&0&1\\1&1&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1\\1&0&1\\1&1&2\\\end{pmatrix}$ $	$ {\rm Rep}(S_3) $, $ {\rm Rep}(S_4, \alpha) $
Rank 4	$ M_{4, 1} $	$ $\begin{pmatrix} 1&0&0&0\\0&1&0&0\\0&0&1&0\\0&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&1&1\\0&2&0&0\\1&0&0&1\\1&0&1&0\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&0&0\\1&2&1&1\\0&1&0&0\\0&1&0&0\\\end{pmatrix}$ $	$ {\rm Rep}(A_4) $
	$ M_{4, 2} $	$ $\begin{pmatrix} 1&0&0&0\\0&1&0&0\\0&0&1&0\\0&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 2&0&0&0\\0&2&0&0\\0&0&2&0\\0&0&0&2\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1&1\\1&0&1&1\\1&1&0&1\\1&1&1&0\\\end{pmatrix}$ $	No
	$ M_{4, 3} $	$ $\begin{pmatrix} 1&0&0&0\\0&1&0&0\\0&0&1&0\\0&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0\\1&1&0&0\\0&0&2&0\\0&0&0&2\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1&1\\1&0&1&1\\1&1&0&1\\1&1&1&0\\\end{pmatrix}$ $	No
	$ M_{4, 4} $	$ $\begin{pmatrix} 0&1&0&0\\1&0&0&0\\0&0&1&0\\0&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0\\1&1&0&0\\0&0&2&0\\0&0&0&2\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&0&1&1\\0&1&1&1\\1&1&0&1\\1&1&1&0\\\end{pmatrix}$ $	No
	$ M_{4, 5} $	$ $\begin{pmatrix} 0&1&0&0\\1&0&0&0\\0&0&0&1\\0&0&1&0\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0\\1&1&0&0\\0&0&1&1\\0&0&1&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1&1\\1&0&1&1\\1&1&0&1\\1&1&1&0\\\end{pmatrix}$ $	$ {\rm Rep}(\mathbb Z_4), \, {\rm Rep}(K_4) $
	$ M_{4, 6} $	$ $\begin{pmatrix} 0&1&0&0\\1&0&0&0\\0&0&0&1\\0&0&1&0\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0\\1&1&0&0\\0&0&1&1\\0&0&1&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&1&1&1\\1&0&1&1\\1&1&1&0\\1&1&0&1\\\end{pmatrix}$ $	No
	$ M_{4, 7} $	$ $\begin{pmatrix} 0&1&0&0\\1&0&0&0\\0&0&0&1\\0&0&1&0\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0\\1&1&0&0\\0&0&1&1\\0&0&1&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&0&1&1\\0&1&1&1\\1&1&1&0\\1&1&0&1\\\end{pmatrix}$ $	$ {\rm Rep}(\mathbb Z_4), \, {\rm Rep}(K_4) $
Rank 5	$ M_{5, 1} $	$ $\begin{pmatrix} 0&1&0&0&0\\1&0&0&0&0\\0&0&0&1&0\\0&0&1&0&0\\0&0&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&0&0&1\\0&0&0&0&1\\0&0&1&1&0\\0&0&1&1&0\\1&1&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&0&1&0\\0&0&1&0&0\\0&1&1&1&1\\1&0&1&1&1\\0&0&1&1&0\\\end{pmatrix}$ $	$ {\rm Rep}(S_4) $
	$ M_{5, 2} $	$ $\begin{pmatrix} 0&1&0&0&0\\1&0&0&0&0\\0&0&0&1&0\\0&0&1&0&0\\0&0&0&0&1\\\end{pmatrix}$ $	$ $\begin{pmatrix} 1&1&0&0&0\\1&1&0&0&0\\0&0&1&1&0\\0&0&1&1&0\\0&0&0&0&2\\\end{pmatrix}$ $	$ $\begin{pmatrix} 0&0&0&1&1\\0&0&1&0&1\\0&1&0&0&1\\1&0&0&0&1\\1&1&1&1&1\\\end{pmatrix}$ $	$ {\rm Rep}(D_4) $

| Show Table

DownLoad: CSV

5. Conclusions

The analysis in this paper shows that the classification of the irreducible based modules of rank up to 5 over the complex representation ring $ r(S_4) $. We also showed that any $ \mathbb{Z}_+ $-modules over the representation ring $ r(G) $ categorified by a module category over the representation category $ {\rm Rep}(G) $ must be a based module. At the end, we present the categorification of based modules over $ r(S_4) $ by module categories over the complex representation category $ {\rm Rep}(S_4) $ of $ S_4 $, using projective representations of specific subgroups of $ S_4 $. We expect that the studies developed here will be helpful in investigations of the structures of module categories over fusion categories. Our future study will focus on the existence of any irreducible based module of rank $ \geq 6 $ over $ r(S_4) $ and classifying irreducible $ \mathbb Z_+ $-modules over $ r(S_4) $, especially for high-rank cases. Also, some other small finite groups may be interesting to consider, e.g., the dihedral group $ D_5 $.

Author contributions

Wenxia Wu: Writing-original draft and editing, conceptualization, software, methodology; Yunnan Li: Topic selection, writing-review and editing, funding acquisition, methodology, supervision. All authors have read and approved the final version of the manuscript for publication.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

We would like to thank Zhiqiang Yu for helpful discussion. This work is supported by Guangdong Basic and Applied Basic Research Foundation (2022A1515010357).

Conflict of interest

The authors declare that there are no conflicts of interest.

References

[1]	CDC—The National Institute for Occupational Safety and Health (NIOSH) (2004). available from: http://www.cdc.gov/niosh/.
[2]	Kopjar N, Garaj-Vrhovac V, Kašuba V, et al. (2009) Assessment of genotoxic risks in Croatian health care workers occupationally exposed to cytotoxic drugs: A multi-biomarker approach. Int J Hyg Environ Health 212: 414-431. doi: 10.1016/j.ijheh.2008.10.001
[3]	Mahboob M, Rahman F, Rekhadevi PV, et al. (2012) Monitoring of Oxidative Stress in Nurses Occupationally Exposed to Antineoplastic Drugs. Toxicol Int 19: 20-24. doi: 10.4103/0971-6580.94510
[4]	Villarini M, Dominici L, Piccinini R, et al. (2011) Assessment of primary, oxidative and excision repaired DNA damage in hospital personnel handling antineoplastic drugs. Mutagenesis 26: 359-369.
[5]	Villarini M, Dominici L, Fatigoni C, et al. (2012) Biological effect monitoring in peripheral blood lymphocytes from subjects occupationally exposed to antineoplastic drugs: assessment of micronuclei frequency. J Occup Health 54: 405-415. doi: 10.1539/joh.12-0038-OA
[6]	Fucic A, Jazbec A, Mijic A, et al. (1998) Cytogenetic consequences after occupational exposure to antineoplastic drugs. Mutat Res Toxicol Environ Mutagen 416: 59-66. doi: 10.1016/S1383-5718(98)00084-9
[7]	Burgaz S, Karahalil B, Bayrak P, et al. (1999) Urinary cyclophosphamide excretion and micronuclei frequencies in peripheral lymphocytes and in exfoliated buccal epithelial cells of nurses handling antineoplastics. Mutat Res 439: 97-104. doi: 10.1016/S1383-5718(98)00180-6
[8]	Sessink RP, Bos RP (1999) Drugs hazardous to healthcare workers. Evaluation of methods for monitoring occupational exposure to cytostatic drugs. Drug Saf Int J Med Toxicol Drug Exp 20: 347-359.
[9]	Bouraoui S, Brahem A, Tabka F, et al. (2011) Assessment of chromosomal aberrations, micronuclei and proliferation rate index in peripheral lymphocytes from Tunisian nurses handling cytotoxic drugs. Environ Toxicol Pharmacol 31: 250-257. doi: 10.1016/j.etap.2010.11.004
[10]	Gulten T, Evke E, Ercan I, et al. (2011) Lack of genotoxicity in medical oncology nurses handling antineoplastic drugs: effect of work environment and protective equipment. Work Read Mass 39: 485-489.
[11]	Buschini A, Villarini M, Feretti D, et al. (2013) Multicentre study for the evaluation of mutagenic/carcinogenic risk in nurses exposed to antineoplastic drugs: assessment of DNA damage. Occup Environ Med 70: 789-794.
[12]	Jackson MA, Stack HF, Waters MD (1996) Genetic activity profiles of anticancer drugs. Mutat Res 355: 171-208. doi: 10.1016/0027-5107(96)00028-0
[13]	Connor TH (2006) Hazardous Anticancer Drugs in Health Care: Environmental Exposure Assessment. Ann N Y Acad Sci 1076: 615-623.
[14]	Kopjar N, Milas I, Garaj-Vrhovac V, et al. (2006) M. Gamulin, Alkaline comet assay study with breast cancer patients: evaluation of baseline and chemotherapy-induced DNA damage in non-target cells. Clin Exp Med 6: 177-190.
[15]	Kiffmeyer T, Hadtstein C (2007) Handling of chemotherapeutic drugs in the or: hazards and safety considerations. Cancer Treat Res 134: 275-290.
[16]	Collins AR (2004) The comet assay for DNA damage and repair: principles, applications, and limitations. Mol Biotechnol 26: 249-261. doi: 10.1385/MB:26:3:249
[17]	Collins AR (2009) Investigating oxidative DNA damage and its repair using the comet assay. Mutat Res 681: 24-32. doi: 10.1016/j.mrrev.2007.10.002
[18]	Laffon B, Teixeira JP, Silva S, et al. (2005) Genotoxic effects in a population of nurses handling antineoplastic drugs, and relationship with genetic polymorphisms in DNA repair enzymes. Am J Ind Med 48: 128-136. doi: 10.1002/ajim.20189
[19]	Dusinska M, Collins AR (2008) The comet assay in human biomonitoring: gene-environment interactions. Mutagenesis 23: 191-205.
[20]	Azqueta A, Shaposhnikov S, Collins A (2009) Detection of oxidised DNA using DNA repair enzymes, In: Anderson, D. and Dhawan A, Comet Assay Toxicol, Royal Society of Chemistry, 58-63.
[21]	Moller P, Knudsen LE, Loft S, et al. (2000) The comet assay as a rapid test in biomonitoring occupational exposure to DNA-damaging agents and effect of confounding factors. Cancer Epidemiol Biomark Prev Publ Am Assoc Cancer Res Cosponsored Am Soc Prev Oncol 9: 1005-1015.
[22]	Collins A.R, Dusinská M, Horváthová E, et al. (2001) Inter-individual differences in repair of DNA base oxidation, measured in vitro with the comet assay. Mutagenesis 16: 297-301.
[23]	Collins A, Oscoz A, Brunborg G, et al. (2008)The comet assay: topical issues. Mutagenesis 23: 143-151.
[24]	Hoeijmakers JH (2001) Genome maintenance mechanisms for preventing cancer. Nature 411: 366-374. doi: 10.1038/35077232
[25]	Boiteux S, Radicella JP (1999) Base excision repair of 8-hydroxyguanine protects DNA from endogenous oxidative stress. Biochimie 81: 59-67. doi: 10.1016/S0300-9084(99)80039-X
[26]	Ersson C (2011) International validation of the comet assay and a human intervention study. Stockholm: Karolinska Institutet.
[27]	Pilger A, Rüdiger HW (2006) 8-Hydroxy-2′-deoxyguanosine as a marker of oxidative DNA damage related to occupational and environmental exposures. Int Arch Occup Environ Health 80: 1-15. doi: 10.1007/s00420-006-0106-7
[28]	Kohno T, Shinmura K, Tosaka M, et al. (1998) Genetic polymorphisms and alternative splicing of the hOGG1 gene, that is involved in the repair of 8-hydroxyguanine in damaged DNA. Oncogene 16: 3219-3225. doi: 10.1038/sj.onc.1201872
[29]	Macpherson P, Barone F, Maga G, et al. (2005) 8-Oxoguanine incorporation into DNA repeats in vitro and mismatch recognition by MutSα. Nucleic Acids Res 33: 5094-5105.
[30]	Hu YC, Ahrendt SA (2005) hOGG1 Ser326Cys polymorphism and G:C-to-T:A mutations: no evidence for a role in tobacco-related non small cell lung cancer. J Int Cancer 114: 387-393. doi: 10.1002/ijc.20730
[31]	Larson RR, Khazaeli MB, DillonHK (2003) A new monitoring method using solid sorbent media for evaluation of airborne cyclophosphamide and other antineoplastic agents. Appl Occup Environ Hyg 18: 120-131. doi: 10.1080/10473220301435
[32]	Castiglia L, Miraglia N, Pieri M, et al. (2008) Evaluation of occupational exposure to antiblastic drugs in an Italian hospital oncological department. J Occup Health 50: 48-56.
[33]	Hedmer M, Jönsson BAG, Nygren O (2004) Development and validation of methods for environmental monitoring of cyclophosphamide in workplaces. J Environ Monit 6: 979-984. doi: 10.1039/b409277e
[34]	Hedmer M, Tinnerberg H, Axmon A, et al. (2008) Environmental and biological monitoring of antineoplastic drugs in four workplaces in a Swedish hospital. Int Arch Occup Environ Health 81: 899-911.
[35]	Kopp B, Crauste-Manciet S, Guibert A, et al. (2013) Environmental and Biological Monitoring of Platinum-Containing Drugs in Two Hospital Pharmacies Using Positive Air Pressure Isolators. Ann Occup Hyg 57: 374-383.
[36]	Schmaus G, Schierl R, Funck S (2002) Monitoring surface contamination by antineoplastic drugs using gas chromatography-mass spectrometry and voltammetry. Am J Health Syst Pharm 59: 956-961.
[37]	Singh N, Lai H (2009) Methods for freezing blood samples at -80 ℃ for DNA damage analysis in human leukocytes, In: Anderson D and Dhawan A, Comet Assay Toxicol, Royal Society of Chemistry, 120-128.
[38]	Duthie SJ, Pirie L, Jenkinson AM, et al. (2002) Cryopreserved versus freshly isolated lymphocytes in human biomonitoring: endogenous and induced DNA damage, antioxidant status and repair capability. Mutagenesis 17: 211-214. doi: 10.1093/mutage/17.3.211
[39]	Collins AR, Azqueta A (2012) Single-Cell Gel Electrophoresis Combined with Lesion-Specific Enzymes to Measure Oxidative Damage to DNA, In: Methods Cell Biol, Elsevier, 69-92. available from; http://linkinghub.elsevier.com/retrieve/pii/B9780124059146000044
[40]	Collins AR (2002) The comet assay. Principles, applications, and limitations. Methods Mol Biol Clifton NJ 203: 163-177.
[41]	Hon C, Chua PP, Danyluk Q, et al. (2013) Examining factors that influence the effectiveness of cleaning antineoplastic drugs from drug preparation surfaces: a pilot study. J Oncol Pharm Pract 20: 210-216.
[42]	Cavallo D, Ursini CL, Perniconi B, et al. (2005) Evaluation of genotoxic effects induced by exposure to antineoplastic drugs in lymphocytes and exfoliated buccal cells of oncology nurses and pharmacy employees. Mutat Res Toxicol Environ Mutagen 587: 45-51. doi: 10.1016/j.mrgentox.2005.07.008
[43]	Hedmer M, Wohlfart G (2012) Hygienic guidance values for wipe sampling of antineoplastic drugs in Swedish hospitals. J Environ Monit 14: 1968-1975. doi: 10.1039/c2em10704j
[44]	Viegas S, Pádua M, Veiga A, et al. (2014) Antineoplastic drugs contamination of workplaces surfaces in two Portuguese hospitals. Environ Monit Assess 186: 7807-18. doi: 10.1007/s10661-014-3969-1
[45]	Collins AR (1999) Oxidative DNA damage, antioxidants, and cancer, BioEssays News Rev. Mol Cell Dev Biol 21: 238-246.
[46]	Cavallo D, Ursini CL, Rondinone B, et al. (2009) Evaluation of a suitable DNA damage biomarker for human biomonitoring of exposed workers. Environ Mol Mutagen: 781-790.
[47]	Digue L, Orsière T, Méo M De, et al. (1999) Evaluation of the genotoxic activity of paclitaxel by the in vitro micronucleus test in combination with fluorescent in situ hybridization of a DNA centromeric probe and the alkaline single cell gel electrophoresis technique (comet assay) in human T-lymphocytes. Environ Mol Mutagen 34: 269-278. doi: 10.1002/(SICI)1098-2280(1999)34:4<269::AID-EM7>3.0.CO;2-D
[48]	Blasiak J, Kowalik J, Małecka-Panas EJ, et al. (2000) DNA damage and repair in human lymphocytes exposed to three anticancer platinum drugs. Teratog Carcinog Mutagen 20: 119-131. doi: 10.1002/(SICI)1520-6866(2000)20:3<119::AID-TCM3>3.0.CO;2-Z
[49]	Ursini CL, Cavallo D, Colombi A, et al. (2006) Evaluation of early DNA damage in healthcare workers handling antineoplastic drugs. Int Arch Occup Environ Health 80: 134-140. doi: 10.1007/s00420-006-0111-x
[50]	Sasaki M, Dakeishi M, Akeishi S, et al. (2008) Assessment of DNA Damage in Japanese Nurses Handling Antineoplastic Drugs by the Comet Assay. J Occup Health 50: 7-12. doi: 10.1539/joh.50.7
[51]	Ündeğer Ü, Başaran N, Kars A, et al. (1999) Assessment of DNA damage in nurses handling antineoplastic drugs by the alkaline COMET assay. Mutat Res Toxicol Environ Mutagen 439: 277-285. doi: 10.1016/S1383-5718(99)00002-9
[52]	Yoshida J, Kosaka H, Tomioka S, et al. (2006) Genotoxic Risks to Nurses from Contamination of the Work Environment with Antineoplastic Drugs in Japan. J Occup Health 48: 517-522. doi: 10.1539/joh.48.517
[53]	Branham MT, Nadin SB, Vargas-Roig LM, et al. (2004) DNA damage induced by paclitaxel and DNA repair capability of peripheral blood lymphocytes as evaluated by the alkaline comet assay. Mutat Res 560: 11-17. doi: 10.1016/j.mrgentox.2004.01.013
[54]	Mader RM, Kokalj A, Kratochvil E, et al. (2009) Longitudinal biomonitoring of nurses handling antineoplastic drugs. J Clin Nurs 18: 263-269. doi: 10.1111/j.1365-2702.2007.02189.x
[55]	Kopjar N, Garaj-Vrhovac V (2001) Application of the alkaline comet assay in human biomonitoring for genotoxicity: a study on Croatian medical personnel handling antineoplastic drugs. Mutagenesis 16: 71-78. doi: 10.1093/mutage/16.1.71
[56]	Maluf SW, Erdtmann B (2000) Follow-up study of the genetic damage in lymphocytes of pharmacists and nurses handling antineoplastic drugs evaluated by cytokinesis-block micronuclei analysis and single cell gel electrophoresis assay. Mutat Res 471: 21-27.
[57]	Kopjar N, Želježić D, Vrdoljak AL, et al. (2007) Irinotecan Toxicity to Human Blood Cells in vitro: Relationship between Various Biomarkers. Basic Clin Pharmacol Toxicol 100: 403-413. doi: 10.1111/j.1742-7843.2007.00068.x
[58]	Rekhadevi PV, Sailaja N, Chandrasekhar M, et al. (2007) Genotoxicity assessment in oncology nurses handling anti-neoplastic drugs. Mutagenesis 22: 395-401.
[59]	Cornetta T, Padua L, Testa A, et al. (2008) Molecular biomonitoring of a population of nurses handling antineoplastic drugs. Mutat Res 638: 75-82. doi: 10.1016/j.mrfmmm.2007.08.017
[60]	Izdes S, Sardas S, Kadioglu E, et al. (2009) Assessment of genotoxic damage in nurses occupationally exposed to anaesthetic gases or antineoplastic drugs by the comet assay. J Occup Health 51: 283-286. doi: 10.1539/joh.M8012
[61]	Rombaldi F, Cassini C, Salvador M, et al. (2008) Occupational risk assessment of genotoxicity and oxidative stress in workers handling anti-neoplastic drugs during a working week. Mutagenesis 24: 143-148.
[62]	Ladeira C, Viegas S, Pádua M, et al. (2014) Assessment of Genotoxic Effects in Nurses Handling Cytostatic Drugs. J Toxicol Environ Health A 77: 879-887. doi: 10.1080/15287394.2014.910158
[63]	Deng H, Zhang M, He J, et al. (2005) Investigating genetic damage in workers occupationally exposed to methotrexate using three genetic end-points. Mutagenesis 20: 351-357. doi: 10.1093/mutage/gei048
[64]	Xing DY, Tan E, Song N, et al. (2001) Ser326Cys polymorphism in hOGG1 gene and risk of esophageal cancer in a Chinese population. Int J Cancer 95: 140-143. doi: 10.1002/1097-0215(20010520)95:3<140::AID-IJC1024>3.0.CO;2-2
[65]	Elahi A, Zheng Z, Park P, et al. (2002) The human OGG1 DNA repair enzyme and its association with orolaryngeal cancer risk. Carcinogenesis 23: 1229-1234. doi: 10.1093/carcin/23.7.1229
[66]	Pawlowska E, Janik-Papis K, Rydzanicz M, et al. (2009) The Cys326 allele of the 8-oxoguanine DNA N-glycosylase 1 gene as a risk factor in smoking- and drinking-associated larynx cancer. Tohoku J Exp Med 219: 269-275. doi: 10.1620/tjem.219.269
[67]	Kim JI, Park YJ, Kim KH, et al. (2003) hOGG1 Ser326Cys polymorphism modifies the significance of the environmental risk factor for colon cancer. World J Gastroenterol 9: 956-960. doi: 10.3748/wjg.v9.i5.956
[68]	Takezaki T, Gao C, Wu J, et al. (2002) hOGG1 Ser(326)Cys polymorphism and modification by environmental factors of stomach cancer risk in Chinese. Int J Cancer 99: 624-627.
[69]	Chen SK, Hsieh WA, Tsai MH, et al. (2003) Age-associated decrease of oxidative repair enzymes, human 8-oxoguanine DNA glycosylases (hOgg1), in human aging. J Radiat Res (Tokyo) 44: 31-35. doi: 10.1269/jrr.44.31
[70]	Aka P, Mateuca R, Buchet JP, et al. (2004) Are genetic polymorphisms in OGG1, XRCC1 and XRCC3 genes predictive for the DNA strand break repair phenotype and genotoxicity in workers exposed to low dose ionising radiations? Mutat Res 556: 169-181.
[71]	Mateuca RA, Roelants M, Iarmarcovai G, et al. (2008) hOGG1(326), XRCC1(399) and XRCC3(241) polymorphisms influence micronucleus frequencies in human lymphocytes in vivo. Mutagenesis 23: 35-41.
[72]	Tarng DC, Tsai TJ, Chen WT, et al. (2001) Effect of Human OGG1 1245C→G Gene Polymorphism on 8-Hydroxy-2'-Deoxyguanosine Levels of Leukocyte DNA among Patients Undergoing Chronic Hemodialysis. J Am Soc Nephrol 12: 2338-2347.

Reader Comments

Your name:*

Email:*
© 2015 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Genetics

Metrics

Article views(5665) PDF downloads(1259) Cited by(4)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(2) / Tables(4)

AIMS Genetics

Relation between DNA damage measured by comet assay and OGG1 Ser326Cys polymorphism in antineoplastic drugs biomonitoring

Related Papers:

Abstract

1. Introduction

2. Preliminaries

2.1. $ \mathbb{Z}_+ $-rings and $ \mathbb{Z}_+ $-modules

2.2. Based rings and based modules

3. Irreducible based modules over $ r(S_4) $

3.1. Irreducible based modules of rank $ \le 3 $ over $ r(S_4) $

3.2. Irreducible based modules of rank $ 4, \, 5 $ over $ r(S_4) $

4. Categorified based modules by module categories over $ { Rep}(S_4) $

4.1. The module categories over $ { Rep}(S_4) $ with trivial twists

4.2. The module categories over $ { Rep}(S_4) $ with nontrivial twists

5. Conclusions

Author contributions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Genetics

Relation between DNA damage measured by comet assay and OGG1 Ser326Cys polymorphism in antineoplastic drugs biomonitoring

Related Papers:

Abstract

1. Introduction

2. Preliminaries

2.1. $ \mathbb{Z}_+ $-rings and $ \mathbb{Z}_+ $-modules

2.2. Based rings and based modules

3. Irreducible based modules over $ r(S_4) $

3.1. Irreducible based modules of rank $ \le 3 $ over $ r(S_4) $

3.2. Irreducible based modules of rank $ 4, \, 5 $ over $ r(S_4) $

4. Categorified based modules by module categories over $ { Rep}(S_4) $

4.1. The module categories over $ { Rep}(S_4) $ with trivial twists

4.2. The module categories over $ { Rep}(S_4) $ with nontrivial twists

5. Conclusions

Author contributions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog